Nonuniform Slip Effect in Wetting Films
Abstract
1. Introduction
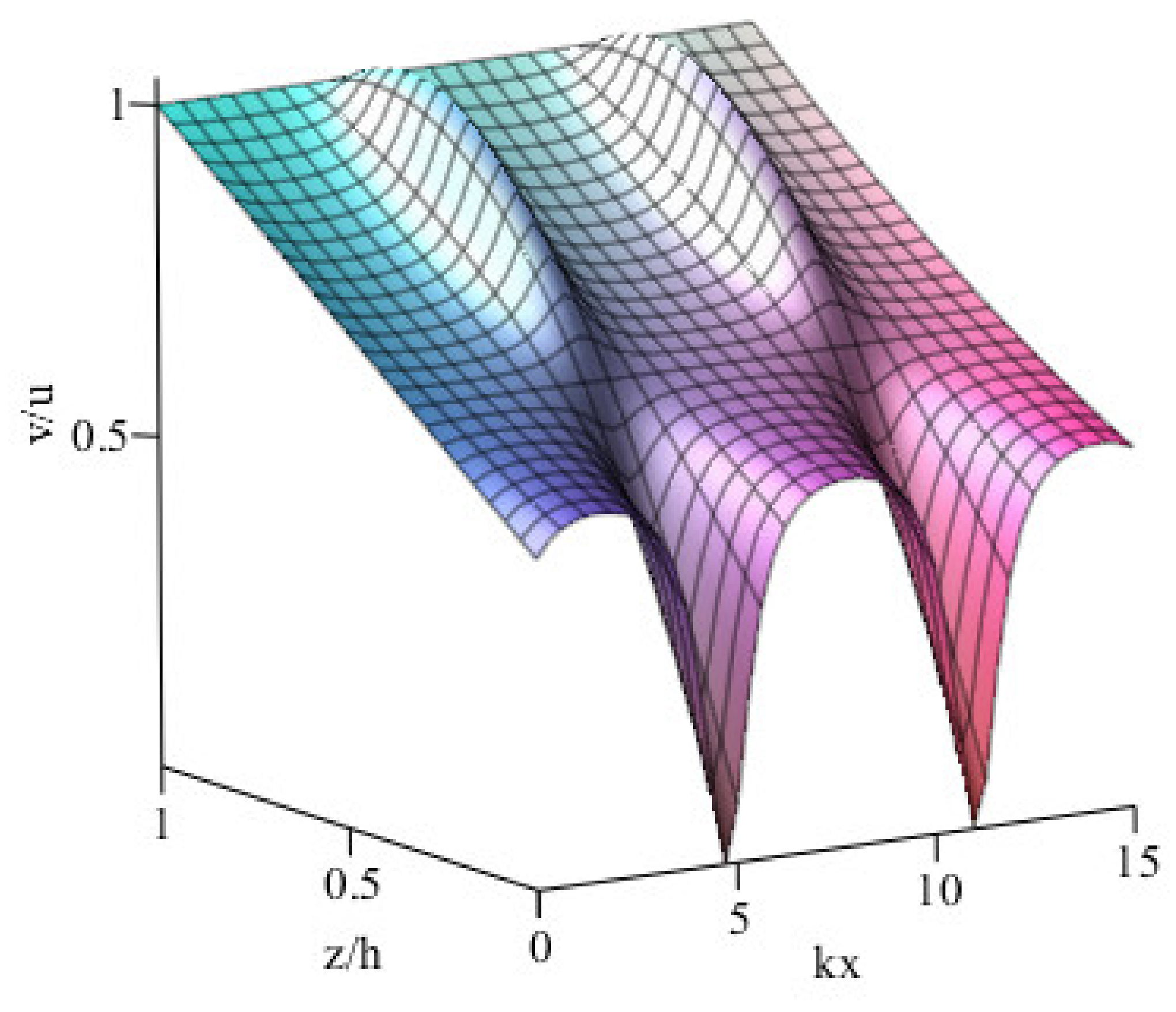
2. Wetting Film Hydrodynamics
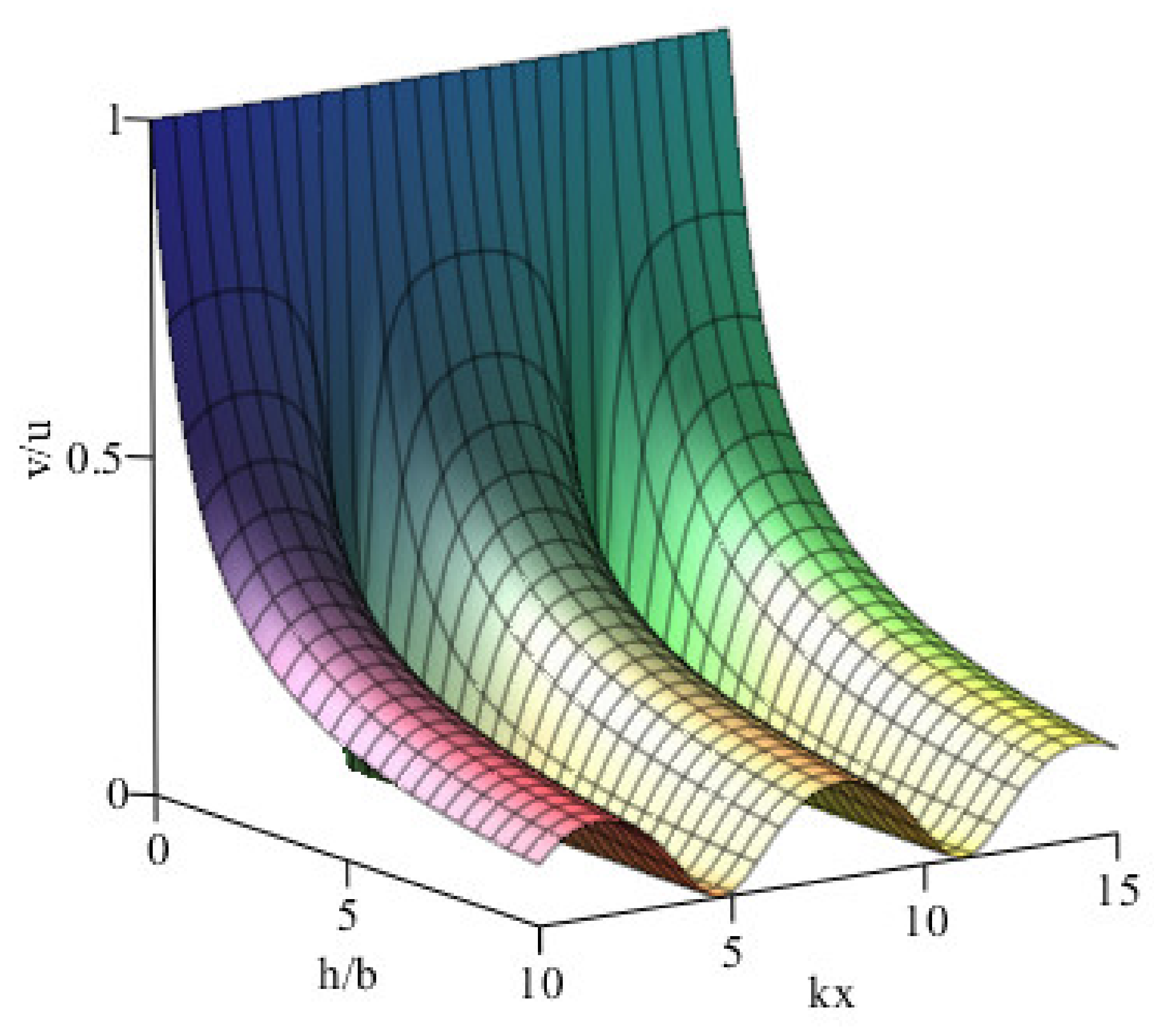
3. Hydrodynamics of Thicker Wetting Films
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Sheludko, A. Thin liquid films. Adv. Colloid Interface Sci. 1967, 1, 391–464. [Google Scholar] [CrossRef]
- Ivanov, I.B. (Ed.) Thin Liquid Films; Dekker: New York, NY, USA, 1988. [Google Scholar]
- Radoev, B.; Stöckelhuber, K.W.; Tsekov, R.; Letocart, P. Wetting film dynamics and stability. In Colloid Stability and Application in Pharmacy; Tadros, T.F., Ed.; Willey: Weinheim, Germany, 2007; pp. 151–172. [Google Scholar]
- Stone, H.A.; Stroock, A.D.; Ajdari, A. Engineering flows in small devices: Microfluidics toward a lab-on-a-chip. Annu. Rev. Fluid Mech. 2004, 36, 381–411. [Google Scholar] [CrossRef]
- Karniadakis, G.; Beskok, A.; Aluru, N. Microflows and Nanoflows: Fundamentals and Simulation; Springer: New York, NY, USA, 2005. [Google Scholar]
- Colosqui, C.E.; Kavousanakis, M.E.; Papathanasiou, A.G.; Kevrekidis, I.G. Mesoscopic model for microscale hydrodynamics and interfacial phenomena: Slip, films, and contact-angle hysteresis. Phys. Rev. E 2013, 87, 13302. [Google Scholar] [CrossRef] [PubMed]
- Lauga, E.; Brenner, M.; Stone, H. Microfluidics: The no-slip boundary condition. In Handbook of Experimental Fluid Mechanics; Tropea, C., Yarin, A., Foss, J.F., Eds.; Springer: New York, NY, USA, 2007; pp. 1219–1240. [Google Scholar]
- Vinogradova, O.I. Slippage of water over hydrophobic surfaces. Int. J. Miner. Process. 1999, 56, 31–60. [Google Scholar] [CrossRef]
- Tsekov, R.; Radoev, B. Surface forces and dynamic effects in thin liquid films on solid interfaces. Int. J. Miner. Process. 1999, 56, 61–74. [Google Scholar] [CrossRef]
- Feuillebois, F.; Bazant, M.Z.; Vinogradova, O.I. Effective slip over superhydrophobic surfaces in thin channels. Phys. Rev. Lett. 2009, 102, 026001. [Google Scholar] [CrossRef] [PubMed]
- Rothstein, J.P. Slip on superhydrophobic surfaces. Annu. Rev. Fluid Mech. 2010, 42, 89–109. [Google Scholar] [CrossRef]
- Wang, C.Y. Flow over a surface with parallel grooves. Phys. Fluids 2003, 15, 1114–1121. [Google Scholar] [CrossRef]
- Davis, J.M.; Troian, S.M. On a generalized approach to the linear stability of spatially nonuniform thin film flows. Phys. Fluids 2003, 15, 1344–1347. [Google Scholar] [CrossRef]
- Asmolov, E.S.; Vinogradova, O.I. Effective slip boundary conditions for arbitrary one-dimensional surfaces. J. Fluid Mech. 2012, 706, 108–117. [Google Scholar] [CrossRef]
- Smith, A.M.; Lovelock, K.R.J.; Gosvami, N.N.; Welton, T.; Perkin, S. Quantized friction across ionic liquid thin films. Phys. Chem. Chem. Phys. 2013, 15, 15317–15320. [Google Scholar] [CrossRef] [PubMed]
- Xu, L.; Bandyopadhyay, D.; Reddy, P.D.S.; Sharma, A.; Joo, S.W. Giant slip induced anomalous dewetting of an ultrathin film on a viscous sublayer. Sci. Rep. 2017, 7, 14776. [Google Scholar] [CrossRef] [PubMed]
- Peschka, D.; Haefner, S.; Marquant, L.; Jacobs, K.; Münch, A.; Wagner, B. Signatures of slip in dewetting polymer films. Proc. Natl. Acad. Sci. USA 2019, 116, 9275–9284. [Google Scholar] [CrossRef] [PubMed]
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsekov, R. Nonuniform Slip Effect in Wetting Films. Coatings 2020, 10, 597. https://doi.org/10.3390/coatings10060597
Tsekov R. Nonuniform Slip Effect in Wetting Films. Coatings. 2020; 10(6):597. https://doi.org/10.3390/coatings10060597
Chicago/Turabian StyleTsekov, Roumen. 2020. "Nonuniform Slip Effect in Wetting Films" Coatings 10, no. 6: 597. https://doi.org/10.3390/coatings10060597
APA StyleTsekov, R. (2020). Nonuniform Slip Effect in Wetting Films. Coatings, 10(6), 597. https://doi.org/10.3390/coatings10060597




