Angular and Spectral Bandwidth Considerations in BRDF Measurements of Interference- and Diffraction-Based Coatings
Abstract
1. Introduction
2. Materials
3. Methodology
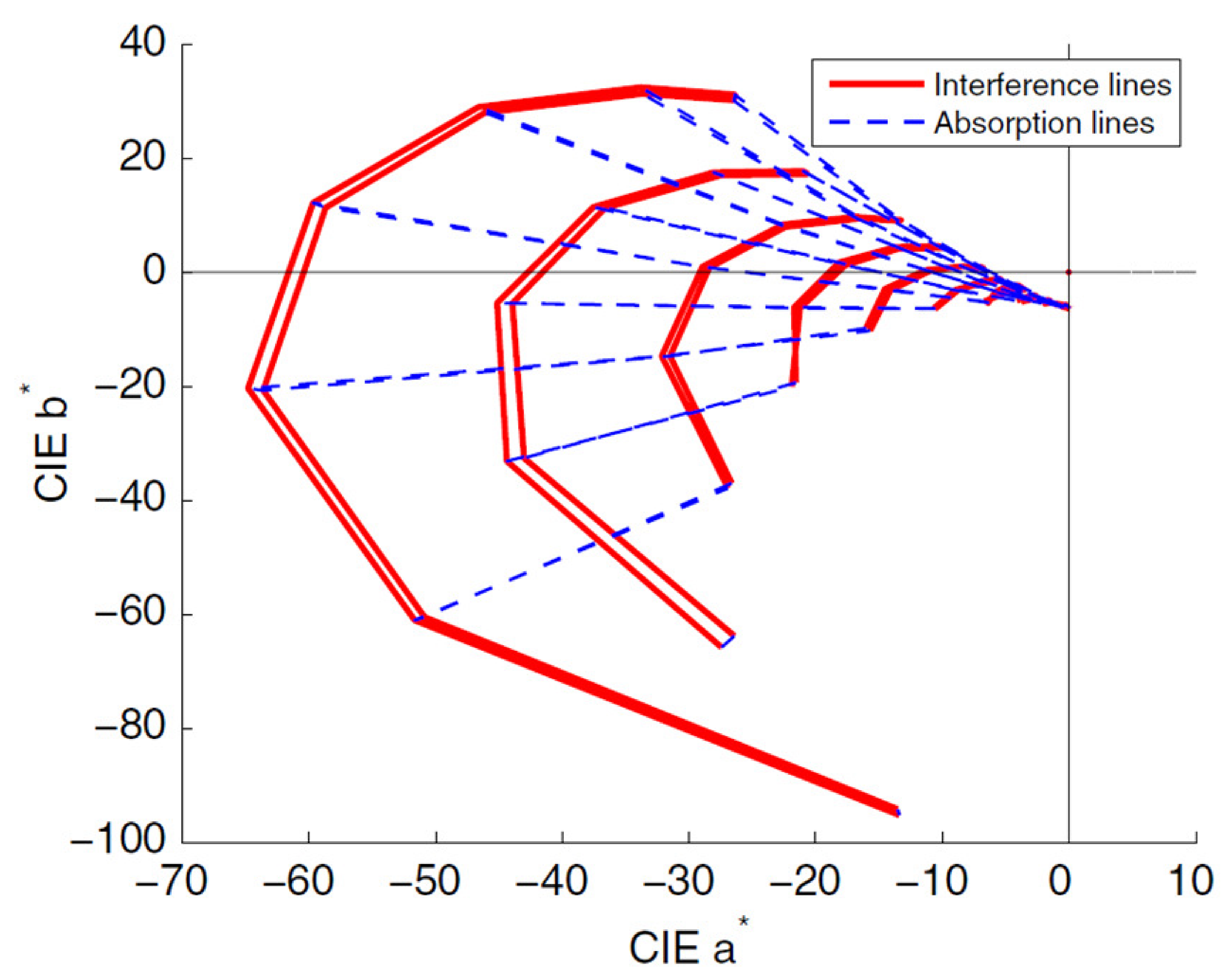
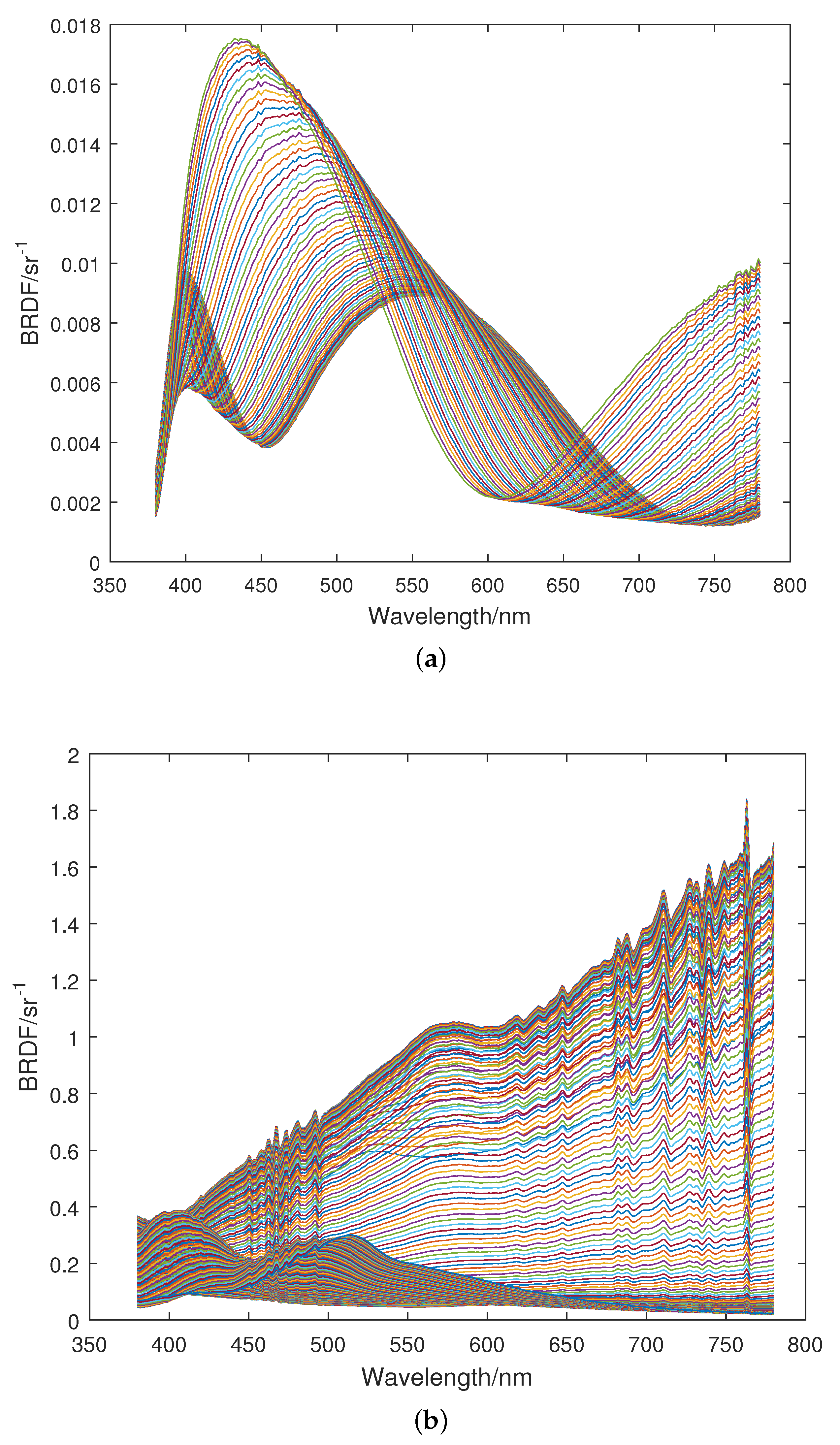
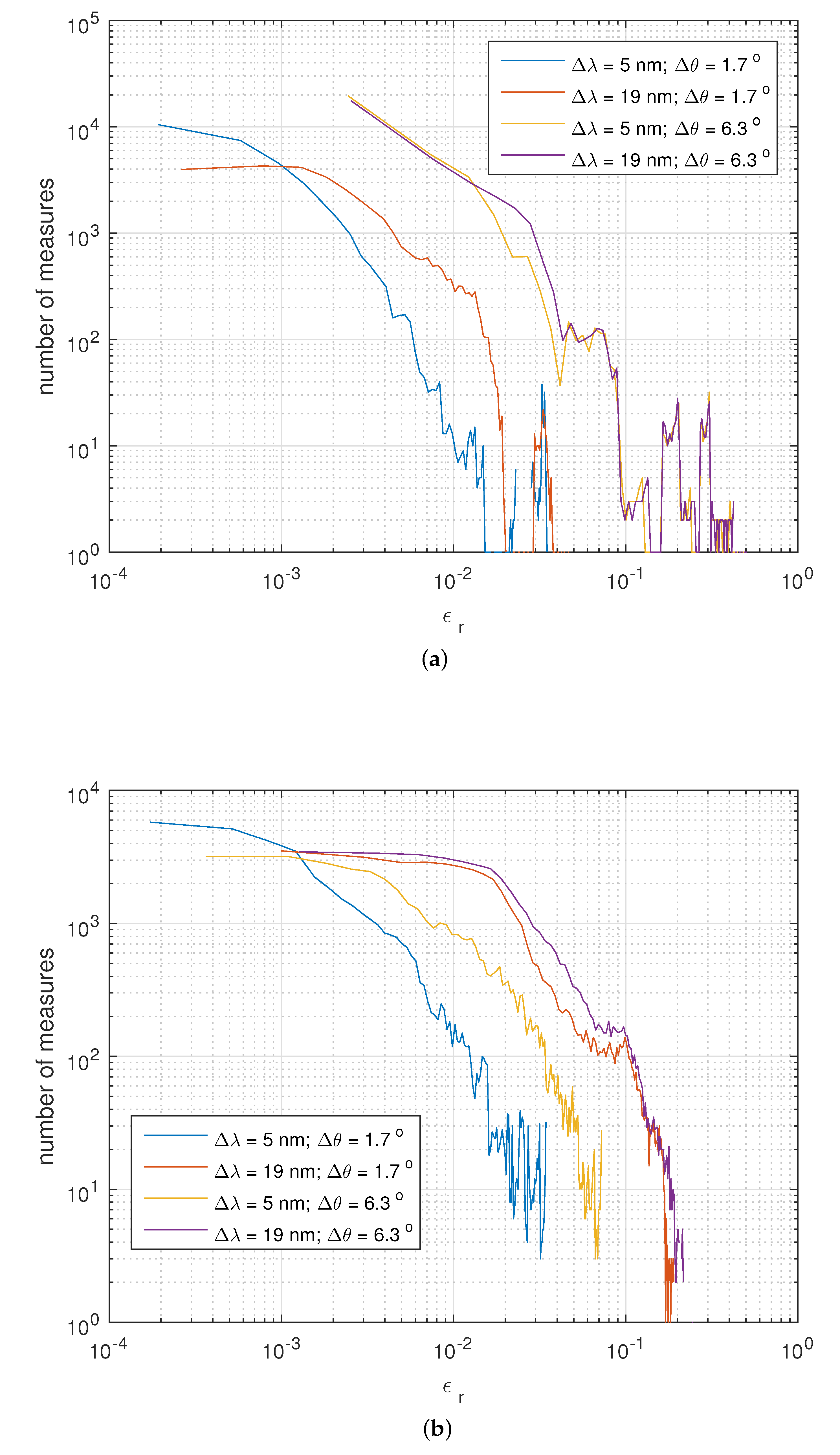
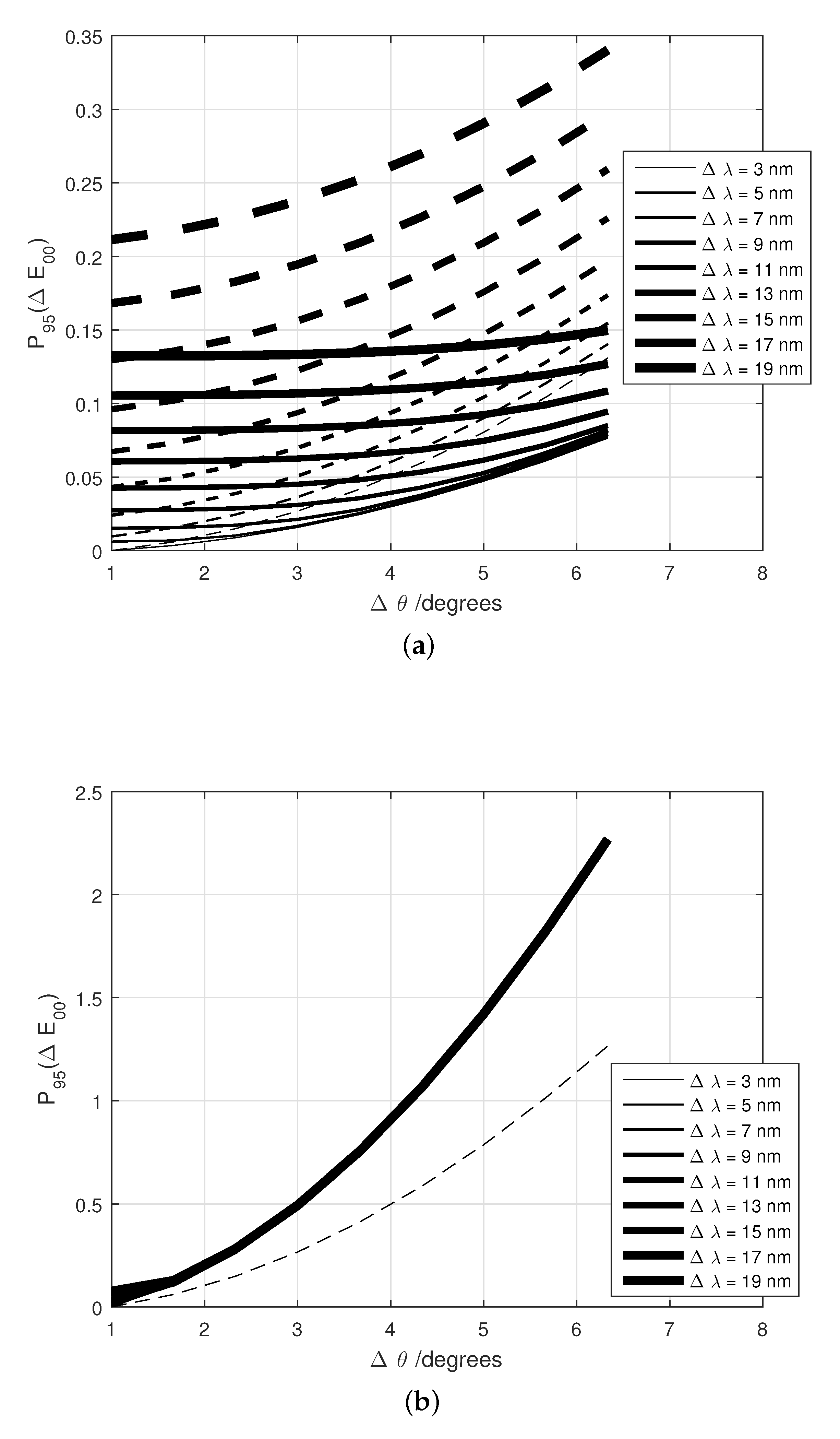
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nicodemus, F.E.; Richmond, J.C.; Hsia, J.J.; Ginsberg, I.W.; Limperis, T. Geometrical Considerations and Nomenclature for Reflectance; US Department of Commerce, National Bureau of Standards: Washington, DC, USA, 1977; Volume 160.
- Klein, G.A. Industrial Color Physics; Springer: Berlin, Germany, 2010; Volume 154. [Google Scholar]
- Maile, F.J.; Pfaff, G.; Reynders, P. Effect pigments—Past, present and future. Prog. Org. Coat. 2005, 54, 150–163. [Google Scholar] [CrossRef]
- Faulkner, E.B.; Schwartz, R.J. High Performance Pigments; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Streitberger, H.J.; Dossel, K.F. Automotive Paints and Coatings; Wiley-Vch: Weinheim, Germany, 2008. [Google Scholar]
- Rösler, M.; Mezger, N.; Dietz, R.; Huber, A. Isoline concepts to find measurement geometries for effect coatings full gonio-color-characterization. Farby i Lakiery 2013, 1, 24–31. [Google Scholar]
- Cramer, W.R.; Gabel, P.W. Measuring special effects. Eur. Coat. J. 2001, 7–8, 34–39. [Google Scholar]
- Kirchner, E.; Cramer, W. Making sense of measurement geometries for multi-angle spectrophotometers. Color Res. Appl. 2012, 37, 186–198. [Google Scholar] [CrossRef]
- Ferrero, A.; Perales, E.; Rabal, A.M.; Campos, J.; Martínez-Verdú, F.M.; Chorro, E.; Pons, A. Color representation and interpretation of special effect coatings. J. Opt. Soc. Am. A 2014, 31, 436–447. [Google Scholar] [CrossRef]
- Kirchner, E.; Ferrero, A. Isochromatic lines as extension of the Helmholtz reciprocity principle for effect paints. J. Opt. Soc. Am. A 2014, 31, 1861–1867. [Google Scholar] [CrossRef]
- Ferrero, A.; Rabal, A.M.; Campos, J.; Pons, A.; Hernanz, M.L. Variables separation of the spectral BRDF for better understanding color variation in special effect pigment coatings. J. Opt. Soc. Am. A 2012, 29, 842–847. [Google Scholar] [CrossRef][Green Version]
- Ferrero, A.; Rabal, A.M.; Campos, J.; Martínez-Verdú, F.; Chorro, E.; Perales, E.; Pons, A.; Hernanz, M.L. Spectral BRDF-based determination of proper measurement geometries to characterize color shift of special effect coatings. J. Opt. Soc. Am. A 2013, 30, 206–214. [Google Scholar] [CrossRef]
- Ferrero, A.; Bernad, B.; Campos, J.; Martínez-Verdú, F.M.; Perales, E.; van der Lans, I.; Kirchner, E. Towards a better understanding of the color shift of effect coatings by densely sampled spectral BRDF measurement. In Measuring, Modeling, and Reproducing Material Appearance; International Society for Optics and Photonics: Bellingham, WA, USA, 2014; Volume 9018, p. 90180K. [Google Scholar]
- Ferrero, A.; Campos, J.; Perales, E.; Martínez-Verdú, F.M.; van der Lans, I.; Kirchner, E. Global color estimation of special-effect coatings from measurements by commercially available portable multiangle spectrophotometers. J. Opt. Soc. Am. A 2015, 32, 1–11. [Google Scholar] [CrossRef]
- Ferrero, A.; Bernad, B.; Campos, J.; Perales, E.; Velázquez, J.L.; Martínez-Verdú, F.M. Color characterization of coatings with diffraction pigments. JOSA A 2016, 33, 1978–1988. [Google Scholar] [CrossRef]
- Rogelj, N.; Poberaj, I.; Gunde, M.K. Goniospectrophotometric space curves of diffraction gratings and their applicability as appearance fingerprints. Appl. Opt. 2013, 52, 8355–8362. [Google Scholar] [CrossRef]
- Cramer, W.R.; Maile, F.J. Rainbows made to order. Eur. Coat. J. 2016, 16, 52–56. [Google Scholar]
- Kettler, W.H.; Sôrmaz, M.; Ehbets, P. Apparatus and Method for Effect Pigment Identification. WO 2018041727A1. 2017. Available online: https://data.epo.org/gpi/EP3500830A1-APPARATUS-AND-METHOD-FOR-EFFECT-PIGMENT-IDENTIFICATION (accessed on 13 October 2020).
- Sperling, U.; Schwarz, P. Device for a Goniometric Examination of the Optical Properties of Surfaces. U.S. Patent 7,276,719, 10 February 2007. [Google Scholar]
- Hünerhoff, D.; Grusemann, U.; Höpe, A. New robot-based gonioreflectometer for measuring spectral diffuse reflection. Metrologia 2006, 43, S11–S16. [Google Scholar] [CrossRef]
- Leloup, F.B.; Forment, S.; Dutré, P.; Pointer, M.R.; Hanselaer, P. Design of an instrument for measuring the spectral bidirectional scatter distribution function. Appl. Opt. 2008, 47, 5454–5467. [Google Scholar] [CrossRef]
- Rabal, A.M.; Ferrero, A.; Campos, J.; Fontecha, J.L.; Pons, A.; Rubiño, A.M.; Corróns, A. Automatic gonio-spectrophotometer for the absolute measurement of the spectral BRDF at in-and out-of-plane and retroreflection geometries. Metrologia 2012, 49, 213–223. [Google Scholar] [CrossRef]
- Obein, G.; Bousquet, R.; Nadal, M.E. New NIST reference goniospectrometer. Proc. SPIE 2005, 5880, 241–250. [Google Scholar]
- Germer, T.A.; Asmail, C.C. Goniometric optical scatter instrument for out-of-plane ellipsometry measurements. Rev. Sci. Instrum. 1999, 70, 3688–3695. [Google Scholar] [CrossRef]
- Baribeau, R.; Neil, W.S.; Côté, E. Development of a robot-based gonioreflectometer for spectral BRDF measurement. J. Mod. Opt. 2009, 56, 1497–1503. [Google Scholar] [CrossRef]
- Nevas, S.; Manoocheri, F.; Ikonen, E. Gonioreflectometer for measuring spectral diffuse reflectance. Appl. Opt. 2004, 43, 6391–6399. [Google Scholar] [CrossRef]
- Ouarets, S.; Ged, G.; Razet, A.; Obein, G. A new gonioreflectometer for the measurement of the bidirectional reflectance distribution function (brdf) at LNE-CNAM. In Proceedings of the CIE 2012 Lighting Quality and energy efficiency, Hangzhou, China, 19–21 September 2012; Volume 5880, pp. 687–691. [Google Scholar]
- Matsapey, N.; Faucheu, J.; Flury, M.; Delafosse, D. Design of a gonio-spectrophotometer for optical characterization of gonio–apparent materials. Meas. Sci. Technol. 2013, 24, 065901. [Google Scholar] [CrossRef]
- Patrick, H.J.; Zarobila, C.J.; Germer, T.A. The NIST Robotic Optical Scatter Instrument (ROSI) and its application to BRDF measurements of diffuse reflectance standards for remote sensing. In Proceedings of the 2013, SPIE Optical Engineering+Applications; International Society for Optics and Photonics: Bellingham, WA, USA, 2013; Volume 8866, p. 886615-12. [Google Scholar]
- Schanda, J. Colorimetry: Understanding the CIE System; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Filip, J.; Vávra, R.; Maile, F.J. Optical analysis of coatings including diffractive pigments using a high-resolution gonioreflectometer. J. Coat. Technol. Res. 2019, 16, 555–572. [Google Scholar] [CrossRef]
- Campos, J.; Corróns, A.; Pons, A.; Corredera, P.; Fontecha, J.L.; Jiménez, J.R. Spectral responsivity uncertainty of silicon photodiodes due to calibration spectral bandwidth. Measure. Sci. Technol. 2001, 12, 1926. [Google Scholar] [CrossRef][Green Version]
- SO/CIE Standard. ISO/CIE 11664-6:2014(E). Colorimetry—Part 6: CIEDE2000 Colour-Difference Formula; International Commission on Illumination (CIE): Vienna, Austria, 2014. [Google Scholar]
- CIE. CIE 15.3:2004: Colorimetry; CIE Central Bureau: Vienna, Austria, 2004. [Google Scholar]

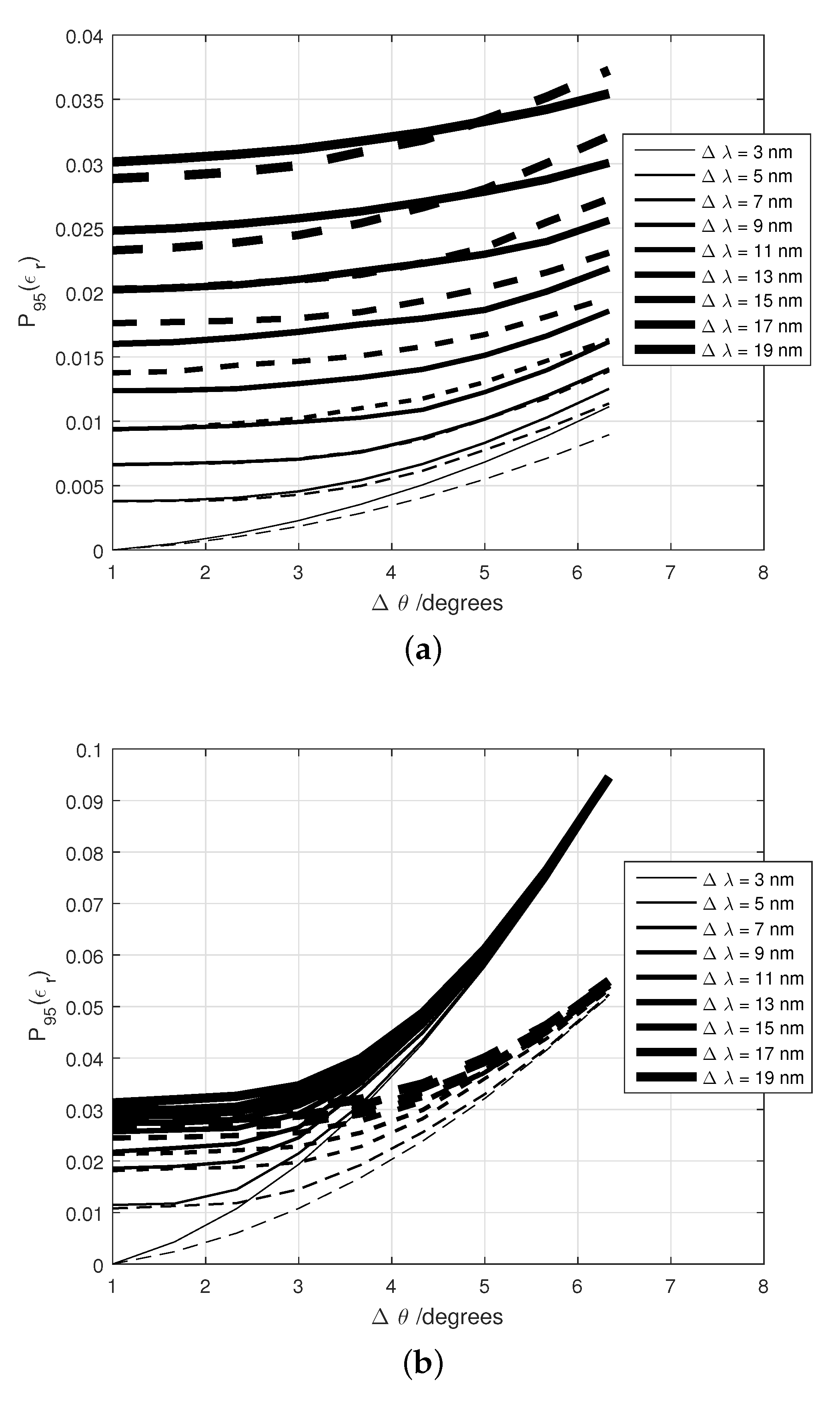
| Recommended Angular/Spectral Bandwidths | Target Relative Uncertainty (k = 2) |
|---|---|
| Goniochromatism Based on Interference Pigments | |
| ≤4°/3 nm | <0.5% |
| ≤5°/7 nm | <1% |
| ≤6°/11 nm | <2% |
| ≤6/17 nm | <3% |
| Goniochromatism based on diffraction pigments | |
| ≤2/3 nm | <1% |
| ≤3°/5 nm | <2% |
| ≤3°/11 nm | <3% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferrero, A.; Campos, J. Angular and Spectral Bandwidth Considerations in BRDF Measurements of Interference- and Diffraction-Based Coatings. Coatings 2020, 10, 1128. https://doi.org/10.3390/coatings10111128
Ferrero A, Campos J. Angular and Spectral Bandwidth Considerations in BRDF Measurements of Interference- and Diffraction-Based Coatings. Coatings. 2020; 10(11):1128. https://doi.org/10.3390/coatings10111128
Chicago/Turabian StyleFerrero, Alejandro, and Joaquín Campos. 2020. "Angular and Spectral Bandwidth Considerations in BRDF Measurements of Interference- and Diffraction-Based Coatings" Coatings 10, no. 11: 1128. https://doi.org/10.3390/coatings10111128
APA StyleFerrero, A., & Campos, J. (2020). Angular and Spectral Bandwidth Considerations in BRDF Measurements of Interference- and Diffraction-Based Coatings. Coatings, 10(11), 1128. https://doi.org/10.3390/coatings10111128





