Effective Boundary Correction for Deterministic Lateral Displacement Microchannels to Improve Cell Separation: A Numerical and Experimental Study
Abstract
1. Introduction
2. Materials and Methods
2.1. The DLD Theory
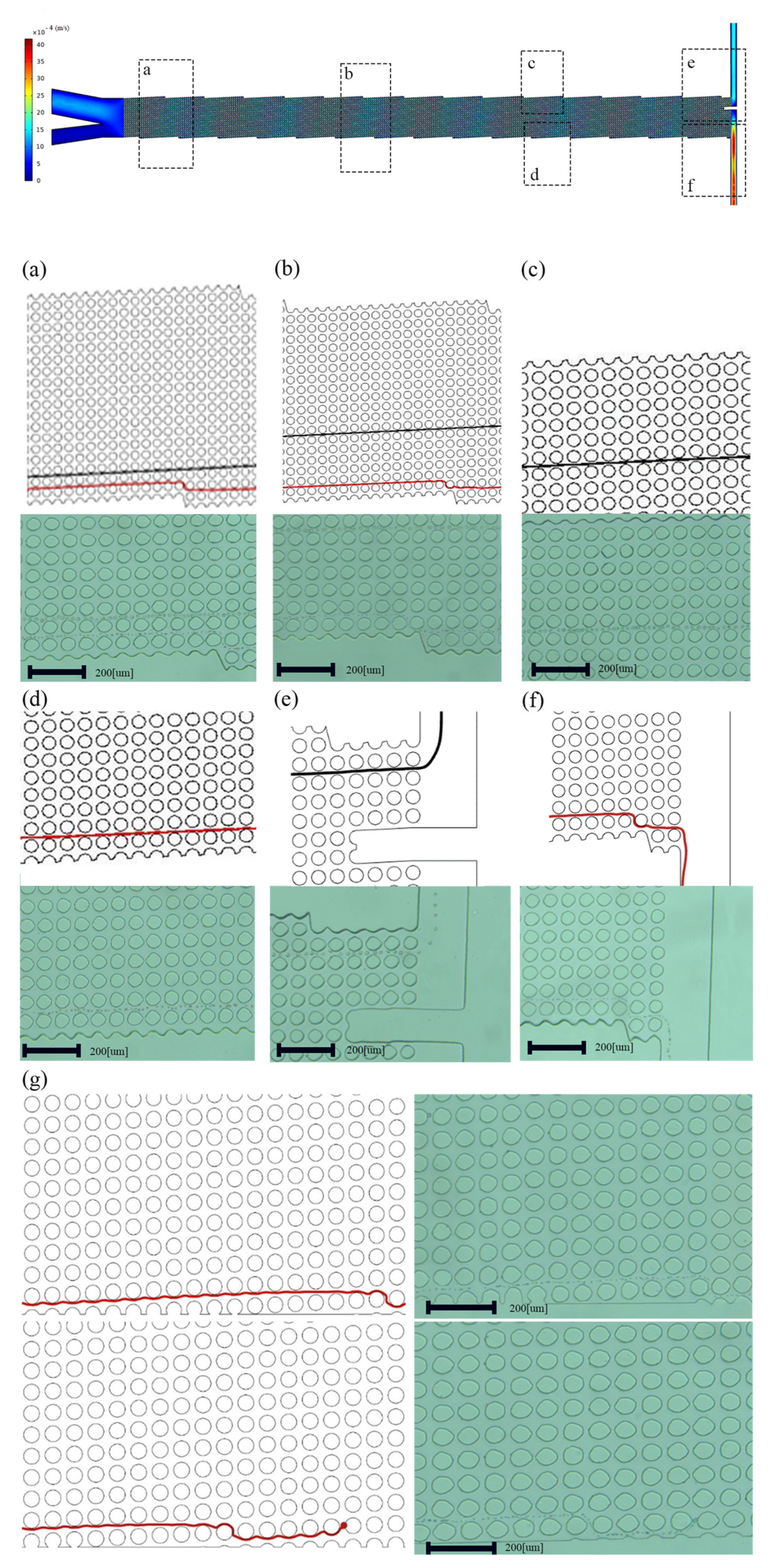
2.2. Developing Boundary Correction
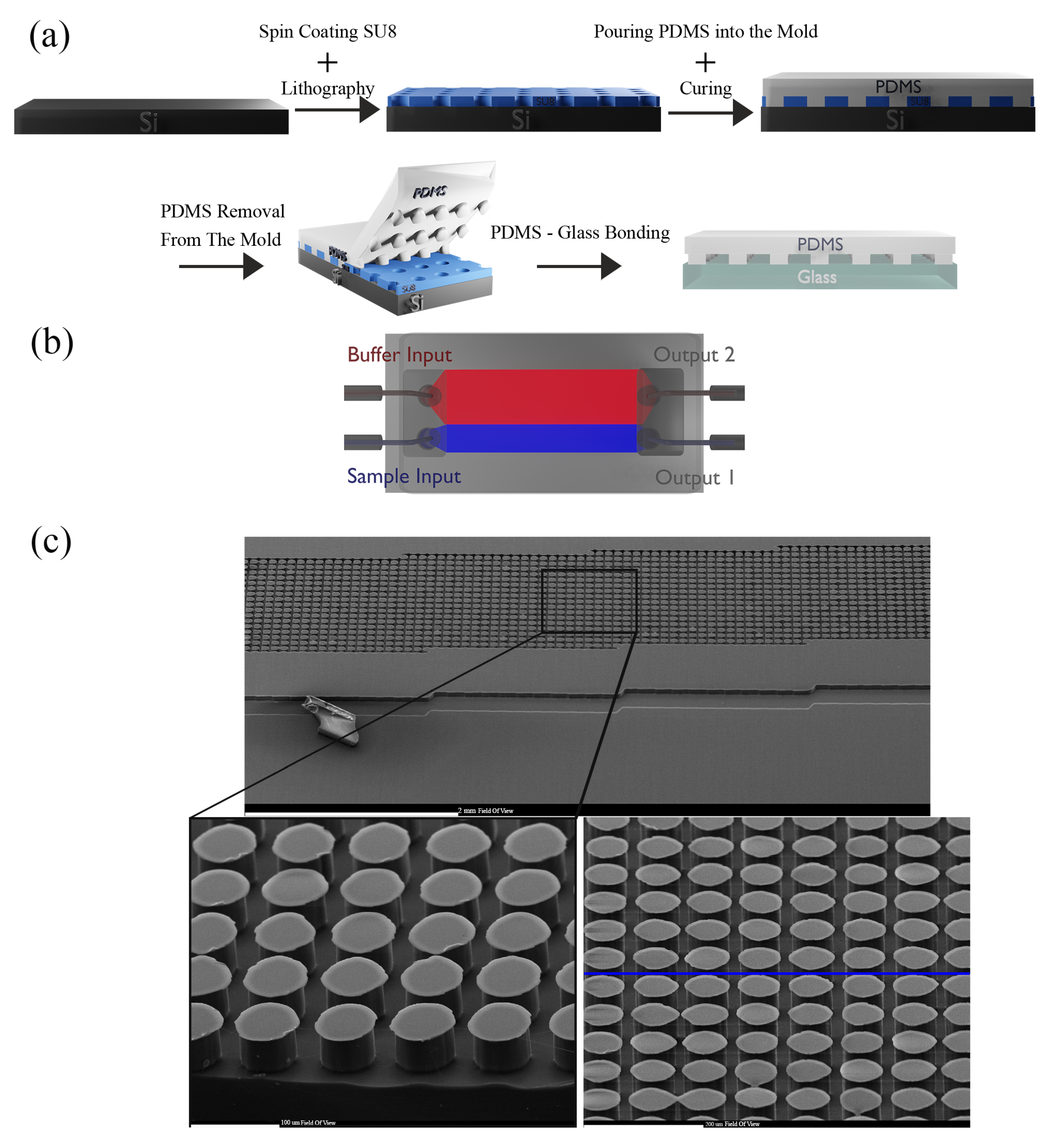
2.3. Fabrication
2.4. Cell Line Sample Preparation
3. Results and Discussion
3.1. Experimental Setups
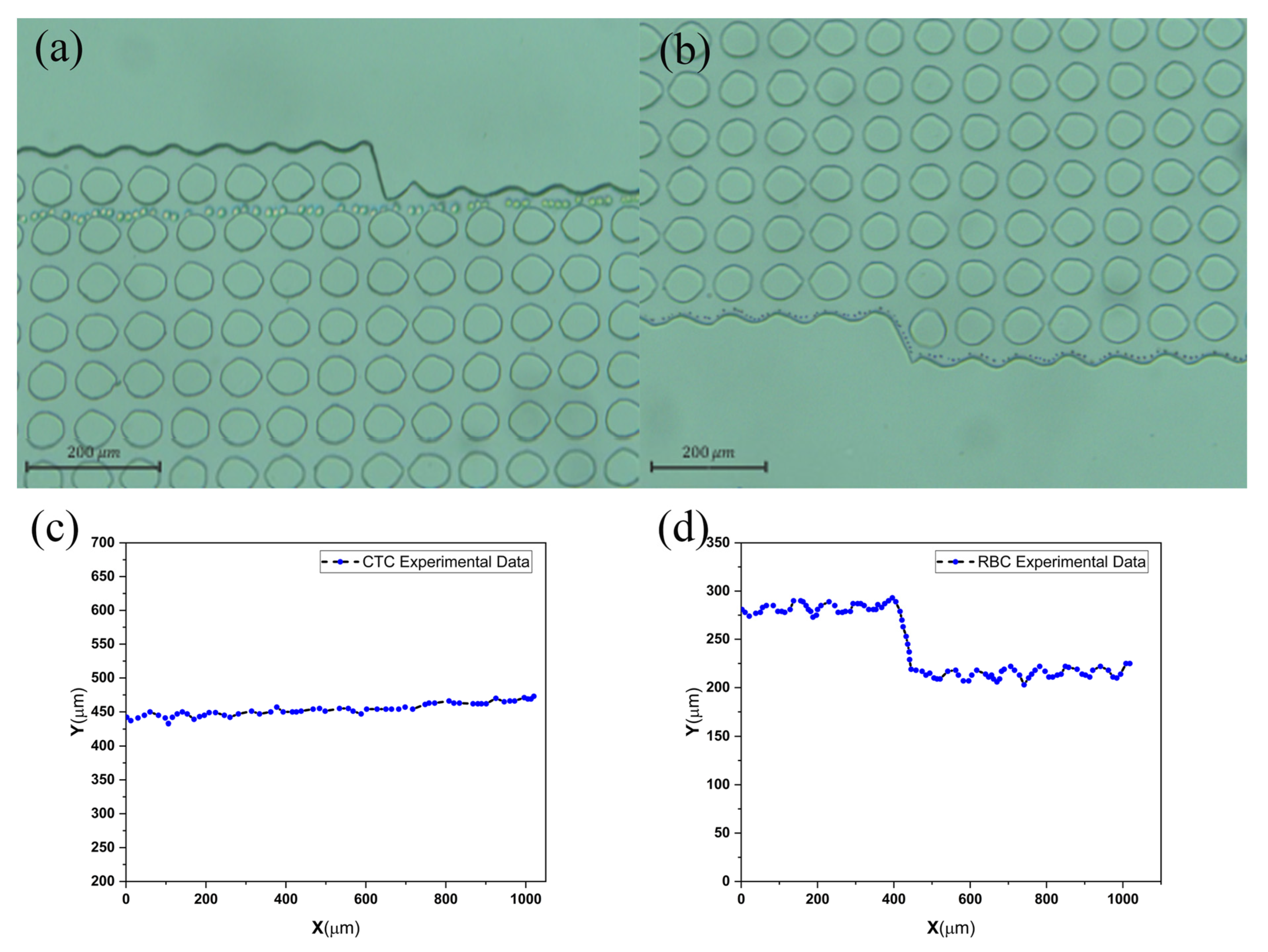
3.2. Tumor Cell Separation Characterization
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Whitesides, G.M. The Origins and the Future of Microfluidics. Nature 2006, 442, 368–373. [Google Scholar] [CrossRef] [PubMed]
- Cho, H.; Kim, J.; Han, K.H. An Assembly Disposable Degassing Microfluidic Device Using a Gas-Permeable Hydrophobic Membrane and a Reusable Microsupport Array. Sens. Actuators B Chem. 2019, 286, 353–361. [Google Scholar] [CrossRef]
- Yager, P.; Edwards, T.; Fu, E.; Helton, K.; Nelson, K.; Tam, M.R.; Weigl, B.H. Microfluidic Diagnostic Technologies for Global Public Health. Nature 2006, 442, 412–418. [Google Scholar] [CrossRef] [PubMed]
- MacDonald, M.P.; Spalding, G.C.; Dholakia, K. Microfluidic Sorting in an Optical Lattice. Nature 2003, 426, 421–424. [Google Scholar] [CrossRef] [PubMed]
- Janasek, D.; Franzke, J.; Manz, A. Scaling and the Design of Miniaturized Chemical-Analysis Systems. Nature 2006, 442, 374–380. [Google Scholar] [CrossRef] [PubMed]
- El-Ali, J.; Sorger, P.K.; Jensen, K.F. Cells on Chips. Nature 2006, 442, 403–411. [Google Scholar] [CrossRef]
- Tabatabaei, S.M.; Pourfath, M.; Fathipour, M. Adsorption Characteristics of Epigenetically Modified DNA Nucleobases on Single-Layer MoS2: A First-Principles Study. J. Appl. Phys. 2018, 124, 134501. [Google Scholar] [CrossRef]
- Tabatabaei, S.M.; Farshchi-Heydari, M.J.; Asad, M.; Fathipour, M. Unravelling the Physisorption Characteristics of H2S Molecule on Biaxially Strained Single-Layer MoS2. Nanoscale Adv. 2019, 1, 3452–3462. [Google Scholar] [CrossRef]
- Yang, D.K.; Yu, L.K.; Kuo, C.T.; Weng, J.H.; Leïchlé, T.; Swami, N.S.; Chen, L.C.; Wei, P.K.; Chou, C.F. Direct in Situ and Real-Time Visualization of Salt-Dependent Thiolated-DNA Immobilization and Biosensing on Gold in Nanofluidic Channels. Sens. Actuators B Chem. 2023, 394, 134303. [Google Scholar] [CrossRef]
- Ghorbani, S.; Bashirpour, M.; Poursafar, J.; Kolahdouz, M.; Neshat, M.; Valinejad, A. Thin Film Tandem Nanoplasmonic Photoconductive Antenna for High Performance Terahertz Detection. Superlattices Microstruct. 2018, 120, 598–604. [Google Scholar] [CrossRef]
- Beigi, F.; Mousavi, M.S.S.; Manteghi, F.; Kolahdouz, M. Doped Nafion-Layered Double Hydroxide Nanoparticles as a Modified Ionic Polymer Metal Composite Sheet for a High-Responsive Humidity Sensor. Appl. Clay Sci. 2018, 166, 131–136. [Google Scholar] [CrossRef]
- Sani, M.M.E.; Aliverdinia, M.; Javidi, R.; Mirhosseini, S.; Zand, M.M. Microstructured Droplet Based Porous Capacitive Pressure Sensor. In Proceedings of the 2022 29th National and 7th International Iranian Conference on Biomedical Engineering, ICBME 2022, Tehran, Iran, 21–22 December 2022; pp. 321–324. [Google Scholar] [CrossRef]
- Lee, Y.S.; Lu, Y.T.; Chang, C.M.; Liu, C.H. Finger-Powered Cell-Sorting Microsystem Chip for Cancer-Study Applications. Sens. Actuators B Chem. 2022, 370, 132430. [Google Scholar] [CrossRef]
- Huang, S.J.; Chang, C.M.; Lu, Y.T.; Liu, C.H. Microfluidic Biochip for Target Tumor Cell and Cell-Cluster Sorting. Sens. Actuators B Chem. 2023, 394, 134369. [Google Scholar] [CrossRef]
- Smith, J.P.; Barbati, A.C.; Santana, S.M.; Gleghorn, J.P.; Kirby, B.J. Microfluidic Transport in Microdevices for Rare Cell Capture. Electrophoresis 2012, 33, 3133–3142. [Google Scholar] [CrossRef] [PubMed]
- Kumar, V.; Rezai, P. Magneto-Hydrodynamic Fractionation (MHF) for Continuous and Sheathless Sorting of High-Concentration Paramagnetic Microparticles. Biomed. Microdevices 2017, 19, 39. [Google Scholar] [CrossRef]
- Autebert, J.; Coudert, B.; Bidard, F.C.; Pierga, J.Y.; Descroix, S.; Malaquin, L.; Viovy, J.L. Microfluidic: An Innovative Tool for Efficient Cell Sorting. Methods 2012, 57, 297–307. [Google Scholar] [CrossRef] [PubMed]
- Bhagat, A.A.S.; Bow, H.; Hou, H.W.; Tan, S.J.; Han, J.; Lim, C.T. Microfluidics for Cell Separation. Med. Biol. Eng. Comput. 2010, 48, 999–1014. [Google Scholar] [CrossRef] [PubMed]
- Rohani, A.; Moore, J.H.; Su, Y.H.; Stagnaro, V.; Warren, C.; Swami, N.S. Single-Cell Electro-Phenotyping for Rapid Assessment of Clostridium Difficile Heterogeneity under Vancomycin Treatment at Sub-MIC (Minimum Inhibitory Concentration) Levels. Sens. Actuators B Chem. 2018, 276, 472–480. [Google Scholar] [CrossRef]
- Khodaee, M.; Dalir, N.; Feghhi, F.; Ansari, N.; Mohammadimasoudi, M.; Goudarzi, A.; Nasiri, A.F.; Kolahdouz, M.; Mohseni, S.M. Enhancement in Electrical Conductivity of Liquid Crystals by Graphene Metal Oxide Composites. Sci. Rep. 2023, 13, 11688. [Google Scholar] [CrossRef]
- Vykoukal, J.; Vykoukal, D.M.; Freyberg, S.; Alt, E.U.; Gascoyne, P.R.C. Enrichment of Putative Stem Cells from Adipose Tissue Using Dielectrophoretic Field-Flow Fractionation. Lab Chip 2008, 8, 1386–1393. [Google Scholar] [CrossRef]
- Song, H.; Rosano, J.M.; Wang, Y.; Garson, C.J.; Prabhakarpandian, B.; Pant, K.; Klarmann, G.J.; Perantoni, A.; Alvarez, L.M.; Lai, E. Continuous-Flow Sorting of Stem Cells and Differentiation Products Based on Dielectrophoresis. Lab Chip 2015, 15, 1320–1328. [Google Scholar] [CrossRef] [PubMed]
- Adams, T.N.G.; Jiang, A.Y.L.; Vyas, P.D.; Flanagan, L.A. Separation of Neural Stem Cells by Whole Cell Membrane Capacitance Using Dielectrophoresis. Methods 2018, 133, 91–103. [Google Scholar] [CrossRef]
- Saneei Mousavi, M.S.; Karami, A.H.; Ghasemnejad, M.; Kolahdouz, M.; Manteghi, F.; Ataei, F. Design of a Remote-Control Drug Delivery Implantable Chip for Cancer Local on Demand Therapy Using Ionic Polymer Metal Composite Actuator. J. Mech. Behav. Biomed. Mater. 2018, 86, 250–256. [Google Scholar] [CrossRef] [PubMed]
- Dykes, J.; Lenshof, A.; Åstrand-Grundström, I.B.; Laurell, T.; Scheding, S. Efficient Removal of Platelets from Peripheral Blood Progenitor Cell Products Using a Novel Micro-Chip Based Acoustophoretic Platform. PLoS ONE 2011, 6, e23074. [Google Scholar] [CrossRef]
- Lenshof, A.; Jamal, A.; Dykes, J.; Urbansky, A.; Åstrand-Grundström, I.; Laurell, T.; Scheding, S. Efficient Purification of CD4+ Lymphocytes from Peripheral Blood Progenitor Cell Products Using Affinity Bead Acoustophoresis. Cytometry A 2014, 85, 933–941. [Google Scholar] [CrossRef] [PubMed]
- Nawaz, A.A.; Chen, Y.; Nama, N.; Nissly, R.H.; Ren, L.; Ozcelik, A.; Wang, L.; McCoy, J.P.; Levine, S.J.; Huang, T.J. Acoustofluidic Fluorescence Activated Cell Sorter. Anal. Chem. 2015, 87, 12051. [Google Scholar] [CrossRef]
- Faridi, M.A.; Ramachandraiah, H.; Iranmanesh, I.; Grishenkov, D.; Wiklund, M.; Russom, A. MicroBubble Activated Acoustic Cell Sorting. Biomed. Microdevices 2017, 19, 23. [Google Scholar] [CrossRef]
- Dehghan Nayeri, F.; Kolahdouz, M.; Asl-Soleimani, E.; Mohajerzadeh, S. Low Temperature Carving of ZnO Nanorods into Nanotubes for Dye-Sensitized Solar Cell Application. J. Alloys Compd. 2015, 633, 359–365. [Google Scholar] [CrossRef]
- Farasat, M.; Aalaei, E.; Kheirati Ronizi, S.; Bakhshi, A.; Mirhosseini, S.; Zhang, J.; Nguyen, N.-T.; Kashaninejad, N. Signal-Based Methods in Dielectrophoresis for Cell and Particle Separation. Biosensors 2022, 12, 510. [Google Scholar] [CrossRef]
- Huang, R.; Barber, T.A.; Schmidt, M.A.; Tompkins, R.G.; Toner, M.; Bianchi, D.W.; Kapur, R.; Flejter, W.L. A Microfluidics Approach for the Isolation of Nucleated Red Blood Cells (NRBCs) from the Peripheral Blood of Pregnant Women. Prenat. Diagn. 2008, 28, 892–899. [Google Scholar] [CrossRef]
- Jing, Y.; Moore, L.R.; Schneider, T.; Williams, P.S.; Chalmers, J.J.; Farag, S.S.; Bolwell, B.; Zborowski, M. Negative Selection of Hematopoietic Progenitor Cells by Continuous Magnetophoresis. Exp. Hematol. 2007, 35, 662–672. [Google Scholar] [CrossRef]
- Karabacak, N.M.; Spuhler, P.S.; Fachin, F.; Lim, E.J.; Pai, V.; Ozkumur, E.; Martel, J.M.; Kojic, N.; Smith, K.; Chen, P.I.; et al. Microfluidic, Marker-Free Isolation of Circulating Tumor Cells from Blood Samples. Nat. Protocols 2014, 9, 694–710. [Google Scholar] [CrossRef]
- Eskandarisani, M.; Aliverdinia, M.; Malakshah, V.M.; Mirhosseini, S.; Zand, M.M. Microstructured Porous Capacitive Bio-pressure Sensor Using Droplet-based Microfluidics. J. Med. Signals Sens. 2024, 13, 71–79. [Google Scholar] [CrossRef]
- Ni, C.; Chen, Y.; Zhou, Y.; Jiang, D.; Ni, Z.; Xiang, N. Inertia-Magnetic Microfluidics for Rapid and High-Purity Separation of Malignant Tumor Cells. Sens. Actuators B Chem. 2023, 397, 134619. [Google Scholar] [CrossRef]
- Ghaderiaram, A.; Bazrafshan, A.; Firouzi, K.; Kolahdouz, M. A Multi-Mode R-TENG for Self-Powered Anemometer under IoT Network. Nano Energy 2021, 87, 106170. [Google Scholar] [CrossRef]
- Xavier, M.; Holm, S.H.; Beech, J.P.; Spencer, D.; Tegenfeldt, J.O.; Oreffo, R.O.C.; Morgan, H. Label-Free Enrichment of Primary Human Skeletal Progenitor Cells Using Deterministic Lateral Displacement. Lab Chip 2019, 19, 513–523. [Google Scholar] [CrossRef]
- Sani, M.M.E.; Aliverdinia, M.; Zand, M.M. Numerical Study of Different Pillar Shapes Using Deterministic Lateral Displacement Method for Particle Separation. In Proceedings of the 2022 30th International Conference on Electrical Engineering, ICEE 2022, Tehran, Iran, 17–19 May 2022; pp. 469–473. [Google Scholar] [CrossRef]
- Kuo, J.N.; Lin, B.Y. Microfluidic Blood-Plasma Separation Chip Using Channel Size Filtration Effect. Microsyst. Technol. 2018, 24, 2063–2070. [Google Scholar] [CrossRef]
- Bowman, T.J.; Drazer, G.; Frechette, J. Inertia and Scaling in Deterministic Lateral Displacement. Biomicrofluidics 2013, 7, 064111. [Google Scholar] [CrossRef]
- Tottori, N.; Nisisako, T. High-Throughput Production of Satellite-Free Droplets through a Parallelized Microfluidic Deterministic Lateral Displacement Device. Sens. Actuators B Chem. 2018, 260, 918–926. [Google Scholar] [CrossRef]
- Murakami, T.; Koiwai, K.; Suzuki, H. Applying Deterministic Lateral Displacement Cell Separation on Immune Cells of Marine Shrimp. Sens. Actuators B Chem. 2021, 347, 130587. [Google Scholar] [CrossRef]
- Huang, L.R.; Cox, E.C.; Austin, R.H.; Sturm, J.C. Continuous Particle Separation through Deterministic Lateral Displacement. Science 2004, 304, 987–990. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.A.; Inglis, D.W.; Morton, K.J.; Lawrence, D.A.; Huang, L.R.; Chou, S.Y.; Sturm, J.C.; Austin, R.H. Deterministic Hydrodynamics: Taking Blood Apart. Proc. Natl. Acad. Sci. USA 2006, 103, 14779–14784. [Google Scholar] [CrossRef] [PubMed]
- Zheng, S.; Yung, R.; Tai, Y.C.; Kasdan, H. Determinstic Lateral Displacement MEMS Device for Continuous Blood Cell Separation. In Proceedings of the IEEE International Conference on Micro Electro Mechanical Systems (MEMS), Miami Beach, FL, USA, 30 January–3 February 2005; pp. 851–854. [Google Scholar] [CrossRef]
- Okano, H.; Konishi, T.; Suzuki, T.; Suzuki, T.; Ariyasu, S.; Aoki, S.; Abe, R.; Hayase, M. Enrichment of Circulating Tumor Cells in Tumor-Bearing Mouse Blood by a Deterministic Lateral Displacement Microfluidic Device. Biomed. Microdevices 2015, 17, 1–11. [Google Scholar] [CrossRef]
- Mehendale, N.; Sharma, O.; D’Costa, C.; Paul, D. A Radial Pillar Device (RAPID) for Continuous and High-Throughput Separation of Multi-Sized Particles. Biomed. Microdevices 2017, 20, 6. [Google Scholar] [CrossRef]
- Chen, Y.; Zeming, K.K.; Hu, X.; Wei, S.; Xu, J.; Yan, H.; Liu, L. Deterministic Flow Cytometry: Rapid Erythrocyte Rheological Profiling from a Drop of Whole Blood. Sens. Actuators B Chem. 2022, 362, 131783. [Google Scholar] [CrossRef]
- Kolahdouz, M.; Maresca, L.; Ostling, M.; Riley, D.; Wise, R.; Radamson, H.H. New Method to Calibrate the Pattern Dependency of Selective Epitaxy of SiGe Layers. Solid. State Electron. 2009, 53, 858–861. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, X. Dielectrophoretic Microfluidic Device for Separation of Red Blood Cells and Platelets: A Model-Based Study. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 89. [Google Scholar] [CrossRef]
- Guzniczak, E.; Otto, O.; Whyte, G.; Chandra, T.; Robertson, N.A.; Willoughby, N.; Jimenez, M.; Bridle, H. Purifying Stem Cell-Derived Red Blood Cells: A High-Throughput Label-Free Downstream Processing Strategy Based on Microfluidic Spiral Inertial Separation and Membrane Filtration. Biotechnol. Bioeng. 2020, 117, 2032–2045. [Google Scholar] [CrossRef] [PubMed]
- Aliverdinia, M.; Eskandarisani, M.; Moghaddam, E.A.; Zand, M. M Numerical Study of Particle Focusing and Concentration under the Effect of Acoustic Waves in a Microchannel. In Proceedings of the 2022 29th National and 7th International Iranian Conference on Biomedical Engineering, ICBME 2022, Tehran, Iran, 21–22 December 2022; pp. 76–80. [Google Scholar] [CrossRef]
- VanDelinder, V.; Groisman, A. Perfusion in Microfluidic Cross-Flow: Separation of White Blood Cells from Whole Blood and Exchange of Medium in a Continuous Flow. Anal. Chem. 2007, 79, 2023–2030. [Google Scholar] [CrossRef]
- Inglis, D.W.; Morton, K.J.; Davis, J.A.; Zieziulewicz, T.J.; Lawrence, D.A.; Austin, R.H.; Sturm, J.C. Microfluidic Device for Label-Free Measurement of Platelet Activation. Lab Chip 2008, 8, 925–931. [Google Scholar] [CrossRef]
- Loutherback, K.; D’Silva, J.; Liu, L.; Wu, A.; Austin, R.H.; Sturm, J.C. Deterministic Separation of Cancer Cells from Blood at 10 ML/Min. AIP Adv. 2012, 2, 042107. [Google Scholar] [CrossRef] [PubMed]
- Au, S.H.; Edd, J.; Stoddard, A.E.; Wong, K.H.K.; Fachin, F.; Maheswaran, S.; Haber, D.A.; Stott, S.L.; Kapur, R.; Toner, M. Microfluidic Isolation of Circulating Tumor Cell Clusters by Size and Asymmetry. Sci. Rep. 2017, 7, 2433. [Google Scholar] [CrossRef] [PubMed]
- Nora Dickson, M.; Tsinberg, P.; Tang, Z.; Bischoff, F.Z.; Wilson, T.; Leonard, E.F. Efficient Capture of Circulating Tumor Cells with a Novel Immunocytochemical Microfluidic Device. Biomicrofluidics 2011, 5, 034119. [Google Scholar] [CrossRef] [PubMed]
- Inglis, D.W.; Herman, N.; Vesey, G. Highly Accurate Deterministic Lateral Displacement Device and Its Application to Purification of Fungal Spores. Biomicrofluidics 2010, 4, 024109. [Google Scholar] [CrossRef] [PubMed]
- Beech, J.P.; Ho, B.D.; Garriss, G.; Oliveira, V.; Henriques-Normark, B.; Tegenfeldt, J.O. Separation of Pathogenic Bacteria by Chain Length. Anal. Chim. Acta 2018, 1000, 223–231. [Google Scholar] [CrossRef] [PubMed]
- Holm, S.H.; Beech, J.P.; Barrett, M.P.; Tegenfeldt, J.O. Separation of Parasites from Human Blood Using Deterministic Lateral Displacement. Lab Chip 2011, 11, 1326–1332. [Google Scholar] [CrossRef] [PubMed]
- Wunsch, B.H.; Smith, J.T.; Gifford, S.M.; Wang, C.; Brink, M.; Bruce, R.L.; Austin, R.H.; Stolovitzky, G.; Astier, Y. Nanoscale Lateral Displacement Arrays for the Separation of Exosomes and Colloids down to 20 Nm. Nat. Nanotechnol. 2016, 11, 936–940. [Google Scholar] [CrossRef] [PubMed]
- Zeming, K.K.; Salafi, T.; Shikha, S.; Zhang, Y. Fluorescent Label-Free Quantitative Detection of Nano-Sized Bioparticles Using a Pillar Array. Nat. Commun. 2018, 9, 1254. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.; Yu, Z.; Chen, D.; Wang, Z.; Miao, J.; Li, Q.; Zhang, D.; Song, J.; Cui, D. Progress in Microfluidics-Based Exosome Separation and Detection Technologies for Diagnostic Applications. Small 2020, 16, 1903916. [Google Scholar] [CrossRef]
- Minc, N.; Fütterer, C.; Dorfman, K.D.; Bancaud, A.; Gosse, C.; Goubault, C.; Viovy, J.L. Quantitative Microfluidic Separation of DNA in Self-Assembled Magnetic Matrixes. Anal. Chem. 2004, 76, 3770–3776. [Google Scholar] [CrossRef]
- Salafi, T.; Zhang, Y.; Zhang, Y. A Review on Deterministic Lateral Displacement for Particle Separation and Detection. Nano-Micro Lett. 2019, 11, 1–33. [Google Scholar] [CrossRef] [PubMed]
- Inglis, D.W.; Davis, J.A.; Austin, R.H.; Sturm, J.C.; Huang, L.R.; Cox, E.C.; Austin, R.H.; Sturm, J.C. Critical Particle Size for Fractionation by Deterministic Lateral Displacement. Lab Chip 2006, 6, 655–658. [Google Scholar] [CrossRef] [PubMed]
- Joensson, H.N.; Uhlén, M.; Svahn, H.A. Droplet Size Based Separation by Deterministic Lateral Displacement—Separating Droplets by Cell-Induced Shrinking. Lab Chip 2011, 11, 1305–1310. [Google Scholar] [CrossRef]
- Tottori, N.; Hatsuzawa, T.; Nisisako, T. Separation of Main and Satellite Droplets in a Deterministic Lateral Displacement Microfluidic Device. RSC Adv. 2017, 7, 35516–35524. [Google Scholar] [CrossRef]
- Dezhkam, R.; Souderijani, A.S.; Shamloo, A.; Eskandarisani, M. and Mashhadian, A. Numerical investigation of centrifugal passive cell separation in three types of serpentine microchannels and comparison with fixed platform. J. Ind. Eng. Chem. 2023, 124, 240–249. [Google Scholar] [CrossRef]
- Jiang, J.; Zhao, H.; Shu, W.; Tian, J.; Huang, Y.; Song, Y.; Wang, R.; Li, E.; Slamon, D.; Hou, D.; et al. An Integrated Microfluidic Device for Rapid and High-Sensitivity Analysis of Circulating Tumor Cells. Sci. Rep. 2017, 7, 42612. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Liu, Z.; Huang, F.; Du, J.; Shu, W.; Feng, H.; Xu, X. Rapid Isolation of Cancer Cells Using Microfluidic Deterministic Lateral Displacement Structure. Biomicrofluidics 2013, 7, 11801. [Google Scholar] [CrossRef]
- Li, N.; Kamei, D.T.; Ho, C.M. On-Chip Continuous Blood Cell Subtype Separation by Deterministic Lateral Displacement. In Proceedings of the 2nd IEEE International Conference on Nano/Micro Engineered and Molecular Systems, IEEE NEMS 2007, Bangkok, Thailand, 16–19 January 2007; pp. 932–936. [Google Scholar] [CrossRef]
- Pariset, E.; Parent, C.; Fouillet, Y.; François, B.; Verplanck, N.; Revol-Cavalier, F.; Thuaire, A.; Agache, V. Separation of Biological Particles in a Modular Platform of Cascaded Deterministic Lateral Displacement Modules. Sci. Rep. 2018, 8, 17762. [Google Scholar] [CrossRef]
- Pariset, E.; Pudda, C.; Boizot, F.; Verplanck, N.; Frédéric, R.C.; Berthier, J.; Thuaire, A.; Agache, V. Purification of Complex Samples: Implementation of a Modular and Reconfigurable Droplet-Based Microfluidic Platform with Cascaded Deterministic Lateral Displacement Separation Modules. PLoS ONE 2018, 13, e0197629. [Google Scholar] [CrossRef]
- Raj, M.K.; Chakraborty, S. PDMS Microfluidics: A Mini Review. J. Appl. Polym. Sci. 2020, 137, 48958. [Google Scholar] [CrossRef]
- Di Toma, A.; Brunetti, G.; Chiriacò, M.S.; Ferrara, F.; Ciminelli, C. A Novel Hybrid Platform for Live/Dead Bacteria Accurate Sorting by On-Chip DEP Device. Int. J. Mol. Sci. 2023, 24, 7077. [Google Scholar] [CrossRef] [PubMed]
- Ho, B.D.; Beech, J.P.; Tegenfeldt, J.O. Cell Sorting Using Electrokinetic Deterministic Lateral Displacement. Micromachines 2020, 12, 30. [Google Scholar] [CrossRef] [PubMed]
- Lu, N.; Tay, H.M.; Petchakup, C.; He, L.; Gong, L.; Maw, K.K.; Leong, S.Y.; Lok, W.W.; Ong, H.B.; Guo, R.; et al. Label-Free Microfluidic Cell Sorting and Detection for Rapid Blood Analysis. Lab Chip 2023, 23, 1226–1257. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.A. Microfluidic Separation of Blood Components through Deterministic Lateral Displacement. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 2008. [Google Scholar]
- Inglis, D. Microfluidic Devices for Cell Separation. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 2007. [Google Scholar]
- Bhagat, A.A.S.; Kuntaegowdanahalli, S.S.; Papautsky, I. Continuous Particle Separation in Spiral Microchannels Using Dean Flows and Differential Migration. Lab Chip 2008, 8, 1906–1914. [Google Scholar] [CrossRef]
- Kuntaegowdanahalli, S.S.; Bhagat, A.A.S.; Kumar, G.; Papautsky, I. Inertial Microfluidics for Continuous Particle Separation in Spiral Microchannels. Lab Chip 2009, 9, 2973–2980. [Google Scholar] [CrossRef]
- Rohani Rad, E.; Farajpour, M.R. Dynamics Analysis of Microparticles in Inertial Microfluidics for Biomedical Applications. J. Comput. Appl. Mech. 2019, 50, 157–164. [Google Scholar] [CrossRef]
- Rohani Rad, E.; Farajpour, M.R. Influence of Taxol and CNTs on the Stability Analysis of Protein Microtubules. J. Comput. Appl. Mech. 2019, 50, 140–147. [Google Scholar] [CrossRef]
- Mirhosseini, S.; Nasiri, A.F.; Khatami, F.; Mirzaei, A.; Aghamir, S.M.K.; Kolahdouz, M. A Digital Image Colorimetry System Based on Smart Devices for Immediate and Simultaneous Determination of Enzyme-Linked Immunosorbent Assays. Sci. Rep. 2024, 14, 2587. [Google Scholar] [CrossRef]
- Wang, C.; Yang, L.; Wang, Z.; He, J.; Shi, Q. Highly Multiplexed Profiling of Cell Surface Proteins on Single Circulating Tumor Cells Based on Antibody and Cellular Barcoding. Anal. Bioanal. Chem. 2019, 411, 5373–5382. [Google Scholar] [CrossRef]



| Dimension | Value |
|---|---|
| Post diameter (Dpost) | 50 [µm] |
| Gap between posts in a row (g) | 50 [µm] |
| Gap between rows (Dy) | g |
| (λ) | G + Dpost |
| Post shifts between nearby rows (Δλ) | 4 [µm] |
| Period (N) | λ/Δλ |
| Post shift ratio (ε) | 1/N |
| Critical diameter (Dc) | 1.4 × g × (ε)0.48 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mirhosseini, S.; Eskandarisani, M.; Faghih Nasiri, A.; Khatami, F.; Mirzaei, A.; Badieirostami, M.; Aghamir, S.M.K.; Kolahdouz, M. Effective Boundary Correction for Deterministic Lateral Displacement Microchannels to Improve Cell Separation: A Numerical and Experimental Study. Biosensors 2024, 14, 466. https://doi.org/10.3390/bios14100466
Mirhosseini S, Eskandarisani M, Faghih Nasiri A, Khatami F, Mirzaei A, Badieirostami M, Aghamir SMK, Kolahdouz M. Effective Boundary Correction for Deterministic Lateral Displacement Microchannels to Improve Cell Separation: A Numerical and Experimental Study. Biosensors. 2024; 14(10):466. https://doi.org/10.3390/bios14100466
Chicago/Turabian StyleMirhosseini, Shaghayegh, Mohammadmahdi Eskandarisani, Aryanaz Faghih Nasiri, Fatemeh Khatami, Akram Mirzaei, Majid Badieirostami, Seyed Mohammad Kazem Aghamir, and Mohammadreza Kolahdouz. 2024. "Effective Boundary Correction for Deterministic Lateral Displacement Microchannels to Improve Cell Separation: A Numerical and Experimental Study" Biosensors 14, no. 10: 466. https://doi.org/10.3390/bios14100466
APA StyleMirhosseini, S., Eskandarisani, M., Faghih Nasiri, A., Khatami, F., Mirzaei, A., Badieirostami, M., Aghamir, S. M. K., & Kolahdouz, M. (2024). Effective Boundary Correction for Deterministic Lateral Displacement Microchannels to Improve Cell Separation: A Numerical and Experimental Study. Biosensors, 14(10), 466. https://doi.org/10.3390/bios14100466






