The Temperature-Sensitive Anisotropic Negative Poisson’s Ratio of Carbon Honeycomb
Abstract
1. Introduction
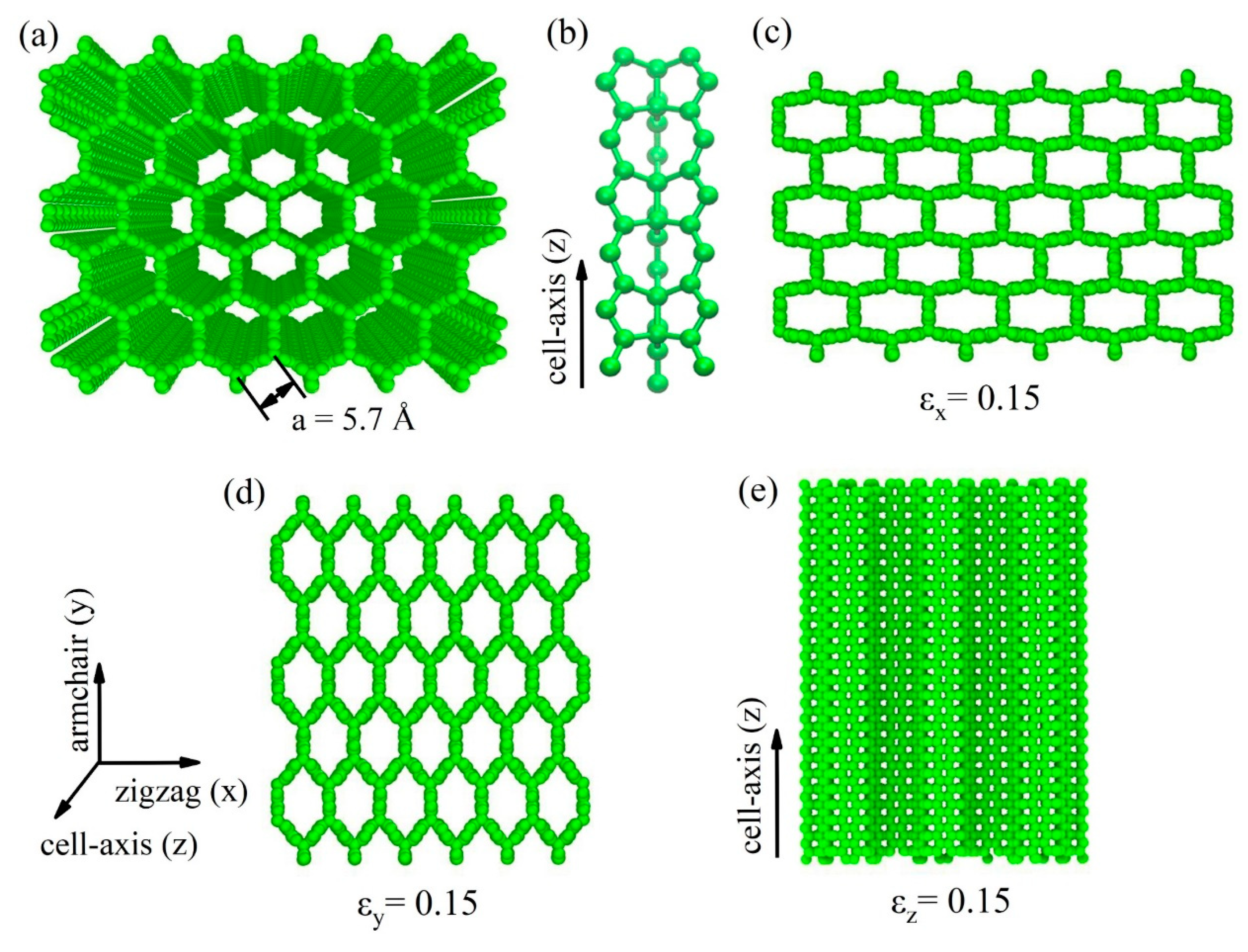
2. Computational Details
3. Results and Discussion
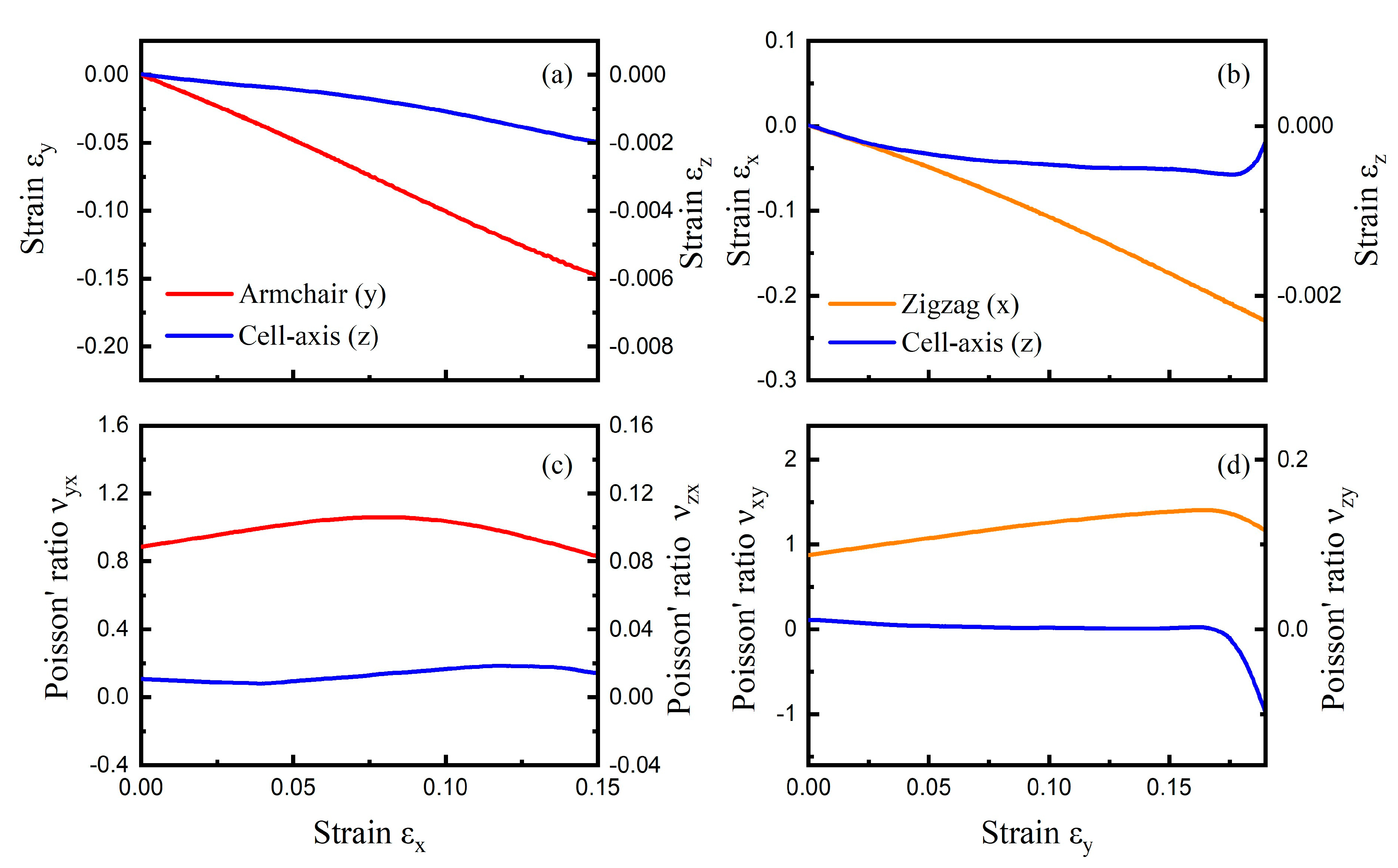
3.1. Tensile Tests
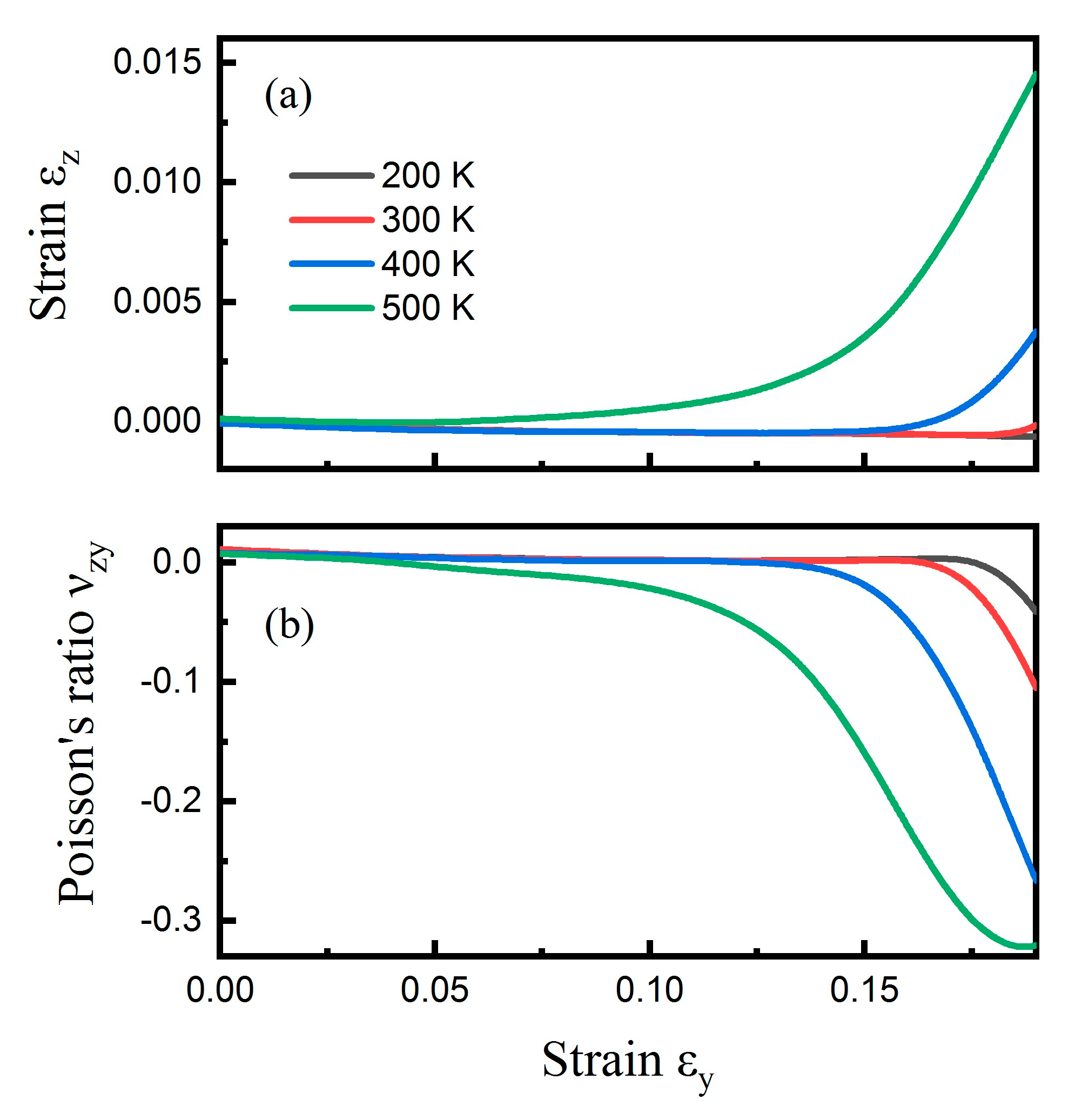
3.2. Temperature Effect for NA Transition
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Data Availability
References
- Evans, K.E. Auxetic polymers: A new range of materials. Endeavour 1991, 15, 170–174. [Google Scholar] [CrossRef]
- Lakes, R.S. Negative-Poisson’s-ratio materials: Auxetic solids. Annu. Rev. Mater. Res. 2017, 47, 63–81. [Google Scholar] [CrossRef]
- Bhullar, S.K. Influence of negative poisson’s ratio on stent applications. Adv. Mater. 2013, 2, 42–47. [Google Scholar] [CrossRef]
- Almgren, R.F. An isotropic three-dimensional structure with Poisson’s ratio = −1. J. Elast. 1985, 15, 427–430. [Google Scholar]
- Pinto, A.M.; Gonçalves, I.C.; Magalhães, F.D. Graphene-based materials biocompatibility: A review. Colloids Surf. B Biointerfaces 2013, 111, 188–202. [Google Scholar] [CrossRef]
- Lira, C.; Scarpa, F.; Rajasekaran, R. A Gradient Cellular Core for Aeroengine Fan Blades Based on Auxetic Configurations. J. Intell. Mater. Syst. Struct. 2011, 22, 907–917. [Google Scholar] [CrossRef]
- Saxena, K.K.; Das, R.; Calius, E.P. Three decades of auxetics research-materials with negative poisson’s ratio: A review. Adv. Eng. Mater. 2016, 18, 1847–1870. [Google Scholar] [CrossRef]
- Wojciechowski, K.W. Constant thermodynamic tension Monte Carlo studies of elastic properties of a two-dimensional system of hard cyclic hexamers. Mol. Phys. 1987, 61, 1247–1258. [Google Scholar] [CrossRef]
- Wojciechowski, K.W. Two-dimensional isotropic system with a negative poisson ratio. Phys. Lett. A 1989, 137, 60–64. [Google Scholar] [CrossRef]
- Du, Y.; Maassen, J.; Wu, W.; Luo, Z.; Xu, X.; Ye, P.D. Auxetic black phosphorus: A 2D material with negative poisson’s ratio. Nano Lett. 2016, 16, 6701–6708. [Google Scholar] [CrossRef]
- Han, J.; Xie, J.; Zhang, Z.; Yang, D.; Si, M.; Xue, D. Negative Poisson’s ratios in few-layer orthorhombic arsenic: First-principles calculations. Appl. Phys. Express 2015, 8, 041801. [Google Scholar] [CrossRef]
- Ulissi, Z.W.; Govind Rajan, A.; Strano, M.S. Persistently auxetic materials: Engineering the poisson ratio of 2D Self-Avoiding membranes under conditions of Non-Zero Anisotropic Strain. ACS Nano 2016, 10, 7542–7549. [Google Scholar] [CrossRef] [PubMed]
- Jiang, J.-W.; Kim, S.Y.; Park, H.S. Auxetic nanomaterials: Recent progress and future development. Appl. Phys. Rev. 2016, 3, 041101. [Google Scholar] [CrossRef]
- Jiang, J.W.; Chang, T.; Guo, X.; Park, H.S. Intrinsic Negative Poisson’s Ratio for Single-Layer Graphene. Nano Lett. 2016, 16, 5286–5290. [Google Scholar] [CrossRef]
- Deng, B.; Hou, J.; Zhu, H.; Liu, S.; Liu, E.; Shi, Y.; Peng, Q. The normal-auxeticity mechanical phase transition in graphene. 2D Mater. 2017, 4, 021020. [Google Scholar] [CrossRef]
- Coluci, V.R.; Hall, L.J.; Kozlov, M.E.; Zhang, M.; Dantas, S.O.; Galvão, D.S.; Baughman, R.H. Modeling the auxetic transition for carbon nanotube sheets. Phys. Rev. B 2008, 78, 115408. [Google Scholar] [CrossRef]
- Ma, Y.J.; Yao, X.F.; Zheng, Q.S.; Yin, Y.J.; Jiang, D.J.; Xu, J.H.; Wei, F.; Zhang, Q. Carbon nanotube films change Poisson’s ratios from negative to positive. Appl. Phys. Lett. 2010, 97, 061909. [Google Scholar] [CrossRef]
- Hall, L.J.; Coluci, V.R.; Galvão, D.S.; Kozlov, M.E.; Zhang, M.; Dantas, S.O.; Baughman, R.H. Sign Change of Poisson’s Ratio for Carbon Nanotube Sheets. Science 2008, 320, 504–507. [Google Scholar] [CrossRef]
- Poncharal, P. Electrostatic Deflections and Electromechanical Resonances of Carbon Nanotubes. Science 1999, 283, 1513–1516. [Google Scholar] [CrossRef]
- Treacy, M.M.J.; Ebbesen, T.W.; Gibson, J.M. Exceptionally high Young’s modulus observed for individual carbon nanotubes. Nature 1996, 381, 678–680. [Google Scholar] [CrossRef]
- Dikin, D.A.; Stankovich, S.; Zimney, E.J.; Piner, R.D.; Dommett, G.H.; Evmenenko, G.; Nguyen, S.T.; Ruoff, R.S. Preparation and characterization of graphene oxide paper. Nature 2007, 448, 457–460. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, S.; Bao, W.; Nika, D.L.; Subrina, S.; Pokatilov, E.P.; Lau, C.N.; Balandin, A.A. Dimensional crossover of thermal transport in few-layer graphene. Nat. Mater. 2010, 9, 555–558. [Google Scholar] [CrossRef]
- Wang, S.; Wu, D.; Yang, B.; Ruckenstein, E.; Chen, H. Semimetallic carbon honeycombs: New three-dimensional graphene allotropes with Dirac cones. Nanoscale 2018, 10, 2748–2754. [Google Scholar] [CrossRef] [PubMed]
- Pang, Z.; Gu, X.; Wei, Y.; Yang, R.; Dresselhaus, M.S. Bottom-up Design of Three-Dimensional Carbon-Honeycomb with Superb Specific Strength and High Thermal Conductivity. Nano Lett. 2017, 17, 179–185. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Wang, C. Buckling of Carbon Honeycombs: A New Mechanism for Molecular Mass Transportation. J. Phys. Chem. C 2017, 121, 8196–8203. [Google Scholar] [CrossRef]
- Zhang, Z.; Kutana, A.; Yang, Y.; Krainyukova, N.V.; Penev, E.S.; Yakobson, B.I. Nanomechanics of carbon honeycomb cellular structures. Carbon 2017, 113, 26–32. [Google Scholar] [CrossRef]
- Gu, X.; Pang, Z.; Wei, Y.; Yang, R. On the influence of junction structures on the mechanical and thermal properties of carbon honeycombs. Carbon 2017, 119, 278–286. [Google Scholar] [CrossRef]
- Park, N.; Ihm, J. Electronic structure and mechanical stability of the graphitic honeycomb lattice. Phys. Rev. B 2000, 62, 7614–7618. [Google Scholar] [CrossRef]
- Krainyukova, N.V.; Zubarev, E.N. Carbon Honeycomb High Capacity Storage for Gaseous and Liquid Species. Phys. Rev. Lett. 2016, 116, 055501. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Donald, W.B.; Shenderova, O.A.; Harrison, J.A.; Stuart, S.J.; Ni, B.; Sinnott, S.B. A second-generation reactive empirical bond order (REBO) potential energy expression for hydrocarbons. J. Phys. Condens. Matter 2002, 14, 783. [Google Scholar]
- Xie, L.; An, H.; He, C.; Qin, Q.; Peng, Q. Mechanical Properties of Vacancy Tuned Carbon Honeycomb. Nanomaterials 2019, 9, 156. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Dong, L.; Lakes, R.S. The properties of copper foams with negative Poisson’s ratio via resonant ultrasound spectroscopy. Phys. Status Solidi (b) 2013, 250, 1983–1987. [Google Scholar] [CrossRef]
- Grima, J.N.; Winczewski, S.; Mizzi, L.; Grech, M.C.; Cauchi, R.; Gatt, R.; Attard, D.; Wojciechowski, K.W.; Rybicki, J. Tailoring Graphene to Achieve Negative Poisson’s Ratio Properties. Adv. Mater. 2015, 27, 1455–1459. [Google Scholar] [CrossRef]
- Hoover, W.G.; Hoover, C.G. Searching for auxetics with DYNA3D and ParaDyn. Phys. Status Solidi (b) 2005, 242, 585–594. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; He, C.; Xie, L.; Peng, Q. The Temperature-Sensitive Anisotropic Negative Poisson’s Ratio of Carbon Honeycomb. Nanomaterials 2019, 9, 487. https://doi.org/10.3390/nano9040487
Wang W, He C, Xie L, Peng Q. The Temperature-Sensitive Anisotropic Negative Poisson’s Ratio of Carbon Honeycomb. Nanomaterials. 2019; 9(4):487. https://doi.org/10.3390/nano9040487
Chicago/Turabian StyleWang, Wenrui, Chenwei He, Lu Xie, and Qing Peng. 2019. "The Temperature-Sensitive Anisotropic Negative Poisson’s Ratio of Carbon Honeycomb" Nanomaterials 9, no. 4: 487. https://doi.org/10.3390/nano9040487
APA StyleWang, W., He, C., Xie, L., & Peng, Q. (2019). The Temperature-Sensitive Anisotropic Negative Poisson’s Ratio of Carbon Honeycomb. Nanomaterials, 9(4), 487. https://doi.org/10.3390/nano9040487





