An Overview of Mechanical Properties of Diamond-like Phases under Tension
Abstract
1. Introduction
2. Simulation Details
3. Results and Discussion
4. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| DLPs | diamond-like phases |
| MD | molecular dynamics |
| CNT | carbon nanotubes |
| RDF | radial distribution function |
References
- Torelli, M.D.; Nunn, N.A.; Jones, Z.R.; Vedelaar, T.; Padamati, S.K.; Schirhagl, R.; Hamers, R.J.; Shames, A.I.; Danilov, E.O.; Zaitsev, A.; et al. High Temperature Treatment of Diamond Particles Toward Enhancement of Their Quantum Properties. Front. Phys. 2020, 8, 205. [Google Scholar] [CrossRef]
- Nunn, N.; Milikisiyants, S.; Torelli, M.D.; Monge, R.; Delord, T.; Shames, A.I.; Meriles, C.A.; Ajoy, A.; Smirnov, A.I.; Shenderova, O.A. Optical and electronic spin properties of fluorescent micro- and nanodiamonds upon prolonged ultrahigh-temperature annealing. J. Vac. Sci. Technol. B 2023, 41, 042206. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.Z.; Lian, C.S.; Xu, J.; Xu, L.F.; Wang, J.T.; Chen, C. Computational prediction of body-centered cubic carbon in an all-six-member ring configuration. Phys. Rev. B 2015, 91, 214106. [Google Scholar] [CrossRef]
- Li, Q.; Ma, Y.; Oganov, A.R.; Wang, H.; Wang, H.; Xu, Y.; Cui, T.; Mao, H.K.; Zou, G. Superhard Monoclinic Polymorph of Carbon. Phys. Rev. Lett. 2009, 102, 175506. [Google Scholar] [CrossRef] [PubMed]
- Mao, W.L.; Mao, H.k.; Eng, P.J.; Trainor, T.P.; Newville, M.; Kao, C.c.; Heinz, D.L.; Shu, J.; Meng, Y.; Hemley, R.J. Bonding Changes in Compressed Superhard Graphite. Science 2003, 302, 425–427. [Google Scholar] [CrossRef] [PubMed]
- Komlenok, M.; Kudryavtsev, O.; Pasternak, D.; Vlasov, I.; Konov, V. Laser printing of diamond nanoparticles with luminescent SiV center. Comput. Opt. 2021, 45, 860–864. [Google Scholar] [CrossRef]
- Liu, P.; Cui, H.; Yang, G.W. Synthesis of Body-Centered Cubic Carbon Nanocrystals. Cryst. Growth Des. 2008, 8, 581–586. [Google Scholar] [CrossRef]
- Knudson, M.D.; Desjarlais, M.P.; Dolan, D.H. Shock-Wave Exploration of the High-Pressure Phases of Carbon. Science 2008, 322, 1822–1825. [Google Scholar] [CrossRef]
- Wang, J.T.; Chen, C.; Kawazoe, Y. New cubic carbon phase via graphitic sheet rumpling. Phys. Rev. B 2012, 85, 214104. [Google Scholar] [CrossRef]
- Bu, K.; Wang, J.T.; Li, Z.Z.; Mizuseki, H.; Kawazoe, Y. A superhard orthorhombic carbon with all six-membered-ring in sp3 bonding networks. Phys. Lett. A 2019, 383, 2809–2812. [Google Scholar] [CrossRef]
- Li, Z.Z.; Wang, J.T.; Mizuseki, H.; Chen, C. Computational discovery of a new rhombohedral diamond phase. Phys. Rev. B 2018, 98, 094107. [Google Scholar] [CrossRef]
- Calderon, H.; Estrada-Guel, I.; Alvarez-Ramírez, F.; Hadjiev, V.; Robles Hernandez, F. Morphed graphene nanostructures: Experimental evidence for existence. Carbon 2016, 102, 288–296. [Google Scholar] [CrossRef]
- Available online: http://sacada.sctms.ru/ (accessed on 12 November 2023).
- Wang, J.T.; Chen, C.; Kawazoe, Y. Phase conversion from graphite toward a simple monoclinic sp3-carbon allotrope. J. Chem. Phys. 2012, 137, 024502. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Z.; Tian, F.; Dong, X.; Li, Q.; Wang, Q.; Wang, H.; Zhong, X.; Xu, B.; Yu, D.; He, J.; et al. Tetragonal Allotrope of Group 14 Elements. J. Am. Chem. Soc. 2012, 134, 12362–12365. [Google Scholar] [CrossRef] [PubMed]
- Mikheev, K.G.; Mogileva, T.N.; Fateev, A.E.; Nunn, N.A.; Shenderova, O.A.; Mikheev, G.M. Low-Power Laser Graphitization of High Pressure—High Temperature Nanodiamond Films. Appl. Sci. 2020, 10, 3329. [Google Scholar] [CrossRef]
- Bagramov, R.; Filonenko, V.; Zibrov, I.; Lyapin, S.; Vlasov, I. Direct Evidence of Catalytic Role of Boron in Graphite-to-Diamond Solid-Phase Conversion under High Pressure. Phys. Status Solidi (RRL)—Rapid Res. Lett. 2020, 14, 2000247. [Google Scholar] [CrossRef]
- Ekimov, E.A.; Kondrina, K.M.; Mordvinova, N.E.; Lebedev, O.I.; Pasternak, D.G.; Vlasov, I.I. High-Pressure, High-Temperature Synthesis of Nanodiamond from Adamantane. Inorg. Mater. 2019, 55, 437–442. [Google Scholar] [CrossRef]
- Yamada, K. Shock synthesis of a new cubic form of carbon. Carbon 2003, 41, 1309–1313. [Google Scholar] [CrossRef]
- Yamada, K.; Tanabe, Y.; Sawaoka, A.B. Allotropes of carbon shock synthesized at pressures up to 15 GPa. Philos. Mag. A 2000, 80, 1811–1828. [Google Scholar] [CrossRef]
- Wang, J.T.; Chen, C.; Kawazoe, Y. Low-Temperature Phase Transformation from Graphite to sp3 Orthorhombic Carbon. Phys. Rev. Lett. 2011, 106, 075501. [Google Scholar] [CrossRef]
- Bewilogua, K.; Hofmann, D. History of diamond-like carbon films—From first experiments to worldwide applications. Surf. Coat. Technol. 2014, 242, 214–225. [Google Scholar] [CrossRef]
- Belenkov, E.A.; Greshnyakov, V.A. Structure, properties, and possible mechanisms of formation of diamond-like phases. Phys. Solid State 2016, 58, 2145–2154. [Google Scholar] [CrossRef]
- Inoi, T.; Baba, K.; Flege, S.; Hatada, R.; Ensinger, W. Preparation of iodine containing diamond-like carbon films by trifluoroiodomethane. Mater. Lett. 2018, 215, 68–70. [Google Scholar] [CrossRef]
- Belenkov, E.A.; Brzhezinskaya, M.M.; Greshnyakov, V.A. Novel carbon diamond-like phases LA5, LA7 and LA8. Diam. Relat. Mater. 2014, 50, 9–14. [Google Scholar] [CrossRef]
- Narojczyk, J.W.; Tretiakov, K.V.; Smardzewski, J.; Wojciechowski, K.W. Hardening of fcc hard-sphere crystals by introducing nanochannels: Auxetic aspects. Phys. Rev. E 2023, 108, 045003. [Google Scholar] [CrossRef] [PubMed]
- Cellini, F.; Lavini, F.; Cao, T.; de Heer, W.; Berger, C.; Bongiorno, A.; Riedo, E. Epitaxial two-layer graphene under pressure: Diamene stiffer than Diamond. FlatChem 2018, 10, 8–13. [Google Scholar] [CrossRef]
- Kano, M. Diamond-Like Carbon Coating Applied to Automotive Engine Components. Tribol. Online 2014, 9, 135–142. [Google Scholar] [CrossRef]
- Wan, S.; Li, D.; Zhang, G.; Tieu, A.K.; Zhang, B. Comparison of the scuffing behaviour and wear resistance of candidate engineered coatings for automotive piston rings. Tribol. Int. 2017, 106, 10–22. [Google Scholar] [CrossRef]
- Wei, P.; He, M.; Ao, W. Molecular dynamics study of the frictional behaviors of diamond-like carbon films. Appl. Phys. A 2021, 127, 652. [Google Scholar] [CrossRef]
- Belenkov, E.A.; Greshnyakov, V.A. Diamond-like phases formed from fullerene-like clusters. Phys. Solid State 2015, 57, 2331–2341. [Google Scholar] [CrossRef]
- Gao, Y.; Cao, T.; Cellini, F.; Berger, C.; de Heer, W.A.; Tosatti, E.; Riedo, E.; Bongiorno, A. Ultrahard carbon film from epitaxial two-layer graphene. Nat. Nanotechnol. 2017, 13, 133–138. [Google Scholar] [CrossRef] [PubMed]
- Greshnyakov, V.; Belenkov, E. Theoretical study of the stability and formation methods of layer diamond-like nanostructures. Lett. Mater. 2020, 10, 457–462. [Google Scholar] [CrossRef]
- Greshnyakov, V.; Belenkov, E. Structure and properties of a chiral polymorph of diamond with a crystal lattice of the SA3 type. Lett. Mater. 2021, 11, 479–484. [Google Scholar] [CrossRef]
- Geng, P.; Branicio, P.S. Atomistic insights on the pressure-induced multi-layer graphene to diamond-like structure transformation. Carbon 2021, 175, 243–253. [Google Scholar] [CrossRef]
- Greshnyakov, V.A. AB INITIO STUDY OF L4-, L3-6-, AND L3-4-6-DIAMOND-LIKE TUBULAR NANOSTRUCTURES. J. Struct. Chem. 2023, 64, 324–334. [Google Scholar] [CrossRef]
- Milyavskiy, V.; Utkin, A.; Zhuk, A.; Yakushev, V.; Fortov, V. Shock compressibility and shock-induced phase transitions of C60 fullerite. Diam. Relat. Mater. 2005, 14, 1920–1923. [Google Scholar] [CrossRef]
- Khishchenko, K.; Milyavskiy, V.; Utkin, A.; Yakushev, V.; Zhuk, A.; Fortov, V. Equation of state and physical–chemical transformations of C60 fullerite at high pressures and temperatures. Diam. Relat. Mater. 2007, 16, 1204–1207. [Google Scholar] [CrossRef]
- Mahnama, M.; Naghdabadi, R. Molecular dynamics simulation of orientation dependency in the shock-induced phase transition of C60 fullerene single crystals into amorphous diamond. Phase Transit. 2013, 87, 271–285. [Google Scholar] [CrossRef]
- Grima, J.N.; Grech, M.C.; Grima-Cornish, J.N.; Gatt, R.; Attard, D. Giant Auxetic Behaviour in Engineered Graphene. Ann. Phys. 2018, 530, 1700330. [Google Scholar] [CrossRef]
- Evans, K.E. Auxetic polymers: A new range of materials. Endeavour 1991, 15, 170–174. [Google Scholar] [CrossRef]
- Almgren, R. An isotropic three-dimensional structure with Poissons ratio = −1. J. Elast. 1985, 15, 427–430. [Google Scholar] [CrossRef]
- Lakes, R. Foam Structures with a Negative Poisson’s Ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef] [PubMed]
- Wojciechowski, K. Constant thermodynamic tension Monte Carlo studies of elastic properties of a two-dimensional system of hard cyclic hexamers. Mol. Phys. 1987, 61, 1247–1258. [Google Scholar] [CrossRef]
- Wojciechowski, K.W.; Brańka, A.C. Negative Poisson ratio in a two-dimensional “isotropic” solid. Phys. Rev. A 1989, 40, 7222–7225. [Google Scholar] [CrossRef] [PubMed]
- Brańka, A.C.; Heyes, D.M.; Wojciechowski, K.W. Auxeticity of cubic materials under pressure. Phys. Status Solidi (b) 2010, 248, 96–104. [Google Scholar] [CrossRef]
- Milton, G.W. Composite-Materials with Poisson Ratios Close to −1. J. Mech. Phys. Solids 1992, 40, 1105–1137. [Google Scholar] [CrossRef]
- Baughman, R.H.; Shacklette, J.M.; Zakhidov, A.A.; Stafström, S. Negative Poisson’s ratios as a common feature of cubic metals. Nature 1998, 392, 362–365. [Google Scholar] [CrossRef]
- Grima, J.N.; Evans, K.E. Auxetic behavior from rotating squares. J. Mater. Sci. Lett. 2000, 19, 1563–1565. [Google Scholar] [CrossRef]
- Kimizuka, H.; Kaburaki, H.; Kogure, Y. Mechanism for negative Poisson ratios over the alpha-beta transition of cristobalite, SiO2: A molecular-dynamics study. Phys. Rev. Lett. 2000, 84, 5548–5551. [Google Scholar] [CrossRef]
- Grima, J.N.; Winczewski, S.; Mizzi, L.; Grech, M.C.; Cauchi, R.; Gatt, R.; Attard, D.; Wojciechowski, K.W.; Rybicki, J. Tailoring Graphene to Achieve Negative Poisson’s Ratio Properties. Adv. Mater. 2014, 27, 1455–1459. [Google Scholar] [CrossRef]
- Lakes, R.S. Negative-Poisson’s-Ratio Materials: Auxetic Solids. Annu. Rev. Mater. Res. 2017, 47, 63–81. [Google Scholar] [CrossRef]
- Sun, W.; Xue, S.; Jiang, J. Molecular dynamics study on the thermal conductivity and ballistic resistance of twisted graphene. Comput. Mater. Sci. 2023, 229, 112436. [Google Scholar] [CrossRef]
- Kim, J.C.; Wi, J.H.; Ri, N.C.; Ri, S.I. Thermal conductivity of graphene/graphane/graphene heterostructure nanoribbons: Non-equilibrium molecular dynamics simulations. Solid State Commun. 2021, 328, 114249. [Google Scholar] [CrossRef]
- Liu, X.; Yu, P.; Lu, C. Mechanical property and thermal conductivity of pentadiamond: A comprehensive molecular dynamics study. Comput. Mater. Sci. 2022, 203, 111039. [Google Scholar] [CrossRef]
- Yu, Y.; Zhang, X.; Bai, L. Nanoindentation and scratching behaviors of diamond-like carbon films by coarse-grained molecular dynamics. Diam. Relat. Mater. 2023, 140, 110516. [Google Scholar] [CrossRef]
- Farzin, A.; Etemadi, M.; Mehran, S.; Rouhi, S. Investigating the mechanical properties of perfect and defective psi-graphene: A molecular dynamics simulation. Mater. Today Commun. 2023, 37, 106908. [Google Scholar] [CrossRef]
- Tersoff, J. Empirical Interatomic Potential for Carbon, with Applications to Amorphous Carbon. Phys. Rev. Lett. 1988, 61, 2879–2882. [Google Scholar] [CrossRef]
- Brenner, D.W. Empirical potential for hydrocarbons for use in simulating the chemical vapor deposition of diamond films. Phys. Rev. B 1990, 42, 9458–9471. [Google Scholar] [CrossRef]
- Stuart, S.J.; Tutein, A.B.; Harrison, J.A. A reactive potential for hydrocarbons with intermolecular interactions. J. Chem. Phys. 2000, 112, 6472–6486. [Google Scholar] [CrossRef]
- van Duin, A.C.T.; Dasgupta, S.; Lorant, F.; Goddard, W.A. ReaxFF: A reactive force field for Hydrocarbons. J. Phys. Chem. A 2001, 105, 9396–9409. [Google Scholar] [CrossRef]
- Los, J.H.; Fasolino, A. Intrinsic long-range bond-order potential for carbon: Performance in Monte Carlo simulations of graphitization. Phys. Rev. B 2003, 68, 024107. [Google Scholar] [CrossRef]
- Savin, A.V.; Kivshar, Y.S.; Hu, B. Suppression of thermal conductivity in graphene nanoribbons with rough edges. Phys. Rev. B 2010, 82, 195422. [Google Scholar] [CrossRef]
- Chung, Y.J.; Lee, G.H.; Beom, H.G. Atomistic Insights into the Phase Transformation of Single-Crystal Silicon during Nanoindentation. Nanomaterials 2022, 12, 2071. [Google Scholar] [CrossRef] [PubMed]
- Marks, N.A.; Cover, M.F.; Kocer, C. The importance of rare events in thin film deposition: A molecular dynamics study of tetrahedral amorphous carbon. Mol. Simul. 2006, 32, 1271–1277. [Google Scholar] [CrossRef]
- Xu, J.; Geng, Y.; Chu, Z.; Hu, Q.; Lei, Y.; Wang, Y. Systematically Study the Tensile and Compressive Behaviors of Diamond-like Carbon. Nanomaterials 2023, 13, 1772. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Dong, B.; Shi, T.; Zhan, H.; Zhang, Y. Atomistic Simulations of the Permeability and Dynamic Transportation Characteristics of Diamond Nanochannels. Nanomaterials 2022, 12, 1785. [Google Scholar] [CrossRef] [PubMed]
- Available online: https://www.lammps.org/ (accessed on 15 December 2023).
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; in’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comput. Phys. Commun. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Orekhov, N.D.; Stegailov, V.V. Molecular dynamics simulation of graphite melting. High Temp. 2014, 52, 198–204. [Google Scholar] [CrossRef]
- Baimova, J.; Rysaeva, L.; Rudskoy, A. Deformation behavior of diamond-like phases: Molecular dynamics simulation. Diam. Relat. Mater. 2018, 81, 154–160. [Google Scholar] [CrossRef]
- Rysaeva, L.K.; Baimova, J.A.; Dmitriev, S.V.; Lisovenko, D.S.; Gorodtsov, V.A.; Rudskoy, A.I. Elastic properties of diamond-like phases based on carbon nanotubes. Diam. Relat. Mater. 2019, 97, 107411. [Google Scholar] [CrossRef]
- Orekhov, N.; Ostroumova, G.; Stegailov, V. High temperature pure carbon nanoparticle formation: Validation of AIREBO and ReaxFF reactive molecular dynamics. Carbon 2020, 170, 606–620. [Google Scholar] [CrossRef]
- Srinivasan, S.G.; van Duin, A.C.T.; Ganesh, P. Development of a ReaxFF Potential for Carbon Condensed Phases and Its Application to the Thermal Fragmentation of a Large Fullerene. J. Phys. Chem. A 2015, 119, 571–580. [Google Scholar] [CrossRef] [PubMed]
- Tersoff, J. Modeling solid-state chemistry: Interatomic potentials for multicomponent systems. Phys. Rev. B 1989, 39, 5566–5568. [Google Scholar] [CrossRef] [PubMed]
- Galiullina, G.M.; Orekhov, N.D.; Stegailov, V.V. Nucleation of carbon nanostructures: Molecular dynamics with reactive potentials. J. Phys. Conf. Ser. 2016, 774, 012033. [Google Scholar] [CrossRef]
- Shenderova, O.A.; Brenner, D.W.; Omeltchenko, A.; Su, X.; Yang, L.H. Atomistic modeling of the fracture of polycrystalline diamond. Phys. Rev. B 2000, 61, 3877–3888. [Google Scholar] [CrossRef]
- Mattoni, A.; Ippolito, M.; Colombo, L. Atomistic modeling of brittleness in covalent materials. Phys. Rev. B 2007, 76, 224103. [Google Scholar] [CrossRef]
- Orekhov, N.D.; Stegailov, V.V. Molecular-dynamics based insights into the problem of graphite melting. J. Phys. Conf. Ser. 2015, 653, 012090. [Google Scholar] [CrossRef]
- Pastewka, L.; Pou, P.; Pérez, R.; Gumbsch, P.; Moseler, M. Describing bond-breaking processes by reactive potentials: Importance of an environment-dependent interaction range. Phys. Rev. B 2008, 78, 161402. [Google Scholar] [CrossRef]
- Latham, C.D.; McKenna, A.J.; Trevethan, T.P.; Heggie, M.I.; Rayson, M.J.; Briddon, P.R. On the validity of empirical potentials for simulating radiation damage in graphite: A benchmark. J. Phys. Condens. Matter 2015, 27, 316301. [Google Scholar] [CrossRef]
- Kuzkin, V.A.; Krivtsov, A.M. Description for mechanical properties of graphene using particles with rotational degrees of freedom. Dokl. Phys. 2011, 56, 527–530. [Google Scholar] [CrossRef]
- Zhu, J.; Han, J.; Liu, A.; Meng, S.; Jiang, C. Mechanical properties and Raman characterization of amorphous diamond films as a function of film thickness. Surf. Coat. Technol. 2007, 201, 6667–6669. [Google Scholar] [CrossRef]
- Hei, L.F.; Lu, F.X.; Li, C.M.; Tang, W.Z.; Chen, G.C.; Song, J.H. A Review on Mechanical Properties of Freestanding Diamond Films. Adv. Mater. Res. 2012, 490–495, 3059–3064. [Google Scholar] [CrossRef]
- Xiong, J.; Liu, L.; Song, H.; Wang, M.; Hu, T.; Zhai, Z.; Yang, B.; Jiang, X.; Huang, N. Mechanical properties evaluation of diamond films via nanoindentation. Diam. Relat. Mater. 2022, 130, 109403. [Google Scholar] [CrossRef]
- Rysaeva, L.K.; Baimova, J.A.; Lisovenko, D.S.; Gorodtsov, V.A.; Dmitriev, S.V. Elastic Properties of Fullerites and Diamond-Like Phases. Phys. Status Solidi (b) 2018, 256, 1800049. [Google Scholar] [CrossRef]
- Lisovenko, D.S.; Baimova, Y.A.; Rysaeva, L.K.; Gorodtsov, V.A.; Dmitriev, S.V. Equilibrium structures of carbon diamond-like clusters and their elastic properties. Phys. Solid State 2017, 59, 820–828. [Google Scholar] [CrossRef]
- Born, M. On the stability of crystal lattices. I. Math. Proc. Camb. Philos. Soc. 1940, 36, 160–172. [Google Scholar] [CrossRef]
- Pastorelli, R.; Ferrari, A.; Beghi, M.; Bottani, C.; Robertson, J. Elastic constants of ultrathin diamond-like carbon films. Diam. Relat. Mater. 2000, 9, 825–830. [Google Scholar] [CrossRef]
- Klein, C.A.; Cardinale, G.F. Young’s modulus and Poisson’s ratio of CVD diamond. Diam. Relat. Mater. 1993, 2, 918–923. [Google Scholar] [CrossRef]
- Savvides, N.; Bell, T. Hardness and elastic modulus of diamond and diamond-like carbon films. Thin Solid Film. 1993, 228, 289–292. [Google Scholar] [CrossRef]
- Savvides, N.; Bell, T.J. Microhardness and Young’s modulus of diamond and diamondlike carbon films. J. Appl. Phys. 1992, 72, 2791–2796. [Google Scholar] [CrossRef]
- Goldstein, R.; Gorodtsov, V.; Lisovenko, D. Mesomechanics of multiwall carbon nanotubes and nanowhiskers. Phys. Mesomech. 2009, 12, 38–53. [Google Scholar] [CrossRef]
- Pigłowski, P.M.; Wojciechowski, K.W.; Tretiakov, K.V. Partial auxeticity induced by nanoslits in the Yukawa crystal. Phys. Status Solidi (RRL)—Rapid Res. Lett. 2016, 10, 566–569. [Google Scholar] [CrossRef]
- Narojczyk, J.W.; Wojciechowski, K.W.; Tretiakov, K.V.; Smardzewski, J.; Scarpa, F.; Piglowski, P.M.; Kowalik, M.; Imre, A.R.; Bilski, M. Auxetic Properties of a f.c.c. Crystal of Hard Spheres with an Array of [001]-Nanochannels Filled by Hard Spheres of Another Diameter. Phys. Status Solidi (b) 2018, 256, 1800611. [Google Scholar] [CrossRef]
- Narojczyk, J.W.; Bilski, M.; Grima, J.N.; Kędziora, P.; Morozow, D.; Rucki, M.; Wojciechowski, K.W. Removing Auxetic Properties in f.c.c. Hard Sphere Crystals by Orthogonal Nanochannels with Hard Spheres of Another Diameter. Materials 2022, 15, 1134. [Google Scholar] [CrossRef]
- Tretiakov, K.V.; Wojciechowski, K.W. Auxetic, Partially Auxetic, and Nonauxetic Behaviour in 2D Crystals of Hard Cyclic Tetramers. Phys. Status Solidi (RRL)—Rapid Res. Lett. 2020, 14, 2000198. [Google Scholar] [CrossRef]
- Pigłowski, P.M.; Narojczyk, J.W.; Wojciechowski, K.W.; Tretiakov, K.V. Auxeticity enhancement due to size polydispersity in fcc crystals of hard-core repulsive Yukawa particles. Soft Matter 2017, 13, 7916–7921. [Google Scholar] [CrossRef]
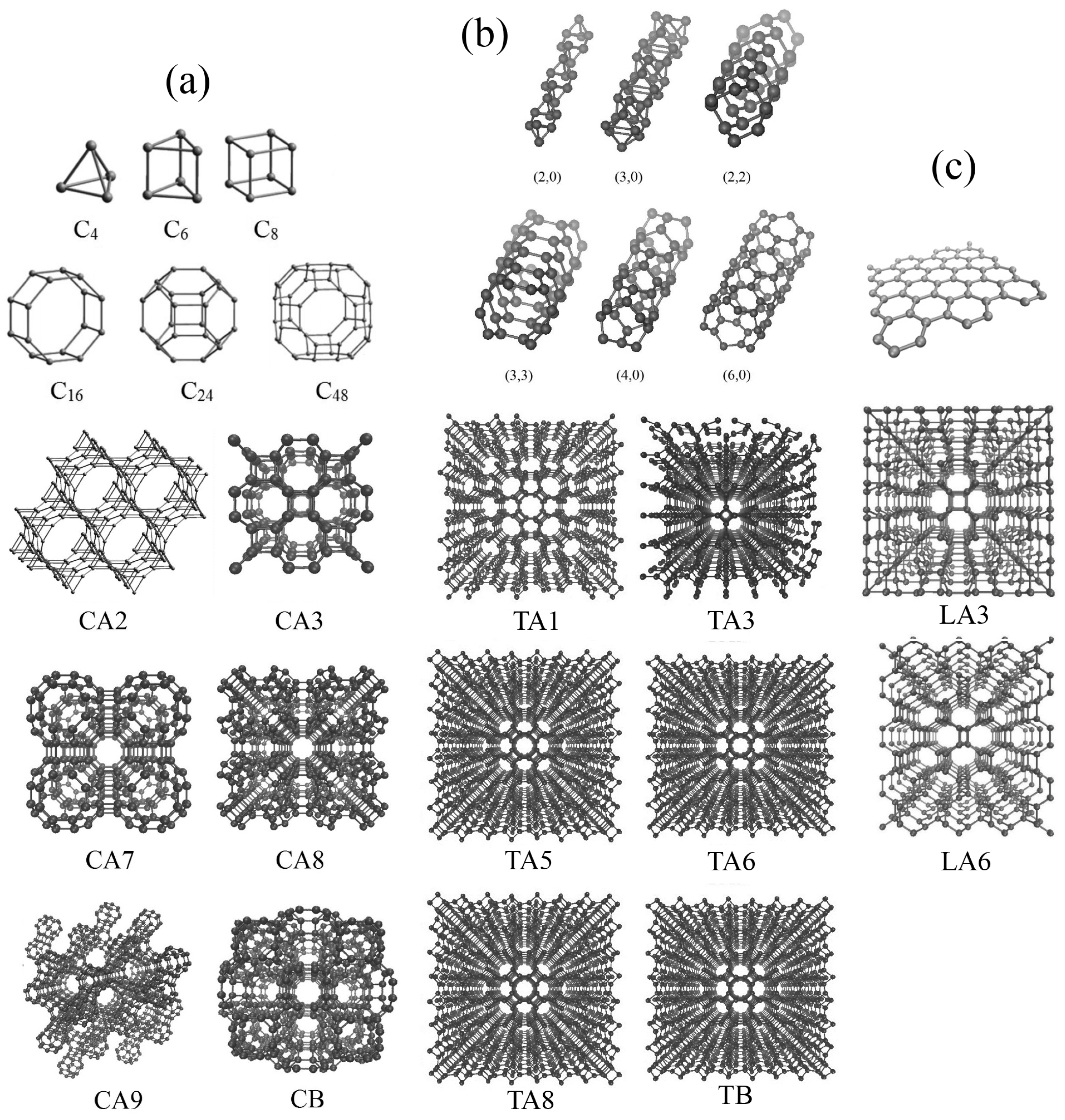
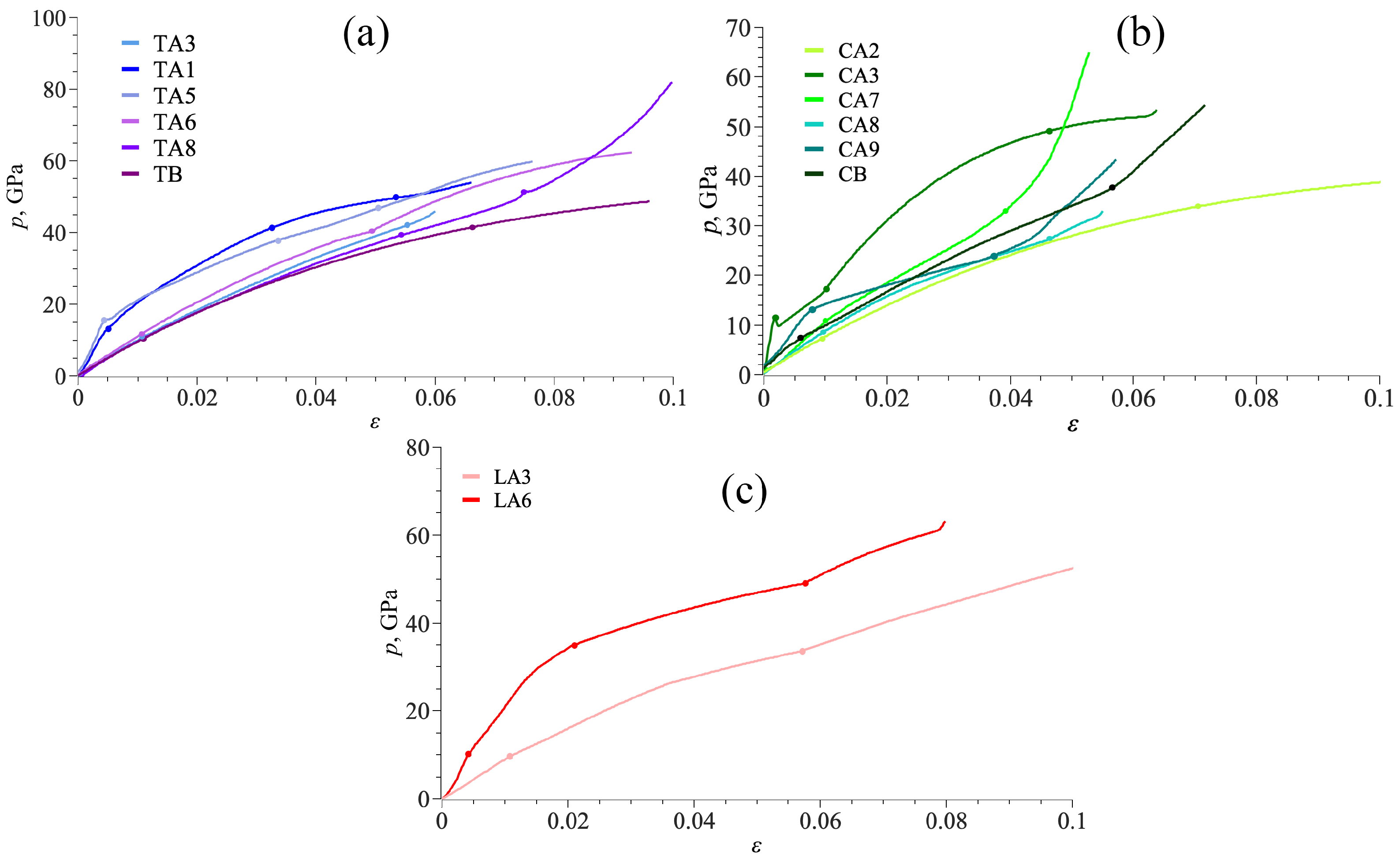
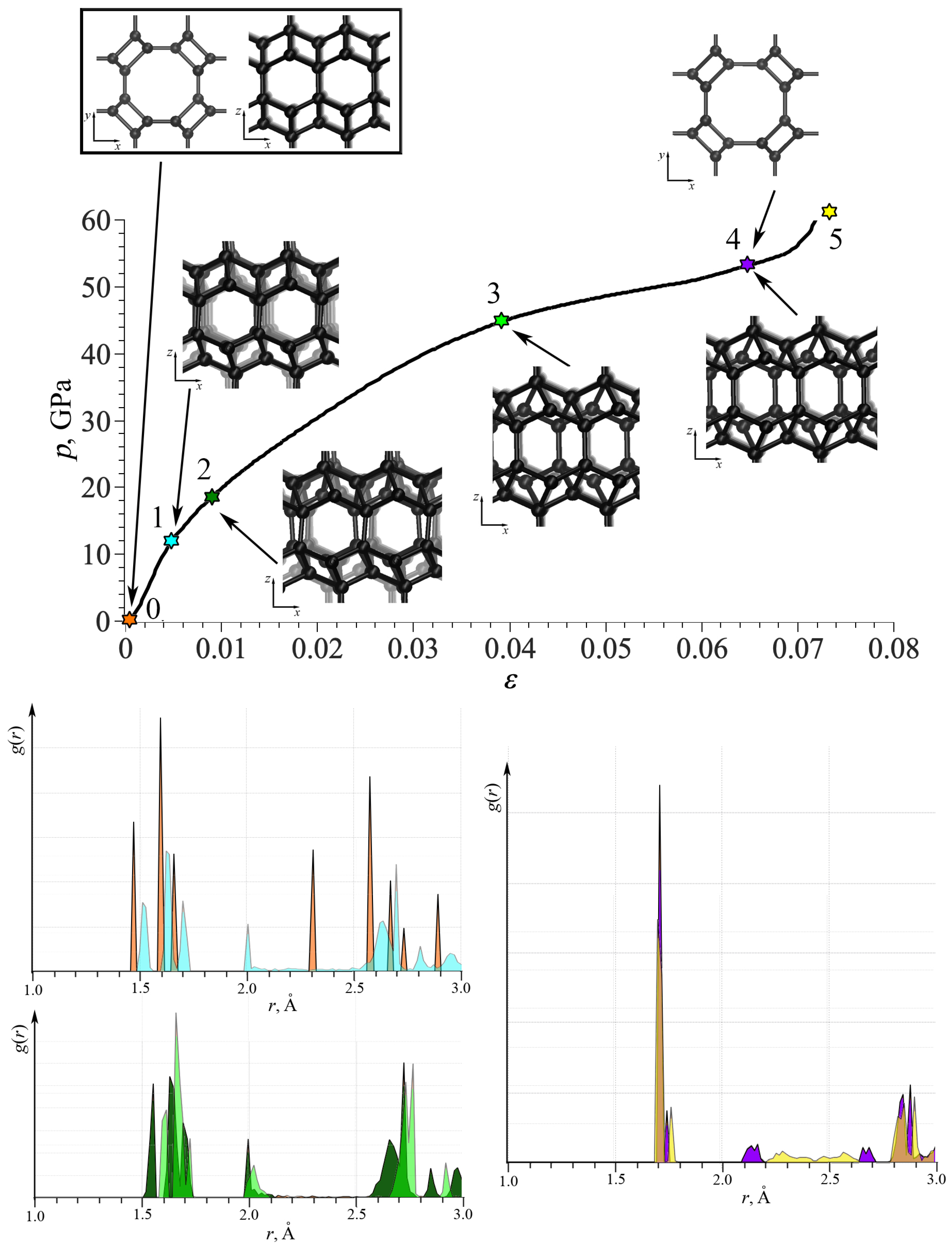
| Structures | Size, Å | , Å | , Å | , Å | , Å | a, Å | c, Å | , g/cm3 |
|---|---|---|---|---|---|---|---|---|
| TA1 | 25 × 25 × 22 | 1.4921 | 1.5638 | 1.5638 | 1.5723 | 6.461 | 2.577 | 2.966 |
| TA3 | 25 × 25 × 22 | 1.5857 | 1.5015 | 1.5625 | 1.5625 | 3.558 | 4.314 | 2.921 |
| TA5 | 30 × 25 × 20 | 1.4846 | 1.5539 | 1.5612 | 1.5832 | 6.917 | 4.406 | 3.027 |
| TA6 | 26 × 28 × 30 | 1.4869 | 1.5410 | 1.5928 | 1.5928 | 7.007 | 4.165 | 3.122 |
| TA8 | 20 × 20 × 22 | 1.4830 | 1.5840 | 1.5587 | 1.6879 | 10.582 | 2.511 | 2.949 |
| TB | 17 × 17 × 19 | 1.4984 | 1.4984 | 1.5152 | 1.5152 | 4.421 | 2.530 | 2.794 |
| Structure | , Å | , Å | , Å | , Å | , Å | a, Å | , g/cm3 |
|---|---|---|---|---|---|---|---|
| CA2 | 17 × 17 × 17 | 1.4411 | 1.5431 | 1.5437 | 1.5726 | 5.037 | 2.203 |
| CA3 | 20 × 20 × 20 | 1.4464 | 1.5702 | 1.5702 | 1.5702 | 4.811 | 2.867 |
| CA7 | 15 × 15 × 15 | 1.5014 | 1.5014 | 1.5665 | 1.5665 | 7.380 | 2.382 |
| CA8 | 20 × 20 × 20 | 1.5047 | 1.5998 | 1.4966 | 1.5833 | 8.967 | 2.656 |
| CA9 | 21 × 32 × 25 | 1.5389 | 1.4830 | 1.6139 | 1.5107 | 12.244 | 2.086 |
| CB | 20 × 20 × 20 | 1.4990 | 1.5404 | 1.4919 | 1.4919 | 9.396 | 2.308 |
| Structure | , Å | , Å | , Å | , Å | , Å | a, Å | c, Å | , g/cm3 |
|---|---|---|---|---|---|---|---|---|
| LA3 | 16 × 16 × 20 | 1.5225 | 1.5225 | 1.5672 | 1.5672 | 4.348 | 2.516 | 3.356 |
| LA6 | 15 × 20 × 20 | 1.4837 | 1.5441 | 1.5441 | 1.5957 | 4.821 | 2.578 | 3.071 |
| DLP | s11 | s12 | s13 | s14 | s22 | s23 | s33 | s44 | s55 | s66 |
|---|---|---|---|---|---|---|---|---|---|---|
| Cubic | ||||||||||
| CA3 | 1.87 ± | −0.44 ± | 2.496 ± | |||||||
| CA7 | 8.12 ± | −3.82 ± | 3.64 ± | |||||||
| CA8 | 1.67 ± | −0.299 ± | 5.91 ± | |||||||
| CA9 | 3.73 ± | −0.9 ± | 7.31 ± | |||||||
| CB | 5.53 ± | −2 ± | 10 ± | |||||||
| Tetragonal | ||||||||||
| TA1 | 2.15 ± | −0.18 ± | −0.52 ± | 2.3 ± | 7.9 ± | 5.51 ± | ||||
| TA3 | 1.9 ± | −0.7 ± | −0.52 ± | 2.58 ± | 7.76 ± | 4.96 ± | ||||
| TA5 | 1.55 ± | −0.5 ± | −0.3 ± | 1.14 ± | 5.6 ± | 2.16 ± | ||||
| TA6 | 1.47 ± | −0.01 ± | −0.13 ± | 0.82 ± | 4.73 ± | 2.77 ± | ||||
| LA3 | 2 ± | −2 ± | −0.04 ± | 8.15 ± | 11.5 ± | 3.44 ± | ||||
| Trigonal | ||||||||||
| TA8 | 1.98 ± | −0.9 ± | −0.06 ± | −0.005 ± | 0.96 ± | 3.88 ± | ||||
| Hexagonal | ||||||||||
| CA2 | 2.51 ± | 0.09 ± | −0.36 ± | 1.92 ± | 18.4 ± | |||||
| TB | 1.65 ± | −0.16 ± | −0.29 ± | 0.99 ± | 10.17 ± | |||||
| Orthorhombic | ||||||||||
| LA6 | 2.45 ± | −1.4 ± | −0.3 ± | 2.2 ± | −0.13 ± | 1.2 ± | 2.1 ± | 2.3 ± | 2.5 ± |
| DLP | c11 | c12 | c13 | c14 | c22 | c23 | c33 | c44 | c55 | c66 |
|---|---|---|---|---|---|---|---|---|---|---|
| Cubic | ||||||||||
| Diam. | 1076 ± 120 | 125 ± 9 | - | - | - | - | - | 563 ± 4 | - | |
| CA3 | 625 ± 10 | 192 ± 12 | - | - | - | - | - | 401 ± 9 | - | |
| CA7 | 750 ± 18 | 667 ± 8 | - | - | - | - | - | 275 ± 4 | - | |
| CA8 | 650 ± 22 | 142 ± 4 | - | - | - | - | - | 169 ± 4 | - | |
| CA9 | 316 ± 11 | 101 ± 8 | - | - | - | - | - | 137 ± 5 | - | |
| CB | 306 ± 10 | 174 ± 4 | - | - | - | - | - | 100 ± 3 | - | |
| Tetragonal | ||||||||||
| TA1 | 652 ± 21 | 31 ± 8 | 196 ± 4 | - | - | - | 461 ± 8 | 130 ± 4 | - | 182 ± 4 |
| TA3 | 706 ± 21 | 294 ± 8 | 165 ± 11 | - | - | - | 463 ± 9 | 129 ± 5 | - | 201 ± 4 |
| TA5 | 820 ± 35 | 350 ± 9 | 226 ± 9 | - | - | - | 989 ± 14 | 179 ± 1 | - | 463 ± 3 |
| TA6 | 1854 ± 128 | 55 ± 2 | −8.59 ± | - | - | - | 1214 ± 24 | 442 ± 12 | - | 221 ± 9 |
| LA3 | 626 ± 20 | 79 ± 2 | 40 ± 1 | - | - | - | 1232 ± 44 | 87 ± | - | 290 ± 6 |
| Trigonal | ||||||||||
| TA8 | 657 ± 12 | 316 ± 4 | 65 ± | 0.5 ± | - | - | 1051 ± 54 | 257 ± 18 | - | - |
| Hexagonal | ||||||||||
| CA2 | 413 ± 18 | −1.6 ± | 87 ± 1 | - | - | - | 555 ± 14 | 161 ± 3 | - | - |
| TB | 600 ± 18 | −30.5 ± | 155 ± 5 | - | - | - | 1067 ± 38 | 3614 ± 141 | - | - |
| Orthorhombic | ||||||||||
| LA6 | 720 ± 33 | 473 ± 1 | 271 ± 4 | - | 761 ± 34 | 229 ± 9 | 933 ± 94 | 454 ± 14 | 423 ± 22 | 395 ± 23 |
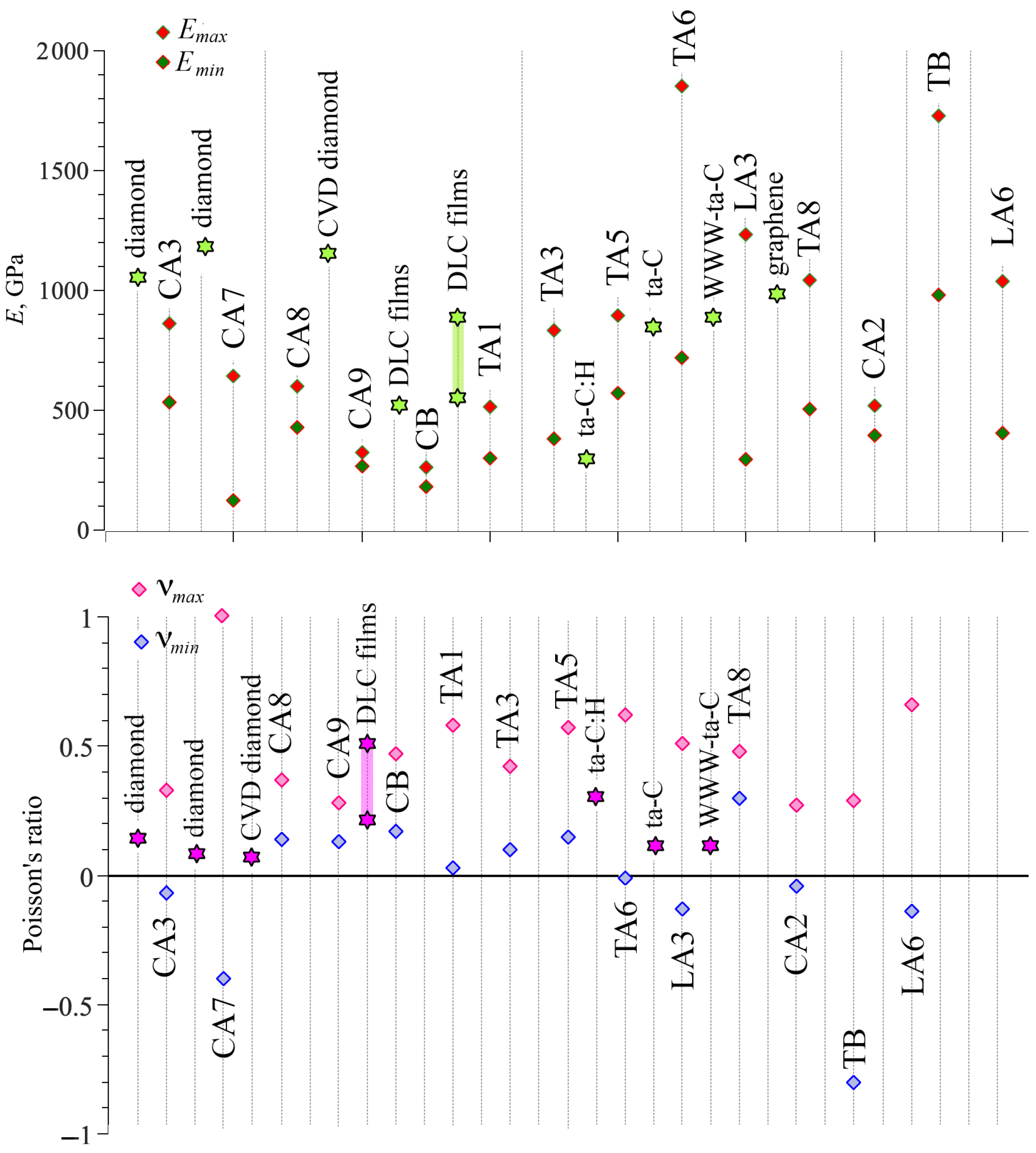
| DLP | |||||
|---|---|---|---|---|---|
| Cubic | |||||
| CA3 | 860 ± 23 | 535 ± 21 | 1.6 ± | 0.33 ± | –0.07 ± |
| CA7 | 644 ± 13 | 123 ± 8 | 5.2 ± | 1.14 ± | –0.4 ± |
| CA8 | 599 ± 19 | 430 ± 13 | 1.4 ± | 0.37 ± | 0.14 ± |
| CA9 | 325 ± 7 | 268 ± 7 | 1.2 ± | 0.28 ± | 0.13 ± |
| CB | 260 ± 3 | 181 ± 3 | 1.4 ± | 0.47 ± | 0.17 ± |
| Tetragonal | |||||
| TA1 | 513 ± 11 | 299 ± 5 | 1.7 ± | 0.58 ± | 0.03 ± |
| TA3 | 834 ± 33 | 380 ± 6 | 2.2 ± | 0.42 ± | 0.1 ± |
| TA5 | 893 ± 19 | 573 ± 173 | 1.5 ± | 0.57 ± | 0.15 ± |
| TA6 | 1852 ± 128 | 717 ± 19 | 2.6 ± | 0.62 ± | –0.01 ± |
| LA3 | 1235 ± 99 | 293 ± 8 | 4.2 ± | 0.51 ± | –0.13 ± |
| Trigonal | |||||
| TA8 | 1043 ± 87 | 504 ± 4 | 2.0 ± | 0.48 ± | 0.3 ± |
| Hexagonal | |||||
| CA2 | 518 ± 18 | 393 ± 13 | 1.3 ± | 0.27 ± | –0.04 ± |
| TB | 1730 ± 77 | 983 ± 4 | 1.6 ± | 0.29 ± | –0.8 ± |
| Orthorhombic | |||||
| LA6 | 1039 ± 77 | 407 ± 24 | 2.5 ± | 0.66 ± | –0.14 ± |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baimova, J.A. An Overview of Mechanical Properties of Diamond-like Phases under Tension. Nanomaterials 2024, 14, 129. https://doi.org/10.3390/nano14020129
Baimova JA. An Overview of Mechanical Properties of Diamond-like Phases under Tension. Nanomaterials. 2024; 14(2):129. https://doi.org/10.3390/nano14020129
Chicago/Turabian StyleBaimova, Julia A. 2024. "An Overview of Mechanical Properties of Diamond-like Phases under Tension" Nanomaterials 14, no. 2: 129. https://doi.org/10.3390/nano14020129
APA StyleBaimova, J. A. (2024). An Overview of Mechanical Properties of Diamond-like Phases under Tension. Nanomaterials, 14(2), 129. https://doi.org/10.3390/nano14020129






