Phase-Field Simulation of Spinodal Decomposition in U-50Zr Metallic Nuclear Fuel
Abstract
1. Introduction
2. Phase-Field Method
2.1. Free Energy Function
2.2. Kinetic Equations
2.3. Equation Solving
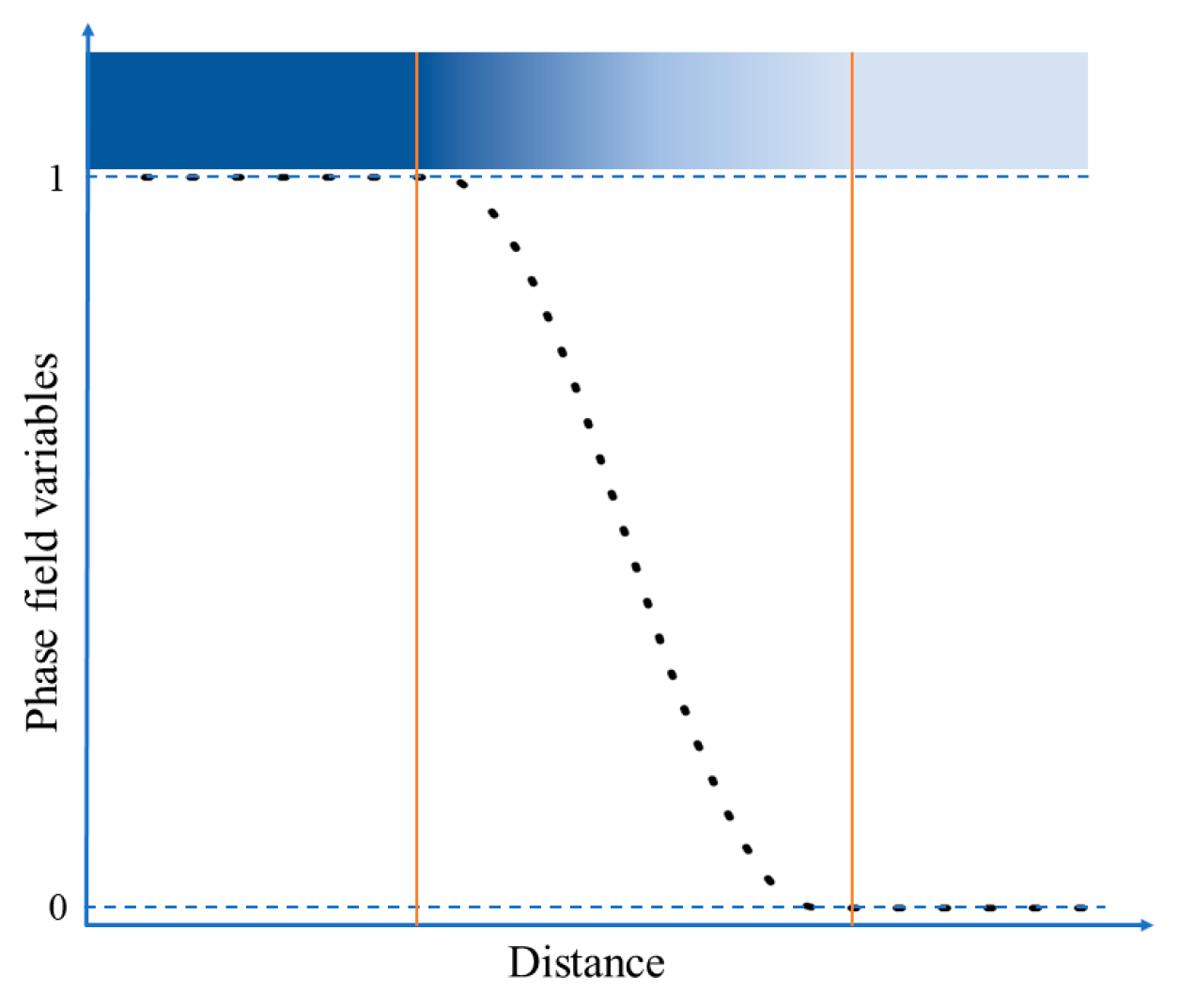
2.4. Reduced Dimensionless Variables
3. Results
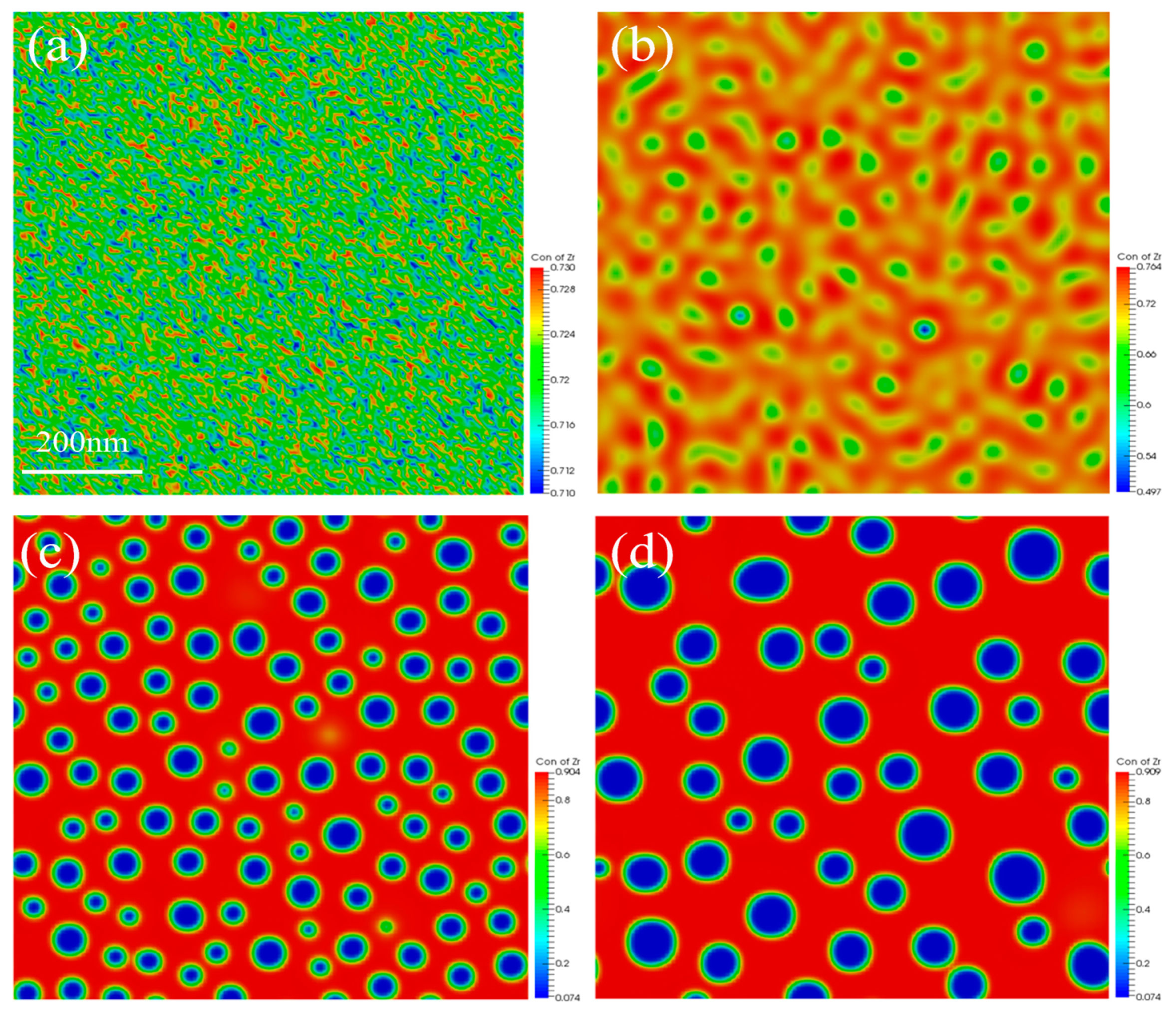
3.1. Spinodal Decomposition within Grain of U-50Zr
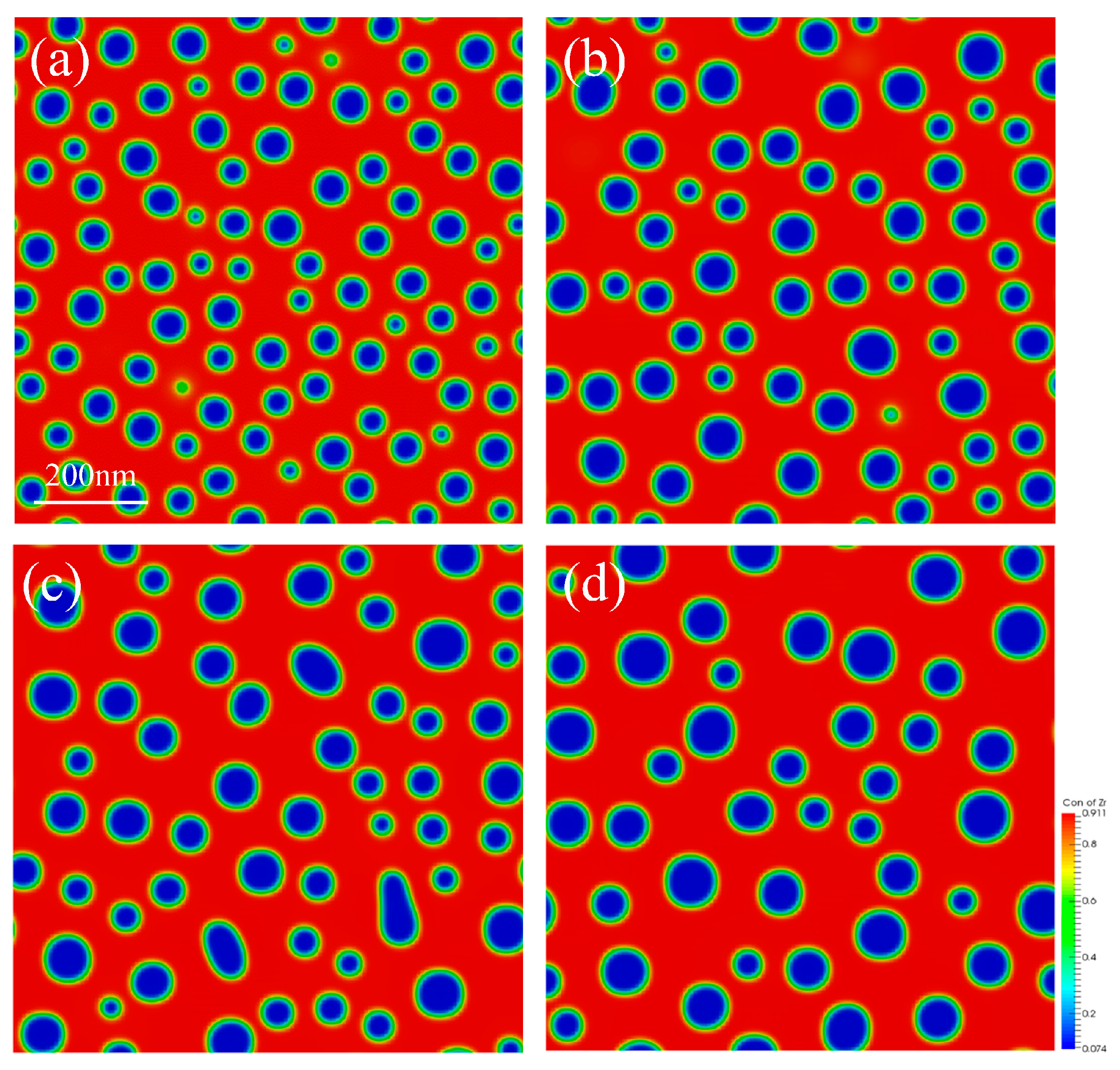
3.2. Effect of Temperature on Spinodal Decomposition
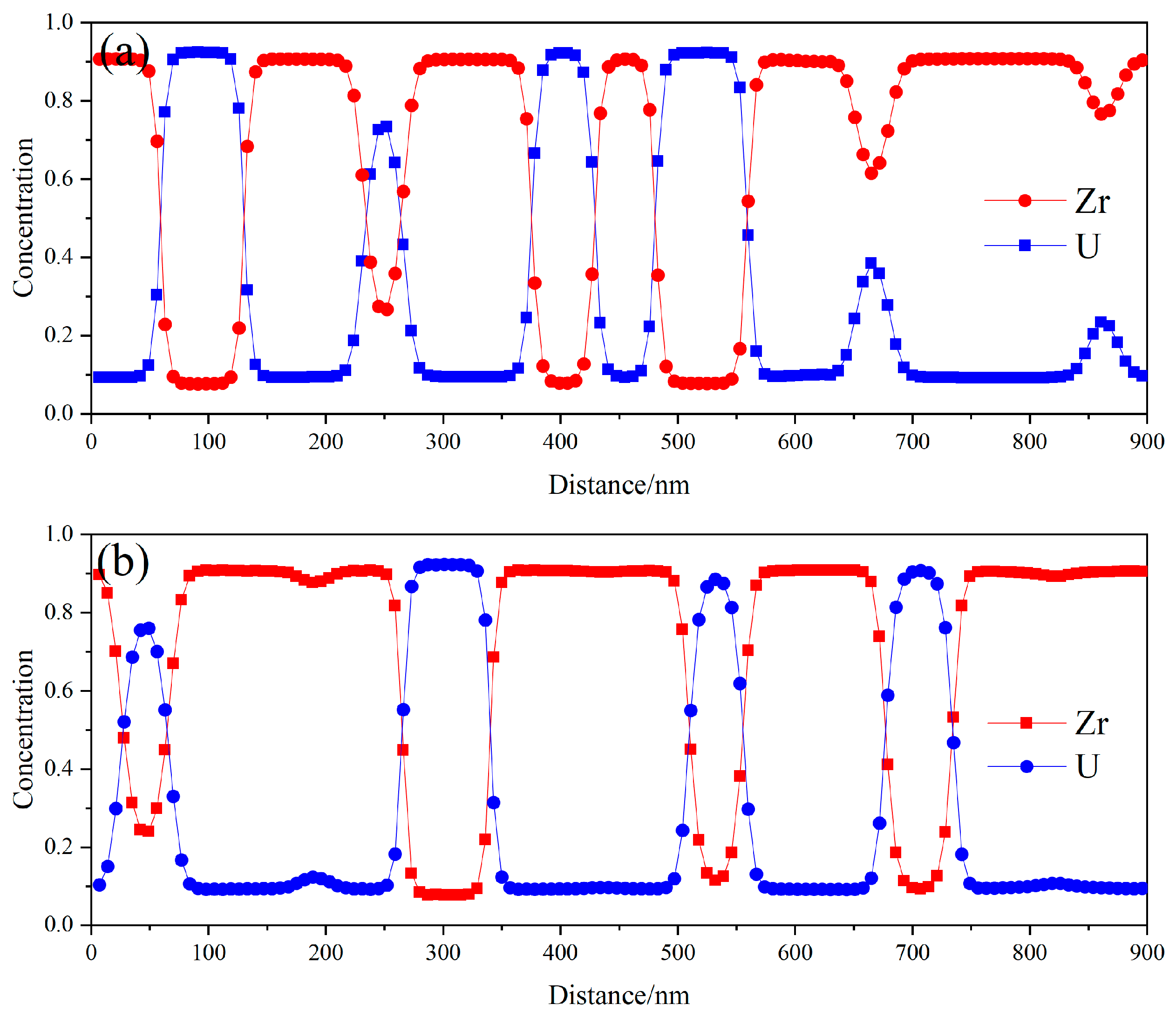
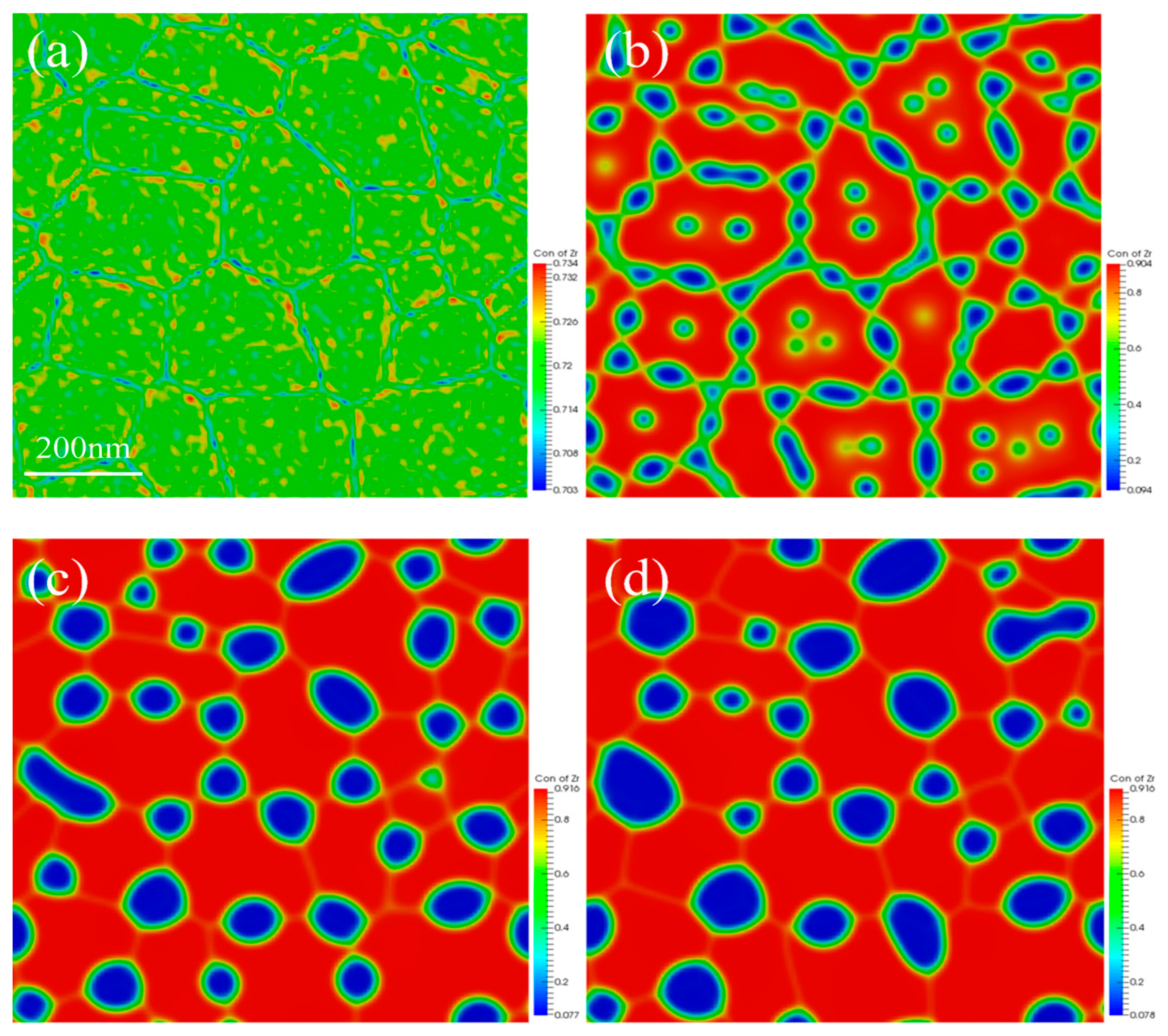
3.3. Effect of Grain Boundaries on Spinodal Decomposition
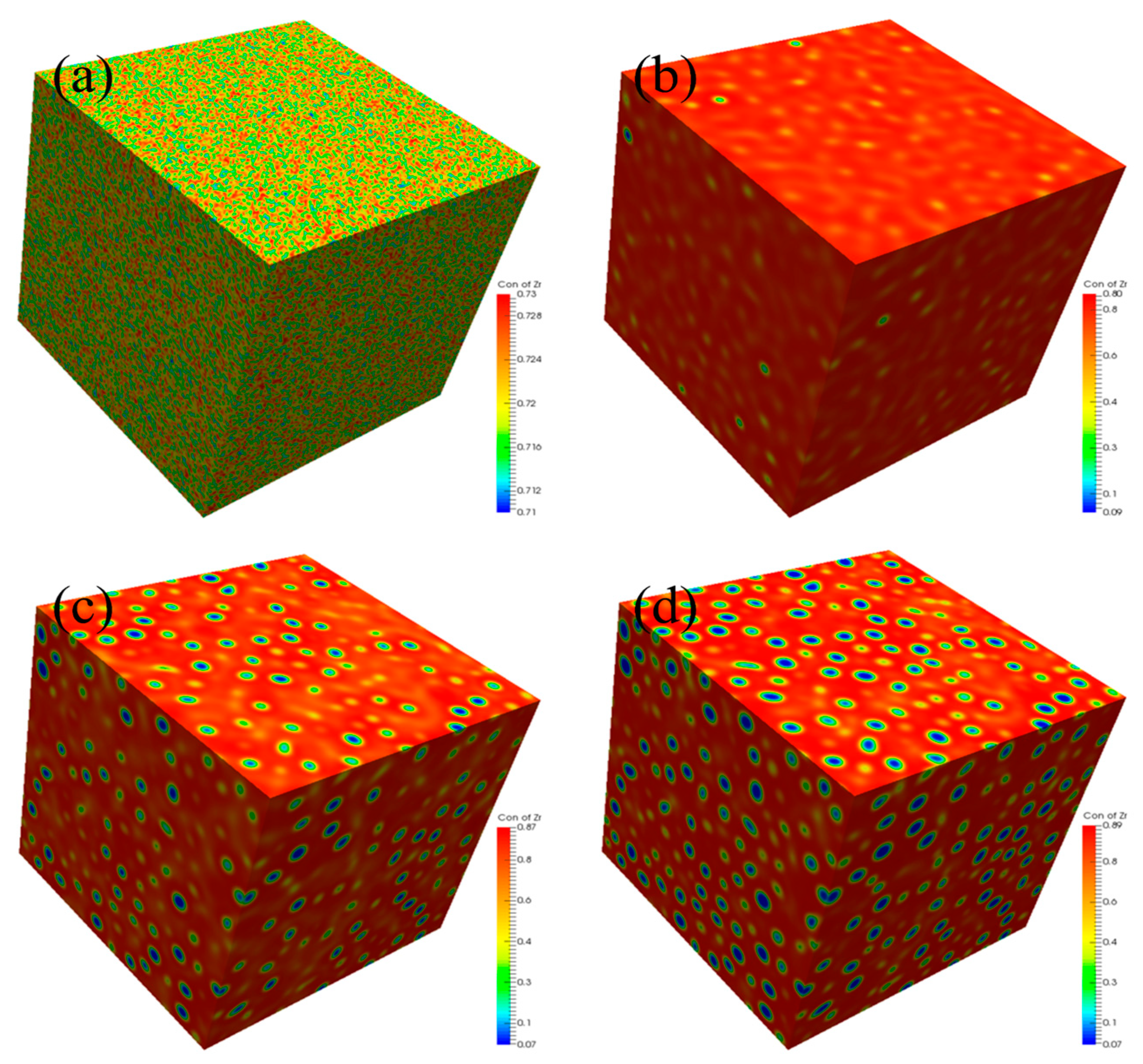
3.4. Three-Dimensional Spinodal Decomposition
4. Conclusions
- (1)
- There are slight composition fluctuations in the U-50Zr alloy as the initial condition of spinodal decomposition. During spinodal decomposition, U atoms in the alloy gradually precipitate and aggregate into U-rich phases. Over time, the precipitated elements begin to coarsen, and the structure evolves from worm-like to spherical. Simultaneously, the precipitate phase continues to grow through the Ostwald ripening mechanism.
- (2)
- The model developed in this study is suitable for the simulation of spinodal decomposition of single crystal U-50Zr alloy in both two-dimensional (2D) and three-dimensional (3D) simulation. The simulation results showed that the temperature has a significant influence on the spinodal decomposition of U-50Zr. As the temperature increases, the time for the spinodal decomposition to reach equilibrium shortens, the rate of phase evolution accelerates, and the rate of coarsening increases. Research has found that temperature mainly affects spinodal decomposition by influencing the atomic diffusion coefficient and the Gibbs free energy of the system.
- (3)
- The 2D simulated results about the spinodal decomposition of polycrystalline U-50Zr alloy showed that GBs have a significant impact on spinodal decomposition. The precipitation phase of spinodal decomposition initially occurs at the GBs, and isolated small spherical U-phases appear within the Zr matrix inside the grains later. As time progresses, large U-rich phases at GBs undergo Ostwald ripening, and grow by absorbing U atoms from the U-rich phase within the grains.
- (4)
- The three-dimensional (3D) spinodal decomposition of U-50Zr single crystal was also simulated, and the simulation results showed that the phases after spinodal decomposition were isolated U-rich phase and matrix Zr-rich phase, respectively. The U-rich phase gradually evolved from an initial worm-like shape to a spherical shape. Due to the influence of surrounding U-rich phases, non-spherical U-rich phases may appear in 3D simulations. However, when the model developed in this study is applied to simulate the 3D spinodal decomposition of polycrystalline alloy, some other factors should be considered.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Basak, C.; Prasad, G.; Kamath, H. An evaluation of the properties of As-cast U-rich U–Zr alloys. J. Alloys Compd. 2009, 480, 857–862. [Google Scholar] [CrossRef]
- Hofman, G.L.; Walters, L.C.; Bauer, T. Metallic fast reactor fuels. Prog. Nucl. Energy 1997, 31, 83–110. [Google Scholar] [CrossRef]
- Landa, A.; Söderlind, P.; Turchi, P. Density-functional study of U–Mo and U–Zr alloys. J. Nucl. Mater. 2011, 414, 132–137. [Google Scholar] [CrossRef]
- Riyas, A.; Mohanakrishnan, P. Studies on physics parameters of metal (U–Pu–Zr) fuelled FBR cores. Ann. Nucl. Energy 2008, 35, 87–92. [Google Scholar] [CrossRef]
- Marques, J. Evolution of nuclear fission reactors: Third generation and beyond. Energy Convers. Manag. 2010, 51, 1774–1780. [Google Scholar] [CrossRef]
- Marcus, G.H. Considering the next generation of nuclear power plants. Prog. Nucl. Energy 2000, 37, 5–10. [Google Scholar] [CrossRef]
- Yao, T.; Wagner, A.R.; Liu, X. On spinodal-like phase decomposition in U–50Zr alloy. Materialia 2020, 9, 100592. [Google Scholar] [CrossRef]
- Yao, T.; Sen, A.; Wagner, A. Understanding spinodal and binodal phase transformations in U-50Zr. Materialia 2021, 16, 101092. [Google Scholar] [CrossRef]
- Zhang, L.; Xiang, Z.; Li, X. Spinodal decomposition in Fe-25Cr-12Co alloys under the influence of high magnetic field and the effect of grain boundary. Nanomaterials 2018, 8, 578. [Google Scholar] [CrossRef]
- Zhu, L.; Li, Y.; Shi, S. Morphology and kinetics evolution of nanoscale phase in Fe–Cr alloys under external strain. Nanomaterials 2019, 9, 294. [Google Scholar] [CrossRef]
- Yan, Z.; Shi, S.; Sang, P. Elastic Strain Relaxation of Phase Boundary of α′ Nanoscale Phase Mediated via the Point Defects Loop under Normal Strain. Nanomaterials 2023, 13, 456. [Google Scholar] [CrossRef] [PubMed]
- Basak, C.; Neogy, S.; Srivastava, D. Disordered bcc γ-phase to δ-phase transformation in Zr-rich U-Zr alloy. Philos. Mag. 2011, 91, 3290–3306. [Google Scholar] [CrossRef]
- Xiong, W.; Xie, W.; Shen, C. Thermodynamic modeling of the U–Zr system–A revisit. J. Nucl. Mater. 2013, 443, 331–341. [Google Scholar] [CrossRef]
- DeNys, T.; Gielen, P. Spinodal decomposition in the Fe− Cr system. Metall. Trans. 1971, 2, 1423–1428. [Google Scholar] [CrossRef]
- Yang, Z.; Liu, W.; Liu, X. Effects of grain boundaries and temperature on spinodal decomposition in a binary Fe-Cr alloy: A phase-field simulation. Ann. Nucl. Energy 2023, 193, 110030. [Google Scholar] [CrossRef]
- Radnóczi, G.; Bokányi, E.; Erdélyi, Z. Size dependent spinodal decomposition in Cu-Ag nanoparticles. Acta Mater. 2017, 123, 82–89. [Google Scholar] [CrossRef]
- Khanolkar, A.; Yao, T.; Hua, Z. In situ monitoring of microstructure evolution during thermal processing of uranium-zirconium alloys using laser-generated ultrasound. J. Nucl. Mater. 2021, 553, 153005. [Google Scholar] [CrossRef]
- Li, Y.; Yan, Z.; Zhou, X. Kinetics of initial phase separation and coarsening of nanoscale phase in Fe–Cr alloys. J. Nucl. Mater. 2017, 497, 154–160. [Google Scholar] [CrossRef]
- Liu, X.; Shen, W.; Liu, W. Phase-Field Simulation of Precipitation and Grain Boundary Segregation in Fe-Cr-Al Alloys under Irradiation. Nanomaterials 2024, 14, 1198. [Google Scholar] [CrossRef]
- Yang, K.; Wang, Y.; Tang, J. Phase Field Study on the Spinodal Decomposition of β Phase in Zr–Nb-Ti Alloys. Materials 2023, 16, 2969. [Google Scholar] [CrossRef]
- Lu, Y.; Ni, X.; Guo, H. A Phase-Field Study of Spinodal Decomposition Impeded by Irradiation in U-Mo and U-Mo-Zr Alloys. Materials 2023, 16, 7546. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.Q.; Yang, W. Computer simulation of the domain dynamics of a quenched system with a large number of nonconserved order parameters: The grain-growth kinetics. Phys. Rev. B 1994, 50, 15752. [Google Scholar] [CrossRef] [PubMed]
- Cahn, J.W.; Hilliard, J.E. Free energy of a nonuniform system. I. Interfacial free energy. J. Chem. Phys. 1958, 28, 258–267. [Google Scholar] [CrossRef]
- Dinsdale, A.T. SGTE data for pure elements. Calphad 1991, 15, 317–425. [Google Scholar] [CrossRef]
- Moelans, N.; Blanpain, B.; Wollants, P. A phase field model for the simulation of grain growth in materials containing finely dispersed incoherent second-phase particles. Acta Mater. 2005, 53, 1771–1781. [Google Scholar] [CrossRef]
- Ke, J.H.; Reese, E.R.; Marquis, E.A. Flux effects in precipitation under irradiation–Simulation of Fe-Cr alloys. Acta Mater. 2019, 164, 586–601. [Google Scholar] [CrossRef]
- Andersson, J.O.; Ågren, J. Models for numerical treatment of multicomponent diffusion in simple phases. J. Appl. Phys. 1992, 72, 1350–1355. [Google Scholar] [CrossRef]
- Mohanty, R.; Bush, J.; Okuniewski, M. Thermotransport in γ (bcc) U–Zr alloys: A phase-field model study. J. Nucl. Mater. 2011, 414, 211–216. [Google Scholar] [CrossRef]
- Allen, S.M.; Cahn, J.W. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metall. 1979, 27, 1085–1095. [Google Scholar] [CrossRef]
- Bian, B.; Zhou, P.; Wen, S. Atomic mobilities and diffusivities in UX (X = Nb, Zr, Ti) bcc alloys. Calphad 2018, 61, 85–91. [Google Scholar] [CrossRef]
- Park, Y.; Newell, R.; Mehta, A. Interdiffusion and reaction between U and Zr. J. Nucl. Mater. 2018, 502, 42–50. [Google Scholar] [CrossRef]
- Hirschhorn, J.; Tonks, M.R.; Aitkaliyeva, A. A study of constituent redistribution in U–Zr fuels using quantitative phase-field modeling and sensitivity analysis. J. Nucl. Mater. 2019, 523, 143–156. [Google Scholar] [CrossRef]
- Beeler, B.; Deo, C.; Baskes, M. First principles calculations of the structure and elastic constants of α, β and γ uranium. J. Nucl. Mater. 2013, 433, 143–151. [Google Scholar] [CrossRef]
- Wang, B.T.; Zhang, P.; Liu, H.Y. First-principles calculations of phase transition, elastic modulus, and superconductivity under pressure for zirconium. J. Appl. Phys. 2011, 109, 063514. [Google Scholar] [CrossRef]
- Flewitt, P.E.J. Phase transformations in niobium 16 to 40% zirconium alloys above the monotectoid temperature—I. Acta Metall. 1974, 22, 47–63. [Google Scholar] [CrossRef]
- Xue, F.; Ji, Y.; Chen, L.Q. Theory of strain phase separation and strain spinodal: Applications to ferroelastic and ferroelectric systems. Acta Mater. 2017, 133, 147–159. [Google Scholar] [CrossRef]
- Soisson, F.; Jourdan, T. Radiation-accelerated precipitation in Fe–Cr alloys. Acta Mater. 2016, 103, 870–881. [Google Scholar] [CrossRef]
- Mishin, Y. Solute drag and dynamic phase transformations in moving grain boundaries. Acta Mater. 2019, 179, 383–395. [Google Scholar] [CrossRef]
- Hofman, G.; Hayes, S.; Petri, M. Temperature gradient driven constituent redistribution in U-Zr alloys. J. Nucl. Mater. 1996, 227, 277–286. [Google Scholar] [CrossRef]
- Fu, E.; Carter, J.; Swadener, G. Size dependent enhancement of helium ion irradiation tolerance in sputtered Cu/V nanolaminates. J. Nucl. Mater. 2009, 385, 629–632. [Google Scholar] [CrossRef]
- Diaz de la Rubia, T.; Zbib, H.M.; Khraishi, T.A. Multiscale modelling of plastic flow localization in irradiated materials. Nature 2000, 406, 871–874. [Google Scholar] [CrossRef] [PubMed]
- Seol, D.; Hu, S.; Li, Y. Three-dimensional phase-field modeling of spinodal decomposition in constrained films. Met. Mater. Int. 2003, 9, 61–66. [Google Scholar] [CrossRef][Green Version]
- Yang, J.; Heogh, W.; Ju, H. Functionally graded structure of a nitride-strengthened Mg2Si-based hybrid composite. J. Magnes. Alloys 2024, 12, 1239–1256. [Google Scholar] [CrossRef]
- Lee, J.; Park, K.; Chang, K. Effect of al concentration on the microstructural evolution of fe-cr-al systems: A phase-field approach. Metals 2020, 11, 4. [Google Scholar] [CrossRef]
- Preis, T.; Virnau, P.; Paul, W. GPU accelerated Monte Carlo simulation of the 2D and 3D Ising model. J. Comput. Phys. 2009, 228, 4468–4477. [Google Scholar] [CrossRef]
- Njifon, C.; Torres, E. Atomistic simulation study of the structure, segregation and stability of grain boundaries in the U-Zr metallic fuel. J. Nucl. Mater. 2023, 583, 154505. [Google Scholar] [CrossRef]
- Li, Y.; Yu, Q.; Ham, S. A phase-field model without artificial curvature effect for the crystal growth simulation. Int. J. Heat Mass Transf. 2023, 203, 123847. [Google Scholar] [CrossRef]
- Molnár, G.; Gravouil, A. 2D and 3D Abaqus implementation of a robust staggered phase-field solution for modeling brittle fracture. Finite Elem. Anal. Des. 2017, 130, 27–38. [Google Scholar] [CrossRef]
- Tóth, G.I.; Tegze, G.R.; Pusztai, T. Polymorphism, crystal nucleation and growth in the phase-field crystal model in 2d and 3d. J. Phys. Condens. Matter Inst. Phys. J. 2010, 22, 364101. [Google Scholar] [CrossRef]


| Parameters | Symbol | Value | Reference |
|---|---|---|---|
| U atomic diffusion coefficient | [30] | ||
| Zr atomic diffusion coefficient | [31] | ||
| Concentration gradient coefficient | |||
| Phase gradient coefficient | [32] | ||
| Phase mobility | L | [32] | |
| U lattice constant | 0.353 nm | [33] | |
| Zr lattice constant | 0.357 nm | [34] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
La, Y.; Wen, C.; Feng, L.; Luo, Y.; Yun, D.; Liu, W. Phase-Field Simulation of Spinodal Decomposition in U-50Zr Metallic Nuclear Fuel. Nanomaterials 2024, 14, 1548. https://doi.org/10.3390/nano14191548
La Y, Wen C, Feng L, Luo Y, Yun D, Liu W. Phase-Field Simulation of Spinodal Decomposition in U-50Zr Metallic Nuclear Fuel. Nanomaterials. 2024; 14(19):1548. https://doi.org/10.3390/nano14191548
Chicago/Turabian StyleLa, Yongxiao, Chunyang Wen, Linna Feng, Yihui Luo, Di Yun, and Wenbo Liu. 2024. "Phase-Field Simulation of Spinodal Decomposition in U-50Zr Metallic Nuclear Fuel" Nanomaterials 14, no. 19: 1548. https://doi.org/10.3390/nano14191548
APA StyleLa, Y., Wen, C., Feng, L., Luo, Y., Yun, D., & Liu, W. (2024). Phase-Field Simulation of Spinodal Decomposition in U-50Zr Metallic Nuclear Fuel. Nanomaterials, 14(19), 1548. https://doi.org/10.3390/nano14191548







