Graphene-Based Dual-Band Metasurface Absorber with High Frequency Ratio
Abstract
1. Introduction
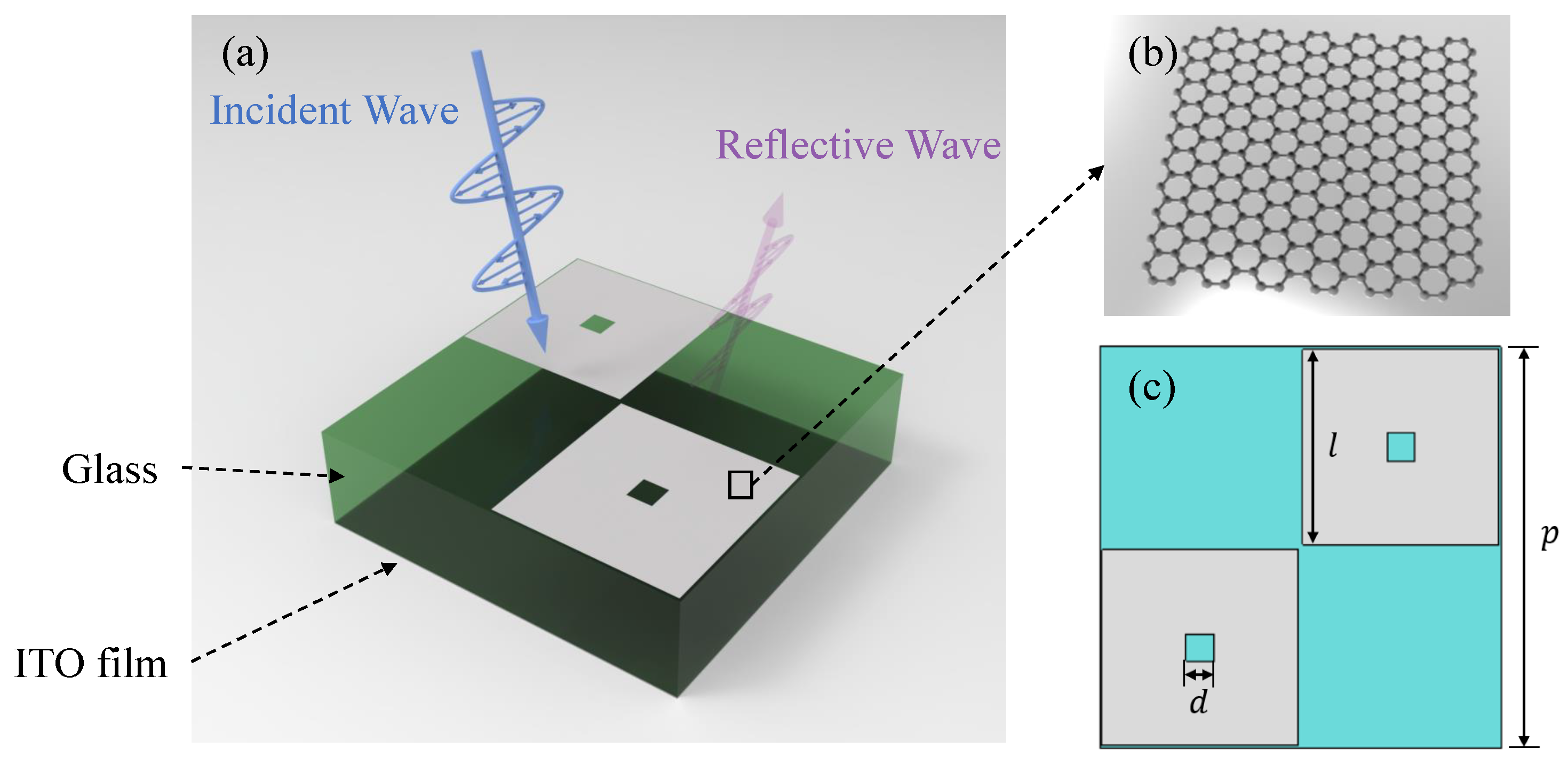
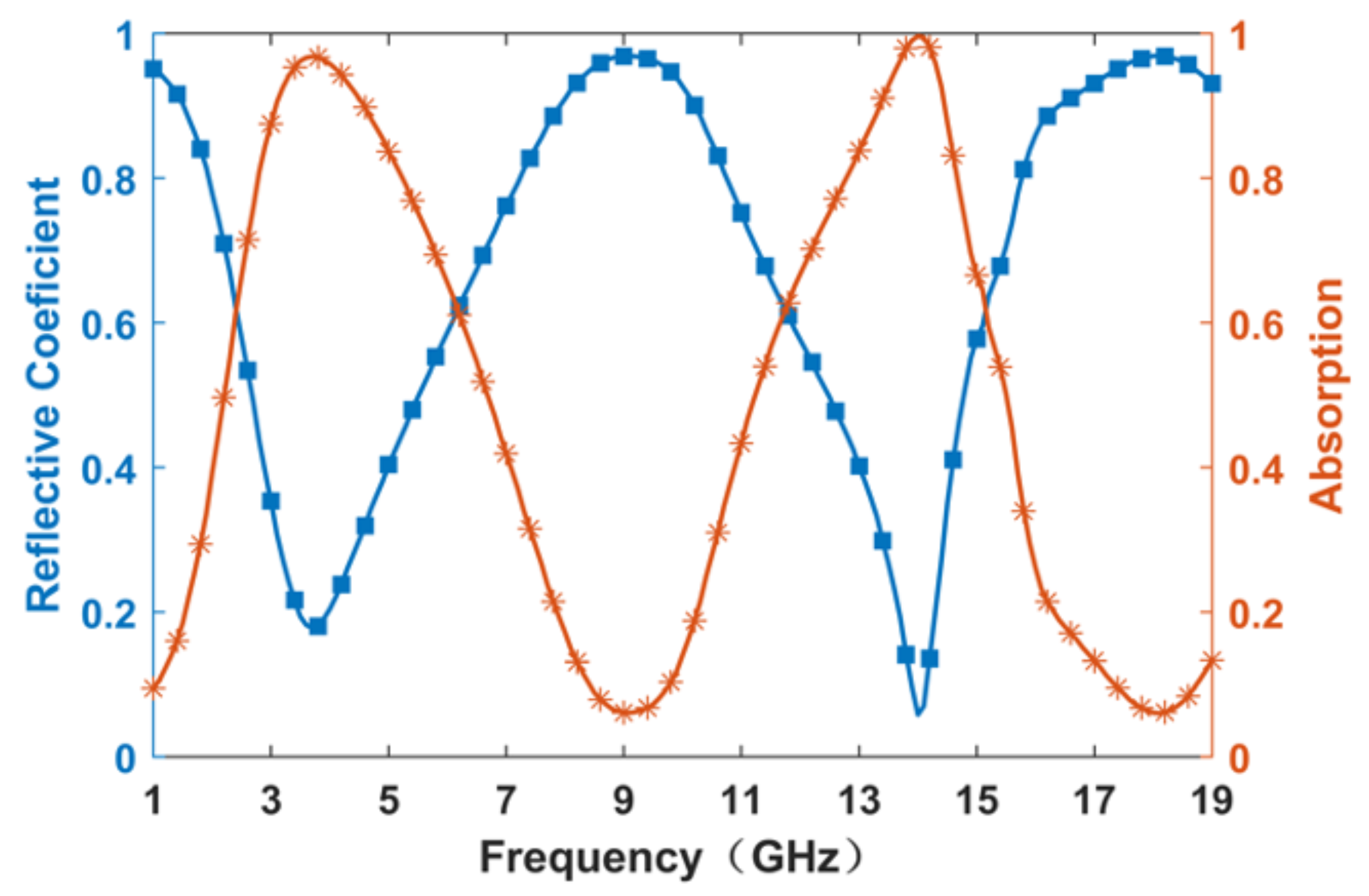
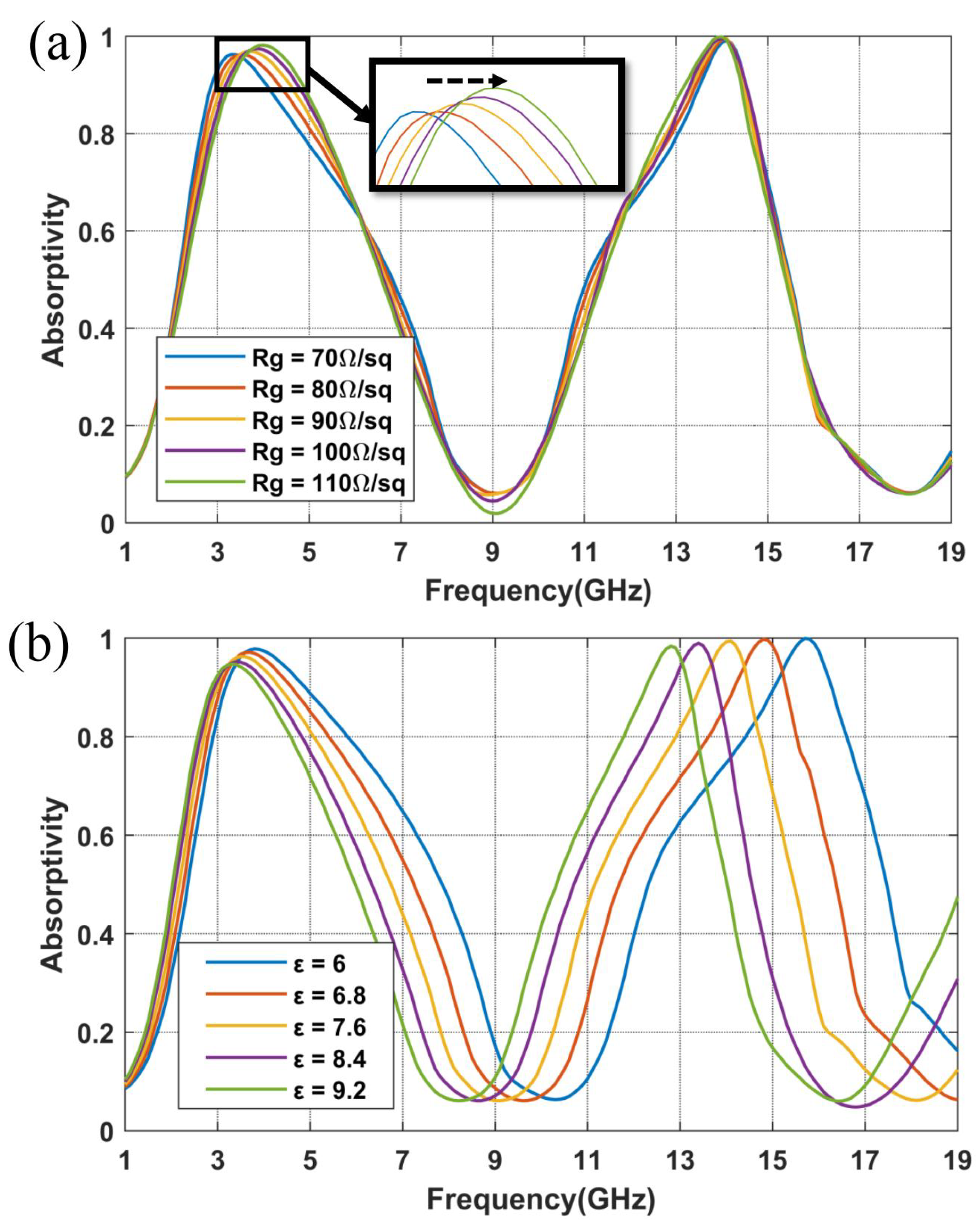
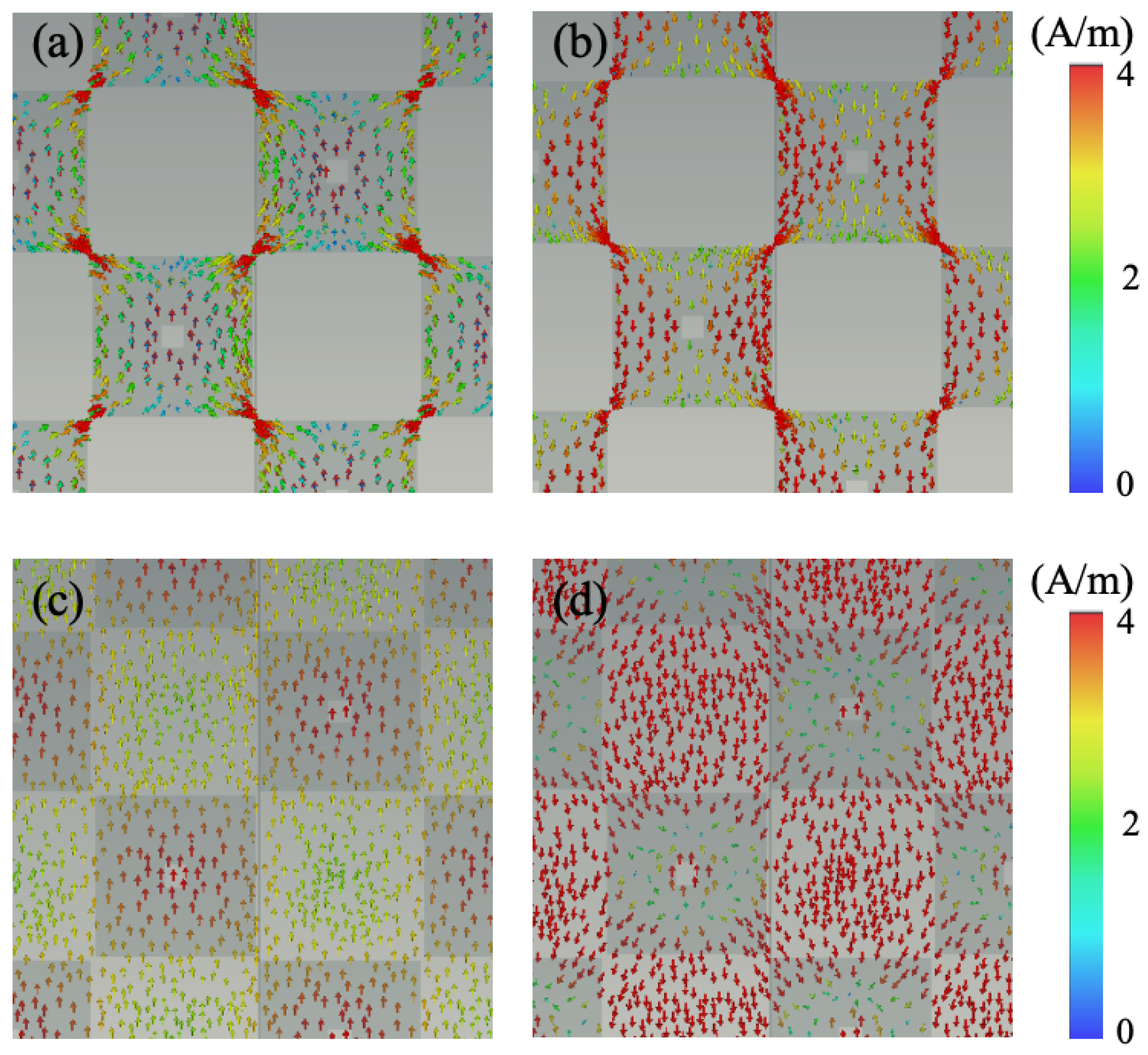
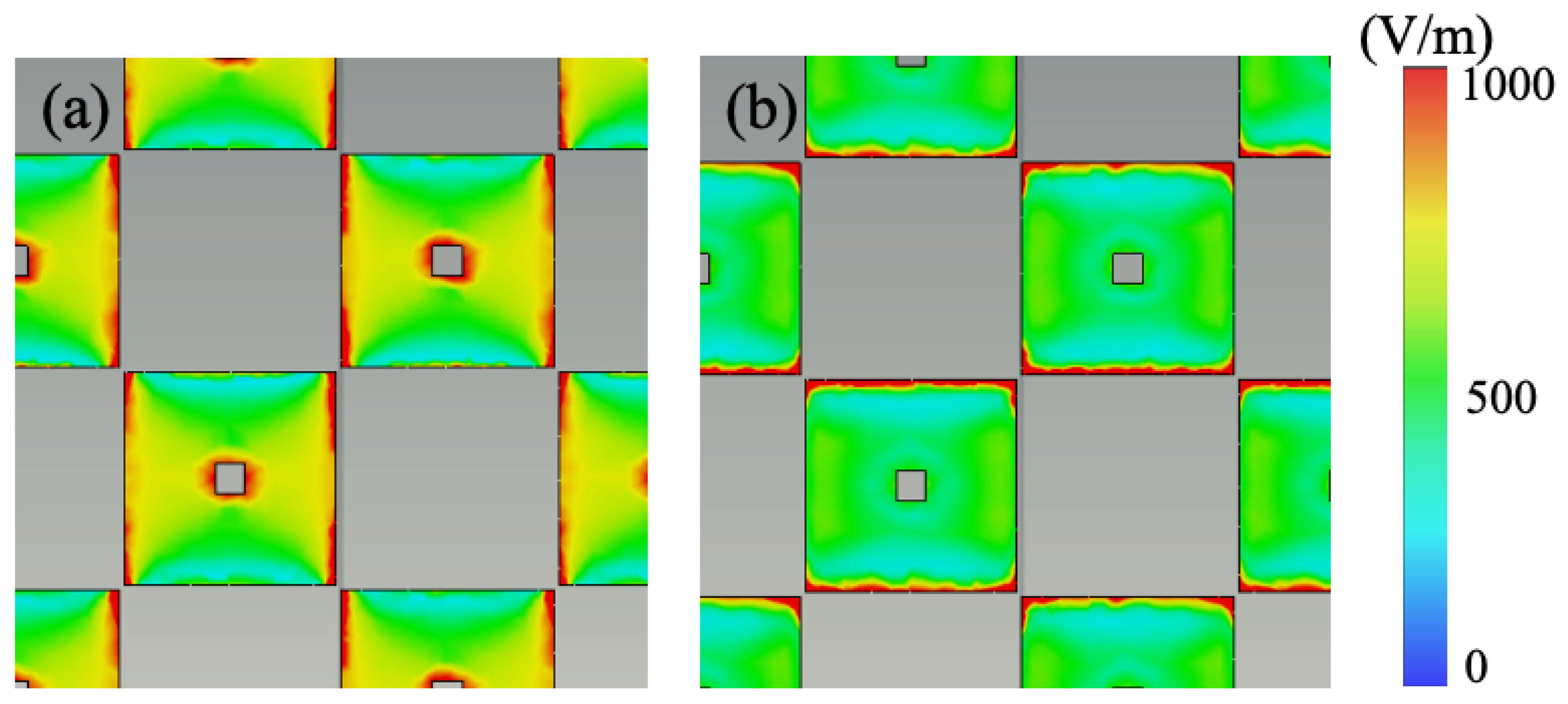
2. Design and Simulation
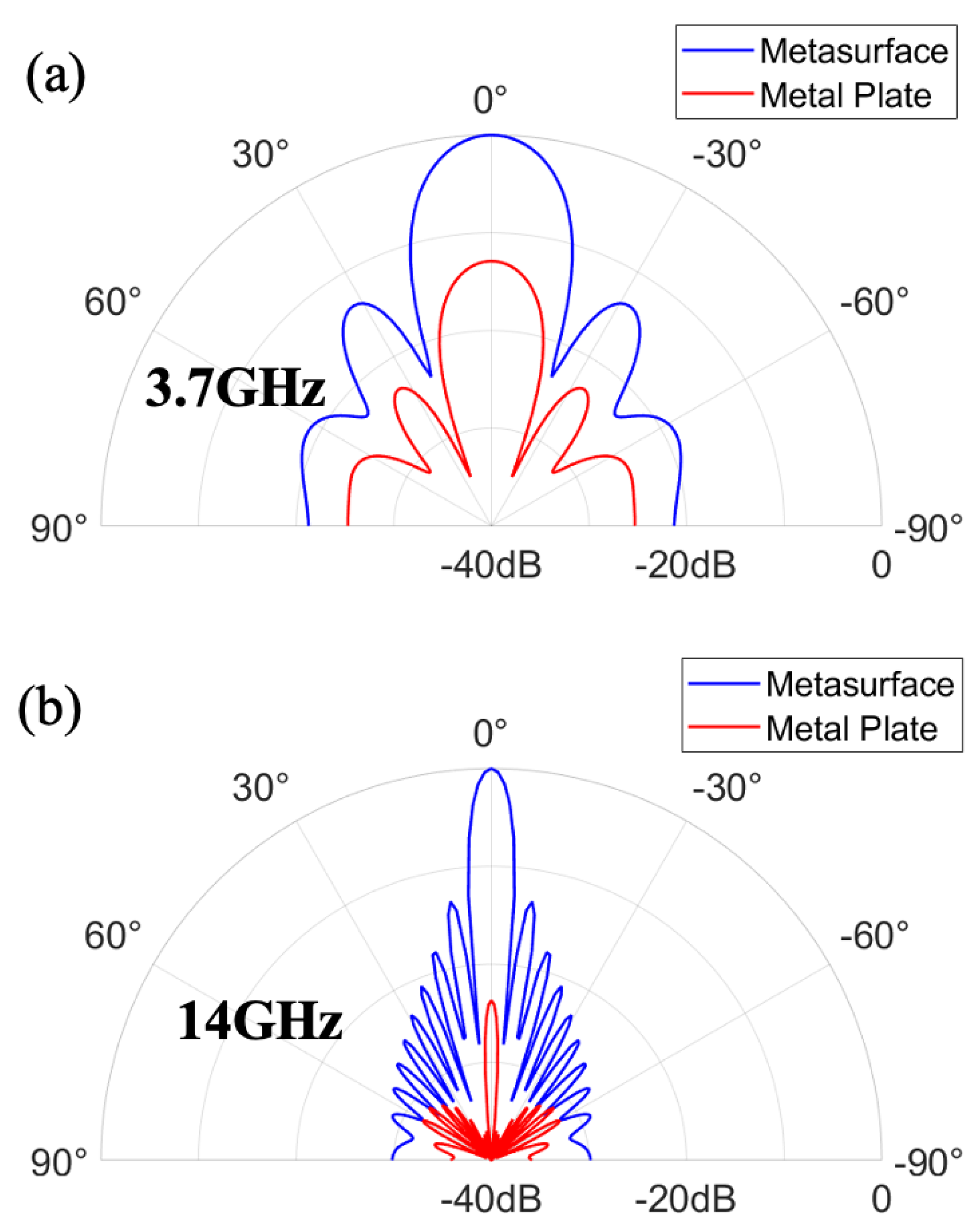
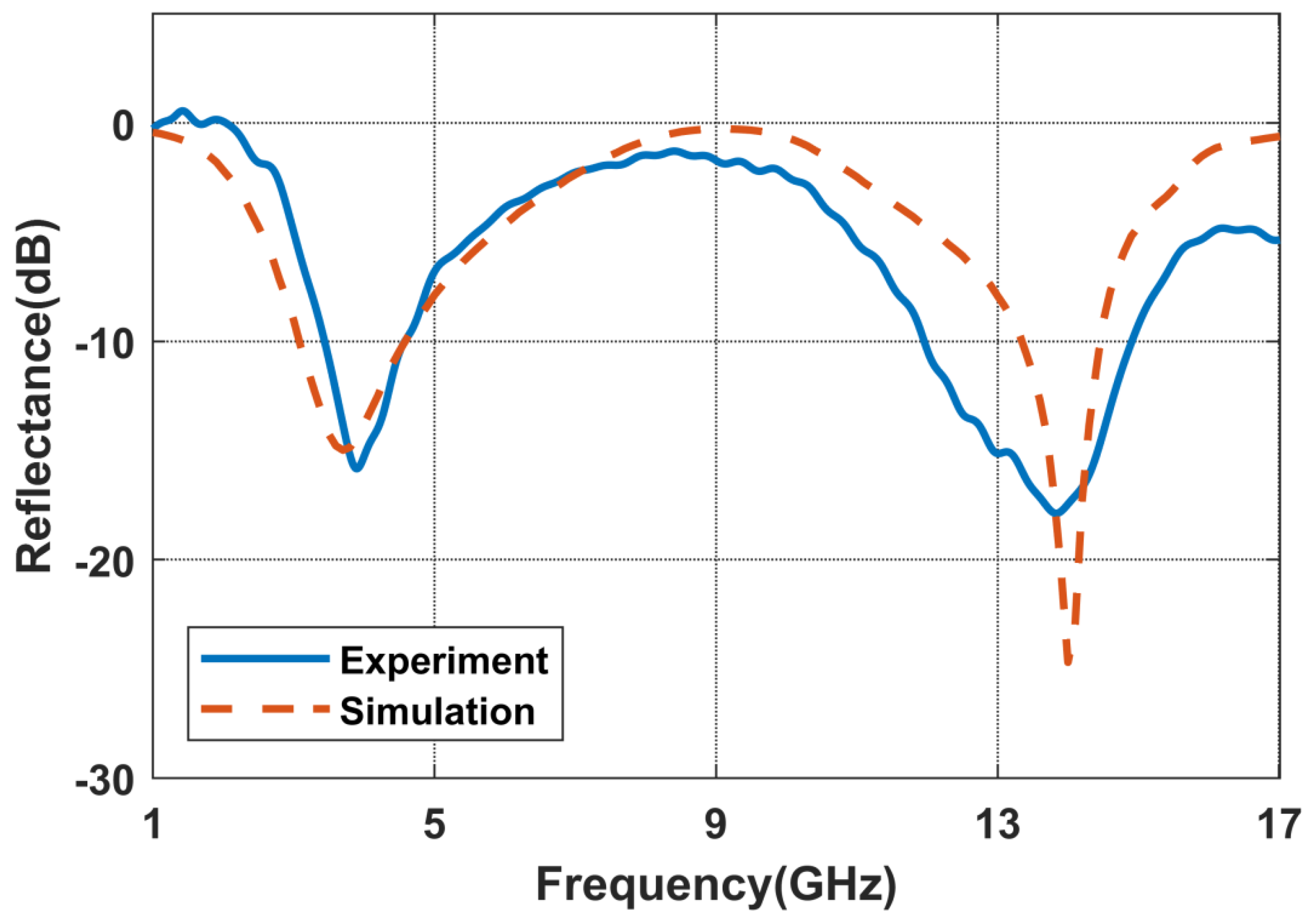
3. Fabrication and Measurement
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef] [PubMed]
- Sun, S.; He, Q.; Xiao, S.; Xu, Q.; Li, X.; Zhou, L. Gradient-index meta-surfaces as a bridge linking propagating waves and surface waves. Nat. Mater. 2012, 11, 426–431. [Google Scholar] [CrossRef] [PubMed]
- Cui, T.J.; Liu, S.; Li, L.L. Information entropy of coding metasurface. Light Sci. Appl. 2016, 5, e16172. [Google Scholar] [CrossRef]
- Chen, K.; Ding, G.; Hu, G.; Jin, Z.; Zhao, J.; Feng, Y.; Jiang, T.; Alù, A.; Qiu, C.W. Directional Janus Metasurface. Adv. Mater. 2020, 32, 1906352. [Google Scholar] [CrossRef] [PubMed]
- Akram, M.R.; Ding, G.; Chen, K.; Feng, Y.; Zhu, W. Ultrathin Single Layer Metasurfaces with Ultra-Wideband Operation for Both Transmission and Reflection. Adv. Mater. 2020, 32, 1907308. [Google Scholar] [CrossRef]
- Wu, X.; Li, Z.; Zhang, Z.; Wang, X.; Si, L.; Zhu, W. Mechanically Reconfigurable Folded Reflectarray Antenna for Variable Near-Field Focusing. IEEE Trans. Antennas Propag. 2023, 71, 10038–10043. [Google Scholar] [CrossRef]
- Lu, W.B.; Wang, J.W.; Zhang, J.; Liu, Z.G.; Chen, H.; Song, W.J.; Jiang, Z.H. Flexible and optically transparent microwave absorber with wide bandwidth based on graphene. Carbon 2019, 152, 70–76. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Z.; Zhang, C.; Shao, L.; Zhu, W. Dynamic manipulation of microwave polarization based on anisotropic graphene meta-device. NPJ 2D Mater. Appl. 2022, 6, 47. [Google Scholar] [CrossRef]
- Tian, J.; Shi, H.; Li, S.; He, C.; Zhu, W. Single-Layer Programmable Metasurface for Manipulating Both Linearly and Circularly Polarized Electromagnetic Waves. ACS Appl. Opt. Mater. 2024, 2, 341–349. [Google Scholar] [CrossRef]
- Ni, X.; Emani, N.K.; Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Broadband Light Bending with Plasmonic Nanoantennas. Science 2012, 335, 427. [Google Scholar] [CrossRef]
- Shao, L.; Zhang, Z.; Wang, X.; He, C.; Si, L.; Rukhlenko, I.D.; Zhu, W. Graphene-based ultralow-profile microwave Fresnel lens. Carbon 2024, 217, 118599. [Google Scholar] [CrossRef]
- Zhang, C.; Xue, T.; Zhang, J.; Liu, L.; Xie, J.; Wang, G.; Yao, J.; Zhu, W.; Ye, X. Terahertz toroidal metasurface biosensor for sensitive distinction of lung cancer cells. Nanophotonics 2022, 11, 101–109. [Google Scholar] [CrossRef]
- Tang, W.; Chen, M.Z.; Dai, J.Y.; Zeng, Y.; Zhao, X.; Jin, S.; Cheng, Q.; Cui, T.J. Wireless Communications with Programmable Metasurface: New Paradigms, Opportunities, and Challenges on Transceiver Design. IEEE Wirel. Commun. 2020, 27, 180–187. [Google Scholar] [CrossRef]
- Shao, L.; Zhu, W. Recent advances in electromagnetic metamaterials and metasurfaces for polarization manipulation. J. Phys. D Appl. Phys. 2024, 57, 343001. [Google Scholar] [CrossRef]
- Li, Z.; Premaratne, M.; Zhu, W. Advanced encryption method realized by secret shared phase encoding scheme using a multi-wavelength metasurface. Nanophotonics 2020, 9, 3687–3696. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Geim, A.K. Graphene: Status and Prospects. Science 2009, 324, 1530–1534. [Google Scholar] [CrossRef] [PubMed]
- Koppens, F.H.L.; Mueller, T.; Avouris, P.; Ferrari, A.C.; Vitiello, M.S.; Polini, M. Photodetectors based on graphene, other two-dimensional materials and hybrid systems. Nat. Nanotechnol. 2014, 9, 780–793. [Google Scholar] [CrossRef]
- Bonaccorso, F.; Sun, Z.; Hasan, T.; Ferrari, A.C. Graphene photonics and optoelectronics. Nat. Photonics 2010, 4, 611–622. [Google Scholar] [CrossRef]
- Shao, L.; Zhu, W. Graphene-derived microwave metamaterials and meta-devices: Emerging applications and properties. Electron 2024, 2, e60. [Google Scholar] [CrossRef]
- Pianelli, A.; Kowerdziej, R.; Dudek, M.; Sielezin, K.; Olifierczuk, M.; Parka, J. Graphene-based hyperbolic metamaterial as a switchable reflection modulator. Opt. Express 2020, 28, 6708–6718. [Google Scholar] [CrossRef] [PubMed]
- Dudek, M.; Kowerdziej, R.; Pianelli, A.; Parka, J. Graphene-based tunable hyperbolic microcavity. Sci. Rep. 2021, 11, 74. [Google Scholar] [CrossRef] [PubMed]
- Shi, H.; Tian, J.; Chen, N.; Zhu, W. Wideband high-efficiency scattering reduction in a graphene based optically transparent and flexible metasurface. Carbon 2024, 225, 119150. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Z.; Shao, L.; Xiao, F.; Zhu, W. Active modulation of electromagnetically induced transparency analog in graphene-based microwave metamaterial. Carbon 2021, 183, 850–857. [Google Scholar] [CrossRef]
- Liu, W.; Hu, B.; Huang, Z.; Guan, H.; Li, H.; Wang, X.; Zhang, Y.; Yin, H.; Xiong, X.; Liu, J.; et al. Graphene-enabled electrically controlled terahertz meta-lens. Photonics Res. 2018, 6, 703–708. [Google Scholar] [CrossRef]
- Chen, H.; Lu, W.B.; Liu, Z.G.; Geng, M.Y. Microwave Programmable Graphene Metasurface. ACS Photonics 2020, 7, 1425–1435. [Google Scholar] [CrossRef]
- Jiang, H.; Wei, J.; Sun, F.; Nie, C.; Fu, J.; Shi, H.; Sun, J.; Wei, X.; Qiu, C.W. Enhanced Photogating Effect in Graphene Photodetectors via Potential Fluctuation Engineering. ACS Nano 2022, 16, 4458–4466. [Google Scholar] [CrossRef]
- Jiang, H.; Fu, J.; Wei, J.; Li, S.; Nie, C.; Sun, F.; Wu, Q.Y.S.; Liu, M.; Dong, Z.; Wei, X.; et al. Synergistic-potential engineering enables high-efficiency graphene photodetectors for near- to mid-infrared light. Nat. Commun. 2024, 15, 1225. [Google Scholar] [CrossRef]
- Fu, J.; Nie, C.; Sun, F.; Li, G.; Shi, H.; Wei, X. Bionic visual-audio photodetectors with in-sensor perception and preprocessing. Sci. Adv. 2024, 10, eadk8199. [Google Scholar] [CrossRef]
- Lee, S.H.; Choi, M.; Kim, T.T.; Lee, S.; Liu, M.; Yin, X.; Choi, H.K.; Lee, S.S.; Choi, C.G.; Choi, S.Y.; et al. Switching terahertz waves with gate-controlled active graphene metamaterials. Nat. Mater. 2012, 11, 936–941. [Google Scholar] [CrossRef]
- Sensale-Rodriguez, B.; Yan, R.; Kelly, M.M.; Fang, T.; Tahy, K.; Hwang, W.S.; Jena, D.; Liu, L.; Xing, H.G. Broadband graphene terahertz modulators enabled by intraband transitions. Nat. Commun. 2012, 3, 780. [Google Scholar] [CrossRef] [PubMed]
- Hanson, G.W. Dyadic Green’s functions and guided surface waves for a surface conductivity model of graphene. J. Appl. Phys. 2008, 103, 064302. [Google Scholar] [CrossRef]
- Zhang, J.; Shao, L.; Li, Z.; Zhang, C.; Zhu, W. Graphene-Based Optically Transparent Metasurface Capable of Dual-Polarized Modulation for Electromagnetic Stealth. ACS Appl. Mater. Interfaces 2022, 14, 31075–31084. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, A.; Chen, N.; Zhu, W.; Chen, Z. Graphene-Based Dual-Band Metasurface Absorber with High Frequency Ratio. Nanomaterials 2024, 14, 1522. https://doi.org/10.3390/nano14181522
Cao A, Chen N, Zhu W, Chen Z. Graphene-Based Dual-Band Metasurface Absorber with High Frequency Ratio. Nanomaterials. 2024; 14(18):1522. https://doi.org/10.3390/nano14181522
Chicago/Turabian StyleCao, Anjie, Nengfu Chen, Weiren Zhu, and Zhansheng Chen. 2024. "Graphene-Based Dual-Band Metasurface Absorber with High Frequency Ratio" Nanomaterials 14, no. 18: 1522. https://doi.org/10.3390/nano14181522
APA StyleCao, A., Chen, N., Zhu, W., & Chen, Z. (2024). Graphene-Based Dual-Band Metasurface Absorber with High Frequency Ratio. Nanomaterials, 14(18), 1522. https://doi.org/10.3390/nano14181522





