Plasmon Resonance in a System of Bi Nanoparticles Embedded into (Al,Ga)As Matrix
Abstract
1. Introduction
2. Calculation Methods and Computational Details
3. Calculation Results and Discussion
3.1. Electronic Structure and Dielectric Function of Bismuth
3.2. Electronic Structure and Dielectric Function of AlGaAs
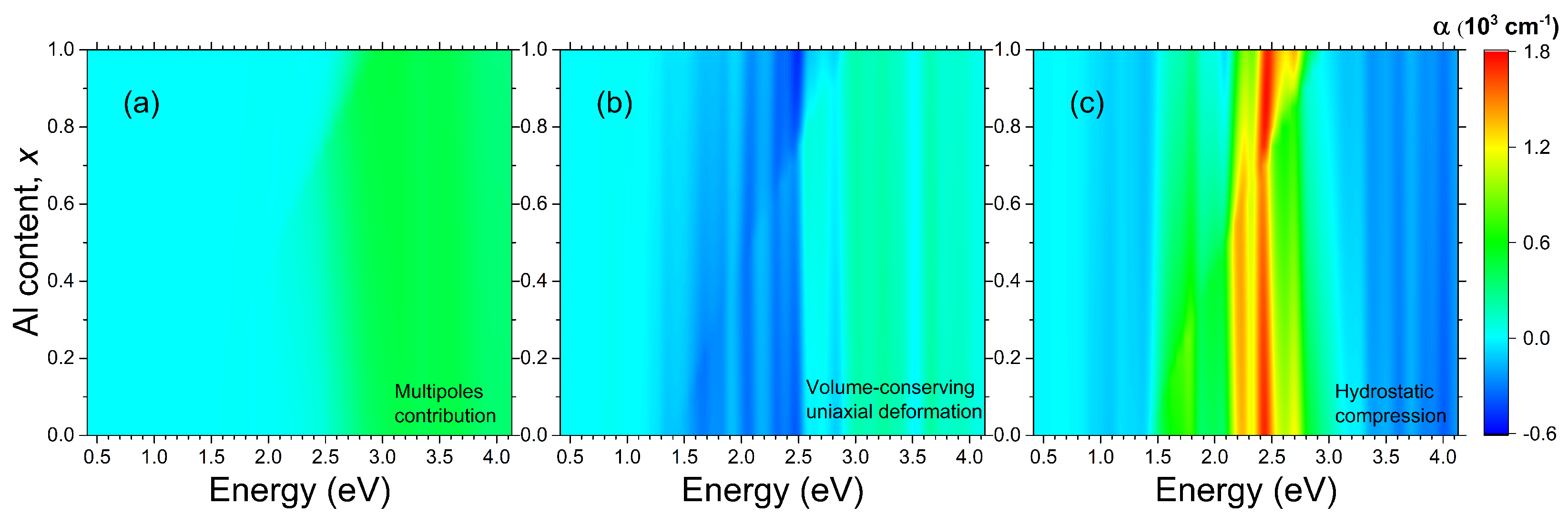
3.3. Localized Surface Plasmons
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| LSPR | Localized surface plasmon resonance. |
| NP | Nanoparticle. |
| MBE | Molecular-beam epitaxy. |
| VPE | Vapor-phase epitaxy. |
| LT | Low temperature. |
| PAW | Projector-augmented wave. |
| GGA-PBE | Perdew–Burke–Ernzerhof generalized gradient approximation. |
| vdW | van der Waals. |
| BL | Bilayer. |
| DFT-D3 | vdW functional with Becke–Johnson damping. |
| mBJ | modified Becke–Johnson semilocal exchange potential. |
| HSE06 | Heyd–Scuseria–Ernzerhof screened hybrid functional. |
| QS | Quasiparticle self-consistent . |
| TRIM | Time reversal-invariant momentum. |
References
- Maier, S. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar]
- Garcia, M.A. Surface plasmons in metallic nanoparticles: Fundamentals and applications. J. Phys. D Appl. Phys. 2011, 44, 283001. [Google Scholar] [CrossRef]
- Wang, L.; Kafshgari, M.H.; Meunier, M. Optical Properties and Applications of Plasmonic-Metal Nanoparticles. Adv. Funct. Mater. 2020, 30, 2005400. [Google Scholar] [CrossRef]
- Rivera, N.; Kaminer, I. Light–matter interactions with photonic quasiparticles. Nature Rev. Phys. 2020, 2, 538–561. [Google Scholar] [CrossRef]
- Koya, A.N.; Romanelli, M.; Kuttruff, J.; Henriksson, N.; Stefancu, A.; Grinblat, G.; De Andres, A.; Schnur, F.; Vanzan, M.; Marsili, M.; et al. Advances in ultrafast plasmonics. Appl. Phys. Rev. 2023, 10, 021318. [Google Scholar] [CrossRef]
- Babicheva, V.E. Optical Processes behind Plasmonic Applications. Nanomaterials 2023, 13, 1270. [Google Scholar] [CrossRef] [PubMed]
- Mandal, P. Application of Plasmonics in Solar Cell Efficiency Improvement: A Brief Review on Recent Progress. Plasmonics 2022, 17, 1247–1267. [Google Scholar] [CrossRef]
- Ibrahim Zamkoye, I.; Lucas, B.; Vedraine, S. Synergistic Effects of Localized Surface Plasmon Resonance, Surface Plasmon Polariton, and Waveguide Plasmonic Resonance on the Same Material: A Promising Hypothesis to Enhance Organic Solar Cell Efficiency. Nanomaterials 2023, 13, 2209. [Google Scholar] [CrossRef]
- Agiotis, L.; Meunier, M. Nonlinear Propagation of Laser Light in Plasmonic Nanocomposites. Laser Photonics Rev. 2022, 16, 2200076. [Google Scholar] [CrossRef]
- Rajamanikandan, R.; Sasikumar, K.; Kosame, S.; Ju, H. Optical Sensing of Toxic Cyanide Anions Using Noble Metal Nanomaterials. Nanomaterials 2023, 13, 290. [Google Scholar] [CrossRef]
- Zhang, C.; Huang, C.; Pu, M.; Song, J.; Zhao, Z.; Wu, X.; Luo, X. Dual-band wide-angle metamaterial perfect absorber based on the combination of localized surface plasmon resonance and Helmholtz resonance. Sci. Rep. 2017, 7, 5652. [Google Scholar] [CrossRef]
- Fleischmann, M.; Hendra, P.; McQuillan, A. Raman spectra of pyridine adsorbed at a silver electrode. Chem. Phys. Lett. 1974, 26, 163–166. [Google Scholar] [CrossRef]
- Bai, S.; Ren, X.; Obata, K.; Ito, Y.; Sugioka, K. Label-free trace detection of bio-molecules by liquid-interface assisted surface-enhanced Raman scattering using a microfluidic chip. Opto-Electron. Adv. 2022, 5, 210121-1–210121-10. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, X.; Li, Y.; Li, C.; Wang, X.; Wu, J.; Li, Y. Handcrafted silver substrates boost surface plasmon resonance for ultra-sensitive lipid analysis. Talanta 2024, 269, 125432. [Google Scholar] [CrossRef] [PubMed]
- Bogdanov, S.; Boltasseva, A.; Shalaev, V. Overcoming quantum decoherence with plasmonics. Science 2019, 364, 532–533. [Google Scholar] [CrossRef]
- Toropov, N.A.; Gladskikh, I.A.; Gladskikh, P.V.; Kosarev, A.N.; Preobrazhenskiĭ, V.V.; Putyato, M.A.; Semyagin, B.R.; Chaldyshev, V.V.; Vartanyan, T.A. Absorption and photoluminescence of epitaxial quantum dots in the near field of silver nanostructures. J. Opt. Technol. 2017, 84, 459–461. [Google Scholar] [CrossRef]
- Liu, X.; Prasad, A.; Nishio, J.; Weber, E.R.; Liliental-Weber, Z.; Walukiewicz, W. Native point defects in low-temperature-grown GaAs. Appl. Phys. Lett. 1995, 67, 279–281. [Google Scholar] [CrossRef]
- Lavrent’eva, L.G.; Vilisova, M.D.; Preobrazhenskii, V.V.; Chaldyshev, V.V. Low-temperature molecular beam epitaxy of GaAs: Influence of crystallization conditions on structure and properties of layers. Crystallogr. Rep. 2002, 47, S118–S127. [Google Scholar] [CrossRef]
- Geursen, R.; Lahiri, I.; Dinu, M.; Melloch, M.R.; Nolte, D.D. Transient enhanced intermixing of arsenic-rich nonstoichiometric AlAs/GaAs quantum wells. Phys. Rev. B 1999, 60, 10926–10934. [Google Scholar] [CrossRef]
- Bert, N.A.; Chaldyshev, V.V.; Musikhin, Y.G.; Suvorova, A.A.; Preobrazhenskii, V.V.; Putyato, M.A.; Semyagin, B.R.; Werner, P. In–Ga intermixing in low-temperature grown GaAs delta doped with In. Appl. Phys. Lett. 1999, 74, 1442–1444. [Google Scholar] [CrossRef][Green Version]
- Chaldyshev, V.V.; Bert, N.A.; Musikhin, Y.G.; Suvorova, A.A.; Preobrazhenskii, V.V.; Putyato, M.A.; Semyagin, B.R.; Werner, P.; Gösele, U. Enhanced As–Sb intermixing of GaSb monolayer superlattices in low-temperature grown GaAs. Appl. Phys. Lett. 2001, 79, 1294–1296. [Google Scholar] [CrossRef][Green Version]
- Warren, A.C.; Woodall, J.M.; Freeouf, J.L.; Grischkowsky, D.; McInturff, D.T.; Melloch, M.R.; Otsuka, N. Arsenic precipitates and the semi-insulating properties of GaAs buffer layers grown by low-temperature molecular beam epitaxy. Appl. Phys. Lett. 1990, 57, 1331–1333. [Google Scholar] [CrossRef]
- Claverie, A.; Liliental-Weber, Z. Structure and orientation of As precipitates in GaAs grown at low temperature by molecular beam epitaxy. Philos. Mag. A 1992, 65, 981–1002. [Google Scholar] [CrossRef]
- Bert, N.A.; Veinger, A.I.; Vilisova, M.D.; Goloshchapov, S.I.; Ivonin, I.V.; Kozyrev, S.V.; Kunitsyn, A.E.; Lavrent’eva, L.G.; Lubyshev, D.I.; Preobrazhenskii, V.V.; et al. Gallium arsenide grown by molecular beam epitaxy at low temperatures: Crystal structure, properties, superconductivity. Phys. Solid State 1993, 35, 1289–1297. [Google Scholar]
- Bert, N.A.; Chaldyshev, V.V.; Cherkashin, N.A.; Nevedomskiy, V.N.; Preobrazhenskii, V.V.; Putyato, M.A.; Semyagin, B.R.; Ushanov, V.I.; Yagovkina, M.A. Metallic AsSb nanoinclusions strongly enriched by Sb in AlGaAsSb metamaterial. J. Appl. Phys. 2019, 125, 145106. [Google Scholar] [CrossRef]
- Wu, M.; Luna, E.; Puustinen, J.; Guina, M.; Trampert, A. Formation and phase transformation of Bi-containing QD-like clusters in annealed GaAsBi. Nanotechnology 2014, 25, 205605. [Google Scholar] [CrossRef] [PubMed]
- Butkutė, R.; Niaura, G.; Pozingytė, E.; Čechavičius, B.; Selskis, A.; Skapas, M.; Karpus, V.; Krotkus, A. Bismuth Quantum Dots in Annealed GaAsBi/AlAs Quantum Wells. Nanoscale Res. Lett. 2017, 12, 436. [Google Scholar] [CrossRef] [PubMed]
- Baladés, N.; Sales, D.; Herrera, M.; Tan, C.; Liu, Y.; Richards, R.; Molina, S. Analysis of Bi distribution in epitaxial GaAsBi by aberration-corrected HAADF-STEM. Nanoscale Res. Lett. 2018, 13, 125. [Google Scholar] [CrossRef] [PubMed]
- Nolte, D.D. Optical scattering and absorption by metal nanoclusters in GaAs. J. Appl. Phys. 1994, 76, 3740–3745. [Google Scholar] [CrossRef]
- Dankowski, S.U.; Streb, D.; Ruff, M.; Kiesel, P.; Kneissl, M.; Knüpfer, B.; Döhler, G.H.; Keil, U.D.; Sorenson, C.B.; Verma, A.K. Above band gap absorption spectra of the arsenic antisite defect in low temperature grown GaAs and AlGaAs. Appl. Phys. Lett. 1996, 68, 37–39. [Google Scholar] [CrossRef]
- Ushanov, V.I.; Chaldyshev, V.V.; Il’inskaya, N.D.; Lebedeva, N.M.; Yagovkina, M.A.; Preobrazhenskii, V.V.; Putyato, M.A.; Semyagin, B.R. Fröhlich resonance in the AsSb/AlGaAs system. Phys. Solid State 2014, 56, 1952–1956. [Google Scholar] [CrossRef]
- Ushanov, V.I.; Chaldyshev, V.V.; Bert, N.A.; Nevedomsky, V.N.; Il’inskaya, N.D.; Lebedeva, N.M.; Preobrazhenskii, V.V.; Putyato, M.A.; Semyagin, B.R. Plasmon resonance in new AsSb–AlGaAs metal–semiconductor metamaterials. Semiconductors 2015, 49, 1587–1591. [Google Scholar] [CrossRef]
- Bert, N.; Ushanov, V.; Snigirev, L.; Kirilenko, D.; Ulin, V.; Yagovkina, M.; Preobrazhenskii, V.; Putyato, M.; Semyagin, B.; Kasatkin, I.; et al. Metal-Semiconductor AsSb-Al0.6Ga0.4As0.97Sb0.03 Metamaterial. Materials 2022, 15, 7597. [Google Scholar] [CrossRef] [PubMed]
- Snigirev, L.; Ushanov, V.; Ivanov, A.; Bert, N.; Kirilenko, D.; Yagovkina, M.; Preobrazhenskii, V.; Putyato, M.; Semyagin, B.; Kasatkin, I.; et al. Structure and optical properties of a composite AsSb-Al0.6Ga0.4As0.97Sb0.03 Metamaterial. Semiconductors 2023, 57, 65–69. [Google Scholar] [CrossRef]
- Silkin, V.M.; Eremeev, S.V.; Ushanov, V.I.; Chaldyshev, V.V. Localized Surface Plasmon Resonance in Metamaterials Composed of As1−zSbz Semimetal Nanoparticles in AlxGa1−xAs1−ySby Semiconductor Matrix. Nanomaterials 2023, 13, 1355. [Google Scholar] [CrossRef] [PubMed]
- Cardona, M.; Greenaway, D.L. Optical Properties and Band Structure of Group IV-VI and Group V Materials. Phys. Rev. 1964, 133, A1685–A1697. [Google Scholar] [CrossRef]
- Lenham, A.P.; Treherne, D.M.; Metcalfe, R.J. Optical Properties of Antimony and Bismuth Crystals. J. Opt. Soc. Am. 1965, 55, 1072–1074. [Google Scholar] [CrossRef]
- Hunderi, O. Optical properties of crystalline and amorphous bismuth films. J. Phys. F Metal Phys. 1975, 5, 2214. [Google Scholar] [CrossRef]
- Werner, W.S.M.; Glantschnig, K.; Ambrosch-Draxl, C. Optical Constants and Inelastic Electron-Scattering Data for 17 Elemental Metals. J. Phys. Chem. Ref. Data 2009, 38, 1013–1092. [Google Scholar] [CrossRef]
- Toudert, J.; Serna, R.; Camps, I.; Wojcik, J.; Mascher, P.; Rebollar, E.; Ezquerra, T.A. Unveiling the Far Infrared-to-Ultraviolet Optical Properties of Bismuth for Applications in Plasmonics and Nanophotonics. J. Phys. Chem. C 2017, 121, 3511–3521. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, L.; Yue, L.; Liang, D.; Chen, X.; Li, Y.; Lu, P.; Shao, J.; Wang, S. Novel Dilute Bismide, Epitaxy, Physical Properties and Device Application. Crystals 2017, 7, 63. [Google Scholar] [CrossRef]
- Richards, R.D.; Bailey, N.J.; Liu, Y.; Rockett, T.B.O.; Mohmad, A.R. GaAsBi: From Molecular Beam Epitaxy Growth to Devices. Phys. Status Solidi 2022, 259, 2100330. [Google Scholar] [CrossRef]
- Mie, G. Beiträge zur Optik trüber Medien, speziell kolloidaler Metallösungen. Ann. Phys. 1908, 330, 377–445. [Google Scholar] [CrossRef]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Adachi, S. GaAs, AlAs and AlxGa1−xAs: Material parameters for use in research and device applications. J. Appl. Phys. 1985, 58, R1–R29. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Koelling, D.D.; Harmon, B.N. A technique for relativistic spin-polarised calculations. J. Phys. C Solid State Phys. 1977, 10, 3107. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comp. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef]
- Becke, A.D.; Johnson, E.R. A simple effective potential for exchange. J. Chem. Phys. 2006, 124, 221101. [Google Scholar] [CrossRef]
- Tran, F.; Blaha, P. Accurate Band Gaps of Semiconductors and Insulators with a Semilocal Exchange-Correlation Potential. Phys. Rev. Lett. 2009, 102, 226401. [Google Scholar] [CrossRef] [PubMed]
- Krukau, A.V.; Vydrov, O.A.; Izmaylov, A.F.; Scuseria, G.E. Influence of the exchange screening parameter on the performance of screened hybrid functionals. J. Chem. Phys. 2006, 125, 224106. [Google Scholar] [CrossRef]
- Gajdoš, M.; Hummer, K.; Kresse, G.; Furthmüller, J.; Bechstedt, F. Linear optical properties in the projector-augmented wave methodology. Phys. Rev. B 2006, 73, 045112. [Google Scholar] [CrossRef]
- Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
- Kawamura, M. FermiSurfer: Fermi-surface viewer providing multiple representation schemes. Comp. Phys. Commun. 2019, 239, 197–203. [Google Scholar] [CrossRef]
- Wyckoff, R.W.G. Crystal Structures; Interscience Publishers: New York, NY, USA, 1963; Volume 1. [Google Scholar]
- Liu, Y.; Allen, R.E. Electronic structure of the semimetals Bi and Sb. Phys. Rev. B 1995, 52, 1566–1577. [Google Scholar] [CrossRef] [PubMed]
- Fu, L.; Kane, C.L. Topological insulators with inversion symmetry. Phys. Rev. B 2007, 76, 045302. [Google Scholar] [CrossRef]
- Zhang, H.J.; Liu, C.X.; Qi, X.L.; Deng, X.Y.; Dai, X.; Zhang, S.C.; Fang, Z. Electronic structures and surface states of the topological insulator Bi1−xSbx. Phys. Rev. B 2009, 80, 085307. [Google Scholar] [CrossRef]
- Aguilera, I.; Friedrich, C.; Blügel, S. Electronic phase transitions of bismuth under strain from relativistic self-consistent GW calculations. Phys. Rev. B 2015, 91, 125129. [Google Scholar] [CrossRef]
- Maltz, M.; Dresselhaus, M.S. Magnetoreflection Studies in Bismuth. Phys. Rev. B 1970, 2, 2877–2887. [Google Scholar] [CrossRef]
- Vecchi, M.P.; Dresselhaus, M.S. Temperature dependence of the band parameters of bismuth. Phys. Rev. B 1974, 10, 771–774. [Google Scholar] [CrossRef]
- Isaacson, R.T.; Williams, G.A. Alfvén-Wave Propagation in Solid-Stae Plasmas. III. Quantum Oscillations of the Fermi Surface of Bismuth. Phys. Rev. 1969, 185, 682–688. [Google Scholar] [CrossRef]
- Brown, R.N.; Mavroides, J.G.; Lax, B. Magnetoreflection in Bismuth. Phys. Rev. 1963, 129, 2055–2061. [Google Scholar] [CrossRef]
- Smith, G.E.; Baraff, G.A.; Rowell, J.M. Effective g Factor of Electrons and Holes in Bismuth. Phys. Rev. 1964, 135, A1118–A1124. [Google Scholar] [CrossRef]
- Ohtsubo, Y.; Perfetti, L.; Goerbig, M.O.; Fèvre, P.L.; Bertran, F.; Taleb-Ibrahimi, A. Non-trivial surface-band dispersion on Bi(111). New J. Phys. 2013, 15, 033041. [Google Scholar] [CrossRef]
- Ohtsubo, Y.; Kimura, S.i. Topological phase transition of single-crystal Bi based on empirical tight-binding calculations. New J. Phys. 2016, 18, 123015. [Google Scholar] [CrossRef]
- Rodriguez-Prieto, A.; Bergara, A.; Silkin, V.M.; Echenique, P.M. Complexity and Fermi surface deformation in compressed lithium. Phys. Rev. B 2006, 74, 172104. [Google Scholar] [CrossRef]
- Errea, I.; Rousseau, B.; Eiguren, A.; Bergara, A. Optical properties of calcium under pressure from first-principles calculations. Phys. Rev. B 2012, 86, 085106. [Google Scholar] [CrossRef]
- Chaldyshev, V.V.; Bert, N.A.; Kolesnikova, A.L.; Romanov, A.E. Stress relaxation scenario for buried quantum dots. Phys. Rev. B 2009, 79, 233304. [Google Scholar] [CrossRef]
- Ioffe Institute NSM Archive: Band Structure and Carrier Concentration of AlGaAs. Available online: https://www.ioffe.ru/SVA/NSM/Semicond/AlGaAs/bandstr.html (accessed on 30 December 2023).
- Jenkins, D.W. Optical constants of AlxGa1−xAs. J. Appl. Phys. 1990, 68, 1848–1853. [Google Scholar] [CrossRef]
- Djurišić, A.B.; Rakić, A.D.; Kwok, P.C.K.; Li, E.H.; Majewski, M.L.; Elazar, J.M. Modeling the optical constants of AlxGa1−xAs alloys. J. Appl. Phys. 1999, 86, 445–451. [Google Scholar] [CrossRef]
- Lin, C.H.; Meese, J. Optical properties of bulk AlxGa1−xAs. J. Appl. Phys. 1993, 74, 6341–6348. [Google Scholar] [CrossRef]
- Tanguy, C. Optical dispersion by Wannier excitons. Phys. Rev. Lett. 1995, 75, 4090. [Google Scholar] [CrossRef] [PubMed]
- Adachi, S. Optical dispersion relations for GaP, GaAs, GaSb, InP, InAs, InSb, AlxGa1−xAs, and In1−xGaxAsyP1−y. J. Appl. Phys. 1989, 66, 6030–6040. [Google Scholar] [CrossRef]
- Scholl, J.A.; Koh, A.L.; Dionne, J.A. Quantum plasmon resonances of individual metallic nanoparticles. Nature 2012, 483, 421–427. [Google Scholar] [CrossRef]
- Wang, Y.W.; Kim, J.S.; Kim, G.H.; Kim, K.S. Quantum size effects in the volume plasmon excitation of bismuth nanoparticles investigated by electron energy loss spectroscopy. Appl. Phys. Lett. 2006, 88, 143106. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ushanov, V.I.; Eremeev, S.V.; Silkin, V.M.; Chaldyshev, V.V. Plasmon Resonance in a System of Bi Nanoparticles Embedded into (Al,Ga)As Matrix. Nanomaterials 2024, 14, 109. https://doi.org/10.3390/nano14010109
Ushanov VI, Eremeev SV, Silkin VM, Chaldyshev VV. Plasmon Resonance in a System of Bi Nanoparticles Embedded into (Al,Ga)As Matrix. Nanomaterials. 2024; 14(1):109. https://doi.org/10.3390/nano14010109
Chicago/Turabian StyleUshanov, Vitalii I., Sergey V. Eremeev, Vyacheslav M. Silkin, and Vladimir V. Chaldyshev. 2024. "Plasmon Resonance in a System of Bi Nanoparticles Embedded into (Al,Ga)As Matrix" Nanomaterials 14, no. 1: 109. https://doi.org/10.3390/nano14010109
APA StyleUshanov, V. I., Eremeev, S. V., Silkin, V. M., & Chaldyshev, V. V. (2024). Plasmon Resonance in a System of Bi Nanoparticles Embedded into (Al,Ga)As Matrix. Nanomaterials, 14(1), 109. https://doi.org/10.3390/nano14010109





