Plasmon Hybridizations in Compound Nanorod–Nanohole Arrays
Abstract
:1. Introduction
2. Materials and Methods
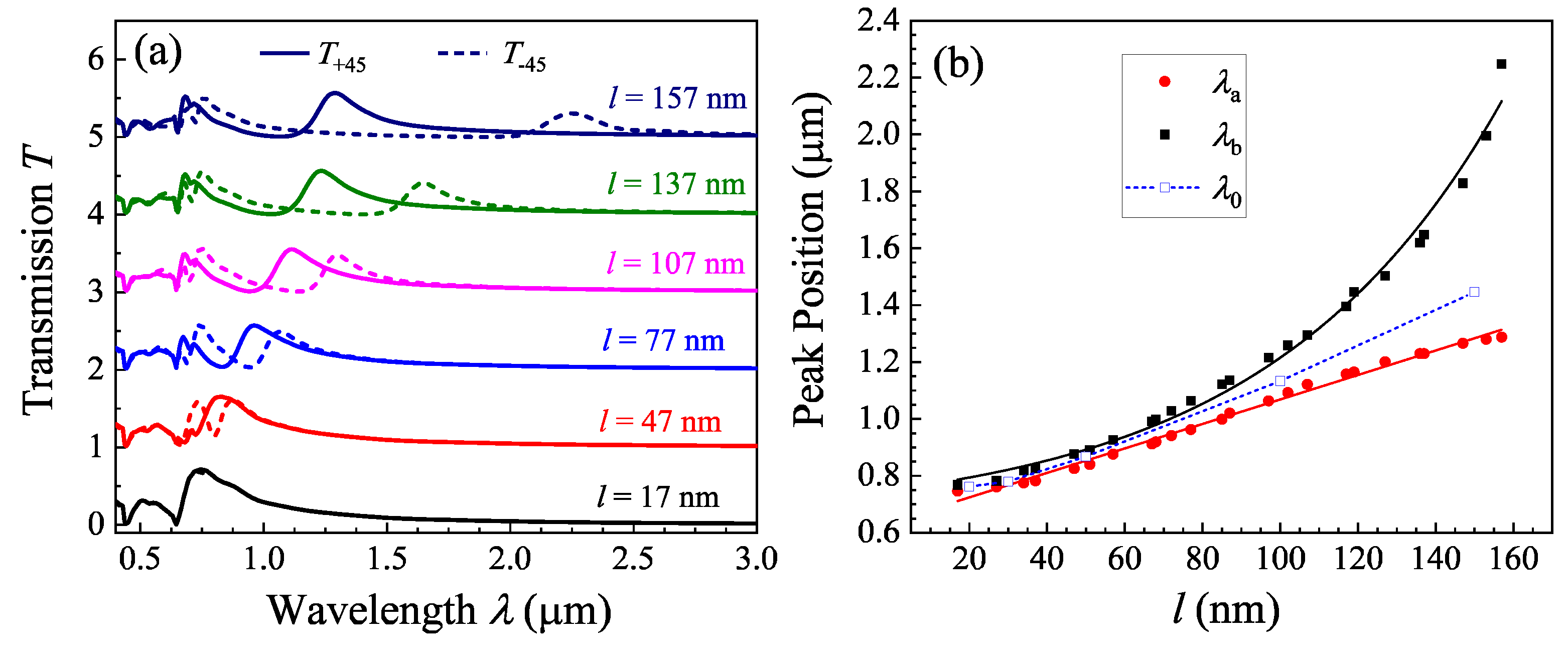
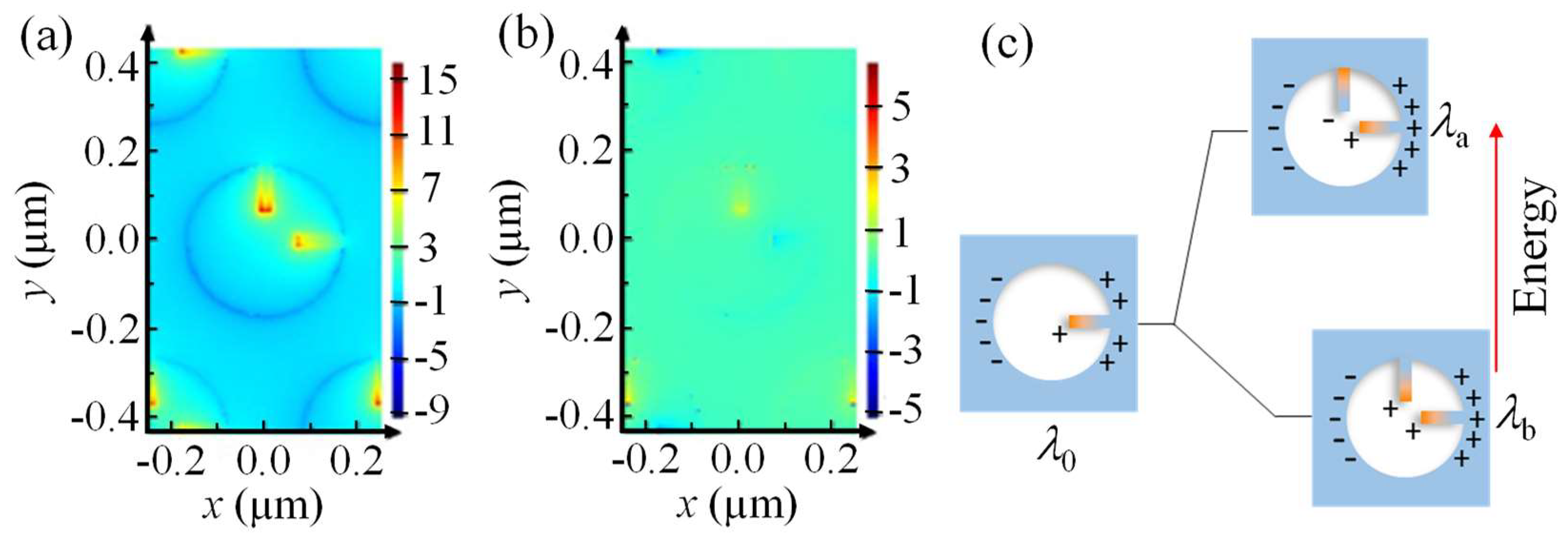
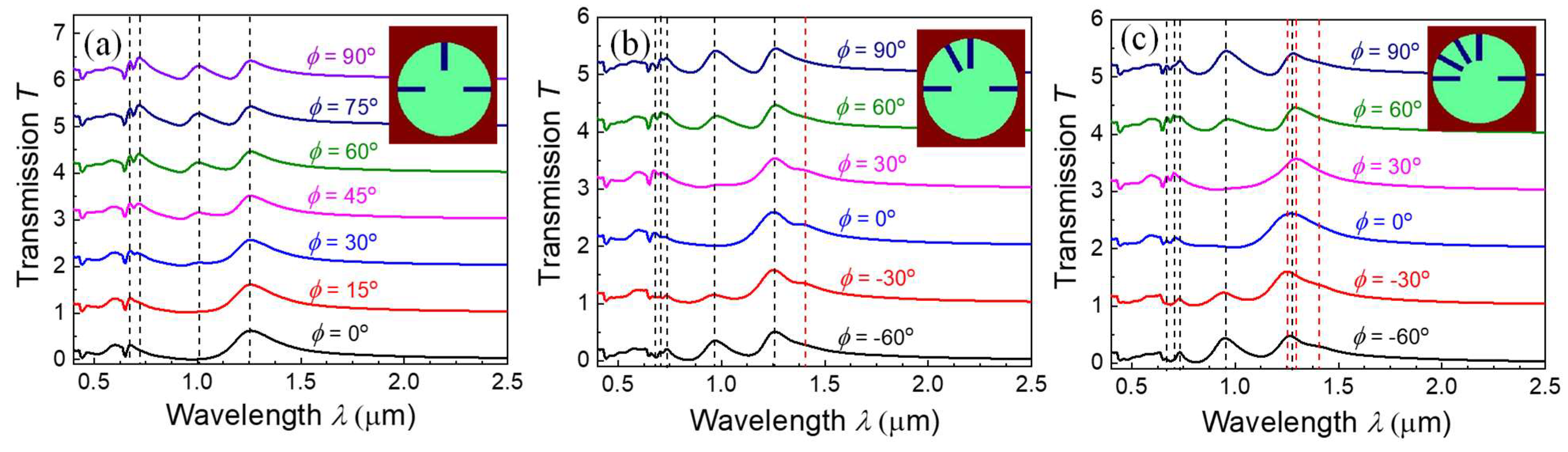
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ebbesen, T.W.; Lezec, H.J.; Ghaemi, H.F.; Thio, T.; Wolff, P.A. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667–669. [Google Scholar] [CrossRef]
- Gordon, R.; Brolo, A.G.; Sinton, D.; Kavanagh, K.L. Resonant optical transmission through hole-arrays in metal films: Physics and applications. Laser Photonics Rev. 2010, 4, 311–335. [Google Scholar] [CrossRef]
- García de Abajo, F.J. Colloquium: Light scattering by particle and hole arrays. Rev. Mod. Phys. 2007, 79, 1267–1290. [Google Scholar] [CrossRef] [Green Version]
- Grupp, D.E.; Lezec, H.J.; Thio, T.; Ebbesen, T.W. Beyond the Bethe Limit: Tunable Enhanced Light Transmission Through a Single Sub-Wavelength Aperture. Adv. Mater. 1999, 11, 860–862. [Google Scholar] [CrossRef]
- Thio, T.; Pellerin, K.M.; Linke, R.A.; Lezec, H.J.; Ebbesen, T.W. Enhanced light transmission through a single subwavelength aperture. Opt. Lett. 2001, 26, 1972–1974. [Google Scholar] [CrossRef] [Green Version]
- Lezec, H.J.; Degiron, A.; Devaux, E.; Linke, R.A.; Martin-Moreno, L.; Garcia-Vidal, F.J.; Ebbesen, T.W. Beaming Light from a Subwavelength Aperture. Science 2002, 297, 820–822. [Google Scholar] [CrossRef]
- Baida, F.I.; Van Labeke, D. Light transmission by subwavelength annular aperture arrays in metallic films. Opt. Commun. 2002, 209, 17–22. [Google Scholar] [CrossRef]
- Fan, W.; Zhang, S.; Minhas, B.; Malloy, K.J.; Brueck, S.R.J. Enhanced Infrared Transmission through Subwavelength Coaxial Metallic Arrays. Phys. Rev. Lett. 2005, 94, 033902. [Google Scholar] [CrossRef]
- Lockyear, M.J.; Hibbins, A.P.; Sambles, J.R.; Lawrence, C.R. Microwave Transmission through a Single Subwavelength Annular Aperture in a Metal Plate. Phys. Rev. Lett. 2005, 94, 193902. [Google Scholar] [CrossRef] [Green Version]
- Salvi, J.; Roussey, M.; Baida, F.I.; Bernal, M.P.; Mussot, A.; Sylvestre, T.; Maillotte, H.; Van Labeke, D.; Perentes, A.; Utke, I.; et al. Annular aperture arrays: Study in the visible region of the electromagnetic spectrum. Opt. Lett. 2005, 30, 1611–1613. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Du, B.; Yang, Y.; Zhang, Y.; Jia, P.; Ebendorff-Heidepriem, H.; Ruan, Y.; Yang, D. Enhancement of extraordinary optical transmission and sensing performance through coupling between metal nanohole and nanoparticle arrays. J. Phys. D Appl. Phys. 2019, 52, 275201. [Google Scholar] [CrossRef]
- Ni, H.; Wang, M.; Shen, T.; Zhou, J. Self-Assembled Large-Area Annular Cavity Arrays with Tunable Cylindrical Surface Plasmons for Sensing. ACS Nano 2015, 9, 1913–1925. [Google Scholar] [CrossRef]
- Weiler, M.; Quint, S.B.; Klenk, S.; Pacholski, C. Bottom-up fabrication of nanohole arrays loaded with gold nanoparticles: Extraordinary plasmonic sensors. Chem. Commun. 2014, 50, 15419–15422. [Google Scholar] [CrossRef] [PubMed]
- Yoo, D.; Mohr, D.A.; Vidal-Codina, F.; John-Herpin, A.; Jo, M.; Kim, S.; Matson, J.; Caldwell, J.D.; Jeon, H.; Nguyen, N.-C.; et al. High-Contrast Infrared Absorption Spectroscopy via Mass-Produced Coaxial Zero-Mode Resonators with Sub-10 nm Gaps. Nano Lett. 2018, 18, 1930–1936. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cai, H.; Meng, Q.; Zhao, H.; Li, M.; Dai, Y.; Lin, Y.; Ding, H.; Pan, N.; Tian, Y.; Luo, Y.; et al. High-Throughput Fabrication of Ultradense Annular Nanogap Arrays for Plasmon-Enhanced Spectroscopy. ACS Appl. Mater. Interfaces 2018, 10, 20189–20195. [Google Scholar] [CrossRef]
- Zhu, A.; Qian, Q.; Yan, Y.; Hu, J.; Zhao, X.; Wang, C. Ultrathin plasmonic quarter waveplate using broken rectangular annular metasurface. Opt. Laser Technol. 2017, 92, 120–125. [Google Scholar] [CrossRef]
- Wang, Y.; Luong, H.; Zhang, Z.; Zhao, Y. Coupling between plasmonic nanohole array and nanorod array: The emerging of a new extraordinary optical transmission mode and epsilon-near-zero property. J. Phys. D Appl. Phys. 2020, 53, 275202. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, Z.; Zhao, Y. The effect of nanorod position on the plasmonic properties of the complex nanorod in nanohole arrays. J. Phys. D Appl. Phys. 2021, 54, 155201. [Google Scholar] [CrossRef]
- Wang, Y.; Ao, S.; Yang, F.; Zhang, Z.; Zhao, Y. Coupling between Surface Plasmon Modes of Single-Layer Complex Silver Nanohole Arrays and Enhancing Index Sensing. ACS Appl. Nano Mater. 2022, 5, 9761–9770. [Google Scholar] [CrossRef]
- Wang, Y.; Chong, H.B.; Zhang, Z.; Zhao, Y. Large-Area Fabrication of Complex Nanohole Arrays with Highly Tunable Plasmonic Properties. ACS Appl. Mater. Interfaces 2020, 12, 37435–37443. [Google Scholar] [CrossRef]
- Wang, Y.; Choi, I.; Zhang, K.; Yang, Y.; Ao, S.; Xue, X.; Fu, W.; Zhang, Z.; Zhao, Y. Highly Conductive Nanograting–Nanohole Structures with Tunable and Dual-Band Spectral Transparency. ACS Appl. Electron. Mater. 2021, 3, 3489–3500. [Google Scholar] [CrossRef]
- Prodan, E.; Radloff, C.; Halas, N.J.; Nordlander, P. A Hybridization Model for the Plasmon Response of Complex Nanostructures. Science 2003, 302, 419–422. [Google Scholar] [CrossRef]
- Yanik, A.A.; Adato, R.; Erramilli, S.; Altug, H. Hybridized nanocavities as single-polarized plasmonic antennas. Opt. Express 2009, 17, 20900–20910. [Google Scholar] [CrossRef] [Green Version]
- Larson, S.; Luong, H.; Song, C.; Zhao, Y. Dipole Radiation-Induced Extraordinary Optical Transmission for Silver Nanorod-Covered Silver Nanohole Arrays. J. Phys. Chem. C 2019, 123, 5634–5641. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: Cambridge, MA, USA, 1991. [Google Scholar]
- Li, Y. Plasmonic Optics: Theory and Applications; SPIE Press: Bellingham, WA, USA, 2017. [Google Scholar]
- Li, Z.-B.; Zhou, W.-Y.; Kong, X.-T.; Tian, J.-G. Polarization dependence and independence of near-field enhancement through a subwavelength circle hole. Opt. Express 2010, 18, 5854–5860. [Google Scholar] [CrossRef] [PubMed]
- Hill, R.T.; Mock, J.J.; Hucknall, A.; Wolter, S.D.; Jokerst, N.M.; Smith, D.R.; Chilkoti, A. Plasmon ruler with angstrom length resolution. ACS Nano 2012, 6, 9237–9246. [Google Scholar] [CrossRef] [Green Version]
- Yoon, J.H.; Yoon, S. Probing interfacial interactions using core-satellite plasmon rulers. Langmuir 2013, 29, 14772–14778. [Google Scholar] [CrossRef] [PubMed]
- Huang, F.M.; Wilding, D.; Speed, J.D.; Russell, A.E.; Bartlett, P.N.; Baumberg, J.J. Dressing plasmons in particle-in-cavity architectures. Nano Lett. 2011, 11, 1221–1226. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Razavi, S.; Zhao, Y. Plasmon Hybridizations in Compound Nanorod–Nanohole Arrays. Nanomaterials 2023, 13, 2135. https://doi.org/10.3390/nano13142135
Razavi S, Zhao Y. Plasmon Hybridizations in Compound Nanorod–Nanohole Arrays. Nanomaterials. 2023; 13(14):2135. https://doi.org/10.3390/nano13142135
Chicago/Turabian StyleRazavi, Shahab, and Yiping Zhao. 2023. "Plasmon Hybridizations in Compound Nanorod–Nanohole Arrays" Nanomaterials 13, no. 14: 2135. https://doi.org/10.3390/nano13142135
APA StyleRazavi, S., & Zhao, Y. (2023). Plasmon Hybridizations in Compound Nanorod–Nanohole Arrays. Nanomaterials, 13(14), 2135. https://doi.org/10.3390/nano13142135






