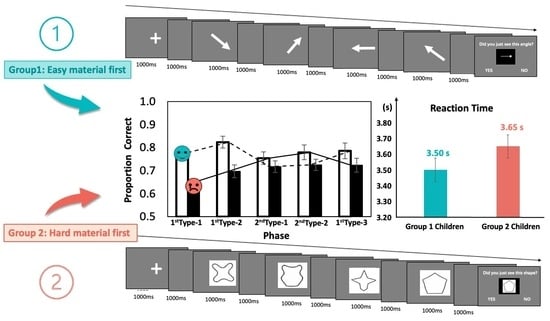
Generalization of Skill for a Working Memory Recognition Procedure in Children: The Benefit of Starting with Easy Materials
Abstract
1. Introduction
2. Working Memory and Task Familiarity
3. The Present Study
4. Material and Methods
4.1. Participants
4.2. Design
4.3. Stimuli and Procedure
4.4. Statistical Analysis
5. Results
5.1. Proportion of Correct Recognition
5.2. Reaction Times
6. Discussion
7. Limitations and Prospects for Research
8. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alloway, Tracy Packiam. 2009. Working memory, but not IQ, predicts subsequent learning in children with learning difficulties. European Journal of Psychological Assessment 25: 92–98. [Google Scholar] [CrossRef]
- Baddeley, Alan. 2012. Working memory: Theories, models, and controversies. Annual review of psychology 63: 1–29. [Google Scholar] [CrossRef] [PubMed]
- Barnett, Susan. M., and Stephen J. Ceci. 2002. When and where do we apply what we learn?: A taxonomy for far transfer. Psychological Bulletin 128: 612. [Google Scholar] [CrossRef]
- Buttle, Heather, and Jane E. Raymond. 2003. High familiarity enhances visual change detection for face stimuli. Perception and Psychophysics 65: 1296–306. [Google Scholar] [CrossRef]
- Chase, William G., and K Anders Ericsson. 1982. Skill and working memory. In Psychology of Learning and Motivation. Cambridge: Academic Press, vol. 16, pp. 1–58. [Google Scholar]
- Chen, Diyu, Hing Yee Eng, and Yuhong Jiang. 2006. Visual working memory for trained and novel polygons. Visual Cognition 14: 37–54. [Google Scholar] [CrossRef]
- Corbin, Lucie, and Valérie Camos. 2011. Improvement of working memory performance by training is not transferable. Europe’s Journal of Psychology 7: 279–94. [Google Scholar] [CrossRef]
- Cowan, Nelson. 2001. The magical number 4 in short-term memory: A reconsideration of mental storage capacity. Behavioral and Brain Sciences 24: 87–114. [Google Scholar] [CrossRef]
- Cowan, Nelson. 2014. Working memory underpins cognitive development, learning, and education. Educational Psychology Review 26: 197–223. [Google Scholar] [CrossRef]
- Cowan, Nelson, Angela M. AuBuchon, Amanda L. Gilchrist, Timothy J. Ricker, and J. Scott Saults. 2011. Age differences in visual working memory capacity: Not based on encoding limitations. Developmental Science 14: 1066–74. [Google Scholar] [CrossRef] [PubMed]
- Cowan, Nelson, Cristy Cartwright, Carrie Winterowd, and Molly Sherk. 1987. An adult model of preschool children’s speech memory. Memory and Cognition 15: 511–17. [Google Scholar] [CrossRef] [PubMed]
- Cowan, Nelson, Emily M. Elliott, J. Scott Saults, Candice C. Morey, Sam Mattox, Anna Hismjatullina, and Andrew R.A. Conway. 2005. On the capacity of attention: Its estimation and its role in working memory and cognitive aptitudes. Cognitive Psychology 51: 42–100. [Google Scholar] [CrossRef] [PubMed]
- Curby, Kim M., and Isabel Gauthier. 2007. A visual short-term memory advantage for faces. Psychonomic Bulletin and Review 14: 620–28. [Google Scholar] [CrossRef]
- Dahlin, Karin I. 2011. Effects of working memory training on reading in children with special needs. Reading and Writing 24: 479–91. [Google Scholar] [CrossRef]
- Daneman, Meredyth, and Patricia A. Carpenter. 1980. Individual differences in working memory and reading. Journal of Verbal Learning and Verbal Behavior 19: 450–66. [Google Scholar] [CrossRef]
- Daneman, Meredy, and Philip M. Merikle. 1996. Working memory and language comprehension: A Meta Analysis. Psychonomic Bulletin and Review 3: 422–433. [Google Scholar] [CrossRef]
- Forsberg, Alicia, Blume Christopher, and Nelson Cowan. 2021. The development of metacognitive accuracy in working memory across childhood. Developmental Psychology 57: 1297–317. [Google Scholar] [CrossRef]
- Forsberg, Alicia, Dominic Guitard, Eryn J. Adams, Duangporn Pattanakul, and Nelson Cowan. 2022. Children’s long-term retention is directly constrained by their working memory capacity limitations. Developmental Science 25: e13164. [Google Scholar] [CrossRef]
- Henry, Lucy A., David J. Messer, and Gilly Nash. 2014. Testing for near and far transfer effects with a short, face-to-face adaptive working memory training intervention in typical children. Infant and Child Development 23: 84–103. [Google Scholar] [CrossRef]
- Hoffmann, Janina A., Lena Bareuther, Roger Schmidt, and Christian Dettmers. 2020. The relation between memory and decision-making in multiple sclerosis patients. Multiple Sclerosis and Related Disorders 37: 101433. [Google Scholar] [CrossRef]
- Holmes, Joni, Susan E. Gathercole, and Darren L. Dunning. 2009. Adaptive training leads to sustained enhancement of poor working memory in children. Developmental Science 12: F9–F15. [Google Scholar]
- Jaeggi, Susanne M., Martin Buschkuehl, John Jonides, and Walter J. Perrig. 2008. Improving fluid intelligence with training on working memory. Proceedings of the National Academy of Sciences 105: 6829–33. [Google Scholar] [CrossRef]
- Kane, Michael J., David Z. Hambrick, and Andrew R. Conway. 2005. Working memory capacity and fluid intelligence are strongly related constructs: Comment on Ackerman, Beier, and Boyle. Psychological Bulletin 131: 66–71. [Google Scholar] [CrossRef]
- Klingberg, Torkel. 2010. Training and plasticity of working memory. Trends in Cognitive Sciences 14: 317–24. [Google Scholar] [CrossRef] [PubMed]
- Klingberg, Torkel, Hans Forssberg, and Helena Westerberg. 2002. Training of working memory in children with ADHD. Journal of Clinical and Experimental Neuropsychology 24: 781–91. [Google Scholar] [CrossRef] [PubMed]
- Kyllonen, Patrick C., and Raymond E. Christal. 1990. Reasoning ability is (little more than) working-memory capacity?! Intelligence 14: 389–433. [Google Scholar] [CrossRef]
- Li, Aedan Y., Jackson C. Liang, Andy C. Lee, and Morgan D. Barense. 2020. The validated circular shape space: Quantifying the visual similarity of shape. Journal of Experimental Psychology: General 149: 949. [Google Scholar] [CrossRef]
- Ly, Alexander, Akash Raj, Alexander Etz, Maarten Marsman, Quentin F. Gronau, and Eric Jan Wagenmakers. 2018. Bayesian reanalyses from summary statistics: A guide for academic consumers. Advances in Methods and Practices in Psychological Science 1: 367–74. [Google Scholar] [CrossRef]
- Melby-Lervåg, Monica, and Charles Hulme. 2013. Is working memory training effective? A meta-analytic review. Developmental Psychology 49: 270. [Google Scholar] [CrossRef]
- Melby-Lervåg, Monica, Thomas S. Redick, and Charles Hulme. 2016. Working memory training does not improve performance on measures of intelligence or other measures of “far transfer” evidence from a meta-analytic review. Perspectives on Psychological Science 11: 512–34. [Google Scholar] [CrossRef]
- Morrison, Alexandra B., and Jason M. Chein. 2011. Does working memory training work? The promise and challenges of enhancing cognition by training working memory. Psychonomic Bulletin and Review 18: 46–60. [Google Scholar] [CrossRef]
- Oberauer, Klaus, Ralf Schulze, Oliver Wilhelm, and Heinz Martin Süß. 2005. Working memory and intelligence—Their correlation and their relation: Comment on Ackerman, Beier, and Boyle. Psychological Bulletin 131: 61–65. [Google Scholar] [CrossRef]
- Peng, Peng, and Douglas Fuchs. 2017. A randomized control trial of working memory training with and without strategy instruction: Effects on young children’s working memory and comprehension. Journal of Learning Disabilities 50: 62–80. [Google Scholar] [CrossRef]
- Peng, Peng, Marcia Barnes, Cuicui Wang, Wei Wang, Shan Li, H. Lee Swanson, William Dardick, and Sha Tao. 2018. A meta-analysis on the relation between reading and working memory. Psychological Bulletin 144: 48–76. [Google Scholar] [CrossRef] [PubMed]
- Rouder, Jeffrey N., Richard D. Morey, Paul L. Speckman, and Jordan M. Province. 2012. Default Bayes factors for ANOVA designs. Journal of Mathematical Psychology 56: 356–74. [Google Scholar] [CrossRef]
- Rudebeck, Sarah R., Daniel Bor, Angharad Ormond, Jill X. O’Reilly, and Andy C. Lee. 2012. A potential spatial working memory training task to improve both episodic memory and fluid intelligence. PLoS ONE 7: e50431. [Google Scholar] [CrossRef] [PubMed]
- Shah, Priti, and Akira Miyake. 1996. The separability of working memory resources for spatial thinking and language processing: An individual differences approach. Journal of Experimental Psychology: General 125: 4. [Google Scholar] [CrossRef] [PubMed]
- Shipstead, Zach, Thomas S. Redick, and Randall W. Engle. 2010. Does working memory training generalize? Psychologica Belgica 50: 245–76. [Google Scholar] [CrossRef]
- Shipstead, Zach, Thomas S. Redick, and Randall W. Engle. 2012. Is working memory training effective? Psychological Bulletin 138: 628. [Google Scholar] [CrossRef]
- Shute, Valerie J. 1991. Who is likely to acquire programming skills? Journal of Educational Computing Research 7: 1–24. [Google Scholar] [CrossRef]
- Stephenson, Clayton L., and Diane F. Halpern. 2013. Improved matrix reasoning is limited to training on tasks with a visuospatial component. Intelligence 41: 341–57. [Google Scholar] [CrossRef]
- Sternberg, Robert J. 2008. Increasing fluid intelligence is possible after all. Proceedings of the National Academy of Sciences 105: 6791–92. [Google Scholar] [CrossRef]
- Swanson, H. Lee, and Margaret Beebe-Frankenberger. 2004. The relationship between working memory and mathematical problem solving in children at risk and not at risk for serious math difficulties. Journal of Educational Psychology 96: 471–91. [Google Scholar] [CrossRef]
- Swanson, H. Lee, and Olga Jerman. 2006. Math disabilities: A selective meta-analysis of the literature. Review of Educational Research 76: 249–74. [Google Scholar] [CrossRef]
- Swanson, H. Lee, Xinhua Zheng, and Olga Jerman. 2009. Working memory, short-term memory, and reading disabilities: A selective meta-analysis of the literature. Journal of Learning Disabilitie 42: 260–87. [Google Scholar] [CrossRef] [PubMed]
- Turner, Marilyn L., and Randall W. Engle. 1989. Is working memory capacity task dependent? Journal of Memory and Language 28: 127–54. [Google Scholar] [CrossRef]
- Von Bastian, Claudia C., and Klaus Oberauer. 2013. Distinct transfer effects of training different facets of working memory capacity. Journal of Memory and Language 69: 36–58. [Google Scholar] [CrossRef]
- Von Bastian, Claudia C., and Klaus Oberauer. 2014. Effects and mechanisms of working memory training: A review. Psychological Research 78: 803–20. [Google Scholar] [CrossRef] [PubMed]

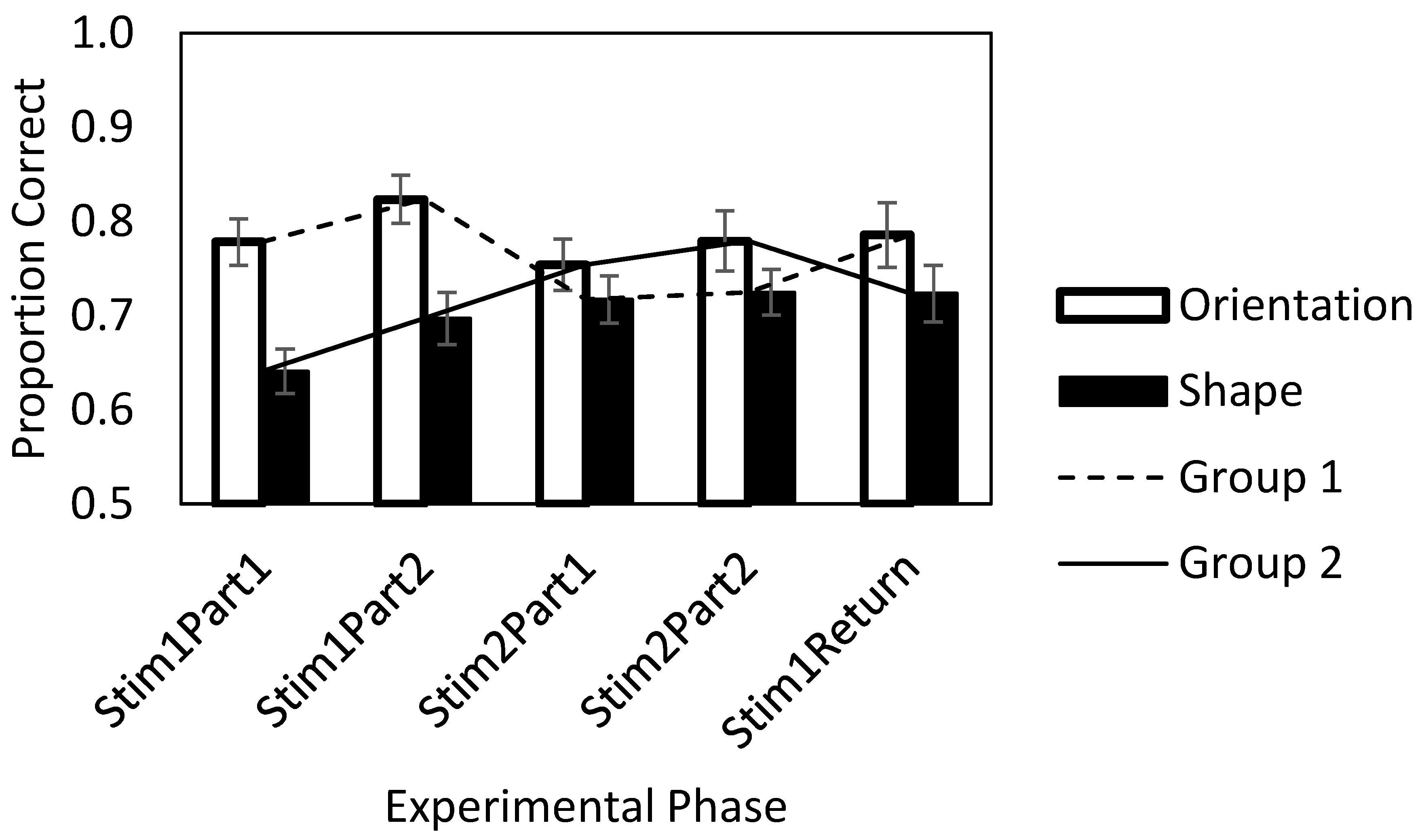
| Group | N | Phase | Stimulus | Proportion Correct | Reaction Time | ||
|---|---|---|---|---|---|---|---|
| Mean | SD | Mean | SD | ||||
| 1 | 35 | Stim1Part1 | Orientation | 0.78 | 0.42 | 3.47 | 1.75 |
| Stim1Part2 | Orientation | 0.82 | 0.38 | 3.37 | 1.73 | ||
| Stim2Part1 | Shape | 0.72 | 0.45 | 3.57 | 1.80 | ||
| Stim2Part2 | Shape | 0.73 | 0.45 | 3.55 | 1.86 | ||
| Stim1Return | Orientation | 0.79 | 0.41 | 3.56 | 2.13 | ||
| Average | 0.77 | 0.42 | 3.50 | 1.86 | |||
| 2 | 35 | Stim1Part1 | Shape | 0.64 | 0.48 | 3.57 | 2.00 |
| Stim1Part2 | Shape | 0.70 | 0.46 | 3.45 | 1.76 | ||
| Stim2Part1 | Orientation | 0.75 | 0.43 | 3.83 | 1.88 | ||
| Stim2Part2 | Orientation | 0.78 | 0.42 | 3.79 | 1.92 | ||
| Stim1Return | Shape | 0.72 | 0.45 | 3.62 | 1.93 | ||
| Average | 0.72 | 0.45 | 3.65 | 1.90 | |||
| Grand average | 0.74 | 0.44 | 3.58 | 1.88 | |||
| Model | Factors | Predictor | df | F | Cohen’s f | p | BFincl of Model | |
|---|---|---|---|---|---|---|---|---|
| 1 | Phase | Phase | 4 | 276 | 1.97 | 0.046 | 0.10 | 0.01 |
| 2 | Group | Group | 1 | 6920 | 20.65 | 0.055 | <0.001 | 796.57 |
| 3 | Phase + Group | Phase | 4 | 276 | 1.97 | 0.046 | 0.10 | 8.67 |
| Group | 1 | 64 | 3.55 | 0.071 | 0.06 | |||
| 4 | Interaction | Interaction | 8 | 272 | 6.38 | 0.110 | <0.001 | 268,376,003 |
| 5 | Phase + interaction | Phase | 4 | 272 | 2.24 | 0.046 | 0.07 | 3,036,658 |
| Interaction | 4 | 272 | 10.51 | 0.100 | <0.001 | |||
| 6 | Group + interaction | Group | 1 | 60 | 2.39 | 0.059 | 0.13 | 238,534,312,357 |
| Interaction | 8 | 272 | 6.38 | 0.110 | <0.001 | |||
| 7 | Phase + Group + interaction | Phase | 4 | 272 | 2.24 | 0.046 | 0.07 | 2,686,584,969 |
| Group | 1 | 60 | 3.49 | 0.071 | 0.07 | |||
| Interaction | 4 | 272 | 10.51 | 0.100 | <0.001 |
| Factor | Method | Formula | BFincl |
|---|---|---|---|
| Phase | 1 | Phase/null model | 0.01 |
| 2 | (Phase + Group)/Group | 0.01 | |
| 3 | All effects/(Group + interaction) | 0.01 | |
| Group | 1 | Group/null model | 796.57 |
| 2 | (Group + interaction)/interaction | 888.81 | |
| 3 | All effects/(Phase + interaction) | 884.72 | |
| Interaction | 1 | Interaction/null model | 268,376,003 |
| 2 | (Group + interaction)/Group | 299,453,294 | |
| 3 | All effects/(Group + Phase) | 310,050,198 |
| G1P2 | G1P3 | G1P5 | G1P6 | G1P7 | G2P2 | G2P3 | G2P5 | G2P6 | |
|---|---|---|---|---|---|---|---|---|---|
| G1P2 | - | ||||||||
| G1P3 | 0.59 | - | |||||||
| G1P5 | 1.33 | 8263.23 | - | ||||||
| G1P6 | 0.34 | 1201.70 | 0.05 | - | |||||
| G1P7 | 0.05 | 0.21 | 6.18 | 1.79 | - | ||||
| G2P2 | - | - | 3.44 | - | - | - | |||
| G2P3 | - | - | - | 0.14 | - | 0.44 | - | ||
| G2P5 | 0.09 | - | - | - | - | 2103.25 | 0.84 | - | |
| G2P6 | - | 0.50 | - | - | - | 771,403.70 | 31.13 | 0.08 | - |
| G2P7 | - | - | - | - | - | 12.39 | 0.09 | 0.09 | 0.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bao, C.; Cowan, N. Generalization of Skill for a Working Memory Recognition Procedure in Children: The Benefit of Starting with Easy Materials. J. Intell. 2023, 11, 56. https://doi.org/10.3390/jintelligence11030056
Bao C, Cowan N. Generalization of Skill for a Working Memory Recognition Procedure in Children: The Benefit of Starting with Easy Materials. Journal of Intelligence. 2023; 11(3):56. https://doi.org/10.3390/jintelligence11030056
Chicago/Turabian StyleBao, Chenye, and Nelson Cowan. 2023. "Generalization of Skill for a Working Memory Recognition Procedure in Children: The Benefit of Starting with Easy Materials" Journal of Intelligence 11, no. 3: 56. https://doi.org/10.3390/jintelligence11030056
APA StyleBao, C., & Cowan, N. (2023). Generalization of Skill for a Working Memory Recognition Procedure in Children: The Benefit of Starting with Easy Materials. Journal of Intelligence, 11(3), 56. https://doi.org/10.3390/jintelligence11030056