Figure 1.
Chemical reactions during the process under study.
Figure 1.
Chemical reactions during the process under study.
Figure 2.
Block diagram of an experimental facility for carbamate thermolysis.
Figure 2.
Block diagram of an experimental facility for carbamate thermolysis.
Figure 3.
Diagram of a laboratory facility for carbamate thermolysis.
Figure 3.
Diagram of a laboratory facility for carbamate thermolysis.
Figure 4.
Geometric model of gas flow: 1—inlet; 2—reaction site; 3—heating side; 4—outlet.
Figure 4.
Geometric model of gas flow: 1—inlet; 2—reaction site; 3—heating side; 4—outlet.
Figure 5.
Geometrical model mesh.
Figure 5.
Geometrical model mesh.
Figure 6.
Comparison of the experimentally obtained temperature profiles along the reactor length at different temperature conditions and carrier gas flow rates with the profiles obtained during the calculation in COMSOL Multiphysics. Continuous lines show the calculated temperature values and experimental points (square points are taken at the carrier gas flow rate of 1.5 L/min, and round points at 3 L/min).
Figure 6.
Comparison of the experimentally obtained temperature profiles along the reactor length at different temperature conditions and carrier gas flow rates with the profiles obtained during the calculation in COMSOL Multiphysics. Continuous lines show the calculated temperature values and experimental points (square points are taken at the carrier gas flow rate of 1.5 L/min, and round points at 3 L/min).
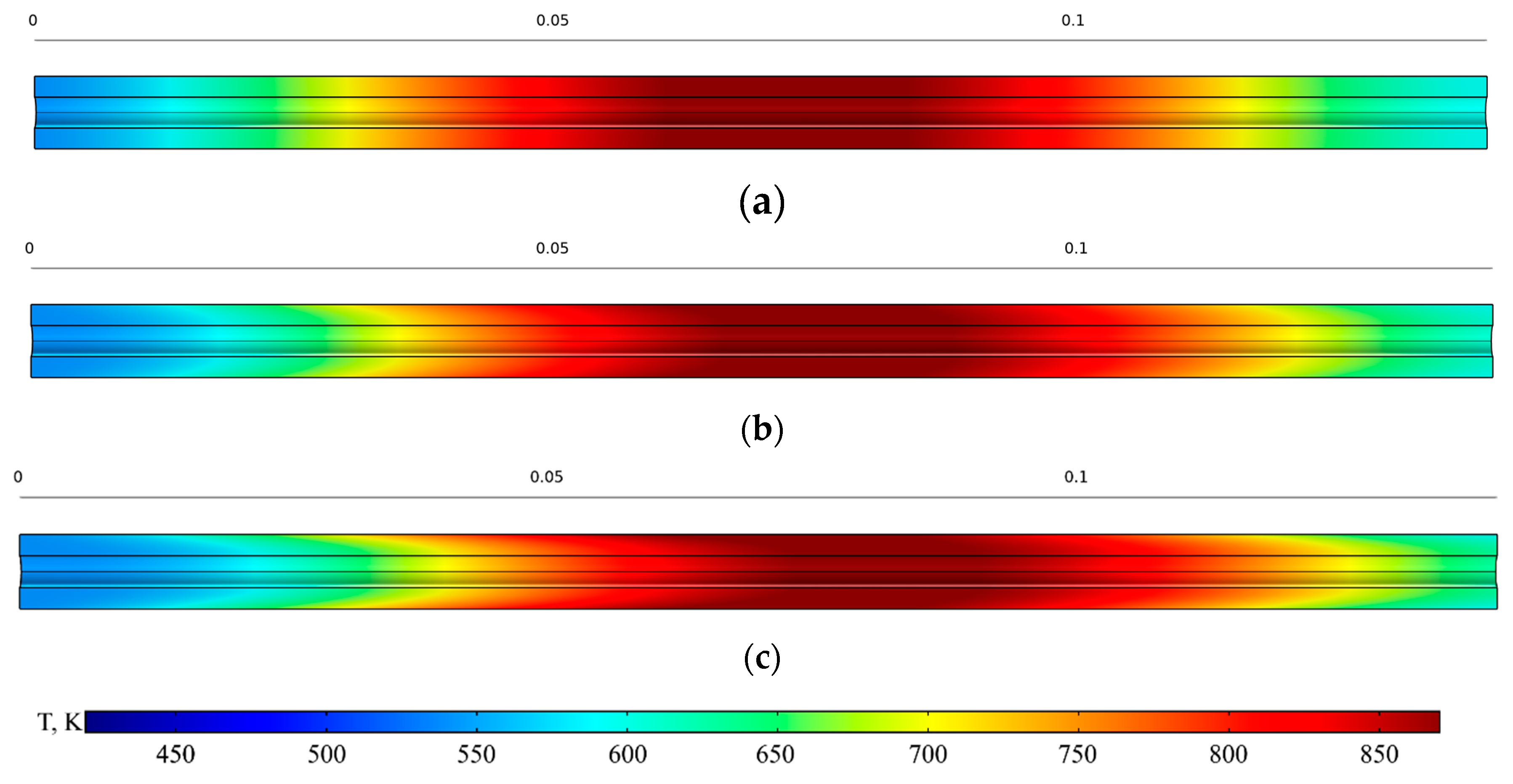
Figure 7.
Temperature profile along the length of the reactor at a temperature of 600 °C and carrier gas flow rates of 0.05 (a), 1.5 (b), and 3.0 (c) L/min.
Figure 7.
Temperature profile along the length of the reactor at a temperature of 600 °C and carrier gas flow rates of 0.05 (a), 1.5 (b), and 3.0 (c) L/min.
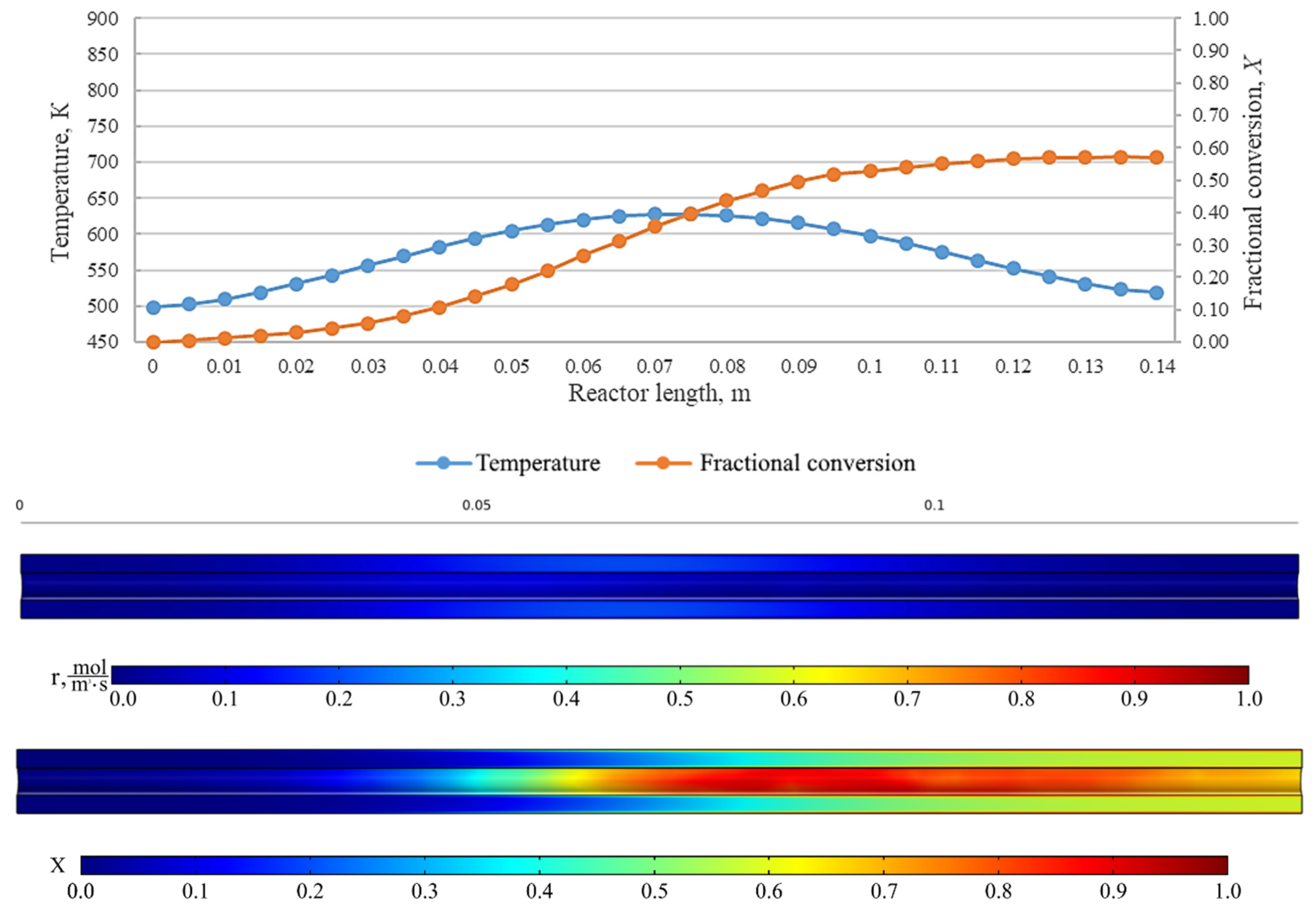
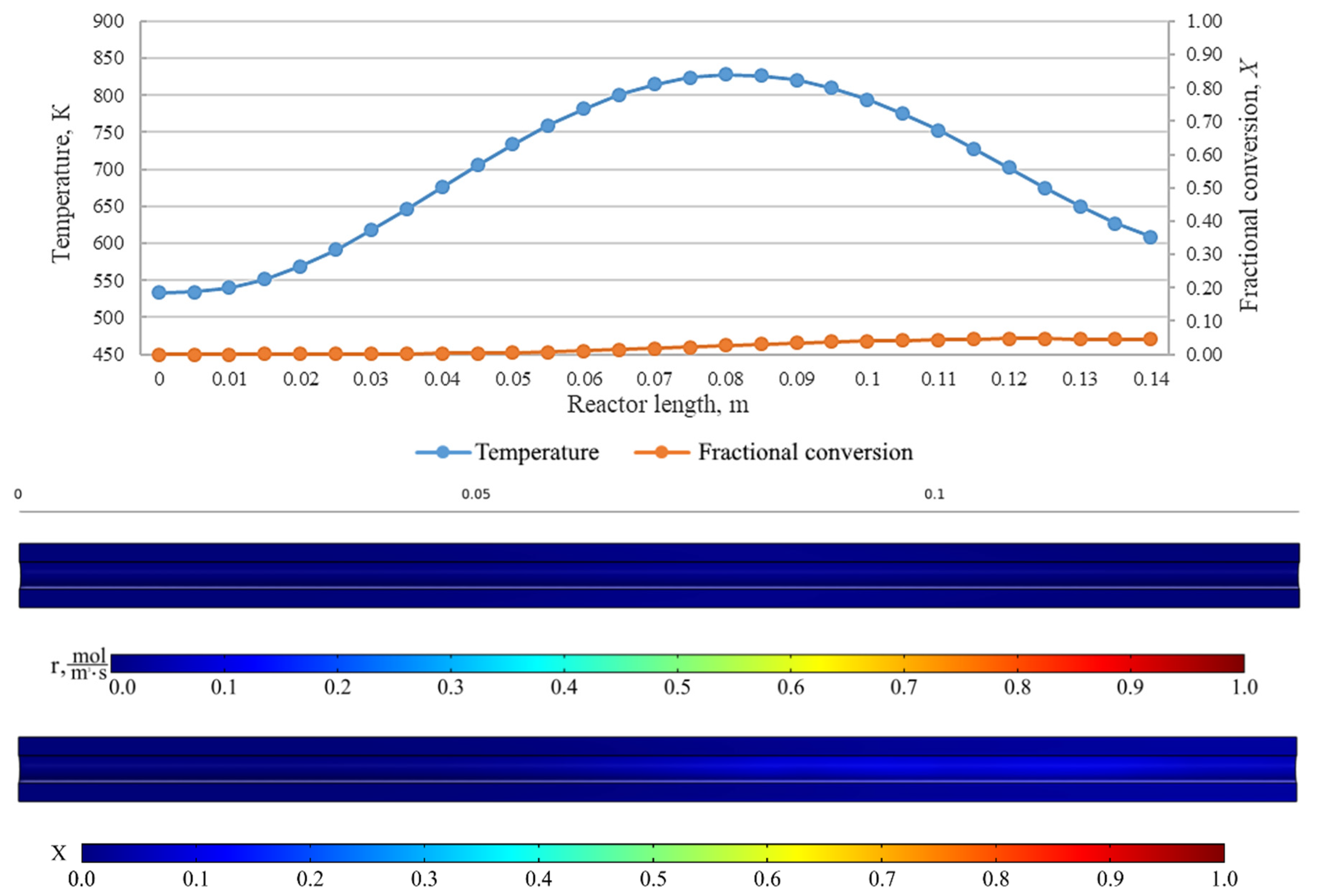
Figure 8.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_350_18 (Tmax = 350 °C, = 0.05 L/min).
Figure 8.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_350_18 (Tmax = 350 °C, = 0.05 L/min).
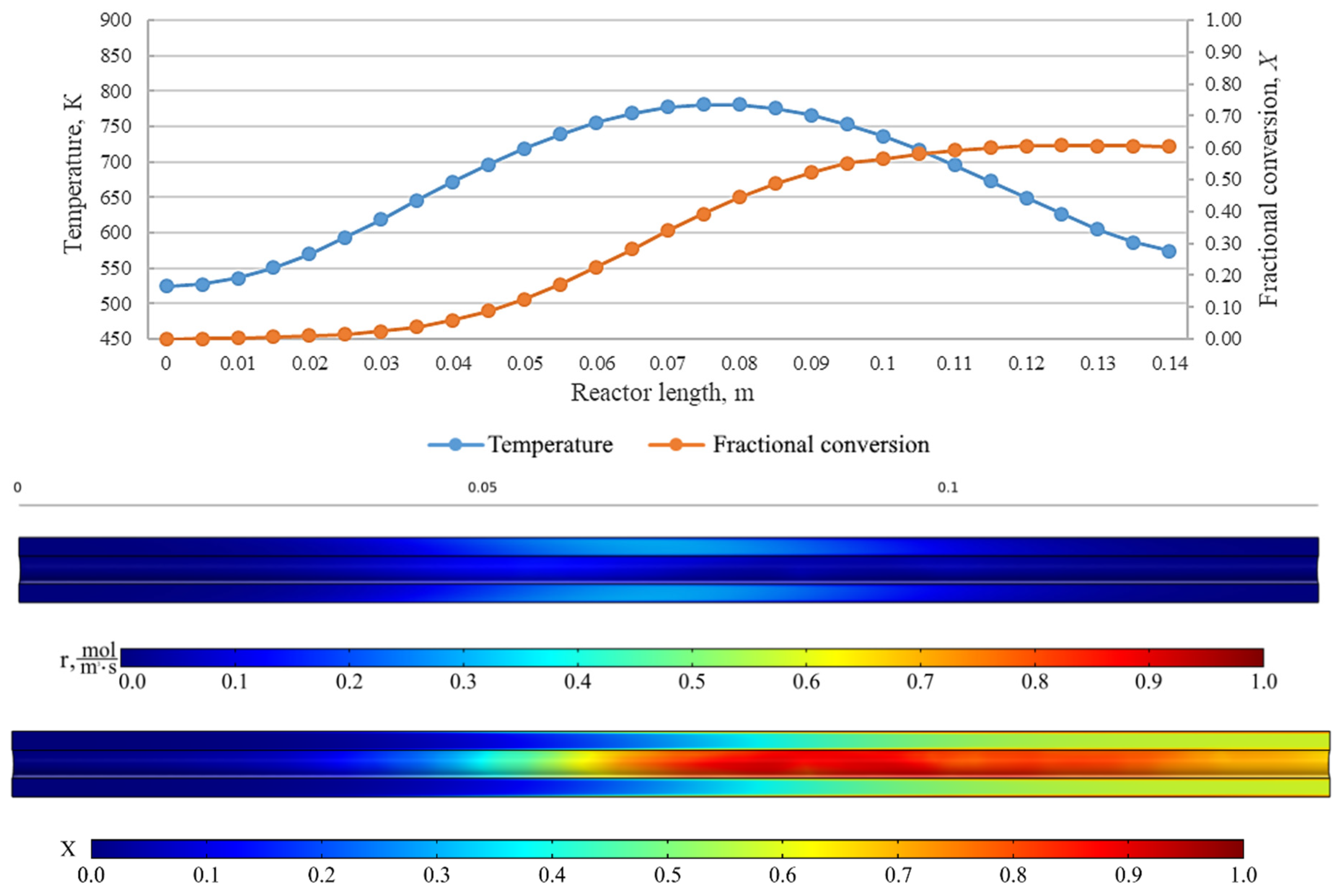
Figure 9.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_350_40 (Tmax = 350 °C, = 0.25 L/min).
Figure 9.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_350_40 (Tmax = 350 °C, = 0.25 L/min).
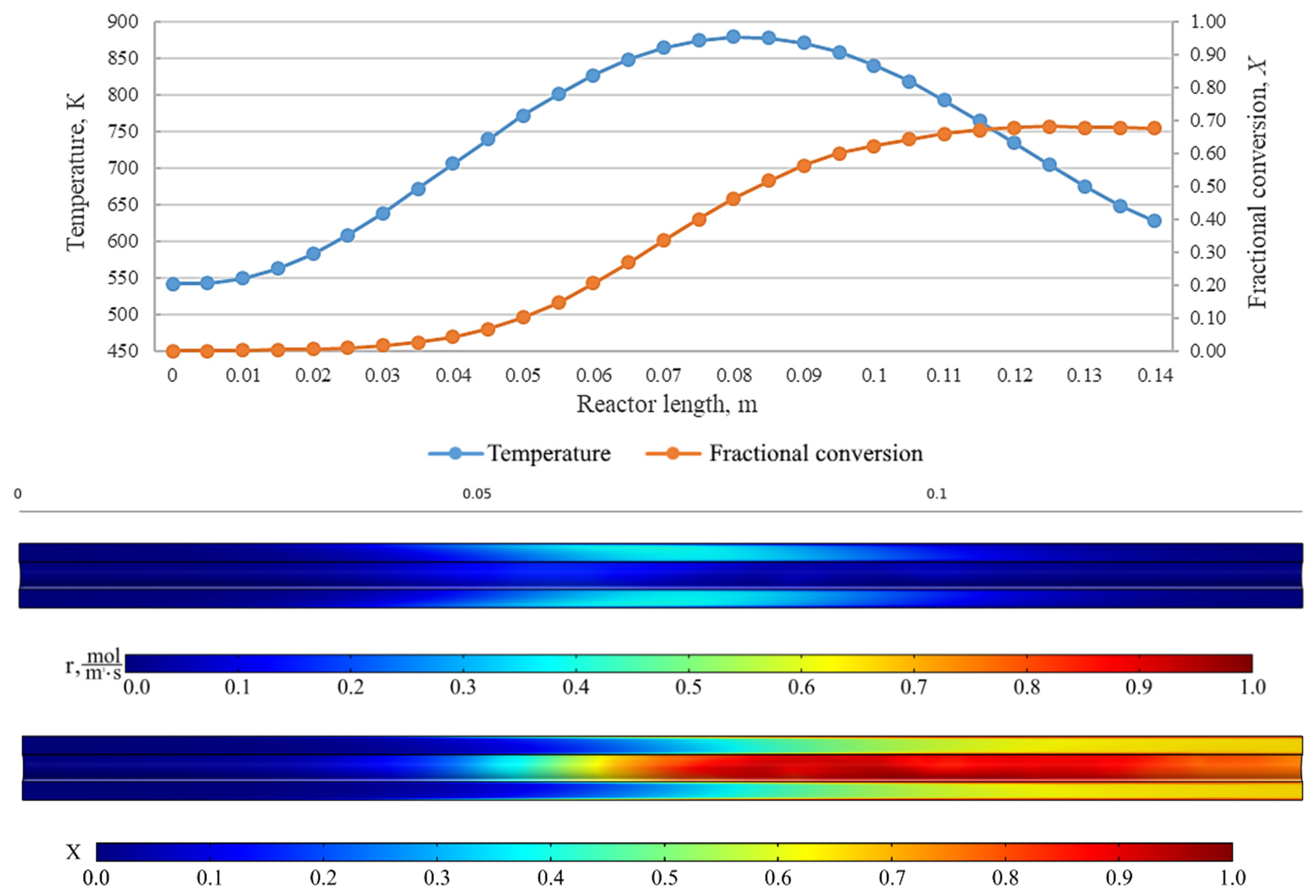
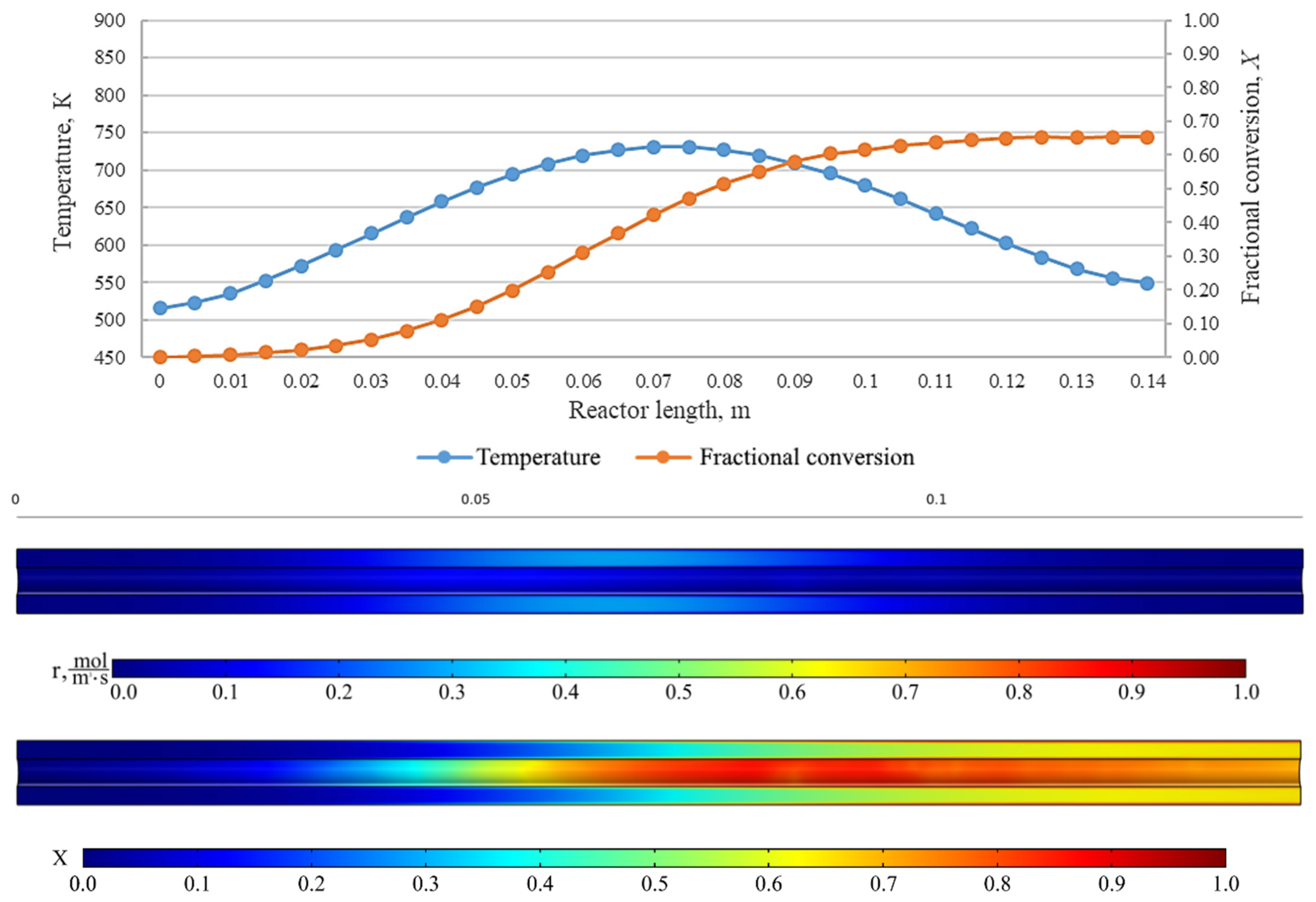
Figure 10.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_500_19 (Tmax = 500 °C, = 0.05 L/min).
Figure 10.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_500_19 (Tmax = 500 °C, = 0.05 L/min).
Figure 11.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_500_43 (Tmax = 500 °C, = 1.5 L/min).
Figure 11.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_500_43 (Tmax = 500 °C, = 1.5 L/min).
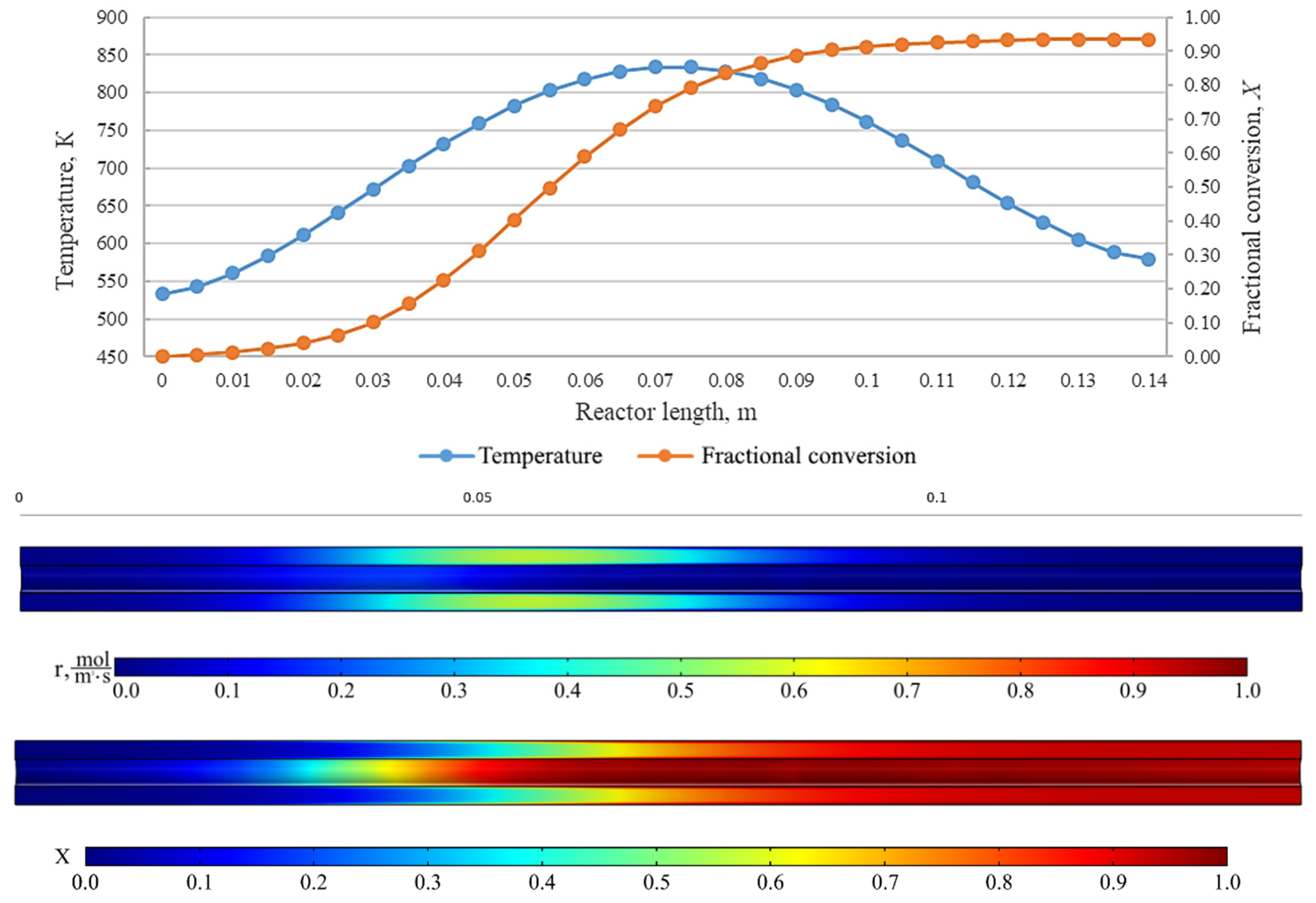
Figure 12.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_600_23 (Tmax = 600 °C, = 0.05 L/min).
Figure 12.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_600_23 (Tmax = 600 °C, = 0.05 L/min).
Figure 13.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_600_37 (Tmax = 600 °C, = 3.0 L/min).
Figure 13.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BnOm_600_37 (Tmax = 600 °C, = 3.0 L/min).
Figure 14.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_550_71 (Tmax = 550 °C, = 0.05 L/min).
Figure 14.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_550_71 (Tmax = 550 °C, = 0.05 L/min).
Figure 15.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_350_72 (Tmax = 350 °C, = 0.1 L/min).
Figure 15.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_350_72 (Tmax = 350 °C, = 0.1 L/min).
Figure 16.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_600_82 (Tmax = 600 °C, = 0.15 L/min).
Figure 16.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_600_82 (Tmax = 600 °C, = 0.15 L/min).
Figure 17.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_550_69 (Tmax = 550 °C, = 3.0 L/min).
Figure 17.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment BuOm_550_69 (Tmax = 550 °C, = 3.0 L/min).
Figure 18.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_450_116 (Tmax = 450 °C, = 0.05 L/min).
Figure 18.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_450_116 (Tmax = 450 °C, = 0.05 L/min).
Figure 19.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_450_127 (Tmax = 450 °C, = 1.0 L/min).
Figure 19.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_450_127 (Tmax = 450 °C, = 1.0 L/min).
Figure 20.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_550_120 (Tmax = 550 °C, = 0.05 L/min).
Figure 20.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_550_120 (Tmax = 550 °C, = 0.05 L/min).
Figure 21.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_550_129 (Tmax = 550 °C, = 3.0 L/min).
Figure 21.
From top to bottom: temperature and fractional conversion curves, profiles of the reaction rate and fractional conversion along the reactor length for the experiment cyGOm_550_129 (Tmax = 550 °C, = 3.0 L/min).
Table 1.
Composition and characteristics of the components of the facility for thermolysis.
Table 1.
Composition and characteristics of the components of the facility for thermolysis.
| Reference Designation | Unit | Number | Characteristics |
|---|
| GFR | Gas flow regulator | 1 | 0.9–180 L/h |
| TS | Thermostat | 1 | T = 25–200 °C |
| F | Fan unit | 1 | — |
| PD | Dosing syringe pump | 1 | V = 10 mL
v = 1–14,000 mL/min |
| HE01, HE02 | Heat exchangers | 2 | F = 20.7 sm2 |
| R | Reactor | 1 | T = 25–450 °C, L = 130 mm, Dout = 10 mm, din = 7 mm |
| PC | Circulating gear pump | 1 | v = 0–30 mL/min |
| S | Separator | 1 | — |
Table 2.
Parameter range for a series of experiments on the thermal decomposition of N-alkyl-O-methylcarbamates.
Table 2.
Parameter range for a series of experiments on the thermal decomposition of N-alkyl-O-methylcarbamates.
| | Maximum Temperature in the Reactor (Tmax), °C |
|---|
| 250 | 300 | 350 | 400 | 450 | 500 | 550 | 600 |
|---|
| Carrier gas flow, L/min | 0.05 | X | X | X | X | X | X | X | X |
| 0.08 | X | X | X | X | X | X | X | X |
| 0.1 | | X | X | X | X | X | X | X |
| 0.15 | | X | X | X | X | X | X | X |
| 0.2 | | | X | X | X | X | X | X |
| 0.25 | | | X | X | X | X | X | X |
| 0.3 | | | | X | X | X | X | X |
| 0.4 | | | | X | X | X | X | X |
| 0.5 | | | | | X | X | X | X |
| 0.75 | | | | | X | X | X | X |
| 1 | | | | | | X | X | X |
| 1.5 | | | | | | X | X | X |
| 2 | | | | | | | X | X |
| 2.5 | | | | | | | X | X |
| 3 | | | | | | | | X |
Table 3.
Results of the kinetic measurements of O-methyl-N-benzyl carbamate thermolysis (1c).
Table 3.
Results of the kinetic measurements of O-methyl-N-benzyl carbamate thermolysis (1c).
| Experiment No. | Experiment Code | Maximum Temperature in the Reactor (Tmax), °C | Residence Time, s | Carrier Gas Flow, L/min | Fractional Conversion, χ |
|---|
| 1 | BnOm_250_38 | 250 | 1.880 | 0.08 | 0.286 ± 0.005 |
| 2 | BnOm_300_39 | 300 | 0.948 | 0.15 | 0.351 ± 0.006 |
| 3 | BnOm_350_18 | 350 | 0.540 | 0.05 | 0.927 ± 0.018 |
| 4 | BnOm_350_40 | 350 | 2.703 | 0.25 | 0.436 ± 0.007 |
| 5 | BnOm_400_20 | 400 | 0.322 | 0.05 | 0.919 ± 0.018 |
| 6 | BnOm_400_41 | 400 | 2.577 | 0.40 | 0.526 ± 0.008 |
| 7 | BnOm_450_21 | 450 | 0.164 | 0.05 | 0.930 ± 0.018 |
| 8 | BnOm_450_42 | 450 | 2.577 | 0.75 | 0.627 ± 0.010 |
| 9 | BnOm_500_19 | 500 | 0.078 | 0.05 | 0.922 ± 0.018 |
| 10 | BnOm_500_43 | 500 | 2.363 | 1.50 | 0.549 ± 0.009 |
| 11 | BnOm_550_22 | 550 | 0.045 | 0.05 | 0.972 ± 0.020 |
| 12 | BnOm_550_44 | 550 | 2.272 | 2.50 | 0.413 ± 0.007 |
| 13 | BnOm_600_23 | 600 | 0.036 | 0.05 | 0.985 ± 0.021 |
| 14 | BnOm_600_24 | 600 | 0.043 | 0.08 | 0.995 ± 0.021 |
| 15 | BnOm_600_26 | 600 | 0.054 | 0.15 | 0.979 ± 0.021 |
| 16 | BnOm_600_27 | 600 | 0.072 | 0.20 | 0.967 ± 0.020 |
| 17 | BnOm_600_29 | 600 | 0.109 | 0.30 | 0.953 ± 0.020 |
| 18 | BnOm_600_30 | 600 | 0.145 | 0.40 | 0.924 ± 0.018 |
| 19 | BnOm_600_31 | 600 | 0.218 | 0.50 | 0.945 ± 0.018 |
| 20 | BnOm_600_32 | 600 | 0.273 | 0.75 | 0.909 ± 0.018 |
| 21 | BnOm_600_33 | 600 | 0.364 | 1.00 | 0.862 ± 0.017 |
| 22 | BnOm_600_34 | 600 | 0.546 | 1.50 | 0.731 ± 0.012 |
| 23 | BnOm_600_35 | 600 | 0.729 | 2.00 | 0.777 ± 0.014 |
| 24 | BnOm_600_36 | 600 | 1.367 | 2.50 | 0.634 ± 0.010 |
| 25 | BnOm_600_37 | 600 | 2.188 | 3.00 | 0.577 ± 0.009 |
Table 4.
Results of the kinetic measurements of O-methyl-N-cyclohexyl carbamate thermolysis (1b).
Table 4.
Results of the kinetic measurements of O-methyl-N-cyclohexyl carbamate thermolysis (1b).
| Experiment No. | Experiment Code | Maximum Temperature in the Reactor (Tmax), °C | Residence Time, s | Carrier Gas Flow, L/min | Fractional Conversion, χ |
|---|
| 1 | cyGOm_250_111 | 250 | 3.007 | 0.05 | 0.031 ± 0.001 |
| 2 | cyGOm_300_110 | 300 | 2.845 | 0.05 | 0.057 ± 0.001 |
| 3 | cyGOm_350_112 | 350 | 2.703 | 0.05 | 0.138 ± 0.003 |
| 4 | cyGOm_350_113 | 350 | 1.351 | 0.10 | 0.085 ± 0.002 |
| 5 | cyGOm_350_114 | 350 | 0.675 | 0.20 | 0.058 ± 0.001 |
| 6 | cyGOm_350_115 | 350 | 0.450 | 0.30 | 0.052 ± 0.001 |
| 7 | cyGOm_400_124 | 400 | 2.577 | 0.05 | 0.319 ± 0.005 |
| 8 | cyGOm_450_116 | 450 | 2.465 | 0.05 | 0.781 ± 0.015 |
| 9 | cyGOm_450_117 | 450 | 1.232 | 0.10 | 0.289 ± 0.005 |
| 10 | cyGOm_450_118 | 450 | 0.616 | 0.20 | 0.149 ± 0.003 |
| 11 | cyGOm_450_119 | 450 | 0.41 | 0.30 | 0.084 ± 0.002 |
| 12 | cyGOm_450_127 | 450 | 0.123 | 1.00 | 0.117 ± 0.002 |
| 13 | cyGOm_500_125 | 500 | 2.363 | 0.05 | 0.823 ± 0.016 |
| 14 | cyGOm_550_120 | 550 | 2.272 | 0.05 | 0.739 ± 0.013 |
| 15 | cyGOm_550_121 | 550 | 1.136 | 0.10 | 0.546 ± 0.009 |
| 16 | cyGOm_550_122 | 550 | 0.567 | 0.20 | 0.482 ± 0.008 |
| 17 | cyGOm_550_123 | 550 | 0.378 | 0.30 | 0.373 ± 0.006 |
| 18 | cyGOm_550_128 | 550 | 0.113 | 1.00 | 0.161 ± 0.003 |
| 19 | cyGOm_550_129 | 550 | 0.037 | 3.00 | 0.093 ± 0.002 |
| 20 | cyGOm_600_126 | 600 | 2.188 | 0.05 | 0.901 ± 0.018 |
Table 5.
Results of the kinetic measurements of O-methyl-N-(n-butyl) carbamate thermolysis (1a).
Table 5.
Results of the kinetic measurements of O-methyl-N-(n-butyl) carbamate thermolysis (1a).
| Experiment No. | Experiment Code | Maximum Temperature in the Reactor (Tmax), °C | Residence Time, s | Carrier Gas Flow, L/min | Fractional Conversion, χ |
|---|
| 1 | BuOm_250_63 | 250 | 3.007 | 0.05 | 0.019 ± 0.001 |
| 2 | BuOm_300_64 | 300 | 2.845 | 0.05 | 0.075 ± 0.002 |
| 3 | BuOm_350_65 | 350 | 2.703 | 0.05 | 0.169 ± 0.004 |
| 4 | BuOm_350_72 | 350 | 1.351 | 0.10 | 0.202 ± 0.005 |
| 5 | BuOm_350_73 | 350 | 0.675 | 0.20 | 0.011 ± 0.001 |
| 6 | BuOm_350_74 | 350 | 0.450 | 0.30 | 0.002 ± 0.001 |
| 7 | BuOm_400_66 | 400 | 2.577 | 0.05 | 0.323 ± 0.007 |
| 8 | BuOm_450_67 | 450 | 2.465 | 0.05 | 0.638 ± 0.015 |
| 9 | BuOm_450_75 | 450 | 1.232 | 0.10 | 0.403 ± 0.009 |
| 10 | BuOm_450_76 | 450 | 0.616 | 0.20 | 0.170 ± 0.004 |
| 11 | BuOm_450_77 | 450 | 0.410 | 0.30 | 0.077 ± 0.002 |
| 12 | BuOm_450_78 | 450 | 0.123 | 1.00 | 0.117 ± 0.003 |
| 13 | BuOm_500_68 | 500 | 2.363 | 0.05 | 0.804 ± 0.021 |
| 14 | BuOm_550_71 | 550 | 2.272 | 0.05 | 0.957 ± 0.027 |
| 15 | BuOm_550_79 | 550 | 1.136 | 0.10 | 0.703 ± 0.016 |
| 16 | BuOm_550_80 | 550 | 0.378 | 0.30 | 0.205 ± 0.005 |
| 17 | BuOm_550_81 | 550 | 0.113 | 1.00 | 0.156 ± 0.004 |
| 18 | BuOm_550_69 | 550 | 0.037 | 3.00 | 0.090 ± 0.002 |
| 19 | BuOm_600_70 | 600 | 2.188 | 0.05 | 0.973 ± 0.027 |
| 20 | BuOm_600_82 | 600 | 0.729 | 0.15 | 0.601 ± 0.014 |
Table 6.
Activation energies and pre-exponential factors of the studied carbamates.
Table 6.
Activation energies and pre-exponential factors of the studied carbamates.
| Parameter | O-methyl-N-benzyl Carbamate | O-methyl-N-butyl
Carbamate | O-methyl-N-cyclohexyl Carbamate |
|---|
| Activation energy, kJ/mol | 58.8 | 52.0 | 55.4 |
| Pre-exponential factor, s−1 | 5.99·105 | 1.25·104 | 2.15·104 |
Table 7.
Coefficients of the reactor temperature profile relationship.
Table 7.
Coefficients of the reactor temperature profile relationship.
| Coefficient | Value | SD |
|---|
| a11 | −2.47 × 10−3 | 6.37 × 10−5 |
| a12 | 4.15 × 10−2 | 4.37 × 10−4 |
| a21 | −3.62 × 10−4 | 1.28 × 10−4 |
| a22 | 6.12 × 10−3 | 4.50 × 10−5 |
| a23 | −1.19 | 1.21 × 10−2 |
| a31 | 0.893 | 2.10 × 10−2 |
| a32 | 7.96 | 0.118 |
| a41 | −6.30 | 0.134 |
| a42 | 8.65 | 0.589 |
| a5 | 23.2 | 0.659 |
| a6 | 194 | 1.16 |
Table 8.
Input parameters for the computational experiments.
Table 8.
Input parameters for the computational experiments.
| Parameter | O-methyl-N-benzyl Carbamate | O-methyl-N-butyl Carbamate | O-methyl-N-cyclohexyl Carbamate |
|---|
| Molecular weight of carbamate component, g/mol | 165.19 | 131.17 | 157.21 |
| Molecular weight of isocyanate component, g/mol | 133.15 | 99.13 | 125.17 |
| Molecular weight of methanol component, g/mol | 32.04 |
| Molecular weight of argon component, g/mol | 39.95 |
| Activation energy, kJ/mol | 58.8 | 52.0 | 55.4 |
| Pre-exponential factor, s−1 | 5.99 × 105 | 1.25 × 104 | 2.15 × 104 |
| Reactor input temperature, °C | 200 |
| Maximum heating temperature in the middle of the reactor, °C | 250–600 |
| Carrier gas flow, L/min | 0.05–3.0 |
Table 9.
Comparison of the experimental fractional conversion of O-methyl N-benzyl carbamate with the data of the calculation experiments.
Table 9.
Comparison of the experimental fractional conversion of O-methyl N-benzyl carbamate with the data of the calculation experiments.
| No. | Experiment Code | Heating Temperature, °C | Gas Consumption, L/min | Experimental Fractional Conversion | Fractional Conversion in COMSOL |
|---|
| 1 | BnOm_250_38 | 250 | 0.08 | 0.286 | 0.386 |
| 2 | BnOm_300_39 | 300 | 0.15 | 0.351 | 0.473 |
| 3 | BnOm_350_18 | 350 | 0.05 | 0.927 | 0.984 |
| 4 | BnOm_350_40 | 350 | 0.25 | 0.436 | 0.571 |
| 5 | BnOm_400_20 | 400 | 0.05 | 0.919 | 1.000 |
| 6 | BnOm_400_41 | 400 | 0.40 | 0.526 | 0.663 |
| 7 | BnOm_450_21 | 450 | 0.05 | 0.930 | 1.000 |
| 8 | BnOm_450_42 | 450 | 0.75 | 0.627 | 0.661 |
| 9 | BnOm_500_19 | 500 | 0.05 | 0.922 | 1.000 |
| 10 | BnOm_500_43 | 500 | 1.50 | 0.549 | 0.603 |
| 11 | BnOm_550_22 | 550 | 0.05 | 0.972 | 1.000 |
| 12 | BnOm_550_44 | 550 | 2.50 | 0.413 | 0.595 |
| 13 | BnOm_600_23 | 600 | 0.05 | 0.985 | 1.000 |
| 14 | BnOm_600_24 | 600 | 0.08 | 0.995 | 1.000 |
| 15 | BnOm_600_26 | 600 | 0.15 | 0.979 | 1.000 |
| 16 | BnOm_600_27 | 600 | 0.20 | 0.967 | 1.000 |
| 17 | BnOm_600_29 | 600 | 0.30 | 0.953 | 0.999 |
| 18 | BnOm_600_30 | 600 | 0.40 | 0.924 | 0.999 |
| 19 | BnOm_600_31 | 600 | 0.50 | 0.945 | 0.999 |
| 20 | BnOm_600_32 | 600 | 0.75 | 0.909 | 0.990 |
| 21 | BnOm_600_33 | 600 | 1.00 | 0.862 | 0.967 |
| 22 | BnOm_600_34 | 600 | 1.50 | 0.731 | 0.898 |
| 23 | BnOm_600_35 | 600 | 2.00 | 0.777 | 0.822 |
| 24 | BnOm_600_36 | 600 | 2.50 | 0.634 | 0.747 |
| 25 | BnOm_600_37 | 600 | 3.00 | 0.577 | 0.675 |
Table 10.
Comparison of the experimental fractional conversion of O-methyl-N-butyl carbamate with the data of the calculation experiments.
Table 10.
Comparison of the experimental fractional conversion of O-methyl-N-butyl carbamate with the data of the calculation experiments.
| No. | Experiment Code | Heating Temperature, °C | Gas Consumption, L/min | Experimental Fractional Conversion | Fractional Conversion in COMSOL |
|---|
| 1 | BtOm_250_63 | 250 | 0.05 | 0.019 | 0.079 |
| 2 | BtOm_300_64 | 300 | 0.05 | 0.075 | 0.164 |
| 3 | BtOm_350_65 | 350 | 0.05 | 0.169 | 0.301 |
| 4 | BtOm_350_72 | 350 | 0.10 | 0.202 | 0.165 |
| 5 | BtOm_350_73 | 350 | 0.20 | 0.011 | 0.087 |
| 6 | BtOm_350_74 | 350 | 0.30 | 0.002 | 0.059 |
| 7 | BtOm_400_66 | 400 | 0.05 | 0.323 | 0.481 |
| 8 | BtOm_450_67 | 450 | 0.05 | 0.638 | 0.671 |
| 9 | BtOm_450_75 | 450 | 0.10 | 0.403 | 0.431 |
| 10 | BtOm_450_76 | 450 | 0.20 | 0.170 | 0.248 |
| 11 | BtOm_450_77 | 450 | 0.30 | 0.077 | 0.174 |
| 12 | BtOm_450_78 | 450 | 1.00 | 0.117 | 0.056 |
| 13 | BtOm_500_68 | 500 | 0.05 | 0.804 | 0.830 |
| 14 | BtOm_550_71 | 550 | 0.05 | 0.957 | 0.916 |
| 15 | BtOm_550_79 | 550 | 0.10 | 0.703 | 0.742 |
| 16 | BtOm_550_80 | 550 | 0.30 | 0.205 | 0.370 |
| 17 | BtOm_550_81 | 550 | 1.00 | 0.156 | 0.132 |
| 18 | BtOm_550_69 | 550 | 3.00 | 0.090 | 0.045 |
| 19 | BtOm_600_70 | 600 | 0.05 | 0.973 | 0.858 |
| 20 | BtOm_600_82 | 600 | 0.15 | 0.601 | 0.731 |
Table 11.
Comparison of the experimental fractional conversion of O-methyl-N-cyclohexyl carbamate with the data of the calculation experiments.
Table 11.
Comparison of the experimental fractional conversion of O-methyl-N-cyclohexyl carbamate with the data of the calculation experiments.
| No. | Experiment Code | Heating Temperature, °C | Gas Consumption, L/min | Experimental Fractional Conversion | Fractional Conversion in COMSOL |
|---|
| 1 | tsGOm_250_111 | 250 | 0.05 | 0.031 | 0.061 |
| 2 | tsGOm_300_110 | 300 | 0.05 | 0.057 | 0.137 |
| 6 | tsGOm_350_112 | 350 | 0.05 | 0.138 | 0.268 |
| 5 | tsGOm_350_113 | 350 | 0.10 | 0.085 | 0.145 |
| 4 | tsGOm_350_114 | 350 | 0.20 | 0.058 | 0.076 |
| 3 | tsGOm_350_115 | 350 | 0.30 | 0.052 | 0.051 |
| 7 | tsGOm_400_124 | 400 | 0.05 | 0.319 | 0.653 |
| 12 | tsGOm_450_116 | 450 | 0.05 | 0.781 | 0.653 |
| 11 | tsGOm_450_117 | 450 | 0.10 | 0.289 | 0.415 |
| 10 | tsGOm_450_118 | 450 | 0.20 | 0.149 | 0.237 |
| 9 | tsGOm_450_119 | 450 | 0.30 | 0.084 | 0.166 |
| 8 | tsGOm_450_127 | 450 | 1.00 | 0.117 | 0.053 |
| 13 | tsGOm_500_125 | 500 | 0.05 | 0.823 | 0.825 |
| 19 | tsGOm_550_120 | 550 | 0.05 | 0.739 | 0.934 |
| 18 | tsGOm_550_121 | 550 | 0.10 | 0.546 | 0.748 |
| 17 | tsGOm_550_122 | 550 | 0.20 | 0.482 | 0.503 |
| 16 | tsGOm_550_123 | 550 | 0.30 | 0.373 | 0.375 |
| 15 | tsGOm_550_128 | 550 | 1.00 | 0.161 | 0.134 |
| 14 | tsGOm_550_129 | 550 | 3.00 | 0.093 | 0.046 |
| 20 | tsGOm_600_126 | 600 | 0.05 | 0.901 | 0.982 |
Table 12.
Statistical processing of the experimental and calculated fractional conversions.
Table 12.
Statistical processing of the experimental and calculated fractional conversions.
| Compound | O-methyl-N-benzyl Carbamate | O-methyl-N-butyl Carbamate | O-methyl-N-cyclohexyl Carbamate |
|---|
| Relative error, % | 4.8 | 5.7 | 6.6 |
| Correlation factor, R2 | 0.9838 | 0.9819 | 0.9599 |
| Fisher’s test, Fobs. | 0.8895 | 1.1668 | 0.9807 |
| Fisher’s test, Fcr. | Upper α/2-quantile | - | 2.1683 | - |
| Lower α/2-quantile | 0.5041 | - | 0.4612 |
| Comparison of | Fobs. ~ Fcr. | Fp > Fcr. (L. α/2) | Fp < Fcr. (U. α/2) | Fp > Fcr. (L. α/2) |
| p | 0.3883 | 0.3701 | 0.4833 |
| Comparison of the p-value with the significance level of α = 0.05 | p > 2α | p > 2α | p > 2α |