Effect of Mesh Sensitivity and Cohesive Properties on Simulation of Typha Fiber/Epoxy Microbond Test
Abstract
1. Introduction
2. Materials and Methods
2.1. FEM
2.2. Material Properties
3. Mesh Sensitivity Analysis
4. Interfacial Debonding
5. Conclusions
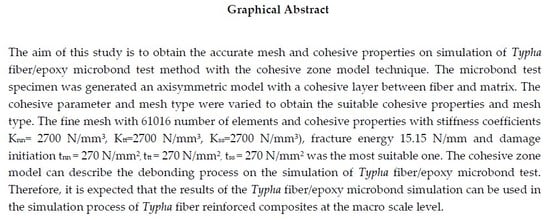
- Fine mesh with 61,016 elements is the most suitable type for the Typha fiber/epoxy microbond simulation test based on the comparison of simulation and experimental results.
- The simulation results agreed well with experimental results of load and displacement curves and interfacial shear strength values when cohesive properties including stiffness coefficients (Knn of 2700 N/mm3, Ktt of 2700 N/mm3, and Kss of 2700 N/mm3), fracture energy of 15.15 N/mm, and damage initiation (tnn = 270 N/mm2, ttt = 270 N/mm2, tss = 270 N/mm2), with fine mesh.
- The cohesive zone model can describe the debonding process during the simulation of the Typha fiber/epoxy microbond test.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Orue, A.; Jauregi, A.; Unsuain, U.; Labidi, J.; Eceiza, A.; Arbelaiz, A. The effect of alkaline and silane treatments on mechanical properties and breakage of sisal fibers and poly (lactic acid)/sisal fiber composites. Compos. Part A Appl. Sci. Manuf. 2016, 84, 186–195. [Google Scholar] [CrossRef]
- Caprino, G.; Carrino, L.; Durante, M.; Langella, A.; Lopresto, V. Low impact behaviour of hemp fibre reinforced epoxy composites. Compos. Struct. 2015, 133, 892–901. [Google Scholar] [CrossRef]
- Faruk, O.; Bledzki, A.K.; Fink, H.-P.; Sain, M. Biocomposites reinforced with natural fibers: 2000–2010. Prog. Polym. Sci. 2012, 37, 1552–1596. [Google Scholar] [CrossRef]
- Zhou, B.; Tu, T.; Kong, F.; Wen, J.; Xu, X. Revised phylogeny and historical biogeography of the cosmopolitan aquatic plant genus Typha (Typhaceae). Sci. Rep. 2018, 8, 8813. [Google Scholar] [CrossRef]
- Ramanaiah, K.; Ratna Prasad, A.V.; Chandra Reddy, K.H. Mechanical Properties and Thermal Conductivity of Typha angustifolia Natural Fiber–Reinforced Polyester Composites. Int. J. Polym. Anal. Charact. 2011, 16, 496–503. [Google Scholar] [CrossRef]
- Bajwa, D.S.; Sitz, E.D.; Bajwa, S.G.; Barnick, A.R. Evaluation of cattail (Typha spp.) for manufacturing composite panels. Ind. Crops Prod. 2015, 75, 195–199. [Google Scholar] [CrossRef]
- Wuzella, G.; Mahendran, A.R.; Bätge, T.; Jury, S.; Kandelbauer, A. Novel, binder-free fiber reinforced composites based on a renewable resource from the reed-like plant Typha sp. Ind. Crops Prod. 2011, 33, 683–689. [Google Scholar] [CrossRef]
- Khalil, H.P.S.A.; Ismail, H.; Rozman, H.D.; Ahmad, M.N. The effect of acetylation on interfacial shear strength between plant fibres and various matrices. Eur. Polym. J. 2001, 37, 1037–1045. [Google Scholar] [CrossRef]
- Chen, J.; Hojjati, M. Microdielectric Analysis and Curing Kinetics of an Epoxy Resin System. Polym. Eng. Sci. 2007, 47, 150–158. [Google Scholar] [CrossRef]
- Ratnam, M.M.; Lim, J.H.; Khalil, H.P.S.A. Study of three-dimensional deformation of a pallet using phase-shiff shadow moiré and finite-element analysis. Exp. Mech. 2005, 45, 9–17. [Google Scholar] [CrossRef]
- Elmatzoglou, M.; Avdelas, A. Numerical Modelling of Double-Steel Plate Composite Shear Walls. Computation 2017, 5, 12. [Google Scholar] [CrossRef]
- Miller, B.; Muri, P.; Rebenfeld, L. A microbond method for determination of the shear strength of a fiber/resin interface. Compos. Sci. Technol. 1987, 28, 17–32. [Google Scholar] [CrossRef]
- Sockalingam, S.; Nilakantan, G. Fiber-matrix interface characterization through the microbond test: A review. Int. J. Aeronaut. Space Sci. 2012, 13, 282–295. [Google Scholar] [CrossRef]
- Kang, S.K.; Lee, D.B.; Choi, N.S. Fiber/epoxy interfacial shear strength measured by the microdroplet test. Compos. Sci. Technol. 2009, 69, 245–251. [Google Scholar] [CrossRef]
- Xiong, X.; Shen, S.Z.; Hua, L.; Liu, J.Z.; Li, X.; Wan, X.; Miao, M. Finite element models of natural fibers and their composites: A review. J. Reinf. Plast. Compos. 2018, 37, 617–635. [Google Scholar] [CrossRef]
- Huzni, S.; Ilfan, M.; Sulaiman, T.; Fonna, S.; Ridha, M.; Arifin, A.K. Finite element modeling of delamination process on composite laminate using cohesive elements. Int. J. Automot. Mech. Eng. 2013, 7, 1023. [Google Scholar] [CrossRef]
- Ash, J.T.; Cross, W.M.; Svalstad, D.; Kellar, J.J.; Kjerengtroen, L. Finite element evaluation of the microbond test: Meniscus effect, interphase region, and vise angle. Compos. Sci. Technol. 2003, 63, 641–651. [Google Scholar] [CrossRef]
- Lin, G.; Geubelle, P.H.; Sottos, N.R. Simulation of fiber debonding with friction in a model composite pushout test. Int. J. Solids Struct. 2001, 38, 8547–8562. [Google Scholar] [CrossRef]
- Meng, Q.; Wang, Z. Prediction of interfacial strength and failure mechanisms in particle-reinforced metal-matrix composites based on a micromechanical model. Eng. Fract. Mech. 2015, 142, 170–183. [Google Scholar] [CrossRef]
- Dugdale, D.S. Yielding of steel sheets containing slits. J. Mech. Phys. Solids 1960, 8, 100–104. [Google Scholar] [CrossRef]
- Barenblatt, G.I. The mathematical theory of equilibrium cracks in brittle fracture. In Advances in Applied Mechanics; Elsevier: Amsterdam, The Netherlands, 1962; Volume 7, pp. 55–129. ISSN 0065-2156. [Google Scholar]
- Rizal, S.; Gopakumar, D.A.; Huzni, S.; Thalib, S.; Syakir, M.I.; Owolabi, F.A.T.; Aprilla, N.A.S.; Paridah, M.T.; Khalil, H.P.S.A. Tailoring the Effective Properties of Typha Fiber Reinforced Polymer Composite via Alkali Treatment. BioResources 2019, 14, 5630–5645. [Google Scholar]
- Selmi, A. Effect of Glass Fiber Content on the Flexural Modulus of Elasticity of Glass-Epoxy Sandwich Composites. Emerg. Trends Eng. Technol. 2014, 2, 187–191. [Google Scholar]
- Dadej, K.; Surowska, B. Analysis of cohesive zone model parameters on response of glass-epoxy composite in mode II interlaminar fracture toughness test. Theory Pract. 2016, 16, 180–188. [Google Scholar]
- Potukuchi, S.K.S. Fracture Analysis of Carbon Fiber/Epoxy Matrix Interface through Microbond and Cruciform Tests. Master’s Thesis, The University of Illinois, Urbana, IL, USA, 2016. Available online: https://www.ideals.illinois.edu/bitstream/handle/2142/90456/POTUKUCHI-THESIS-2016.pdf?sequence=1&isAllowed=y (accessed on 30 December 2019).
- Żmudzki, J.; Walke, W.; Chladek, W. Influence of model discretization density in FEM numerical analysis on the determined stress level in bone surrounding dental implants. In Information Technologies in Biomedicine; Springer: Berlin/Heidelberg, Germany, 2008; pp. 559–567. [Google Scholar]
- Liu, Y.; Glass, G. Effects of Mesh Density on Finite Element Analysis; SAE Technical Paper; SAE International: Warrendale, PA, USA, January 2013. [Google Scholar]
- Schellekens, J.C.J.; De Borst, R. A non-linear finite element approach for the analysis of mode-I free edge delamination in composites. Int. J. Solids Struct. 1993, 30, 1239–1253. [Google Scholar] [CrossRef]
- Soto, A.; González, E.V.; Maimí, P.; Turon, A.; de Aja, J.R.S.; de la Escalera, F.M. Cohesive zone length of orthotropic materials undergoing delamination. Eng. Fract. Mech. 2016, 159, 174–188. [Google Scholar] [CrossRef]
- Zhou, Y.; Fan, M.; Chen, L. Interface and bonding mechanisms of plant fibre composites: An overview. Compos. Part B Eng. 2016, 101, 31–45. [Google Scholar] [CrossRef]
- Hao, J.; Zhao, Y.; Luo, Y.; Wang, Y.; Chen, D. Experiments and finite element simulation of interfacial properties for monofilament composites. New Carbon Mater. 2014, 29, 176–185. [Google Scholar] [CrossRef]










| Mesh Type | Seed Mesh | Typha Fiber and Matrix | Cohesive Layer | Total | |
|---|---|---|---|---|---|
| CAX4 | CAX3 | COHAX4 | |||
| Coarse | 0.005 | 2256 | 183 | 1675 | 4114 |
| Medium | 0.003 | 5896 | 230 | 1675 | 7801 |
| Fine | 0.001 | 58,324 | 1017 | 1675 | 61,016 |
| Very Fine | 0.0005 | 18,8491 | 3145 | 1675 | 193,311 |
| Material | Young’s Modulus (GPa) | Poisson’s Ratio |
|---|---|---|
| Typha fiber | 0.88 | 0.35 |
| Epoxy resin | 3.7 | 0.4 |
| Variations | Stiffness Coefficients (N/mm3) | Fracture Energy (N/mm) | Damage Initiation (N/mm2) | ||||
|---|---|---|---|---|---|---|---|
| Knn | Kss | Ktt | GIc | tnn | tss | ttt | |
| VR1 | 2700 | 2700 | 2700 | 14.65 | 270 | 270 | 270 |
| VR2 | 2700 | 2700 | 2700 | 15.15 | 270 | 270 | 270 |
| VR3 | 3000 | 3000 | 3000 | 15.00 | 250 | 250 | 250 |
| VR4 | 3500 | 3500 | 3500 | 14.00 | 270 | 270 | 270 |
| VR5 | 5000 | 5000 | 5000 | 10.50 | 267 | 267 | 267 |
| VR6 | 8000 | 8000 | 8000 | 5.50 | 200 | 200 | 200 |
| VR7 | 10,000 | 10,000 | 10,000 | 5.00 | 250 | 250 | 250 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ikramullah; Afrizal, A.; Huzni, S.; Thalib, S.; Abdul Khalil, H.P.S.; Rizal, S. Effect of Mesh Sensitivity and Cohesive Properties on Simulation of Typha Fiber/Epoxy Microbond Test. Computation 2020, 8, 2. https://doi.org/10.3390/computation8010002
Ikramullah, Afrizal A, Huzni S, Thalib S, Abdul Khalil HPS, Rizal S. Effect of Mesh Sensitivity and Cohesive Properties on Simulation of Typha Fiber/Epoxy Microbond Test. Computation. 2020; 8(1):2. https://doi.org/10.3390/computation8010002
Chicago/Turabian StyleIkramullah, Andri Afrizal, Syifaul Huzni, Sulaiman Thalib, H. P. S. Abdul Khalil, and Samsul Rizal. 2020. "Effect of Mesh Sensitivity and Cohesive Properties on Simulation of Typha Fiber/Epoxy Microbond Test" Computation 8, no. 1: 2. https://doi.org/10.3390/computation8010002
APA StyleIkramullah, Afrizal, A., Huzni, S., Thalib, S., Abdul Khalil, H. P. S., & Rizal, S. (2020). Effect of Mesh Sensitivity and Cohesive Properties on Simulation of Typha Fiber/Epoxy Microbond Test. Computation, 8(1), 2. https://doi.org/10.3390/computation8010002