Theoretical Prediction of Electronic Structures and Phonon Dispersion of Ce₂XN₂ (X = S, Se, and Te) Ternary

Abstract

A systematic study of structural, electronic, vibrational properties of new ternary dicerium selenide dinitride, Ce₂SeN₂ and predicted compounds—Ce₂SN₂ and Ce₂TeN₂—is performed using first-principles calculations within Perdew–Burke–Ernzerhof functional with Hubbard correction. Our calculated results for structural parameters nicely agree to the experimental measurements. We predict that all ternary dicerium chalcogenide nitrides are thermodynamically stable. The predicted elastic constants and related mechanical properties demonstrate its profound mechanical stability as well. Moreover, our results show that Ce₂XN₂ are insulator materials. Trends of the structural parameters, electronic structures, and phonon dispersion are discussed in terms of the characteristics of the Ce (4f) states.

1. Introduction

In recent years, remarkable progress has been made in the field of synthesis of novel multinary nitrides. They are known to exhibit a wide variety of physical properties as well as magnetic, electronic, optical, and catalytic properties [1,2,3,4]. Exploring their structure–bonding properties relationships remains the priority of many scientists in the field of solid-state chemistry [5]. Important works toward understanding structural diversity and complexity of lanthanide compounds have been made by Schleid and co-workers [6,7]. It was suggested that this heteroanionic system possesses a structural versatility comparable to that of oxynitrides and nitride halides. This area has been also extended to Li containing chalcogenide nitrides [8] to find new fast ion conductors with trivalent cations. Moreover, ternary compounds based on lanthanoid chalcogenide nitrides exhibit surprising crystal structures [7], whereas the binary rocksalt-type mononitrides LnN [7] display octahedrally surrounded cations and anions.

Recently, DiSalvo and co-workers introduced a new phase containing a tetravalent cation [9,10]. Subsequently, Dong and DiSalvo [11] synthesized a new ternary dicerium selenide dinitride using single crystal X-ray structure determination. Thus, to support the experimental findings reported by Dong and DiSalvo [11], we investigated using first-principles based methods to study the structural and electronic properties of the new ternary Ce₂SeN₂. We have also extended our theoretical works on the Ce₂SN₂ and Ce₂TeN₂ ternary phases in order to predict their crystal parameters, electronic structures, bonding characterization, and phonon dispersion. The role of changing X (= S, Se, or Te) on the structural properties and electronic structures as well as the bonding properties within the series of 2:1:2 compounds can be addressed from comparative analyses of the charges and formation energies. The purpose of this work is to present such a detail study for the Ce₂XN₂ ternaries with X = S, Se, or Te.

2. Computational Details

The total energy and electronic structure have been calculated within the density functional formalism using the projector augmented wave (PAW) approach [12] as implemented in the Vienna ab initio Simulation Package (VASP) [13]. The interaction between the core electrons and the valence electrons is described with the projector augmented wave potentials. The Perdew–Burke–Ernzerhof (PBE) [14] version of the generalized gradient approximation (GGA) is used for the exchange-correlation potential. On-site Hubbard interaction between the highly localized 5f electrons was treated within the rotationally invariant form [15] of GGA + U, a combination of the standard GGA and a Hubbard parameter U. The effective on-site Coulomb interaction energy U (U − J), is applied to the Ce 5f electrons (6 eV and 1 eV, respectively), as suggested in [16]. A plane waves energy cutoff of 500 eV was used. Structure relaxations were performed with 8 × 8 × 4 k-point grids, while the electronic properties were calculated by 16 × 16 × 8 k-point grids in the Monkhorst–Pack scheme [17]. During the geometry optimizations, all atoms were allowed to relax until the Hellmann–Feynman forces were smaller than 0.01 eV/Å. Both lattice vectors and atomic positions were fully relaxed. From the calculation, we determined the trends of electronic charge transfers by means of the analysis of the charge density obtained from the self-consistent calculations using the (AIM) theory approach [18] developed by Bader who devised an intuitive way of separating molecules into element atoms as based purely on the electronic charge density.

3. Results and Discussion

The new ternary dicerium selenide dinitride, Ce₂SeN₂, crystallizes in the trigonal space group P-31m (No. 157). The unit cell parameters were found to be a = 4.0772(3) Å, c = 7.0477(7) Å, with α = β = 90 and γ = 120 [11] with Z = 1. Projections of the Ce₂SeN₂ structure are presented in Figure 1. The crystal structure is built up of Ce atoms forming a hexagonal closed packing along the c-axis. The Se atoms occupy all the octahedral voids between every other pair of Ce layers, while the N atoms fill all the tetrahedral voids between the remaining pairs of Ce layers. In addition, each Ce atom is surrounded by three Se atoms and four N atoms [11] (see Figure 1). However, in this work, we also extended our calculation to study the hypothetical compounds like Ce₂SN₂ and Ce₂TeN₂.

The crystal structure contains variable internal coordinates characterizing the 2:1:2 trigonal structure, namely z_Ce and z_N whereby Ce is found at (2d) 1/3, 2/3, z_Ce with z_Ce = 0.7153 and N at (1a) 1/3, 2/3, z_N with 1/3, 2/3, z_N = 0.3744 while X = S, Se, and Te is at (2a) 0, 0, 0 (the experimental lattice and positional parameters can be found in the original literature [11]). Full geometry optimization of all three ternaries showed a preserved crystal symmetry and space group positions and calculated z_Ce and z_N changed by less than 2%. The relaxed structural parameters are reported in

Table 1. Calculated structural parameters, a, c, relaxed atomic parameters, z, formation energy, ΔE_f, and charges, Q of Ce₂SN₂, Ce₂SeN₂, and Ce₂TeN₂ compounds. The elastic constants C_ij, bulk modulus B_H, shear modulus, G_H, and Young’s modulus, E, are also shown.

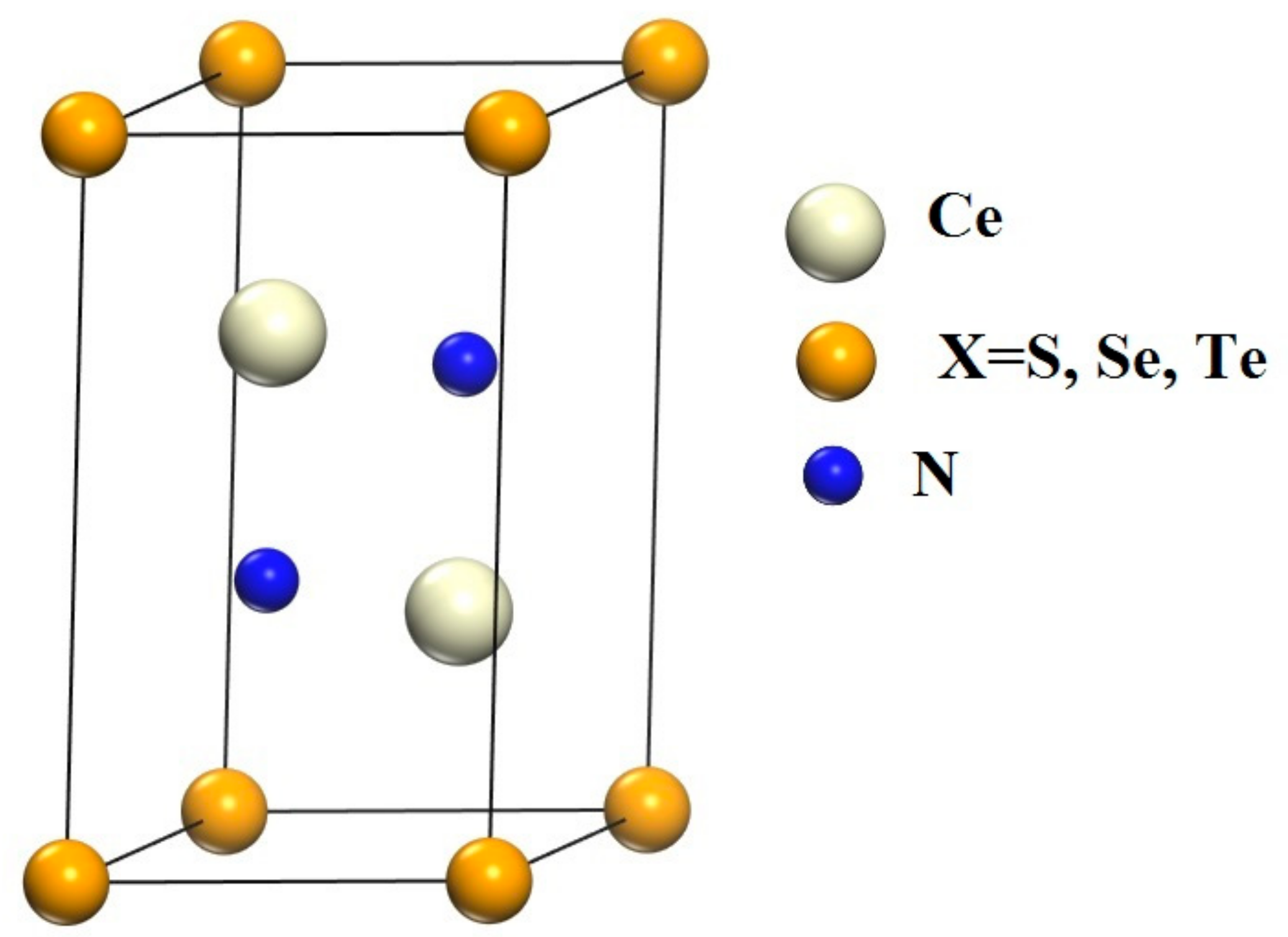
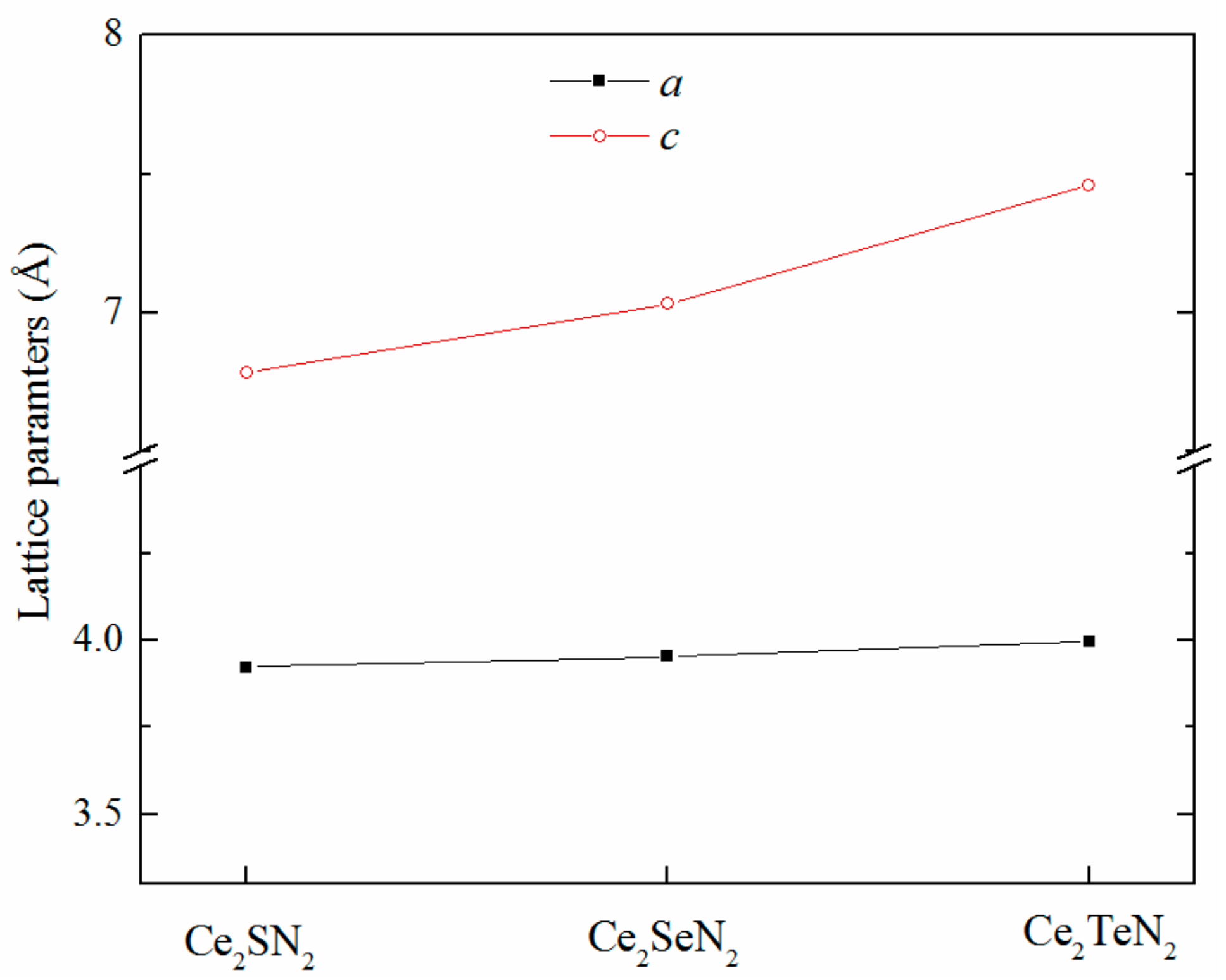
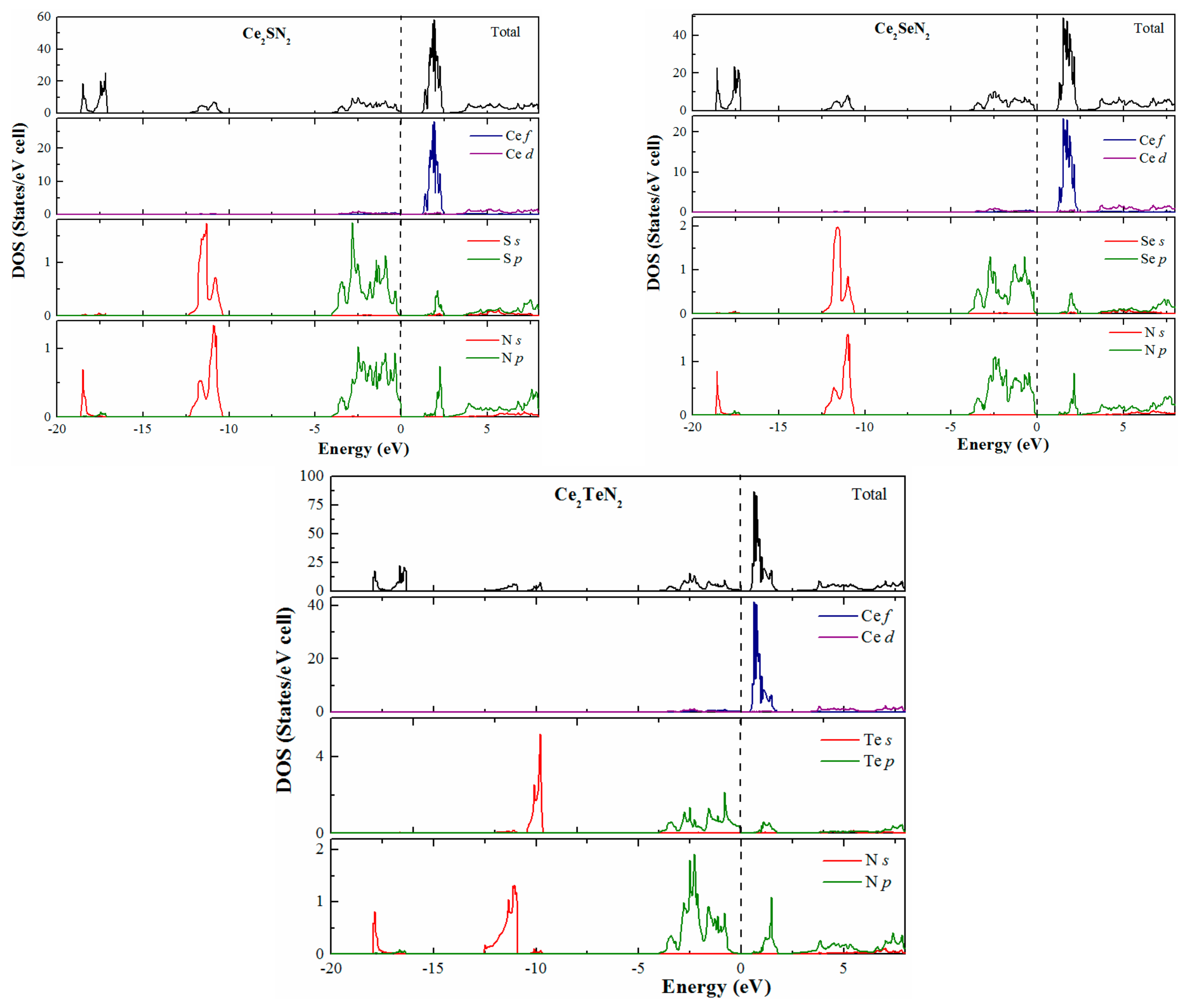
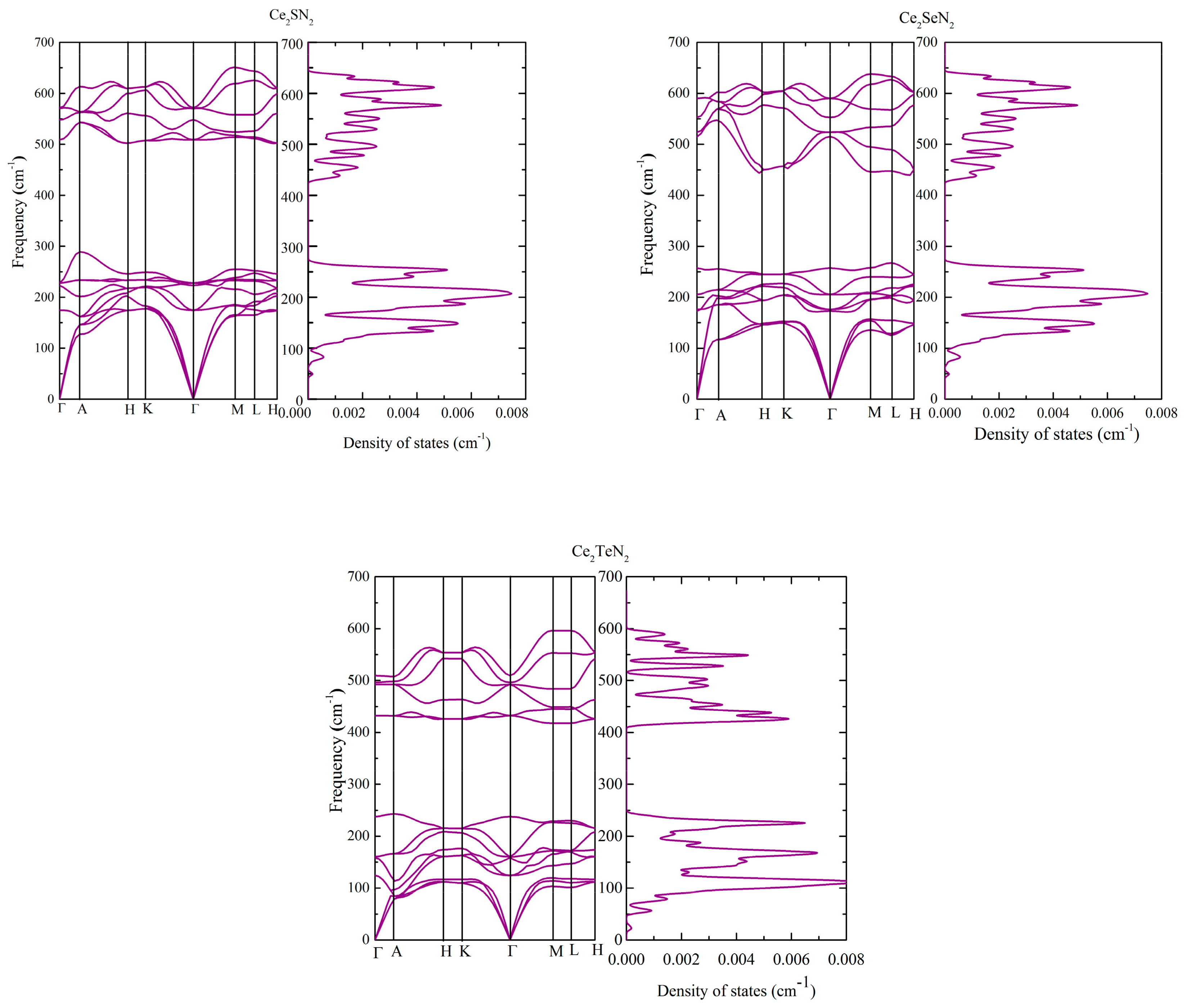
	Ce2SeN2		Ce2SN2	Ce2TeN2
	This work	Exp. [11]	This work
a (Å)	3.953	4.077	3.923	3.996
c (Å)	7.032	7.047	6.785	7.461
zCe,N	zCe = 0.7026 zN = 0.3716	zCe = 0.7153 zN = 0.3744	zCe = 0.7115 zN = 0.3681	zCe = 0.7115 zN = 0.3681
ΔEf (eV)	−2.56		−3.65	−2.58
Q (e)	QCe = +1.96 QSe = −0.99 QN = −1.46		QCe = +1.99 QS = −1.07 QN = −1.45	QCe = +1.84 QTe = −0.56 QN = −1.56
C11 (GPa)	202.9		220.8	183.6
C12 (GPa)	94.3		93.7	86.3
C13 (GPa)	75.1		72.4	83.9
C14 (GPa)	2.9		1.0	6.3
C33 (GPa)	185.7		184.5	207.0
C44 (GPa)	80.6		84.8	76.1
BH (GPa)	119.7		121.6	120.1
GH (GPa)	65.1		71.7	60.0
E (GPa)	165.3		179.7	154.3

along with the experimental results and displayed in Figure 2. In Table 1, it was found that our calculated bond lengths agree well with the experimental data [9]. Our results show that the a is very close, the difference is better observed for c. The trends can be seen more easily: on going from Ce₂SN₂ to Ce₂SeN₂ to Ce₂TeN₂, it is seen that the lattice constants increase (see Figure 2); the increase of electrons number of the chalcogen elements when moving down along the columns of periodic table results in an increase of the lattice parameters. The lattice constant shows an increase in accordance with the large size of the chalcogen atom.

We then extracted the formation energies and the charge transfers of the different compounds from our theoretical calculations. The latter are computed from the charge density analysis with the AIM theory presented above, as reported in Table 1. The charge analysis shows that Ce is the most electropositive elements whereas S, Se, Te, and N elements are more electronegative. The formation energy, ΔE_f, was calculated using the following formulation [19]:

$$\Delta E_f=E_{tot}\left(C{e}_{2}X{N}_2\right)-\frac{1}{2}\left[E_{tot}\left(Ce\right)+E_{tot}\left(X\right)+\frac{1}{2}{E}_{tot}\left(N_2\right)\right]$$

(1)

Here $E_{tot}\left(C{e}_{2}X{N}_2\right)$ is the total energy of the Ce₂XN₂ and, $E_{tot}\left(Ce\right)$, $E_{tot}\left(X\right)$ and $E_{tot}\left(N_2\right)$ represent the isolated atomic energies of the individual metal species in crystalline form and molecular nitrogen N₂, respectively. Our calculated formation energy values are also reported in Table 1. The formation energy values are negative, indicating their intrinsic stability.

To investigate the strength and mechanical stability of Ce₂XN₂, we calculated the stiffness elastic constants C_ij, as shown in Table 1. The elastic stability is checked by the whole set of elastic stiffness constants that satisfies the Born–Huang criterion [20] defined for trigonal structure, i.e., $C_{11}>\left|C_{12}\right|$, $\left(C_{11}+C_{12}\right)C_{33}>2C_{13}^2$, $\left(C_{11}-C_{12}\right)C_{44}>2C_{14}^2$. After obtaining elastic constants, the polycrystalline bulk modulus (B_H), shear modulus (G_H), Young’s modulus (E), and Poisson’s ratio (ν) are calculated from the Voigt–Reuss–Hill (VRH) approximations [21,22]. The calculated polycrystalline elastic constants of ternary nitrides are summarized in Table 1. We notice that Ce₂XN₂ ternaries exhibit a large value of C₁₁ and a small value of C₄₄, implying a low G_H and E despite diving a relatively large B_H.

In order to explain the electronic structure of new ternaries Ce₂XN₂ with X = S, Se, and Te, we calculated the total and projected densities of state curves at the predicted equilibrium lattice constants within the frame work of GGA + U, as shown in Figure 3. The vertical line is the Fermi level (E_F). It can be seen from total DOS graphs that the effect of U on the electronic properties is essentially limited to the unoccupied f band and this does not change the electronic structures especially occupied states [16]. The overall DOS profiles are almost similar for all compounds. Our predicted results indicate that Ce₂XN₂ are an insulator. The PDOS shows several interesting features. At the bottom of the valence band—i.e., between −20 and −15 eV—the first valence band is involved essentially from the N s states. The next bands occur between −13 and −10 eV is mostly due to N s and S/Se/Te s states. The region at the bottom of the valence-band, i.e., between −13 and −8 eV, is originated from S/Se/Te p states and Ce 5d states. The upper region of the valence-band is mostly dominated by N 2p states with contributions of S/Se/Te p states and Ce f states, whereas the narrow empty band situated above the Fermi level is dominated by unoccupied Ce 4f. It can be observed that the N 2p states hybridize strongly with the S/Se/Te p states. The respective energy gaps between the valance-band top and the unoccupied Ce 4f (Ce 5d) band bottom show that the three ternaries are insulating with gap magnitudes of 1.16 eV (2.60 eV), 1.08 (2.43 eV), and 0.35 eV (2.08 eV) for Ce₂SN₂, Ce₂SeN₂, and Ce₂TeN₂ respectively. The calculated electronic structures show that bandgap decreases as atomic number increases from S to Se to Te elements.

The bonding characteristics with Ce₂XN₂ ternary are discussed based on the charge density difference map, as shown in Figure 4. This is calculated by subtracting the electron density of Ce, (S, Se, and Te) and N atoms rigidly separated in the geometry they have in total ternary system [23,24,25]. At self-consistent energy calculations, the charge transfer is from Ce towards N and X in Ce₂XN₂ in agreement with the above computed quantities using the charge density analysis. Similar behavior is observed in the case of CeO₂ [16]. We also show for all compounds that the Ce–N bonds have a strong ionic character as well as a covalent nature due to the interaction between Ce f/X p and N p orbitals. We conclude that ionic bonding is the main determinant of the chemical bonding in Ce₂XN₂, although the covalency is stronger in the sense of orbital participation in forming the valence state.

Ce₂XN₂ has symmetry group of P-3m1 (No. 164), its point group is D_3d (−3m). In the standard notation, the mechanical representation can lead to distinguish of acoustic and optic active modes as: M = 2A_1g + 3A_2u + 3E_u + 2E_g, where two acoustic modes are centered in Γ as Γ_acoustic = A_2u + E_u and eight optic mode as: Γ_optic = 2A_1g + 2A_2u + 2E_u + 2E_g. From these modes, three infrared are active modes: 2A_2u + 2E_u (Acoustic modes not included) and three Raman modes are active as: 2A_1g + 2E_g. When, we employ the polarization selection rules for Raman and Hyper-Raman processes, we found the following Hyper-Raman active modes: 2A_2u + 2E_u (Acoustic modes not included). Calculations of the lattice dynamics of the present nitrides within the first-principles calculations give rich information about the phonon eigenmodes and might be used to support the assignment of the experimentally observed features in the Raman and infrared spectra, when available in the future. In the present work, the calculations of the total energy and Hellmann–Feynman forces were carried out using the CASTEP [26,27]. Vanderbilt ultrasoft pseudopotentials were used to describe the ionic cores. The GGA with PBE [14] functional is adopted. The plane-wave cutoff energy was set at 400 eV. The Brillouin zone integration was sampled using 8 × 8 × 4 k-point grid. We calculated the phonon dispersion relations for Ce₂XN₂ by using the Hellmann–Feynman forces in the small displacement along the optimized structures with the ground-state energy, as shown in Figure 5. In the present calculation, the maximal magnitude of the Hellmann–Feynman forces is less than 0.0004 eV/Å. At Γ point, we observe LO–TO splitting induced by the coupling of phonon modes and the electric field. Furthermore, from all the dispersion curves, no imaginary phonon frequency was observed in the considered BZ which confirms the dynamic stability of the considered nitrides. A large phonon band gap with 175 cm⁻¹, centered on 350 cm⁻¹, is evident for all three nitrides.

4. Conclusions

In summary, we have studied the structural, electronic, and vibrational properties for new ternaries Ce₂SeN₂, Ce₂SN₂, and Ce₂TeN₂ using first-principles DFT+U calculation. It has been shown that the structural parameters determined after relaxation are in good agreement with the experimental data. Our predictions show that all ternaries are energetically and mechanically stable. Interestingly, we find from electronic structures DFT + U calculations that these chalcogenide nitrides are insulators. We also found that the binding in Ce₂XN₂ has a strong ionic character as well as a covalent nature due to the interaction between Ce f/X p and N p orbitals. We have also reported and discussed vibrational spectra. They show the dynamical stability of the considered nitrides.