Energetic Study of Clusters and Reaction Barrier Heights from Efficient Semilocal Density Functionals
Abstract
:1. Introduction
2. Results
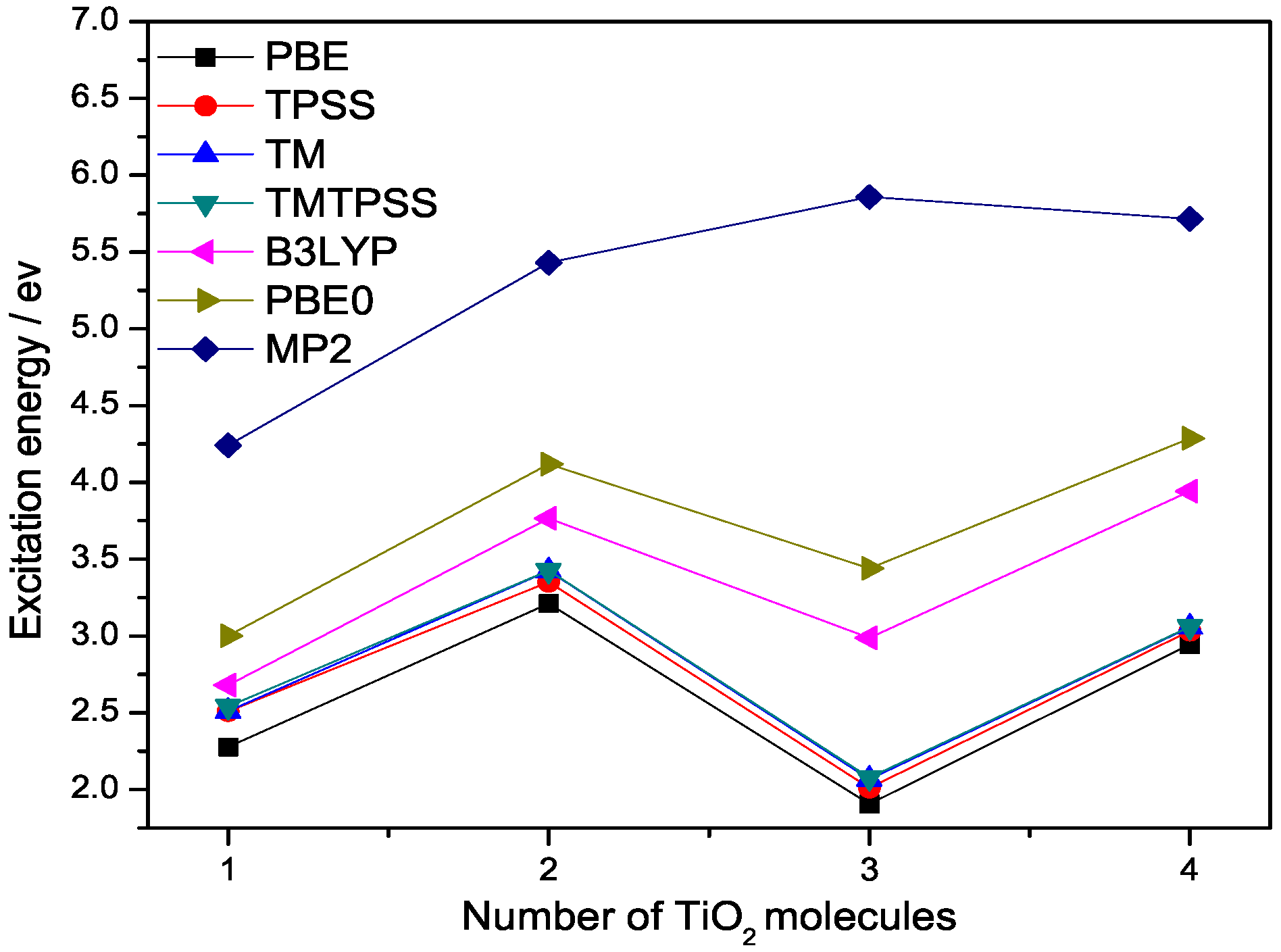
2.1. Binding Energies and Excitation Energy of Titanium Dioxide Cluster
2.2. Binding Energies and Excitation Energy of Water Cluster
2.3. BH76 Barrier Heights
3. Materials and Methods
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133–A1136. [Google Scholar] [CrossRef]
- Parr, R.G.; Yang, W. Density-Functional Theory of Atoms and Molecules; Oxford University Press: Oxford, UK, 1989. [Google Scholar]
- Vosko, H.; Wilk, L.; Nusair, M. Accurate spin-dependent electron liquid correlation energies for local spin density calculations: A critical analysis. Can. J. Phys. 1980, 58, 1200–1201. [Google Scholar] [CrossRef]
- Perdew, J.P.; Wang, Y. Accurate and simple analytic representation of the electron-gas correlation energy. Phys. Rev. B 1992, 45, 13244–13249. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Truhlar, D.G. A new local density functional for main-group thermochemistry, transition metal bonding, thermochemical kinetics, and noncovalent interactions. J. Chem. Phys. 2006, 125, 194101. [Google Scholar] [CrossRef] [PubMed]
- Tao, J.; Perdew, J.P.; Staroverov, V.N.; Scuseria, G.E. Climbing the density functional ladder: Nonempirical meta-generalized gradient approximation designed for molecules and solids. Phys. Rev. Lett. 2003, 91, 146401. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Ruzsinszky, A.; Perdew, J.P. Strongly constrained and appropriately normed semilocal density functional. Phys. Rev. Lett. 2015, 115, 036402. [Google Scholar] [CrossRef] [PubMed]
- Constantin, L.A.; Perdew, J.P.; Tao, J. Meta-generalized gradient approximation for the exchange-correlation hole with an application to the jellium surface energy. Phys. Rev. B 2006, 73, 205104. [Google Scholar] [CrossRef]
- Tao, J.; Springborg, M.; Perdew, J.P. Properties of the exchange hole under an appropriate coordinate transformation. J. Chem. Phys. 2003, 119, 6457–6502. [Google Scholar] [CrossRef]
- Tao, J.; Mo, Y. Accurate semilocal density functional for condensed-matter physics and quantum chemistry. Phys. Rev. Lett. 2016, 117, 073001. [Google Scholar] [CrossRef] [PubMed]
- Mo, Y.; Tian, G.; Car, R.; Staroverov, V.N.; Scuseria, G.E.; Tao, J. Performance of a nonempirical density functional on molecules and hydrogen-bonded complexes. J. Chem. Phys. 2016, 145, 234306. [Google Scholar] [CrossRef] [PubMed]
- Mo, Y.; Car, R.; Staroverov, V.N.; Scuseria, G.E.; Tao, J. Assessment of a nonempirical semilocal density functional on solids and surfaces. Phys. Rev. B. 2017, 95, 035118. [Google Scholar] [CrossRef]
- Gratzel, M. Photoelectrochemical cells. Nature 2001, 414, 338–344. [Google Scholar] [CrossRef] [PubMed]
- Tyo, E.C.; Vajda, S. Catalysis by clusters with precise numbers of atoms. Nature Nanotech. 2015, 10, 577–588. [Google Scholar] [CrossRef] [PubMed]
- Cernuto, G.; Masciocchi, N.; Cervellino, A.; Colonna, G.M.; Guagliardi, A. Size and shape dependence of the photocatalytic activity of TiO2 nanocrystals: A total scattering Debye function study. J. Am. Chem. Soc. 2011, 133, 3114–3119. [Google Scholar] [CrossRef] [PubMed]
- Cho, D.; Ko, K.C.; Lamiel-Garcia, O. Effect of size and structure on the ground-state and excited-state electronic structure of TiO2 Nanoparticles. J. Chem. Theory Comput. 2016, 12, 3751–3763. [Google Scholar] [CrossRef] [PubMed]
- Mo, Y.; Tian, G.; Tao, J. Performance of a nonempirical exchange functional from the density matrix expansion: Comparative study with different correlation. Phys. Chem. 2017. resubmitted. [Google Scholar]
- Tian, G.; Mo, Y.; Tao, J. Performance of a nonempirical semilocal density-functional on noncovalent interactions. J. Chem. Phys. 2017. resubmitted. [Google Scholar]
- Qu, Z.; Kroes, G.J. Theoretical study of the electronic structure and stability of titanium dioxide clusters (TiO2)n with n = 1–9. J. Phys. Chem. B 2006, 110, 8998–9007. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Wang, L.S. Electronic structure of titanium oxide clusters: TiOy (y = 1–3) and (TiO2)n (n = 1–4). J. Chem. Phys. 1997, 107, 8221–8228. [Google Scholar] [CrossRef]
- Zhai, H.J.; Wang, L.S. Probing the Electronic Structure and Band Gap Evolution of Titanium Oxide Clusters (n = 1–10) Using Photoelectron Spectroscopy. J. Am. Chem. Soc. 2007, 129, 3022–3026. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Armstrong, G. Supercooled water: Ice maybe. Nat. Chem. 2010, 2, 256. [Google Scholar] [CrossRef]
- Moore, E.B.; Molinero, V. Structural transformation in supercooled water controls the crystallization rate of ice. Nature 2011, 479, 506–508. [Google Scholar] [CrossRef] [PubMed]
- Xantheas, S.S.; Burnham, C.J.; Harrison, R.J. Development of transferable interaction models for water. II. Accurate energetics of the first few water clusters from first principles. J. Chem. Phys. 2002, 116, 1493–1499. [Google Scholar] [CrossRef]
- Liu, K.; Cruzan, J.D.; Saykally, R.J. Water clusters. Science 1996, 271, 929–931. [Google Scholar] [CrossRef]
- Pradzynski, C.C.; Forck, R.M.; Zeuch, T.; Slavíček, P.; Buck, U. A fully size-resolved perspective on the crystallization of water clusters. Science 2012, 337, 1529–1532. [Google Scholar] [CrossRef] [PubMed]
- Gillan, M.J.; Alfé, D.; Michaelides, A. Perspective: How good is DFT for water? J. Chem. Phys. 2016, 144, 130901. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S. Accurate description of van der Waals complexes by density functional theory including empirical corrections. J. Comput. Chem. 2004, 25, 1463–1473. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 2006, 27, 1787–1799. [Google Scholar] [CrossRef] [PubMed]
- Santra, B.; Michaelides, A.; Fuchs, M.; Tkatchenko, A.; Filippi, C.; Scheffler, M. On the accuracy of density-functional theory exchange-correlation functionals for H bonds in small water clusters. II. The water hexamer and van der Waals interactions. J. Chem. Phys. 2008, 129, 194111. [Google Scholar] [CrossRef] [PubMed]
- Hahn, P.H.; Schmidt, W.G.; Seino, K.; Preuss, M.; Bechstedt, F.; Bernholc, J. Optical absorption of water: Coulomb effects versus hydrogen bonding. Phys. Rev. Lett. 2005, 94, 037404. [Google Scholar] [CrossRef] [PubMed]
- Fang, C.; Li, W.-F.; Koster, R.S.; Klime, J.; van Blaaderen, A.; van Huis, M.A. The accurate calculation of the band gap of liquid water by means of GW corrections applied to plane-wave density functional theory molecular dynamics simulations. Phys. Chem. Chem. Phys. 2015, 17, 365–375. [Google Scholar] [CrossRef] [PubMed]
- Warren, S.G. Optical constants of ice from the ultraviolet to the microwave. Appl. Opt. 1984, 23, 1206–1225. [Google Scholar] [CrossRef] [PubMed]
- Minton, A.P. Far-ultraviolet spectrum of ice. J. Phys. Chem. 1971, 75, 1162–1164. [Google Scholar] [CrossRef]
- Painter, L.R.; Birkhoff, R.D.; Arakawa, E.T. Collective oscillation in liquid water. J. Chem. Phys. 1969, 51, 243. [Google Scholar] [CrossRef]
- Seki, M.; Kobayashi, K.; Nakahara, J. Optical spectra of hexagonal ice. J. Phys. Soc. Jpn. 1981, 50, 2643–2648. [Google Scholar] [CrossRef]
- Shibaguchi, T.; Onuki, H.; Onaka, R. Electronic structures of water and ice. J. Phys. Soc. Jpn. 1977, 42, 152–154. [Google Scholar] [CrossRef]
- Engel, E.A.; Monserrat, B.; Needs, R.J. Vibrational renormalisation of the electronic band gap in hexagonal and cubic ice. J. Chem. Phys. 2015, 143, 244708. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Gonzlez-Garca, N.; Truhlar, D.G. Benchmark Database of barrier heights for heavy atom transfer, nucleophilic substitution, association, and unimolecular reactions and its use to test theoretical methods. J. Phys. Chem. A 2005, 109, 2012–2018. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Haunschild, R.; Xiao, B.; Bulik, I.W.; Scuseria, G.E.; Perdew, J.P. Semilocal and hybrid meta-generalized gradient approximations based on the understanding of the kinetic-energy-density dependence. J. Chem. Phys. 2013, 138, 044113. [Google Scholar] [CrossRef] [PubMed]
- Cohen, A.J.; Mori-Snchez, P.; Yang, W. Challenges for Density Functional Theory. Chem. Rev. 2012, 112, 289. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision A.02; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Levy, M.; Perdew, J.P. Hellmann-Feynman, virial, and scaling requisites for the exact universal density functionals. Shape of the correlation potential and diamagnetic susceptibility for atoms. Phys. Rev. A 1985, 32, 2010. [Google Scholar] [CrossRef]
- Oliver, G.L.; Perdew, J.P. Spin-density gradient expansion for the kinetic energy. Phys. Rev. A 1979, 20, 397. [Google Scholar] [CrossRef]
- Johnson, E.R.; Becke, A.D.; Sherrill, C.D.; DiLabio, G.A. Oscillations in meta-generalized-gradient approximation potential energy surfaces for dispersion-bound complexes. J. Chem. Phys. 2009, 131, 034111. [Google Scholar] [CrossRef] [PubMed]


| N | PBE | TPSS | TM | TMTPSS | B3LYP | PBE0 | MP2 |
|---|---|---|---|---|---|---|---|
| 2 | 55.54 | 56.94 | 59.37 | 59.09 | 57.49 | 59.55 | 73.97 |
| 3 | 74.16 | 76.18 | 79.79 | 79.45 | 77.09 | 80.11 | 98.75 |
| 4 | 84.38 | 87.28 | 91.42 | 91.00 | 87.86 | 91.67 | 113.74 |
| ME | 24.13 | 22.02 | 18.63 | 18.97 | 21.34 | 18.38 | |
| MAE | 24.13 | 22.02 | 18.63 | 18.97 | 21.34 | 18.38 |
| N | PBE | PBE-D3BJ | TPSS | TPSS-D3BJ | TM | TMTPSS | B3LYP | PBE0 | MP2 |
|---|---|---|---|---|---|---|---|---|---|
| 2 | |||||||||
| 3 | −17.05 | −18.44 | −15.72 | −17.43 | −16.29 | −15.56 | −14.97 | −16.42 | −15.82 |
| 4 | −30.64 | −32.92 | −28.65 | −31.43 | −28.22 | −26.91 | −27.04 | −29.35 | −27.63 |
| 5 | −40.41 | −43.29 | −37.97 | −41.45 | −36.77 | −35.08 | −35.74 | −38.75 | −36.31 |
| ME | −2.19 | −3.93 | −0.65 | −2.77 | −0.39 | 0.59 | 0.54 | −1.27 | |
| MAE | 2.19 | 3.93 | 0.70 | 2.77 | 0.39 | 0.59 | 0.54 | 1.27 |
| Method | LSDA | PBE | TPSS | TM | TMTPSS | B3LYP | M06L | PBE0 | SCAN |
|---|---|---|---|---|---|---|---|---|---|
| ME | −14.78 | −8.66 | −8.14 | −7.08 | −6.86 | −4.15 | −3.9 | −3.68 | −7.7 |
| MAE | 14.88 | 8.71 | 8.17 | 7.08 | 6.86 | 4.28 | 4.1 | 3.99 | 7.7 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, G.; Mo, Y.; Tao, J. Energetic Study of Clusters and Reaction Barrier Heights from Efficient Semilocal Density Functionals. Computation 2017, 5, 27. https://doi.org/10.3390/computation5020027
Tian G, Mo Y, Tao J. Energetic Study of Clusters and Reaction Barrier Heights from Efficient Semilocal Density Functionals. Computation. 2017; 5(2):27. https://doi.org/10.3390/computation5020027
Chicago/Turabian StyleTian, Guocai, Yuxiang Mo, and Jianmin Tao. 2017. "Energetic Study of Clusters and Reaction Barrier Heights from Efficient Semilocal Density Functionals" Computation 5, no. 2: 27. https://doi.org/10.3390/computation5020027
APA StyleTian, G., Mo, Y., & Tao, J. (2017). Energetic Study of Clusters and Reaction Barrier Heights from Efficient Semilocal Density Functionals. Computation, 5(2), 27. https://doi.org/10.3390/computation5020027





