Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials
Abstract
1. Introduction
2. Numerical Solution
2.1. Polymer Thermoelastic Solution (PTES)
2.2. Fractional Size- and Temperature-Dependent Solution (FSTDS)
2.3. Nonlinear Nonlocal Elasticity Solution (NNES)
- (I)
- The nonlocal kernel must depend on the internal length;
- (II)
- ;
- (II)
- It should satisfy , .
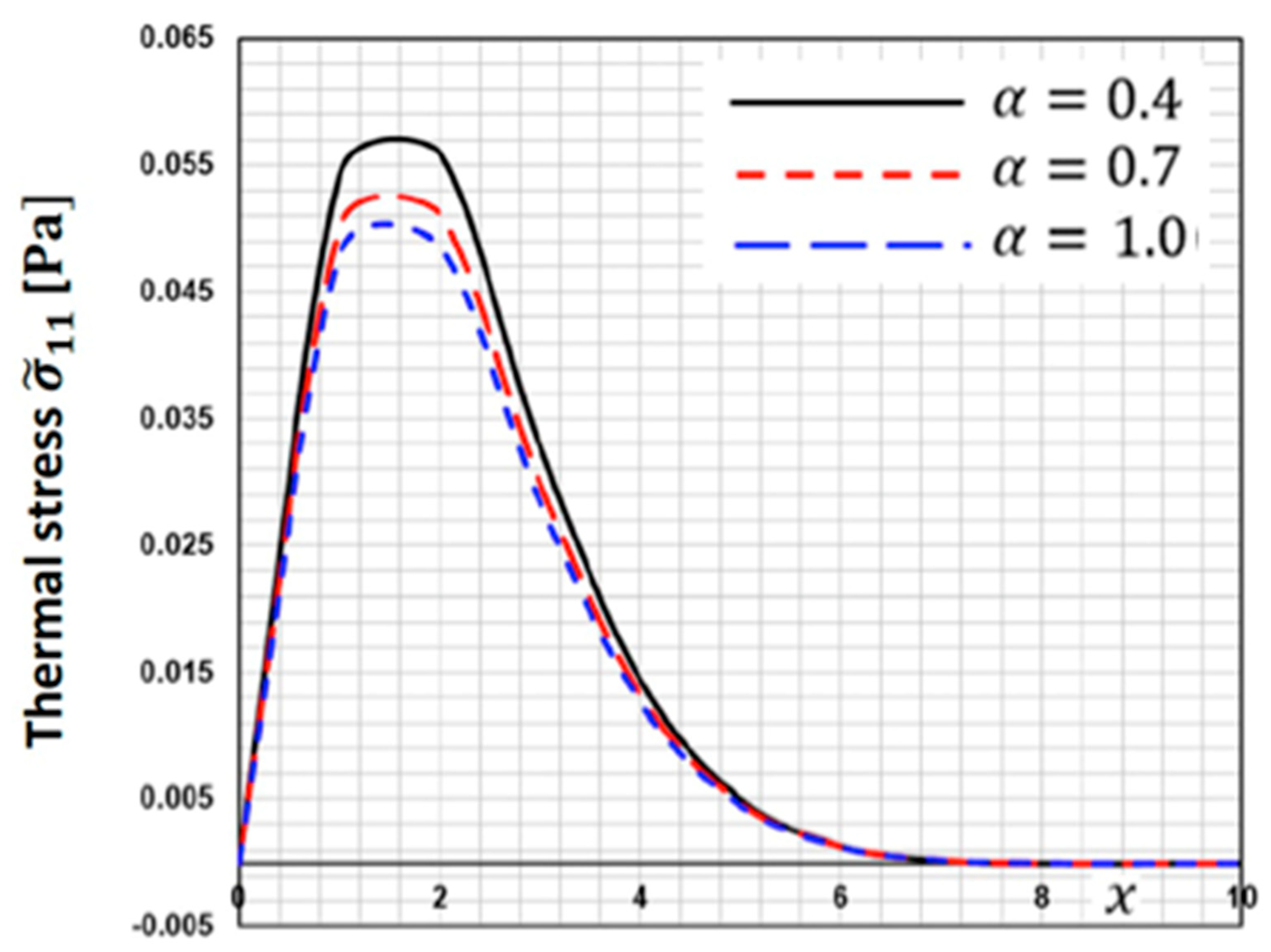
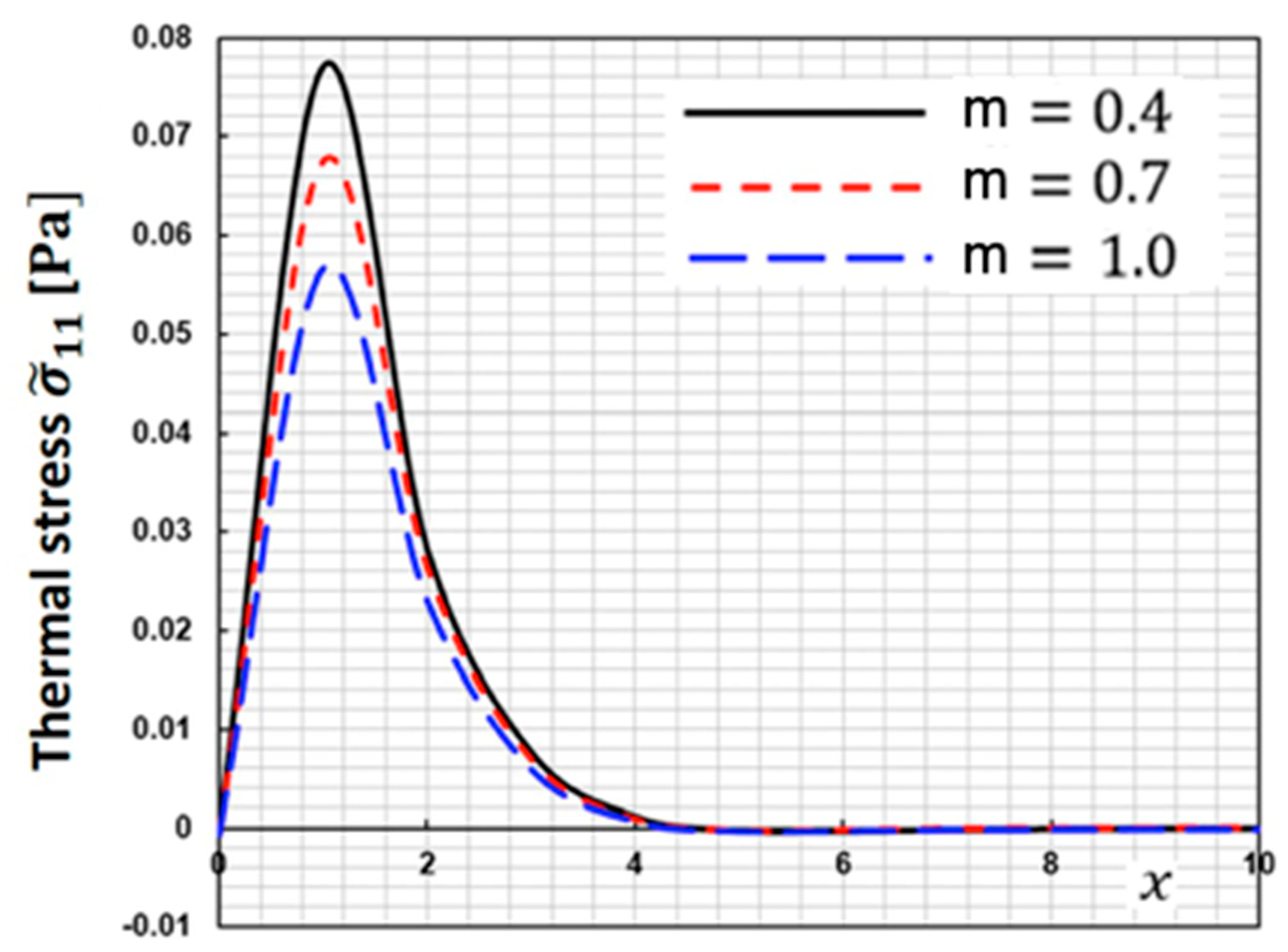
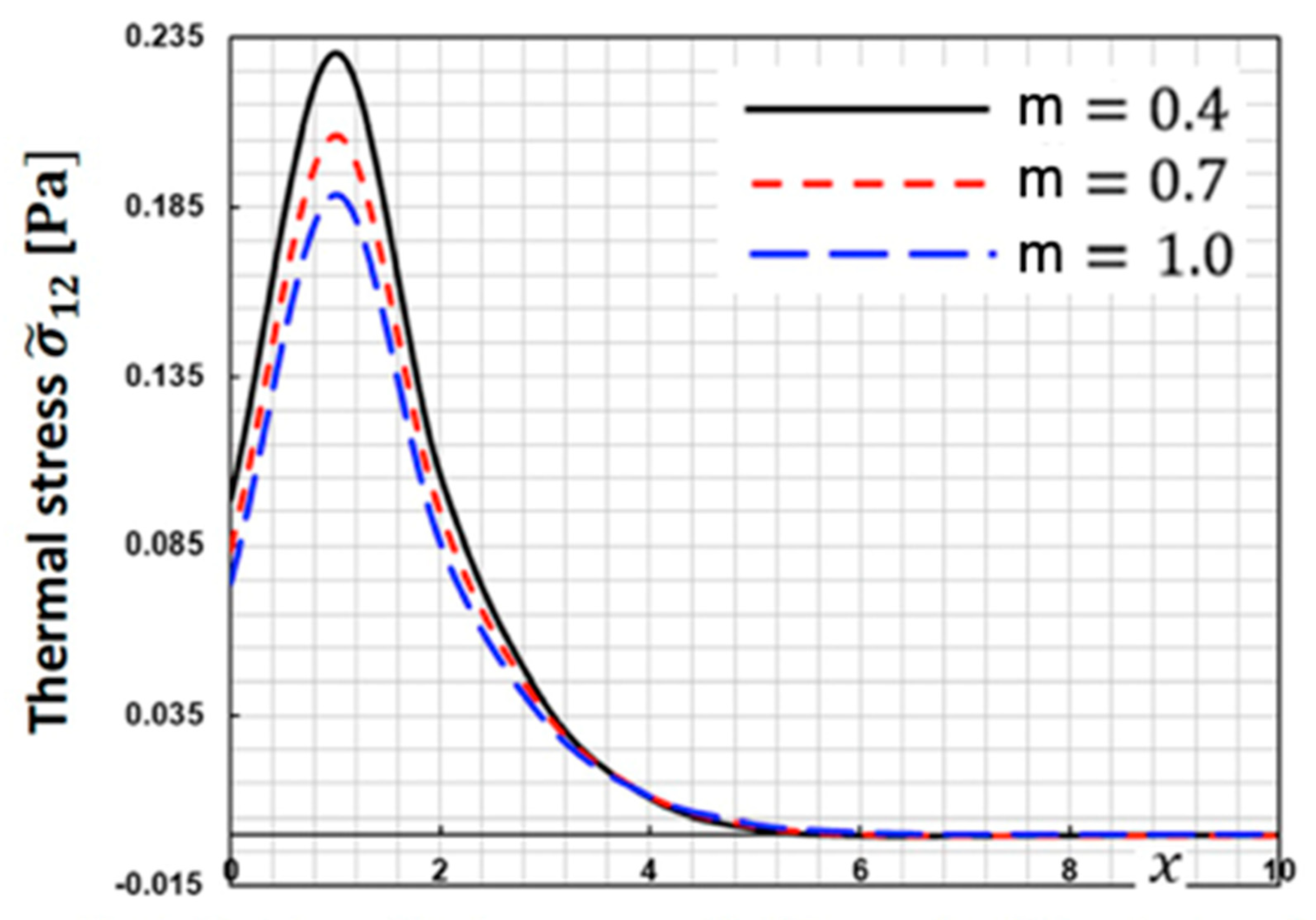
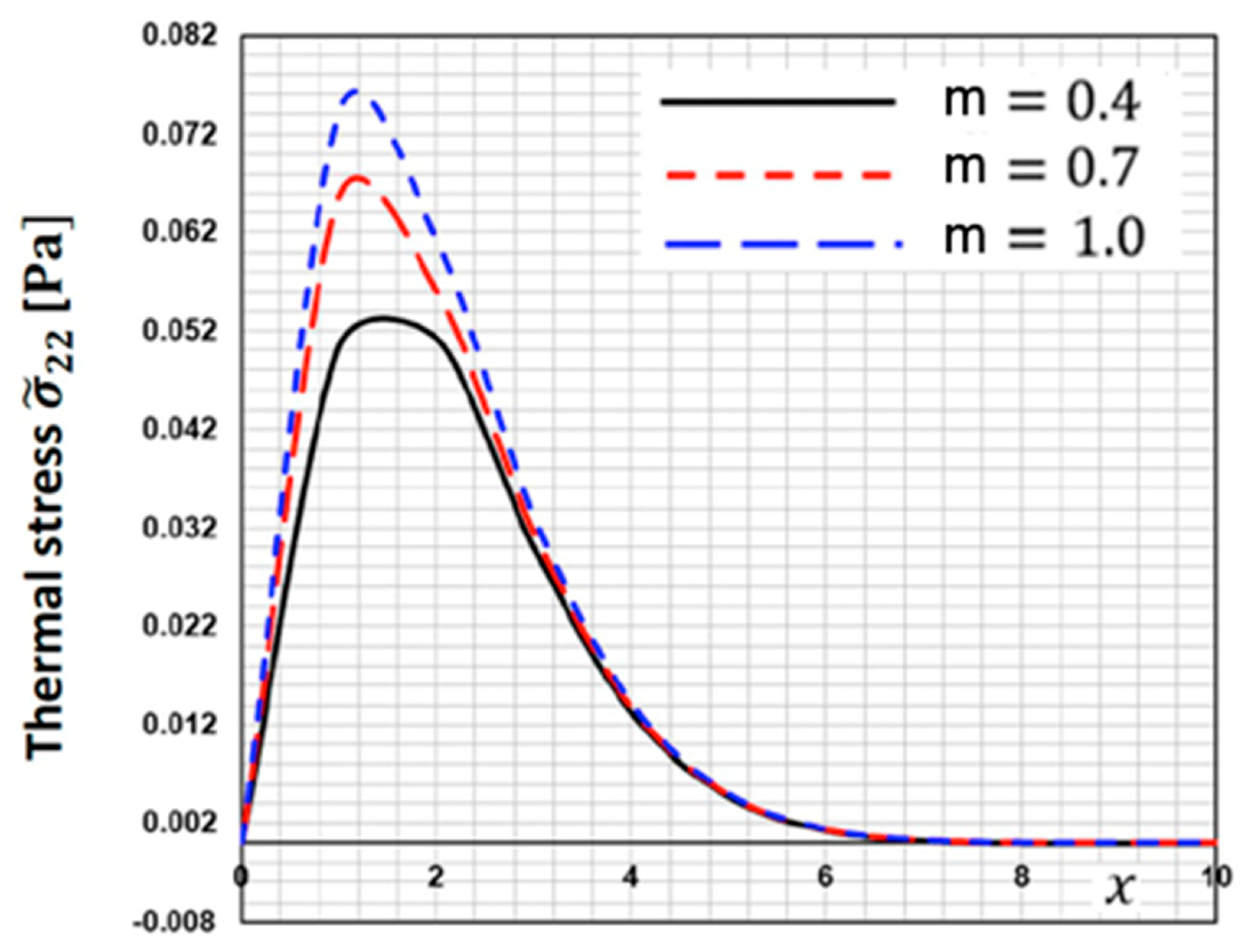
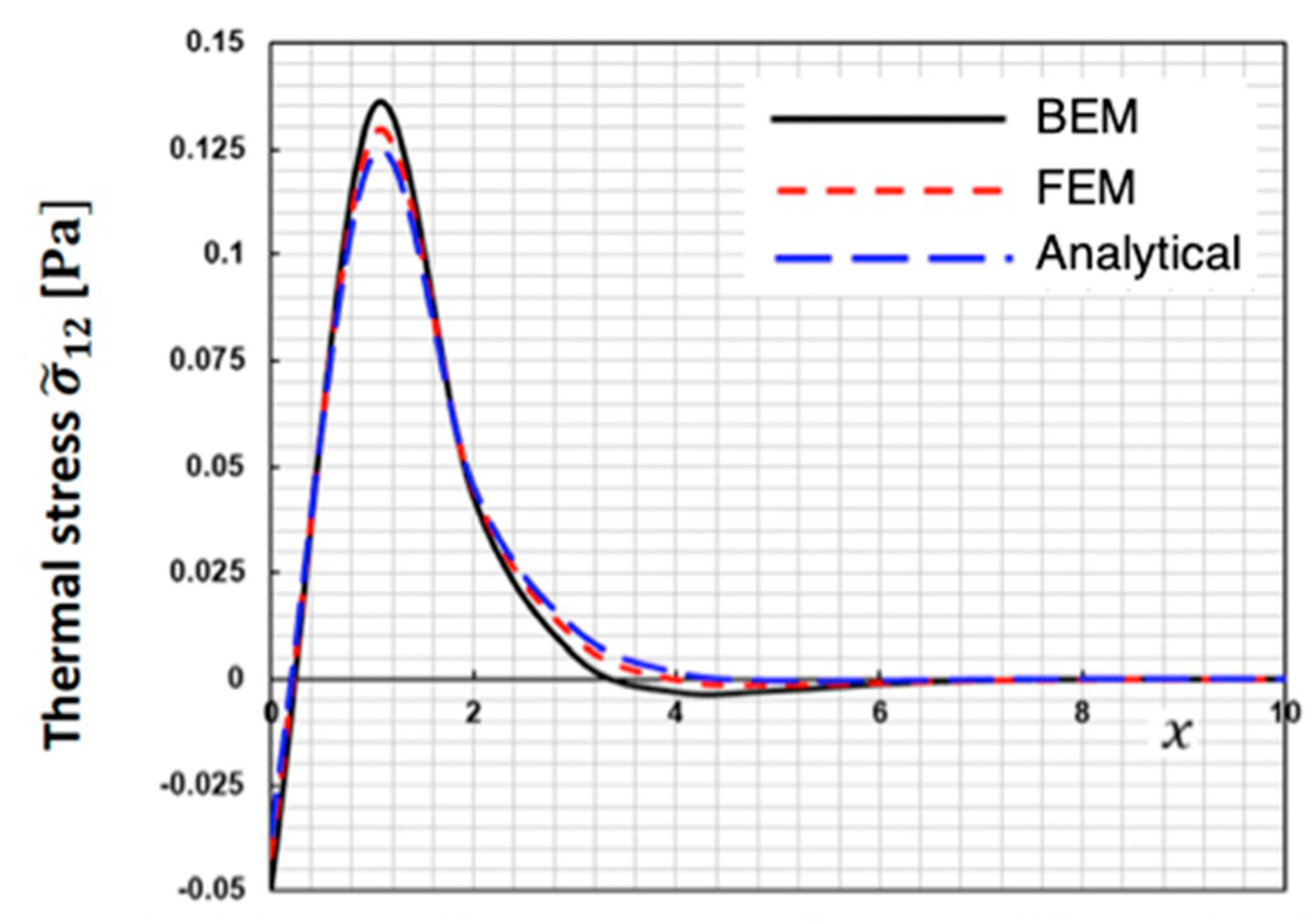
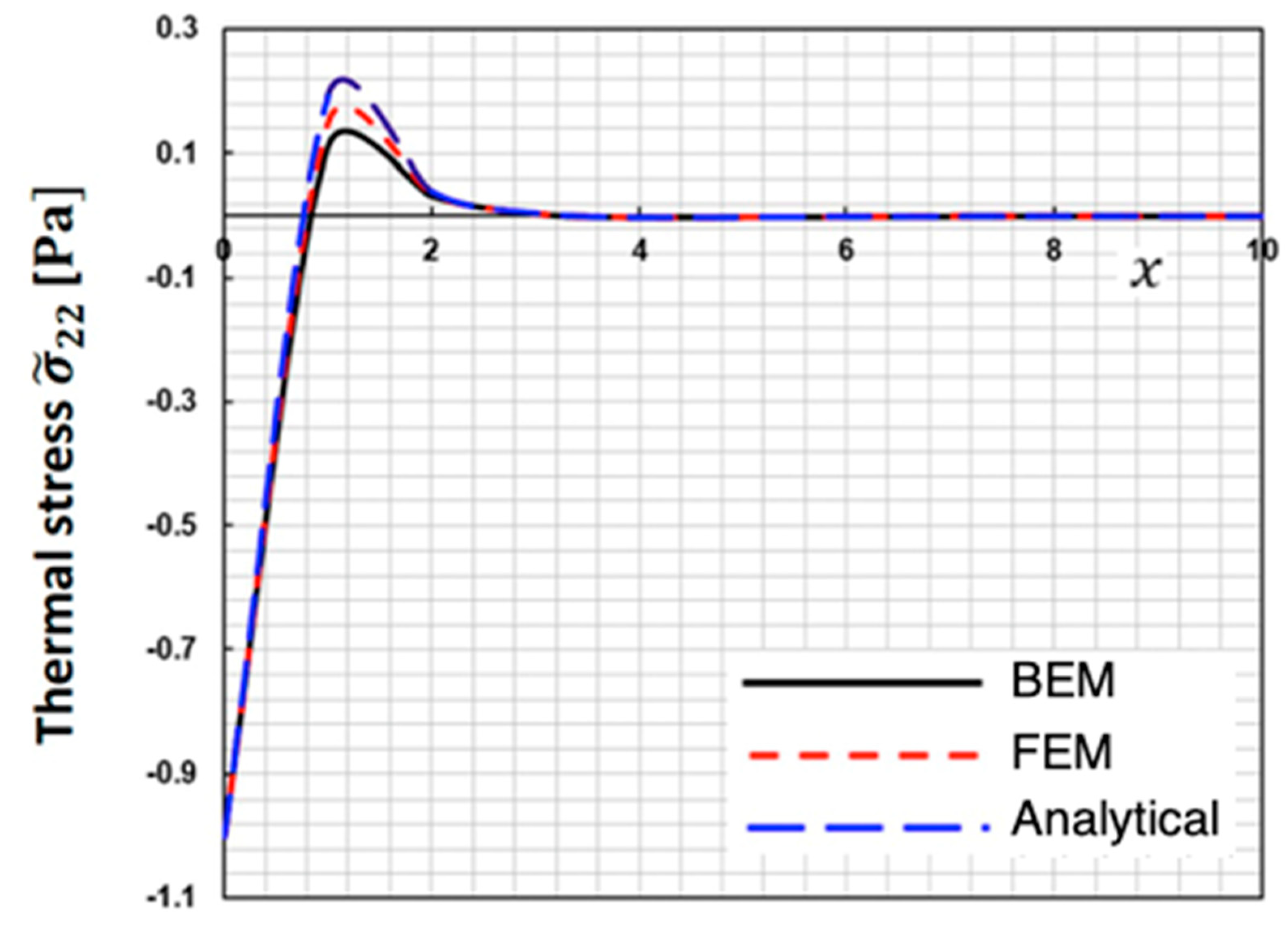
3. Numerical Results and Discussion
4. Conclusions
- A new fractional boundary element model was used to solve the nonlinear nonlocal size- and temperature-dependent thermoelastic problems of anisotropic fibrous polymer nanomaterials.
- Th proposed BEM technique was used first to solve the anisotropic fibrous polymer nanoparticle problem. Then, we used the solution of the anisotropic fibrous polymer nanomaterial problem to solve the nonlinear nonlocal thermoelasticity problem.
- The nonlocal elastic technique separates the displacement field into a complementary component and a particular component.
- The overall displacement is obtained using the boundary element technique, which solves a Navier-type problem, whereas the individual displacement is derived using local radial points.
- The new modified shift-splitting (NMSS) technique which reduces memory and processing time requirements was used to solve linear systems created by BEM.
- The numerical findings were depicted graphically to display the influences of the fractional and graded parameters on the thermal stresses of anisotropic fibrous polymer nanomaterials.
- The numerical findings also show the differences between the regularized, generalized modified shift-splitting, and new modified shift-splitting iterative methods, and they verified the validity, accuracy, and effectiveness of the developed fractional boundary element technique.
- The main advantages of the current HBEM model are its generality and simplicity. The numerical findings supported the claim that the proposed method offers more advantages than other domain discretization techniques.
- A comparison of the computational resources needed to solve nonlinear nonlocal thermoelastic problems of anisotropic fibrous polymer nanomaterials was performed for current fractional BEM and the finite element method (FEM).
- The findings of this paper contribute to the development of mathematical models that can be applied in food packaging, phones, soda and water bottles, films, agriculture, biomedical devices, coating, paints, blending, airplanes, textile fibers, the automotive industry, consumer goods, industrial, recreational vehicles, effective actuators, fluorescence imaging, photodynamic therapy, hydrogels, electronic devices, engineering resins and polyolefins, and computers, among others.
- In future work, we suggest expanding the boundary element technique proposed in this research for applications in three-dimensional thermoelastic problems of anisotropic fibrous polymer nanomaterials, which include multilayer difficulties, complex geometries, and the inclusion of convective factors.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Thermal expansion | Pseudo mean curvature tensor | ||
| Thermal shear strain | Internal length of considered material | ||
| Boundary | Couple traction | ||
| Kronecker delta function | Monomials number | ||
| Strain tensor | True couple-stress vector | ||
| Couple stress parameter | Pseudo couple-stress tensor | ||
| Yield stress | Functionally graded parameter | ||
| Total force-stress tensor | Outward unit normal vector | ||
| Symmetric force-stress tensor | Nodes number | ||
| Skew-symmetric force-stress tensor | P | Pressure | |
| Rotation | Point couple kernel function | ||
| Spherical region | Monomials | ||
| Shape parameters | External heat source | ||
| Heat capacity | Euclidian distance | ||
| Point force kernel function | Radius of spherical region | ||
| Fourth-order constant stiffness tensor | Radial basis function | ||
| Young’s modulus | Traction | ||
| Body force vector | Displacement vector | ||
| Strain hardening | Kelvin fundamental solution | ||
| I | Identity tensor | Poisson’s ratio | |
| Boltzmann’s constant | Evaluation point | ||
| Mean curvature vector | Center point | ||
| Field point |
References
- Kong, B.; Liu, R.; Guo, J.; Lu, L.; Zhou, Q.; Zhao, Y. Tailoring micro/nano-fibers for biomedical applications. Bioact. Mater. 2023, 19, 328–347. [Google Scholar] [CrossRef]
- Deng, R.; Luo, Z.; Rao, Z.; Lin, Z.; Chen, S.; Zhou, J.; Zhu, Q.; Liu, X.; Bai, Y.; Quan, D. Decellularized extracellular matrix containing electrospun fibers for nerve regeneration: A comparison between core–shell structured and preblended composites. Adv. Fiber Mater. 2022, 4, 503–519. [Google Scholar] [CrossRef]
- Lu, C.; Shao, H.; Bi, S.; Chen, N.; Shao, G.; Jiang, J. Estimation of transverse thermoelastic properties of polyimide fibers based on micromechanical models. Compos. Sci. Technol. 2024, 250, 110541. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Ahmad, H.; Nofal, T.A.; Abu-Zinadah, H. Thermo-viscoelastic fractional model of rotating nanobeams with variable thermal conductivity due to mechanical and thermal loads. Mod. Phys. Lett. B 2021, 35, 2150297. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Marin, M. The size-dependent thermoelastic vibrations of nanobeams subjected to harmonic excitation and rectified sine wave heating. Mathematics. Mathematics 2020, 8, 1128. [Google Scholar] [CrossRef]
- Huang, K.; Xu, W. A Nonlinear Nonlocal Thermoelasticity Euler–Bernoulli Beam Theory and Its Application to Single-Walled Carbon Nanotubes. Nanomaterials 2023, 13, 721. [Google Scholar] [CrossRef] [PubMed]
- Pryjmaková, J.; Kaimlová, M.; Hubáček, T.; Švorčík, V.; Siegel, J. Nanostructured materials for artificial tissue replacements. Int. J. Mol. Sci. 2020, 21, 2521. [Google Scholar] [CrossRef]
- Mosaad, K.E.; Shoueir, K.R.; Saied, A.H.; Dewidar, M.M. New prospects in nano phased co-substituted hydroxyapatite enrolled in polymeric nanofiber mats for bone tissue engineering applications. Ann. Biomed. Eng. 2021, 49, 2006–2029. [Google Scholar] [CrossRef] [PubMed]
- Li, C.; Lu, Y.; He, T. An investigation into size-dependent dynamic mechanical-diffusion responses of multi-layered laminated sandwich-like nanocomposites under shock loadings of molar concentration for vibration control based on nonlocal diffusion-elasticity theory. Mech. Adv. Mater. Struct. 2023, 30, 647–660. [Google Scholar] [CrossRef]
- Tchepemen, N.; Balasubramanian, S.; Kanagaraj, N.; Kengne, E. Modulational instability in a coupled nonlocal media with cubic, quintic and septimal nonlinearities. Nonlinear Dyn. 2023, 111, 20311–20329. [Google Scholar] [CrossRef]
- Arash, B.; Unger, R.; Exner, W.; Rolfes, R. A finite deformation gradient-enhanced damage model for nanoparticle/polymer nanocomposites: An atomistically-informed multiscale approach. Compos. Struct. 2021, 258, 113211. [Google Scholar] [CrossRef]
- Soleiman, A.; Abouelregal, A.E.; Fahmy, M.A.; Sedighi, H.M. Thermomechanical Behavior of Functionally Graded Nanoscale Beams Under Fractional Heat Transfer Model with a Two-Parameter Mittag-Leffler Function. Iran. J. Sci. Technol. Trans. Mech. Engineering. 2023. [Google Scholar] [CrossRef]
- Fahmy, M.A. A new BEM modeling algorithm for size-dependent thermopiezoelectric problems in smart nanostructures. CMC-Comput. Mater. Contin. 2021, 69, 931–944. [Google Scholar] [CrossRef]
- Fahmy, M.A. Fractional Temperature-Dependent BEM for Laser Ultrasonic Thermoelastic Propagation Problems of Smart Nanomaterials. Fractal Fract. 2023, 7, 536. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Rabih, M.N.A.; Alharbi, H.A.; Megahid, S.F. A modified couple stress model to analyze the effect of size-dependent on thermal interactions in rotating nanobeams whose properties change with temperature. Math. Mech. Solids 2024. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Mohamed, B.O.; Sedighi, H.M. Thermoelastic deformation properties of non-localized and axially moving viscoelastic Zener nanobeams. Adv. Nano Res. 2024, 16, 141–154. [Google Scholar] [CrossRef]
- Fahmy, M.A. A new boundary element formulation for modeling and simulation of three-temperature distributions in carbon nanotube fiber reinforced composites with inclusions. Math. Methods Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Fahmy, M.A. 3D Boundary Element Model for Ultrasonic Wave Propagation Fractional Order Boundary Value Problems of Functionally Graded Anisotropic Fiber-Reinforced Plates. Fractal Fract. 2022, 6, 247. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Almehmadi, M.M. Boundary element analysis of rotating functionally graded anisotropic fiber-reinforced magneto-thermoelastic composites. Open Eng. 2022, 12, 313–322. [Google Scholar] [CrossRef]
- Fahmy, M.A. Three-Dimensional Boundary Element Strategy for Stress Sensitivity of Fractional-Order Thermo-Elastoplastic Ultrasonic Wave Propagation Problems of Anisotropic Fiber-Reinforced Polymer Composite Material. Polymers 2022, 14, 2883. [Google Scholar] [CrossRef]
- Ren, B.; Wu, C.T.; Seleson, P.; Zeng, D.; Nishi, M.; Pasetto, M. An FEM-Based Peridynamic Model for Failure Analysis of Unidirectional Fiber-Reinforced Laminates. J. Peridynamics Nonlocal Model. 2022, 4, 139–158. [Google Scholar] [CrossRef]
- Eshmatov, B.K.; Abdikarimov, R.; Amabili, M.; Vatin, N.I. Nonlinear vibrations and dynamic stability of viscoelastic anisotropic fiber reinforced plates. Mag. Civ. Eng. 2023, 118, 11811. [Google Scholar] [CrossRef]
- Rubinstein, M.; Panyukov, S. Elasticity of Polymer Networks. Macromolecules 2002, 35, 6670–6686. [Google Scholar] [CrossRef]
- Hematiyan, M.R. Exact transformation of a wide variety of domain integrals into boundary integrals in boundary element method. Commun. Numer. Methods Eng. 2008, 24, 1497–1521. [Google Scholar] [CrossRef]
- Hadjesfandiari, A.R.; Dargush, G.F. Fundamental solutions for isotropic size-dependent couple stress elasticity. Int. J. Solids Struct. 2013, 50, 1253–1265. [Google Scholar] [CrossRef]
- Lazar, M.; Maugin, G.A.; Aifantis, E.C. On a theory of nonlocal elasticity of biHelmholtz type and some applications. Int. J. Solids Struct. 2006, 43, 1404–1421. [Google Scholar] [CrossRef]
- Polizzotto, C.; Fushi, P.; Pisano, A.A. A strain-difference-based nonlocal elasticity model. Int. J. Solids Struct. 2004, 41, 2383–2401. [Google Scholar] [CrossRef]
- Liu, G.R.; Gu, Y.T. A meshfree method: Meshfree weak-strong (MWS) form method for 2-D solids. Comput. Mech. 2003, 33, 2–14. [Google Scholar] [CrossRef]
- Schwartz, M.; Niane, N.; Njiwa, R.K. A simple solution method to 3D integral nonlocal elasticity: Isotropic-BEM coupled with strong form local radial point interpolation. Eng. Anal. Bound. Elements 2011, 36, 606–612. [Google Scholar] [CrossRef]
- Brebbia, C.A.; Dominguez, J. Boundary Elements. An Introductory Course; Computational Mechanics Publications: Southampton, UK, 1992. [Google Scholar]
- Liu, G.R.; Gu, Y.T. A local radial point interpolation method (LRPIM) for free vibration analyses of 2-D solids. J. Sound Vib. 2001, 246, 29–46. [Google Scholar] [CrossRef]
- Badahmane, A. Regularized preconditioned GMRES and the regularized iteration method. Appl. Numer. Math. 2020, 152, 159–168. [Google Scholar] [CrossRef]
- Victoria, L.; del Castillo, C.D.; Rinaldi, C. Effect of Sample Concentration on the Determination of the Anisotropy Constant of Magnetic Nanoparticles. IEEE Trans. Magn. 2010, 46, 852–859. [Google Scholar] [CrossRef]
- Huang, Z.G.; Wang, L.G.; Xu, Z.; Cui, J.J. The generalized modified shift-splitting preconditioners for nonsymmetric saddle point problems. Appl. Math. Comput. 2017, 299, 95–118. [Google Scholar] [CrossRef]
- Ardeshiry, M.; Goughery, H.S.; Pour, H.N. New modified shift-splitting preconditioners for non-symmetric saddle point problems. Arab. J. Math. 2020, 9, 245–257. [Google Scholar] [CrossRef]
- Sidhardh, S.; Patnaik, S.; Semperlotti, F. Thermodynamics of fractional-order nonlocal continua and its application to the thermoelastic response of beams. Eur. J. Mech./A Solids 2021, 88, 104238. [Google Scholar] [CrossRef]
- Kumar, H.; Mukhopadhyay, S. Size-dependent thermoelastic damping analysis in nanobeam resonators based on Eringen’s nonlocal elasticity and modified couple stress theories. J. Vib. Control. 2022, 29, 1510–1523. [Google Scholar] [CrossRef]
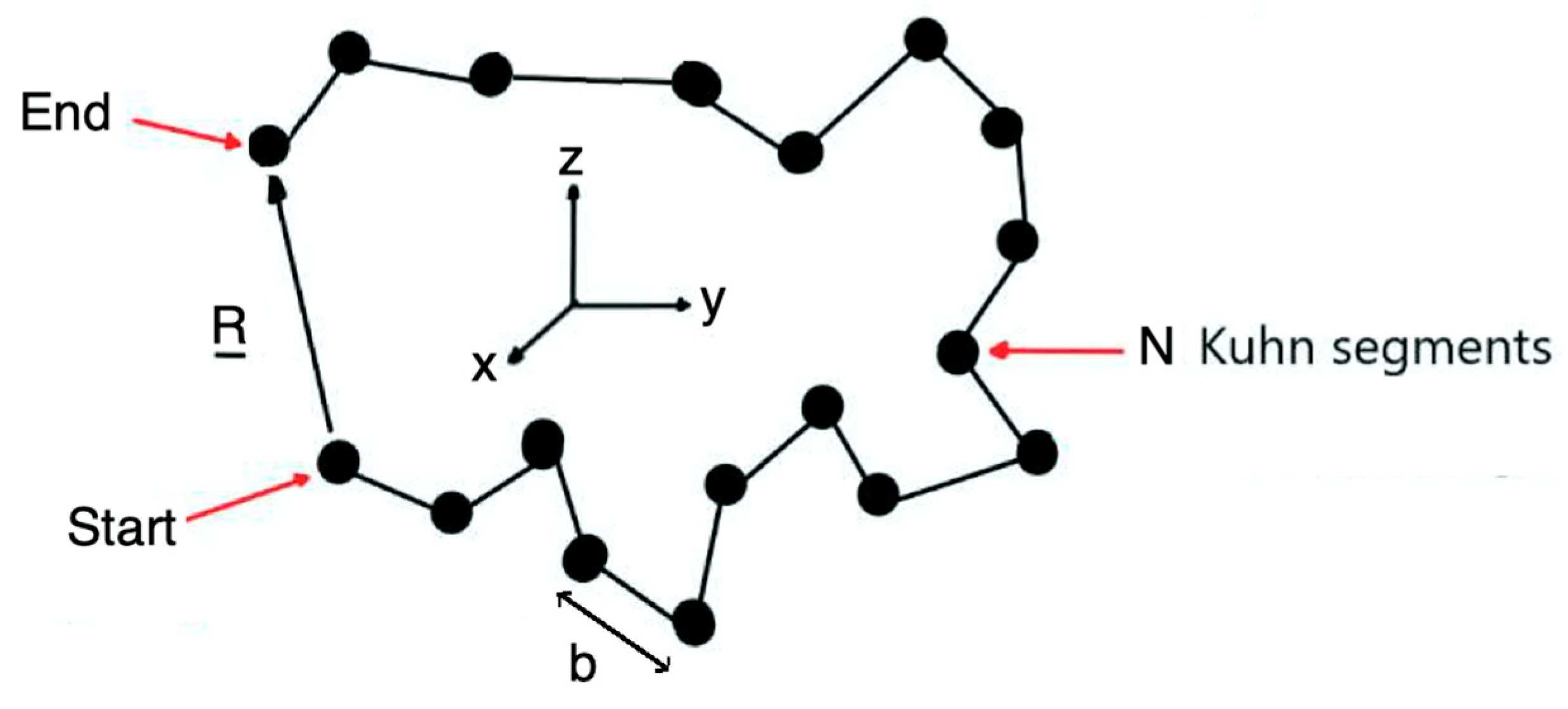
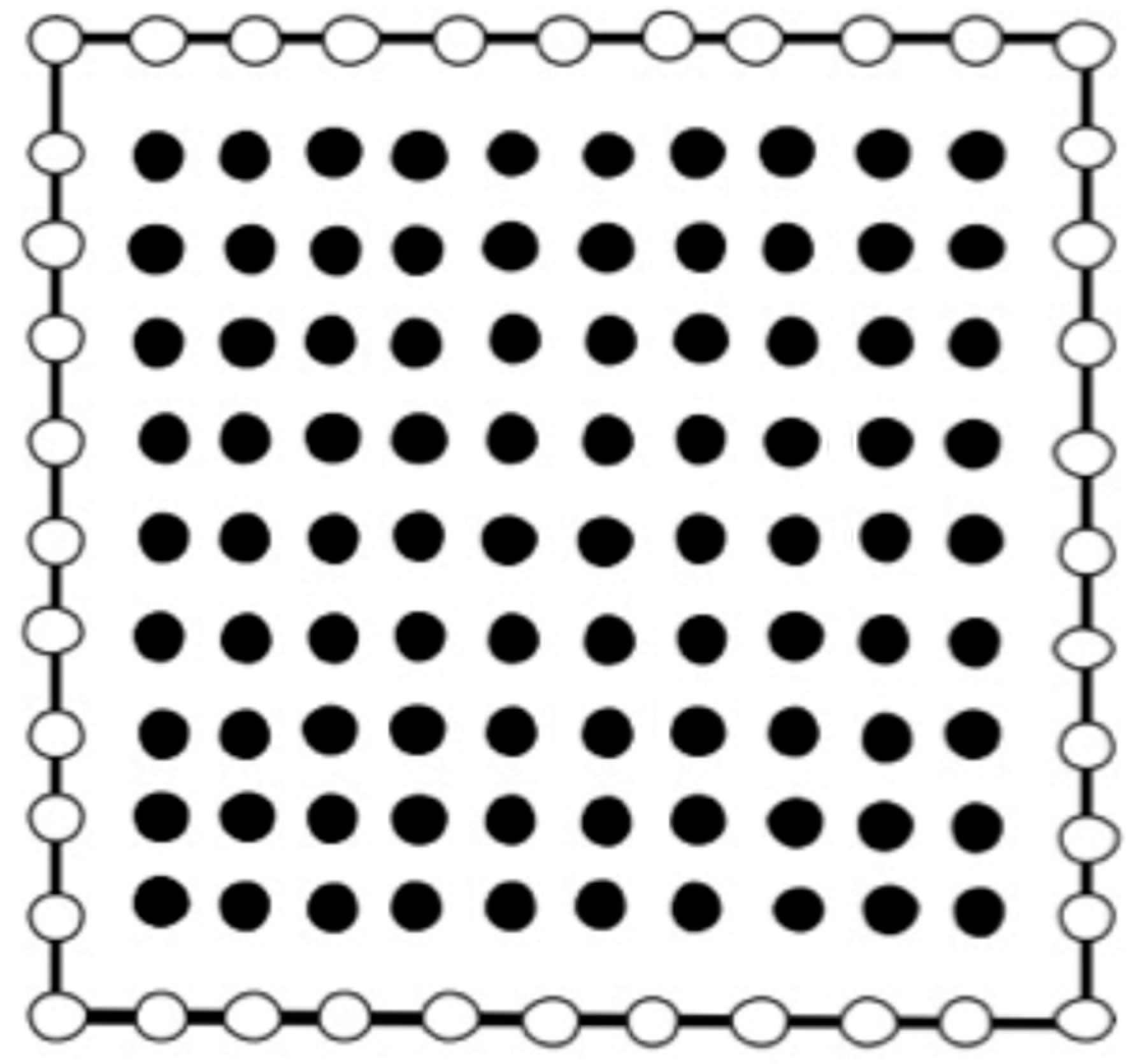



| Regularized | GMSS | NMSS | |||||
|---|---|---|---|---|---|---|---|
| Discretization Level | Preconditioning Level | CPU Time | Iteration Number | CPU Time | Iteration Number | CPU Time | Iteration Number |
| 1 (34) | 0 | 0.08 | 6 | 0.06 | 6 | 0.04 | 6 |
| 2 (68) | 0 | 0.24 | 7 | 0.20 | 7 | 0.16 | 7 |
| 1 | 0.20 | 5 | 0.16 | 5 | 0.12 | 5 | |
| 3 (136) | 0 | 0.64 | 14 | 0.54 | 12 | 0.42 | 10 |
| 1 | 0.56 | 10 | 0.46 | 8 | 0.34 | 6 | |
| 2 | 0.48 | 8 | 0.38 | 6 | 0.26 | 4 | |
| 4 (272) | 0 | 2.58 | 16 | 2.46 | 14 | 1.88 | 12 |
| 1 | 2.38 | 12 | 2.24 | 10 | 1.56 | 8 | |
| 2 | 2.12 | 10 | 1.92 | 8 | 1.42 | 6 | |
| 3 | 1.96 | 8 | 1.76 | 6 | 1.36 | 3 | |
| 5 (544) | 0 | 12.48 | 22 | 10.26 | 20 | 7.82 | 16 |
| 1 | 11.28 | 19 | 9.84 | 17 | 6.98 | 14 | |
| 2 | 10.48 | 17 | 9.42 | 14 | 6.15 | 12 | |
| 3 | 9.46 | 14 | 8.96 | 11 | 5.94 | 10 | |
| 4 | 8.96 | 11 | 8.42 | 9 | 5.24 | 7 | |
| 6 (1088) | 0 | 50.26 | 24 | 44.46 | 22 | 38.40 | 18 |
| 1 | 46.48 | 21 | 40.48 | 18 | 34.64 | 15 | |
| 2 | 42.48 | 17 | 36.26 | 15 | 30.24 | 13 | |
| 3 | 38.64 | 15 | 32.48 | 13 | 26.56 | 11 | |
| 4 | 34.86 | 13 | 28.86 | 11 | 22.32 | 9 | |
| 5 | 30.64 | 11 | 24.64 | 9 | 18.84 | 3 | |
| BEM | FEM | |
|---|---|---|
| Number of nodes | 60 | 40,000 |
| Number of elements | 25 | 14,000 |
| CPU time [min.] | 3 | 140 |
| Memory [Mbyte] | 1 | 120 |
| Disc space [Mbyte] | 0 | 180 |
| Accuracy of results [%] | 1.2 | 2.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fahmy, M.A.; Toujani, M. Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials. Computation 2024, 12, 117. https://doi.org/10.3390/computation12060117
Fahmy MA, Toujani M. Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials. Computation. 2024; 12(6):117. https://doi.org/10.3390/computation12060117
Chicago/Turabian StyleFahmy, Mohamed Abdelsabour, and Moncef Toujani. 2024. "Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials" Computation 12, no. 6: 117. https://doi.org/10.3390/computation12060117
APA StyleFahmy, M. A., & Toujani, M. (2024). Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials. Computation, 12(6), 117. https://doi.org/10.3390/computation12060117







