1. Introduction
Reissner–Nordstrom blackholes are constituted of one singularity point and two horizons, i.e., an inner Cauchy horizon and an event horizon. The embedding diagram of the Reissner–Nordstrom blackhole for a constant-angle section is obtained as in [
1]
Differently from single-horizon blackholes and so, in particular, from asymptotically flat spacetime blackhole solutions [
2], for a Reissner–Nordstrom spacetime blackhole solution, it is possible to provide a tortoise-coordinate extension, i.e., an extension of the radial coordinate
r into the complex plane as a function of the real component and of the imaginary one. In more detail, let be
x the real component and
y the imaginary one: the tortoise-coordinate
z is defined as
An analytical discussion leads to the definition of
and
as tow surfaces, respectively. Two groups of contours on each surface are possible, i.e.,
with
i.e., such that
and
are two surfaces. The Stokes lines are defined as
on
from [
3]; the horizons must stay within the closed lines of the Stokes lines.
The numerical calculation of the Stokes lines of a Reissner–Nordstrom blackhole (in four spacetime dimensions) is depicted in ([
4] Figure 4), leftmost panel.
It is therefore necessary to outline that the singularity, the inner horizon and the event horizon correspond, respectively, to the three poles of the variable
z from Equation (
2).
This analysis is complemented after the study of [
5], in which the imaginary part of the frequency is found to be not periodic.
The Stokes portraits of regular blackholes are introduced in [
6].
The generalised Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element and a cosmological-constant term were pointed out as enjoying innovative characterisations.
The stability properties of the generalised Reissner–Nordstrom spacetimes with a cosmological-constant term both in the deSitter case and in the anti-deSitter case have been studied in [
7].
The Minkowski inequality of the Reissner–Nordstrom–anti-deSitter spacetimes is presented in [
8].
The generalised Reissner–Nordstrom spacetimes endowed with a schwarzschild solid-angle element and a linear term were delineated after the following interesting properties.
In [
9], a Reissner–Nordstrom spacetime endowed with a linear term was presented and the three horizons were hinted at; the possibility of merging of the horizons was not included in the analysis.
In [
10], the features of a Reissner–Nordstrom spacetime endowed with a ‘quintessential’ component is qualitatively analysed as the mass and the charge are assumed as small quantities; ‘approximate Lie symmetries’ are implemented.
The generalised Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element, a linear term and a cosmological constant were delineated to exhibit crucial features.
In [
11], different types of spacetimes are qualitatively depicted and the features of the horizons are qualitatively described.
Some of the features of the generalised Kottler spacetimes and their parameter spaces were studied in [
12,
13].
In the present study, we analyse the generalised Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element, a cosmological constant; the generalised Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element, a linear term; and the generalised Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element, a linear term and a cosmological constant.
The analytical expressions of the radii are spelled out within a new parameterisation.
The well-posedness of the new analytical expressions of the radii is discussed.
The coordinate-singularity-avoiding coordinate extensions are newly found and provided in the instances where the cosmological-constant term is present: the tortoise-coordinate transformation is newly demonstrated to apply to the generalised Reissner–Nordstrom spacetimes, thus giving rise to new understanding of the geometries.
In the case of a generalised Reissner–Nordstrom spacetime with a linear-term parameter, the conditions for the vanishing of the explicit imaginary part of the radii are set.
In the three instances, the parameter space of the models is newly set and newly fully constrained.
The manuscript is aimed at examining the physical features of these spacetimes; the spacetimes endowed with a deSittter-like case and those endowed with an anti-deSitter-like term are newly demonstrated to exhibit very different intrinsic geometrical properties. The geometrical role of the linear term is newly explored within the Reissner–Nordstrom generalised spacetimes.
The paper is organised as follows.
In
Section 1, some features of the Reissner–Nordstrom spacetimes are recalled; in particular, the implementation of the tortoise-coordinate transformation and the study of the horizons is recapitulated.
In
Section 2, the generalised Reissner–Nordstrom spacetimes chosen are described; a comparison with the studies of the phenomenological parameters involved in the schemes is summarised.
In
Section 3, the generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild solid-angle element, with a cosmological-constant term, are newly studied: the expressions of the analytical radii are spelled out in a new characterisation; the coordinate-singularity-avoiding coordinate extensions are newly found, to which the tortoise-coordinate transformation is therefore newly demonstrated to apply; the parameter space of the model is newly established and constrained. The results newly significantly reveal that the deSitter case and the anti-deSitter case are denoted after new significantly distinct properties.
In
Section 4, the generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild solid-angle element, with a linear term, are newly investigated: the expressions of the radii are spelled out in a new parameterisation; the physical radii are newly individuated; the parameter space of the model is newly set and constrained.
In
Section 5, the generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild solid-angle element, with a linear term and a cosmological-constant term, are newly examined: the expressions of the radii are written in a new parameteirsation, the coordinate-singularity-avoiding coordinate extensions are newly found, for which the tortoise-coordinate transformation is thus newly revealed to apply; the parameter space of the model is newly indicated and constrained. The results newly demonstrate that the deSitter-like case and the anti-deSitter-like one exhibit very different, non-trivial properties; the presence of the linear term is newly demonstrated to be qualified after intrinsic geometrical properties.
In
Section 7, the quantum features of Reissner–Nordstrom spacetimes are recalled with the aim of comparison with the perturbation approach presented in
Section 1.
In
Section 8, remarks about the newly found results are presented.
2. The Generalised Reissner–Nordstrom Spacetimes
A generalisation of the Reissner–Nordstrom spacetimes endowed with a Schwarzschild solid-angle element is presented in [
14] as
Particular values of the summation in Equation (
6) are to be chosen.
The ranges of the parameters
of the metrics were studied in [
15] as
and analysed in [
16,
17,
18].
The spacetimes are discussed in [
19] as far as their geometrical features are concerned.
In [
14], two particular solutions of the Reissner–Nordstrom–deSitter blackhole spacetime
corresponding to a Reissner–Nordstrom–deSitter spacetime
were found to have an outer horizon of deSitter kind at
with
, and an inner horizon of the blackhole kind was found at
with
.
In the present study, the generalisation
is chosen. In more detail, the parameters selected will allow one to describe generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild sold-angle element, with a cosmological-constant term
; generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild sold-angle element, with a linear-term parameter term
; and generalised Reissner–Nordstrom spacetimes, endowed with a Schwarzschild sold-angle element, with a linear-term parameter
and with a cosmological-constant term
. The linear-term-parameter generalisation will be confirmed to be consistent as a small modification of the Schwarzschild radius. The deSitter cases and the anti-deSitter cases will be newly found to qualify the generalised spacetimes in a different manner, according to the two different instances.
3. The Generalised Reissner–Nordstrom Spacetimes with a Cosmological Constant
The generalised Reissner–Nordstrom spacetimes with a cosmological-constant term, endowed with a Schwarzschild solid-angle element, are written after the square line element
with
being the cosmological-constant term; i.e., a generalised Reissner–Nordstrom–deSitter spacetime is obtained in the case
, while a generalised Reissner–Nordstrom–anti-deSitter spacetime is described in the case
.
The equation
is solved as the formal expressions of the four radii
with the functions
m and
n defined as
and
3.1. Discussion of the Solutions of the Generalised Reissner–Nordstrom Spacetimes with a Cosmological-Constant Term
The well-posedness of the solutions of Equation (12) are discussed in the following.
The non-vanishing nature of the denominators
m in the summands of the radii of Equation (12) is as follows
This way, the new following physically consistent constraints are obtained
From Equation (16), one newly learns that the limit to a vanishing cosmological constant is non-trivial.
Furthermore, differently, one newly learns also that the limit to a vanishing parameter is well posed: its physical implementation is the restoration of the Schwarzschild radius in the presence of a cosmological constant; this role is modified after the definition of the new coordinate-singularity-avoiding coordinate extension.
In particular, Equation (16d) defines the modifications to the physical meaning of the Schwarzschild radius, which, in their turn, further constrain the parameter space.
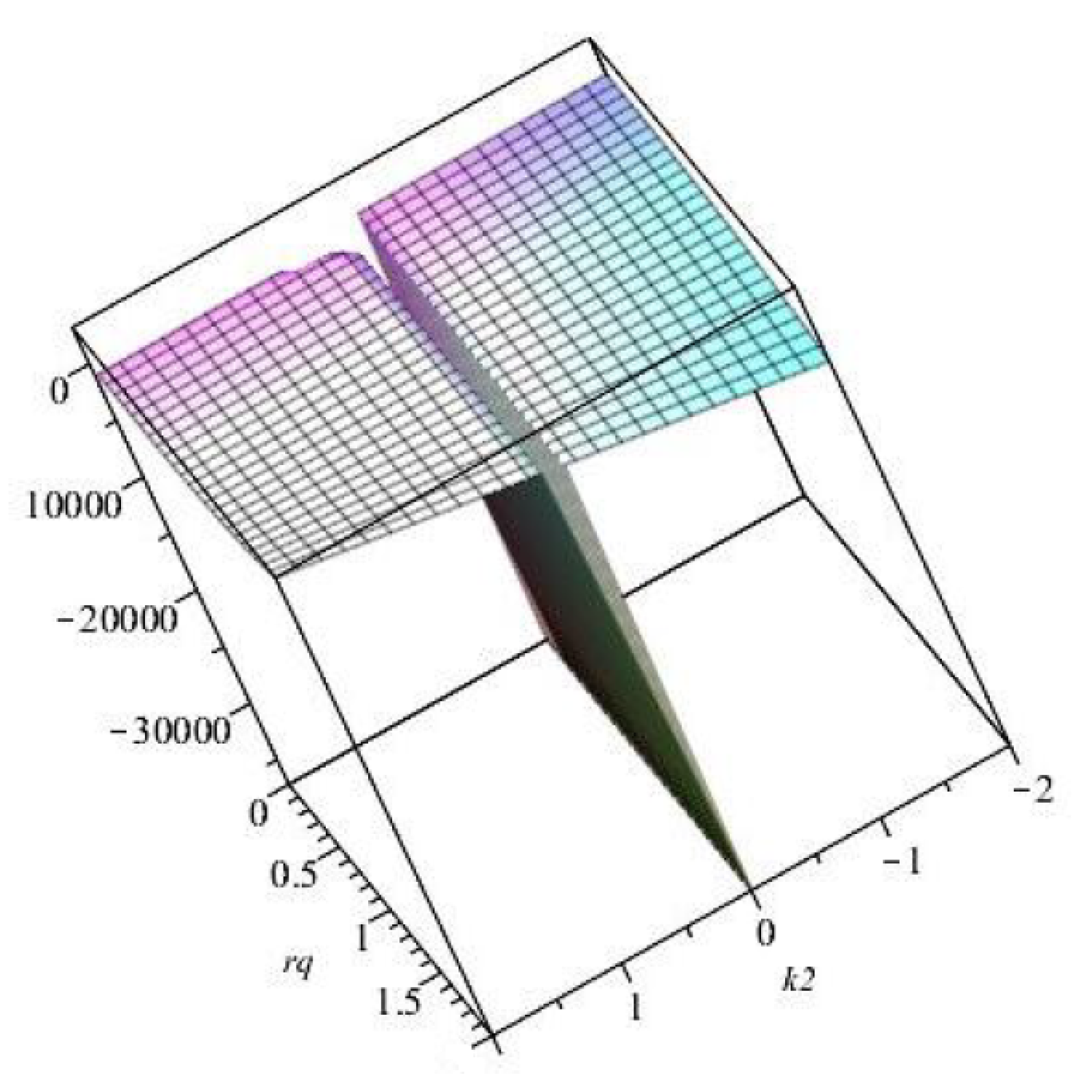
The conditions in Equation (16d) are plotted in
Figure 1.
The new constraints from Equation (16) imply that there is a profound difference between the geometries of the deSitter characterisation and those of the anti-deSitter one, as can be seen in
Figure 1.
Moreover, one new region of the parameter space of the scheme is delineated, which is already ruled out.
The non-vanishing nature of the denominators
m is formulated as follows
Requesting
, i.e., requesting the most general scheme, further implies
From Equation (
18), a new constraint on the parameter space is found, which involves the possible values of
.
The choice of an equality in Equation (
18) delineates a new particular family of generalised Reissner–Nordstrom spacetimes with a cosmological constant, which has not been explored yet. More specifically, the new family of generalised Reissner–Nordstrom spacetimes with a cosmological constant admits two new physically different cases, in which the cosmological-constant term acts in a different manner and whose geometric properties have not been investigated yet.
Furthermore, Equation (16d) automatically further implies the new following constraint on the parameter space which newly relates
and
in a new non-trivial manner; i.e.,
for which, in more detail, one demonstrates that the limit to a Schwarzschild spacetime or to a generalised Schwarzschild spacetime are not only highly non-trivial, but also depend momentously on the sign of the cosmological constant; i.e., the generalised Reissner–Nordstrom geometries are found to admit a representation characterised after the new constraints.
More specifically, the request (where the inequality is learnt from the analysis in the above) implies a new lower bound of in the deSitter case.
Requesting , i.e., requesting the most general scheme, further implies possible modifications of the roles and of the values of the square length : form the new definition of the new coordinates-singularity avoiding coordinates extension, one will learn that the modifications of the square length are only small; furthermore, one will learn that such modifications are intrinsically related with the (small) modifications of the value of the Schwarzschild radius.
The generalised Reissner–Nordstrom spacetimes with a cosmological constant therefore are distinguished in a different manner; more specifically, the role of the cosmological constant is that of modifying the expression of the Schwarzschild radius and that of the length in a new non-trivial manner, which, furthermore, act differently in the deSitter case and in the anti-deSitter case.
The numerator of the summands in Equation (12) is studied as follows.
3.2. Merging of the Radii
For the sake of comparison with the more general results of
Section 5, the solutions of Equation (12) are simplified and the four radii are demonstrated to become the two horizons as follows
with the functions
and
Indeed, the first summand is calculated to vanish for real values of all the parameters but that of the cosmological-constant term; i.e., it vanishes for consisting of a real part and a (positive) imaginary one: the differences between the deSitter case and the anti-deSitter case are here newly understood also as far as the multivaluedness of the tortoise-coordinate extension is concerned. It is therefore newly verified that the tortoise-coordinate extension is here well posed.
3.3. New Coordinate-Singularity-Avoiding Coordinate Extension of the Generalised Reissner–Nordstrom Spacetimes with a Cosmological Constant
The new coordinate-singularity-avoiding coordinate extension is found to be
with
Indeed, it is crucial to remark that
The coordinate-extension Equation (
24) is valid for small values of
only; the cosmological-constant term is therefore newly demonstrated to modify the value of the Schwarzschild radius only slightly.
It is thus possible to implement the tortoise-coordinate transformation to study the radii.
5. Generalised Reissner–Nordstrom Spacetimes with a Linear Term and a Cosmological-Constant Term
The generalised Reissner–Nordstrom spacetimes with a linear term and a cosmological-constant term, endowed with a Schwarzschild solid-angle element, are written after the square line element
where
is the parameter characterising the linear term and
is the cosmological-constant term, which is, for the moment, not specified as having positive values or as having negative values. The
equation of the spacetime (
39) is calculated to admit the analytical solutions, which are here newly parameterised as
where the following definitions have been taken:
and
5.1. Discussion of the Radii Solutions
The denominators of the radii solutions of Equation (40) are well posed when
The equation
is discussed as
which is solved after the values
which is well posed for
The discussion of the equality in the latter shows that there are regions of the parameter space which have to be excluded since their expression is multiplied by the imaginary unit.
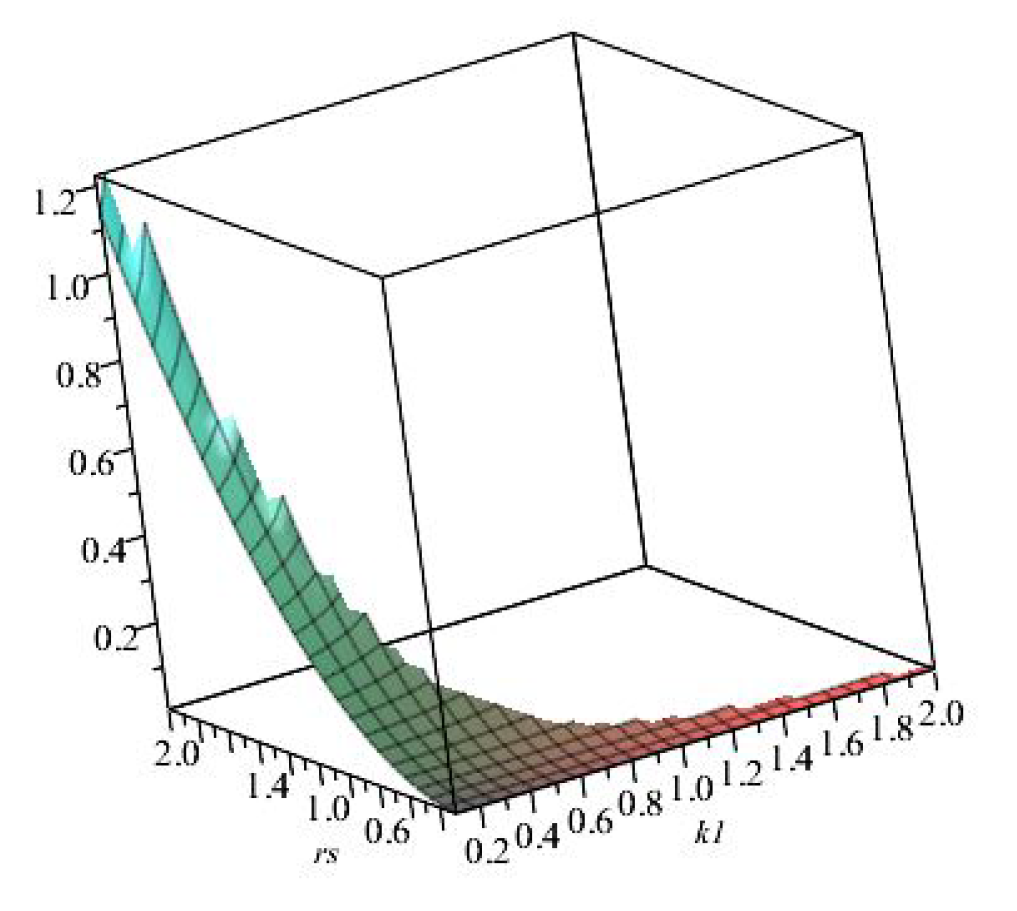
In particular, from the discussion of Equation (
45) with respect to the square length
, the region to be excluded is depicted in
Figure 4.
Furthermore, the value has to be excluded from the investigation, as will be explained after the explanations of the roles of the parameters and with respect to the definition of the Schwarzschild radius, for the implementation of the tortoise coordinates, for the study of the blackhole spacetime in a non-perturbation way.
A new particular family of solutions from Equation (40) is
which is solved after the relation for the Reissner–Nordstrom squared length
when evaluated at
; Equation (
49) therefore becomes
Furthermore, one must stipulate
5.2. The Physical Horizons
The two physical horizons are obtained from Equation (40) after stipulating that the opportune quantities vanish, i.e.,
and
because of Equation (
51).
It is important to remark that the condition Equation (
53) does not depend on the square length
.
Equation (
53) is solved as
Imposing
is realised in the instances
The horizons are therefore found as
From Equation (
54) and from Equation (55), the new constraints on the Schwarzscihld radius, on
and on
are obtained.
Furthermore, the new roles of the parameters characterising the value of the Schwarzshild radius are outlined.
The parameter space is therefore studied at the realness of the square roots.
It is possible to remark that the two horizons of Equation (56) exhibit different properties in the deSitter case and in the anti-deSitter case.
The different properties exhibited in the deSitter case and those demonstrated in the anti-deSitter case also involve the linear-term parameter
The method presented in [
20] applies only to a Schwarzschild–deSitter spacetime.
In [
21], the ‘trapping horizons’ are described only for Reissner–Nordstrom–deSitter spacetimes in the special case of
and in the special case
.
In the present analysis, the manner of merging of the radii to create the two horizons is newly defined according to the ratio . The new constraints of the new functions a and f on the parameter space further complete the new establishment of the parameter space of the model.
5.3. New Coordinate-Singularity-Avoiding Coordinate Extension of the Generalised Reissner–Nordstrom Spacetimes with a Linear Term and with a Cosmological Constant
The new coordinate-singularity-avoiding coordinate extensions are written as
with the value of
being
This way, the expansion is balanced after the newly found initial value
of the new radial variable
as
The new coordinate-extension Equation (
57) is apt for small values of
and
only; the linear-term parameter and the cosmological-constant term are thus demonstrated to modify the value of the Schwarzschild radius in a new manner. The newly found generalised Reissner–Nordstrom–deSitter geometry and the generalised Reissner–Nordstrom–anti-deSitter one are newly explored; the two geometries are found to be different, the difference being highly non-trivial due to the non-linearity of the Einstein field equations.
One therefore newly learns that the limit to a vanishing cosmological constant is non-trivial.
Moreover, in a different manner, it is also possible to infer the well-posed-ness of the limit to a vanishing value of the parameter; indeed, its physical understanding is the accomplishment in the restoration of the role of the Schwarzschild radius in the presence of a cosmological- constant term; this interpretation is modified after the implementation of the new coordinate-singularity-avoiding coordinate extension.
It is therefore possible to apply the tortoise-coordinate transformation to analyse the radii.
6. Outlook
The present work is aimed at studying some particular features of the generalised Reissner–Nordstrom blackhole spacetimes. The new methodology used is the findings of the analytical radii, the discussion of their well-posedness and the conditions for the obtention of the physical horizons; from the study, new features of the generalised spacetimes are found and new constraints on the parameter spaces of the models are established.
Three particular cases of interest have been investigated.
Concerning the generalised cosmological Reissner–Nordstrom spacetime with a cosmological constant, endowed with a Schwarzschild solid-angle element, the results found complete studies in the literature by adding new qualifications and new relationships among the Reissner–Nordstrom parameters and the cosmological constant; the findings far and away generalise the pioneering work [
21], in which only particular aspects of the Schwarzschild–deSitter spacetime were outlined; the study was not followed after further investigations. Furthermore, the present analysis of this spacetimes is complemented with the new definition of the coordinate-singularity-avoiding coordinate extensions, which allows one to implement the tortoise-coordinate transformation; the new coordinate extensions generalise the Nairai transformation for the considered case: in particular, the role of the cosmological constant in modifying the other parameters only slightly is newly found. A new particular family of generalised Reissner–Nordstrom spacetimes with a cosmological constant is found and studied. All the results are demonstrated to hold in the Reissner–Nordstrom–deSitter case and in the Reissner–Nordstrom–anti-deSitter case in a new different non-trivial manner, which further clarifies new features of the generalised deSitter spaces and those of the generalised anti-deSitter spaces.
The exploration of the generalised Reissner–Nordstrom spacetimes with a linear term is motivated by a particular case in [
14] and the related literature. In this case, the analytical radii are found and discussed and the expressions of the physical horizons are presented. The analysed generalised spacetime is therefore qualified after new relations among the parameters characterising the solution, as well as new constraints of the parameter space of the model.
The generalised Reissner–Nordstrom spacetimes with a linear term and a cosmological constant, endowed with a Schwarzschild solid-angle element, are studied analytically after [
14]. As a result, the analytical expressions of the radii are found and their well-posedness is discussed; the expressions of the physical horizons are presented. The new aspects of the relations among the parameters qualifying the solution are investigated and the new parameter space is set. A new particular family of solutions is found and explored. The new coordinate-singularity-avoiding coordinate extension is newly found to be consistent with the Nairai transformation and allows one to further apply the tortoise-coordinate transformation; the coordinate-singularity-avoiding coordinate extensions newly characterise the role of the parameters of the generalised model in modifying the Reissner–Nordstrom parameters only slightly: furthermore, differently from the case of the generalised Reissner–Nordstrom spacetimes with a cosmological-constant term, the initial values of the coordinates are calculated to be non-trivial.
The specificities of the newly introduced methodology consist also in analysing the physical spacetimes non-perturbatively.
The gathering of the results allows one to implement further qualifications and further investigations.
8. Remarks
In the present paper, the generalised Reissner–Nordstrom spacetimes have been analysed in three particular instances: the generalised Reissner–Nordstrom spacetimes with a cosmological constant, the generalised Reissner–Nordstrom spacetimes with a linear term and the Reissner–Nordstrom spacetimes with a linear term and a cosmological-constant term.
The generalised Reissner–Nordstrom spacetime with a cosmological constant helped us reveal the highly non-trivial differences between the deSitter case and the anti-deSitter case. The differences are outlined not only in the definition of the radii, but also as far as the coordinate-singularity-avoiding coordinate extension is concerned. A comparison with the perturbation approach is accomplished after exploring the features of the cosmological-constant term . A particular new specific family of such spacetime blackhole solutions, corresponding to , is found. The analytical expressions of the physical horizons are set within the appropriate constraints of the parameter space.
The generalised Reissner–Nordstrom spacetimes with a linear term have been analysed. The three radii are found, which are determined after the reduced quartic equation, and the conditions for the description of the physical spacetime two physical horizons are displayed. The geometry role of the linear term, , is here newly investigated with respect to the previous interpretations as quintessence and as non-dynamical dark energy.
Cases of generalised Reissner–Nordstrom spacetime with a linear term and a cosmological constant have been explored. The well-posedness of the four radii is discussed, as well as the description of the two physical horizons. A new family of particular solutions is newly found, according to the new parameterisation of the blackhole spacetime radii and horizons, and the parameter space of these solutions is newly inspected. The coordinate-singularity-avoiding coordinate extension is found; in particular, the non-trivial value of the initial conditions of the radial variable is studied. The comparison with the generalised Reissner–Nordstrom spacetimes with a cosmological-constant term is therefore accomplished after the recognition of the features which are exhibited within the discussion of the physical conditions.
The analysis of the role of the lienar.term parameter and of that of the cosmological term parameter in the modifications of the physical implementation of the Schwarzschild radius open the way to the comparison with analysis of the comparison with [
44], which was developed for the sake of possible descriptions of particular types of galaxy rotation curves.
The paper is organised as follows.
In
Section 1, the generalised Reissner–Nordstrom spacetimes are introduced; in particular, the perturbation approach is presented, also with respect to the definition of the tortoise-coordinate system.
In
Section 2, the generalised Reissner–Nordstrom spacetimes are present from a geometrical point of view. Among the generalisations possible, three of them were taken into account, whose physical expression is straightforwardly juxtaposed with the generalised Schwarzschild spacetimes within the same instances of physical definition.
In
Section 3, the generalised Reissner–Nordstrom spacetimes with a cosmological-constant term are newly studied. The two physical horizons are exhibited as analytically descending from the non-trivial four radii, which are the solutions of the
equation. The parameter space of the model is set and constrained. The coordinate-singularity-avoiding coordinate extension is found. The results are demonstrated to hold in a different manner in the deSitter case and in the anti-deSitter case, whose specific aspects are found to be significantly different. The solutions are also rewritten in a manner so as to render easy the comparison with the case of a generalised Reissner–Nordstrom spacetime with a linear term and a cosmological-constant term.
In
Section 4, generalised Reissner–Nordstrom spacetimes with a linear term are investigated. The particular features of the reduced-quartic
equation are outlined as implying three radii; the constraints for the obtention of the two physical radii are discussed. The parameter space of the scheme is established and constrained. The role of the linear term is here contrasted with that of the case of the generalised Schwarzschild spacetimes, in which such a role is straightforwardly interpreted as a small modification of the Schwarzschild radius.
In
Section 5, the generalised Reissner–Nordstrom spacetimes with a linear term and a cosmological-constant term are examined. The four radii are found; the well-posedness of the solutions is scrutinised and the conditions of the definition of the two physical radii are researched. The parameter space of the model is defined and constrained and also compared with the cases of the generalised Reissner–Nordstrom spacetimes with a cosmological-constant term. The differences between the deSitter case and the anti-deSitter case are set for comparison. A prospective systematic study of the possible quantum implementations of the geometries allows for the definitions of new cosmological settings also at the semiclassicalisation epoch.
In
Section 7, some features of the (generalised) Reissner–Nordstrom spacetimes, such as the Minkowski inequality, are reviewed for the sake of comparison with the Schouten classification and the Petrov classification within the framework of an analysis of asymptotical behaviour; furthermore, the quantum features of generalised Reissner–Nordstrom spacetimes are recalled for comparison with the perturbation approach as well as for prospective studies.
In
Section 8, the main features of the generalised chosen Reissner–Nordstrom spacetimes are compared with further examples.