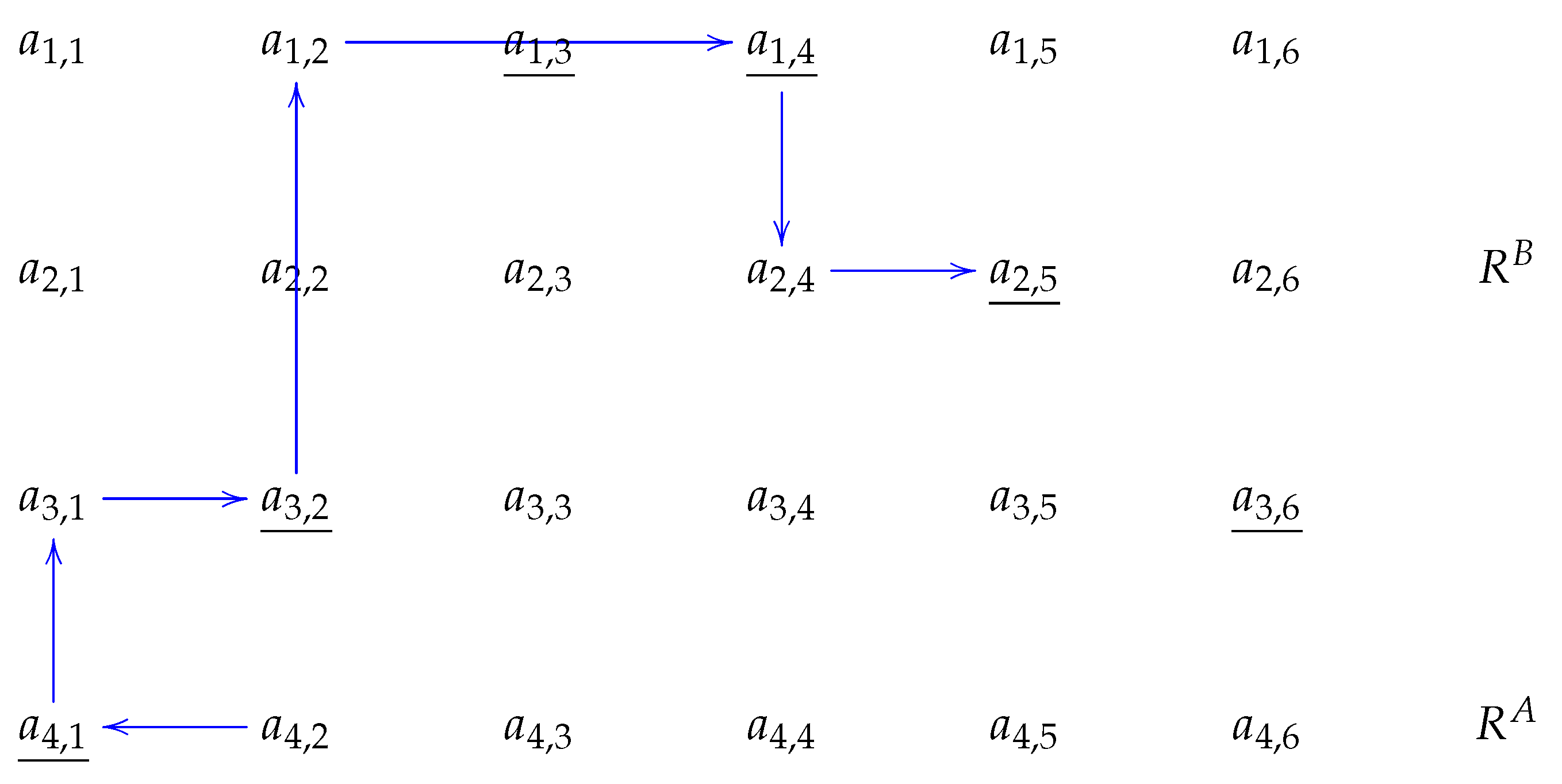Solutions of the Yang–Baxter Equation and Automaticity Related to Kronecker Modules

Abstract

The Kronecker algebra $\mathcal{K}$ is the path algebra induced by the quiver with two parallel arrows, one source and one sink (i.e., a quiver with two vertices and two arrows going in the same direction). Modules over $\mathcal{K}$ are said to be Kronecker modules. The classification of these modules can be obtained by solving a well-known tame matrix problem. Such a classification deals with solving systems of differential equations of the form $Ax=B{x}^{\prime }$, where A and B are $m\times n$, $\mathbb{F}$-matrices with $\mathbb{F}$ an algebraically closed field. On the other hand, researching the Yang–Baxter equation (YBE) is a topic of great interest in several science fields. It has allowed advances in physics, knot theory, quantum computing, cryptography, quantum groups, non-associative algebras, Hopf algebras, etc. It is worth noting that giving a complete classification of the YBE solutions is still an open problem. This paper proves that some indecomposable modules over $\mathcal{K}$ called pre-injective Kronecker modules give rise to some algebraic structures called skew braces which allow the solutions of the YBE. Since preprojective Kronecker modules categorize some integer sequences via some appropriated snake graphs, we prove that such modules are automatic and that they induce the automatic sequences of continued fractions.

1. Introduction

An automorphism R over a vector space V is a solution of the Yang–Baxter equation, if it satisfies the following identity (1) known as the braided equation, i.e.,

$$(R\otimes i{d}_V)\circ (i{d}_V\otimes R)\circ (R\otimes i{d}_V)=(i{d}_V\otimes R)\circ (R\otimes i{d}_V)\circ (i{d}_V\otimes R)$$

(1)

is satisfied on $V\otimes V\otimes V$.

Equation (1) was introduced in 1967 by Yang in two short papers written with the purpose of generalizing previous works on theoretical physics. Shortly afterwards, Baxter introduced such an equation in a paper regarding statistical mechanics. It is worth noting that giving a complete classification of the YBE solutions remains an open problem [1,2].

YBE research is a trending topic in several fields of mathematics. Its investigation has influenced areas such as Hopf algebras, quantum computing, cryptography, knot theory, non-associative algebras, etc. For instance, Civino et al. [3] used the cryptanalysis of substitution–permutation networks to give a non-degenerate involutive set-theoretical solutions of the YBE via some algebraic structures named braces. YBE was used by Chen [4] to generate braiding quantum gates helpful in topological quantum computing and Kauffman et al. [5] proved that the solutions of the YBE give rise to universal gates in a quantum computer.

It is worth pointing out that Nichita et al. [6,7,8] introduced the Yang–Baxter operators of the form $R_{\delta ,\kappa ,ϵ}^{\mathfrak{A}}:\mathfrak{A}\otimes \mathfrak{A}\to \mathfrak{A}\otimes \mathfrak{A}$, such that $x\otimes y\mapsto \delta xy\otimes 1+\kappa 1\otimes xy-ϵx\otimes y$, where for a field $\mathbb{F}$, $\mathfrak{A}$ is a unitary associative $\mathbb{F}$-algebra. Furthermore,

• $\kappa \ne 0$ if $\delta =ϵ\ne 0$;
• $\delta \ne 0$ if $\kappa =ϵ\ne 0$;
• $ϵ\ne 0$ if $\delta =\kappa =0$.

In particular, $R_{\delta ,\kappa ,ϵ}^{\mathfrak{A}}$ gives the universal quantum gate

$$\left(\begin{array}{cccc}1& 0& 0& 0\\ 0& 0& 1& 0\\ 0& 1& 1& 0\\ 0& 0& 0& -1\end{array}\right)$$

Nichita [7] defined another Yang–Baxter operator, which generate identities in Jordan algebras after appropriated specializations.

On the other hand, the representation theory of the Kronecker algebra is a subject of great interest in the matrix problem theory. Kronecker and Weierstrass found out the indecomposable modules associated with this algebra by solving the following matrix problem over an algebraically closed field $\mathbb{F}$ [9,10,11]. In this case, we denote by $M=(X,Y)$, $R_i^M$, $C_j^X$, $C_k^Y$ a matrix M (called Kronecker matrix) consisting of two matrix blocks X and Y with the same size, the ith row of the matrix M, and the jth (kth) column of the matrix block X (Y).

Kronecker Problem

Finding the canonical Jordan forms of matrices of type M with respect to the following transformations:

• Row permutations;
• Additions of the form $f_h{R}_h^M+f_i{R}_i^M$, with $f_h,f_i\ne 0$, $f_h,f_i\in \mathbb{F}$;
• Simultaneous column permutations of $C_j^X$ and $C_k^X$ within the matrix block X, and $C_j^Y$ and $C_k^Y$ within the matrix block Y;
• Multiplications of the form $f(C_j^X,C_j^Y)=(f{C}_j^X,f{C}_j^Y)$, where $0\ne f\in \mathbb{F}$.

If the Kronecker matrices $M=(A,B)$ and $M^{\prime }=(A^{\prime },B^{\prime })$ can be transformed one into the other by means of elementary transformations, then they are said to be equivalent or isomorphic as Kronecker modules.

Recently, Espinosa [12] found new invariants associated with preprojective and pre-injective Kronecker modules (i.e., non-regular Kronecker modules) in their investigations regarding the categorization of real sequences in the sense of Ringel et al. [13,14]. So-called Kronecker snake graphs are examples of such invariants. This paper proves that some snake graphs arising from pre-injective Kronecker modules (called helices or pre-injective Kronecker snake graphs) induce skew braces. In other words, we prove that appropriated snake graphs associated with indecomposable Kronecker modules induce the solutions of YBE.

Automaticity associated with different algebraic structures is a widely studied topic. The seminal work by Turing regarding the classification of numbers is perhaps one of the most remarkable works regarding this subject [15]. He classified real numbers as computable or uncomputable. Accordingly, computable numbers are real numbers whose k-adic expansion ($k\ge 2$) can be produced by a Turing machine. It is worth noting that automata are one of the most basic computation models, and that if a sequence $\mathfrak{a}={\left(a_n\right)}_{n\ge 0}$ is generated by a k-automaton, then the sequence $\mathfrak{a}$ is said to be automatic [16,17].

According to Shallit et al. [17], automatic sequences have strong relationships with number theory. These interactions allow many results in transcendence theory to be proven with positive characteristics. In particular, Adamczewski et al. [18] proved that Liouville numbers cannot be generated by a finite automaton, thus answering a conjecture proposed by Shallit [16].

Relationships between the theory of the representation of algebras and the automata theory were given by Rees [19], who proved that strings and bands (associated with indecomposable modules over monomial algebras) can be generated by an automaton. In this paper, it is proven that the preprojective Kronecker modules give rise to some automatic categories and that the sequences of some continued fractions associated with such modules are automatic.

1.1. Motivations

Since their introduction, Kronecker modules have been a source of a plethora of applications in diverse science fields [10,11]. Particularly, these have been used to solve differential equations [20]. The generalizations of the Kronecker matrix problem give rise to the well-known Krawtchouk matrices with applications in quantum computing, statistics, combinatorics, coding theory, probability, etc. [21]. On the other hand, relationships between automata theory, the number theory, and the theory of representation of algebras is a topic of great interest among many mathematicians [16,17].

It is worth noting that the categorization of integer sequences in the sense of Ringel et al. [13,14] allows interpreting numbers in sequences as the invariants of objects of a given category (for instance, modules over path algebras or quiver representations). Automatic sequences give rise to automatic objects in these categories. This paper proves that integer sequences categorized by preprojective Kronecker modules are automatic.

Investigations regarding YBE have influenced the research in knot theory, quantum computing, quantum mechanics, Hopf algebras, cryptography, etc. [3,6,7,8]. This paper proves that pre-injective Kronecker modules give rise to the skew braces used to generate the solutions of the YBE.

1.2. Contributions

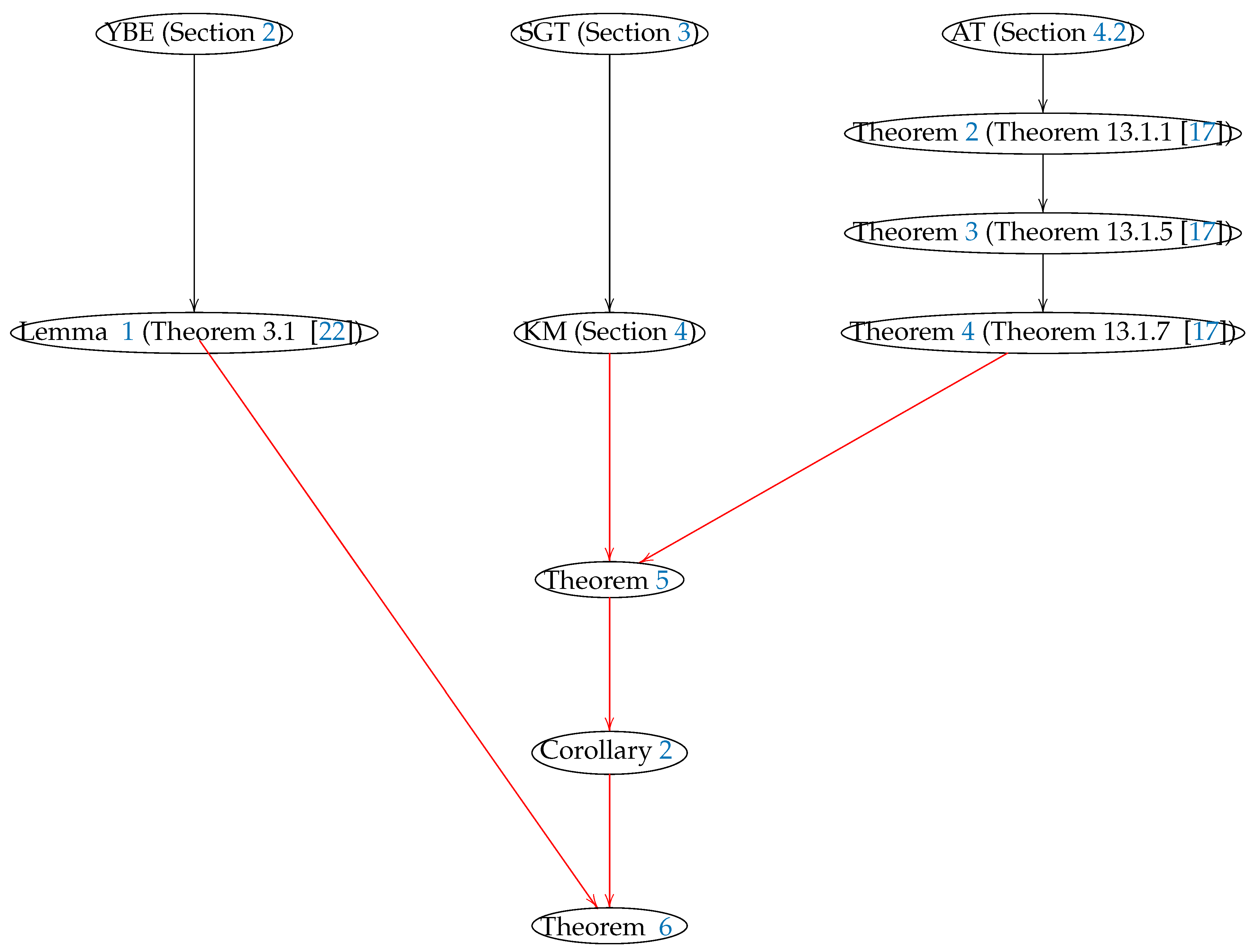
The main results of this paper are Theorem 5, Corollary 2, and Theorem 6. These are illustrated as the targets of red arrows in Figure 1, which shows how the different theories are related to each other to obtain our results. We use the acronyms AT, Cr, KM, Lm, Sect, SGT, Th, and YBE for automata theory, corollary, Kronecker modules, lemma, section, snake graph theory, theorem and Yang–Baxter equation, respectively.

Theorem 5 proves that the categories of type $\langle P_1,\dots ,P_t\rangle$ generated by a finite number of preprojective Kronecker modules are automatic. Corollary 2 proves that some sequences of continued fractions (arising from the preprojective Kronecker modules) are automatic. Theorem 6 proves that pre-injective Kronecker modules give rise to skew braces which, according to Vendramin et al. [22], generate solutions of the YBE.

The organization of this paper is as follows: the main definitions and notations are given in Section 2; we reiterate the definitions and notations regarding YBE in Section 2; the snake graphs are shown in Section 3; the Kronecker modules are elaborated in Section 4; and the automatic sequences and automatic categories are discussed in Section 4.2 and Section 4.3. Finally, we present the main results in Section 5 and the concluding remarks are given in Section 6.

Figure 1. The main results presented in this paper (targets of red arrows) allow a connection to be established between YBE theory, the representation theory of the Kronecker algebra, the automata theory, and the snake graph theory. We use the acronyms AT, Cr, KM, Lm, Sect, SGT, Th, and YBE for automata theory, corollary, Kronecker modules, lemma, section, snake graph theory, theorem, and Yang–Baxter equation, respectively.

Figure 1. The main results presented in this paper (targets of red arrows) allow a connection to be established between YBE theory, the representation theory of the Kronecker algebra, the automata theory, and the snake graph theory. We use the acronyms AT, Cr, KM, Lm, Sect, SGT, Th, and YBE for automata theory, corollary, Kronecker modules, lemma, section, snake graph theory, theorem, and Yang–Baxter equation, respectively.

2. Preliminaries

This section recalls some basic definitions and results regarding YBE, braces, and Kronecker modules, which are helpful for a better understanding of this paper.

Yang–Baxter Equation and Its Solutions

This section makes a brief introduction to some of the methods used to solve the YBE [6,7,8,23,24,25,26,27].

Drinfeld [28] proposed that the set-theoretical YBE be solved. Solutions of these kinds of equations are given by quadratic sets, which are pairs of the form $(X,r)$, where X is a set and $r:X\times X\to X\times X$ is a bijective map that satisfies the corresponding braided Equation (2).

$$(r\times i{d}_X)\circ (i{d}_X\times r)\circ (r\times i{d}_X)=(i{d}_X\times r)\circ (r\times i{d}_X)\circ (i{d}_X\times r)$$

(2)

meaning that a solution $(X,r)$ written as $r(x,y)=({\sigma }_x\left(y\right),{\tau }_y\left(x\right))$, for all $(x,y)\in X\times X$ is said to be non-degenerate, provided that ${\sigma }_x$ and ${\tau }_x$ are bijective maps for any $x\in X$. It is involutive if $r^2=i{d}_{X\times X}$ [24].

One of the best approaches to solve the non-degenerate involutive set-theoretical solutions of the YBE was introduced by Rump [25,26], who define some algebraic structures called braces. According to Cedó, Jespers and Oknińiski [23], a left brace is an Abelian group $(A,+)$ endowed with another group structure, defined by a rule $(a,b)\mapsto ab$ that satisfies the compatibility conditions for all $a,b,c\in A$.

$$a(b+c)+a=ab+ac$$

(3)

Right braces are defined in the same fashion. In such a case, the compatibility condition has the form $a+a(b+c)=ab+ac$.

Note that, if X is finite, then an involutive solution of the braided equation is right non-degenerate if and only if it is left non-degenerate. It is worth noticing that the non-degenerate involutive set-theoretical solutions of the YBE were given by Etingof et al. and Gateva-Ivanova and Van den Bergh [29,30] by associating a group $G(X,r)$ with the solution $(X,r)$ [23]. Afterwards, Ballester-Bolinches et al. [27] used the Cayley graph of some subgroups $\mathcal{G}(X,r)$ (of the symmetric group on X denoted ${\mathrm{Sym}}_X$) associated with the solutions $(X,r)$ of the YBE to define the left braces.

For $a\in G$, we define ${\rho }_a,{\lambda }_a\in {\mathrm{Sym}}_G$ by

$$\begin{array}{cc}\hfill {\rho }_a\left(b\right)& =ba-a,\hfill \\ \hfill {\lambda }_a\left(b\right)& =ab-a.\hfill \end{array}$$

(4)

Rump proved the following result.

Lemma 1

(Lemma 4.1, [23], Propositions 2 and 3 [25]). Let G be a left brace. The following properties hold.

1. 

$a{\lambda }_a^{-1}\left(b\right)=b{\lambda }_b^{-1}\left(a\right)$;

2. 

${\lambda }_a{\lambda }_{{\lambda }_a^{-1}\left(b\right)}={\lambda }_b{\lambda }_{{\lambda }_b^{-1}\left(a\right)}$;

3. 

The map $r:G\times G\to G\times G$ defined by $r(x,y)=({\lambda }_x\left(y\right),{\lambda }_{{\lambda }_x\left(y\right)}^{-1}\left(x\right))$ is a non-degenerate involutive set-theoretical solution of the YBE.

We remind that Vendramin and Guarnieri [22] introduced the notion of skew brace. According to them, a skew left brace A is a group (written multiplicatively) with an additional group structure given by $(a,b)\mapsto a\circ b$ such that

$$a\circ \left(bc\right)=(a\circ b)a^{-1}(a\circ c)$$

(5)

holds for all $a,b,c\in A$, where $a^{-1}$ denotes the inverse of a with respect to the group structure given by $(a,b)\mapsto ab$.

Left braces are examples of skew braces.

The following results describes the non-degenerate involutive set-theoretical solutions of the YBE in terms of skew braces.

Theorem 1

(Theorem 3.1. [22]). Let A be a skew left brace. Then, $r_A:A\times A\to A\times A$, $r_A(a,b)=({\lambda }_a\left(b\right),{\lambda }_{{\lambda }_a\left(b\right)}^{-1}({(a\circ b)}^{-1}a(a\circ b)))$ is a non-degenerate solution of the YBE. Furthermore, $r_A$ is involutive if and only if $ab=ba$ for all $a,b\in A$.

3. Snake Graphs

Snake graphs are finite-connected planar graphs consisting of uniform adjacent square tiles. Two consecutive tiles $T_i$ and $T_{i+1}$ are cemented by gluing either the northern edge of $T_i$ with the southern edge of $T_{i+1}$ or the eastern edge of $T_i$ with the western edge of $T_{i+1}$ [31,32,33].

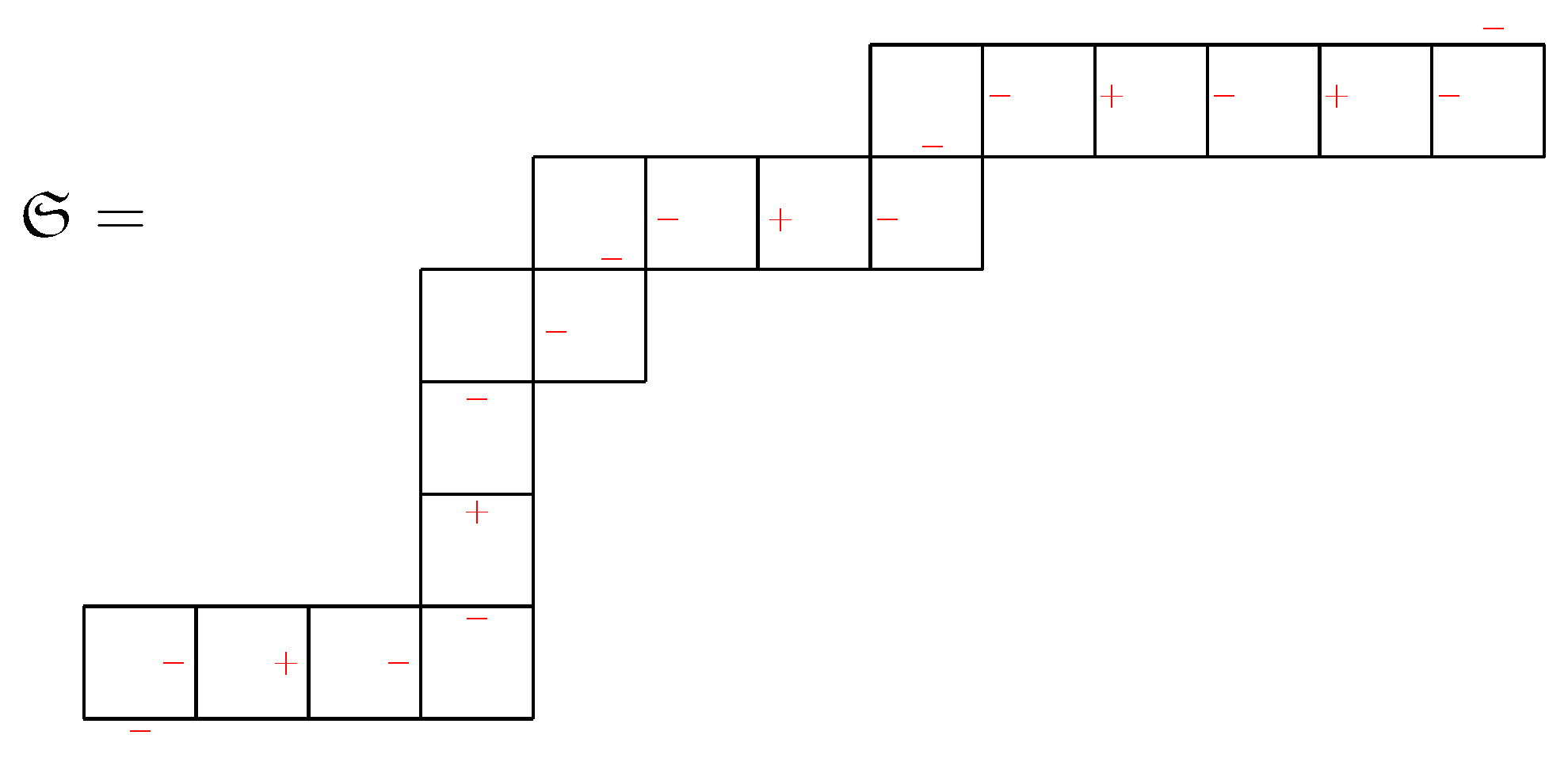
A snake graph is said to be horizontal straight (vertical straight) if the gluing process is only applied to the eastern–western edges (northern–southern) of its tiles. Any snake graph $\mathcal{G}$ is a union of a finite number of straight snake graphs ${\mathcal{G}}_1,\dots ,{\mathcal{G}}_k$, if $|{\mathcal{G}}_i|=n_i$, then we write $\mathfrak{S}={\mathfrak{S}}_f(n_1,n_2,\dots ,n_k)$. Figure 2 shows an example of the snake graph ${\mathfrak{S}}_f(4,4,2,2,4,2,6)$.

According to Schiffler et al. [31,32,33], if a snake graph $\mathfrak{S}$ consists of tiles $T_1,T_2,\dots ,T_k$. Then, there is an associated sequence of functions ${\mathcal{S}}_k=\{f_1,f_2,\dots ,f_k\}$, such that $f_i:T_i=\{e_w,e_s,e_e,e_n\}\to \{+,-\}$, $f_i\left(e_w\right)=f_i\left(e_n\right)\in \{+,-\}$, $f_i\left(e_w\right)\ne f_i\left(e_s\right)=f_i\left(e_e\right)\in \{+,-\}$, where $e_w$, $e_s$, $e_e$, $e_n$ denote the west, south, east, and north edges of tile $T_i$, respectively.

Note that the values of $f_i$ given by the internal edges, $f_1\left(e_s\right)$, $f_k\left(y\right)$, $y\in \{e_e,e_n\}$, and tiles $T_1,T_2,\dots ,T_k$ completely determine the snake graph $\mathfrak{S}$. For instance, if $f_i\left(e_w\right)=+$, then $f_i\left(e_n\right)=+$, and $f_i\left(e_e\right)=f_i\left(e_s\right)=-$. Figure 3 shows an example of a sequence ${\mathcal{S}}_k$ associated with the snake graph ${\mathfrak{G}}_f(4,4,2,2,4,2,6)$.

A positive finite continued fraction is a function

$$\begin{array}{cc}\hfill [g_1,g_2,\dots ,g_n]& =g_1+\frac{1}{g_2+\frac{1}{g_3+\frac{1}{g_4+\frac{1}{\ddots +\frac{1}{g_n}}}}}\hfill \end{array}$$

(6)

on n variables $g_1,g_2,\dots ,g_n$, $g_i\in {\mathbb{Z}}_{\ge 1}$.

Positive continued fractions are determined by their convergents denoted by $[g_1,g_2,\dots ,g_m]$, $1\le m\le n$. Note that n is finite if and only if the continued fraction gives a rational number denoted by $[g_1,g_2,\dots ,g_n]$.

Schiffler et al. [31,32,33] proved that there is a bijective correspondence between the set of positive continued fractions and the set of snake graphs via sequence ${\mathcal{S}}_k$. They denoted by $\mathfrak{S}[g_1,g_2,\dots ,g_n]$ the unique snake graph defined by the continued fraction $[g_1,g_2,\dots ,g_n]$. As an example, the snake graph of the continued fraction $[2,1,2,1,4,1,3,1,1,1,2]$ is ${\mathfrak{G}}_f(4,4,2,2,4,2,6)=\mathfrak{G}[2,1,2,1,4,1,3,1,1,1,2]$, as shown in Figure 3.

4. The Kronecker Problem

This section describes the solutions to the Kronecker problem formulated in the introduction. Snake graphs are invariants associated with such solutions.

The Kronecker problem was solved by Weierstrass in 1867 for some particular cases and by Kronecker in 1890 for the complex numbers field case. Solutions to this problem are classified as regular or non-regular (preprojective or pre-injective) [9,10,11].

Solutions to the Kronecker problem correspond to the indecomposable modules over the algebra $\Lambda =\left(\begin{array}{cc}\mathbb{F}& {\mathbb{F}}^2\\ 0& \mathbb{F}\end{array}\right)$, where $\mathbb{F}$ is a field and the multiplication is given by the formula

$$\left(\begin{array}{cc}{f}_{11}& f_{12}\\ 0& f_{22}\end{array}\right)\left(\begin{array}{cc}{g}_{11}& g_{12}\\ 0& g_{22}\end{array}\right)=\left(\begin{array}{cc}{f}_{11}{g}_{11}& f_{11}{g}_{12}+f_{12}{g}_{22}\\ 0& f_{22}{g}_{22}\end{array}\right)$$

(7)

Finite dimensional right $\Lambda$-modules are called Kronecker modules, and every such a module can be identified with a quadruple $\mathfrak{L}=L_1\stackrel{\stackrel{k_1}{\to }}{\underset{k_2}{\to }}{L}_2$, where $L_1$ and $L_2$ are the vector spaces $\mathfrak{L}\left(\begin{array}{cc}1& 0\\ 0& 0\end{array}\right)$, $\mathfrak{L}\left(\begin{array}{cc}0& 0\\ 0& 1\end{array}\right)$, $k_1$ and $k_2$ are linear maps defined by $k_1\left(x\right)=x\left(\begin{array}{cc}0& i\\ 0& 0\end{array}\right)$, $k_2\left(x\right)=x\left(\begin{array}{cc}0& j\\ 0& 0\end{array}\right)$, for $x\in L_1$, and $\{i,j\}$ is the standard basis of ${\mathbb{F}}^2$.

The category of Kronecker modules is categorically equivalent to the category of Kronecker matrices, so indecomposable Kronecker modules can be determined by solving the Kronecker matrix problem described in the introduction of this paper. Two Kronecker matrices $M=(A,B)$ and $M^{\prime }=(A^{\prime },B^{\prime })$ are said to be equivalent (or isomorphic as Kronecker modules) if one can be obtained from the other via matrix transformations.

It is worth noting that the Auslander–Reiten quiver associated with the Kronecker algebra $\Lambda$ has three components, which are the preprojective component containing all the indecomposable projective modules; the pre-injective component containing all the indecomposable injective modules; and the regular component. We let $(n+1,n)$ ($(n,n+1)$) denote a preprojective Kronecker module (pre-injective Kronecker module) whose associated Kronecker matrix has $n+1$ (n) rows and n ($n+1$) columns. The following matrices $\mathrm{II}$ ($\mathrm{III}$) show the standard form of the canonical pre-injective (preprojective) Kronecker modules.

$$\mathrm{II}={\mathrm{III}}^{*}=\left(\begin{array}{cccccccccccc}1& 0& \dots & \dots & 0& |& 0& 1& 0& \dots & 0& 0\\ 0& 1& \dots & \dots & 0& |& 0& 0& 1& \dots & 0& 0\\ 0& 0& 1& \dots & 0& |& 0& 0& 0& 1& \dots & 0\\ ⋮& ⋮& ⋮& & ⋮& ⋮& ⋮& ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& \dots & 1& 0& |& 0& 0& \ddots & \dots & 0& 1\end{array}\right)$$

(8)

$$\mathrm{III}={\mathrm{II}}^{*}=\left(\begin{array}{cccccccccccc}0& 0& \dots & \dots & 0& |& 1& 0& \dots & \dots & 0& 0\\ 1& 0& \dots & \dots & 0& |& 0& 1& \dots & \dots & 0& 0\\ 0& 1& \dots & \dots & 0& |& 0& 0& 1& \dots & 0& 0\\ ⋮& \ddots & \ddots & & ⋮& ⋮& ⋮& ⋮& ⋮& \ddots & ⋮& ⋮\\ 0& 0& \dots & 1& 0& |& 0& 0& \ddots & \dots & 0& 1\\ 0& 0& 0& \dots & 1& |& 0& 0& \dots & \dots & 0& 0\end{array}\right)$$

(9)

Each non-regular indecomposable Kronecker module $M=(A,B)$ has an associated finite set of directed graphs (directed paths) called helices by Espinosa [12]. To construct such graphs, the 1’s in the canonical non-regular Kronecker modules are called pivoting vertices or pivoting entries. Then, the helices are constructed by connecting with horizontal and vertical arrows alternatively two-element sets of entries. For instance, the sets $(a_1,b_1),(b_1,b_2),(b_2,a_2),\dots$ with $a_i\in A$, $b_j\in B$, $a_1\in R_1^A$ are connected first with a horizontal arrow then with a vertical arrow, and so on. In this case, the entries $b_1,a_2,b_3,a_4,\dots$ are pivoting entries and the process (of constructing the helix) ends once the helix has visited all the rows of the matrix M. The reader is referred to [9,12] for a more detailed description of a helix construction.

In [9], Espinosa et al. proved that the number of helices associated with the preprojective Kronecker module $(n+1,n)$ is $h_n^p=n!\lceil \frac{n}{2}\rceil$, and they used this result to categorize the integer sequence A052558 in the sense of Ringel and Fahr [13,14]. They also noted that each helix is given by a word $W_p$ of the form $ABBAABB\dots$ defined by the way that the helix visits the entries of the matrix M. Figure 4 is an example of a helix given by the word $ABBAABBA$.

Figure 5 shows examples of the helices associated with the indecomposable pre-injective Kronecker module $(2,3)$.

Note that each helix defines a snake graph; in such a case, the first horizontal arrow induced a horizontal straight snake graph whose tiles are given by the entries occurring from $a_1$ to $b_1$, the first vertical straight snake graph is given by the entries from $b_1$ to $b_2$ and so on. Henceforth, we assumed that the helices associated with preprojective and pre-injective Kronecker modules are snake graphs.

4.1. Automatic Sequences and Automatic Categories

A deterministic finite automaton (DFA) [17] is defined as a 5-tuple

$$M=(Q,\Sigma ,\delta ,q_0,F)$$

(10)

where

• Q is a finite set of states;
• $\Sigma$ is the finite input alphabet;
• $\delta :Q\times \Sigma \to Q$ is the transition function;
• $q_0\in Q$ is the initial state;
• $F\subseteq Q$ is the set of accepting states.

If the empty word $\epsilon \in \Sigma$, then $\delta (q,\epsilon )=q$ for all $q\in Q$. Furthermore, for all $q\in Q$, $x\in {\Sigma }^{*}$, and $a\in \Sigma$. It holds that

$$\delta (q,xa)=\delta \left(\delta \right(q,x),a).$$

(11)

The language $\mathcal{L}\left(M\right)$ accepted by M is defined in such a way that

$$\mathcal{L}\left(M\right)=\{w\in {\Sigma }^{*}\mid \delta (q_0,w)\in F\}.$$

(12)

A state q of a DFA is said to be reachable if there exists $x\in {\Sigma }^{*}$ such that $\delta (q_0,x)=q$, and it is unreachable otherwise [16,17,18].

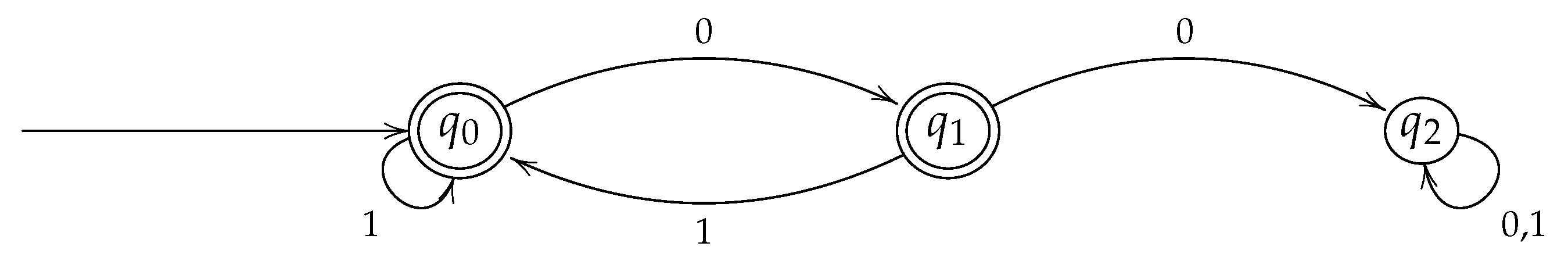
A DFA can be represented by a directed graph, a letter indicates the new state of the machine if the given letter is read. By convention, the initial state is drawn with an unlabeled arrow entering the state, and accepting states are drawn with double circles. For instance, let us consider the automaton $\mathcal{A}$ for which

• $Q=\{q_0,q_1,q_2\}$,
• $\Sigma ={\{0,1\}}^{*}$,
• $\delta (q_0,0)=q_1$,   $\delta (q_0,1)=q_0$,   $\delta (q_1,0)=q_2$,   $\delta (q_1,1)=q_0$,   $\delta (q_2,0)=\delta (q_2,1)=q_2$.
• $F=\{q_0,q_1\}$.

The following Figure 6 shows the graphical representation of the automaton $\mathcal{A}$, which accepts all strings over $\{0,1\}$ that do not contain two consecutive 0s.

4.2. Automatic Sequences

According to Shallit et al. [17], research on automatic sequences dates back to the 1960s with Büchi’s work [34], who attempted to prove that the set of powers of an integer $n\ge 2$ is k-automatic if and only if n and k are multiplicatively dependent. They also reiterate that Cobham [35] was the first to study k-automatic sequences systematically and that Deshouillers coined the term automatic sequence in 1979.

A DFA with output (DFAO) is a DFA M with two additional parameters $\Delta$ and $\tau$, such that $\Delta$ is the output alphabet and $\tau :Q\to \Delta$ is the output function. This machine induces a function $f_M:{\Sigma }^{*}\to \Delta$ such that $f_M\left(w\right)=\tau \left(\delta (q_0,w)\right)$. $f_M$ is said to be a finite-state function.

Note that, if $M=(Q,\Sigma ,\delta ,q_0,\Delta ,\tau )$, is a DFAO then the set $\{w\in {\Sigma }^{*}\mid \tau \left(\delta (q_0,w)\right)=d\}$ is a regular language.

A sequence ${\left(a_n\right)}_{n\ge 0}$ over a finite alphabet $\Delta$ is k-automatic if there is a k-DFAO, $M=(Q,{\Sigma }_k,\delta ,q_0,\Delta ,\tau )$, such that $a_n=\tau \left(\delta (q_0,w)\right)$ for all $n\ge 0$ and all w with ${\left[w\right]}_k=n$, i.e., ${\Sigma }_k$ the set $\{0,1,2,\dots ,k-1\}$ and ${\left[w\right]}_k={\displaystyle \sum _{1\le i\le t}}{a}_i{k}^{t-i}$ if $w=a_1{a}_2\dots a_t\in {\Sigma }^{*}$ [17].

Let $(k,b)$ be integers $\ge 2$. Let r be a real number, and suppose that $r=a_0+{\displaystyle \sum _{i\ge 1}}{a}_i{b}^{-i}$ for $i\ge 0$ and $0\le a_i<b$. Then, r is said to be $(k,b)$-automatic if the sequence of digits ${\left(a_i\right)}_{i\ge 0}$ is a k-automatic sequence. We let $L(k,b)$ denote the set of all $(k,b)$-automatic reals.

The Baum–Sweet sequence $a={\left(a_n\right)}_{n\ge 0}=110110010100100110010\dots$ is an example of an automatic sequence (see Figure 7).

The sequence ${\left(C_n\right)}_{n\ge 0}=0,1,2,1,2,2,0,1,2,2,0,2,0,0,0,1,\dots$ is 2-automatic. It coincides with the sequence of Catalan numbers modulo 4.

A subset $S\subseteq \mathbb{N}$ is k-automatic if there exists a regular language $L\subseteq {\Sigma }_k^{*}$ such that $S={\left[L\right]}_k=\{{\left[w\right]}_k\in w\in L\}$.

The following results regard automatic sequences.

Theorem 2

(Theorem 13.1.1, [17]). If r is a rational number, then $r\in L(k,b)$; for all $k,b\ge 2$, i.e., r is a $(k,b)$-automatic real number.

Theorem 3

(Theorem 13.1.5, [17]). If $r,s\in L(k,b)$ then $r+s\in L(k,b)$.

Corollary 1

(Theorem 13.1.7, [17]). The set $L(k,b)$ constitutes a vector space over the rational numbers.

Theorem 4

(Theorem 14.6.2, [17]). The sequence ${(\left(\genfrac{}{}{0pt}{}{n}{m}\right)modd)}_{m,n\ge 0}$ is k-automatic if and only if the integers d and k are powers of the same prime number p. In this case, the sequence is $p^j$-automatic for any $j\ge 0$.

It is worth pointing out that the class of k-automatic sets is closed under union, intersection complement, and set addition.

4.3. Automatic Categories

Let $\mathcal{C}$ be a Krull–Schmidt category whose indecomposable objects are automatic sets (i.e., they can be obtained as outputs of a DFA or a DFAO), then $\mathcal{C}$ is said to be an automatic category. Particularly, suppose a k-automatic sequence $\mathfrak{g}$ of real numbers is categorized (in the sense of Ringel and Fahr [13,14]) by the indecomposable objects of the category $\mathcal{C}$. In that case, it is said to be an k-automatic category with respect to the sequence $\mathfrak{g}$. For instance, Rees [19] proved that the string and band modules associated with a monomial algebra are automatic. To do that, she built an automaton that recognizes strings. It is worth pointing out that a unique band is associated with the Kronecker algebra. We recall that a sequence of letters (arrows) $\mathfrak{s}=a_n{a}_{n-1}\dots a_2{a}_1$ associated with a monomial algebra $\Lambda =\mathbb{F}Q/I$ is a string (of length n) if the following conditions hold:

• $t\left(a_j\right)=s\left(a_{j+1}\right)$, for all $1\le j\le n$;
• $a_j\ne a_j^{-1}$, note that $t\left(a_j\right)=s(a_j^{-1})$,   $s\left(a_j\right)=t(a_j^{-1})$;
• For all $1\le i<i+k\le n$, neither the sequence $a_{j+k}\dots a_j$ nor the sequence $u_j^{-1}\dots u_{j+k}^{-1}$ is contained in I.

A string $\mathfrak{b}$ of length $n\ge 1$ is cyclic if $s\left(\mathfrak{b}\right)=t\left(\mathfrak{b}\right)$. If additionally, there is no string $\mathfrak{s}$ such that the m-fold concatenation $\mathfrak{s}\mathfrak{s}\dots \mathfrak{s}$ equals $\mathfrak{b}$. Then, $\mathfrak{b}$ is a primitive cyclic string. If a primitive cyclic string $\mathfrak{b}=b_n\dots b_1$ is such that ${\mathfrak{b}}^m\ne 0$ for all $m\ge 1$ and $b_1$ is also an inverse letter whilst $b_n$ is a direct letter, then $\mathfrak{b}$ is a band.

Given a Krull–Schmidt category $\mathcal{C}$, a full subcategory L of $\mathcal{C}$ closed under direct sums and direct summands (and isomorphisms) will be called an object class in $\mathcal{C}$ [10]. In such a case, L is a Krull–Schmidt category, and is uniquely determined by the indecomposable objects belonging to L. $\langle M\rangle$ is the smallest object class containing M. $\langle M_1,M_2,\dots ,M_t\rangle$ is the smallest object classes, comprising $M_1,\dots ,M_t$ as elements.

5. Main Results

This section gives the main results of this paper. Firstly, we prove that, for $n>1$ fixed, the category $\langle (n+1,n)\rangle$ is automatic. In the last section, we introduce the skew brace induced by the helices associated with pre-injective Kronecker modules.

5.1. Automaticity Associated with Kronecker Modules

The following result proves that preprojective Kronecker modules give rise to automatic categories as a consequence of the Theorems 2—4 and Corollary 1.

Theorem 5.

For $t>1$ fixed, the category ${\langle (n+1,n)\rangle }_{2\le n\le t}$ is automatic.

Proof. 

For $2\le n\le t$, each helix ${\mathcal{H}}_{(n,a_{i_0{j}_0},p_{i_1{j}_1})}$ (associated with the preprojective Kronecker module $(n+1,n)$) starting and ending at the vertices ($a_{i_0{j}_0}\in i_A$ and $p_{i_1{j}_1}\in P_A$ ($P_B$) if n is odd (even)) define a DFA $(Q,\Sigma ,\delta ,q_0,F)$ such that

• The set of states Q is given by the entries of the preprojective Kronecker module $(n+1,n)$. We assume that the helix starts in an entry $a_{i_0{j}_0}\in i_A$ and ends in a vertex $p_{i_1{j}_1}\in P_X$, $X\in \{A,B\}$.
• The language $\Sigma ={\{A,B\}}^{*}\cup \left\{\epsilon \right\}$.
• The transition function $\delta :\Sigma \times Q\to Q$ is given by the arrows in the helix ${\mathcal{H}}_{(n,a_{i_0{j}_0},p_{i_1{j}_1})}$. We let ${\mathcal{H}}_0$ (${\mathcal{H}}_1$) be the corresponding set of vertices (arrows), ${\omega }_n=ABBAA\dots XXY$, and $X,Y\in \{A,B\}$ denotes the word associated with the helix such that $|{\omega }_n|=2n+2$.

  $$\delta (w,x)=\left\{\begin{array}{cc}\epsilon ,\hfill & \mathrm{for}\mathrm{any}w\in \Sigma ,x\in \{a_{i_{0}j},b_{i_{0}s}\mid j\ne j_0,1\le s\le n\}\mathrm{or}x\in {\left({\mathcal{H}}_0\right)}^c.\hfill \\ p_{i_1{j}_1},\hfill & \mathrm{if}w={\omega }_n=ABBAABB\dots XXYX,Y\in \{A,B\},\left|{\omega }_n\right|=2n.\hfill \\ s\left(Z\right),\hfill & \mathrm{if}w=ABB\dots XY\subset w^{\prime }=wZ=ABB\dots XYZ\subset {\omega }_n.\hfill \\ \epsilon ,\hfill & \mathit{if}\left|w\right|>2n+2\mathrm{or}w\mathrm{is}\mathrm{not}\mathrm{a}\mathrm{subword}\mathrm{associated}\mathrm{with}\mathrm{the}\mathrm{helix}.\hfill \\ x,\hfill & \mathrm{if}w=\epsilon .\hfill \end{array}\right.$$
• The initial state $q_0=a_{i_0{j}_0}$.
• The set of final states $F=\left\{p_{i_1{j}_1}\right\}$.

Since, preprojective Kronecker modules can be obtained via the union of helices, which are isomorphic as graphs. □

Note that the automaton $(Q,\Sigma ,\delta ,q_0,F)$ defined by the helix shown in Figure 4 is given by the following identities:

• $Q=\{p_{i,j}\mid 1\le i\le 4,1\le j\le 6\}$.
• $\Sigma ={\{A,B\}}^{*}\cup \left\{\epsilon \right\}$.
• $$\begin{array}{cc}\hfill \delta (AB,p_{4,2})& =p_{4,1},\delta (ABB,p_{4,1})=p_{3,1},\delta (ABBA,p_{3,1})=p_{3,2},\hfill \\ \hfill \delta (ABBAA,P_{3,2})& =p_{1,2},\delta (ABBAAB,p_{1,2})=p_{1,4},\hfill \\ \hfill \delta (ABBAABB,p_{1,4})& =p_{2,4},\delta (ABBAABBA,p_{2,4})=p_{2,5}\hfill \\ \hfill \delta (w,\epsilon )& =w,\mathrm{for}\mathrm{any}w\in \Sigma ,\hfill \\ \hfill \delta (w,x)& =\epsilon ,\mathrm{otherwise}.\hfill \end{array}$$

  (13)
• $q_0=p_{4,2}$.
• $F=\left\{p_{2,5}\right\}$.

Let us now introduce the sequences of continued fractions ${\mathfrak{g}}_{(n,k)}$ such that, for $n>2$ fixed and $2\le q\le n$, it holds that ${\mathfrak{g}}_{(n,k)}=[2,a_{n_1},2,a_{n_2},2,a_{n_3},3,a_{n_1},3,a_{n_4},3,a_{n_1},\dots ,3,b_n,2]$, $b_n=a_{n_1},a_{n_4}$, where the sequences $a_{n_i}$, $1\le i\le 4$ only consist of 1s and satisfy the following conditions:

• $|a_{n_1}|=n-2$.
• $|a_{n_2}|=k-3$.
• $|a_{n_3}|=n-k$.
• $|a_{n_4}|=n$.
• $$b_n=\left\{\begin{array}{cc}{a}_{n_1},\hfill & \mathrm{if}n\mathrm{is}\mathrm{odd},\hfill \\ a_{n_4},\hfill & \mathrm{if}n\mathrm{is}\mathrm{even}.\hfill \end{array}\right.$$

Corollary 2.

For $n>2$ and $2\le k\le n$, the sequence ${\mathfrak{g}}_{(n,k)}$ is automatic.

Proof. 

For $n>2$ and $2\le k\le n$, the fixed continued fraction

$${\mathfrak{g}}_{(n,k)}=[2,a_{n_1},a_{n_2},a_{n_3},3,a_{n_1},3,a_{n_4},3,a_{n_1},\dots ,3,b_n,2]$$

(14)

gives rise to a snake graph of the form

$$\mathcal{G}={\mathfrak{G}}_f\left(\right|a_{n_1}|+3,|a_{n_2}|+3,|a_{n_3}|+3,2,|a_{n_1}|+3,2,|a_{n_4}|+3,2,|a_{n_1}|+3,2,\dots ,2,|b_n|+3).$$

(15)

Vertical straight snake graphs of length 2 appear as transitions of the form $(1,3)$.

Now, we fix a preprojective Kronecker module with the form $(n+1,n)$ and assume that its entries $a_{i,j}$ determine tiles in such a way that the snake graph $\mathcal{G}$ corresponds to the helix with vertices

$a_{1,1}$; $a_{1,n+1}$; $a_{k,n+1}$; $a_{k,k-1}$; $a_{k-1,k-1};\dots$; $a_{k-t,n+(k-t)}$; $a_{k-(t+1),n+(k-t)}$; $a_{k-(t+1),k-(t+2)}$; $a_{k-(t+2),k-(t+2)}$, $1\le t\le k-2$.

Sequence ${\mathfrak{g}}_{(n,k)}$ is generated by an automaton $\mathfrak{A}=(Q,\Sigma ,q_0,F,\Sigma ,\delta )$ such that

• $Q=(n+1,n)=\{a_{i,j}\mid 1\le i\le n,1\le j\le n+1\}$.
• $\Sigma ={\{A,B\}}^{*}\cup \left\{\epsilon \right\}$.
• $q_0=a_{1,1}$.
• $F=\{a_{2,1},a_{2,n+2}\}$.
• The transition function $\delta :\Sigma \times Q\to Q$ is defined in such a way that for $1\le t\le k-2$, it holds that

  $$\delta (w,x)=\left\{\begin{array}{cc}{a}_{1,n+1},\hfill & \mathrm{if}w=AB,x=a_{1,1},\hfill \\ a_{k,n+1},\hfill & \mathrm{if}w=ABB,x=a_{1,n+1},\hfill \\ a_{k,k-1},\hfill & \mathrm{if}w=ABBA,x=a_{k,n+1},\hfill \\ a_{k-1,k-1},\hfill & \mathrm{if}w=ABBAA,x=a_{k,k-1},\hfill \\ a_{k-t,n+(k-t)},\hfill & \mathrm{if}w=ABBAA\dots AAB,x=a_{k-t,k-t},\hfill \\ a_{k-(t+1),n+(k-t)},\hfill & \mathrm{if}w=ABBAA\dots AABB,x=a_{k-t,n+(k-t)},\hfill \\ a_{k-(t+1),k-(t+2)},\hfill & \mathrm{if}w=ABBAA\dots AABBA,x=a_{k-(t+1),n+(k-t)},\hfill \\ a_{k-(t+2),k-(t+2)},\hfill & \mathrm{if}w=ABBAA\dots AABBAA,x=a_{k-(t+1),k-(t+2)},\hfill \\ x,\hfill & \mathrm{Otherwise}.\hfill \end{array}\right.$$
  In particular, $\delta (w,x)=x$ if $\left|w\right|>2k$ or $\left|w\right|<2k$ and w does not encode a subpath of a helix associated with the sequence ${\mathfrak{g}}_{(n,k)}$, (Figure 8 shows an example of an automaton that recognizes the sequence ${\mathfrak{g}}_{(n,k)}$).
  We note that the automaton $\mathfrak{A}$ recognizes words of the form $ABBAA\dots BBA$ or $ABBAA\dots AAB$ of length $\left|w\right|=2k$, $2\le k\le n$. □

Figure 8. Example of an automaton accepting the terms of the sequence ${\mathfrak{g}}_{(n,k)}$. $q_0=a_{1,1}$, $q_1=a_{1,n+1}$, $q_2=a_{k,n+1}$, and so on.

Figure 8. Example of an automaton accepting the terms of the sequence ${\mathfrak{g}}_{(n,k)}$. $q_0=a_{1,1}$, $q_1=a_{1,n+1}$, $q_2=a_{k,n+1}$, and so on.

5.2. Skew Braces Associated with Kronecker Modules

The result presented in this section proves that helices associated with pre-injective Kronecker modules give rise to skew braces. In this case, we assume that the matrix form of such modules are given as in identities (8) and (9), i.e., a pre-injective module P can be written as a matrix block $P=[E\mid F]$, where E and F are $n\times n+1$ matrices.

We let $({\mathfrak{H}}_{(n,n+1)},\circ )$ denote the set of helices associated with the pre-injective Kronecker module $(n,n+1)$ endowed with an operation ∘ (multiplication). In such a case, each helix $\mathfrak{h}$ can be written in the form:

$$\mathfrak{h}=\left(e_{p_{t_1}{q}_{s_1}}{f}_{p_{t_1}{q}_{s_2}},f_{p_{t_2}{q}_{s_2}}{e}_{p_{t_2}{q}_{s_3}},e_{p_{t_3}{q}_{s_3}}{f}_{p_{t_3}{q}_{s_4}},\dots ,l_{p_{t_n}{q}_{s_n}}{l}_{p_{t_n}{q}_{s_{n+1}}}\right)$$

(16)

where starting vertices are entries in the null column of matrix E, the $p_{t_i}’s$ visit all the rows of the indecomposable, $q_{s_1}=n+1$, $p_{t_i}\ne p_{t_j}$ if $i\ne j$ and $l\in \{e,f\}$.

∘ is defined in such a way that if $\mathfrak{h},{\mathfrak{h}}^{\prime }\in {\mathfrak{H}}_{(n,n+1)}$ then

$$\begin{array}{cc}\hfill \mathfrak{h}& =\left(e_{p_{t_1}{q}_{s_1}}{f}_{p_{t_1}{q}_{s_2}},f_{p_{t_2}{q}_{s_2}}{e}_{p_{t_2}{q}_{s_3}},e_{p_{t_3}{q}_{s_3}}{f}_{p_{t_3}{q}_{s_4}},\dots ,l_{p_{t_n}{q}_{s_n}}{l}_{p_{t_n}{q}_{s_{n+1}}}\right),\hfill \\ \hfill {\mathfrak{h}}^{\prime }& =\left(e_{p_{t_1}^{\prime }{q}_{s_1}^{\prime }}^{\prime }{f}_{p_{t_1}^{\prime }{q}_{s_2}^{\prime }}^{\prime },f_{p_{r_2}^{\prime }{q}_{s_2}^{\prime }}^{\prime }{e}_{p_{t_2}^{\prime }{q}_{s_3}^{\prime }}^{\prime },e_{p_{t_3}^{\prime }{q}_{s_3}^{\prime }}^{\prime }{f}_{p_{r_3}^{\prime }{q}_{s_4}^{\prime }}^{\prime },\dots ,l_{p_{t_n}^{\prime }{q}_{s_n}^{\prime }}^{\prime }{l}_{p_{t_n}^{\prime }{q}_{s_{n+1}}^{\prime }}^{\prime }\right)\hfill \end{array}$$

(17)

then

$$\mathfrak{h}\circ {\mathfrak{h}}^{\prime }=\left(e_{p_{t_{p_{t_1}^{\prime }}}{q}_{s_1}}^{\prime }{f}_{p_{t_{p_{t_1}^{\prime }}}{q}_{s^1(*)}}^{\prime },f_{p_{t_{p_{r_2}^{\prime }}}{q}_{s^1(*)}}^{\prime }{e}_{p_{t_{p_{r_2}^{\prime }}}{q}_{s^2(*)}}^{\prime },\dots ,l_{p_{t_{p_{r_n}^{\prime }}}{q}_{s^n(*)}}^{\prime }{l}_{p_{r_{p_{r_n}^{\prime }}}{q}_{s^{n+1}(*)}}^{\prime }\right)$$

(18)

with $l^{\prime }\in \{e,f\}$ and $p_{t_{p_{t_n}^{\prime }}}-q_{s^{n+1}(*)}=p_{t_n}^{\prime }-q_{s_n+1}$ or equivalently

$$q_{s^{n+1}(*)}=p_{t_{p_{t_n}^{\prime }}}-p_{t_n}^{\prime }+q_{s_n+1}.$$

It is possible to endow ${\mathfrak{H}}_{(n,n+1)}$ with another operation + (addition) by bearing in mind that the map f

$\left(e_{p_{t_1}{q}_{s_1}}{f}_{p_{t_1}{q}_{s_2}},f_{p_{t_2}{q}_{s_2}}{e}_{p_{t_2}{q}_{s_3}},e_{p_{t_3}{q}_{s_3}}{f}_{p_{t_3}{q}_{s_4}},\dots ,l_{p_{r_n}{q}_{s_n}}{l}_{p_{r_n}{q}_{s_{n+1}}}\right)\stackrel{f}{\to }(\begin{array}{ccccc}1& 2& 3& \dots & n\\ p_{t_1}& p_{t_2}& p_{t_3}& \dots & p_{t_n}\end{array})$ defines a bijection between ${\mathfrak{H}}_{(n,n+1)}$ and the symmetric set $S_n$. Henceforth, we assume that the notation $f^{-1}\left({\pi }_{\mathfrak{h}}\right)={\mathfrak{h}}_{\pi }\in {\mathfrak{H}}_{(n,n+1)}$, if ${\pi }_{\mathfrak{h}}\in S_n$.

+ is defined in such a way that, if $\mathfrak{h},{\mathfrak{h}}^{\prime }\in {\mathfrak{H}}_{(n,n+1)}$, then $\mathfrak{h}+{\mathfrak{h}}^{\prime }={\mathfrak{h}}_{{\pi }_2{\pi }_1}$ if $\mathfrak{h}={\mathfrak{h}}_{{\pi }_1}$ and ${\mathfrak{h}}^{\prime }={\mathfrak{h}}_{{\pi }_2}$ with ${\pi }_1,{\pi }_2\in S_n$.

The following result proves that helices associated with pre-injective Kronecker modules induce a skew brace, thus constituting a solution of the Yang–Baxter equation according to Lemma 1 and Theorem 1.

Theorem 6.

For $n>1$ fixed, the set of helices $({\mathfrak{H}}_{(n,n+1)},+,\circ )$ endowed with the addition + and multiplication ∘ as defined above is a skew brace.

Proof. 

Firstly, we will prove that $({\mathfrak{H}}_{(n,n+1)},+)$ and $({\mathfrak{H}}_{(n,n+1)},\circ )$ are groups. To do that, it suffices to note that, by definition, ${\mathfrak{H}}_{(n,n+1)}$ is closed under addition and multiplication. Since it is easy to see that these operations are associative. We will focus on the description of the corresponding units and inverses.

The identity element $1_n$ is a helix defined in the following fashion:

$$1_n=\left\{\begin{array}{cc}\left(e_{1n+1}{f}_{12},f_{22}{e}_{22},e_{32}{f}_{34},\dots ,l_{nn-1}{l}_{nn+1}\right)\hfill & \mathrm{if}n\mathrm{is}\mathrm{odd}\hfill \\ \left(e_{1n+1}{f}_{12},f_{22}{e}_{22},e_{32}{f}_{34},\dots ,l_{nn}{l}_{nn}\right)\hfill & \mathrm{if}n\mathrm{is}\mathrm{even}\hfill \end{array}\right.$$

(19)

Note that, $1_n={\mathfrak{h}}_e$ where $e=i{d}_{S_n}$ is the identity of the symmetry group $S_n$. The multiplicative inverse of a helix

$$\mathfrak{h}=\left(e_{p_{t_1}{q}_{s_1}}{f}_{p_{t_1}{q}_{s_2}},f_{p_{t_2}{q}_{s_2}}{e}_{p_{t_2}{q}_{s_3}},e_{p_{t_3}{q}_{s_3}}{f}_{p_{t_3}{q}_{s_4}},\dots ,l_{p_{t_n}{q}_{s_n}}{l}_{p_{t_n}{q}_{s_{n+1}}}\right)$$

is a helix ${\mathfrak{h}}^{-1}$ defined in such a way that

$${\mathfrak{h}}^{-1}=\left(e_{p_{t_1}^{\prime }{q}_{s_1}^{\prime }}^{\prime }{f}_{p_{t_1}^{\prime }{q}_{s_2}^{\prime }}^{\prime },f_{p_{t_2}^{\prime }{q}_{s_2}^{\prime }}^{\prime }{e}_{p_{t_2}^{\prime }{q}_{s_3}^{\prime }}^{\prime },e_{p_{t_3}^{\prime }{q}_{s_3}^{\prime }}^{\prime }{f}_{p_{t_3}^{\prime }{q}_{s_4}^{\prime }}^{\prime },\dots ,l_{p_{t_n}^{\prime }{q}_{s_n}^{\prime }}^{\prime }{l}_{p_{t_n}^{\prime }{q}_{s_{n+1}}^{\prime }}^{\prime }\right)$$

where $p_{t_{p_{t_i}^{\prime }}}=i$, for all $1\le i\le n$.

On the other hand, ${\mathfrak{h}}_e=0$ provided that

$${\mathfrak{h}}_e+\mathfrak{h}={\mathfrak{h}}_e+{\mathfrak{h}}_{\pi }={\mathfrak{h}}_{\pi e}={\mathfrak{h}}_{\pi }=\mathfrak{h},\mathrm{for}\mathrm{any}\mathfrak{h}\in {\mathfrak{H}}_{(n,n+1)}\mathrm{and}\mathrm{some}\pi \in S_n.$$

(20)

For any $\mathfrak{h}={\mathfrak{h}}_{\pi }$ with $\pi \in S_n$, it holds that

$${\mathfrak{h}}_{\pi }+{\mathfrak{h}}_{{\pi }^{-1}}={\mathfrak{h}}_{\pi {\pi }^{-1}}={\mathfrak{h}}_e=\mathfrak{h}.$$

(21)

Thus, $-{\mathfrak{h}}_{\pi }={\mathfrak{h}}_{{\pi }^{-1}}=-\mathfrak{h}$.

Finally, we note that for all helices $\mathfrak{h},{\mathfrak{h}}^{\prime },{\mathfrak{h}}^{″}\in {\mathfrak{H}}_{(n,n+1)}$ with $\mathfrak{h}={\mathfrak{h}}_{{\pi }_i}$, ${\mathfrak{h}}^{\prime }={\mathfrak{h}}_{{\pi }_j}$, ${\mathfrak{h}}^{″}={\mathfrak{h}}_{{\pi }_k}$ for some ${\pi }_i,{\pi }_j,{\pi }_k\in S_n$, it holds that;

$$\begin{array}{cc}\hfill {\mathfrak{h}}_{{\pi }_i}+({\mathfrak{h}}_{{\pi }_j}\circ {\mathfrak{h}}_{{\pi }_k})& ={\mathfrak{h}}_{{\pi }_i}+{\mathfrak{h}}_{{\pi }_j{\pi }_k}={\mathfrak{h}}_{{\pi }_j{\pi }_k{\pi }_i}={\mathfrak{h}}_{{\pi }_i}+{\mathfrak{h}}_{{\pi }_k}+{\mathfrak{h}}_{{\pi }_j}.\hfill \\ \hfill ({\mathfrak{h}}_{{\pi }_i}+{\mathfrak{h}}_{{\pi }_j})\circ \left({\mathfrak{h}}_{{\pi }_i}^{-1}\right)\circ ({\mathfrak{h}}_{{\pi }_i}+{\mathfrak{h}}_{{\pi }_k})& ={\mathfrak{h}}_{{\pi }_j{\pi }_i}\circ {\mathfrak{h}}_{{\pi }_i^{-1}}\circ {\mathfrak{h}}_{{\pi }_k{\pi }_i}={\mathfrak{h}}_{{\pi }_j{\pi }_i{\pi }_i^{-1}{\pi }_k{\pi }_i}={\mathfrak{h}}_{{\pi }_j{\pi }_k{\pi }_i}.\hfill \end{array}$$

(22)

Thus, $({\mathfrak{H}}_{(n,n+1)},+\circ )$ is a skew brace. □

Remark 1.

We note that some details included in the proof of Theorem 6 can be omitted, taking into account that, according to Vendramin et al. [22], groups give rise to skew braces, also called almost trivial skew braces by Koch et al. [36]. However, for the sake of clarity, we prove that $({\mathfrak{h}}_{(n,n+1)},+,\circ )$ satisfies all the properties that make it a skew brace.

5.3. Discussion

This paper provides new applications of the non-regular modules over the Kronecker algebra. On the one hand, snake graphs associated with preprojective Kronecker modules allow proving the automaticity of some continued fraction sequences. On the other hand, snake graphs associated with pre-injective Kronecker modules give rise to particular classes of skew braces that define the set-theoretical solutions of the Yang–Baxter equation (see Lemma 1 and Theorem 1).

As an example, the following are the elements of $({\mathfrak{H}}_{(3,4)},+,\circ )$, where $h_{\left(ijk\right)}$ denotes the helix associated with the permutation $\left(\begin{array}{ccc}1& 2& 3\\ i& j& k\end{array}\right)$, $i,j,k\in \{1,2,3\}$ denoted $\left(\begin{array}{ccc}i& j& k\end{array}\right)$;

$$h_1=h_{\left(123\right)}=\left(e_{14}{f}_{12},f_{22}{e}_{22},e_{32}{f}_{34}\right)$$

$$h_2=h_{\left(132\right)}=\left(e_{14}{f}_{12},f_{32}{e}_{33},e_{23}{f}_{23}\right)$$

$$h_3=h_{\left(213\right)}=\left(e_{24}{f}_{23},f_{13}{e}_{11},e_{31}{f}_{34}\right)$$

$$h_4=h_{\left(231\right)}=\left(e_{24}{f}_{23},f_{33}{e}_{33},e_{13}{f}_{12}\right)$$

$$h_5=h_{\left(321\right)}=\left(e_{34}{f}_{34},f_{24}{e}_{22},e_{12}{f}_{12}\right)$$

$$h_6=h_{\left(312\right)}=\left(e_{34}{f}_{34},f_{14}{e}_{11},e_{21}{f}_{23}\right).$$

Some products

$$\begin{array}{cc}\hfill h_1\circ h_2& =\left(e_{14}{f}_{12},f_{22}{e}_{22},e_{32}{f}_{34}\right)\circ \left(e_{14}{f}_{12},f_{32}{e}_{33},e_{23}{f}_{23}\right)\hfill \\ \hfill & =\left(e_{14}{f}_{12},f_{32}{e}_{33},e_{23}{f}_{23}\right)=h_2=h_1+h_2.\hfill \end{array}$$

$$\begin{array}{cc}\hfill h_3\circ h_3& =\left(e_{24}{f}_{23},f_{13}{e}_{11},e_{31}{f}_{34}\right)\circ \left(e_{24}{f}_{23},f_{13}{e}_{11},e_{31}{f}_{34}\right)\hfill \\ \hfill & =\left(e_{14}{f}_{12},f_{22}{e}_{22},e_{32}{f}_{34}\right)=h_1=h_3+h_3.\hfill \end{array}$$

The Cayley table of $({\mathfrak{H}}_{3,4},\circ )$ appears as follows:

∘	$h_1$	$h_2$	$h_3$	$h_4$	$h_5$	$h_6$
$h_1$	$h_1$	$h_2$	$h_3$	$h_4$	$h_5$	$h_6$
$h_2$	$h_2$	$h_1$	$h_6$	$h_5$	$h_4$	$h_3$
$h_3$	$h_3$	$h_4$	$h_1$	$h_2$	$h_6$	$h_5$
$h_4$	$h_4$	$h_3$	$h_5$	$h_6$	$h_2$	$h_1$
$h_5$	$h_5$	$h_6$	$h_4$	$h_3$	$h_1$	$h_2$
$h_6$	$h_6$	$h_5$	$h_2$	$h_1$	$h_3$	$h_4$

The Cayley table of $({\mathfrak{H}}_{3,4},+)$ has the following shape:

+	$h_1$	$h_2$	$h_3$	$h_4$	$h_5$	$h_6$
$h_1$	$h_1$	$h_2$	$h_3$	$h_4$	$h_5$	$h_6$
$h_2$	$h_2$	$h_1$	$h_4$	$h_3$	$h_6$	$h_5$
$h_3$	$h_3$	$h_6$	$h_1$	$h_5$	$h_4$	$h_2$
$h_4$	$h_4$	$h_5$	$h_2$	$h_6$	$h_3$	$h_1$
$h_5$	$h_5$	$h_4$	$h_6$	$h_2$	$h_1$	$h_3$
$h_6$	$h_6$	$h_3$	$h_5$	$h_1$	$h_2$	$h_4$

6. Concluding Remarks

This paper explored new interactions between the representation theory of the Kronecker algebra and studies dealing with the Yang–Baxter equation and automatic sequences. On the one hand, it is proven that preprojective Kronecker modules are automatic in the sense that a suitable automaton generates them. Actually, it is possible to conclude that Krull–Schmidt categories generated by a finite number of preprojective Kronecker modules are automatic. This result is obtained provided that any non-regular module over the Kronecker algebra has an associated set of snake graphs, as such snake graphs allow one to prove that some sequences of continued fractions are also automatic.

On the other hand, it is proven that the snake graphs associated with pre-injective Kronecker modules give rise to the solutions of the Yang–Baxter equation. To do that, such a set of snake graphs is endowed with two non-commutative operations, making it a skew brace.

Future Work

The following are interesting tasks to carry out in the future.

• To define automatic sequences based on invariants of indecomposable modules of different Krull–Schmidt categories.
• To determine the braces via solutions of generalized matrix problems.