Relations of Society Concepts and Religions from Wikipedia Networks

Abstract

We analyze the Google matrix of directed networks of Wikipedia articles related to eight recent Wikipedia language editions representing different cultures (English, Arabic, German, Spanish, French, Italian, Russian, Chinese). Using the reduced Google matrix algorithm, we determine relations and interactions of 23 society concepts and 17 religions represented by their respective articles for each of the eight editions. The effective Markov transitions are found to be more intense inside the two blocks of society concepts and religions while transitions between the blocks are significantly reduced. We establish five poles of influence for society concepts (Law, Society, Communism, Liberalism, Capitalism) as well as five poles for religions (Christianity, Islam, Buddhism, Hinduism, Chinese folk religion) and determine how they affect other entries. We compute inter-edition correlations for different key quantities providing a quantitative analysis of the differences or the proximity of views of the eight cultures with respect to the selected society concepts and religions.

1. Introduction

During human society evolution, several concepts of society have been developed. Their relations and interactions are studied by social sciences, as discussed, for example in [1,2]. Among these concepts there are those linked to social society structure, as, e.g., law, education, culture, and political formations, as, e.g., capitalism, socialism, communism and others. These concepts represent social concepts of society. Other concepts are represented by religions, which play an important role in human society formation. The roles and relations of religions and human societies have been studied and investigated from various view points including history, philosophy, psychology, archaeology, economy and other sciences (see, e.g., [3,4,5,6,7,8,9] and Refs. therein). However, the mathematical analysis of these relations is essentially absent since it is rather difficult to use and apply rigorous mathematical methods to human sciences and religions. In this work, we try to develop a mathematical framework to investigate religions and society concepts relations based on the Wikipedia network analysis. We consider a directed network composed of Wikipedia articles (nodes) with generated hyperlinks (citations) between them. By two decades ago, the free online Wikipedia has superseded old encyclopedias such as Encyclopedia Britannica [10] in quality and volume of articles related to scientific topics [11]. At present, the academic analysis of information contained in Wikipedia finds more and more applications as reviewed and described in [12,13,14,15,16]. Thus, Wikipedia contains an enormous diverse database of human knowledge that we use to determine relations between religions and society concepts obtained on purely mathematical grounds. Of course, religions are also human concepts related to society but for clarity we consider two groups of concepts formed by society: society social concepts and religions.

We analyze Wikipedia networks of different language editions by the Google matrix approach and PageRank algorithm, which was originally developed and applied by Brain and Page for the World Wide Web search analysis [17,18]. We also use additional algorithms such as CheiRank [19,20,21] and the reduced Google matrix (REGOMAX) [22]. Various applications of these algorithms with an analysis of the importance and interactions of various Wikipedia articles and groups of articles have been reported for historical figures [20,23], world universities [20,24], politicians [22], banks and world countries [25] and other examples. The REGOMAX algorithm is particularly useful since it allows one to determine effective interactions between nodes of a selected group taking into account direct and all indirect pathways between these nodes via the global network with a size exceeding by orders of magnitude the number of selected group nodes. These tools have been shown to operate efficiently not only for Wikipedia networks but also for the world trade network of UN COMTRADE [26] and the MetaCore network of protein–protein interactions [27]. A pedestrian explanation and mathematical foundations of the REGOMAX algorithm are presented below (see Section 3.3).

In this work, we focus on the directed networks obtained from eight actual Wikipedia language editions: English (EN), Arab (AR), German (DE), Spanish (ES), French (FR), Italian (IT), Russian (RU) and Chinese (ZH). We consider that these eight networks describe eight different cultures since a language is one of the most important elements of culture. The eight networks are generated from Wikipedia edition dumps of 1 October 2024 and the main network parameters are given in

Table 1. Table of the 8 used Wikipedia editions (code in 2nd column) in different languages (1st column) and corresponding network data where N denotes the number of network nodes (3rd column), $N_{\mathsf{\ell }}$ the total number of links (4th column) and $N_d$ the number of dangling nodes (5th column). The last column provides the ratio $N_{\mathsf{\ell }}/N$. All networks were created from Wikipedia xml-dump files dated from 1 October 2024 (excluding redirection and special technical nodes and links).

Language	Edition	N	${\mathit{N}}_{\mathsf{\ell }}$	${\mathit{N}}_{\mathit{d}}$	${\mathit{N}}_{\mathsf{\ell }}/\mathit{N}$
English	EN	6,891,535	185,658,675	18,444	26.9
Arabic	AR	1,242,011	16,433,487	20,467	13.2
German	DE	2,946,636	79,189,123	20,099	26.9
Spanish	ES	1,916,240	41,324,254	4243	21.6
French	FR	2,638,634	76,118,849	3567	28.8
Italian	IT	1,884,339	49,495,890	7622	26.3
Russian	RU	2,002,167	43,375,388	7630	21.7
Chinese	ZH	1,444,719	20,682,593	58,250	14.3

.

For our analysis, we select from Wikipedia 40 articles about human concepts composed of 23 society concepts and 17 religions (and branches of religions) given in

Table 2. Table of the subset of $N_r=40$ selected Wikipedia articles (nodes) from the English Wikipedia edition EN with 23 society articles ($K_g=1,\dots ,23$) and 17 religion articles ($K_g=24,\dots ,40$) both separated by an additional horizontal line. All data in this table refer to the English Wikipedia edition EN. Here, $K_g$ represents the global index of this group (1st column) obtained by first PageRank ordering the 23 society nodes and, subsequently, PageRank ordering the 17 religion nodes. The other columns correspond to the group-local K- and $K^{*}$-rank indices (2nd and 3rd columns), the exact (English) Wikipedia article title (4th column), a short two-character code for each article (5th column), network global $K_{\mathrm{M}}$- and $K_{\mathrm{M}}^{*}$-rank indices (6th and 7th columns) and a subgroup (pole) index (8th column). In this table, PageRank ordering K- and $K^{*}$-indices were computed using the English Wikipedia edition EN. The two-character code for each article is by default obtained from the first two characters of the title with some modifications for the 2nd character to avoid double codes or one of the 8 codes already used for the 8 Wikipedia language editions (see 2nd column in Table 1). The subgroup index defines for each society and religion block five subgroups and the label “(T)” defines a top pole node for each subgroup. The two-character codes, the pole index and top node label are used later in the network diagrams presented and discussed in Section 4.3.

${\mathit{K}}_{\mathit{g}}$	K	${\mathit{K}}^{*}$	Title	Code	${\mathit{K}}_{\mathbf{M}}$	${\mathit{K}}_{\mathbf{M}}^{*}$	Subgroup
1	7	28	Law	LA	387	101,448	1(T)
2	10	36	Education	ED	531	323,268	2
3	11	15	Communism	CM	690	26,967	3(T)
4	12	19	Democracy	DM	750	41,331	4
5	13	16	Liberalism	LI	755	28,568	4(T)
6	14	21	Socialism	SO	837	42,483	3
7	16	20	Ecology	EL	988	41,611	1
8	17	3	Politics	PO	1072	4444	1
9	18	9	Culture	CU	1190	10,959	2
10	19	22	Nazism	NA	1234	49,141	5
11	20	30	Capitalism	CA	1282	117,558	5(T)
12	22	34	Republic	RE	1652	283,031	4
13	25	11	Monarchy	MR	1903	15,001	2
14	27	27	Fascism	FA	2089	89,729	5
15	28	4	Society	SY	2448	6032	2(T)
16	29	33	Economy	EC	2560	261,158	5
17	31	5	Anarchism	AN	3663	8827	3
18	33	39	Money	MO	4624	425,841	5
19	34	40	Oligarchy	OL	5759	1,200,109	2
20	35	8	Civilization	CI	6035	9853	2
21	36	37	Autocracy	AU	6074	324,067	2
22	38	35	Materialism	MA	7709	316,619	1
23	40	31	Idealism	ID	11,579	130,647	1
24	1	6	Catholic Church	CC	60	8870	1
25	2	2	Christianity	CH	85	1307	1(T)
26	3	7	Islam	IS	96	9758	2(T)
27	4	17	Buddhism	BU	188	28,678	3(T)
28	5	10	Hinduism	HI	255	11,219	4(T)
29	6	18	Eastern Orthodox Church	EO	353	31,721	1
30	8	12	Judaism	JU	401	16,492	1
31	9	13	Protestantism	PR	405	22,902	1
32	15	24	Sunni Islam	SU	890	63,317	2
33	21	1	Jainism	JA	1361	275	4
34	23	25	Sikhism	SI	1659	64,136	4
35	24	32	Confucianism	CO	1794	139,315	3
36	26	14	Shia Islam	SM	1945	26,758	2
37	30	23	Taoism	TA	2576	50,597	3
38	32	29	Shinto	SH	3999	116,232	5
39	37	26	Chinese folk religion	CF	6105	65,071	5(T)
40	39	38	Oriental Orthodox Churches	OO	9789	331,323	1

. These 40 concepts are analyzed for each of eight Wikipedia edition networks. We show that the REGOMAX algorithm allows us to determine nontrivial interactions among the 23 selected society concepts, 17 religions and also relations between these two groups for each of the eight networks of Table 1. Furthermore, the obtained results also allow to quantitatively characterize the correlations between these eight cultures.

This paper is composed as follows: In Section 2, we present some technical details about the used data sets, and in Section 3 the mathematical tools used for network and correlation analysis are briefly reviewed. Section 4 presents and provides a detailed discussion of our results, and Section 5 provides the final discussion and conclusion.

Additional material, additional figures and data files are available at [28].

2. Data Sets of Wikipedia Networks

2.1. Construction of the Wikipedia Networks

To construct the Wikipedia networks for the different language editions of Table 1, we downloaded the official xml-dump files for each edition (versions with all pages and articles in a single file) with time stamp of 1 October 2024. First, we extracted for a given edition the titles of all main content articles with namespace key value being 0, thus excluding technical Wikipedia articles of type Category, Help, File, Wikemedia, etc., having other namespace key values. Also, titles corresponding to a redirection to another existing article were not taken into account. This procedure provides the list of all article titles/network nodes with a technical index given by the initial order of the xml-dump file, which is typically roughly alphabetical (but not exactly). This technical initial index, while being important to store the network links and for the different computation codes, will not be used in this work to characterize specific articles/nodes. Instead, we will use the PageRank index $K_M$ (see below) to characterize different nodes.

In a second step, for each article we determined the links to other articles in the same Wikipedia edition. These links are (with some technical complications) available in the xml-dump files as clear text article titles but sometimes it is necessary to capitalize the first character of a link name to identify the proper article title, which is always capitalized for the first character, and the corresponding node index. This capitalization procedure is a technical nontrivial operation for accented or special characters (with multibyte utf8 codes), e.g., in the case of French or Russian article titles, for which we used the library Utf8proc [29]. Links to redirection titles, outside webpages or other Wikipedia editions were not taken into account. Multiple links between two articles, in particular several links pointing to a particular position inside the target article, were counted as a single unique link. Links from an article to itself were kept but also only as a single link.

In this way, we obtained the Wikipedia networks with full “official” lists of article titles for the 8 editions and tables of links, with some key values shown in Table 1, e.g., with typical node numbers between $N\approx 6.9\times {10}^6$ (for the English Wikipedia edition EN) and $N\approx 1.2\times {10}^6$ (for the Arabic Wikipedia edition AR) and typical link numbers between $N_{\mathsf{\ell }}\approx 1.9\times {10}^8$ (EN) and $N\approx 1.6\times {10}^7$ (AR). The ratio $N_{\mathsf{\ell }}/N$ between both has typical values of 13–27. Table 1 also shows the number of dangling nodes $N_d$ corresponding to nodes having no outgoing link to another Wikipedia article of the same edition. These nodes require special treatment in the Google matrix approach (see next section) and the typical values are $N_d\sim {10}^3$–${10}^5$, which represents a very small fraction of the full number of nodes $N\sim {10}^6$–${10}^7$.

2.2. Edition-Specific Subsets

Starting with English Wikipedia, we select a group of 40 articles about 23 society concepts and 17 religions (or branches of religions) shown in Table 2. The order of these articles is fixed by first PageRank ordering the 23 society nodes and then the 17 religion nodes providing the group index $K_g$. Then, for each of the other editions, we determine an equivalent group using the official Wikipedia links between two different editions. We note that most EN-Wikipedia articles (all among the group of Table 2) provide a language submenu at the top, allowing a reader to select the exactly corresponding article in another language (another Wikipedia edition) if it exists. For this, we downloaded the source html files of the 40 EN-articles and extracted from these files the corresponding article titles of the other editions. This information is indeed contained in these source files in the other language submenu where the corresponding articles in other language editions can be selected from a given English Wikipedia page. It turns out that for all the other 7 language editions and each of the 40 EN-articles of Table 2, we were able to find the corresponding article associated to the other edition (for some other language Wikipedia editions, not used in this work here, the number of found “translated” articles may be less than 40).

We note that this procedure is important and that simple linguistic title translation may lead to wrong articles in the other edition. For example, the EN-article “Society” ($K_g=15$ in Table 2) translates linguistically to “Gesellschaft” in German but the DE-article “Gesellschaft” corresponds more to a technical article with links to several other DE-articles using the word “Gesellschaft” in the title and for different contexts (e.g., sociology, ethonology, state-law etc.). However, the official inter-edition Wikipedia link from the EN-article “Society” to the DE-Wikipedia edition provides the title “Gesellschaft (Soziologie)” which is a different DE-Wikipedia article than “Gesellschaft”. There are some other similar examples like this, also for FR-Wikipedia. In particular, both articles about the Orthodox Church with very specific differences between them (with $K_g=29,40$ in Table 2), require use of the official inter-edition Wikipedia links as simple linguistic translation may easily lead to the wrong article.

In this work, we will for convenience always use (in Tables and Figures below) the English article titles given in Table 2, even when we speak about another edition. Of course, the network index values of the 40 group nodes, necessary for the computation of the reduced Google matrix (see below), were correctly determined individually for each edition using the properly translated group list for the same edition, eventually using article titles in special character sets (especially for AR, RU, ZH).

In

Table 3. Table of local K- and $K^{*}$-indices of the subset of $N_r=40$ selected Wikipedia articles (nodes) of Table 2 obtained from the networks of all 8 Wikipedia editions of Table 1. For each edition (other than EN) a subset of $N_r=40$ articles was selected by using the official Wikipedia translation of the titles of Table 2 for EN to the titles of the corresponding edition in the other language (AR to ZH). For each edition-specific group, the reduced matrix $G_R$, PageRank and CheiRank vector were computed using the corresponding edition Wikipedia network providing local K- and $K^{*}$-indices visible with two values $K;K^{*}$ per entry in columns 3 to 10 (for the 8 editions). The columns 1 and 2 provide the index $K_g$ and the short code for each node defined in Table 2. The additional horizontal line separates the society nodes ($K_g\le 23$) from the religion nodes ($K_g\ge 24$). The group nodes of each edition are also visible in Figure 1 and Figure 2, showing the global Wikipedia network structure for each edition in the $ln\left(K_{\mathrm{M}}\right)$-$ln\left(K_{\mathrm{M}}^{*}\right)$ plane.

${\mathit{K}}_{\mathit{g}}$	Code/	EN	AR	DE	ES	FR	IT	RU	ZH
	Node	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$	$\mathit{K};{\mathit{K}}^{*}$
1	LA	7; 28	5; 18	17; 8	5; 15	5; 37	5; 22	15; 14	3; 22
2	ED	10; 36	15; 3	25; 40	11; 30	19; 33	26; 28	20; 26	8; 7
3	CM	11; 15	16; 22	13; 23	18; 10	10; 2	12; 13	16; 9	13; 1
4	DM	12; 19	10; 12	6; 7	6; 22	11; 14	11; 10	13; 19	12; 12
5	LI	13; 16	22; 16	12; 22	15; 35	13; 13	16; 14	18; 28	25; 19
6	SO	14; 21	21; 28	18; 17	21; 9	18; 4	15; 23	10; 15	16; 16
7	EL	16; 20	27; 15	20; 28	22; 12	17; 17	22; 26	21; 21	28; 30
8	PO	17; 3	4; 26	10; 25	7; 31	6; 11	8; 31	14; 37	4; 28
9	CU	18; 9	17; 17	8; 15	12; 17	14; 21	10; 19	12; 32	7; 34
10	NA	19; 22	25; 13	2; 13	23; 13	12; 22	21; 11	28; 12	32; 38
11	CA	20; 30	20; 21	23; 10	20; 23	22; 16	19; 20	22; 11	23; 10
12	RE	22; 34	18; 35	21; 38	17; 27	8; 31	20; 30	19; 36	15; 23
13	MR	25; 11	23; 37	14; 6	19; 6	21; 32	17; 21	17; 1	17; 9
14	FA	27; 27	29; 31	22; 11	24; 5	27; 6	9; 3	26; 8	33; 20
15	SY	28; 4	7; 24	19; 26	16; 34	26; 39	18; 40	11; 35	22; 32
16	EC	29; 33	12; 33	7; 27	3; 38	20; 40	4; 6	7; 33	14; 39
17	AN	31; 5	30; 7	27; 4	26; 1	28; 1	38; 24	30; 3	29; 5
18	MO	33; 39	26; 40	26; 14	25; 36	24; 23	29; 37	24; 20	20; 27
19	OL	34; 40	37; 38	31; 39	33; 39	30; 35	31; 38	36; 34	36; 14
20	CI	35; 8	24; 8	35; 36	30; 21	32; 27	30; 33	27; 31	24; 25
21	AU	36; 37	36; 36	36; 37	37; 40	37; 38	37; 39	39; 40	30; 29
22	MA	38; 35	38; 27	33; 30	35; 14	35; 29	34; 17	33; 23	39; 26
23	ID	40; 31	40; 25	38; 33	36; 37	38; 10	32; 32	35; 39	38; 36
24	CC	1; 6	3; 14	1; 16	1; 2	3; 26	1; 1	4; 27	10; 13
25	CH	2; 2	2; 1	3; 9	2; 3	1; 12	2; 4	2; 4	1; 17
26	IS	3; 7	1; 2	4; 1	4; 4	2; 3	3; 5	1; 7	5; 11
27	BU	4; 17	11; 11	11; 3	9; 7	7; 5	25; 8	5; 6	2; 3
28	HI	5; 10	13; 10	16; 5	14; 8	15; 7	14; 9	8; 5	9; 24
29	EO	6; 18	19; 19	15; 20	13; 16	16; 20	13; 15	23; 16	34; 15
30	JU	8; 12	9; 4	5; 12	8; 11	9; 9	6; 2	6; 13	18; 37
31	PR	9; 13	6; 9	9; 19	10; 18	4; 15	7; 18	3; 18	6; 31
32	SU	15; 24	8; 5	24; 2	27; 32	23; 36	23; 34	9; 30	21; 18
33	JA	21; 1	34; 32	34; 31	34; 19	34; 8	36; 16	34; 17	27; 33
34	SI	23; 25	28; 20	30; 21	29; 20	29; 18	27; 27	32; 10	31; 35
35	CO	24; 32	32; 34	28; 34	32; 29	33; 34	33; 29	31; 29	19; 4
36	SM	26; 14	14; 6	32; 24	31; 24	25; 25	24; 25	25; 22	26; 21
37	TA	30; 23	31; 29	29; 18	28; 25	31; 19	28; 12	29; 24	11; 2
38	SH	32; 29	35; 30	37; 29	38; 28	36; 24	35; 7	38; 2	35; 8
39	CF	37; 26	39; 39	40; 32	40; 26	39; 30	40; 35	40; 38	40; 6
40	OO	39; 38	33; 23	39; 35	39; 33	40; 28	39; 36	37; 25	37; 40

, we summarize the group local PageRank and CheiRank indices K and $K^{*}$ (see next section) for all 40 subset nodes and all 8 Wikipedia editions of Table 1, using for each edition the properly translated group for this edition as described above.

3. Google Matrix Algorithms

3.1. Google Matrix Construction

We construct the Google matrix G of the different Wikipedia networks with N nodes (articles) in the usual way [17,18,21]. First, we define the adjacency matrix A by $A_{ij}=1$ if node j points to node i (if there is a link from the Wikipedia article j to the article i) and $A_{ij}=0$ otherwise. Then, the stochastic matrix S of Markov transitions is defined by $S_{ij}=A_{ij}/{\sum }_l{A}_{lj}$ for columns j with ${\sum }_l{A}_{lj}>0$. For dangling nodes j, having no outgoing links, i.e., with ${\sum }_l{A}_{lj}=0$, we simply define $S_{ij}=1/N$ for all values of i. The columns of S are sum normalized, i.e., ${\sum }_l{S}_{lj}=1$, and it conserves the total probability when the matrix S is multiplied to an arbitrary probability vector P, i.e., ${\sum }_i\tilde{P}\left(i\right)={\sum }_{i}P\left(i\right)=1$ if $\tilde{P}=SP$.

Finally, the Google matrix elements are defined as

$$G_{ij}=\alpha S_{ij}+(1-\alpha )/N$$

(1)

where $0.5\le \alpha <1$ is the damping factor for which we choose the usual standard value $\alpha =0.85$ [18,21]. The columns of G are also column sum normalized and it also conserves the probability in the same way as the matrix S.

Physically, this matrix describes a stochastic process where a random surfer jumps over the network. With a probability $\alpha$ the surfer jumps randomly from his actual page j to a random page page i among the pages with existing links $j\to i$, and with a complementary probability $\left(1-\alpha \right)$, he jumps to an arbitrary random node of the network. For dangling nodes, he jumps immediately to an arbitrary random node. The damping factor allows one to connect all isolated communities and avoids a situation where the random surfer is trapped inside a small finite subset of nodes with no links going outside this subset.

3.2. PageRank, CheiRank

Due to the damping factor, the stochastic process defined by G converges, according to the Perron–Frobenius theorem. In the long term, this limits to a stationary probability $P={lim}_{t\to \infty }{G}^t{P}_0$, where $P_0$ is an arbitrary initial probability vector (with real values, $P_0\left(i\right)\ge 0$, ${\sum }_i{P}_0\left(i\right)=1$ and the same holds also for the stationary limit $P\left(i\right)$). The stationary vector P, in the following also called PageRank vector, satisfies the eigenvalue equation $GP=\lambda P=P$ with the maximal eigenvalue $\lambda =1$ of G. One can show from (1) that all other (real or complex) eigenvalues $\lambda$ of G satisfy the inequality $\left|\lambda \right|\le \alpha$, providing a spectral gap $1-\alpha$ ensuring the exponential convergence to the steady state vector and the iteration $G^t{P}_0$ indeed provides a reliable numerical method to compute the PageRank.

The value of the PageRank component $P\left(i\right)$ represents the importance of the node i, which is essentially proportional to the number of all incoming links $j\to i$ [18] but also weighted with the importance $P\left(j\right)$ of the source nodes j. It is also useful to determine the rank index $K_M\left(i\right)=1,2,3,\dots$ of a node i by ordering the nodes i with decreasing values of $P\left(i\right)$. This index is also called PageRank index such that $K_M\left(i\right)=1$ for maximal $P\left(i\right)$, $K_M\left(i\right)=N$ for minimal $P\left(i\right)$ and more generally $K_M\left(i\right)<K_M\left(j\right)$, corresponding to $P\left(i\right)>P\left(j\right)$. The Google search engine actually uses this PageRank index to select the presentation order of search results, which typically results in rather long lists of web pages.

Following [19,20], we can also consider the network obtained by the inversion of all the directions of links of the original network with a resulting Google matrix noted $G^{*}$ (which is different from the simple transpose $G^T$ due to different column-sum normalizations and different sets of dangling nodes). The PageRank vector associated to $G^{*}$ is called CheiRank vector and noted $P^{*}$ with a CheiRank ordering index $K_M^{*}\left(i\right)$. The value of $P^{*}\left(i\right)$ is typically proportional to the number of outgoing links $i\to j$ with some weight factors being $P^{*}\left(j\right)$.

It is also useful to introduce a 2DRank index $K_2$, which orders nodes on the PageRank–CheiRank plane by order of their appearance in a square of increasing size $K_M=K_M^{*}$ starting from $K_M=K_M^{*}=1$ (see details in [20]). The coarse-grained density of the distribution of nodes in the $K_M$-$K_M^{*}$ plane, eventually in log-scale, provides also a useful graphical presentation of the network nodes; see also Figure 1 and Figure 2 below. In these Figures the numbers at the color bar correspond to $W(K_{\mathrm{M}},K_{\mathrm{M}}^{*})/W_{\mathrm{cut}}$ where $W_{\mathrm{cut}}=maxW(K_{\mathrm{M}},K_{\mathrm{M}}^{*})/16$ and values of $W(K_{\mathrm{M}},K_{\mathrm{M}}^{*})>W_{\mathrm{cut}}$ have been saturated to $W_{\mathrm{cut}}$. The non-linear color scale (corresponding to $x^8$ if $x\in [0,1]$ represents the linear scale of the visible color bar) and the saturation at $W_{\max }/16$ have been chosen in order to increase the visibility of low-density values.

In this work, we note by $K_M$ ($K_M^{*}$) the PageRank index for the full (inverted) network while K ($K^{*}$) denotes a reduced limited index for the group(s) of 40 nodes corresponding to Table 2 and Table 3, i.e., with values $K=1,\dots ,40$ ($K^{*}=1,\dots ,40$). These local group indices K and $K^{*}$ can be computed by different methods, e.g., by direct extraction from $K_M$ and $K_M^{*}$ by attributing the values of K ($K^{*}$) with increasing values of $K_M$ ($K_M^{*}$), e.g., $K=1$ for the subset node with minimal $K_M$ value, $K=2$ for the subset node with the second minimal $K_M$ value, etc. We used, however, a slightly different method that consists simply of reducing the PageRank vector to the subset, with new index values being $1,\dots ,40$, and then computing K by ordering the components of this reduced (or projected) PageRank vector (and similarly for $K^{*}$ using a reduced CheiRank vector). A third, more complicated method is to compute the projected PageRank from the reduced Google (see next section). Table 3 summarizes these local indices K and $K^{*}$ for the 8 editions and their associated subsets of 40 nodes.

Thus, the index $K_M$ is the order index of PageRank vector, which is ordered by monotonically decreasing probability $P\left(K_M\right)$, thus the maximal probability is at $K_M=1$, next is at $K_M=2$, etc. Recall that $1\le K_M\le N$ is the index of the whole matrix G of a Wikipedia network of size N. The same procedure is applied for index ${K^{*}}_M$ of CheiRank probability vector $P^{*}\left({K^{*}}_M\right)$ of the whole matrix $G^{*}$ of size N. The indices $K,K^{*}$ are constructed in the same way but for the PageRank and CheiRank vectors of a reduced Google matrix of size 40, thus $1\le K,K^{*}\le 40$.

3.3. Reduced Google Matrix

A pedestrian explanation of the REGOMAX algorithm without formulas can be found by an interested reader in Ref. [27]. Below we give the mathematical foundations of REGOMAX.

In [22], a method and algorithm (REGOMAX) was introduced to define and compute a reduced Google matrix for a selected subset of $N_{\mathrm{r}}$ nodes (with $N_r\sim {10}^2$–${10}^3$ being typically of modest size), taking into account both direct links between two nodes of this subset and also indirect links between two such nodes using pathways along nodes outside this subset. We note that the contributions of indirect links are very important and their omission may lead to erroneous results, as was demonstrated in [23] for a directed network of historical figures of Wikipedia previously studied in [30].

For this approach, it is convenient to write the Google matrix G and the PageRank vector P of the global network as

$$G=\left(\begin{array}{cc}{G}_{\mathrm{rr}}& G_{\mathrm{rs}}\\ G_{\mathrm{sr}}& G_{\mathrm{ss}}\end{array}\right),P=\left(\begin{array}{c}{P}_{\mathrm{r}}\\ P_{\mathrm{s}}\end{array}\right)$$

(2)

where the label “$\mathrm{r}$” refers to the nodes of the reduced network of $N_{\mathrm{r}}$ subset nodes and “$\mathrm{s}$” to the other $N_{\mathrm{s}}=N-N_{\mathrm{r}}$ nodes, which form the complementary network acting as an effective “scattering network”. The PageRank eigenvalue equation $GP=P$ implies [22] that the projected PageRank $P_{\mathrm{r}}$ can be computed from

$$G_{\mathrm{R}}{P}_{\mathrm{r}}=P_{\mathrm{r}}$$

(3)

where the reduced Gooogle matrix of size $N_{\mathrm{r}}\times N_{\mathrm{r}}$ is given by

$$G_{\mathrm{R}}=G_{\mathrm{rr}}+G_{\mathrm{rs}}{(\mathbf{1}-G_{\mathrm{ss}})}^{-1}{G}_{\mathrm{sr}}=G_{\mathrm{rr}}+G_{\mathrm{pr}}+G_{\mathrm{qr}}.$$

(4)

This matrix is also a stochastic matrix with columns being sum normalized. For practical reasons, we renormalize $P_{\mathrm{r}}$ such that ${\sum }_j{P}_{\mathrm{r}}\left(j\right)=1$ where the sum runs over all nodes of the small subset. In (4), the first term $G_{\mathrm{rr}}$ accounts for all direct links between two nodes A and C in the subset and the second term represents all indirect links between these two nodes using a chain of links from A to $B_1$, then from $B_1$ to $B_2$, …, and then from $B_m$ to C where the intermediate nodes $B_1,\dots ,B_m$ belong to the complementary scattering network of $N_{\mathrm{s}}$ nodes. Such a pathway corresponds to the term $G_{\mathrm{rs}}{G}_{\mathrm{ss}}^m{G}_{\mathrm{sr}}$ that is obtained by expanding the above matrix inverse in a geometric series over such terms. We note that the matrix $G_{\mathrm{ss}}$ has a leading eigenvalue ${\lambda }_c$ close to 1 but smaller than 1 (if $N_{\mathrm{r}}>0$) such that the matrix inverse is well defined. The second term can be furthermore decomposed [22] in two contributions $G_{\mathrm{pr}}+G_{\mathrm{qr}}$, where $G_{\mathrm{pr}}$ is a rank-1 matrix obtained by extracting from the matrix inverse the contribution of the leading eigenvector of $G_{\mathrm{ss}}$ (which is rather close to $P_{\mathrm{s}}$), and $G_{\mathrm{qr}}$ is the remaining contribution, which can be numerically efficiently computed by a rapid convergent geometric series over a certain specific matrix obtained from $G_{\mathrm{ss}}$ by a projection on the space bi-orthogonal to the leading eigenvector of $G_{\mathrm{ss}}$ (see [22] for details). Initially, this decomposition was introduced to find an efficient numerical algorithm to obtain $G_{\mathrm{R}}$ but it turns out that it also useful in terms of interpretation. The term $G_{\mathrm{pr}}$, while having a dominant weight, has a very simple structure with nearly identical columns being close to $P_{\mathrm{r}}$ (all columns are proportional with factors close to 1). This term is essentially determined by the leading eigenvector of $G_{\mathrm{ss}}$ (see [22] for an explicit formula). More details about the REGOMAX algorithm are given in [22], with applications to specific selected sets of Wikipedia and other networks described in [24,25,26,27],

Due to the simple structure of $G_{\mathrm{pr}}$, it is the other matrix $G_{\mathrm{qr}}$, smaller in numerical weight, which represents the most nontrivial information related to indirect hidden transitions. We also define the matrix $G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, which is obtained from $G_{\mathrm{qr}}$ with its diagonal elements being replaced by zero. We note that each component can be characterized by its weight being $W_{\mathrm{R}}$, $W_{\mathrm{pr}}$, $W_{\mathrm{rr}}$, $W_{\mathrm{qr}}$ and ($W_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$) for $G_{\mathrm{R}}$, $G_{\mathrm{pr}}$, $G_{\mathrm{rr}}$, $G_{\mathrm{qr}}$ and ($G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$), respectively, and which is defined as the sum of all matrix elements divided by its size $N_{\mathrm{r}}$. (Since $G_{\mathrm{R}}$ is also column-sum normalized we always have $W_{\mathrm{R}}=1$.) Studies for examples of reduced Google matrices associated to various directed networks can be found in [22,24,25,26,27].

We note that the first equivalence relation in (4) is similar to the Schur complement in linear algebra (see e.g., [31]). Issai Schur introduced the Schur complement in 1917 (see history in [31]) and later it found a variety of applications [31,32]. However, the expansion in the form of three matrix components, given by the second equivalence relation in (4), with the physical sense of each component, was introduced only in [22].

In this work, we apply the reduced Google matrix approach to the group(s) of 40 Wikipedia articles shown in Table 2 and Table 3 and introduced in the last section. For all these cases, we computed the reduced Google matrix and its different components, as well as the reduced PageRank vector $P_{\mathrm{r}}$ and the group local PageRank index K (with values $K=1,\dots ,40$). The same can also be performed for the inverted network with Google matrix $G^{*}$. However, in this work we present only results for the reduced Google matrix associated to G and not to $G^{*}$ (except for the local index $K^{*}$, which can also be obtained more directly by extraction from $K_M^{*}$ or by ordering $P_{\mathrm{r}}^{*}$, which is defined in a similar way as $P_{\mathrm{r}}$).

We present the positions of 40 articles in the PageRank–CheiRank plane for all 8 editions in Figure 1 and Figure 2; the reduced Google matrix and its components in Figure 3, Figure 4, Figure 5, Figure 6 and Figure 7 and related network diagrams in Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14 and Figure 15 for all 8 editions.

Finally, we note that the algorithms discussed above in Section 3.2 and Section 3.3 are not new; they are presented to clarify the notations and to introduce the reader to the framework of methods used in these studies. Thus, the REGOMAX algorithm with three matrix components has been introduced in [22] and applied to various directed networks in [24,25,26,27]. In this work, we simply use these algorithms to investigate the relations of social concepts and religions in Wikipedia networks.

3.4. Inter-Edition Correlator Quantities

It is interesting to compare the different quantities we compute, such as $P_{\mathrm{r}}$, K, $G_{\mathrm{R}}$, etc., between the different Wikipedia editions of Table 1. For this, we consider different types of correlators for which we give a brief review below.

For example, for two given data sets $X_i$ and $Y_i$ of size $n_s$ with $i=1,\dots ,n_s$, the Pearson correlation coefficient is defined as [33]

$${\rho }_{XY}={\displaystyle \frac{\langle (X-\langle X\rangle )(Y-\langle Y\rangle )\rangle }{{\sigma }_X{\sigma }_Y}}={\displaystyle \frac{\langle XY\rangle -\langle X\rangle \langle Y\rangle }{{\sigma }_X{\sigma }_Y}}$$

(5)

where $\langle f(X,Y)\rangle ={\sum }_{i}f(X_i,Y_i)/n_s$ is the average over an arbitrary function $f(X,Y)$ and ${\sigma }_X=\sqrt{\langle X^2\rangle -{\langle X\rangle }^2}$, ${\sigma }_Y=\sqrt{\langle Y^2\rangle -{\langle Y\rangle }^2}$ is the standard deviation of X and Y, respectively. For ${\rho }_{XY}>0$ (${\rho }_{XY}<0$), one can state (for a given index i) that it is more likely that $Y_i>\langle Y\rangle$ ($Y_i<\langle Y\rangle$) if $X_i>\langle X\rangle$. In other words, deviations from the average values for X and Y have probably the same (opposite) sign if ${\rho }_{XY}>0$ (${\rho }_{XY}<0$).

The Kendall rank correlation coefficient is defined as [34]

$${\tau }_{XY}={\displaystyle \frac{2n_c}{n_p}}-1$$

(6)

where $n_p=n_s(n_s-1)/2$ is the number of all possible pairs $(X_i,Y_i)$, $(X_j,Y_j)$ with $i<j$ and $n_c$ is the number of concordant pairs such that either $X_i<X_j$ and $Y_i<Y_j$ or $X_i>X_j$ and $Y_i>Y_j$ (same sorting order between the pair). Here, we have ${\tau }_{XY}=1$ (${\tau }_{XY}=-1$) if both data sets have the same (reverse) sorting order.

In the (end of the) next section and in Figure 16, we present and discuss results for both correlators ${\rho }_{q\left(i\right)q\left(j\right)}$ and ${\tau }_{q\left(i\right)q\left(j\right)}$ as $8\times 8$ matrices where $i,j=1,\dots ,8$ represent index values for the 8 Wikipedia editions and $q\left(i\right)$ a data set/quantity computed for the given Wikipedia edition with index value i. Concerning the correlator (5), we consider five quantities $q\left(i\right)$ being the reduced PageRank vector $P_{\mathrm{r}}$ (all vector coefficients with $n_s=40$), the reduced Google matrix $G_{\mathrm{R}}$ (all matrix elements with $n_s={40}^2$), the religion or society sub-block of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ (all sub-block matrix elements with $n_s={17}^2$ or $n_s={23}^2$, respectively) and also the local PageRank index K (all K values with $n_s=40$). For K, we also compute the Kendall rank correlator (6) which is actually equivalent to the Kendall rank correlator for $P_{\mathrm{r}}$ since the local ranking index K is obtained by ordering the values of $P_{\mathrm{r}}$, i.e., two K vectors for two different editions have the exact same number of concordant pairs as the related two $P_{\mathrm{r}}$ vectors. We mention that mathematically both correlator quantities can take in theory values between $-1$ (perfect anti-correlation) and $+1$ (perfect correlation) while values close to 0 indicate small or absent correlations. However, for the 6 correlator quantities mentioned above only positive correlator values appear (actually with minimal values above $0.33$; see Figure 16 for more details).

We note that the algorithms described in Section 3.1, Section 3.2 and Section 3.3 use only links between Wikipedia articles. This approach was shown to provide important and useful information about nodes of various directed networks being also at the foundations of the Google search engine [17,18]. The validity of these algorithms is confirmed by various network studies reported in [21,22,23,24,25,26,27].

4. Results

4.1. Article Location on PageRank–CheiRank Plane

We first discuss some results for the local ranking indices K and $K^{*}$ given in Table 3 for the eight edition cases and the 40 edition-specific selected articles. Concerning EN-Wikipedia, we note that the top six PageRank positions in the subset of Table 2 are taken by religions, with Catholic Church at $K=1$, while the first society concept Law only appears at $K=7$. This tendency is preserved in the other seven editions where religions still take the top 3–4 PageRank positions while society concepts such as Liberalism only appear at $K=5$ for the AR, ES, FR and IT editions. There are two exceptions, one being Nazism at $K=2$ for DE, which is clearly linked to German history, and surprisingly also for ZH, where the top society concept is Law at $K=3$ being significantly above Communism at $K=13$. For EN, the top society concept is also Law at $K=7$, and for RU it is Economy at $K=7$.

Among religions, the top $K=1$ position is taken by Catholic Church for EN, DE, ES and IT and Christianity is at $K=1$ for FR and ZH (Buddhism is at $K=2$ for ZH). Somewhat surprisingly, for RU Islam is at the top PageRank position $K=1$ and Christianity is at $K=2$. This is probably related to a significant Muslim population in Russia that is, however, significantly smaller than its Christian population. Islam is the top religion for the AR-Wikipedia edition, which appears to be rather natural. However, here Christianity and Catholic Church appear at $K=2$ and $K=3$, respectively.

Concerning the CheiRank index $K^{*}$, which characterizes the communicative properties of a node, the situation is more mixed. Thus, we have at $K^{*}=1$: Jainism for EN; Christianity for AR; Islam for DE, Anarchism for ES and FR; Catholic Church for IT; Monarchy for RU and Communism for ZH. We attribute such a mixing to stronger fluctuations of outgoing links as discussed in [21].

Concerning the global ranking indices $K_M$ and $K_M^{*}$ for the full network, we show the density of their distribution in the $ln\left(K_{\mathrm{M}}\right)$-$ln\left(K_{\mathrm{M}}^{*}\right)$ plane in Figure 1 for EN, AR, DE and ES and Figure 2 for FR, IT, RU and ZH. The overall density structure is rather comparable for all eight editions with a certain asymmetry between both axis. However, the precise top $K_M$ and $K_M^{*}$ positions (isolated small red or green squares) are very specific for each edition.

Furthermore, the locations of the 40 edition-specific selected articles of Table 2 and Table 3 are also shown in Figure 1 and Figure 2. We see that, for all editions, religion nodes (white crosses corresponding to $24\le K_g\le 40$) have typically higher PageRank positions (lower $K_M$ values) as compared to social concepts (red crosses corresponding to $1\le K_g\le 23$), i.e., typically the top religion nodes appear to be more important than the top society nodes. In particular, for the EN-edition the top religion PageRank index is about 6 times smaller as compared to the top social concept PageRank index, as can be seen from Table 2. For the other editions, we have similar ratios of $K_M$ between the top religion and the top society nodes, which can be seen from the difference of the horizontal positions between the first white cross and the first red cross (difference of $ln{K}_M$) in the different panels of Figure 1 and Figure 2.

4.2. Matrix Components of REGOMAX Algorithm

We have computed the different reduced Google matrix components (as described in Section 3.3) for the eight Wikipedia editions and using for each edition the edition-specific group of 40 articles given in Table 2 and Table 3 (as explained in Section 2.2). The presentation order of the nodes in the groups is given by the index $K_g$, which was obtained by separately PageRank ordering the 23 nodes for society concepts and the 17 nodes for religions (or branches of religions) for the EN-Wikipedia edition. However, for the other editions there are different K and $K^{*}$ indices but for practical reasons we keep the same initial node order $K_g$ (obtained from EN-Wikipedia) when presenting the different matrix components in the figures below.

Recall that $G_{\mathrm{R}}$ is, according to (4), given by the sum $G_{\mathrm{rr}}+G_{\mathrm{pr}}+G_{\mathrm{qr}}$ where $G_{\mathrm{rr}}$ represents the direct links, $G_{\mathrm{pr}}+G_{\mathrm{qr}}$ indirect links with $G_{\mathrm{pr}}$ being a rank-1 matrix (with columns being rather close to the projected PageRank $P_{\mathrm{r}}$) taking into account the contributions of the leading eigenvector in the complementary scattering space (see [22] for details) and $G_{\mathrm{qr}}$ is obtained from the other contributions and contains interesting nontrivial information about indirect links. Numerically, $G_{\mathrm{pr}}$ is quite dominant in this sum with relative weights $W_{\mathrm{pr}}=0.91$–$0.97$ depending on the edition but it has a very simple structure. The interesting properties of the reduced Google matrix are contained in the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ being the sum of $G_{\mathrm{rr}}$ and $G_{\mathrm{qr}}$ (with diagonal elements of the latter replaced by 0). For two editions (EN and ZH), we present results for the four matrices $G_{\mathrm{R}}$, $G_{\mathrm{pr}}$, $G_{\mathrm{rr}}$ and $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ while for the other six editions we limit ourselves to $G_{\mathrm{R}}$ and $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$.

Below we will focus our discussion on the most interesting case $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$. To simplify this discussion, we also introduce for this matrix the sub-blocks A (left top diagonal society block), B (right top block with links from religion to society), C (left bottom block with links from society to religion) and D (bottom right diagonal religion block). In particular, we determine the strongest matrix element for each block, the sum of matrix elements per block and the ratios $R(C,A)$, $R(D,B)$, $R(D,A)$ for these sums. In the next Subsection, we will see that the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ can also be exploited to generate effective networks of friends and followers.

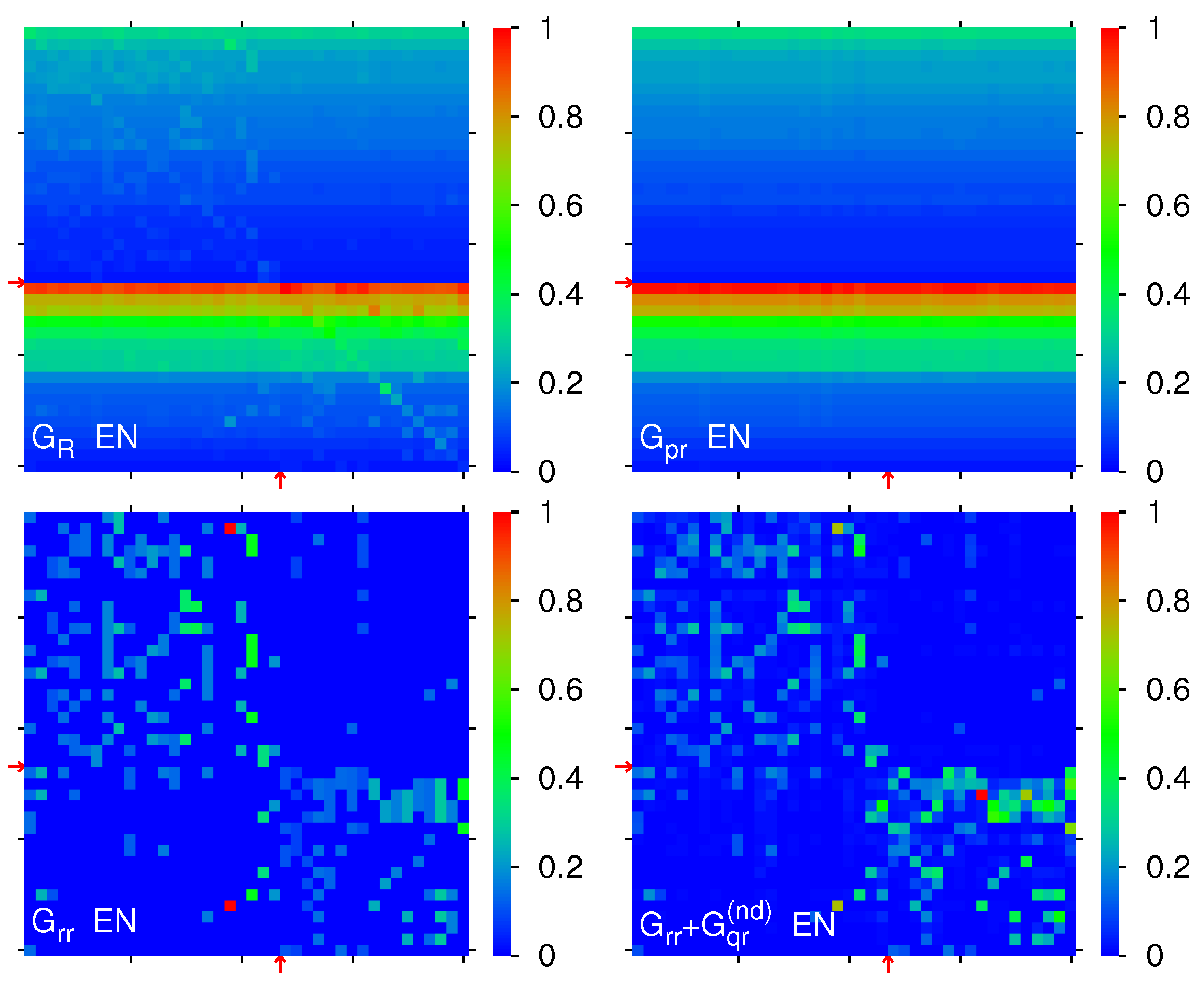
In Figure 3, we show color density plots for $G_{\mathrm{R}}$, $G_{\mathrm{pr}}$, $G_{\mathrm{rr}}$ and $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ for the edition EN with numerical weights being $W_{\mathrm{R}}=1$, $W_{\mathrm{rr}}=0.0144$, $W_{\mathrm{pr}}=0.9661$ and $W_{\mathrm{qr}}=0.0194$ ($W_{\mathrm{rr}}+W_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}=0.0280$). The color plot of $G_{\mathrm{pr}}$ is essentially composed of rows of equal color with matrix elements $G_{\mathrm{pr}}(i,j)\approx P_{\mathrm{r}}\left(i\right)$ for all columns j. Here, the sequence of row colors illustrates the separate PageRange order in the two blocks. The row with red color corresponds to the top PageRank node Catholic Church with $K=1$, $K_g=24$ and the first row in the religion block, and the rows below at $K_g=25,\dots ,29$ with orange or (strong) green color correspond to $K=2,\dots ,6$. The color plot of $G_{\mathrm{R}}$ is similar in appearance, due to the strong weight of $G_{\mathrm{pr}}$, but with additional peaks at isolated positions with largest elements of $G_{\mathrm{rr}}$ or $G_{\mathrm{qr}}$ (including some quite strong diagonal elements of $G_{\mathrm{qr}}$, especially in the religion block).

For $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, the strongest matrix elements $G_{\mathrm{rr}}(i,j)+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}(i,j)$ for each block A, B, C and D (for links $i\leftarrow j$) correspond to 0.0126 (Education ← Oligarchy, A); 0.0021 (Economy ← Chinese folk religion, B); 0.0125 (Shia Islam ← Oligarchy, C) and 0.0172 (Islam ← Sunni Islam, D). The last value appears as a sum of a direct link and also a stronger indirect link from Sunni Islam to Islam while the two links from Oligarchy to Education or Shia Islam result from direct links also clearly visible in $G_{\mathrm{rr}}$.

We also observe that the two diagonal blocks A and D seem rather decoupled with significantly smaller links in the off-diagonal blocks B and C, which is also confirmed by the sum ratios $R(C,A)=0.2778$, $R(B,D)=0.0996$ and $R(D,A)=0.8998$. The value of $R(D,A)$ is higher due to the ratio of areas ${(17/23)}^2\approx 0.55$, showing that the transitions inside the religion block D are more intense comparing to the society concepts block A; this difference is also related to the particularly strong maximal element in the D block (see above). The decoupling between the two blocks, which is also confirmed for the other seven Wikipedia editions discussed below, seems to be surprising in view of the important historical role played by religions in society formation. However, on the other side there is a well-known statement from the Bible, “Render unto Caesar the things that are Caesar’s, and unto God the things that are God’s” (Bible Matthew 22:21 [35]) that may be partially at the origin of this result. Also, in certain countries, e.g., France, the separation between the state and religions is fixed by law.

Figure 4 shows the matrices $G_{\mathrm{R}}$ and $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ for the two editions $AR$ and $DE$. For AR we have the weights $W_{\mathrm{pr}}=0.9153,W_{\mathrm{rr}}=0.0452$ and $W_{\mathrm{qr}}=0.03936$ with higher weights of $G_{\mathrm{rr}}$ and $G_{\mathrm{qr}}$ as compared to EN. For AR, the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.1067 (Monarchy ← Autocracy, A); 0.0063 (Law ← Catholic Church, B); 0.0132 (Islam ← Law, C) and 0.0524 (Taoism ← Confucianism, D). The first element of this list is related to the fact that several Islamic countries are monarchies. The sum ratios are $R(C,A)=0.1311$, $R(B,D)=0.1053$ and $R(D,A)=0.7031$. Here, in the panel of $G_{\mathrm{R}}$ one sees a strong red row at $K_g=26$ for the top PageRank node Islam.

In a similar way, we obtain for DE $W_{\mathrm{pr}}=0.9582,W_{\mathrm{rr}}=0.04200$ and $W_{\mathrm{qr}}=0.0217$ and here the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.0327 (Democracy ← Oligarchy, A); 0.0137 (Communism ← Chinese folk religion, B); 0.0058 (Islam ← Republic, C) and 0.0209 (Hinduism ← Jainism, D). The sum ratios are $R(C,A)=0.1364$, $R(B,D)=0.1847$ and $R(D,A)=0.8417$. As for EN, there is a red row for $G_{\mathrm{R}}$ at $K_g=24$ for Catholic Church.

For the ES edition (top panels of Figure 5), we find $W_{\mathrm{pr}}=0.9372$, $W_{\mathrm{rr}}=0.0269$ and $W_{\mathrm{qr}}=0.0358$ and the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.0222 (Oligarchy ← Autocracy, A); 0.0117 (Society ← Confucianism, B); 0.0082 (Buddhism ← Materialism, C) and 0.0346 (Islam ← Sunni Islam, D). Here, we have in the D block a second very strong matrix element with value 0.0296 (Islam ← Shia Islam). The sum ratios are given by $R(C,A)=0.2170$, $R(B,D)=0.1403$ and $R(D,A)=1.0227$. As for EN and DE, there is a red row for $G_{\mathrm{R}}$ at $K_g=24$ for Catholic Church.

For FR (bottom panels of Figure 5), we have $W_{\mathrm{pr}}=0.9572$, $W_{\mathrm{rr}}=0.0197$ and $W_{\mathrm{qr}}=0.0229$ and the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to: 0.0222 (Culture ← Society, A); 0.0101 (Politics ← Confucianism, B); 0.0178 (Christianity ← Autocracy, C) and 0.0198 (Buddhism ← Chinese folk religion, D). There are additional strong matrix elements 0.0191 (Capitalism ← Economy, A), 0.0179 (Education ← Economy, A), 0.0176 (Communism ← Economy, A), 0.0189 (Taoism ← Chinese folk religion, B) and 0.0170 (Monarchy ← Autocracy, A). We attribute the last link and the top C link from Autocracy to Christianity to the baptême de Clovis when the king of France Clovis I accepted the Christian religion around the year 500. The sum ratios are $R(C,A)=0.1894$, $R(B,D)=0.1395$ and $R(D,A)=1.1519$. There is a red row for $G_{\mathrm{R}}$ at $K_g=25$, $K=1$ for Christianity along with strong orange rows at $K_g=26$, $K=2$ (Islam) and $K_g=24$, $K=3$ (Catholic Church).

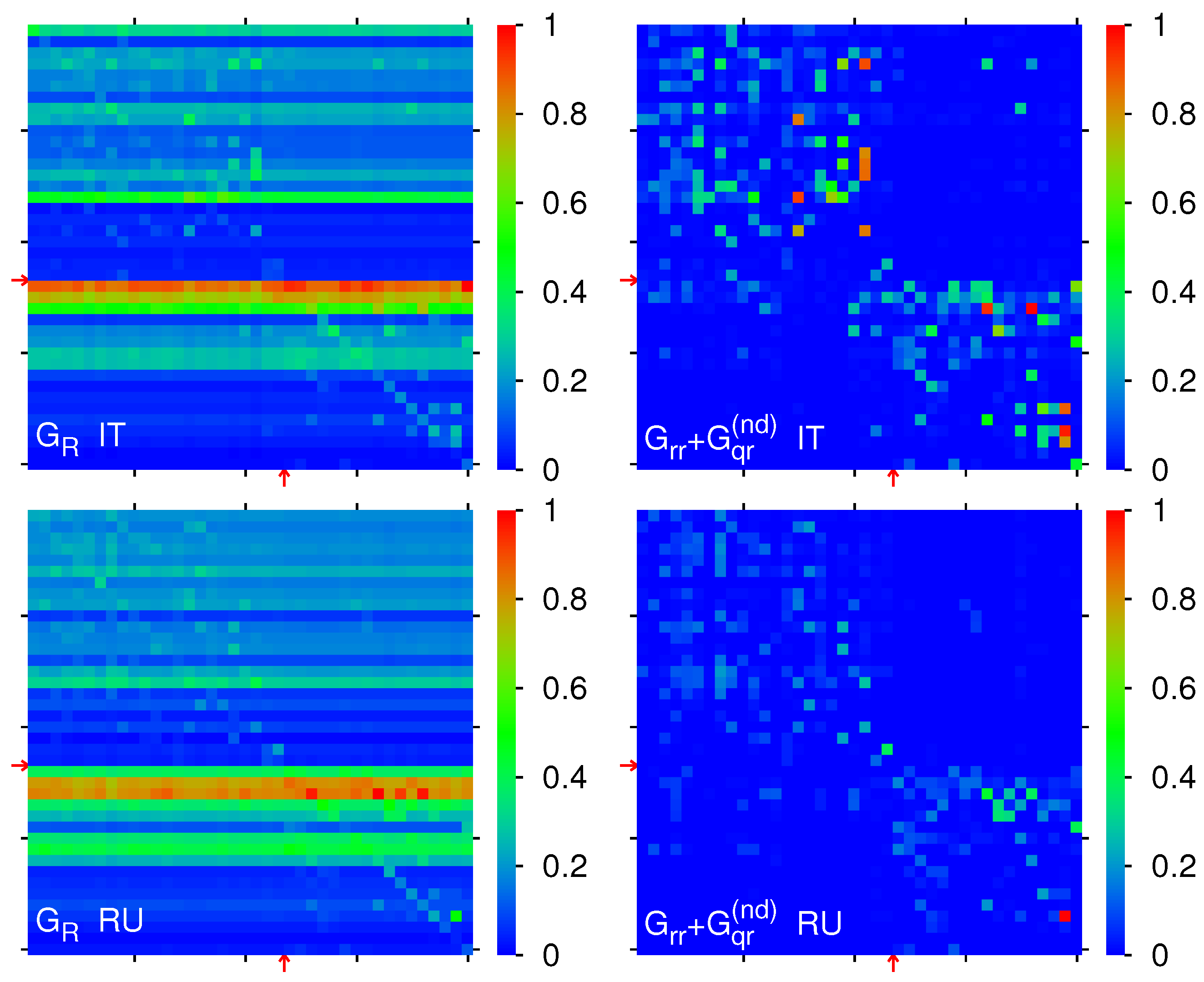
For the IT edition (top panels of Figure 6), the matrix weights are $W_{\mathrm{pr}}=0.9393$, $W_{\mathrm{rr}}=0.0297$ and $W_{\mathrm{qr}}=0.0308$ and the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.0298 (Economy ← Society, A); 0.0108 (Democracy ← Sunni Islam, B); 0.0095 (Christianity ← Idealism, C) and 0.0326 (Islam ← Shia Islam, D). Furthermore, there are significant numbers of additional strong matrix elements in the D-block: 0.0313 (Taoism ← Chinese folk religion); 0.0307 (Islam ← Sunni Islam); 0.0285 (Confucianism ← Chinese folk religion) and 0.0260 (Shinto ← Chinese folk religion). Also, there are more in the A-block: 0.0292 (Democracy ← Autocracy); 0.0280 (Fascism ← Autocracy); 0.0278 (Monarchy ← Autocracy); 0.0273 (Oligarchy ← Autocracy); 0.0272 (Culture ← Society) and 0.0266 (Republic ← Autocracy). The latter are probably related to Italian history. The sum ratios are given by $R(C,A)=0.1372$, $R(B,D)=0.1159$ and $R(D,A)=0.6477$ and there are two strong rows for $G_{\mathrm{R}}$ being red ($K_g=24$, $K=1$, Catholic Church) and orange ($K_g=25$, $K=2$, Christianity).

For RU (bottom panels of Figure 6), we have $W_{\mathrm{pr}}=0.9515$, $W_{\mathrm{rr}}=0.0217$ and $W_{\mathrm{qr}}=0.0267$ and the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.0223 (Materialism ← Idealism, A); 0.0043 (Capitalism ← Protestantism, B); 0.0066 (Buddhism ← Civilization, C) and 0.0569 (Taoism ← Chinese folk religion, D). Here, we have the sum ratios $R(C,A)=0.1519$, $R(B,D)=0.0928$ and $R(D,A)=0.7268$ and in the $G_{\mathrm{R}}$ panel we see two orange-red rows at $K_g=26$, $K=1$ (Islam) and, with slightly smaller values, at $K_g=25$, $K=2$ (Christianity).

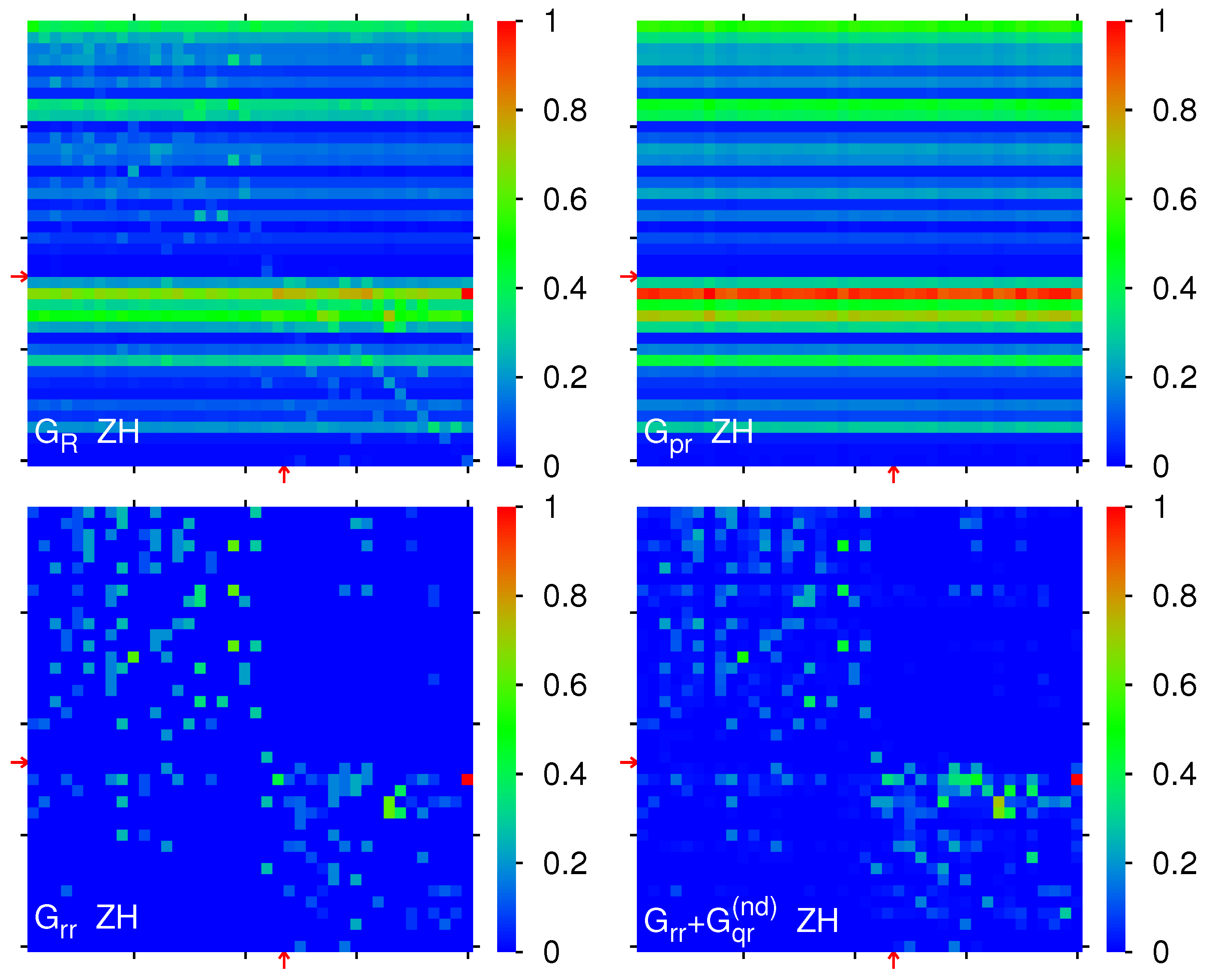
Finally, in Figure 7 we show the matrices for $G_{\mathrm{R}}$, $G_{\mathrm{pr}}$, $G_{\mathrm{rr}}$ and $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ of the ZH edition. Here, the weights are $W_{\mathrm{pr}}=0.9233,W_{\mathrm{rr}}=0.0333$ and $W_{\mathrm{qr}}=0.0432$ ($W_{\mathrm{rr}}+W_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}=0.0658$). For ZH, the strongest matrix elements of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ per block correspond to 0.0341 (Fascism ← Nazism, A); 0.0097 (Education ← Judaism, B); 0.0219 (Christianity ← Idealism, C) and 0.0653 (Christianity ← Oriental Orthodox Churches, D) and the block-sum ratios are $R(C,A)=0.2639$, $R(B,D)=0.1319$ and $R(D,A)=0.8458$. In the panel of $G_{\mathrm{pr}}$, we see a strong red row at $K_g=25$, $K=1$ (Christianity), which appears less pronounced (between orange and strong green) in the other panel of $G_{\mathrm{R}}$. This is mainly because the very strong maximal direct matrix element in the D-block of $G_{\mathrm{rr}}$ (and of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ corresponding to the link Christianity ← Oriental Orthodox Churches) shifts the maximum value in the color plot, defining the red color, which reduces the color scale of other matrix elements. We note that the structure of the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ follows for ZH mainly $G_{\mathrm{rr}}$ for the strongest transitions.

The results of this Subsection show that for the matrices $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ of all eight editions there are indeed multiple significant transitions inside both blocks of society concepts and of religions. However, the transitions between these two blocks are, roughly by a factor 5–10, smaller if we compare the sums of matrix elements of the off-diagonal block to the sums over the diagonal blocks. The ratio of transition strengths of the two diagonal blocks is typically $R(D,B)$ somewhat larger than the ratio ${(17/23)}^2\approx 0.55$ of block areas $D,B$. This shows that transitions between religions are on average a bit stronger than those between society concepts.

4.3. Network Structure Inside Social Concepts and Religions

In this section, we present effective network diagrams for friends and followers based on the information contained in the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, or more precisely in its two diagonal blocks A for society concepts and D for religions. Since, according to the results of the last Subsection, these two blocks are rather well decoupled with only weak links between them, we will present separate network diagrams for each block. Network diagrams of (nearly) the same style were, for example, used in [22] (for groups of political leaders in the Wikipedia network), [25] (for banks and countries in the Wikipedia network) and [27] (for a specific fibrosis-related protein group in the MetaCore network of proteins).

However, for convenience, we present here the construction method of these network diagrams. Assume we have a small matrix g with elements $g(i,j)$ being either a reduced Google matrix or one of its components (e.g., $G_{\mathrm{R}}$, $G_{\mathrm{rr}}$, $G_{\mathrm{qr}}$ or $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$) or a certain sub-block of such a matrix (e.g., society or religion sub-blocks A or D of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ shown in the previous section). First, we choose in the list of nodes (associated to this matrix or block) five top nodes representing five different subgroups based on some categorization criteria (depending on the set of nodes and the context) and we attribute each other node of this list to exactly one of the five subgroups (based on some criteria and the context). In the following, we will use for these subgroups the notation poles as a synonym for “center of interest”, which is a typical use of this expression in the French language. For each pole, we also define some presentation color.

To construct the effective friend network (see below for the other case of follower networks), we first draw a main circle (thin gray line) and place the five top nodes uniformly on this circle with some label and the corresponding color. Then, we select for each top node j (also called level-1 nodes) the four strongest friends i (level-2 nodes) with strongest outgoing links $j\to i$, i.e., with largest matrix elements $g(i,j)$ in the same column j of this matrix. Each of these strongest friends, if not yet present in the diagram as another level-1 node, is placed on a smaller secondary circle around the top level-1 node associated to it and we draw thick black arrows from the level-1 nodes to their friends. It is possible that a new level-2 node appears as a friend of several initial level-1 nodes. In this case, we try first to place this level-2 node on the circle of the level-1 node with same color (same pole) if possible, i.e., if this level-2 node is indeed a friend of the level-1 node of the same color. Only if this is not possible, we place it on the circle of another level-1 node (first level-1 node of different color that has the given level-2 node as friend). If a level-1 node has a friend that is already present in the diagram as another level-1 node, we simply draw a thick black arrow from the former to the latter and do not modify the position of the latter.

The procedure is repeated for all (newly added) level-2 nodes with smaller circles around them on which we place their (up to) four strongest friends (level-3 nodes if newly added) and with the same rule for preferential placement on a circle of a parent node of same color. Now, we draw thin red arrows from the level-2 nodes to their friends. In the case if such a friend is already present in the network (as level-1 or level-2 node), its position is not modified and we only draw the thin red arrow from his parent node to it. We also mention that only non-empty circles with at least one node on them are drawn; i.e., if a given node has no newly added friends (i.e., all its friends are already in the diagram), then we will not draw an empty circle around it.

At this stage, we typically stop the procedure for simplicity. Even if we continue this procedure with level-4, level-5 nodes, etc., the number of newly added nodes quickly decreases and when there are no newly added nodes the procedure converges to a stable final diagram. This can happen actually quite early so that there is typically no big difference in diagrams limited to level-3 nodes and those with higher-level nodes. In particular, for the diagrams given below, the number of newly added level-3 nodes is typically already quite small (much smaller than the theoretical limit $5\times 4\times 4=80$) also because the available set of nodes is limited from the very beginning, even significantly smaller than the theoretical level-3 limit. In some of the diagrams below, there are even no newly added level-3 nodes (if absence of smallest level-3 circles) and we have convergence to a stable diagram at level-2, i.e., all friends of level-2 nodes are already present in the diagram as former level-1 or level-2 nodes. In case of convergence, the last stage does not add new nodes but it still adds arrows from the most recently added nodes in the previous stage to their friends (which are already present in the network diagram).

The construction of follower network diagrams is essentially the same with two modifications: (i) At each level k, we select for each level-k node i (typically only $k=1,2$ in our case) the four strongest followers j (as possible level-$(k+1)$ node if not yet present in the diagram) with strongest incoming links $i\leftarrow j$ defined by the largest matrix elements $g(i,j)$ in the same row i. (ii) Arrows (thick black or thin red) are drawn with inverted directions from followers (level-$(k+1)$ nodes) to parents (level-k nodes), e.g., there are some arrows from a circle node to its center node while in the friend diagrams we have arrows from the circle center to the outside nodes on the circle (note in case of multiple parent nodes or pre-existing friends or followers, we have typically a significant number of other types of arrows between different circles).

In this work, we present figures for the network diagrams constructed from the two diagonal blocks A for society-related nodes and D for religions of the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ for the eight different Wikipedia editions. Since there are two friend and follower diagrams for each case, we have four network diagrams per edition presented in a figure with four panels. The subgroups or poles together with their respective five top nodes for both society and religion cases are given in Table 2 (eighth column) and this table also contains a two-letter code for each node (fifth column) used as a node label in the diagrams.

For society concepts, we choose the five top pole nodes Law, Society, Communism, Liberalism and Capitalism with respective node colors being olive, (dark) green, cyan, blue and indigo. We have tried to attribute the members of the poles based on the context and logical proximity to the top node, e.g., Education and Culture are attributed to the second pole of Society; Ecology and Politics belong to the first pole of Law and Socialism and Anarchism are attributed to the third pole for Communism. In certain cases, this attribution is a bit arbitrary and other choices would have been possible. We also tried to assure that each pole has a certain minimum number of members.

For the religion nodes, we choose the five top pole religions Christianity, Islam, Buddhism, Hinduism and Chinese folk religion (same colors as for the society top nodes in this order) and with its pole members being related to branches of religions or sub-religions. Here, we have attributed Judaism to the first Christianity pole with other members being Catholic Church, Protestantism and both nodes about the Orthodox Church.

In the following, we will more precisely call these poles also “initial poles” in order to distinguish them from “natural poles”, which may emerge naturally by certain clusters in a network diagram. Quite often, natural poles and initial poles are very similar but in certain cases natural poles are composed of nodes from several initial poles.

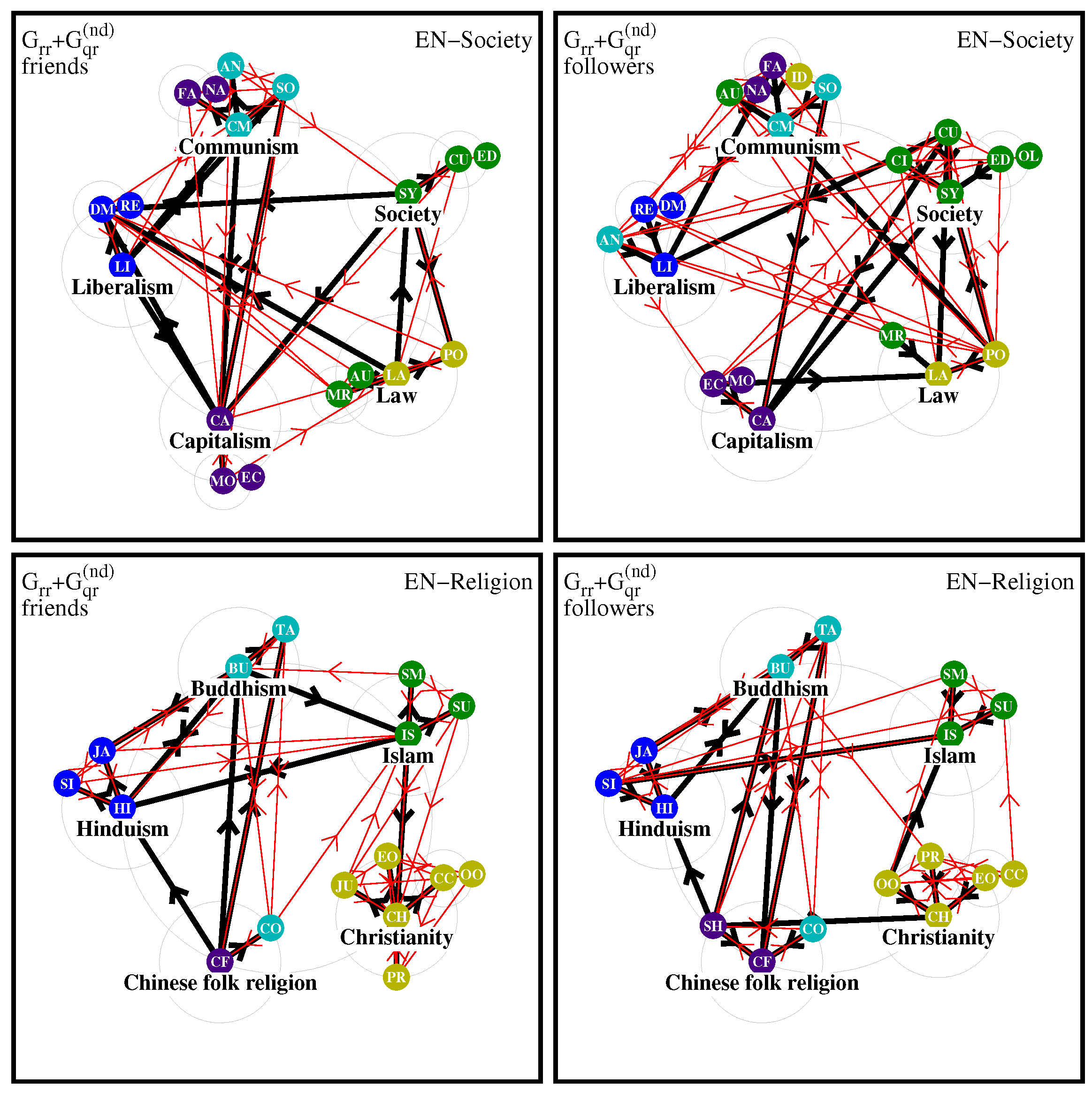
Specifically, for the EN edition, whose network diagrams are shown in Figure 8, we can identify in the friend society diagram the formation of five natural poles (which may slightly deviate from the initial poles) with main members being (1T) Law, Politics, Monarchy and Autocracy (two initial poles); (2T) Society, Culture and Education (one initial pole); (3T) Communism, Socialism, Anarchism, Nazism and Fascism (two initial poles); (4T) Liberalism, Democracy and Republic (one initial pole) and (5T) Capitalism, Money and Economy (one initial pole). Thus, the natural pole Communism has the largest number of diagram members even if it is composed of nodes belonging to two different initial poles.

The diagram of followers has the natural poles (1T) Law, Politics and Monarchy (two initial poles); (2T) Society, Culture, Education, Civilization and Oligarchy (one initial pole); (3T) Communism, Socialism, Fascism, Nazism, Autocracy and Idealism (five initial poles); (4T) Liberalism, Democracy, Republic and Anarchism (two initial poles) and (5T) Capitalism, Money and Economy (one initial pole). Thus, again the strongest natural pole is formed around Communism.

For the diagram of religion friends we have from Figure 8 the natural pole members (1T) Christianity, Catholic Church, Eastern Orthodox Church, Judaism, Protestantism and Oriental Orthodox Churches (one initial pole); (2T) Islam, Sunni Islam and Shia Islam (one initial pole); (3T) Buddhism and Taoism (one initial pole); (4T) Hinduism, Jainism and Sikhism (one initial pole) and (5T) Chinese folk religion and Confucianism (two initial poles). The strongest pole is Christianity; however, it is somehow isolated having strong links only from Islam while the poles of Islam, Buddhism, Hinduism and Chinese folk religion have more active interconnections.

For the diagram of religion followers, we find (1T) Christianity, Eastern Orthodox Church, Protestantism, Oriental Orthodox Churches and Catholic Church (one initial pole); (2T) Islam, Sunni Islam and Shia Islam (one initial pole); (3T) Buddhism and Taoism (one initial pole); (4T) Hinduism, Jainism and Sikhism (one initial pole) and (5T) Chinese folk religion, Confucianism and Shinto (two initial poles). Here, the strongest pole is again Christianity, and now it is less isolated with connections to Islam and Chinese folk religion. At the same time, we see here more intense links between religions from Asia (3T, 4T, 5T) forming a strongly interconnected religion group.

The diagram of friends for society concepts of AR, whose network diagrams are shown Figure 9, has a reduced number of nodes as compared to EN in Figure 8, but the poles are more interconnected by strong links. Interestingly, the Communism pole does not include Fascism and Nazism in contrast to the EN edition. For the diagram of society followers, the natural pole with largest number of nodes is Law with seven members and four initial poles. The Communism pole includes only Socialism and Anarchism in contrast to EN where the (natural) pole of Capitalism also includes Fascism and Nazism.

For the AR diagram of friends for religions in Figure 9, we see that the Christianity pole contains a larger number of members as in the EN case but there are more links between poles and the Christianity pole is not as isolated as it is for EN. However, for the diagram of followers, the Christianity pole remains more isolated compared to the EN-edition, and also there are no strong black links between Christianity and Islam.

For the DE edition, the diagrams are presented in Figure 10. For the diagram of friends for society concepts, the strongest natural pole is Liberalism with Democracy, Fascism, Nazism, Monarchy and Oligarchy (three initial poles), while for EN Fascism and Nazism are included in the Communism pole; also for DE the poles are more densely interconnected as compared to EN. For the diagram of followers, we again see a significant difference with EN, thus Fascism and Nazism are included in Liberalism pole while they are in the Communism pole for EN.

For the DE religion diagrams, we have denser interconnections between the five poles as compared to EN. In the case of followers, there are no strong links between Islam and Christianity but there are many (level-2) red links between their respective pole members.

For the ES edition, the network diagrams are given in Figure 11. Its society friend diagram is similar to EN but there are fewer nodes in the Liberalism pole (i.e., the direct friends of Liberalism are the other four level-1 top nodes), also Fascism and Nazism are absent. For the case of followers, there are fewer pole members for Society and Communism but more for Law and Liberalism; Nazism is attributed to the Liberalism pole, which is different from the EN case where Nazism is absent.

For the religion diagrams of ES in Figure 11, the case of friends is similar to those of the EN edition but there are fewer links between the Islam and Christianity poles.

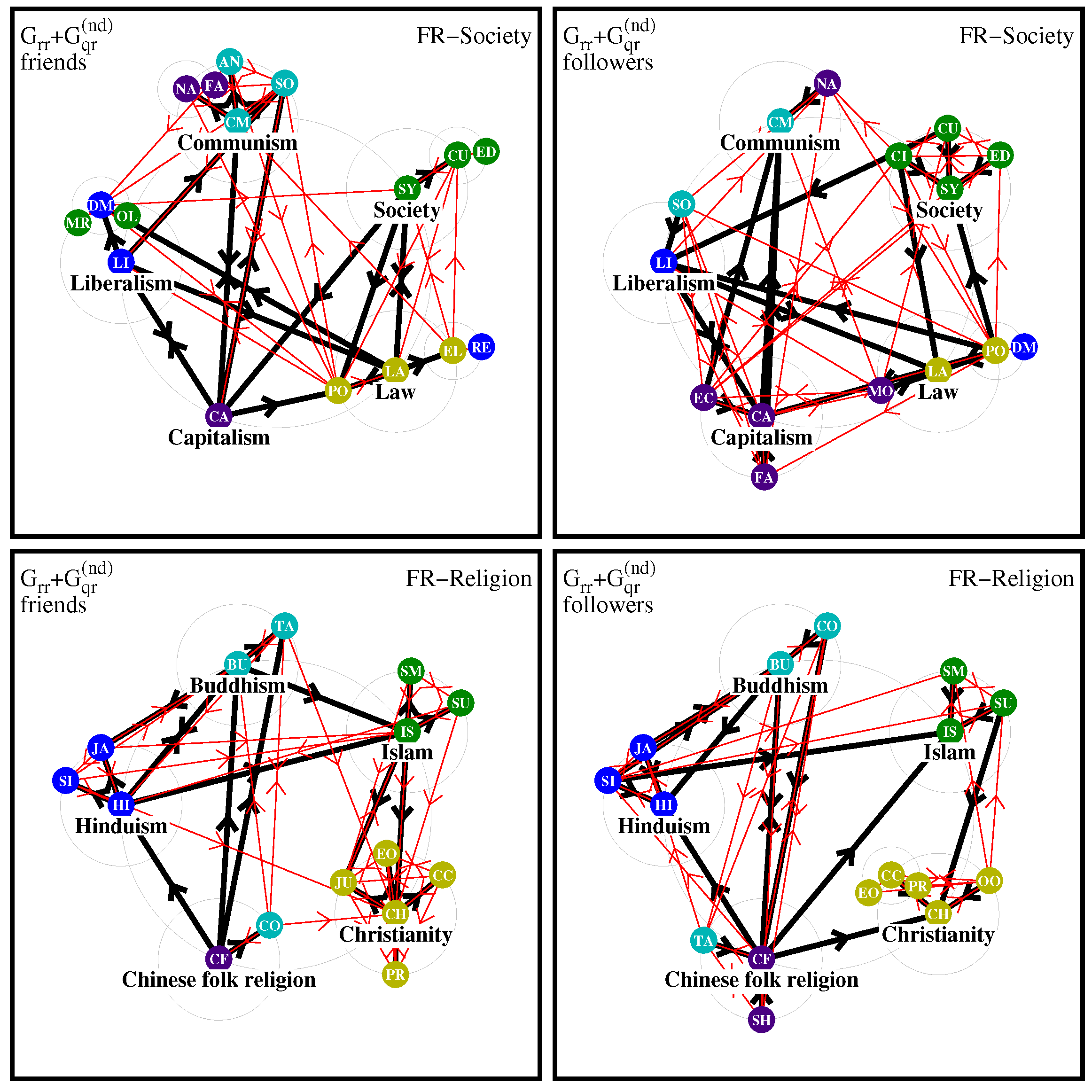
For the FR edition, the network diagrams are shown in Figure 12. Here, the society friend diagram is similar to the case of EN but with fewer links between the Society and Liberalism poles; as for EN, the nodes Nazism and Fascism belong to the Communism natural pole. For the case of followers, the Communism pole has only one node of Nazism (from another initial pole), while for EN this pole contains six members including Fascism and Nazism. The religion diagrams of FR are quite similar with those of EN.

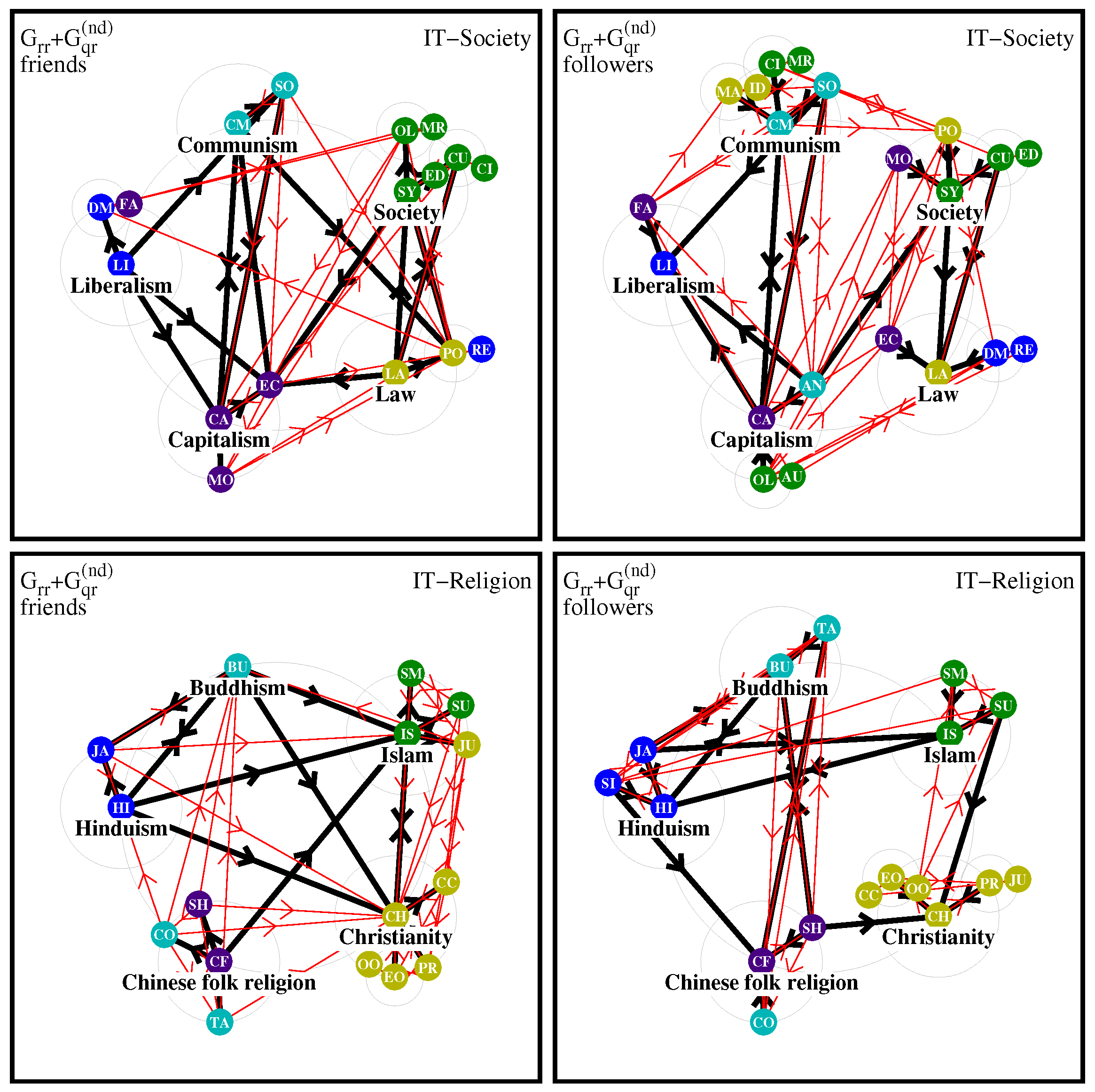
The network diagrams for the IT edition are presented in Figure 13. For the society friend diagram, the largest pole is Society including Culture, Education, Civilization, Oligarchy and Monarchy (all nodes from the same initial pole); the Liberalism pole includes Democracy and Fascism while Communism has only Socialism. This makes the last two poles rather different from the EN edition. The Capitalism pole is the same as for the EN case; the Law pole contains only Politics and Republic. For the society follower network of IT, the highest number of members is in the pole of Communism including Socialism, Materialism, Idealism, Civilization and Monarchy (three initial poles). In both society friend and follower diagrams, the node Fascism is attributed to Liberalism and while Nazism is absent, which constitutes a drastic difference with the EN case.

In the religion friend diagram of IT, the node Christianity has the highest number of nodes and it is strongly linked with Islam, Buddhism and Hinduism, in contrast to EN where this pole is more isolated. The diagram of followers is similar to those of the EN edition and Christianity remains the strongest pole.

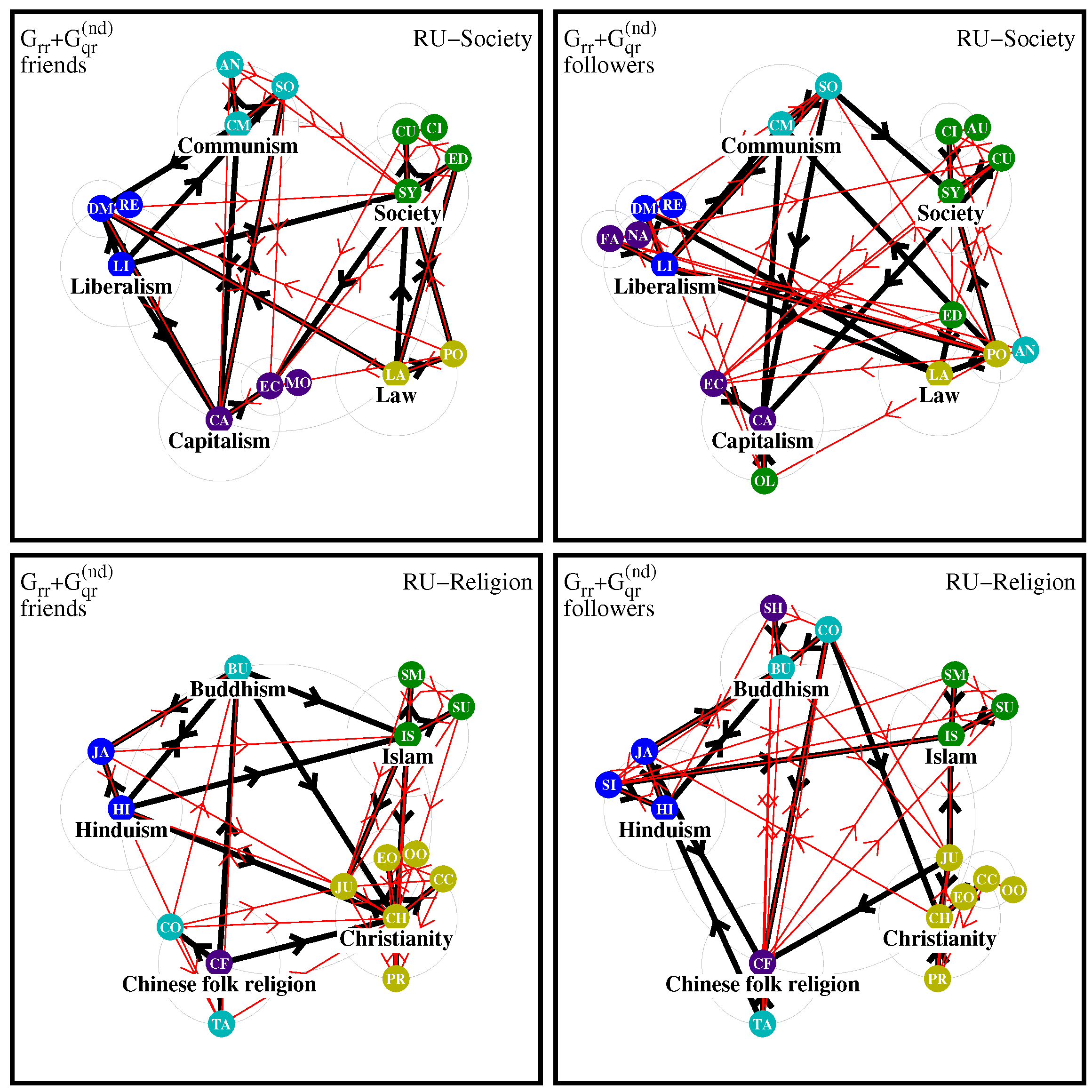
In Figure 14, we show the network diagrams for the RU edition. Here, the society friend diagram is similar to EN but without Fascism or Nazism in the diagram, also the poles Law and Society have a bit fewer included nodes. In the diagram of followers, Fascism and Nazism are included in the Liberalism pole, in contrast to the EN edition where these two nodes are included in the Communism pole.

For the religion friend diagram, Christianity has the largest number of nodes including Judaism linked also from Islam and this pole is less isolated than in the EN edition. For the diagram of religion followers, Christianity is still the largest pole with six nodes including Judaism pointing to Islam but in other aspects this diagram is similar to the EN edition.

Finally, for the edition for ZH the diagrams are presented in Figure 15. In the society friend diagram, the strongest pole is Law including Politics, Civilization, Autocracy, Monarchy, Economy and surprisingly Fascism. We note that for ZH the node Law has the unusual local Rank value $K=3$ for a society node, which are normally well behind the religion nodes in PageRank order. The Communism pole includes only Socialism being well linked to the Society pole, in contrast to the EN case; the Liberalism and Capitalism poles are similar to EN. In the society followers diagram, the strongest pole is again Law with six nodes and three initial poles; Fascism and Nazism are included in the Liberalism pole.

For the religion friend diagram of ZH, the strongest pole is Christianity with six nodes being well connected to other poles, in contrast to the EN case; at the same time the interlinks between Asian religion poles Buddhism, Hinduism and Chinese folk religion are denser as compared to EN. In the religion follower diagram, the strongest pole is also Christianity with six nodes, the diagram structure is similar to EN with a larger number of links between the Islam and Hinduism poles.

Among the main results of Section 4.2 and Section 4.3, we point out the following: (R1) The REGOMAX algorithm established matrix transitions between 40 articles of society concepts and religions for eight language editions of Wikipedia. (R2) We find that the weights of links between sector of social concepts and sector of religions are on average a factor of 5 weaker compared to those inside each of these two sectors; this indicates a relatively weak influence of one sector on another one. (R3) We established five main poles of influence for each sector and determined main links between them; these poles are Law, Society, Communism, Liberalism and Capitalism for the sector of society concepts and Christianity, Islam, Buddhism, Hinduism and Chinese folk religion for the sector of religions These networks are described in Section 4.3. (R4) We established that concepts of Nazism and Fascism were attributed to different poles by different editions. For example, they are attributed to Communism by the English edition, to Capitalism by Arabic edition and Liberalism by German edition. We discuss the proximity and differences of cultures in the next Subsection from a complementary view point.

4.4. Proximity and Differences of Cultures

Let us summarize the most important differences and similarities between the eight cultures represented by the eight Wikipedia language editions obtained in the last Subsection by analyzing the different network diagrams.

First, for the society diagrams the English and French cultures attribute the two nodes Fascism and Nazism to the Communism pole while they are attributed to the Capitalism pole by the Arabic culture and to the Liberalism pole by the German, Spanish (partially), Italian (partially), Russian (for followers) and Chinese (for followers) cultures.

Concerning the religion diagrams, the Christianity pole seems to be rather isolated in the English culture with other links only from the Islam pole (in the friend diagram). On the other hand, for the other cultures the Christianity pole is well connected not only with the Islam pole but also with the other poles of Hinduism, Buddhism and Chinese folk religion. For a majority of cultures, the three poles of the above Asian religions have a higher density of links between them as compared to the Islam and Christianity poles.

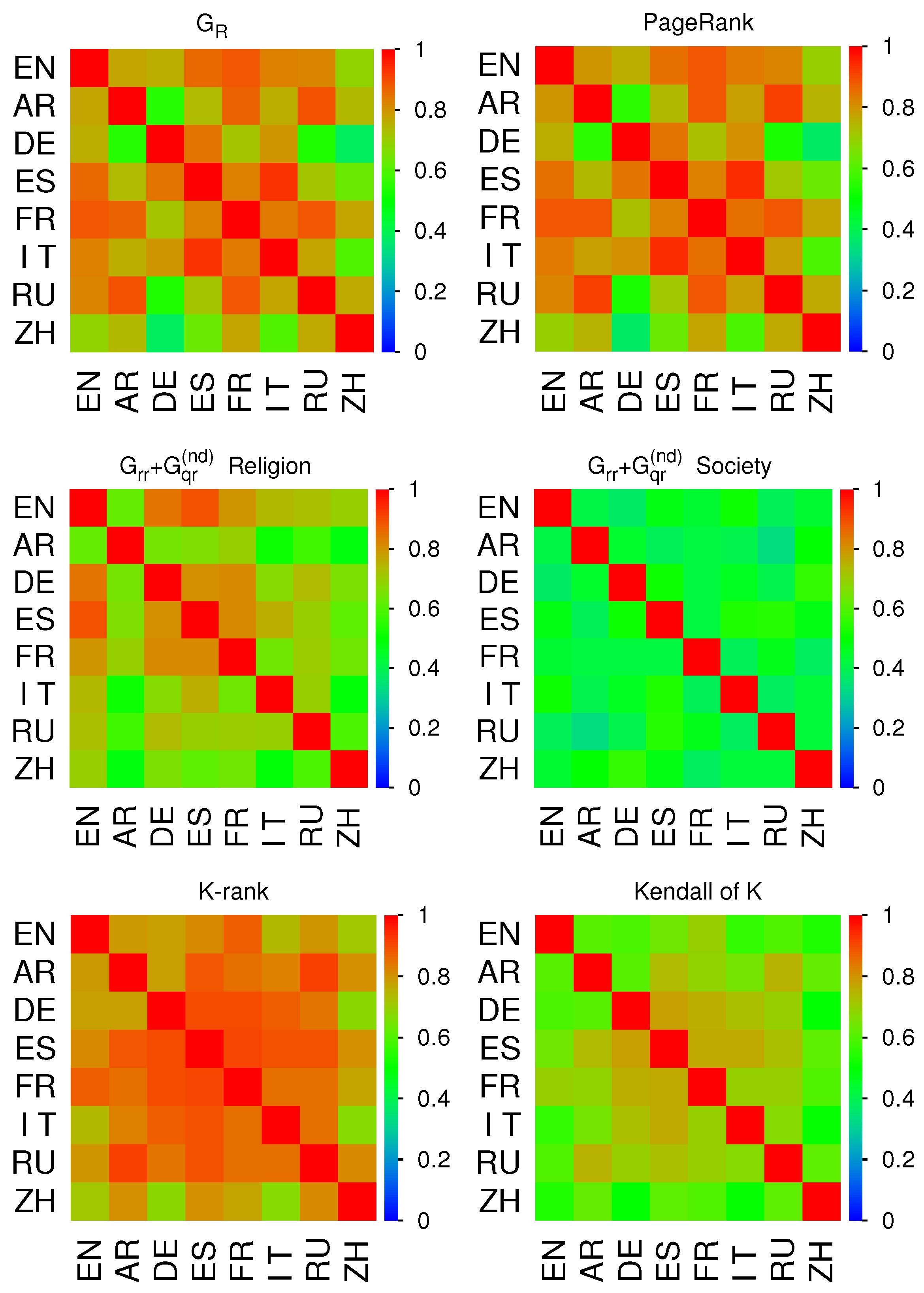
To determine quantitatively the proximity of the eight cultures, we compute the correlators for certain key quantities shown in Figure 16. The six panels of Figure 16 provide $8\times 8$-matrix density plots for different inter-edition correlators with five panels for the Pearson correlator of five quantities being the matrix $G_{\mathrm{R}}$, the (group local) PageRank vector $P_{\mathrm{r}}$, the religion and society sub-blocks for $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ and the local PageRank index K and one additional panel for the Kendall correlator of K. The precise definitions of these correlator quantities with some additional technical details are given in Section 3.4 and we note that for such correlator quantities the minimal mathematical possible value is $-1$, for the case of two data sets with strong anti-correlations, while values close to 0 indicate weak or absent correlations and values close to $+1$ correspond to strong correlations.

First, we observe that generally all eight Wikipedia editions seem to be rather well correlated with a big majority of correlator values being above 0.5 and only a few values close to 0.33. The two correlators associated to $G_{\mathrm{R}}$ and $P_{\mathrm{r}}$ (top row of Figure 16) are very close, which is plausible due to the typical strong numerical weight of $G_{\mathrm{pr}}$ in $G_{\mathrm{R}}$ and the fact that the columns of $G_{\mathrm{pr}}$ are close to $P_{\mathrm{r}}$. Here, the correlations of DE between AR, RU and ZH seem minimal (still with values ∼0.5) and also ZH seems to be less correlated to the other editions (with some fluctuations). The other inter-edition correlations are typically quite strong ∼0.8 with the largest values of $0.93$–$0.94$ for the correlation between ES and IT.

More specifically, for the $G_{\mathrm{R}}$-correlator and EN the closest other editions are FR (0.882) and ES (0.858); for AR the largest values are with RU (0.896) and FR (0.867), which appears to be plausible due to the, at least partial, importance of Islam in these three cultures. For DE, the two closest cultures are ES (0.845) and IT (0.803); for ES they are IT (0.933) and EN 0.858); for FR they are RU (0.885) and EN (0.882); for IT they are ES (0.933) and FR (0.842); for RU they are AR (0.896) and FR (0.885) and finally for ZH they are FR (0.774) and RU (0.767). For the very similar $P_{\mathrm{r}}$-correlator, this list of closest two editions is identical with only slightly different correlator values.

Concerning the religion block of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ (center left panel of Figure 16), we see that AR and ZH have globally the weakest correlations to the other cultures, with values ∼0.5, which seems natural due to the importance of their specific religions. On the other hand here, we have a block of four strongly correlated editions of EN, DE, ES and FR between them, with values ≥0.8, while IT and RU have typical “intermediate” correlations ∼0.7.

More explicitly, for this case the closest cultures to EN are ES (0.892) and DE (0.844); for AR they are FR (0.699) and ES (0.662) with relatively low values; for DE they are EN (0.844), FR (0.814) and ES (0.806); for ES they are EN (0.892), FR (0.814) and DE (0.806); for FR they are DE (0.814), ES (0.814) and EN (0.801); for IT they are ES (0.76) and EN (0.746); for RU they are DE (0.737) and EN (0.73) and finally the strongest correlator of ZH is to EN (0.694).

The society block of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ (center right panel of Figure 16) shows the “weakest” general correlations of all correlator quantities with (off-diagonal) values being typically ∼0.5 with the strongest off-diagonal element being 0.565 between DE and ZH, which is due to two strong matrix elements in $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$ due to the links from Oligarchy to Democracy and Monarchy for both editions. Here, the AR-RU correlator represents the minimal correlator value $0.332$ for all editions and all correlator quantities.

The Pearson and Kendall correlators of the PageRank index K (bottom row of Figure 16) appear to have roughly a similar relative structure as the Pearson correlators of $G_{\mathrm{R}}$ and $P_{\mathrm{r}}$ (top row of Figure 16). However, here the overall values are significantly stronger (lower) for the Pearson (Kendall) K-correlator in comparison to the top row values. For the Kendall correlator of K and the two editions EN and ZN, there is an additional suppression of the correlator values to other editions, which are mostly close to ∼0.5. Furthermore, for both K-correlators, we have a block of five editions DE, ES, FR, IT and RU of relatively strong correlations between them and AR has somewhat intermediate correlations to this block, while EN and ZH seem to be a bit more separated from this block (but still with significant correlator values).

5. Discussion and Conclusions

In this work, we presented the Google matrix analysis of Wikipedia networks constructed from eight language editions (EN, AR, DE, ES, FR, IT, RU, ZH) collected on 1 October 2024 and with key properties given in Table 1. Specifically, we analyzed the relations and interactions between 40 article entries about 23 society concepts and 17 religions or branches of religions (see Table 2 and Table 3). Using the PageRank and CheiRank vectors it is possible to establish a ranking of these 40 articles based on either their importance or their communicativeness, respectively. We found that globally in this group the articles related to religion are located at higher PageRank positions, implying higher importance, than the society-related articles including Law, Society, Liberalism, Capitalism, Communism, etc. Exceptions are the articles of Nazism with second PageRank position in the German edition and Law with third PageRank position in the Chinese edition.

Using the established REGOMAX algorithm [22], we computed for each edition the reduced Google matrix $G_{\mathrm{R}}$ and its components, which describe the direct and indirect transitions between all 40 entries (nodes), the latter taking into account all indirect pathways using nodes outside the group of 40 articles via the huge global matrix of the whole Wikipedia network. We found that the two diagonal blocks for society and religion nodes of the matrix $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, representing direct and “interesting” indirect links, nearly decouple with significantly smaller transitions between these two blocks.

Therefore, the interactions between society concepts and religions are relatively weak for all eight editions even if the historical role of religions on society development is well known. We conjecture that this is partially related to the well known Bible statement, “Render unto Caesar the things that are Caesar’s, and unto God the things that are God’s” (Bible Matthew 22:21 [35]) but there may also be other reasons.

We also extracted effective network friend and follower diagrams from the two diagonal blocks of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, providing a compact description of relations inside either the sector of society concepts or inside the sector of religions. For example, depending on the edition, the concepts of Fascism and Nazism are attributed to different influence poles such as Communism (EN, FR), Liberalism (DE, IT, RU, ZH) or Capitalism (AR). For the sector of religions, we noted that for some editions the Christianity pole is rather isolated from other religion poles (e.g., EN) while for most other editions it is well connected to other poles of Buddhism, Hinduism, Islam and Chinese folk religion. For a majority of editions, the links between Asian religions represented by the poles of Buddhism, Hinduism and Chinese folk religion are stronger than the links of the two Christianity and Islam poles.

Finally, we also provided a quantitative analysis of inter-edition correlators for various key quantities (reduced Google matrix components or blocks, PageRank vector or Index, etc.), which allows one to determine the proximity or distance of different cultures, represented by the Wikipedia language editions, with respect to their views on the 40 selected Wikipedia articles. For example, for $G_{\mathrm{R}}$ the Arabic (Chinese) culture has the strongest correlations with the Russian (French) culture and the German edition is closest to the Spanish and Italian editions. If we consider the religion diagonal block of $G_{\mathrm{rr}}+G_{\mathrm{qr}}^{\left(\mathrm{nd}\right)}$, we have the strongest culture proximity between EN and ES. Generally speaking, the overall inter-edition correlations are rather large, with most values above $0.5$ often close to 0.8–0.9 and only a few minimal values close to 0.33.

The performed Google matrix analysis allows us to establish mathematical network structures of relations between 40 social concepts and religions. The obtained reduced Google matrix gives the quantitative strength of their interactions that are presented in Figure 3, Figure 4, Figure 5, Figure 6 and Figure 7 for eight Wikipedia editions. We also obtained the related network structure for social concepts and religions depicted in Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14 and Figure 15. This REGOMAX data allow us to determine mathematical proximity of eight cultures summarized in Figure 16. We hope that these mathematical results can be a useful complement for sociological and historical studies of relations between social concepts and religions.

In conclusion, we presented a mathematical network analysis of relations and interactions of 23 society concepts and 17 religions for eight Wikipedia editions allowing to extract nontrivial features of these relations. We note that the described REGOMAX approach is generic and can be applied to any selected subset (topic) of modest size of Wikipedia articles. For example, this can be a set of concepts of philosophy, science and technology.