Decision-Making for Project Delivery System with Related-Indicators Based on Pythagorean Fuzzy Weighted Muirhead Mean Operator

Abstract

An appropriate project delivery system plays an essential role in sustainable construction project management. Due to the complexity of practical problems and the ambiguity of human thinking, selecting an appropriate project delivery system (PDS) is an enormous challenge for owners. This paper aims to develop a PDS selection method to deal with the related-indicators case by combining the advantages of Pythagorean fuzzy sets (PFSs) and Pythagorean fuzzy weighted Muirhead mean (PFWMM) operators. The contributions of this paper are as follows: (1) This study innovatively introduced the PFWMM operator to deal with PDS selection problems for the case of the relevance among all indicators affecting PDSs selection in a complex environment. (2) A new method of solving indicators’ weights was proposed to adapt to the related-indicators PDS selection problem, through investigating the differences between the ideal PDS and the alternative PDS under all indicators. (3) A decision-making framework for PDS selection was constructed by comprehensive use of the advantages of PFSs and the PFWMM operator in dealing with related-indicators PDS decision-making problems. An example of selecting a PDS is exhibited to illustrate the effectiveness and applicability of the proposed method.

1. Introduction

With the development of the construction industry in the national economy, problems such as a complicated external environment, low project profits, and fierce competition have attracted the attention of researchers as well as people working in the construction industry. Scientific decision-making in construction project bidding is becoming increasingly essential for construction enterprises. Project delivery systems (PDSs) give a framework for the implementation of the project, which commonly includes design-bid-build (DBB), design-build (DB), construction management (CM), engineering procurement construction (EPC), etc. [1,2]. Different forms have different characteristics, and therefore, selecting an appropriate form of a PDS is important for the profitability and success of the project’s schedule, cost, quality, and contract management [3,4,5,6]. Moreover, choosing the proper PDS directly affects the achievement of performance goals of the construction project. Therefore, it is a matter of cardinal significance for owners to pay close attention to selecting an appropriate PDS [5].

Many research works have been conducted on PDS selection [2,7,8,9], and the information characterized by the indicators cannot be fully reflected, because of the fuzziness of human thinking and the complexity of the objective. The fuzzy set has widely been used to express the evaluation information in PDS selection. For example, An et al. [1] established a group decision-making model for PDS selection under the interval intuitionistic fuzzy setting, wherein a new weight determination for a decision-maker is introduced by using the information utility level. Su et al. [10] developed a project procurement method selection with interval neutrosophic sets from a multi-criteria decision-making view. Mafakheri et al. [11] utilized the Analytic Hierarchy Process (AHP) interval to determine the interval priorities for alternative PDSs, which were then ranked using rough set theory. Su et al. [12] developed three similarity measures and established an improved TOPSIS PDS decision-making method based on those measures under the Pythagorean fuzzy environment. Based on multi-agent systems, Zhu et al. [13] constructed a decision-making simulation model for the PDS selection. They analyzed the influence of multiple factors impacting the PDS decision: the project attribute characteristics, the policy and market environment, the owner’s ability and preference, and the contractor technology capabilities. The existing research gave a broad theoretical foundation. Comparing with other fuzzy set theories, the Pythagorean fuzzy number (PFN) can be easily seen to describe psychological behavior more effectively when experts provide evaluation information. Moreover, the indicators affecting PDS selection are independent by default, but they are interrelated in practice, as the indicators are usually related to each other more or less.

To deal with the related-indicators case, Peng and Yuan [14] proposed the Choquet integral operator to fuse Pythagorean fuzzy information. To capture the interrelationships among fused PFNs, Liang et al. [15] proposed a number of Pythagorean fuzzy geometric Bonferroni mean operators and discussed their desirable properties. Wei and Lu [16] also developed a family of Pythagorean fuzzy Maclaurin symmetric mean operators. Zhang et al. [17] investigated the generalized Bonferroni mean in the Pythagorean fuzzy environment and proposed a family of generalized Pythagorean fuzzy Bonferroni mean operators. Li et al. [18] developed a Pythagorean fuzzy power Muirhead mean, and the weighted the Pythagorean fuzzy power Muirhead mean operators to consider the relationships between fused data and the interrelationships between all aggregated values. Generally, the Bonferroni mean (BM) [19], the Heronian mean (HM) [20], and the Muirhead mean (MM) [21] can complete this function. However, Liu and Li [22] pointed out that the MM has some advantages over the BM and the HM. The main limitation both of the BM and the HM is that they can only consider the interrelationships between two attributes and cannot capture the case of three or more than three attributes with relevance. Moreover, some existing aggregation operators, such as the BM and the Maclaurin symmetric mean (MSM) [23], are special cases of the MM. Furthermore, the MM has a parameter vector, which can increase the flexibility in aggregation processes; that is, capturing the overall interrelationship among the multiple aggregated arguments is the advantage of the MM operator, and the MM operator is a generalization of most existing operators.

To summarize, there is a research gap in the existing research on PDS selection. There is a lack of consideration of the relevance among indicators affecting PDS selection, and therefore the result of the PDS selection will be more or less distorted. That is, the existing method of selecting a PDS cannot give a relatively objective result, since the operators do not take into account the relevance among indicators.

Therefore, the objective of this research is to develop an indicator-related PDS selection method. Furthermore, Pythagorean fuzzy set theory and Pythagorean fuzzy Muirhead mean (PFWMM) operators given in [24] are comprehensively used in this study. The main work of this paper includes three aspects: (1) The PFWMM operator is innovatively introduced to deal with PDS selection problems for the case of the relevance among all indicators affecting the PDS’s selection under a fuzzy environment. (2) A new method to calculate indicators’ weights was proposed by investigating the differences between the ideal PDS and an alternative PDS under all indicators. (3) A decision-making framework for PDS selection was constructed by the comprehensive use of the advantages of Pythagorean fuzzy sets (PFSs) and the PFWMM operator in dealing with related-indicators PDS decision-making problems. Finally, an example using the proposed method was applied to selecting a PDS.

The rest of this paper is organized as follows: The preliminaries for PDS selection, including the use of a Pythagorean fuzzy number and a weighted Pythagorean fuzzy Muirhead mean operator is provided in Section 2. In Section 3, the PDS selection method based on the PFWMM is constructed. An example study applying the proposed PDS selection method is given in Section 4. The comparative analysis and the conclusions are presented in Section 5 and Section 6, respectively.

2. Preliminaries

This section presents the preliminaries for PDS selection, which includes two subsections: (1) the concept and correlative operations for a Pythagorean fuzzy number, and (2) the definitions and theorems on the weighted Pythagorean fuzzy Muirhead mean operator. These are the fundamental theories for establishing the related-indicators PDS selection.

2.1. Pythagorean Fuzzy Number

This subsection provides some basic concepts, definitions, and operational laws of a PFN, which are utilized in the analysis.

Definition 1

([25]). If $X$ is a fixed set, then

$$\mathrm{P}=\left\{\langle x,{\mu }_{\mathrm{P}}\left(x\right),v_{\mathrm{P}}\left(x\right)\rangle |x\in X\right\}$$

is called a Pythagorean fuzzy Set (PFS) on $X$, where ${\mu }_{\mathrm{P}}\left(x\right)$ and $v_{\mathrm{P}}\left(x\right)$ are the membership degree and non-membership degree of $x\in X$, respectively, that is, ${\mu }_{\mathrm{P}}$: $X\to \left[0,1\right]$, $v_{\mathrm{P}}$: $X\to \left[0,1\right]$, and $0\le {\mu }_{\mathrm{P}}^2+v_{\mathrm{P}}^2\le 1$, $\forall x\in X$. Furthermore, ${\pi }_{\mathrm{P}}\left(x\right)=\sqrt{1-{\mu }_{\mathrm{P}}^2\left(x\right)-v_{\mathrm{P}}^2\left(x\right)}$, $\forall x\in X$, denotes the hesitation degree of the element$x\in X$ to $\mathrm{P}$. In particular, if ${\pi }_{\mathrm{P}}\left(x\right)=0$ for $\forall x\in X$, then $\mathrm{P}$ is the typical fuzzy set. For brevity, $\alpha =\left({\mu }_{\alpha },v_{\alpha }\right)$ indicates the Pythagorean fuzzy number (PFN) [26], and ${\mu }_{\alpha }\in \left[0,1\right]$, $v_{\alpha }\in \left[0,1\right]$, and $0\le {\mu }_{\alpha }^2+v_{\alpha }^2\le 1$.

Definition 2

([27]). Let $\alpha$ be a PFN; a score function $\mathrm{S}$ of the PFN $\alpha$ is defined as follows:

$$\mathrm{S}\left(\alpha \right)=\frac{1}{2}\left(1+{\mu }_{\alpha }^2-v_{\alpha }^2\right),\mathrm{S}\left(\alpha \right)\in \left[0,1\right].$$

(1)

Definition 3

([26]). Let $\alpha$ be a PFN, an accuracy function $\mathrm{H}$ of the PFN $\alpha$ is defined as follows:

$$\mathrm{H}\left(\alpha \right)={\mu }_{\alpha }^2-v_{\alpha }^2,\mathrm{H}\left(\alpha \right)\in \left[0,1\right].$$

(2)

Generally, the larger the value of the score function, the larger the value of the PFN. However, when the values of the score functions between two PFNs are equal, the larger the value of the accuracy function, the larger the value of the PFN. Therefore, we define the comparison method for two PFNs.

Definition 4

([27]). Let ${\alpha }_1=\langle {\mu }_1,v_1\rangle$ and ${\alpha }_2=\langle {\mu }_2,v_2\rangle$ be any two PFNs, then their score functions are $\mathrm{S}\left({\alpha }_1\right)=\frac{1}{2}\left(1+{\mu }_{{\alpha }_1}^2-v_{{\alpha }_1}^2\right)$ and $\mathrm{S}\left({\alpha }_2\right)=\frac{1}{2}\left(1+{\mu }_{{\alpha }_2}^2-v_{{\alpha }_2}^2\right)$. Accuracy functions are $\mathrm{H}\left({\alpha }_1\right)={\mu }_{{\alpha }_1}^2+v_{{\alpha }_1}^2$ and $\mathrm{H}\left({\alpha }_2\right)={\mu }_{{\alpha }_2}^2+v_{{\alpha }_2}^2$, and the comparison rules between two ${\alpha }_1$ and ${\alpha }_2$ are:

(1) 

If$\mathrm{S}\left({\alpha }_1\right)<\mathrm{S}\left({\alpha }_2\right)$, then${\alpha }_1<{\alpha }_2$;

(2) 

If$\mathrm{S}\left({\alpha }_1\right)=\mathrm{S}\left({\alpha }_2\right)$, then

• If$\mathrm{H}\left({\alpha }_1\right)<\mathrm{H}\left({\alpha }_2\right)$, then ${\alpha }_1<{\alpha }_2$;
• If$\mathrm{H}\left({\alpha }_1\right)>\mathrm{H}\left({\alpha }_2\right)$, then ${\alpha }_1>{\alpha }_2$.

Definition 5

([28]). Let $\alpha =\left({\mu }_{\alpha },v_{\alpha }\right)$, ${\alpha }_1=\left({\mu }_{{\alpha }_1},v_{{\alpha }_1}\right)$ and ${\alpha }_2=\left({\mu }_{{\alpha }_2},v_{{\alpha }_2}\right)$ be three PFNs, $\lambda >0$, and some of their basic operations are as follows:

(A1) 

${\alpha }_1\oplus {\alpha }_2=\left(\sqrt{{\mu }_{{\alpha }_1}^2+{\mu }_{{\alpha }_2}^2-{\mu }_{{\alpha }_1}^2\cdot {\mu }_{{\alpha }_2}^2},v_{{\alpha }_1}\cdot v_{{\alpha }_2}\right)$;

(A2) 

${\alpha }_1\otimes {\alpha }_2=\left({\mu }_{{\alpha }_1}\cdot {\mu }_{{\alpha }_2},\sqrt{v_{{\alpha }_1}^2+v_{{\alpha }_2}^2-v_{{\alpha }_1}^2\cdot v_{{\alpha }_2}^2}\right)$;

(A3) 

$\lambda \alpha =\left(\sqrt{1-{\left(1-{\mu }_{\alpha }^2\right)}^{\lambda }},v_{\alpha }^{\lambda }\right)$;

(A4) 

${\alpha }^{\lambda }=\left({\mu }_{\alpha }^{\lambda },\sqrt{1-{\left(1-v_{\alpha }^2\right)}^{\lambda }}\right)$;

(A5) 

${\alpha }^c=\left(v_{\alpha },{\mu }_{\alpha }\right)$, which is the complement operation of$\alpha$, where $c>0$.

Furthermore, Zhang and Xu [29] also proposed a Pythagorean fuzzy distance measure for PFNs.

Definition 6

([29]). Let ${\alpha }_1=\left({\mu }_{{\alpha }_1},v_{{\alpha }_1}\right)$ and ${\alpha }_2=\left({\mu }_{{\alpha }_2},v_{{\alpha }_2}\right)$ be two PFNs. Then, the distance between them is defined as follows:

$$d\left({\alpha }_1,{\alpha }_2\right)=\frac{1}{2}\left(\left|{\mu }_{{\alpha }_1}^2-{\mu }_{{\alpha }_2}^2\right|+\left|v_{{\alpha }_1}^2-v_{{\alpha }_2}^2\right|+\left|{\pi }_{{\alpha }_1}^2-{\pi }_{{\alpha }_2}^2\right|\right).$$

(3)

Definition 7

([30]). Assume that ${\alpha }_i=\left({\mu }_i,v_i\right)$ $\left(i=1,2,\dots ,n\right)$ is a PFN set and $w=\left(w_1,w_2,\dots ,w_n\right)$ is the weight vector, where $w_i\in \left[0,1\right]$ and ${\displaystyle \sum _{i=1}^n{w}_i}=1$, then a Pythagorean fuzzy weighted aggregate (PFWA) operator is expressed as:

$$\mathrm{PFWA}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)=\alpha \left({\displaystyle \sum _{i=1}^n{w}_i{\mu }_i},{\displaystyle \sum _{i=1}^n{w}_i{v}_i}\right).$$

(4)

2.2. Pythagorean Fuzzy Weighted Muirhead Mean Operator

Definition 8

([21]). Let $S=\left\{{\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right\}$ be a set of real numbers, and $\left[\lambda \right]=\left({\lambda }_1,{\lambda }_2,\dots ,{\lambda }_n\right)\in R$, then the Muirhead mean operator is defined as follows:

$${\mathrm{MM}}^{\lambda }\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n!}{\displaystyle \sum _{\vartheta \in S_n}{\displaystyle \prod _{j=1}^n{\alpha }_{\vartheta \left(j\right)}^{{\lambda }_j}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}},$$

(5A)

where $\vartheta \left(j\right)$ $\left(j=1,2,\dots ,n\right)$ is any permutation of $\left(1,2,\dots ,n\right)$, and $S_n=\left(\vartheta \left(1\right),\vartheta \left(2\right),\dots ,\vartheta \left(n\right)\right)$.

Moreover, there are some special cases of the MM operator with different vectors as follows:

① If $\lambda =\left(1,1,0,\dots ,0\right)$, then Equation (5A) reduces to the following equation:

$${\mathrm{MM}}^{\left(1,1,0,\dots ,0\right)}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n\left(n-1\right)}{\displaystyle \sum _{i\underset{i\ne j}{,j=1}}^n{\alpha }_i{\alpha }_j}\right)}^{\frac{1}{2}},$$

(5B)

which is the BM operator.

② If $\lambda =\left(1,0,\dots ,0\right)$, then Equation (5A) reduces to the following equation:

$${\mathrm{MM}}^{\left(1,0,\dots ,0\right)}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)=\frac{1}{n}{\displaystyle \sum _{j=1}^n{\alpha }_j},$$

(5C)

which is the arithmetic averaging operator.

③ If $\lambda =\left(1/n,1/n,\dots ,1/n\right)$, then Equation (5A) reduces to the following equation:

$${\mathrm{MM}}^{\left(1/n,\dots ,1/n\right)}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\displaystyle \prod _{j=1}^n{\alpha }_j^{1/n}},$$

(5D)

which is the geometric averaging operator.

④ If $\lambda =\stackrel{k}{\overbrace{(1,1,\cdots ,1,}}\stackrel{n-k}{\overbrace{0,0,\dots ,0)}}$, then Equation (5A) reduces to the following equation:

$${\mathrm{MM}}^{\stackrel{k}{\overbrace{(1,1,\cdots ,1,}}\stackrel{n-k}{\overbrace{0,0,\dots ,0)}}}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{\underset{1\le i_1\prec \cdots \prec i_k\le n}{\oplus }\underset{j=1}{\overset{k}{\otimes }}{\alpha }_{ij}}{C_n^k}\right)}^{\frac{1}{k}},$$

(5E)

which is the Maclaurin symmetric mean (MSM) operator.

Therefore, the advantage of the MM operator is that it can capture the overall interrelationships among the multiple aggregated arguments from the above special cases of the MM operator.

Definition 9

([24]). Let ${\alpha }_j=\left({\mu }_j,v_j\right)$ $\left(j=1,2,\dots ,n\right)$ denote a set of PFNs, and $\left[\lambda \right]=\left({\lambda }_1,{\lambda }_2,\dots ,{\lambda }_n\right)\in R$ is a parameter vector. Then the Pythagorean fuzzy Muirhead mean operator (PFMM) is defined as follows:

$${\mathrm{PFMM}}^{\lambda }\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n!}{\displaystyle \sum _{\vartheta \in S_n}{\displaystyle \prod _{j=1}^n{\alpha }_{\vartheta \left(j\right)}^{{\lambda }_j}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}},$$

(6)

where $\vartheta \left(j\right)$ $\left(j=1,2,\dots ,n\right)$ is any permutation of $\left(1,2,\dots ,n\right)$, and $S_n=\left(\vartheta \left(1\right),\vartheta \left(2\right),\dots ,\vartheta \left(n\right)\right)$.

Theorem 1

([24]). Let ${\alpha }_j=\left({\mu }_j,v_j\right)$ $\left(j=1,2,\dots ,n\right)$ denote a set of PFNs, then the aggregated value of them obtained by using PFMM is also a PFN, and

$${\mathrm{PFMM}}^{\lambda }\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n!}{\displaystyle \sum _{\vartheta \in S_n}{\displaystyle \prod _{j=1}^n{\alpha }_{\vartheta \left(j\right)}^{{\lambda }_j}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}}$$

$$=({\left(\sqrt{1-{\left({\displaystyle {\prod }_{\vartheta \in S_n}\left(1-{\displaystyle \prod _{j=1}^n{\mu }_{\vartheta \left(j\right)}^{2{\lambda }_j}}\right)}\right)}^{\frac{1}{n!}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}},\sqrt{1-{\left(1-{\left({\displaystyle \prod _{\vartheta \in S_n}\left(1-{\displaystyle \prod _{j=1}^n{\left(1-v_{\vartheta \left(j\right)}^2\right)}^{{\lambda }_j}}\right)}\right)}^{\frac{1}{n!}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}}}).$$

Tang et al. [24] indicated that the PFMM operator has three properties: (1) idempotency; (2) monotonicity; and (3) boundedness. Tang et al. [24] also proposed the Pythagorean fuzzy weighted Muirhead mean (PFWMM) operator, which overcomes the limitation of the PFMM not considering the importance of the aggregated arguments in the process of aggregation.

Definition 10

([24]). Let ${\alpha }_j=\left({\mu }_j,v_j\right)$ $\left(j=1,2,\dots ,n\right)$ denote a set of PFNs, and the weight vector be $W=\left(w_1,w_2,\dots ,w_n\right)$, where $w_j\in \left[0,1\right]$ and ${\displaystyle {\sum }_{j=1}^n{w}_j}=1$, and $\left[\lambda \right]=\left({\lambda }_1,{\lambda }_2,\dots ,{\lambda }_n\right)\in R$. Then the Pythagorean fuzzy weighted Muirhead mean (PFWMM) operator is defined as follows:

$${\mathrm{PFWMM}}_w^{\lambda }\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n!}{\displaystyle \sum _{\vartheta \in S_n}{\displaystyle \prod _{j=1}^n{\left(n{w}_{\vartheta \left(j\right)}{\alpha }_{\vartheta \left(j\right)}\right)}^{{\lambda }_j}}}\right)}^{\frac{1}{{\displaystyle {\sum }_{j=1}^n{\lambda }_j}}}.$$

(7)

Theorem 2

([24]). Let ${\alpha }_j=\left({\mu }_j,v_j\right)$ $\left(j=1,2,\dots ,n\right)$ denote a set of PFNs, then the aggregated value of them obtained by using the PFWMM is also a PFN, and

$${\mathrm{PFWMM}}^{\lambda }\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\left(\frac{1}{n!}{\displaystyle \sum _{\vartheta \in S_n}{\displaystyle \prod _{j=1}^n{\left(n{w}_{\vartheta \left(j\right)}{\alpha }_{\vartheta \left(j\right)}^{}\right)}^{{\lambda }_j}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}}$$

$$\begin{gathered} =({\left(\sqrt{1-{\left({\displaystyle {\prod }_{\vartheta \in S_n}\left(1-{\displaystyle \prod _{j=1}^n{\left(1-{\left(1-{\mu }_{\vartheta \left(j\right)}^2\right)}^{n{w}_{\vartheta \left(j\right)}}\right)}^{{\lambda }_j}}\right)}\right)}^{\frac{1}{n!}}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}}, \\ \sqrt{1-{\left(1-{\left({\displaystyle \prod _{\vartheta \in S_n}\left(1-{\displaystyle \prod _{j=1}^n{\left(1-v_{\vartheta \left(j\right)}^{2n{w}_{\vartheta \left(j\right)}}\right)}^{{\lambda }_j}}\right)}\right)}^{\frac{1}{n!}}\right)}^{\frac{1}{{\displaystyle \sum _{j=1}^n{\lambda }_j}}}}). \end{gathered}$$

There are two properties for the PFWMM operator: monotonicity and boundedness, and the detailed proof is shown in Tang et al. [24].

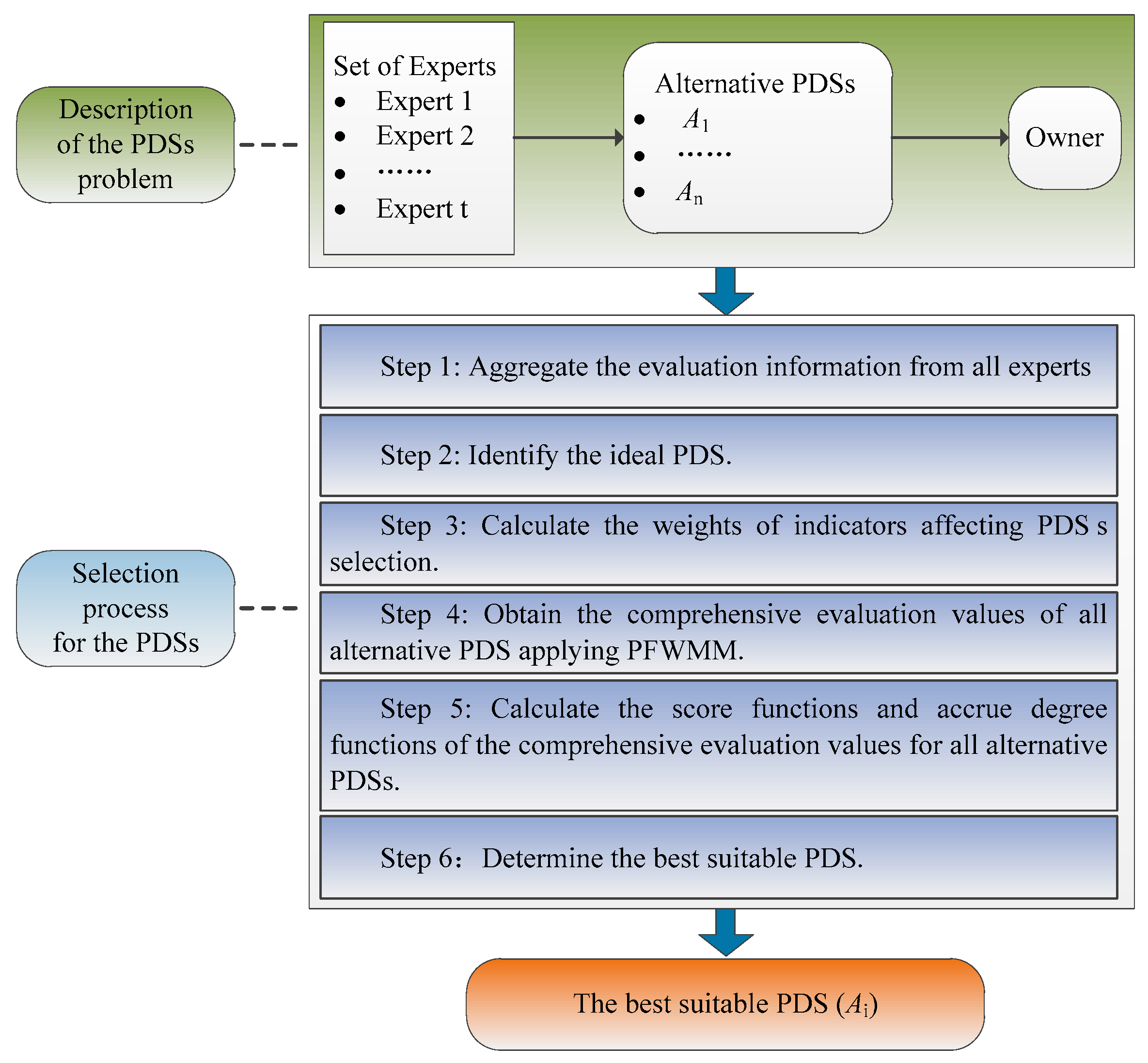
3. Decision-Making Method for PDS Selection Based on PFWMM

In the Pythagorean fuzzy environment, let $A=\left\{A_1,A_2,\dots ,A_n\right\}$ be an alternative PDS set, and $C=\left\{C_1,C_2,\dots ,C_m\right\}$ be a set of the indicators affecting PDS selection, where the weight of each indicator is $w_j\in \left[0,1\right]$, the weight vector of the indicator set is $W=\left\{w_1,w_2,\dots ,w_m\right\}$, and ${\displaystyle \sum _{j=1}^m{w}_j}=1$. It is assumed that $t$ experts give evaluation values characterized by the PFNs of each PDS under different indicators. If the PNF $e_{ij}^{\left(l\right)}=\langle {\mu }_{ij}^{\left(l\right)},{\nu }_{ij}^{\left(l\right)}\rangle$ denotes the evaluation value of the $i\mathrm{th}$ PDS under the $j\mathrm{th}$ indicator from the $l\mathrm{th}$ expert, where ${\mu }_{ij}^{\left(l\right)}$ and $v_{ij}^{\left(l\right)}$ denote membership and non-membership degrees, respectively. Thus, the evaluation matrix can be obtained as follows:

$$E=\left(\begin{array}{ccc}{e}_{11}& \cdots & e_{1m}\\ ⋮& \ddots & ⋮\\ e_{n1}& \cdots & e_{nm}\end{array}\right),$$

(8)

where $e_{ij}=\langle {\mu }_{ij},v_{ij}\rangle$ is the aggregate information of the $i\mathrm{th}$ PDS under the $j\mathrm{th}$ indicator from $t$ experts, where $i=1,2,\dots ,n$, $j=1,2,\dots ,m$ and $l=1,2,\dots ,t$.

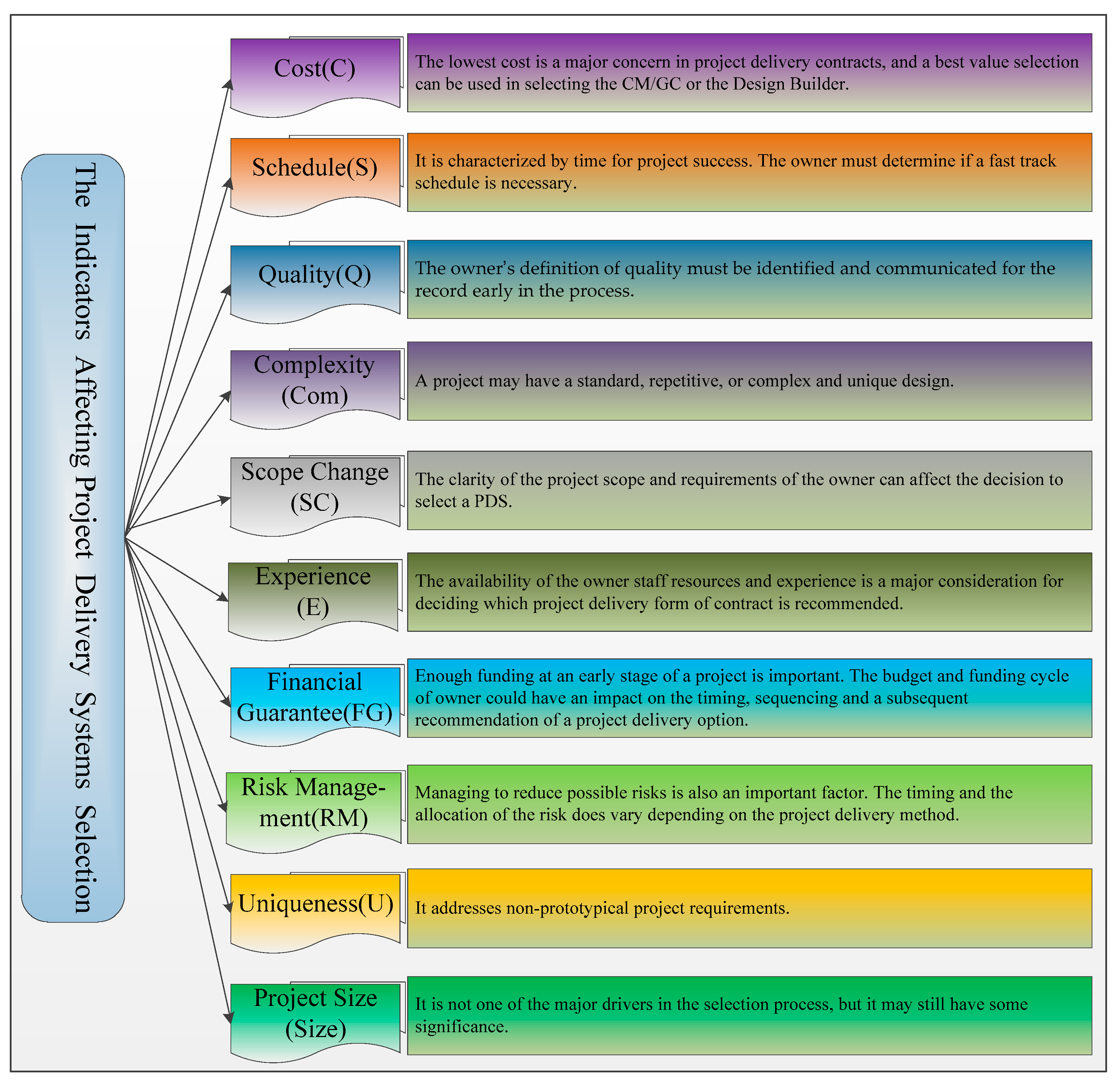
3.1. Construct the Decision-Making Indicator System for PDS Selection

For a given construction project, the alternative PDS can be chosen from design-bid-build (DBB), design-build (DB), construction management (CM), and engineering procurement construction (EPC). Many indicators affect a suitable PDS selection [11,12]; the indicators and their interpretation are shown as in Figure 1.

3.2. Determine Weights of Indicators Affecting PDSs Selection

Many approaches can be used to determine the weights of the indicators, for example, the entropy method [31], the analytic hierarchy process [32], and the best worst method [33]. They have their own advantages, but they also have some deficiencies for the related-indicators case. To fully consider the effect of correlativity among indicators, we establish a new method of solving weights of indicators by investigating the differences between the ideal PDS and the alternative PDSs under all indicators, which would be a basis to determine weights of indicators. In this way, the effect of related-indicators is relatively diminished. We describe the approach to solve the calculation of the weights of the indicators below:

Definition 11.

Let$e_{ij}=\langle {\mu }_{ij},v_{ij}\rangle$$(i=1,2,\dots ,n$, $j=1,2,\dots ,m)$ be the evaluation value of the$i\mathrm{th}$alternative PDS under the$j\mathrm{th}$indicator, and$e_j^{*}=\langle {\mu }_j^{*},v_j^{*}\rangle$be the evaluation value of the ideal PDS under the$j\mathrm{th}$indicator. If$d_j\left(A_i,A^{*}\right)$denotes the distance measure between the$i\mathrm{th}$alternative PDS and the ideal PDS under the$j\mathrm{th}$indicator, then the weight of the indicator is as follows:

$$\{\begin{array}{c}{w}_1=w_1=\cdots =w_m=1/m,\\ w_j=\frac{1/D_j}{{\displaystyle {\sum }_{j=1}^n\left(1/D_j\right)}},\end{array}\begin{array}{c}\\ \end{array}\begin{array}{c}\underset{}{D_j=0}\\ \underset{}{D_j\ne 0}\end{array},$$

(9)

where$D_j={\displaystyle \sum _{i=1}^n{d}_j\left(A_i,A^{*}\right)}$, which is the sum of all distance measures between alternative PDSs and the ideal PDS for the$j\mathrm{th}$indicator. Obviously,$w_j\in \left[0,1\right]$and ${\displaystyle \sum _{j=1}^m{w}_j}=1$.

3.3. Aggregate the Evaluation Information from All Experts

From the Pythagorean fuzzy weighted averaging (PFWA) operator in Definition 7, the evaluation information from all experts is aggregated and the comprehensive evaluation information for the PDS selection is obtained. Therefore, the aggregate information of the $i\mathrm{th}$ PDS under the $j\mathrm{th}$ indicator from $t$ experts can be obtained by Equation (6) $e_{ij}=e_{ij}\left(e_{ij}^{\left(1\right)},e_{ij}^{\left(2\right)},\dots ,e_{ij}^{\left(t\right)}\right)=e_{ij}\left({\displaystyle \sum _{l=1}^t{W}_l{\mu }_{ij}^{\left(l\right)}},{\displaystyle \sum _{l=1}^l{W}_l{v}_{ij}^{\left(l\right)}}\right)$, where $W_l$ is the weight of the $l\mathrm{th}$ expert, $i=1,2,\dots ,n$, $j=1,2,\dots ,m$ and $l=1,2,\dots ,t$. Moreover, the averaging weighting method is applied for convenience, that is, $W_1=W_2=\cdots =W_t=1/t$.

3.4. The Selection Procedure for the Alternative PDSs

For a given PDS selection problem, fixed experts are invited to select PDSs based on determined indicators affecting PDS selection. As mentioned above, the alternative PDSs set is $A=\left(A_1,A_2,\dots ,A_n\right)$, the set of indicators is $C=\left(C_1,C_2,\dots ,C_m\right)$, and the weight vector of criteria is $w=\left(w_1,w_2,\dots ,w_m\right)$. There are $t$ experts who are invited to give the evaluation information for the PDS selection, and the evaluation information matrix is $E^{\left(l\right)}$ from the $l\mathrm{th}$ expert. According to the above illustration, we present a selection process for alternative PDSs, as shown in Figure 2.

Step 1: Aggregate the evaluation information from all experts.

If the evaluation information matrix from the $l\mathrm{th}$ expert is $E^{\left(l\right)}={\left(e_{ij}^{\left(l\right)}\right)}_{n\times m}={\left({\mu }_{ij}^{\left(l\right)},v_{ij}^{\left(l\right)}\right)}_{n\times m}$, then the aggregated evaluation information matrix is $E={\left(e_{ij}\right)}_{n\times m}$ using Definition 7, where $e_{ij}=$$e_{ij}\left({\displaystyle \sum _{l=1}^t{W}_l{\mu }_{ij}^{\left(l\right)}},{\displaystyle \sum _{l=1}^t{W}_l{v}_{ij}^{\left(l\right)}}\right)$ and $W_1=W_2=\cdots =W_t=1/t$.

Step 2: Identify the ideal PDS.

An ideal PDS can be expressed by using the maximum evaluation value for the benefit criteria and the minimum evaluation value for the cost criteria. If the sets of benefit criteria and cost criteria are $B=\left\{\right\}$ and $P$, respectively, then the ideal PDS $A^{*}=\left(e_1^{*},e_2^{*},\dots ,e_m^{*}\right)$ is represented as:

$$A^{*}=\left(e_1^{*},e_2^{*},\dots ,e_m^{*}\right),$$

(10)

where $e_j^{*}=\underset{i}{\max }\langle {\mu }_{ij},v_{ij}\rangle$ for $C_j\in B$, and $e_j^{*}=\underset{i}{\min }\langle {\mu }_{ij},v_{ij}\rangle$ for $C_j\in P$.

Step 3: Calculate the weights of the indicators affecting the PDSs selection.

From Equation (9), the distance measure between alternative PDSs and the ideal PDS under the $j\mathrm{th}$ indicator is calculated as follows:

$$d_j\left(A_i,A^{*}\right)=\frac{1}{2}\left(\left|{\mu }_{ij}^2-{\mu }_j^{*}{}^2\right|+\left|v_{ij}^2-v_j^{*}{}^2\right|+\left|{\pi }_{ij}^2-{\pi }_j^{*}{}^2\right|\right).$$

Therefore, the weight of the $j\mathrm{th}$ indicator from Equation (7) is

$$\{\begin{array}{c}{w}_1=w_1=\cdots =w_m=1/m,\\ w_j=\frac{1/D_j}{{\displaystyle {\sum }_{j=1}^n\left(1/D_j\right)}},\end{array}\begin{array}{c}\\ \end{array}\begin{array}{c}\underset{}{D_j=0}\\ \underset{}{D_j\ne 0}\end{array},$$

where $D_j={\displaystyle \sum _{i=1}^n{d}_j\left(A_i,A^{*}\right)}$.

Step 4: Obtain the comprehensive evaluation values of all alternative PDSs, applying the PFWMM.

Aggregating evaluation values ${\tilde{e}}_{ij}$$\left(j=1,2,\dots ,m\right)$ of the $i\mathrm{th}$ alternative PDS under $j$ indicators affecting the PDS selection according to Equation (5A) in Definition 10, the comprehensive evaluation value of the $i\mathrm{th}$ alternative PDS is obtained.

Step 5: Calculate the score functions and accrue degree functions of the comprehensive evaluation values for all alternative PDSs.

To compare the comprehensive evaluation values for all alternative PDSs, their score functions and their accrued degree functions are calculated, and the calculation method and comparison rule are shown in Definition 4.

Step 6: Determine the best suitable PDS.

According to the outcome of the comparisons in Step 5, a bigger comprehensive evaluation value corresponds to a better alternative PDS, therefore, the best suitable PDS is selected.

4. Case Study

For a real-world infrastructure project, there are four PDSs including construction management (CM), engineering procurement construction (EPC), design-build (DB), design-bid-build (DBB) for selection. The indicators affecting the PDS selection are shown in Section 3.1. They are cost (C), schedule (S), quality (Q), complexity (Com), scope change (SC), experience (E), financial guarantee (FG), risk management (RM), uniqueness (U), and project size (Size). Firstly, the cost (C), schedule (S) and quality (Q) are inter-conditioned and mutually constraining relationships. If the schedule of the project is compressed, the costs will be increased, and the construction quality of the project will be affected. Secondly, complexity (Com) and project size (Size) are generally relevant, as well. Furthermore, the financial guarantee (FG) affects cost (C), schedule (S), and quality (Q). If the owners have abundant experience in project management, then it will have a positive influence on risk management (RM), cost (C), schedule (S), and quality (Q).

To ensure the reliability and availability of data, five experienced experts, including an engineer, an academic, a contractor, a consultant and an owner in this field were invited to act as the decision makers. The work process generally included the following procedure: (1) The owners introduced their capacity and the goal of project. (2) The construction site was further investigated, and the related principals described the whole project in detail. (3) A score chart and score criterion, determined in advance, were used by every invited expert to provide the evaluation information of this project, and the aggregated information of all experts was used as the final evaluation information.

In this selection process, the set $A=\left\{A_1,A_2,A_3,A_4\right\}$ of delivery options is the four PDSs (CM, EPC, DBB, and DB), and the set $C=\left\{C_1,C_2,C_3,C_4,C_5,C_6,C_7,C_8,C_9,C_{10}\right\}$ denotes the set of ten indicators (i.e., C, S, Q, Com, SC, E, FG, RM, U, and Size), where cost indicators are C, S, Com, SC, U, Size, and benefit indicators are Q, E, FG, RM. We assume that $\left(u_{ij}^{\left(l\right)},v_{ij}^{\left(l\right)}\right)$ $(i=1,2,3,4;$ $j=1,2,\dots ,10;$ $l=1,2,3,4,5)$ is the evaluation value from the $l\mathrm{th}$ expert to delivery option $A_i$ with respect to criteria $C_j$; and $E_{4\times 10}^{\left(l\right)}={\left(E_{ij}^{\left(l\right)}\right)}_{4\times 10}={\left(u_{ij}^{\left(l\right)},v_{ij}^{\left(l\right)}\right)}_{4\times 10}$ $\left(l=1,2,3,4,5\right)$ denotes the PFN evaluation matrix from the $l\mathrm{th}$ expert. They are as follows:

$$\begin{array}{l}{E}_{4\times 10}^{\left(1\right)}=(\begin{array}{ccccc}\mathrm{p}\left(0.7,0.2\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.6,0.2\right)& \mathrm{p}\left(0.6,0.2\right)\\ \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.8,0.4\right)\\ \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.5,0.3\right)\\ \mathrm{p}\left(0.5,0.2\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.7,0.3\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.4,0.4\right)& \mathrm{p}\left(0.6,0.3\right)\\ \mathrm{p}\left(0.6,0.2\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.7,0.2\right)\\ \mathrm{p}\left(0.9,0.3\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.7,0.2\right)& \mathrm{p}\left(0.4,0.5\right)\\ \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.4,0.2\right)& \mathrm{p}\left(0.6,0.4\right)\end{array})\end{array}\end{array}\end{array};$$

$$\begin{array}{l}{E}_{4\times 10}^{\left(2\right)}=(\begin{array}{ccccc}\mathrm{p}\left(0.5,0.7\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.6,0.7\right)\\ \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.9,0.2\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.7,0.5\right)\\ \mathrm{p}\left(0.4,0.8\right)& \mathrm{p}\left(0.3,0.6\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.5,0.7\right)\\ \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.4,0.8\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.6,0.7\right)\\ \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.5,0.7\right)& \mathrm{p}\left(0.9,0.3\right)\\ \mathrm{p}\left(0.5,0.8\right)& \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.9,0.3\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.4,0.6\right)\\ \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.9,0.2\right)\end{array})\end{array}\end{array}\end{array};$$

$$\begin{array}{l}{E}_{4\times 10}^{\left(3\right)}=(\begin{array}{ccccc}\mathrm{p}\left(0.8,0.7\right)& \mathrm{p}\left(0.7,0.6\right)& \mathrm{p}\left(0.7,0.6\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.6,0.6\right)\\ \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.7,0.6\right)& \mathrm{p}\left(0.6,0.6\right)& \mathrm{p}\left(0.5,0.7\right)& \mathrm{p}\left(0.5,0.6\right)\\ \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.5,0.8\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.3,0.8\right)& \mathrm{p}\left(0.3,0.8\right)\\ \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.5,0.6\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.5,0.8\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.7,0.7\right)& \mathrm{p}\left(0.7,0.6\right)\\ \mathrm{p}\left(0.5,0.5\right)& \mathrm{p}\left(0.5,0.5\right)& \mathrm{p}\left(0.7,0.6\right)& \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.6,0.6\right)\\ \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.5,0.7\right)& \mathrm{p}\left(0.5,0.7\right)& \mathrm{p}\left(0.4,0.8\right)& \mathrm{p}\left(0.3,0.8\right)\\ \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.6,0.8\right)& \mathrm{p}\left(0.7,0.7\right)& \mathrm{p}\left(0.5,0.8\right)& \mathrm{p}\left(0.7,0.6\right)\end{array})\end{array}\end{array}\end{array};$$

$$\begin{array}{l}{E}_{4\times 10}^{\left(4\right)}=(\begin{array}{ccccc}\mathrm{p}\left(0.4,0.5\right)& \mathrm{p}\left(0.4,0.5\right)& \mathrm{p}\left(0.4,0.4\right)& \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.6,0.5\right)\\ \mathrm{p}\left(0.9,0.4\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.8,0.7\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.5,0.4\right)\\ \mathrm{p}\left(0.6,0.7\right)& \mathrm{p}\left(0.6,0.6\right)& \mathrm{p}\left(0.6,0.6\right)& \mathrm{p}\left(0.4,0.3\right)& \mathrm{p}\left(0.2,0.3\right)\\ \mathrm{p}\left(0.1,0.3\right)& \mathrm{p}\left(0.2,0.7\right)& \mathrm{p}\left(0.2,0.3\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.8,0.3\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.4,0.5\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.5,0.4\right)\\ \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.8,0.4\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.8,0.3\right)\\ \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.4,0.3\right)& \mathrm{p}\left(0.8,0.5\right)& \mathrm{p}\left(0.8,0.3\right)& \mathrm{p}\left(0.7,0.5\right)\\ \mathrm{p}\left(0.3,0.4\right)& \mathrm{p}\left(0.8,0.2\right)& \mathrm{p}\left(0.2,0.3\right)& \mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.2,0.5\right)\end{array})\end{array}\end{array}\end{array};$$

$$\begin{array}{l}{E}_{4\times 10}^{\left(5\right)}=(\begin{array}{ccccc}\mathrm{p}\left(0.7,0.5\right)& \mathrm{p}\left(0.4,0.6\right)& \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.3,0.5\right)& \mathrm{p}\left(0.8,0.3\right)\\ \mathrm{p}\left(0.5,0.2\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.5,0.4\right)\\ \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.5,0.2\right)& \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.5,0.4\right)& \mathrm{p}\left(0.6,0.3\right)\\ \mathrm{p}\left(0.6,0.4\right)& \mathrm{p}\left(0.7,0.2\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.4,0.2\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.7,0.6\right)& \mathrm{p}\left(0.3,0.8\right)& \mathrm{p}\left(0.4,0.5\right)& \mathrm{p}\left(0.3,0.6\right)\\ \mathrm{p}\left(0.7,0.4\right)& \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.5,0.4\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.6,0.4\right)\\ \mathrm{p}\left(0.6,0.3\right)& \mathrm{p}\left(0.6,0.5\right)& \mathrm{p}\left(0.3,0.7\right)& \mathrm{p}\left(0.5,0.3\right)& \mathrm{p}\left(0.6,0.5\right)\\ \mathrm{p}\left(0.7,0.2\right)& \mathrm{p}\left(0.5,0.2\right)& \mathrm{p}\left(0.7,0.3\right)& \mathrm{p}\left(0.8,0.2\right)& \mathrm{p}\left(0.7,0.2\right)\end{array})\end{array}\end{array}\end{array}.$$

According to the selection procedure for PDSs, the steps are as follows:

Step 1:

Construct the Pythagorean fuzzy evaluation matrix by aggregating the evaluation information of the five experts by Definition 5, where the weights of every expert are $W_1=W_2=\cdots =W_5=0.2$. The Pythagorean fuzzy evaluation matrix is determined as follows:

$$\begin{array}{l}{E}_{4\times 10}={\left({\mathrm{p}}_{ij}\left({\displaystyle \sum _{l=1}^5{W}_l{u}_{ij}^{\left(l\right)}},{\displaystyle \sum _{l=1}^5{W}_l{v}_{ij}^{\left(l\right)}}\right)\right)}_{4\times 10}\\ \begin{array}{cc}& \end{array}=(\begin{array}{ccccc}\mathrm{p}\left(0.62,0.52\right)& \mathrm{p}\left(0.56,0.58\right)& \mathrm{p}\left(0.60,0.44\right)& \mathrm{p}\left(0.58,0.46\right)& \mathrm{p}\left(0.64,0.46\right)\\ \mathrm{p}\left(0.72,0.40\right)& \mathrm{p}\left(0.76,0.38\right)& \mathrm{p}\left(0.68,0.50\right)& \mathrm{p}\left(0.62,0.48\right)& \mathrm{p}\left(0.60,0.46\right)\\ \mathrm{p}\left(0.58,0.60\right)& \mathrm{p}\left(0.48,0.50\right)& \mathrm{p}\left(0.66,0.44\right)& \mathrm{p}\left(0.56,0.42\right)& \mathrm{p}\left(0.42,0.48\right)\\ \mathrm{p}\left(0.46,0.38\right)& \mathrm{p}\left(0.60,0.48\right)& \mathrm{p}\left(0.62,0.38\right)& \mathrm{p}\left(0.66,0.42\right)& \mathrm{p}\left(0.56,0.44\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.60,0.38\right)& \mathrm{p}\left(0.64,0.50\right)& \mathrm{p}\left(0.58,0.54\right)& \mathrm{p}\left(0.58,0.44\right)& \mathrm{p}\left(0.54,0.52\right)\\ \mathrm{p}\left(0.68,0.38\right)& \mathrm{p}\left(0.62,0.42\right)& \mathrm{p}\left(0.70,0.44\right)& \mathrm{p}\left(0.60,0.48\right)& \mathrm{p}\left(0.72,0.36\right)\\ \mathrm{p}\left(0.64,0.52\right)& \mathrm{p}\left(0.56,0.46\right)& \mathrm{p}\left(0.66,0.50\right)& \mathrm{p}\left(0.62,0.40\right)& \mathrm{p}\left(0.48,0.58\right)\\ \mathrm{p}\left(0.62,0.44\right)& \mathrm{p}\left(0.66,0.46\right)& \mathrm{p}\left(0.64,0.40\right)& \mathrm{p}\left(0.64,0.44\right)& \mathrm{p}\left(0.62,0.38\right)\end{array})\end{array}\end{array}\end{array}.$$

Step 2:

From Equation (10), the ideal PDS is obtained:

$$\begin{array}{l}{A}^{*}=(\begin{array}{ccccc}\mathrm{p}\left(0.58,0.60\right)& \mathrm{p}\left(0.56,0.58\right)& \mathrm{p}\left(0.66,0.44\right)& \mathrm{p}\left(0.58,0.46\right)& \mathrm{p}\left(0.42,0.48\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{ccccc}\mathrm{p}\left(0.68,0.38\right)& \mathrm{p}\left(0.66,0.46\right)& \mathrm{p}\left(0.70,0.44\right)& \mathrm{p}\left(0.60,0.48\right)& \mathrm{p}\left(0.48,0.58\right)\end{array})\end{array}\end{array}.$$

Step 3:

Using Equations (7) and (9), the weight vector of indicators affecting the PDS selection is

$$\begin{array}{l}w=(\begin{array}{ccccc}0.0522& 0.0609& 0.1269& 0.1161& 0.0594\end{array}\\ \begin{array}{cc}& \end{array}\begin{array}{ccccc}0.1073& 0.1332& 0.1015& 0.1823& 0.0602\end{array})\end{array}.$$

Step 4:

Firstly, the normalized matrix ${\tilde{E}}_{4\times 10}={\left({\tilde{e}}_{ij}\right)}_{4\times 10}$ should be obtained by ${\tilde{e}}_{ij}=e_{ij}=\left(u_{ij},v_{ij}\right)$ for the benefit indicator, and by ${\tilde{e}}_{ij}=e_{ij}^c=\left(v_{ij},u_{ij}\right)$,

$$\begin{array}{l}{\tilde{E}}_{4\times 10}={\left({\mathrm{p}}_{ij}\left({\displaystyle \sum _{l=1}^5{W}_l{u}_{ij}^{\left(l\right)}},{\displaystyle \sum _{l=1}^5{W}_l{v}_{ij}^{\left(l\right)}}\right)\right)}_{4\times 10}\\ \begin{array}{cc}& \end{array}=(\begin{array}{ccccc}\mathrm{p}\left(0.52,0.62\right)& \mathrm{p}\left(0.58,0.56\right)& \mathrm{p}\left(0.60,0.44\right)& \mathrm{p}\left(0.46,0.58\right)& \mathrm{p}\left(0.46,0.64\right)\\ \mathrm{p}\left(0.40,0.72\right)& \mathrm{p}\left(0.38,0.76\right)& \mathrm{p}\left(0.68,0.50\right)& \mathrm{p}\left(0.48,0.62\right)& \mathrm{p}\left(0.46,0.60\right)\\ \mathrm{p}\left(0.60,0.58\right)& \mathrm{p}\left(0.50,0.48\right)& \mathrm{p}\left(0.66,0.44\right)& \mathrm{p}\left(0.42,0.56\right)& \mathrm{p}\left(0.48,0.42\right)\\ \mathrm{p}\left(0.38,0.46\right)& \mathrm{p}\left(0.48,0.60\right)& \mathrm{p}\left(0.62,0.38\right)& \mathrm{p}\left(0.42,0.66\right)& \mathrm{p}\left(0.44,0.56\right)\end{array}\\ \begin{array}{ccc}& & \begin{array}{cc}& \begin{array}{ccccc}\mathrm{p}\left(0.60,0.38\right)& \mathrm{p}\left(0.64,0.50\right)& \mathrm{p}\left(0.58,0.54\right)& \mathrm{p}\left(0.44,0.58\right)& \mathrm{p}\left(0.52,0.54\right)\\ \mathrm{p}\left(0.68,0.38\right)& \mathrm{p}\left(0.62,0.42\right)& \mathrm{p}\left(0.70,0.44\right)& \mathrm{p}\left(0.48,0.60\right)& \mathrm{p}\left(0.36,0.72\right)\\ \mathrm{p}\left(0.64,0.52\right)& \mathrm{p}\left(0.56,0.46\right)& \mathrm{p}\left(0.66,0.50\right)& \mathrm{p}\left(0.40,0.62\right)& \mathrm{p}\left(0.58,0.48\right)\\ \mathrm{p}\left(0.62,0.44\right)& \mathrm{p}\left(0.66,0.46\right)& \mathrm{p}\left(0.64,0.40\right)& \mathrm{p}\left(0.44,0.64\right)& \mathrm{p}\left(0.38,0.62\right)\end{array})\end{array}\end{array}\end{array},$$

Then the comprehensive evaluation values of all alternative PDSs can be obtained, as shown in

Table 1. The comprehensive evaluation values, obtained by taking different settings of the parameter vector $\lambda$.

	$\mathit{\lambda }=\left(1,0,\dots ,0\right)$	$\mathit{\lambda }=\left(1,1,0,\dots ,0\right)$	$\mathit{\lambda }=\left(1/10,\dots ,1/10\right)$
$A_1$	$\langle 0.2072,0.9272\rangle$	$\langle 0.3653,0.5547\rangle$	$\langle 0.1980,0.9361\rangle$
$A_2$	$\langle 0.2399,0.9195\rangle$	$\langle 0.4582,0.5062\rangle$	$\langle 0.2337,0.9244\rangle$
$A_3$	$\langle 0.2061,0.9267\rangle$	$\langle 0.3658,0.5511\rangle$	$\langle 0.1854,0.9373\rangle$
$A_4$	$\langle 0.2202,0.9176\rangle$	$\langle 0.4097,0.4897\rangle$	$\langle 0.2032,0.9241\rangle$

, where different parameter vectors are shown, namely $\lambda =\left(1,0,\dots ,0\right)$, $\lambda =\left(1,1,0,\dots ,0\right)$, and $\lambda =\left(1/10,\dots ,1/10\right)$.

Step 5:

According to Equations (1) and (2), the score functions of the comprehensive evaluation values for all alternative PDSs can be obtained, as shown in

Table 2. The score functions values by taking different parameter vector $\lambda$.

	$\mathbf{S}\left({\mathit{A}}_1\right)$	$\mathbf{S}\left({\mathit{A}}_2\right)$	$\mathbf{S}\left({\mathit{A}}_3\right)$	$\mathbf{S}\left({\mathit{A}}_4\right)$
$\lambda =\left(1,0,0,\dots ,0\right)$	0.0916	0.1061	0.0918	0.1032
$\lambda =\left(1,1,0,\dots ,0\right)$	0.4129	0.4768	0.4150	0.4640
$\lambda =\left(1/10,\dots ,1/10\right)$	0.0815	0.1000	0.0779	0.0937

.

Step 6:

According to the outcome of the comparisons shown in Table 2 and Definition 4, the rank of the four PDSs is: ① when the parameter vector $\lambda =\left(1,0,\dots ,0\right)$, the rank of the four PDSs is: $A_2>A_4>A_3>A_1$; ② when the parameter vector $\lambda =\left(1,1,0,\dots ,0\right)$, the rank of the four PDSs is: $A_2>A_4>A_3>A_1$; ③ when the parameter vector $\lambda =\left(1/10,\dots ,1/10\right)$, the rank of the four PDSs is: $A_2>A_4>A_1>A_3$.

That is, the EPC is the best suitable PDS. The ranking order is slightly different since the parameter vector $\lambda$ has taken different values. However, the best option is constant, regardless of the variation in the parameter vector $\lambda$.

This selection result is made by considering the related indicators. Firstly, the cost (C), schedule (S) and quality (Q) are inter-conditioned and mutually constraining relationships. If the schedule of the project is compressed, the costs will be increased, and the construction quality of project would be affected. Secondly, complexity (Com) and project size (Size) are generally relevant, too. Moreover, the financial guarantee (FG) affects cost (C), schedule (S), and quality (Q). If the owners have abundant experience in project management, then it will have a positive influence on risk management (RM), cost (C), schedule (S), and quality (Q). The proposed decision-making method makes the PDS selection more valid and feasible.

5. Comparative Analysis

In order to state the advantage of the proposed method, this section gives a comparative analysis with the existing method. We employed the aggregation operators such as the PFWA operator [29], the PFWG operator [29], the symmetric Pythagorean fuzzy weighted averaging (SPFWA) operator [34] and the symmetric Pythagorean fuzzy weighted geometric (SPFWG) operator [34], which cannot capture the interrelationship among all indicators. Let ${\alpha }_j=\langle {\mu }_j,v_j\rangle$ $\left(j=1,2,\dots ,n\right)$ be a collection of PFNs; the PFWA, PFWG, SPFWA and SPFWG are defined as follows:

$$\mathrm{PFWA}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)=\left(w_1{\alpha }_1\right)\oplus \left(w_2{\alpha }_2\right)\oplus \cdots \oplus \left(w_n{\alpha }_n\right),$$

$$\mathrm{PFWG}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)={\alpha }_1^{w_1}\otimes {\alpha }_2^{w_2}\otimes \cdots \otimes {\alpha }_n^{w_n},$$

$$\mathrm{SPFWG}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)=\left(w_1\odot {\alpha }_1\right)〚+〛\left(w_2\odot {\alpha }_2\right)〚+〛\cdots 〚+〛\left(w_n\odot {\alpha }_n\right),$$

$$\mathrm{SPFWA}\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n\right)=\left(w_1\cdot {\alpha }_1\right)\dot{+}\left(w_2\cdot {\alpha }_2\right)\dot{+}\cdots \dot{+}\left(w_n\cdot {\alpha }_n\right),$$

where

$${\alpha }_i〚+〛{\alpha }_j=\left(\frac{{\mu }_{{\alpha }_i}{\mu }_{{\alpha }_j}}{{\left[\left(1-{\mu }_{{\alpha }_i}^2\right)\left(1-{\mu }_{{\alpha }_j}^2\right)+{\mu }_{{\alpha }_i}^2{\mu }_{{\alpha }_j}^2\right]}^{1/2}},\frac{v_{{\alpha }_i}{v}_{{\alpha }_j}}{{\left[\left(1-v_{{\alpha }_i}^2\right)\left(1-v_{{\alpha }_j}^2\right)+v_{{\alpha }_i}^2{v}_{{\alpha }_j}^2\right]}^{1/2}}\right),$$

$$\lambda \odot {\alpha }_j=\left(\frac{{\mu }_{{\alpha }_j}^{\lambda }}{{\left[{\left(1-{\mu }_{{\alpha }_j}^2\right)}^{\lambda }+{\mu }_{{\alpha }_j}^{2\lambda }\right]}^{1/2}},\frac{v_{{\alpha }_j}^{\lambda }}{{\left[{\left(1-v_{{\alpha }_j}^2\right)}^{\lambda }+v_{{\alpha }_j}^{2\lambda }\right]}^{1/2}}\right),$$

$${\alpha }_i\dot{+}{\alpha }_j=\left({\left[\frac{1-\left(1-{\mu }_{{\alpha }_i}^2\right)\left(1-{\mu }_{{\alpha }_j}^2\right)}{2-\left(1-{\mu }_{{\alpha }_i}^2\right)\left(1-{\mu }_{{\alpha }_j}^2\right)-{\mu }_{{\alpha }_i}^2{\mu }_{{\alpha }_j}^2}\right]}^{\frac{1}{2}},{\left[\frac{1-\left(1-v_{{\alpha }_i}^2\right)\left(1-v_{{\alpha }_j}^2\right)}{2-\left(1-v_{{\alpha }_i}^2\right)\left(1-v_{{\alpha }_j}^2\right)-v_{{\alpha }_i}^2{v}_{{\alpha }_j}^2}\right]}^{\frac{1}{2}}\right),$$

$$\lambda \cdot {\alpha }_j=\left({\left[\frac{1-{\left(1-{\mu }_{{\alpha }_j}^2\right)}^{\lambda }}{2-{\left(1-{\mu }_{{\alpha }_j}^2\right)}^{\lambda }-{\mu }_{{\alpha }_j}^{2\lambda }}\right]}^{\frac{1}{2}},{\left[\frac{1-{\left(1-v_{{\alpha }_j}^2\right)}^{\lambda }}{2-{\left(1-v_{{\alpha }_j}^2\right)}^{\lambda }-v_{{\alpha }_j}^{2\lambda }}\right]}^{\frac{1}{2}}\right).$$

The comprehensive evaluation values are shown in

Table 3. The comprehensive evaluation values with different operators.

Operator	Alternatives
	${\mathit{A}}_1$	${\mathit{A}}_2$	${\mathit{A}}_3$	${\mathit{A}}_4$
PFWA	$\langle 0.5961,0.4698\rangle$	$\langle 0.6688,0.4319\rangle$	$\langle 0.5934,0.4671\rangle$	$\langle 0.6258,0.4232\rangle$
PFWG	$\langle 0.5941,0.3701\rangle$	$\langle 0.6623,0.3490\rangle$	$\langle 0.5808,0.3674\rangle$	$\langle 0.6206,0.3401\rangle$
SPFWG	$\langle 0.9828,0.4715\rangle$	$\langle 0.9978,0.4329\rangle$	$\langle 0.9748,0.4692\rangle$	$\langle 0.9886,0.4237\rangle$
SPFWA	$\langle 0.6054,0.5650\rangle$	$\langle 0.6964,0.5046\rangle$	$\langle 0.5957,0.5855\rangle$	$\langle 0.6519,0.5002\rangle$

. The ordering of the PDS selection can be obtained according to the comparative rule in Definition 4. The values of all score functions are calculated, as shown in

Table 4. The score functions values of all alternatives with different operators.

Operator	The Score Functions Values				Ordering
	$\mathbf{S}\left({\mathit{A}}_1\right)$	$\mathbf{S}\left({\mathit{A}}_2\right)$	$\mathbf{S}\left({\mathit{A}}_3\right)$	$\mathbf{S}\left({\mathit{A}}_4\right)$
PFWA	0.5673	0.6304	0.5670	0.6063	$A_2>A_4>A_1>A_3$
PFWG	0.6080	0.6584	0.6012	0.6347	$A_2>A_4>A_3>A_1$
SPFWG	0.8718	0.9041	0.8651	0.8989	$A_2>A_4>A_1>A_3$
SPFWA	0.5236	0.6152	0.5061	0.5874	$A_2>A_4>A_1>A_3$

.

Table 4 shows that the EPC is the best PDS using the PFWA, PFWG, SPFWA and SPFWG operators, though their ranking results are slightly different. Recall that for the ranking result of the PFWMM operator used in the proposed method, the best PDS is also EPC. However, the PFWA, PFWG, SPFWG and SPFWA operators cannot consider the interrelationship among all indicators affecting PDS selection. The PFWMM operator used in the proposed method can capture the interrelationship, and the ranking orders change with the parameter in the PFWMM operator using the proposed method, though the optimal PDS is constant. In summary, the proposed method using the PFWMM operator is more accordant with the practical PDS problem.

6. Conclusions

The project delivery system (PDS) has a significant effect on project implementation. Due to the complexity of the objective world and the ambiguity of human thinking in the actual PDS selection process, there are more or fewer relationships among all indicators affecting the PDS selection. It is an enormous challenge for owners to select an appropriate PDS. In recent years, several researchers have proposed aggregation operators with the PFS and applied them to deal with the PDS decision-making problems. Unfortunately, existing research has not considered the relevance among all indicators affecting the PDS selection. However, the relationships between these indicators really exist. If the decision-makers ignore the related-indicators in the planning stage of the construction project, it will cause the selected PDS to be distorted. The PFS and PFWMM show the significant advantages in dealing with uncertainty information and aggregating the evaluation information for related-indicators cases in the decision-making process. Generally, the PFS can handle imprecise and ambiguous information and manage complex uncertainties in applications, and the PFWMM operator aggregates evaluation information of indicators with relevance in the PDS selection process. Motivated by such considerations, the contributions of this paper are as follows: (1) Considering the existence of the relevance among all indicators affecting the selection of a PDS in a complex environment, this study innovatively introduced the PFWMM operator to deal with PDS selection problems. (2) In order to adapt to the related-indicators PDS selection problem, a new method of solving for indicators’ weights was proposed, using the PFWMM operator. (3) A decision-making framework for PDS selection with the related-indicators case was constructed by full use of the advantages of PFSs and PFWMM operators in dealing with decision-making problems. Finally, comparing the proposed method using the PFWMM with the PFWA, PFWG, SPFWA and SPFWG operators, the best PDS is the EPC, from the ranking results of the five methods, though there are some slight differences. Moreover, a practical example of the PDS selection is given and it showed the effectiveness and applicability of the proposed method.

Comparing with the existing methods, the proposed PDS selection method considers the correlation among indicators affecting the PDS selection, which extends the applied scope of the decision making theory. In future research, it is necessary to extend methods and theories to other MCDM problems. Additional operator theories should be developed and extended to other fuzzy sets, such as supplier selection, risk assessment, and environment evaluation problems under interval Pythagorean fuzzy sets or triangle intuitionistic fuzzy sets, etc.