Model Uncertainties for Soil-Structure Interaction in Offshore Wind Turbine Monopile Foundations
Abstract
1. Introduction
2. Wave Loads
2.1. Load Model for Vertical Surface Piercing Circular Cylinders
2.2. Linear and Nonlinear Wave Kinematics
2.3. Load Scenarios
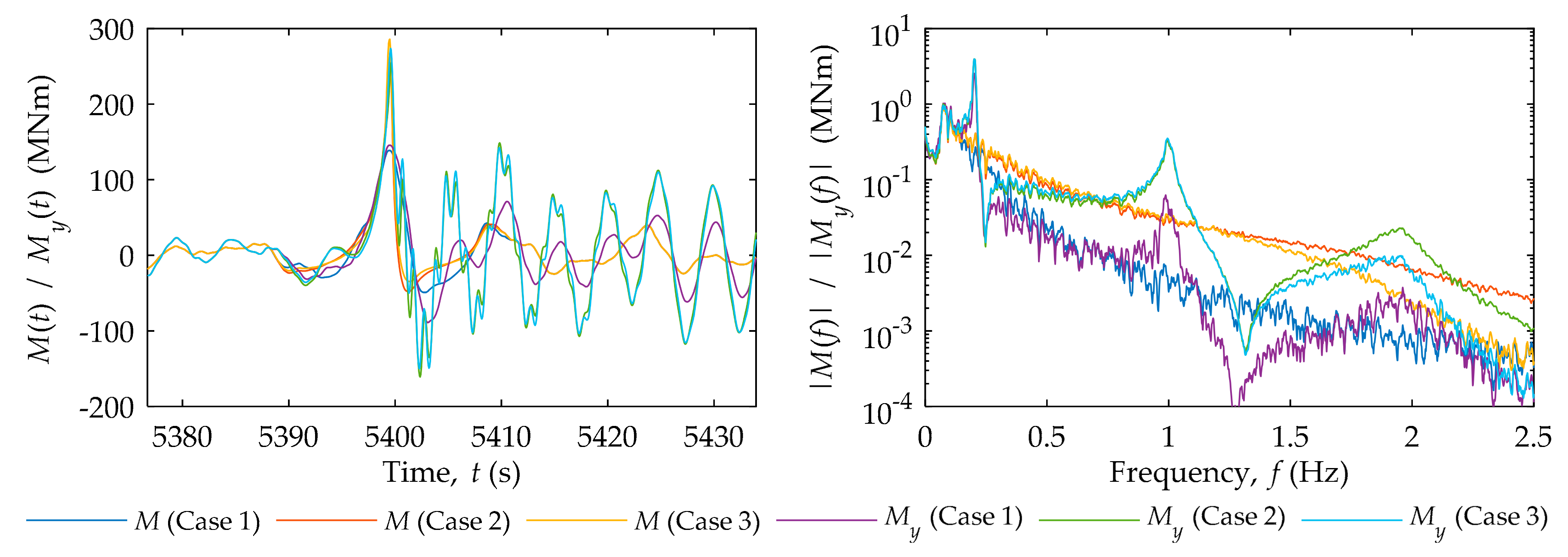
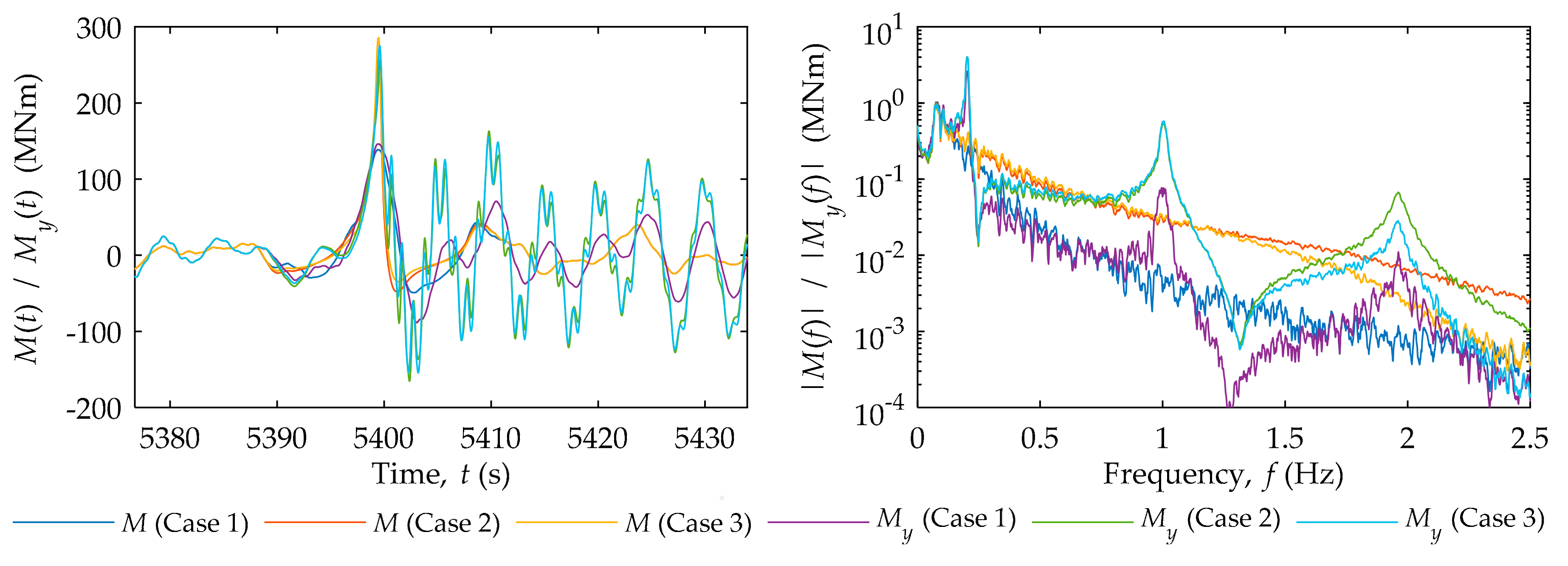
- Load Case 1: Linear irregular waves with significant wave height m, peak wave period s. The duration of the simulated time series is 3 h corresponding to around 1000 waves. Loads are calculated by Morison’s equation without slamming contribution.
- Load Case 2: Same as Load Case 1 but where one wave in the irregular wave train is substituted by a stream function wave with m, s corresponding approximately to the maximum wave in the irregular series (Rayleigh distributed wave heights). This leads to a highly nonlinear wave that is merged into and out of the irregular wave by a cosine taper window. The regular wave is fully active for one wave period and merged in and out in half a period to either side.
- Load Case 3: Same as Load Case 2 but including slamming loads for the stream-function wave.
3. Lumped-Parameter Models for the Foundation
3.1. Semi-Analytical Model of Monopile Response in the Frequency Domain
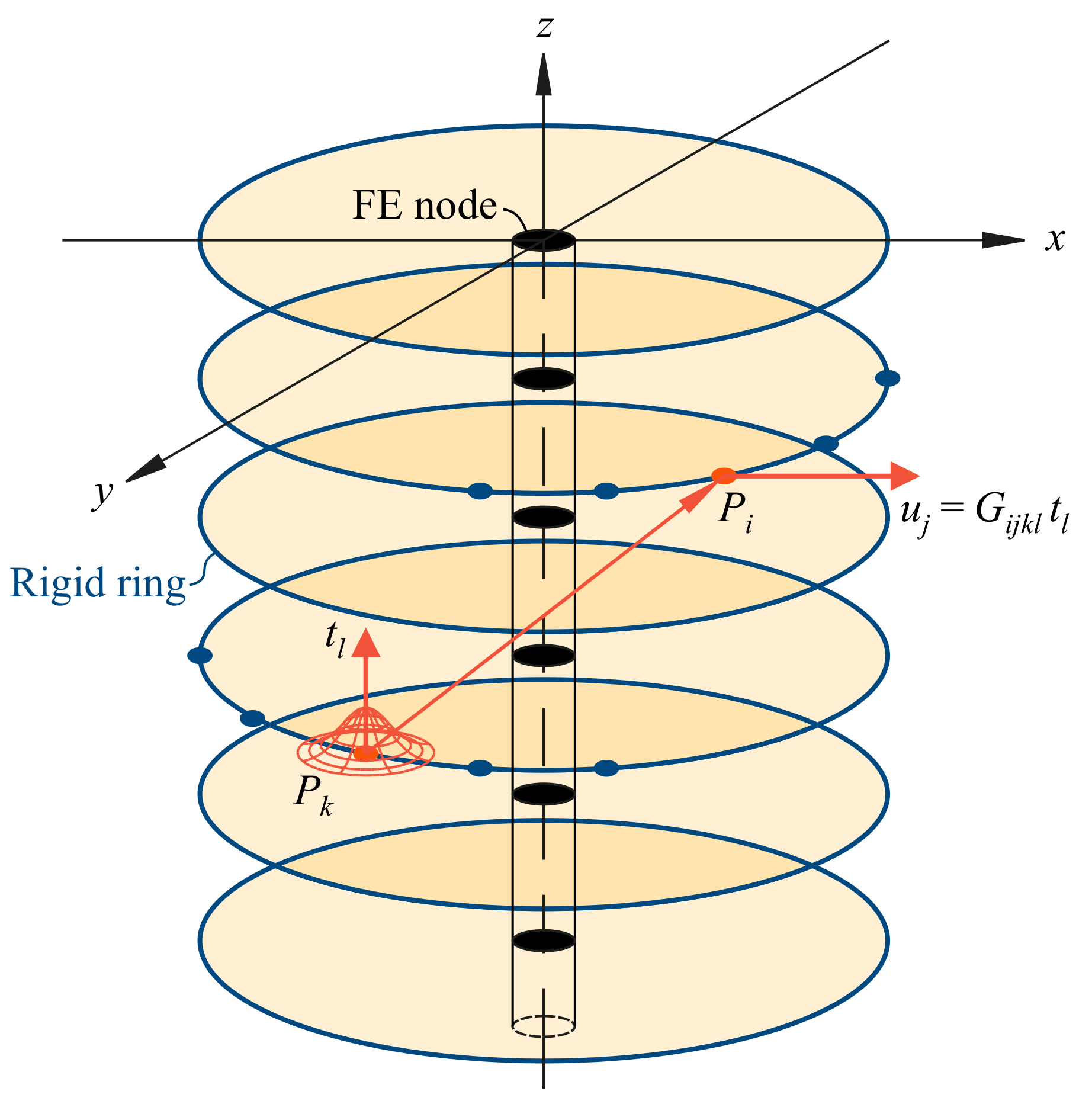
- The mid-surface of the monopile wall is discretized into a total of points, forming a ring with 18 points at the level of each FE node as illustrated in Figure 1. Each point has three translational degrees of freedom.
- Three modes of rigid-body motion (translations, , , and rotation, ) are prescribed for each of the rigid rings, defining the rigid-body motion matrix of dimensions, .
- The displacements at receiver point in direction due to a unit-magnitude load applied at source point in direction are determined for all combinations of source and receiver points and assembled into the Green’s function matrix . It is noted that this matrix is not symmetrical, but that reciprocity of the Green’s function implies that .
- The load magnitudes of the contact forces associated with the rigid-body modes of the rings are determined as . Hence, the dynamic stiffness matrix for the soil interacting with the pile becomes .
- The dynamic stiffness matrix of the pile is found as , where , and are the static stiffness, viscous damping and consistent mass matrices obtained from the FE model of the pile using two-node beam elements with Hermitian interpolation. Then, provides the dynamic stiffness of the pile–soil system.
- A matrix, , is constructed with dimensions, . A identity matrix is put into the submatrix associated with the three degrees of freedom (d.o.f.) at the pile cap, and the remaining entries are set to zero. Afterwards, the matrix is taken as the submatrix of associated with the three d.o.f. of the pile cap. Hence, provides a dynamic stiffness matrix for the pile cap at the angular frequency .
3.2. Consistent Lumped-Parameter Models of the Monopile Cap Response
3.3. Properties of the Model for the Structure, Foundation and Subsoil
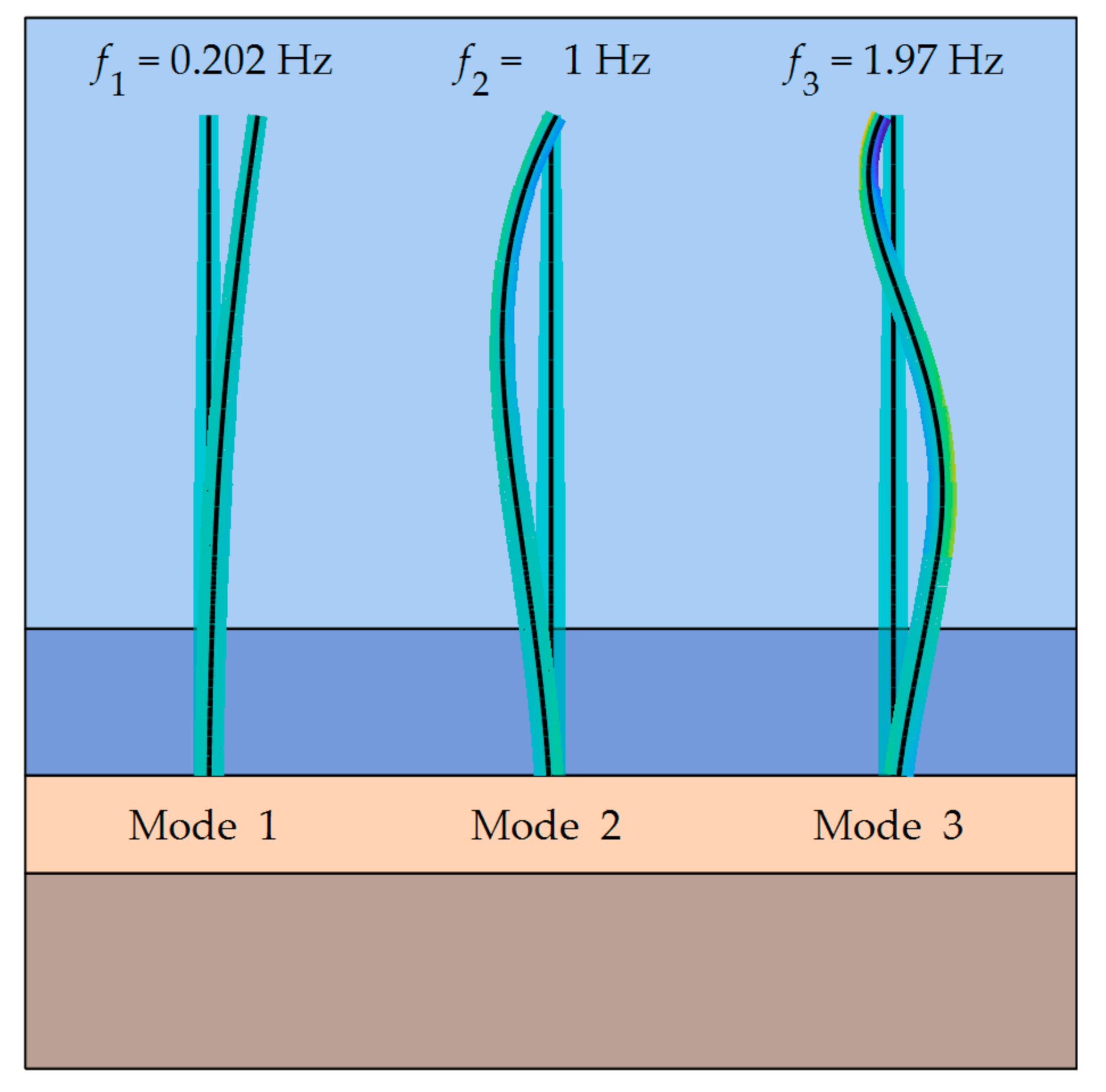
3.4. Calibration and Test of Lumped-Parameter Model
4. Influence of Model Simplifications
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Damgaard, M.; Zania, V.; Andersen, L.V.; Ibsen, L.B. Effects of soil-structure interaction on real time dynamic response of offshore wind turbines on monopiles. Eng. Struct. 2014, 75, 388–401. [Google Scholar] [CrossRef]
- Byrne, B.; Mcadam, R.; Burd, H.; Houlsby, G.; Martin, C.; Beuckelaers, W.; Zdravkovic, L.; Taborda, D.M.G.; Potts, D.; Jardine, R.; et al. PISA: New Design Methods for Offshore Wind Turbine Monopiles. In Proceedings of the Royal Geographical Society 8th International Conference (OSIG 2017), London, UK, 12–14 September 2017; Society for Underwater Technology: London, UK, 2017; pp. 142–161. [Google Scholar]
- Thieken, K.; Achmus, M.; Lemke, K. A new static p-y approach for piles with arbitrary dimensions in sand. Geotechnik 2015, 38, 267–288. [Google Scholar] [CrossRef]
- Vahdatirad, M.J.; Bayat, M.; Andersen, L.V.; Ibsen, L.B. Probabilistic finite element stiffness of a laterally loaded monopile based on an improved asymptotic sampling method. J. Civ. Eng. Manag. 2015, 21, 503–513. [Google Scholar] [CrossRef]
- Andersen, L.V.; Vahdatirad, M.J.; Sichani, M.T.; Sørensen, J.D. Natural frequencies of wind turbines on monopile foundations in clayey soils-A probabilistic approach. Comput. Geotech. 2012, 43, 1–11. [Google Scholar] [CrossRef]
- Versteijlen, W.G.; Metrikine, A.V.; van Dalen, K.N. A method for identification of an effective Winkler foundation for large-diameter offshore wind turbine support structures based on in-situ measured small-strain soil response and 3D modelling. Eng. Struct. 2016, 124, 221–236. [Google Scholar] [CrossRef]
- Amar Bouzid, D.; Bhattacharya, S.; Otsmane, L. Assessment of natural frequency of installed offshore wind turbines using nonlinear finite element model considering soil-monopile interaction. J. Rock Mech. Geotech. Eng. 2018, 10, 333–346. [Google Scholar] [CrossRef]
- Achmus, M.; Abdel-Rahman, K.; Kuo, Y. Numerical modelling of large diameter steel piles under monotonic and cyclic horizontal loading. In Numerical Models in Geomechanics; Taylor & Francis: London, UK, 2007. [Google Scholar] [CrossRef]
- Wolf, J.; Meek, J.; Sung, C. Cone models for a pile foundation. In Piles under Dynamic Loads; Geotechnical Special Publications; ASCE: New York, NY, USA, 1992; Volume 34, pp. 94–113. [Google Scholar]
- Wolf, J.P. Consistent lumped-parameter models for unbounded soil: Frequency-independent stiffness, damping and mass matrices. Earthq. Eng. Struct. Dyn. 1991, 20, 33–41. [Google Scholar] [CrossRef]
- Wolf, J.P. Consistent lumped-parameter models for unbounded soil: Physical representation. Earthq. Eng. Struct. Dyn. 1991, 20, 11–32. [Google Scholar] [CrossRef]
- Cook, R.D.; Malkus, D.S.; Plesha, M.E.; Witt, R.J. Concepts and Applications of Finite Element Analysis; John Wiley & Sons, Inc.: New York, NY, USA, 2002. [Google Scholar]
- Morison, J.R.; Johnson, J.W.; Schaaf, S.A. The Force Exerted by Surface Waves on Piles. J. Pet. Technol. 1950, 2, 149–154. [Google Scholar] [CrossRef]
- Det Norske Veritas. DNV-RP-J101: Design of Offshore Wind Turbine Structures; Det Norske Veritas: Hovik, Norway, 2014. [Google Scholar]
- Wheeler, J.D. Method for Calculating Forces Produced by Irregular Waves. J. Pet. Technol. 1970, 22, 359–367. [Google Scholar] [CrossRef]
- Rienecker, M.M.; Fenton, J.D. A Fourier approximation method for steady water waves. J. Fluid Mech. 1981, 104, 119. [Google Scholar] [CrossRef]
- Rendon, E.A.; Manuel, L. Long-term loads for a monopile-supported offshore wind turbine. Wind Energy 2014, 17, 209–223. [Google Scholar] [CrossRef]
- Senanayake, A.; Manuel, L.; Gilbert, R.B. Estimating natural frequencies of monopile supported offshore wind turbines using alternative P-Y models. In Proceedings of the Annual Offshore Technology Conference, Houston, TX, USA, 1–4 May 2017; Volume 6. [Google Scholar]
- Andersen, L.; Clausen, J. Impedance of surface footings on layered ground. Comput. Struct. 2008, 86, 72–87. [Google Scholar] [CrossRef]
- Liingaard, M.; Andersen, L.; Ibsen, L.B. Impedance of flexible suction caissons. Earthq. Eng. Struct. Dyn. 2007, 36, 2249–2271. [Google Scholar] [CrossRef]
- Andersen, L.V. Influence of dynamic soil-structure interaction on building response to ground vibration. In Numerical Methods in Geotechnical Engineering: Proceedings of the 8th European Conference on Numerical Methods in Geotechnical Engineering NUMGE, Delft, The Netherlands, 18–20 June 2014; CRC Press: Boca Raton, FL, USA, 2014; Volume 2, pp. 1087–1092. [Google Scholar]
- Andersen, L. Assessment of lumped-parameter models for rigid footings. Comput. Struct. 2010, 88, 1333–1347. [Google Scholar] [CrossRef]
- Damgaard, M.; Andersen, L.V.; Ibsen, L.B. Computationally efficient modelling of dynamic soil-structure interaction of offshore wind turbines on gravity footings. Renew. Energy 2014, 68, 289–303. [Google Scholar] [CrossRef]

| Segment | Length (m) | Radius (mm) | Wall Thickness (mm) | Mesh Size (m) | ||
|---|---|---|---|---|---|---|
| Tower Seg. 1 | 15.0 | 2000 | 24 | 350,000 | 75,000,000 | 5.0 |
| Tower Seg. 2 | 15.0 | 2200 | 24 | 5.0 | ||
| Tower Seg. 3 | 15.0 | 2400 | 24 | 5000 | 10,000 | 5.0 |
| Tower Seg. 4 | 15.0 | 2600 | 24 | 5.0 | ||
| Tower Seg. 5 | 15.0 | 2800 | 24 | 5000 | 10,000 | 5.0 |
| Tower Seg. 6 | 15.0 | 3000 | 24 | 5.0 | ||
| Supp. Struct. Seg. 1 | 15.0 | 3000 | 80 | 5000 | 10,000 | 1.0 |
| Supp. Struct. Seg. 2 | 30.0 | 3000 | 80 | 5000 | 10,000 | 2.0 |
| Emb. Pile Seg. 1 | 40.0 | 3000 | 80 | 1.0 |
| Layer | Depth (m) | Shear Modulus (MPa) | Poisson’s Ratio (−) | Mass Density (kg/m3) | Loss Factor (−) | Shear Wave Speed (m/s) |
|---|---|---|---|---|---|---|
| Layer 1: Soft soil | 20.00 | 20.00 | 0.450 | 2000 | 0.050 | 100.0 |
| Layer 2: Hard soil | ∞ | 80.00 | 0.450 | 2000 | 0.030 | 200.0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andersen, L.V.; Andersen, T.L.; Manuel, L. Model Uncertainties for Soil-Structure Interaction in Offshore Wind Turbine Monopile Foundations. J. Mar. Sci. Eng. 2018, 6, 87. https://doi.org/10.3390/jmse6030087
Andersen LV, Andersen TL, Manuel L. Model Uncertainties for Soil-Structure Interaction in Offshore Wind Turbine Monopile Foundations. Journal of Marine Science and Engineering. 2018; 6(3):87. https://doi.org/10.3390/jmse6030087
Chicago/Turabian StyleAndersen, Lars Vabbersgaard, Thomas Lykke Andersen, and Lance Manuel. 2018. "Model Uncertainties for Soil-Structure Interaction in Offshore Wind Turbine Monopile Foundations" Journal of Marine Science and Engineering 6, no. 3: 87. https://doi.org/10.3390/jmse6030087
APA StyleAndersen, L. V., Andersen, T. L., & Manuel, L. (2018). Model Uncertainties for Soil-Structure Interaction in Offshore Wind Turbine Monopile Foundations. Journal of Marine Science and Engineering, 6(3), 87. https://doi.org/10.3390/jmse6030087







