Influence of Load Variation on the Flow Field and Stability of the Francis Turbine
Abstract
:1. Introduction
2. Mathematical Model
2.1. Fundamental Governing Equation
2.2. Turbulence Model
3. Construction of Geometric Model and Division of Mesh
3.1. Three-Dimensional Geometrical Model
3.2. Grid Division and Independence Verification
3.3. Boundary Condition
4. Analysis of Calculation Results
4.1. Internal Flow Field Analysis
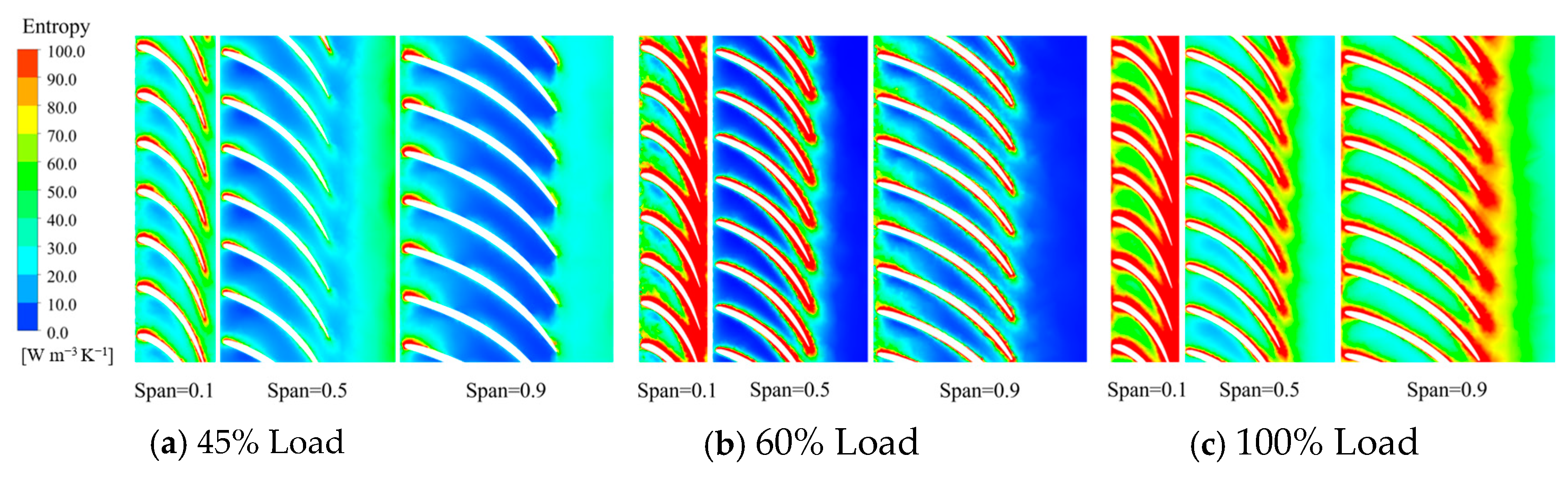
4.1.1. Vaneless Space
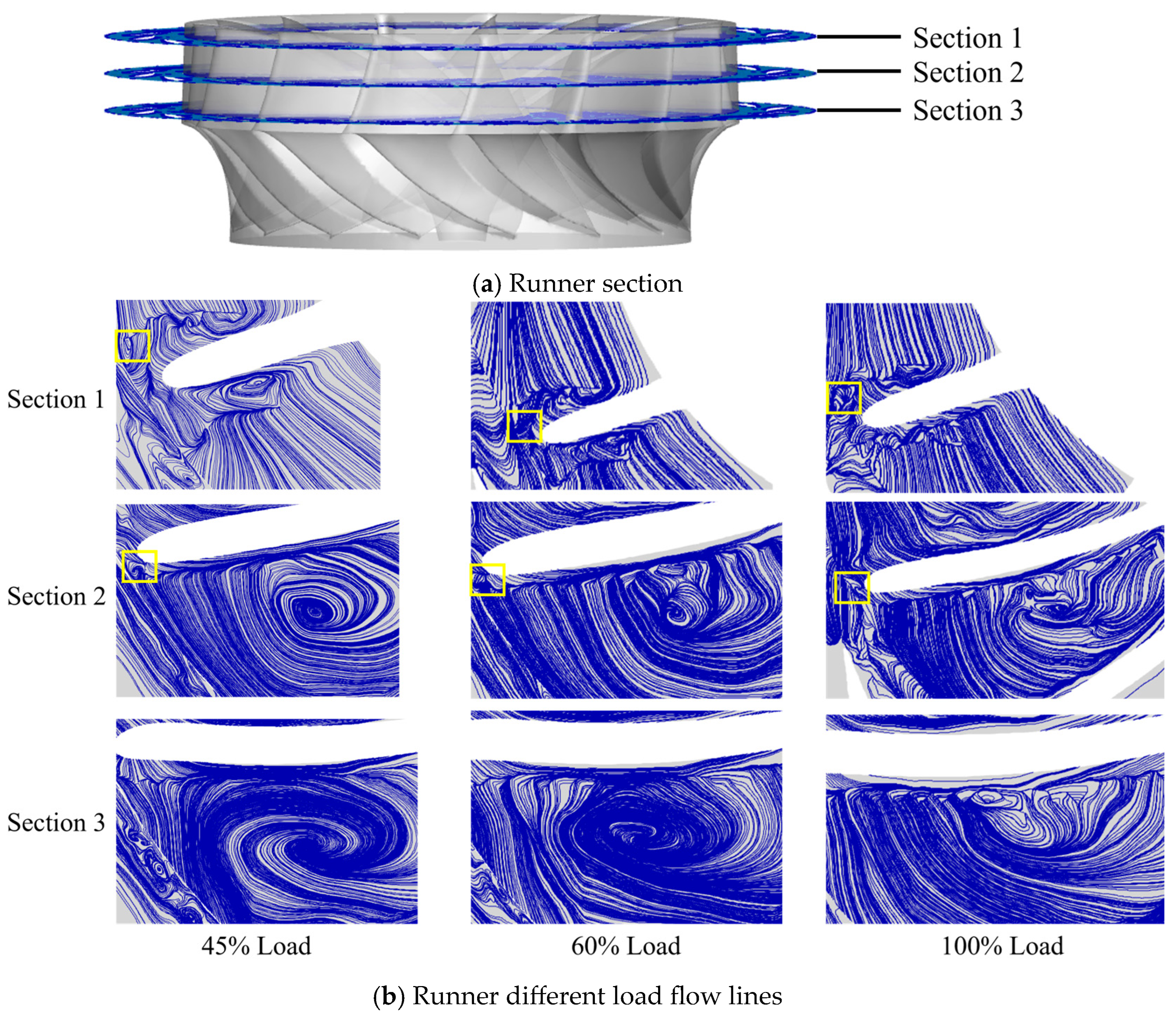
4.1.2. Runner
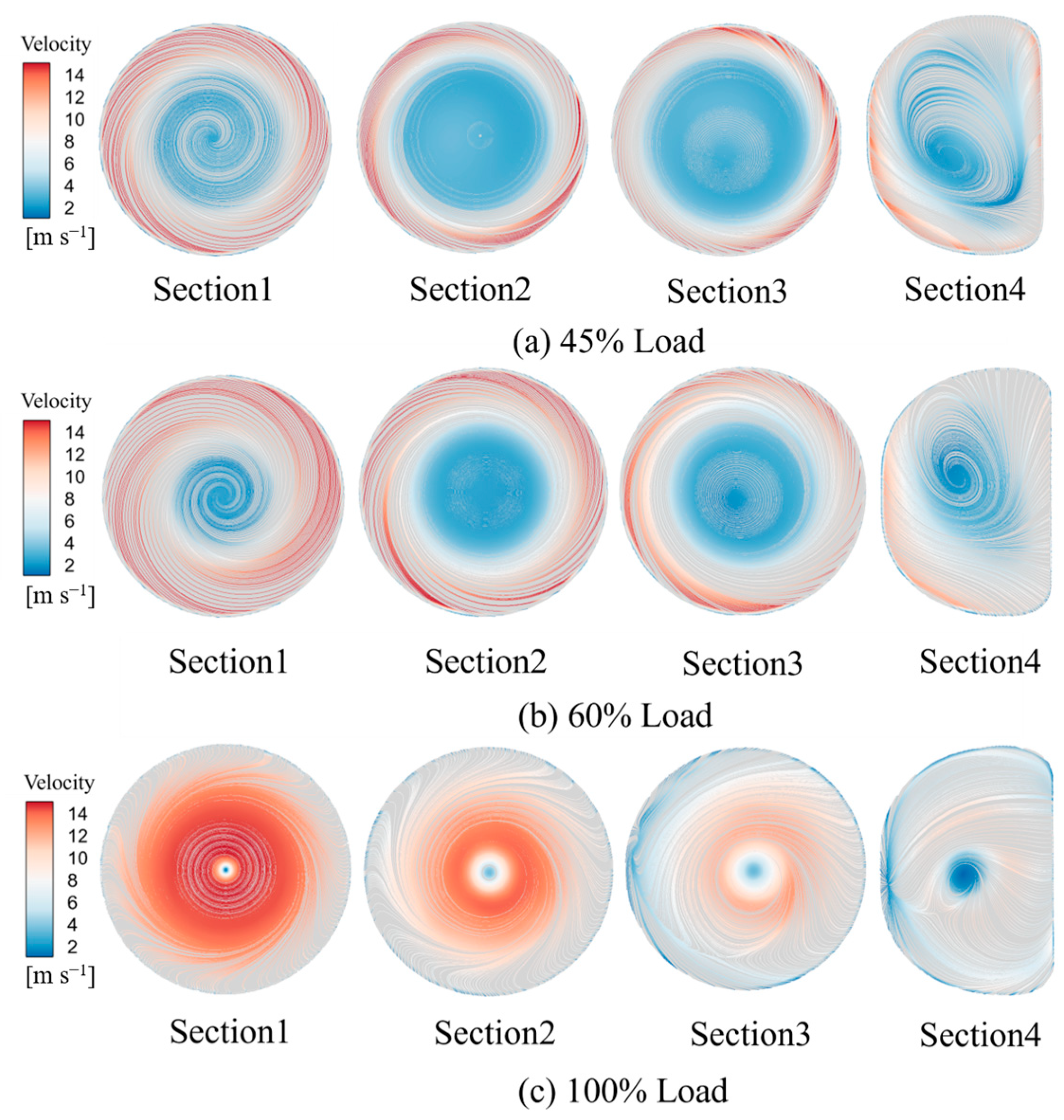
4.1.3. Draft Tube
4.2. Pressure Pulsation Analysis
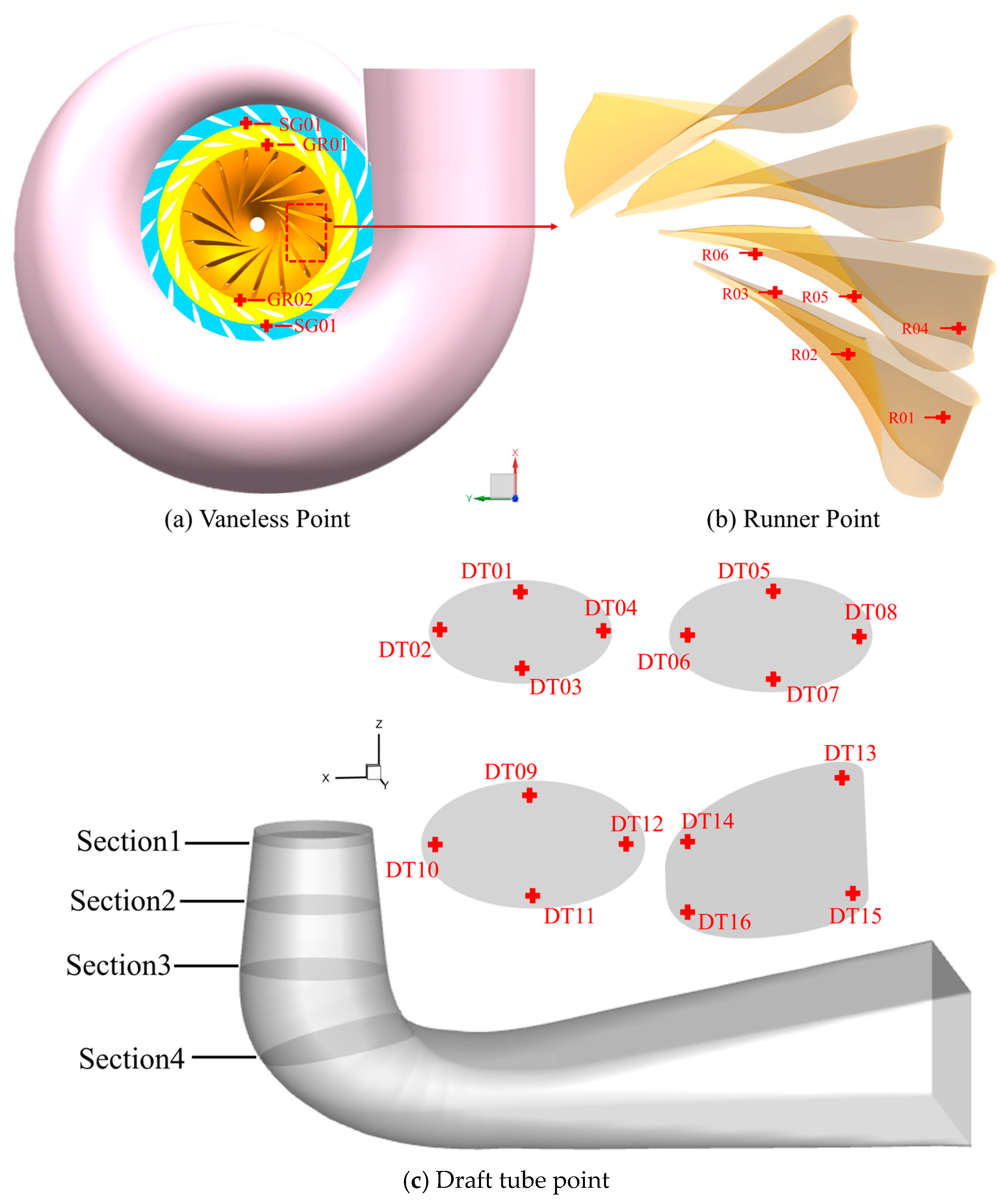
4.2.1. Monitoring Point Setting
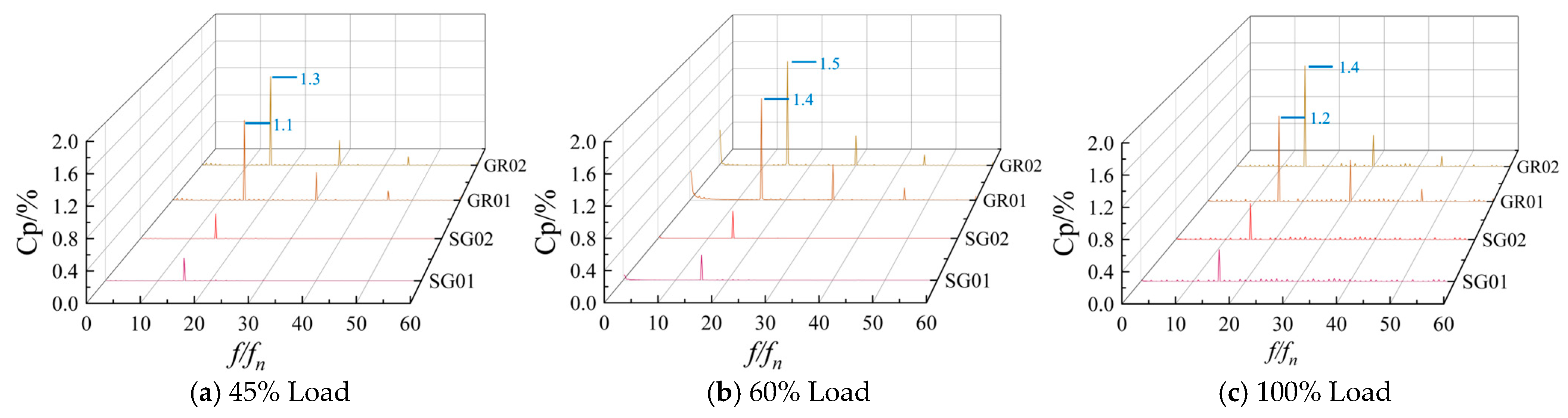
4.2.2. Vaneless Space
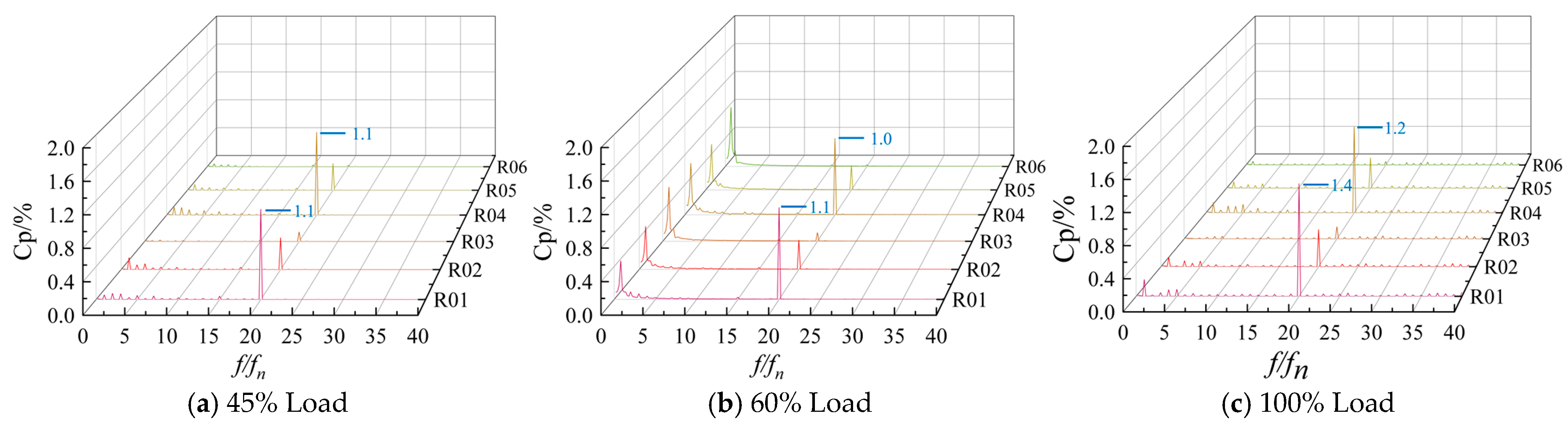
4.2.3. Runner
4.2.4. Draft Tube
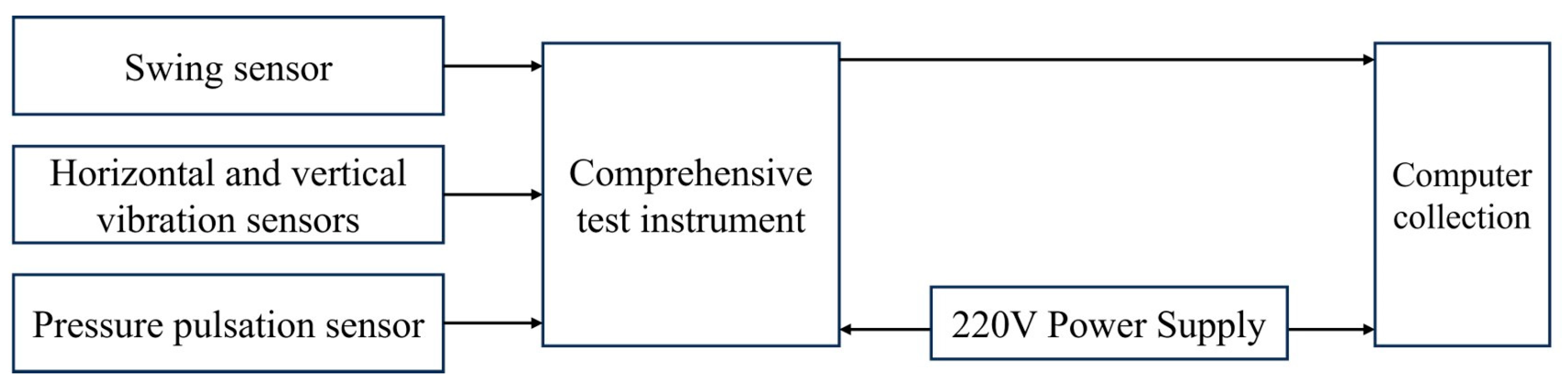
5. Analysis of the Test Results
5.1. Pressure Pulsation Within the Vaneless Region
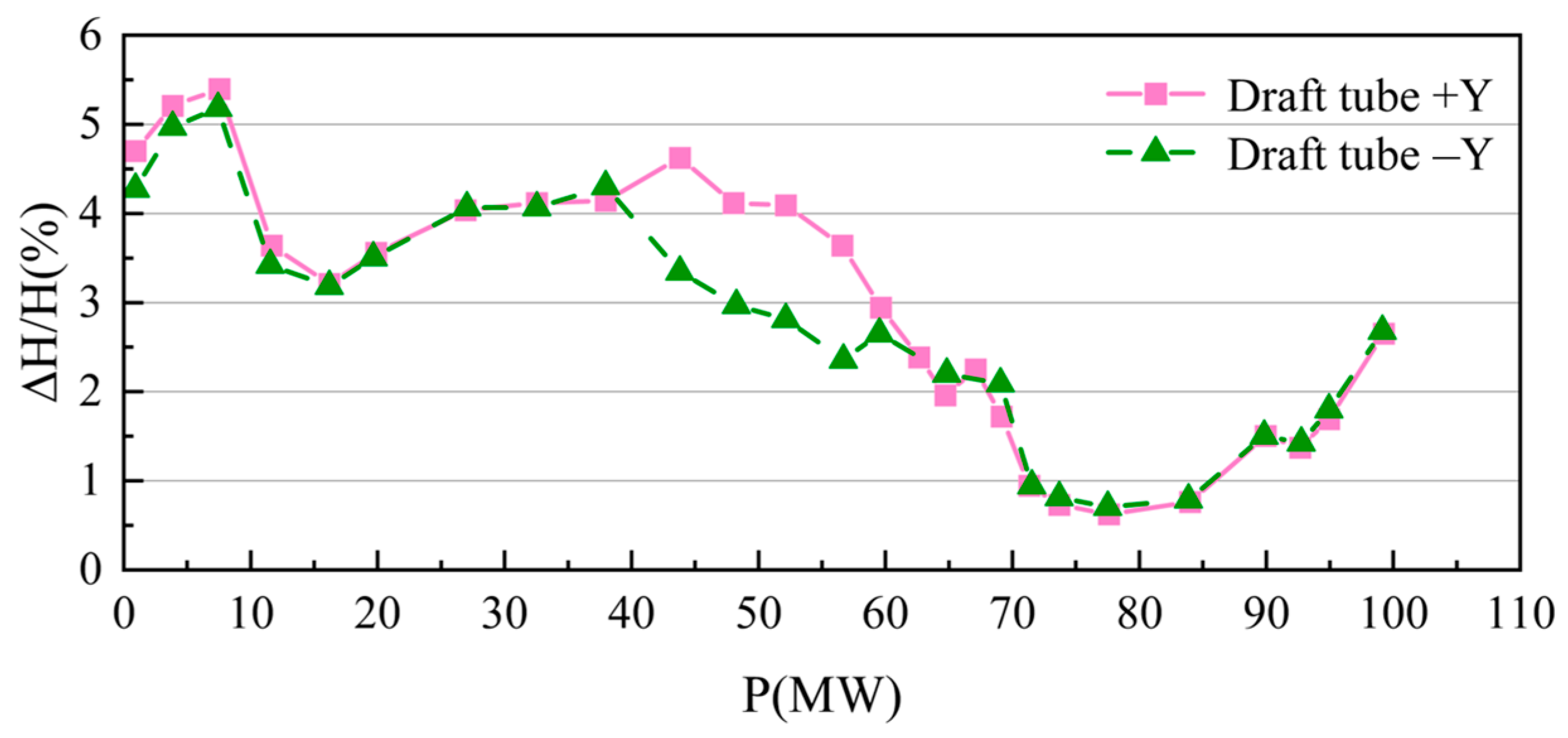
5.2. Pressure Pulsation of the Draft Tube
6. Conclusions
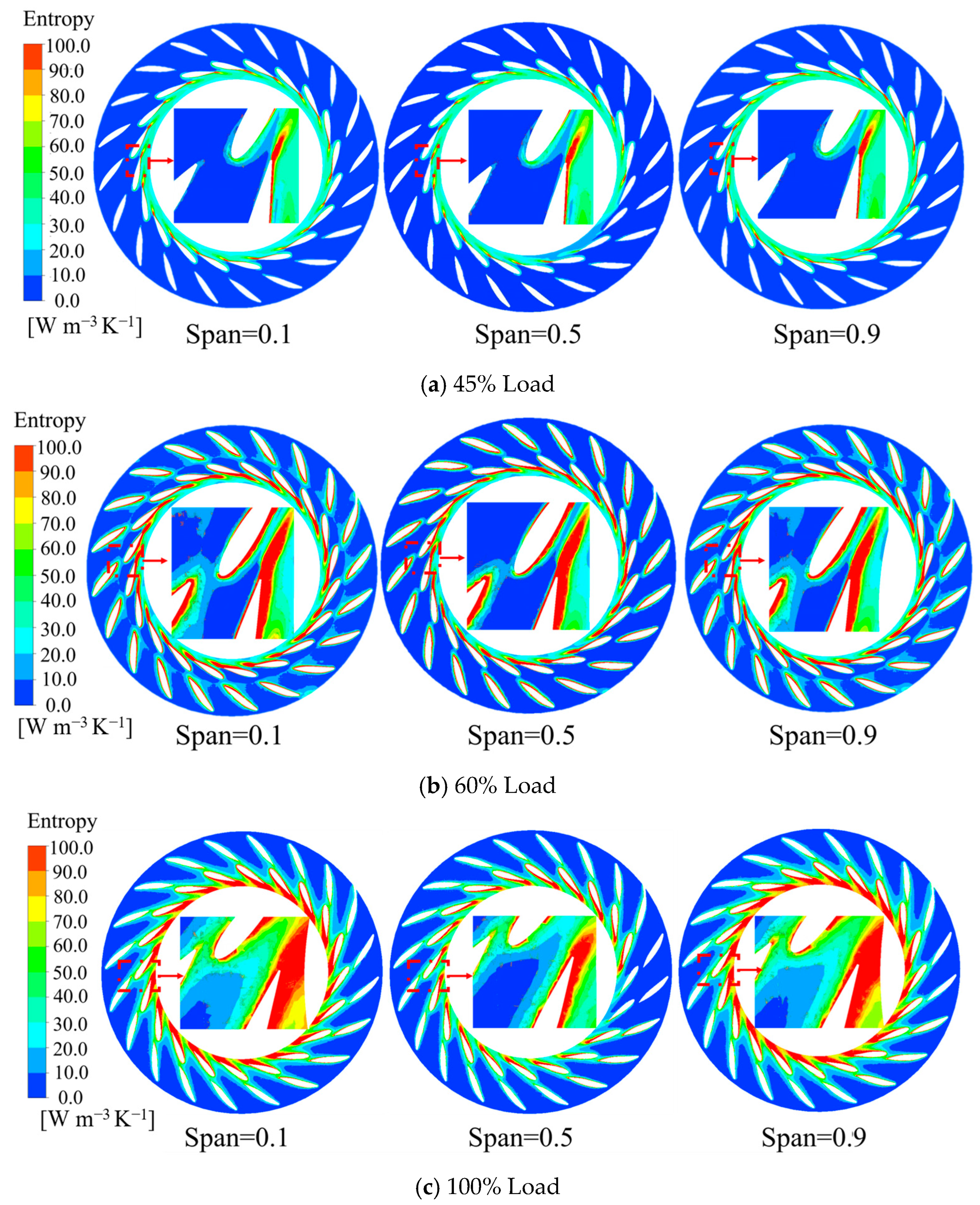
- The draft tube accounts for the majority of the turbine’s energy dissipation, ranging from about 72.7% to 95.9%. The peak dissipation occurs at the 45% load condition. The energy loss between the vaneless region and the runner is mainly due to backflow and eddy currents caused by flow separation. At full load (100%), the energy loss in the vaneless region and the runner exceeds that observed at 45% and 60% loads.
- The pressure fluctuations caused by the vortex belt are the primary contributors to the unstable operation of the unit. At 45% and 100% load conditions, the draft tube is mainly affected by dynamic and static interference, rotor-generated single and double frequencies, and low-frequency vortex belt oscillations. Under 60% load, the pressure pulsation in the draft tube is predominantly driven by the vortex band at a frequency of 0.2 fn, with its amplitude notably higher than that observed at 45% and 100% load conditions. Measures can be taken to reduce or eliminate hydraulic losses, such as introducing air into the draft tube, modifying the water flow within it, or controlling the eccentricity of the vortex belt.
- The relative amplitude of pressure pulsation in the draft tube ranged from 0.5% to 5.5%, markedly higher than that observed in the vaneless region. Additionally, the amplitude was more pronounced under partial load conditions, consistent with the findings from numerical simulations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| SST | Shear Stress Transport |
| CFD | Computational Fluid Dynamics |
| DES | Detached-Eddy Simulation |
| BEP | Best Efficiency Point |
| SG | Stay vane to Guide vane |
| GR | Guide vane to Runner |
| R | Runner |
| DT | Draft Tube |
References
- Chen, H.; Lu, Y.; Liu, K.; Zhang, Z.; Li, H.; Huang, X.; Zhao, W.; Wang, Z. Study on the Internal Flow Characteristics of Long and Short Blade Runners of a 1000 MW Francis Turbine under Different Opening Conditions. Processes 2023, 11, 1796. [Google Scholar] [CrossRef]
- Li, S.; Yao, B.; Pang, J.; Liu, D.; Chengmei, D.; Jiang, D.; Wang, H.; Gang, Y.; Cheng, H.; Liu, X. Research on the technical improvement of the turbine runner of a power station based on improving stability. Energy Sci. Eng. 2024, 12, 4582–4597. [Google Scholar] [CrossRef]
- Pang, J.; Yao, B.; Liu, X.; Li, J.; Xiao, M.; Jiang, D.; Tang, W.; Zhou, L.; Lai, Z. Influence of guide vane opening on channel vortex and pressure pulsation in Francis turbine runners. Phys. Fluids 2024, 36, 043325. [Google Scholar] [CrossRef]
- Pang, J.; Liu, H.; Liu, X.; Yang, H.; Peng, Y.; Zeng, Y.; Yu, Z. Study on sediment erosion of high head Francis turbine runner in Minjiang River basin. Renew. Energy 2022, 192, 849–858. [Google Scholar] [CrossRef]
- Pang, J.; Chang, X.; Gang, Y.; Zhou, Z.; Xiang, W.; Zhou, L.; Liu, X.; Wang, Z. Design and Study of a Sediment Erosion Test Device for a Single-Flow Channel in the Guide Apparatus of a Reaction Hydraulic Turbine. J. Mar. Sci. Eng. 2024, 12, 777. [Google Scholar] [CrossRef]
- Liang, A.; Li, H.; Zhang, W.; Yao, Z.; Zhu, B.; Wang, F. Study on pressure fluctuation and rotating stall characteristics in the vaneless space of a pump-turbine in pump mode. J. Energy Storage 2024, 94, 112385. [Google Scholar] [CrossRef]
- Li, H.; Yang, J.; Zhang, W.; Hu, L.; Liang, A.; Yao, Z. Energy performance and unsteady gas-liquid flow characteristics of a multiphase rotodynamic pump: An experiment. Appl. Energy 2024, 375, 124112. [Google Scholar] [CrossRef]
- Hu, L.; Liang, A.; Li, H.; Zhang, W.; Zhu, B. Impact of rotor–stator axial spacing on the gas–liquid–solid flow characteristics of a multiphase rotodynamic pump based on the Euler multi-fluid model. Phys. Fluids 2024, 36, 063314. [Google Scholar] [CrossRef]
- Wang, H.; Pang, J.; Lai, Z.; Li, S.; Jiang, D.; Wang, X.; Xiang, P.; Yao, B.; Zeng, Y.; Liu, X. Influence of guide vane skirt structure on sediment erosion of high-head turbine in high hardness and sandy river. Phys. Fluids 2024, 36, 103317. [Google Scholar] [CrossRef]
- Wang, H.; Pang, J.; Liu, X.; Zhou, Z.; Gang, Y.; Lai, Z.; Wang, J.; Qin, B. Analysis on cause of erosion of guide vane of high-head Francis turbine in sandy river. Energy Sci. Eng. 2024, 12, 3704–3717. [Google Scholar] [CrossRef]
- Ren, H.; Yu, B.; Wang, K.; Wang, L. Analysis of Flow and Pressure Plusation in Draft Tube of Francis Turbine under Different Guide Vane Opening. Pearl River 2023, 44, 126–133. [Google Scholar]
- Wang, H.; Zheng, Y.; Sun, A.R. The influent of guide vane opening on pressure pulsation characteristics and flow- induced noise in Francis turbine. South-North Water Transf. Water Sci. Technol. 2018, 16, 186–192. [Google Scholar]
- Zuo, Z.; Liu, S.; Liu, D.; Qin, D.Q.; Wu, Y.L. Numerical analyses of pressure fluctuations induced by interblade vortices in a model Francis turbine. J. Hydrodyn. Ser. B 2015, 27, 513–521. [Google Scholar] [CrossRef]
- Trivedi, C.; Gandhi, K.B.; Cervantes, J.M.; Dahlhaug, O.G. Experimental investigations of a model Francis turbine during shutdown at synchronous speed. Renew. Energy 2015, 83, 828–836. [Google Scholar] [CrossRef]
- Hu, D.; Cheng, Y.; Zhang, P.; Wang, X.; Ding, J.; Zhang, X. Distribution features of flow patterns and pressure pulsations of pump-turbine in five operating modes on the four-quadrant plane. Front. Energy Res. 2022, 10, 880293. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, L.; Chen, J.; Zhang, Z.; Jia, K.; Feng, K.; Wang, X. Influence of rotational speed on performance of low specific speed hydraulic turbine in turbine mode. Adv. Mech. Eng. 2022, 14, 16878132221100652. [Google Scholar] [CrossRef]
- Minakov, A.; Platonov, D.; Dekterev, A.; Sentyabov, A.V.; Zakharov, A.V. The numerical simulation of low frequency pressure pulsations in the high-head Francis turbine. Comput. Fluids 2015, 111, 197–205. [Google Scholar] [CrossRef]
- Jamali, R.; Sohani, A.; Hemmatpour, K.; Behrang, M.; Ghobeity, A. Experimental study of pressure pulsation in a large-scale hydropower plant with Francis turbine units and a common penstock. Energy Convers. Manag. X 2022, 16, 100308. [Google Scholar] [CrossRef]
- Unterluggauer, J.; Sulzgruber, V.; Doujak, E.; Bauer, C. Experimental and numerical study of a prototype Francis turbine startup. Renew. Energy 2020, 157, 1212–1221. [Google Scholar] [CrossRef]
- Kumar, S.; Cervantes, M.J.; Gandhi, B.K. Rotating vortex rope formation and mitigation in draft tube of hydro turbines–A review from experimental perspective. Renew. Sustain. Energy Rev. 2021, 136, 110354. [Google Scholar] [CrossRef]
- Joy, J.; Raisee, M.; Cervantes, M.J. Experimental investigation of an adjustable guide vane system in a Francis turbine draft tube at part load operation. Renew. Energy 2023, 210, 737–750. [Google Scholar] [CrossRef]
- Wang, W.; Chen, Q.; Yan, D.; Geng, D. A novel comprehensive evaluation method of the draft tube pressure pulsation of Francis turbine based on EEMD and information entropy. Mech. Syst. Signal Process. 2019, 116, 772–786. [Google Scholar] [CrossRef]
- Yamamoto, K.; Müller, A.; Favrel, A.; Avellan, F. Physical mechanism of interblade vortex development at deep part load operation of a Francis turbine. J. Fluids Eng. 2019, 141, 111113. [Google Scholar] [CrossRef]
- Wang, F.; Liao, C.; Tang, X. Numerical simulation of pressure fluctuations in a large Francis turbine runner. Chin. J. Mech. Eng. 2012, 25, 1198–1204. [Google Scholar] [CrossRef]
- Platonov, V.D.; Maslennikova, V.A.; Dekterev, A.D.; Minakov, A.V.; Abramov, A.V. Experimental study of pressure pulsations in the flow duct of a medium-size model hydroelectric generator with Francis turbine. Thermophys. Aeromechanics 2018, 25, 21–30. [Google Scholar] [CrossRef]
- Schmandt, B.; Herwig, H. Internal flow losses: A fresh look at old concepts. Fluids Eng.-Trans. ASME 2011, 133, 051201. [Google Scholar] [CrossRef]
- Yu, Z.-F.; Yan, Y.; Wang, W.-Q.; Liu, X.-S. Entropy production analysis for vortex rope of a Francis turbine using hybrid RANS/LES method. Int. Commun. Heat Mass Transf. 2021, 127, 105494. [Google Scholar] [CrossRef]
- Yu, Z.-F.; Wang, W.-Q.; Yan, Y.; Liu, X.-S. Energy loss evaluation in a Francis turbine under overall operating conditions using entropy production method. Renew. Energy 2021, 169, 982–999. [Google Scholar] [CrossRef]
- Yu, Z.-F.; Wang, W.-Q.; Yan, Y.; Wang, H.-Y.; Wu, W.-L. Evaluating energy-efficiency improvement of variable-speed operation with the help of entropy: A case study of low-head Francis turbine. Sustain. Energy Technol. Assess. 2022, 53, 102468. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, L.; Yin, G.; Guan, C. Research on Unsteady Hydraulic Features of a Francis Turbine and a Novel Method for Identifying Pressure Pulsation Transmission Path. Water 2019, 11, 1216. [Google Scholar] [CrossRef]
- Zhou, X.; Wu, H.; Shi, C. Numerical and experimental investigation of the effect of baffles on flow instabilities in a Francis turbine draft tube under partial load conditions. Adv. Mech. Eng. 2019, 11, 1687814018824468. [Google Scholar] [CrossRef]
- Luo, Z.; Nie, C.; Lv, S.; Guo, T.; Gao, S. The effect of J-groove on vortex suppression and energy dissipation in a draft tube of Francis turbine. Energies 2022, 15, 1707. [Google Scholar] [CrossRef]
- Trivedi, C.; Cervantes, J.M.; Gandhi, K.B. Investigation of a High Head Francis Turbine at Runaway Operating Conditions. Energies 2016, 9, 149. [Google Scholar] [CrossRef]
- Liu, D.; Li, Z.; Xu, L.; Li, J.; Yang, Y.; Wang, X.; Pang, J.; Liu, X. Vortex motion in vaneless space and runner passage of pump-turbine in S-shaped region. Phys. Fluids 2024, 36, 025115. [Google Scholar] [CrossRef]
- Xu, L.; Kan, K.; Zheng, Y.; Liu, D.; Binama, M.; Xu, Z.; Yan, X.; Guo, M.; Chen, H. Rotating stall mechanism of pump-turbine in hump region: An insight into vortex evolution. Energy 2024, 292, 130579. [Google Scholar] [CrossRef]
- Zhang, D.; Wu, S.; Shi, W.; Pan, D.; Yao, J.; Zhang, G. Application and experiment of different turbulence models for simulating tip leakage vortex in axial flow pump. Editor. Off. Trans. Chin. Soc. Agric. Eng. 2013, 29, 46–53. [Google Scholar]
- Ren, Y.; Liu, H.; Shu, M.; Wu, X. Analysis of applicability of turbulence models in performance prediction for centrifugal pumps at the off-design conditions. Fluid Mach. 2012, 40, 18–22. [Google Scholar]
- Zhang, F.; Appiah, D.; Hong, F.; Zhang, J.; Yuan, S.; Adu-Poku, K.A.; Wei, X. Energy loss evaluation in a side channel pump under different wrapping angles using entropy production method. Int. Commun. Heat Mass Transf. 2020, 113, 104526. [Google Scholar] [CrossRef]
- Zhang, Z.; Lu, Y.; Zhu, R.; Wang, Z.; Li, X. Analysis of hydraulic loss and entropy production characteristics of 1000 MW Francis turbines under multi-operating condition. J. Drain. Irrig. Mach. Eng. (JDIME) 2024, 42, 1142–1149. (In Chinese) [Google Scholar]
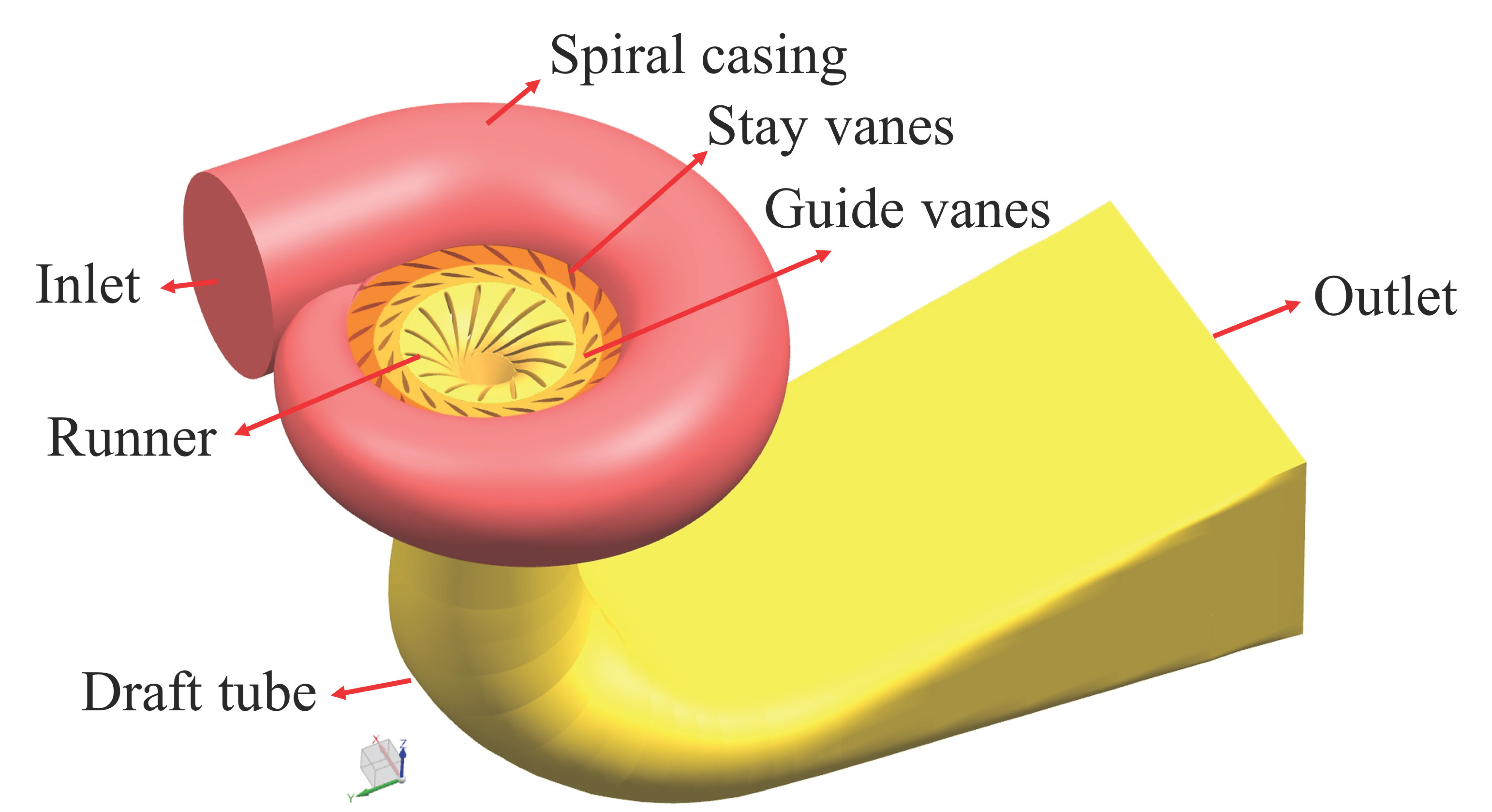

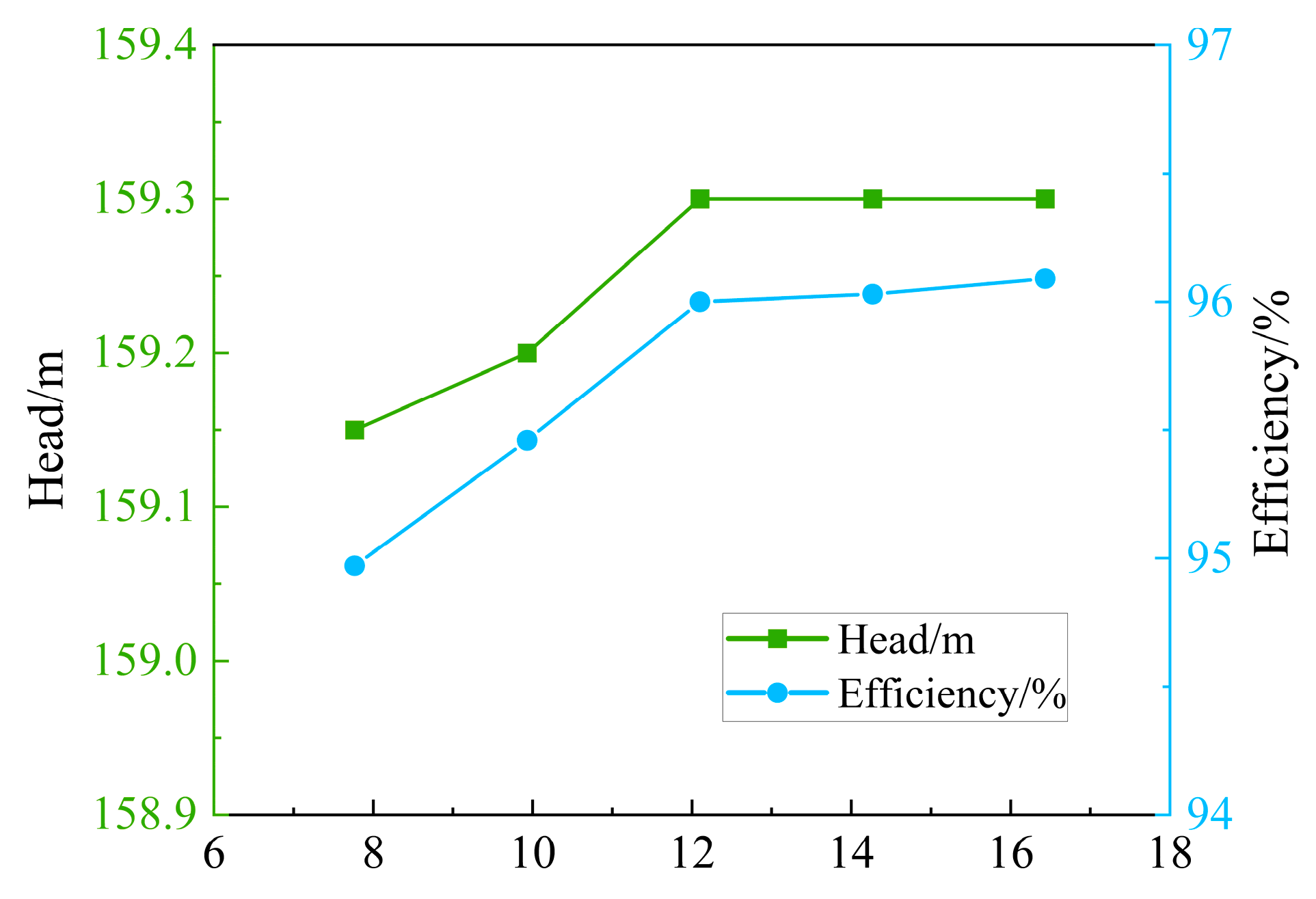
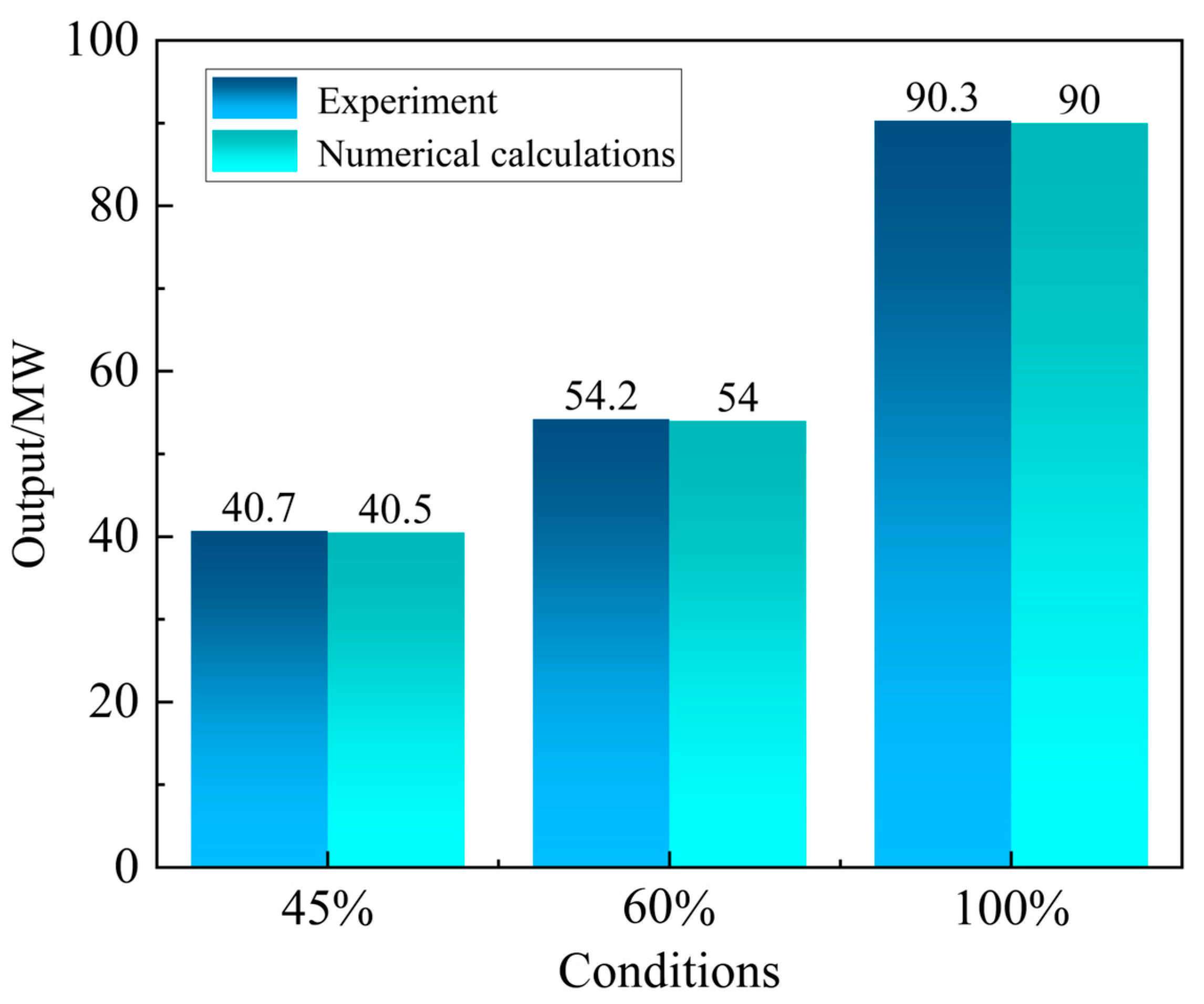





| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Rated Head Hr (m) | 159.3 | Number of runner blades | 15 |
| Rated output P (MW) | 90 | Number of stay vanes | 20 |
| Rated Speed nr (r/min) | 272.7 | Number of guide vanes | 20 |
| Rated Discharge Qr (m3/s) | 62.7 | Runner inlet diameter D1 (mm) | 2975 |
| Suction Height Hs (m) | −3 | Runner outlet diameter D2 (mm) | 2472 |
| 45% | 60% | 100% | |
|---|---|---|---|
| Vaneless | The entropy production at the trailing edge of the guide vane is minimal. | The entropy production around the stay vane is higher than that of the other two operating conditions | The entropy production range of the trailing edge of the guide vane is the largest, and the two ends are higher than the middle |
| Runner | There is almost no high-entropy production area in the tail of the blades | Span = 0.9 The entropy yield range of the blade tail was the largest | |
| Draft tube | High-entropy production areas occur at both ends of the proximal wall | High entropy production areas occur at both ends of the proximal wall, and the range of elbow segments is the largest | The high-entropy production zones are symmetrically distributed about the centerline of the straight conical section. |
| 45% | 60% | 100% | |
|---|---|---|---|
| Vaneless | A low-frequency pressure ripple of 0.2 fn is observed, with the largest amplitude of pressure ripple resulting from dynamic and static interference. | ||
| Runner | A low-frequency pressure pulsation of 0.8 fn occurs | The amplitude of pressure fluctuation caused by dynamic and static interference is the largest | |
| Draft tube | The amplitude of the pressure ripple and the extent of the vortex zone are clearly observable. | The pressure ripples caused by the vortex belt exhibit the largest amplitude. | The maximum value is the amplitude of the pressure ripple due to the dynamic and static interference |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Pang, J.; Dan, C.; Xiang, W.; Yi, X.; Liu, X. Influence of Load Variation on the Flow Field and Stability of the Francis Turbine. J. Mar. Sci. Eng. 2025, 13, 316. https://doi.org/10.3390/jmse13020316
Li S, Pang J, Dan C, Xiang W, Yi X, Liu X. Influence of Load Variation on the Flow Field and Stability of the Francis Turbine. Journal of Marine Science and Engineering. 2025; 13(2):316. https://doi.org/10.3390/jmse13020316
Chicago/Turabian StyleLi, Shenhui, Jiayang Pang, Chengmei Dan, Wenping Xiang, Xutao Yi, and Xiaobing Liu. 2025. "Influence of Load Variation on the Flow Field and Stability of the Francis Turbine" Journal of Marine Science and Engineering 13, no. 2: 316. https://doi.org/10.3390/jmse13020316
APA StyleLi, S., Pang, J., Dan, C., Xiang, W., Yi, X., & Liu, X. (2025). Influence of Load Variation on the Flow Field and Stability of the Francis Turbine. Journal of Marine Science and Engineering, 13(2), 316. https://doi.org/10.3390/jmse13020316






