Experimental and Simulation Studies on Protective Structures in Floating Dock
Abstract
1. Introduction
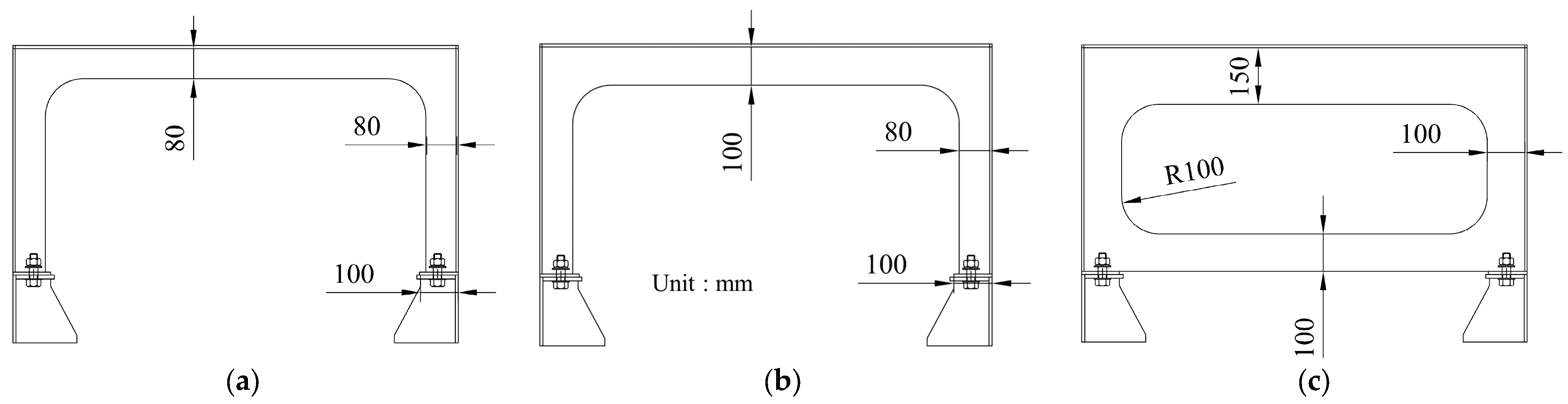
2. Protective Structures
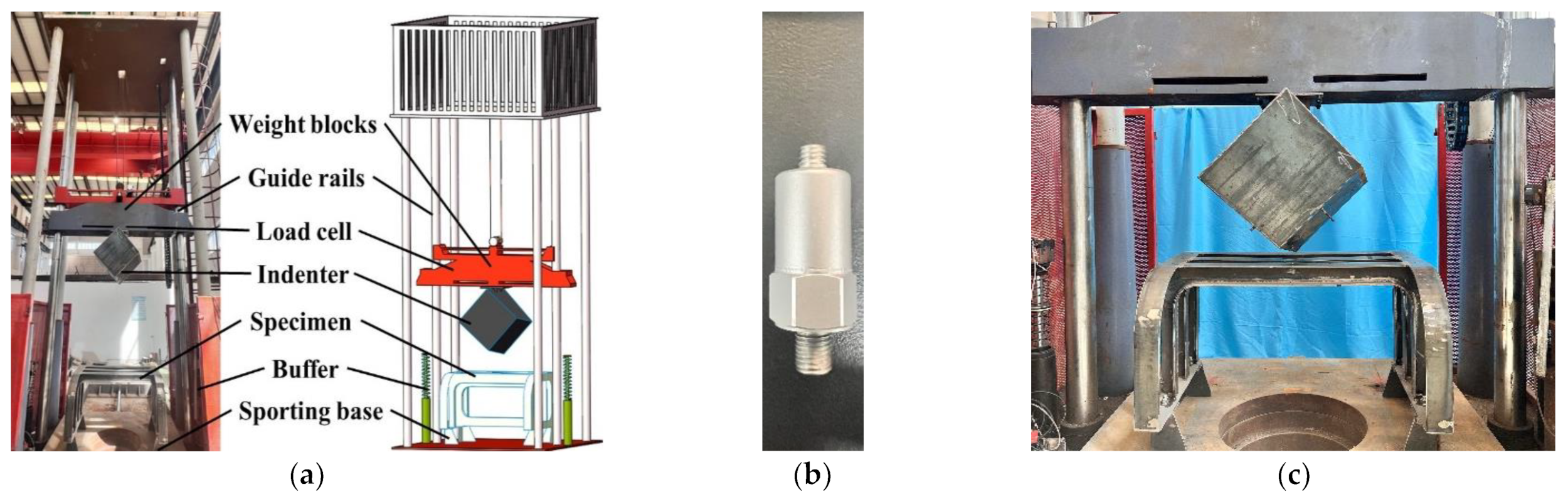
3. Falling Weight Impact Test
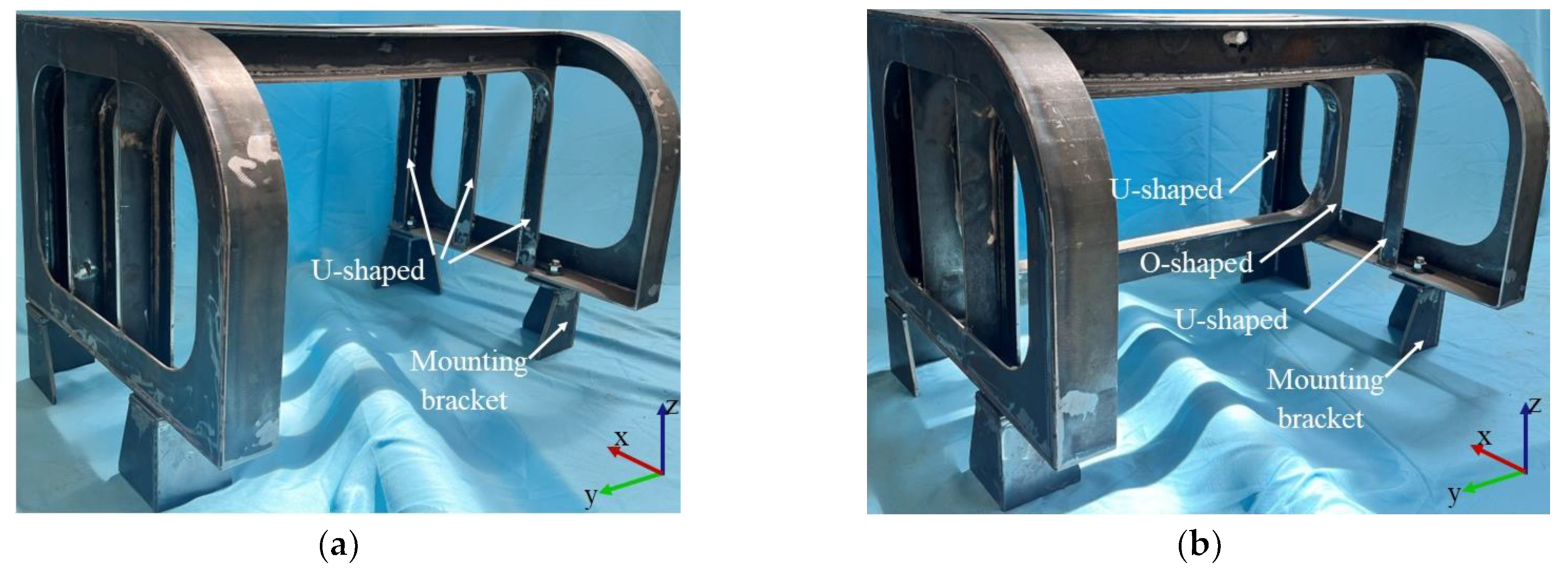
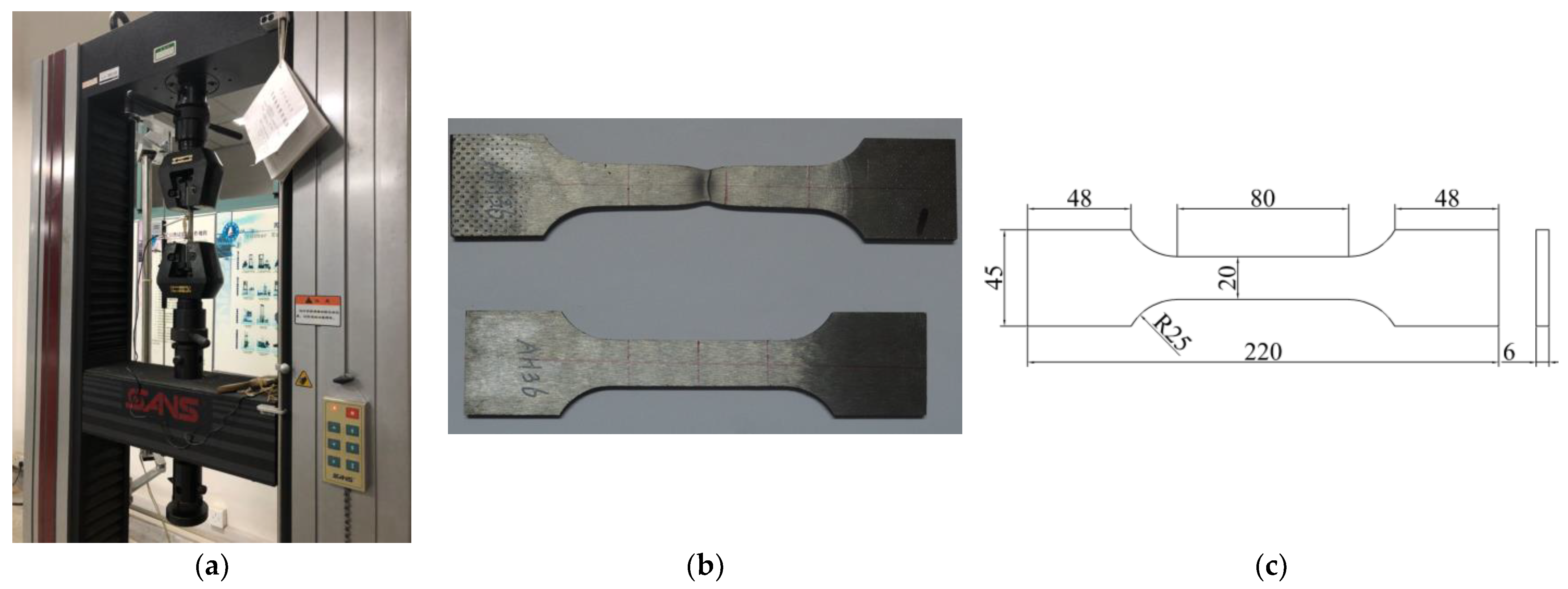
3.1. Test Specimens Employed in Experiments
3.2. Indenter Used in Experimental Testing
4. Test Results
4.1. Deformation Modes of Structure
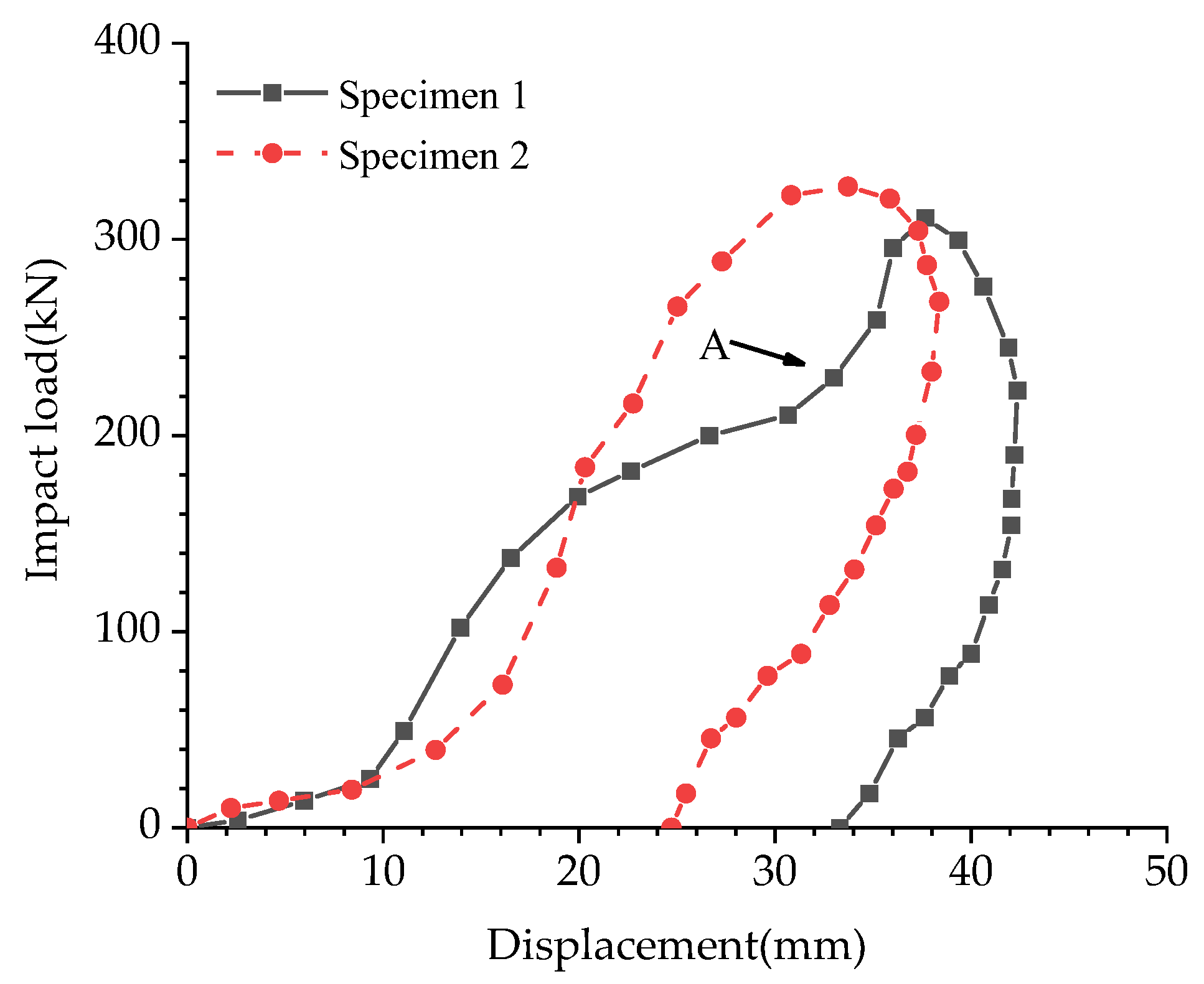
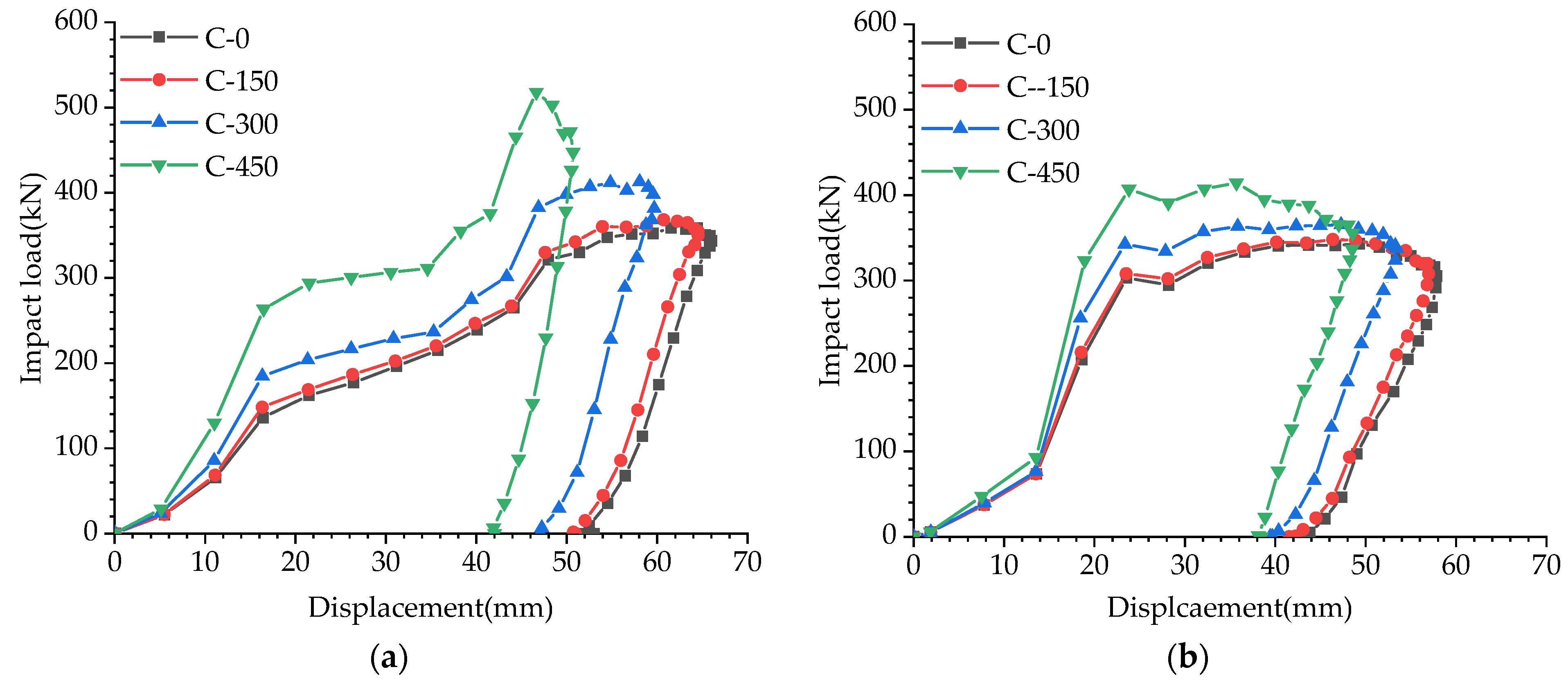
4.2. Impact Load
5. Numerical Simulations
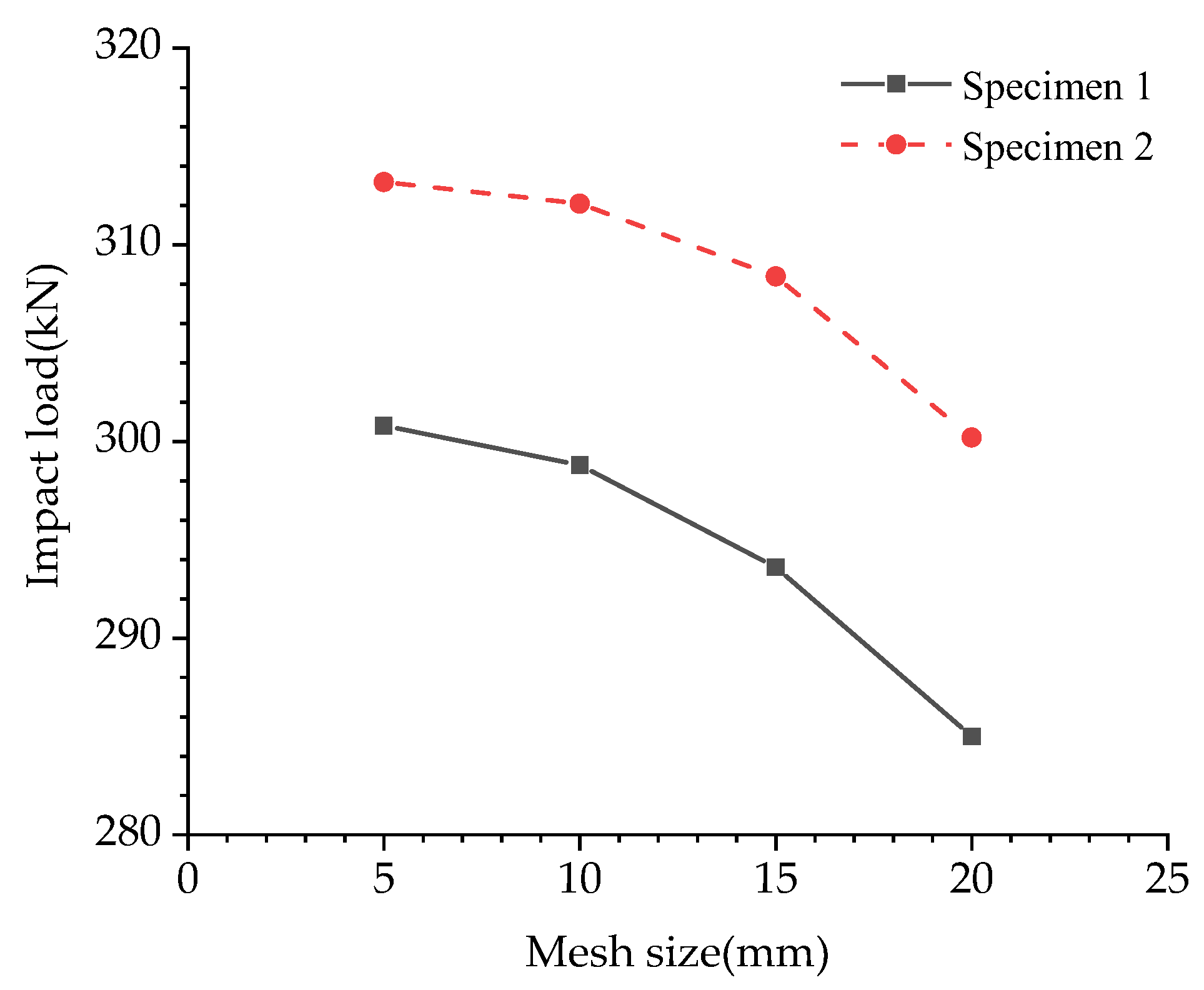
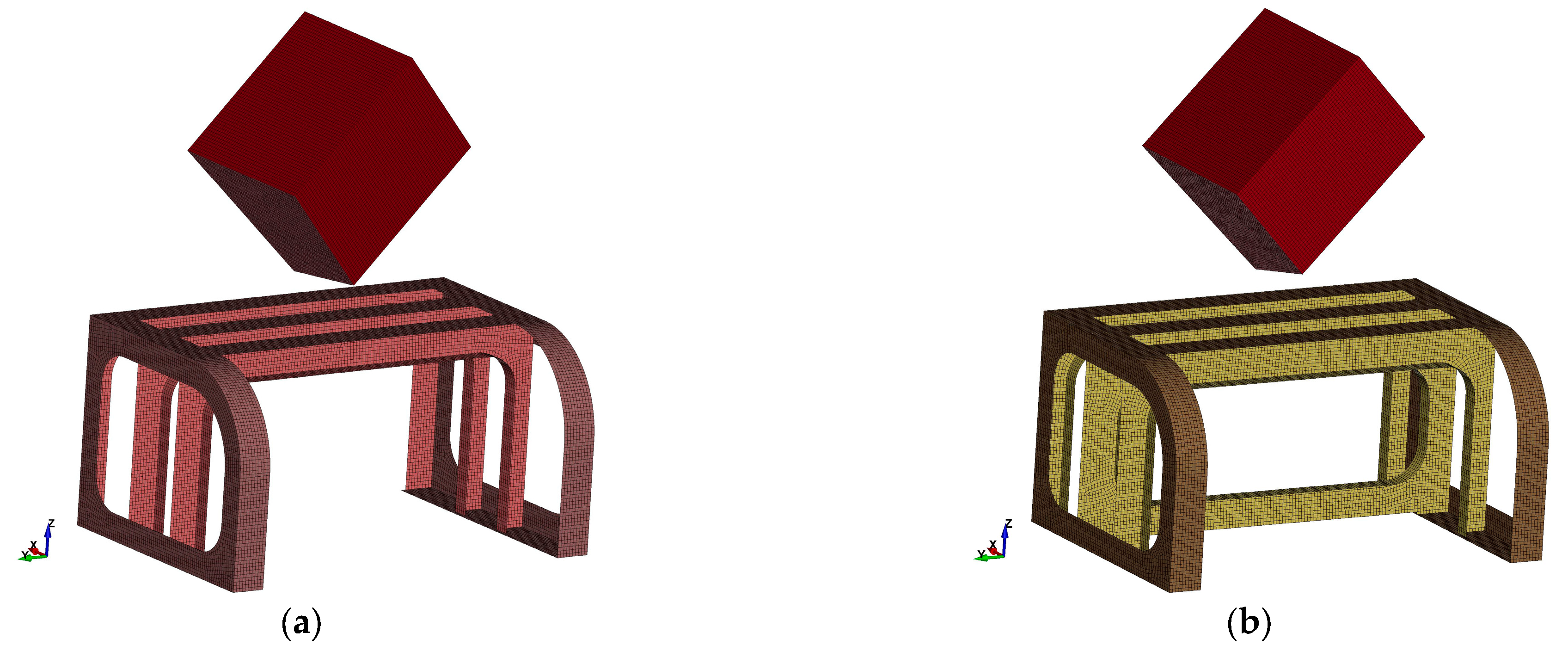
5.1. FE Models
5.2. Results and Comparisons
5.2.1. Deformation Modes
5.2.2. Impact Load
6. Parameter Sensitivity Analysis
6.1. Effect of Initial Impact Velocity
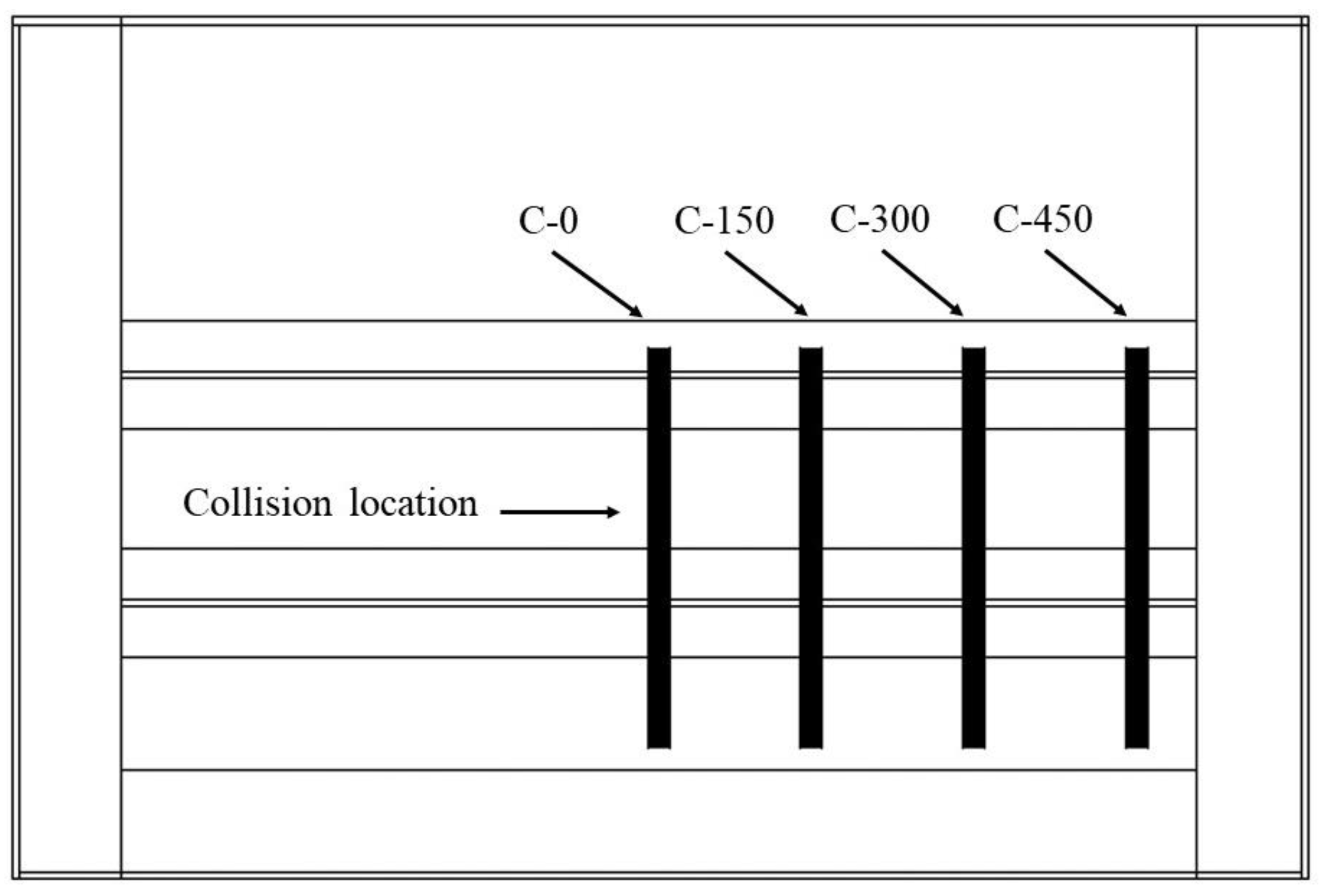
6.2. Effect of Initial Impact Locations
7. Conclusions
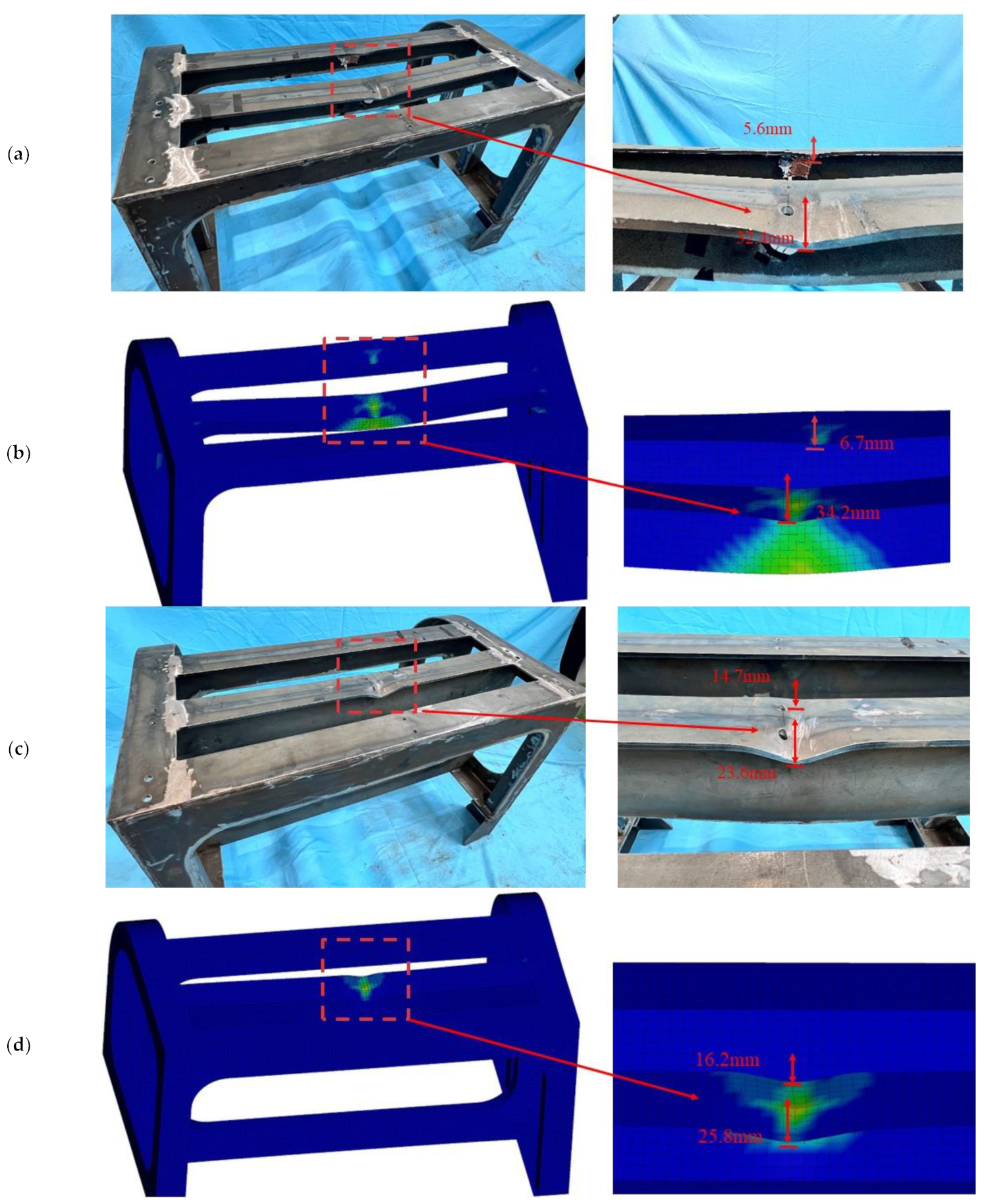
- At an impact speed of 3.89 m/s, Specimen 1 exhibited indentation deformation in the mid-section contact area and overall sinking of the frame. Additionally, the middle position of the upper section panel of Specimen 1 also showed slight deformation due to the deeper impact depth, with the ram impacting the upper section again. Deformation in Specimen 2 occurred only on Frame B, exhibiting indentation deformation, with no other areas contacting the ram. Moreover, the localized deformation in Specimen 2 was “saddle-shaped”.
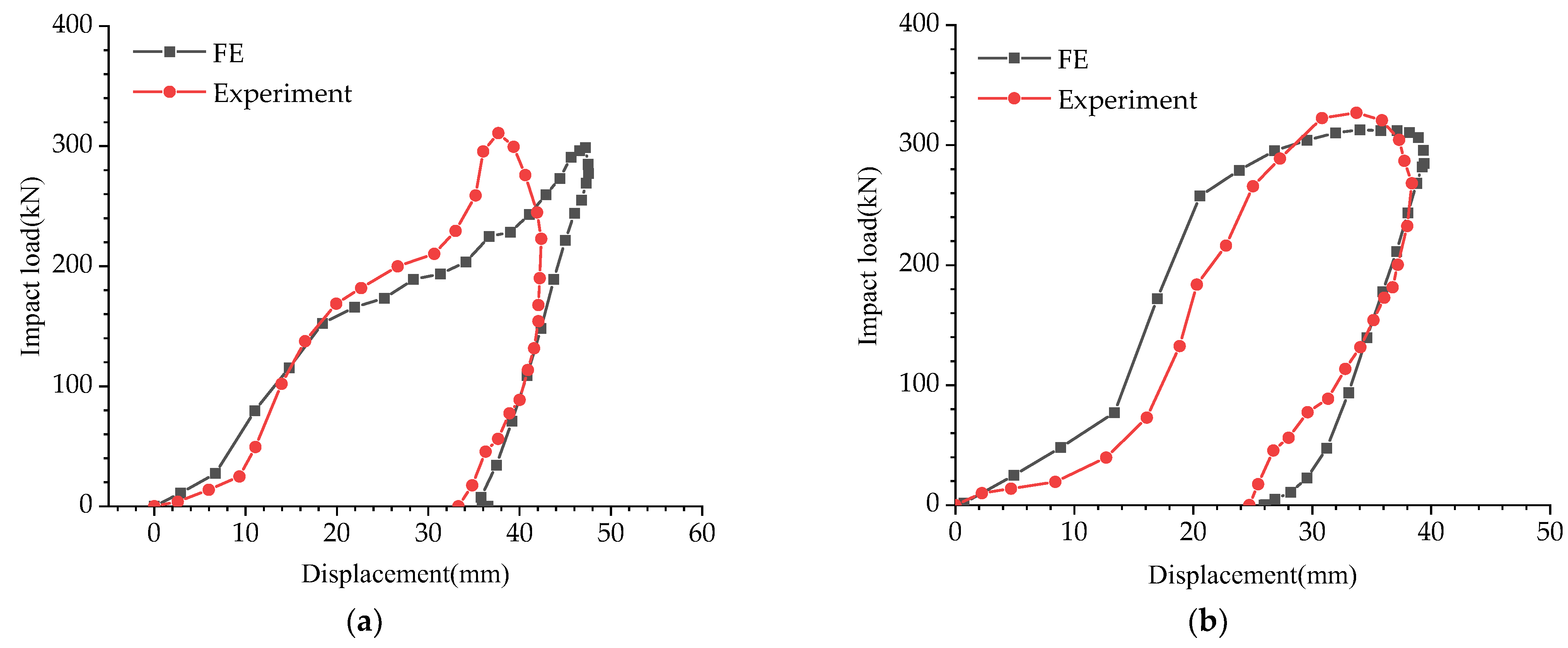
- The finite element analysis accurately predicted and captured the deformation modes of the two anti-collision structures. The maximum indentation depth and peak load in the mid-section contact area were very close to the experimental results. Specifically, the maximum indentation depth error for Specimen 1 was 5.5%, and for Specimen 2 it was 9.3%. The peak load errors for both specimens were also below 5%, demonstrating the accuracy of the finite element predictions.
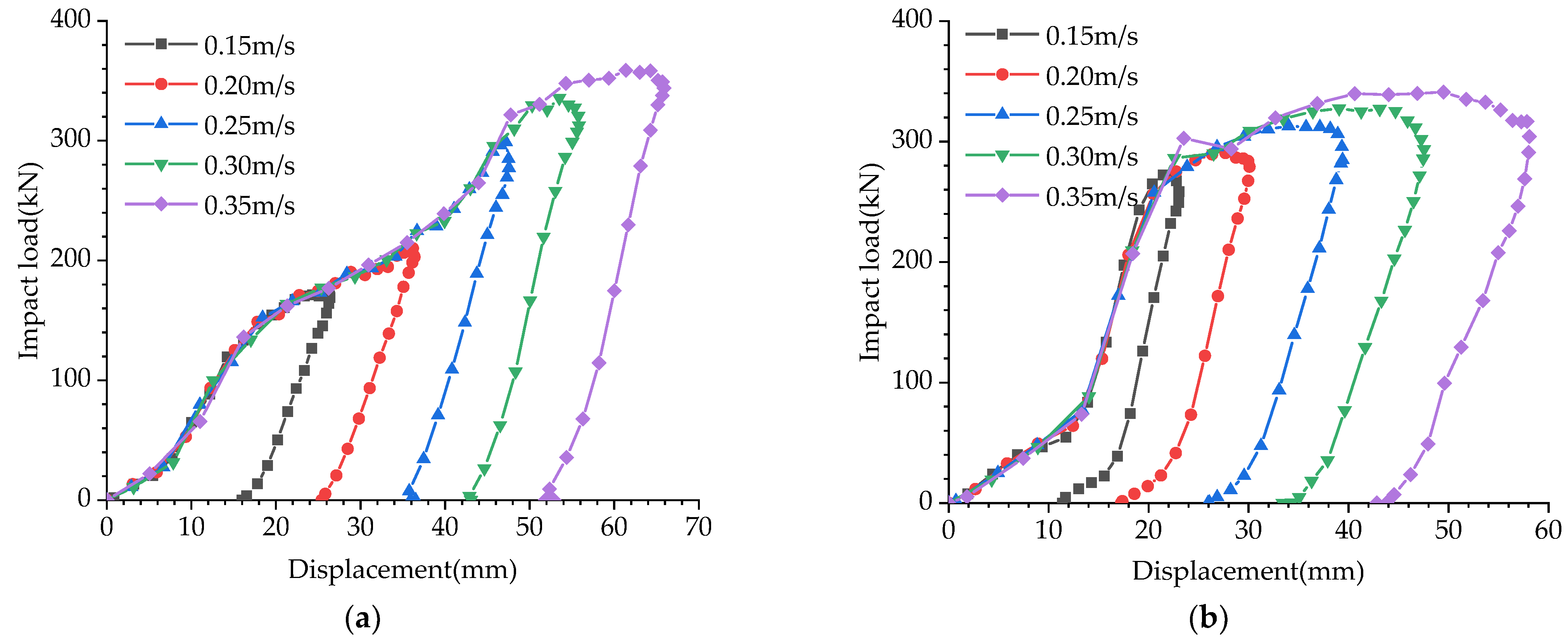
- As the impact speed increased within the range of 2.5 m/s to 3.5 m/s, Specimen 1 exhibited overall structural deformation, with a maximum indentation depth of 52.26 mm. In contrast, Specimen 2 showed localized indentation deformation at all five speeds, with a maximum indentation depth of 43.26 mm. Both the damage deformation patterns and the maximum indentation depth data indicate that Specimen 2 has superior impact resistance.
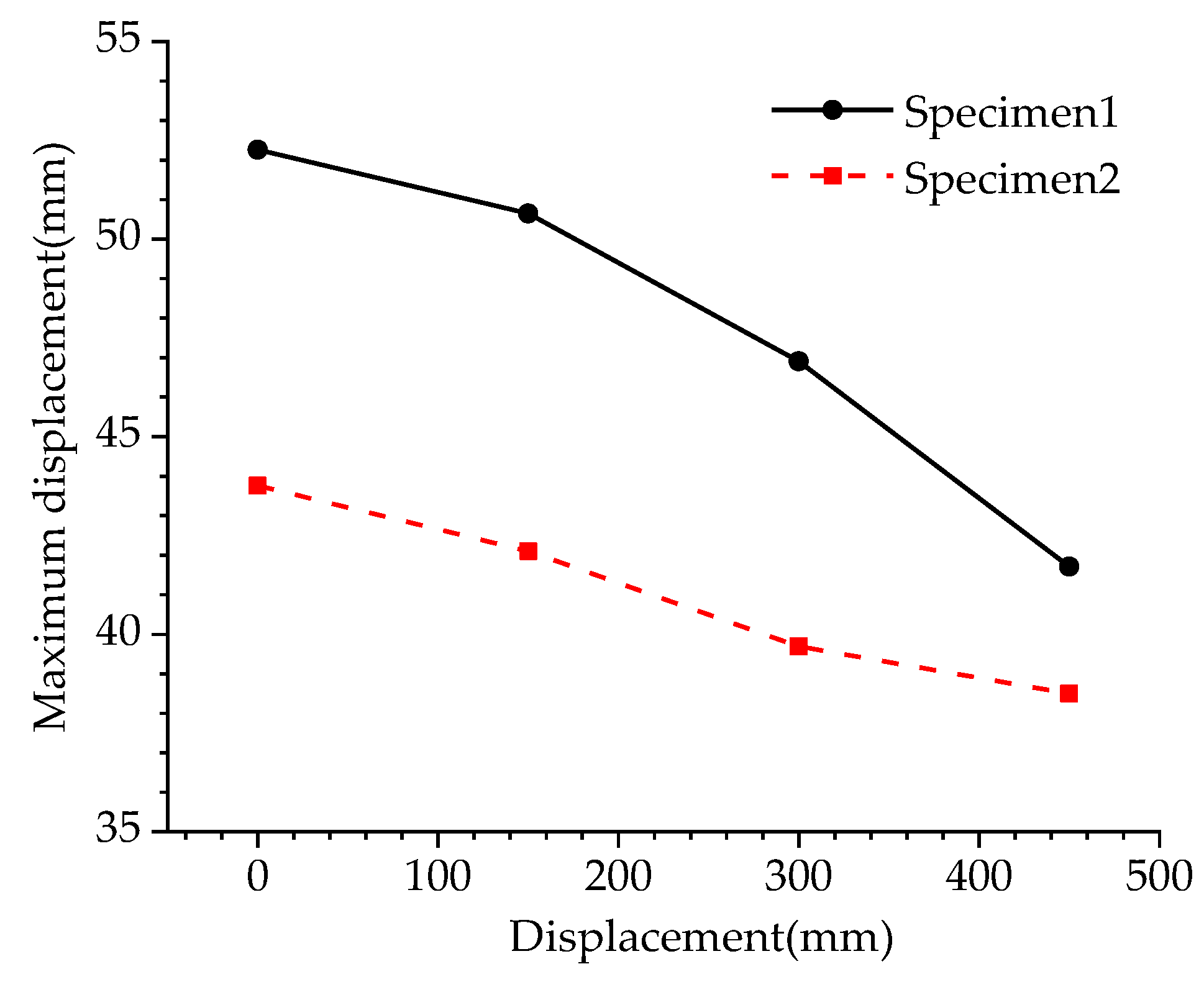
- Finite element simulations of collisions at different positions showed that the dynamic responses of the two specimens with different structures were slightly affected by the impact location. As the impact location moved from the center to the end, the maximum indentation depth of Specimen 1 decreased from 52.26 mm to 41.71 mm, while that of Specimen 2 decreased relatively less, from 43.26 mm to 38.50 mm. This indicates that the O-shaped structure is less sensitive to impact location compared to the U-shaped structure. Additionally, the trend shows that for both specimens, as the impact location moves further from the midspan, the indentation depth decreases due to the increasing involvement of the support part in resisting the impact.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- House, D. Dry Docking and Shipboard Maintenance; Routledge: London, UK, 2015; ISBN 9781317438434. [Google Scholar]
- Wankhede, A. Dry Dock, Types of Dry Docks & Requirements for Dry Dock. Last Modified 9 January 2021. 2021. Available online: https://www.marineinsight.com/guidelines/dry-dock-types-of-dry-docks-requirements-for-dry-dock/#Types_of_Dry_Dock (accessed on 24 March 2024).
- Kaup, M.; Łozowicka, D.; Blatnický, M. Selected Problems of Ship Safety in the Process of Docking. New Trends Prod. Eng. 2018, 1, 43–49. [Google Scholar] [CrossRef]
- Korotaev, V.V.; Pantiushin, A.V.; Serikova, M.G.; Anisimov, A.G. Deflection Measuring System for Floating Dry Docks. Ocean Eng. 2016, 117, 39–44. [Google Scholar] [CrossRef]
- Zhang, J.; Wen, X.; Ong, M.C. Development of a Floating Dock Numerical Model and the Ballast Water Distribution Strategy. In Proceedings of the ASME 2023 42nd International Conference on Ocean, Offshore and Arctic Engineering, Melbourne, Australia, 11–16 June 2023. [Google Scholar]
- Wen, X.; García Conde, A.; Zhang, J.; Ong, M.C. Dynamic Analysis of a Floating Dock under Accidental Conditions. Appl. Ocean Res. 2024, 144, 103908. [Google Scholar] [CrossRef]
- Njumo, D.A. Stability Failure Analysis in the Operations of Floating Dry Docks. Int. J. Marit. Eng. 2017, 159, 41–61. [Google Scholar] [CrossRef]
- Zhou, S.; Fang, H.; Zhang, X.; Zhu, L.; Wang, S. Ship Collision Performance of a Flexible Anti-Collision Device Designed with Fiber-Reinforced Rubber Composites. Eng. Struct. 2024, 302, 117472. [Google Scholar] [CrossRef]
- Cho, H.R.; Kim, H.J.; Park, S.M.; Park, D.K.; Yun, S.H.; Paik, J.K. Effect of Solid Rubber Fenders on the Structural Damage Due to Collisions between a Ship-Shaped Offshore Installation and an Offshore Supply Vessel. Ships Offshore Struct. 2023, 18, 1037–1059. [Google Scholar] [CrossRef]
- Park, S.M.; Kim, H.J.; Cho, H.R.; Kong, K.H.; Park, D.K.; Paik, J.K. Effect of Pneumatic Rubber Fenders on the Prevention of Structural Damage during Collisions between a Ship-Shaped Offshore Installation and a Shuttle Tanker Working Side-by-Side. Ships Offshore Struct. 2023, 18, 596–608. [Google Scholar] [CrossRef]
- Liu, C.; Hao, E.; Zhang, S. Optimization and Application of a Crashworthy Device for the Monopile Offshore Wind Turbine against Ship Impact. Appl. Ocean Res. 2015, 51, 129–137. [Google Scholar] [CrossRef]
- Pan, J.; Peng, H.; Gan, J.; Zhang, W.; Li, X. Study on Crashworthiness Performance of an Airbag Anti-Collision Device for Vessel-Bridge Collision. In Proceedings of the ASME 2023 42nd International Conference on Ocean, Offshore and Arctic Engineering, Melbourne, Australia, 11–16 June 2023. [Google Scholar]
- Shiraishi, S.; Nagai, K.; Akiyama, H. Durability Test and Statistical Evaluation of Rubber Fenders for Vessel Berthing. J. JSCE 2021, 9, 71–85. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Yang, L.; Huang, D.; Zhang, Z.; Chen, G. An Impact Dynamics Analysis on a New Crashworthy Device against Ship–Bridge Collision. Int. J. Impact Eng. 2008, 35, 895–904. [Google Scholar] [CrossRef]
- Wang, J.J.; Song, Y.C.; Wang, W.; Tu, L.F. Evaluation of Composite Crashworthy Device for Pier Protection against Barge Impact. Ocean Eng. 2018, 169, 144–158. [Google Scholar] [CrossRef]
- Wang, W.; Morgenthal, G.; Kraus, M. Numerical Evaluation of a Novel Crashworthy Device for Pier Protection from Barge Impact. Eng. Struct. 2020, 212, 110535. [Google Scholar] [CrossRef]
- Wang, J.J.; Song, Y.C.; Wang, W.; Chen, C.J. Evaluation of Flexible Floating Anti-Collision Device Subjected to Ship Impact Using Finite-Element Method. Ocean Eng. 2019, 178, 321–330. [Google Scholar] [CrossRef]
- Zhou, L.; Li, H.; Wei, J.; Pu, X.; Mahunon, A.D.; Jiang, L. Design and Simulation Analysis of a New Type of Assembled UHPC Collision Avoidance. Appl. Sci. 2020, 10, 4555. [Google Scholar] [CrossRef]
- Yan, H.; Jia, E.; Fang, H.; Zhu, L.; Zhang, X.; Dai, Z. Impact Dynamics Analyses on an Innovative Fiber-Reinforced Rubber Composite Bumper System for Bridge Protection. Thin-Walled Struct. 2024, 195, 111331. [Google Scholar] [CrossRef]
- Park, H.-Y.; Kim, J.; Kuwahara, S. Cyclic Behavior of Shear-Type Hysteretic Dampers with Different Cross-Sectional Shapes. J. Constr. Steel Res. 2021, 187, 106964. [Google Scholar] [CrossRef]
- Chen, Y.; Ye, D.; Zhang, L. Analytical Development and Experimental Investigation of the Casting Multi-Plate Damper (CMPD). Eng. Struct. 2022, 250, 113402. [Google Scholar] [CrossRef]
- Zheng, K.; Xu, X. Experimental and Numerical Study on the Mechanical Behavior of Composite Steel Structure under Explosion Load. Materials 2021, 14, 246. [Google Scholar] [CrossRef] [PubMed]
- GB/T228.1-2010; Metallic Materials-Tensile Testing-Part 1: Method of Test at Room Temperature. China Standard Press: Beijing, China, 2011.
- Alsos, H.S.; Amdahl, J.; Hopperstad, O.S. On the Resistance to Penetration of Stiffened Plates, Part II: Numerical Analysis. Int. J. Impact Eng. 2009, 36, 875–887. [Google Scholar] [CrossRef]
- Liu, K.; Lu, Y.; Wang, Z.; Wang, G. (George) An Experimental, Numerical and Analytical Study on Deformation Mechanisms of Web Girders in a Collision or Grounding Incident. Ships Offshore Struct. 2019, 14, 839–852. [Google Scholar] [CrossRef]

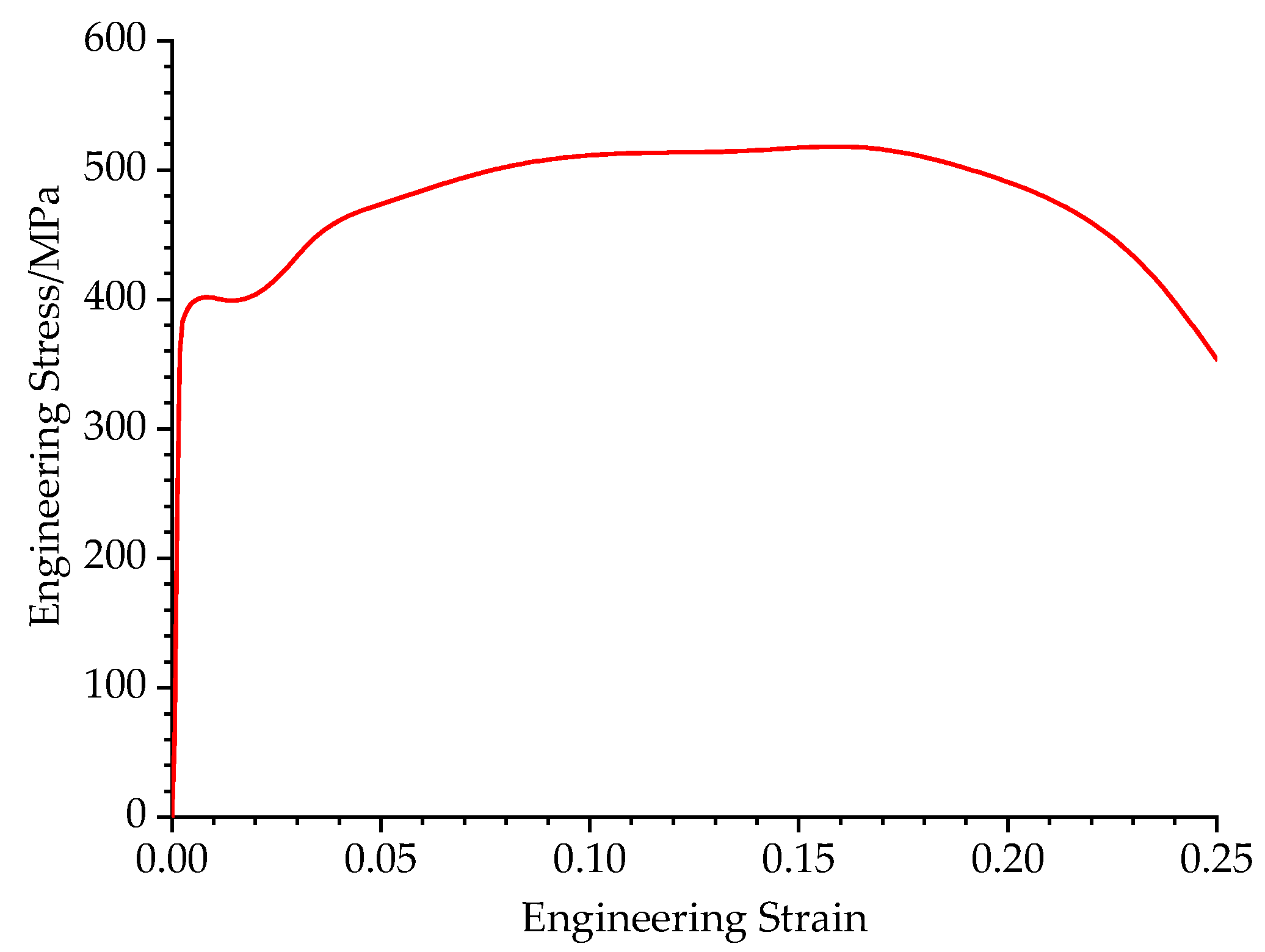


| Property | Value | Unit |
|---|---|---|
| Density ρ | 7850 | kg/m3 |
| Young’s modulus Ε | 212 | GPa |
| Poisson’s ratio υ | 0.26 | - |
| Yield strength σY | 355 | MPa |
| Ultimate tensile strength σu | 510 | MPa |
| Specimen | Maximum Displacement (mm) | Discrepancy | Peak Load (kN) | Discrepancy | ||
|---|---|---|---|---|---|---|
| Experiment | FE | Experiment | FE | |||
| Specimen 1 | 32.4 | 34.2 | 5.5% | 310.9 | 298.8 | 3.9% |
| Specimen 2 | 23.6 | 25.8 | 9.3% | 327.0 | 312.1 | 4.6% |
| Impact Cases | Impact Velocity (m/s) | Impact Energy (kJ) | Drop Height (mm) |
|---|---|---|---|
| V-0.15 | 2.34 | 2.6 | 278 |
| V-0.20 | 3.11 | 4.7 | 495 |
| V-0.25 | 3.89 | 7.3 | 773 |
| V-0.30 | 4.67 | 10.1 | 1114 |
| V-0.35 | 5.45 | 14.4 | 1518 |
| Impact Energy Cases | Final Deformation of Frame B in Specimen 1 | Final Deformation of Frame B in Specimen 2 |
|---|---|---|
| V-0.15 |  |  |
| V-0.20 |  |  |
| V-0.25 |  |  |
| V-0.30 |  |  |
| V-0.35 |  |  |
| Impact Location Cases | Final Deformation of Frame B in Specimen 1 | Final Deformation of Frame B in Specimen 2 |
|---|---|---|
| C-0 |  |  |
| C-150 |  |  |
| C-300 | 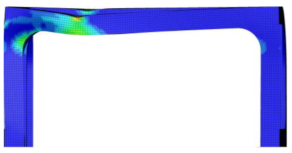 |  |
| C-450 |  |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Liu, K.; Liu, J.; Meng, Q.; Qiu, W.; Zong, S. Experimental and Simulation Studies on Protective Structures in Floating Dock. J. Mar. Sci. Eng. 2024, 12, 1311. https://doi.org/10.3390/jmse12081311
Wang Z, Liu K, Liu J, Meng Q, Qiu W, Zong S. Experimental and Simulation Studies on Protective Structures in Floating Dock. Journal of Marine Science and Engineering. 2024; 12(8):1311. https://doi.org/10.3390/jmse12081311
Chicago/Turabian StyleWang, Zhengyao, Kun Liu, Jingqiao Liu, Qingao Meng, Weijian Qiu, and Shuai Zong. 2024. "Experimental and Simulation Studies on Protective Structures in Floating Dock" Journal of Marine Science and Engineering 12, no. 8: 1311. https://doi.org/10.3390/jmse12081311
APA StyleWang, Z., Liu, K., Liu, J., Meng, Q., Qiu, W., & Zong, S. (2024). Experimental and Simulation Studies on Protective Structures in Floating Dock. Journal of Marine Science and Engineering, 12(8), 1311. https://doi.org/10.3390/jmse12081311






