A Simplified Method for the Evaluation of Floating-Body Motion Responses over a Sloping Bottom
Abstract
1. Introduction
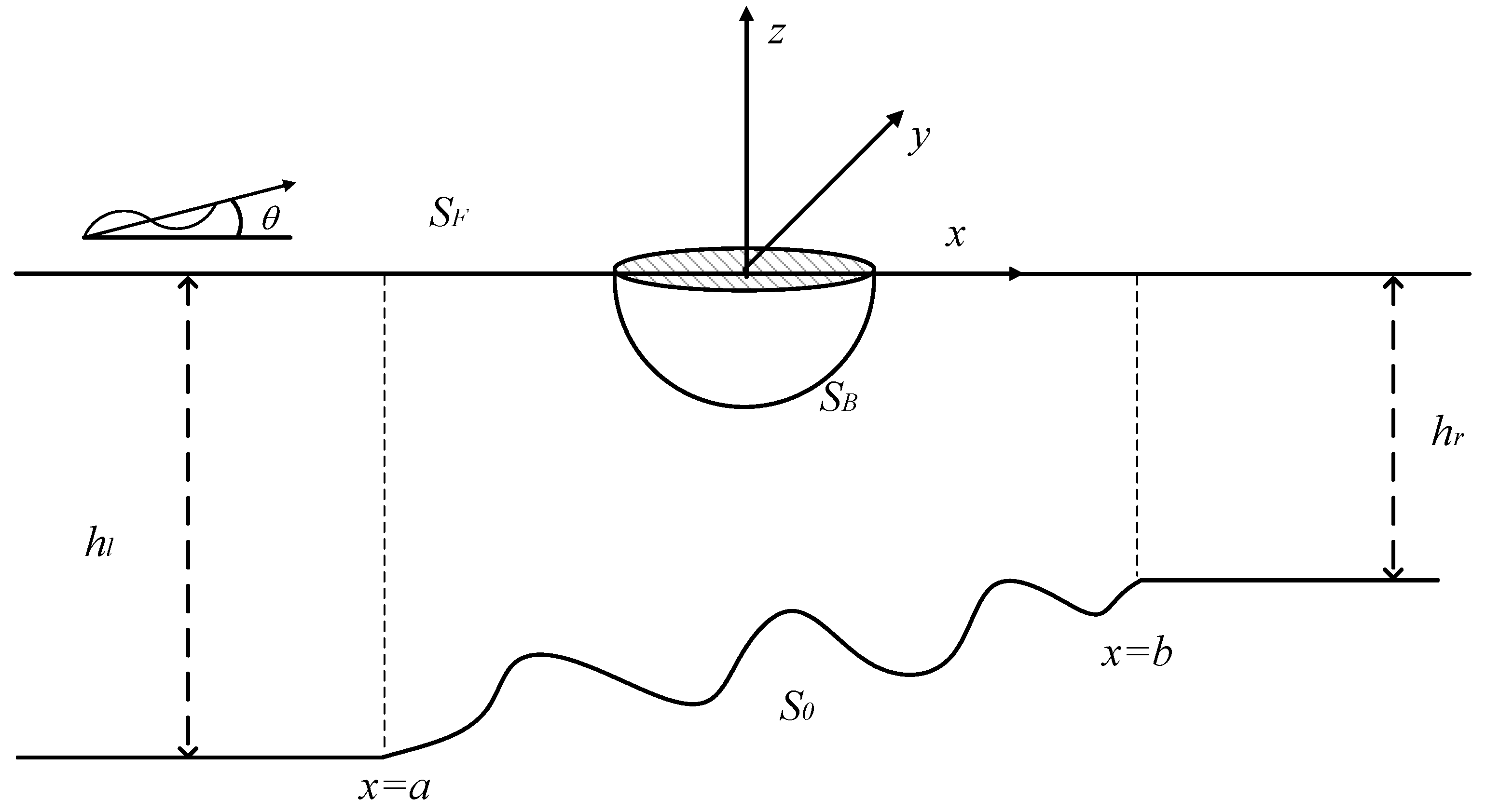
2. Governing Equations and Boundary Conditions
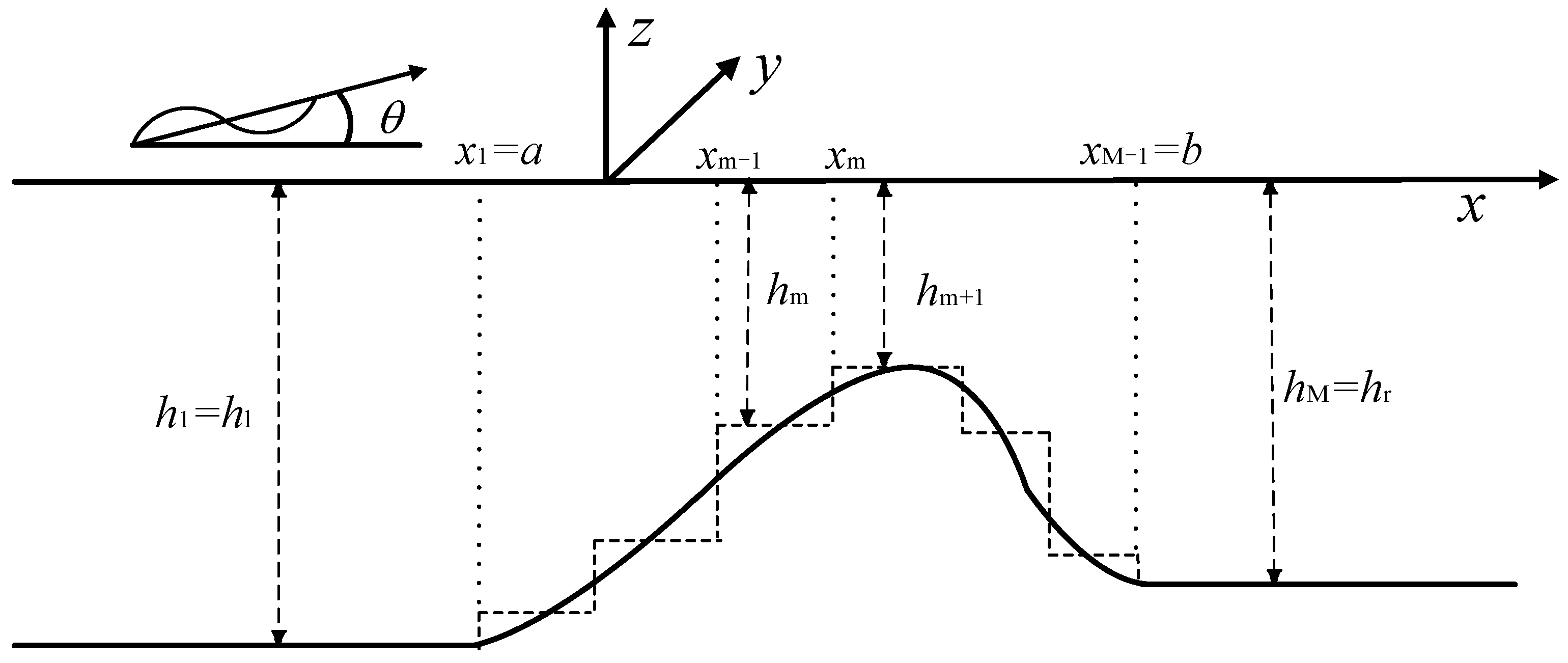
3. A Simplified Method Combining the Eigenfunction Matching Method with the Finite-Depth Green’s Function
3.1. Incident Wave Problem for Sloping Bottom Environment
3.2. Diffraction and Radiation Problems Considering the Sloping Seabed
4. Results and Discussion
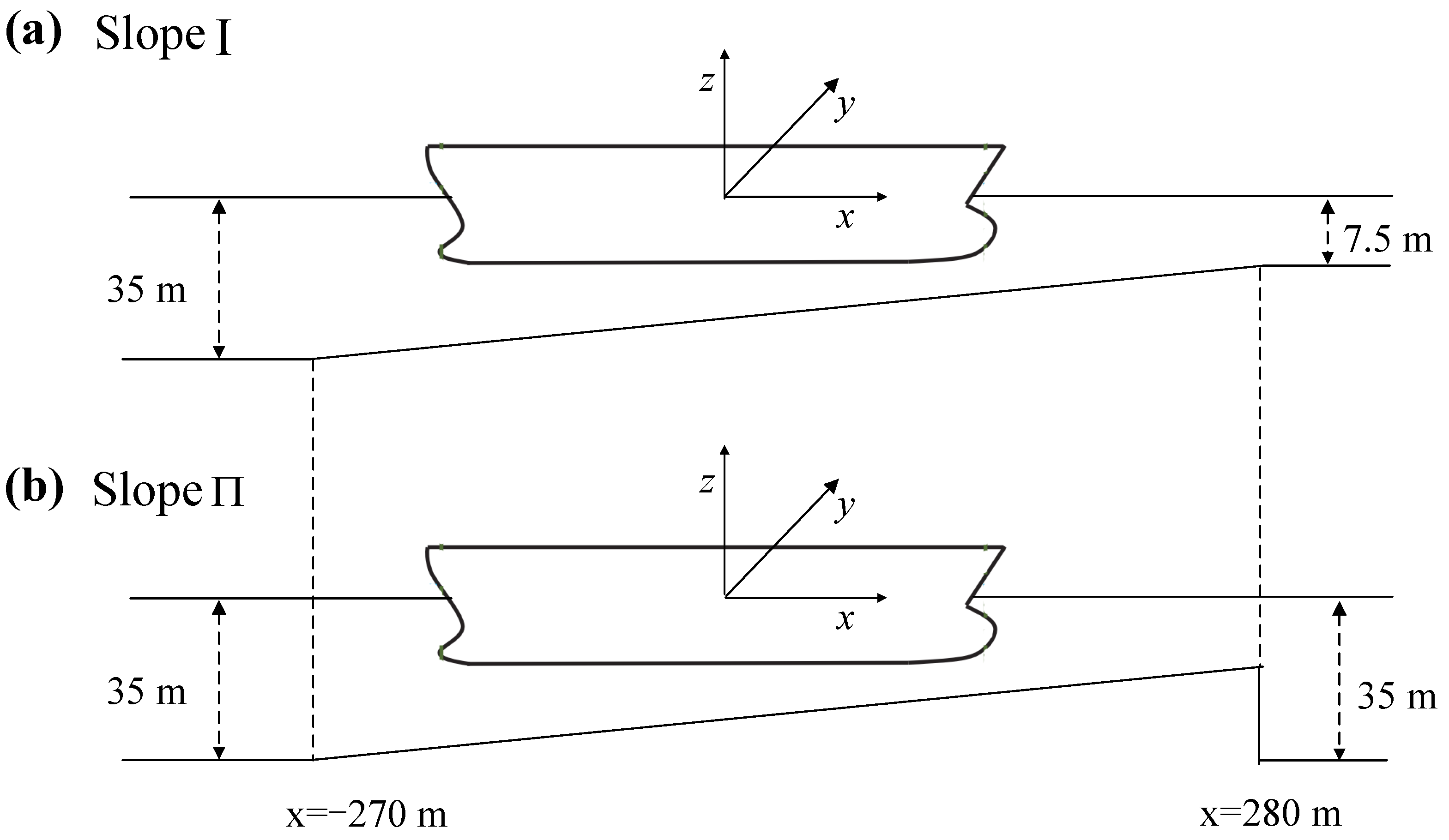
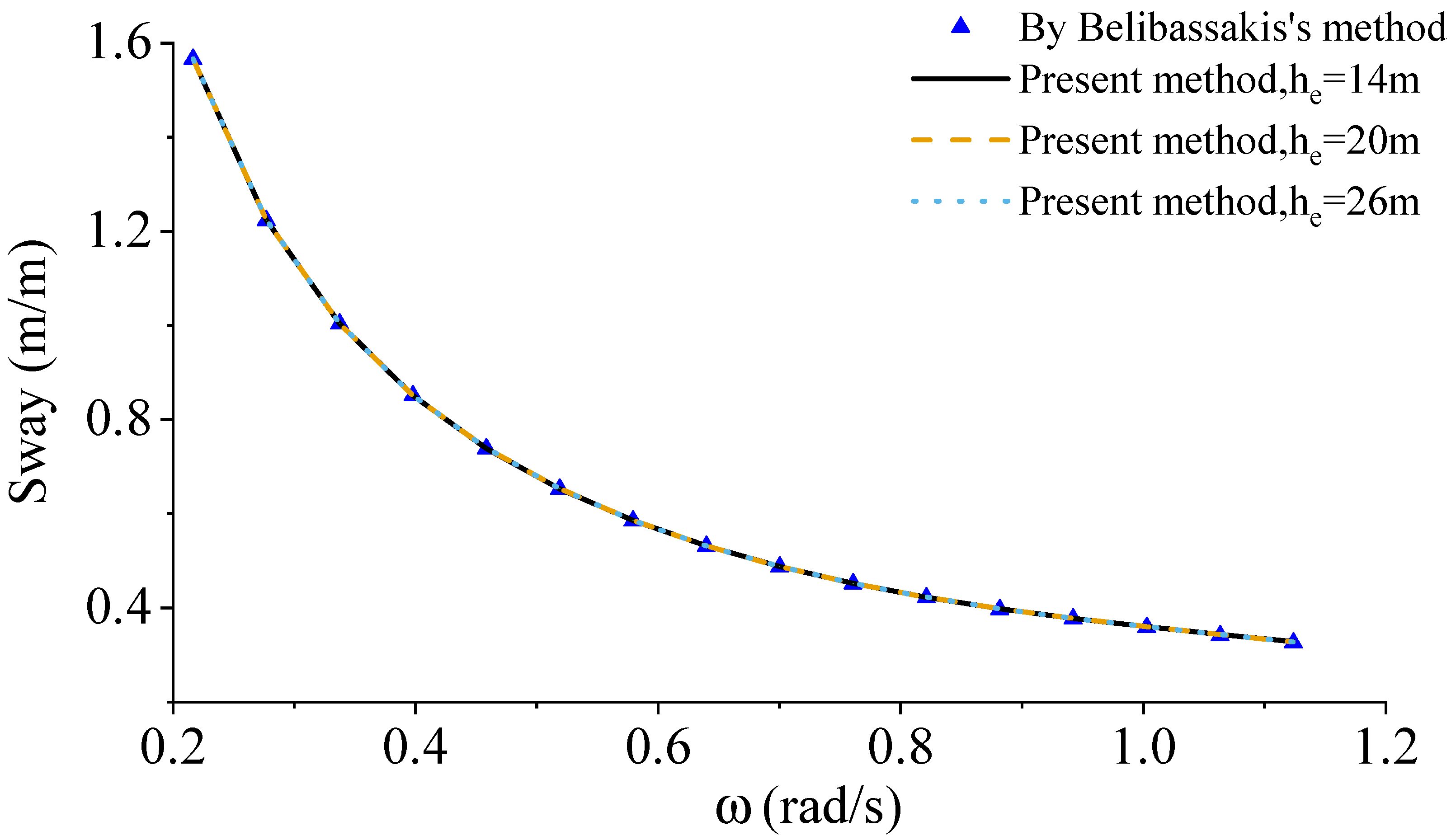
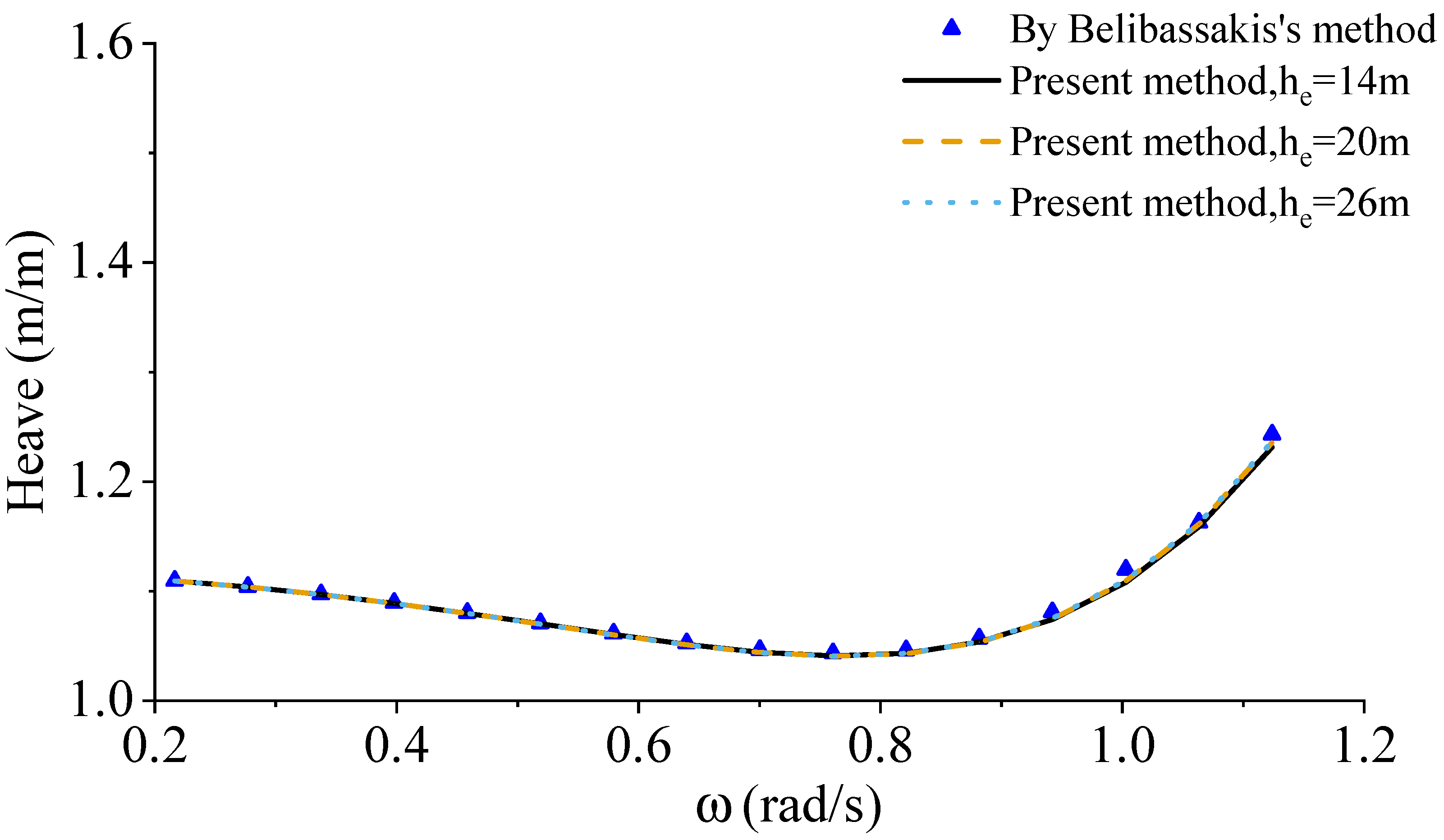
4.1. Comparison with Numerical Results for an LNG Ship
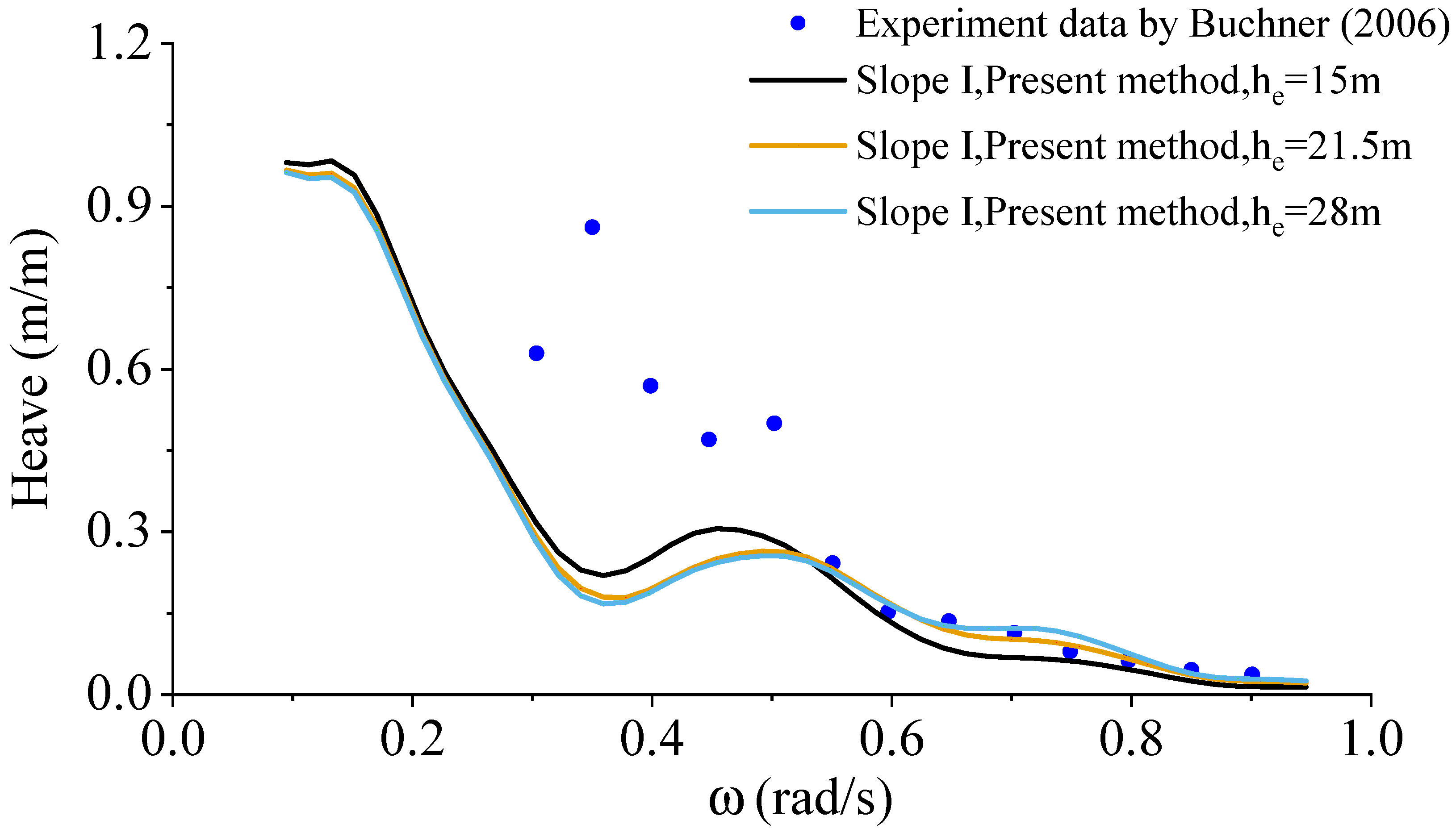
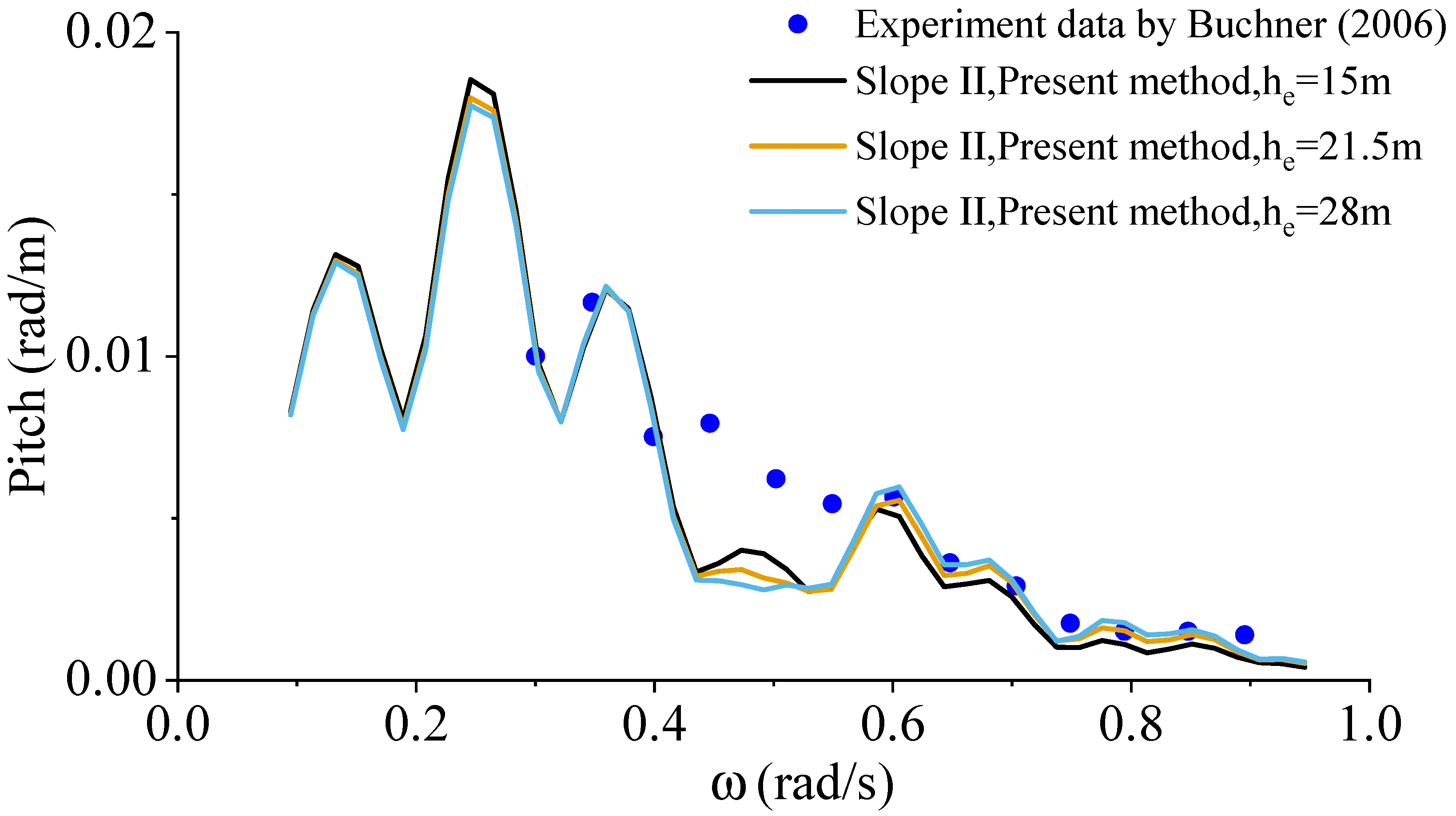
4.2. Comparison with Experimental Results for an LNG Ship
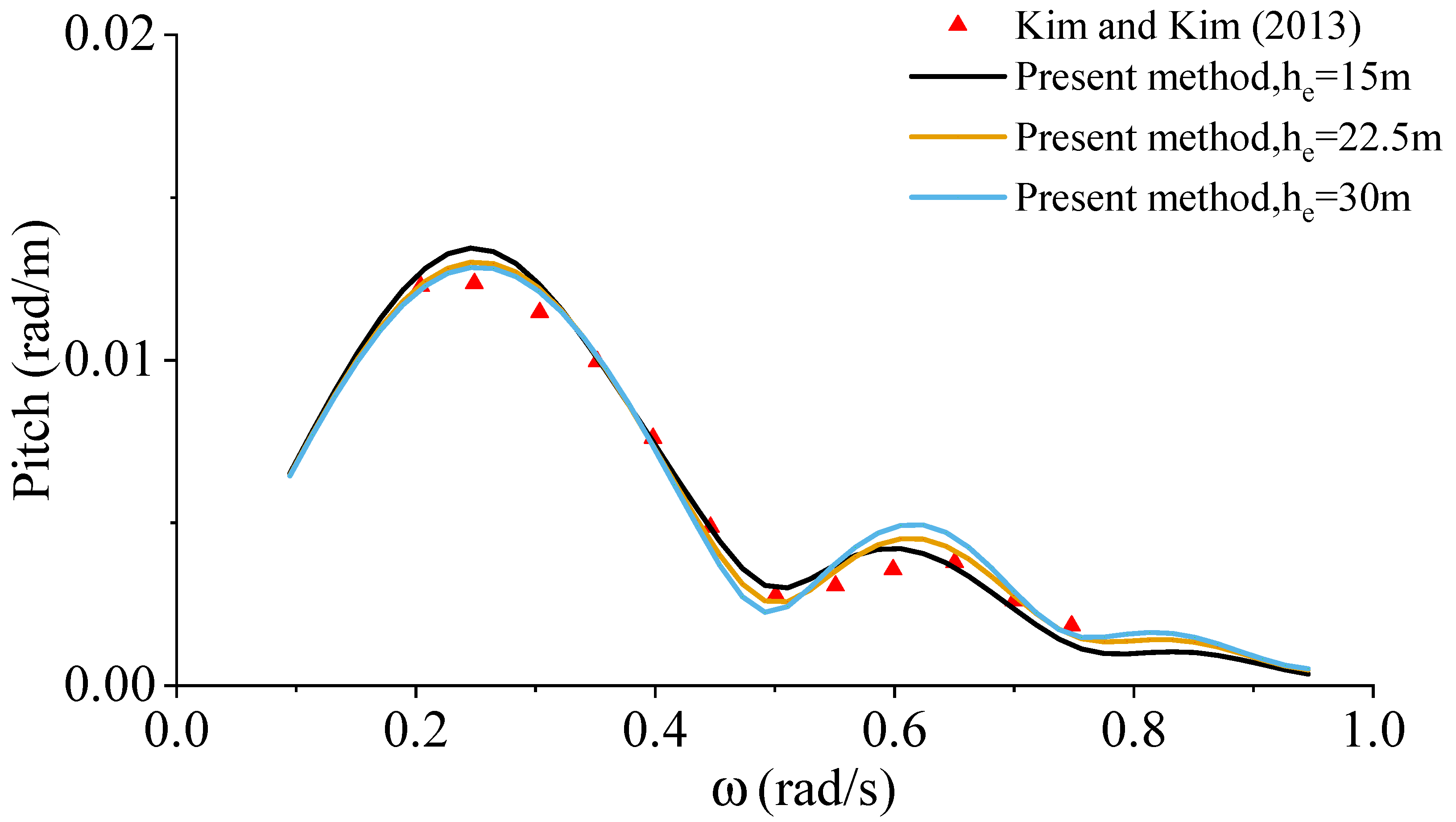
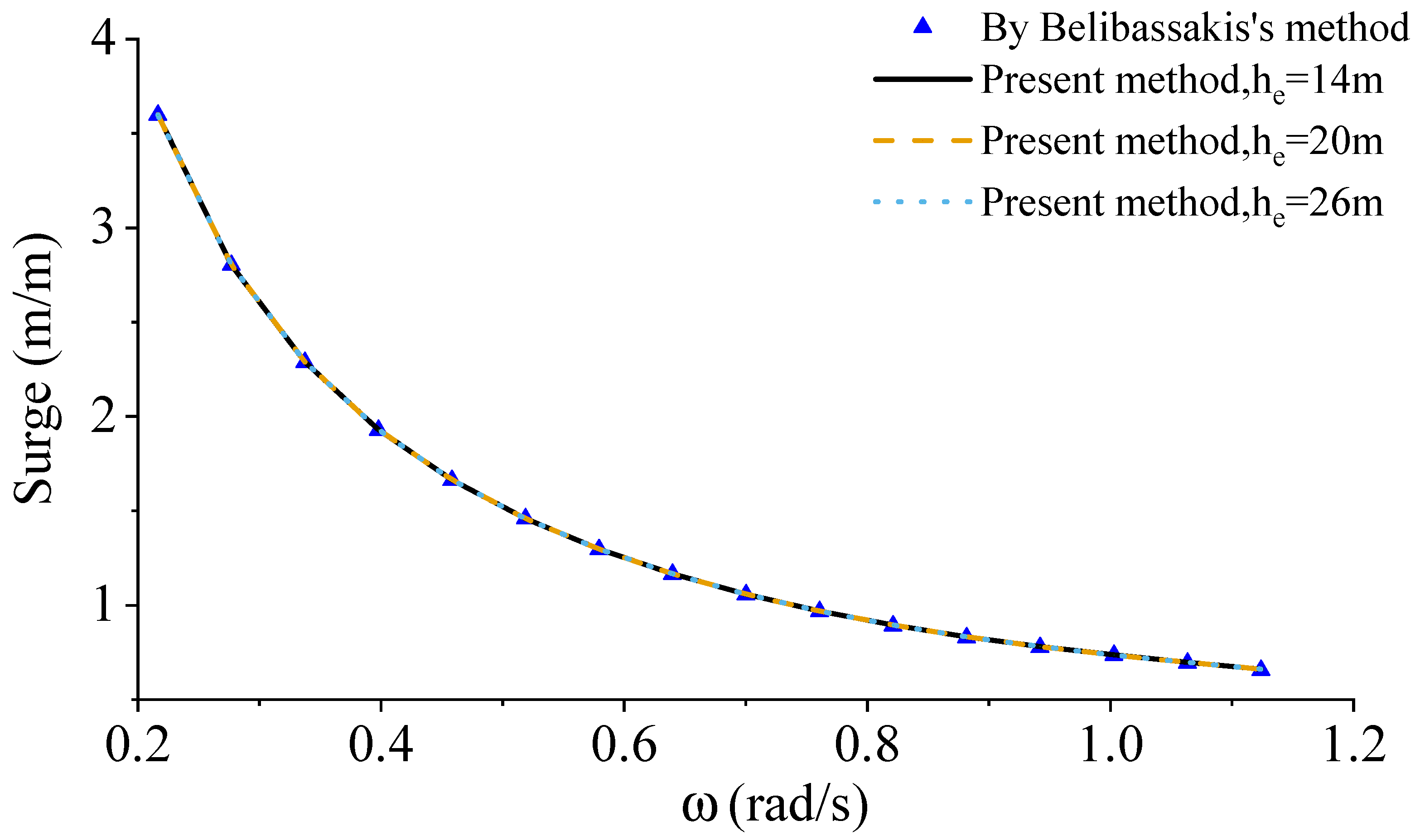
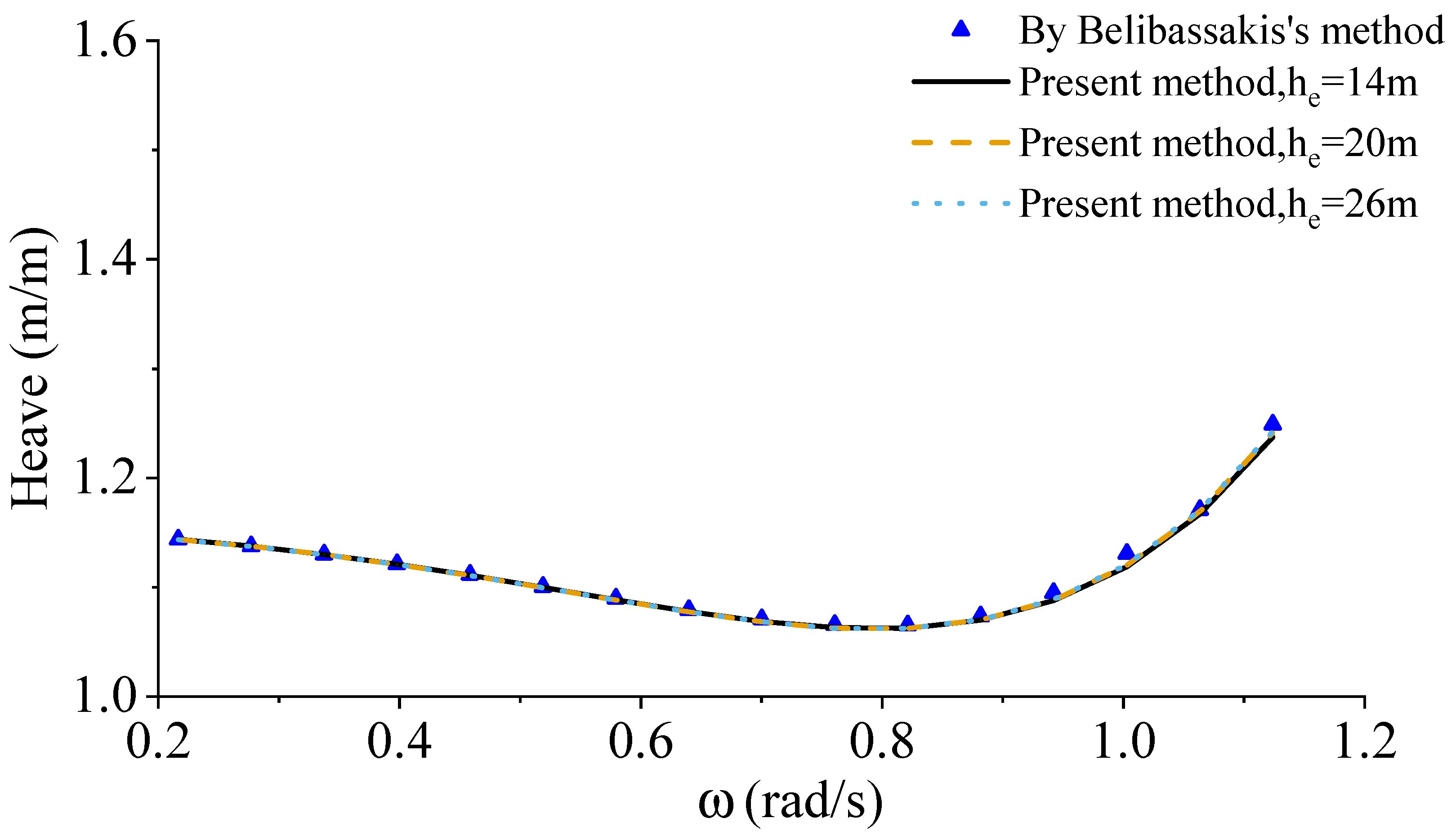
4.3. Comparison with Numerical Results for a Floating Hemisphere
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Water depth of the left flat bottom | |
| Water depth of the right flat bottom | |
| Wave velocity potential | |
| Incident wave potential | |
| Diffraction wave potential | |
| Radiation wave potential | |
| Wave frequency | |
| g | Gravity acceleration |
| n | Unit normal vector |
| Body velocity in the normal direction | |
| Eigenvalue of the dispersion relation | |
| G | Green’s function |
| Source strength | |
| Equivalent constant depth | |
| r | Distance between the source and field points |
| Distance between the field point and the image of the source point | |
| RAO | Response amplitude operator |
| BEM | Boundary element method |
| FDGF | Finite-depth Green’s function |
| 2D | Two-dimensional |
| 3D | Three-dimensional |
| F-K | Froude–Krylov |
| EMM | Eigenfunction matching method |
References
- Oortmerssen, G.V. The motions of a ship in swallow water. Ocean Eng. 1976, 3, 221–255. [Google Scholar] [CrossRef]
- Feng, A.; Tang, P.; You, Y.; Liu, K. Finite Water Depth Effect on Wave-Body Problems Solved by Rankine Source Method. J. Ocean Univ. China 2017, 16, 191–199. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Xu, S.; Ding, A. Motion Response of a Moored Semi-Submersible-Type Single Module of a VLFS in Multi-Slope Shallow Water. Int. J. Offshore Polar Eng. 2017, 27, 397–405. [Google Scholar] [CrossRef]
- Zheng, Y.H.; You, Y.G.; Shen, Y.M. On the radiation and diffraction of water waves by a rectangular buoy. Ocean Eng. 2004, 31, 1063–1082. [Google Scholar] [CrossRef]
- Zheng, Y.; Shen, Y.; You, Y.; Wu, B.; Jie, D. On the radiation and diffraction of water waves by a rectangular structure with a sidewall. Ocean Eng. 2004, 31, 2087–2104. [Google Scholar] [CrossRef]
- Yang, X.-Y.; Zhang, H.-S.; Li, H.-T. Wave radiation and diffraction by a floating rectangular structure with an opening at its bottom in oblique seas. J. Hydrodyn. 2017, 29, 1054–1066. [Google Scholar] [CrossRef]
- Wu, B.-J.; Zheng, Y.-H.; You, Y.-G.; Sun, X.-Y.; Chen, Y. On diffraction and radiation problem for a cylinder over a caisson in water of finite depth. Int. J. Eng. Sci. 2004, 42, 1193–1213. [Google Scholar] [CrossRef]
- Hulme, A. The wave forces on a floating hemisphere undergoing forced periodic oscillations. J. Fluid Mech. 1982, 121, 443–463. [Google Scholar] [CrossRef]
- Newman, J.N. Algorithms for the free-surface Green function. J. Eng. Math. 1985, 19, 57–67. [Google Scholar] [CrossRef]
- Chen, X.B. Hydrodynamics in offshore and naval applications-Part I. In Proceedings of the Keynote Lecture of 6th International Conference on Hydrodynamics, Perth, WA, Australia, 24–26 November 2004. [Google Scholar]
- Linton, C. Rapidly convergent representations for Green’functions for Laplace’equation. Proc. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 1999, 455, 1767–1797. [Google Scholar] [CrossRef]
- Liu, Y.; Iwashita, H.; Hu, C. A calculation method for finite depth free-surface green function. Int. J. Nav. Archit. Ocean Eng. 2015, 7, 375–389. [Google Scholar] [CrossRef]
- Lee, C.H. WAMIT Theory Manual; Massachusetts Institute of Technology, Department of Ocean Engineering: Cambridge, MA, USA, 1995. [Google Scholar]
- Israeli, M.; Orszag, S.A. Approximation of radiation boundary conditions. J. Comput. Phys. 1981, 41, 115–135. [Google Scholar] [CrossRef]
- Filippas, E.S.; Belibassakis, K.A. Hydrodynamic analysis of flapping-foil thrusters operating beneath the free surface and in waves. Eng. Anal. Bound. Elem. 2014, 41, 47–59. [Google Scholar] [CrossRef]
- Zhang, X.; Beck, R.F. Computations for large-amplitude two-dimensional body motions. J. Eng. Math. 2007, 58, 177–189. [Google Scholar] [CrossRef]
- Shen, Y.M.; Zheng, Y.H.; You, Y.G. On the radiation and diffraction of linear water waves by a rectangular structure over a sill. Part I. Infinite domain of finite water depth. Ocean Eng. 2005, 32, 1073–1097. [Google Scholar] [CrossRef]
- Liu, X.; Wang, X.; Xu, S.; Ding, A. Influences of a varying sill at the seabed on two-dimensional radiation of linear water waves by a rectangular buoy. J. Offshore Mech. Arct. Eng. 2020, 142, 041202. [Google Scholar] [CrossRef]
- Feng, A.; Bai, W.; Price, W.G. Two-dimensional wave radiation and diffraction problems in a flat or sloping seabed environment. J. Fluids Struct. 2017, 75, 193–212. [Google Scholar] [CrossRef]
- Murai, M.; Inoue, Y.; Nakamura, T. The prediction method of hydroelastic response of VLFS with sea bottom topographical effects. In Proceedings of the Thirteenth International Offshore and Polar Engineering Conference, Honolulu, HI, USA, 25–30 May 2003. [Google Scholar]
- Buchner, B. The Motions of a Ship on a Sloped Seabed. In Proceedings of the 25th International Conference on Offshore Mechanics and Arctic Engineering, Hamburg, Germany, 4–9 June 2006; pp. 339–347. [Google Scholar]
- Ferreira, M.; Newman, J. Diffraction effects and ship motions on an artificial seabed. In Proceedings of the 24th International Workshop on Water Waves and Floating Bodies, Zelenogorsk, Russia, 19–22 April 2009. [Google Scholar]
- Hauteclocque, G.D.; Rezende, F.; Giorgiutti, Y.; Chen, X.B. Wave Kinematics and Seakeeping Calculation With Varying Bathymetry. In Proceedings of the ASME 2009 28th International Conference on Ocean, Offshore and Arctic Engineering, Honolulu, HI, USA, 31 May–5 June 2009. [Google Scholar]
- Belibassakis, K.A. A boundary element method for the hydrodynamic analysis of floating bodies in variable bathymetry regions. Eng. Anal. Bound. Elem. 2008, 32, 796–810. [Google Scholar] [CrossRef]
- Athanassoulis, G.A.; Belibassakis, K.A. A consistent coupled-mode theory for the propagation of small-amplitude water waves over variable bathymetry regions. J. Fluid Mech. 1999, 75, 275–301. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Athanassoulis, G.A. Three-dimensional Green’s function for harmonic water waves over a bottom topography with different depths at infinity. J. Fluid Mech. 2004, 510, 267–302. [Google Scholar] [CrossRef]
- Ding, J.; Tian, C.; Wu, Y.S.; Li, Z.W.; Ling, H.J.; Ma, X.Z. Hydroelastic analysis and model tests of a single module VLFS deployed near islands and reefs. Ocean Eng. 2017, 144, 224–234. [Google Scholar] [CrossRef]
- Kim, T.; Kim, Y. Numerical analysis on floating-body motion responses in arbitrary bathymetry. Ocean Eng. 2013, 62, 123–139. [Google Scholar] [CrossRef]
- Yang, P.; Li, Z.; Wu, Y.; Wen, W.; Ding, J.; Zhang, Z. Boussinesq-Hydroelasticity coupled model to investigate hydroelastic responses and connector loads of an eight-module VLFS near islands in time domain. Ocean Eng. 2019, 190, 106418. [Google Scholar] [CrossRef]
- Roseau, M. Asymptotic Wave Theory; Elsevier: Amsterdam, The Netherlands, 2012; Volume 20. [Google Scholar]
- Berkhoff, J. Computation of combined refraction—Diffraction. In Proceedings of the 13th International Conference on Coastal Engineering, Vancouver, BC, Canada, 10–14 July 1972. [Google Scholar]
- Kirby, J.T. A general wave equation for waves over rippled beds. J. Fluid Mech. 1986, 162, 171–186. [Google Scholar] [CrossRef]
- Newman, J. Propagation of water waves over an infinite step. J. Fluid Mech. 1965, 23, 399–415. [Google Scholar] [CrossRef]
- Liu, X.; Wang, X.; Xu, S. A DMM-EMM-RSM hybrid technique on two-dimensional frequency-domain hydroelasticity of floating structures over variable bathymetry. Ocean Eng. 2020, 201, 107135. [Google Scholar] [CrossRef]
- Zou, Z. Water Wave Theories and Their Applications; Chinese Science Press: Beijing, China, 2005. [Google Scholar]
- Massel, S.R. Extended refraction-diffraction equation for surface waves. Coast. Eng. 1993, 19, 97–126. [Google Scholar] [CrossRef]
- John, F. On the motion of floating bodies II. Simple harmonic motions. Commun. Pure Appl. Math. 1950, 3, 45–101. [Google Scholar] [CrossRef]
- Yang, P.; Gu, X.; Tian, C.; Cheng, X.; Ding, J. Numerical study of 3D pulsating source green function of finite water depth. In Proceedings of the ASME 2014 33rd International Conference on Ocean, Offshore and Arctic Engineering. American Society of Mechanical Engineers Digital Collection, San Francisco, CA, USA, 8–13 June 2014. [Google Scholar]
- Liu, Y.; Cong, P.; Gou, Y.; Yoshida, S.; Kashiwagi, M. Enhanced Endo’s approach for evaluating free-surface Green’s function with application to wave-structure interactions. Ocean Eng. 2020, 207, 107377. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Wang, X.; Wang, L.; Miao, Q. Numerical investigation on motion responses of a floating hemisphere over a sloping bottom. J. Offshore Mech. Arct. Eng. 2021, 143, 051903. [Google Scholar] [CrossRef]







| Parameter | Unit | Value |
|---|---|---|
| Length | m | 274 |
| Beam | m | 44.2 |
| Draft | m | 11 |
| Displacement | m3 | 97120 |
| Longitudinal center of gravity | m | −1.06 from midship |
| Vertical center of gravity | m | 5.3 above still water plane |
| Roll radius of gyration | m | 15.2 |
| Pitch radius of gyration | m | 68.5 |
| Parameter | Unit | Value |
|---|---|---|
| Diameter | m | 10 |
| Displacement | m3 | 261.8 |
| Vertical center of gravity | m | 0 |
| Roll radius of gyration | m | 3.16 |
| Pitch radius of gyration | m | 3.16 |
| Yaw radius of gyration | m | 3.16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Gu, K.; Qian, Z.; Ding, S.; Wang, K.; Wang, H.; Sun, C. A Simplified Method for the Evaluation of Floating-Body Motion Responses over a Sloping Bottom. J. Mar. Sci. Eng. 2024, 12, 756. https://doi.org/10.3390/jmse12050756
Liu X, Gu K, Qian Z, Ding S, Wang K, Wang H, Sun C. A Simplified Method for the Evaluation of Floating-Body Motion Responses over a Sloping Bottom. Journal of Marine Science and Engineering. 2024; 12(5):756. https://doi.org/10.3390/jmse12050756
Chicago/Turabian StyleLiu, Xiaolei, Kun Gu, Zhijia Qian, Sheng Ding, Kan Wang, Hao Wang, and Chen Sun. 2024. "A Simplified Method for the Evaluation of Floating-Body Motion Responses over a Sloping Bottom" Journal of Marine Science and Engineering 12, no. 5: 756. https://doi.org/10.3390/jmse12050756
APA StyleLiu, X., Gu, K., Qian, Z., Ding, S., Wang, K., Wang, H., & Sun, C. (2024). A Simplified Method for the Evaluation of Floating-Body Motion Responses over a Sloping Bottom. Journal of Marine Science and Engineering, 12(5), 756. https://doi.org/10.3390/jmse12050756






