The Formation of 2D Holograms of a Noise Source and Bearing Estimation by a Vector Scalar Receiver in the High-Frequency Band
Abstract
1. Introduction
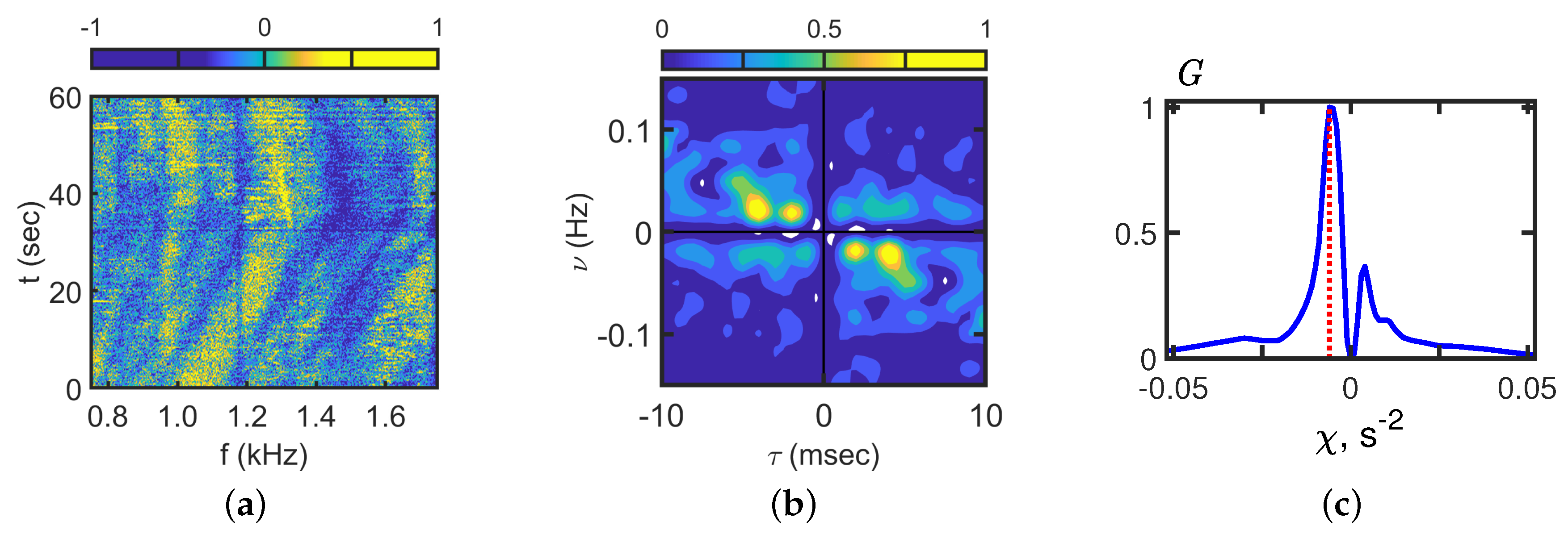
2. Holographic Method of Signal Processing in a Shallow-Water Waveguide
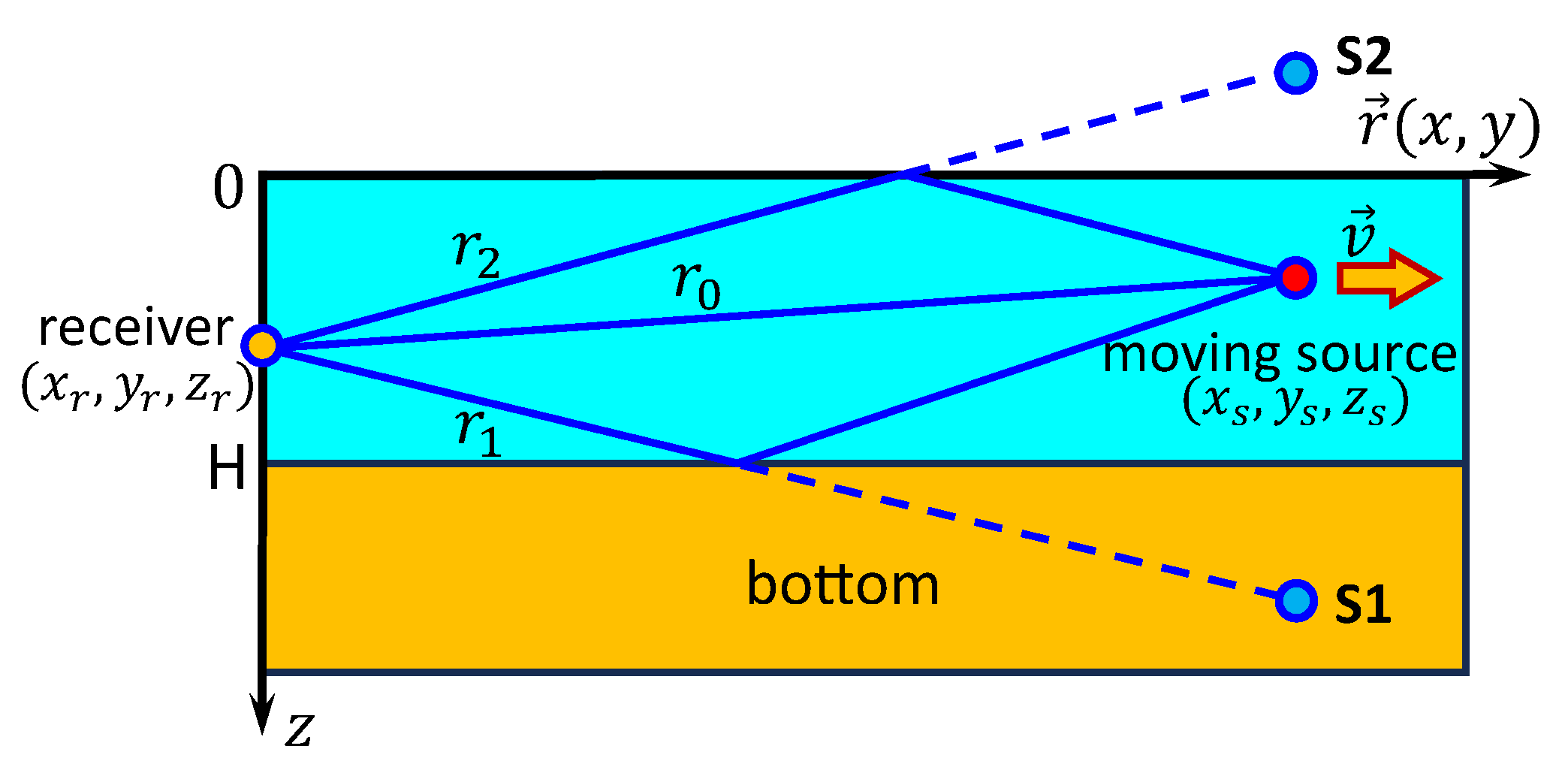
2.1. High-Frequency Sound Field of a Moving Source in Shallow Water
2.2. The 2D Interferograms of the Moving Source Formed by a Vector Scalar Receiver
2.3. The 2D Holograms of the Moving Source Formed by a Vector Scalar Receiver
2.4. Detection of the Moving Source
- If this condition is satisfied, the source is in the waveguide;
- If the condition is not satisfied, the source is absent.
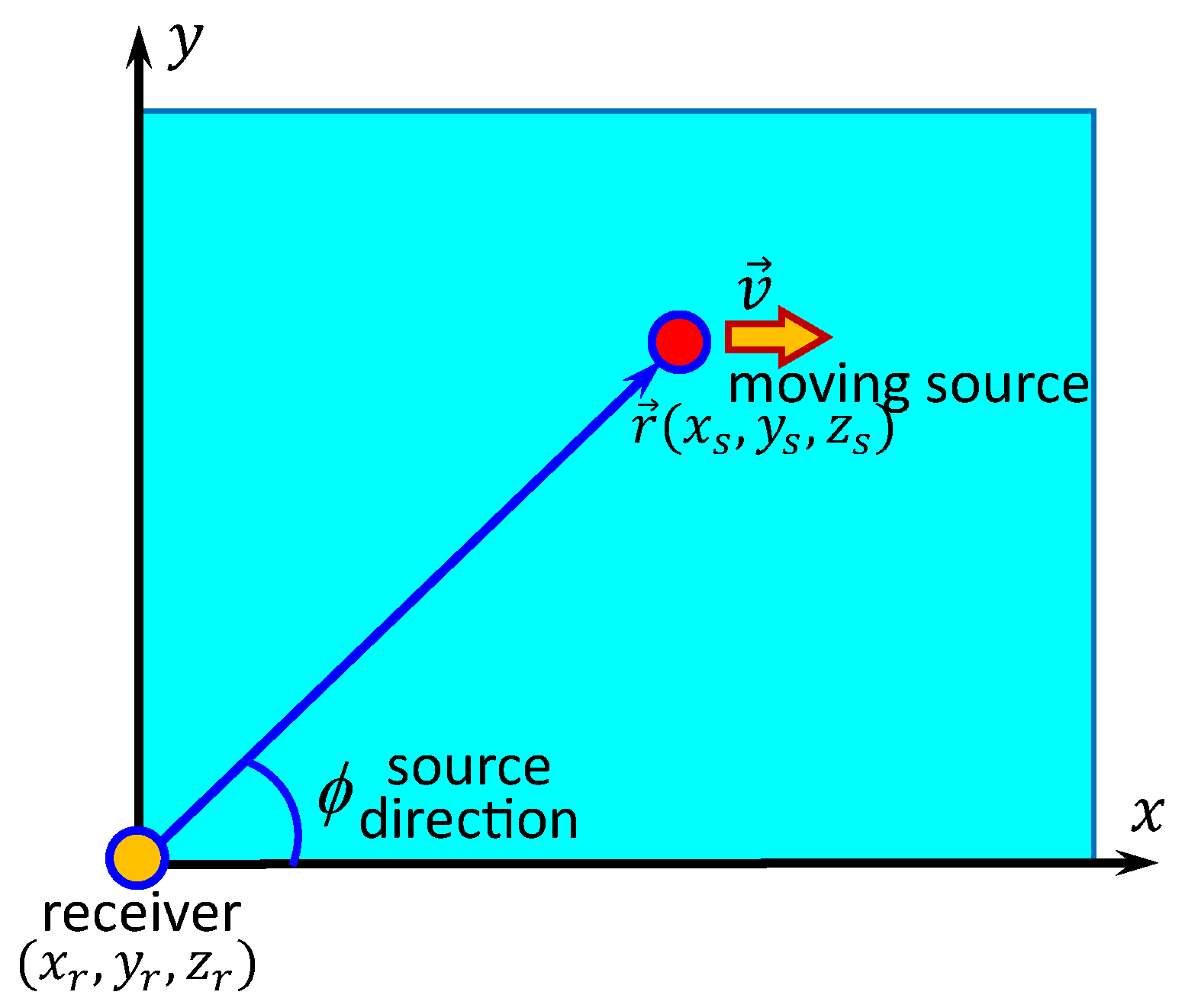
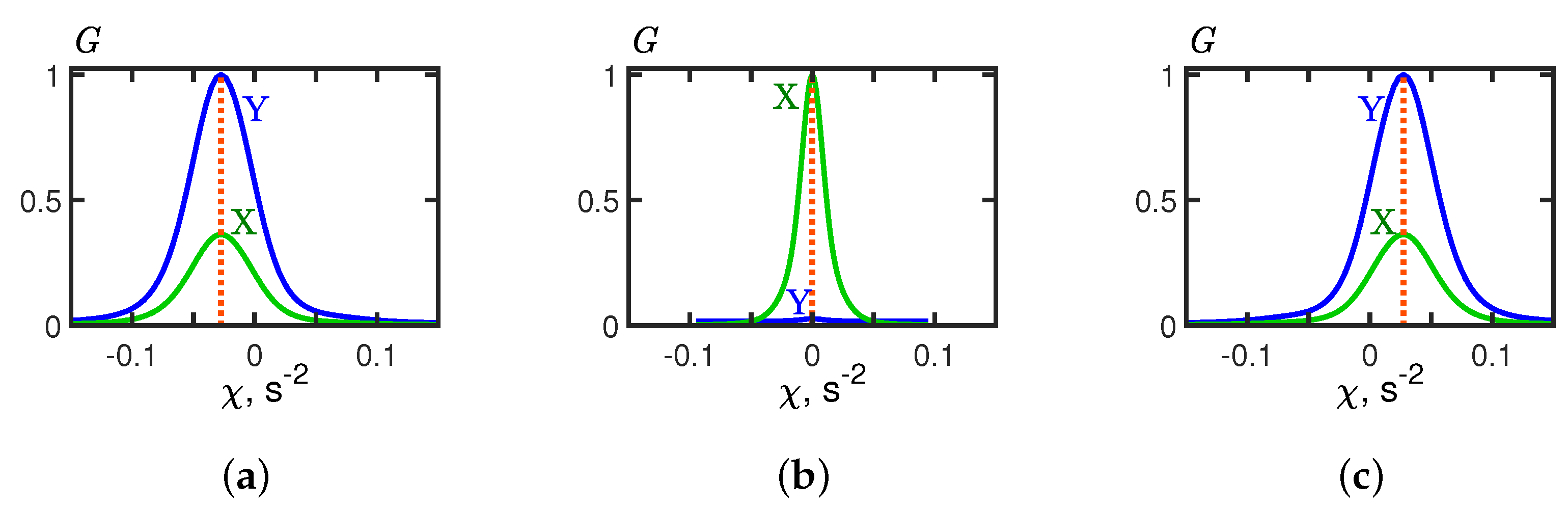
2.5. Source Bearing Estimation
- If , , then the source is located in the first quadrant of the VSR;
- If , , then the source is located in the second quadrant of the VSR;
- If , , then the source is located in the third quadrant of the VSR;
- If , , then the source is located in the fourth quadrant of the VSR.
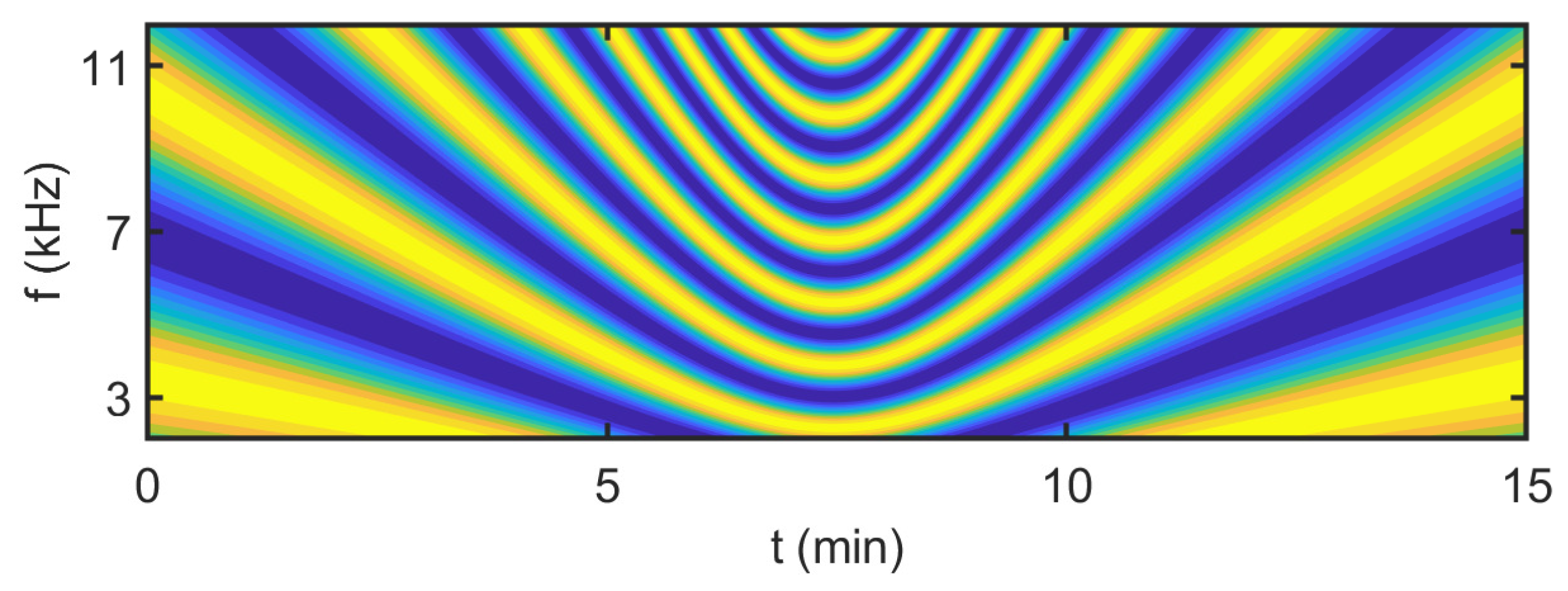
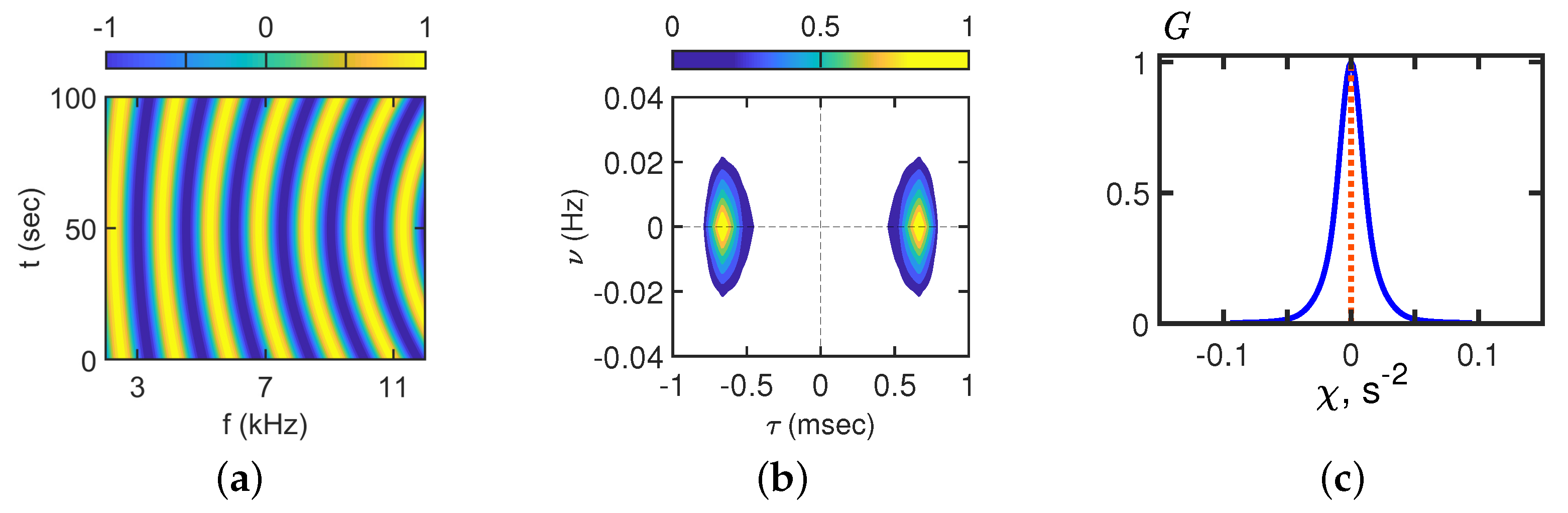
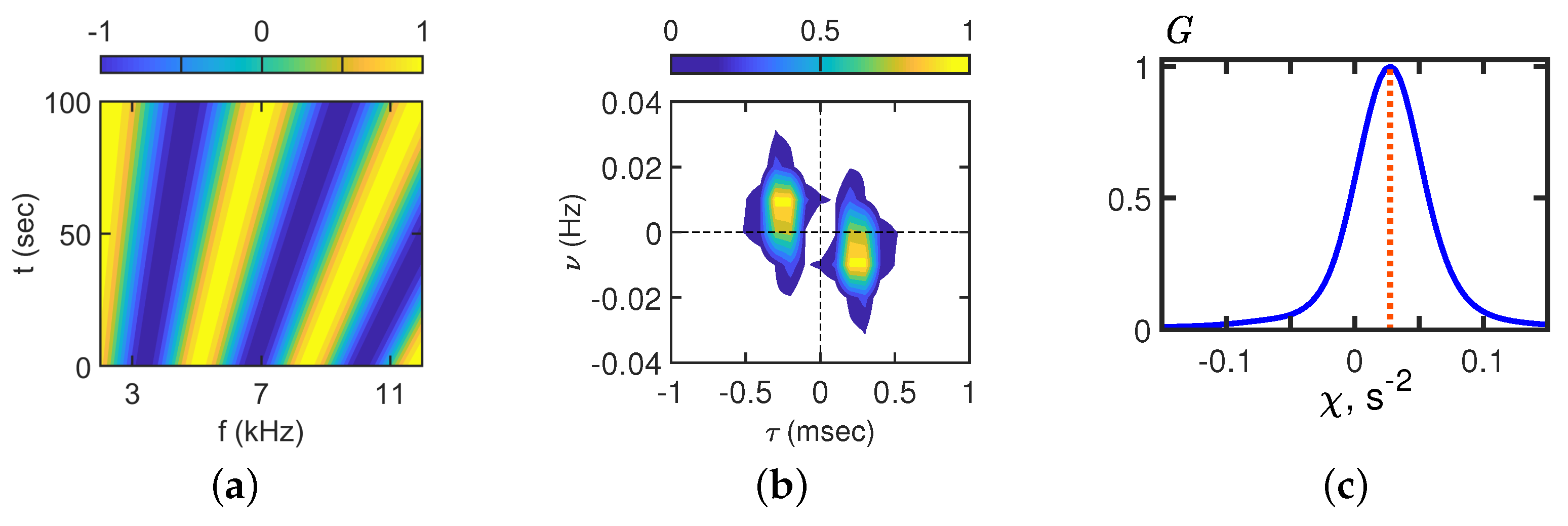
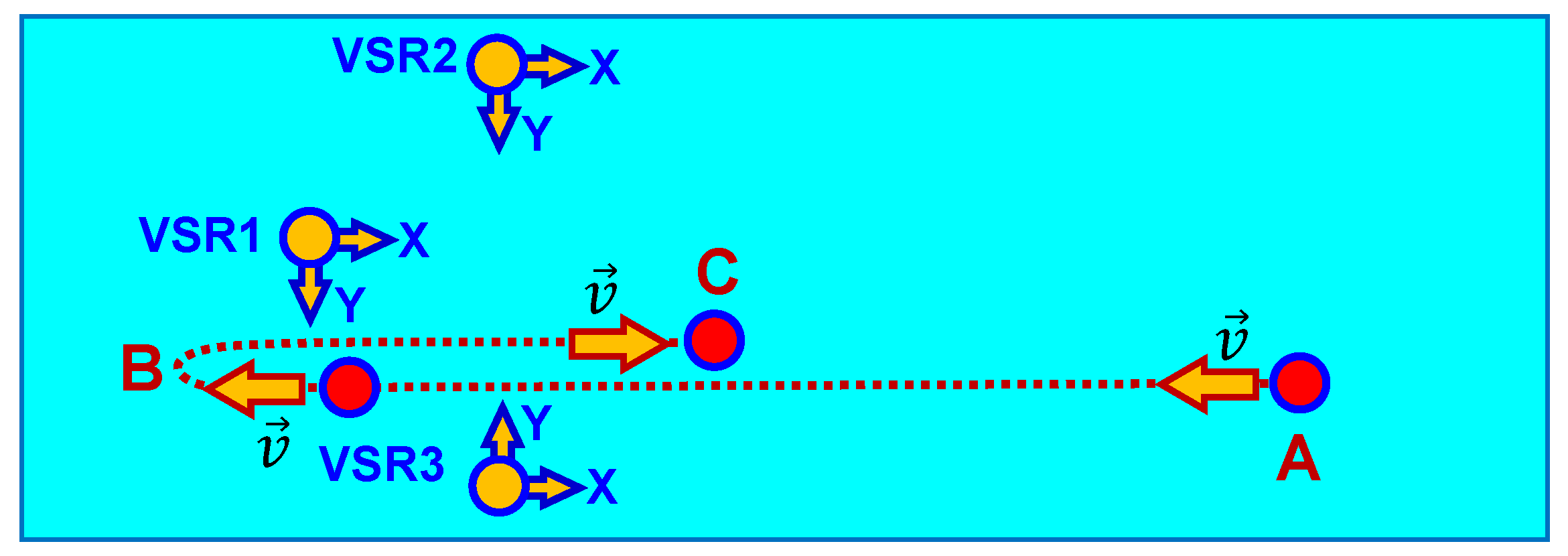
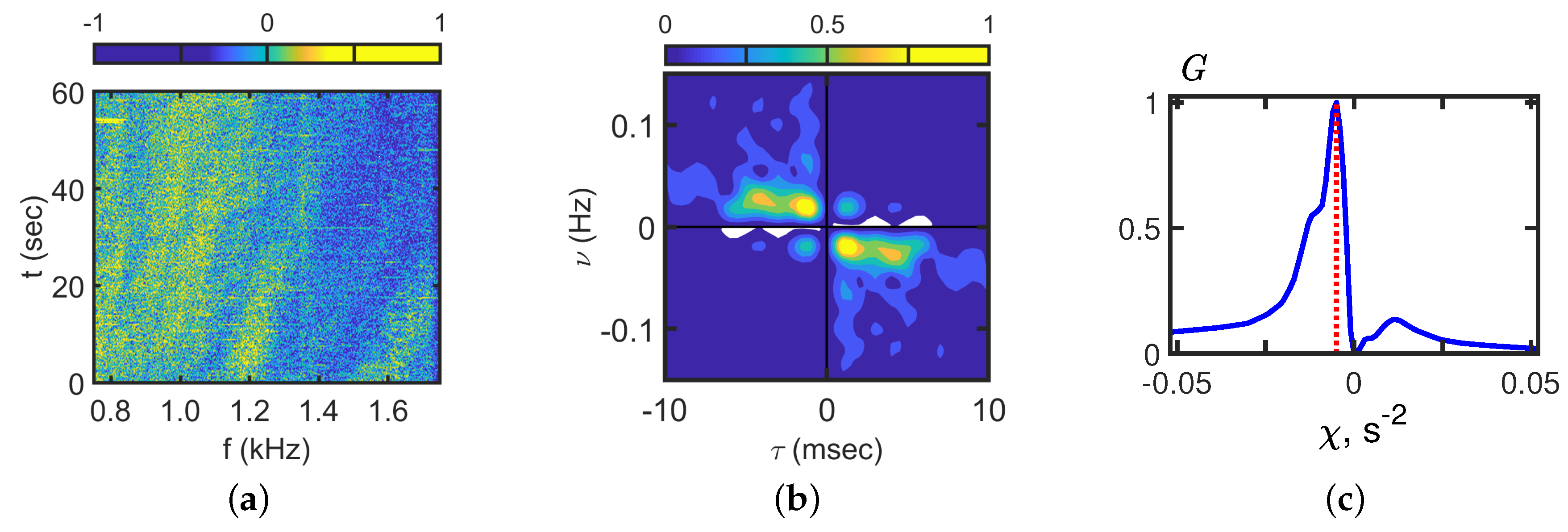
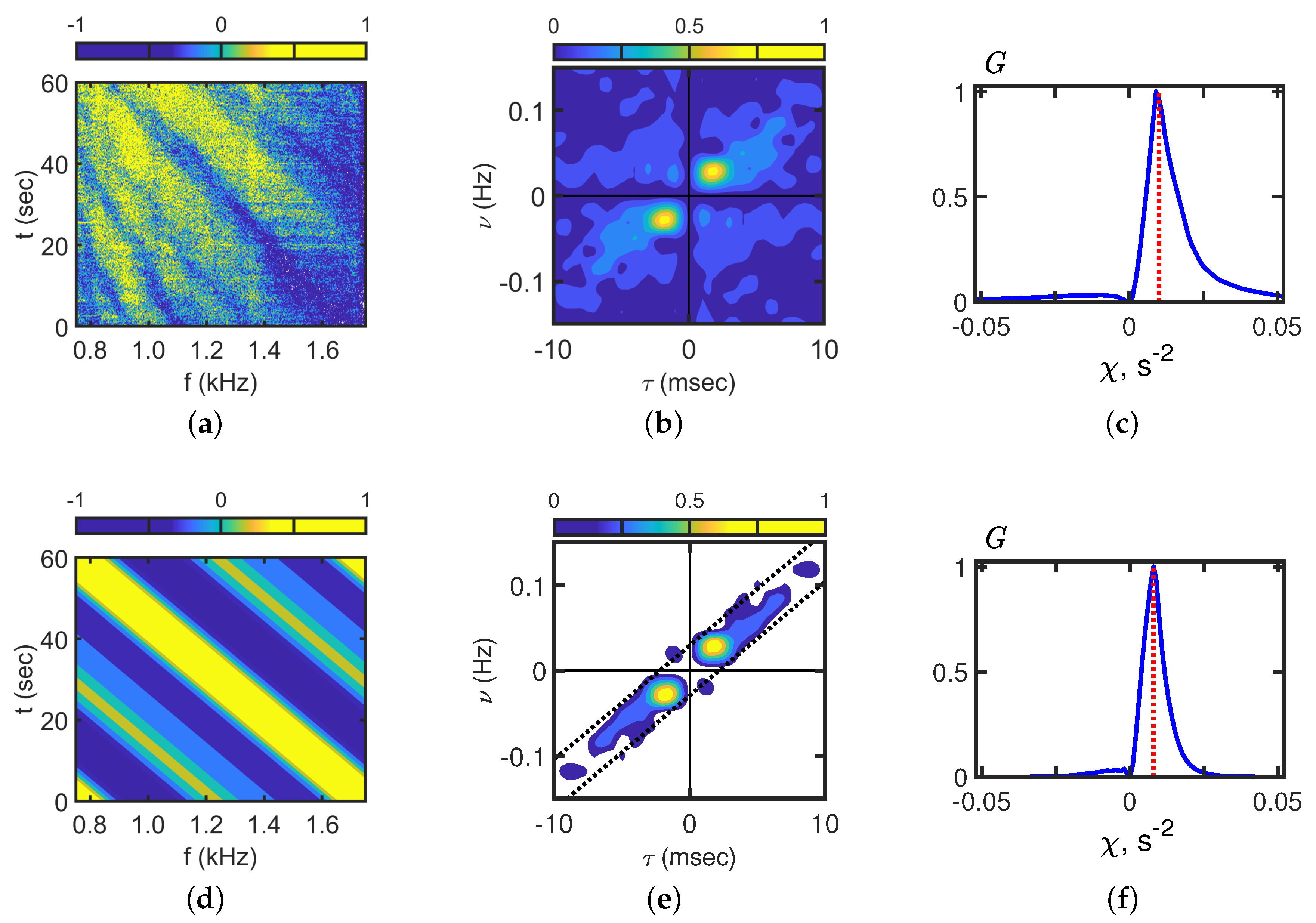
3. Numerical Simulation Results
- The receiver is located at point: ( m, m, m);
- The source velocity: m/s;
- The motion time: = 0–15 min;
- The motion starting point: A ( m, m, m), m;
- The motion traverse point: B ( m, m, m), m;
- The motion finish point: C ( m, m, m), m.
- The interferograms , , and have the same slope of the interference fringes as ;
- The focal points are on the same straight line with the same angular coefficients in the hologram domain of , , and as in the hologram domain of ;
- The angular distributions of the holograms , , and have an extreme value at the same points.
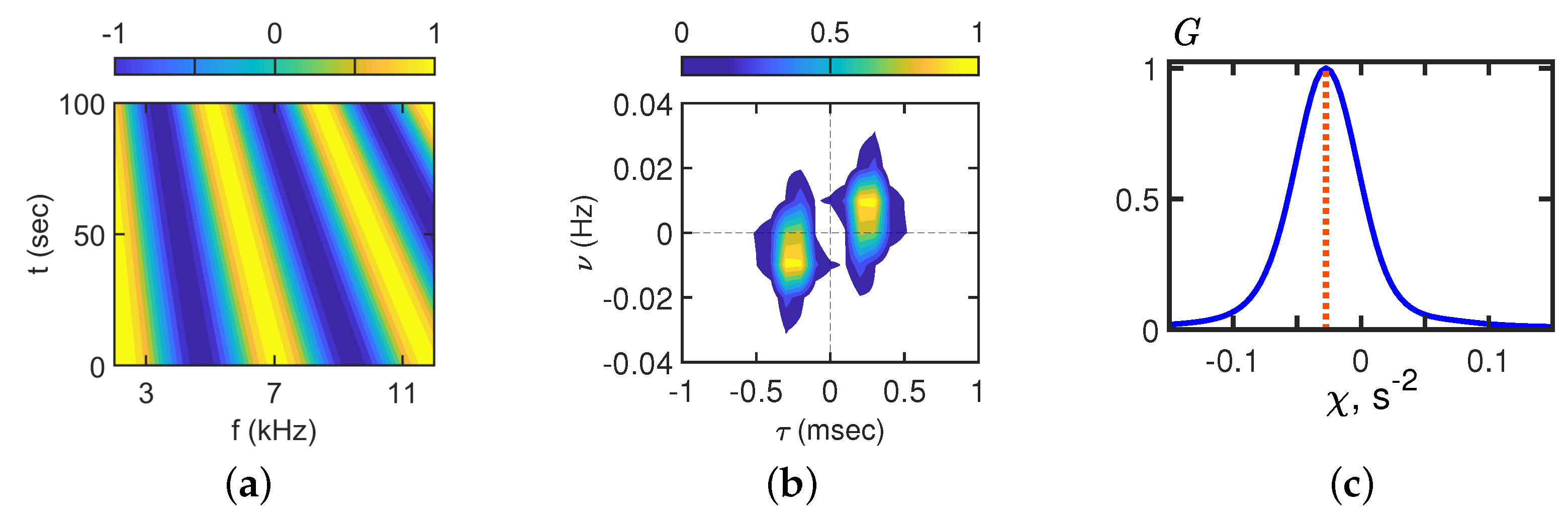
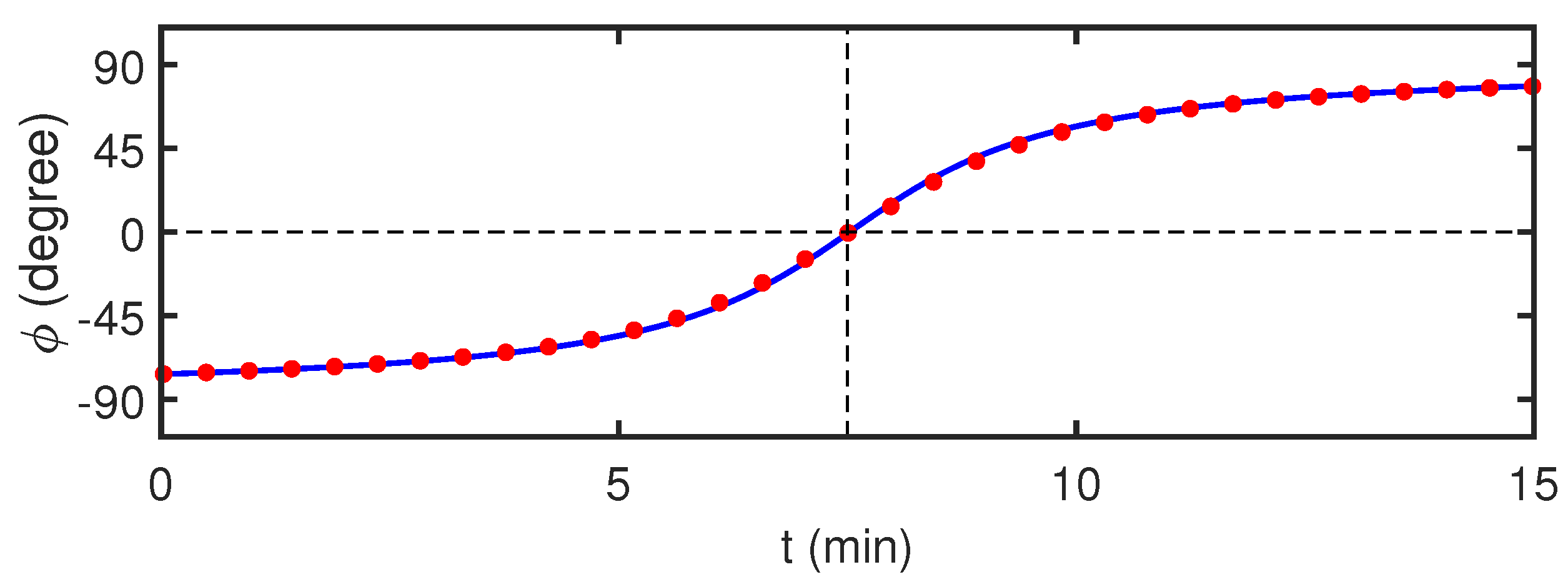
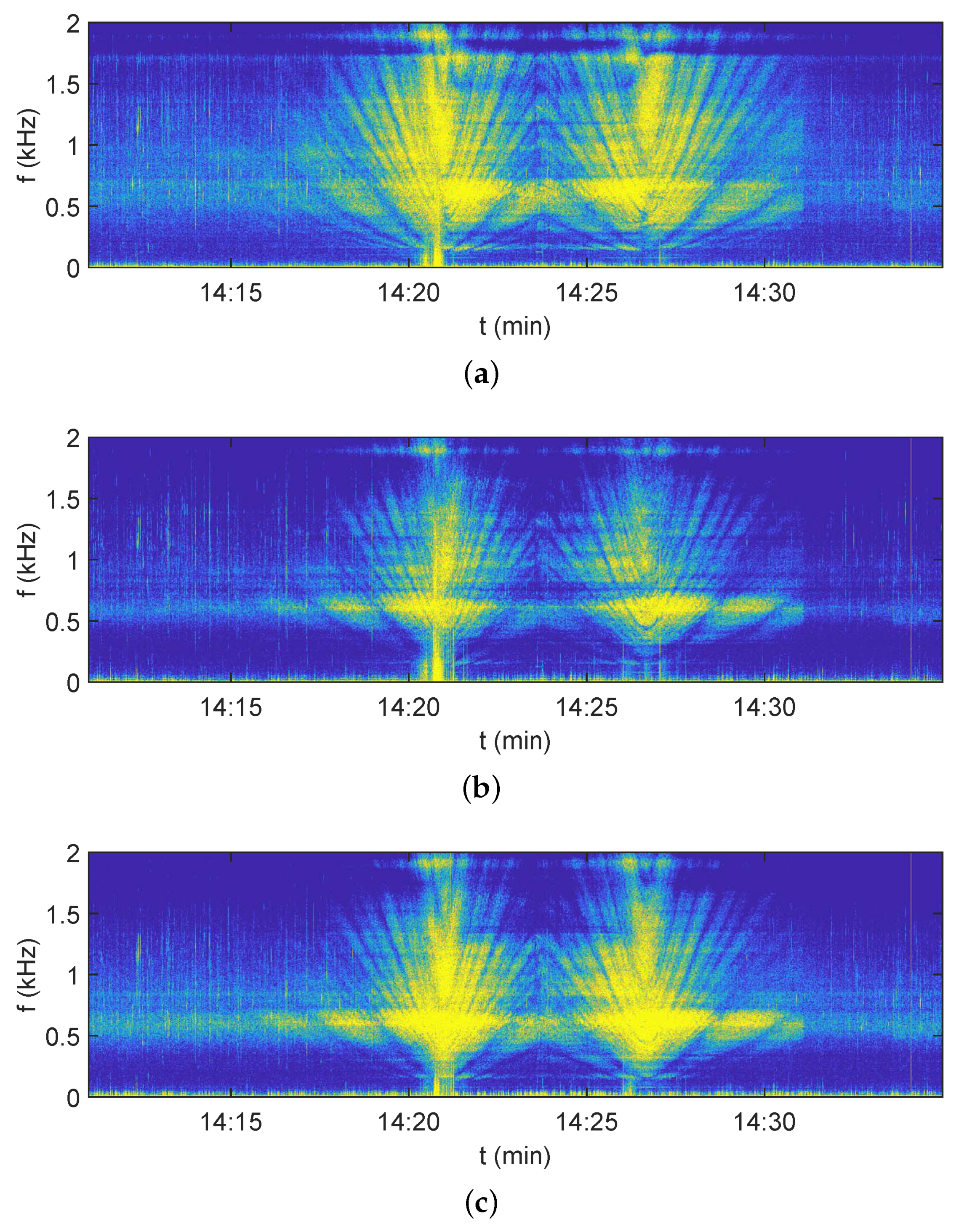
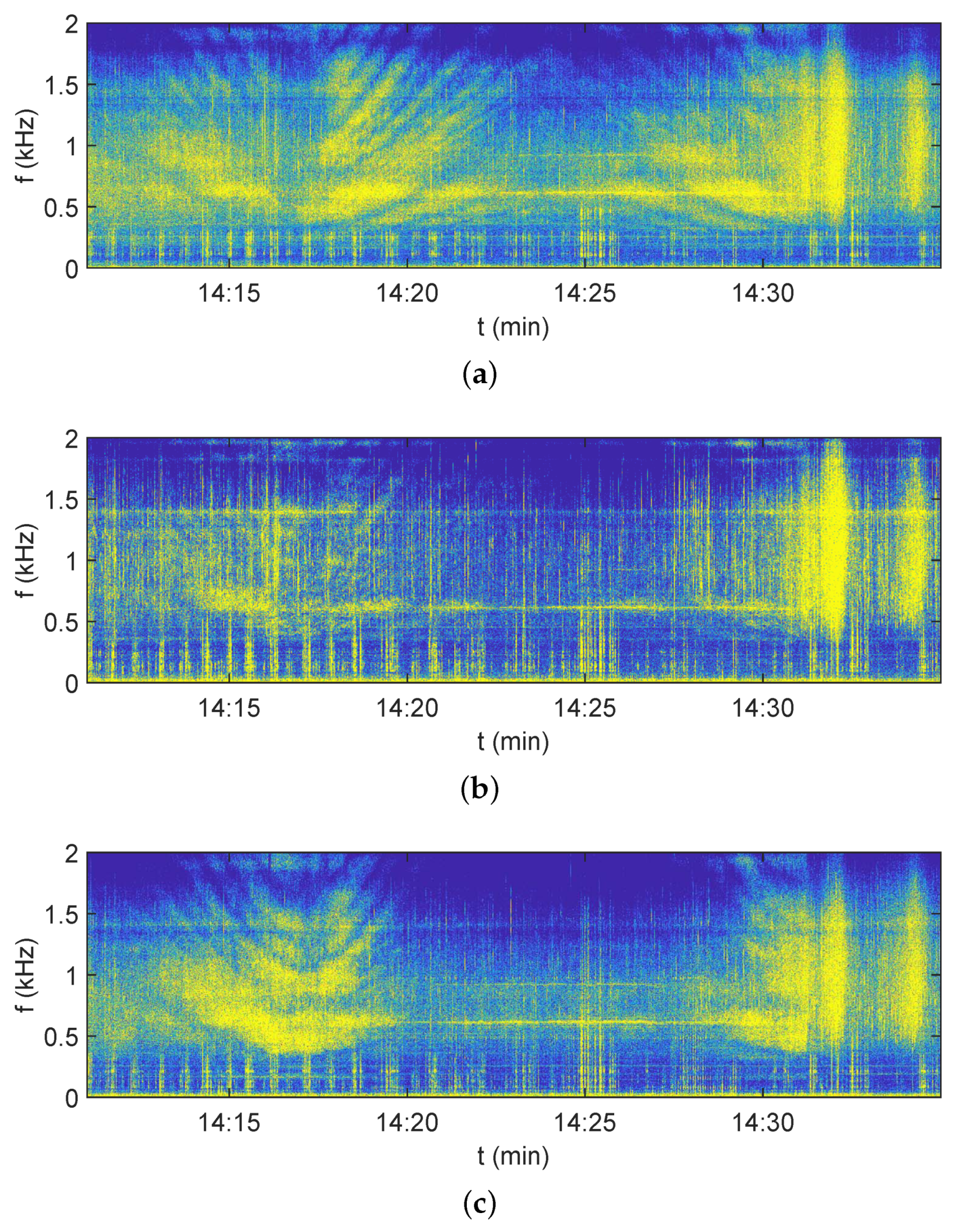
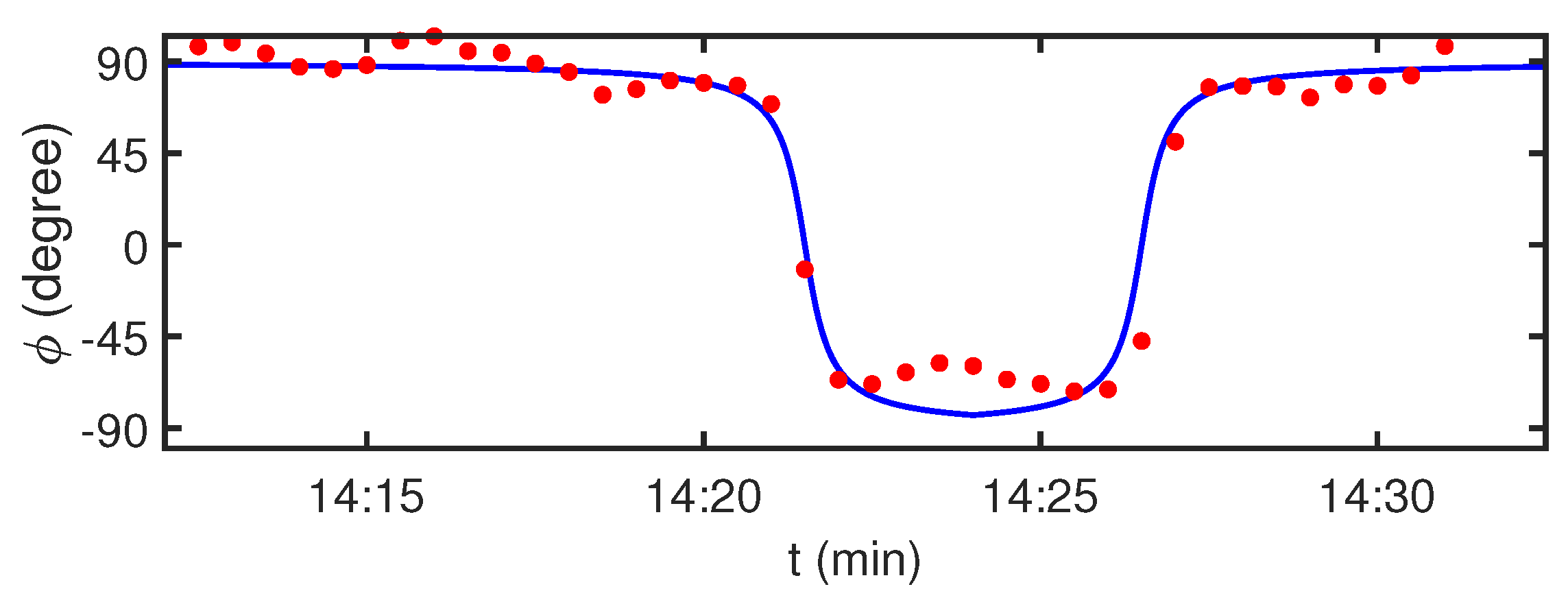
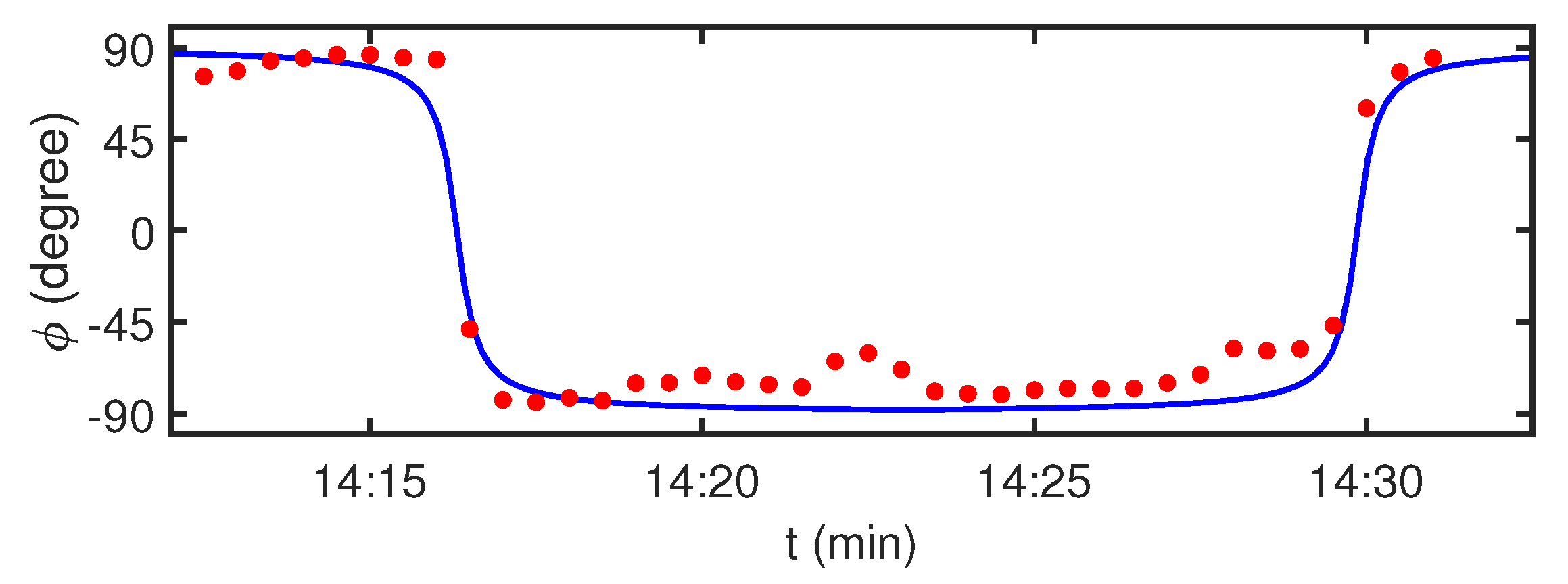
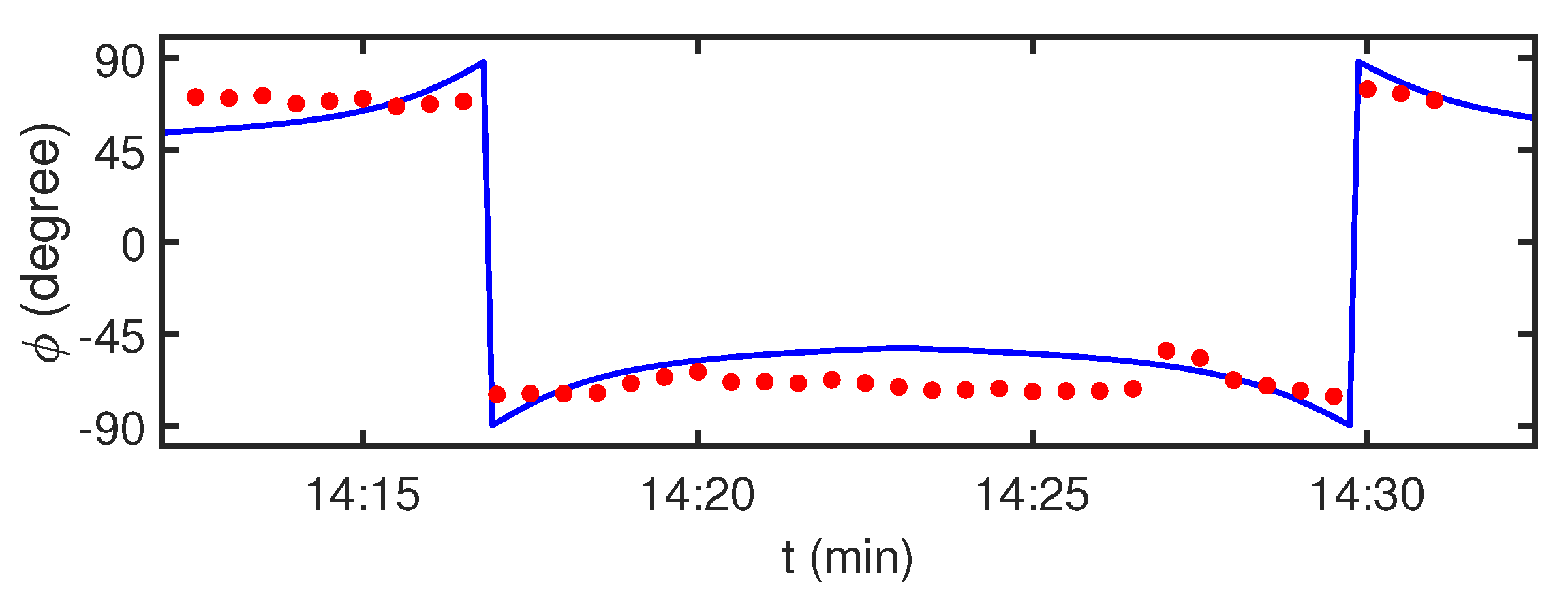
4. Experimental Results
- Motion time: = 0–18 min;
- Motion starting point: A;
- Motion start time: t = 14:13;
- Motion first track: along a straight line from point A to point B;
- Motion first track time: 14:13–14:24;
- Motion turning point: B;
- Motion turning time: 14:24;
- Motion second track: along a straight line from point B to point C;
- Motion second track time: 14:24–14:31;
- Motion finish point: C;
- Motion finish time: 14:31;
- Distance between point A and receiver VSR1 m;
- Distance between point A and receiver VSR2 m;
- Distance between point A and receiver VSR3 m;
- Distance between receiver VSR1 and receiver VSR2 m;
- Distance between receiver VSR1 and receiver VSR3 m;
- Distance between receiver VSR2 and receiver VSR3 m;
- Distance between receiver VSR1 and line connecting the receivers VSR2 and VSR3 m.
5. Conclusions
- The generation of 2D interferograms (, , , ) in the frequency-time domain ;
- The formatting of 2D holograms (, , , ) in the time-frequency domain ;
- The detection of the moving source using angular distributions (, , , ) of 2D holograms;
- The estimate of the bearing of the moving source by the ratio of the angular distributions of the 2D holograms (, ,).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| MFP | matched-field processing; |
| ISP | interferometric signal processing; |
| HSP | holographic signal processing; |
| VSR | vector scalar receiver; |
| VSRn | vector scalar receiver with number n; |
| 2D | two-dimensional; |
| 3D | three-dimensional; |
| 2D FT | two-dimensional Fourier transform. |
References
- Chuprov, S. Interference structure of a sound field in a layered ocean. Ocean Acoust. Curr. State 1982, 71–91. [Google Scholar]
- Weston, D.; Stevens, K. Interference of wide-band sound in shallow water. J. Sound Vibr. 1972, 21, 57–64. [Google Scholar] [CrossRef]
- Grachev, G.; Wood, J. Theory of acoustic field invariants in layered waveguides. Acoust. Phys. 1993, 39, 33–35. [Google Scholar]
- Orlov, E.F.; Sharonov, G.A. Interference of Sound Waves in the Ocean; Dal’nauka: Vladivostok, Russia, 1998. [Google Scholar]
- Ianniello, J. Recent developments in sonar signal processing. IEEE Signal Proc. Mag. 1998, 15, 27–40. [Google Scholar]
- Kuperman, W.A.; D’Spain, G.L. Ocean acoustic interference phenomena and signal processing. In Ocean Acoustic Interference Phenomena and Signal Processing 621; American Institute of Physics: San Francisco, CA, USA, 2002. [Google Scholar]
- Baggeroer, A.B.; Kuperman, W.A.; Schmidt, H. Matched field processing: Source localization in correlated noise as an optimum parameter estimation problem. J. Acoust. Soc. Am. 1998, 83, 571–587. [Google Scholar] [CrossRef]
- Jackson, D.R.; Ewart, T.E. The effect of internal waves on matched-field processing. J. Acoust. Soc. Am. 1994, 96, 2945–2955. [Google Scholar] [CrossRef]
- Dosso, S.E.; Nielsen, P.L.; Wilmut, M.J. Data error covariance in matched-field geoacoustic inversion. J. Acoust. Soc. Am. 2006, 119, 208–219. [Google Scholar] [CrossRef]
- Sazontov, A.G.; Malekhanov, A.I. Matched field signal processing in underwater sound channels. Acoust. Phys. 2015, 61, 213–230. [Google Scholar] [CrossRef]
- D’Spain, G.L.; Kuperman, W.A. Application of waveguide invariants to analysis of spectrograms from shallow water environments that vary in range and azimuth. J. Acoust. Soc. Am. 1999, 106, 2454–2468. [Google Scholar] [CrossRef]
- Heaney, K.; Cox, H. Rapid geoacoustic characterization for limiting environmental uncertainty for sonar system performance prediction. In Impact of Littoral Environmental Variability on Acoustic Predictions and Sonar Performance; Pace, N., Jensen, F.B., Eds.; Kluwer Academic: Dordrecht, The Netherlands, 2002; pp. 123–130. [Google Scholar]
- Thode, A.M. Source ranging with minimal environmental information using a virtual receiver and waveguide invariant theory. J. Acoust. Soc. Am. 2000, 108, 1582–1594. [Google Scholar] [CrossRef]
- Hodgkiss, W.; Song, H.; Kuperman, W.; Akal, T.; Ferla, C.; Jackson, D. A long-range and variable focus phase-conjugation experiment in a shallow water. J. Acoust. Soc. Am. 1999, 105, 1597–1604. [Google Scholar] [CrossRef]
- Thode, A.M.; Kuperman, W.A.; D’Spain, G.L.; Hodgkiss, W.S. Localization using Bartlett matched-field processor sidelobes. J. Acoust. Soc. Am. 2000, 107, 278–286. [Google Scholar] [CrossRef]
- Yang, T.C. Motion compensation for adaptive horizontal line array processing. J. Acoust. Soc. Am. 2003, 113, 245–260. [Google Scholar] [CrossRef]
- Yang, T.C. Beam intensity striations and applications. J. Acoust. Soc. Am. 2003, 113, 1342–1352. [Google Scholar] [CrossRef]
- Rouseff, D.; Leigh, C.V. Using the waveguide invariant to analyze Lofargrams. In Proceedings of the Oceans ’02 MTS/IEEE, Biloxi, MI, USA, 29–31 October 2002; Volume 4, pp. 2239–2243. [Google Scholar]
- Rouseff, D.; Spindel, R.C. Modeling the waveguide invariant as a distribution. AIP Conf. Proc. Amer. Inst. Phys. 2002, 621, 137–150. [Google Scholar]
- Baggeroer, A.B. Estimation of the distribution of the interference invariant with seismic streamers. AIP Conf. Proc. Amer. Inst. Phys. 2002, 621, 151–170. [Google Scholar]
- Heaney, K.D. Rapid geoacoustic characterization using a surface ship of opportunity. IEEE J. Ocean. Engrg. 2004, 29, 88–99. [Google Scholar] [CrossRef]
- Cockrell, K.L.; Schmidt, H. Robust passive range estimation using the waveguide invariant. J. Acoust. Soc. Am. 2010, 127, 2780–2789. [Google Scholar] [CrossRef]
- Rouseff, D.; Zurk, L.M. Striation-based beam forming for estimating the waveguide invariant with passive sonar. J. Acoust. Soc. Am. Express Lett. 2011, 130, 76–81. [Google Scholar] [CrossRef]
- Harrison, C.H. The relation between the waveguide invariant, multipath impulse response, and ray cycles. J. Acoust. Soc. Am. 2011, 129, 2863–2877. [Google Scholar] [CrossRef]
- Emmetiere, R.; Bonnel, J.; Gehant, M.; Cristol, X.; Chonavel, T. Understanding deep-water striation patterns and predicting the waveguide invariant as a distribution depending on range and depth. J. Acoust. Soc. Am. 2018, 143, 3444–3454. [Google Scholar] [CrossRef] [PubMed]
- Emmetiere, R.; Bonnel, J.; Cristol, X.; Gehant, M.; Chonavel, T. Passive source depth discrimination in deep-water. IEEE J. Select. Topics Signal Process. 2019, 13, 185–197. [Google Scholar] [CrossRef]
- Wang, N. Dispersionless transform and potential application in ocean acoustics. In Proceedings of the 10th Western Pacific Acoustics Conference, Beijing, China, 21–23 September 2009. [Google Scholar]
- Gao, D.; Wang, N. Dispersionless transform and signal enhancement application. In Proceedings of the 2th International Conference on Shallow Water Acoustic, Shanghai, China, 16–20 September 2009. [Google Scholar]
- Gao, D.; Wang, N. Dispersionless transform and signal enhancement application. In Proceedings of the 3th Oceanic Acoustics Conference, Beijing, China, 21–25 May 2012. [Google Scholar]
- Guo, X.; Yang, K.; Ma, Y.; Yang, Q. A source range and depth estimation method based on modal dedispersion transform. Acta Phys. Sin. 2016, 65, 214302. [Google Scholar]
- Zhang, S.; Zhang, Y.; Gao, S. Passive acoustic location with de-dispersive transform. In Proceedings of the 16th Western China Acoustics Conference, Leshan, China, 14–16 November 2016. [Google Scholar]
- Lee, S.; Makris, N.C. A new invariant method for instantaneous source range estimation in an ocean waveguide from passive beam-time intensity data. J. Acoust. Soc. Am. 2004, 116, 2646. [Google Scholar] [CrossRef]
- Lee, S.; Makris, N.C. The array invariant. J. Acoust. Soc. Am. 2006, 119, 336–351. [Google Scholar] [CrossRef]
- Lee, S. Efficient Localization in a Dispersive Waveguide: Applications in Terrestrial Continental Shelves and on Europa. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2006. [Google Scholar]
- Duan, R.; Yang, K.; Li, H.; Yang, Q.; Wu, F.; Ma, Y. A performance study of acoustic interference structure applications on source depth estimation in deep water. J. Acoust. Soc. Am. 2019, 145, 903–916. [Google Scholar] [CrossRef] [PubMed]
- Song, H.C.; Byun, G. Extrapolating Green’s functions using the waveguide invariant theory. J. Acoust. Soc. Am. 2020, 147, 2150–2158. [Google Scholar] [CrossRef] [PubMed]
- Byun, G.; Song, H.C. Adaptive array invariant. J. Acoust. Soc. Am. 2020, 148, 925–933. [Google Scholar] [CrossRef]
- Song, H.C.; Byun, G. An overview of array invariant for source-range estimation in shallow water. J. Acoust. Soc. Am. 2022, 151, 2336–2352. [Google Scholar] [CrossRef]
- Knobles, D.P.; Neilsen, T.B.; Wilson, P.S.; Hodgkiss, W.S.; Bonnel, J.; Lin, Y.T. Maximum entropy inference of seabed properties using waveguide invariant features from surface ships. J. Acoust. Soc. Am. 2022, 151, 2885–2896. [Google Scholar] [CrossRef]
- Yao, Y.; Sun, C.; Liu, X. Application of Waveguide Invariant Theory to Analysis of Interference Phenomenon in Deep Ocean. Acoustics 2020, 2, 595–604. [Google Scholar] [CrossRef]
- Kim, S.; Cho, S.; Jung, S.-K.; Choi, J.W. Passive Source Localization Using Acoustic Intensity in Multipath-Dominant Shallow-Water Waveguide. Sensors 2021, 21, 2198. [Google Scholar] [CrossRef] [PubMed]
- Su, X.; Qin, J.; Yu, X. Interference Pattern Anomaly of an Acoustic Field Induced by Bottom Elasticity in Shallow Water. J. Mar. Sci. Eng. 2023, 11, 647. [Google Scholar] [CrossRef]
- Li, X.; Sun, C. Source Depth Discrimination Using Intensity Striations in the Frequency–Depth Plane in Shallow Water with a Thermocline. Remote Sens. 2024, 16, 639. [Google Scholar] [CrossRef]
- Pang, J.; Gao, B. Application of a Randomized Algorithm for Extracting a Shallow Low-Rank Structure in Low-Frequency Reverberation. Remote Sens. 2023, 15, 3648. [Google Scholar] [CrossRef]
- Li, P.; Wu, Y.; Guo, W.; Cao, C.; Ma, Y.; Li, L.; Leng, H.; Zhou, A.; Song, J. Striation-Based Beamforming with Two-Dimensional Filtering for Suppressing Tonal Interference. J. Mar. Sci. Eng. 2023, 11, 2117. [Google Scholar] [CrossRef]
- Li, P.; Wu, Y.; Ma, Y.; Cao, C.; Leng, H.; Zhou, A.; Song, J. Prefiltered Striation-Based Beamforming for Range Estimation of Multiple Sources. J. Mar. Sci. Eng. 2023, 11, 1550. [Google Scholar] [CrossRef]
- Kuz’kin, V.M.; Pereselkov, S.A.; Kuznetsov, G.N. Spectrogram and localization of a sound source in a shallow sea. Acoust. Phys. 2017, 63, 449–461. [Google Scholar]
- Pereselkov, S.A.; Kuz’kin, V.M. Interferometric processing of hydroacoustic signals for the purpose of source localization. J. Acoust. Soc. Am. 2022, 151, 666–676. [Google Scholar] [CrossRef]
- Ehrhardt, M.; Pereselkov, S.A.; Kuz’kin, V.M.; Kaznacheev, I.; Rybyanets, P. Experimental observation and theoretical analysis of the low-frequency source interferogram and hologram in shallow water. J. Sound Vibr. 2023, 544, 117388. [Google Scholar] [CrossRef]
- Kuz’kin, V.M.; Pereselkov, S.A.; Kuznetsov, G.N.; Grigor’ev, V.A. Resolving power of the Interferometric method of source localization. Phys. Wave Phenom. 2018, 26, 150–159. [Google Scholar] [CrossRef]
- Kuz’kin, V.M.; Pereselkov, S.A.; Matvienko, Y.V.; Lyakhov, G.A.; Tkachenko, S.A. Noise source detection in an oceanic waveguide using interferometric processing. Phys. Wave Phenom. 2020, 28, 68–74. [Google Scholar] [CrossRef]
- Badiey, M.; Kuz’kin, V.M.; Pereselkov, S.A. Interferometry of hydrodynamics of oceanic shelf caused by intensive internal waves. Fundam. Appl. Hydrophys. 2020, 1, 45–55. [Google Scholar]
- Pereselkov, S.; Kuz’kin, V.; Ehrhardt, M.; Tkachenko, S.; Rybyanets, P.; Ladykin, N. Three-Dimensional Modeling of Sound Field Holograms of a Moving Source in the Presence of Internal Waves Causing Horizontal Refraction. J. Mar. Sci. Eng. 2023, 11, 1922. [Google Scholar] [CrossRef]
- Kuz’kin, V.M.; Matvienko, Y.V.; Pereselkov, S.A.; Prosovetskii, D.Y.; Kaznacheeva, E.S. Mode Selection in Oceanic Waveguides. Phys. Wave Phenom. 2022, 30, 111–118. [Google Scholar] [CrossRef]
- Pereselkov, S.; Kuz’kin, V.; Lyakhov, G.; Tkachenko, S.; Kaznacheeva, E. Adaptive Algorithms for Interferometric Processing. Phys. Wave Phenom. 2020, 28, 267–273. [Google Scholar]
- Pereselkov, S.A.; Kuz’kin, V.M.; Kaznacheev, I.V.; Kutsov, M.V.; Lyakhov, G.A. Interferometry in Acoustic Information Processing by Using Extended Antennas and Space-Time Analogy. Phys. Wave Phenom. 2020, 28, 326–332. [Google Scholar]
- Gordienko, V.A. Vector-Phase Methods in Acoustics; FIZMATLIT: Moscow, Russia, 2007. (In Russian) [Google Scholar]
- Nehorai, A.; Paldi, E. Acoustic vector sensor array processing. J. Acoust. Soc. Am. 1994, 51, 1479–1491. [Google Scholar] [CrossRef]
- Cao, J.; Liu, J.; Wang, J.; Lai, X. Acoustic vector sensor: Reviews and future perspectives. IET Signal Process. 2017, 11, 1–9. [Google Scholar] [CrossRef]
- Shi, J.; Dosso, S.E.; Sun, D.; Liu, Q. Geoacoustic inversion of the acoustic-pressure vertical phase gradient from a single vector sensor. J. Acoust. Soc. Am. 2019, 146, 3159–3173. [Google Scholar] [CrossRef]
- Wang, W.; Li, X.; Zhang, K.; Shi, J.; Shi, W.; Ali, W. Robust Direction Finding via Acoustic Vector Sensor Array with Axial Deviation under Non-Uniform Noise. J. Mar. Sci. Eng. 2022, 10, 1196. [Google Scholar] [CrossRef]
- Bozzi, F.A.; Jesus, S.M. Vector Sensor Steering-Dependent Performance in an Underwater Acoustic Communication Field Experiment. Sensors 2022, 22, 8332. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Zhang, G.; Wang, R.; Rong, H.; Yang, B. Acoustic Vector Sensor Multi-Source Detection Based on Multimodal Fusion. Sensors 2023, 23, 1301. [Google Scholar] [CrossRef] [PubMed]
- Rashid, R.; Zhang, E.; Abdi, A. Underwater Acoustic Signal Acquisition and Sensing Using a Ring Vector Sensor Communication Receiver: Theory and Experiments. Sensors 2023, 23, 6917. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Da, L.; Wang, C.; Yuan, M.; Zhang, Y.; Zhuo, J. Passive ranging of a moving target in the direct-arrival zone in deep sea using a single vector hydrophone. J. Acoust. Soc. Am. 2023, 154, 2426–2439. [Google Scholar] [CrossRef]
- Qiao, G.; Liu, Q.; Liu, S.; Muhammad, B.; Wen, M. Symmetric Connectivity of Underwater Acoustic Sensor Networks Based on Multi-Modal Directional Transducer. Sensors 2021, 21, 6548. [Google Scholar] [CrossRef]
- Jensen, F.B.; Kuperman, W.A.; Porter, M.B.; Schmidt, H. Computational Ocean Acoustics; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Brekhovskikh, L.M.; Lysanov, Y.P. Fundamentals of Ocean Acoustics; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pereselkov, S.; Kuz’kin, V.; Ehrhardt, M.; Matvienko, Y.; Tkachenko, S.; Rybyanets, P. The Formation of 2D Holograms of a Noise Source and Bearing Estimation by a Vector Scalar Receiver in the High-Frequency Band. J. Mar. Sci. Eng. 2024, 12, 704. https://doi.org/10.3390/jmse12050704
Pereselkov S, Kuz’kin V, Ehrhardt M, Matvienko Y, Tkachenko S, Rybyanets P. The Formation of 2D Holograms of a Noise Source and Bearing Estimation by a Vector Scalar Receiver in the High-Frequency Band. Journal of Marine Science and Engineering. 2024; 12(5):704. https://doi.org/10.3390/jmse12050704
Chicago/Turabian StylePereselkov, Sergey, Venedikt Kuz’kin, Matthias Ehrhardt, Yurii Matvienko, Sergey Tkachenko, and Pavel Rybyanets. 2024. "The Formation of 2D Holograms of a Noise Source and Bearing Estimation by a Vector Scalar Receiver in the High-Frequency Band" Journal of Marine Science and Engineering 12, no. 5: 704. https://doi.org/10.3390/jmse12050704
APA StylePereselkov, S., Kuz’kin, V., Ehrhardt, M., Matvienko, Y., Tkachenko, S., & Rybyanets, P. (2024). The Formation of 2D Holograms of a Noise Source and Bearing Estimation by a Vector Scalar Receiver in the High-Frequency Band. Journal of Marine Science and Engineering, 12(5), 704. https://doi.org/10.3390/jmse12050704






