A Spatial Correlation Identification Model for Coherent Structure Extraction and Three-Dimensional Visualization
Abstract
1. Introduction
2. Materials and Methods
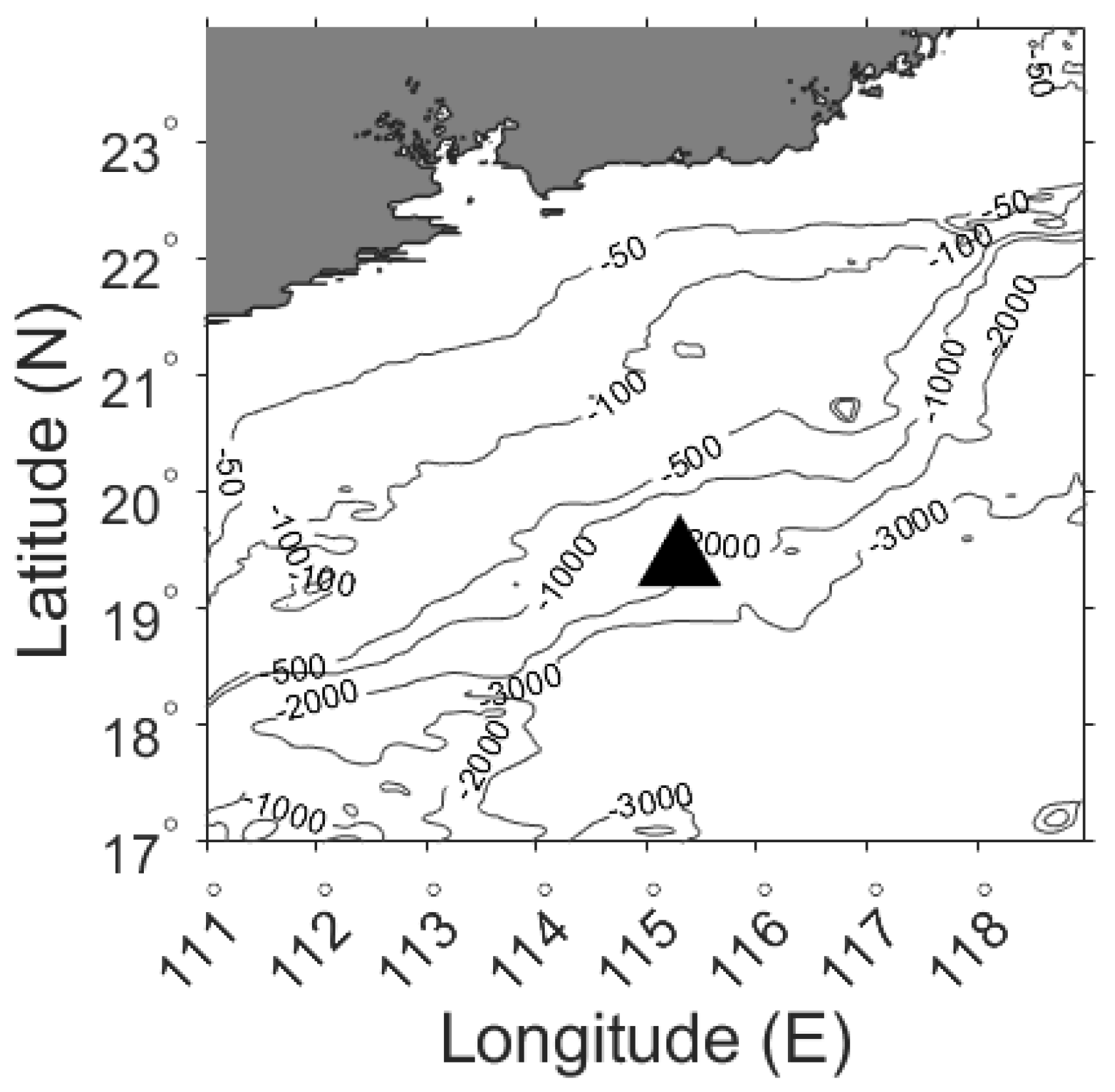
2.1. Measurements
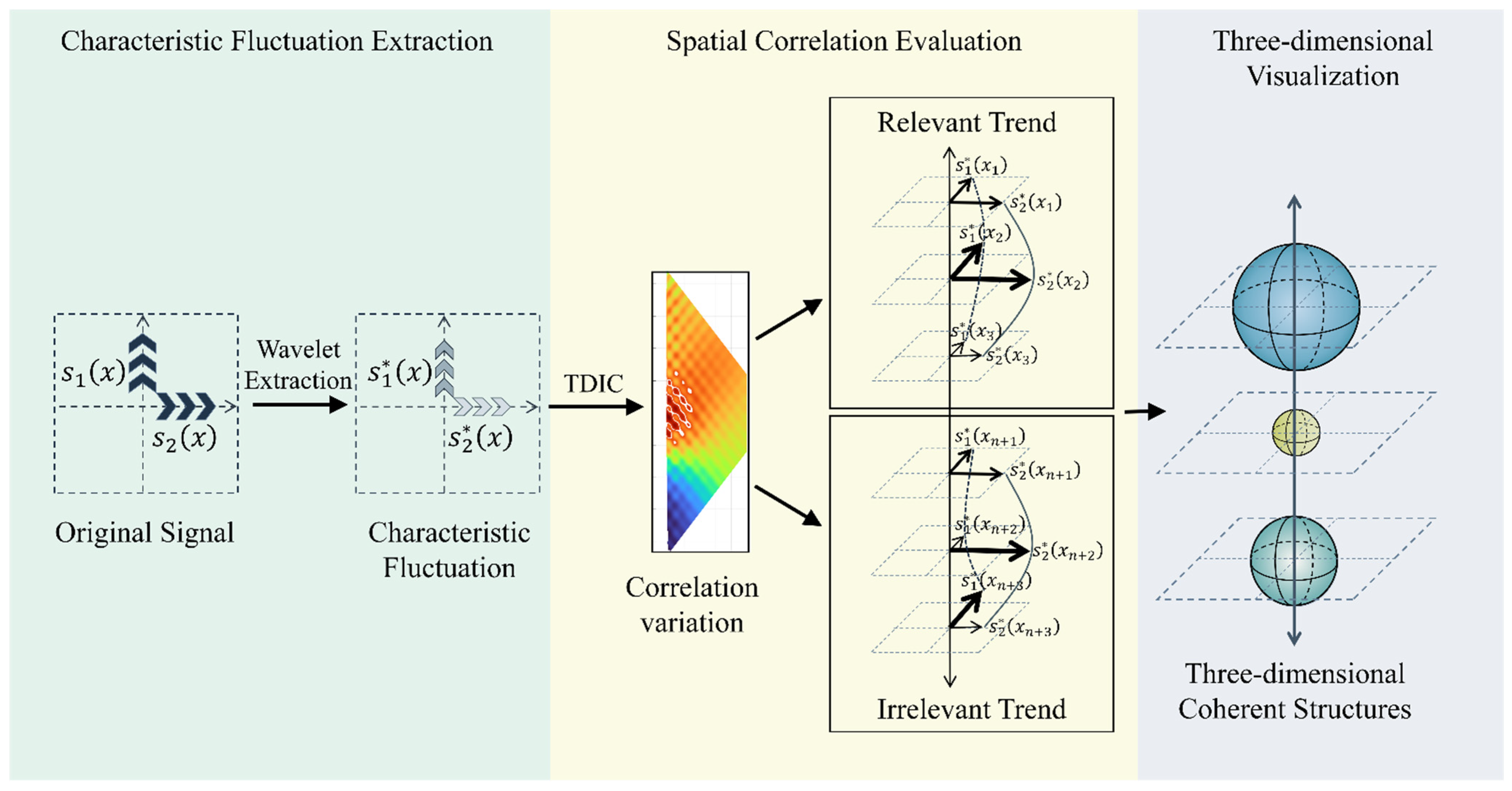
2.2. Methods
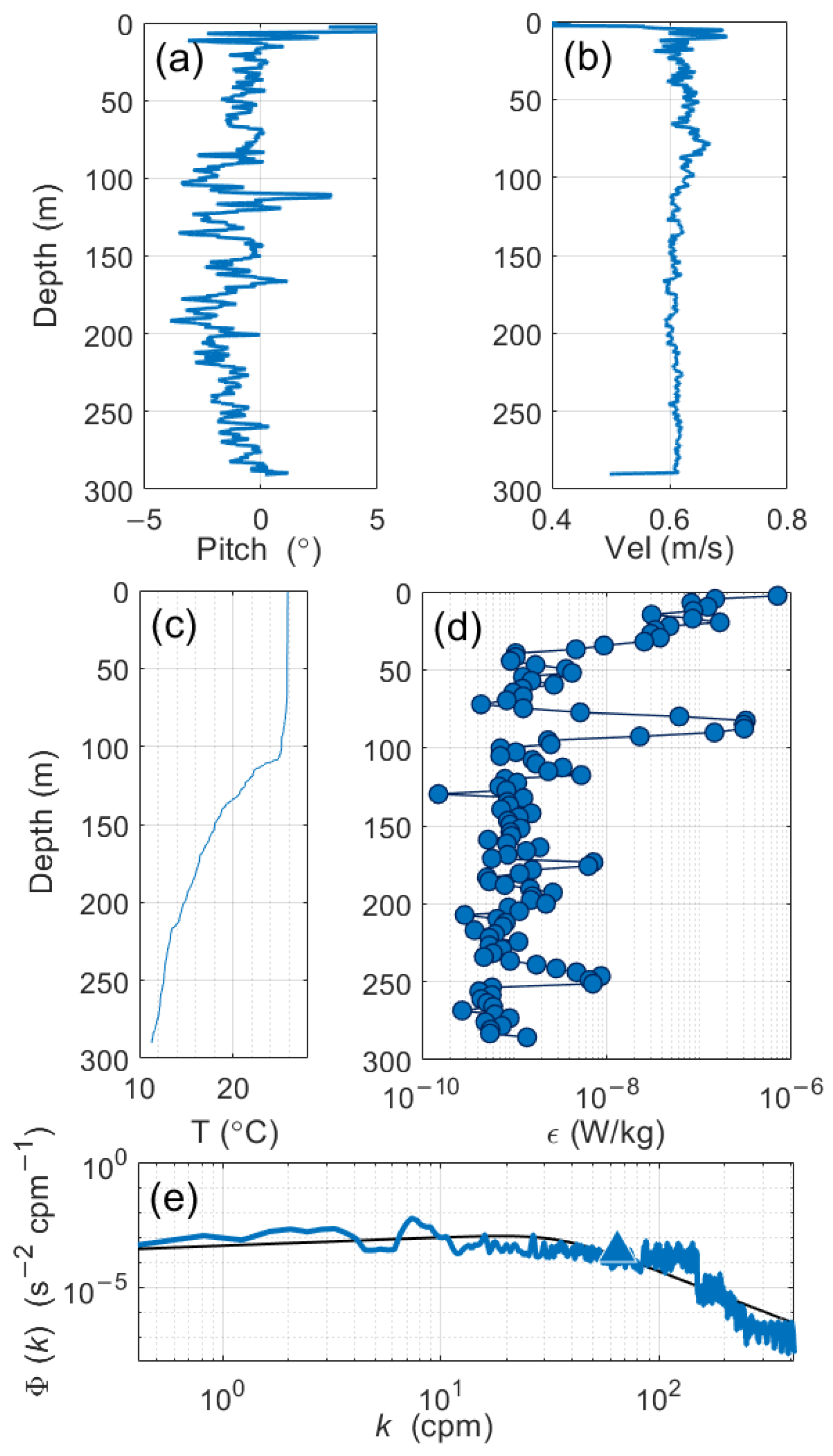
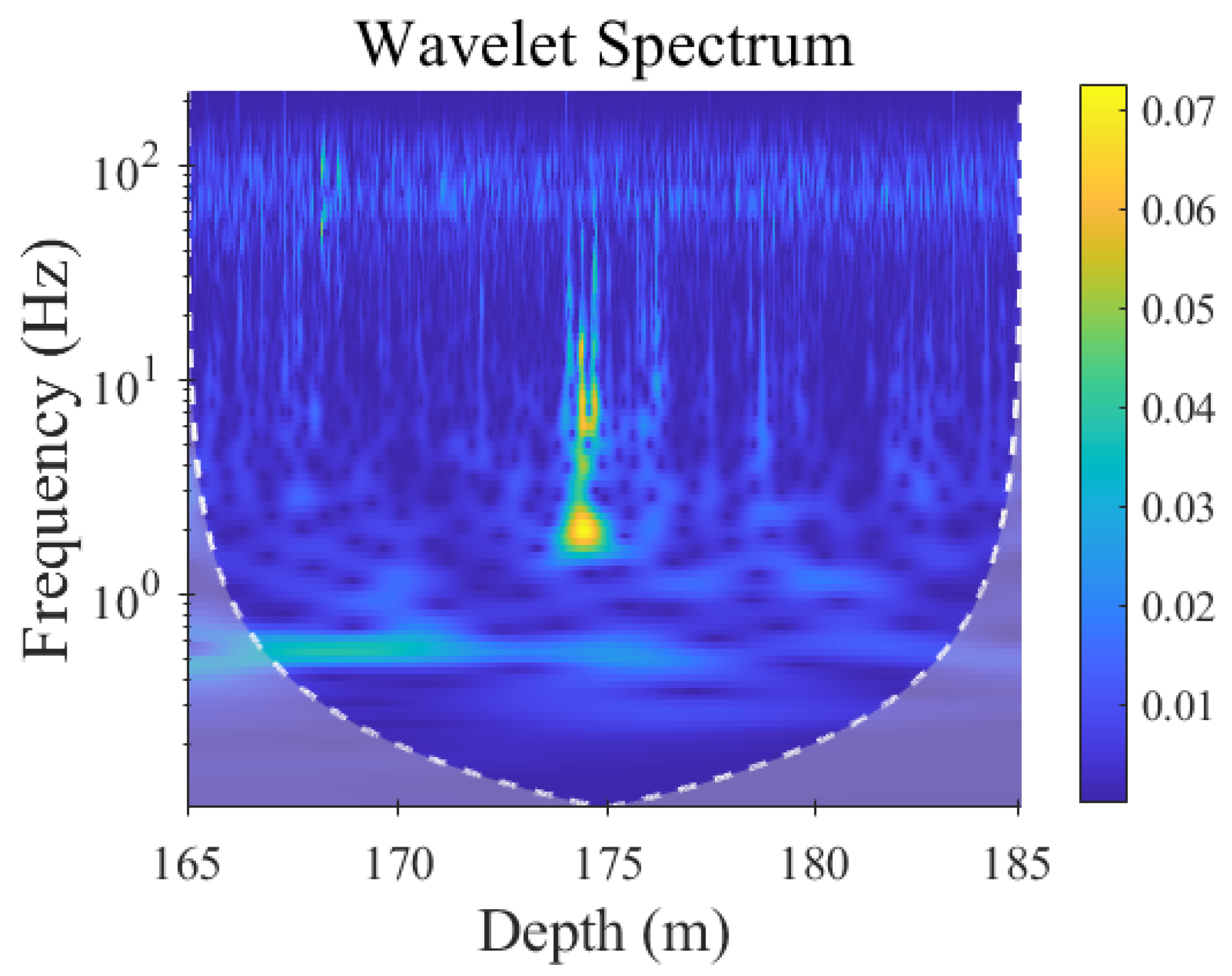
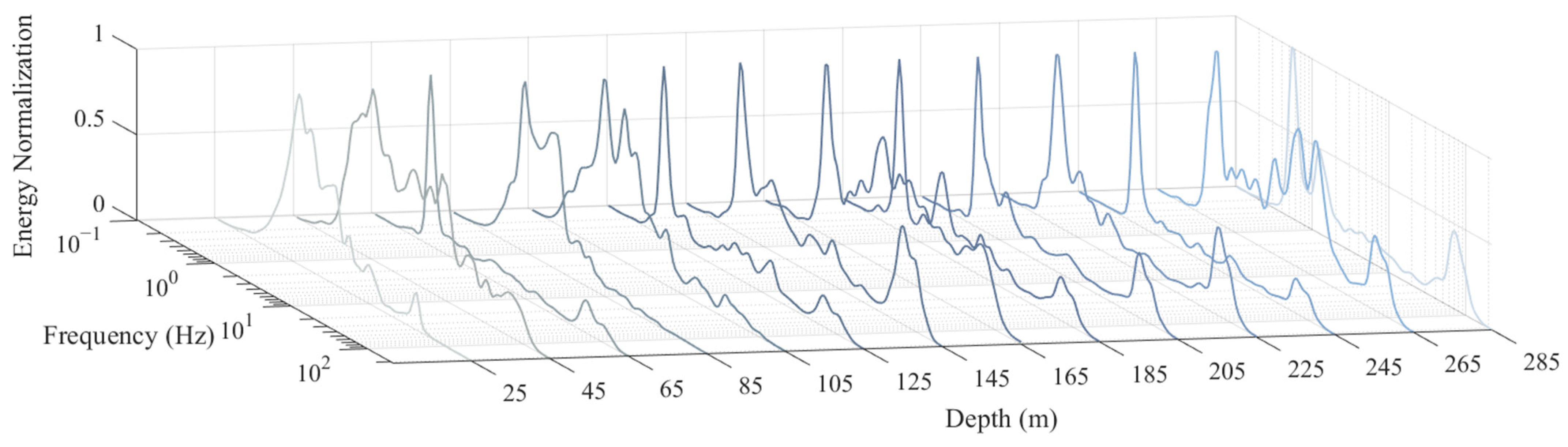
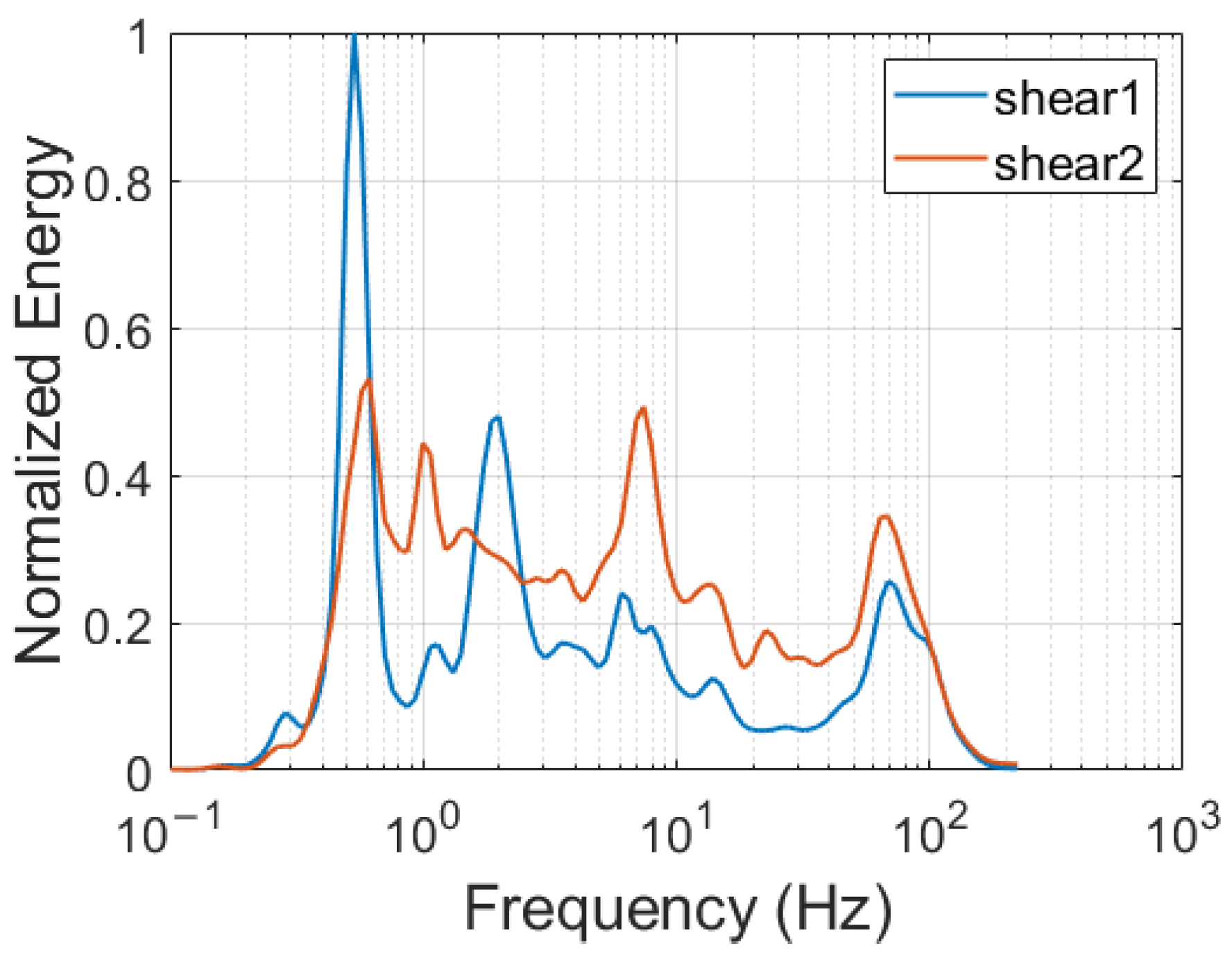
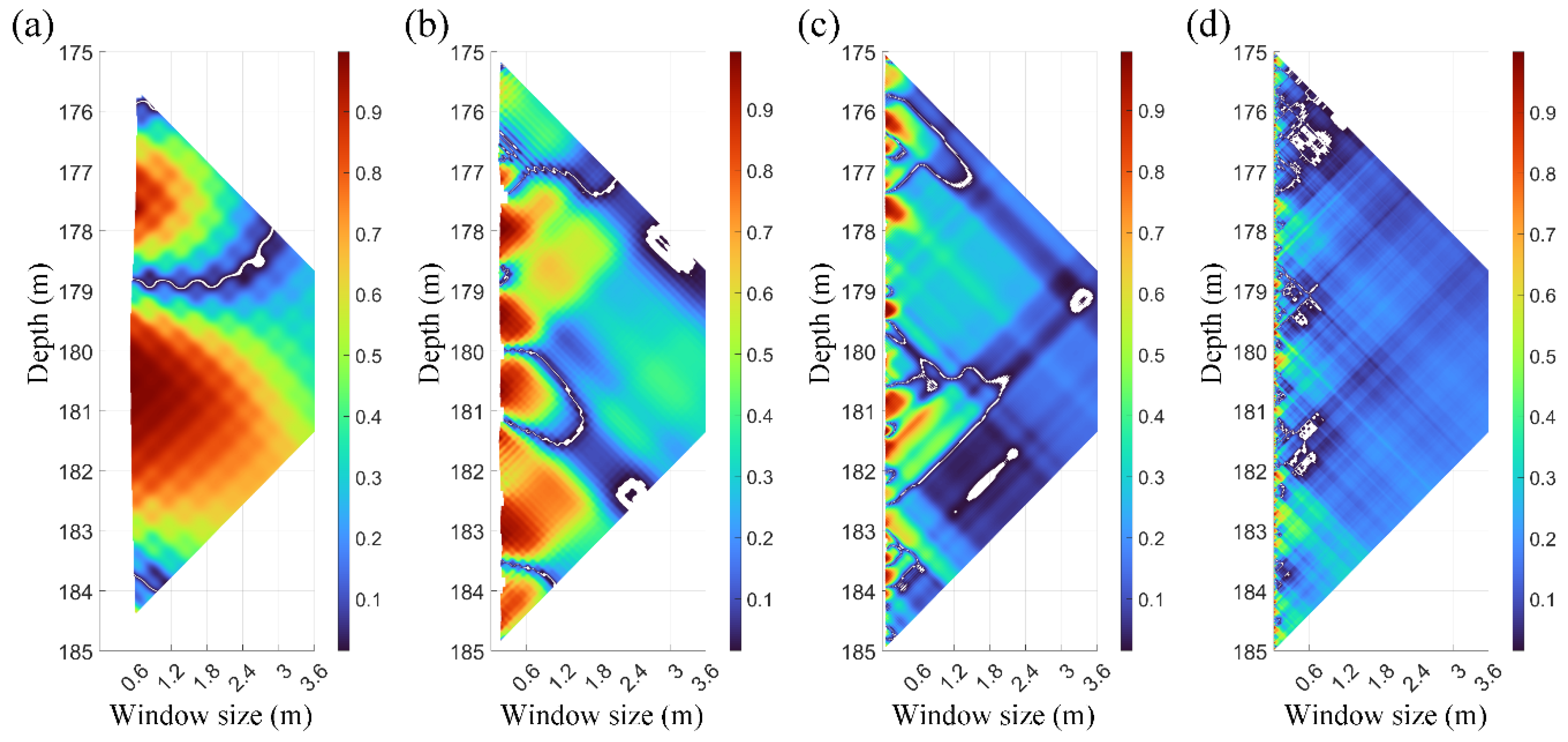
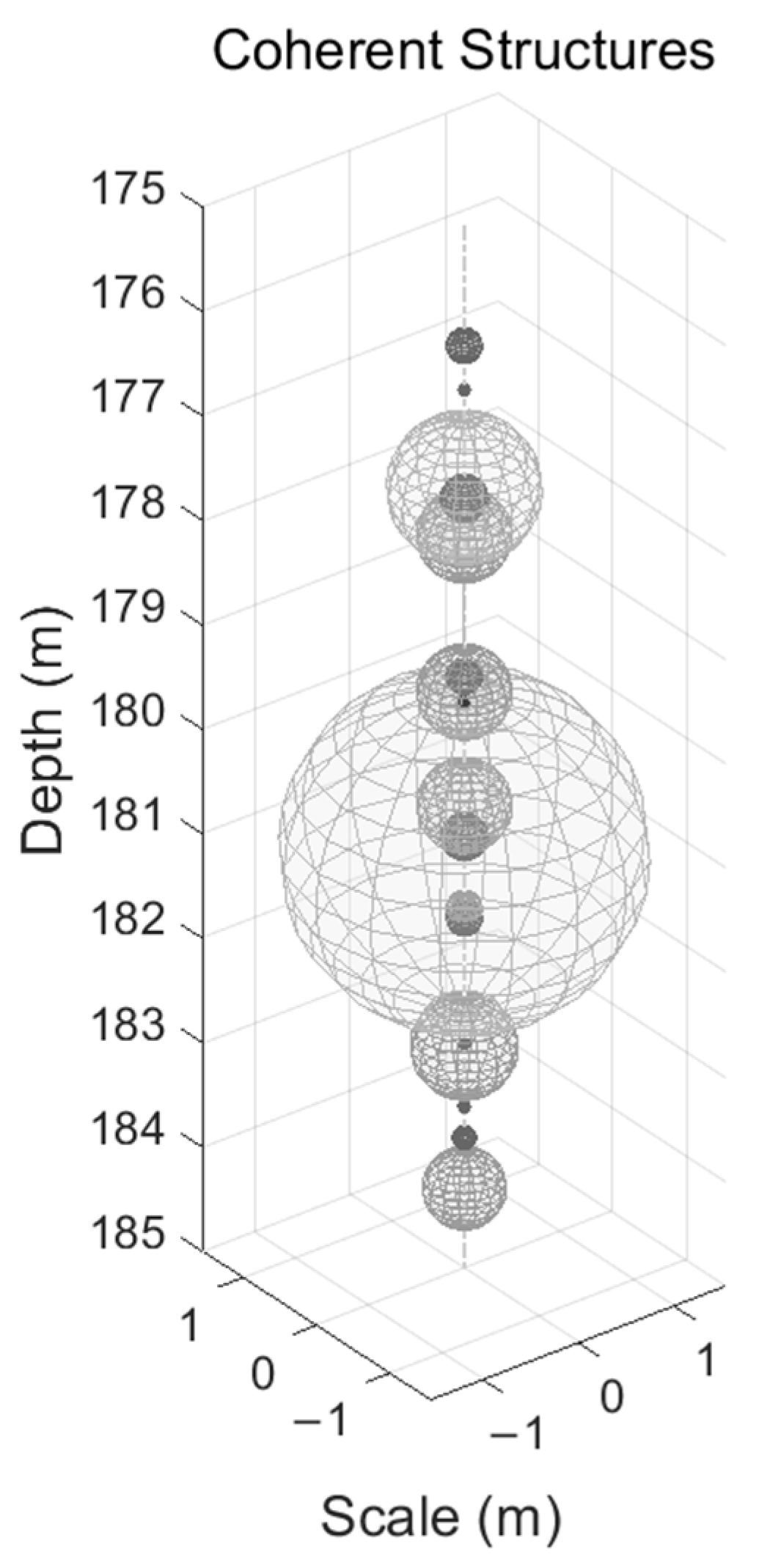
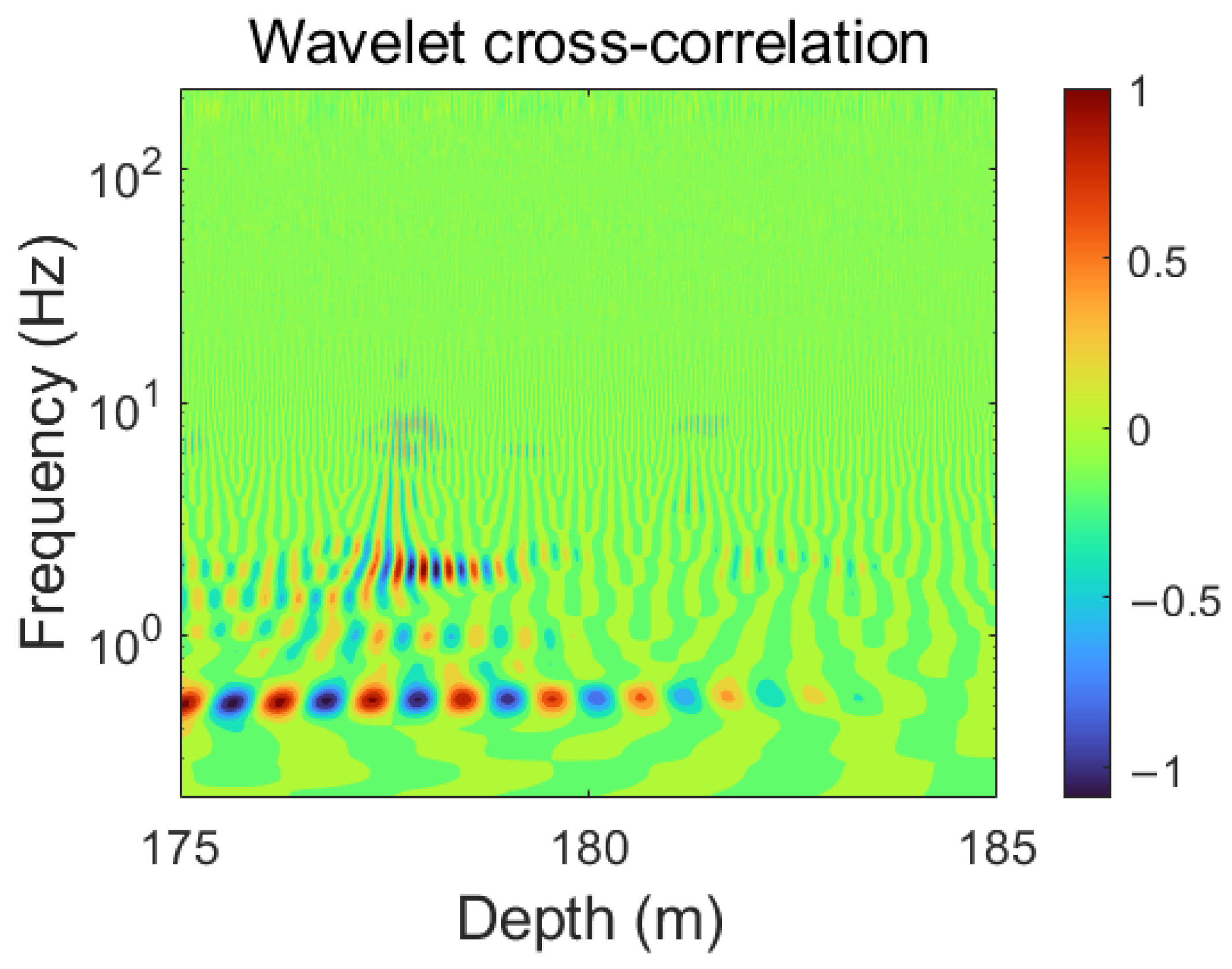
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nguyen, D.B.; Wu, P.R.; Monico, R.O.; Chen, G.N. Dynamic mode decomposition for large-scale coherent structure extraction in shear flows. IEEE Trans. Vis. Comput. Graph. 2023, 29, 1531–1544. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Z.Y.; Koken, M.; Constantinescu, G. Approximate methodology to account for effects of coherent structures on sediment entrainment in rans simulations with a movable bed and applications to pier scour. Adv. Water Resour. 2018, 120, 65–82. [Google Scholar] [CrossRef]
- Nolan, P.J.; Foroutan, H.; Ross, S.D. Pollution transport patterns obtained through generalized lagrangian coherent structures. Atmosphere 2020, 11, 168. [Google Scholar] [CrossRef]
- Sandeepan, B.S.; Rakesh, P.T.; Venkatesan, R. Observation and simulation of boundary layer coherent roll structures and their effect on pollution dispersion. Atmos. Res. 2013, 120, 181–191. [Google Scholar] [CrossRef]
- Shi, Y.; Zeng, Q.C.; Liu, L.; Cheng, X.L.; Hu, F. Important role of turbulent wind gust and its coherent structure in the rapid removal of urban air pollution. Environ. Res. Commun. 2022, 4, 075001. [Google Scholar] [CrossRef]
- Talke, S.A.; Horner-Devine, A.R.; Chickadel, C.C.; Jessup, A.T. Turbulent kinetic energy and coherent structures in a tidal river. J. Geophys. Res.-Ocean. 2013, 118, 6965–6981. [Google Scholar] [CrossRef]
- Farooq, S.; Huarte-Espinosa, M.; Ostilla-Mónico, R. Large-scale structures in high-Reynolds-number rotating Waleffe flow. J. Fluid Mech. 2020, 884, A26. [Google Scholar] [CrossRef]
- Li, X.; Hu, F.; Pu, Y.F.; Al-Jiboori, M.H.; Hu, Z.X.; Hong, Z.X. Identification of coherent structures of turbulence at the atmospheric surface layer. Adv. Atmos. Sci. 2002, 19, 687–698. [Google Scholar]
- Peacock, T.; Haller, G. Lagrangian coherent structures the hidden skeleton of fluid flows. Phys. Today 2013, 66, 41–47. [Google Scholar] [CrossRef]
- Saddoughi, S.G.; Veeravalli, S.V. Local isotropy in turbulent boundary layers at high Reynolds number. J. Fluid Mech. 2006, 348, 201–245. [Google Scholar] [CrossRef]
- Khurshid, S.; Donzis, D.A.; Sreenivasan, K.R. Slow spectral transfer and energy cascades in isotropic turbulence. J. Fluid Mech. 2021, 908, A21. [Google Scholar] [CrossRef]
- Siddiqui, M.; Loewen, M.R.; Asher, W.E.; Jessup, A.T. Coherent structures beneath wind waves and their influence on air-water gas transfer. J. Geophys. Res. Ocean. 2004, 109, C03024. [Google Scholar] [CrossRef]
- Gurka, R.; Liberzon, A.; Hetsroni, G. Pod of vorticity fields: A method for spatial characterization of coherent structures. Int. J. Heat Fluid Flow 2006, 27, 416–423. [Google Scholar] [CrossRef]
- Li, D.D.; Zhao, B.D.; Wang, J.W. Data-driven identification of coherent structures in gas-solid system using proper orthogonal decomposition and dynamic mode decomposition. Phys. Fluids 2023, 35, 013321. [Google Scholar] [CrossRef]
- Yin, S.; Fan, Y.F.; Sandberg, M.; Li, Y.G. PIV based POD analysis of coherent structures in flow patterns generated by triple interacting buoyant plumes. Build. Environ. 2019, 158, 165–181. [Google Scholar] [CrossRef]
- Bulusu, K.V.; Plesniak, M.W. Shannon entropy-based wavelet transform method for autonomous coherent structure identification in fluid flow field data. Entropy 2015, 17, 6617–6642. [Google Scholar] [CrossRef]
- Gong, X.; Ma, X.Y.; Fan, Z.Y.; Zhang, X.; Jiang, N. Wavelet analysis of the coherent structures in airfoil leading-edge separation control by bionic coverts. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2023, 237, 2076–2089. [Google Scholar] [CrossRef]
- Lucia, C.; Gassmann, M.I. Wavelet analysis of coherent structures above maize and soybean crops. Bound.-Layer Meteor. 2022, 184, 231–249. [Google Scholar]
- Lotfy, E.R.; Zaki, S.A.; Harun, Z. Modulation of the atmospheric turbulence coherent structures by mesoscale motions. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 178. [Google Scholar] [CrossRef]
- Ostilla-Mónico, R.; Verzicco, R.; Grossmann, S.; Lohse, D. The near-wall region of highly turbulent Taylor-Couette flow. J. Fluid Mech. 2016, 788, 95–117. [Google Scholar] [CrossRef]
- Hunt, J.C.R.; Wray, A.A.; Moin, P. Eddies, streams, and convergence zones in turbulent flows. In Studying Turbulence Using Numerical Simulation Databases, 2; NASA: Washington, DC, USA, 1988. [Google Scholar]
- Perry, A.E.; Chong, M.S. A description of eddying motions and flow patterns using critical-point concepts. Ann. Rev. Fluid Mech. 2003, 19, 125–155. [Google Scholar] [CrossRef]
- Haller, G. Lagrangian Coherent Structures. Annu. Rev. Fluid Mech. 2015, 47, 137–162. [Google Scholar] [CrossRef]
- Martins, F.; Sciacchitano, A.; Rival, D.E. Detection of vortical structures in sparse Lagrangian data using coherent-structure colouring. Exp. Fluids 2021, 62, 69. [Google Scholar] [CrossRef]
- Schlueter-Kuck, K.L.; Dabiri, J.O. Coherent structure colouring: Identification of coherent structures from sparse data using graph theory. J. Fluid Mech. 2017, 811, 468–486. [Google Scholar] [CrossRef]
- Acevedo, O.C.; Costa, F.D.; Oliveira, P.; Puhales, F.S.; Degrazia, G.A.; Roberti, D.R. The influence of submeso processes on stable boundary layer similarity relationships. J. Atmos. Sci. 2014, 71, 207–225. [Google Scholar] [CrossRef]
- Basu, S.; Porte-Agel, F.; Foufoula-Georgiou, E.; Vinuesa, J.F.; Pahlow, M. Revisiting the local scaling hypothesis in stably stratified atmospheric boundary-layer turbulence: An integration of field and laboratory measurements with large-eddy simulations. Bound.-Layer Meteor. 2006, 119, 473–500. [Google Scholar] [CrossRef]
- Conangla, L.; Cuxart, J.; Soler, M.R. Characterisation of the nocturnal boundary layer at a site in northern Spain. Bound.-Layer Meteor. 2008, 128, 255–276. [Google Scholar] [CrossRef]
- Diabil, H.A.; Li, X.K.; Abdalla, I.E. Numerical study of a separated boundary layer transition over two and three dimensional geometrical shapes. World Sci. Eng. Acad. Soc. 2017, 16, 45–72. [Google Scholar]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. Digit. Arch. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Jiang, X.Y. Revisiting coherent structures in low-speed turbulent boundary layers. Appl. Math. Mech.-Engl. Ed. 2019, 40, 261–272. [Google Scholar] [CrossRef]
- Zhou, J.; Adrian, R.J.; Balachandar, S.; Kendall, T.M. Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 1999, 387, 353–396. [Google Scholar] [CrossRef]
- Hu, H.B.; Du, P.; Huang, S.H.; Wang, Y. Extraction and verification of coherent structures in near-wall turbulence. Chin. Phys. B 2013, 22, 74703. [Google Scholar] [CrossRef]
- Wu, J.Y.; Nichols, A.; Krynkin, A.; Croft, M. Objective phase-space identification of coherent turbulent structures in 1d time series data. J. Hydraul. Res. 2022, 60, 811–825. [Google Scholar] [CrossRef]
- Zheng, D.; Zhang, T.; Xing, J.; Mei, J.Q. Improvement of the HHT method and application in weak vortex signal detection. Meas. Sci. Technol. 2007, 18, 2769–2776. [Google Scholar] [CrossRef]
- Abraham, A.; Hong, J.R. Characterization of atmospheric coherent structures and their impact on a utility-scale wind turbine. Flow 2022, 2, E5. [Google Scholar] [CrossRef]
- Alekseenko, S.V.; Abdurakipov, S.S.; Hrebtov, M.Y.; Tokarev, M.P.; Dulin, V.M.; Markovich, D.M. Coherent structures in the near-field of swirling turbulent jets: A tomographic PIV study. Int. J. Heat Fluid Flow 2018, 70, 363–379. [Google Scholar] [CrossRef]
- Sun, J.; Gao, T.D.; Fan, Y.; Chen, W.Y.; Xuan, R.X. The modulation of particles on coherent structure of turbulent boundary layer in dilute liquid-solid two-phase flow with PIV. Powder Technol. 2019, 344, 883–896. [Google Scholar] [CrossRef]
- Tang, Z.Q.; Jiang, N.; Schröder, A.; Geisler, R. Tomographic PIV investigation of coherent structures in a turbulent boundary layer flow. Acta Mech. Sin. 2012, 28, 572–582. [Google Scholar] [CrossRef]
- Liu, C.Q.; Wang, Y.Q.; Yang, Y.; Duan, Z.W. New omega vortex identification method. Sci. China-Phys. Mech. Astron. 2016, 59, 684711. [Google Scholar] [CrossRef]
- Sakurai, T.; Yoshimatsu, K.; Schneider, K.; Farge, M.; Morishita, K.; Ishihara, T. Coherent structure extraction in turbulent channel flow using boundary adapted wavelets. J. Turbul. 2017, 18, 352–372. [Google Scholar] [CrossRef]
- Bolado-Penagos, M.; Sala, I.; Gomiz-Pascual, J.J.; González, C.J.; Izquierdo, A.; Alvarez, O.; Vázquez, A.; Bruno, M.; van Haren, H. Analysis of internal soliton signals and their eastward propagation in the Alboran sea: Exploring the effect of subinertial forcing and fortnightly variability. Prog. Oceanogr. 2023, 217, 103077. [Google Scholar] [CrossRef]
- van Haren, H.; Bakker, R.; Witte, Y.; Laan, M.; van Heerwaarden, J. Half a cubic hectometer mooring array of 3000 temperature sensors in the deep sea. J. Atmos. Ocean. Technol. 2021, 38, 1585–1597. [Google Scholar] [CrossRef]
- Yang, C.F.; Chi, W.C.; van Haren, H.; Lin, C.R.; Kuo, B.Y. Tracking deep-sea internal wave propagation with a differential pressure gauge array. Sci. Rep. 2021, 11, 23311. [Google Scholar] [CrossRef]
- Bulusu, K.V.; Plesniak, M.W. Secondary flow morphologies due to model stent-induced perturbations in a 180° curved tube during systolic deceleration. Exp. Fluids 2013, 54, 1493. [Google Scholar] [CrossRef]
- Fang, R.Z.; Wang, Y.; Lan, C.X.; Zhang, Z.J.; Zheng, D.; Lan, G.D.; Wang, B.M. Detecting near-surface coherent structure characteristics using wavelet transform with different meteorological elements. J. Trop. Meteorol. 2020, 26, 453–460. [Google Scholar]
- Protzko, D.E.; Guimond, S.R.; Jackson, C.R.; Sapp, J.W.; Jelenak, Z.; Chang, P.S. Documenting coherent turbulent structures in the boundary layer of intense hurricanes through wavelet analysis on IWRAP and SAR data. IEEE Trans. Geosci. Remote Sens. 2023, 61, 4105316. [Google Scholar] [CrossRef]
- Chen, X.; Wu, Z.; Huang, N.E. The time-dependent intrinsic correlation based on the empirical mode decomposition. Adv. Adapt. Data Anal. 2010, 2, 1000047. [Google Scholar] [CrossRef]
- Johny, K.; Pai, M.L.; Adarsh, S. A multivariate EMD-LSTM model aided with time dependent intrinsic cross-correlation for monthly rainfall prediction. Appl. Soft. Comput. 2022, 123, 108941. [Google Scholar] [CrossRef]
- Luo, J.; Wang, N.A.; Zheng, Z.C.; Li, T.X.; He, S.Q.; Tarolli, P. Tillage-induced microtopography alters time-dependent intrinsic correlation of runoff and sediment yield. Soil Tillage Res. 2022, 221, 105423. [Google Scholar] [CrossRef]
- Medina, O.D.; Schmitt, F.G.; Calif, R.; Germain, G.; Gaurier, B. Turbulence analysis and multiscale correlations between synchronized flow velocity and marine turbine power production. Renew. Energy 2017, 112, 314–327. [Google Scholar] [CrossRef]
- Hadjighasem, A.; Karrasch, D.; Teramoto, H.; Haller, G. A spectral clustering approach to Lagrangian vortex detection. APS Meet. Abstr. 2015, 93, 063107. [Google Scholar] [CrossRef]
- Derian, P.; Heas, P.; Memin, E. Wavelets to reconstruct turbulence multifractals from experimental image sequences, TSFP7. In Proceedings of the 7th International Symposium on Turbulence and Shear Flow Phenomena, Ottawa, ON, Canada, 28–31 July 2011; Begel House Inc.: New York, NY, USA, 2011. [Google Scholar]
- Goodman, L.; Levine, E.R.; Lueck, R.G. On measuring the terms of the turbulent kinetic energy budget from an AUV. J. Atmos. Ocean. Technol. 2006, 23, 977–990. [Google Scholar] [CrossRef]
- Nasmyth, P.W. Oceanic Turbulence; University of British Columbia: Vancouver, BC, USA, 1970. [Google Scholar]
- Vassilicos, J.C. Dissipation in Turbulent Flows. Annu. Rev. Fluid Mech. 2015, 47, 95–114. [Google Scholar] [CrossRef]
- Wolk, F.; Yamazaki, H.; Seuront, L.; Lueck, R.G. A new free-fall profiler for measuring biophysical microstructure. J. Atmos. Ocean. Technol. 2002, 19, 780–793. [Google Scholar] [CrossRef]
- Luan, X.; Mao, B.B.; Ren, X.M.; Yang, H.; Teng, Y.R.; Song, D.L. Turbulent cascade in the multi-layered network. In Proceedings of the OCEANS 2019—Marseille, Marseille, France, 17–20 June 2019. [Google Scholar]
- Li, H. Identification of coherent structure in turbulent shear flow with wavelet correlation analysis. J. Fluids Eng.-Trans. ASME 1998, 120, 778–785. [Google Scholar] [CrossRef]
- Yule, A.J. Large-scale structure in the mixing layer of a round jet. J. Fluid Mech. 1978, 89, 413–432. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mao, B.; Yang, H.; Sun, F.; Zhang, Y.; Zhang, X. A Spatial Correlation Identification Model for Coherent Structure Extraction and Three-Dimensional Visualization. J. Mar. Sci. Eng. 2024, 12, 483. https://doi.org/10.3390/jmse12030483
Mao B, Yang H, Sun F, Zhang Y, Zhang X. A Spatial Correlation Identification Model for Coherent Structure Extraction and Three-Dimensional Visualization. Journal of Marine Science and Engineering. 2024; 12(3):483. https://doi.org/10.3390/jmse12030483
Chicago/Turabian StyleMao, Beibei, Hua Yang, Fei Sun, Ying Zhang, and Xinrui Zhang. 2024. "A Spatial Correlation Identification Model for Coherent Structure Extraction and Three-Dimensional Visualization" Journal of Marine Science and Engineering 12, no. 3: 483. https://doi.org/10.3390/jmse12030483
APA StyleMao, B., Yang, H., Sun, F., Zhang, Y., & Zhang, X. (2024). A Spatial Correlation Identification Model for Coherent Structure Extraction and Three-Dimensional Visualization. Journal of Marine Science and Engineering, 12(3), 483. https://doi.org/10.3390/jmse12030483




