Bucket Size Optimization for Metering Device in Garlic Planter Using Discrete Element Method

Abstract

In this study, the discrete element method was used to optimize the bucket size for the metering device in a garlic planter for enhancing the productivity of garlic farming according to the garlic size. Statistical information concerning the actual shape of garlic cloves was incorporated, and the mechanical properties of garlic were determined using the bulk density, sliding test, and repose angle test for enhancing the fidelity of the simulation model. The optimal bucket size achieving the target plant rate of 97.5% was determined using the developed discrete element model for the three garlic size groups. The linear search method was used for optimization, and batch simulation was performed to validate the optimized results and confirm the performance index of the metering device. A Gaussian distribution based on statistical information accounted for the various garlic sizes in each group. Finally, a metering test verified the reliability of the optimization technique. The differences between the simulation and test results were within 10% for all performance indices, including missing plant rate, multi-plant rate, and planting rate, indicating the high reliability of the analysis model. Subsequently, the larger garlic groups (Groups 2 and 3) exhibited metering performance close to the target plant rate.

1. Introduction

The United Nations has identified zero hunger as one of the sustainable development goals to be achieved by 2030. Consequently, global interest in enhancing agricultural production rates has been growing [1]. However, in countries such as South Korea, the aging population and decreasing rural population pose challenges to these goals. One potential solution is the automation and mechanization of agriculture, which is particularly effective for labor-intensive crops. Garlic, a typical such crop, is a case in point; garlic planting is particularly strenuous and accounts for 15% of the total labor in garlic farming [2]. The mechanization of garlic planting could alleviate the manpower shortage, enhance the planting rate per hour, and increase overall production.

Garlic planters can be classified based on their power source and divided into self-propelled and tractor-attached types. They can also be categorized based on the posture of the garlic cloves to be planted, including upright planting, in which the root of the garlic cloves faces downwards, and lie-down planting, in which the garlic cloves are placed horizontally on the ground using free fall [3]. One planter component, the metering device, transfers garlic cloves from the hopper to the planting unit, which then plants them in the field based on the planting method. This is followed by soil covering and mulching. The metering device is a crucial component of the garlic planter. It plays a significant role in controlling the missing plant and multi-plant rates, key performance indicators of the garlic planter [4]. The missing plant rate refers to the frequency at which garlic cloves fail to be transferred to the planting unit, while the multi-plant rate indicates the frequency at which two or more garlic cloves are transferred to the planting unit simultaneously. Both performance indicators can cause significant losses for farmers and must be minimized. A high missing plant rate can result in a reduction in total production and/or additional labor required to replant in the missed area, while a high multi-plant rate leads to unnecessary waste of garlic cloves and can result in interference of the growth between several garlic cloves planted in a limited space, leading to reduced total production.

To reduce the rates of missing plants and multiple plants, an optimal design that considers the performance index of the metering device is necessary. It is imperative to anticipate the missing plant and multi-plant rates of the metering device during the development stage and supplement the design accordingly based on the results to achieve the optimal design of the metering device. Performance indicators of metering devices can be predicted through experimental methods using test devices and simulation methods. While performance prediction through experimentation offers the advantage of high accuracy when performed under the same conditions as the actual operation, it requires a significant amount of time and resources to build a test device, thereby limiting the production of samples that cover a wide range of design variables. Conversely, simulation-based methods offer high accuracy when using validated analysis models and, as there is no limit on the design range, can predict the performance of the metering device across a wide range of design parameters. Therefore, an analytical method that employs simulation is appropriate for optimal design beyond simple performance comparison.

To analyze the performance of garlic planters, various studies have been conducted utilizing both experimental and simulation methods. Kang et al. [5] conducted a yield comparison experiment as a testing method to determine the effects of planting angle and planting depth on garlic growth. The results confirmed that garlic yield was highest at planting angles of 45° or 90° and planting depths of 2–4 cm. Additionally, to improve the performance of the metering device, Kang et al. [3] experimentally determined the missing plant rate based on the bucket size. The results indicated that the bucket size of the metering device significantly affects the performance index. Zilpilwar et al. [6] determined the optimal cup size and operating speed of a cup-feed-type metering device through an experimental method similar to that in the aforementioned study. The authors considered the planting rate, planting interval, and clove damage rate. Zhang et al. [7] conducted one-factor and multifactor experiments to optimally design key components of a spoon-clamping type planter and optimize working parameters. As mentioned above, experimental studies offer high prediction accuracy using actual metering devices. However, these studies have limited applicability of predictive models depending on the sample range and expense of test equipment. Additionally, due to the restricted selection range of design variables, the scope for optimal design is limited.

In addressing the limitations of the experimental method, studies have been conducted using simulations to predict the performance of garlic planters. Im et al. [8] classified garlic samples into four groups based on size and developed a 3D computer-aided design (CAD) model using statistical results on garlic clove shape. The optimal bucket size of the metering device was then determined using the developed CAD model and multi-body dynamics model according to the size of the garlic cloves. Although the fidelity of the model was increased by incorporating the shape of actual garlic cloves in the analysis, validation of the determined optimal bucket size and simulation model was not performed. Guo et al. [9] analyzed the single seed rate, multiple seed rate, and missing seed rate of the metering device according to the adjustment size and operation speed using the discrete element method (DEM). This resulted in a single-seed metering performance qualification rate of over 80%. An actual field test was performed to validate the applicability of the optimal design, and the performance of the metering device met the required standards. While the authors derived the optimal design value using DEM, they did not consider the various sizes of garlic in the analysis model nor incorporate the actual shape of garlic cloves.

To minimize the missing seed rate of a finger clip plate garlic seed-metering device, Wang & Sun et al. [4] utilized the commercial software EDEM as a further investigation that executed optimal design using the DEM. The study scrutinized the missing seed and single seed rates based on the opening diameter of the device, operating speed, and the population number of garlic cloves. Moreover, a regression model was constructed via a Box–Behnken center combination test. However, the analysis model did not consider the actual shape of the target garlic, which was the garlic grown in Pizhou, and cloves were depicted as multi-spheres. The low accuracy of the resulting contact force calculation limits the validity of the study, similar to Guo et al. [9].

As another machine for the mechanization of garlic farming, Yu et al. [10] optimized the parameters for the root-cutting operation of the garlic harvester to minimize the root clearance rate and bulb damage. To achieve this optimization, they developed a mathematical model for assessing the root clearance rate and bulb damage.

Research has been conducted to enhance planting performance not only in garlic but also in other crops [11,12,13,14,15,16,17]. However, studies that utilized experimental approaches have limitations in design variables or operating conditions and are applicable only within specific sample ranges [3,5,6,7]. Previous studies that used simulation models executed optimal designs without design variable constraints; however, in some cases, the analysis model was not validated [8], and the actual shape of garlic cloves was not considered in the analysis model, constraining its reliability [10,11].

In this investigation, we aimed to surpass the limitations of prior research by incorporating the actual shape of garlic cloves into the discrete element model to determine the optimal bucket size of the metering device. We optimized by categorizing the garlic cloves into three groups, considering their size distribution. Furthermore, we conducted a metering simulation to validate the optimization results and validated the reliability of the optimization technique by comparing the outcomes with the actual test results. To incorporate the actual shape of garlic cloves, we adopted the statistical data proposed by Im et al. [8] into the discrete element model. We performed angle of repose and sliding tests to identify the mechanical parameters of the analysis model. Using the developed discrete element model, we determined the optimal bucket size to meet the target planting rate under the same operating conditions as the actual planter. We employed the sequential search method as the optimization technique and found the optimal solution by gradually reducing the bucket size. We conducted a metering simulation to validate that the determined bucket size satisfied the required performance index, we conducted a metering simulation and validated the optimization technique through a metering test. As a result of the metering simulation, the performance index of the required level was nearly achieved, and the reliability of the optimization technique was confirmed as the difference between the test result and simulation result in all performance indexes was within 10%.

Thus, in this study, we developed a discrete element model with high fidelity and executed the optimal design of the garlic planter metering device using the developed model. During actual metering operation, the optimization result of each group almost satisfied the target plant rate, and the proposed methodology can be used to optimize metering devices for other crops.

2. Materials and Methods

In this study, the DEM was employed to optimize the bucket size of the metering device of the garlic planter. To accurately represent the actual shape of garlic cloves in the analysis model, a 3D mesh model was created using the 3D CAD model for each of the three garlic size groups, which was previously developed by Im et al. [8]. Subsequently, the discrete element model parameters were determined through bulk density measurement, sliding test, and repose angle test. The optimal bucket size for each group was determined using the developed discrete element model, and a metering simulation was conducted to validate the optimal bucket size. Finally, an actual metering experiment was conducted to validate the optimization technique.

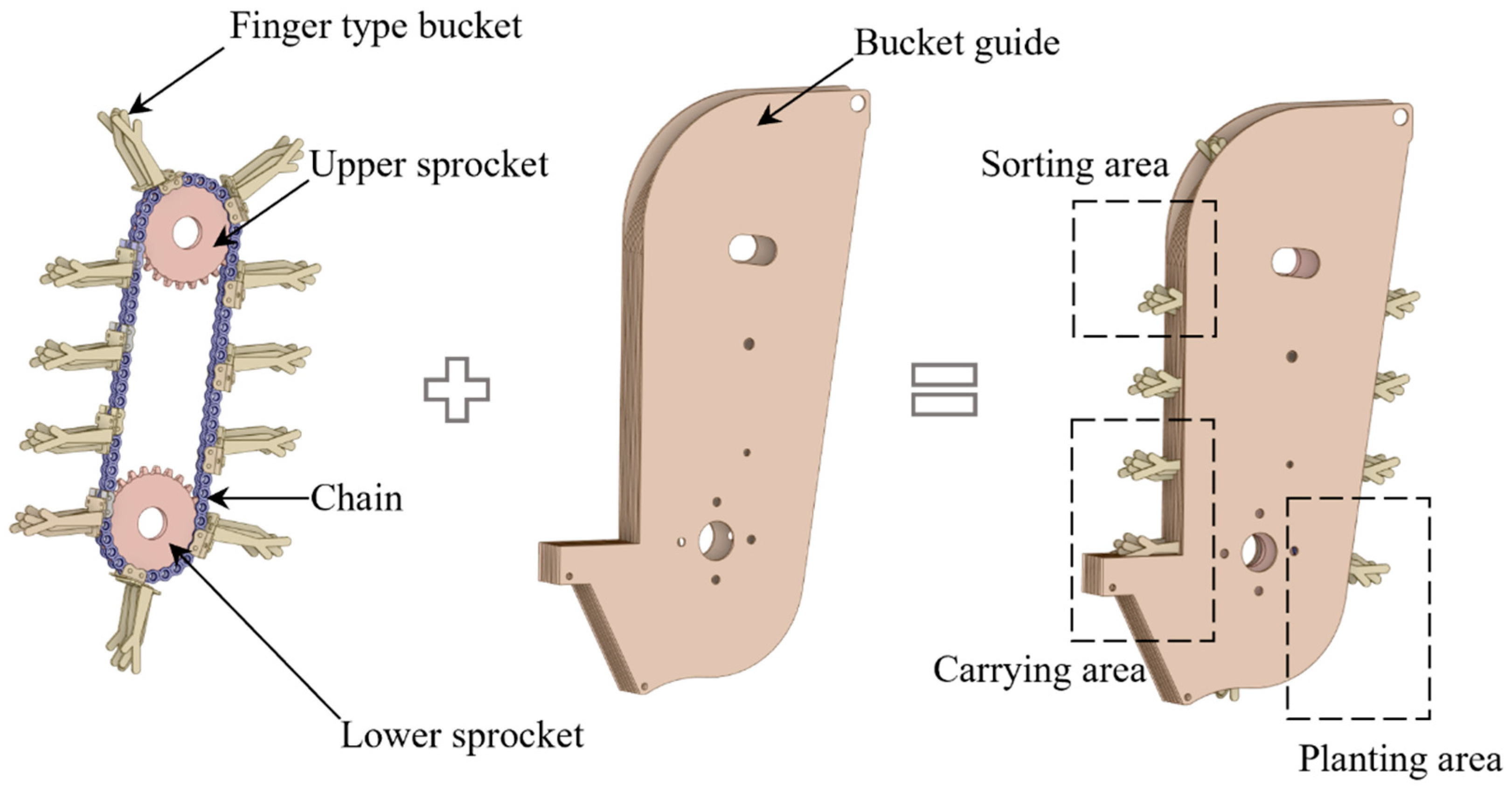
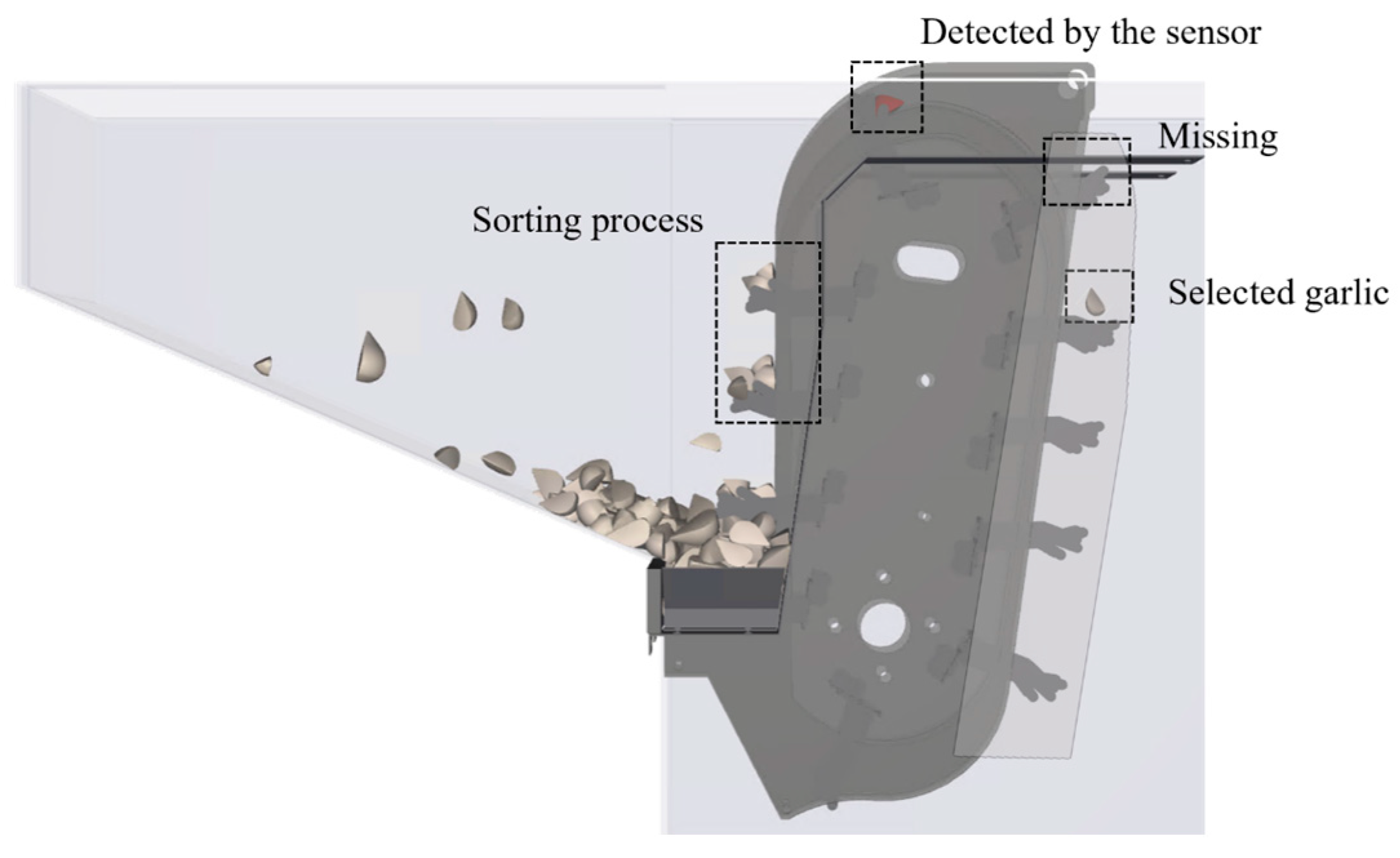
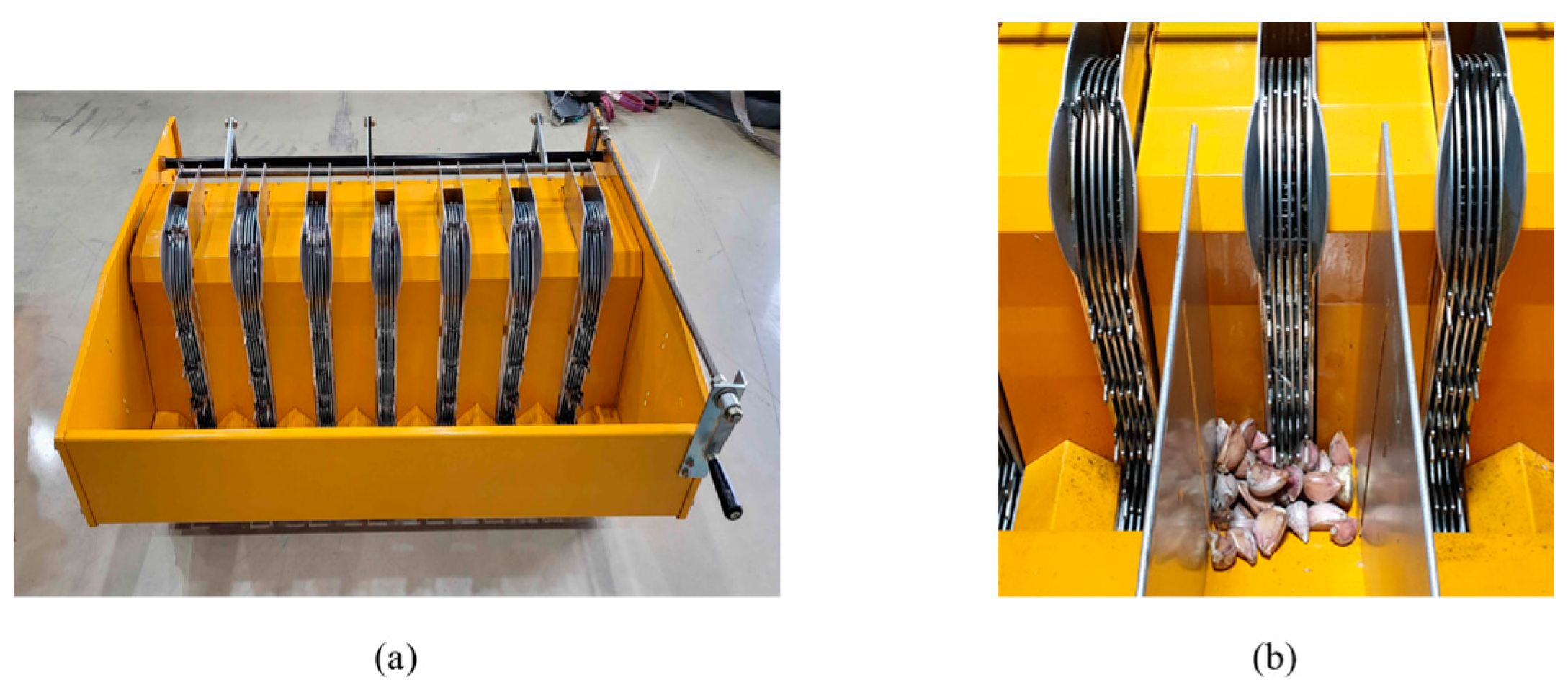
2.1. Metering Device Working Principle

The finger-type bucket metering device utilized in this study comprises the components illustrated in Figure 1. The finger-type bucket is affixed to the chain and derives its power from the sprocket. The lower sprocket is connected to the support wheel of the planter, and as the vehicle advances, it rotates in a clockwise direction owing to the frictional force on the ground. The bucket moves in unison with the rotation of the sprocket, collects the garlic cloves from the hopper in the carrying area, and transports them to the planting area. Throughout this process, the bucket size is determined based on the bucket guide setting, and according to the bucket size, any excess garlic cloves in the sorting area fall back into the hopper. The garlic that passes through the sorting area without falling rotates along the pitch diameter of the sprocket and undergoes a 180° phase shift as the bucket moves. As the phase shifts, gravity causes the garlic to fall onto the back of the finger-type bucket, which is one cycle ahead, and it is then planted through free fall while the planter traverses the planting area.

The bucket size varies based on the setting angle (α) of the bucket guide, as illustrated in Figure 2a, and the bucket guide rotates around the same rotation center as the lower sprocket, as shown in the figure. The position of the chain and sprocket does not change in accordance with the bucket guide settings, and the buckets affixed to the chain always follow the same trajectory. The bucket trajectory can be defined as the combination of the outermost points traced while the bucket moves, as indicated by the dotted line in Figure 2b. As the trajectory of the bucket is consistent, the bucket size (l) changes as the relative position of the guide changes. The bucket size can be defined as the minimum distance between the bucket trajectory and bucket guide, as shown in Figure 2b. When the center of gravity of the placed garlic cloves surpasses the bucket trajectory by a specific distance, the cloves fall under the influence of their weight.

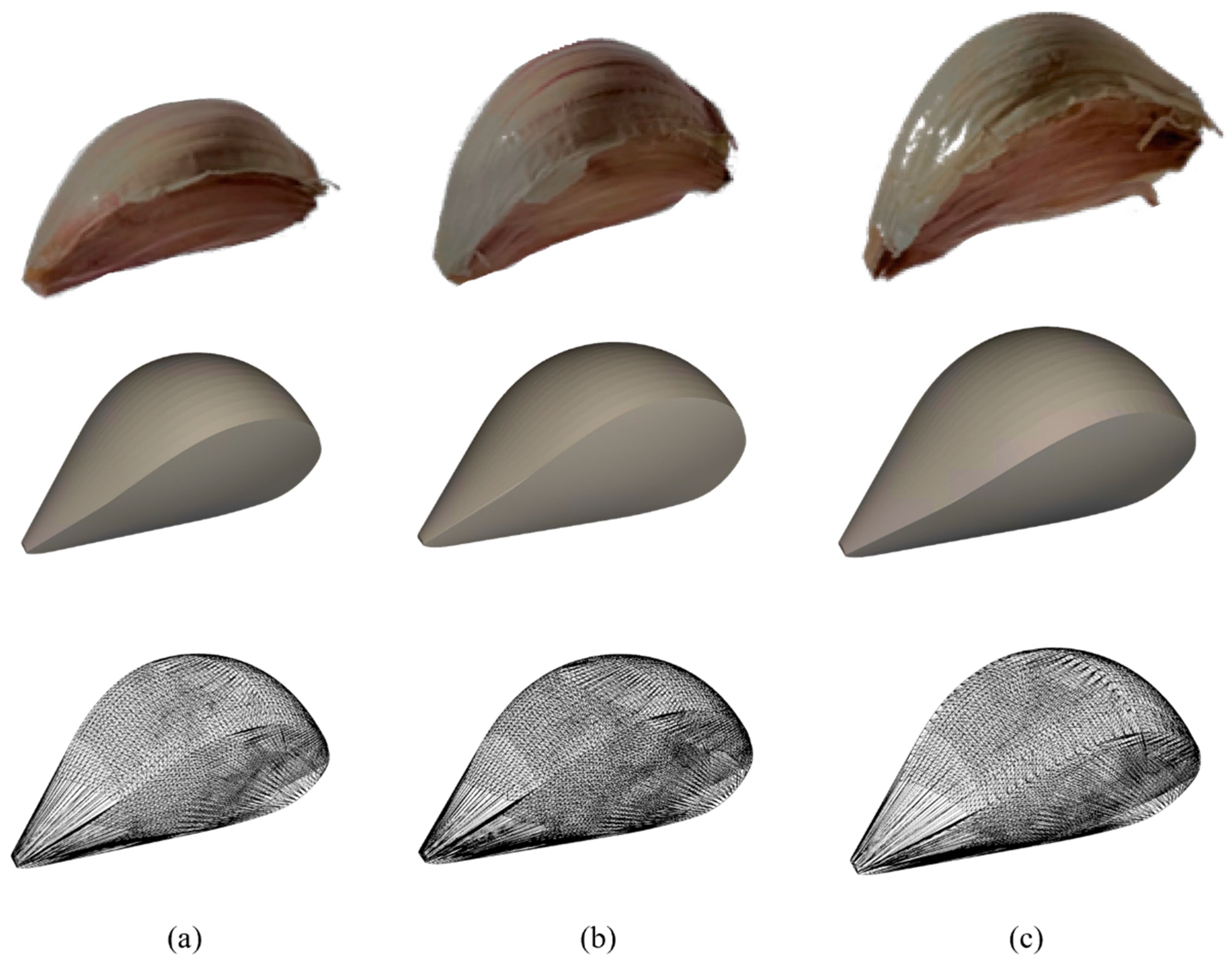
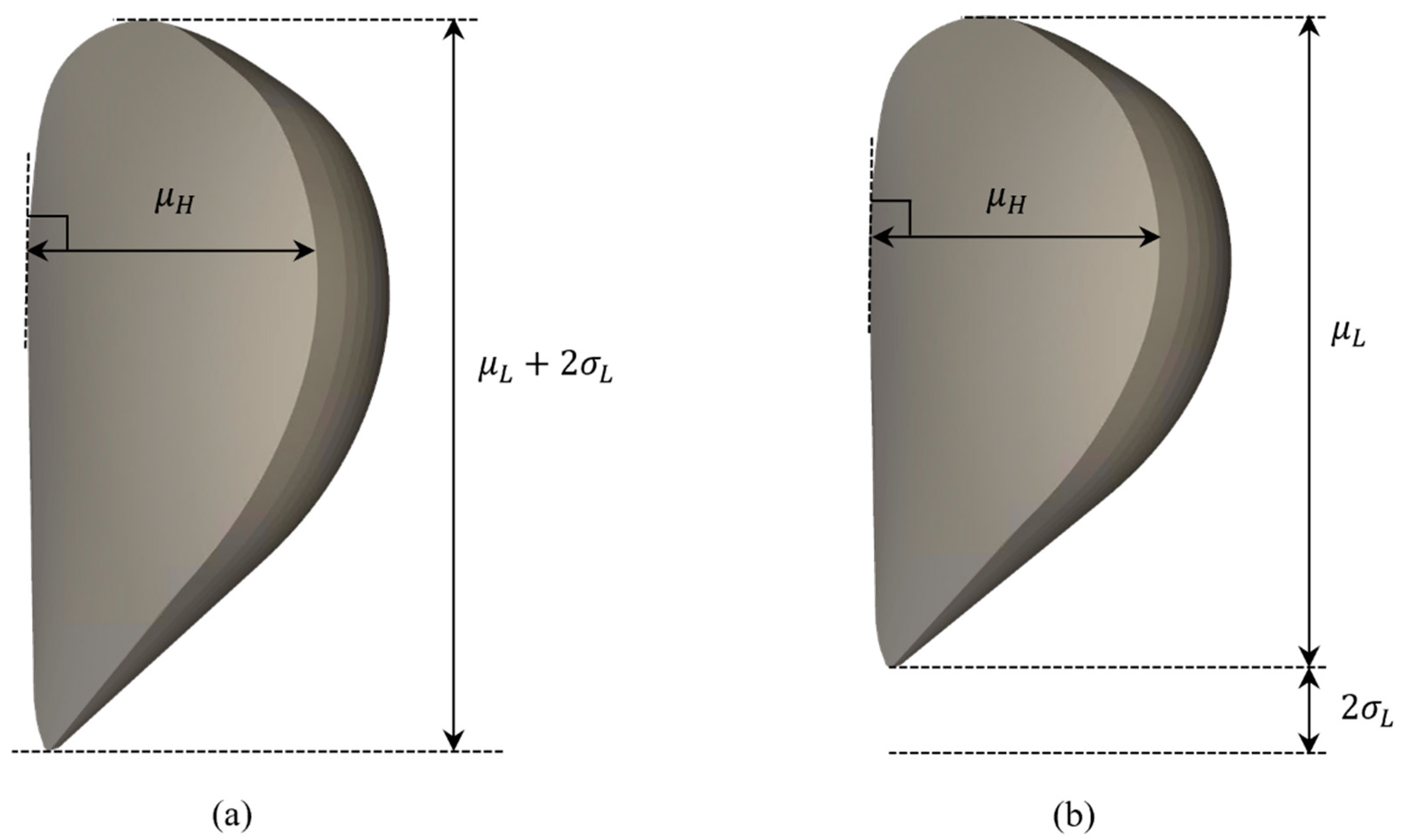
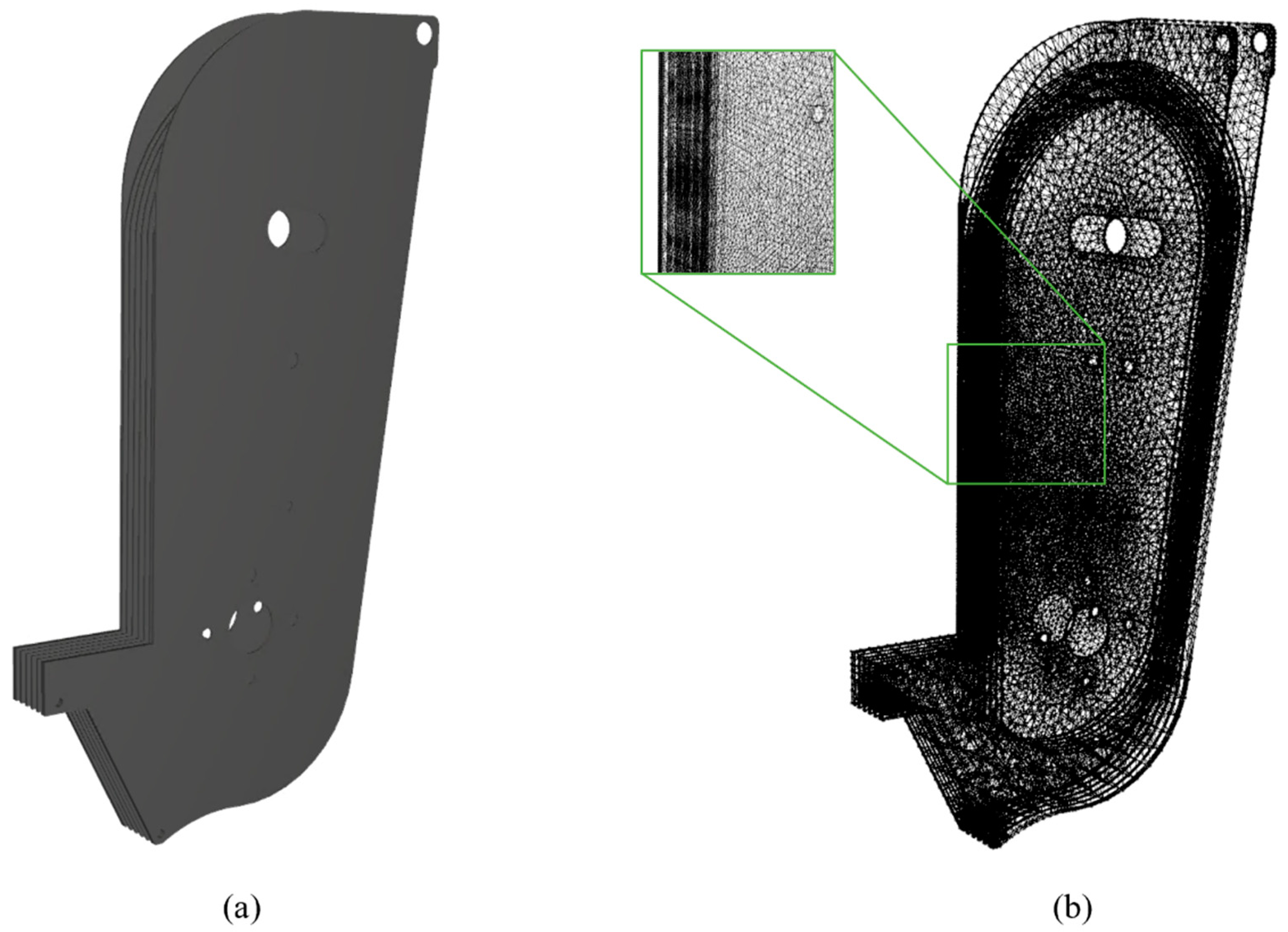
2.2. 3D Mesh Modeling

In this investigation, as the target garlic, we used garlic from the cold Uiseong region, identical to the sample used by Im et al. [8], and produced a 3D mesh model of garlic cloves using the 3D CAD model proposed in that study. Figure 3 displays samples of the actual garlic cloves of each group alongside the corresponding 3D CAD and mesh models for contact force calculation. The commercial software ANSYS Rocky can accommodate the actual particle shape in the analysis model by importing the mesh file (in STL format) to define the nodes required for contact force calculation (ANSYS Rocky, [18]). Excessively refined nodes demand a significant amount of computation and the analysis time can increase exponentially. Conversely, excessively coarse nodes can produce numerical errors during calculation. Therefore, selecting the appropriate number of nodes is critical. To ensure the accuracy and convergence of contact calculation, we utilized 3912 nodes for Group 1, 4730 nodes for Group 2, and 4497 nodes for Group 3 in the model, determined through trial-and-error procedures.

The 3D CAD model of garlic cloves used in this study comprises the elongated model, as presented in Figure 4a, and the average model, as shown in Figure 4b. To obtain statistical information about the shape of garlic cloves, Im et al. [8] measured the height and length of 160 garlic samples and developed a 3D CAD model to represent the shape of each group based on the statistical results. Similarly, we used an elongated model with an elongated length $\left({\mu }_L+{2\sigma }_L\right)$ and average height $\left({\mu }_H\right)$ was used to determine the optimal bucket size, as was executed by Im et al. [8]. For the metering simulation, an average model with an average length $\left({\mu }_L\right)$ and average height $\left({\mu }_H\right)$ of the groups was employed. During metering work, mechanical vibration aligns the garlic cloves parallel to the finger-type bucket, and the length of the garlic cloves determines the location of their center of gravity. The length of the garlic clove model was set to ${\mu }_L+{2\sigma }_L$, and the minimum bucket size allowing the clove to pass through the sorting area was determined to achieve the target planting rate of 97.5%. Further details are excluded as they are beyond the scope of this thesis. The average model presented in Figure 4b was utilized for the metering simulation described in Section 2.6. To validate that the optimal bucket size indeed satisfies the plant rate of 97.5%, we conducted a metering simulation. At this juncture, the average model was scaled up and down according to the Gaussian distribution to test the optimal bucket size for various garlic sizes.

When constructing a discrete element model, it is necessary to define a mesh as a calculation node for the garlic cloves and the mechanical device with which they come into contact during planting. The garlic cloves come into contact with finger-type buckets and bucket guides, as shown in Figure 5 and Figure 6. For this component, 8166 computational nodes were defined, as depicted in Figure 5b. Similarly, Figure 6a illustrates the 3D model of the bucket guide with 37,268 computational nodes, as shown in Figure 6b. The bucket guide consists of several plates, and contact with the garlic occurs in the anterior part, which is enlarged in Figure 6b. Therefore, a denser mesh was constructed in the contact area to efficiently calculate contact forces.

2.3. DEM Parameter Determination

It is essential to define discrete element parameters that precisely reflect the mechanical properties of actual garlic cloves to ensure the high accuracy of the discrete element model. These parameters include properties that reflect the mechanical characteristics of the particle itself, such as bulk density, Young’s modulus, and Poisson’s ratio, as well as parameters related to interactions with other mechanical devices, such as static friction coefficient and restitution coefficient. The parameters for the interaction between garlic and steel were determined to simulate the mechanical interaction with an actual steel device. We also determined the parameters for the interaction between acrylic and garlic to calibrate the repose angle. For visualization, we used an acrylic cylinder for the repose angle test and for the discrete element model for calibration.

Table 1. DEM parameter determination.

Category	Property	Value	Unit	Determination
Garlic	Bulk density	605.45	kg/m3	Directly measured
	Young’s modulus	13.38	MPa	Park et al. [19]
	Poisson’s ratio	0.16	-	Park et al. [19]
Garlic-garlic	Static friction coefficient	0.46	-	Repose angle calibration
	Tangential ratio	1	-	Default
	Restitution coefficient	0.50	-	Park et al. [19]
Acryl-garlic	Static friction coefficient	0.4	-	Sliding test
	Tangential ratio	1	-	Default
	Restitution coefficient	0.65	-	Yu et al. [20]
Steel-garlic	Static friction coefficient	0.31	-	Sliding test
	Tangential ratio	1	-	Default
	Restitution coefficient	0.65	-	Yu et al. [20]

summarizes the discrete element parameters and methods used to determine these parameters.

The bulk density of garlic was directly measured using an acrylic cylinder, as depicted in Figure 7. In this study, the garlic cloves were used with their peels intact, resembling their natural state for planting. However, this preservation of the peels led to a notable disparity in true density. The presence of a gap between the garlic (white part) and the peel resulted in an overestimation of the clove’s volume, consequently leading to imprecise measurements. By determining the bulk density of a specific set of garlic cloves, it becomes feasible to obtain a representative parameter more effortlessly. The values for Young’s modulus and Poisson’s ratio proposed by Park et al. [19] were employed. The value suggested by Yu et al. [20] for the restitution coefficient was used as an interaction parameter. The static friction coefficients and friction angle between steel–garlic, and acryl–garlic were determined via a sliding test, as shown in Figure 8. The garlic clove was placed on a plate made of the material to be measured, which was then slowly tilted; the angle at which the garlic clove began to move was considered the friction angle $\left(\varphi \right)$ and the static friction coefficient was defined as $\tan \left(\varphi \right).$ Guo et al. [9] applied the same method to determine the static friction coefficient between garlic and steel plate. Finally, since fabricating a garlic plate is infeasible, the garlic–garlic static friction coefficient was determined through repose angle calibration.

The repose angle calibration refers to the process of adjusting the garlic–garlic friction coefficient of the model such that the same value as the repose angle obtained through the actual test is obtained in the simulation result. For accurate calibration, fixed values should be used for the rest of the parameters except for the friction coefficient [21]. In this study, as shown in Table 1, calibration was performed after fixing the remaining values except for the garlic–garlic static friction coefficient. For the repose angle test, a cylinder lifting test (CLT) was performed, as shown in Figure 9 [22]. A total of eight repose angles were measured at 45° intervals in one test, and the reliability of the test results was improved by repeating the test three times. The measurement of the repose angle involved using a digital protractor attached to a steel plate. The steel plate was adjusted to be parallel to the pile of garlic cloves, and the angle of the steel plate was measured. When using large-sized garlic, the reproducibility of test results may not be secured owing to limited boundary conditions; hence, Group 1, which has the smallest size, was used. In addition, the CLT method can be affected by the lifting velocity according to the friction coefficient between the cylinder and the garlic [23]. Therefore, the dynamic effect was removed by performing CLT at a speed of 5 mm/s [22]. Consequently, an average of 30.53° and a standard deviation of 1.26° were obtained.

Figure 10a shows the actual repose angle test, and Figure 10b illustrates the response angle simulation for calibration purposes. An analysis was conducted by incrementally increasing the garlic–garlic friction coefficient by 0.1 from 0.3 to match the repose angle obtained from the test. Figure 11 shows the simulation results. Using data interpolation, it was confirmed that when the friction coefficient was 0.46, the angle of repose of 30.53° matched the test results.

2.4. DEM Contact Model

2.4.1. Normal Force Model: Hysteretic Linear Spring Model

The study employed the hysteretic linear spring model proposed by Walton and Braun [23] to define contact in the normal direction of the discrete element model. This model is characterized by a rapid computation speed of plastic energy dissipation and is suitable as a model for compressible materials, such as garlic (ESSS Rocky [24]). The formula used to calculate the contact force in the normal direction is as follows.

$$F_n^t=\left\{\begin{array}{c}\min \left(K_{nl}{s}_n^t,F_n^{t-∆t}+K_{nu}∆s_n\right) if ∆s_n\ge 0\\ \max \left(F_n^{t-∆t}+K_{nu}∆s_n,\lambda K_{nl}{s}_n^t\right) if ∆s_n<0\end{array}\right.,$$

(1)

$$∆s_n=s_n^t-s_n^{t-∆t},$$

(2)

where:

$F_n^t$ is the normal elastic-plastic contact force at the current time $t$ (N)

$F_n^{t-∆t}$ is the normal elastic-plastic contact force at the previous time $t-∆t$ (N)

$∆s_n$ is the change in the contact normal overlap during the current time (mm)

$K_{nl}$ is the loading contact stiffness (N/mm)

$K_{nu}$ is the unloading contact stiffness (N/mm)

$\lambda$ is a dimensionless small constant (its value in Rocky is 0.001) (-).

$$\frac{1}{K_{nl}}=\left\{\begin{array}{c}\frac{1}{K_{nl,p_1}}+\frac{1}{K_{nl,p_2}} for particle - particle contact\\ \frac{1}{K_{nl,p}}+\frac{1}{K_{nl,p}} for particle - boundary contact\end{array}\right.$$

(3)

$$K_{nu}=\frac{K_{nl}}{{\epsilon }^2}$$

(4)

Here:

$K_{nl,p_1}$ is the loading contact stiffness of particle one (N/mm)

$K_{nl,p_2}$ is the loading contact stiffness of particle two (N/mm)

$\epsilon$ is the coefficient of restitution (-)

$$K_{nl,p}=E_{p}L$$

(5)

$$K_{nl,b}=E_{b}L$$

(6)

Here:

$E_p$ is the particle material’s bulk Young’s modulus (Pa)

$E_b$ is the boundary’s bulk Young’s modulus (Pa)

$L$ is the particle size (mm)

2.4.2. Tangential Force Model: Linear Spring Coulomb Limit Model

Calculating the contact force in the tangential direction utilizes a linear spring Coulomb limit model. This model is categorized as an elastic-frictional model; the tangential force for purely elastic contact is computed using Equations (7) and (8), while the final tangential force uses Equation (9). This step is necessary as the tangential contact force must not surpass the Coulomb friction force.

$$F_{\tau ,e}^t=F_{\tau }^{t-∆t}-K_{\tau }∆s_{\tau }$$

(7)

Here:

$F_{\tau ,e}^t$ is the value of the tangential force at the current time (N)

$F_{\tau }^{t-∆t}$ is the value of the tangential force at the previous time (N)

$∆s_{\tau }$ is the tangential relative displacement of the particles during the timestep (mm)

$K_{\tau }$ is the tangential stiffness, which is defined as:

$$K_{\tau }=r_K{K}_{nl},$$

(8)

where:

$r_K$ is the tangential stiffness ratio (-).

$$F_{\tau }^t=\min \left(\left|F_{\tau ,e}^t\right|,\mu F_n^t\right)\frac{F_{\tau ,e}^t}{\left|F_{\tau ,e}^t\right|},$$

(9)

where:

$\mu$ is the friction coefficient (-).

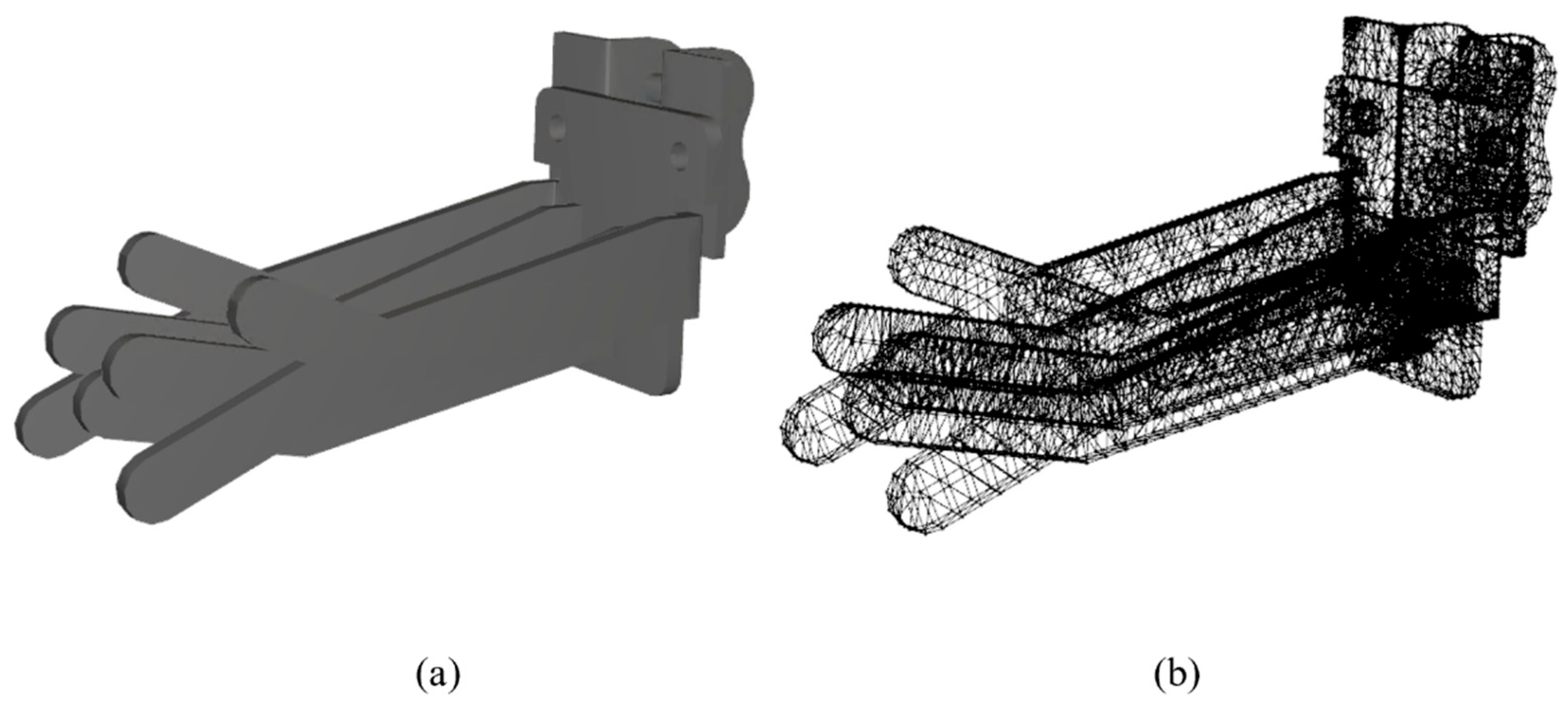
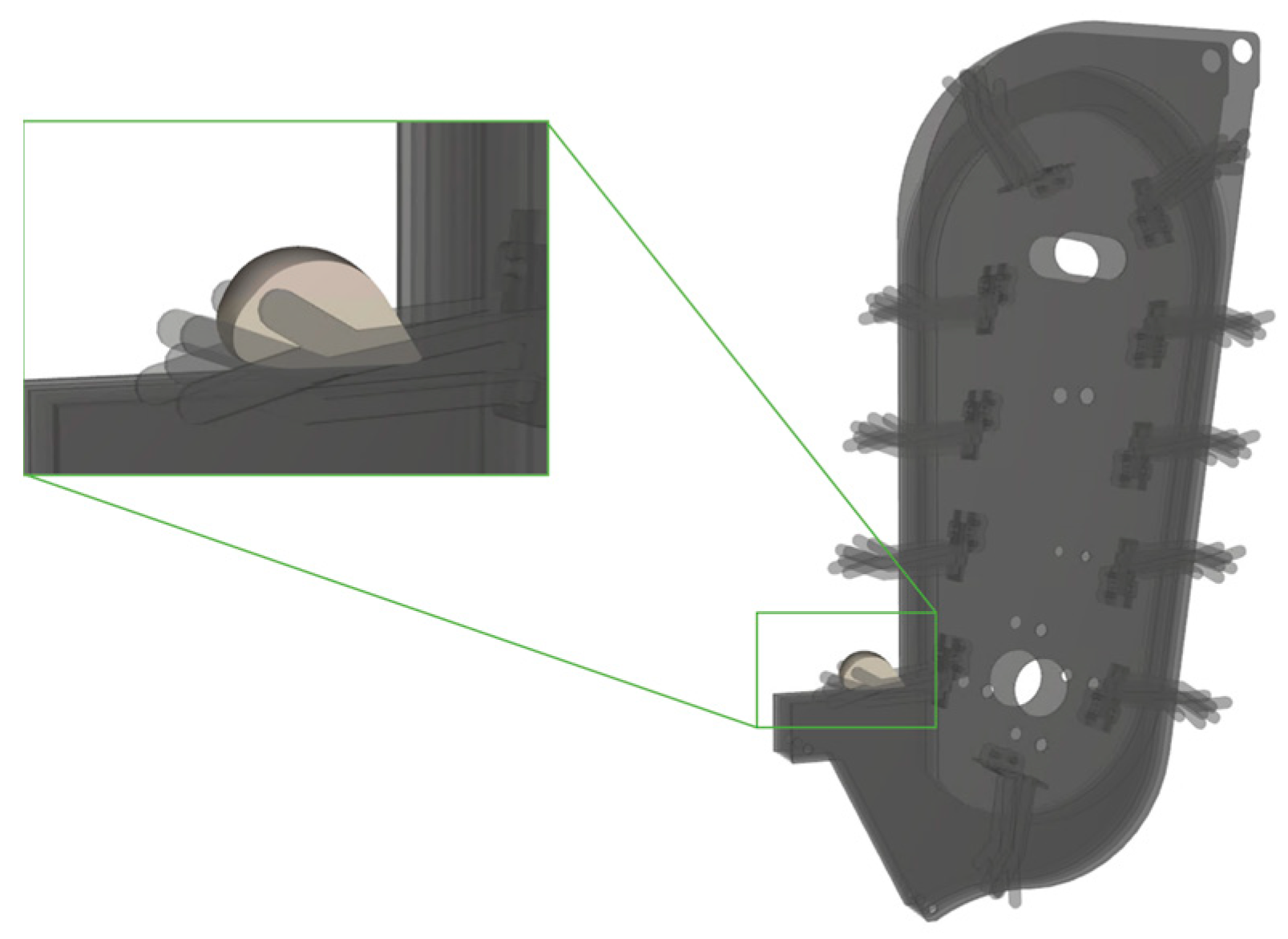
2.5. Bucket Size Optimization Using DEM

A simulation model was created to optimize the bucket size of the metering device using the commercial software ANSYS Rocky for the DEM [18]. The bucket optimization method proposed by Im et al. [8] was employed to determine the optimal bucket size for each group. There exists a linear relationship between garlic size and optimal bucket size, and the sequential search method was used to optimize the design parameter by linearly decreasing the bucket size [8,25]. In the sorting process of the metering device, excess garlic cloves, which are more than one clove, are preferable to fall into the hopper via the guide. The rate at which the bucket was exhibited to be too small to contain a clove was defined as the missing plant rate. The target metering device is required to achieve a plant rate of 97.5% or more while simultaneously minimizing the multi-plant rate, as described in Equation (13). Although a bucket size larger than necessary is advantageous for achieving a plant rate of 97.5% or more, it may increase the multi-plant rate. Therefore, it is desirable to determine the smallest bucket size that satisfies the target plant rate.

$$Q=\left(1-\frac{N_2}{N}\right)\times 100,$$

(10)

$$M=\frac{N_1}{N}\times 100,$$

(11)

$$C=\frac{N_2}{N}\times 100,$$

(12)

where:

$Q$ is plant rate (%)

$M$ is multi-plant rate (%)

$C$ is missing plant rate (%)

$N$ is the total number of trials (-)

$N_1$ is the number of metering trials with multiple cloves (-)

$N_2$ is the number of metering trials with empty bucket (-)

$$\begin{gathered} minimize:M \\ subject to: Q\ge 97.5 \end{gathered}$$

(13)

The elongated model depicted in Figure 4a was utilized to achieve the target plant rate. The model was constructed by setting the length that determines the center of gravity of garlic cloves as ${\mu }_L+{2\sigma }_L$. Assuming a normal distribution of garlic clove lengths, all cloves, except for the top 2.5% of the group, are successfully planted if they pass safely through the metering device [8]. Figure 12 illustrates the bucket size optimization simulation and displays the initial posture of the garlic clove for simulation purposes. The most unfavorable condition for the metering operation was selected as the initial location of the garlic clove to attain the target plant rate. The mechanical vibration and geometrical characteristics of the finger-type bucket ensure that garlic cloves are always positioned parallel to the bucket; when the center of gravity faces outward, it is disadvantageous for planting. Furthermore, due to the rolling phenomenon, when the round side of the garlic clove faces the bottom, the center of gravity moves inside the bucket trajectory, which can be beneficial for metering. Therefore, the flat side was arranged to face the bottom for the most unfavorable condition.

The dynamics model provided by ANSYS Rocky was utilized, and a rotational motion of 30 rpm was set on the lower sprocket, consistent with the actual operating conditions to establish the operation of the metering device. To carry out the sequential search method, the garlic clove model was positioned in the initial location in the most unfavorable orientation for planting, and multiple simulations were conducted while incrementally increasing the metering device guide angle (α) depicted in Figure 2a by 0.1°. The optimal bucket size was determined as the size immediately prior to the garlic clove of each group falling into the hopper via the guide. The design parameter interval was established to ensure applicability to the actual system as 0.1°, adjustable by the user.

2.6. Metering Simulation Using DEM

To validate the optimal bucket size of each group obtained through Section 2.5, a metering simulation was conducted using the DEM. The bucket size of the metering device was set to the optimum value obtained earlier, and the lower sprocket was rotated at 30 rpm, identical to the actual operating condition. Figure 13 depicts the metering simulation, demonstrating the sorting process that garlic cloves go through after being placed in the hopper. By incorporating the virtual sensor into the simulation model, as shown in Figure 13, the number of garlic cloves in the bucket that passed through the sorting area was analyzed in the time domain. The garlic clove model for metering simulation was based on statistical information on the shape of garlic cloves and used the average model illustrated in Figure 4b. Furthermore, for various sizes, garlic clove models were scaled up or down based on a Gaussian distribution and randomly placed in the hopper to perform metering simulations for various sizes. The software provides a scale distribution method to consider different sizes when the mean and standard deviation information is available [18]. The morphological statistical information of garlic cloves to define the Gaussian distribution was based on the values provided by Im et al. [8].

2.7. Metering Experiment

An actual metering experiment was conducted using a real metering device to validate the reliability of the discrete element analysis model and optimization technique. Figure 14a illustrates the actual garlic planter, while Figure 14b depicts the 1-row metering test machine. A boundary plate was installed on a 7-row metering device to create a 1-row metering test, establishing the same boundary conditions as the simulation model. Fifty garlic cloves were selected from each group, and a metering test was conducted with the sprocket rotating at 30 rpm, using the optimal bucket size as in the metering simulation. The test verified the plant rate (Q), multi-plant rate (M), and missing plant rate (C), and to ensure the reliability of the test results, it was repeated five times. The average value of each result was compared to the simulation result to validate the optimization methodology.

3. Results and Discussions

3.1. Bucket Size Optimization & Metering Simulation

This study aimed to optimize the bucket size of the metering device by initially positioning the garlic clove model under the most unfavorable conditions for metering. The optimal solution was then determined by gradually reducing the bucket size using the sequential search method. The design parameter interval was set at 0.1°, which the user can adjust to ensure system applicability. The optimization process revealed that the optimal bucket size increased with the size of the garlic clove, and it was found that Group 1 had an optimal bucket size of 16.11 mm, Group 2 had 22.75 mm, and Group 3 had 23.64 mm.

Table 2. Optimal bucket size for each group of garlic.

Group	Optimum Bucket Size l (mm)
Group 1	16.11
Group 2	22.75
Group 3	23.64

summarizes the results of the bucket size optimization. A batch simulation of the metering operation was conducted to validate the accuracy of optimization results. Once the optimal bucket size was obtained through the sequential search method, a metering simulation at the optimal setting was conducted for garlic of various sizes in each group, and the results are shown in

Table 3. Results of metering simulation.

Group	No. Trials	Plant Rate (Q)	Multi-Plant Rate (M)	Missing Plant Rate (C)
Group 1	40	92.50%	10.00%	7.50%
Group 2	37	97.30%	2.70%	2.70%
Group 3	43	97.70%	2.30%	2.30%

. Some 40 metering operations were performed for Group 1, 37 for Group 2, and 43 for Group 3 to enhance the reliability of the metering performance results.

Except for Group 1, all groups were able to nearly achieve the target plant rate of 97.5%. Moreover, the multi-plant rate demonstrated a low level of under 3%. Group 1, being the smallest in size, exhibited the highest multi-plant rate at 10% and demonstrated poor metering performance. It is believed that the overlapping planting of more than one garlic clove occurred in the width direction of the finger-type bucket for Group 1, as shown in Figure 15. Conversely, in the case of Groups 2 and 3, this phenomenon did not occur because the thickness of the garlic clove was large, considering the bucket width. As such, they were deemed the most suitable groups for applying the metering device.

3.2. Experimental Result

To validate the bucket size optimization technique, an actual metering test was conducted, as depicted in Figure 14, and the results are illustrated in

Table 4. Results of the metering experiment.

Group	Test No	Plant Rate (Q)	Multi-Plant Rate (M)	Missing Plant Rate (C)
Group 1	1	89.29%	0.00%	10.71%
	2	89.09%	1.82%	10.91%
	3	90.91%	0.00%	9.09%
	4	89.29%	0.00%	10.71%
	5	94.00%	6.00%	6.00%
	Average	90.44%	1.47%	9.56%
Group 2	1	100.00%	2.04%	0.00%
	2	97.92%	6.25%	2.08%
	3	97.96%	4.08%	2.04%
	4	95.92%	2.04%	4.08%
	5	98.04%	0.00%	1.96%
	Average	97.97%	2.85%	2.03%
Group 3	1	97.56%	2.44%	2.44%
	2	97.62%	0.00%	2.38%
	3	93.18%	0.00%	6.82%
	4	93.18%	0.00%	6.82%
	5	93.18%	0.00%	6.82%
	Average	94.95%	0.49%	5.05%

. The test was executed under conditions identical to the analysis model, including bucket size and rotational speed, to validate the optimization process using simulation. The test was repeated five times using 50 garlic cloves in each group to enhance the reliability of the test results. Average plant rates of 90.44% for Group 1, 97.97% for Group 2, and 94.95% for Group 3 were confirmed. It was observed that all groups except Group 1 came close to the target plant rate of 97.5%. Additionally, it was confirmed that Group 1 had a multi-plant rate of 1.47%, Group 2 had a rate of 2.85%, and Group 3 had a rate of 0.49%. These findings demonstrate significantly improved metering performance compared to the test results of Kang et al. [3].

Table 5. Comparison between simulation and experiment results.

Group	Index	Simulation	Experiment	Difference
Group 1	Plant rate (Q)	92.50%	90.44%	2.10%
	Multi-plant rate (M)	10.00%	1.50%	8.50%
	Missing plant rate (C)	7.50%	9.60%	−2.10%
Group 2	Plant rate (Q)	97.30%	97.97%	−0.70%
	Multi-plant rate (M)	2.70%	2.90%	−0.20%
	Missing plant rate (C)	2.70%	2.00%	0.70%
Group 3	Plant rate (Q)	97.70%	94.95%	2.80%
	Multi-plant rate (M)	2.30%	0.50%	1.80%
	Missing plant rate (C)	2.30%	5.10%	−2.80%

presents the simulation and test results for the plant rate, multi-plant rate, and missing plant rate. It was confirmed that the plant rate for both results had a maximum error of 2.8% for Group 3. The difference between the two results for the multi-plant rate was 8.5% for Group 1, 0.2% for Group 2, and 1.8% for Group 3. Lastly, for the missing plant rate, a relatively low level of error was confirmed, with a maximum error of 2.8% for Group 3. For Group 1, due to the relatively narrow width of cloves, multiple garlic cloves could be stacked in the bucket, as shown in Figure 15, and the metering performance was affected by both the width of the bucket and the length of the garlic cloves. The bucket size optimization technique applied in this study considered the length of garlic cloves as the only independent variable and not the effect of bucket width. Consequently, both simulation and test results displayed poor metering performance, and the reliability of the optimal solution was not fully guaranteed for Group 1. As a result, there was a relatively significant difference between the analysis and test results in Group 1 compared to those of Groups 2 and 3. Upon comparing the analysis and test results, it was determined that the analysis model predicted the metering performance of the actual metering device within 10% of all performance indicators, including plant rate, multi-plant rate, and missing plant rate. Particularly for Groups 2 and 3, the high reliability of the analysis model was confirmed, showing a difference in the metering performance of a maximum of 2.8% (plant rate of Group 3).

4. Conclusions

In this investigation, the bucket size of the garlic planter metering device was optimized by utilizing the DEM, considering the size of various garlic cloves. To enhance the precision of the analysis model, the actual shape of the garlic cloves was incorporated using a 3D mesh, and the mechanical properties of garlic were found through various tests, such as the angle of repose test and sliding test. Subsequently, the optimal bucket size was determined for the three garlic size groups using the sequential search method based on the developed model. A metering simulation was carried out to validate the optimal value, and finally, a physical test was conducted under the same conditions to ensure the reliability of the optimization technique. The primary findings of the study are summarized as follows.

(1)

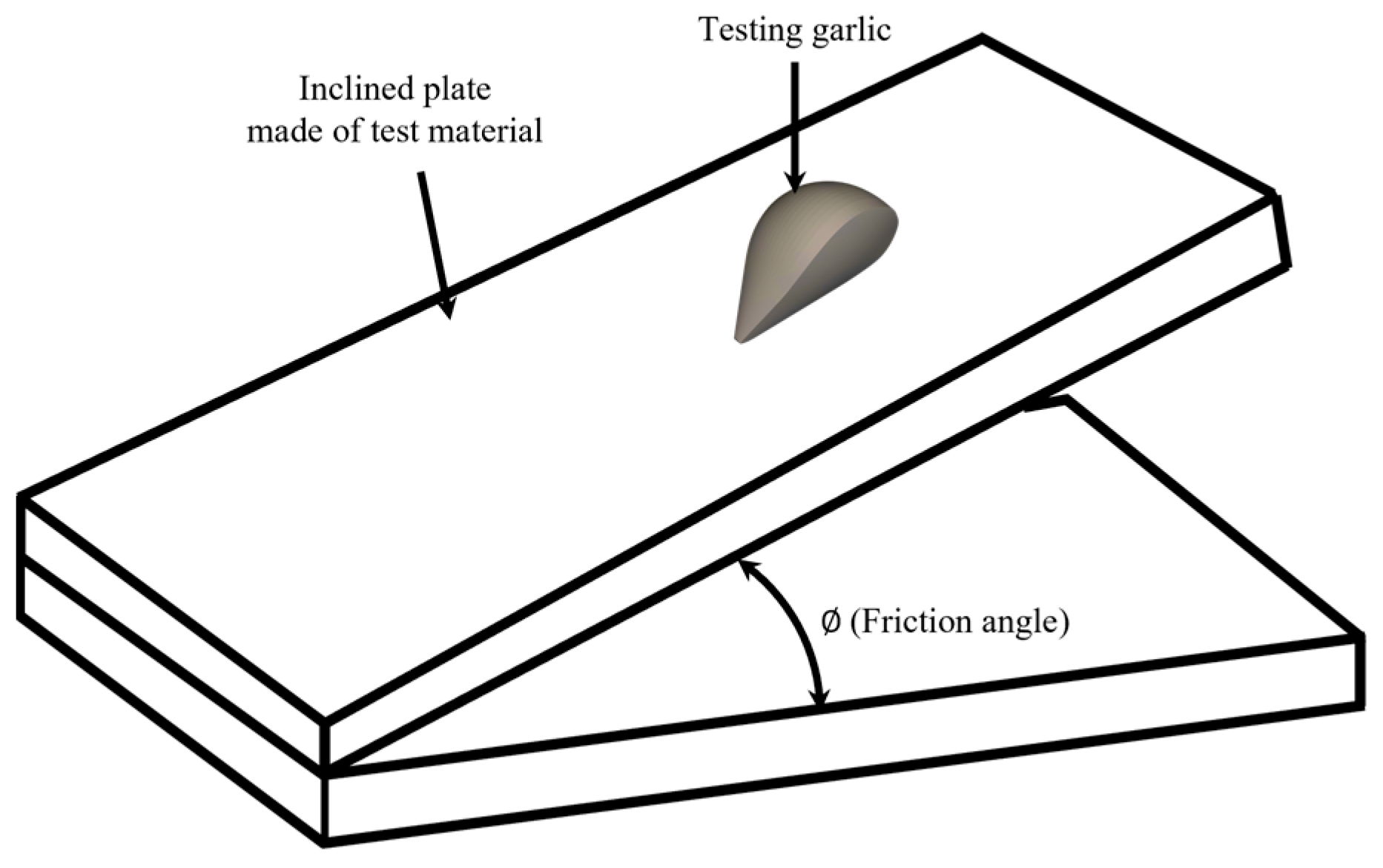
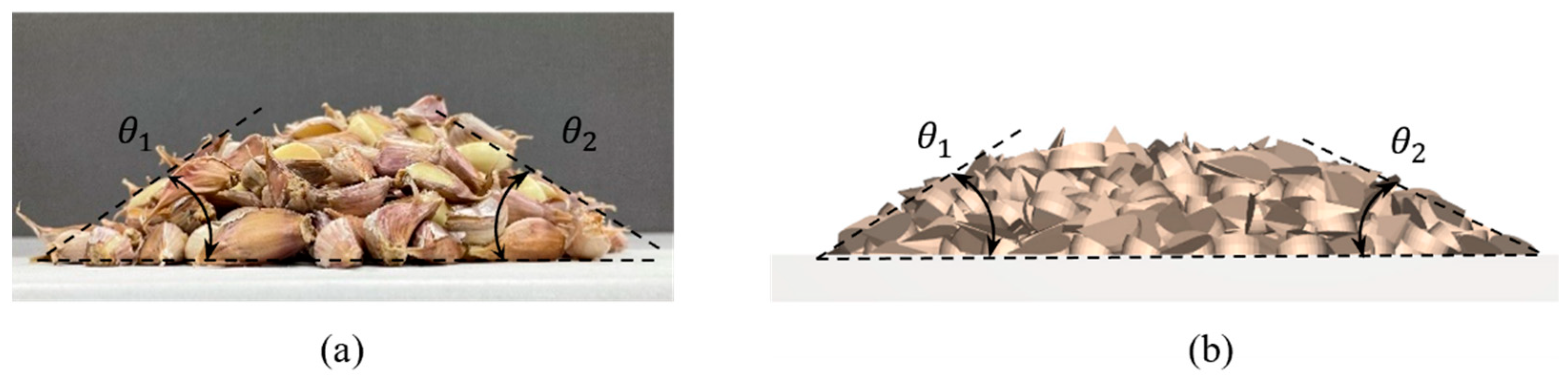
Calibration was executed by conducting a repose angle test to establish the garlic-garlic friction coefficient. It was ascertained that the simulation model and the test produced the same repose angle when the garlic-garlic friction coefficient was 0.46.

(2)

Bucket size optimization was carried out to achieve the target metering rate of 97.5%. Subsequently, it was determined that Group 1 had an optimal bucket size of 16.11 mm, Group 2 had that of 22.75 mm, and Group 3 had that of 23.64 mm.

(3)

To validate the reliability of the determined optimal bucket size, a metering simulation was conducted, which showed that the plant rate was 90.44% for Group 1, 97.97% for Group 2, and 94.95% for Group 3. The results indicate that Groups 2 and 3 achieved a seeding rate close to the target value of 97.5%.

(4)

To validate the reliability of the optimization methodology, an actual metering test was conducted under identical conditions. Upon comparing the metering performance, it was confirmed that all metering performances exhibited differences of less than 10%. Notably, in Groups 2 and 3, the difference in metering performance was within 2.8%, thus confirming the reliability of the optimization technique.

(5)

Regarding Group 1, as depicted in Figure 15, the garlic clove widths were relatively narrow, leading to an overlap phenomenon. Consequently, the metering performance prediction exhibits low accuracy, and the metering performance is inferior to Groups 2 and 3.

The DEM was employed to confirm the optimal bucket size for each garlic group, and during actual metering work, the optimal bucket size resulted in nearly satisfactory target plant rates. Moreover, the proposed methodology enables the optimal design of various agricultural implements with similar mechanisms. Notably, this study solely considered the length of the finger-type bucket in the bucket optimization process, while the width was not taken into account. As a result, while Groups 2 and 3 met the target plant rate, Group 1 did not. Future studies will enhance the optimization completeness by incorporating the width as well as the bucket size.