Nickel Chalcogenide Nanoparticles-Assisted Photothermal Solar Driven Membrane Distillation (PSDMD)
Abstract
:1. Introduction
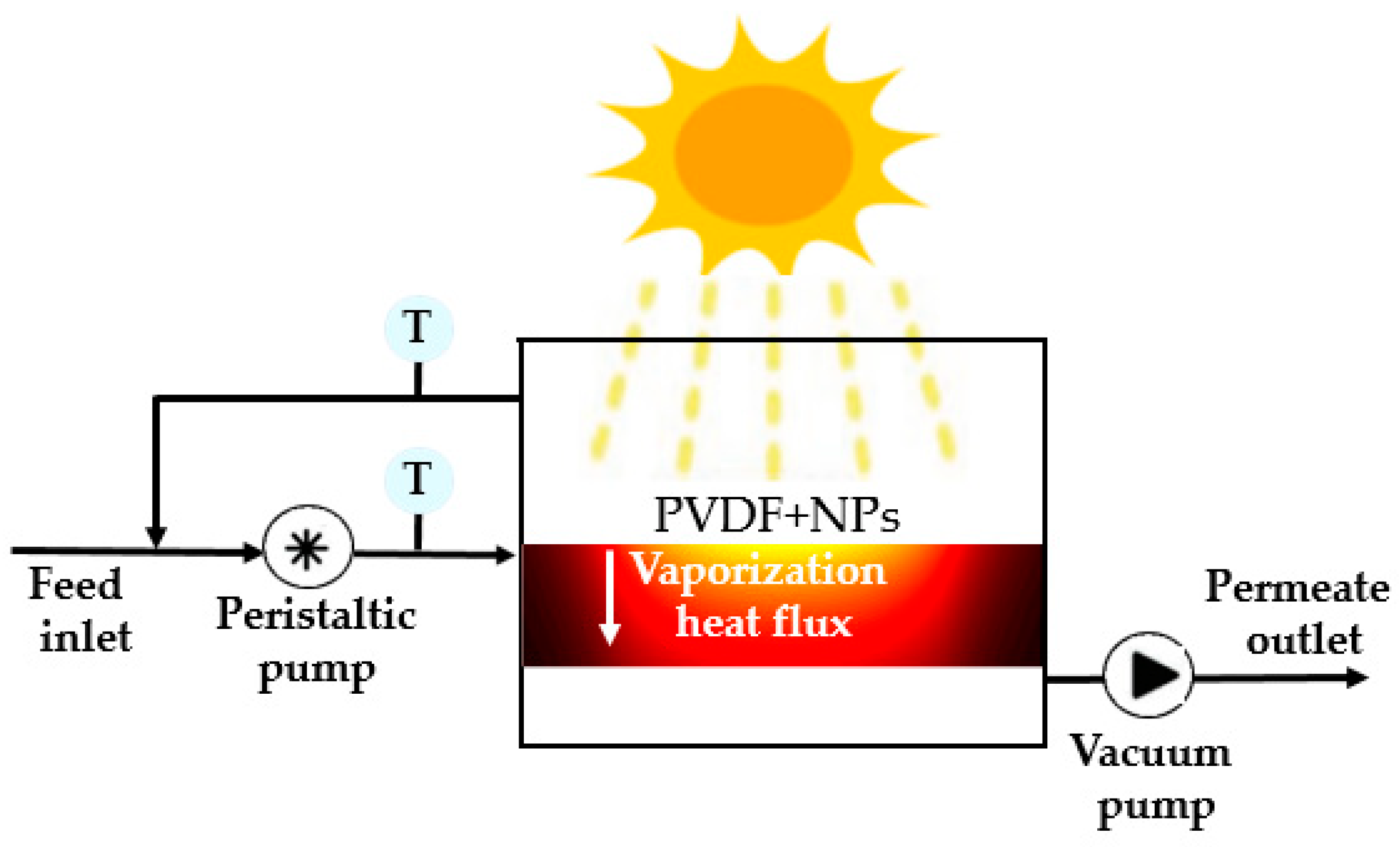
2. Materials and Methods
3. Results and Discussions
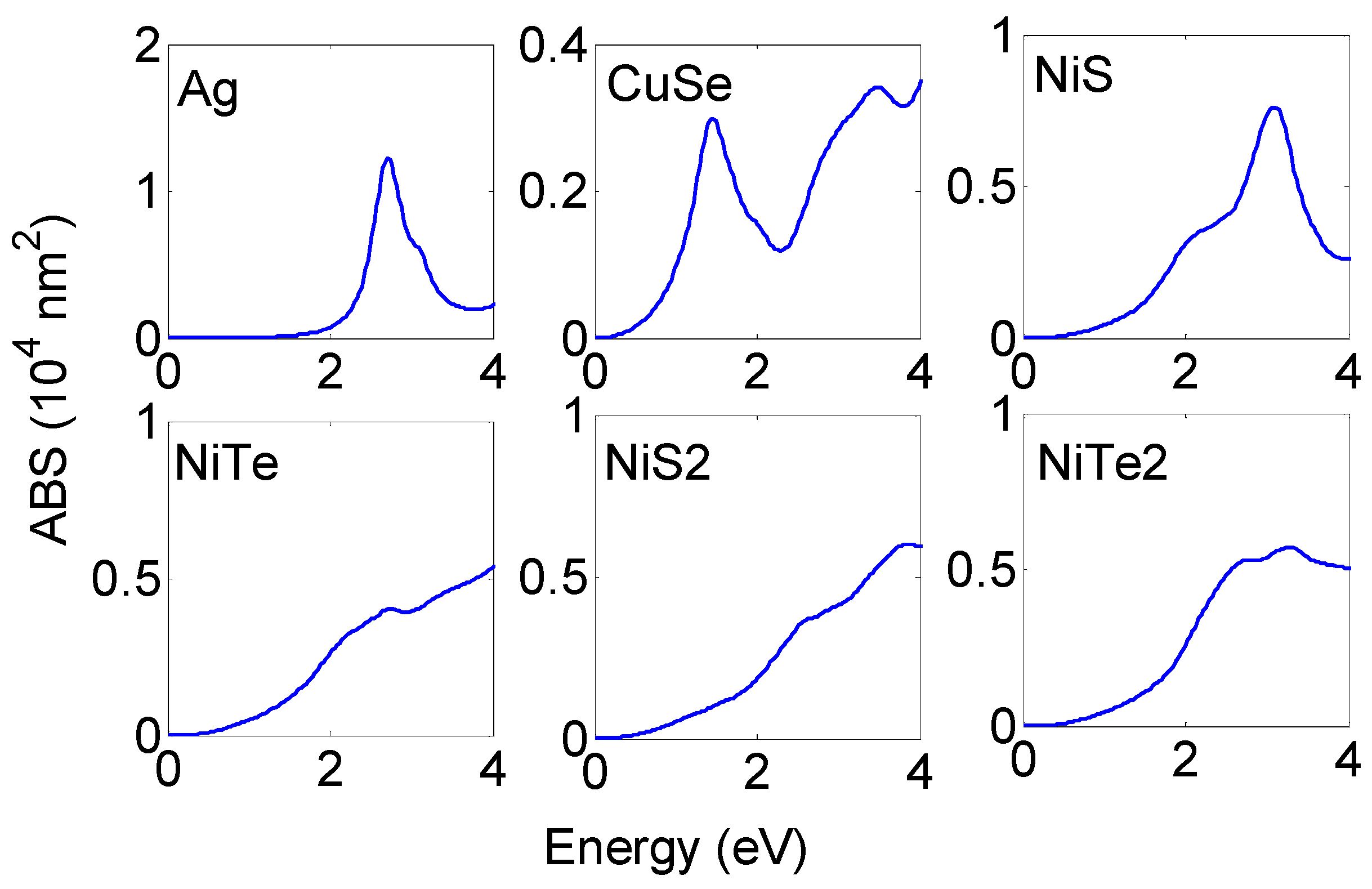
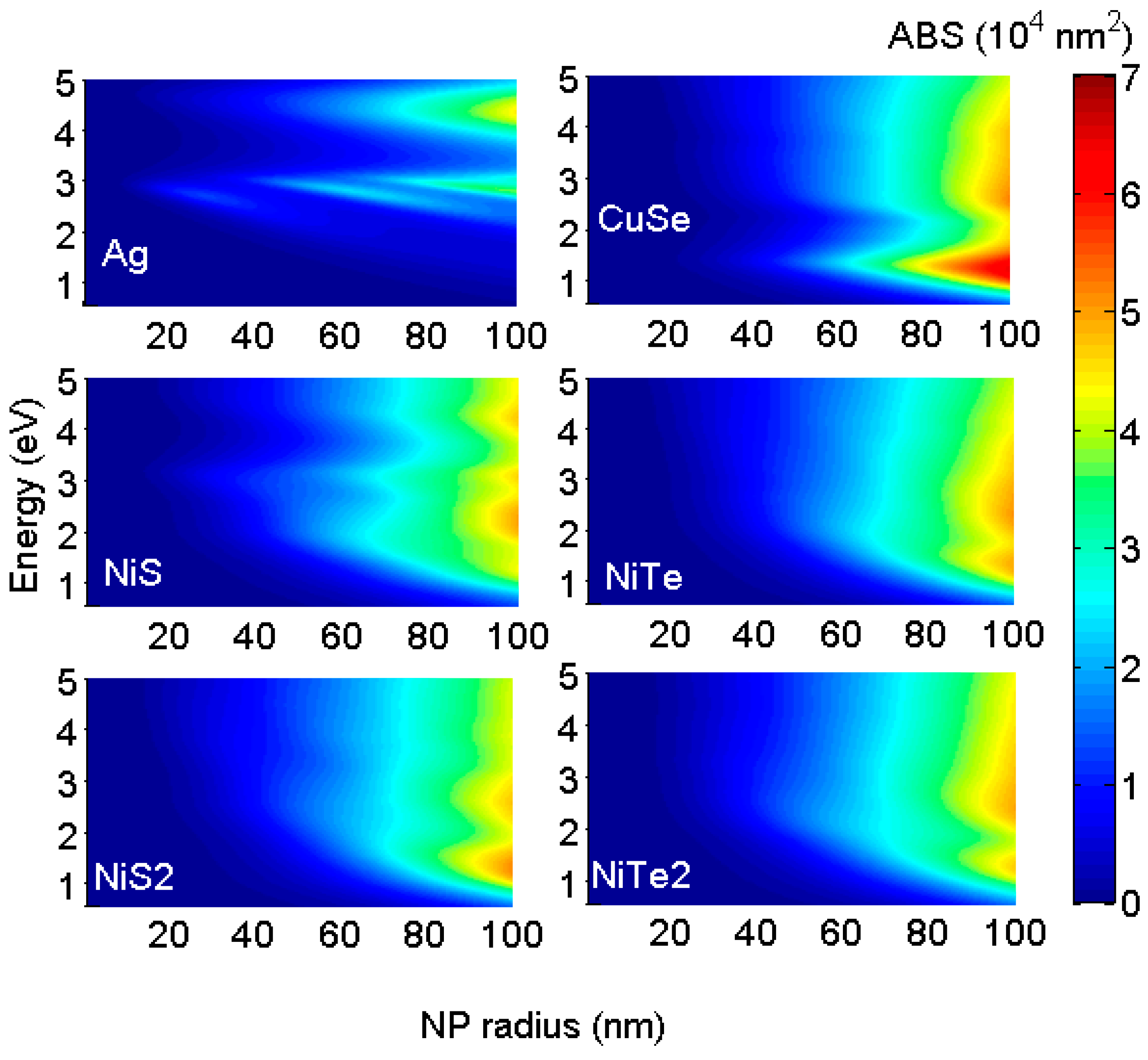
3.1. Nanoparticles Absorption Cross Section
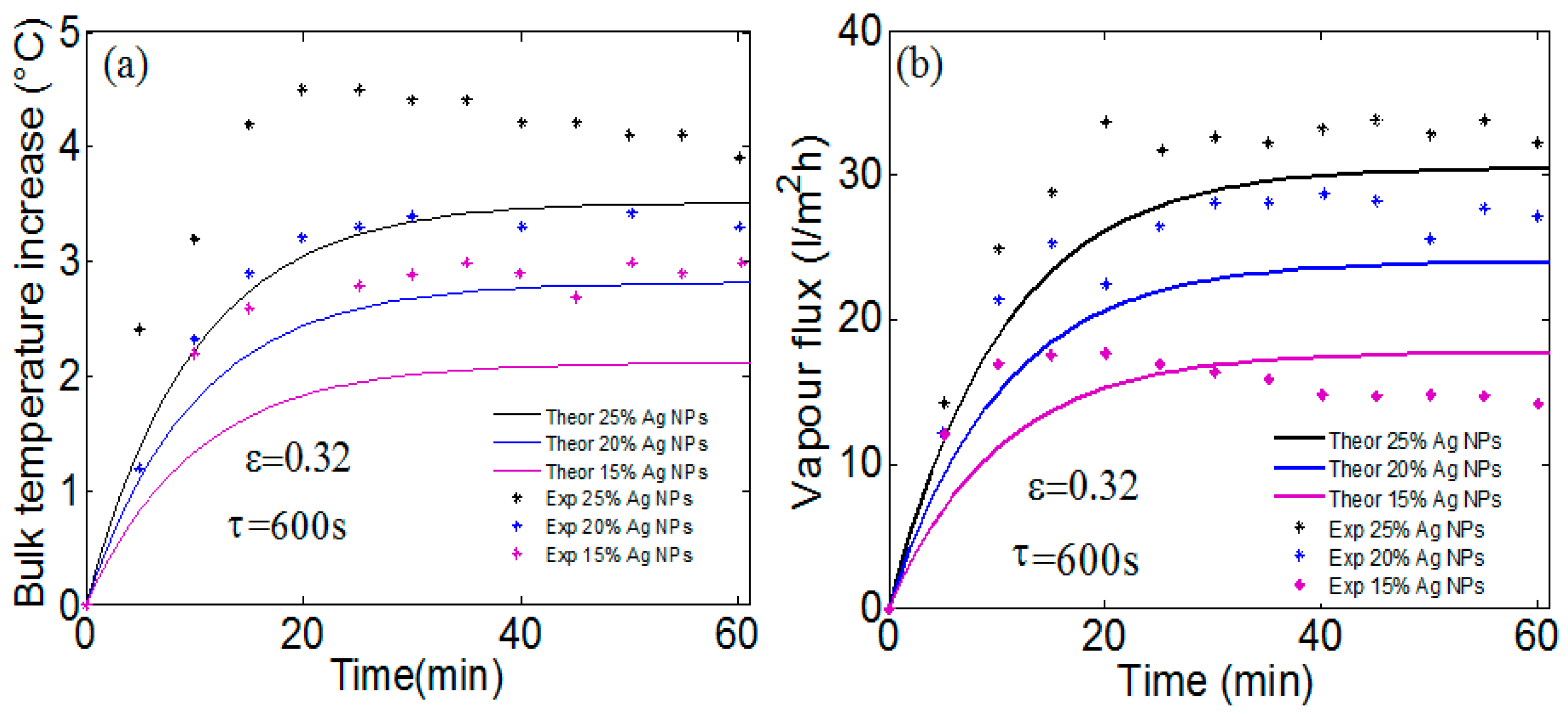
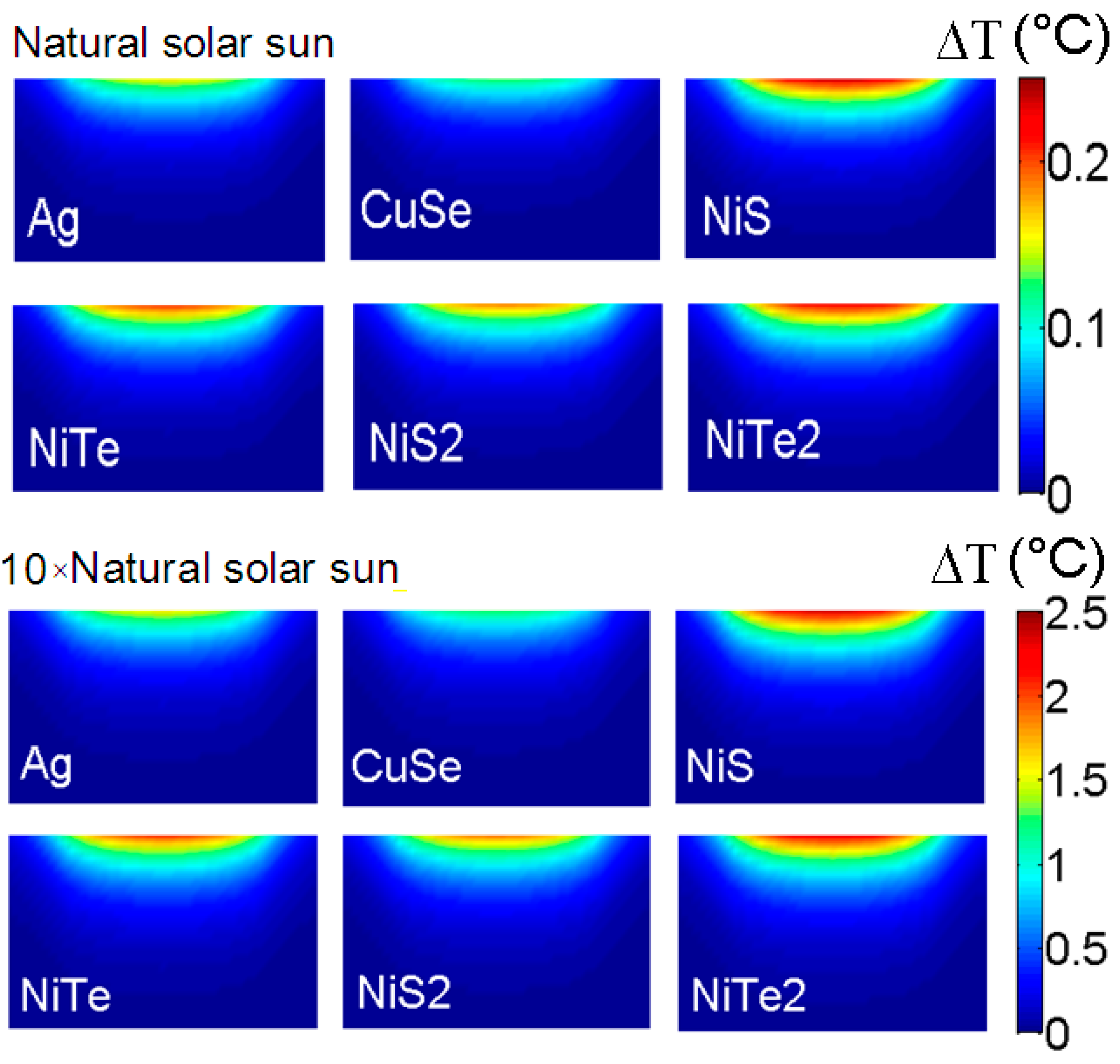
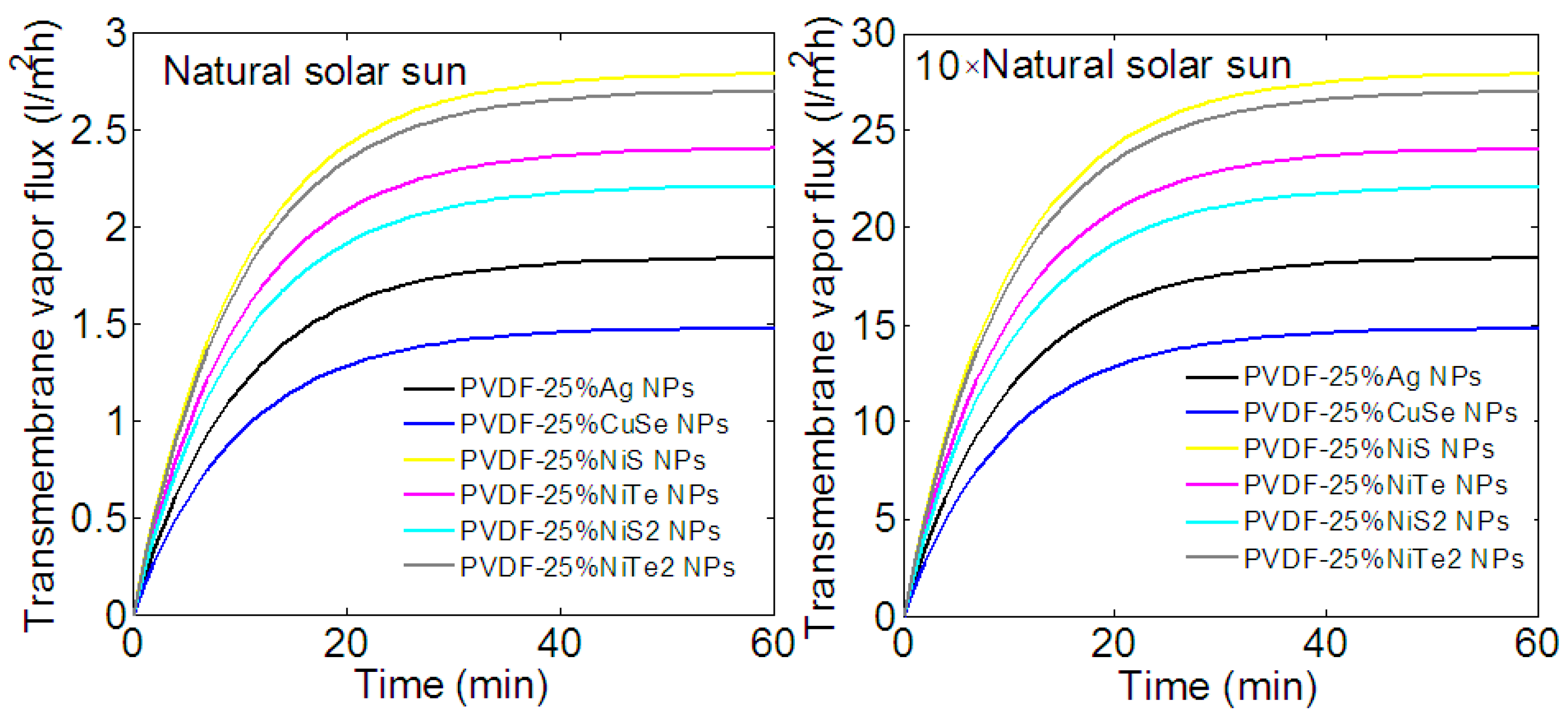
3.2. Photothermal Membrane Temperature and Vapor Flux
3.3. Membrane Efficiency
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhou, L.; Tan, Y.; Wang, J.; Xu, W.; Yuan, Y.; Cai, W.; Zhu, S.; Zhu, J. 3D self-assembly of aluminium nanoparticles for plasmon-enhanced solar desalination. Nat. Photonics 2016, 10, 393–398. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The Future of Seawater Desalination: Energy, Technology, and the Environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Service, R.F. Desalination Freshens Up. Science 2006, 313, 1088–1090. [Google Scholar] [CrossRef] [PubMed]
- Greenlee, L.F.; Lawler, D.F.; Freeman, B.D.; Marrot, B.; Moulin, P. Reverse osmosis desalination: Water sources, technology, and today’s challenges. Water Res. 2009, 43, 2317–2568. [Google Scholar] [CrossRef]
- Lee, K.P.; Arnot, T.C.; Mattia, D. A review of reverse osmosis membrane materials for desalination—Development to date and future potential. J. Membr. Sci. 2011, 370, 1–22. [Google Scholar] [CrossRef]
- Al-Shammiri, M.; Safar, M. Multi-effect distillation plants: State of the art. Desalination 1999, 126, 45–59. [Google Scholar] [CrossRef]
- Kiss, A.A.; Flores Landaeta, S.J.; Infante Ferreira, C.A. Towards energy efficient distillation technologies—Making the right choice. Energy 2012, 47, 531–542. [Google Scholar] [CrossRef]
- Abdelkareem, M.A.; Assad, M.E.; Sayed, E.T.; Soudan, B. Recent progress in the use of renewable energy sources to power water desalination plants. Desalination 2018, 435, 97–113. [Google Scholar] [CrossRef]
- Hogan, P.A.; Fane, A.G.; Morrison, G.L. Desalination by solar heated membrane distillation. Desalination 1991, 81, 81–90. [Google Scholar] [CrossRef]
- Lawson, K.W.; Lloyd, D.R. Review: Membrane distillation. J. Membr. Sci. 1997, 124, 1–25. [Google Scholar] [CrossRef]
- Gryta, M. Effectiveness of Water Desalination by Membrane Distillation Process. Membranes 2012, 2, 415–429. [Google Scholar] [CrossRef] [PubMed]
- Drioli, E.; Ali, A.; Macedonio, F. Membrane distillation: Recent developments and perspectives. Desalination 2015, 356, 56–84. [Google Scholar] [CrossRef]
- Wang, P.; Chung, T.-S. Recent advances in membrane distillation processes. J. Membr. Sci. 2015, 474, 39–56. [Google Scholar] [CrossRef]
- El-Bourawi, M.S.; Ding, Z.; Ma, R.; Khayet, M. A framework for better understanding membrane distillation separation process. J. Membr. Sci. 2006, 285, 4–29. [Google Scholar] [CrossRef]
- Schofield, R.; Fane, A.G.; Fell, C.J.D.; Macoun, R. Factors affecting flux in membrane distillation. Desalination 1990, 77, 279–294. [Google Scholar] [CrossRef]
- Politano, A.; Di Profio, G.; Fontananova, E.; Sanna, V.; Cupolillo, A.; Curcio, E. Overcoming temperature polarization in membrane distillation by thermoplasmonic effects activated by Ag nanofillers in polymeric membranes. Desalination 2019, 451, 192–199. [Google Scholar] [CrossRef]
- Elmaghraoui, D.; Politano, A.; Jaziri, S. Photothermal response of plasmonic nanofillers for membrane distillation. J. Chem. Phys. 2020, 152, 114102. [Google Scholar] [CrossRef]
- Santoro, S.; Avci, A.H.; Politano, A.; Curcio, E. The advent of thermoplasmonic membrane distillation. Chem. Soc. Rev. 2022, 51, 6087–6125. [Google Scholar] [CrossRef]
- Dongare, P.D.; Alabastri, A.; Pedersen, S.; Zodrow, K.R.; Hogan, N.J.; Neumann, O.; Wu, J.; Wang, T.; Deshmukh, A.; Elimelech, M.; et al. Nanophotonics-enabled solar membrane distillation for off-grid water purification. Proc. Natl. Acad. Sci. USA 2017, 114, 6936–6941. [Google Scholar] [CrossRef]
- Santoro, S.; Aquino, M.; Seo, D.H.; Laan, T.V.D.; Lee, M.; Yun, J.S.; Park, M.J.; Bendavid, A.; Shon, H.K.; Avci, A.H.; et al. Dimensionally controlled graphene-based surfaces for photothermal membrane crystallization. J. Colloid Interface Sci. 2022, 623, 607–616. [Google Scholar] [CrossRef]
- Han, X.; Wang, W.; Zuo, K.; Chen, L.; Yuan, L.; Liang, J.; Li, Q.; Ajayan, P.M.; Zhao, Y.; Lou, J. Bio-derived ultrathin membrane for solar driven water purification. Nano Energy 2019, 60, 567–575. [Google Scholar] [CrossRef]
- Dongare, P.D.; Alabastri, A.; Neumanna, O.; Nordlander, P.; Halas, N.J. Solar thermal desalination as a nonlinear optical process. Proc. Natl. Acad. Sci. USA 2019, 116, 13183–13187. [Google Scholar] [CrossRef]
- Gao, M.; Peh, C.K.; Meng, F.L.; Ho, G.W. Photothermal Membrane Distillation toward Solar Water Production. Small Methods 2021, 5, 2001200. [Google Scholar] [CrossRef]
- Ho, C.D. Solar-Assisted Membrane Distillation. Membranes 2022, 12, 304. [Google Scholar] [CrossRef] [PubMed]
- Miao, Q.; Zhang, Y.; Cong, S.; Guo, F. Experimental Investigation on Floating Solar-Driven Membrane Distillation Desalination Modules. Membranes 2021, 11, 304. [Google Scholar] [CrossRef] [PubMed]
- Mustakeem, M.; El-Demellawi, J.K.; Obaid, M.; Ming, F.; Alshareef, H.N.; Ghaffour, N. MXene-Coated Membranes for Autonomous Solar-Driven Desalination. ACS Appl. Mater. Interfaces 2022, 14, 5265–5274. [Google Scholar] [CrossRef]
- Zhang, B.; Wong, P.W.; Guo, J.; Zhou, Y.; Wang, Y.; Sun, J.; Jiang, M.; Wang, Z.; An, A.K. Transforming Ti3C2Tx MXene’s intrinsic hydrophilicity into superhydrophobicity for efficient photothermal membrane desalination. Nat. Commun. 2022, 13, 3315. [Google Scholar] [CrossRef]
- CK, P.P.; Kumaresan, G.; Abraham, R.; Santosh, R.; Velraj, R. Effect of Teflon-Coated PVDF Membrane on the Performance of a Solar-Powered Direct Contact Membrane Distillation System. Sustainability 2022, 14, 6895. [Google Scholar] [CrossRef]
- Shin, D.; Kang, G.; Gupta, P.; Behera, S.; Lee, H.; Urbas, A.M.; Park, W.; Kim, K. Thermoplasmonic and Photothermal Metamaterials for Solar Energy applications. Adv. Opt. Mater. 2018, 6, 1800317. [Google Scholar] [CrossRef]
- Xue, G.; Chen, Q.; Lin, S.; Duan, J.; Yang, P.; Liu, K.; Li, J.; Zhou, J. Highly Efficient Water Harvesting with Optimized Solar Thermal Membrane Distillation Device. Glob. Chall. 2018, 2, 1800001. [Google Scholar] [CrossRef]
- Zhong, W.; Hou, J.; Yang, H.-C.; Chen, V. Superhydrophobic membranes via facile bio-inspired mineralization for vacuum membrane distillation. J. Membr. Sci. 2017, 540, 98–107. [Google Scholar] [CrossRef]
- Li, Q.; Beier, L.J.; Tan, J.; Brown, C.; Lian, B.; Zhong, W.; Wang, Y.; Ji, C.; Dai, P.; Li, T.; et al. An integrated, solar-driven membrane distillation system for water purification and energy generation. Appl. Energy 2019, 237, 534–548. [Google Scholar] [CrossRef]
- Wu, J.; Zodrow, K.R.; Szemraj, P.B.; Li, Q. Photothermal nanocomposite membranes for direct solar membrane distillation. J. Mater. Chem. A 2017, 578, 23712. [Google Scholar] [CrossRef]
- Lia, W.; Chenb, Y.; Yaoc, L.; Rena, X.; Lia, Y.; Denga, L. Fe3O4/PVDF-HFP photothermal membrane with in-situ heating for sustainable, stable and efficient pilot-scale solar-driven membrane distillation. Desalination 2020, 478, 114288. [Google Scholar] [CrossRef]
- Chen, Y.R.; Xin, R.; Huang, X.; Zuo, K.; Tung, K.-L.; Li, Q. Wetting-resistant photothermal nanocomposite membranes for direct solar membrane distillation. J. Membr. Sci. 2021, 620, 118913. [Google Scholar] [CrossRef]
- Guan, Y.F.; Huang, B.C.; Qian, C.; Wang, L.F.; Yu, H.Q. Improved PVDF membrane performance by doping extracellular polymeric substances of activated sludge. Water Res. 2017, 113, 89–96. [Google Scholar] [CrossRef]
- Yang, R.; Li, R.; Zhang, L.; Xu, Z.; Kang, Y.; Xue, P. Facile synthesis of hollow mesoporous nickel sulfide nanoparticles for highly efficient combinatorial photothermal–chemotherapy of cancer. J. Mater. Chem. B 2020, 8, 7766. [Google Scholar] [CrossRef]
- Zhanga, X.; Wua, J.; Williamsb, G.R.; Niua, S.; Qiana, Q.; Li-Min Zhu, L.M. Functionalized MoS2-nanosheets for targeted drug delivery and chemo-photothermal therapy. Colloids Surf. B Biointerfaces 2019, 173, 101–108. [Google Scholar] [CrossRef]
- Wang, X.-M.; Huang, L.; Wang, Y.-J.; Xuan, L.; Li, W.W.; Tian, L.-J. Highly efficient near-infrared photothermal antibacterial membrane with incorporated biogenic CuSe nanoparticles. Chem. Eng. J. 2021, 405, 126711. [Google Scholar] [CrossRef]
- Abramovich, S.; Dutta, D.; Rizza, C.; Santoro, S.; Aquino, M.; Cupolillo, A.; Occhiuzzi, J.; La Russa, M.F.; Ghosh, B.; Farias, D.; et al. NiSe and CoSe Topological Nodal-Line Semimetals: A Sustainable Platform for Efficient Thermoplasmonics and Solar-Driven Photothermal Membrane Distillation. Small 2022, 18, 2201473. [Google Scholar] [CrossRef]
- Tavker, N.; Sharma, M. Designing of waste fruit peels extracted cellulose supported molybdenum sulfide nanostructures for photocatalytic degradation of RhB dye and industrial effluent. J. Environ. Manag. 2020, 255, 109906. [Google Scholar] [CrossRef] [PubMed]
- Ajibade, P.A.; Oluwalana, A.E.; Andrew, F.P. Morphological Studies, Photocatalytic Activity, and Electrochemistry of Platinum Disulfide Nanoparticles from Bis (morpholinyl-4-carbodithioato)-platinum(II). ACS Omega 2020, 5, 27142–27153. [Google Scholar] [CrossRef] [PubMed]
- Ge, Y.C.; Gao, S.P.; Dong, P.; Baines, R.; Ajayan, P.M.; Ye, M.X.; Shen, J.F. Insight into the hydrogen evolution reaction of nickel dichalcogenide nanosheets: Activities related to non-metal ligands. Nanoscale 2017, 9, 5538–5544. [Google Scholar] [CrossRef] [PubMed]
- De Silva, U.; Masud, J.; Zhang, N.; Hong, Y.; Liyanage, W.P.R.; Zaeem, M.A.; Nath, M. Nickel telluride as a bifunctional electrocatalyst for efficient water splitting in alkaline medium. J. Mater. Chem. A 2018, 6, 7608–7622. [Google Scholar] [CrossRef]
- Gao, M.R.; Xu, Y.F.; Jiang, J.; Yu, S.H. Nanostructured metal chalcogenides: Synthesis, modification, and applications in energy conversion and storage devices. Chem. Soc. Rev. 2013, 42, 2986–3017. [Google Scholar] [CrossRef]
- Reddy, P.L.; Deshmukh, K.; Kovářík, T.; Reiger, D.; Nambiraj, N.A.; Lakshmipathy, R.; Pasha, S.K. Enhanced dielectric properties of green synthesized Nickel Sulphide (NiS) nanoparticles integrated polyvinylalcohol nanocomposites. Mater. Res. Express 2020, 7, 064007. [Google Scholar] [CrossRef]
- Shen, L.; Chen, Z.X. Critical review of the impact of tortuosity on diffusion. Chem. Eng. Sci. 2007, 62, 3748–3755. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous electron gas. Phys. Rev. B 1964, 136, 864. [Google Scholar]
- Draxel, C.A.; Sofo, J.O. Linear optical properties of solids within the full-potential linearized augmented plane wave method. Comput. Phys. Commun. 2006, 175, 1. [Google Scholar]
- Schwarz, K.; Blaha, P.; Trickey, S.B. Electronic structure of solids with WIEN2k. Mol. Phys. 2010, 108, 3147. [Google Scholar]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation. Phys. Rev. 1965, 140, A1133. [Google Scholar]
- Yu, P.; Cardona, M. Fundamentals of Semiconductors Physics and Materials Properties; Springer: Berlin, Germany, 2010. [Google Scholar]
- Lide, R. David, CRC Handbook of Chemistry and Physics, 85th ed.; CRS Press: Boca Raton, FL, USA, 2004; p. 6. [Google Scholar]
- Buonomenna, M.G.; Lopez, L.C.; Favia, P.; d’Agostino, R.; Gordano, A.; Drioli, E. New PVDF membranes: The effect of plasma surface modification on retention in nanofiltration of aqueous solution containing organic compounds. Water Res. 2007, 41, 4309–4316. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elmaghraoui, D.; Ben Amara, I.; Jaziri, S. Nickel Chalcogenide Nanoparticles-Assisted Photothermal Solar Driven Membrane Distillation (PSDMD). Membranes 2023, 13, 195. https://doi.org/10.3390/membranes13020195
Elmaghraoui D, Ben Amara I, Jaziri S. Nickel Chalcogenide Nanoparticles-Assisted Photothermal Solar Driven Membrane Distillation (PSDMD). Membranes. 2023; 13(2):195. https://doi.org/10.3390/membranes13020195
Chicago/Turabian StyleElmaghraoui, Donia, Imen Ben Amara, and Sihem Jaziri. 2023. "Nickel Chalcogenide Nanoparticles-Assisted Photothermal Solar Driven Membrane Distillation (PSDMD)" Membranes 13, no. 2: 195. https://doi.org/10.3390/membranes13020195
APA StyleElmaghraoui, D., Ben Amara, I., & Jaziri, S. (2023). Nickel Chalcogenide Nanoparticles-Assisted Photothermal Solar Driven Membrane Distillation (PSDMD). Membranes, 13(2), 195. https://doi.org/10.3390/membranes13020195






