The Steady State Characteristics of Multicomponent Diffusion in Micro- and Mesoporous Media for Adsorbable and Nonadsorbable Species
Abstract
:1. Introduction
2. Materials and Methods
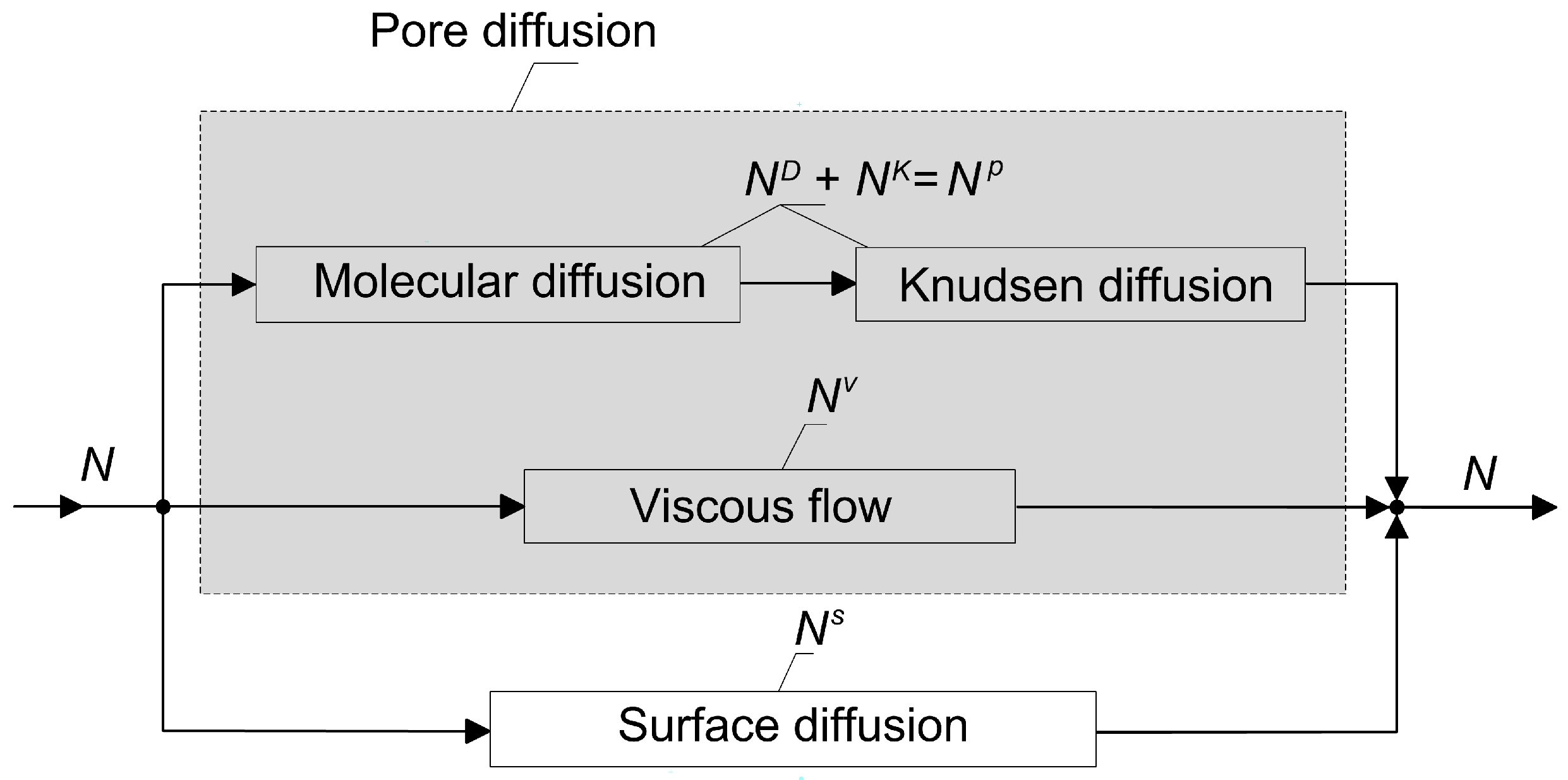
2.1. Description of the Dynamics of Complex Multicomponent Diffusion
2.2. An Alternative Model of Surface Diffusion
2.3. Method for Determining Steady States
- (a)
- adopt tentative values of Np and Ns;
- (b)
- assume the boundary conditions (35), (37) for ξ = 0;
- (c)
- integrate systems of ordinary differential Equations (33) and (34) from ξ = 0 to ξ = 1;
- (d)
- verify the fulfilment of the boundary conditions (36), (38) for ξ = 1;
- (e)
- if the boundary conditions are not met, improve the values of Np and Ns e.g., with the help of Newton’s overriding algorithm and return to point (b);
- (f)
- if the boundary conditions are met, it means that the profiles of all 2 × K variables, i.e., p(ξ) and q(ξ) = q [p(ξ)] and the molar flux densities corresponding to pore diffusion Np and surface diffusion Ns describe the determined steady state. Thence it is possible to calculate Ntot = Np + Ns.
3. Results and Discussion
- (a)
- To propose a mathematical model for the determination of steady states of complex multicomponent diffusion;
- (b)
- To verify the method.
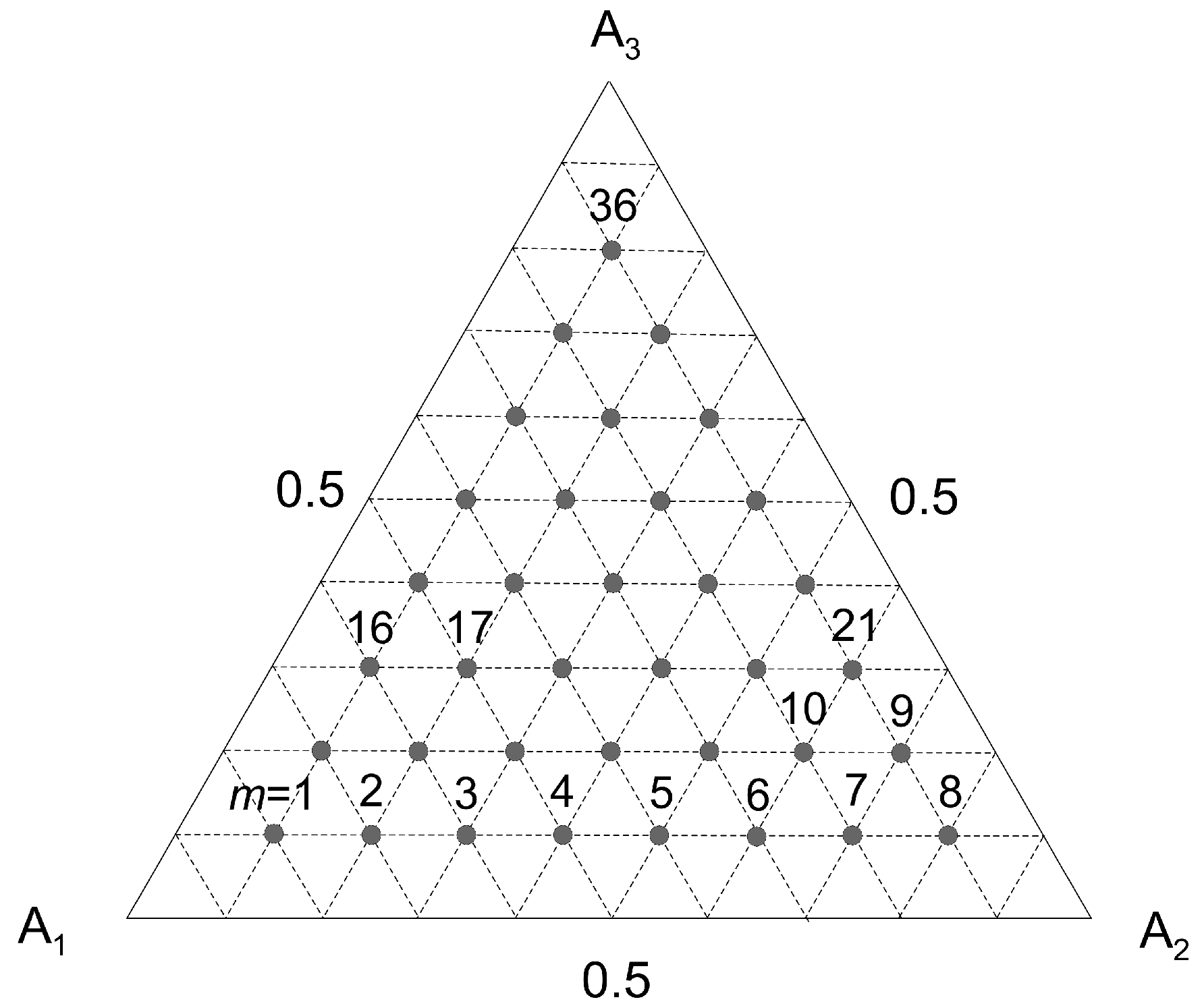
3.1. Selection of Diffusion Systems and Process Parameters
- (a)
- diffusion of the ternary solution {A1, A2, A3} = {CH4, CO2, N2} through microporous activated carbon (set “I”);
- (b)
- diffusion of the ternary solution {A1, A2, A3} = {CO2, C3H8, N2} through microporous glass Vycor (set “II”).
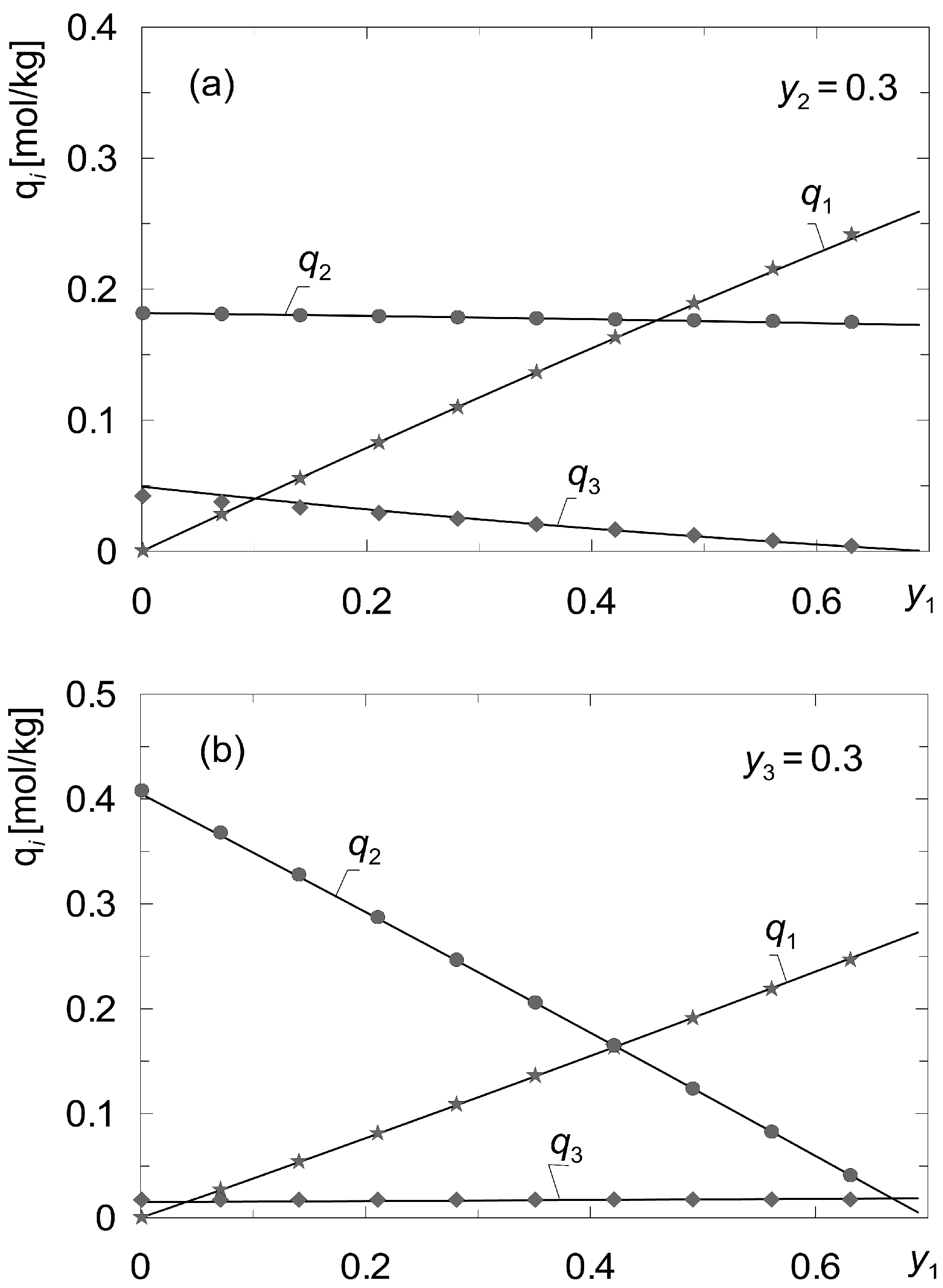
3.2. Thermodynamic Equilibrium in Selected Diffusion Systems
- (1)
- Assume the total pressure p, the gas phase composition yi and calculate the partial pressures pi = pyi.
- (2)
- Calculate the approximate value of the reduced spreading pressure η using the formula.
- (3)
- Estimate the approximate values of the hypothetical pressures as
- (4)
- Solve a system of K + 1 algebraic equations with respect to η and
- (5)
- Calculate the solid phase concentrations qi using the relationship
- (a)
- for data set no. “I”: b1 = 2.719 × 10−6; b2 = 3.106 × 10−6; b3 = 1.952 × 10−6 Pa−1;
- (b)
- for data set no. “II”: b1 = 2.206 × 10−6; b2 = 6.394 × 10−6 Pa−1.
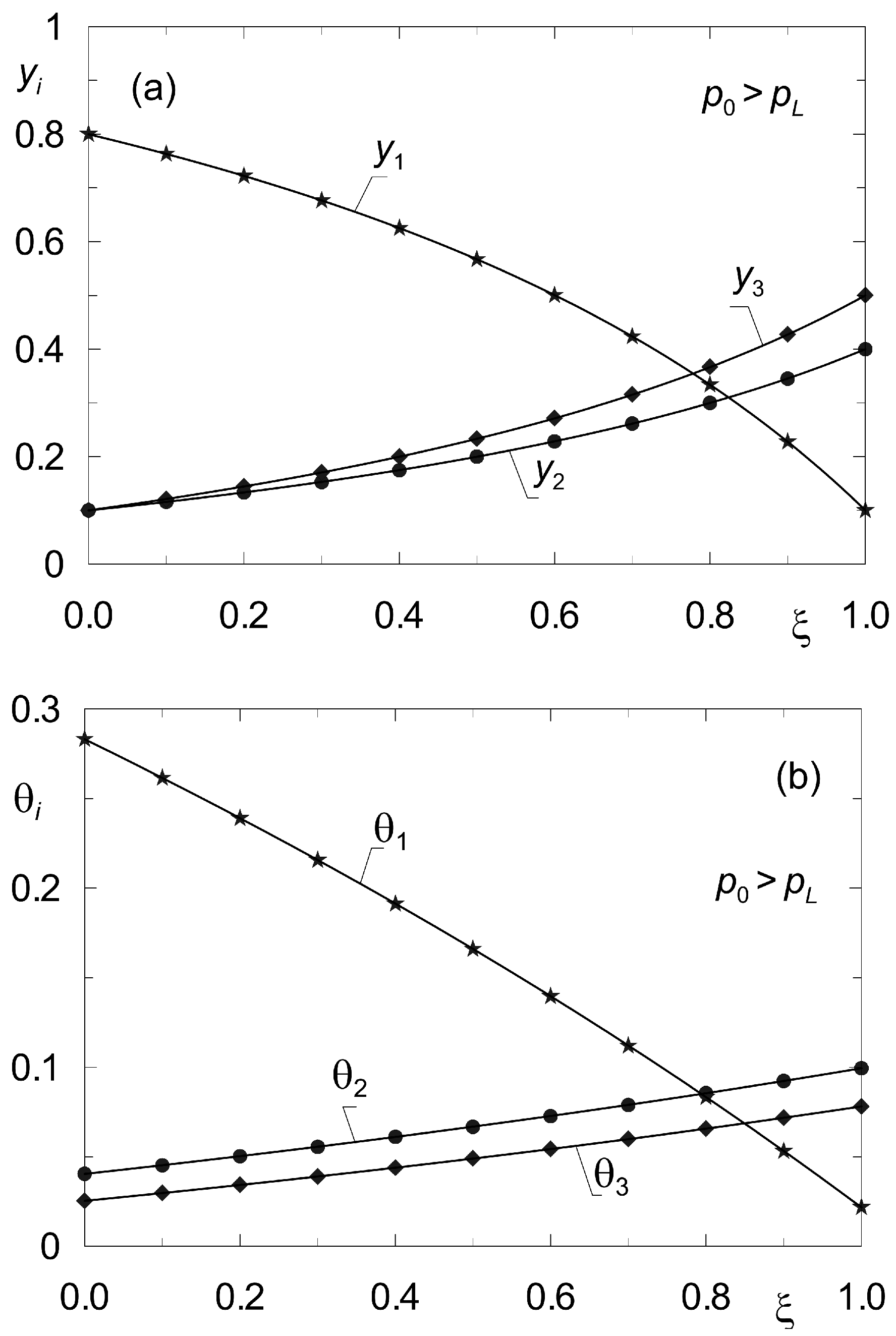
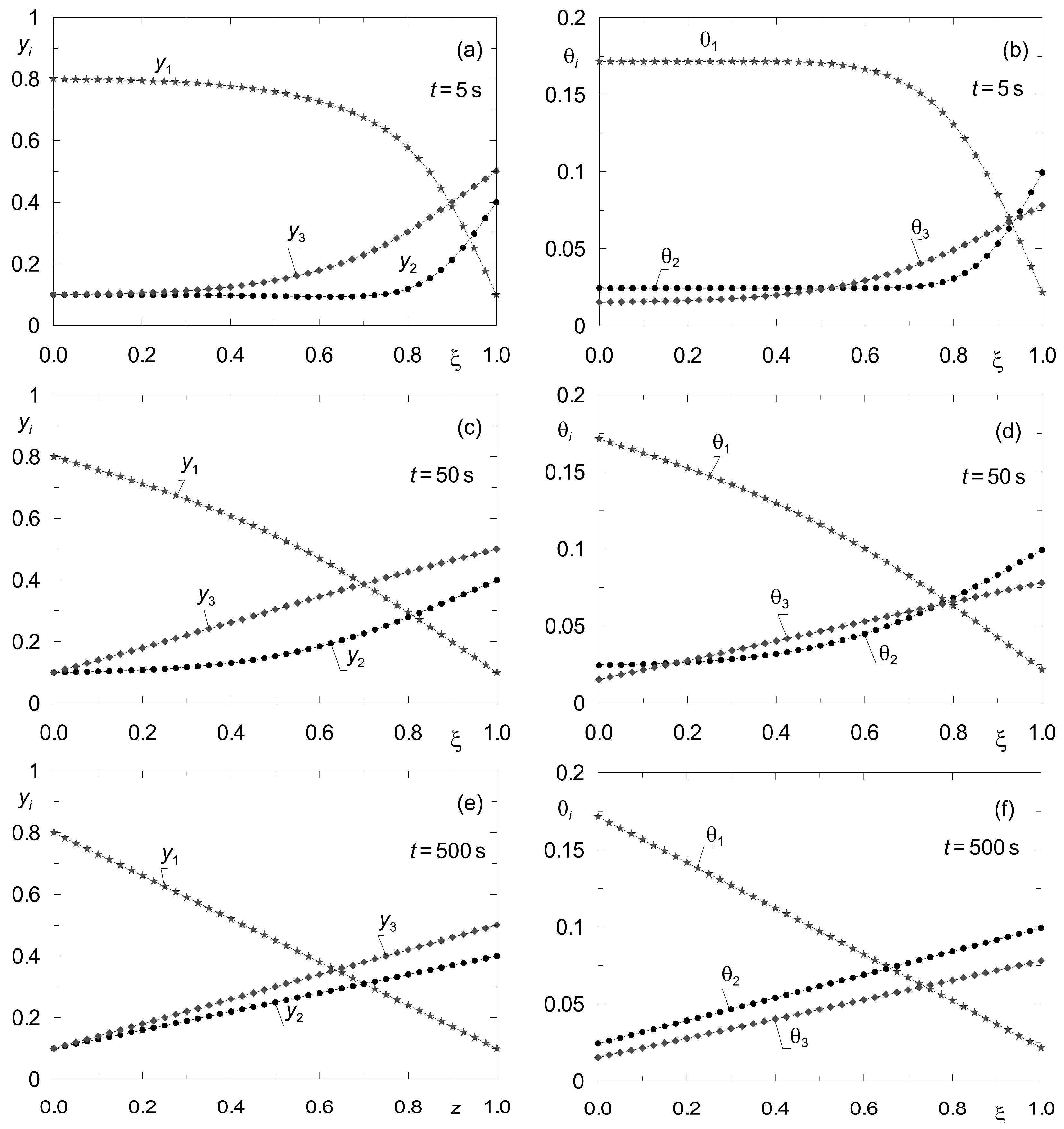
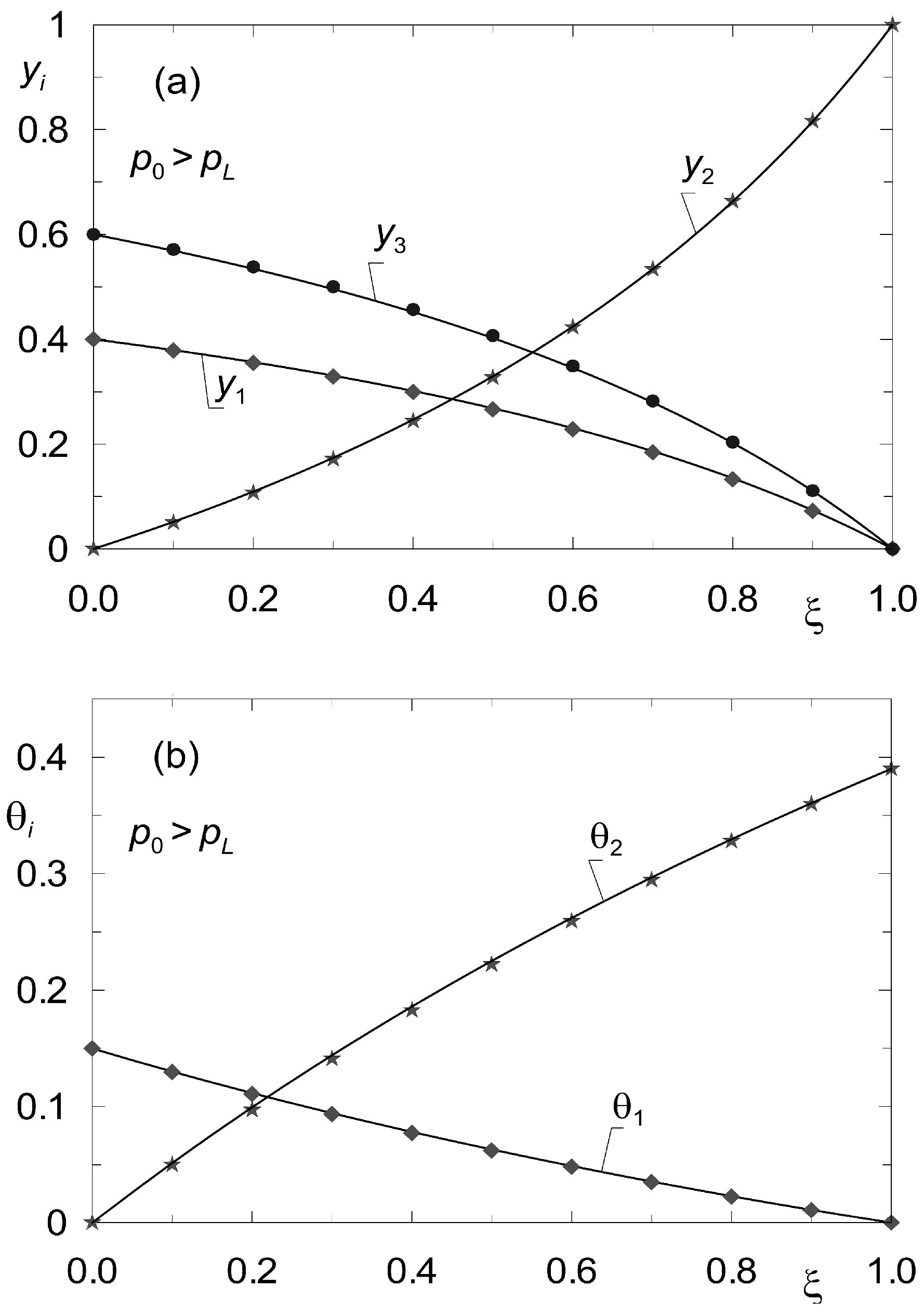
3.3. Verification of the Steady State Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| a | specific area of porous solid (m2⋅kg−1) |
| Ai | i-th component |
| bi | constant in Langmuir multicomponent isotherm (Pa−1) |
| constant in Langmuir isotherm for pure species (Pa−1) | |
| e | effective permeability parameter (m2) |
| dp | pore diameter, (m) |
| Dij | binary Maxwell–Stefan diffusion coefficient in gas phase (m2⋅s−1) |
| Maxwell–Stefan surface diffusivity of unary i-th species (m2⋅s−1) | |
| Maxwell–Stefan counter-sorption diffusivity (m2⋅s−1) | |
| DKn,i | Knudsen diffusion coefficient of i-th species (m2⋅s−1) |
| fi | fugacity coefficient |
| He | Henry constant for adsorption (mol⋅kg−1⋅Pa−1) |
| K | number of species |
| L | thickness of porous membrane (m) |
| m | shape coefficient |
| Mi | molar mass of species i (kg⋅mol−1) |
| Np | vector of pore molar fluxes (mol⋅m−2⋅s−1) |
| Ns | vector of surface molar fluxes (mol⋅m−2⋅s−1) |
| Ntot | vector of total diffusive molar fluxes (mol⋅m−2⋅s−1) |
| p | total pressure (Pa) |
| pi | partial pressure of i-th component (Pa) |
| qi | adsorbed phase concentration of i-th component (mol⋅kg−1) |
| q* | vector of saturation capacities (mol⋅kg−1) |
| R | universal gas constant (J⋅mol−1⋅K−1) |
| t | time (s) |
| T | temperature (K) |
| xi | molar fraction of i-th component in adsorbed phase |
| yi | molar fraction of i-th component in gas phase |
| z | spatial coordinate (m) |
| Greek letters | |
| α | degree of confinement |
| γι | activity coefficient of i-th species |
| Γ | matrix of thermodynamic correction factors |
| ε | porosity |
| η | viscosity (Pa⋅s) |
| η | reduced spreading pressure (mol⋅kg−1) |
| θι | fractional surface occupancy of component i |
| λ | vector of model parameters |
| µ | chemical potential (J⋅mol−1) |
| ξ | dimensionless spatial coordinate |
| π | spreading pressure (J⋅m−2) |
| ρσ | skeletal membrane density (kg⋅m−3) |
| τ | tortuosity |
| Superscripts | |
| * | equilibrium value |
| ° | standard state or pure species |
| e | effective value |
| g | gas phase |
| p | pore diffusion |
| s | surface diffusion |
| Subscripts | |
| i | i-th species in mixture |
| m | m-th point onto selected lattice |
| n | nonadsorbable species |
| s | solid phase |
| tot | total molar flux |
| 0, L | refer to z = 0 or z = L, respectively |
References
- Krishna, R.; Wesselingh, J.A. The Maxwell-Stefan approach to mass transfer. Chem. Eng. Sci. 1997, 52, 861–911. [Google Scholar] [CrossRef]
- Solsvik, J.; Jakobsen, H.A. Modeling of multicomponent mass diffusion in porous spherical pellets: Application to steam methane reforming and methanol synthesis. Chem. Eng. Sci. 2011, 66, 1986–2000. [Google Scholar] [CrossRef]
- Guo, W.Y.; Wuo, W.Z.; Luo, M.; Xiao, W.D. Modelling of diffusion and reaction in monolithic catalysts for the methanol-to-propylene process. Fuel Process. Technol. 2013, 108, 133–138. [Google Scholar] [CrossRef]
- Lei, K.L.; Ma, H.; Zhang, H.; Ying, W.; Fang, D. One-dimensional isothermal multicomponent diffusion-reaction model and its application to methanol synthesis over commercial Cu-based catalyst. Pol. J. Chem. Technol. 2015, 17, 103–109. [Google Scholar] [CrossRef]
- Wu, K.L.; Li, X.F.; Wang, C.; Yu, W.; Chen, Z. Model for surface diffusion of adsorbed gas in nanopores of shale gas reservoirs. Ind. Eng. Chem. Res. 2015, 54, 3225–3236. [Google Scholar] [CrossRef]
- Bertei, A.; Nicolella, C. Common inconsistencies in modeling gas transport in porous electrodes: The dusty-gas model and the Fick law. J. Power Sources 2015, 279, 133–137. [Google Scholar] [CrossRef]
- Yang, B.; Kang, Y.; You, L.; Li, X.; Chen, Q. Measurement of the surface diffusion coefficient for adsorbed gas in the fine mesopores and micropores of shale organic matter. Fuel 2016, 181, 793–804. [Google Scholar] [CrossRef]
- Wernert, V.; Nguyen, K.L.; Levitz, P.; Coasne, B.; Denoyel, R. Impact of surface diffusion on transport through porous materials. J. Chromatogr. A 2022, 1665, 462823. [Google Scholar] [CrossRef]
- Welti-Chanes, J.; Vergara-Balderas, F.; Bermudez-Aguirre, D. Transport phenomena in food engineering: Basic concepts and advances. J. Food Eng. 2005, 67, 113–128. [Google Scholar] [CrossRef]
- Geankoplis, C.J. Transport Processes and Separation Process Principles, 4th ed.; Prentice-Hall: Boston, MA, USA, 2003. [Google Scholar]
- Lin, H.; He, Z.; Sun, Z.; Vu, J.; Ng, A.; Mohammed, M.; Kniep, J.; Merkel, T.C.; Wu, T.; Lambrecht, R.C. CO2-selective membrane for hydrogen production and CO2 capture—Part I: Membrane development. J. Membr. Sci. 2014, 457, 149–161. [Google Scholar] [CrossRef]
- Westerterp, K.R. Multifunctional reactors. Chem. Eng. Sci. 1992, 47, 2195–2206. [Google Scholar] [CrossRef]
- Dautzenberg, F.M.; Mukherjee, M. Process intensification using multifunctional reactors. Chem. Eng. Sci. 2001, 56, 251–267. [Google Scholar] [CrossRef]
- Doraiswamy, L.K.; Üner, D. Chemical Reaction Engineering: Beyond the Fundamentals, 1st ed.; CRC Press: New York, NY, USA, 2014. [Google Scholar]
- Liu, Z.; Emami-Meybodi, H. Apparent diffusion coefficient for adsorption-controlled gas transport in nanoporous media. Chem. Eng. J. 2022, 450, 138105. [Google Scholar] [CrossRef]
- Mason, E.A.; Malinauskas, A.P. Gas Transport in Porous Media: The Dusty Gas Model, 1st ed.; Elsevier: Amsterdam, The Netherlands; New York, NY, USA, 1983. [Google Scholar]
- Do, D.D. Adsorption Analysis: Equilibria and Kinetics, 1st ed.; Imperial College Press: London, UK, 1998. [Google Scholar]
- Vaartstra, G.; Lu, Z.; Grossman, J.C.; Wang, E.N. Numerical validation of the dusty-gas model for binary diffusion in low aspect ratio capillaries. Phys. Fluids 2021, 33, 121701. [Google Scholar] [CrossRef]
- Krishna, R. Multicomponent surface diffusion of adsorbed species: A description based on the generalized Maxwell-Stefan equations. Chem. Eng. Sci. 1990, 45, 1779–1791. [Google Scholar] [CrossRef]
- Krishna, R. Problems and pitfalls in the use of Fick formulation for intraparticle diffusion. Chem. Eng. Sci. 1993, 48, 845–861. [Google Scholar] [CrossRef]
- Krishna, R. A unified approach to the modeling of intraparticle diffusion in adsorption processes. Gas Sep. Purif. 1993, 7, 91–104. [Google Scholar] [CrossRef]
- Van den Broeke, L.J.P.; Krishna, R. Experimental verification of the Maxwell-Stefan theory for micropore diffusion. Chem. Eng. Sci. 1995, 50, 2507–2522. [Google Scholar] [CrossRef]
- Tawalbeh, M.; Al-Ismaily, M.; Kruczek, B.; Tezel, F.H. Modeling the transport of CO2, N2, and their binary mixtures through highly permeable silicalite-1 membranes using Maxwell−Stefan equations. Chemosphere 2021, 263, 127935. [Google Scholar] [CrossRef]
- Do, H.D.; Do, D.D. Maxwell-Stefan analysis of multicomponent transient diffusion in a capillary and adsorption of hydrocarbons in activated carbon particle. Chem. Eng. Sci. 1998, 53, 1239–1252. [Google Scholar] [CrossRef]
- Tuchlenski, A.; Uchytil, P.; Seidel-Morgenstein, A. An experimental study of combined gas phase and surface diffusion in porous glass. J. Membr. Sci. 1998, 140, 165–184. [Google Scholar] [CrossRef]
- Yang, J.; Čermáková, J.; Uchytil, P.; Hamel, C.; Seidel-Morgenstern, A. Gas phase transport, adsorption and surface diffusion in a porous glass membrane. Catal. Today 2005, 104, 344–351. [Google Scholar] [CrossRef]
- Jackson, R. Transport in Porous Catalysts, 1st ed.; Elsevier Scientific Publishing Company: Amsterdam, The Netherlands; Oxford, UK; New York, NY, USA, 1977. [Google Scholar]
- Krishna, R. Investigating the validity of the Knudsen diffusivity prescription for mesoporous and macroporous materials. Ind. Eng. Chem. Res. 2016, 55, 4749–4759. [Google Scholar] [CrossRef]
- Fuller, E.N.; Schettler, P.D.; Giddings, J.C. A new method for prediction of binary gas phase diffusion coefficients. Ind. Eng. Chem. 1966, 58, 19–27. [Google Scholar] [CrossRef]
- Poling, B.E.; Prausnitz, J.M.; O’Connel, J.P. The Properties of Gases and Liquids, 5th ed.; McGraw-Hill: New York, NY, USA; Toronto, ON, Canada, 2001. [Google Scholar]
- Krishna, R.; van Baten, J.M. An investigation of the characteristics of Maxwell-Stefan diffusivities of binary mixtures in silica nanopores. Chem. Eng. Sci. 2009, 64, 870–882. [Google Scholar] [CrossRef]
- Krishna, R.; van Baten, J.M. Unified Maxwell-Stefan description of binary mixture diffusion in micro- and meso-porous materials. Chem. Eng. Sci. 2009, 64, 3159–3178. [Google Scholar] [CrossRef]
- Ruthven, D.M. Principles of Adsorption and Adsorption Processes, 1st ed.; John Wiley & Sons: New York, NY, USA, 1984. [Google Scholar]
- Ruthven, D.M.; DeSisto, W.; Higgins, S. Diffusion in a mesoporous silica membrane: Validity of the Knudsen diffusion model. Chem. Eng. Sci. 2009, 64, 3201–3203. [Google Scholar] [CrossRef]
- Bhatia, S.K.; Nicholson, D. Comments on “Diffusion in a mesoporous silica membrane: Validity of the Knudsen diffusion model”, by Ruthven, D.M., et al. Chem. Eng. Sci. 2009, 64, 3201–3203, Erratum in Chem. Eng. Sci. 2010, 65, 4519–4520. [Google Scholar] [CrossRef]
- Ruthven, D.M. Letter to the editor. Response to comments from S.K. Bhatia and D. Nicholson. Chem. Eng. Sci. 2010, 65, 4521–4522. [Google Scholar] [CrossRef]
- Bhatia, S.K.; Nicholson, D. Some pitfalls in the use of the Knudsen equation in modelling diffusion in nanoporous materials. Chem. Eng. Sci. 2011, 66, 284–293. [Google Scholar] [CrossRef]
- Krishna, R.; van Baten, J.M. Influence of adsorption on the diffusion selectivity for mixture permeation across mesoporous membranes. J. Membr. Sci. 2011, 369, 545–549. [Google Scholar] [CrossRef]
- Krishna, R.; van Baten, J.M. Investigating the validity of the Knudsen prescription for diffusivities in a mesoporous covalent organic framework. Ind. Eng. Chem. Res. 2011, 50, 7083–7087. [Google Scholar] [CrossRef]
- Bizon, K.; Tabiś, B. Phenomenological and numerical issues concerning dynamics of nonisobaric multicomponent diffusion of gases in microporous media. Chem. Proc. Eng. 2021, 42, 223–234. [Google Scholar] [CrossRef]
- Seydel, R. Practical Bifurcation and Stability Analysis: From Equilibrium to Chaos, 2nd ed.; Springer: New York, NY, USA; Budapest, Hungary, 1994. [Google Scholar]
- Frey, D.D.; Rodrigues, A.E. Explicit calculation of multicomponent equilibria for ideal adsorbed solutions. AIChE J. 1994, 40, 182–186. [Google Scholar] [CrossRef]
- Landa, H.O.R.; Flockerzi, D.; Seidel-Morgenstern, A. A method for efficiently solving the IAST equations with an application to adsorber dynamics. AIChE J. 2013, 59, 1263–1277. [Google Scholar] [CrossRef]
- Hamdi, S.; Schiesser, W.E.; Griffiths, G.W. Method of lines. Scholarpedia 2010, 2, 2859. [Google Scholar] [CrossRef]


| Porous Material | ρs (kg·m−3) | dp (m) | ε |
|---|---|---|---|
| Microporous activated carbon [22] (Set “I”) | 2100 | 5.2 × 10−10 | 0.64 |
| Vycor microporous glass [25] (Set “II”) | 2057 | 4.7 × 10−9 | 0.284 |
| Parameter | Set “I” [22] | Set “II” [25] | Value |
|---|---|---|---|
| T | 345 | 343 | K |
| y01 | 0.80 | 0.40 | − |
| y02 | 0.10 | 0.00 | − |
| yL1 | 0.10 | 0.00 | − |
| yL2 | 0.40 | 0.10 | − |
| 1.1647× 10−5 | 1.193 × 10−5 | m2⋅s−1 | |
| 1.4095 × 10−5 | 2.109 × 10−5 | m2⋅s−1 | |
| 1.0650 ×10−5 | 1.495 × 10−5 | m2⋅s−1 | |
| 1.375 × 10−10 | 2.80 × 10−9 | m2⋅s−1 | |
| 5.819 × 10−11 | 4.00 × 10−9 | m2⋅s−1 | |
| 3.174 × 10−10 | − | m2⋅s−1 | |
| 1.248 × 10−5 | 1.696 × 10−5 | Pa⋅s | |
| 1.809 × 10−5 | 9.464 × 10−6 | Pa⋅s | |
| 1.968 × 10−5 | 1.936 × 10−5 | Pa⋅s | |
| L | 10−3 | 10−3 | m |
| Quantity | A1 = CH4 | A2 = CO2 | A3 = N2 |
|---|---|---|---|
| 1.8 | 2.4 | 0.38 | |
| 2.7 × 10−6 | 3.0 × 10−6 | 3.0 × 10−6 |
| Quantity | A1 = CO2 | A2 = C3H8 | A3 = N2 |
|---|---|---|---|
| 1.531 | 0.3395 | 0 | |
| 1.91 × 10−6 | 7.14 × 10−6 | 0 |
| A1 = CH4 | A2 = CO2 | A3 = N2 | |
|---|---|---|---|
| (mol⋅m−2⋅s−1) | 7.103 × 10−4 | −1.838 × 10−4 | −3.072 × 10−4 |
| (mol⋅m−2⋅s−1) | 1.788 × 10−4 | −5.252 × 10−5 | −3.114 × 10−5 |
| Ntot,i (mol⋅m−2⋅s−1) | 8.891 × 10−4 | −2.363 × 10−4 | −3.383 × 10−4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bizon, K.; Boroń, D.; Tabiś, B. The Steady State Characteristics of Multicomponent Diffusion in Micro- and Mesoporous Media for Adsorbable and Nonadsorbable Species. Membranes 2022, 12, 921. https://doi.org/10.3390/membranes12100921
Bizon K, Boroń D, Tabiś B. The Steady State Characteristics of Multicomponent Diffusion in Micro- and Mesoporous Media for Adsorbable and Nonadsorbable Species. Membranes. 2022; 12(10):921. https://doi.org/10.3390/membranes12100921
Chicago/Turabian StyleBizon, Katarzyna, Dominika Boroń, and Bolesław Tabiś. 2022. "The Steady State Characteristics of Multicomponent Diffusion in Micro- and Mesoporous Media for Adsorbable and Nonadsorbable Species" Membranes 12, no. 10: 921. https://doi.org/10.3390/membranes12100921
APA StyleBizon, K., Boroń, D., & Tabiś, B. (2022). The Steady State Characteristics of Multicomponent Diffusion in Micro- and Mesoporous Media for Adsorbable and Nonadsorbable Species. Membranes, 12(10), 921. https://doi.org/10.3390/membranes12100921








