New Four Points Initialization for Digital Image Correlation in Metal-Sheet Strain Measurements
Abstract
:1. Introduction
2. Theoretical Bases
2.1. Normalized Cross Correlation (NCC)
2.2. Superficial Strain Estimation
3. Materials and Methods
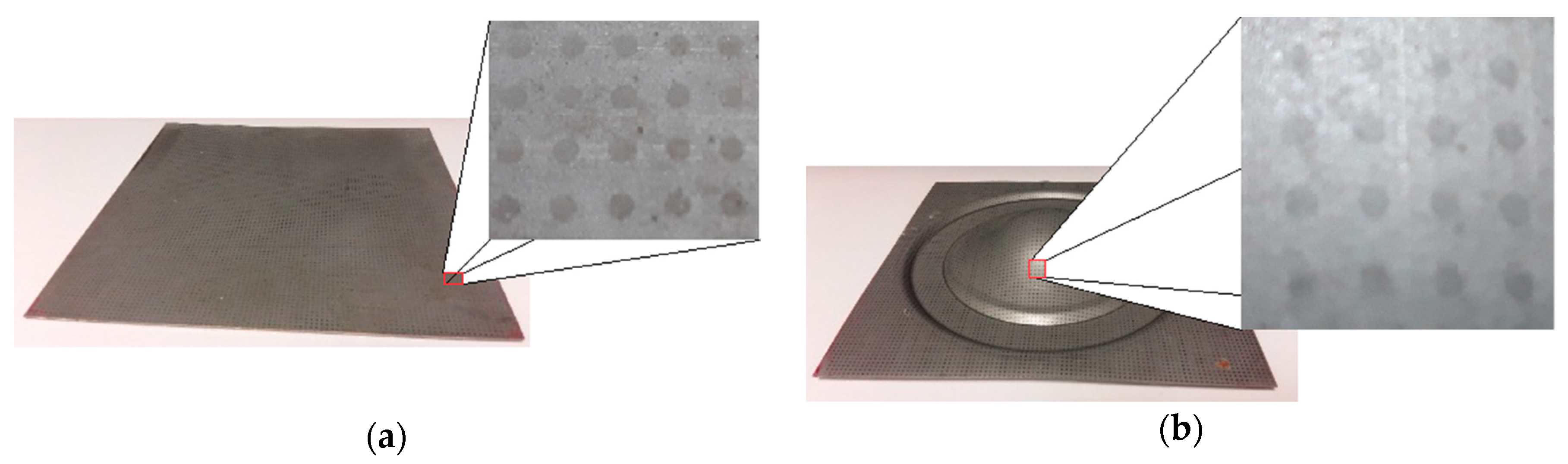
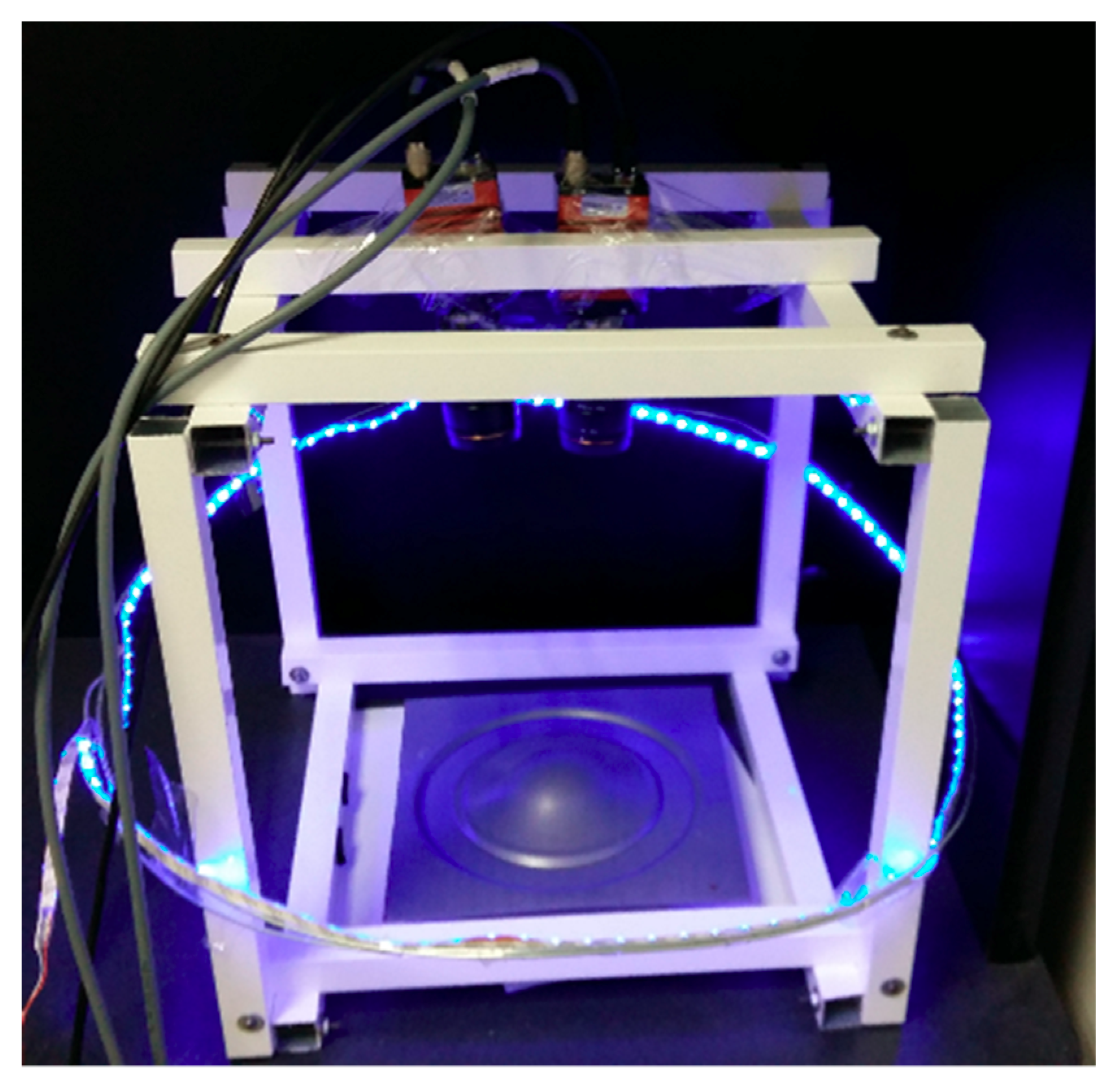
3.1. Materials
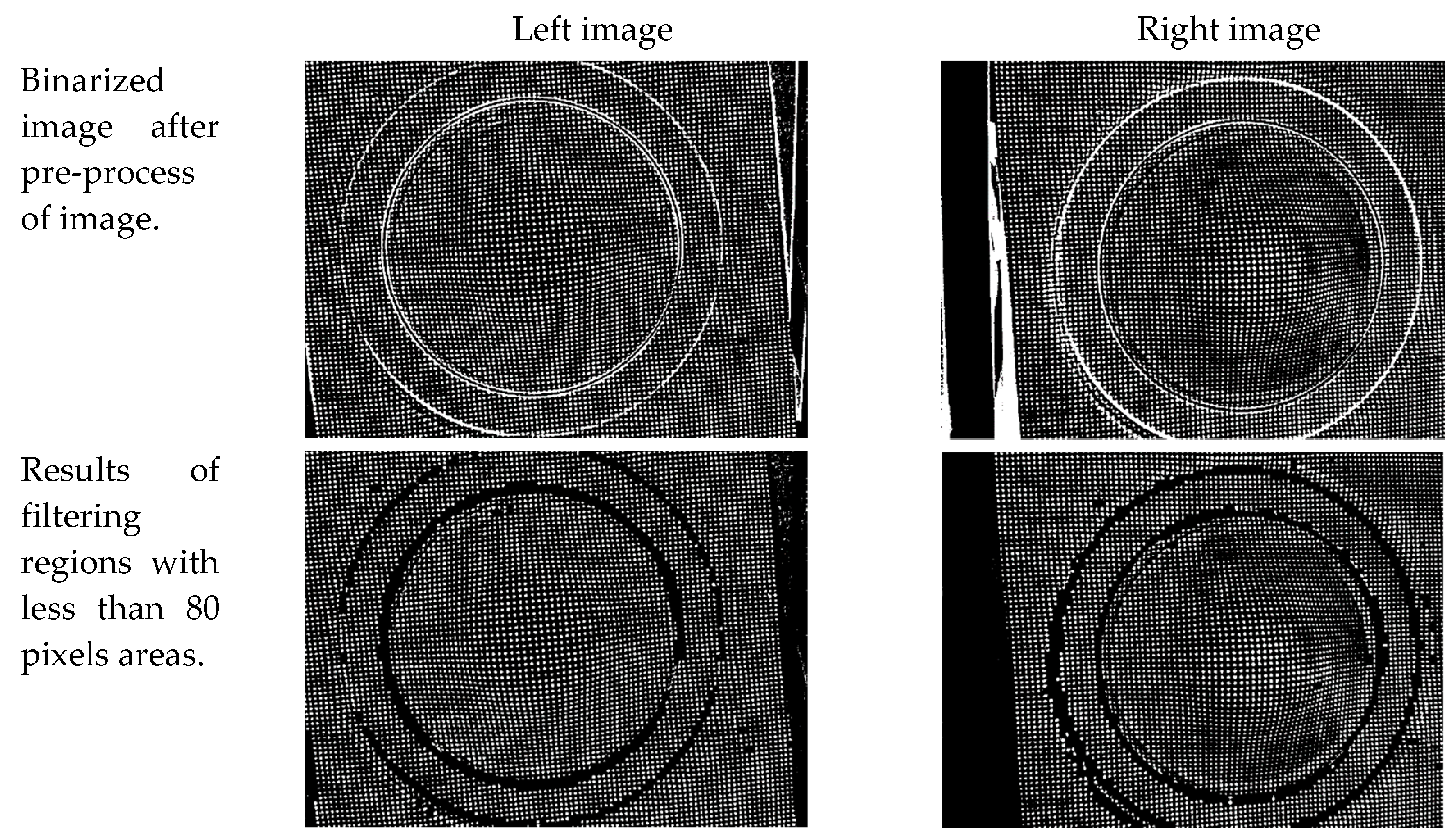
3.2. Method
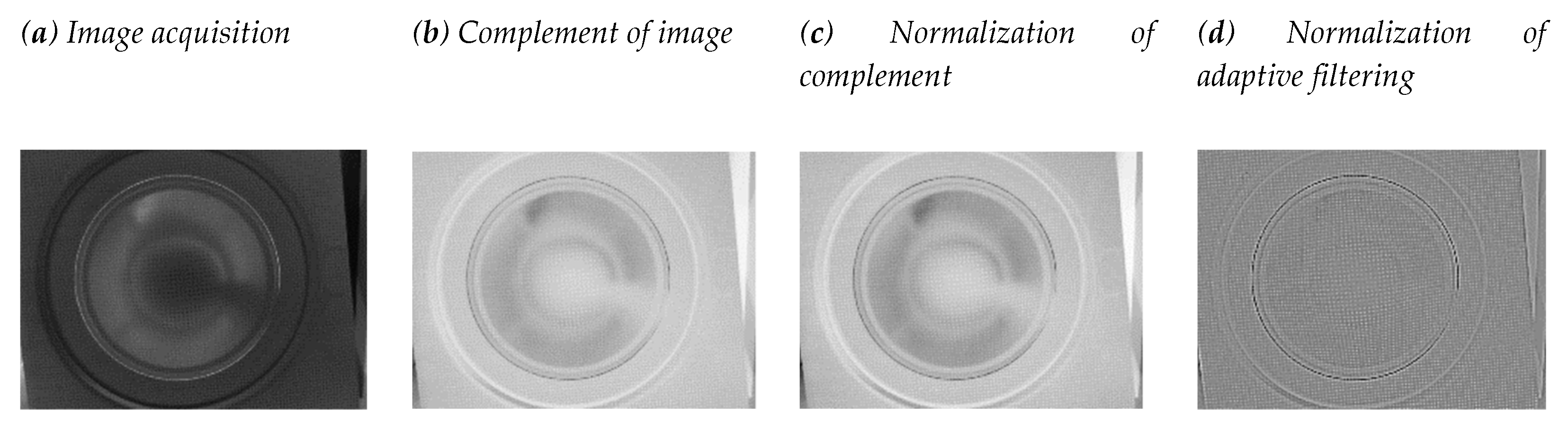
- (a)
- Stamping of known circle grid on the unformed metal sheet.
- (b)
- Deformation of the metal-sheet through the mechanical stamping process.
- (c)
- Calibration of cameras.
- (d)
- Illumination of the piece with LED blue light for measuring.
- (e)
- Capture of stereo images.
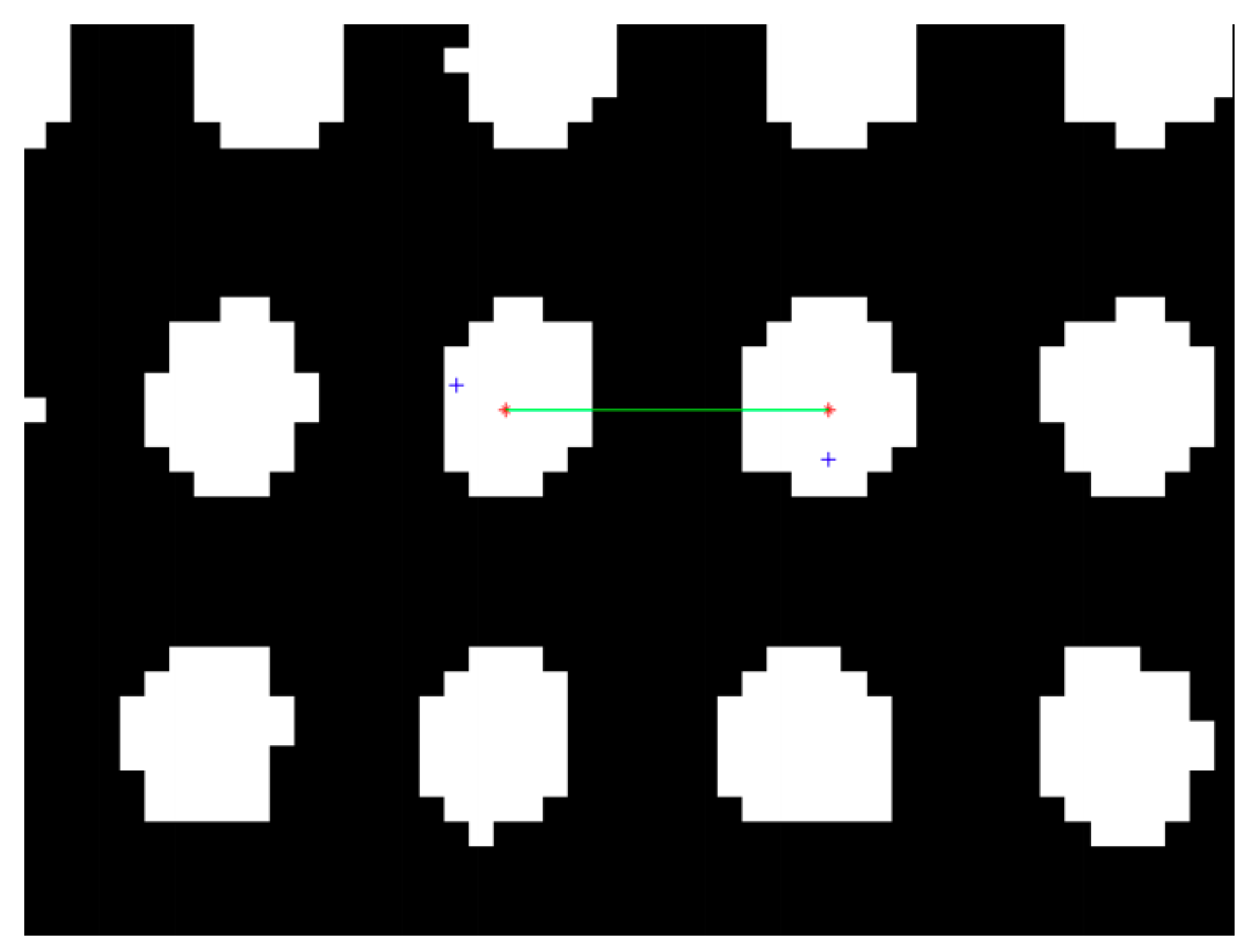
- (f)
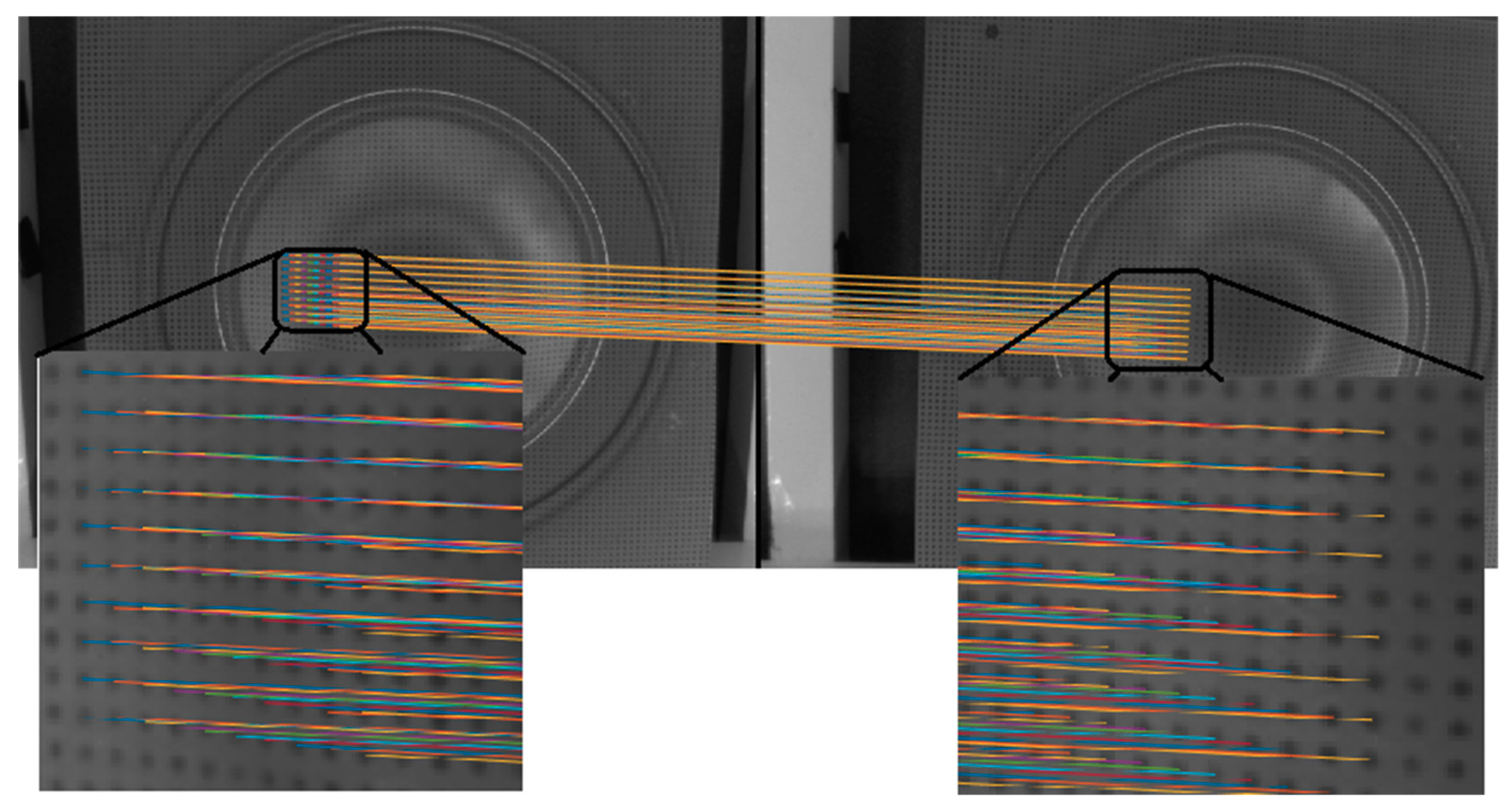
- Selection of four landmarks.
- (g)
- Search for neighbor’s centroid.
- (h)
- Calculation of the NCC in the proposed neighborhood.
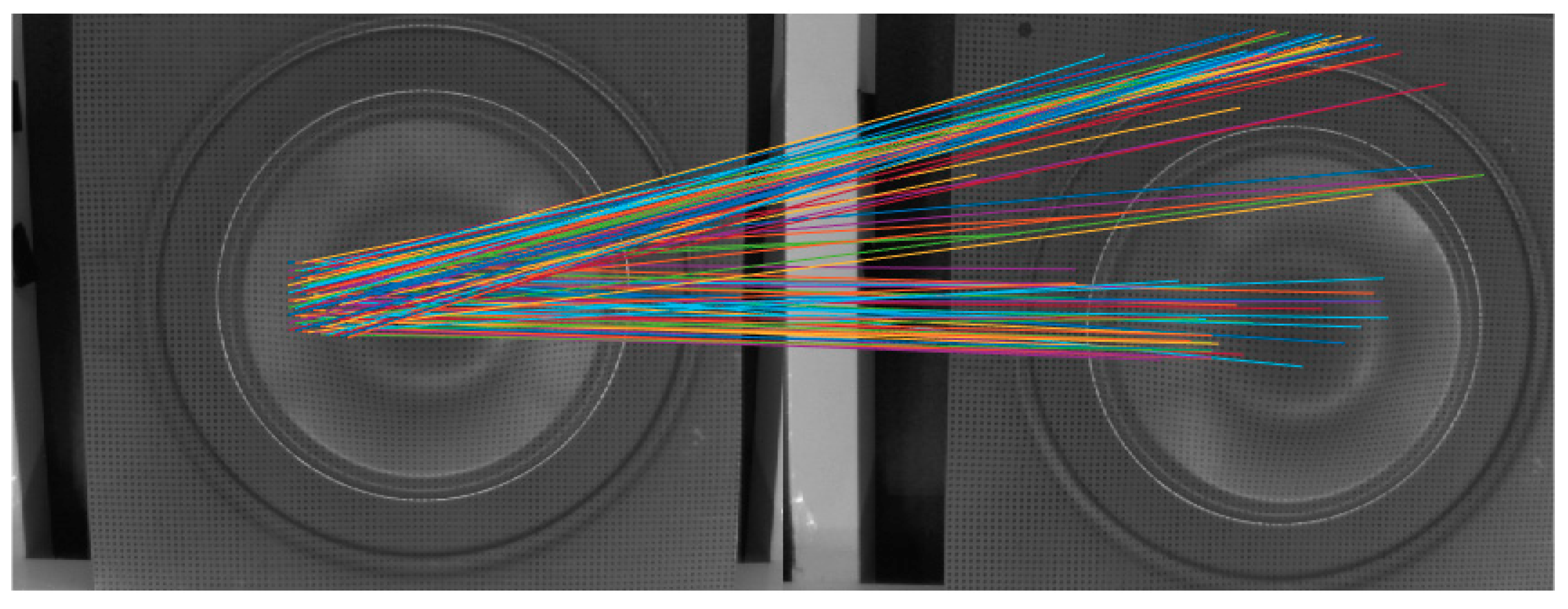
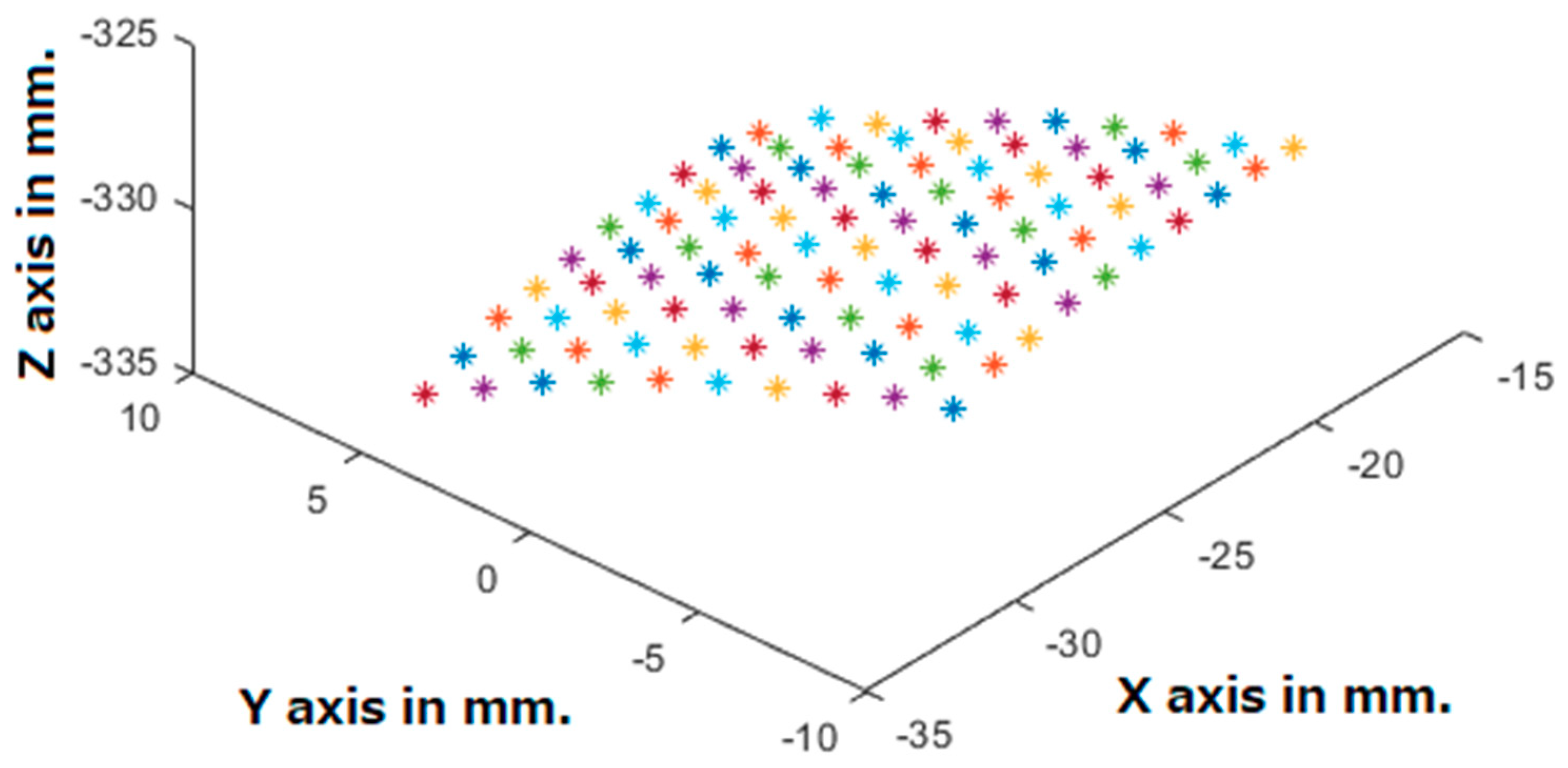
- (i)
- Triangulation of the points to obtain their position in 3D space.
- (j)
- Strain estimation from averaging the centroids’ differences with the four neighbors using Equation (6).
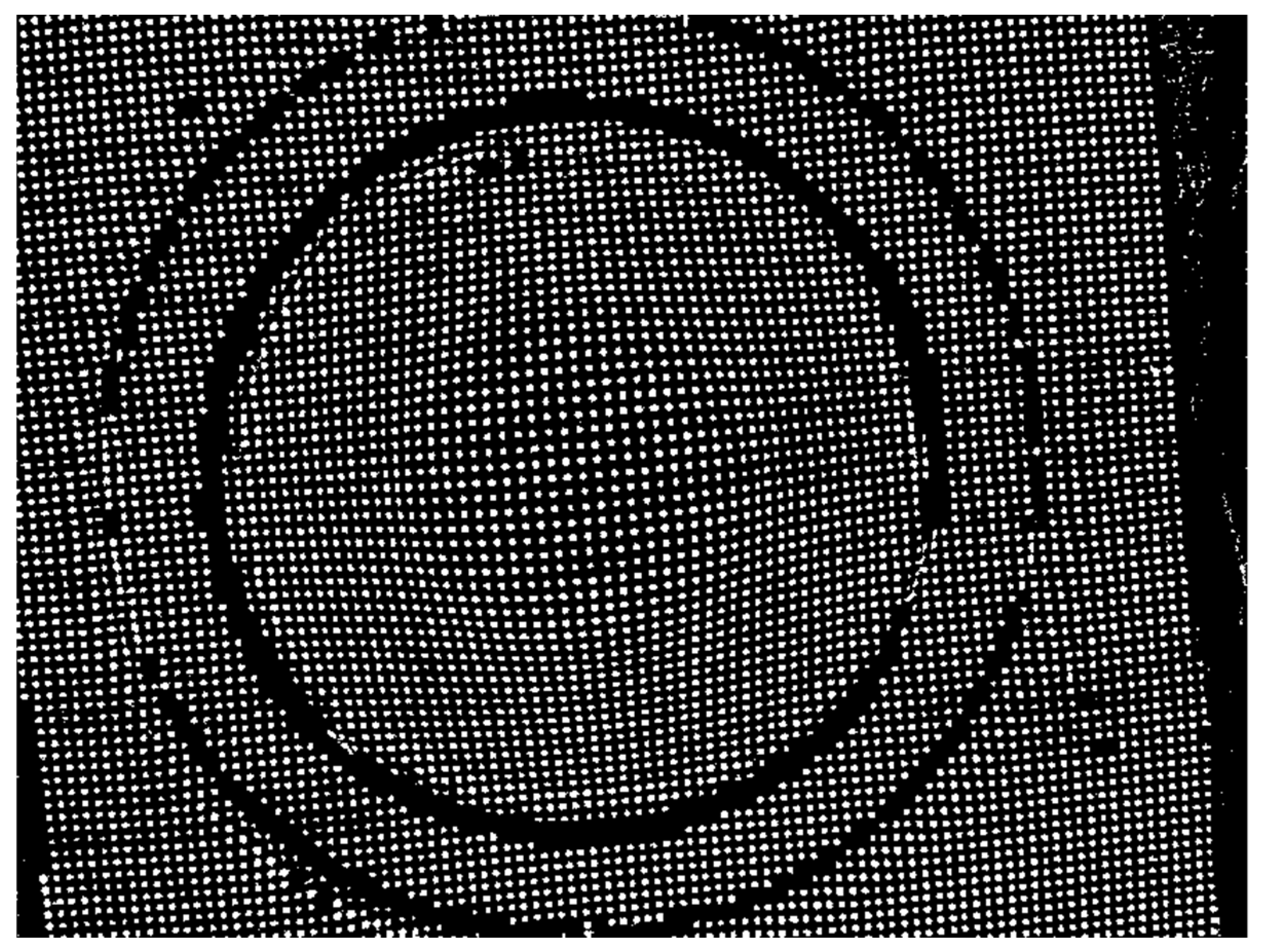
3.3. Four-Points Initialization
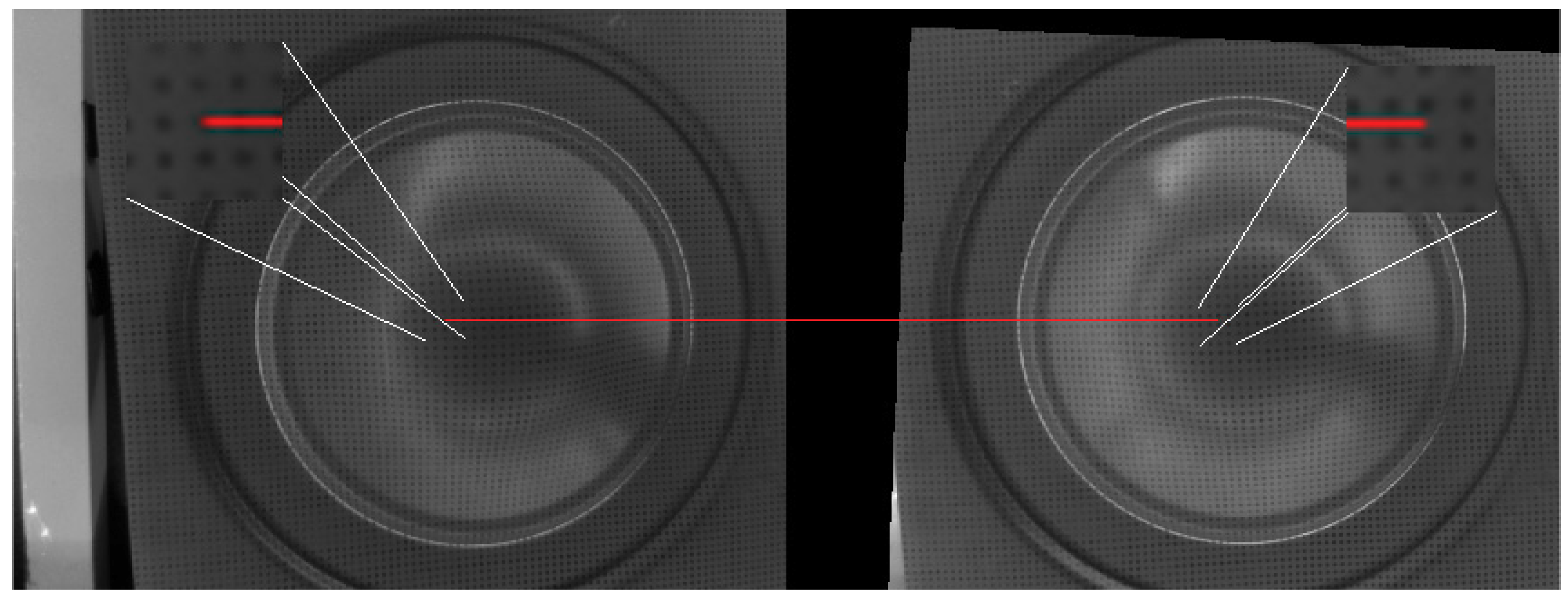
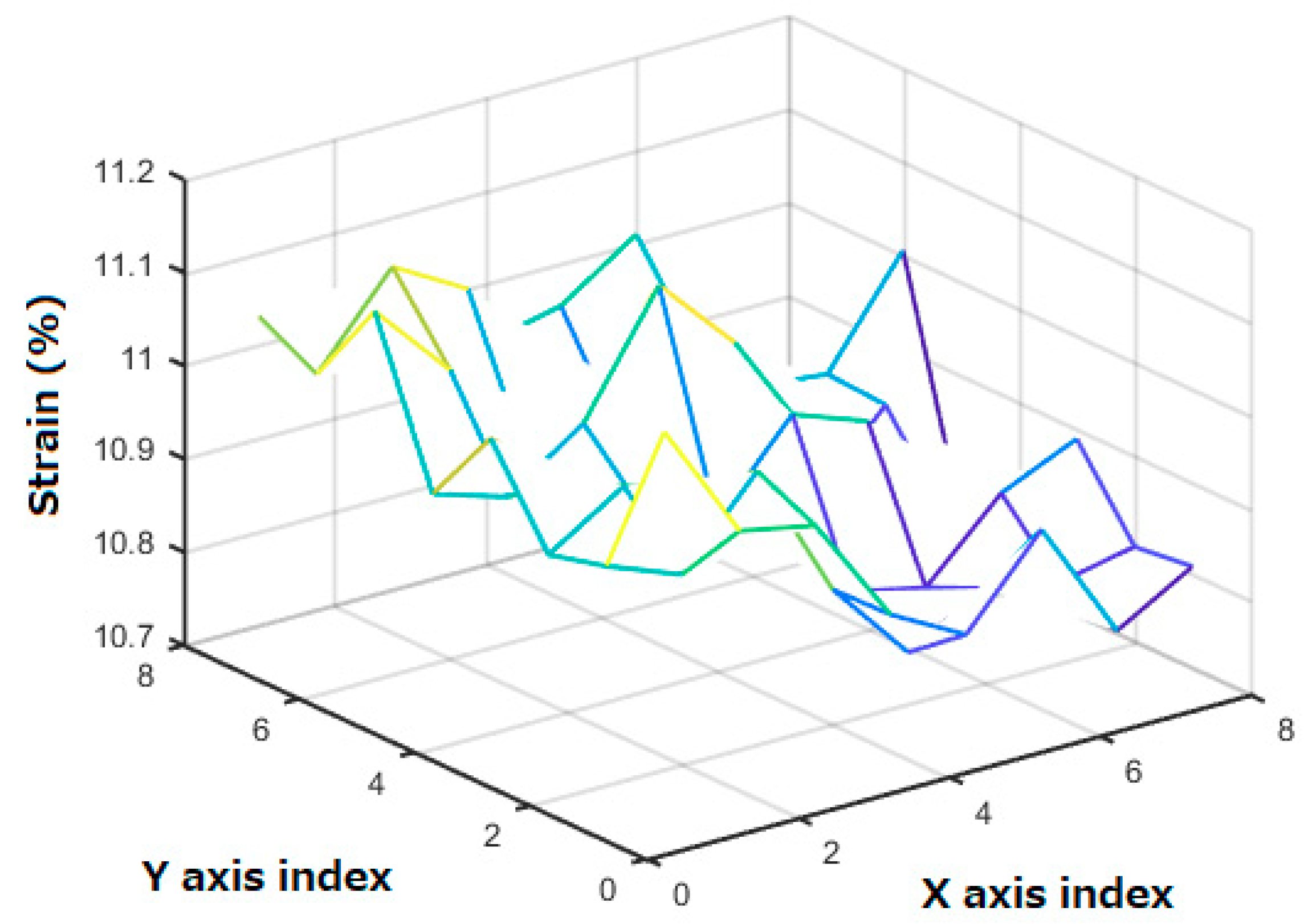
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A

References
- Choi, J.; Choi, B.; Heo, S.; Oh, Y.; Shin, S. Numerical modeling of the thermal deformation during stamping process of an automotive body part. Appl. Therm. Eng. 2018, 118, 159–172. [Google Scholar] [CrossRef]
- Lee, H.H.; Yoon, J.I.; Kim, H.S. Single-roll angular-rolling: A new continuous severe plastic deformation process for metal sheets. Scr. Mater. 2018, 146, 204–207. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Kwon, S.J.; Kwon, O.H.; Kim, Y.S. Mechanical Properties Identification of Sheet Metals by 2D-Digital Image Correlation Method. Procedia Eng. 2017, 184, 381–389. [Google Scholar] [CrossRef]
- Blaber, J.; Adair, B.; Antoniou, A. Ncorr: Open-Source 2D Digital Image Correlation Matlab Software. Exp. Mech. 2015, 55, 1105–1122. [Google Scholar] [CrossRef]
- Kazuki, K.; Takuma, M.; Shuichi, A. Measurement of Super-Pressure Balloon Deformation with Simplified Digital Image Correlation. Appl. Sci. 2018, 8, 9. [Google Scholar] [CrossRef]
- Solav, D.; Moerman, K.M.; Jaeger, A.M.; Genovese, K.; Herr, H.M. MultiDIC: An Open-Source Toolbox for Multi-View 3D Digital Image Correlation. IEEE Access 2018, 16, 30520–30535. [Google Scholar] [CrossRef]
- Xinxing, S.; Xiangjun, D.; Chen, Z.; He, X. Real-time 3D digital image correlation method and its application in human pulse monitoring. Appl. Opt. 2016, 55, 696–704. [Google Scholar] [CrossRef]
- Genovese, K.; Sorgente, D. A morphing-based scheme for large deformation analysis with stereo-DIC. Opt. Lasers Eng. 2018, 104, 159–172. [Google Scholar] [CrossRef]
- Reu, P.L.; Toussaint, E.; Jones, E.; Bruck, H.A.; Iadicola, M.; Balcaen, R.; Turner, D.Z.; Siebert, T.; Lava, P.; Simonsen, M. DIC Challenge: Developing Images and Guidelines for Evaluating Accuracy and Resolution of 2D Analyses. Exp. Mech. 2017, 58, 1067–1099. [Google Scholar] [CrossRef]
- Grédiac, M.; Blaysat, B.; Sur, F. A Robust-to-Noise Deconvolution Algorithm to Enhance Displacement and Strain Maps Obtained with Local DIC and LSA. Exp. Mech. 2018, 1, 1–25. [Google Scholar] [CrossRef]
- Bruck, H.A.; McNeill, S.R.; Sutton, M.A.; Peters, W.H. Digital image correlation using Newton–Raphson method of partial differential correction. Exp. Mech. 1989, 29, 261–267. [Google Scholar] [CrossRef]
- Cheng, P.; Sutton, M.A.; Schreier, H.W.; McNeill, S.R. Full-field speckle pattern image correlation with B-spline deformation function. Exp. Mech. 2002, 42, 344–352. [Google Scholar] [CrossRef]
- Kammers, A.D.; Daly, S. Small-scale patterning methods for digital image correlation under scanning electron microscopy. Meas. Sci. Technol. 2011, 22, 125501. [Google Scholar] [CrossRef]
- Rubino, V.; Lapusta, N.; Rosakis, A.; Leprince, S.; Avouac, J. Static laboratory earthquake measurements with the digital image correlation method. Exp. Mech. 2015, 55, 77–94. [Google Scholar] [CrossRef]
- Dickinson, A.S.; Taylor, A.C.; Ozturk, H.; Browne, M. Experimental validation of a finite element model of the proximal femur using digital image correlation and a composite bone model. J. Biomech. Eng. 2011, 133, 014504. [Google Scholar] [CrossRef]
- Zhang, D.; Eggleton, C.; Arola, D. Evaluating the mechanical behavior of arterial tissue using digital image correlation. Exp. Mech. 2002, 42, 409–416. [Google Scholar] [CrossRef] [Green Version]
- Franck, C.; Maskarinec, S.A.; Tirrell, D.A.; Ravichandran, G. Three-dimensional traction force microscopy: A new tool for quantifying cell-matrix interactions. PLoS ONE 2011, 6, e17833. [Google Scholar] [CrossRef]
- Wang, H.; Lai, W.; Antoniou, A.; Bastawros, A. Application of digital image correlation for multiscale biomechanics. In Handbook of Imaging in Biological Mechanics; CRC Press: Oxfords, UK, 2014; pp. 141–151. [Google Scholar]
- Carroll, J.D.; Abuzaid, W.; Lambros, J.; Sehitoglu, H. High resolution digital image correlation measurements of strain accumulation in fatigue crack growth. Int. J. Fatigue 2013, 57, 140–150. [Google Scholar] [CrossRef]
- Tong, W. Detection of plastic deformation patterns in a binary aluminum alloy. Exp. Mech. 1997, 37, 452–459. [Google Scholar] [CrossRef]
- Rehrl, C.; Kleber, S.; Antretter, T.; Pippan, R. A methodology to study crystal plasticity inside a compression test sample based on image correlation and EBSD. Mater. Charact. 2011, 62, 793–800. [Google Scholar] [CrossRef]
- Daly, S.; Ravichandran, G.; Bhattacharya, K. Stress-induced martensitic phase transformation in thin sheets of Nitinol. Acta Mater. 2007, 55, 3593–3600. [Google Scholar] [CrossRef]
- Reedlunn, B.; Daly, S.; Hector, L.; Zavattieri, P.; Shaw, J. Tips and tricks for characterizing shape memory wire part 5: Full-field strain measurement by digital image correlation. Exp. Technol. 2013, 37, 62–78. [Google Scholar] [CrossRef]
- Bastawros, A.; Bart-Smith, H.; Evans, A. Experimental analysis of deformation mechanisms in a closed-cell aluminum alloy foam. J. Mech. Phys. Solids 2000, 48, 301–322. [Google Scholar] [CrossRef]
- Bart-Smith, H.; Bastawros, A.-F.; Mumm, D.; Evans, A.; Sypeck, D.; Wadley, H. Compressive deformation and yielding mechanisms in cellular Al alloys determined using X-ray tomography and surface strain mapping. Acta Mater. 1998, 46, 3583–3592. [Google Scholar] [CrossRef]
- Antoniou, A.; Onck, P.; Bastawros, A.F. Experimental analysis of compressive notch strengthening in closed-cell aluminum alloy foam. Acta Mater. 2004, 52, 2377–2386. [Google Scholar] [CrossRef]
- Jerabek, M.; Major, Z.; Lang, R. Strain determination of polymeric materials using digital image correlation. Polym. Test. 2010, 29, 407–416. [Google Scholar] [CrossRef]
- Wang, Y.; Cuitiño, A.M. Full-field measurements of heterogeneous deformation patterns on polymeric foams using digital image correlation. Int. J. Solids. Struct. 2002, 39, 3777–3796. [Google Scholar] [CrossRef]
- Reddy, B.S.; Chatterji, B.N. An FFT-Based Technique for Translation, Rotation, and Scale-Invariant Image Registration. IEEE Trans. Image Proc. 1996, 5, 1266–1271. [Google Scholar] [CrossRef]
- Min, J.; Stoughton, T.B.; Carsley, J.E.; Lin, J. Comparison of DIC methods of determining forming limit strains. Procedia Manuf. 2017, 7, 668–674. [Google Scholar] [CrossRef]
- Tsai, T.R. A versatile camera calibration technique for high-accuracy 3D machine vision metrology using off-the-shelf TV cameras and lenses. IEEE J. Robot. Autom. 1987, RA-3, 323–344. [Google Scholar] [CrossRef]
- Barranco-Gutiérrez, A.I.; Martínez-Díaz, S.; Gómez-Torres, J.L. Visión estereoscópica con Matlab y OpenCV, 1st ed.; Pearson Education: Mexico City, Mexico, 2018; pp. 10–53. [Google Scholar]
- Zhang, Z. A flexible new technique for camera calibration. IEEE Trans. Pattern Anal. Mach. Int. 2000, 22, 1330–1334. [Google Scholar] [CrossRef] [Green Version]
- Cofaru, C.; Philips, W.; Paepegem, W.V. A novel speckle pattern Adaptive digital image correlation approach with robust strain calculation. Opt. Laser Eng. 2012, 50, 187–198. [Google Scholar] [CrossRef]
- Garcia, D.; Orteu, J.J.; Penazzi, L. A combined temporal tracking and stereo-correlation technique for accurate measurement of 3D displacements: Application to sheet metal forming. J. Mater. Proc. Technol. 2002, 125–126, 736–742. [Google Scholar] [CrossRef]
- Shi, J.; Chen, F.; Lu, J.; Chen, G. An evolutionary image matching approach. Appl. Soft Comput. 2013, 13, 3060–3065. [Google Scholar] [CrossRef]
- Cyrille, B.; Philippe, D. Automatic Camera Calibration. U.S. Patents US20160350921 A1, 2 May 2017. [Google Scholar]
- Jia, Z.; Yang, J.; Liu, W.; Wang, F.; Liu, Y.; Wang, L.; Fan, C.; Zhao, K. Improved camera calibration method based on perpendicularity compensation for binocular stereo vision measurement system. Opt. Expres 2015, 23, 15205–15223. [Google Scholar] [CrossRef] [PubMed]
- García-Rodenas, L.A.; Araujo, P.; Bruyère, V.I.E.; Morando, P.J.; Regazzoni, A.E.; Blesa, M.A. A Model for the Dissolution of Metal Oxides Mediated by Heterogeneous Charge Transfer. Anales de la Asociación Química Argentina, 2004, Volume 92, n.1-3. Available online: http://www.scielo.org.ar/scielo.php?script=sci_arttext&pid=S0365-03752004000100007 (accessed on 16 April 2019).
- Computational Vision at Caltech. Camera Calibration Toolbox for Matlab. Available online: http://www.vision.caltech.edu/bouguetj/calib_doc/ (accessed on 24 February 2019).
- Matlab Company. Image Processing Toolbox™ User’s Guide; Mathworks: Sherborn, MA, USA, 2018; pp. 6-18–6-60. Available online: https://www.mathworks.com/help/images/ (accessed on 16 April 2019).


| Characteristics | Speckle Pattern | Circle Pattern |
|---|---|---|
| The normalized correlation matches well with the patterns. | YES | NO |
| Mark deformation is assumed as irregular. | NO | YES |
| A pixel can represent a mark. | Some cases | YES |
| Independence of the distance between the cameras and the specimen. | Some cases | YES |
| Position Indexes | j = 1 | j = 2 | j = 3 | j = 4 | j = 5 | j = 6 | j = 7 | j = 8 |
|---|---|---|---|---|---|---|---|---|
| i = 1 | 10.4623 | 10.334 | 10.2988 | 10.1945 | 10.2066 | 10.2369 | 10.1932 | 10.1931 |
| i = 2 | 10.2814 | 10.2721 | 10.3268 | 10.2013 | 10.1416 | 10.2001 | 10.1461 | 10.209 |
| i = 3 | 10.2739 | 10.3202 | 10.2906 | 10.2571 | 10.1665 | 10.1584 | 10.2454 | 10.2757 |
| i = 4 | 10.3615 | 10.2886 | 10.2542 | 10.2351 | 10.3094 | 10.2866 | 10.2113 | 10.217 |
| i = 5 | 10.2692 | 10.282 | 10.2885 | 10.4412 | 10.3556 | 10.2272 | 10.2494 | 10.158 |
| i = 6 | 10.4365 | 10.3514 | 10.2451 | 10.4047 | 10.361 | 10.2592 | 10.3007 | 10.3751 |
| i = 7 | 10.3587 | 10.4371 | 10.3885 | 10.1398 | 10.215 | 10.2778 | 10.1877 | 10.3029 |
| i = 8 | 10.3664 | 10.3276 | 10.3599 | 10.2999 | 10.314 | 10.3569 | 10.2394 | 10.172 |
| Position Indexes | j = 1 | j = 2 | j = 3 | j = 4 | j = 5 | j = 6 | j = 7 | j = 8 |
|---|---|---|---|---|---|---|---|---|
| i = 1 | 11.2903 | 9.6774 | 9.3548 | 9.6774 | 8.871 | 8.0645 | 8.0645 | 7.2581 |
| i = 2 | 10.4839 | 8.871 | 7.2581 | 8.0645 | 8.0645 | 5.6452 | 4.8387 | 5.6452 |
| i = 3 | 6.4516 | 8.0645 | 8.5484 | 7.7419 | 6.4516 | 6.4516 | 6.4516 | 6.4516 |
| i = 4 | 8.0645 | 9.6774 | 9.6774 | 7.2581 | 6.4516 | 6.4516 | 5.6452 | 6.4516 |
| i = 5 | 8.0645 | 10.1613 | 10.1613 | 5.6452 | 5.6452 | 6.4516 | 5.6452 | 7.2581 |
| i = 6 | 11.2903 | 11.2903 | 8.0645 | 7.2581 | 8.0645 | 10.4839 | 8.0645 | 10.9677 |
| i = 7 | 13.7097 | 10.4839 | 8.871 | 8.871 | 8.871 | 9.6774 | 8.871 | 12.9032 |
| i = 8 | 9.6774 | 6.4516 | 7.2581 | 8.0645 | 10.4839 | 7.2581 | 6.4516 | 8.871 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barranco-Gutiérrez, A.-I.; Padilla-Medina, J.-A.; Perez-Pinal, F.J.; Prado-Olivares, J.; Martínez-Díaz, S.; Gutiérrez-Frías, O.-O. New Four Points Initialization for Digital Image Correlation in Metal-Sheet Strain Measurements. Appl. Sci. 2019, 9, 1691. https://doi.org/10.3390/app9081691
Barranco-Gutiérrez A-I, Padilla-Medina J-A, Perez-Pinal FJ, Prado-Olivares J, Martínez-Díaz S, Gutiérrez-Frías O-O. New Four Points Initialization for Digital Image Correlation in Metal-Sheet Strain Measurements. Applied Sciences. 2019; 9(8):1691. https://doi.org/10.3390/app9081691
Chicago/Turabian StyleBarranco-Gutiérrez, Alejandro-Israel, José-Alfredo Padilla-Medina, Francisco J. Perez-Pinal, Juan Prado-Olivares, Saúl Martínez-Díaz, and Oscar-Octavio Gutiérrez-Frías. 2019. "New Four Points Initialization for Digital Image Correlation in Metal-Sheet Strain Measurements" Applied Sciences 9, no. 8: 1691. https://doi.org/10.3390/app9081691
APA StyleBarranco-Gutiérrez, A.-I., Padilla-Medina, J.-A., Perez-Pinal, F. J., Prado-Olivares, J., Martínez-Díaz, S., & Gutiérrez-Frías, O.-O. (2019). New Four Points Initialization for Digital Image Correlation in Metal-Sheet Strain Measurements. Applied Sciences, 9(8), 1691. https://doi.org/10.3390/app9081691








