Indices to Determine the Reliability of Rocks under Fatigue Load Based on Strain Energy Method
Abstract
1. Introduction
2. Classification and Calculation Methods of Rock Strain Energy by Tests Results
2.1. Classifications and Methods of CalculatingStrain Energy
2.2. Testing Schemes and Experimental Data
3. Test Results
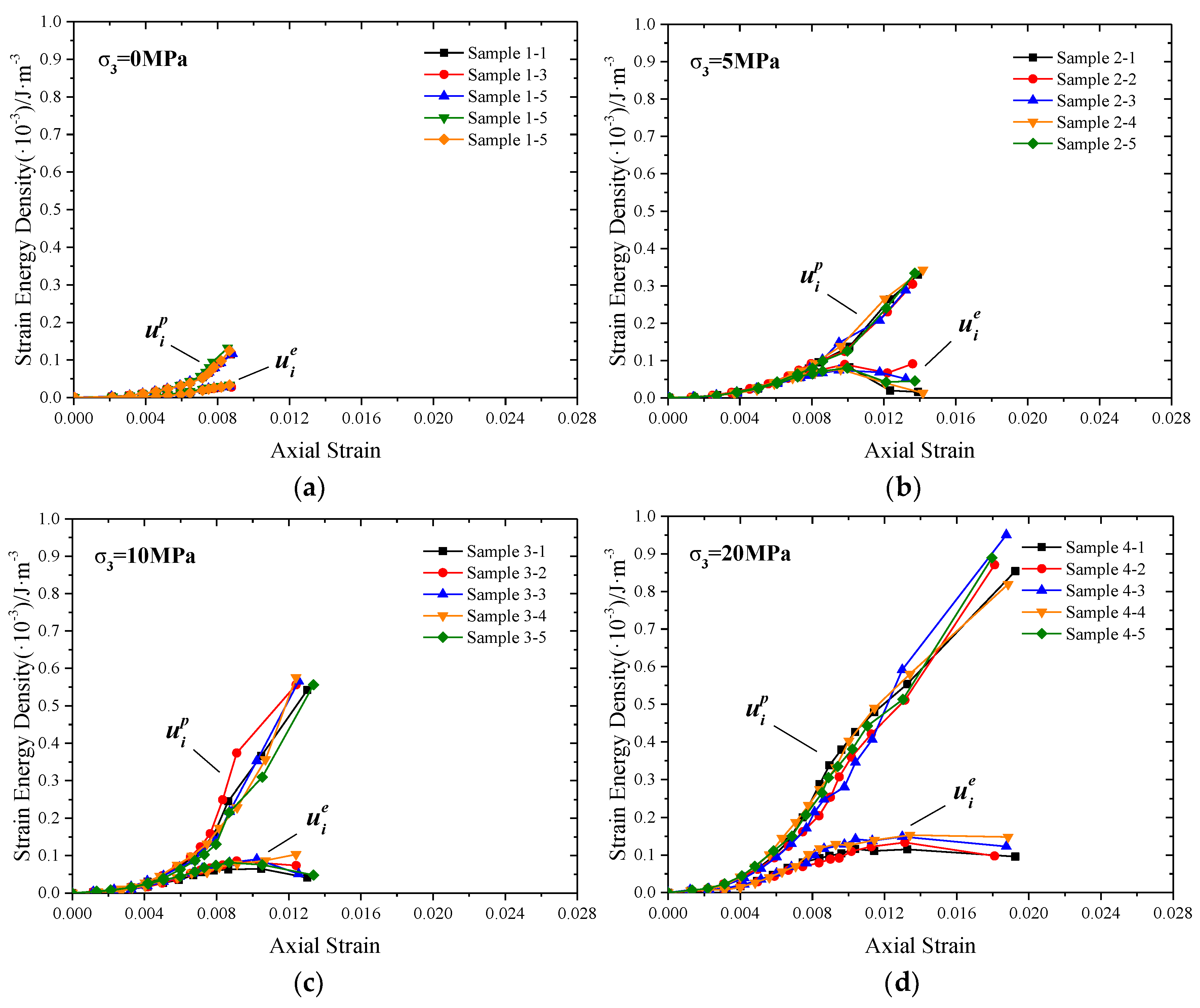
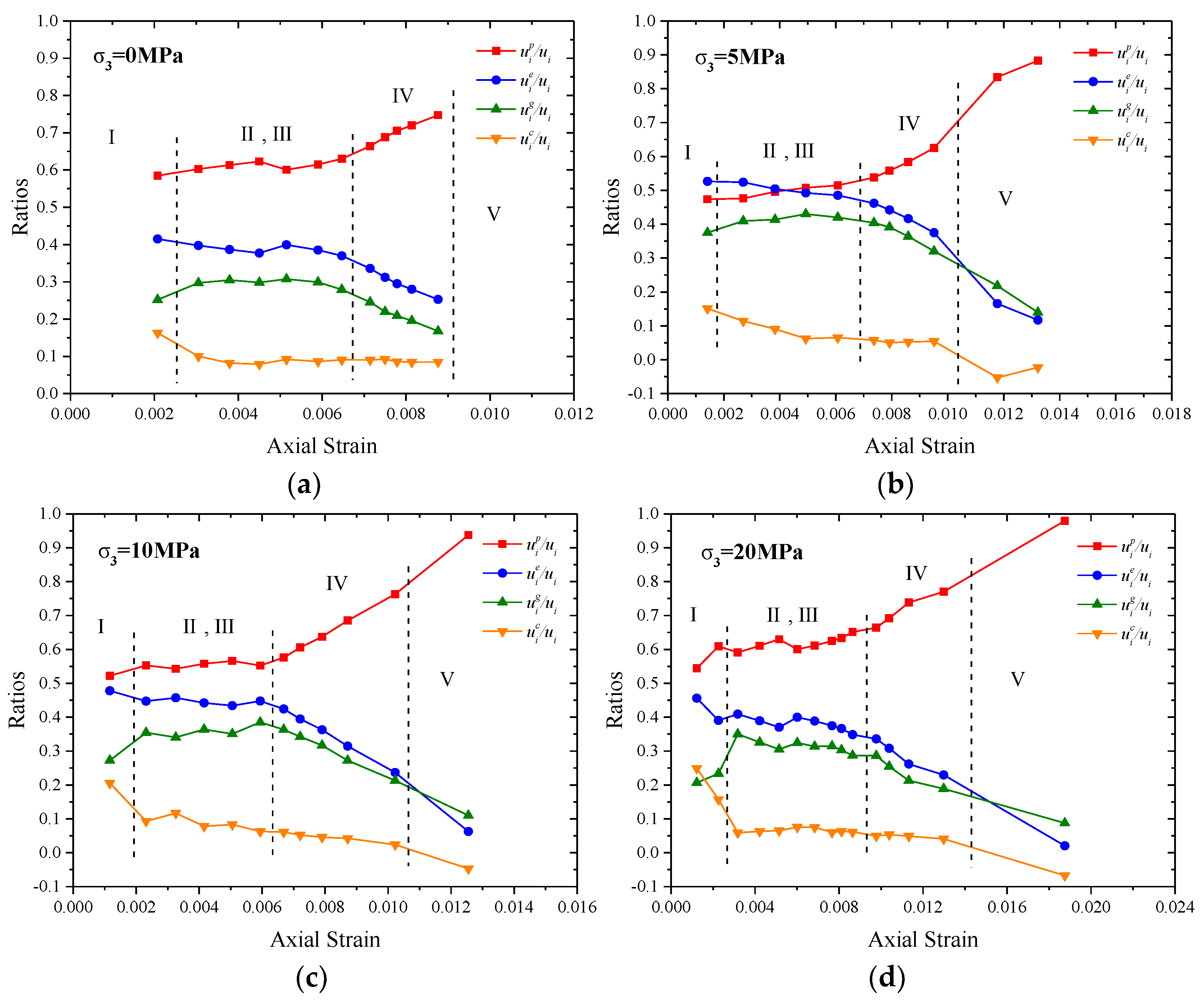
3.1. The Evolution of Strain Energy
3.2. The Distribution of Strain Energy
- (1)
- Throughout the deformation process, the proportion of the same type of strain energy to the total strain energy under different confining pressures is concentrated in a certain range and shows the same growth trend.
- (2)
- Before the yield stage, the ratio of and to the is balanced. After entering the yield stage, the proportion of and increases and decreases rapidly, respectively.
- (3)
- The ratio of to rises rapidly to a certain level at the beginning of deformation, and remains relatively stable until it enters the yield stage. After entering the yield stage, the ratio of to decreases rapidly.
- (4)
- Except the first two points, the proportion of in the pre-peak stages almost remains a constant value, and decreases rapidly in the post-peak stage.
3.3. Discussions
4. Verification and Extension of Strain Energy Index
4.1. The Relationship between Strain Energy and the Deformationmechanism of Rocks
4.2. Collection of Experimental Results from PreviousStudies
5. Conclusions
- (1)
- The elastic strain energy density of rock is composed of grain strain energy density and crack strain energy density according to the new division method. Both grain strain energy density and crack strain energy density increase before rock failure, but the growth rate of grain strain energy density decreases after it enters the yield stage.
- (2)
- From the point of view of energy distribution, the distribution of grain strain energy density is stable before the yield stage and the proportion of grain strain energy density decreases after entering the yield stage. This indicates that more strain energy density is allocated to the plastic strain energy density, which results in the decrease of grain strain energy density. This phenomenon is consistent with the deformation mechanism of cracks expansion after rock enters the yield stage. However, the distribution of crack strain energy is stable during the pre-peak deformation stage, and it decreases only after the peak stage. We believe that this phenomenon is related to the integrity of the rock because no matter how many cracks grow in the rock, as long as the rock has not failed, the restorable part of the crack is coordinated with the total strain energy density.
- (3)
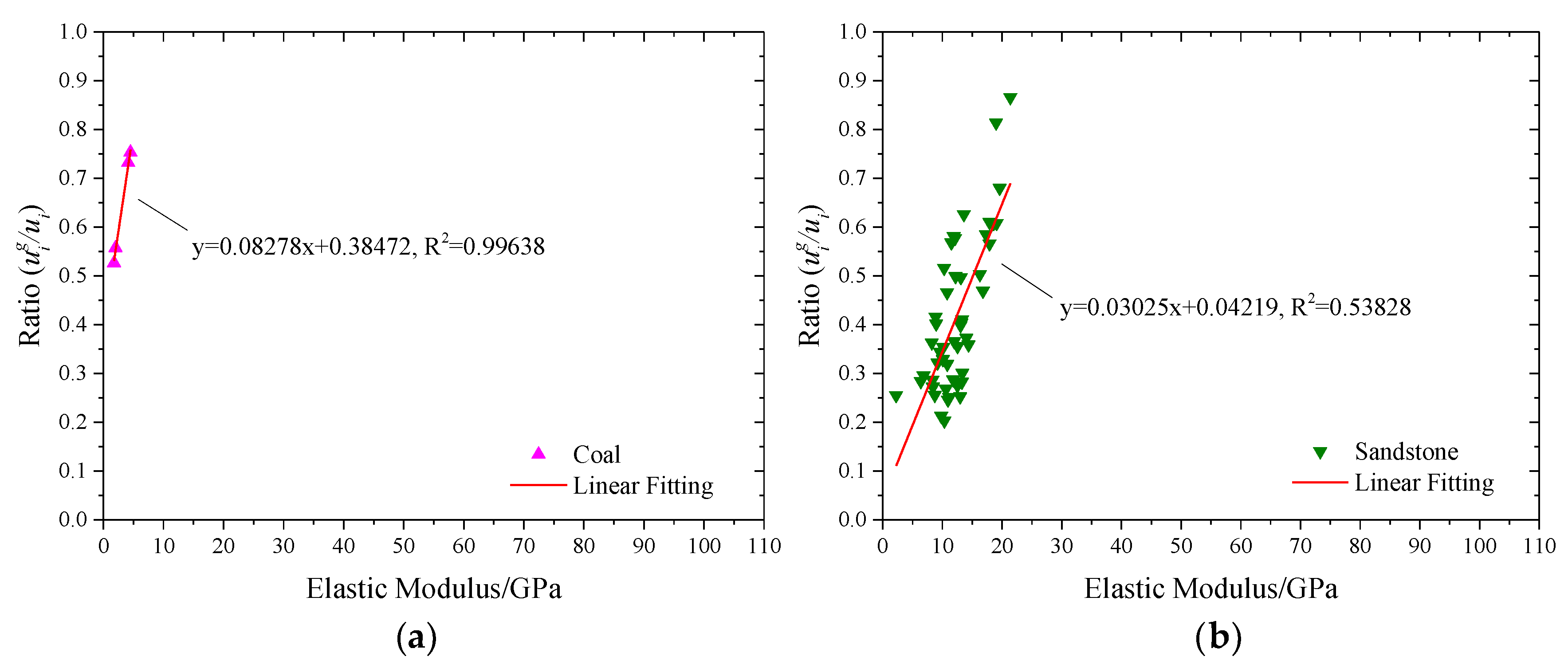
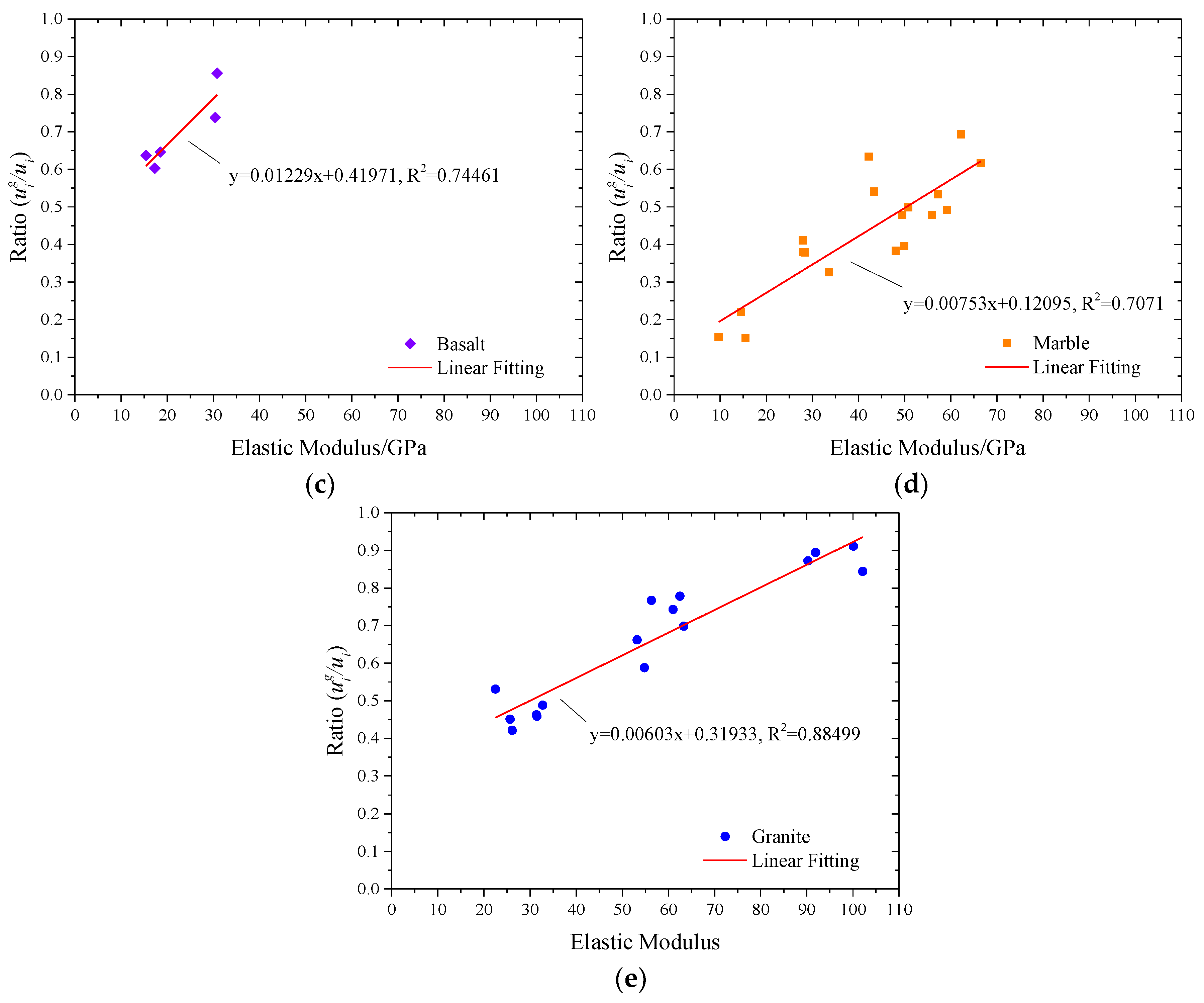
- Through the analysis of the data obtained from other literatures, it is found that the proportion of grain strain energy density to total strain energy density can be used as the index to determine the stability of rock. The proportion of crack strain energy density to total strain energy density can be used as the index to determine rock failure.
- (4)
- The proportion of grain strain energy density to total strain energy density is concentrated in the same rock type, which has linear relationship with elastic modulus. The slope of the linear equation between different rocks and elastic modulus will decrease with the increase of uniaxial compression strength of rock under normal conditions.
- (5)
- The proportion of crack strain energy density to total strain energy density is stable in the range of 0.04~0.13, which is independent of rock type and elastic modulus.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gu, D. Fundamentals of Rock Engineering Geomechanics; Science Press: Beijing, China, 1979. [Google Scholar]
- MARTIN; CHANDLER. The progressive fracture of Lac du Bonnet granite. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1994, 31, 643–659. [Google Scholar] [CrossRef]
- Meifeng, C.; Manchao, H.; Dongyan, L. Rock Mechanics and Engineering; Science Press: Beijing, China, 2002; pp. 52–61. [Google Scholar]
- Wang, S. Geological nature of rock and its deduction for rock mechanics. Chin. J. Rock Mech. Eng. 2009, 28, 433–450. [Google Scholar]
- Lockner, D. The role of acoustic emission in the study of rock fracture. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 883–899. [Google Scholar] [CrossRef]
- Yamada, I.; Masuda, K.; Mizutani, H. Electromagnetic and acoustic emission associated with rock fracture. Phys. Earth Planet. Inter. 1989, 57, 157–168. [Google Scholar] [CrossRef]
- Rudajev, V.; Vilhelm, J.; Lokajı́Ček, T. Laboratory studies of acoustic emission prior to uniaxial compressive rock failure. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 2000, 37, 699–704. [Google Scholar] [CrossRef]
- Shah, K.R.; Labuz, J.F. Damage mechanisms in stressed rock from acoustic emission. J. Geophys. Res. Solid Earth 1995, 100, 15527–15539. [Google Scholar] [CrossRef]
- Wawersik, W.R.; Fairhurst, C. A study of brittle rock fracture in laboratory compression experiments. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1970, 7, 561–575. [Google Scholar] [CrossRef]
- Carpinteri, A.; Lacidogna, G.; Corrado, M.; Battista, E.D. Cracking and crackling in concrete-like materials: A dynamic energy balance. Eng. Fract. Mech. 2016, 155, 130–144. [Google Scholar] [CrossRef]
- Iturrioz, I.; Lacidogna, G.; Carpinteri, A. Acoustic emission detection in concrete specimens: Experimental analysis and simulations by a lattice model. Int. J. Damage Mech. 2013, 23, 327–358. [Google Scholar] [CrossRef]
- Zhu, W.; Feng, C. Constitutive model of energy dissipation and its application to stability analysis of ship lock slope in three gorges project. Chin. J. Rock Mech. Eng. 2000, 19, 261–264. [Google Scholar]
- Wang, J.A.; Park, H.D. Comprehensive prediction of rockburst based on analysis of strain energy in rocks. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2001, 16, 49–57. [Google Scholar] [CrossRef]
- Xiao, J.Q.; Ding, D.X.; Jiang, F.L.; Xu, G. Fatigue damage variable and evolution of rock subjected to cyclic loading. Int. J. Rock Mech. Min. Sci. 2010, 47, 461–468. [Google Scholar] [CrossRef]
- Hua, A. Energy analysis of surrounding rocks in underground engineering. Chin. J. Rock Mech. Eng. 2003, 22, 1054–1059. [Google Scholar]
- Yu, M.H. Advances in strength theories for materials under complex stress state in the 20th Century. Adv. Mech. 2004, 34, 529–560. [Google Scholar] [CrossRef]
- Heping, X.; Yang, J.; Liyun, L.; Ruidong, P. Energy mechanism of deformation and failure of rock masses. Chin. J. Rock Mech. Eng. 2008, 27, 1729–1740. [Google Scholar]
- Carpinteri, A.; Corrado, M.; Lacidogna, G. Three different approaches for damage domain characterization in disordered materials: Fractal energy density, b-value statistics, renormalization group theory. Mech. Mater. 2012, 53, 15–28. [Google Scholar] [CrossRef]
- Xie, H.P.; Yang, J.U.; Li-Yun, L.I. Criteria for strength and structural failure of rocks based on energy dissipation and energy release principles. Chin. J. Rock Mech. Eng. 2005, 24, 3003–3010. [Google Scholar]
- Cai, M.; Dong, J.I.; Guo, Q. Study of rockburst prediction based on in-situ stress measurement and theory of energy accumulation caused by mining disturbance. Chin. J. Rock Mech. Eng. 2013, 32, 1973–1980. [Google Scholar]
- Zhang, Z.; Feng, G. Experimental investigations on energy evolution characteristics of coal,sandstone and granite during loading process. J. China Univ. Min. Technol. 2015, 44, 416–422. [Google Scholar]
- Jiang, C.; Duan, M.; Yin, G.; Wang, J.G.; Lu, T.; Xu, J.; Zhang, D.; Huang, G. Experimental study on seepage properties, AE characteristics and energy dissipation of coal under tiered cyclic loading. Eng. Geol. 2017, 221, 114–123. [Google Scholar] [CrossRef]
- Meng, Q.; Zhang, M.; Han, L.; Pu, H.; Chen, Y. Acoustic Emission Characteristics of Red Sandstone Specimens Under Uniaxial Cyclic Loading and Unloading Compression. Rock Mech. Rock Eng. 2018, 51, 1–20. [Google Scholar] [CrossRef]
- Cook, N.G.W. The failure of rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1965, 2, 389–403. [Google Scholar] [CrossRef]
- Wawersik, W.R.; Brace, W.F. Post-failure behavior of a granite and diabase. Rock Mech. 1971, 3, 61–85. [Google Scholar] [CrossRef]
- Bukowska, M. Post-peak failure modulus in problems of mining geo-mechanics. J. Min. Sci. 2013, 49, 731–740. [Google Scholar] [CrossRef]
- Bogusz, A.; Bukowska, M. Stress-strain characteristics as a source of information on the destruction of rocks under the influence of load. J. Sustain. Min. 2015, 14, 46–54. [Google Scholar] [CrossRef]
- Pasternak, E.; Dyskin, A.V.; Sevel, G. Chains of oscillators with negative stiffness elements. J. Sound Vib. 2014, 333, 6676–6687. [Google Scholar] [CrossRef]
- Pasternak, E.; Dyskin, A. Dynamic Instability in Geomaterials Associated with the Presence of Negative Stiffness Elements. In Bifurcation and Degradation of Geomaterials in the New Millennium; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Zhang, X.; Tahmasebi, P. Micromechanical evaluation of rock and fluid interactions. Int. J. Greenh. Gas Control 2018, 76, 266–277. [Google Scholar] [CrossRef]
- Bingye, X.; Xinsheng, L. Application Elastic- Plastic Mechanics; Tsinghua University Press: Beijing, China, 1995. [Google Scholar]
- Xingguang, Z.; Pengfei, L.; Like, M.; Rui, S.; Ju, W. Damage and dilation characteristics of deep granite at beishan under cyclic loading-unloading conditions. Chin. J. Rock Mech. Eng. 2014, 33, 1740–1748. [Google Scholar]
- Guo, S.; Qi, S.; Zhan, Z.; Zheng, B. Plastic-strain-dependent strength model to simulate the cracking process of brittle rocks with an existing non-persistent joint. Eng. Geol. 2017, 231, 114–125. [Google Scholar] [CrossRef]
- Zhang, C.; Tu, S.; Zhang, L. Analysis of Broken Coal Permeability Evolution Under Cyclic Loading and Unloading Conditions by the Model Based on the Hertz Contact Deformation Principle. Transp. Porous Med. 2017, 119, 1–16. [Google Scholar] [CrossRef]
- Fengqiang, G.; Jingyi, Y.; Xibing, L. A new criterion of rock burst proneness based on the linear energy storage law and the residual elastic energy index. Chin. J. Rock Mech. Eng. 2018, 37, 1993–2014. [Google Scholar]
- Bagde, M.N.; Petroš, V. Fatigue and dynamic energy behaviour of rock subjected to cyclical loading. Int. J. Rock Mech. Min. Sci. 2009, 46, 200–209. [Google Scholar] [CrossRef]
- Hui, Z.; Fanjie, Y.; Chuanqing, Z.; Rongchao, X.; Kai, Z. An elastoplastic coupling mechanical model for marble considering confining. Chin. J. Rock Mech. Eng. 2012, 31, 2389–2399. [Google Scholar]
- Ma, L.J.; Liu, X.Y.; Wang, M.Y.; Xu, H.F.; Hua, R.P.; Fan, P.X.; Jiang, S.R.; Wang, G.A.; Yi, Q.K. Experimental investigation of the mechanical properties of rock salt under triaxial cyclic loading. Int. J. Rock Mech. Min. Sci. 2013, 62, 34–41. [Google Scholar] [CrossRef]
- Zhou, Z.L.; Zhi-Bo, W.U.; Xi-Bing, L.I.; Xiang, L.I.; Chun-De, M.A. Mechanical behavior of red sandstone under cyclic point loading. Trans. Nonferrous Met. Soc. China 2015, 25, 2708–2717. [Google Scholar] [CrossRef]
- Li, D.; Sun, Z.; Xie, T.; Li, X.; Ranjith, P.G. Energy evolution characteristics of hard rock during triaxial failure with different loading and unloading paths. Eng. Geol. 2017, 228, 270–281. [Google Scholar] [CrossRef]
- Brace, W.F.; Paulding, B.W.; Scholz, C. Dilatancy in the fracture of crystalline rocks. J. Geophys. Res. 1966, 71, 3939–3953. [Google Scholar] [CrossRef]
- Bieniawski, Z.T. Mechanism of brittle fracture of rock: Part I—Theory of the fracture process. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1967, 4, 395–406. [Google Scholar] [CrossRef]
- You, M. Experimental study on strengthening of marble specimen in cyclic loading of uniaxial or pseudo-triaxial compression. Chin. J. Solid Mech. 2008, 29, 66–72. [Google Scholar]
- Fukun, X.; Zhiliang, S.; Gang, L.; Ze, Z.; Fengrui, Z. Relationship between hysteresis loop and elastoplastic strain energy during cyclic loading and unloading. Chin. J. Rock Mech. Eng. 2014, 33, 1791–1797. [Google Scholar]
- Zhen, L.I.; Zhou, H.; Yang, F.J.; Zhao, H.B.; Zhong-Liang, R.U. Elastoplastic coupling strain definition and constitutive function. Rock Soil Mech. 2018, 39, 917–925. [Google Scholar]
- Zhizhen, Z.; Feng, G. Research on nonlinear characteristics of rock energy evolution under uniaxial compression. Chin. J. Rock Mech. Eng. 2012, 31, 1198–1207. [Google Scholar]
- Meng, Q.; Zhang, M.; Han, L.; Pu, H.; Nie, T. Effects of Acoustic Emission and Energy Evolution of Rock Specimens Under the Uniaxial Cyclic Loading and Unloading Compression. Rock Mech. Rock Eng. 2016, 49, 1–14. [Google Scholar] [CrossRef]
- Zhu, X.; Li, Y.; Wang, C.; Sun, X.; Liu, Z. Deformation Failure Characteristics and Loading Rate Effect of Sandstone Under Uniaxial Cyclic Loading and Unloading. Geotech. Geol. Eng. 2018, 1–8. [Google Scholar] [CrossRef]
- Heap, M.J.; Vinciguerra, S.; Meredith, P.G. The evolution of elastic moduli with increasing crack damage during cyclic stressing of a basalt from Mt. Etna volcano. Tectonophysics 2009, 471, 153–160. [Google Scholar] [CrossRef]
- Yang, S.Q.; Xu, P.; Ranjith, P.G.; Chen, G.F.; Jing, H.W. Evaluation of creep mechanical behavior of deep-buried marble under triaxial cyclic loading. Arabian J. Geosci. 2015, 8, 6567–6582. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, K.; Feng, X.T. Experimental study on progressive yielding of marble. Mater. Res. Innov. 2015, 15, s143–s146. [Google Scholar] [CrossRef]
- Jun, L.; Da-wei, H.; Hui, Z.; Jing-jing, L.; Tao, L.; Lin-ken, S. Gas permeability of granite in triaxial cyclic compression tests. Rock Soil Mech. 2018, 40, 1–8. [Google Scholar]
- Zhang, Z.; Zhu, J.; Wang, B.; Feng, Z.; Bo, L.U.; Zhang, L.; Jiang, Y. The damage and shear dilation property evolution based on energy dissipation mechanism of gneissic granite. Chin. J. Rock Mech. Eng. 2018, 37, 3441–3448. [Google Scholar]
- Peng, Z.; Hongguang, J.I.; Sun, L.; Zhang, Z.; Yu, G.; Hua, J.; Chengjiang, L.I. Experimental study of characteristics of irreversibility and fracture precursors of acoustic emission in rock under different confining pressures. Chin. J. Rock Mech. Eng. 2016, 35, 1333–1340. [Google Scholar]
- China, MOHURD. Code for Hydropower Engineering Geological Investigation. Available online: http://www.mohurd.gov.cn/wjfb/201702/t20170224_230737.html (accessed on 18 August 2016).
- China, MOHURD. Standard for Engineering Classification of Rock Mass; China Planning Press: Beijing, China, 2015. Available online: http://www.mohurd.gov.cn/wjfb/201508/t20150829_224347.html (accessed on 27 August 2014).
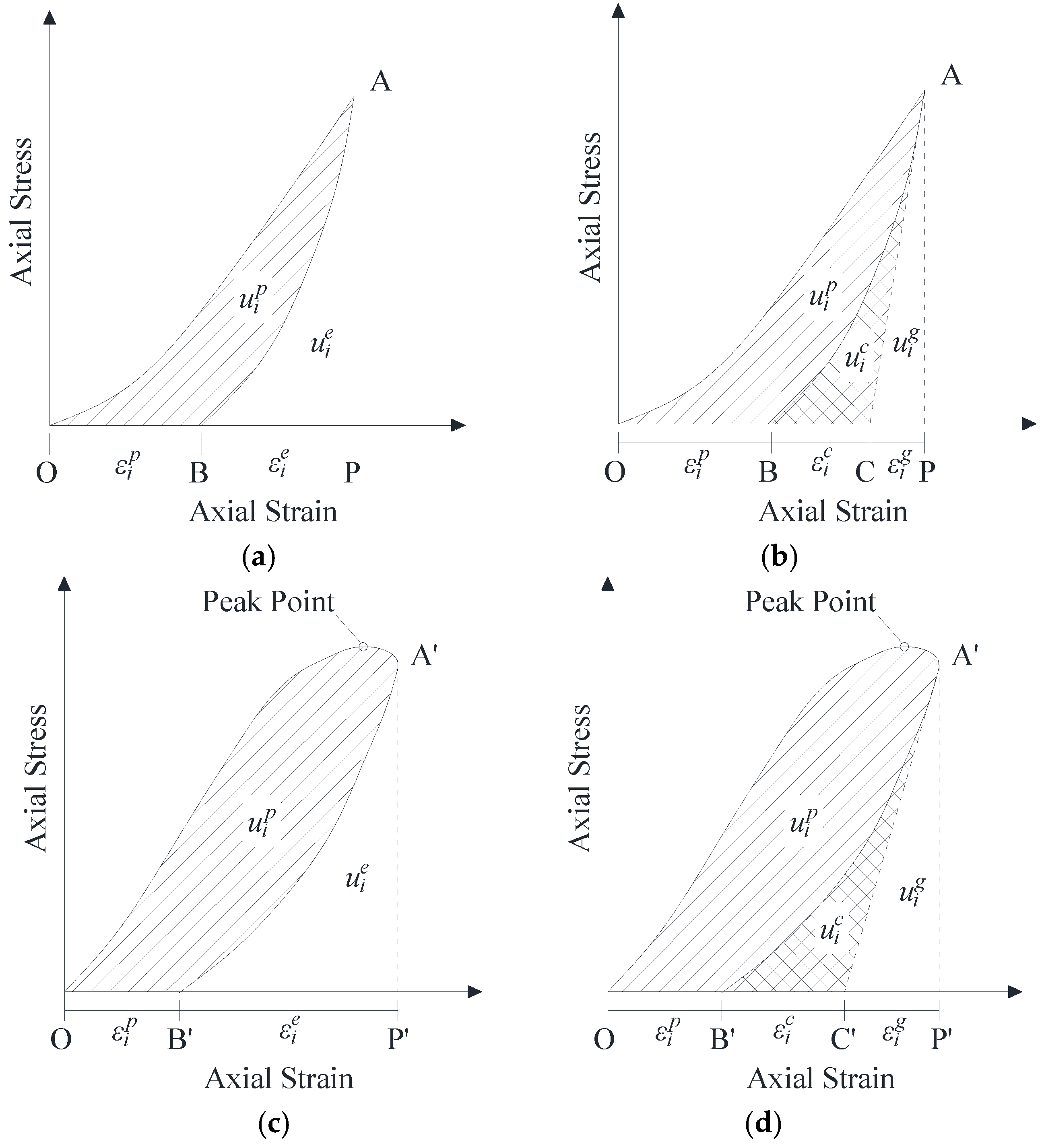
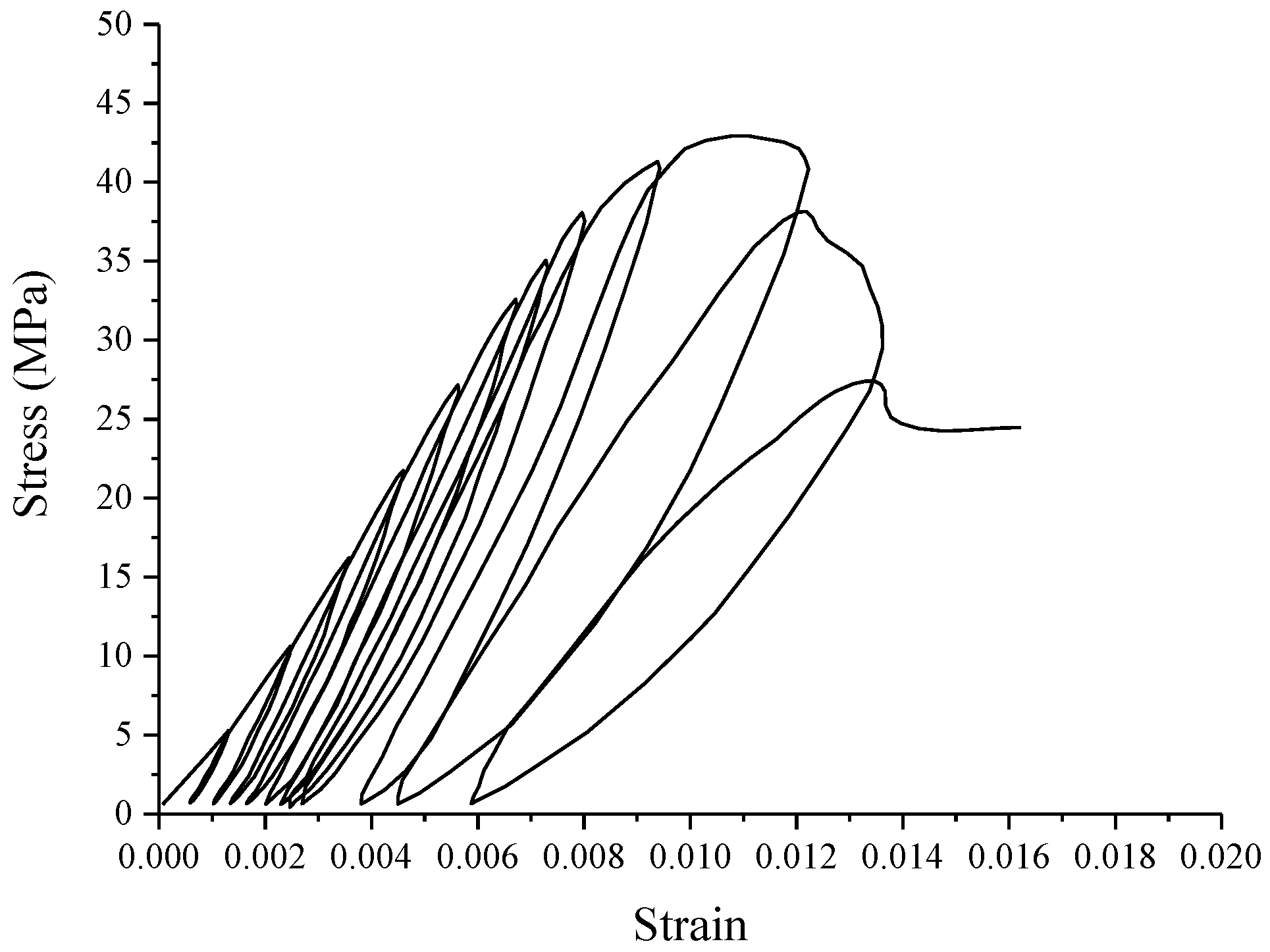


| σ3 (MPa) | E (GPa) | σcd (MPa) | εcd | σp (MPa) | εp |
|---|---|---|---|---|---|
| 0 | 2.79 | 18.69 | 0.0064 | 24.92 | 0.0093 |
| 5 | 4.42 | 30.95 | 0.0068 | 38.15 | 0.0109 |
| 10 | 5.27 | 38.23 | 0.0071 | 49.75 | 0.0118 |
| 20 | 8.75 | 51.84 | 0.0083 | 67.87 | 0.0161 |
| No. | Rock Type | Number of Data | σ3 (MPa) | References |
|---|---|---|---|---|
| 1 | Marble | 6 | 0 | [43] |
| 2 | Marble | 4 | 40 | [44] |
| 3 | Granite | 4 | 10 | [32] |
| 4 | Coal | 1 | 0 | [44] |
| 5 | Coal | 1 | 10 | [21] |
| 6 | Sandstone | 1 | 10 | [21] |
| 7 | Granite | 1 | 10 | [21] |
| 8 | Marble | 1 | 5 | [37] |
| 9 | Sandstone | 1 | 0 | [45] |
| 10 | Sandstone | 1 | 0 | [17] |
| 11 | Sandstone | 2 | 0 | [46] |
| 12 | Sandstone | 6 | 0 | [47] |
| 13 | Sandstone | 1 | 0 | [48] |
| 14 | Basalt | 5 | 0 | [49] |
| 15 | Granite | 1 | 0 | [2] |
| 16 | Sandstone | 37 | 0 | [23] |
| 17 | Coal | 2 | 3 | [22] |
| 18 | Marble | 1 | 20 | [50] |
| 19 | Marble | 1 | 35 | [50] |
| 20 | Marble | 1 | 50 | [50] |
| 21 | Marble | 2 | 5 | [51] |
| 22 | Marble | 1 | 20 | [51] |
| 23 | Marble | 1 | 40 | [51] |
| 24 | Granite | 3 | 2 | [52] |
| 25 | Granite | 1 | 5 | [52] |
| 26 | Granite | 1 | 7 | [52] |
| 27 | Granite | 1 | 40 | [53] |
| 28 | Granite | 1 | 1 | [54] |
| 29 | Granite | 1 | 10 | [54] |
| 30 | Granite | 1 | 20 | [54] |
| 31 | Granite | 1 | 30 | [54] |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, H.; Wang, S.; Pei, X.; Chen, W. Indices to Determine the Reliability of Rocks under Fatigue Load Based on Strain Energy Method. Appl. Sci. 2019, 9, 360. https://doi.org/10.3390/app9030360
Fu H, Wang S, Pei X, Chen W. Indices to Determine the Reliability of Rocks under Fatigue Load Based on Strain Energy Method. Applied Sciences. 2019; 9(3):360. https://doi.org/10.3390/app9030360
Chicago/Turabian StyleFu, Huanran, Sijing Wang, Xiangjun Pei, and Weichang Chen. 2019. "Indices to Determine the Reliability of Rocks under Fatigue Load Based on Strain Energy Method" Applied Sciences 9, no. 3: 360. https://doi.org/10.3390/app9030360
APA StyleFu, H., Wang, S., Pei, X., & Chen, W. (2019). Indices to Determine the Reliability of Rocks under Fatigue Load Based on Strain Energy Method. Applied Sciences, 9(3), 360. https://doi.org/10.3390/app9030360





