A Switching Hybrid Dynamical System: Toward Understanding Complex Interpersonal Behavior
Abstract
1. Introduction
2. Continuous Dynamics
2.1. The Haken-Kelso-Bunz (HKB) Model
2.2. Identity of Synchronization Modes Revealed by the Relative Phase Region During Interpersonal Competition
3. Continuous to Discrete Dynamics: Return Map
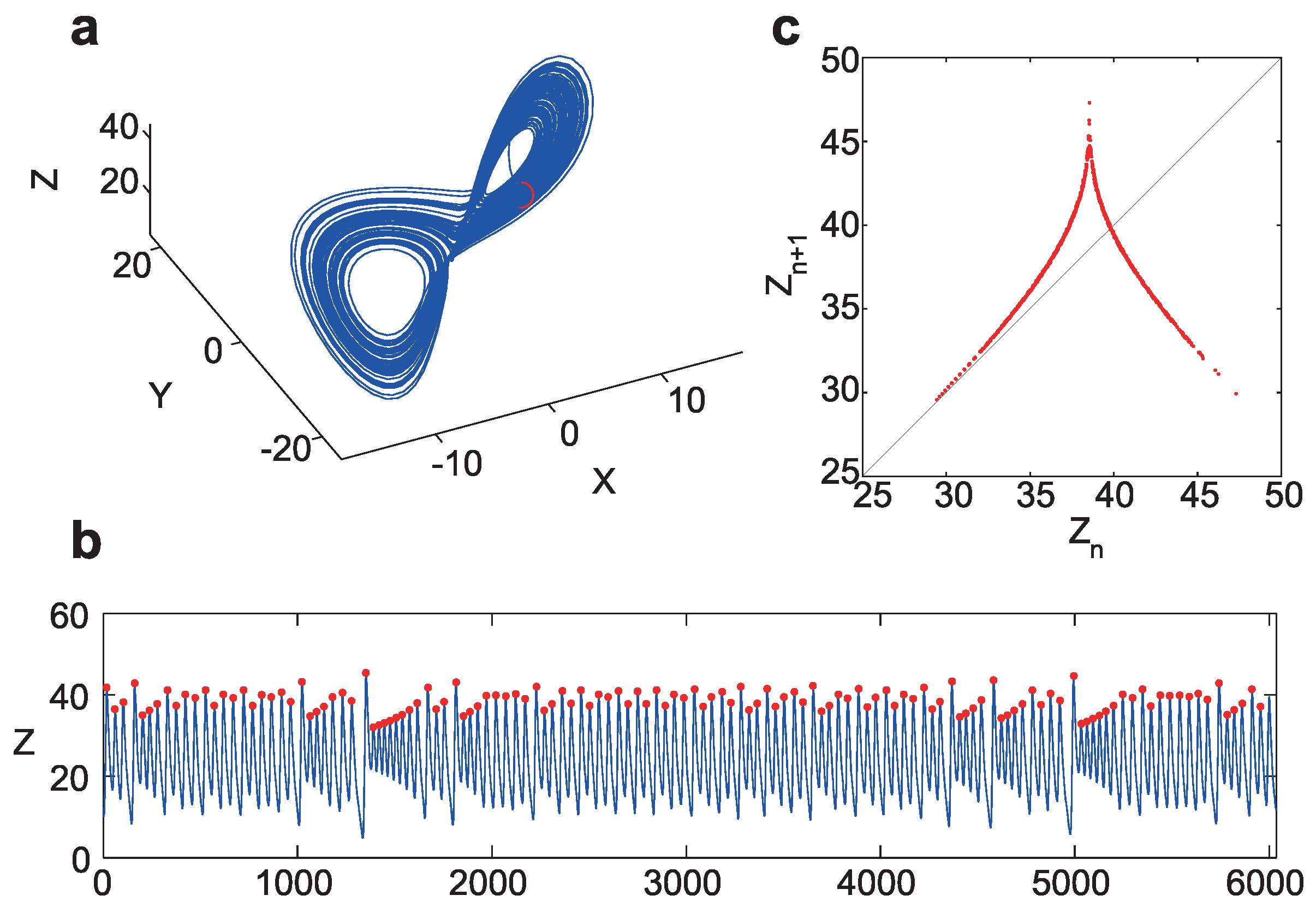
3.1. Lorenz Map
3.2. Identification of Coordination Patterns by the Return Map during Interpersonal Competition
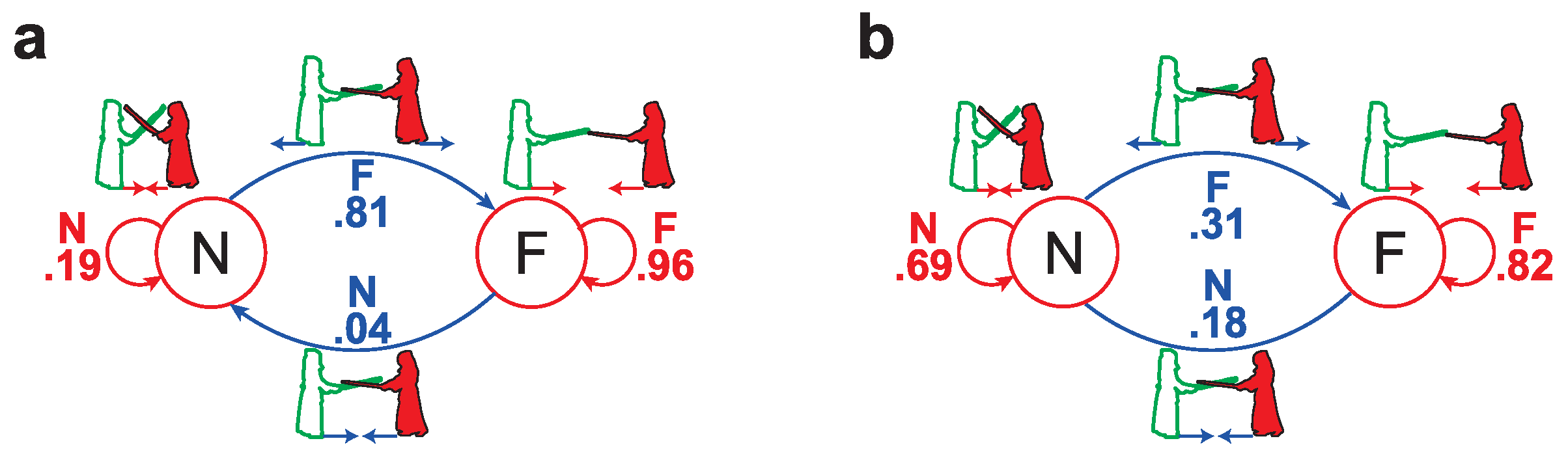
3.3. Identification of Switching Pattern for Expertise via the State Transition Probability
4. Continuous to Discrete Dynamics: Switching Dynamics
4.1. Switching Dynamics
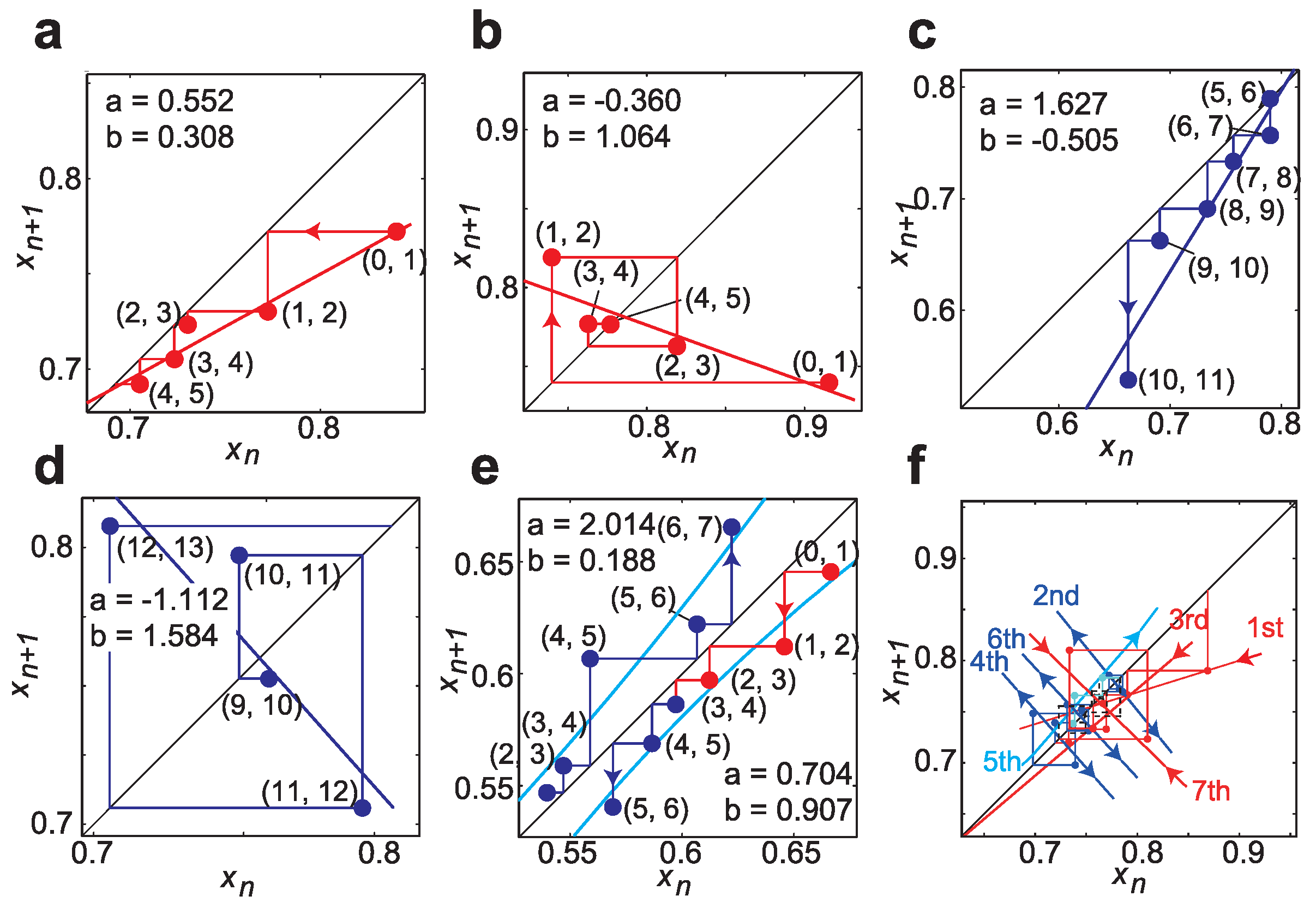
4.2. Underlying Simple Rule for Complex Striking Actions as per the Poincaré Map
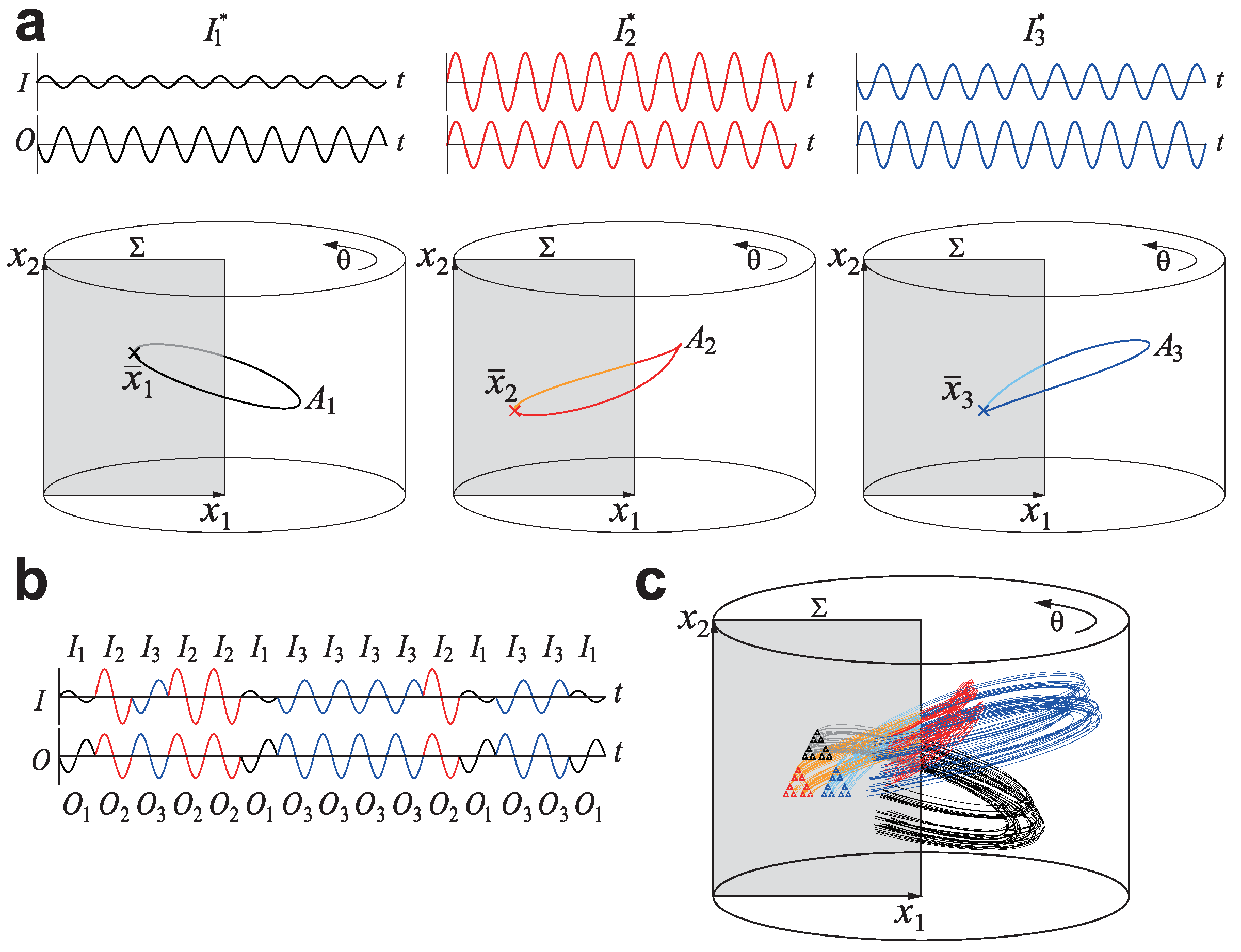
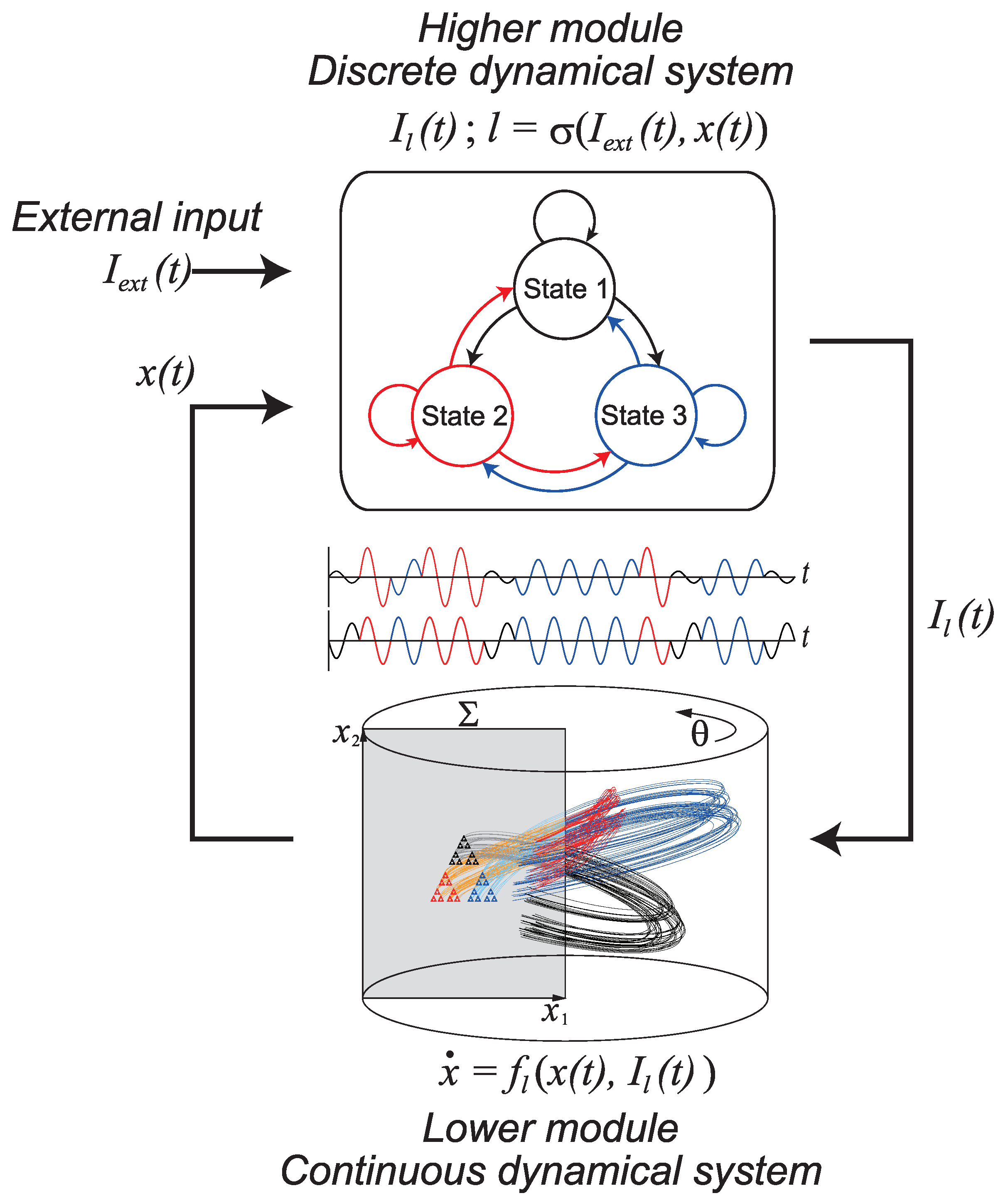
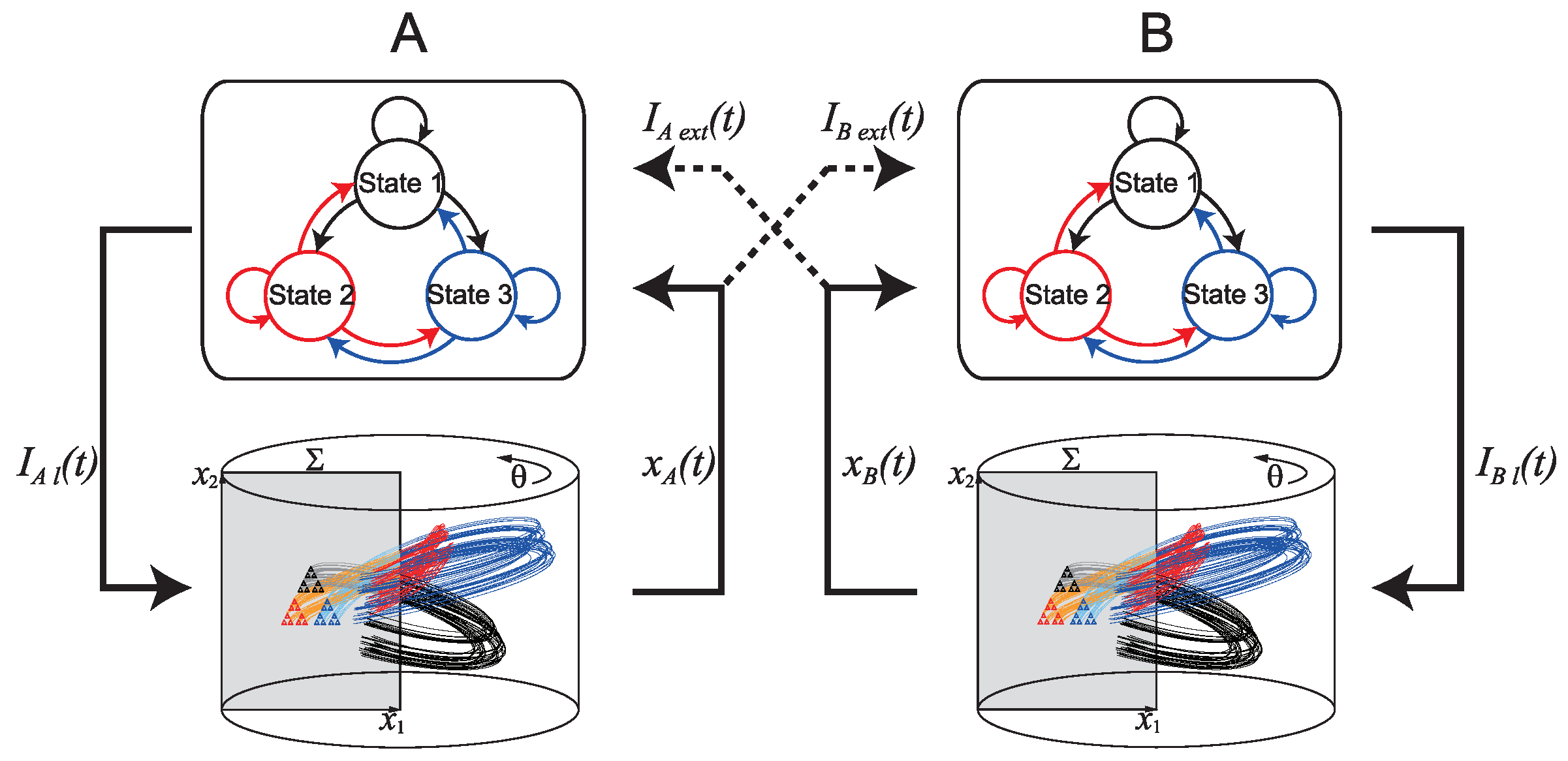
5. Switching Hybrid Dynamics
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kelso, J.A.S. Phase transitions and critical behavior in human bimanual coordination. Am. J. Physiol. 1984, 246, R1000–R1004. [Google Scholar] [CrossRef]
- Bennett, M.; Schatz, M.F.; Rockwood, H.; Wiesenfeld, K. Huygens’ clock. Proc. R. Soc. A Math. Phys. Eng. Sci. 2002, 458, 563–579. [Google Scholar] [CrossRef]
- Strogatz, S. SYNC: The Emerging Science of Spontaneous Order; Hyperion Books: New York, NY, USA, 2003. [Google Scholar]
- Haken, H. Synergetics: An Introduction, Non-Equilibrium Phase Transitions and Self-Organization in Physics, Chemistry and Biology, 2nd ed.; Springer: Berlin, Germany, 1978. [Google Scholar]
- Haken, H.; Kelso, J.A.S.; Bunz, H. A theoretical model of phase transitions in human hand movements. Biol. Cybern. 1985, 51, 347–356. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, R.C.; Carello, C.; Turvey, M.T. Phase transitions and critical fluctuations in the visual coordination of rhythmic movements between people. J. Exp. Psychol. Hum. Percept. Perform. 1990, 16, 227–247. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, R.C.; Turvey, M.T. Phase-entrainment dynamics of visually coupled rhythmic movements. Biol. Cybern. 1994, 70, 369–376. [Google Scholar] [CrossRef] [PubMed]
- Kuramoto, Y. Chemical Oscillations, Waves, and Turbulence; Springer: Berlin, Germany, 1984. [Google Scholar]
- Néda, Z.; Ravasz, E.; Brechet, Y.; Vicsek, T.; Barabási, A.L. Self-organizing processes: the sound of many hands clapping. Nature 2000, 403, 850–851. [Google Scholar] [CrossRef] [PubMed]
- Frank, T.D.; Richardson, M.J. On a test statistics for the Kuramoto order parameter of synchronization: An illustration for group synchronization during rocking chairs. Phys. D 2010, 239, 2084–2092. [Google Scholar] [CrossRef]
- Richardson, M.J.; Garcia, R.L.; Frank, T.D.; Gergor, M.; Marsh, K.L. Measuring group synchrony: A cluster-phase method for analyzing multivariate movement time-series. Front. Physiol. 2012, 3, 405. [Google Scholar] [CrossRef] [PubMed]
- Aihara, I.; Takeda, R.; Mizumoto, T.; Otsuka, T.; Takahashi, T.; Okuno, H.G.; Aihara, K. Complex and transitive synchronization in a frustrated system of calling frogs. Phys. Rev. E 2011, 83, 031913. [Google Scholar] [CrossRef] [PubMed]
- Golubitsky, M.; Stewart, I. The Symmetry Perspective: From Equilibrium to Chaos in Phase Space and Physical Space; Birkhäuser: Basel, Switzerland, 2002. [Google Scholar]
- Yokoyama, K.; Yamamoto, Y. Three people can synchronize as coupled oscillators during sports activities. PLoS Comput. Biol. 2011, 7, e1002181. [Google Scholar] [CrossRef]
- Gray, R. ‘Markov at the bat’: A model of cognitive processing in baseball batters. Psychol. Sci. 2002, 13, 542–547. [Google Scholar] [CrossRef] [PubMed]
- McGarry, T.; Franks, I.M. A stochastic approach to predicting competition squash match-play. J. Sports Sci. 1994, 12, 537–584. [Google Scholar] [CrossRef] [PubMed]
- McGarry, T.; Franks, I. Development, application, and limitation of a stochastic Markov model in explaining championship squash performance. Res. Q. Exerc. Sport 1996, 67, 406–415. [Google Scholar] [CrossRef] [PubMed]
- Kelso, J.A.S. Dynamic Patterns: The Self-Organization of Brain and Behavior; The MIT Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Coey, C.; Varlet, M.; Schmidt, R.C.; Richardson, M.J. Effects of movement stability and congruency on the emergence of spontaneous interpersonal coordination. Exp. Brain Res. 2011, 211, 483–493. [Google Scholar] [CrossRef] [PubMed]
- Fine, J.M.; Amazeen, E.L. Interpersonal Fitts’ law: When two perform as one. Exp. Brain Res. 2011, 211, 459–469. [Google Scholar] [CrossRef] [PubMed]
- Richardson, M.J.; Marsh, K.L.; Schmidt, R.C. Effects of visual and verbal interaction on unintentional interpersonal coordination. J. Exp. Psychol. Hum. Percept. Perform. 2005, 31, 62–79. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, R.C.; O’Brien, B. Evaluating the dynamics of unintended interpersonal coordination. Ecol. Psychol. 1997, 9, 189–206. [Google Scholar] [CrossRef]
- Yamanishi, J.; Kawato, M.; Suzuki, R. Two coupled oscillators as a model for the coordinated finger tapping by both hands. Biol. Cybern. 1980, 37, 219–225. [Google Scholar] [CrossRef]
- Scholz, J.P.; Kelso, J.A.S. A quantitative approach to understanding the formation and change of coordinated movement patterns. J. Mot. Behav. 1989, 21, 122–144. [Google Scholar] [CrossRef]
- Pikovsky, A.; Rosenblum, M.; Kurths, J. Phase synchronization in regular and chaotic system: a tutorial. Int. J. Bifurc. Chaos 2000, 10, 2291–2306. [Google Scholar] [CrossRef]
- Pikovsky, A.; Rosenblum, M.; Kurths, J. Synchronization: A Universal Concept in Nonlonear Sciences; Cambridge University Press: New York, NY, USA, 2001. [Google Scholar]
- Carvalho, J.; Araújo, D.; Travassos, B.; Esteves, P.; Pessanha, L.; Pereira, F.; Davids, K. Dynamics of players relative positioning during baseline rallies in tennis. J. Sports Sci. 2013, 31, 1596–1605. [Google Scholar] [CrossRef] [PubMed]
- Lames, M. Modelling the interaction in game sports—Relative phase and moving correlations. J. Sports Sci. Med. 2006, 5, 556–560. [Google Scholar] [PubMed]
- Palut, Y.; Zanone, P.G. A dynamical analysis of tennis: Concepts and data. J. Sports Sci. 2005, 23, 1021–1032. [Google Scholar] [CrossRef] [PubMed]
- McGarry, T. Identifying patterns in squash contests using dynamical analysis and human perception. Int. J. Perform. Anal. Sport 2006, 6, 134–147. [Google Scholar] [CrossRef]
- Chow, J.Y.; Seifert, L.; Hérault, R.; Chia, S.J.Y.; Lee, M.C.Y. A dynamical system perspective to understanding badminton singles game play. Hum. Mov. Sci. 2014, 33, 70–87. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Okumura, M.; Yokoyama, K.; Kijima, A. Interpersonal Coordination in Competitive Sports Contexts: Martial Arts. In Interpersonal Coordination and Performance in Social Systems; Passos, P., Davids, K., Chow, J.Y., Eds.; Routledge: Oxford, UK, 2016; pp. 179–194. [Google Scholar]
- Strogatz, S.H. Nonlinear Dynamics and Chaos; Westview Press: Cambridge, MA, USA, 1994. [Google Scholar]
- Okumura, M.; Kijima, A.; Kadota, K.; Yokoyama, K.; Suzuki, H.; Yamamoto, Y. A critical interpersonal distance switches between two coordination modes in kendo matches. PLoS ONE 2012, 7, e51877. [Google Scholar] [CrossRef]
- Kijima, A.; Kadota, K.; Yokoyama, K.; Okumura, M.; Suzuki, H.; Schmidt, R.C.; Yamamoto, Y. Switching dynamics in an interpersonal competition brings about ‘Deadlock’ synchronization of players. PLoS ONE 2012, 7, e47911. [Google Scholar] [CrossRef]
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Yokoyama, K.; Okumura, M.; Kijima, A.; Kadota, K.; Gohara, K. Joint action syntax in Japanese martial arts. PLoS ONE 2013, 8, e72436. [Google Scholar] [CrossRef]
- Gohara, K.; Okuyama, A. Dynamical systems excited by temporal inputs: Fractal transition between excited attractors. Fractals 1999, 7, 205–220. [Google Scholar] [CrossRef]
- Gohara, K.; Okuyama, A. Fractal transition: Hierarchical structure and noise effect. Fractals 1999, 7, 313–326. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Gohara, K. Continuous hitting movements modeled from the perspective of dynamical systems with temporal input. Hum. Mov. Sci. 2000, 19, 341–371. [Google Scholar] [CrossRef]
- Nishikawa, J.; Gohara, K. Automata on fractal sets observed in hybrid dynamical systems. Int. J. Bifurc. Chaos 2008, 18, 3665–3678. [Google Scholar] [CrossRef]
- Miller, E.K. The prefrontal cortex and cognitive control. Nat. Rev. Neurosci. 2000, 1, 59–65. [Google Scholar] [CrossRef] [PubMed]
- Sakagami, M.; Tsutsui, K. The hierarchical organization of decision making in the primate prefrontal cortex. Neurosci. Res. 1999, 34, 79–89. [Google Scholar] [CrossRef]
- Ditzinger, T.; Haken, H. Oscillations in the perception of ambiguous patterns. Biol. Cybern. 1989, 61, 279–287. [Google Scholar] [CrossRef]
- Drew, T.; Võ, M.L.H.; Wolfe, J.M. The invisible Gorilla strikes again: sustained inattentional blindness in expert observers. Psychol. Sci. 2013, 24, 1848–1853. [Google Scholar] [CrossRef]
- Gregoriou, G.G.; Gotts, S.J.; Zhou, H.; Desimone, R. High-frequency, long-range coupling between prefrontal and visual cortex during attention. Science 2009, 324, 1207–1210. [Google Scholar] [CrossRef]
- Neisser, U.; Becklen, R. Selective looking: attending to visually specified events. Cogn. Psychol. 1975, 7, 480–494. [Google Scholar] [CrossRef]
- Alder, D.; Ford, P.R.; Causer, J.; Williams, A.M. The coupling between gaze behavior and opponent kinematics during anticipation of badminton shots. Hum. Mov. Sci. 2014, 37, 167–179. [Google Scholar] [CrossRef]
- Vaeyens, R.; Lenoir, M.; Philippaerts, R.M.; Williams, A.M. Mechanisms underpinning successful decision making in skilled youth soccer players: an analysis of visual search behaviors. J. Mot. Behav. 2007, 39, 395–408. [Google Scholar] [CrossRef] [PubMed]
- Williams, A.M.; Ward, P.; Knowles, J.M.; Smeeton, N.J. Anticipation skill in a real-world task: measurement, training, and transfer in tennis. J. Exp. Psychol. Appl. 2002, 8, 259–270. [Google Scholar] [CrossRef]
- Araújo, D.; Davids, K.; Serpa, S. An ecological approach to expertise effects in decision-making in a simulated sailing regatta. Psychol. Sport Exerc. 2005, 6, 671–692. [Google Scholar] [CrossRef]
- Araújo, D.; Davids, K.; Hristovski, R. The ecological dynamics of decision making in sport. Psychol. Sport Exerc. 2006, 7, 653–676. [Google Scholar] [CrossRef]
- Thura, D.; Cos, I.; Trung, J.; Cisek, P. Context-dependent urgency influences speed-accuracy trade-offs in decision making and movement execution. J. Neurosci. 2014, 34, 16442–16454. [Google Scholar] [CrossRef]
- Buzsáki, G. Neural syntax: cell assemblies, synapsembles, and readers. Neuron 2010, 68, 362–385. [Google Scholar] [CrossRef] [PubMed]
- Hirakawa, T.; Suzuki, H.; Okumura, M.; Gohara, K.; Yamamoto, Y. Switching dynamics between two movement patterns varies according to time interval. Int. J. Bifurc. Chaos 2016, 26, 1630026. [Google Scholar] [CrossRef]
- Hirakawa, T.; Suzuki, H.; Gohara, K.; Yamamoto, Y. Inverse proportional relationship between switching-time length and fractal-like structure for continuous tracking movement. Int. J. Bifurc. Chaos 2017, 27, 1730040. [Google Scholar] [CrossRef]
- Suzuki, H.; Yamamoto, Y. Robustness to temporal constraint explains expertise in ball-over-net sports. Hum. Mov. Sci. 2015, 41, 193–206. [Google Scholar] [CrossRef]




© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yamamoto, Y.; Kijima, A.; Okumura, M.; Yokoyama, K.; Gohara, K. A Switching Hybrid Dynamical System: Toward Understanding Complex Interpersonal Behavior. Appl. Sci. 2019, 9, 39. https://doi.org/10.3390/app9010039
Yamamoto Y, Kijima A, Okumura M, Yokoyama K, Gohara K. A Switching Hybrid Dynamical System: Toward Understanding Complex Interpersonal Behavior. Applied Sciences. 2019; 9(1):39. https://doi.org/10.3390/app9010039
Chicago/Turabian StyleYamamoto, Yuji, Akifumi Kijima, Motoki Okumura, Keiko Yokoyama, and Kazutoshi Gohara. 2019. "A Switching Hybrid Dynamical System: Toward Understanding Complex Interpersonal Behavior" Applied Sciences 9, no. 1: 39. https://doi.org/10.3390/app9010039
APA StyleYamamoto, Y., Kijima, A., Okumura, M., Yokoyama, K., & Gohara, K. (2019). A Switching Hybrid Dynamical System: Toward Understanding Complex Interpersonal Behavior. Applied Sciences, 9(1), 39. https://doi.org/10.3390/app9010039



