1. Introduction
Direct numerical simulation of many complex multi-scale problems, such as turbulence in fluids, requires the use of adequately refined grids to resolve the structure and the dynamics of the smallest scales. However, in many situations the limits of available computing power, both in terms of memory requirements and of integration time, force the choice of the grid to a step size which is at (or even over) the edge of the acceptable resolution. In these situations the physics is described within a few computational nodes and the resolution properties of the discretization are a crucial factor. A similar situation is encountered in Large Eddy Simulation (LES) of turbulence. In this case, the smallest scale resolved by the discretization is deliberately greater than the smallest physical scales having significant energy, since the effects of the latter are modelled through the specification of a suitable subgrid model. In this case, the resolution of the discretization (intended as the number of mesh points required for a given accuracy at a given wave length) is even more important, since the numerical errors have to be small as compared to the subgrid energy contribution.
In these and in many other cases, high-order methods are usually recommended, since they generally provide lower levels of numerical errors for the grids employed. In recent years high order implicit compact schemes have been extensively used for flow problems, as an alternative to high order explicit finite-difference schemes and to spectral (or pseudospectral) schemes. After the 1992 seminal paper by S. K. Lele [
1], in which a comprehensive and systematic presentation of compact schemes is reported, they have been extensively used in fluid flow calculations, both in compressible and incompressible cases. Their success is due to several favourable properties which place them in between the flexibility typical of explicit finite difference schemes and the resolution properties of global spectral schemes.
Although the influential paper by Lele is considered as the starting point for the subsequent huge research activity on compact schemes and on their applications, there is a quite interesting production on this subject well before the nineties, whose character is, in some sense, in line with the approaches taken in this paper. Actually, implicit compact derivatives were already used in numerical analysis well before the seventies (cfr. for example [
2]); however, their main application was limited to the finite-difference approximation of ordinary differential equations. The fourth-order implicit Nyström formula, known as Simpson’s rule, can indeed be viewed as an application of the classical Padé scheme to a system of first order ordinary differential equations. The first mention in the open literature of the opportunity of using implicit compact formulæ for the numerical approximation of partial differential equations seems to be due to S. Orzsag and M. Israeli [
3], who attribute to H. O. Kreiss the suggestion. In that paper, they fully recognized the main favorable characteristics of compact schemes, namely the reduced truncation error (with respect to the analogous explicit formulæ) the reduced width of the stencil, which eases the implementation of boundary conditions, and the low computational cost. The idea of using implicit formulæ for the computation of spatial derivatives can be possibly traced back to the finite difference formulation of some finite element methods based on Hermite cubics, which had growing popularity at that time (see the interesting observation made at p. 59 of ref. [
4]).
The authoritative suggestion contained in the review of Orszag and Israeli sparked many studies on the development and test of compact schemes. Almost simultaneously compact schemes were applied with success to the wave equation [
5], to parabolic equations [
6] and to different nonlinear fluid mechanics problems [
7]. In this last paper also the problem of boundary conditions is investigated, and a series of asymmetric formulæ for the boundary is presented. In these early years the focus was not only on the applications of compact schemes, but also on the development of a theoretical framework in which new schemes could be derived. In a series of papers, Rubin and co-workers [
8,
9,
10] firstly estabilished that many compact schemes can be derived by employing the theory of spline interpolation. They showed, for example, that the classical Padé fourth-order formula for first derivative can be obtained by analytically differentiating the cubic spline interpolation, thus recognizing that compact schemes could be derived through a theory of polynomial interpolation in physical space, which has to be necessarily implicit. They also obtained formulæ for second derivatives and for the case of nonuniform mesh, and applied their schemes to a variety of fluid flow problems. Ciment et al. [
11] developed the theory of the “Operator Compact Implicit Method”, in which new classes of compact schemes were derived for parabolic problems by applying the compact technique to the full spatial convection-diffusion operator. Adam [
12], while investigating the problem of boundary condition closures, proposed also a new method for obtaining a second derivative approximation for interior points through the so-called “explicit elimination”, in which the second derivative is expressed as a function of the values and of the computed set of first derivatives, in a way which shares some similarities with the technique adopted in this paper. Finally, Chang and Shirer [
13] introduced for the first time the formulæ for compact interpolation and for compact staggered derivatives in their applications to geostrophic adjustment problem and to the nonlinear two-dimensional vortex-advection problem. In the first application, they also compared the various schemes by computing the discrete dispersion relation of the gravity waves, in an analysis which is practically equivalent to the Fourier analysis of the modified wavenumber, which is now a standard tool for the evaluation of the resolution properties of difference schemes.
The polynomial approach for the derivation of compact schemes has been pursued in further occasions during the past two decades. Chu and Fan [
14,
15] used the theory of Hermite interpolants, based on first and second derivative data, to obtain the expressions for their so-called CCD schemes, in which both first and second derivatives appear as unknowns in the generic scheme. Coppola and Meola [
16] developed a new theory of Local Matched Reconstructions, which generalizes the spline interpolation theory and is more suited to the systematic generation of all the classical collocated compact schemes. In that paper the authors employ this theory also to derive new classes of compact schemes.
In this paper, we present a strategy for the generation of several compact derivatives starting from the computation of a single set of compact schemes. The idea is motivated by the growing interest in the application of compact schemes to Navier-Stokes equations [
17,
18,
19,
20,
21] and is here applied to a typical incompressible Navier-Stokes solver with a staggered arrangement of the variables. The convective term is discretized by using the skew-symmetric form, which is a mandatory choice for energy-stable simulations in the context of compact-difference schemes. In such a situation, the computation of convective and diffusive terms in the the momentum equation requires the evaluation of staggered derivatives and interpolations of velocity components, together with the collocated second derivative. It will be shown that an efficient and economic way of obtaining all these quantities can be devised, which is based on the computation of a single set of compact derivatives, i.e., through the solution of a single tridiagonal system.
The paper is organized as follows. In
Section 2 the Hermitian interpolation theory is presented for fourth-order schemes, and then extended to high-order methods. The characteristics of the novel schemes are analyzed in
Section 3, while in
Section 4 the implementation into an incompressible Navier-Stokes algorithm is discussed.
Section 5 presents numerical results. Concluding remarks are given in
Section 6.
2. Problem Formulation and Derivation of New Compact Schemes
In this section, we present the procedure for obtaining the whole set of compact formulæ needed in a typical staggered Navier-Stokes solver, starting from the computation of a single set of collocated compact derivatives. We will firstly describe how to construct the formulæby employing the classical fourth-order Padé scheme as a starting point. The extension to higher-order schemes is straightforward and will be presented in
Section 2.4. Moreover, the procedure will be illustrated with reference to a uniform mesh; the extension to non-uniform meshes is also in principle straightforward, but will be not considered here. Hereinafter, when referring to derivatives, we will use the terms
collocated and
staggered to denote that the derivatives are computed in the same location, or shifted with respect to the original data set respectively.
Let us assume that the dependent variable
is discretized on an uniform mesh
with
and
. For interior points, the classical fourth-order Padé scheme reads:
with truncation error
. This equation is a typical choice for high-order compact approximations of the first derivative on a collocated mesh. The set of nodal derivatives is obtained by solving a tridiagonal linear system, for which standard highly-efficient methods are available. The computational cost associated to this scheme, for a system of
N equations, can be roughly estimated to be
floating point operations. This value is obtained by summing the cost of the computation of the right-hand side (
) to that of a standard Thomas solver for the tridiagonal system (
,
22]). Being a central method, the Padé scheme is free of numerical dissipation when employed in linear problems with periodic boundary conditions. In the non-periodic case it requires the specification of one non-symmetric scheme for each boundary node, in the case in which the dependent variable naturally falls on the boundary. In a typical staggered Navier-Stokes solver, however, this scheme cannot be used, since the quantities known at nodes
have to be either interpolated or differentiated at the staggered locations
. For this problem, the analogous staggered fourth order compact scheme is given by:
with truncation error
. Please note that in Equations (
1) and (
2), as well as in all the other classical compact formulæ considered in the following, the convention that the left-hand side coefficients sum to one is assumed. This convention is alternative to the common choice for which the coefficient of the central term (
or
) equals to one; it has been adopted with the aim of obtaining a fair comparison of the truncation errors among the various compact and explicit formulæ considered in this work. Equation (
2) is again a centred non-dissipative scheme. Again, one non-symmetric scheme is required for each boundary node when the dependent variable falls on the boundary. The computational cost associated to this scheme can be again estimated to be
operations.
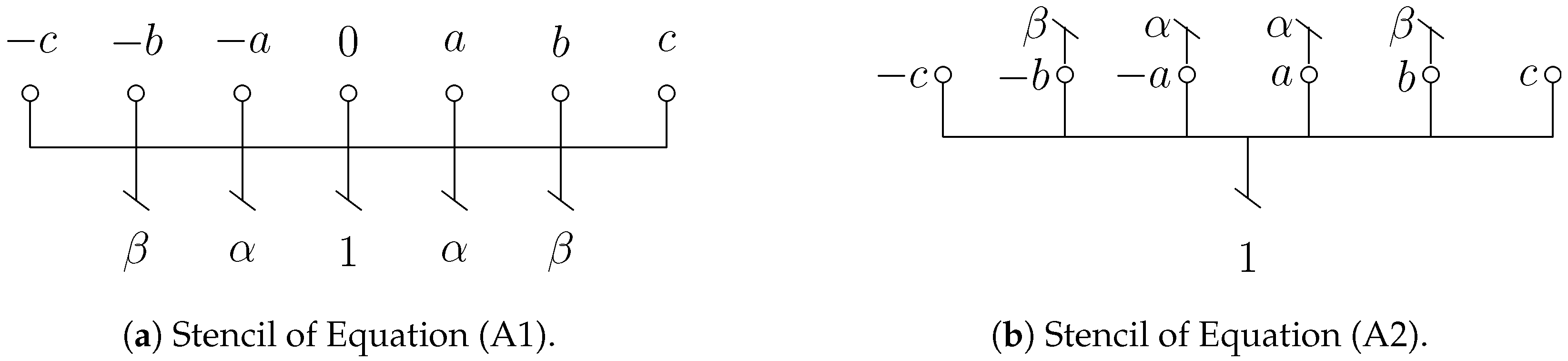
In what follows, each scheme will be referred to by a short acronym of the form
n1L1L2-D
n2, where
is an integer equal to the formal order of accuracy of the scheme,
is either C or S for collocated and staggered schemes respectively,
is E, C, or H for explicit, compact, and proposed Hermitian schemes, and
is the order of derivation of the left-hand side of the scheme (0 for interpolation schemes). For instance, Equations (
1) and (
2) are referred to by the acronyms 4CC-D1 and 4SC-D1 respectively. Moreover, it will be useful to refer to a graphical representation of the various numerical schemes that will be introduced; each variable appearing in the finite-difference formula is depicted with a different symbol, depending on its order of derivation, and in a different position with respect to the stencil, depending on its role in the scheme. The explicit data
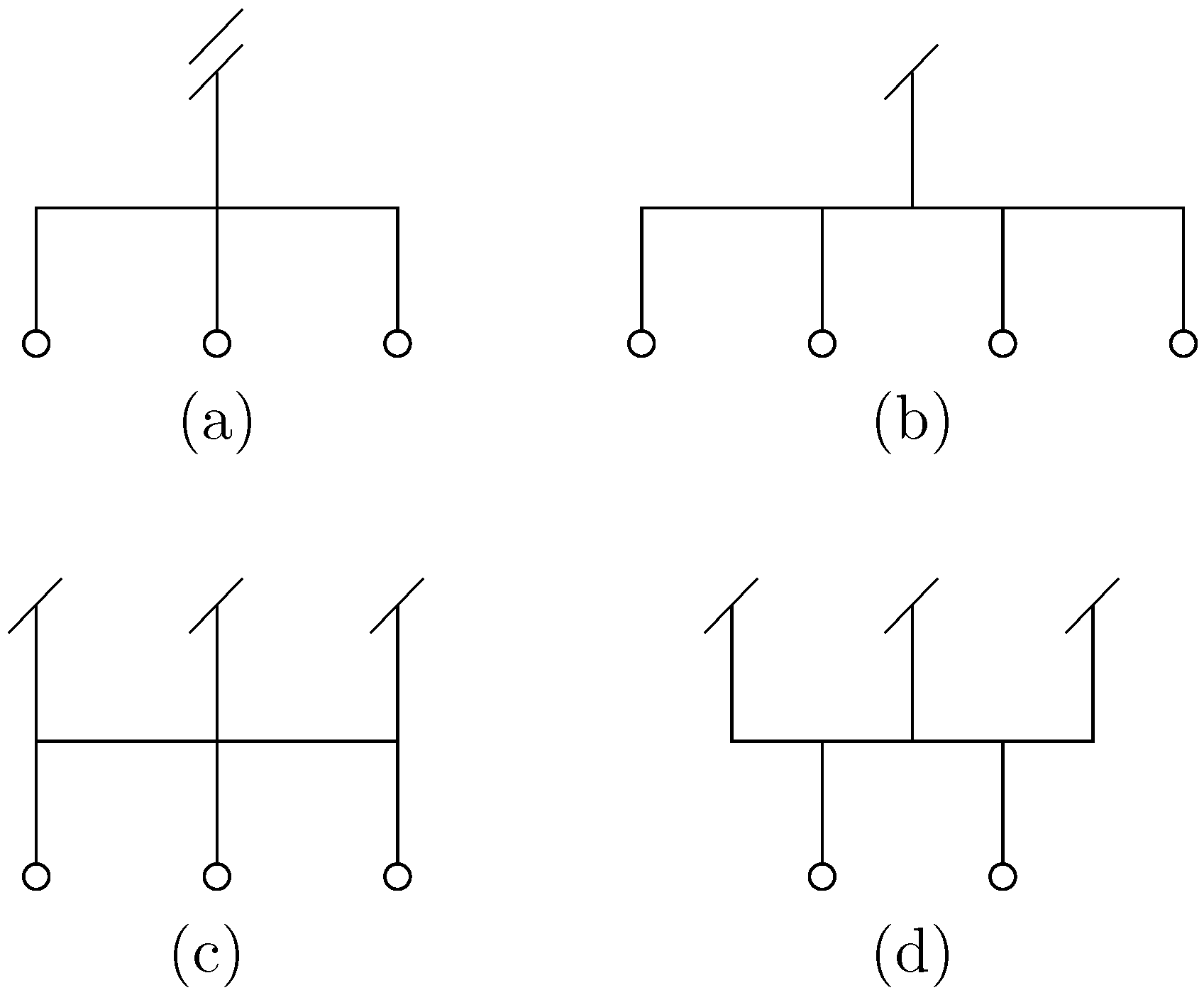
are depicted as small circles, while first and successive derivatives are denoted by a corresponding number of inclined dashes. If a variable is a known quantity in the formula, its position in the graphical representation falls within the lower part, below the horizontal line representing the mesh. If it is an unknown, it is positioned above the mesh, in the upper part of the sketch.
In
Figure 1, some examples are reported for different classical schemes, both explicit and compact. The weights appearing in the numerical formula can be also reported in the sketch as associated to each variable. When such weights are omitted, it is assumed that the drawing represents the (unique) maximum-order scheme attainable on that stencil.
Figure 1a represents the classical second-order explicit scheme for the second derivative, while
Figure 1b depicts the staggered explicit fourth-order scheme (4SE-D1) given by:
The procedure proposed in this paper is based on the observation that the Padé scheme here considered, Equation (
1), can be obtained (as virtually all compact schemes) by evaluating the analytical derivative at the node
of a local polynomial reconstruction
for the unknown function
f, as it is done for explicit finite difference formulæ. In the case of compact schemes, the implicit character of the relation between the set of grid nodes derivatives and the nodal values
, suggests that local polynomial representations of the unknown function
f relative to adjacent nodes have to be linked by implicit relations. This representation is in general not unique. It is well known, for instance, that in the particular case of the Padé scheme, the classical third-degree cubic spline gives a consistent interpolation, in the sense that its analytical first derivative at node
returns Equation (
1) [
10]. In what follows, we will consider a different set of local interpolations which is consistent with the compact scheme in Equation (
1). This set is given by the class of polynomials
, of degree four, determined by imposing the explicit conditions
together with the implicit conditions
It is easy to verify that the derivative at node
of the local polynomial expansion
, which can be assumed to be defined on the interval
, furnishes again Equation (
1). This approach was exploited in [
16] in order to give an alternative derivation of many of the classical compact formulæ. New interpolation procedures were also investigated in that paper, based on the enforcement of more general implicit conditions. The theory developed therein generalizes the classical spline interpolation, and led to new classes of compact–type schemes.
In this paper, we adopt a slightly different standpoint, in which the implicit polynomial approach is not employed as a tool to justify the derivation of Equation (
1), or of similar collocated formulæ. Here, the set of local polynomial approximations
consistent with Equation (
1) is used as the basis for the calculation of all the compact approximations needed in a staggered Navier-Stokes algorithm, which is typically constituted by staggered interpolations and first derivatives, and by collocated second derivatives.
The polynomials
determined by the conditions of Equations (
4)–(
6) can be conveniently obtained by performing an Hermitian interpolation of the grid values
and of the derivatives
, once these last ones have been obtained by solving the tridiagonal system associated to Equation (
1). Of course, this last procedure is equivalent to the one outlined above, in which the explicit and implicit conditions in Equations (
4)–(
6) for the whole set of local polynomials
are enforced. Please note that in the present procedure we assume that the values
are assigned, while the compact scheme in Equation (
1) is used to get a larger number of information, which is then exploited to feed the higher-degree Hermitian interpolation. The computation of the polynomial functions
would allow one to have a complete local approximation of the function
f in the interval
. Any information on the interpolated values, or on first or higher-order derivatives, at any point near
can be obtained analytically by evaluating
or its derivatives at the selected point. The evaluation of
and of its first derivative at staggered points and the evaluation of
at grid points, leads to new compact-type formulæ, which can be obtained explicitly, once the single compact scheme in Equation (
1) has been computed.
2.1. Schemes for Staggered First Derivative
The numerical formulæ for the first derivatives
, at staggered grid points
, based on the Hermite polynomial
, can be directly evaluated as a linear combination of nodal values
and nodal first derivatives
for
. Since the six values
and
, are related by Equation (
1), only five of them can be chosen independently for the construction of the the fourth-degree polynomial
. Although any choice of five data among them is equivalent, for what concerns the evaluation of first derivative at the staggered point
, the selection of the values
and
is particularly convenient, since for this set of data the coefficient of the value
results to be zero. This is a consequence of the symmetry of the problem: the first derivative at
of the third-degree polynomial fitting the data
and
is coincident with the derivative at the same point of the whole family of fourth-degree polynomials fitting the same data. This is similar to what happens for a linear interpolation between two points
and
, which shares the same first derivative at
with the whole family of parabolas that fit the same data.
For uniform meshes, the formula to compute the staggered first derivative based on collocated data and first derivatives (4SH-D1, H standing for
Hermitian) assumes the simple form:
The combination of Equations (
1) and (
7) gives a fourth-order scheme for the staggered first derivative at interior points with global truncation error
. The standard reference for comparison for this scheme is the staggered fourth order Padé scheme of Equation (
2), which has a comparable truncation error, while the explicit scheme of Equation (
3) has truncation error
. For non-periodic boundary conditions, Equation (
1) has to be replaced by suitable non-symmetric compact formulæ at the boundary nodes, while Equation (
7) remains unaltered near boundaries. The computational cost of Equation (
7) can be estimated to be
. The graphical sketch of the proposed scheme, which is built upon the successive application of Equations (
1) and (
7), is given in
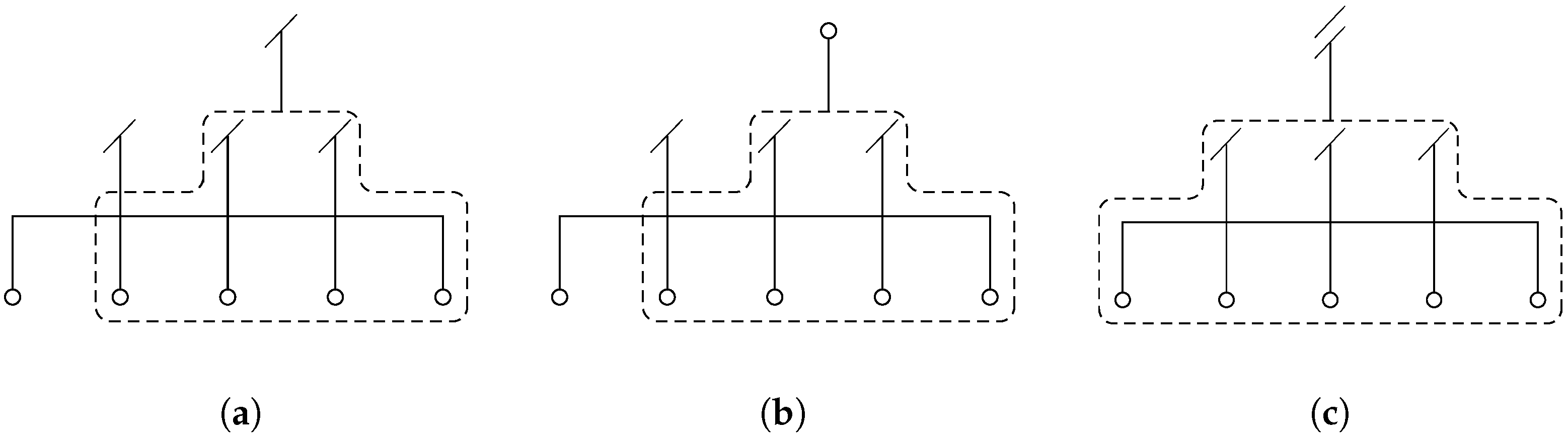
Figure 2a.
A quick inspection of Equations (
1) and (
7) reveals that both express essentially the same relation between the five values in the sets
and
respectively, the only difference being the spacing (
h in the former,
in the latter). Nonetheless, the two schemes differ in the crucial choice of known and unknown terms in the aforementioned sets, thus the two corresponding equations are presented separately ([
19], cf. Equations (20) and (21)). We take the opportunity to stress that any finite difference formula can be obtained by requesting that a linear combination of the desired nodal values—of derivatives of various orders—be proportional to
, being
p the minimum order of accuracy of the scheme. This approach is the most general and easily allows the computation of the coefficients used in several class of unconventional schemes [
14,
15,
23].
2.2. Schemes for Interpolation
The interpolation scheme can be obtained with a procedure similar to that described for staggered first derivative. The evaluation of the local Hermitian polynomial
at node
gives a consistent approximation of the function value, which is based on a fourth-degree polynomial. However, in this case the procedure produces a non-symmetric formula, which causes the interpolation scheme to be dissipative. To avoid this circumstance, one can derive a symmetric scheme either by taking the arithmetic mean of the two interpolation formulæ given by the polinomials
and
at node
, or by evaluating the interpolation
based on the reduced set of values
. The two approaches give respectively the schemes 6SH-D0 and 4SH-D0 (D0 stands for
interpolation):
with global truncation errors
and
, when Equation (
1) is used for first derivatives at the right hand side of Equations (
8) and (
9). In the case of interpolation, the third-degree polynomial based on the data
furnishes a fourth-order approximation of the function
f at any point interior to the interval
. This suggests that Equation (
9), which gives a fourth-order formula for
, is to be preferred to Equation (
8) for consistency to other fourth-order approximations, and will be the baseline choice in the following. Equation (
9) has a computational cost of
operations and its graphical representation is given in
Figure 2b. The reference comparison formula is the classical fourth-order compact interpolation scheme, 4SC-D0,
which has truncation error
.
2.3. Schemes for Second Derivative
The evaluation of the second derivative of
at node
leads to the 4CH-D2 scheme:
which was already derived by Adam [
12]. The combination of Equations (
1) and (
11) is again a centred fourth-order formula with truncation error
. The graphical sketch of the proposed scheme, which is built upon the successive application of Equations (
1) and (
11), is given in
Figure 2c. The computation of the explicit scheme in Equation (
11) requires
operations. The reference fourth-order scheme for collocated second derivative, 4CC-D2 is:
with truncation error
.
2.4. Higher-Order Schemes
Higher-order schemes can be obtained in a similar way as has been done for the fourth-order case by considering higher-degree polynomial interpolations. The starting set of data has to be larger and, in order to save accuracy, derivatives employed in the Hermite interpolation have to be calculated with higher precision. By limiting ourselves to tridiagonal systems, sixth- and eighth-order basic set of derivatives can be computed through the schemes 6CC-D1 and 8CC-D1 (extensions of the classical Padé scheme, 4CC-D1),
with truncation errors
and (1/17,640)
h8fIX respectively.
The numerical scheme for the staggered first derivatives
, can be evaluated as a linear combination of nodal first derivatives
for
and of nodal values
with
, in the case of the sixth-order scheme 6SH-D1, and with
in the case of the eighth-order scheme 8SH-D1. For a uniform mesh these formulæ read
The combination of Equations (
13) and (
15) gives a sixth-order scheme for the staggered first derivative at interior points, while the combination of Equations (
14) and (
16) gives an eighth-order scheme. The references for comparison are the classical sixth- and eighth- order staggered compact schemes, 6SC-D1 and 8SC-D1:
The same approach can be used to determine new schemes for interpolants and second derivatives, which are based on collocated schemes for first derivatives. Specifically, the sixth-order scheme for Hermitian interpolation, 6SH-D0, has been already presented in Equation (
8) whereas the eighth-order one, 8SH-D0, is given by the following formula,
Equations (
8) and (
19) can be combined with Equations (
13) and (
14), thus producing a sixth- and a eighth-order scheme for the interpolant at interior points. The corresponding classic schemes for compact interpolation are 6SC-D0 and 8SC-D0,
Similarly, sixth- and eighth-order schemes 6CH-D2 and 8CH-D2 can be obtained by combining the Hermitian formulæ
with Equations (
13) and (
14), respectively. The references for comparison are the following classic formulæ (6CC-D2 and 8CC-D2),
In
Figure 3, the graphical representation of the new schemes of Equation (
15), Equations (
8) and (
22) are reported.
4. Application to Incompressible Navier-Stokes Equations
The motion of an incompressible fluid is governed by the Navier-Stokes equations,
where
P is the pressure, Re is the Reynolds number,
are the nondimensional cartesian components of the velocity field, and
the components of any given velocity source/sink field. Discretization of Equations (
35) and (
36) follows here a standard semi-discrete approach [
26,
27,
28], in which the momentum equation is discretized in space and then advanced in time without taking the pressure term into account; subsequently, a Poisson equation is solved for the pressure; finally the divergence-free velocity at the following time level is obtained by correcting with the gradient of pressure.
In Equation (
35) the nonlinear convective term is expressed in the so-called
advective form. By employing the continuity Equation (
36), it can be equivalently expressed in
divergence form (i.e., as the divergence of the dyadic tensor
), or in
skew-symmetric form, in which the arithmetic mean of divergence and advective forms is considered. This distinction has little importance on the continuous level, once all the analytical manipulations of standard calculus are assumed to be valid. However, the direct discretization of each form behave usually differently, since the standard rules of calculus (even the simplest ones, as the product rule) are in general not guaranteed to be valid for discrete operators.
The choice of the form in which the convective term is discretized is usually dictated by the behaviour of the discrete set of equations with respect to induced balance equations. The preservation of some global quadratic invariants, such as, for instance, kinetic energy integrated over the whole domain (in the inviscid limit and for periodic or homogeneous flux boundary conditions), is an important target for stable and reliable simulations at high Reynolds numbers [
29]. Different strategies have been developed in order to fulfill this requirement, some of which are briefly recalled in the following section. A more in-depth discussion on this and related topics can be found in [
30].
4.1. Spatial Discretization
Equations (
35) and (
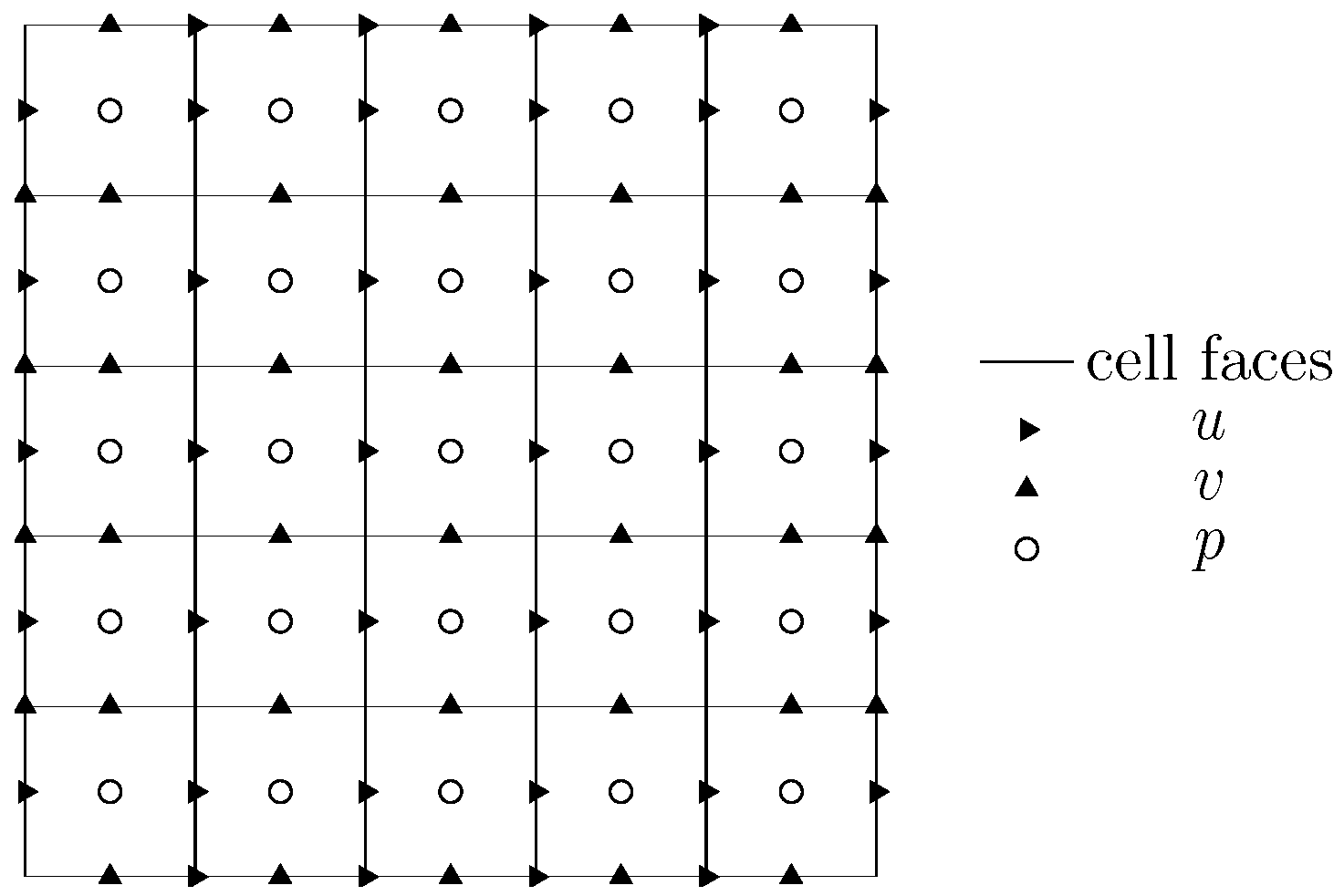
36) are firstly discretized on a cartesian spatial mesh, which will be here assumed to be uniform. As it is very common in the numerical discretization of incompressible Navier-Stokes equations, the variables are arranged on a staggered layout, in which velocity components are located on the normal faces of the cells and pressure nodes are positioned at the centers (
Figure 5). The staggered arrangement of the variables has been often preferred to the simpler collocated or regular arrangements for incompressible flows, because it provides a more robust link between the discrete variables and avoids the odd-even decoupling of the pressure (also known as pressure checker-boarding problem) [
26]. Moreover, it is well known that discrete operators based on explicit and symmetric central differences can easily provide primary (i.e., on mass and momentum) and secondary (e.g., on global kinetic energy) conservation on staggered grids [
29]. This is an important topic, since a spatial discretization which is able to enforce conservation of global kinetic energy on a discrete level usually permits to avoid the onset of nonlinear instabilities arising from the accumulation of aliasing errors associated to the discrete evaluation of nonlinear terms [
31,
32]. The well known second order Harlow-Welch discretization procedure is the simplest example of staggered discretization globally conserving kinetic energy. Moreover, it directly discretizes the
divergence form of the convective term, which has several advantages, in terms of local primary conservation properties and reduced computational cost.
The Harlow-Welch procedure has been extended also to higher order central explicit schemes, both on uniform and variable meshes [
29,
33]. When dealing with implicit (central) compact schemes, however, it is not clear if the discretization of the nonlinear convective term in Equation (
35) can be performed directly on the divergence form and still retaining the global conservation of kinetic energy at the discrete level. In this case, the employment of the so-called
skew-symmetric splitting is mandatory, as in the case of regular layouts, since it automatically guarantees conservation of global kinetic energy on a semi-discrete level [
29]. Recently, other approaches have been proposed, in which the beneficial properties of the skew-symmetric splitting are obtained by properly alternating the more economic divergence and advective forms inside the stages of a multistage time integration procedure [
34,
35,
36]. In this paper, we will not consider these more sophisticated techniques, and will instead rely on the classical skew-symmetric form for the convective term.
One-dimensional derivatives of zeroth, first, and second order are used to discretize spatial operators involved in the computation of the non-linear convective as well as linear viscous terms in Equation (
35). While the latter does not require any special attention, the former does, due to its non linearity. On a discrete level, the skew-symmetric form leads a discretization of the
i-component of the convective term in momentum equation Equation (
35) of the form (cfr. Equations (87) and (89) of Morinishi et al. [
29]):
where
is the finite difference derivative in direction
and overbars denote finite difference iterpolations. In our case both differentiations and interpolations are performed by compact formulæ.
Hence, on a fully staggered arrangement of variables, the two addends in (
37) are calculated by the following combination of interpolations and differentiantions:
Divergence form
- 1
components i and j of velocity are iterpolated in the direction j and i respectively;
- 2
the product is performed;
- 3
the staggered derivative of the product in direction j is computed.
Advective form
- 1
components i and j are stagger-differentiated and iterpolated in the directions j and i respectively;
- 2
the product is performed;
- 3
the product is interpolated along direction j.
As regards both point 1 and 2 it is clear that “pure” products are performed at cell centers, whereas mixed products at cell corners (cell edges in 3D). The same holds for the advective form. From
Figure 5 one sees that this procedure approximates the convective term on the locations of the variables
. Please note that the interpolated quantities used to compute the divergence form (step 1) can be reused in the computation of the advective form (step 2).
The divergence and gradient operators required in the pressure correction process (cf.
Section 4.2) are built based on one-dimensional derivatives as well. Please note that for consistency, the Laplacian operator comes as a consequence of the discrete product
. The main and essential difference with respect to the choice made for the convective and viscous terms is that in this paper the one-dimensional derivatives are explicit, instead of compact, with the same order of accuracy. The reason is that compact schemes would have led to a full matrix discretizing the Laplacian operator, which would have been impractical [
37]. Please note that this approach, despite the stencil of explicit schemes being wider, only requires one non-symmetric boundary scheme (in the case of 4th order of accuracy) for the application of both divergence and gradient operators.
4.2. Time Integration
Time advancement is accomplished through an explicit Runge-Kutta method. Althoug after spatial discretization the Navier-Stokes equations reduce to a system of ordinary Differential Algebraic Equations, the introduction of a projection operator for the velocity field onto the divergence free subspace, formally conducts to a system of Ordinary Differential Equations, to which standard time integrators can be applied. In this context, the projection step has to be executed at each stage of the RK [
38]. By employing a standard notation, the time integration procedure with reference to a generic
s-stage explicit Runge-Kutta method can be expressed as:
where
and
are the vectors containing the discretized velocity and pressure fields on the three-dimensional grid,
is the right hand side of momentum equation, without the pressure term, once discretized in space and ∇ is the discrete nabla operator, assuming the meaning of discrete divergence or gradient operator, depending on the context. This classical approach of projecting each intermediate field in the context of an explicit RK method (cf. Equation (38)) is quite costly with respect to multisteps methods, since each projection requires the solution of an elliptic equation. More convenient strategies which make use of an extrapolation of the pseudo-pressure and perform only the final projection (
39) are possible [
39,
40].
6. Conclusions
In the context of finite difference approximation of Navier-Stokes equations, staggered grid arrangements are usually employed, because they possess several favourable properties due to a more robust coupling between the discrete sets of variables. Moreover, when dealing with high Reynolds number flows, or in any case in which one is forced to a step size that is at the edge of (or even over) the acceptable resolution, high order and in particular compact schemes are possibly preferred for their improved resolving capabilities. Finally, in these circumstances the discrete approximation of the nonlinear convective term has to be performed by resorting to the so-called skew-symmetric form, in which two different expressions of the convective term are calculated and then averaged. This choice is practically unavoidable if one wants to prevent the nonlinear instability due to the accumulation of aliasing errors.
These considerations show that staggered Navier-Stokes solvers based on compact schemes have several issues. They must compute different interpolations and staggered first derivatives of the velocity field, as well as collocated second derivatives for the approximation of the viscous term. The number of derivatives and interpolations required for each computational node is increased with respect to standard formulations, and each of these approximations needs different closure schemes near the boundaries.
In this paper, a novel procedure to efficiently deal with these difficulties is presented. In this method, a whole set of different compact derivatives and interpolations is computed by explicit formulæ resting on a single preliminarly computed compact approximation. Although a complete optimization of the application of the general theory to an incompressible Navier-Stokes solver has not been attempted, it has been shown that the procedure is cost effective and that conducts to novel compact schemes with interesting resolution properties. Moreover, it simplifies the treatment of boundary conditions, since only one boundary closure is required for the whole set of approximations.
It is interesting to stress that although this paper has focused on the staggered discretization of incompressible flows, the proposed new method can be in principle equally applied to compressible flows. Numerical discretization of compressible flows has been usually carried out by considering regular or collocated arrangements of the variables. Staggered layouts have been considered as unnecessary, because of the absence of spurious modes for the pressure and in light of an increased programming difficulty. However, recently staggered discretizations have been proposed also for compressible flows, with the aim of alleviating the inherent instability of the simulations at high Reynolds numbers [
17,
45]. In such situations compact schemes and skew-symmetric form of nonlinear convective terms are adopted, and the method here presented applies with slight modifications also to these situations.
As a final comment, it is worth noting that, besides the application here proposed to staggered Navier-Stokes solvers, the theory developed is believed to have a remarkable interest by itself. It estabilishes that sets of compact approximations can be obtained by explicit evaluations based on the computation of a single compact scheme. By resorting to a theory of implicit interpolation, it reveals that a single set of compact derivatives can be associated to a wider family of schemes, whose computation can be efficiently done by explicit formulæ. In some sense, the procedure illustrated can be seen as a prefactorization of a whole family of schemes involving a single basic implict formula and different explicit evaluations.