SAR Interferogram Filtering of Shearlet Domain Based on Interferometric Phase Statistics
Abstract
:1. Introduction
2. Phase Filtering of Shearlet Domain Based on Interferometric Phase Statistics
2.1. Interferometric Phase Statistics
2.2. Phase Model
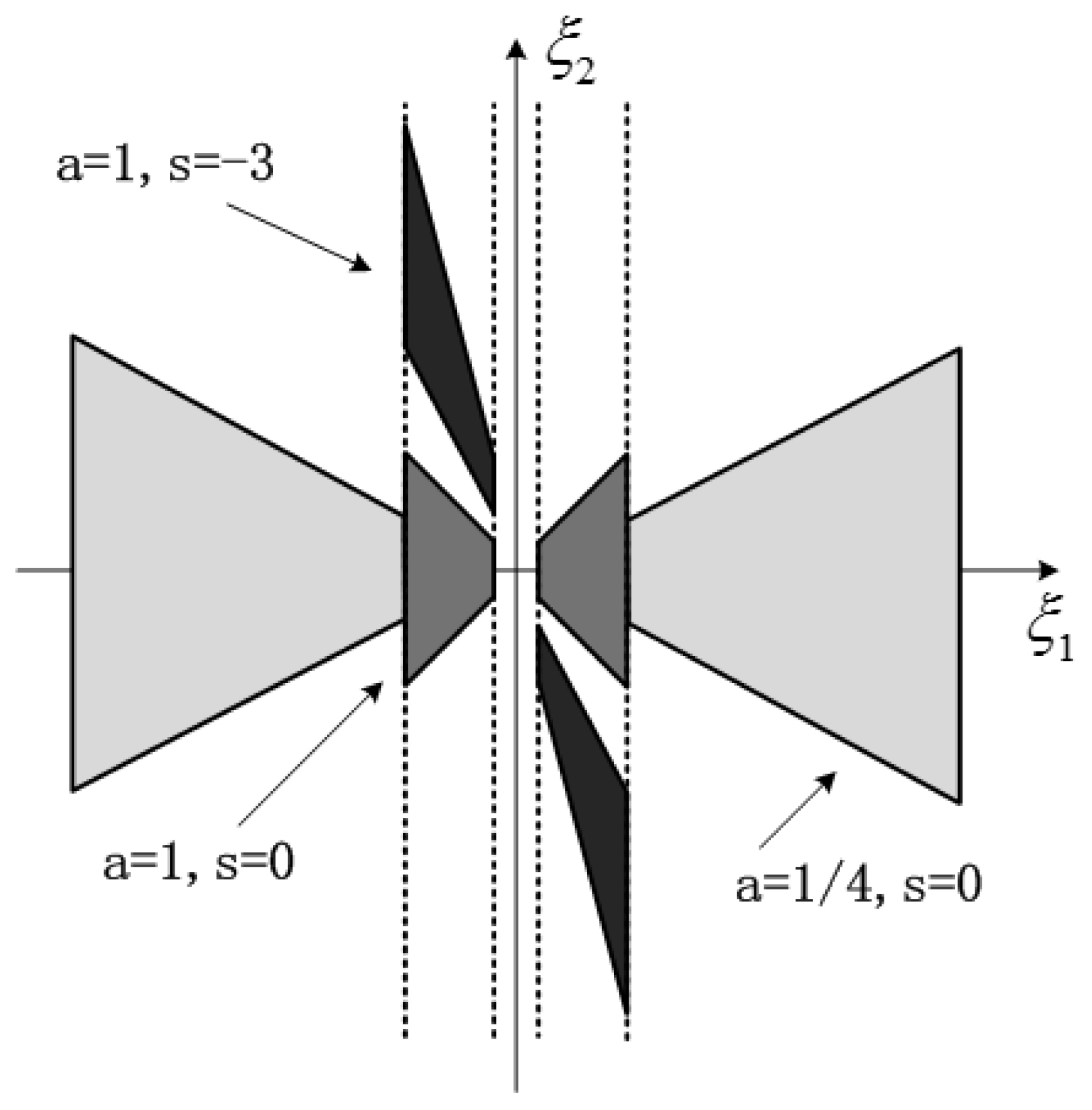
2.3. Interferometric Phase Shearlet Transform
- are well localized;
- have parabolic scaling;
- are highly directionally sensitive;
- are spatially localized;
- and are optimally sparse.
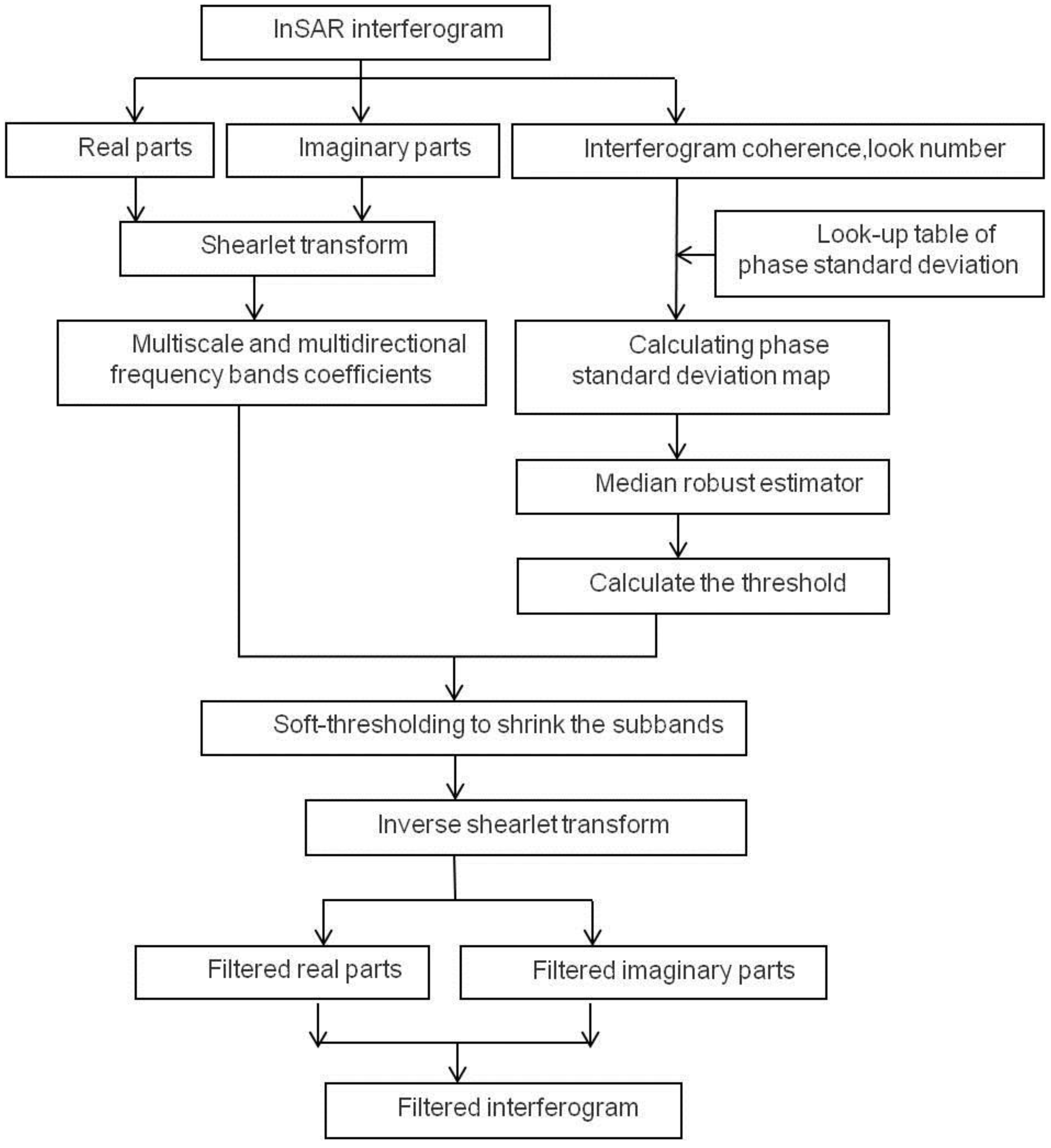
2.4. The Proposed Filter
- (1)
- The real and imaginary parts of InSAR interferogram are processed by the shearlet transform, which are then decomposed into multiscale and multidirectional frequency bands , where is scale index, is direction index.
- (2)
- The threshold is then determined by the following procedure.
- Create a phase standard deviation look-up table between different coherence and multi-look number, as shown in Table 1.
- For a given interferogram, the standard deviation map can be generated pixel by pixel using the look-up table of the coherence and multi-look.
- Median robust estimator
- Calculate the threshold , for . denotes the average energy distribution of white noise in the shearlet coefficient on scale in direction . is the standard deviation defined by the proposed method.
- (3)
- After the new shrinkage threshold of every shearlet coefficient is obtained, we use the soft-thresholding formulation to shrink the shearlet coefficients.
- (4)
- Then the real and imaginary parts of the shearlet coefficients are processed by the inverse shearlet transform and the filtered real and imaginary parts are combined to get the filtered interferogram.
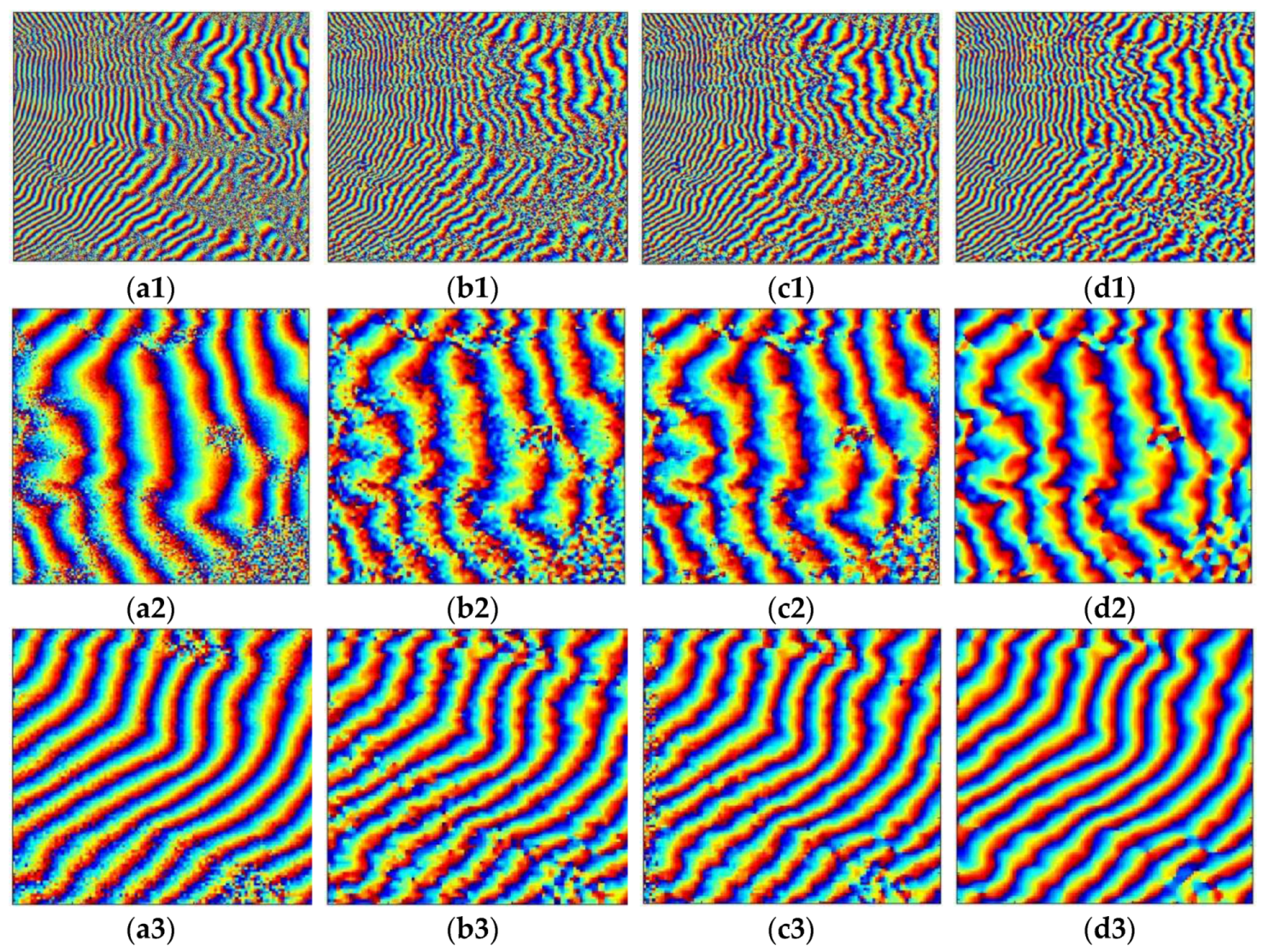
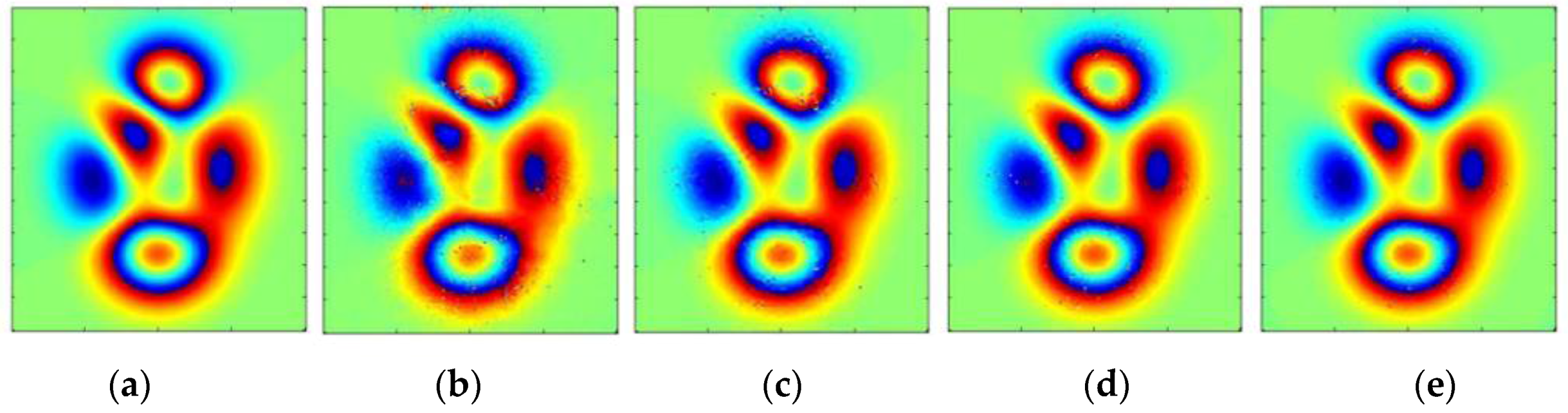
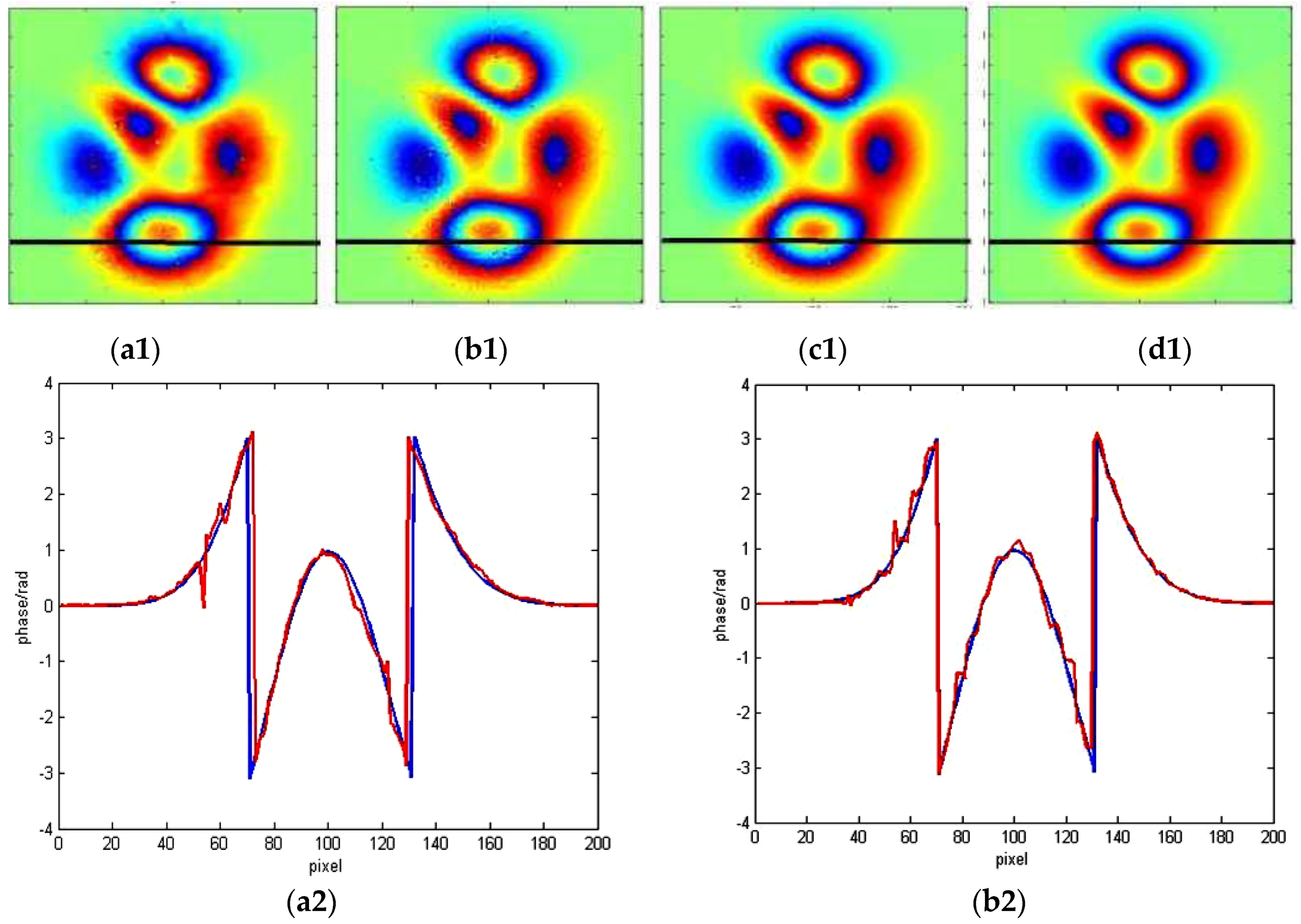
3. Validation with Simulation Data
4. Validation with Real Data
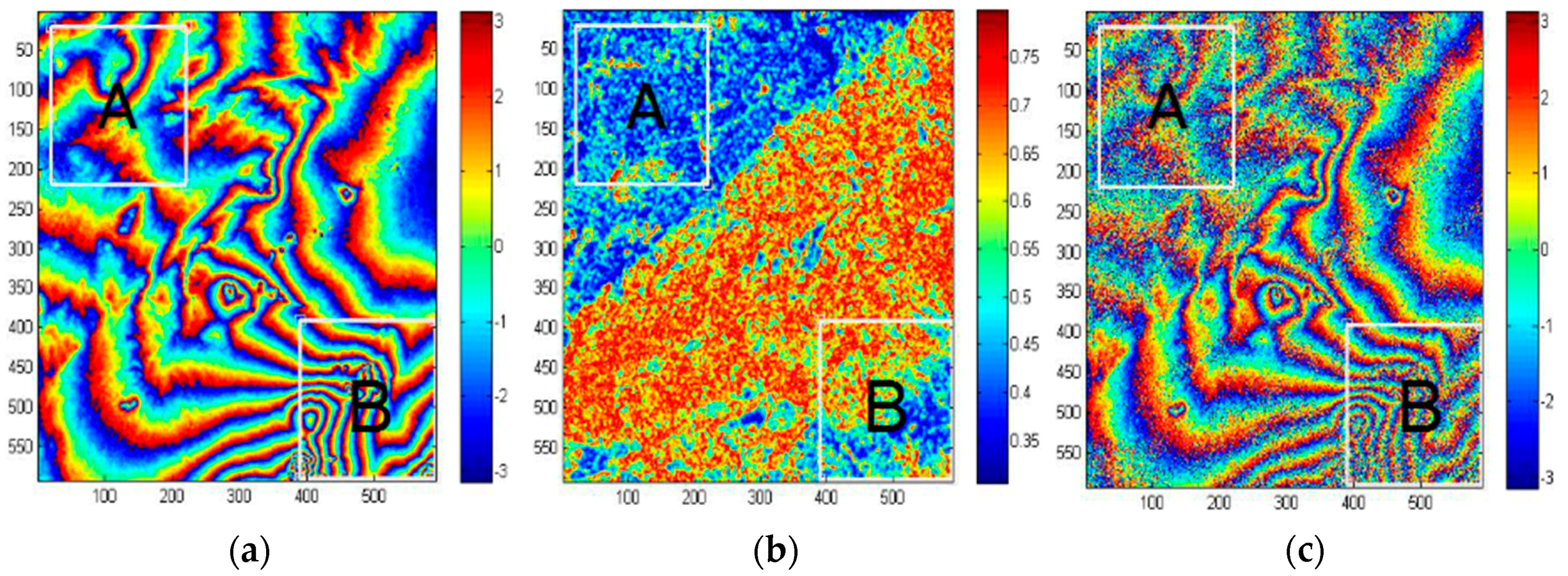
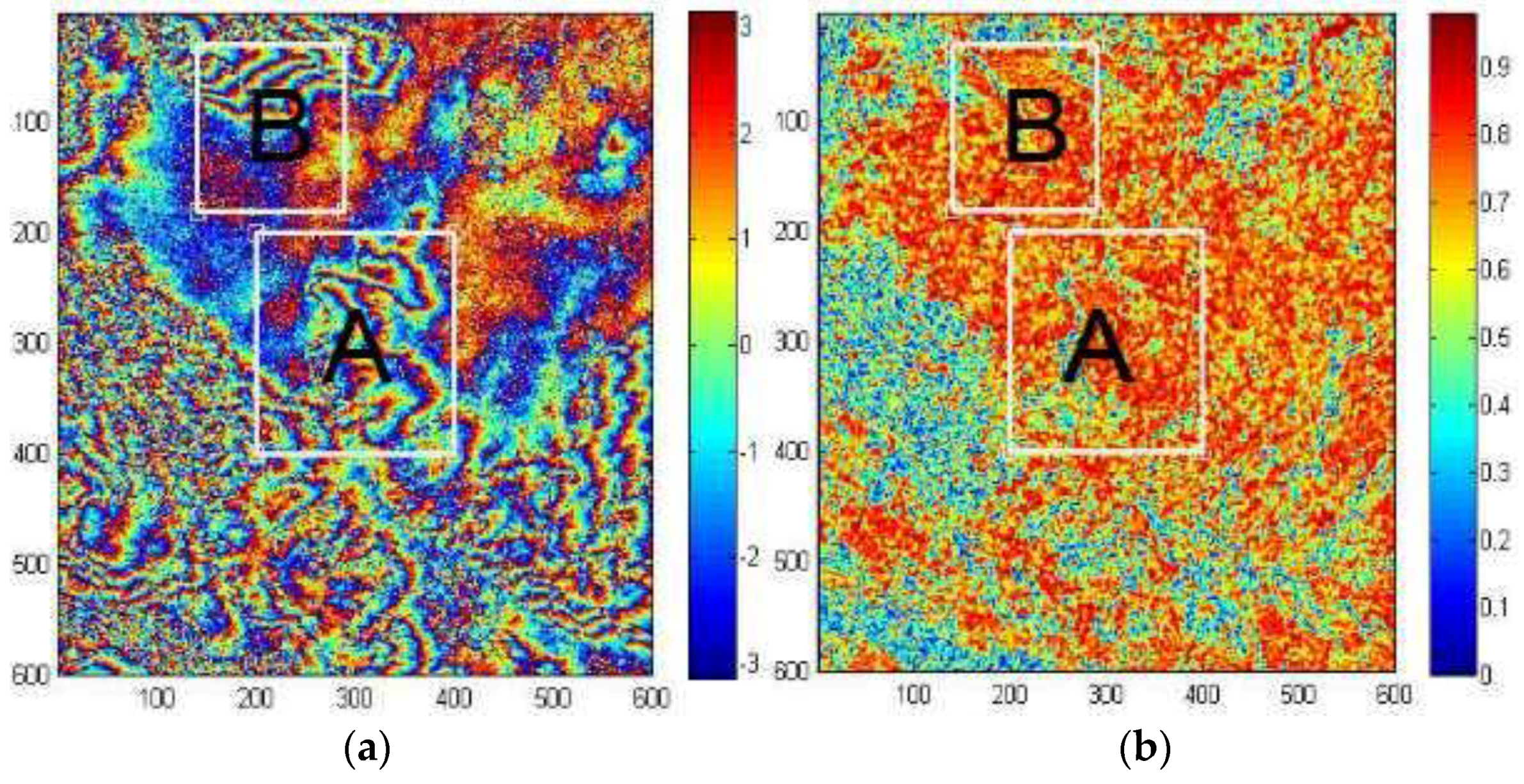
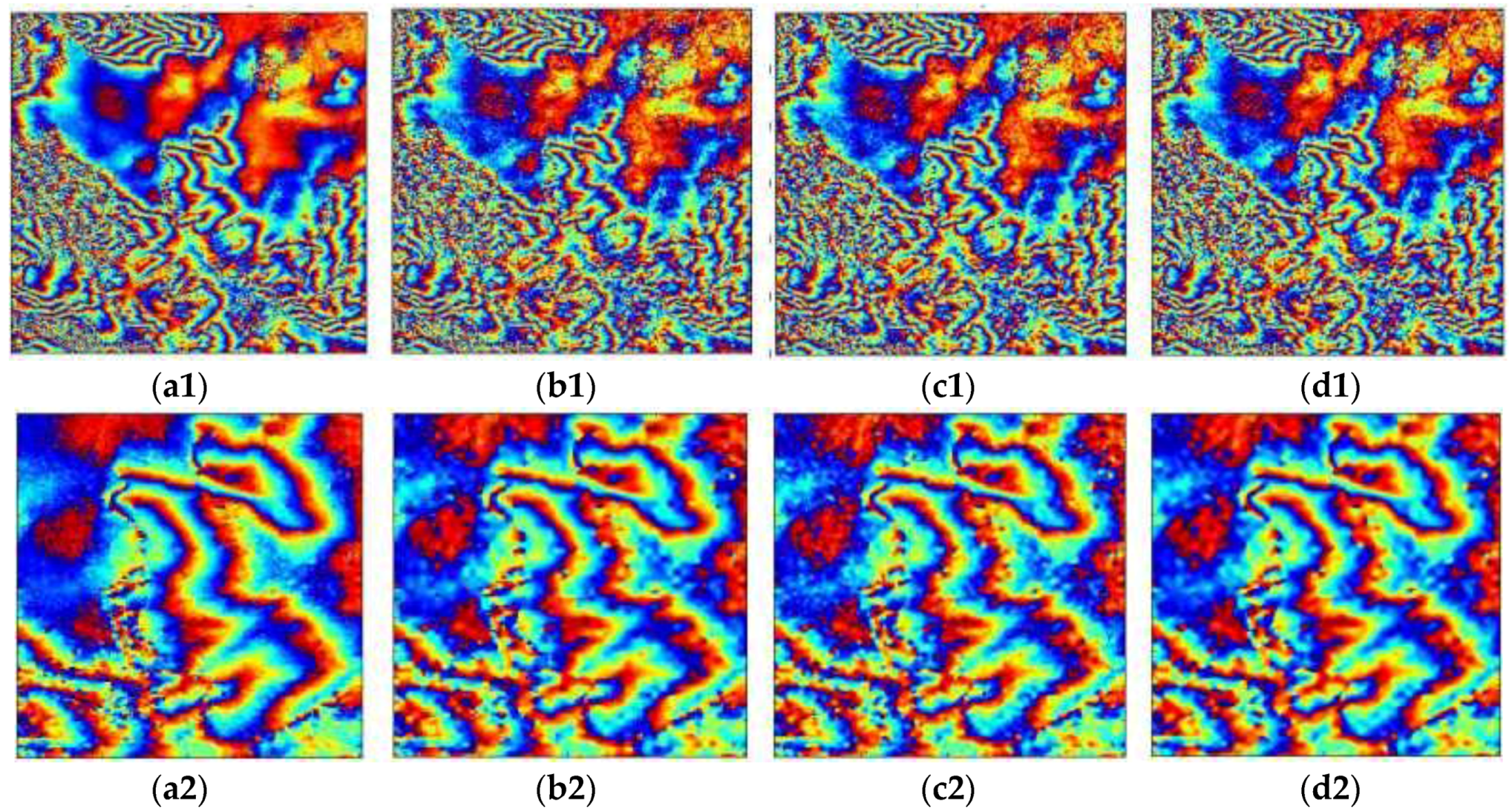
4.1. Validation with the Interferogram over the Etna Volcano
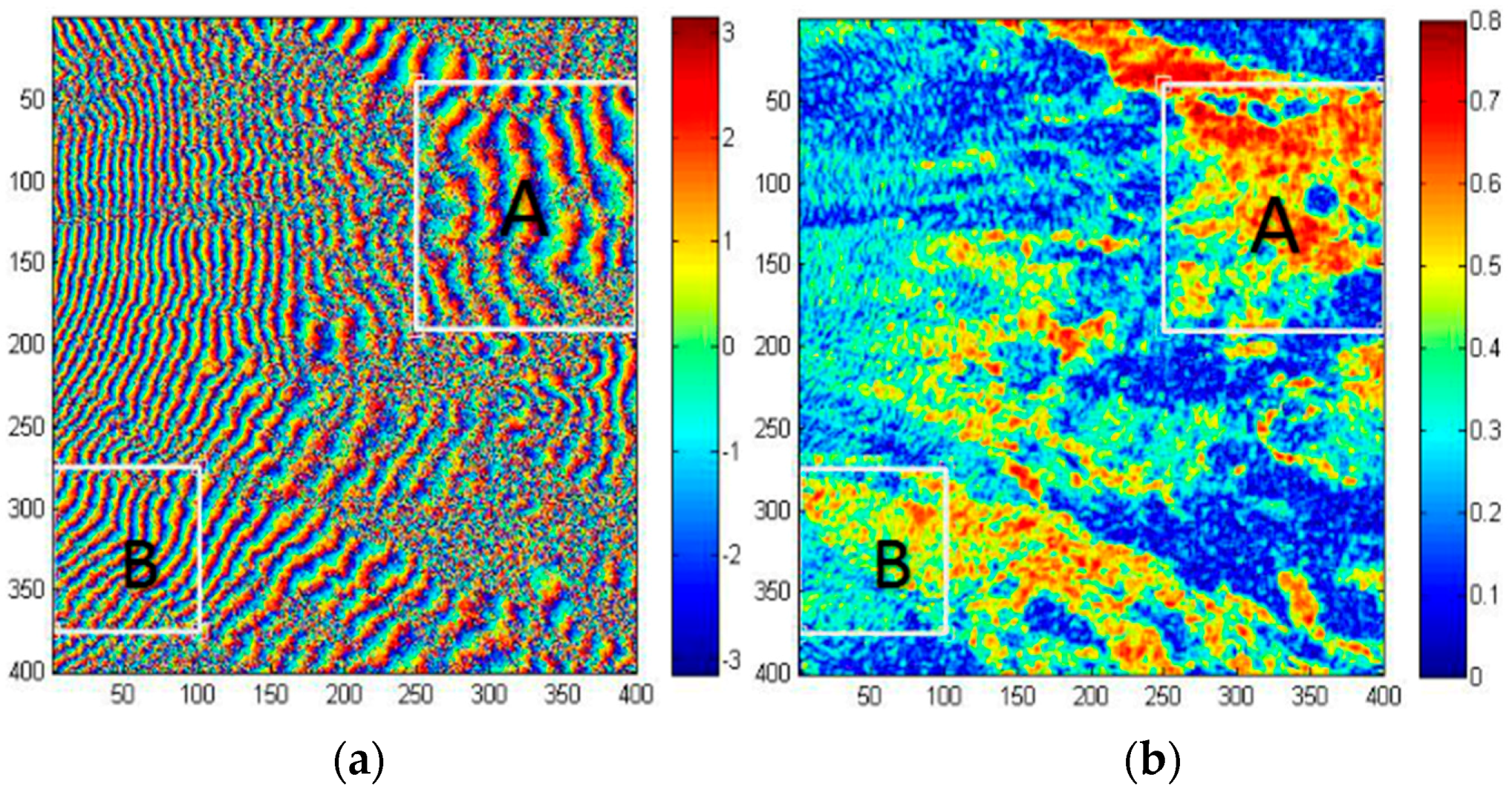
4.2. Validation with the Interferogram over Hong Kong
5. Discussion
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Berardino, P.; Fornaro, G.; Lanari, R. A new algorithm for surface deformation monitoring based on small baseline differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2375–2383. [Google Scholar] [CrossRef]
- Song, R.; Guo, H.; Liu, G.; Perski, Z.; Yue, H. Improved Goldstein SAR Interferogram Filter Based on Adaptive Neighborhood Technique. IEEE Geosci. Remote Sens. Lett. 2015, 12, 2114–2118. [Google Scholar]
- Wang, Y.; Huang, H.; Dong, Z.; Wu, M. Modified patch-based locally optimal Wiener method for interferometric SAR phase filtering. ISPRS J. Photogramm. Remote Sens. 2016, 114, 10–23. [Google Scholar] [CrossRef]
- Lin, X.; Li, F.; Meng, D.; Hu, D.; Ding, C. Nonlocal SAR Interferometric Phase Filtering Through Higher Order Singular Value Decomposition. IEEE Geosci. Remote Sens. Lett. 2015, 12, 806–810. [Google Scholar] [CrossRef]
- Suo, Z.; Zhang, J.; Li, M.; Zhang, Q. Improved InSAR Phase Noise Filter in Frequency Domain. IEEE Trans. Geosci. Remote Sens. 2016, 54, 1185–1195. [Google Scholar] [CrossRef]
- Lee, J.S.; Papathanassiou, K.P.; Ainsworth, T.L.; Grunes, M.R.; Reigber, A. A new technique for noise filtering of SAR interferometric phase images. IEEE Trans. Geosci. Remote Sens. 1998, 36, 1456–1465. [Google Scholar]
- Chao, C.F.; Chen, K.S.; Lee, J.S. Refined filtering of interferometric phase from InSAR data. IEEE Trans. Geosci. Remote Sens. 2013, 51, 5313–5323. [Google Scholar] [CrossRef]
- Fu, S.H.; Long, X.J.; Xia, Y. Directionally adaptive filter for synthetic aperture radar interferometric phase images. IEEE Trans. Geosci. Remote Sens. 2013, 51, 552–559. [Google Scholar] [CrossRef]
- Baselice, F.; Ferraioli, G.; Pascazio, V.; Schirinzi, G. Joint InSAR DEM and deformation estimation in a Bayesian framework. In Proceedings of the 2014 IEEE Geoscience and Remote Sensing Symposium (IGARSS), Quebec City, QC, Canada, 13–18 July 2014; pp. 398–401.
- Danudirdjo, D.; Hirose, A. InSAR image regularization and DEM error correction with fractal surface scattering model. IEEE Trans. Geosci. Remote Sens. 2015, 53, 1427–1439. [Google Scholar] [CrossRef]
- Goldstein, R.M.; Werner, C.L. Radar Interferogram Filtering for Geophysical Application. Geophys. Res. Lett. 1998, 25, 4035–4038. [Google Scholar] [CrossRef]
- Baran, I.; Stewart, M.P.; Kampes, B.M. A Modification to the Goldstein Radar Interferogram Filter. IEEE Trans. Geosci. Remote Sens. 2003, 41, 2114–2118. [Google Scholar] [CrossRef]
- Li, Z.W.; Ding, X.L.; Huang, C. Improved filtering parameter determination for the Goldstein radar interferogram filter. ISPRS J. Photogramm. Remote Sens. 2008, 63, 621–634. [Google Scholar] [CrossRef]
- Wang, Q.S.; Huang, H.F.; Yu, A.X.; Dong, Z. An efficient and adaptive approach for noise filtering of SAR interferometric phase images. IEEE Trans. Geosci. Remote Sens. Lett. 2011, 8, 1140–1144. [Google Scholar] [CrossRef]
- Ferretti, A.; Fumagalli, A.; Novali, F.; Prati, C. A new algorithm for processing interferometric data-stacks: SqueeSAR. IEEE Trans. Geosci. Remote Sens. 2011, 49, 3460–3470. [Google Scholar] [CrossRef]
- Parizzi, A.; Brcic, R. Adaptive InSAR stack multilooking exploiting amplitude statistics: A comparison between different techniques and practical results. IEEE Trans. Geosci. Remote Sens. Lett. 2011, 8, 441–445. [Google Scholar] [CrossRef]
- Fornaro, G.; Reale, D.; Verde, S. Adaptive spatial multilooking and temporal multilinking in SBAS interferometry. In Proceedings of the IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Munich, Germany, 22–27 July 2012; pp. 1892–1895.
- Pepe, A.; Yang, Y.; Manzo, M.; Lanari, R. Improved EMCF-SBAS processing chain based on advanced techniques for the noise-filtering and selection of small baseline multi-look DInSAR interferograms. IEEE Trans. Geosci. Remote Sens. 2015, 53, 4394–4417. [Google Scholar] [CrossRef]
- Martinez, C.L.; Canovas, X.F.; Chandra, M. SAR interferometric phase noise reduction using wavelet transform. Electron. Lett. 2001, 37, 649–651. [Google Scholar] [CrossRef]
- Martínez, C.L.; Fàbregas, X. Modeling and reduction of SAR interferometric phase noise in the wavelet domain. IEEE Trans. Geosci. Remote Sens. 2003, 40, 2553–2566. [Google Scholar] [CrossRef]
- Zha, X.; Fu, R.; Dai, Z.; Liu, B. Noise reduction in interferograms using the wavelet packet transform and Wiener filtering. IEEE Geosci. Remote Sens. Lett. 2008, 5, 404–408. [Google Scholar]
- Abdallah, W.B.; Abdelfattah, R. Two-dimensional wavelet algorithm for interferometric synthetic aperture radar phase filtering enhancement. J. Appl. Remote Sens. 2015, 9, 096061. [Google Scholar] [CrossRef]
- Xu, G.; Xing, M.; Xia, X.; Zhang, L.; Liu, Y.; Bao, Z. Sparse regularization of interferometric phase and amplitude for In SAR image formation based on Bayesian representation. IEEE Trans. Geosci. Remote Sens. 2015, 53, 2123–2136. [Google Scholar] [CrossRef]
- Easley, G.; Labate, D.; Lim, W.Q. Sparse directional image representations using the discrete shearlet transform. Appl. Comput. Harmon. Anal. 2008, 25, 25–46. [Google Scholar] [CrossRef]
- Zebker, H.A.; Villasenor, J. Decorrelation in interferometric radar echoes. IEEE Trans. Geosci. Remote Sens. 1992, 30, 950–959. [Google Scholar] [CrossRef]
- Martinez, C.L.; Pottier, E. On the extension of multidimensional speckle noise model from single-look to multilook SAR image. IEEE Trans. Geosci. Remote Sens. 2007, 45, 305–320. [Google Scholar] [CrossRef]
- Lee, J.S.; Hopple, K.W.; Mango, S.A.; Miller, R. Intensity and phase statistics of multilook polarimetric interferometric SAR imagery. IEEE Trans. Geosci. Remote Sens. 1994, 32, 1017–1028. [Google Scholar]
- Sarabandi, K. Derivation of phase statistics from the Müeller matrix. Radio Sci. 1992, 27, 553–560. [Google Scholar] [CrossRef]
- Li, Z.W.; Ding, X.L.; Zheng, D.W. Least squares-based filter for remote sensing image noise reduction. IEEE Trans. Geosci. Remote Sens. 2008, 46, 2044–2049. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, H. Comparisons of Speckle Noise Filtering Methods on Interferometric Synthetic Aperture Radar Images. J. Comput. 2014, 9, 908–915. [Google Scholar] [CrossRef]
- Cunha, A.L.; Zhou, J.; Do, M.N. The Nonsubsampled Contourlet Transform: Theory, Design, and Applications. IEEE Trans. Image Process. 2006, 15, 3089–3101. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Deng, C.; Wang, S. Shearlet-Based Adaptive Shrinkage Threshold for Image Denoising. In Proceedings of the International Conference on E-Business and E-Government (ICEE 2010), Guangzhou, China, 7–9 May 2010.
- Zhang, B.; Sun, L.X.; Yu, H.B.; Xin, Y.; Cong, Z.B. A method for improving wavelet threshold denoising in laser-induced breakdown spectroscopy. Spectrochim. Acta B 2015, 107, 32–44. [Google Scholar] [CrossRef]
- Ghiglia, D.C.; Pritt, M.D. Two-Dimensional Phase Unwrapping: Theory, Algorithms, and Software; Wiley: New York, NY, USA, 1998. [Google Scholar]
- Li, Z.L.; Zou, W.B.; Ding, X.L.; Chen, Y.Q. A quantitative measure for the quality of InSAR interferograms based on phase differences. Photogramm. Eng. Remote Sens. 2004, 70, 1131–1137. [Google Scholar] [CrossRef]





| Coherence | Multi-Look Number | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | ... | |
| 0.001 | 1.813 | 1.813 | 1.813 | 1.812 | 1.812 | 1.812 | 1.812 | 1.811 | 1.811 | 1.811 | ... |
| 0.002 | 1.812 | 1.812 | 1.811 | 1.810 | 1.810 | 1.809 | 1.809 | 1.809 | 1.808 | 1.808 | ... |
| 0.003 | 1.812 | 1.810 | 1.809 | 1.808 | 1.808 | 1.807 | 1.807 | 1.806 | 1.805 | 1.805 | ... |
| 0.004 | 1.811 | 1.809 | 1.808 | 1.807 | 1.806 | 1.805 | 1.804 | 1.803 | 1.803 | 1.802 | ... |
| 0.005 | 1.810 | 1.808 | 1.806 | 1.805 | 1.803 | 1.802 | 1.801 | 1.801 | 1.800 | 1.799 | ... |
| 0.006 | 1.809 | 1.806 | 1.804 | 1.803 | 1.801 | 1.800 | 1.799 | 1.798 | 1.797 | 1.796 | ... |
| 0.007 | 1.808 | 1.805 | 1.803 | 1.801 | 1.799 | 1.798 | 1.796 | 1.795 | 1.794 | 1.793 | ... |
| 0.008 | 1.807 | 1.804 | 1.801 | 1.799 | 1.797 | 1.795 | 1.794 | 1.792 | 1.797 | 1.790 | ... |
| 0.009 | 1.806 | 1.802 | 1.800 | 1.797 | 1.795 | 1.793 | 1.791 | 1.790 | 1.788 | 1.787 | ... |
| 0.010 | 1.805 | 1.801 | 1.798 | 1.795 | 1.793 | 1.791 | 1.789 | 1.787 | 1.785 | 1.784 | ... |
| ... | ... | ... | ... | ... | ... | ... | ... | ... | ... | ... | ... |
| Filter | Positive Residues | Negative Residues | Residue Reduction (%) | PSD (×105) | SPD (×105) | RMSE |
|---|---|---|---|---|---|---|
| Unfiltered | 10,005 | 10,008 | - | 3.4254 | 2.5439 | - |
| Goldstein | 5430 | 5431 | 45.73 | 2.5304 | 1.9945 | 0.2820 |
| Wavelet | 2817 | 2824 | 71.81 | 1.9773 | 1.7258 | 0.1824 |
| Lee | 2339 | 2342 | 76.61 | 1.8647 | 1.6119 | 0.1705 |
| Proposed | 790 | 792 | 92.09 | 1.3952 | 1.3223 | 0.1693 |
| Filter | Positive Residues | Negative Residues | Residue Reduction (%) | PSD (×105) | SPD (×105) | RMSE |
|---|---|---|---|---|---|---|
| Unfiltered | 9901 | 9905 | - | 6.1753 | 4.5503 | - |
| Goldstein | 3556 | 3559 | 64.08 | 3.4275 | 2.6937 | 0.1711 |
| Wavelet | 2248 | 2241 | 77.34 | 2.8216 | 2.3629 | 0.1499 |
| Lee | 2667 | 2671 | 73.04 | 3.3403 | 2.7315 | 0.1117 |
| Proposed | 1825 | 1826 | 81.56 | 2.5054 | 2.2158 | 0.0979 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, Y.; Zhu, J.; Fu, H.; Xie, Q.; Xu, B.; Zhang, B. SAR Interferogram Filtering of Shearlet Domain Based on Interferometric Phase Statistics. Appl. Sci. 2017, 7, 141. https://doi.org/10.3390/app7020141
He Y, Zhu J, Fu H, Xie Q, Xu B, Zhang B. SAR Interferogram Filtering of Shearlet Domain Based on Interferometric Phase Statistics. Applied Sciences. 2017; 7(2):141. https://doi.org/10.3390/app7020141
Chicago/Turabian StyleHe, Yonghong, Jianjun Zhu, Haiqiang Fu, Qinghua Xie, Bing Xu, and Bing Zhang. 2017. "SAR Interferogram Filtering of Shearlet Domain Based on Interferometric Phase Statistics" Applied Sciences 7, no. 2: 141. https://doi.org/10.3390/app7020141
APA StyleHe, Y., Zhu, J., Fu, H., Xie, Q., Xu, B., & Zhang, B. (2017). SAR Interferogram Filtering of Shearlet Domain Based on Interferometric Phase Statistics. Applied Sciences, 7(2), 141. https://doi.org/10.3390/app7020141







