A Multi-Scale Investigation of Sandy Red Clay Degradation Mechanisms During Wet–Dry Cycles and Their Implications for Slope Stability
Abstract
1. Introduction
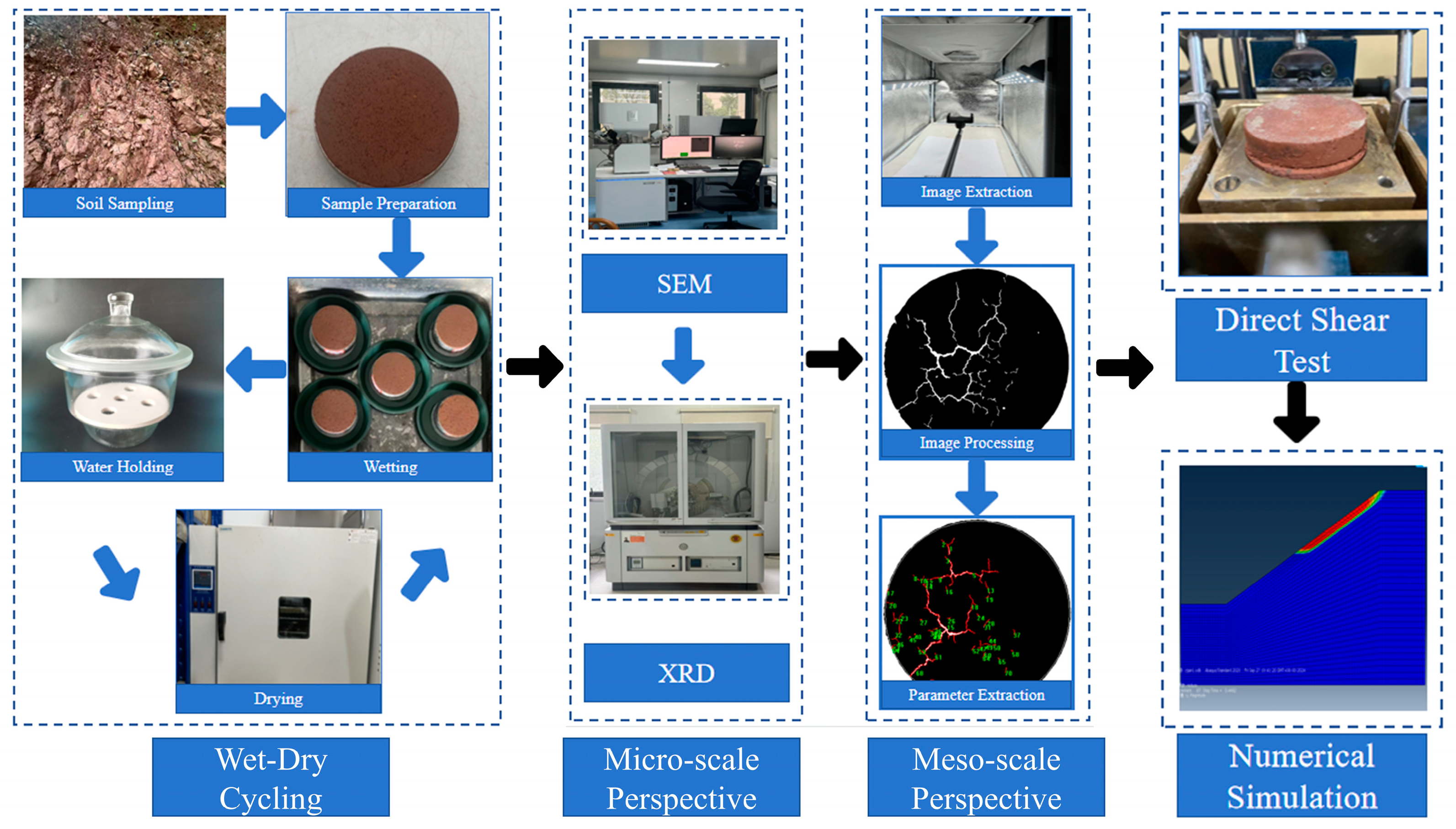
2. Experimental Materials and Methods
2.1. Experimental Materials and Preparation
2.2. Experimental Methods and Procedures
2.3. Wet–Dry Cycle Scheme
3. Damage Characteristics
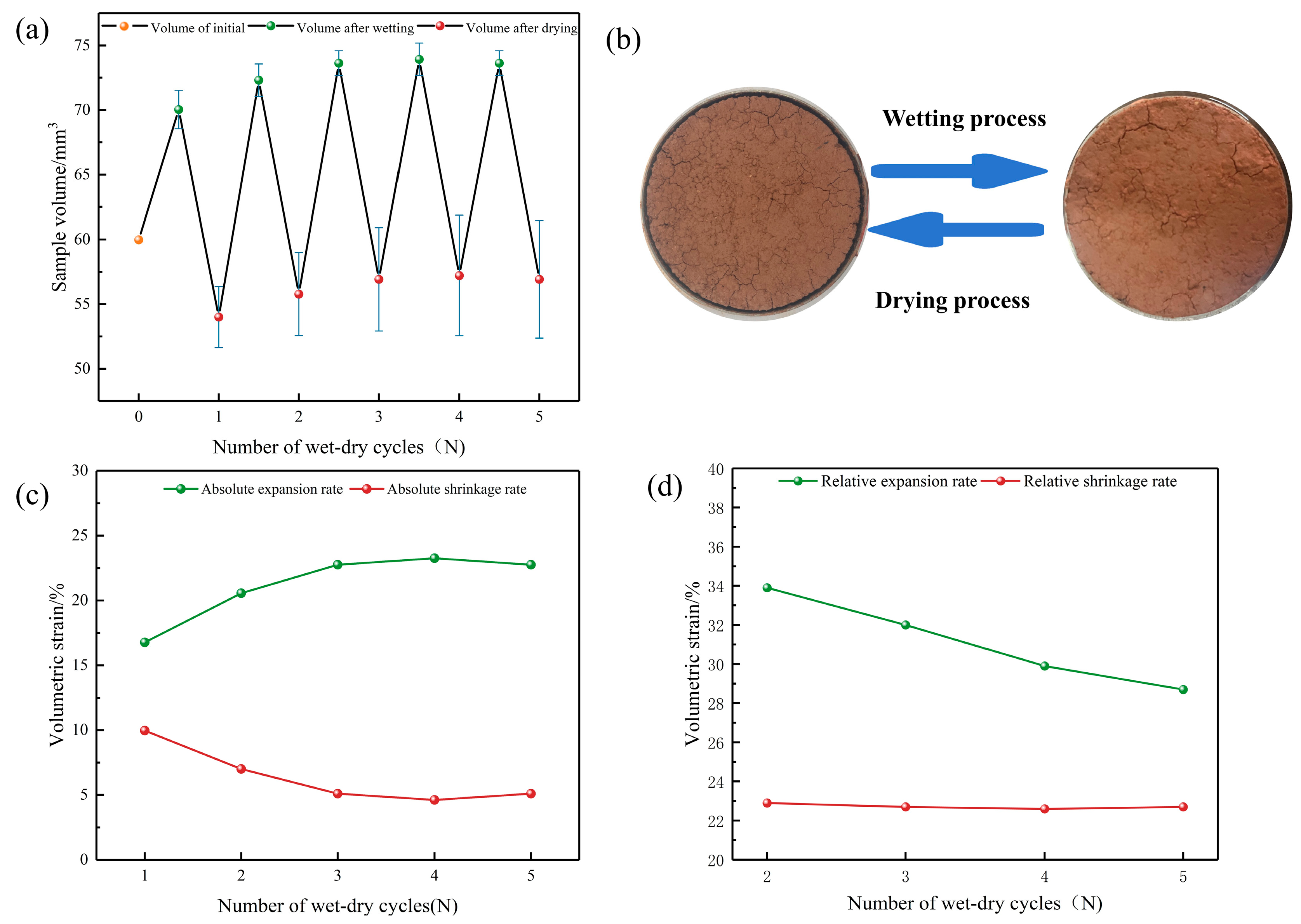
3.1. Expansion and Shrinkage Properties
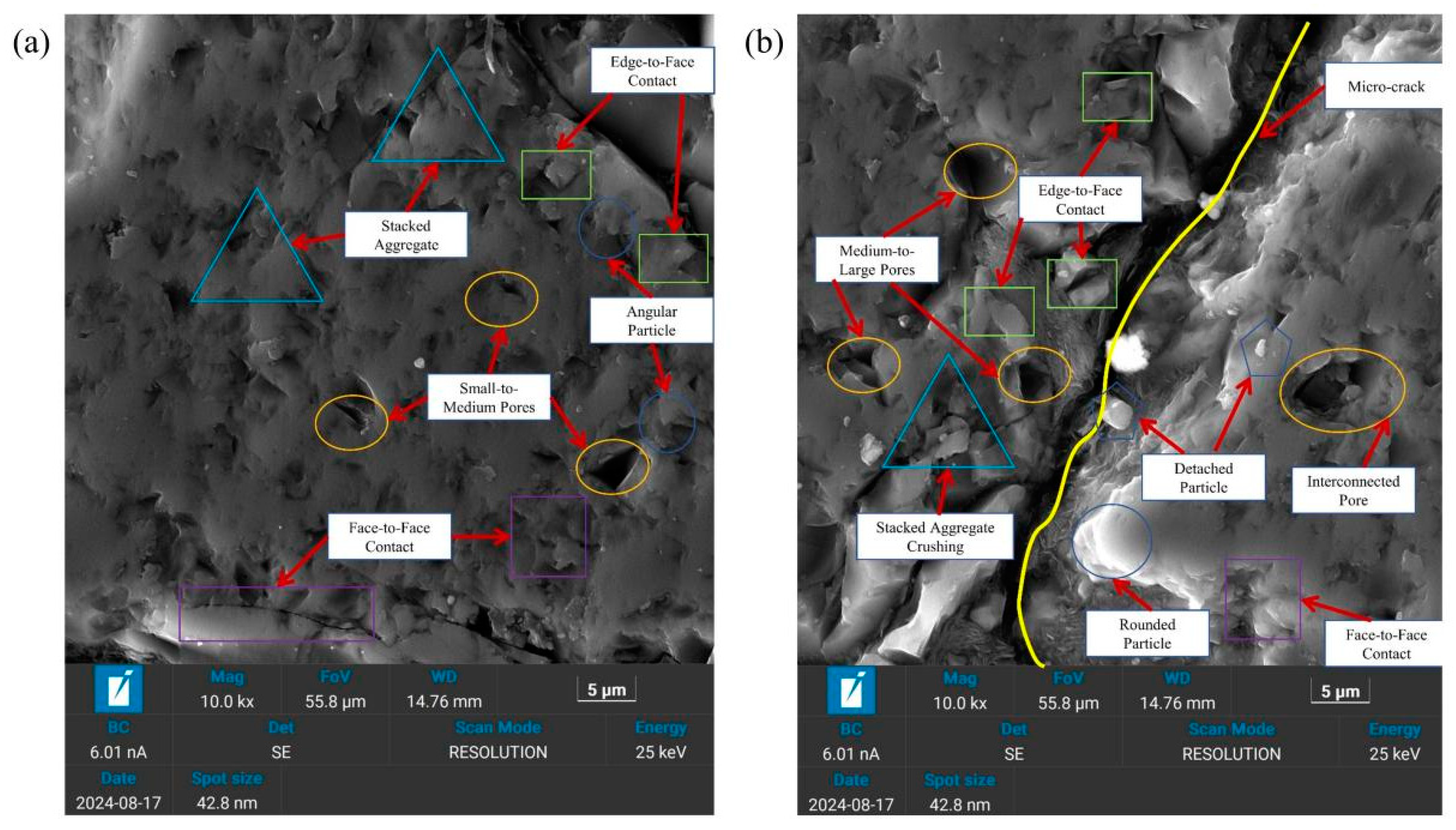
3.2. Microscale Damage
3.2.1. Microscale Physical Damage
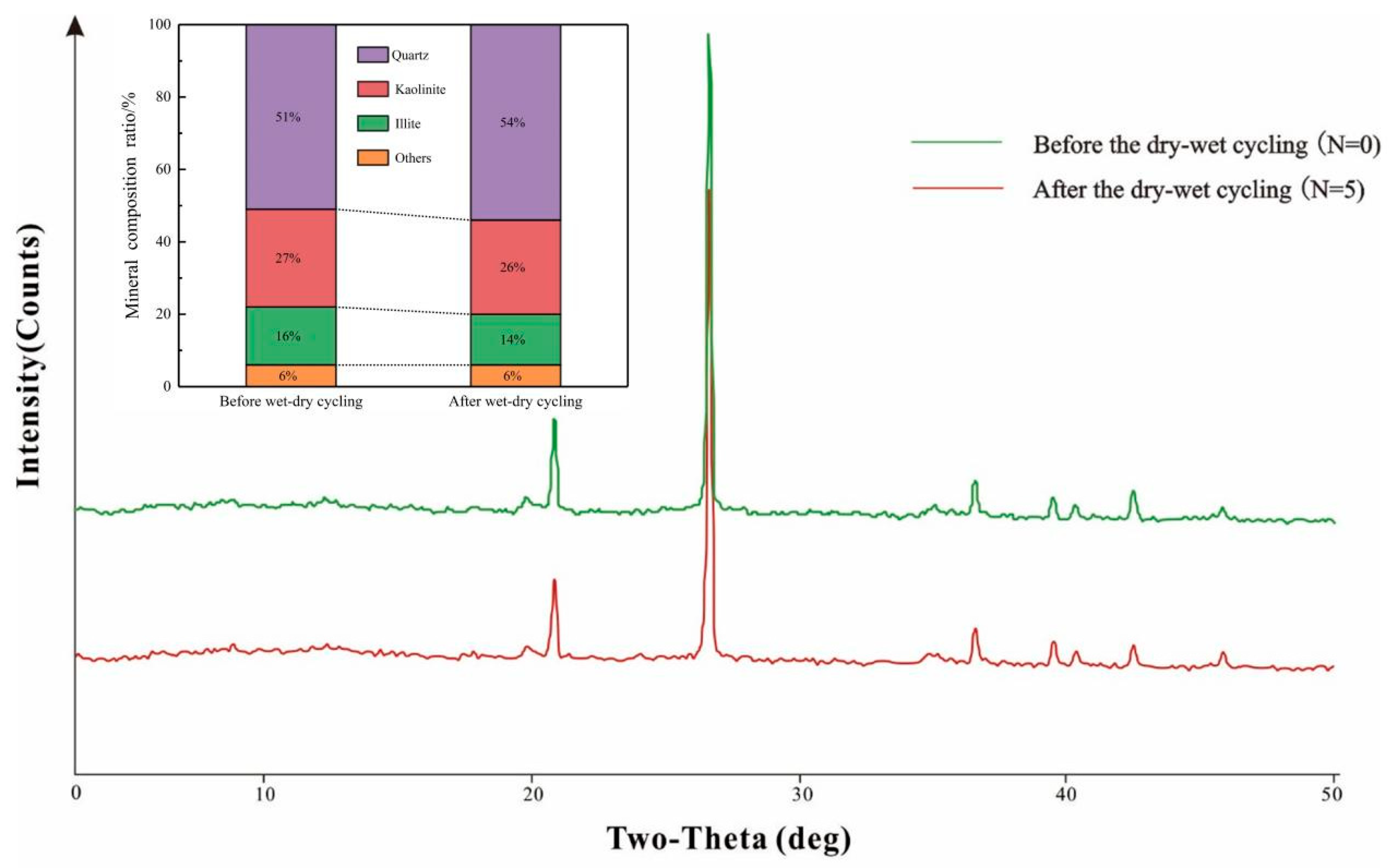
3.2.2. Microscale Chemical Damage
3.3. Mesoscale Damage
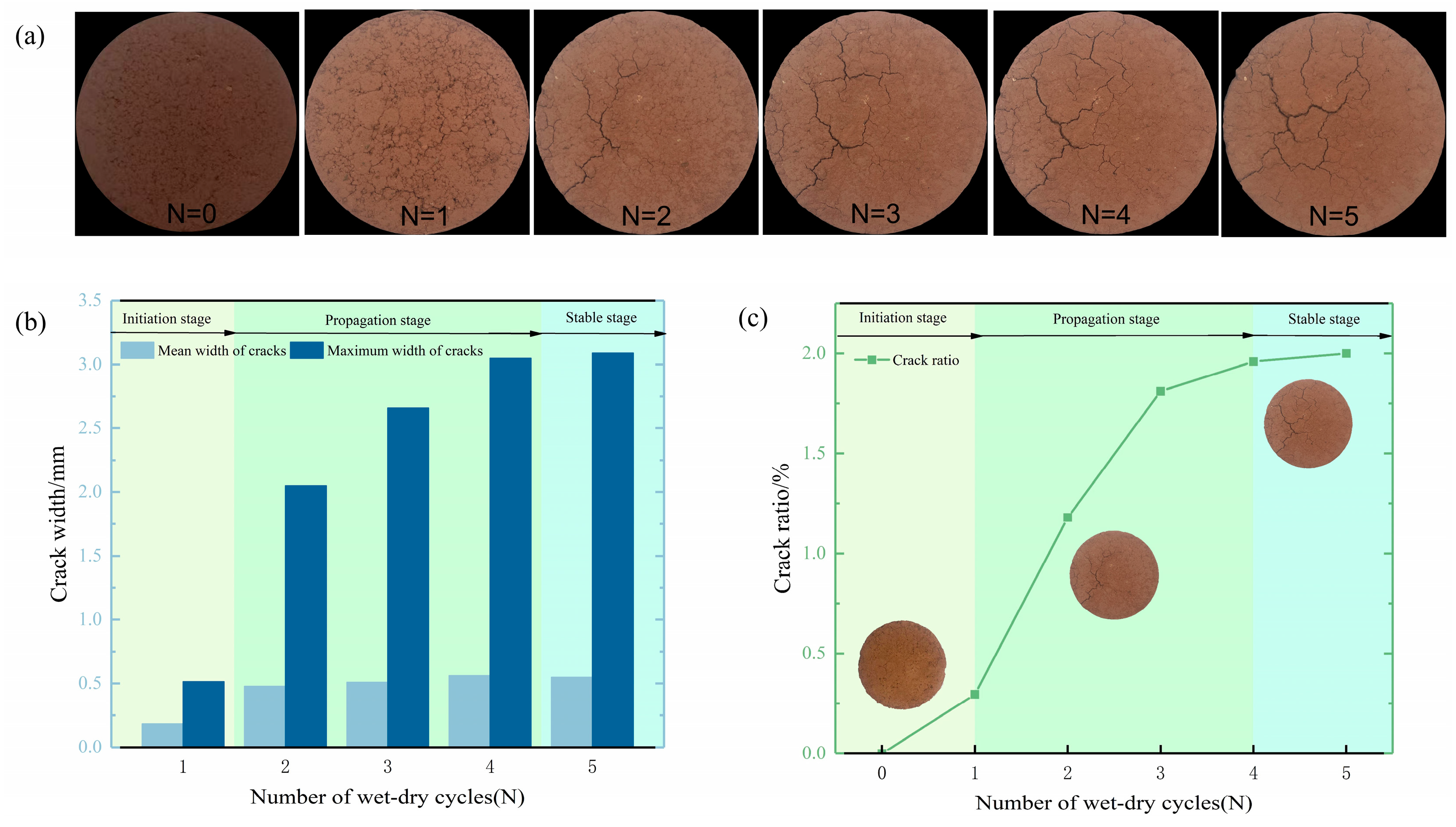
3.4. Macroscale Damage
4. Discussion on the Mechanism of Damage
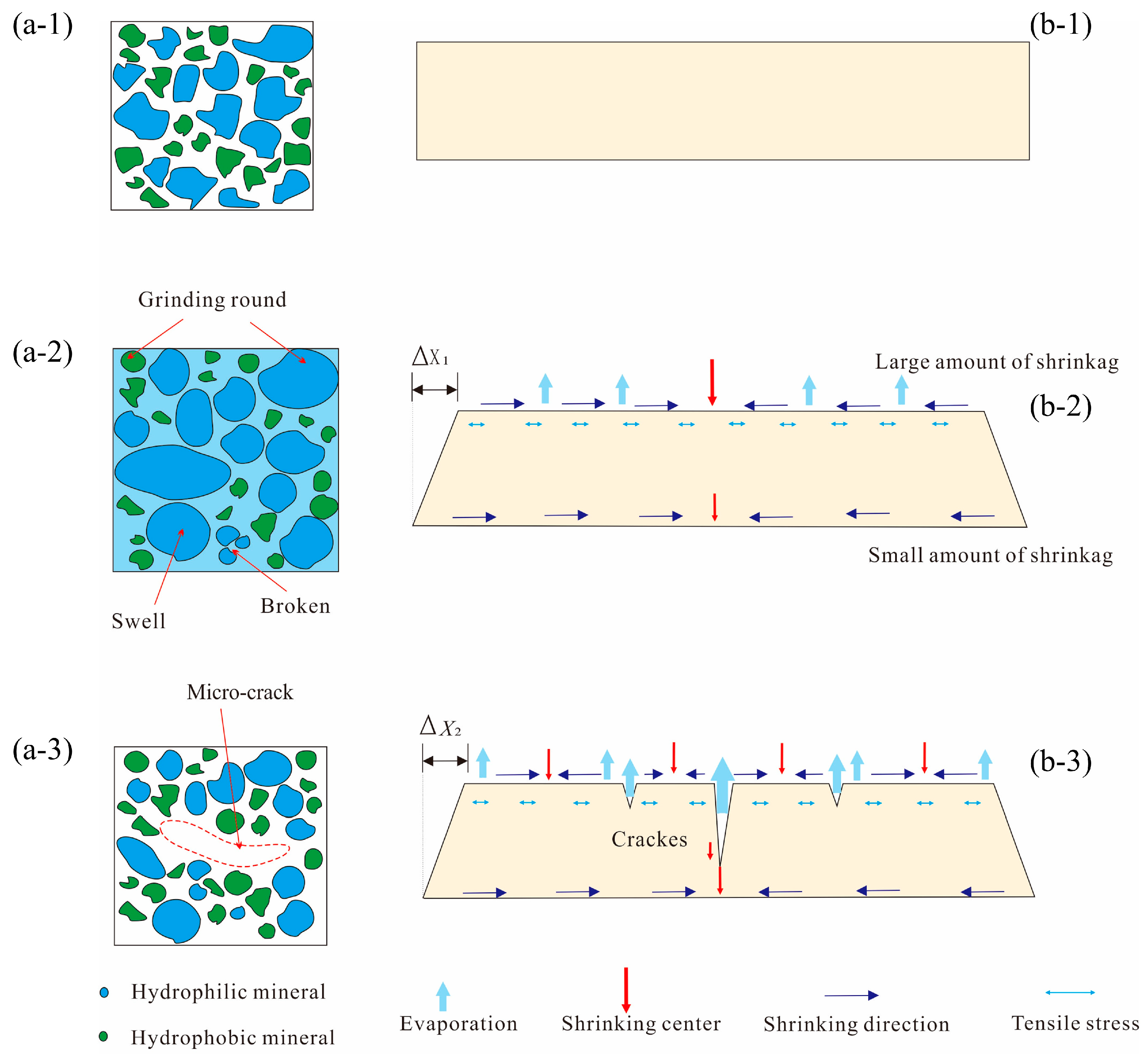
4.1. Mechanism of Microscale Damage
4.2. Mechanism of Mesoscale Damage
4.3. Mechanism of Macroscale Damage
5. Wet–Dry Cycling Effects on Slope Stability
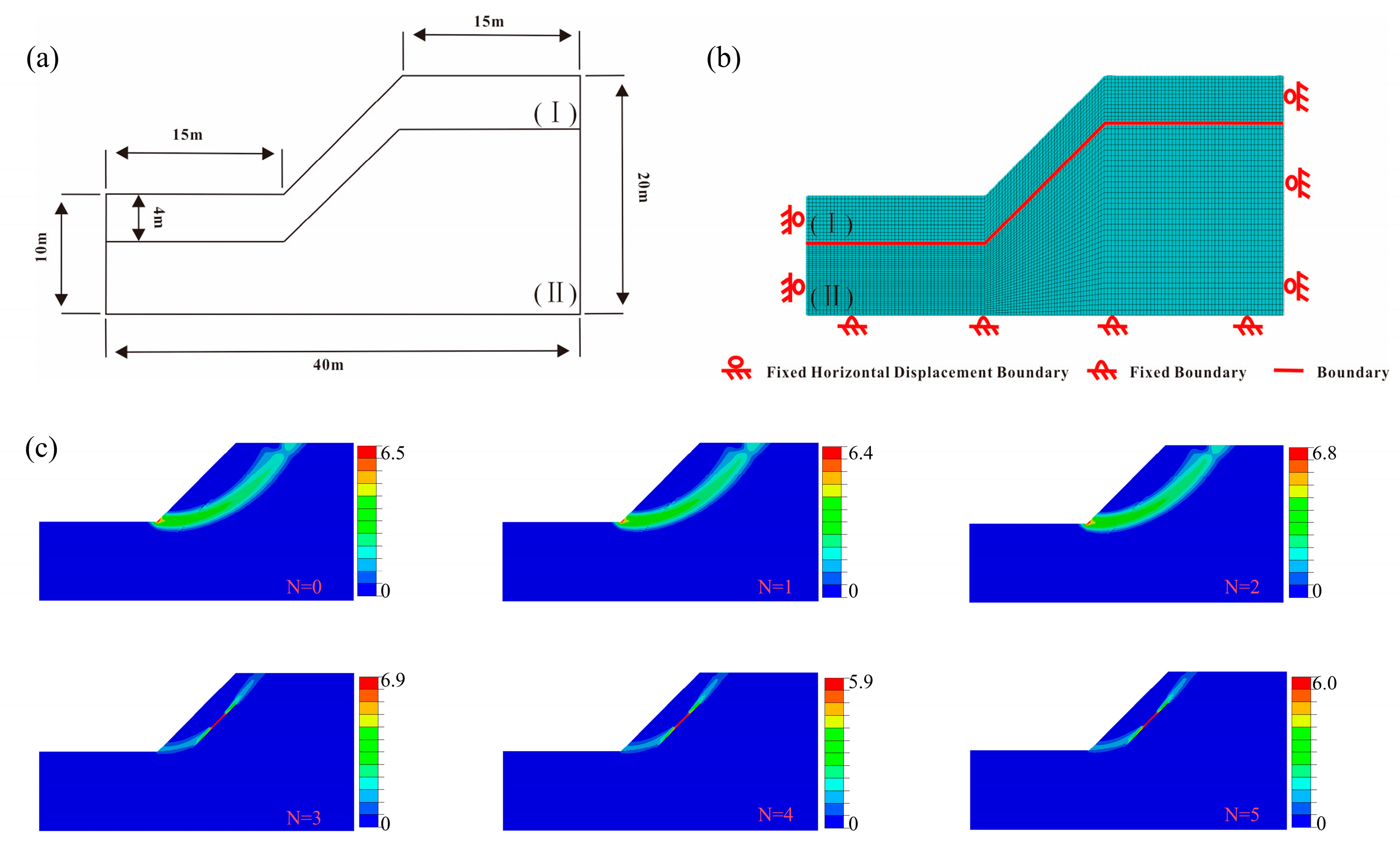
5.1. Model Construction
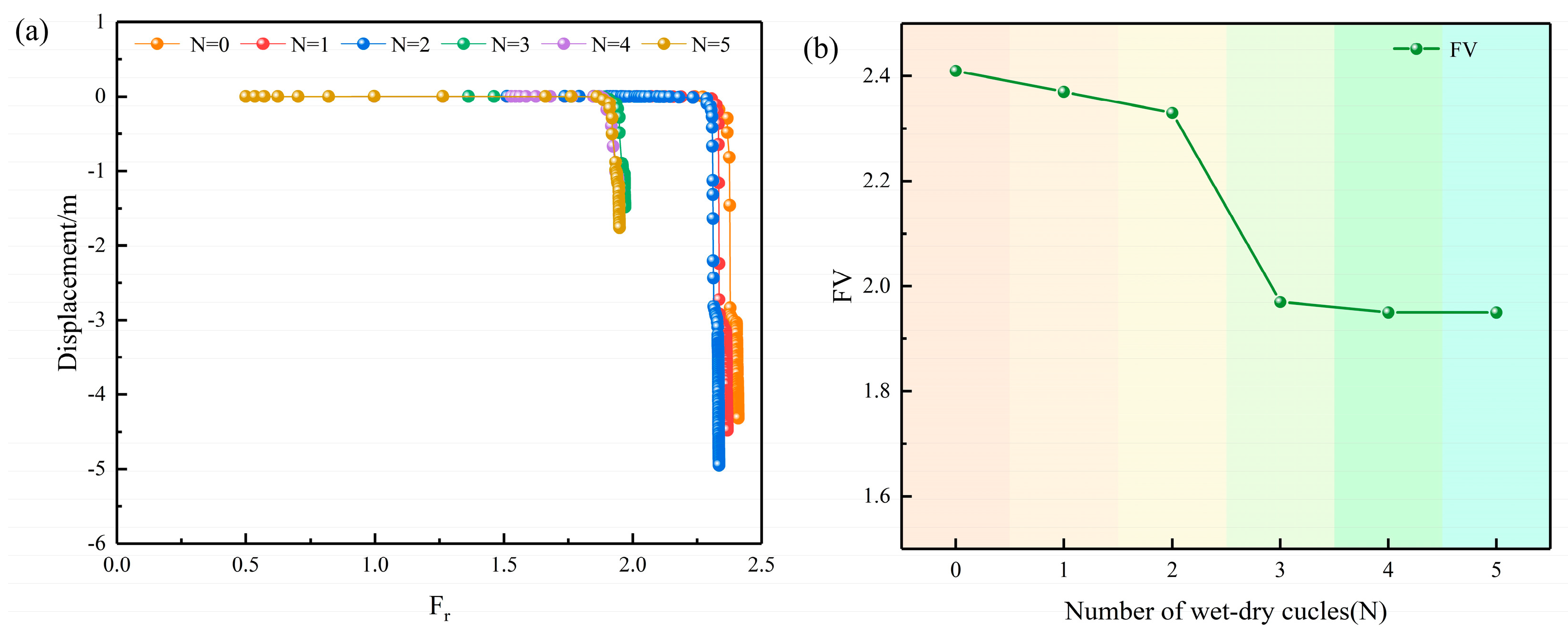
5.2. Model Computation
5.3. Preventive and Curative Measures
6. Conclusions and Prospect
6.1. Conclusions
6.2. Prospect
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, M.Y.; Ji, F.; Hong, Z.-S.; Shi, X.S. Changing law of permeability coefficient during compression for reconstituted sandy clays. Mar. Georesour. Geotechnol. 2024, 42, 1651–1659. [Google Scholar] [CrossRef]
- Shi, X.S.; Zhao, J.; Gao, Y. A homogenization-based state-dependent model for gap-graded granular materials with fine-dominated structure. Int. J. Numer. Anal. Methods Geomech. 2021, 45, 1007–1028. [Google Scholar] [CrossRef]
- Zeng, Y.; Shi, X.; Xiong, H.; Chen, W.; Bian, X. Elastoplastic modeling of sandy clays based on equivalent void ratio concept. Int. J. Geomech. 2023, 23, 04023123. [Google Scholar] [CrossRef]
- He, F.; Tan, S.; Liu, H. Mechanism of rainfall induced landslides in Yunnan Province using multi-scale spatiotemporal analysis and remote sensing interpretation. Microprocess. Microsyst. 2022, 90, 104502. [Google Scholar] [CrossRef]
- Zhao, X.; Li, G.; Zhao, Z.-F.; Li, C.-X.; Chen, Q.; Ye, X. Identifying the spatiotemporal characteristics of individual red bed landslides: A case study in Western Yunnan, China. J. Mt. Sci. 2022, 19, 1748–1766. [Google Scholar] [CrossRef]
- Pejon, O.J.; Zuquette, L.V. Analysis of cyclic swelling of mudrocks. Eng. Geol. 2002, 67, 97–108. [Google Scholar] [CrossRef]
- Estabragh, A.R.; Moghadas, M.; Javadi, A.A. Effect of different types of wetting fluids on the behaviour of expansive soil during wetting and drying. Soils Found. 2013, 53, 617–627. [Google Scholar] [CrossRef]
- Basma, A.A.; Al-Homoud, A.S.; Malkawi, A.I.H.; Al-Bashabsheh, M.A. Swelling-shrinkage behavior of natural expansive clays. Appl. Clay Sci. 1996, 11, 211–227. [Google Scholar] [CrossRef]
- Guo, P.; Wang, Y.; Zhang, X.; Ma, X.; Deng, S.; Qiu, Y. Investigation on elastic–plastic deformation and mechanical failure of varied-moisture expansive soil subjected to dry–wet cycles. Environ. Earth Sci. 2024, 83, 433. [Google Scholar] [CrossRef]
- Chaosheng, T.; Bin, S. Swelling and shrinkage behaviour of expansive soil during wetting-drying cycles. Chin. J. Geotech. Eng. 2011, 33, 1376–1384. [Google Scholar]
- Jury, W.A.; Horton, R. Soil Physics; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Xu, J.; Li, Y.; Ren, C.; Lan, W. Damage of saline intact loess after dry-wet and its interpretation based on SEM and NMR. Soils Found. 2020, 60, 911–928. [Google Scholar]
- Chen, X.; He, P.; Qin, Z. Damage to the microstructure and strength of altered granite under wet–dry cycles. Symmetry 2018, 10, 716. [Google Scholar]
- Niu, Z.-L.; Xu, J.; Li, Y.-F.; Wang, Z.-F.; Wang, B. Strength deterioration mechanism of bentonite modified loess after wetting–drying cycles. Sci. Rep. 2022, 12, 3130. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Bao, W.; Tian, L.; Huang, Z.; Chen, R. Study on the effect of pore structure changes induced by freeze-thaw-wetting-drying cycles on water retention characteristics of compacted loess. Constr. Build. Mater. 2024, 455, 139213. [Google Scholar]
- Ni, W.-K.; Yuan, K.-Z.; Lü, X.-F.; Yuan, Z.-H. Comparison and quantitative analysis of microstructure parameters between original loess and remoulded loess under different wetting-drying cycles. Sci. Rep. 2020, 10, 5547. [Google Scholar]
- Xie, Y.; Zhang, B.; Liu, B.; Zeng, Z.; Zhang, Y.; Zheng, Y. Shrinkage cracking and strength deterioration of red clay under cyclic drying and wetting. Alex. Eng. J. 2022, 61, 2574–2588. [Google Scholar] [CrossRef]
- Liu, Y.; Dai, F. A review of experimental and theoretical research on the deformation and failure behavior of rocks subjected to cyclic loading. J. Rock Mech. Geotech. Eng. 2021, 13, 1203–1230. [Google Scholar]
- Zaidi, M.; Ahfir, N.-D.; Alem, A.; Taibi, S.; El Mansouri, B.; Zhang, Y.; Wang, H. Use of X-ray computed tomography for studying the desiccation cracking and self-healing of fine soil during drying–wetting paths. Eng. Geol. 2021, 292, 106255. [Google Scholar] [CrossRef]
- Tang, C.-S.; Cui, Y.-J.; Shi, B.; Tang, A.-M.; Liu, C. Desiccation and cracking behaviour of clay layer from slurry state under wetting–drying cycles. Geoderma 2011, 166, 111–118. [Google Scholar]
- Zhou, R.; Wang, B.-T.; Han, S.-Y.; Wang, D.-Y.; Zhang, F.-H. Mechanisms of crack development and strength deterioration in compacted expansive soils under controlled wetting-drying conditions. Eng. Fail. Anal. 2024, 159, 108133. [Google Scholar]
- Yun, C.; Chan-fu, W.; Geng, N. Effect of wetting and drying cycles on shear strength of karst red clay. Rock Soil Mech. 2017, 38, 191–196. [Google Scholar]
- Gu, D.; Liu, H.; Gao, X.; Huang, D.; Zhang, W. Influence of cyclic wetting–drying on the shear strength of limestone with a soft interlayer. Rock Mech. Rock Eng. 2021, 54, 4369–4378. [Google Scholar]
- Noor-E-Khuda, S. Influence of wetting–drying cycles on compressive and flexural strength of cement mortar and CFRP-mortar bond strength. Constr. Build. Mater. 2021, 271, 121513. [Google Scholar] [CrossRef]
- Hua, W.; Dong, S.; Li, Y.; Xu, J.; Wang, Q. The influence of cyclic wetting and drying on the fracture toughness of sandstone. Int. J. Rock Mech. Min. Sci. 2015, 78, 331–335. [Google Scholar]
- Zhang, J.J.; Guang, Z. Study of the Fissures, Volume Change and Permeability of Expansive Soil Under Wetting and Drying Cycles. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2010. [Google Scholar]
- Bai, Y.; Ye, W.; Wu, Y.; Chen, Y. Multiscale analysis of the strength deterioration of loess under the action of drying and wetting cycles. Adv. Mater. Sci. Eng. 2021, 2021, 6654815. [Google Scholar]
- Qin, Y.; Li, G.; Chen, X.; Fan, K. Study on shear strength and structure of Malan loess under wetting–drying cycles. Arab. J. Geosci. 2021, 14, 2854. [Google Scholar]
- Huihui, X.; Zhenhao, X.; Qingbing, L.; Guiyang, H. Evolution of peak and residual strengths of weakly expansive soils under wet-dry cyclic paths. Geotechnical 2019, 40 (Suppl. S1), 245–252. [Google Scholar]
- Estabragh, A.; Parsaei, B.; Javadi, A. Laboratory investigation of the effect of cyclic wetting and drying on the behaviour of an expansive soil. Soils Found. 2015, 55, 304–314. [Google Scholar]
- Chai, Z.Y.; Zhang, P.; Guo, J.Q.; Kang, T.H. Swelling anisotropy and cyclic swelling-shrinkage of argillaceous rock. Rock Soil Mech. 2014, 35, 347–350. [Google Scholar]
- Tian, B.G.; Cheng, Q.; Tang, C.S.; Shi, B. Healing behaviour of desiccation cracks in a clayey soil subjected to different wetting rates. Eng. Geol. 2022, 313, 106973. [Google Scholar]
- Zhang, Y.; Lu, J.; Han, W.; Xiong, Y.; Qian, J. Effects of moisture and stone content on the shear strength characteristics of soil-rock mixture. Materials 2023, 16, 567. [Google Scholar] [CrossRef] [PubMed]
- Kang, Q.; Xia, Y.; Li, X.; Zhang, W.; Feng, C. Study on the effect of moisture content and dry density on shear strength of silty clay based on direct shear test. Adv. Civ. Eng. 2022, 2022, 2213363. [Google Scholar] [CrossRef]
- Wang, Q.; Zhou, Y.; Su, W.; Xie, J.; Xu, Y.; Liu, Y.; Ye, W. Study on the disintegration characteristics of expansive stiff clay: With consideration of expansion-disintegration interaction. Bull. Eng. Geol. Environ. 2024, 83, 446. [Google Scholar] [CrossRef]
- Chen, L.; Lu, L. Investigation on the characteristics of volumetric change during the wet-dry cycle of the soil. Chin. J. Undergr. Space Eng. 2013, 9, 229–235. [Google Scholar]
- Chaosheng, T.; Cui, Y.; Tang, A.M.; Shi, B. Volumetric shrinkage characteristics of soil during drying. Chin. J. Geotech. Eng. 2011, 33, 1271–1279. [Google Scholar]
- Leng, T.; Tang, C.; Xu, D.; Li, Y.; Zhang, Y.; Wang, K.; Shi, B. Advance on the engineering geological characteristics of expansive soil. J. Eng. Geol. 2018, 26, 112–128. [Google Scholar]
- Akgün, H.; Türkmenoğlu, A.G.; Kelam, A.A.; Yousefi-Bavil, K.; Öner, G.; Koçkar, M.K. Assessment of the effect of mineralogy on the geotechnical parameters of clayey soils: A case study for the Orta County, Çankırı, Turkey. Appl. Clay Sci. 2018, 164, 44–53. [Google Scholar] [CrossRef]
- Zhu, H.; Zhang, Y.; Li, Z.; Xue, X. Study on crack development and micro-pore mechanism of expansive soil improved by coal gangue under drying–wetting cycles. Materials 2021, 14, 6546. [Google Scholar] [CrossRef]
- Zhou, Z.; Cai, X.; Cao, W.; Li, X.; Xiong, C. Influence of water content on mechanical properties of rock in both saturation and drying processes. Rock Mech. Rock Eng. 2016, 49, 3009–3025. [Google Scholar] [CrossRef]
- Wu, K.; Zhao, C.; Zhang, W.; Wu, H.; Wang, Y.; Yu, Y. Swelling-shrinking characteristics and irreversible deformation of expansive soil during wetting-drying cycles. J. Harbin Inst. Technol. 2016, 48, 121–127. [Google Scholar]
- Qi, J.; Vermeer, P.A.; Cheng, G. A review of the influence of freeze-thaw cycles on soil geotechnical properties. Permafr. Periglac. Process. 2006, 17, 245–252. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and non-associated visco-plasticity and plasticity in soil mechanics. Geotechnique 1975, 25, 671–689. [Google Scholar]
- Lin, P.; Liu, X.; Hu, S.; Li, P. Large deformation analysis of a high steep slope relating to the Laxiwa Reservoir, China. Rock Mech. Rock Eng. 2016, 49, 2253–2276. [Google Scholar]
- Griffiths, D.V.; Fenton, G.A. Probabilistic slope stability analysis by finite elements. J. Geotech. Geoenviron. Eng. 2004, 130, 507–518. [Google Scholar]
- Matsui, T.; San, K.C. Finite element slope stability analysis by shear strength reduction technique. Soils Found. 1992, 32, 59–70. [Google Scholar]
- Gupta, V.; Bhasin, R.K.; Kaynia, A.M.; Kumar, V.; Saini, A.S.; Tandon, R.S.; Pabst, T. Finite element analysis of failed slope by shear strength reduction technique: A case study for Surabhi Resort Landslide, Mussoorie township, Garhwal Himalaya. Geomat. Nat. Hazards Risk 2016, 7, 1677–1690. [Google Scholar]
- Dong, Y.; Liao, Z.; Wang, J.; Liu, Q.; Cui, L. Potential failure patterns of a large landslide complex in the Three Gorges Reservoir area. Bull. Eng. Geol. Environ. 2023, 82, 41. [Google Scholar] [CrossRef]
- Yin, Z.; Yuan, J.; Wei, J.; Cao, X.; Liu, H.; Xu, B. Influences of fissures on slope stability of expansive soil. Chin. J. Geotech. Eng. 2012, 34, 2155–2161. [Google Scholar]
- Zhou, J.; Xu, H.-Z.; Hu, W.-J. Impact of wetting-drying cycle effects on stability of expansive soil slopes. Chin. J. Geotech. Eng. 2013, 35, 152–156. [Google Scholar]
- Peng, X.; Li, J.; Li, Y.; Jiang, J. Slope instability judgment criteria in FEM based on strength reduction method. In Proceedings of the 2020 5th International Conference on Materials Science, Energy Technology and Environmental Engineering, Shanghai, China, 7–9 August 2020; IOP Publishing: Bristol, UK, 2020; Volume 571, p. 012104. [Google Scholar]
- Zhang, H.; Yang, J.; Wang, L.; Xu, Y.; Lan, S.; Luo, J.; Chang, Z. Experimental and numerical investigation on failure mechanism of expansive soil subgrade slope. Sci. Rep. 2023, 13, 19795. [Google Scholar]
- Wang, H.; Wang, Y.; Jin, F. Stability of Expansive Soil Slopes under Wetting–Drying Cycles Based on the Discrete Element Method. Water 2024, 16, 861. [Google Scholar] [CrossRef]
- Li, T.; Chen, G. Analysis of factors influencing anti-slip pile support in tunnel landslide systems for tunnels with different burial depths. Transp. Geotech. 2023, 42, 101079. [Google Scholar] [CrossRef]
- Gao, J.; Zhang, H.; Xie, X.; Zhang, Y. Study on the mechanical properties and microstructure of geogrid under different materials and temperatures. Fibers Polym. 2023, 23, 1753–1762. [Google Scholar]
- Ma, J.; Liu, X.; Yuan, S.; Xu, J.; Chen, F.; Yang, X. Multi-scale investigation on curing time effect of lime stabilized red mudstone as fill material for high-speed railway subgrade. Constr. Build. Mater. 2024, 443, 137749. [Google Scholar] [CrossRef]
- Lin, G.; Liu, W.; Zhao, J.; Fu, P. Experimental investigation into effects of lignin on sandy loess. Soils Found. 2023, 63, 101359. [Google Scholar]



| Sampling Depth/m | Natural Density/g·cm−3 | Natural Water Content | Natural Dry Density/g·cm−3 |
|---|---|---|---|
| 4–6 | 2.03 | 18.1% | 1.72 |
| Zone | Modulus of Deformation /(kpa) | Internal Friction Angle/(°) | Cohesion /(kpa) | Volume Weight /(kN/m3) | Poisson’s Ratio | |
|---|---|---|---|---|---|---|
| Zone I | 0 | 105 | 24.50 | 48.4 | 20.3 | 0.30 |
| 1 | 105 | 24.10 | 44.6 | 20.3 | 0.30 | |
| 2 | 105 | 23.86 | 36.8 | 20.3 | 0.30 | |
| 3 | 105 | 23.84 | 28.8 | 20.3 | 0.30 | |
| 4 | 105 | 24.05 | 25.7 | 20.3 | 0.30 | |
| 5 | 105 | 23.46 | 24.4 | 20.3 | 0.30 | |
| Zone II | 105 | 24.50 | 48.4 | 20.3 | 0.30 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, W.; Liu, Z.; Kong, Z.; Jing, L.; Xiao, R. A Multi-Scale Investigation of Sandy Red Clay Degradation Mechanisms During Wet–Dry Cycles and Their Implications for Slope Stability. Appl. Sci. 2025, 15, 4085. https://doi.org/10.3390/app15084085
Xie W, Liu Z, Kong Z, Jing L, Xiao R. A Multi-Scale Investigation of Sandy Red Clay Degradation Mechanisms During Wet–Dry Cycles and Their Implications for Slope Stability. Applied Sciences. 2025; 15(8):4085. https://doi.org/10.3390/app15084085
Chicago/Turabian StyleXie, Wei, Zhenguo Liu, Zhigang Kong, Lu Jing, and Rui Xiao. 2025. "A Multi-Scale Investigation of Sandy Red Clay Degradation Mechanisms During Wet–Dry Cycles and Their Implications for Slope Stability" Applied Sciences 15, no. 8: 4085. https://doi.org/10.3390/app15084085
APA StyleXie, W., Liu, Z., Kong, Z., Jing, L., & Xiao, R. (2025). A Multi-Scale Investigation of Sandy Red Clay Degradation Mechanisms During Wet–Dry Cycles and Their Implications for Slope Stability. Applied Sciences, 15(8), 4085. https://doi.org/10.3390/app15084085





