Stability Analysis of the Longitudinal Slope Linear Shield Tunnel Excavation Face
Abstract
1. Introduction
2. Materials and Methods for Longitudinal Slope Shield Tunneling
2.1. Transparent Soil Model Test for Longitudinal Slope Shield Tunneling
2.1.1. Transparent Soil Materials
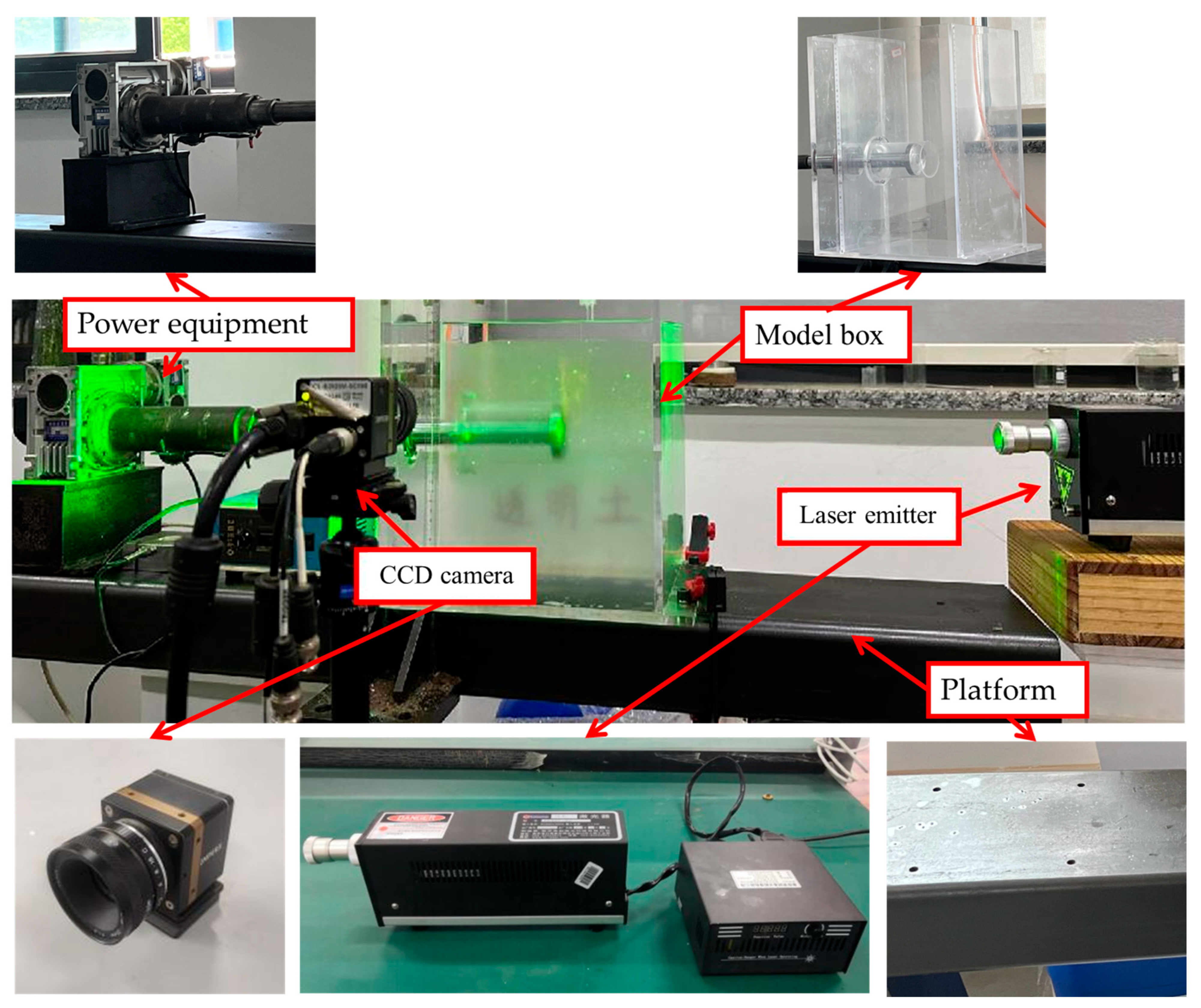
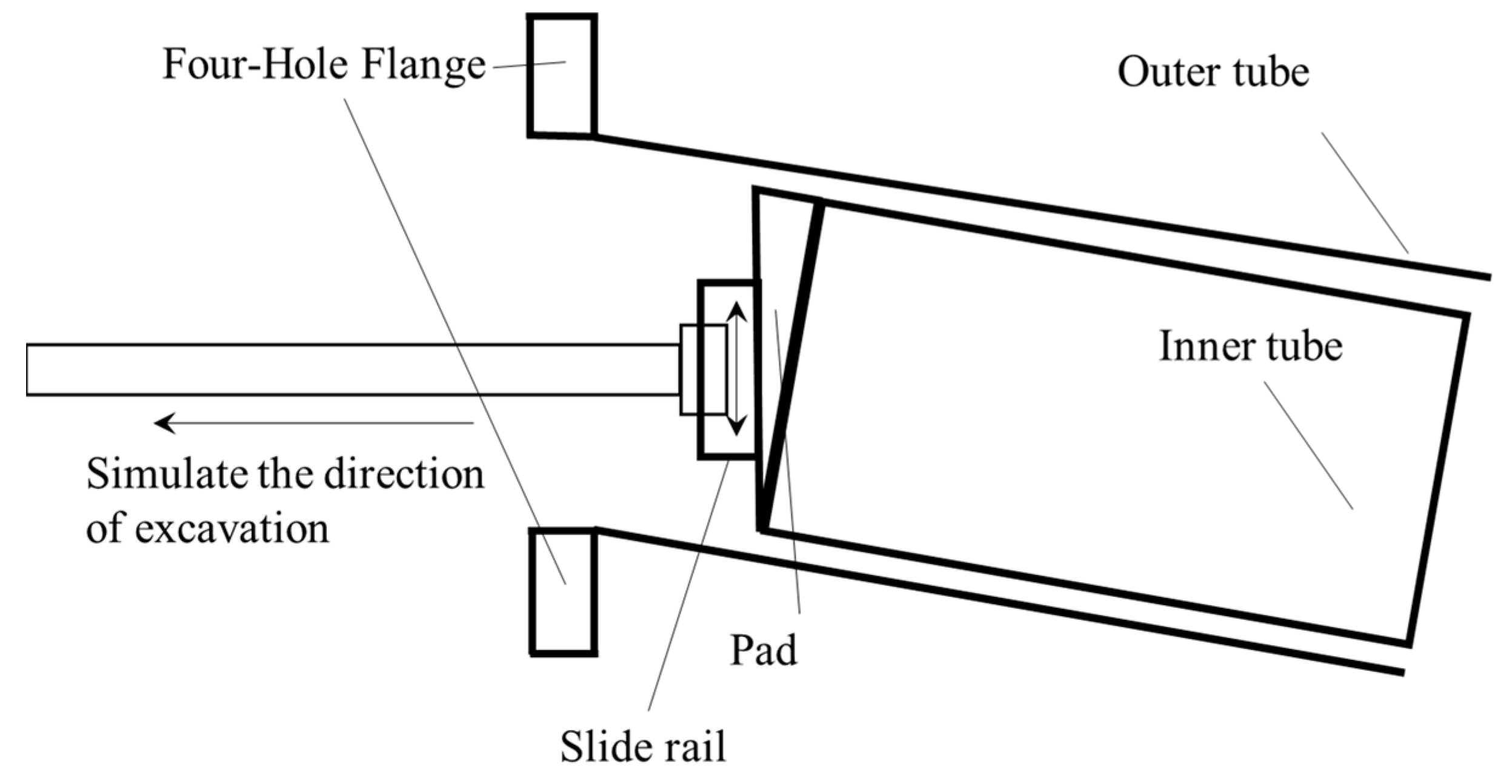
2.1.2. Test Equipment
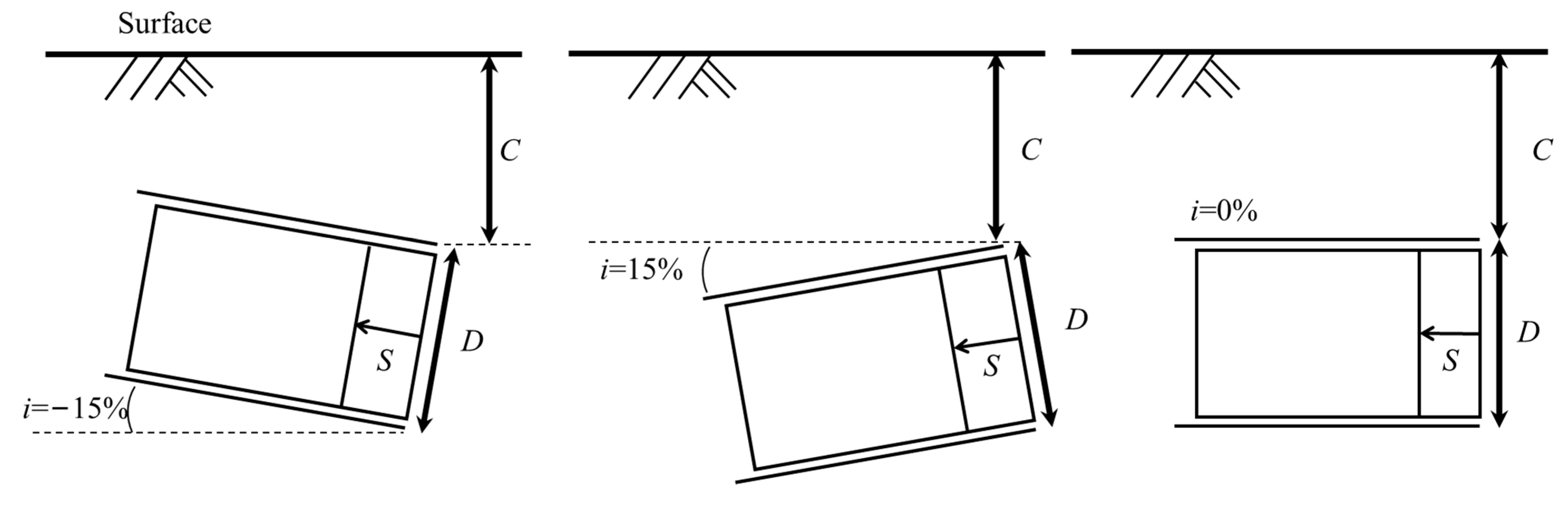
2.1.3. Test Procedure and Working Conditions
2.2. Numerical Simulation Analysis of Longitudinal Tunnel
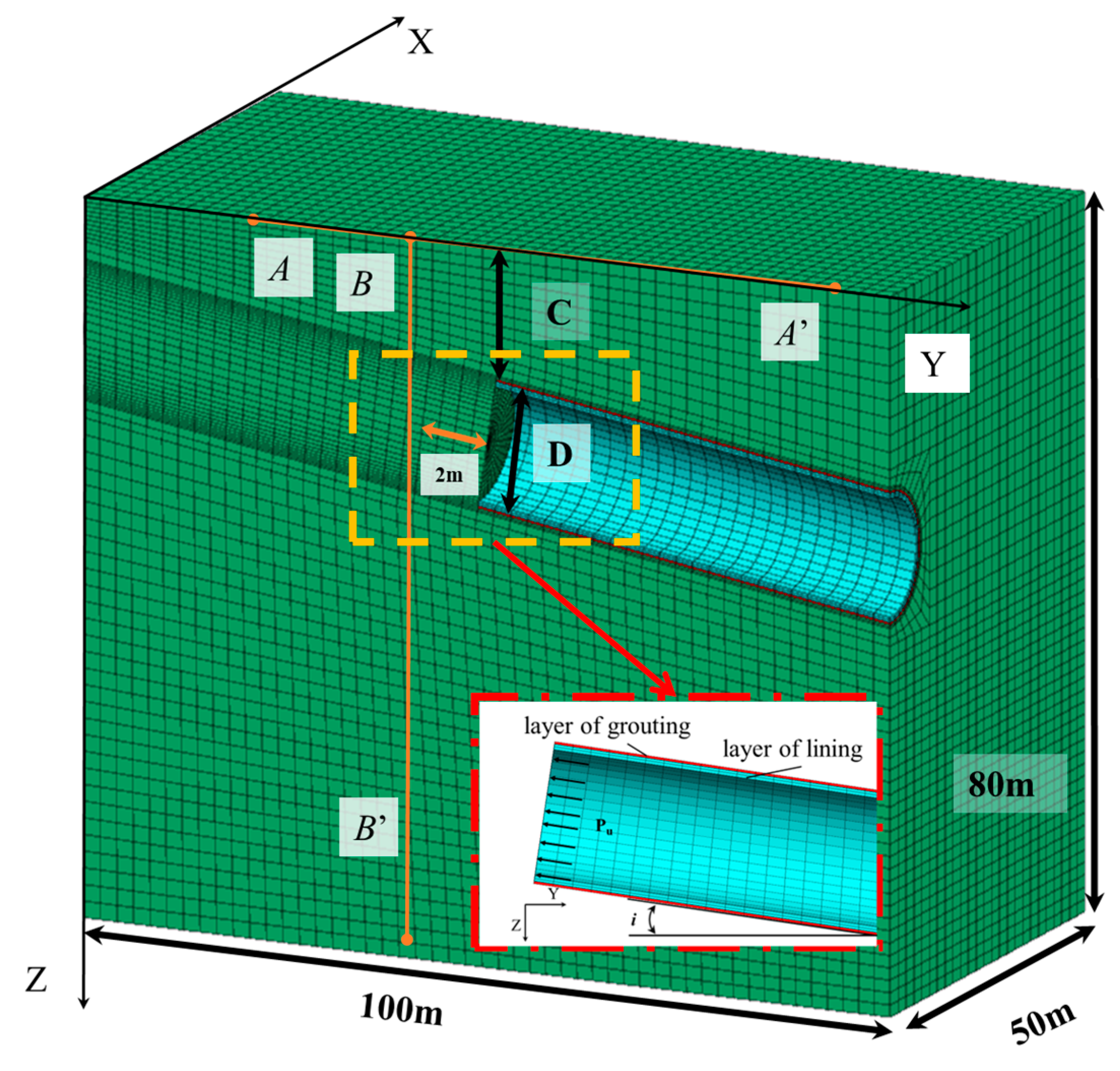
2.2.1. Numerical Simulation Modeling
2.2.2. Numerical Simulation Methods and Numerical Simulation Parameters
3. Results and Discussion
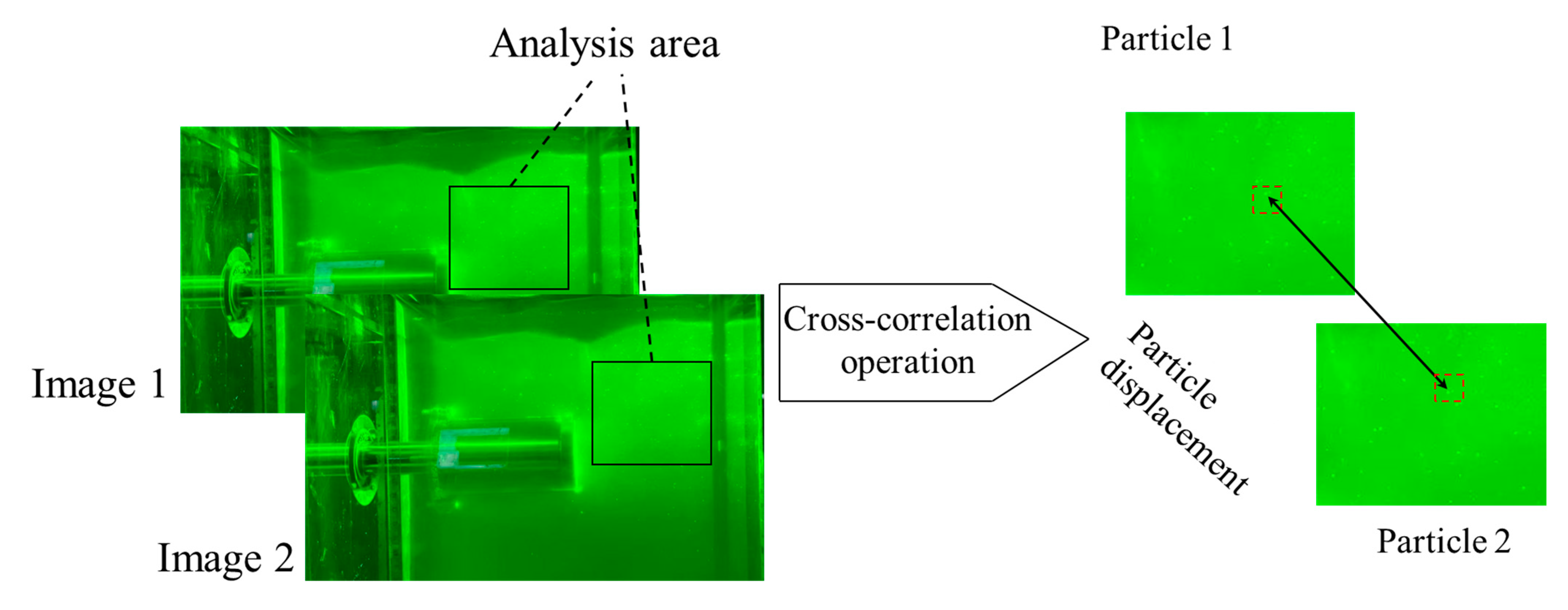
3.1. Results and Discussion of Transparent Soil Experiment
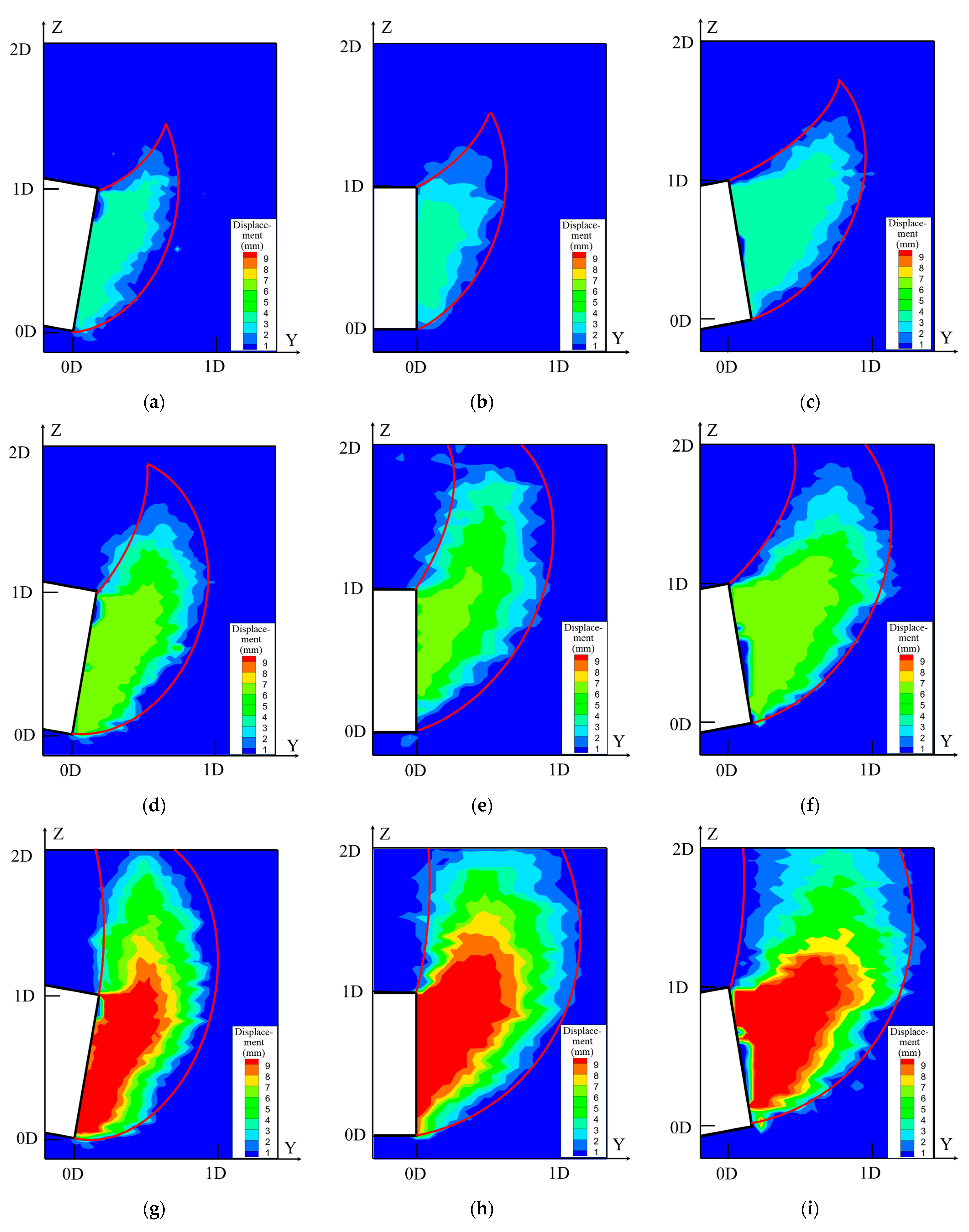
3.2. Validation and Comparison of Numerical Simulation Results
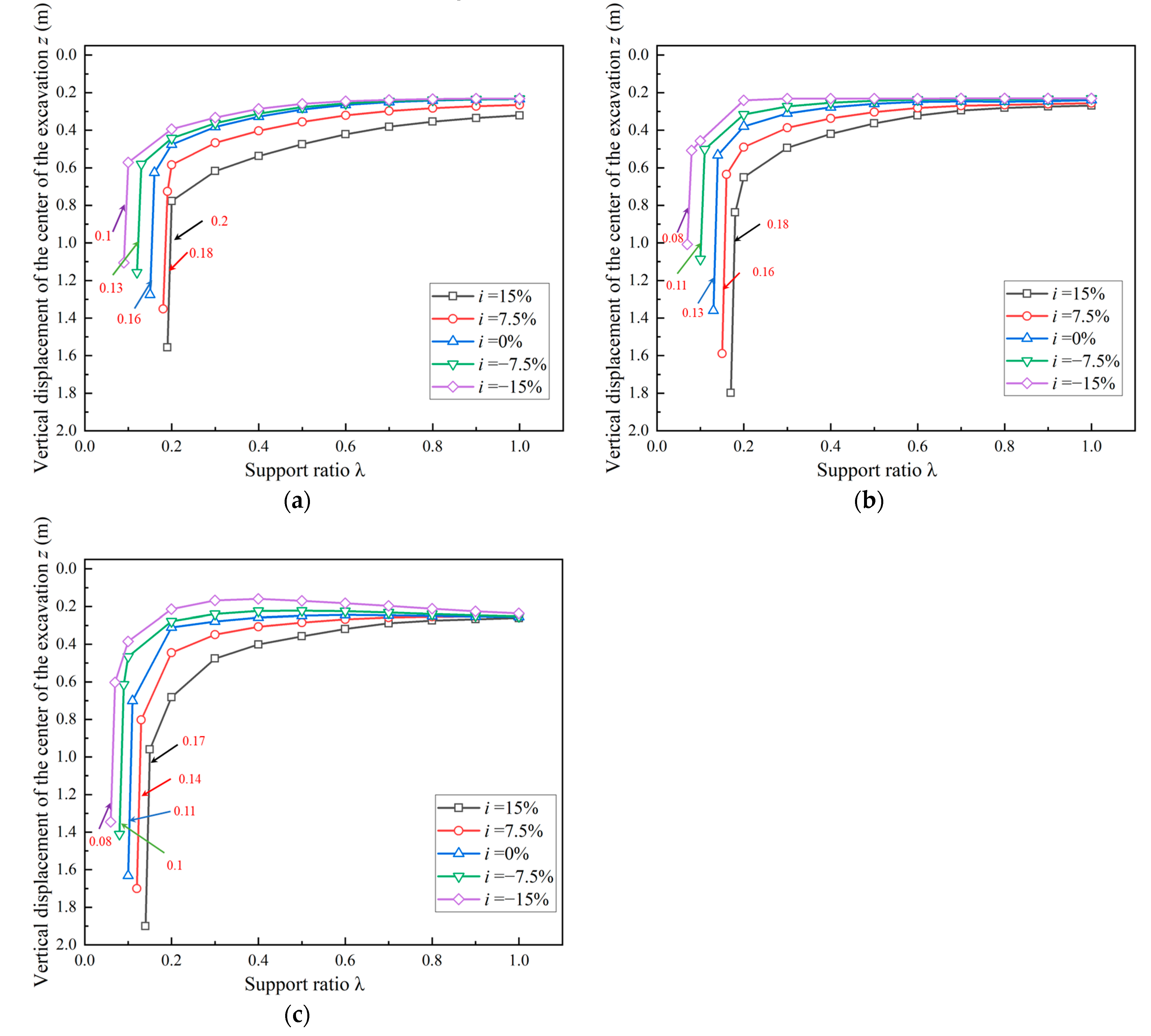
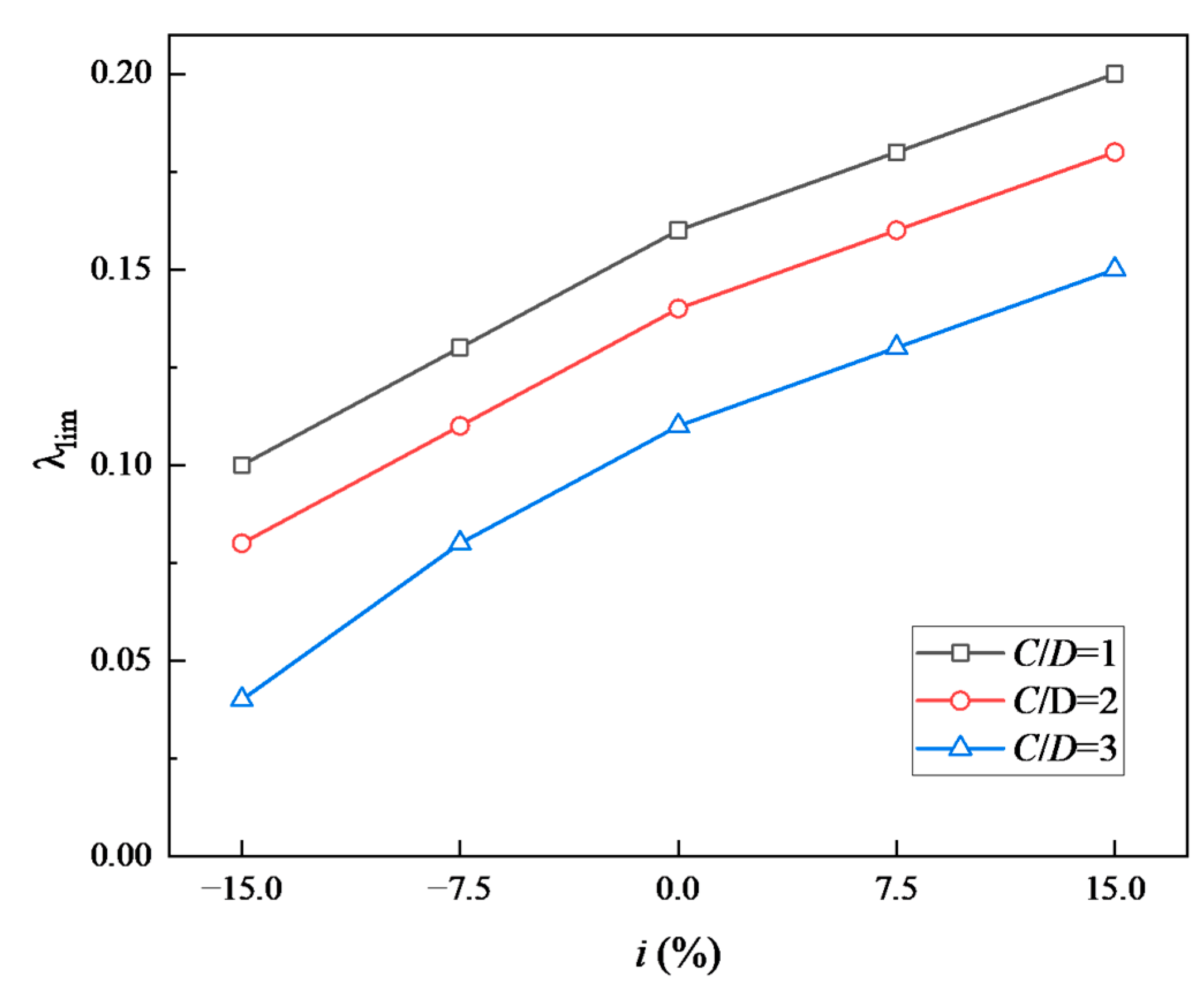
3.3. Results and Discussion of Ultimate Support Ratio in Numerical Simulation Results
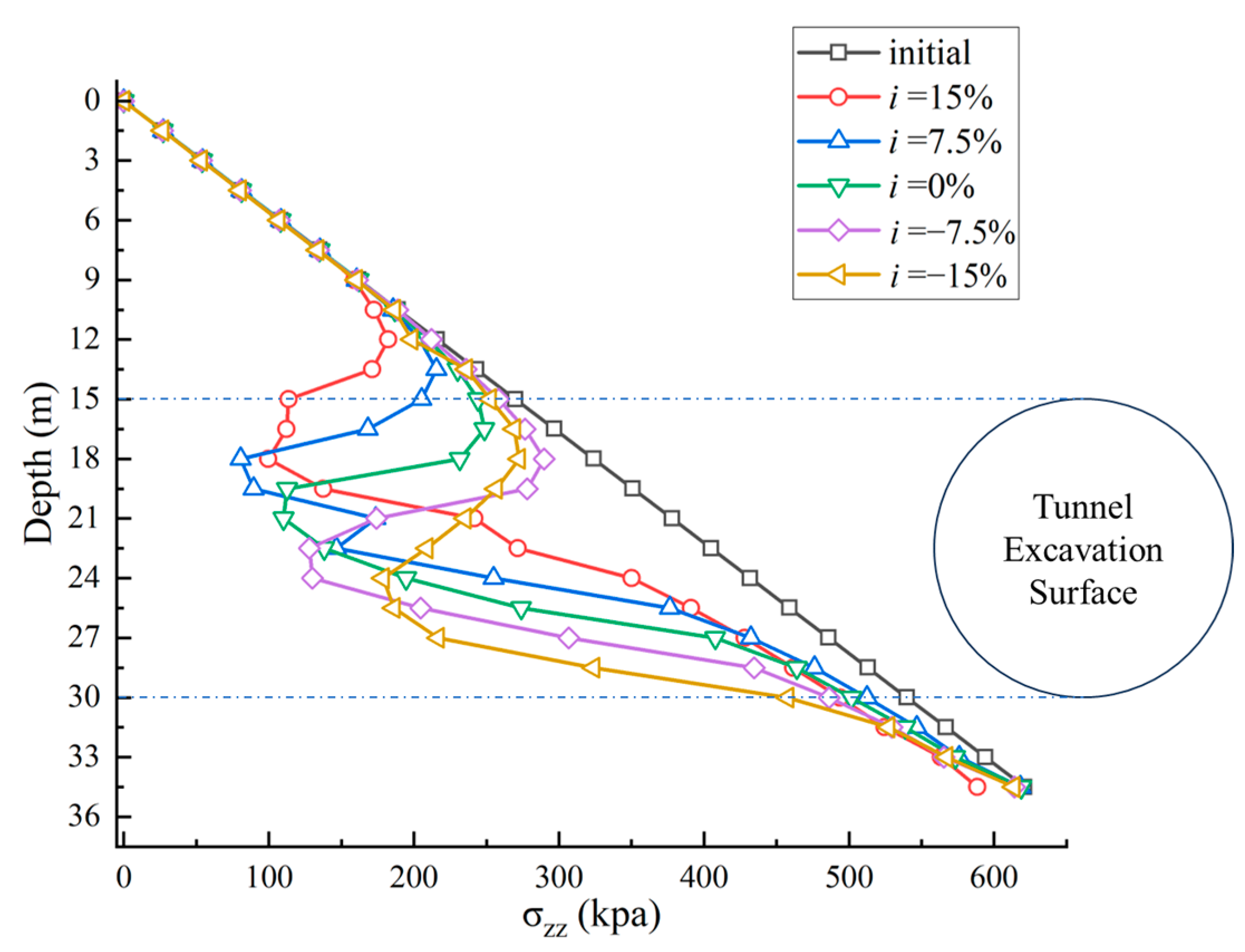
3.4. Results and Discussion of Vertical Stresses in Numerical Simulation Results
4. Conclusions and Future Work
4.1. Conclusions
4.2. Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Han, J.; Wang, J.; Cheng, C.; Zhang, C.; Liang, E.; Wang, Z.; Song, J.-J.; Leem, J. Mechanical Response and Parametric Analysis of a Deep Excavation Structure Overlying an Existing Subway Station: A Case Study of the Beijing Subway Station Expansion. Front. Earth Sci. 2023, 10, 1079837. [Google Scholar] [CrossRef]
- Jia, P.; Zhao, W.; Khoshghalb, A.; Ni, P.; Jiang, B.; Chen, Y.; Li, S. A New Model to Predict Ground Surface Settlement Induced by Jacked Pipes with Flanges. Tunn. Undergr. Space Technol. 2020, 98, 103330. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, M.; Li, J.; Wang, Z.; Tong, J.; Liu, D. Safety Factor Analysis of a Tunnel Face with an Unsupported Span in Cohesive-Frictional Soils. Comput. Geotech. 2020, 117, 103221. [Google Scholar] [CrossRef]
- Chen, Y.; Tan, L.; Xiao, N.; Liu, K.; Jia, P.; Zhang, W. The Hydro-Mechanical Characteristics and Micro-Structure of Loess Enhanced by Microbially Induced Carbonate Precipitation. Geomech. Energy Environ. 2023, 34, 100469. [Google Scholar] [CrossRef]
- Qarmout, M.; König, D.; Gussmann, P.; Thewes, M.; Schanz, T. Tunnel Face Stability Analysis Using Kinematical Element Method. Tunn. Undergr. Space Technol. 2019, 85, 354–367. [Google Scholar] [CrossRef]
- De Buhan, P.; Cuvillier, A.; Dormieux, L.; Maghous, S. Face Stability of Shallow Circular Tunnels Driven under the Water Table: A Numerical Analysis. Int. J. Numer. Anal. Methods Geomech. 1999, 23, 79–95. [Google Scholar] [CrossRef]
- Zou, J.; Chen, G.; Qian, Z. Tunnel Face Stability in Cohesion-Frictional Soils Considering the Soil Arching Effect by Improved Failure Models. Comput. Geotech. 2019, 106, 1–17. [Google Scholar] [CrossRef]
- Han, K.; Wang, L.; Su, D.; Hong, C.; Chen, X.; Lin, X.-T. An Analytical Model for Face Stability of Tunnels Traversing the Fault Fracture Zone with High Hydraulic Pressure. Comput. Geotech. 2021, 140, 104467. [Google Scholar] [CrossRef]
- Dias, D.; Janin, J.-P.; Soubra, A.-H.; Kastner, R. Three-Dimensional Face Stability Analysis of Circular Tunnels by Numerical Simulations. In GeoCongress 2008: Characterization, Monitoring, and Modeling of GeoSystems; American Society of Civil Engineers: Reston, VI, USA, 2012; pp. 886–893. [Google Scholar] [CrossRef]
- Li, P.; Zou, H.; Wang, F.; Xiong, H. An Analytical Mechanism of Limit Support Pressure on Cutting Face for Deep Tunnels in the Sand. Comput. Geotech. 2020, 119, 103372. [Google Scholar] [CrossRef]
- Fernández, F.; Rojas, J.E.G.; Vargas, E.A.; Velloso, R.Q.; Dias, D. Three-Dimensional Face Stability Analysis of Shallow Tunnels Using Numerical Limit Analysis and Material Point Method. Tunn. Undergr. Space Technol. 2021, 112, 103904. [Google Scholar] [CrossRef]
- Chen, R.P.; Tang, L.J.; Ling, D.S.; Chen, Y.M. Face Stability Analysis of Shallow Shield Tunnels in Dry Sandy Ground Using the Discrete Element Method. Comput. Geotech. 2011, 38, 187–195. [Google Scholar] [CrossRef]
- Wang, J.; Feng, K.; Wang, Y.; Lin, G.; He, C. Soil Disturbance Induced by EPB Shield Tunnelling in Multilayered Ground with Soft Sand Lying on Hard Rock: A Model Test and DEM Study. Tunn. Undergr. Space Technol. 2022, 130, 104738. [Google Scholar] [CrossRef]
- Idinger, G.; Aklik, P.; Wu, W.; Borja, R.I. Centrifuge Model Test on the Face Stability of Shallow Tunnel. Acta Geotech. 2011, 6, 105–117. [Google Scholar] [CrossRef]
- Ahmed, M.; Iskander, M. Evaluation of Tunnel Face Stability by Transparent Soil Models. Tunn. Undergr. Space Technol. 2012, 27, 101–110. [Google Scholar] [CrossRef]
- Senent Domínguez, S.; García Luna, R.; Sánchez Lázaro, M.; Jiménez Rodriguez, R. Tunnel Face Stability Laboratory Tests in Sand Considering Surface Settlements. In Proceedings of the XVII European Conference on Soil Mechanics and Geotechnical Engineering|XVII European Conference on Soil Mechanics and Geotechnical Engineering, Reykjavík, Iceland, 1–6 of September 2019; E.T.S.I. Caminos, Canales y Puertos (UPM): Reykjavík, Iceland, 2019. [Google Scholar]
- Kirsch, A. Experimental Investigation of the Face Stability of Shallow Tunnels in Sand. Acta Geotech. 2010, 5, 43–62. [Google Scholar] [CrossRef]
- Huo, M.; Chen, W.; Yuan, J.; Wu, G.; Li, Y.; Liu, Y. Experimental Investigation and Limit Analysis of Shield Tunnel Face Failure Mechanism in Sand. Undergr. Space 2025, 22, 137–152. [Google Scholar] [CrossRef]
- Xiang, Y.; Liu, H.; Zhang, W.; Chu, J.; Zhou, D.; Xiao, Y. Application of Transparent Soil Model Test and DEM Simulation in Study of Tunnel Failure Mechanism. Tunn. Undergr. Space Technol. 2018, 74, 178–184. [Google Scholar] [CrossRef]
- Ma, S.; Duan, Z.; Huang, Z.; Liu, Y.; Shao, Y. Study on the Stability of Shield Tunnel Face in Clay and Clay-Gravel Stratum through Large-Scale Physical Model Tests with Transparent Soil. Tunn. Undergr. Space Technol. 2022, 119, 104199. [Google Scholar] [CrossRef]
- Zhang, W.; Zhong, H.; Xiang, Y.; Wu, D.; Zeng, Z.; Zhang, Y. Visualization and Digitization of Model Tunnel Deformation via Transparent Soil Testing Technique. Undergr. Space 2022, 7, 564–576. [Google Scholar] [CrossRef]
- Ads, A.; Iskander, M.; Bless, S.; Omidvar, M. Visualizing the Effect of Fin Length on Torpedo Anchor Penetration and Pullout Using a Transparent Soil. Ocean. Eng. 2020, 216, 108021. [Google Scholar] [CrossRef]
- Mollon, G.; Dias, D.; Soubra, A.H. Rotational failure mechanism for the face stability analysis of tunnels driven by a pressurized shield. Int. J. Numer. Anal. MethodsGeomech. 2011, 35, 1363–1388. [Google Scholar]
- Cheng, C.; Jia, P.; Zhao, W.; Ni, P.; Bai, Q.; Wang, Z.; Lu, B. Experimental and Analytical Study of Shield Tunnel Face in Dense Sand Strata Considering Different Longitudinal Inclination. Tunn. Undergr. Space Technol. 2021, 113, 103950. [Google Scholar] [CrossRef]
- Weng, X.; Sun, Y.; Yan, B.; Niu, H.; Lin, R.; Zhou, S. Centrifuge Testing and Numerical Modeling of Tunnel Face Stability Considering Longitudinal Slope Angle and Steady State Seepage in Soft Clay. Tunn. Undergr. Space Technol. 2020, 101, 103406. [Google Scholar] [CrossRef]
- Chen, C.N.; Huang, W.-Y.; Tseng, C.-T. Stress Redistribution and Ground Arch Development during Tunneling. Tunn. Undergr. Space Technol. 2011, 26, 228–235. [Google Scholar] [CrossRef]


| Material Type | Density γ (g/cm3) | Friction Angle φ (°) | Cohesive Force c (kPa) |
|---|---|---|---|
| Transparent soil | 1.8 | 20 | 5 |
| No. | Setback Amount S (mm) | i (%) |
|---|---|---|
| 1-1 | 3 mm | −15% |
| 1-2 | 0% | |
| 1-3 | 15% | |
| 2-1 | 6 mm | −15% |
| 2-2 | 0% | |
| 2-3 | 15% | |
| 3-1 | 9 mm | −15% |
| 3-2 | 0% | |
| 3-3 | 15% |
| Material Type | Density γ (kN/m3) | Dynamic Young’s Modulus E (GPa) | Poisson’s Ratio μ | Friction Angle φ (°) | Cohesive Force c (kPa) |
|---|---|---|---|---|---|
| soil | 18 | 0.05 | 0.33 | 20 | 5 |
| Grout | 25 | 20 | 0.2 | - | - |
| Lining | 25 | 0.015 | 0.2 | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, M.; Gu, S. Stability Analysis of the Longitudinal Slope Linear Shield Tunnel Excavation Face. Appl. Sci. 2025, 15, 4083. https://doi.org/10.3390/app15084083
Zhang M, Gu S. Stability Analysis of the Longitudinal Slope Linear Shield Tunnel Excavation Face. Applied Sciences. 2025; 15(8):4083. https://doi.org/10.3390/app15084083
Chicago/Turabian StyleZhang, Mengxi, and Shengwei Gu. 2025. "Stability Analysis of the Longitudinal Slope Linear Shield Tunnel Excavation Face" Applied Sciences 15, no. 8: 4083. https://doi.org/10.3390/app15084083
APA StyleZhang, M., & Gu, S. (2025). Stability Analysis of the Longitudinal Slope Linear Shield Tunnel Excavation Face. Applied Sciences, 15(8), 4083. https://doi.org/10.3390/app15084083





