Optimization of Adhesive Joint Design in Timber–Glass Systems: Enhancing Structural Performance with Primer Treatment
Abstract
1. Introduction
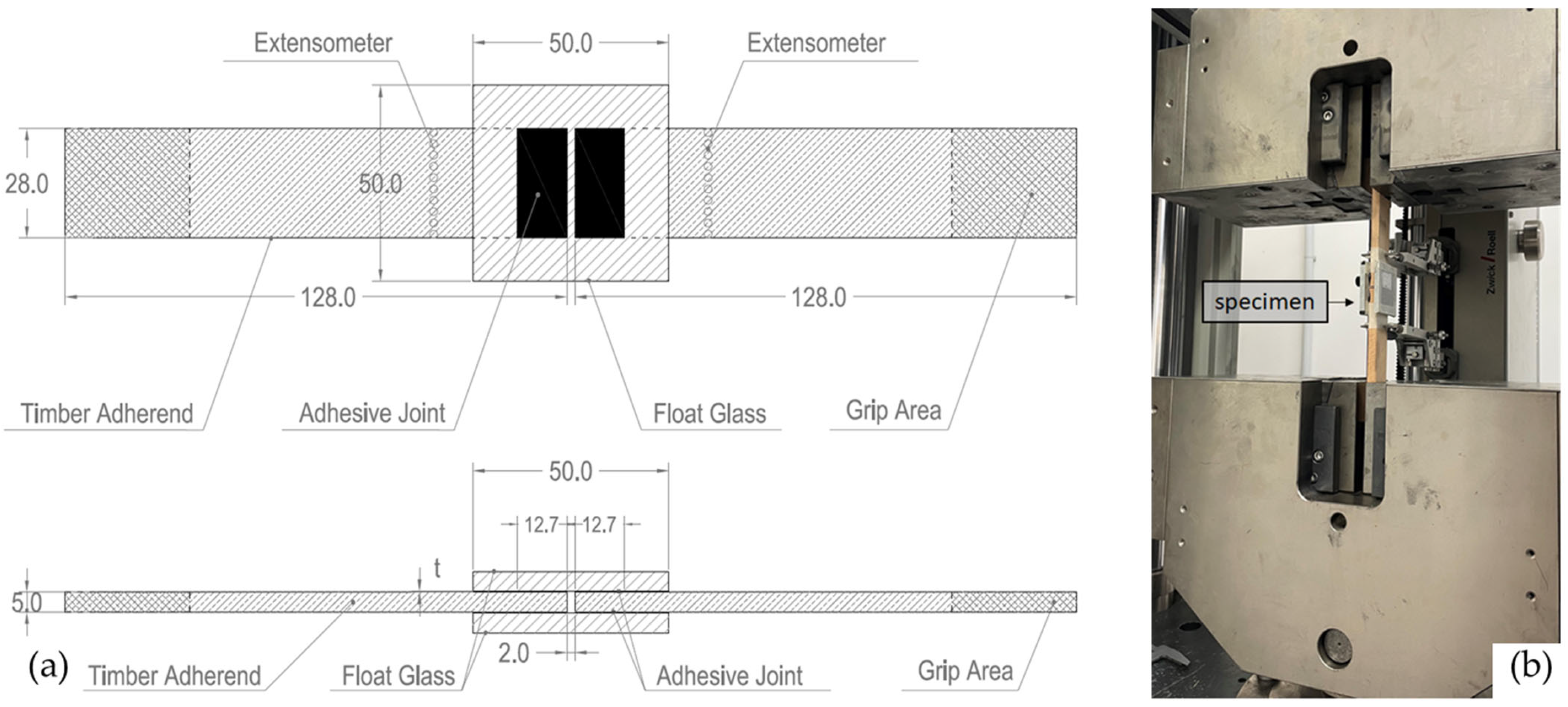
2. Materials and Methods
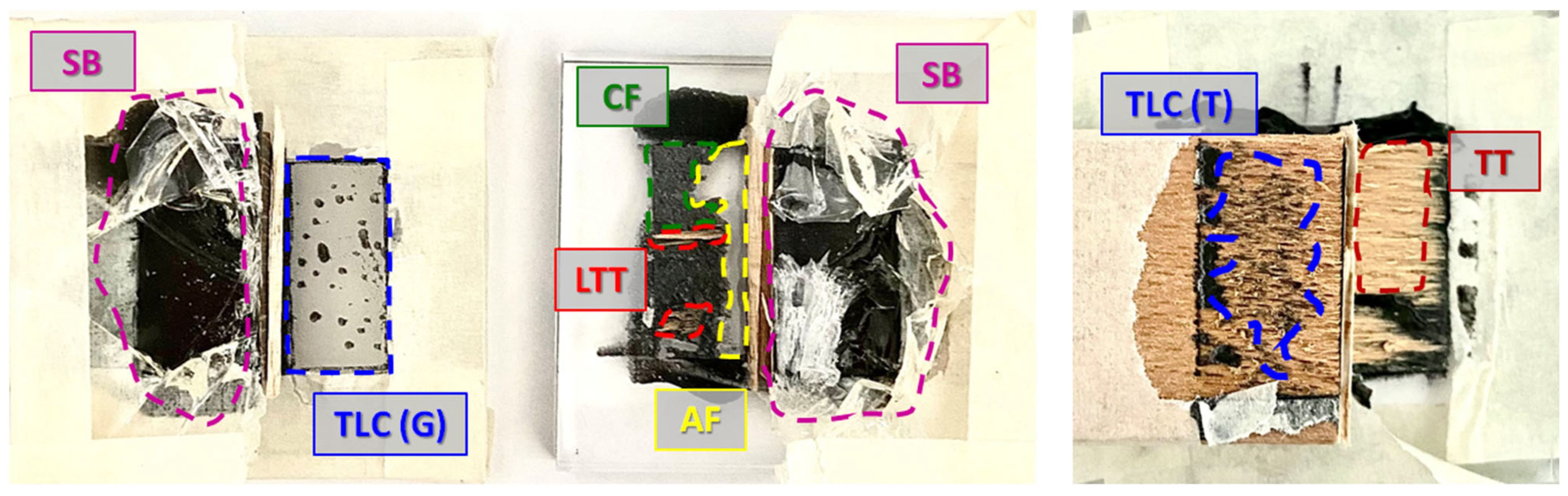
- Adhesive Failure (AF): Failure occurring at the interface between the adhesive and the adherend when the resistance of the interface (adhesion strength) is less than that of the adherend.
- Cohesive Failure (CF): Failure occurring within the adhesive; therefore, the latter is present on both fracture surfaces.
- Thin-Layer Cohesive Failure (TLC): Cohesive failure occurring very close to the adhesive—substrate interface, characterized by a “light dusting” of the adhesive on one substrate surface and a thick layer of the adhesive left on the other.
- Timber-Tear Failure (TT): Failure occurring exclusively within the timber component, characterized by the presence of timber fibers on both ruptured surfaces.
- Light-Timber-Tear Failure (LTT): Timber failure characterized by a thin layer of timber fibers visible on the adhesive.
- Stock-Break Failure (SB): Breaking of the glass substrate outside the adhesively bonded joint region, often occurring near it.
- Mixed Failure (MF): Any combination of two or more of the previous classes of failure modes described.
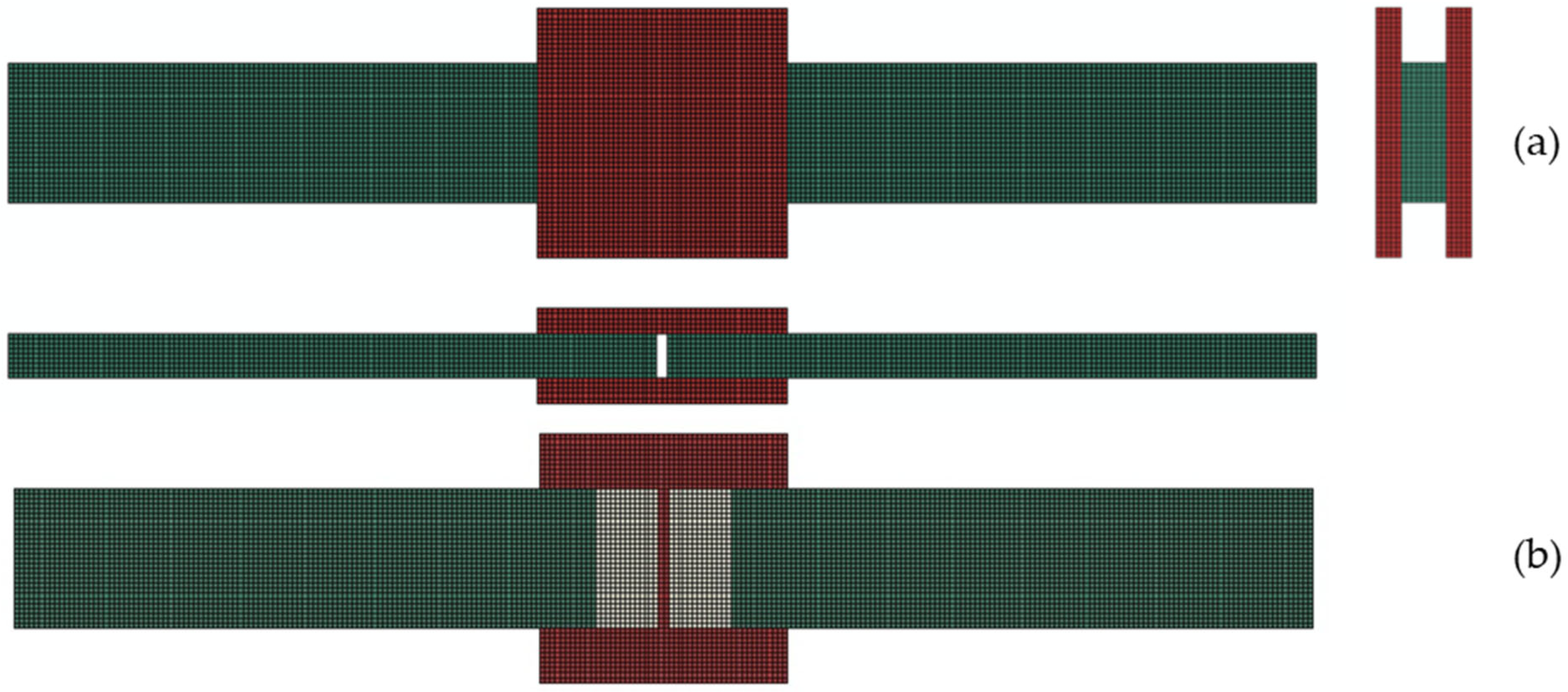
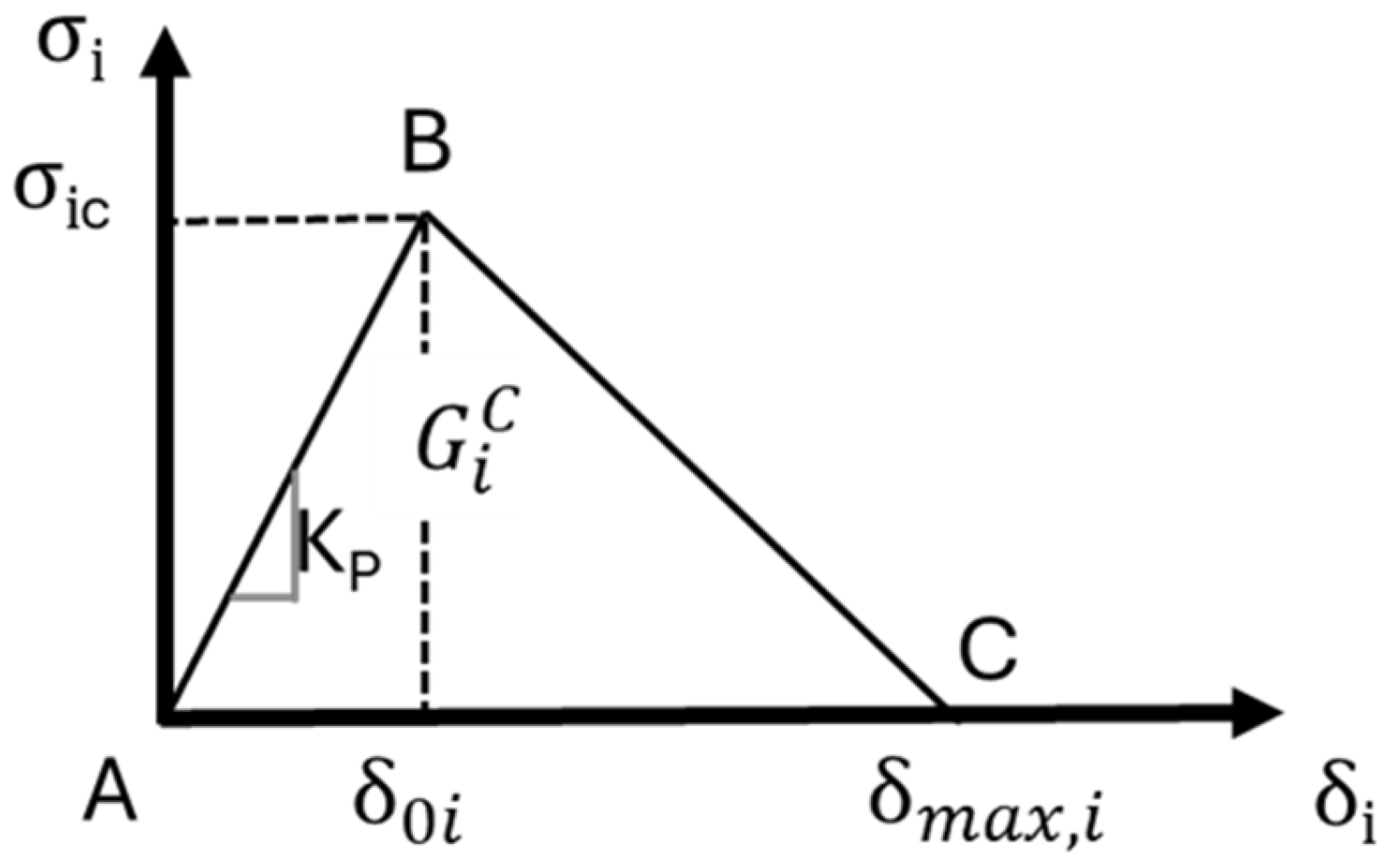
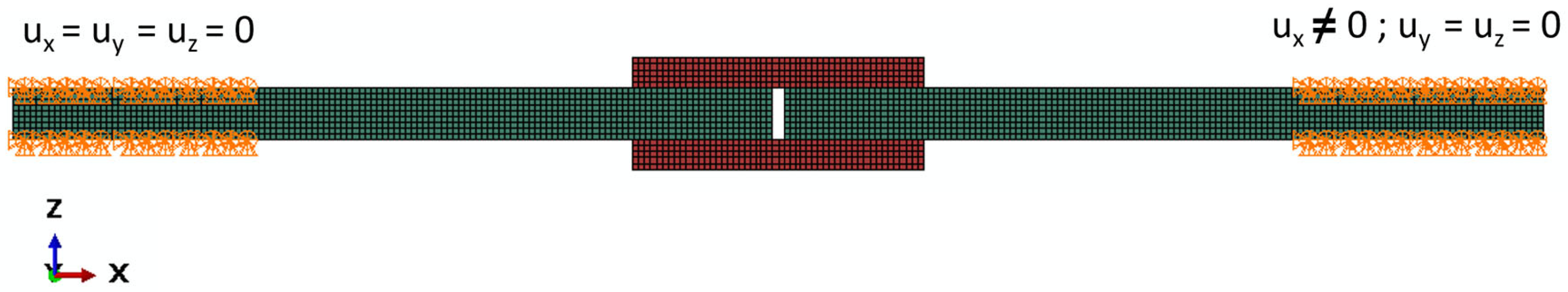
3. FE Model
4. Results and Discussion
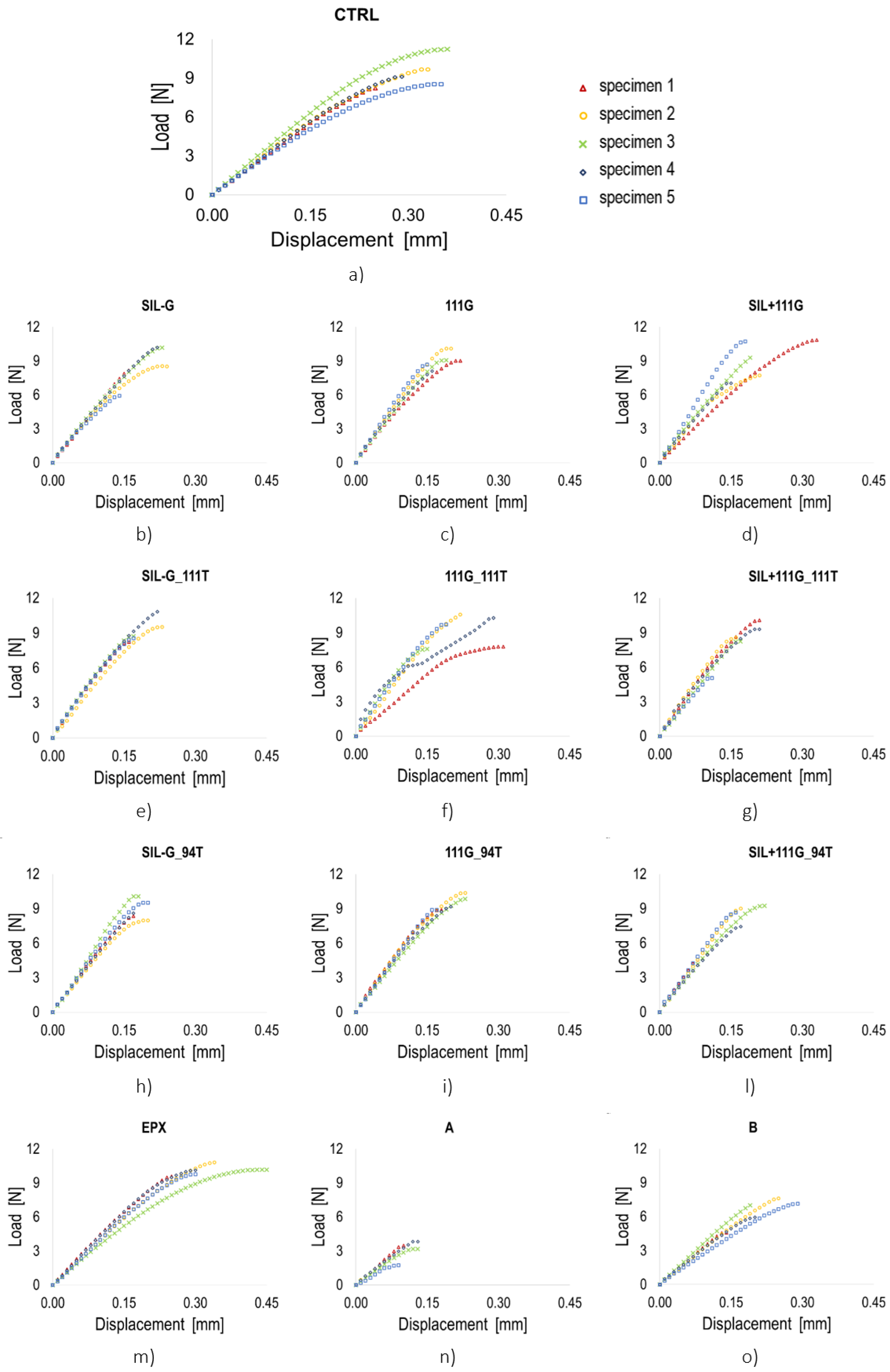
4.1. Experimental Results
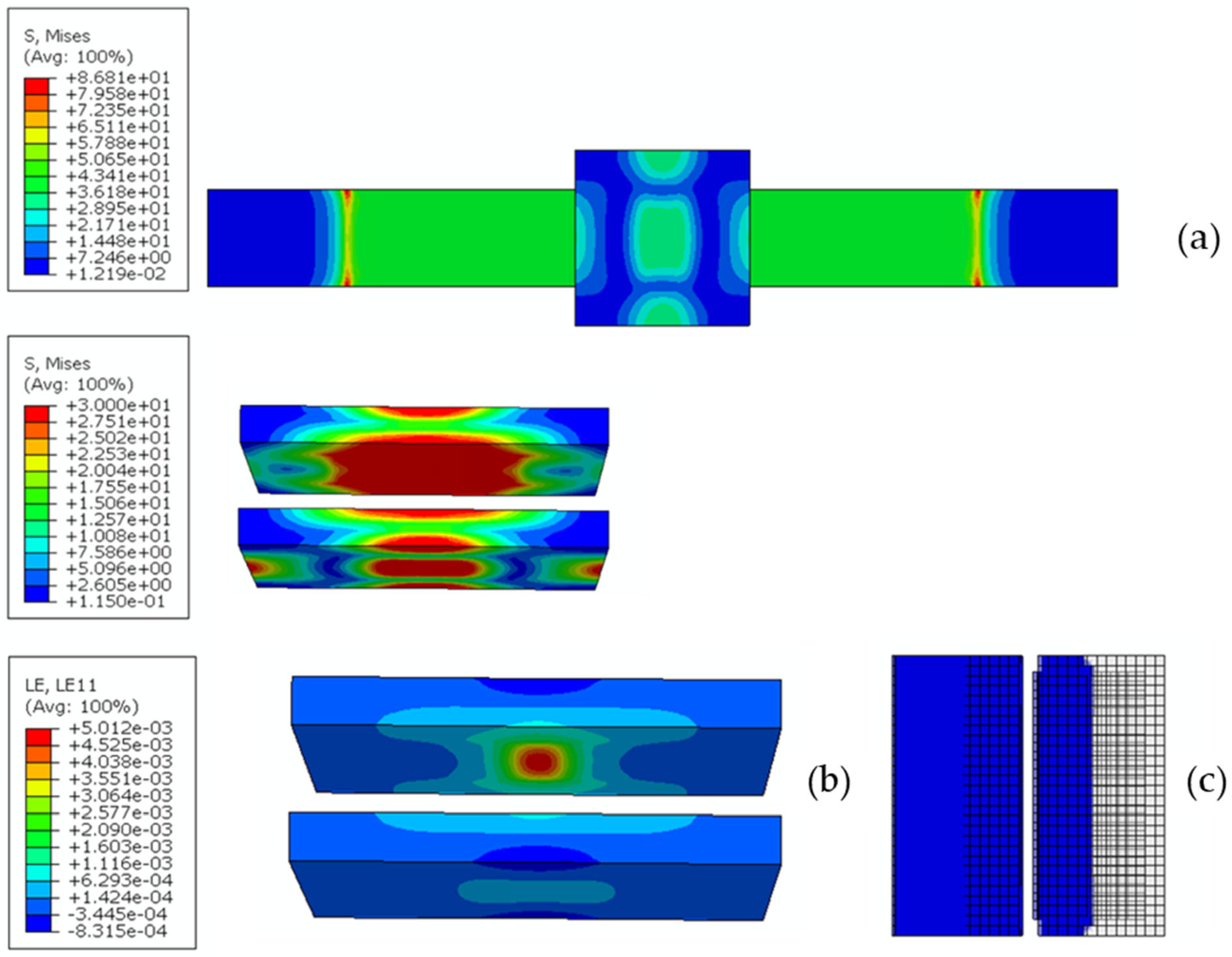
4.2. Numerical Results and Experimental Data Comparison
5. Conclusions
- (1)
- The pre-treatments on the glass and/or timber side of the bonded joint reduce the maximum displacement, shear stress, and shear strain. They may also contribute to increase the maximum failure load of the joint, but this behavior is not observed for all the tested series; in particular, adhesion promoters (111 and 94) seem to have a better effect than a moisture inhibitor (Silane) in this respect. As a consequence of the decrease in the ultimate displacement, all pre-treated series have higher stiffness than the untreated ones. These treatments, in fact, improve surface adhesion at the adherend—adhesive interface, which in turn enhances load transmission between the adherents up to the maximum permissible stress of the glass, before the adhesive failure (AF) occurs. This aspect of stiffness is relevant according to the different fields of application (i.e., depending on the displacements that the joint can bear, a combination is more or less suitable for a specific purpose).
- (2)
- The behavior of the painted joint strongly depends on the type of paint used on the glass: a paint of the same nature of the adhesive (i.e., epoxy paint) can increase the ultimate load but has no significant effect on the remaining considered parameters (as summarized in Table 8), nor on the type of failure mechanisms. Conversely, the use of an acrylic paint causes a worsening of the overall mechanical behavior and shifts the collapse mode from glass failure to interface failure.
- (3)
- According to the results of the numerical modeling, it can be concluded that the simulation appears to be more representative of all those series where adhesive promoters are used. This aspect can be attributed to the fact that the usage of adhesive promoters reduces the effects on the joint structural behavior of the uncertainties affecting all specimens because of the manufacturing process, which cannot be easily included in the modeling.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vallée, T.; Tannert, T.; Fecht, S. Adhesively bonded connections in the context of timber engineering—A Review. J. Adhes. 2017, 93, 257–287. [Google Scholar] [CrossRef]
- Martens, K.; Caspeele, R.; Belis, J. Development of composite glass beams—A review. Eng. Struct. 2015, 101, 1–15. [Google Scholar] [CrossRef]
- Tannert, T.; Gerber, A.; Vallee, T. Hybrid adhesively bonded timber-concrete-composite floors. Int. J. Adhes. Adhes. 2020, 97, 102490. [Google Scholar] [CrossRef]
- MacHalická, K.; Horčičková, I.; Eliášová, M. Shear adhesive connections for glass structures. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2015; Volume 96. [Google Scholar] [CrossRef]
- Freytag, B. Glass-concrete composite technology. Struct. Eng. Int. J. Int. Assoc. Bridge Struct. Eng. 2004, 14, 111–117. [Google Scholar] [CrossRef]
- Speranzini, E.; Agnetti, S. Strengthening of glass beams with steel reinforced polymer (SRP). Compos. B Eng. 2014, 67, 280–289. [Google Scholar] [CrossRef]
- Hildebrand, J.; Werner, F. Glass-plastic hybrid construction. In Advances in Engineering Structures, Mechanics & Construction; Springer: Dordrecht, The Netherlands, 2006. [Google Scholar]
- Adams, R.D.; Peppiatt, N.A. Stress analysis of adhesive-bonded lap joints. J. Strain Anal. 1974, 9, 158–196. [Google Scholar] [CrossRef]
- Machalická, K.; Vokáč, M.; Eliášová, M. Influence of artificial aging on structural adhesive connections for façade applications. Int. J. Adhes. Adhes. 2018, 83, 168–177. [Google Scholar] [CrossRef]
- Jasiūnas, L.; Peck, G.; Bridžiuvienė, D.; Miknius, L. Mechanical, thermal properties and stability of high renewable content liquefied residual biomass derived bio-polyurethane wood adhesives. Int. J. Adhes. Adhes. 2020, 101, 102618. [Google Scholar] [CrossRef]
- Bartkowiak, M.; Czech, Z.; Mozelewska, K.; Nowak, M. Influence of thermal reactive crosslinking agents on the tack, peel adhesion, and shear strength of acrylic pressure-sensitive adhesives. Polym. Test. 2020, 90, 106603. [Google Scholar] [CrossRef]
- Banea, M.D.; Da Silva, L.F.M.; Campilho, R.D.S.G. Effect of temperature on the shear strength of aluminium single lap bonded joints for high temperature applications. J. Adhes. Sci. Technol. 2014, 28, 1367–1381. [Google Scholar] [CrossRef]
- Pethrick, R.A. Design and ageing of adhesives for structural adhesive bonding—A review. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2015, 229, 349–379. [Google Scholar] [CrossRef]
- Jeevi, G.; Nayak, S.K.; Abdul Kader, M. Review on adhesive joints and their application in hybrid composite structures. J. Adhes. Sci. Technol. 2019, 33, 1497–1520. [Google Scholar] [CrossRef]
- Pequeno, J.M.; Cruz, P.J.S. Structural Timber-Glass Linear System: Characterization & Architectural Potentialities. In Proceedings of the Glass Performance Days 2009—11th International Conference, Tampere, Finland, 12–15 June 2009; pp. 344–348. [Google Scholar]
- Eriksson, J.; Ludvigsson, M.; Dorn, M.; Enquist, B.; Serrano, E. Load bearing timber glass composites—A WoodWisdom-Net project for innovative building system. In COST Action TU0905 Mid-Term Conference on Structural Glass; CRC Press: Boca Raton, FL, USA, 2013; p. 269. [Google Scholar] [CrossRef]
- Cruz, P.J.S.; Pequeno, J. Timber-Glass Composite Beams: Mechanical Behaviour & Architectural Solutions. Challenging Glass 2008, 22, 23. [Google Scholar]
- Vallee, T.; Grunwald, C.; Milchert, L.; Fecht, S. Design and dimensioning of a complex timber-glass hybrid structure: The IFAM pedestrian bridge. Glass Struct. Eng. 2016, 1, 3–18. [Google Scholar] [CrossRef]
- Blyberg, L.; Lang, M.; Lundstedt, K.; Schander, M.; Serrano, E.; Silfverhielm, M.; Stålhandske, C. Glass, timber and adhesive joints—Innovative load bearing building components. Constr. Build. Mater. 2014, 55, 470–478. [Google Scholar] [CrossRef]
- Marchione, F.; Agliata, R.; Munafò, P. Application of adhesive technology to a new type of glazed panel for curtain walls with an integrated frame. TEMA 2022, 8, 108–116. [Google Scholar] [CrossRef]
- Blyberg, L.; Serrano, E.; Enquist, B.; Sterley, M. Adhesive joints for structural timber/glass applications: Experimental testing and evaluation methods. Int. J. Adhes. Adhes. 2012, 35, 76–87. [Google Scholar] [CrossRef]
- Kozłowski, M.; Kadela, M.; Hulimka, J. Numerical Investigation of Structural Behaviour of Timber-Glass Composite Beams. Procedia Eng. 2016, 161, 990–1000. [Google Scholar] [CrossRef]
- Rudawska, A. Adhesive joint strength of hybrid assemblies: Titanium sheet-composites and aluminium sheet-composites Experimental and numerical verification. Int. J. Adhes. Adhes. 2010, 30, 574–582. [Google Scholar] [CrossRef]
- Van Lancker, B.; Dispersyn, J.; De Corte, W.; Belis, J. Durability of adhesive glass-metal connections for structural applications. Eng. Struct. 2016, 126, 237–251. [Google Scholar] [CrossRef]
- Sousa, J.M.; Correia, J.R.; Firmo, J.P.; Cabral-Fonseca, S.; Gonilha, J. Effects of thermal cycles on adhesively bonded joints between pultruded GFRP adherends. Compos. Struct. 2018, 202, 518–529. [Google Scholar] [CrossRef]
- Schneider, B.; Beber, V.C.; Schweer, J.; Brede, M.; Mayer, B. An experimental investigation of the fatigue damage behaviour of adhesively bonded joints under the combined effect of variable amplitude stress and temperature variation. Int. J. Adhes. Adhes. 2018, 83, 41–49. [Google Scholar] [CrossRef]
- Min, J.; Wan, H.; Carlson, B.E.; Lin, J.; Sun, C. Application of laser ablation in adhesive bonding of metallic materials: A review. Opt. Laser Technol. 2020, 128, 106188. [Google Scholar] [CrossRef]
- Žigon, J.; Kovač, J.; Zaplotnik, R.; Saražin, J.; Šernek, M.; Petrič, M.; Dahle, S. Enhancement of strength of adhesive bond between wood and metal using atmospheric plasma treatment. Cellulose 2020, 27, 6411–6424. [Google Scholar] [CrossRef]
- Worpenberg, C.; Stiesch, M.; Eisenburger, M.; Breidenstein, B.; Busemann, S.; Greuling, A. The effect of surface treatments on the adhesive bond in all-ceramic dental crowns using four-point bending and dynamic loading tests. J. Mech. Behav. Biomed. Mater. 2023, 139, 105686. [Google Scholar] [CrossRef]
- Guo, L.; Liu, J.; Xia, H.; Li, X.; Zhang, X.; Yang, H. Effects of surface treatment and adhesive thickness on the shear strength of precision bonded joints. Polym. Test. 2021, 94, 107063. [Google Scholar] [CrossRef]
- Zou, X.; Liu, L.; Chen, T.; Wu, L.; Chen, K.; Kong, L.; Wang, M. Laser surface treatment to enhance the adhesive bonding between steel and CFRP: Effect of laser spot overlapping and pulse fluence. Opt. Laser Technol. 2023, 159, 109002. [Google Scholar] [CrossRef]
- Consiglio Nazionale Delle Ricerche (CNR). CNR-DT 210/2013—Istruzioni per la Progettazione, l’Esecuzione ed il Controllo di Costruzioni con Elementi Strutturali di Vetro; Consiglio Nazionale Delle Ricerche (CNR): Rome, Italy, 2013. [Google Scholar]
- ISO 13061-6; Physical and Mechanical Properties of Wood—Test Methods for Small Clear Wood Specimens—Part 6: Determination of Ultimate Tensile Stress Parallel to Grain. International Standard ISO: Geneva, Switzerland, 2014.
- Marchione, F.; Chiappini, G.; Munafò, P. Effect of temperature and relative humidity on the shear performance of double-lap adhesive joints between steel and glass adherends. J. Build. Eng. 2022, 45, 103546. [Google Scholar] [CrossRef]
- 3MTM. Scotch-WeldTMStructural Adhesive 7240 B/A FR Preliminary Product Data Sheet. 2018. Available online: https://multimedia.3m.com/mws/media/1884344O/3m-scotch-weld-structural-adhesive-7240-ba-fr-product-data-sheet-english.pdf (accessed on 17 January 2025).
- Marchione, F.; Munafò, P. Experimental investigation on timber-glass double-lap adhesive joints. Int. J. Adhes. Adhes. 2021, 106, 102818. [Google Scholar] [CrossRef]
- ASTM, D3528-96; Standard Test Method for Strength Properties of Double Lap Shear Adhesive Joints by Tension Loading. ASTM International: West Conshohocken, PA, USA, 2016.
- ISO 4587:2003; Adhesives—Determination of Tensile Lap-Shear Strength of Rigid-to-Rigid Bonded Assemblies. ISO: Geneva, Switzerland, 2003.
- ASTM, D5573; Standard Practice for Classifying Failure Modes in Fiber-Reinforced-Plastic (FRP) Joints 1. ASTM International: West Conshohocken, PA, USA, 1999.
- Lamanna, G.; Perrella, M.; Opran, C.G. Numerical and Experimental Investigation on the Influence of Tightening in a Hybrid Single Lap Joint. Macromol. Symp. 2021, 396, 2100010. [Google Scholar] [CrossRef]
- Lamanna, G.; Opran, C.G. Numerical Characterization of Pretensioning of a Hybrid Joint Under Longitudinal Load. Macromol. Symp. 2021, 396, 2100009. [Google Scholar] [CrossRef]
- Lamanna, G.; Ion, S.M.; Opran, C.G. Flexural Effects Evaluation on Hybrid Joints Under Uniaxial Tensile Load. Macromol. Symp. 2021, 396, 2100007. [Google Scholar] [CrossRef]
- Caputo, F.; Lamanna, G.; Soprano, A. Effects of tolerances on the structural behavior of a bolted hybrid joint. Key Eng. Mater. 2012, 488–489, 565–568. [Google Scholar] [CrossRef]
- Dassault Systèmes. Abaqus/CAE User Manual v. 2021; Dassault Systèmes: Paris, France, 2021. [Google Scholar]
- Long, H.; Ma, H.; Wei, Y.; Liua, Y. Investigating the scale effect in adhesive joint mechanics using gradient theories. Int. J. Mech. Sci. 2021, 198, 106348. [Google Scholar] [CrossRef]


| Glass [32] | Beechwood [33] | |||||
|---|---|---|---|---|---|---|
| Thermal Coeff. of Expansion | Young Modulus | Tensile Strength | Density | Moisture Content | Young Modulus | Tensile Strength |
| α (°C−1) | E (GPa) | σR (MPa) | ρ (kg/m3) | % | E (GPa) | σR (MPa) |
| 9 × 10−6 | 75 | 30 | 720 | 15 | 1.5 | 90 |
| Chemical nature | two-part | toughened epoxy base + modified amine accelerator | |
| Viscosity | - | thixotropic | |
| Work life | Wl | (min) | 45 |
| Application temperature | At | (°C) | 15 ÷ 30 |
| Glass transition temperature | Tg | (°C) | 66.87 |
| Service temperature | St | (°C) | −40 ÷ 80 |
| Shear strength | τ | (MPa) | 6.2–24.3 * |
| Young modulus in compression | Et | (MPa) | 3500–4000 |
| Use | - | semi-structural | |
| Epoxy-Based | Acrylic-Based—Type A | Acrylic-Based—Type B | |
|---|---|---|---|
| Color | silver | black | black |
| Type | 2-compound pearlescent paint for glass | bilayer is 100% solvent-based, high coverage | High Solids, solvent-based 2-component, satin finish |
| Composition | 1 part paint + 0.6 diluent 1 + 0.45 catalyst 2 | 1 part paint + 0.5 diluent 3 | 1 part paint + 0.2 catalyst 4 |
| Auxiliary components | 1 DT 810 Epoxy Thinner by Visa Colors 2 AM 85 Glossy Hardener | 3 disolvente acrìlico RU RAC medio | 4 Catalizador DCP9156, high adherence, by Racing colors (included with the paint) |
| Silane | 111 | 94 | ||
|---|---|---|---|---|
| Main ingredient | (% by Weight) | isopropyl alcohol (80–95) * | propan-2-ol (98–100) | cyclohexane (30–60) * |
| Boiling point | (°C) | 82.2 | 82.4 | 76.7 |
| Flashpoint | (°C) | 11.7 | 11 | −17.2 |
| Density | (g/mL) | 0.80 | 0.79 | 0.82 |
| Vapor Pressure | (mmHg) | 43 (at 25 °C) | 330 (at 20 °C) | 68 (at 20 °C) |
| Relative Vapor Density | 2.07 | 2.1 | no data available | |
| CTRL | Untreated |
| SIL-G | Silane on the glass side |
| 111G | Primer 111 on the glass side |
| SIL + 111G | Silane + Primer 111 on the glass side |
| SIL-G_111T | Silane on the glass side and Primer 111 on the timber side |
| 111G-111T | Primer 111 on the glass side and Primer 111 on the timber side |
| SIL + 111G_111T | Silane + Primer 111 on the glass side and Primer 111 on the timber side |
| SIL-G_94T | Silane on the glass side and Primer 94 on the timber side |
| 111G_94T | Primer 111 on the glass side and Primer 94 on the timber side |
| SIL + 111G_94T | Silane + Primer 111 on the glass side and Primer 94 on the timber side |
| EPX | Glass treated with epoxy paint |
| A | Glass treated with acrylic paint—type A |
| B | Glass treated with acrylic paint—type B |
| Longitudinal Young’s modulus | En | [MPa] | 4000 |
| Transversal Young’s moduli | Es = Et | [MPa] | 1350 |
| Nominal stress I mode | [MPa] | 42 | |
| Nominal stress II mode | [MPa] | 15 | |
| Nominal stress III mode | [MPa] | 15 | |
| Critical fracture energy, mode I | Jm−2 | 380 | |
| Critical fracture energy, mode II | Jm−2 | 190 | |
| Critical fracture energy, mode III | Jm−2 | 190 |
| Series | Specimen 1 | Specimen 2 | Specimen 3 | Specimen 4 | Specimen 5 |
|---|---|---|---|---|---|
| CTRL | SB + TLC (G) | SB | SB + AF (G) + CF + LTT | SB | SB + CF + LTT |
| SIL-G | SB | SB + TT + TLC (T) | SB + LTT + TLC (T) | SB + TT | TT + LTT + TLC (T/G) |
| 111G | SB + TT + TLC (G) | SB + TLC (T/G) + LTT | SB + TT + TLC (G) | SB | SB + TT |
| SIL + 111G | SB + LTT + TLC (T) | SB | SB | SB | SB + LTT + TLC (T) |
| SIL-G_111T | SB | SB + TLC (T) + LTT+ AF (G) | CF + LTT + TLC (T/G) | SB | SB + TT + LTT + TLC (T) |
| 111G_111T | SB + TT | AF (G) + LTT + TLC (T) | SB + LTT + TLC (T) | SB | SB + TLC (T) + LTT |
| SIL + 111G_111T | TT + LTT | SB + TT | SB + TT | LTT | SB + TLC (T) + LTT |
| SIL-G_94T | SB | TLC (T) + LTT | SB + TT + TLC (T) | SB | SB + TLC (T) + LTT |
| 111G_94T | TLC (G) + LTT | SB + LTT + TLC (T) | SB + LTT + TLC (T) | SB + LTT + TLC (T) | SB + TT |
| SIL + 111G_94T | SB + TLC (T) | SB + TT + LTT + TLC (T) | SB + LTT + TT + TLC (T) | SB + TLC (T) + LTT | SB + LTT + TLC (T) |
| EPX | SB | SB | SB + CF + LTT + TLC (T) | SB + LTT + TLC (T) | SB + LTT + TLC (T) |
| A | AF (G) + TLC (G) | AF (G) + TLC (G) | AF (G) | AF (G) | AF (G) |
| B | TLC (G) + AF (G) | TLC (G) + SB + AF (G) | TLC (G) + AF (G) | TLC (G) + AF (G) | TLC (G) + AF (G) |
| Series | Load max | Displ max | τmax | γmax | k | Failure Modes [39] |
|---|---|---|---|---|---|---|
| (kN) | (mm) | (MPa) | (-) | (kN/mm) | ||
| CTRL | 8.90 ± 0.64 | 0.31 ± 0.05 | 7.26 ± 0.50 | 1.09 ± 0.16 | 30.39 ± 3.66 | 3 MF + 2 SB |
| SIL-G | 8.61 ± 1.06 | 0.20 ± 0.05 | 6.67 ± 0.83 | 0.66 ± 0.16 | 44.62 ± 5.97 | 4 MF + 1 SB |
| 111G | 9.01 ± 0.73 | 0.18 ± 0.03 | 6.98 ± 0.57 | 0.62 ± 0.08 | 50.73 ± 5.97 | 4 MF + 1 SB |
| SIL + 111G | 9.13 ± 1.47 | 0.18 ± 0.03 | 7.07 ± 1.14 | 0.63 ± 0.04 | 46.21 ± 10.89 | 3 SB + 2 MF |
| SIL-G_111T | 9.18 ± 1.04 | 0.19 ± 0.03 | 7.11 ± 0.80 | 0.58 ± 0.09 | 49.88 ± 4.48 | 3 MF + 2 SB |
| 111G_111T | 9.20 ± 1.41 | 0.23 ± 0.07 | 7.13 ± 1.09 | 0.66 ± 0.21 | 43.19 ± 11.77 | 4 MF + 1 SB |
| SIL + 111G_111T | 9.06 ± 0.82 | 0.17 ± 0.02 | 7.02 ± 0.64 | 0.53 ± 0.07 | 50.27 ± 3.02 | 4 MF + 1 LTT |
| SIL-G_94T | 8.91 ± 0.87 | 0.18 ± 0.01 | 6.90 ± 0.67 | 0.60 ± 0.04 | 50.18 ± 6.09 | 3 MF + 2 SB |
| 111G_94T | 9.44 ± 0.65 | 0.20 ± 0.03 | 7.32 ± 0.50 | 0.68 ± 0.11 | 48.50 ± 4.85 | 5 MF |
| SIL + 111G_94T | 8.59 ± 0.81 | 0.18 ± 0.03 | 6.66 ± 0.63 | 0.63 ± 0.10 | 48.46 ± 6.14 | 5 MF |
| EPX | 10.10 ± 0.47 | 0.29 ± 0.04 | 7.82 ± 0.36 | 1.17 ± 0.30 | 34.87 ± 3.20 | 3 MF + 2 SB |
| A | 2.72 ± 1.12 | 0.09 ± 0.03 | 2.11 ± 0.87 | 0.30 ± 0.12 | 30.06 ± 6.21 | 3 AF (G) + 2 MF |
| B | 6.46 ± 0.72 | 0.21 ± 0.05 | 5.01 ± 0.55 | 0.70 ± 0.16 | 31.45 ± 4.75 | 5 MF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agliata, R.; De Luca, A.; Caputo, F.; Marchione, F.; Sepe, R.; Munafò, P. Optimization of Adhesive Joint Design in Timber–Glass Systems: Enhancing Structural Performance with Primer Treatment. Appl. Sci. 2025, 15, 1616. https://doi.org/10.3390/app15031616
Agliata R, De Luca A, Caputo F, Marchione F, Sepe R, Munafò P. Optimization of Adhesive Joint Design in Timber–Glass Systems: Enhancing Structural Performance with Primer Treatment. Applied Sciences. 2025; 15(3):1616. https://doi.org/10.3390/app15031616
Chicago/Turabian StyleAgliata, Rosa, Alessandro De Luca, Francesco Caputo, Francesco Marchione, Raffaele Sepe, and Placido Munafò. 2025. "Optimization of Adhesive Joint Design in Timber–Glass Systems: Enhancing Structural Performance with Primer Treatment" Applied Sciences 15, no. 3: 1616. https://doi.org/10.3390/app15031616
APA StyleAgliata, R., De Luca, A., Caputo, F., Marchione, F., Sepe, R., & Munafò, P. (2025). Optimization of Adhesive Joint Design in Timber–Glass Systems: Enhancing Structural Performance with Primer Treatment. Applied Sciences, 15(3), 1616. https://doi.org/10.3390/app15031616










