Nonlinear Analysis and Reliability Analysis of Multilink Mechanism Considering Mixed Clearance
Abstract
1. Introduction
2. Creation of Kinematic Pair Clearance Model
2.1. Assumptions About the Clearance Model
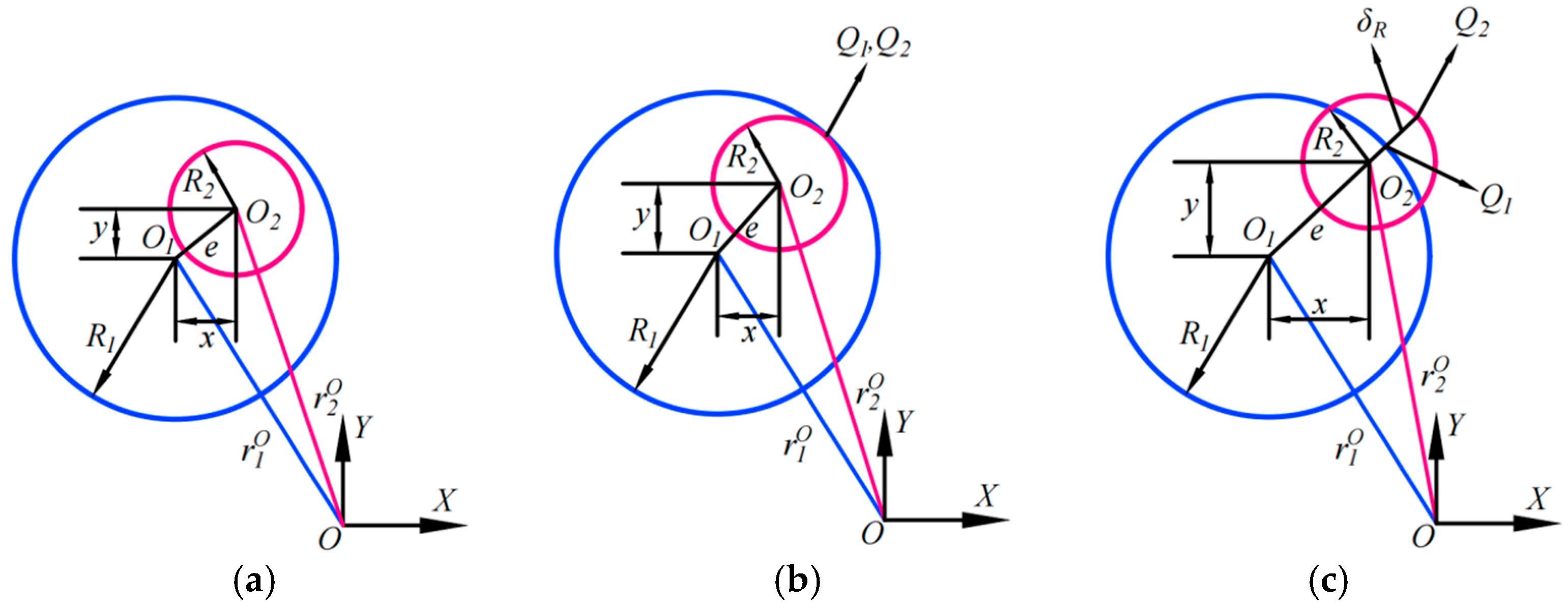
2.2. Creation of Kinematic Model for Revolute Joint Clearance
2.2.1. Collision Force Modeling in the Normal Direction
2.2.2. Constructing a Model for Tangential Friction
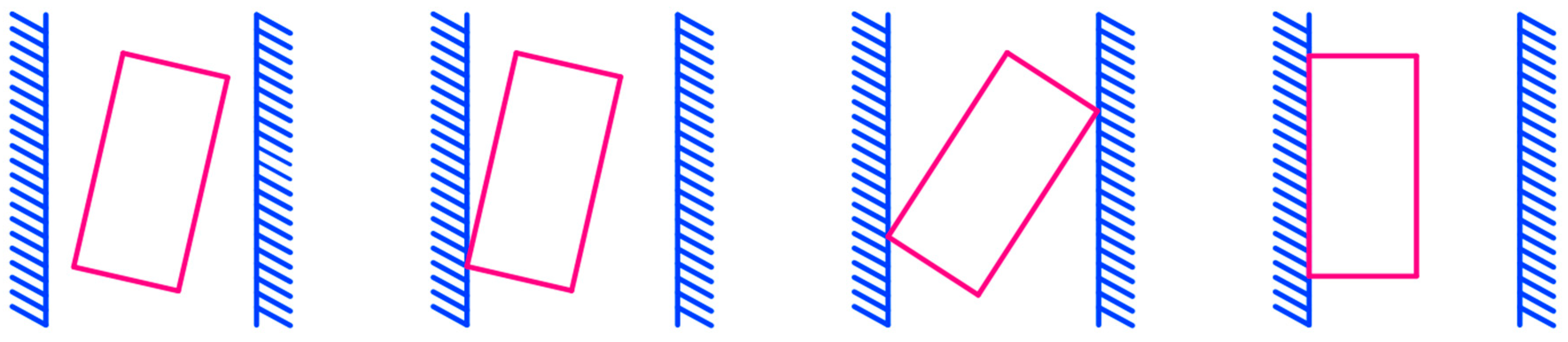
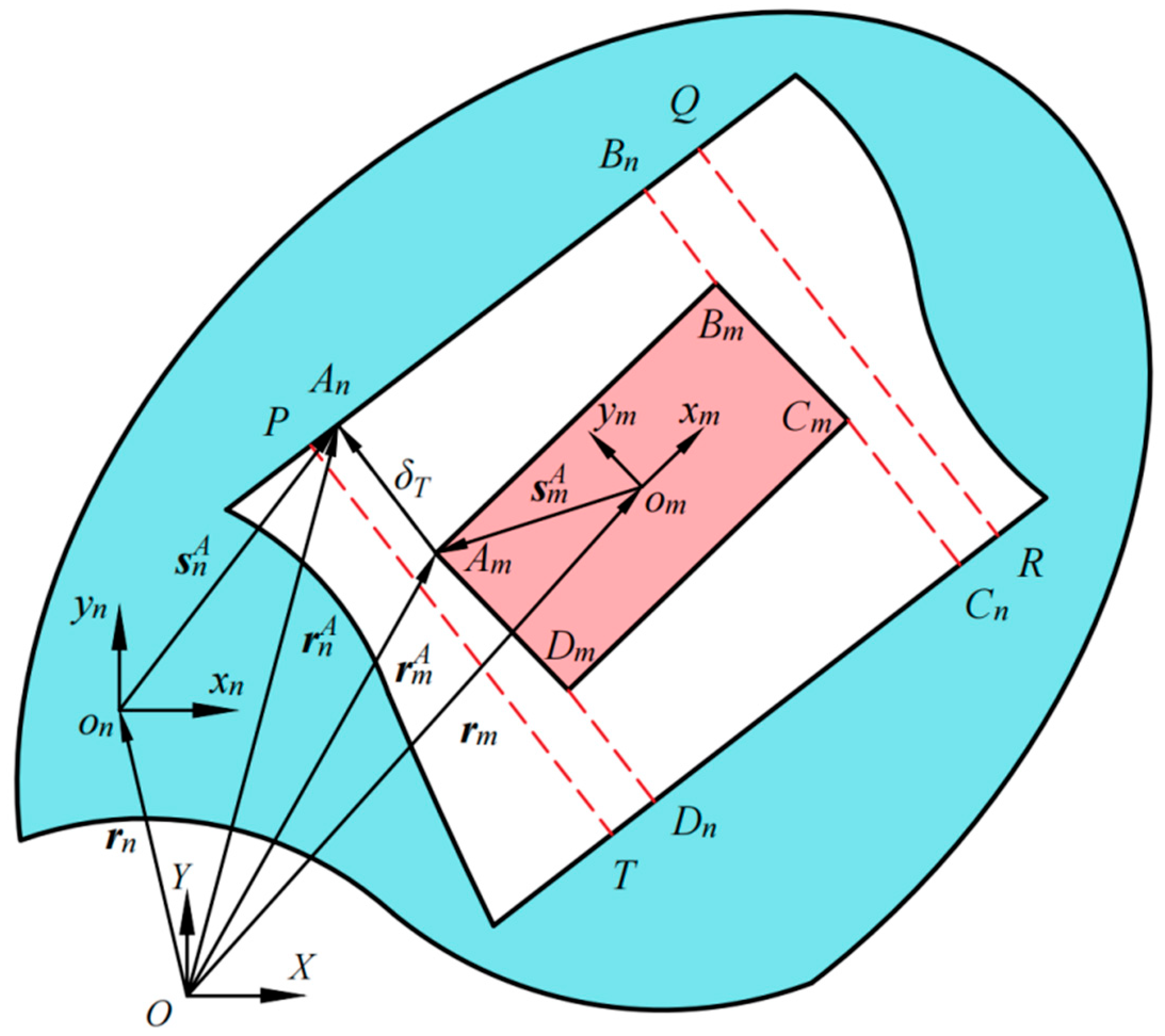
2.3. Establishment of a Kinematic Model for the Clearance in Prismatic Joints
2.3.1. Establishment of Normal Impact Force Model
2.3.2. Establishment of a Tangential Friction Model
3. Establishment of Rigid Body Dynamics Model for Mechanisms with Clearances
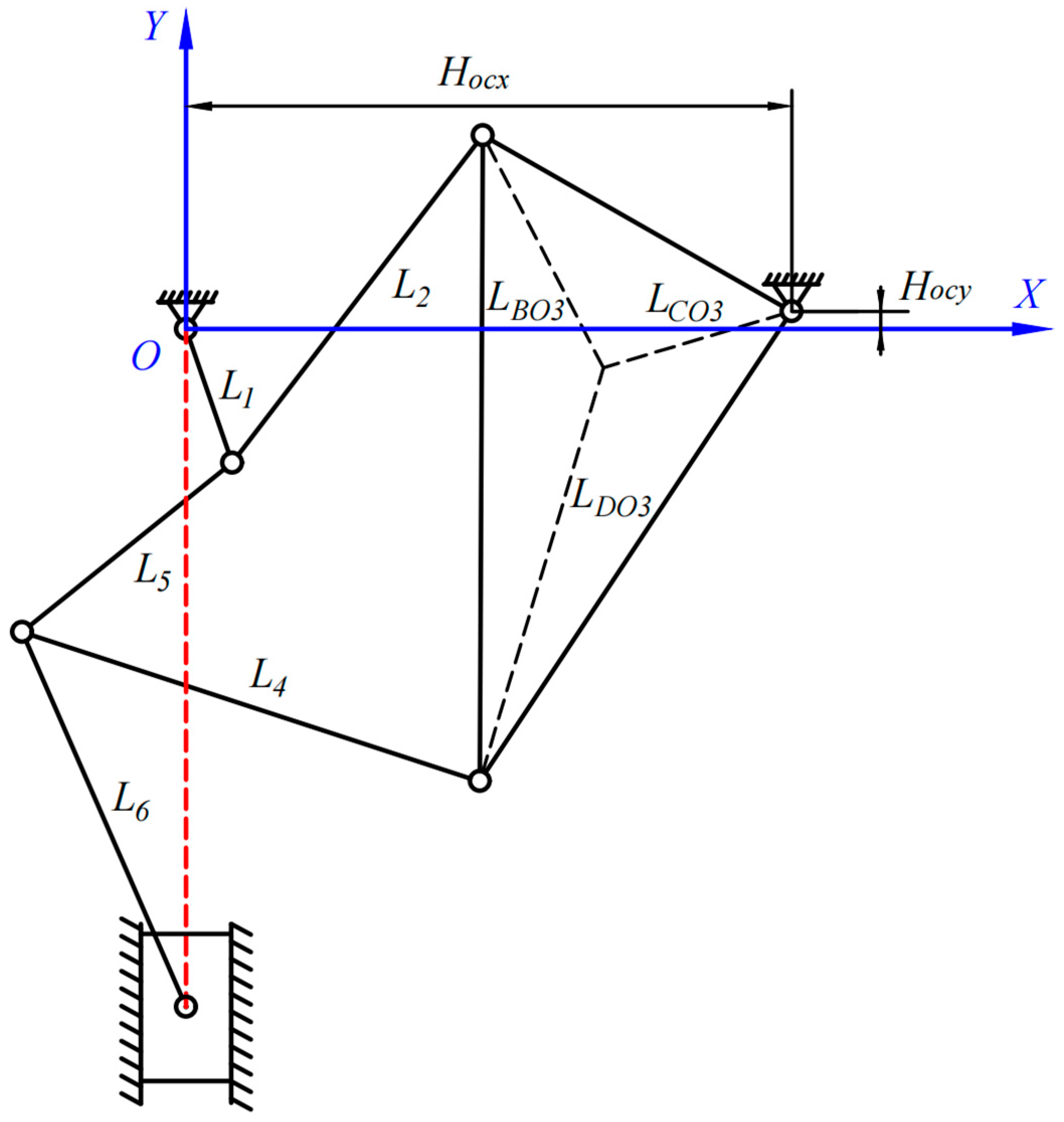
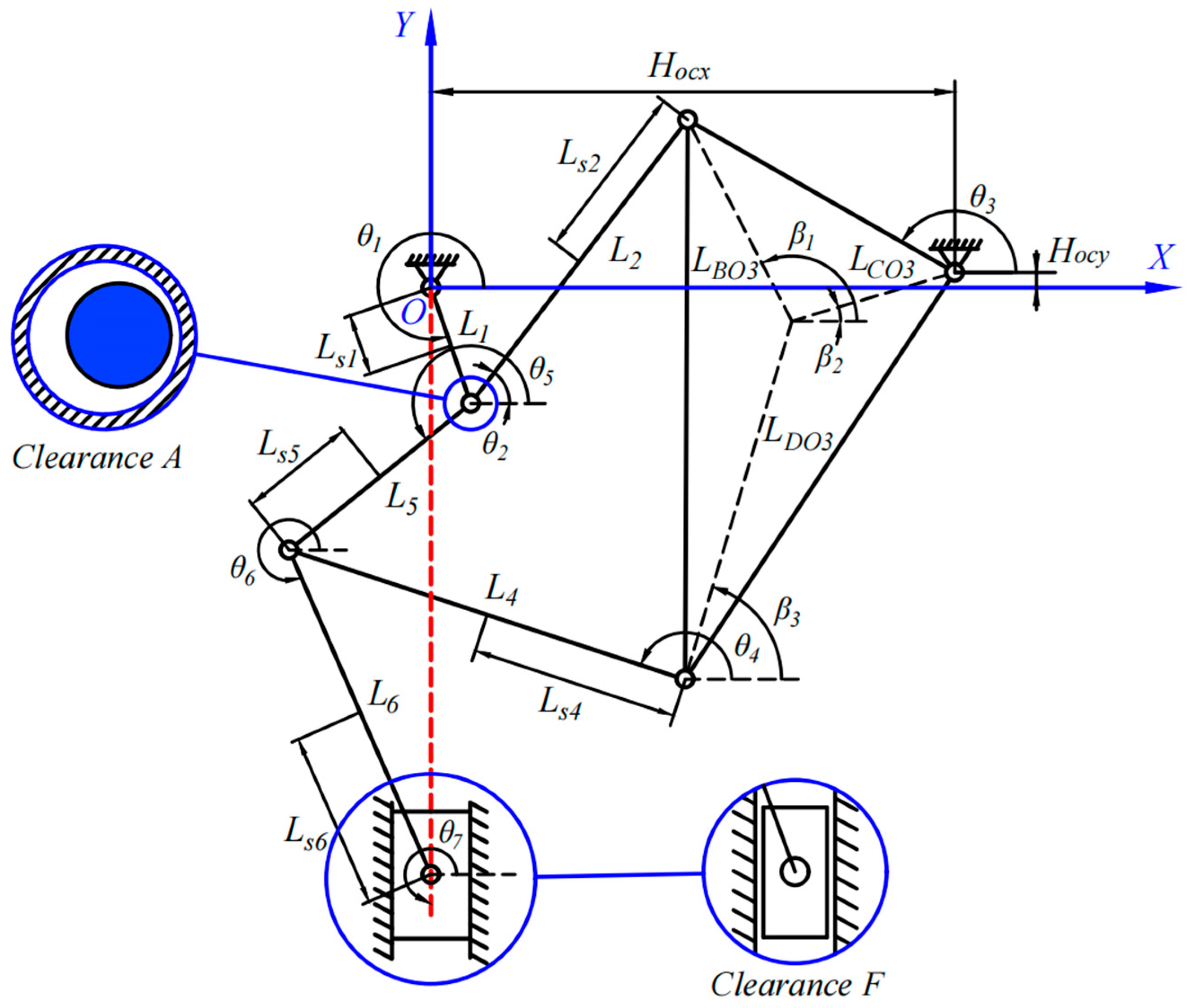
3.1. Structural Characteristics of an Eight-Bar Mechanism
3.2. Establishment of a Rigid Body Dynamics Model with Mixed Clearances
4. Dynamic Precision Reliability Modeling of Multilink Mechanisms with Clearances
4.1. The Theory of Stress–Strength Interference
4.2. Mechanism Dynamically Precise Reliability Model
5. Response Analysis of Rigid Body Dynamics of Clearance Containing Mechanism
5.1. Simulation Parameters for an Eight-Bar Mechanism with Clearance
5.2. Impact of Different Clearance Types on the Rigid Body Dynamic Response in a Mechanism
5.3. Effect of Combined Clearances on Rigid Body Dynamic Response of Mechanism
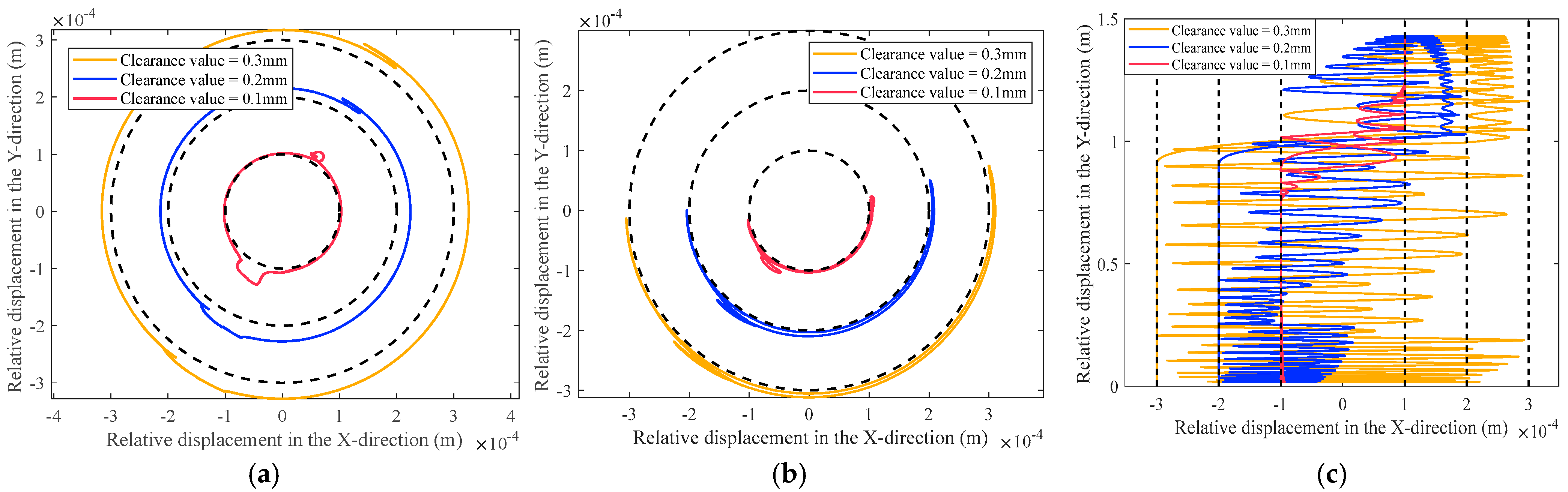
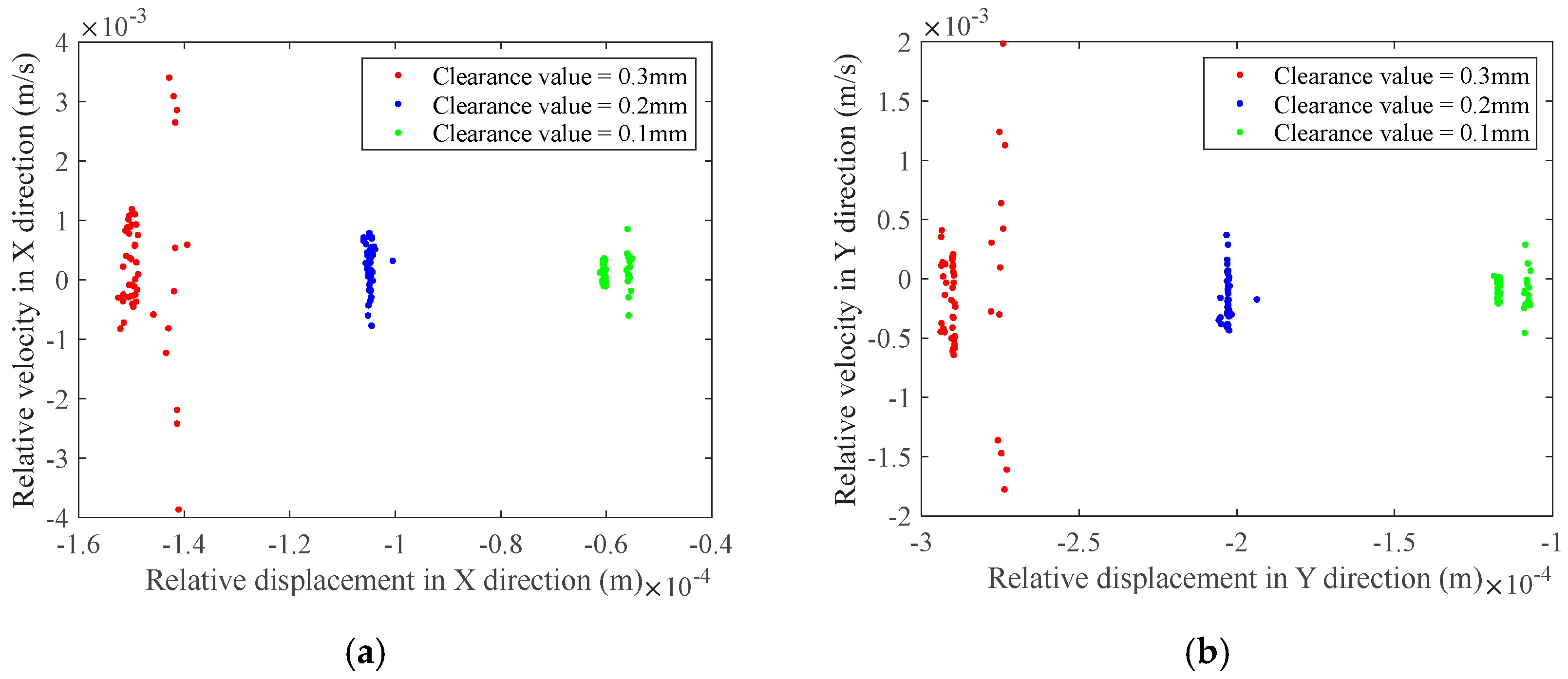
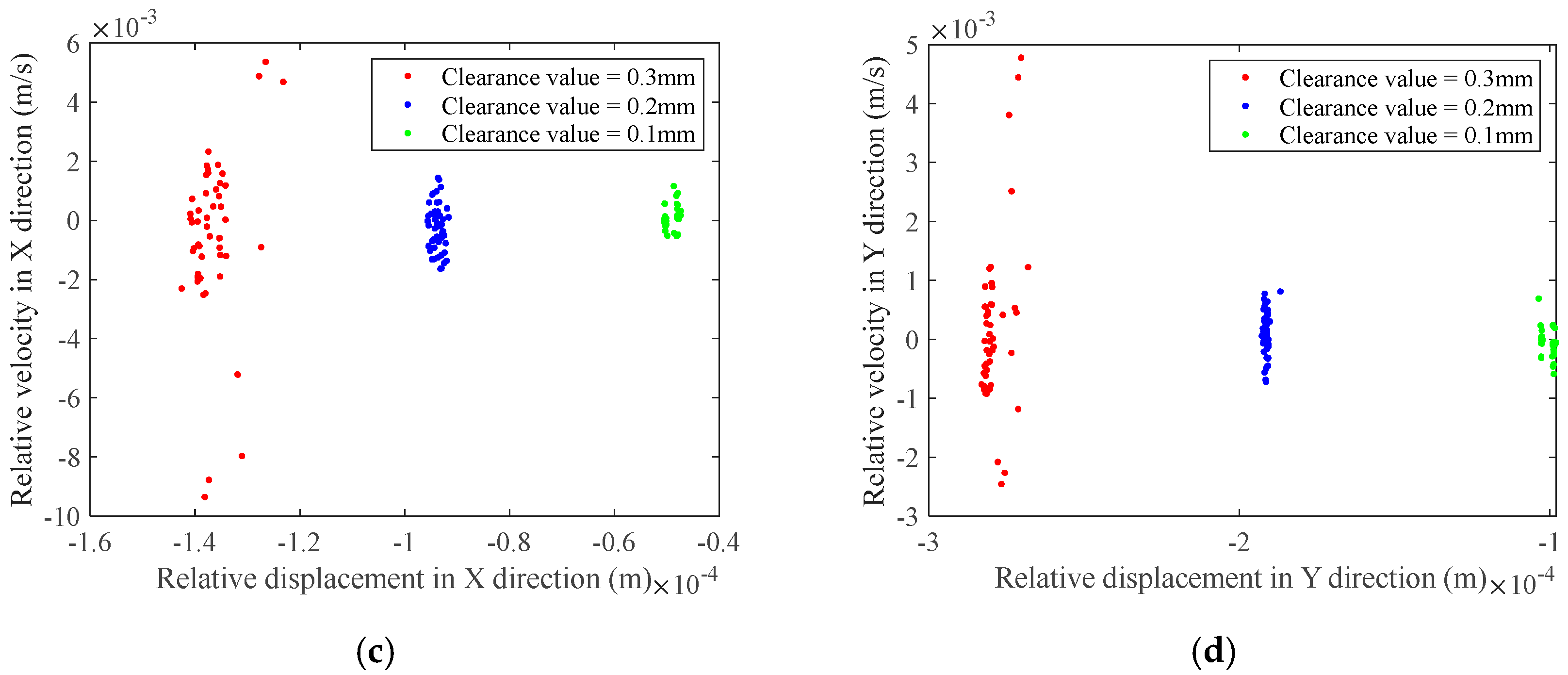
5.3.1. Effect of Clearance Values in the Response of Rigid Body Dynamics of Mixed Clearance Mechanism
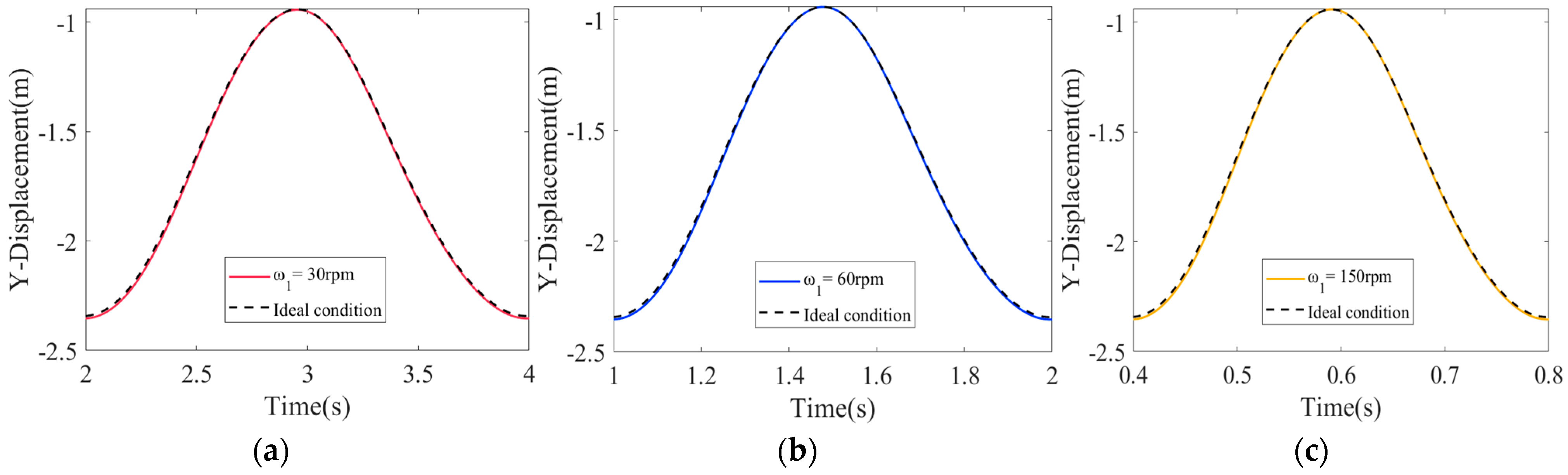
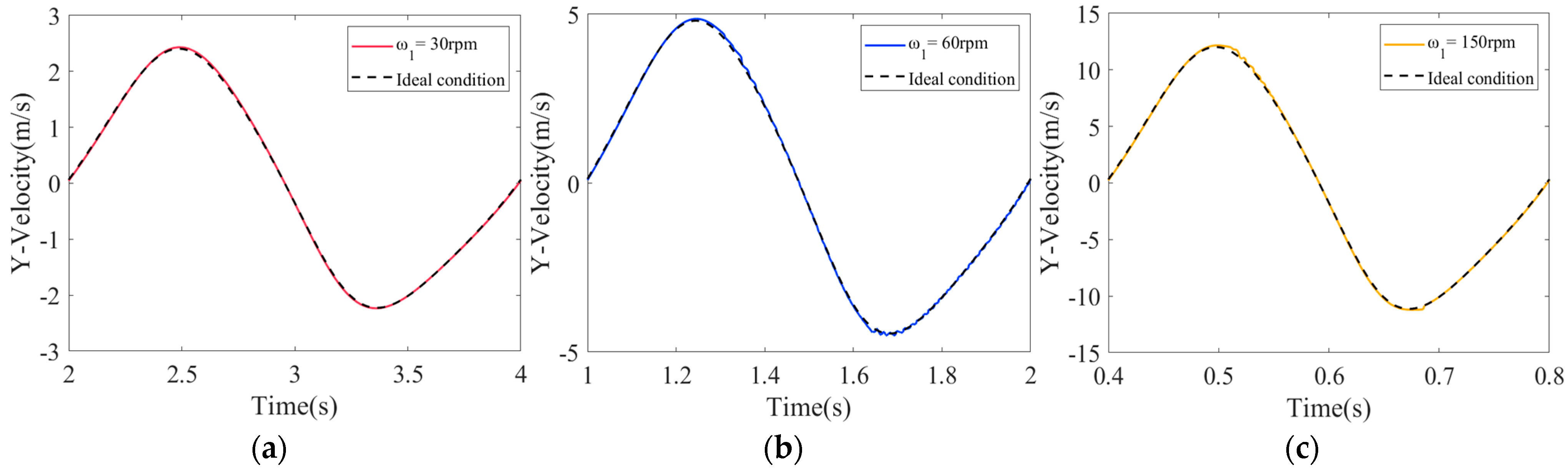
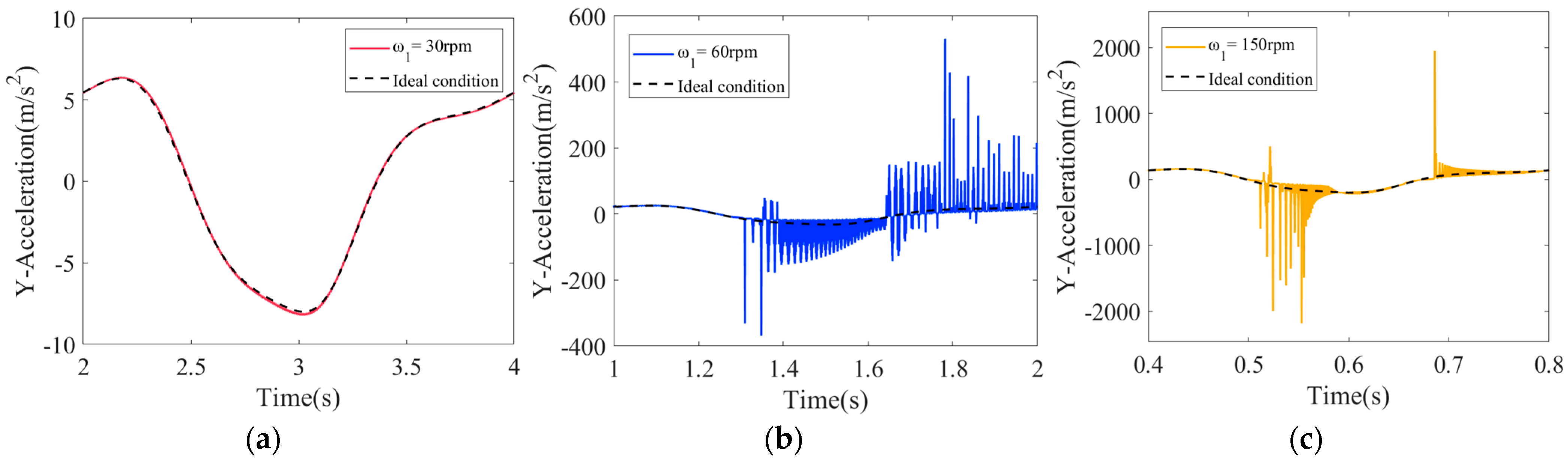
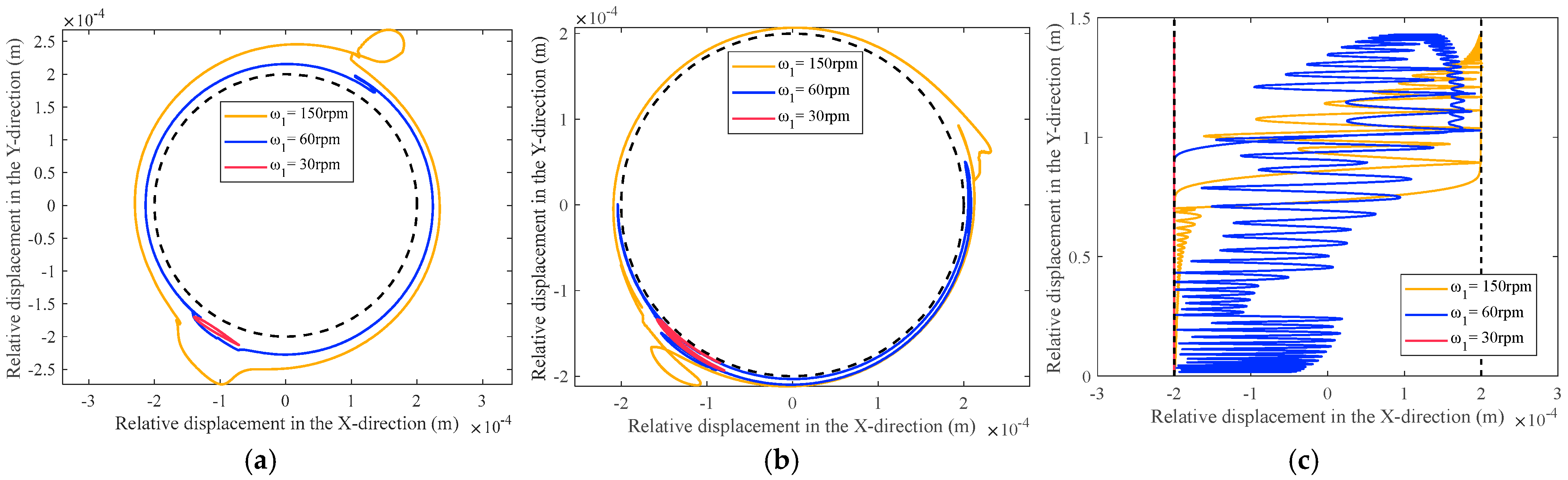
5.3.2. Effect of Driving Speed on Dynamic Response of the Rigid Body of a Mixed Clearance Mechanism
6. Nonlinear Characterizations of Rigid Multilink Mechanism with Clearances
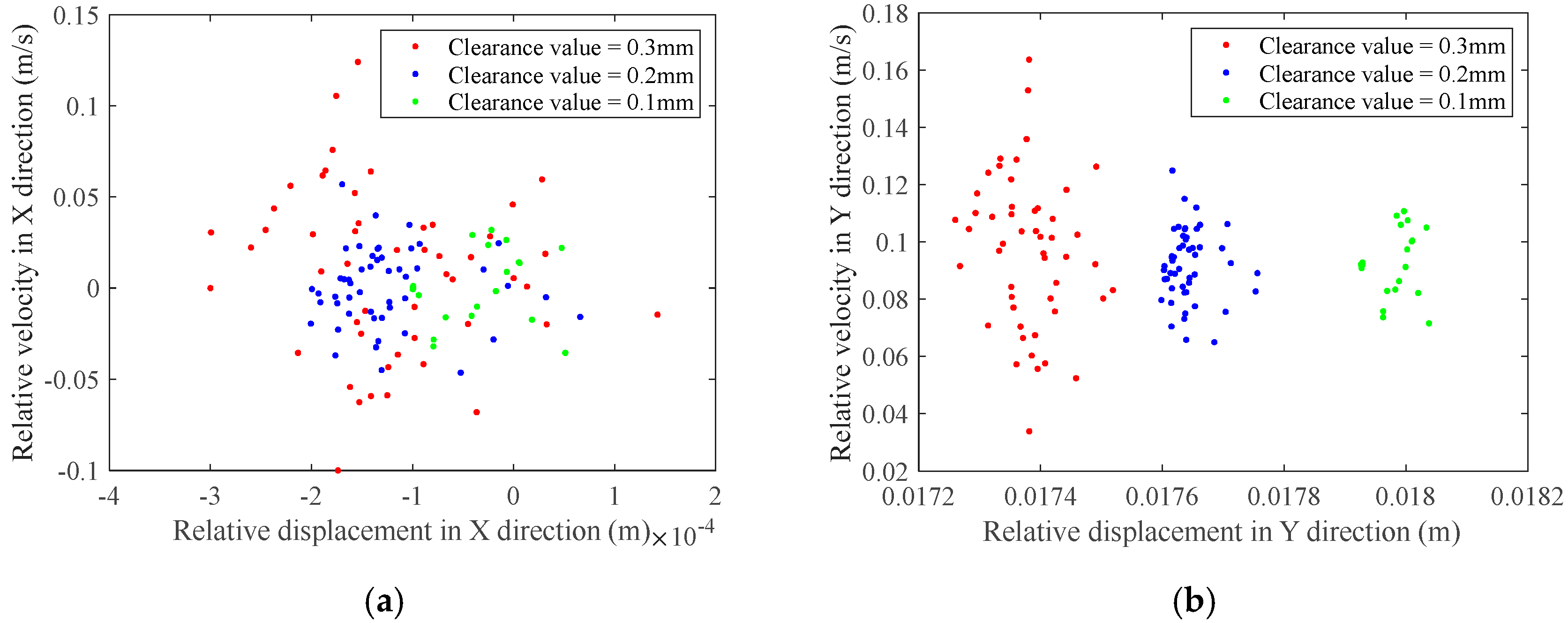
6.1. Effect of Clearance Values on Nonlinearity Characteristics of Rigourous Multilink Structures with Mixed Clearances
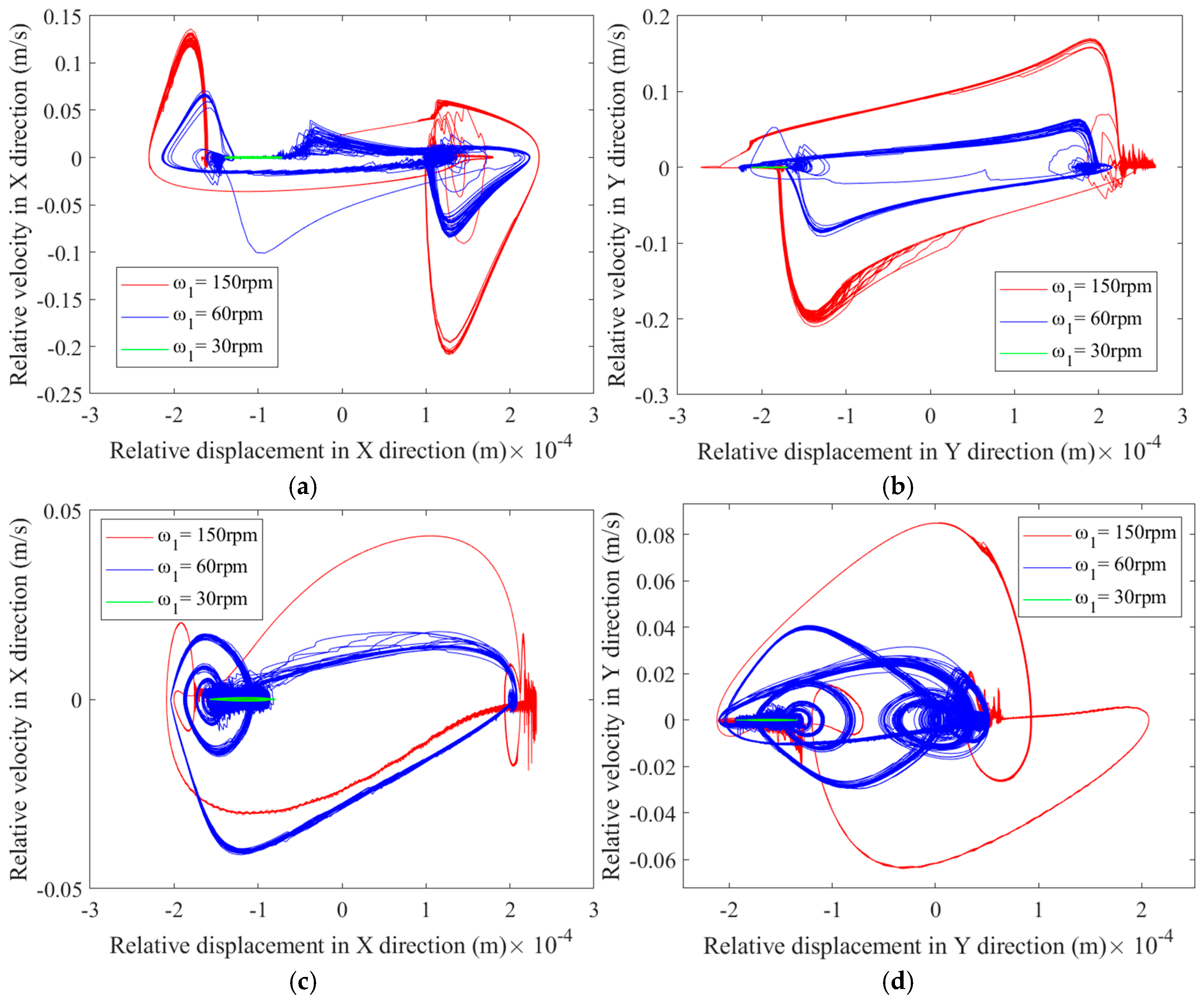
6.2. Driving Speed Effect on Mixed Clearance Rigid Multilink Mechanism Nonlinear Characteristics
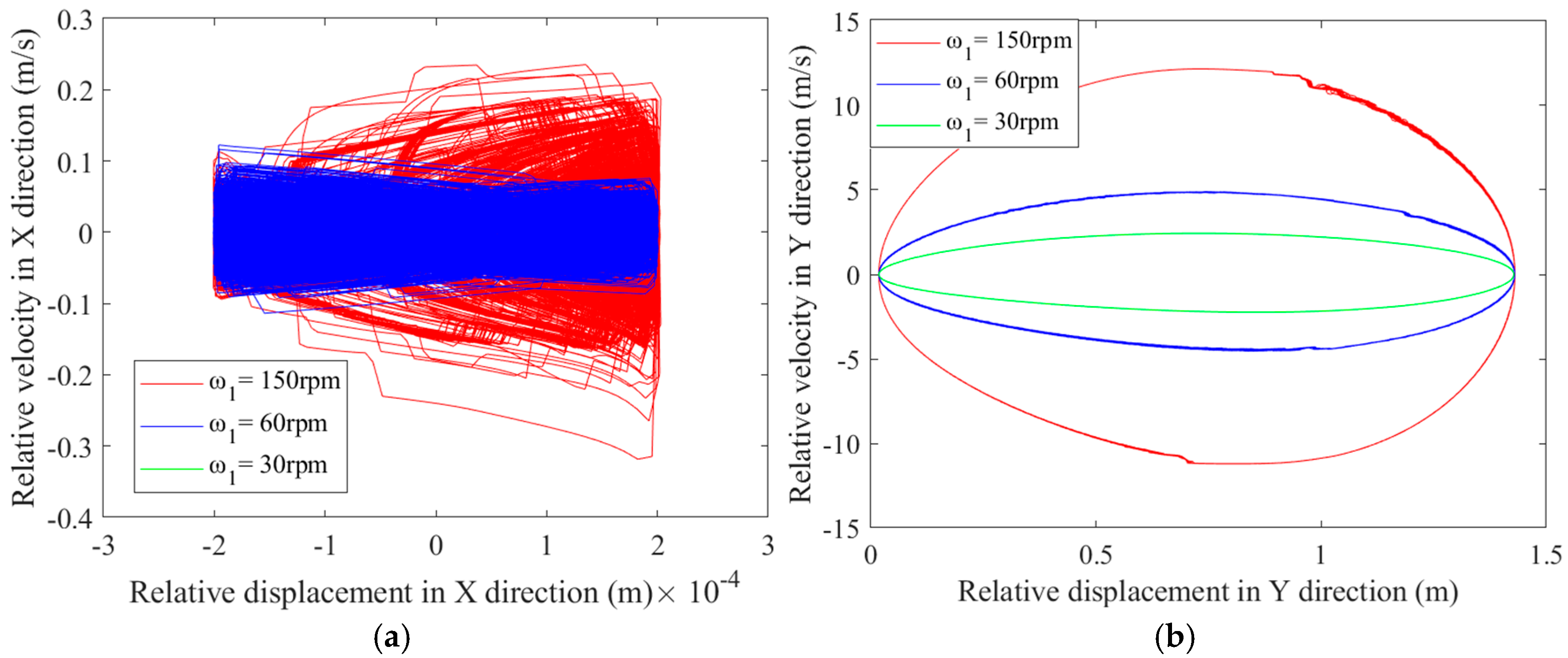
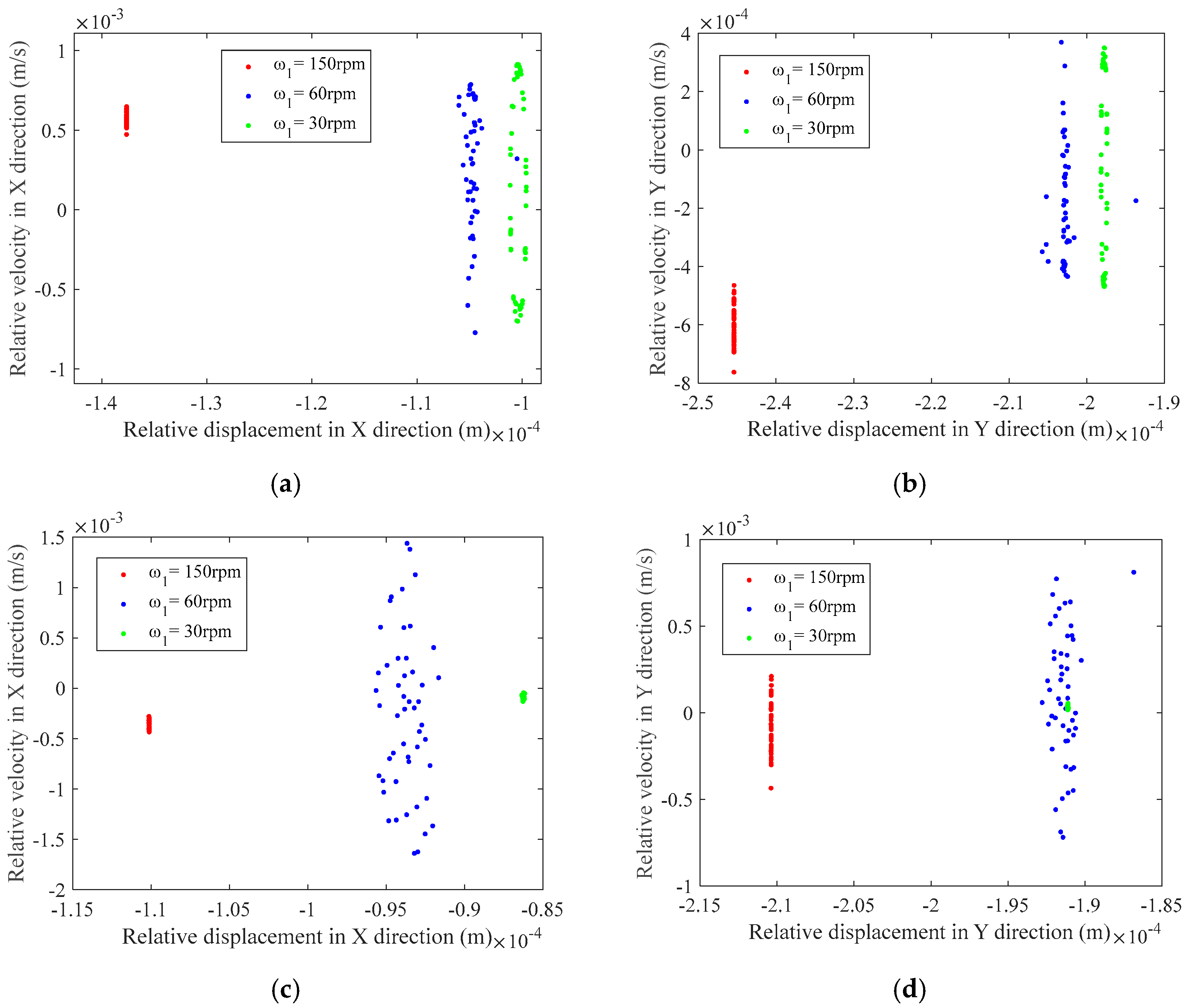
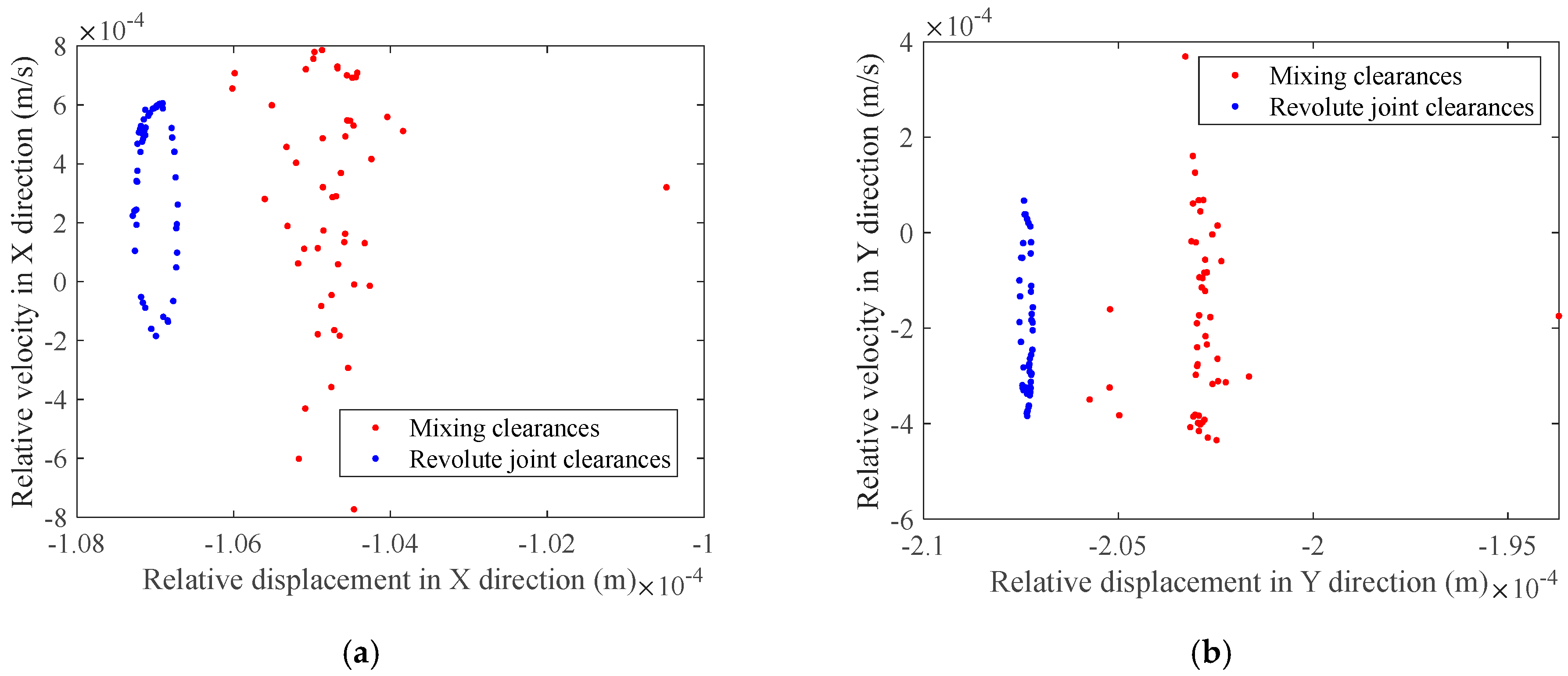
6.3. The Influence of the Prismatic Joint Clearance on the Nonlinear Characteristics at the Revolute Joint Clearance
7. Dynamic Precision Reliable Analysis of Multilink Structure with Clearance
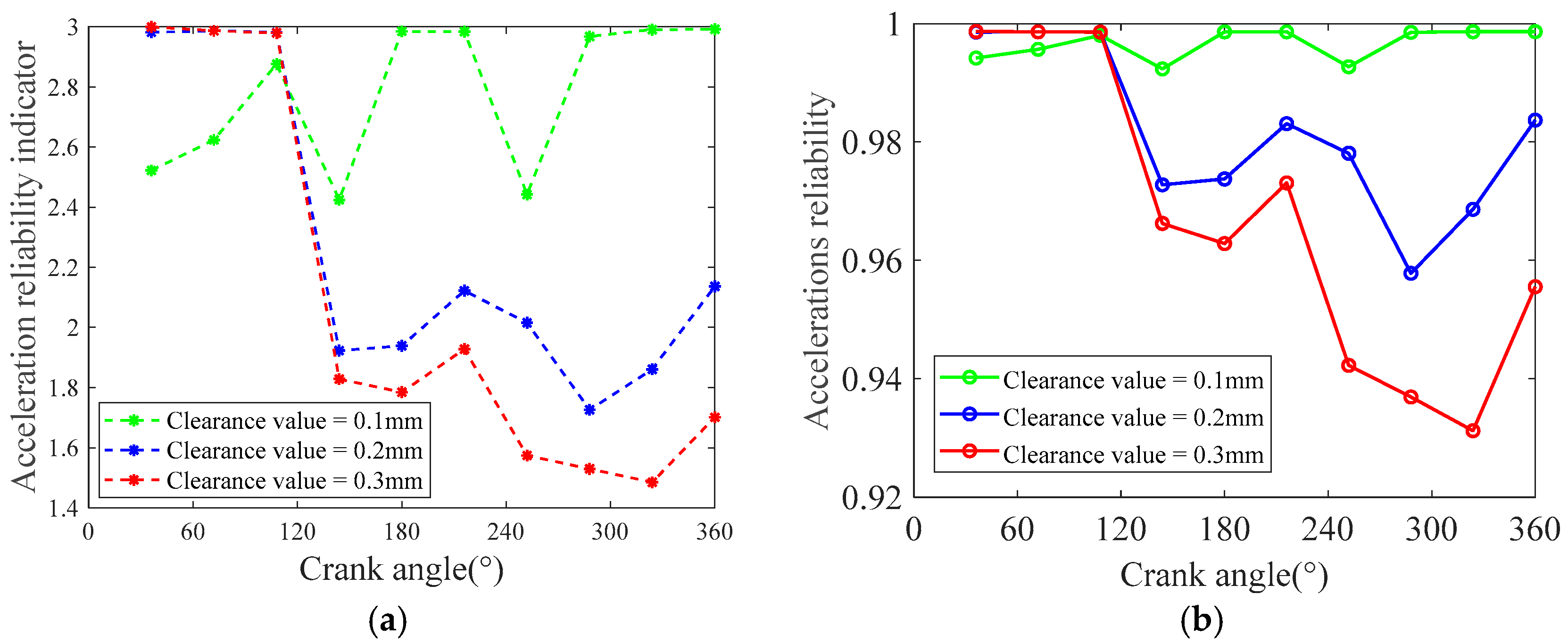
7.1. Influence of Clearance Values on Reliable Dynamic Accuracy of Hybrid Clearance Mechanisms
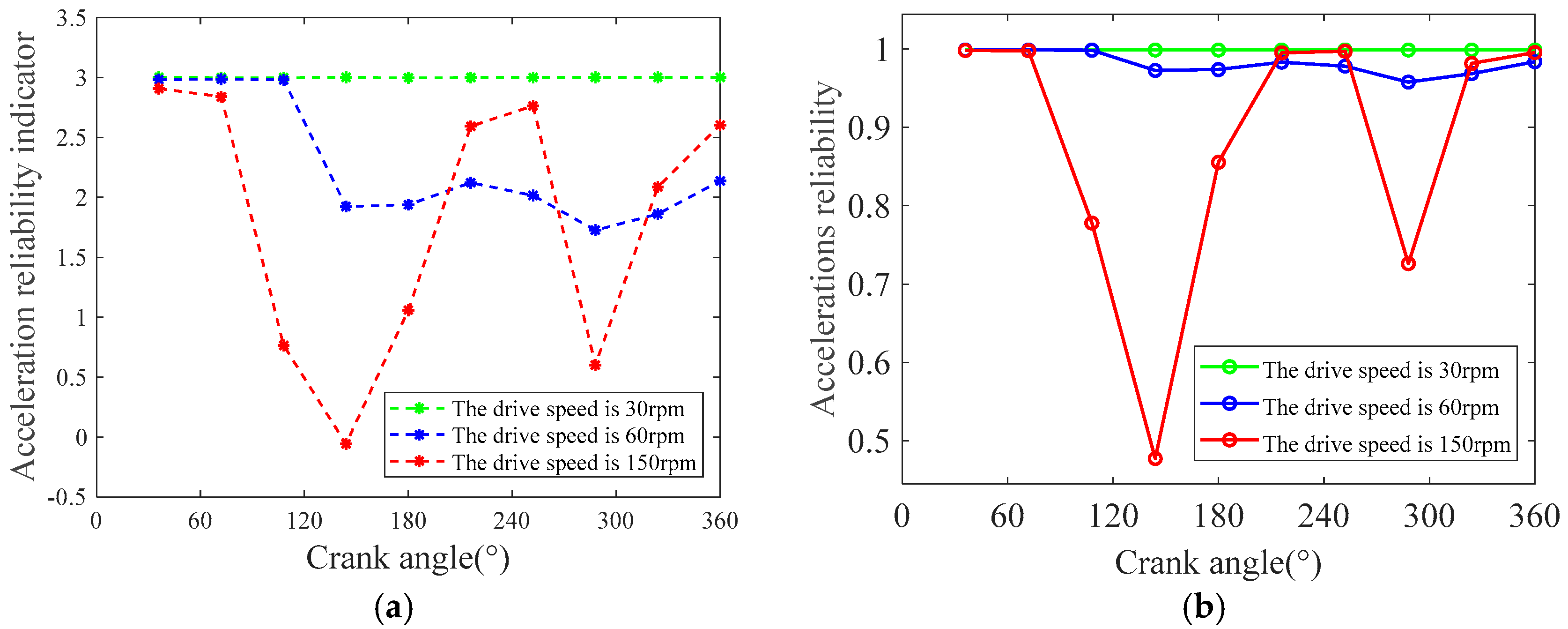
7.2. Influence of Travelling Speed on Reliability of the Dynamic Accuracy of a Mixed Clearance Mechanism
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Song, Z.D.; Yang, X.J.; Li, B.; Xu, W.F.; Hu, H. Modular dynamic modeling and analysis of planar closed-loop mechanisms with clearance joints and flexible links. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 231, 522–540. [Google Scholar] [CrossRef]
- Korayem, M.H.; Dehkordi, S.F.; Mehrjooee, O. Nonlinear analysis of open-chain flexible manipulator with time-dependent structure. Adv. Space Res. 2022, 69, 1027–1049. [Google Scholar] [CrossRef]
- Mehrjooee, O.; Dehkordi, S.F.; Korayem, M.H. Dynamic modeling and extended bifurcation analysis of flexible-link manipulator. Mech. Based Des. Struct. Mach. 2020, 48, 87–110. [Google Scholar] [CrossRef]
- Chen, X.L.; Jiang, S.; Wang, S.Y.; Deng, Y. Dynamics analysis of planar multi-DOF mechanism with multiple revolute clearances and chaos identification of revolute clearance joints. Multibody Syst. Dyn. 2019, 47, 317–345. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, F.; Li, Y.Y. Reliability optimization design of a planar multi-body system with two clearance joints based on reliability sensitivity analysis. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 1369–1382. [Google Scholar] [CrossRef]
- Xiang, W.W.K.; Yan, S.Z.; Wu, J.N.; Niu, W.D. Dynamic response and sensitivity analysis for mechanical systems with clearance joints and parameter uncertainties using Chebyshev polynomials method. Mech. Syst. Signal Process. 2020, 138, 106551. [Google Scholar] [CrossRef]
- Hou, Y.L.; Deng, Y.J.; Zeng, D.X. Dynamic modelling and properties analysis of 3RSR parallel mechanism considering spherical joint clearance and wear. J. Cent. South Univ. 2021, 28, 712–727. [Google Scholar] [CrossRef]
- Li, Y.Y.; Chen, G.P.; Sun, D.Y.; Gao, Y.; Wang, K. Dynamic analysis and optimization design of a planar slider-crank mechanism with flexible components and two clearance joints. Mech. Mach. Theory 2016, 99, 37–57. [Google Scholar] [CrossRef]
- Bai, Z.; Liu, T.; Li, J.; Zhao, J. Numerical and experimental study on dynamic characteristics of planar mechanism with mixed clearances. Mech. Based Des. Struct. Mach. 2023, 51, 6142–6165. [Google Scholar] [CrossRef]
- Tan, H.; Hu, Y.; Li, L. A continuous analysis method of planar rigid-body mechanical systems with two revolute clearance joints. Multibody Syst. Dyn. 2017, 40, 347–373. [Google Scholar] [CrossRef]
- Olyaei, A.A.; Ghazavi, M.R. Stabilizing slider-crank mechanism with clearance joints. Mech. Mach. Theory 2012, 53, 17–29. [Google Scholar] [CrossRef]
- Zheng, X.; Li, J.; Wang, Q.; Liao, Q. A methodology for modeling and simulating frictional translational clearance joint in multibody systems including a flexible slider part. Mech. Mach. Theory 2019, 142, 103594. [Google Scholar] [CrossRef]
- Zheng, E.; Wang, T.; Guo, J.; Zhu, Y.; Lin, X.; Wang, Y.; Kang, M. Dynamic modeling and error analysis of planar flexible multilink mechanism with clearance and spindle-bearing structure. Mech. Mach. Theory 2019, 131, 234–260. [Google Scholar] [CrossRef]
- Lu, J.; Jiang, J.; Li, J.; Wen, M. Analysis of dynamic mechanism and global response of vehicle shimmy system with multi-clearance joints. J. Vib. Control 2018, 24, 2312–2326. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, J.; Wang, H. Dynamic performance of over-constrained planar mechanisms with multiple revolute clearance joints. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 3524–3537. [Google Scholar] [CrossRef]
- Farahan, S.B.; Ghazavi, M.R.; Rahmanian, S. Bifurcation in a planar four-bar mechanism with revolute clearance joint. Nonlinear Dyn. 2017, 87, 955–973. [Google Scholar] [CrossRef]
- Hou, Y.; Wang, Y.; Jing, G.; Deng, Y.; Zeng, D.; Qiu, X. Chaos phenomenon and stability analysis of RU-RPR parallel mechanism with clearance and friction. Adv. Mech. Eng. 2018, 10, 1–15. [Google Scholar] [CrossRef]
- Wang, Z.; Tian, Q.; Hu, H.; Flores, P. Nonlinear dynamics and chaotic control of a flexible multibody system with uncertain joint clearance. Nonlinear Dyn. 2016, 86, 1571–1597. [Google Scholar] [CrossRef]
- Erkaya, S.; Dogan, S. A comparative analysis of joint clearance effects on articulated and partly compliant mechanisms. Nonlinear Dyn. 2015, 81, 323–341. [Google Scholar] [CrossRef]
- Flores, P.; Leine, R.; Glocker, C. Modeling and analysis of planar rigid multibody systems with translational clearance joints based on the non-smooth dynamics approach. Multibody Syst. Dyn. 2010, 23, 165–190. [Google Scholar] [CrossRef]
- Megahed, S.M.; Haroun, A.F. Analysis of the Dynamic Behavioral Performance of Mechanical Systems with Multi-Clearance Joints. J. Comput. Nonlinear Dyn. 2012, 7, 011005. [Google Scholar] [CrossRef]
- Qian, M.; Qin, Z.; Yan, S.; Zhang, L. A comprehensive method for the contact detection of a translational clearance joint and dynamic response after its application in a crank-slider mechanism. Mech. Mach. Theory 2020, 145, 103734. [Google Scholar] [CrossRef]
- Skrinjar, L.; Slavic, J.; Boltezar, M. A validated model for a pin-slot clearance joint. Nonlinear Dyn. 2017, 88, 131–143. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, X.; Zhang, X.; Mo, J. Dynamic analysis of a 3-(P)RR parallel mechanism by considering joint clearances. Nonlinear Dyn. 2017, 90, 405–423. [Google Scholar] [CrossRef]
- Rahmanian, S.; Ghazavi, M.R. Bifurcation in planar slider-crank mechanism with revolute clearance joint. Mech. Mach. Theory 2015, 91, 86–101. [Google Scholar] [CrossRef]
- Liu, X.; Qi, C.K.; Lin, J.F.; Li, D.J.; Gao, F. An Actuation Acceleration-Based Kinematic Modeling and Parameter Identification Approach for a Six-Degrees-of-Freedom 6-PSU Parallel Robot with Joint Clearances. J. Mech. Robot. 2025, 17, 011015. [Google Scholar] [CrossRef]
- Wu, L.J.; Marghitu, D.B.; Zhao, J. Nonlinear Dynamics Response of a Planar Mechanism with Two Driving Links and Prismatic Pair Clearance. Math. Probl. Eng. 2017, 2017, 6343135. [Google Scholar] [CrossRef]
- Ting, K.L.; Hsu, K.L. Clearance-Induced Position Uncertainty of Planar Linkages and Parallel Manipulators. J. Mech. Des. 2017, 139, 082301. [Google Scholar]
- Xiao, S.; Liu, S.; Wang, H.; Lin, Y.; Song, M.; Zhang, H. Nonlinear dynamics of coupling rub-impact of double translational joints with subsidence considering the flexibility of piston rod. Nonlinear Dyn. 2020, 100, 1203–1229. [Google Scholar] [CrossRef]
- Jiang, S.; Chen, X. Test study and nonlinear dynamic analysis of planar multi-link mechanism with compound clearances. Eur. J. Mech. A Solids 2021, 88, 104252. [Google Scholar] [CrossRef]
- Muvengei, O.; Kihiu, J.; Ikua, B. Dynamic analysis of planar rigid-body mechanical systems with two-clearance revolute joints. Nonlinear Dyn. 2013, 73, 259–273. [Google Scholar] [CrossRef]
- Xiao, L.; Yan, F.; Chen, T.; Zhang, S.; Jiang, S. Study on nonlinear dynamics of rigid-flexible coupling multi-link mechanism considering various kinds of clearances. Nonlinear Dyn. 2023, 111, 3279–3306. [Google Scholar] [CrossRef]
- Jiang, S.; Wang, T.; Xiao, L. Experiment research and dynamic behavior analysis of multi-link mechanism with wearing clearance joint. Nonlinear Dyn. 2022, 109, 1325–1340. [Google Scholar] [CrossRef]
- Jiang, S.; Zhao, M.; Liu, J.; Lin, Y.; Xiao, L.; Jia, Y. Dynamic response and nonlinear characteristics of multi-link mechanism with clearance joints. Arch. Appl. Mech. 2023, 93, 3461–3493. [Google Scholar] [CrossRef]
- Ting, K.L.; Hsu, K.L.; Yu, Z.; Wang, J. Clearance-induced output position uncertainty of planar linkages with revolute and prismatic joints. Mech. Mach. Theory 2017, 111, 66–75. [Google Scholar] [CrossRef]
- Zhan, Z.H.; Zhang, X.M.; Zhang, H.D.; Chen, G.C. Unified motion reliability analysis and comparison study of planar parallel manipulators with interval joint clearance variables. Mech. Mach. Theory 2019, 138, 58–75. [Google Scholar] [CrossRef]
- Yu, Z.T.; Ting, K.L.; Hsu, K.L.; Wang, J. Uncertainty of coupler point position of slider crank mechanisms. J. Mech. Des. 2016, 138, 033301. [Google Scholar]
- Chen, X.L.; Sun, C.H. Dynamic modeling of spatial parallel mechanism with multi-spherical joint clearances. Int. J. Adv. Robot. Syst. 2019, 16, 1729881419866902. [Google Scholar] [CrossRef]
- Isaac, F.; Marques, F.; Dourado, N. Finite element modeling of three-dimensional dry revolute joints for multibody dynamic simulations. Multibody Sys. Dyn. 2019, 45, 293–313. [Google Scholar] [CrossRef]
- Alshaer, B.J.; Lankarani, H.M. Exact analytical formulation for dynamic load analysis of imperfectly lubricated journal bearings in multibody systems. Multibody Syst. Dyn. 2024, 202, 105773. [Google Scholar]
- Zhang, S.; Gao, Y.K.; Yang, J. Dynamic modeling and analysis of vehicle scissor door mechanisms incorporating mixed clearance joints using a hybrid contact force model. Multibody Sys. Dyn. 2024, 61, 509–538. [Google Scholar] [CrossRef]
- Lin, J.; Cheng, Z.; Fang, P. Dynamics research and chaos identification in rigid-flexible coupling multi-link mechanisms with irregular wear clearances. Nonlinear Dyn. 2023, 111, 12983–13015. [Google Scholar]
- Jiang, S.; Liu, J.; Yang, Y. Experimental research and dynamics analysis of multi-link rigid-flexible coupling mechanisms with multiple lubrication clearances. Arch. Appl. Mech. 2023, 93, 2749–2780. [Google Scholar] [CrossRef]
- Flores, P.; Ambrósio, J.; Claro, J.C.P.; Lankarani, H.M. Translational Joints With Clearance in Rigid Multibody Systems. J. Comput. Nonlinear Dyn. 2007, 3, 011007. [Google Scholar] [CrossRef]
- Li, Y.Y.; Wang, C.; Huang, W.H. Dynamics analysis of planar rigid-flexible coupling deployable solar array system with multiple revolute clearance joints. Mech. Syst. Signal Process. 2019, 117, 188–209. [Google Scholar] [CrossRef]
- Wang, G.; Wang, L. Dynamics investigation of spatial parallel mechanism considering rod flexibility and spherical joint clearance. Mech. Mach. Theory 2019, 137, 83–107. [Google Scholar] [CrossRef]
- Erkaya, S.; Doğan, S.; Ulus, Ş. Effects of Joint Clearance on the Dynamics of a Partly Compliant Mechanism: Numerical and Experimental Studies. Mech. Mach. Theory 2015, 88, 125–140. [Google Scholar] [CrossRef]
- Whitney, D.E. Mechanical Assemblies: Their Design, Manufacture, and Role in Product Development; Oxford University Press: New York, NY, USA, 2004. [Google Scholar]












| Joint Type | Normal Impact Force Model | Tangential Friction Force Model |
|---|---|---|
| Revolute joint | L-N model | Modified Coulomb friction force model |
| Prismatic joint | Hertz Model and L-N Model | Modified Coulomb friction force model |
| Component | Mass/kg | ) | ||
|---|---|---|---|---|
| Rod1 | J1 | |||
| Rod2 | J2 | |||
| Rod3 | J3 | |||
| Rod4 | J4 | |||
| Rod5 | J5 | |||
| Rod6 | J6 | |||
| Rod7 | J7 | |||
| Parameter | Length/mm | Parameter | Length/mm |
|---|---|---|---|
| 205 | 628 | ||
| 1200 | 1020.17 | ||
| 1454.48 | 606.2 | ||
| 1200 | 1322.46 | ||
| 1256 | 1346 | ||
| 102.5 | 327 | ||
| 600 | 34.36 | ||
| 727.24 | 108.25 | ||
| 600 | 87.41 |
| Parameter | Value |
|---|---|
| 15 | |
| 200 | |
| 100 | |
| 20 | |
| 0.2 | |
| 0.9 | |
| 210 | |
| 0.28 |
| Parameter | Clearance (mm) | Direction | Horizontal Range (mm) | Vertical Range (mm) |
|---|---|---|---|---|
| 0.1 | X | [−1.19 × 10−4, 1.24 × 10−4] | [−6.88 × 10−2, 4.82 × 10−2] | |
| Y | [−1.27 × 10−4, 1.19 × 10−4] | [−5.98 × 10−2, 4.62 × 10−2] | ||
| 0.2 | X | [−2.14 × 10−4, 2.23 × 10−4] | [−1.01 × 10−1, 7.09 × 10−2] | |
| Y | [−2.27 × 10−4, 2.16 × 10−4] | [−9.11 × 10−2, 6.36 × 10−2] | ||
| 0.3 | X | [−3.16 × 10−4, 3.24 × 10−4] | [−1.60 × 10−1, 9.71 × 10−2] | |
| Y | [−3.26 × 10−4, 3.18 × 10−4] | [−1.22 × 10−1, 8.73 × 10−2] | ||
| 0.1 | X | [−1.06 × 10−4, 1.10 × 10−4] | [−2.38 × 10−2, 1.43 × 10−2] | |
| Y | [−1.11 × 10−4, 2.59 × 10−5] | [−1.56 × 10−2, 2.29 × 10−2] | ||
| 0.2 | X | [−2.03 × 10−4, 2.08 × 10−4] | [−4.10 × 10−2, 1.72 × 10−2] | |
| Y | [−2.29 × 10−4, 5.26 × 10−5] | [−2.96 × 10−2, 4.06 × 10−2] | ||
| 0.3 | X | [−3.05 × 10−4, 3.10 × 10−4] | [−5.78 × 10−2, 4.11 × 10−2] | |
| Y | [−3.11 × 10−4, 1.46 × 10−4] | [−4.75 × 10−2, 7.08 × 10−2] | ||
| prismatic joint F | 0.1 | X | [−1.00 × 10−4, 1.01 × 10−4] | [−1.10 × 10−1, 1.24 × 10−1] |
| Y | [1.81 × 10−2, 1.42] | [−4.52, 4.88] | ||
| 0.2 | X | [−2.00 × 10−4, 2.00 × 10−4] | [−1.13 × 10−1, 1.22 × 10−1] | |
| Y | [1.74 × 10−2, 1.42] | [−4.54, 4.90] | ||
| 0.3 | X | [−3.00 × 10−4, 3.01 × 10−4] | [−1.50 × 10−1, 1.51 × 10−1] | |
| Y | [1.71 × 10−2, 1.43] | [−4.58, 4.94] |
| Parameter | Speed (rpm) | Direction | Horizontal Range (mm) | Vertical Range (mm) |
|---|---|---|---|---|
| 30 | X | [−1.39 × 10−4, −7.13 × 10−5] | [−9.64 × 10−4, 9.19 × 10−4] | |
| Y | [−2.12 × 10−4, −1.70 × 10−4] | [7.14 × 10−4, 7.10 × 10−4] | ||
| 60 | X | [−2.13 × 10−4, 2.23 × 10−4] | [−1.01 × 10−1, 7.09 × 10−2] | |
| Y | [−2.27 × 10−4, 2.15 × 10−4] | [−9.11 × 10−2, 6.336 × 10−2] | ||
| 150 | X | [−2.29 × 10−4, 2.34 × 10−4] | [−2.08 × 10−1, 1.35 × 10−1] | |
| Y | [−2.72 × 10−4, 2.67 × 10−4] | [−2.10 × 10−1, 1.69 × 10−1] | ||
| 30 | X | [−1.57 × 10−4, −7.97 × 10−5] | [−5.13 × 10−4, 5.22 × 10−4] | |
| Y | [−1.93 × 10−4, −1.33 × 10−4] | [−3.57 × 10−4, 3.50 × 10−4] | ||
| 60 | X | [−2.03 × 10−4, 2.07 × 10−4] | [−4.10 × 10−2, 1.72 × 10−2] | |
| Y | [−2.09 × 10−4, 5.26 × 10−5] | [−2.95 × 10−2, 4.07 × 10−2] | ||
| 150 | X | [−2.08 × 10−4, 2.31 × 10−4] | [−3.06 × 10−2, 4.32 × 10−2] | |
| Y | [−2.11 × 10−4, 2.06 × 10−4] | [−6.38 × 10−2, 8.48 × 10−2] | ||
| prismatic joint F | 30 | X | [−2.00 × 10−4, 2.00 × 10−4] | [−1.12 × 10−7, 8.53 × 10−8] |
| Y | [1.74 × 10−2, 1.42] | [−2.23, 2.42] | ||
| 60 | X | [−2.00 × 10−4, 2.00 × 10−4] | [−1.13 × 10−2, 1.22 × 10−1] | |
| Y | [1.74 × 10−2, 1.42] | [−4.54, 4.90] | ||
| 150 | X | [−2.00 × 10−4, 2.01 × 10−4] | [−3.18 × 10−1, 2.35 × 10−1] | |
| Y | [1.76 × 10−2, 1.43] | [−11.16, 12.16] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lian, Y.; Zhu, J.; Zuo, Q.; Cai, M.; Jiang, S. Nonlinear Analysis and Reliability Analysis of Multilink Mechanism Considering Mixed Clearance. Appl. Sci. 2025, 15, 5774. https://doi.org/10.3390/app15105774
Lian Y, Zhu J, Zuo Q, Cai M, Jiang S. Nonlinear Analysis and Reliability Analysis of Multilink Mechanism Considering Mixed Clearance. Applied Sciences. 2025; 15(10):5774. https://doi.org/10.3390/app15105774
Chicago/Turabian StyleLian, Yuyang, Jianuo Zhu, Quanzhi Zuo, Mingyang Cai, and Shuai Jiang. 2025. "Nonlinear Analysis and Reliability Analysis of Multilink Mechanism Considering Mixed Clearance" Applied Sciences 15, no. 10: 5774. https://doi.org/10.3390/app15105774
APA StyleLian, Y., Zhu, J., Zuo, Q., Cai, M., & Jiang, S. (2025). Nonlinear Analysis and Reliability Analysis of Multilink Mechanism Considering Mixed Clearance. Applied Sciences, 15(10), 5774. https://doi.org/10.3390/app15105774





