Thermal–Electrical Optimization of Lithium-Ion Battery Conductor Structures Under Extreme High Amperage Current
Abstract
1. Introduction
2. Structural Optimization Method with Multiphysical Field Coupling
2.1. Theoretical Basis of Optimization Method
2.2. Finite Element Analysis Method
- (a)
- Control Group (C0 Group): Unthickened connecting piece and tab, serving as the baseline.
- (b)
- Test Group 1 (T1 Group): Thickened connecting piece and tab, used to assess the impact of cross-sectional area on temperature variation.
- (c)
- Test Group 2 (T2 Group): Thickened connecting piece and tab, along with welded reinforcement structural part, designed to evaluate the effect of contact area enhancement on temperature changes.
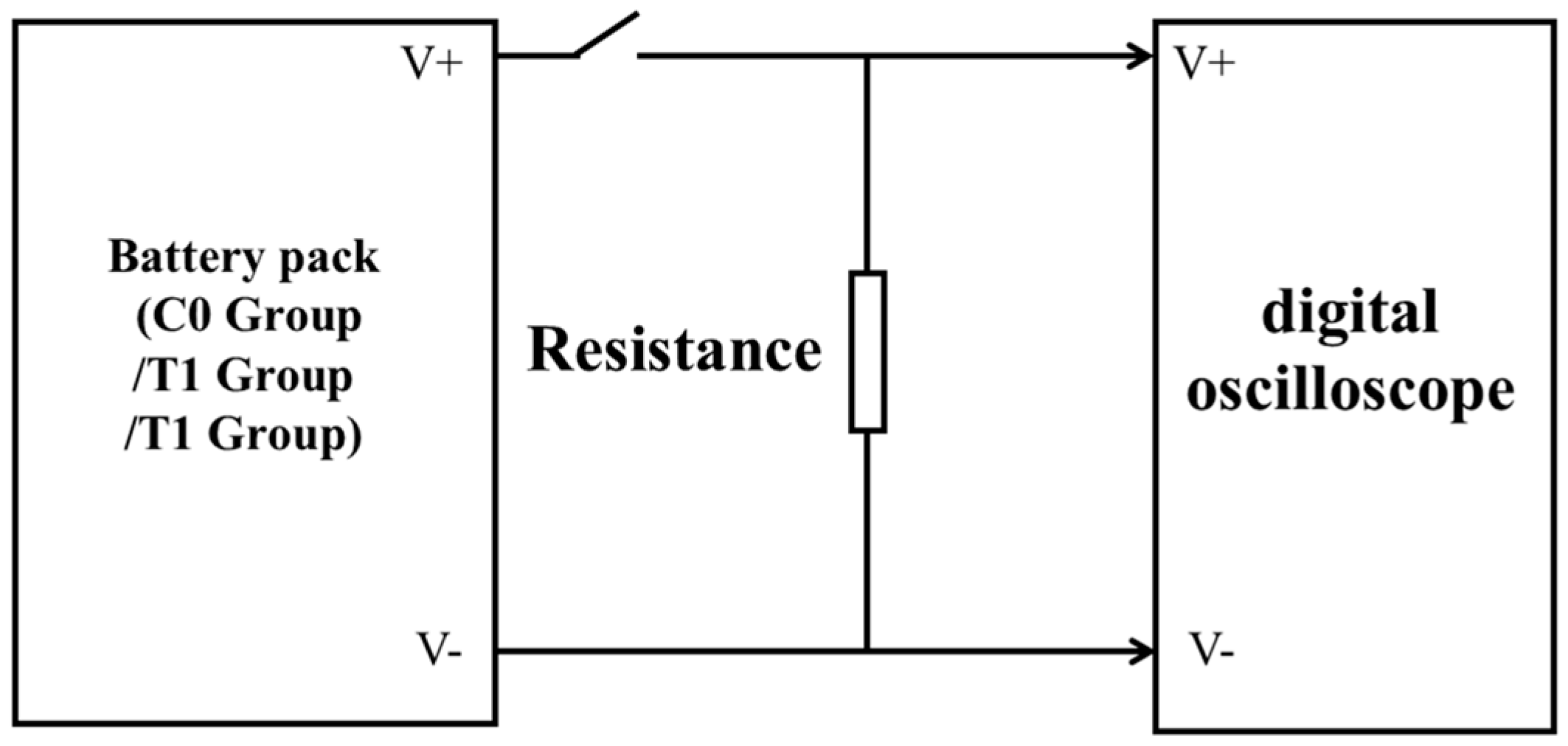
2.3. Experimental Validation Methods
- Allow the battery to rest for a period of time (1 h);
- Charge the battery at a constant current of 0.5 C until it is fully charged, then allow it to cool naturally to room temperature;
- Perform a discharge test on the series resistance of the battery pack at room temperature, maintaining the same current until the detection voltage becomes abnormal, and the open circuit condition is met.
3. Simulation Results and Optimizing Parameter Determination
4. Experimental Verification Results
5. Discussion
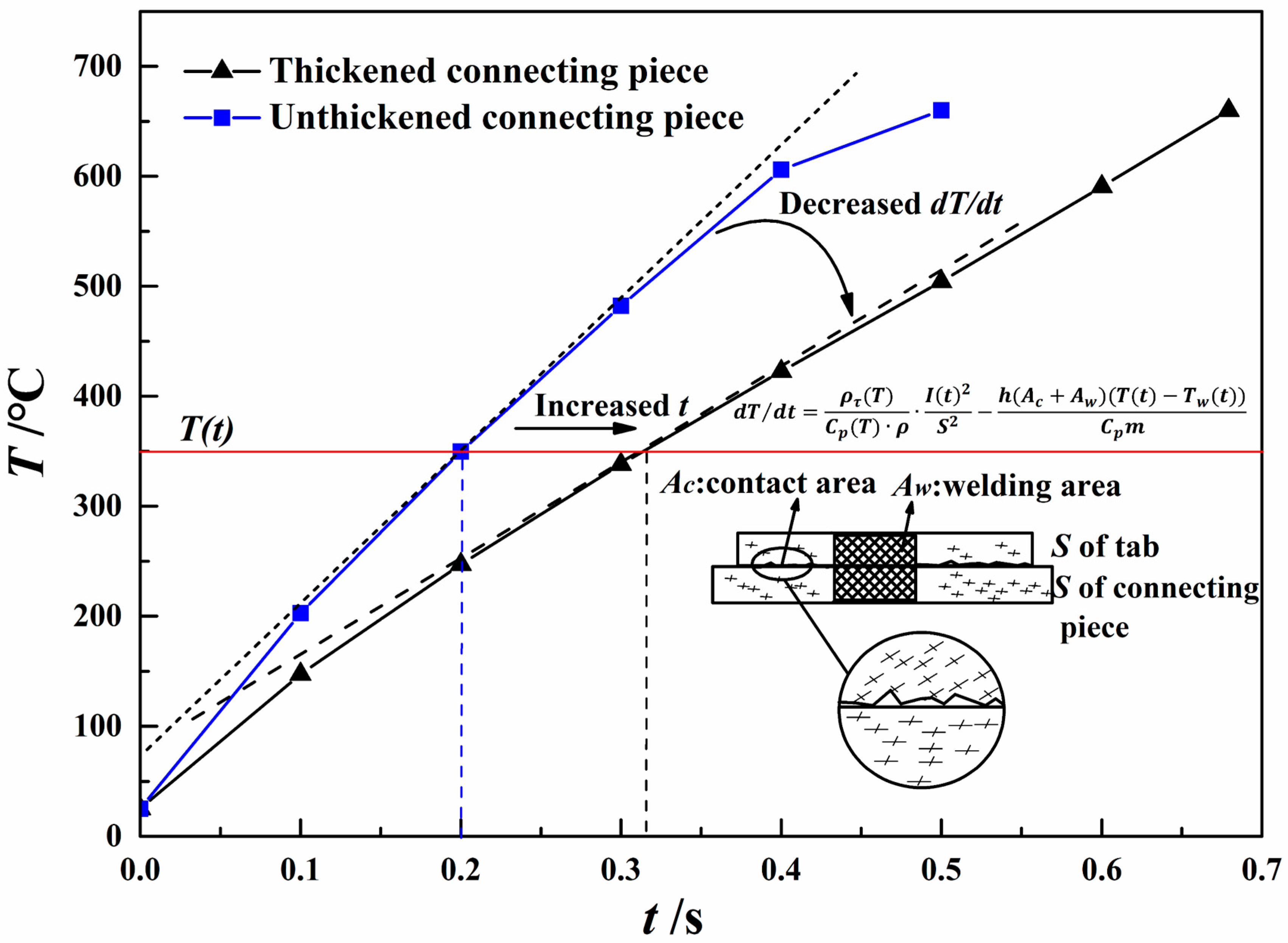
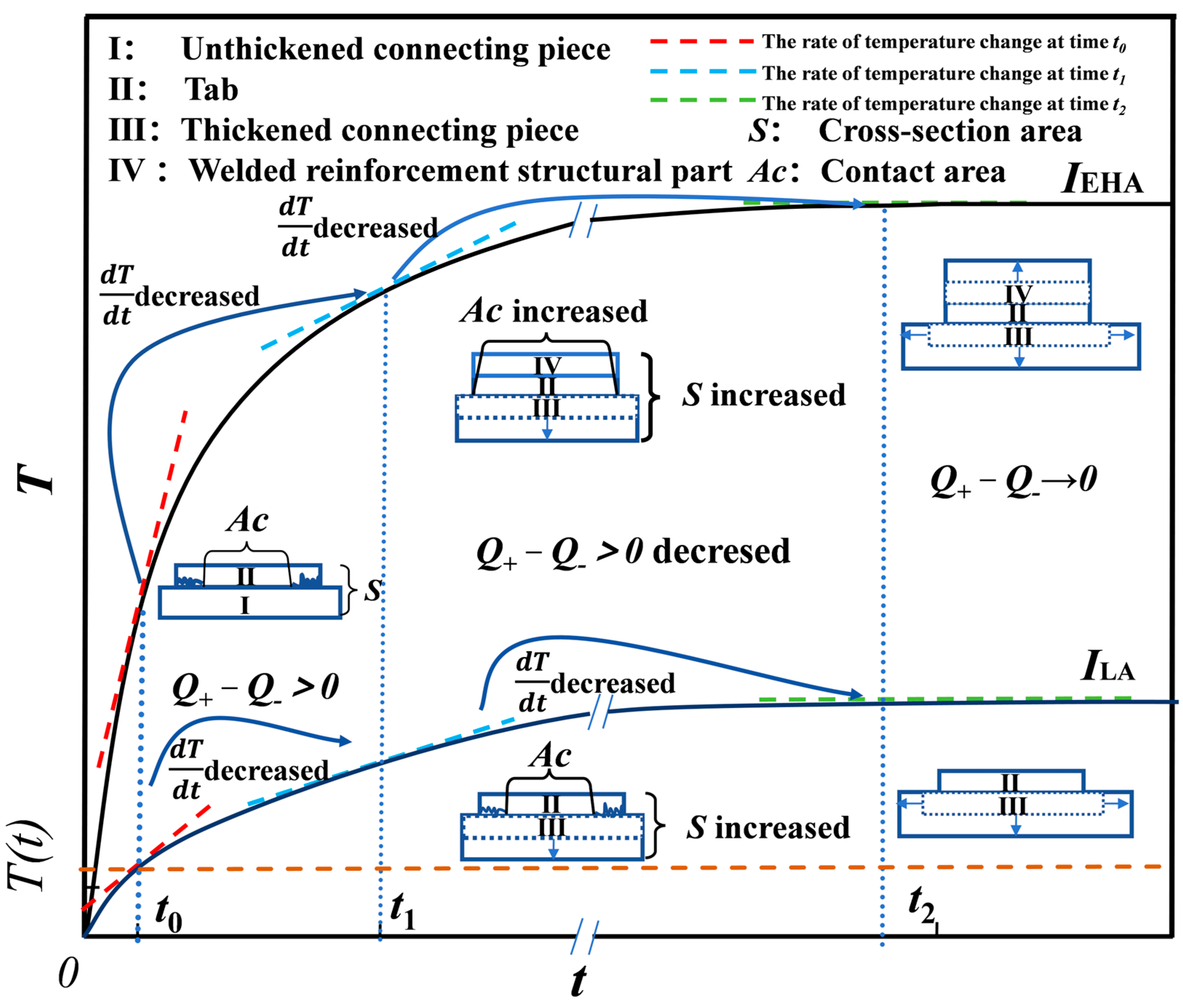
5.1. Dynamic Effect of Heat Generation and Heat Dissipation of Conductor Structures Under Extreme High-Amperage Condition
5.2. Applications and Limitations
6. Conclusions
- Through a comprehensive evaluation method involving theoretical derivation, multiphysics coupling finite element analysis, and experimental verification, it has been confirmed that both the cross-sectional area and the contact area of the conductor structures are closely linked to the battery’s ability to withstand extreme high-amperage current condition.
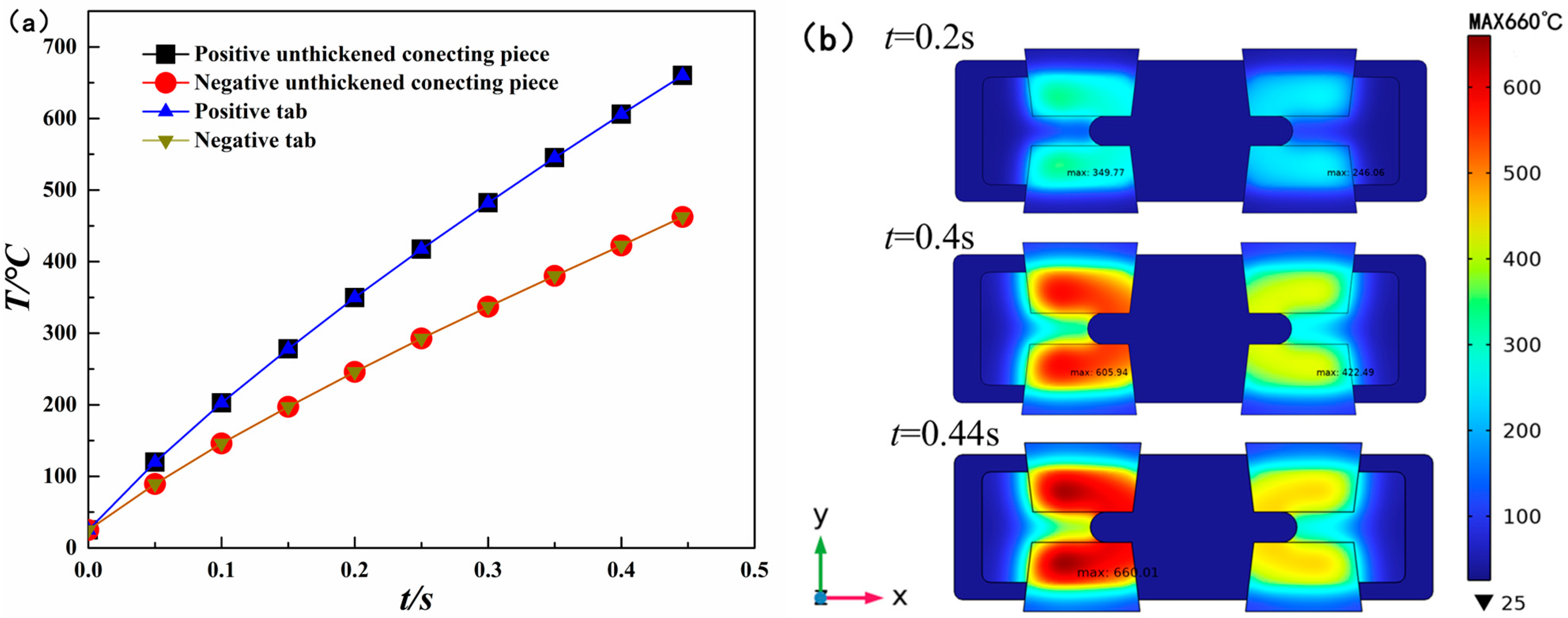
- Under extreme high-current conditions and fixed welding areas, the positive conductor structure reaches the fusing temperature threshold of 660 °C within 0.45 s, indicating rapid heat accumulation. By increasing the conductor’s cross-sectional area by 50%, the current-carrying duration improves modestly from 0.75 s to 0.8 s, demonstrating that enlarging the cross-sectional area alone is not sufficient for substantial thermal performance enhancement.
- The dynamic effects of heat generation and heat dissipation in conductor structures differ markedly from those observed under low-current conditions. For low currents, increasing the cross-sectional area promotes thermal balance. However, for extreme high-amperage currents, it is necessary to simultaneously adjust both the cross-sectional area (to reduce heat generation) and the contact area (to enhance heat dissipation efficiency) to balance the difference between heat generation and heat dissipation.
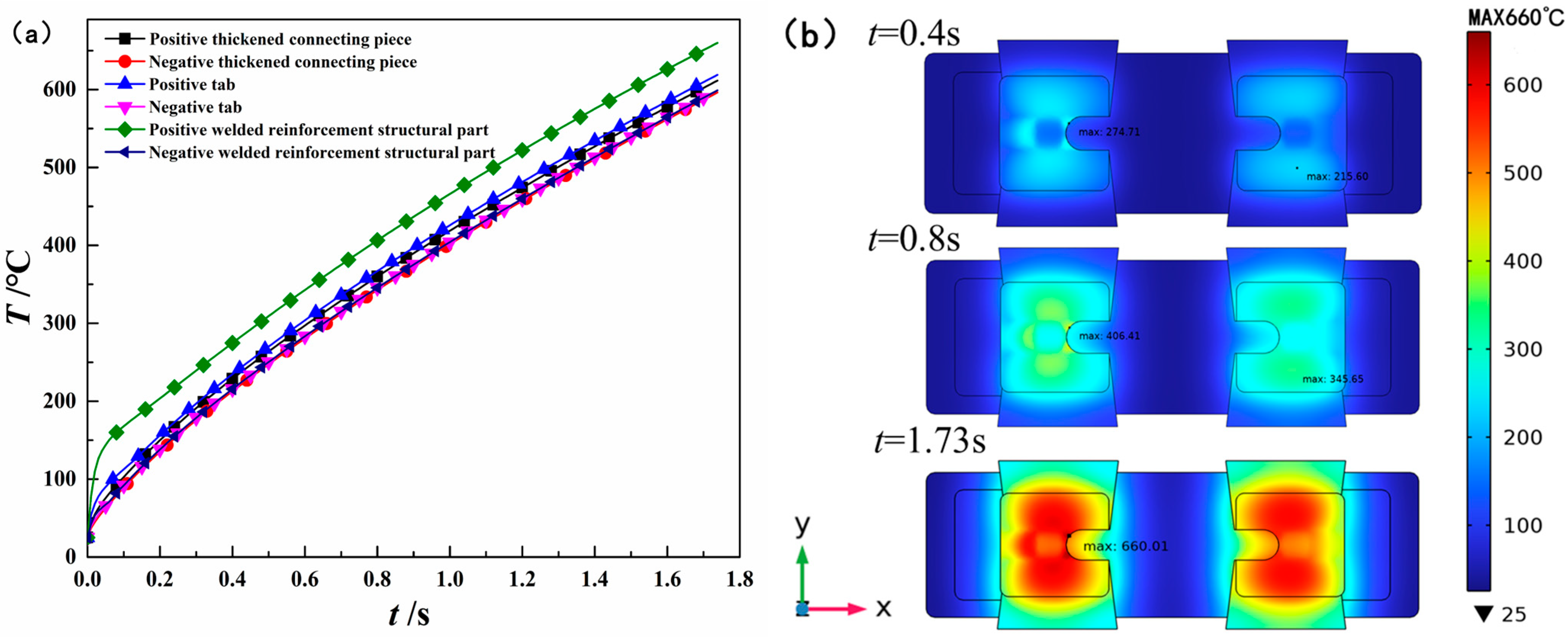
- By introducing a welded reinforcement structure, the thermal conduction path transitions from a “welding-dominant” to a “welding–contact synergy” model. This optimization involves a similar increase in cross-sectional area compared to the traditional design and an additional 100% increase in contact area, ultimately extending the current-carrying duration to 1.73 s (230% of traditional design’s current-carrying duration). This synergy between structure and thermal balance significantly enhances the current-handling capacity of the conductor.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wenjun, L.; Hangyu, X.; Qi, Y.; Jiuming, L.; Zhenyu, Z.; Shengbin, W.; Jiayue, P.; Bin, Z.; Xianglei, C.; Zhen, Z. Development of Strategies for High-Energy-Density Lithium Batteries. Energy Storage Sci. Technol. 2020, 9, 448–478. [Google Scholar]
- Domínguez-Navarro, J.A.; Dufo-López, R.; Yusta-Loyo, J.M.; Artal-Sevil, J.S.; Bernal-Agustín, J.L. Design of an Electric Vehicle Fast-Charging Station with Integration of Renewable Energy and Storage Systems. Int. J. Electr. Power Energy Syst. 2019, 105, 46–58. [Google Scholar] [CrossRef]
- Akram, U.; Nadarajah, M.; Shah, R.; Milano, F. A Review on Rapid Responsive Energy Storage Technologies for Frequency Regulation in Modern Power Systems. Renew. Sustain. Energy Rev. 2020, 120, 109626. [Google Scholar] [CrossRef]
- Wang, W.; Rui, C.; Wei, W.; Wugui, W. Design and Verification of a Current-Pulsed Power Supply for Special Loads. Chin. J. Ship Res. 2019, 14, 30–36. [Google Scholar]
- Ouyang, D.; Chen, M.; Huang, Q.; Weng, J.; Wang, Z.; Wang, J. A Review on the Thermal Hazards of the Lithium-Ion Battery and the Corresponding Countermeasures. Appl. Sci. 2019, 9, 2483. [Google Scholar] [CrossRef]
- Feng, X.; Zheng, S.; Ren, D.; He, X.; Wang, L.; Cui, H.; Liu, X.; Jin, C.; Zhang, F.; Xu, C.; et al. Investigating the Thermal Runaway Mechanisms of Lithium-Ion Batteries Based on Thermal Analysis Database. Appl. Energy 2019, 246, 53–64. [Google Scholar] [CrossRef]
- Tao, J.; Wang, S.; Cao, W.; Fernandez, C.; Blaabjerg, F. A Comprehensive Review of Multiple Physical and Data-Driven Model Fusion Methods for Accurate Lithium-Ion Battery Inner State Factor Estimation. Batteries 2024, 10, 442. [Google Scholar] [CrossRef]
- Chen, Z.; Liu, W.; Xia, T.; Pan, E. Consistency Evaluation for Lithium-Ion Battery Energy Storage Systems Based on Approximate Low-Rank Representation and Hypersphere Concentration. IEEE Trans. Ind. Electron. 2024, 72, 4811–4821. [Google Scholar] [CrossRef]
- Ba, L.M.; Tang, K.; Kang, L.B.; Pan, G.H.; He, G.L. Thermal Effects and Structure Optimization of High Power Li-Ion Batteries. Chin. J. Power Sources 2018, 42, 3–7. [Google Scholar]
- Kong, L.; Li, Y.; Feng, W. Strategies to Solve Lithium Battery Thermal Runaway: From Mechanism to Modification. Electrochem. Energy Rev. 2021, 4, 633–679. [Google Scholar] [CrossRef]
- Finegan, D.P.; Darcy, E.; Keyser, M.; Tjaden, B.; Heenan, T.M.M.; Jervis, R.; Bailey, J.J.; Vo, N.T.; Magdysyuk, O.V.; Drakopoulos, M.; et al. Identifying the Cause of Rupture of Li-Ion Batteries during Thermal Runaway. Adv. Sci. 2018, 5, 1700369. [Google Scholar] [CrossRef]
- Tang, Y.; Zhang, Y.; Li, W.; Ma, B.; Chen, X. Rational Material Design for Ultrafast Rechargeable Lithium-Ion Batteries. Chem. Soc. Rev. 2015, 44, 5926–5940. [Google Scholar] [CrossRef]
- Xue, N.; Du, W.; Martins, J.R.R.A.; Shyy, W. Lithium-Ion Batteries: Thermomechanics, Performance, and Design Optimization. In Handbook of Clean Energy Systems; Wiley Online Library: Minneapolis, MN, USA, 2015; pp. 1–16. [Google Scholar] [CrossRef]
- Zaghib, K.; Dontigny, M.; Guerfi, A.; Charest, P.; Rodrigues, I.; Mauger, A.; Julien, C.M. Safe and Fast-Charging Li-Ion Battery with Long Shelf Life for Power Applications. J. Power Sources 2011, 196, 3949–3954. [Google Scholar] [CrossRef]
- Wang, J.; Sun, X. Understanding and Recent Development of Carbon Coating on LiFePO 4 Cathode Materials for Lithium-Ion Batteries. Energy Environ. Sci. 2012, 5, 5163–5185. [Google Scholar] [CrossRef]
- Cheng, G.; Gao, L.; Gu, H.; Wang, K. Developing and Fast Charge Performance of High Power Li-Ion Battery. Batter. Bimon. 2019, 049, 94–97. [Google Scholar]
- He, T.; Liu, W.; Mai, M.; Qi, J.; Lei, Y. Design of Fast Charging Electrolyte for Lithium Iron Phosphate. SINO-Glob. Energy 2024, 29, 29–35. [Google Scholar]
- Lei, S.; Zeng, Z.; Cheng, S.; Xie, J. Fast-charging of Lithium-ion Batteries: A Review of Electrolyte Design Aspects. Batter. Energy 2023, 2, 20230018. [Google Scholar] [CrossRef]
- Ai, X.; Song, K.; Guo, M.; Liu, X.; Zhang, Y.; Hu, B. Effect of Amorphous Carbon Coated Graphite Anode on Fast Charge Performance of Lithium Battery. Carbon N. Y. 2023, 4, 1–6. [Google Scholar]
- Balogun, M.-S.; Zhu, Y.; Qiu, W.; Luo, Y.; Huang, Y.; Liang, C.; Lu, X.; Tong, Y. Chemically Lithiated TiO 2 Heterostructured Nanosheet Anode with Excellent Rate Capability and Long Cycle Life for High-Performance Lithium-Ion Batteries. ACS Appl. Mater. Interfaces 2015, 7, 25991–26003. [Google Scholar] [CrossRef]
- Wang, D.; Liu, H.; Li, M.; Wang, X.; Bai, S.; Shi, Y.; Tian, J.; Shan, Z.; Meng, Y.S.; Liu, P.; et al. Nanosheet-Assembled Hierarchical Li4Ti5O12 Microspheres for High-Volumetric-Density and High-Rate Li-Ion Battery Anode. Energy Storage Mater. 2019, 21, 361–371. [Google Scholar] [CrossRef]
- Afaynou, I.; Faraji, H.; Choukairy, K.; Khallaki, K.; Akrour, D. Effectiveness of a PCM-Based Heat Sink with Partially Filled Metal Foam for Thermal Management of Electronics. Int. J. Heat Mass Transf. 2024, 235, 126196. [Google Scholar] [CrossRef]
- Kaliaperumal, M.; Dharanendrakumar, M.S.; Prasanna, S.; Abhishek, K.V.; Chidambaram, R.K.; Adams, S.; Zaghib, K.; Reddy, M.V. Cause and Mitigation of Lithium-Ion Battery Failure—A Review. Materials 2021, 14, 5676. [Google Scholar] [CrossRef] [PubMed]
- Zhu, P.; Gastol, D.; Marshall, J.; Sommerville, R.; Goodship, V.; Kendrick, E. A Review of Current Collectors for Lithium-Ion Batteries. J. Power Sources 2021, 485, 229321. [Google Scholar] [CrossRef]
- Zhang, M.; Liu, L.; Stefanopoulou, A.; Siegel, J.; Lu, L.; He, X.; Ouyang, M. Fusing Phenomenon of Lithium-Ion Battery Internal Short Circuit. J. Electrochem. Soc. 2017, 164, A2738–A2745. [Google Scholar] [CrossRef]
- Grandjean, T.; Barai, A.; Hosseinzadeh, E.; Guo, Y.; McGordon, A.; Marco, J. Large Format Lithium Ion Pouch Cell Full Thermal Characterisation for Improved Electric Vehicle Thermal Management. J. Power Sources 2017, 359, 215–225. [Google Scholar] [CrossRef]
- Lai, Y.; Du, S.; Ai, L.; Ai, L.; Cheng, Y.; Tang, Y.; Jia, M. Insight into Heat Generation of Lithium Ion Batteries Based on the Electrochemical-Thermal Model at High Discharge Rates. Int. J. Hydrogen Energy 2015, 40, 13039–13049. [Google Scholar] [CrossRef]
- Jiang, F.; Peng, P.; Sun, Y. Thermal Analyses of LiFePO4/Graphite Battery Discharge Processes. J. Power Sources 2013, 243, 181–194. [Google Scholar] [CrossRef]
- Drake, S.J.; Martin, M.; Wetz, D.A.; Ostanek, J.K.; Miller, S.P.; Heinzel, J.M.; Jain, A. Heat Generation Rate Measurement in a Li-Ion Cell at Large C-Rates through Temperature and Heat Flux Measurements. J. Power Sources 2015, 285, 266–273. [Google Scholar] [CrossRef]
- Basu, S.; Patil, R.S.; Ramachandran, S.; Hariharan, K.S.; Kolake, S.M.; Song, T.; Oh, D.; Yeo, T.; Doo, S. Non-Isothermal Electrochemical Model for Lithium-Ion Cells with Composite Cathodes. J. Power Sources 2015, 283, 132–150. [Google Scholar] [CrossRef]
- Jin, Y.; Zhao, W.; Li, Z.; Liu, B.; Liu, L. Modeling and Simulation of Lithium-Ion Battery Considering the Effect of Charge-Discharge State. J. Phys. Conf. Ser. 2021, 1907, 012003. [Google Scholar] [CrossRef]
- Panchal, S.; Rashid, M.; Long, F.; Mathew, M.; Fraser, R.; Fowler, M. Degradation Testing and Modeling of 200 Ah LiFePO4 Battery; SAE Technical Paper 2018-01-0441; SAE: Detroit Michigan, MI, USA, 3 April 2018. [Google Scholar] [CrossRef]
- Burke, A.; Miller, M. Life Cycle Testing of Lithium Batteries for Fast Charging and Second-Use Applications. In Proceedings of the 2013 World Electric Vehicle Symposium and Exhibition (EVS27), Barcelona, Spain, 17–20 November 2013; pp. 1–10. [Google Scholar]
- Ferguson, S.; Brenner, C.; Cox, J.; Foote, B.; Hensley, K.; Ruth, N. Saft’s Xcelion 6T® 28V Lithium Ion Batteries for Military Vehicles. In Proceedings of the 2017 Ndia Ground Vehicle Systems Engineering and Technology Symposium, Power & Mobility (P&M) Technical Session, Novi, MI, USA, 8–10 August 2017. [Google Scholar]
- Bingkun, G. Lithium-Ion Batteries; Central South University Press: Changsha, China, 2005; ISBN 7-81061-5632-7. [Google Scholar]
- Scrosati, B. Lithium Batteries: Science and Technology. Electrochim. Acta 2004, 49, 2215–2216. [Google Scholar] [CrossRef]
- Bo, L. Research on the Safety Performance of Lithium-Ion Batteries; Central South University Press: Changsha, China, 2014; ISBN 9787548712435. [Google Scholar]
- Maher, K.; Boumaiza, A.; Amin, R. Understanding the Heat Generation Mechanisms and the Interplay between Joule Heat and Entropy Effects as a Function of State of Charge in Lithium-Ion Batteries. J. Power Sources 2024, 623, 235504. [Google Scholar] [CrossRef]
- Lei, S.; Yanzhe, X.; Zhuoran, Z. Research of Heating Power of Lithium-Ion Battery Based on Discharging Curve. Mar. Electr. Electron. Technol. 2022, 42, 61–64. [Google Scholar] [CrossRef]
- Mama, M.; Solai, E.; Capurso, T.; Danlos, A.; Khelladi, S. Comprehensive Review of Multi-Scale Lithium-Ion Batteries Modeling: From Electro-Chemical Dynamics up to Heat Transfer in Battery Thermal Management System. Energy Convers. Manag. 2025, 325, 119223. [Google Scholar] [CrossRef]
- Tang, M.; Wu, C.; Peng, W.; Han, R.; Zhang, S.; Wang, D. Numerical Simulation Study on the Impact of Convective Heat Transfer on Lithium Battery Air Cooling Thermal Model. Appl. Therm. Eng. 2024, 257, 124220. [Google Scholar] [CrossRef]
- Sun, H.; Tian, W.; Yue, J.; Su, F. Lithium-Ion Battery Heterogeneous Electrochemical-Thermal-Mechanical Multiphysics Coupling Model and Characterization of Microscopic Properties. J. Power Sources 2025, 629, 235970. [Google Scholar] [CrossRef]
- Alkhedher, M.; Al Tahhan, A.B.; Yousaf, J.; Ghazal, M.; Shahbazian-Yassar, R.; Ramadan, M. Electrochemical and Thermal Modeling of Lithium-Ion Batteries: A Review of Coupled Approaches for Improved Thermal Performance and Safety Lithium-Ion Batteries. J. Energy Storage 2024, 86, 111172. [Google Scholar] [CrossRef]
- Wang, Y.; Ni, R.; Jiang, X.; Yin, M.; Zhang, D.; Xie, Z. An Electrochemical-Mechanical Coupled Multi-Scale Modeling Method and Full-Field Stress Distribution of Lithium-Ion Battery. Appl. Energy 2023, 347, 121444. [Google Scholar] [CrossRef]
- Liu, Y.; Tang, S.; Li, L.; Liu, F.; Jiang, L.; Jia, M.; Ai, Y.; Yao, C.; Gu, H. Simulation and Parameter Identification Based on Electrochemical- Thermal Coupling Model of Power Lithium Ion-Battery. J. Alloys Compd. 2020, 844, 156003. [Google Scholar] [CrossRef]
- Qiu, C.; He, G.; Shi, W.; Zou, M.; Liu, C. The Polarization Characteristics of Lithium-Ion Batteries under Cyclic Charge and Discharge. J. Solid State Electrochem. 2019, 23, 1887–1902. [Google Scholar] [CrossRef]
- Zhao, D.; Chen, W. Analysis of Polarization and Thermal Characteristics in Lithium-Ion Battery with Various Electrode Thicknesses. J. Energy Storage 2023, 71, 108159. [Google Scholar] [CrossRef]
- Yang, F.; Qiao, Y.; Gao, B.; Duan, P.; Zhu, Y. Investigation on Li-Ion Battery Charging Polarization Characteristics and Influence Factors. Ionics 2016, 22, 1603–1610. [Google Scholar] [CrossRef]
- Xie, W.; Guo, P.; Gao, X. Elucidating the Rate Limitation of Lithium-Ion Batteries under Different Charging Conditions through Polarization Analysis. J. Energy Storage 2024, 82, 110554. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, M.; Yang, D. Investigation of the Polarization Effect in Lithium Iron Phosphate Battery for Electric Vehicles. In Proceedings of the 2014 IEEE Conference and Expo Transportation Electrification Asia-Pacific (ITEC Asia-Pacific), Beijing, China, 31 August–3 September 2014; pp. 1–5. [Google Scholar] [CrossRef]
- Wimarshana, B.; Bin-Mat-Arishad, I.; Fly, A. A Multi-Step Parameter Identification of a Physico-Chemical Lithium-Ion Battery Model with Electrochemical Impedance Data. J. Power Sources 2023, 580, 233400. [Google Scholar] [CrossRef]
- Li, Z.; Gao, M.; Zhao, X.; Cai, X.; Zhang, Y. Heat Generation Effect and Failure Mechanism of Pouch-Type Lithium-Ion Battery under over-Discharge for Electric Vehicle. J. Energy Storage 2024, 76, 109759. [Google Scholar] [CrossRef]
- Taheri, P.; Hsieh, S.; Bahrami, M. Investigating Electrical Contact Resistance Losses in Lithium-Ion Battery Assemblies for Hybrid and Electric Vehicles. J. Power Sources 2011, 196, 6525–6533. [Google Scholar] [CrossRef]
- Mo, J.; Zhang, G.; Zhang, J.; Mo, C.; Wang, B.; Guo, S.; Jiang, R.; Liu, J.; Peng, K. Effect of Cold Welding on the Inconsistencies and Thermal Safety of Battery Modules Based on a Constructed Discharge Model. Appl. Energy 2025, 377, 124570. [Google Scholar] [CrossRef]
- Brand, M.J.; Schmidt, P.A.; Zaeh, M.F.; Jossen, A. Welding Techniques for Battery Cells and Resulting Electrical Contact Resistances. J. Energy Storage 2015, 1, 7–14. [Google Scholar] [CrossRef]


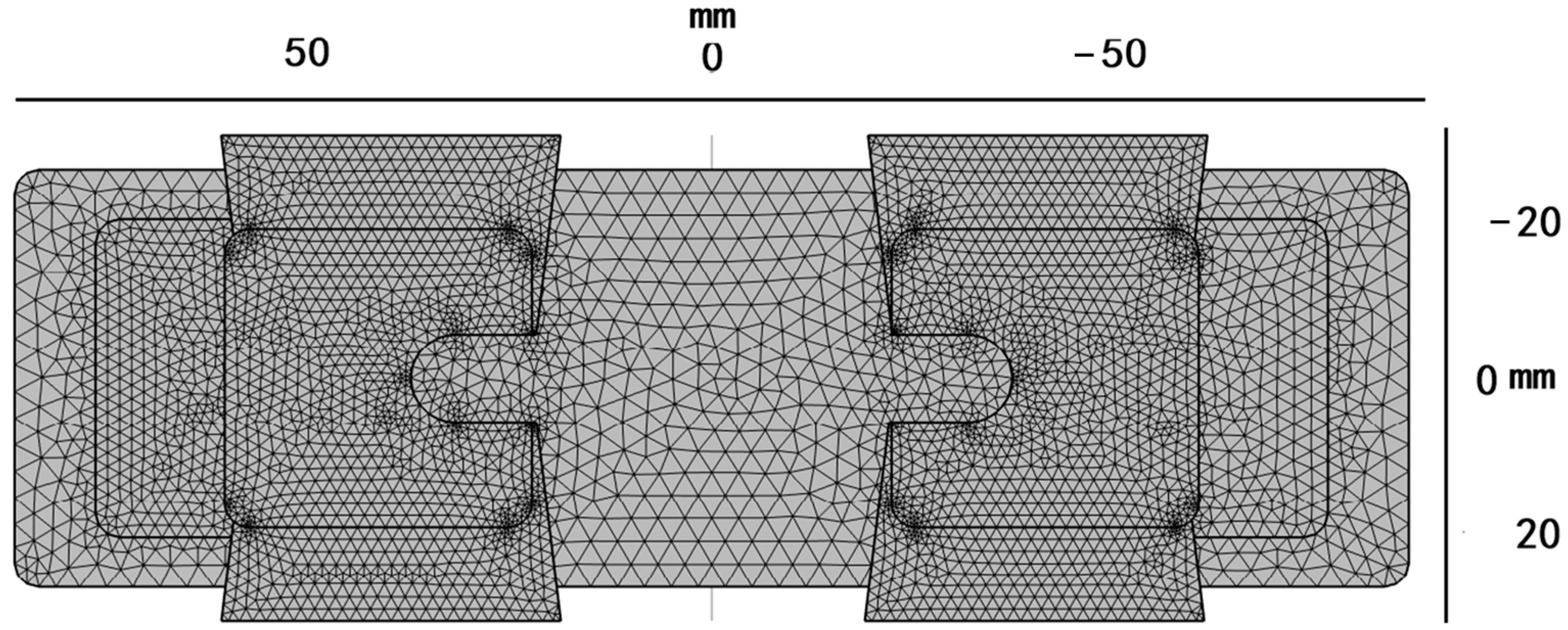

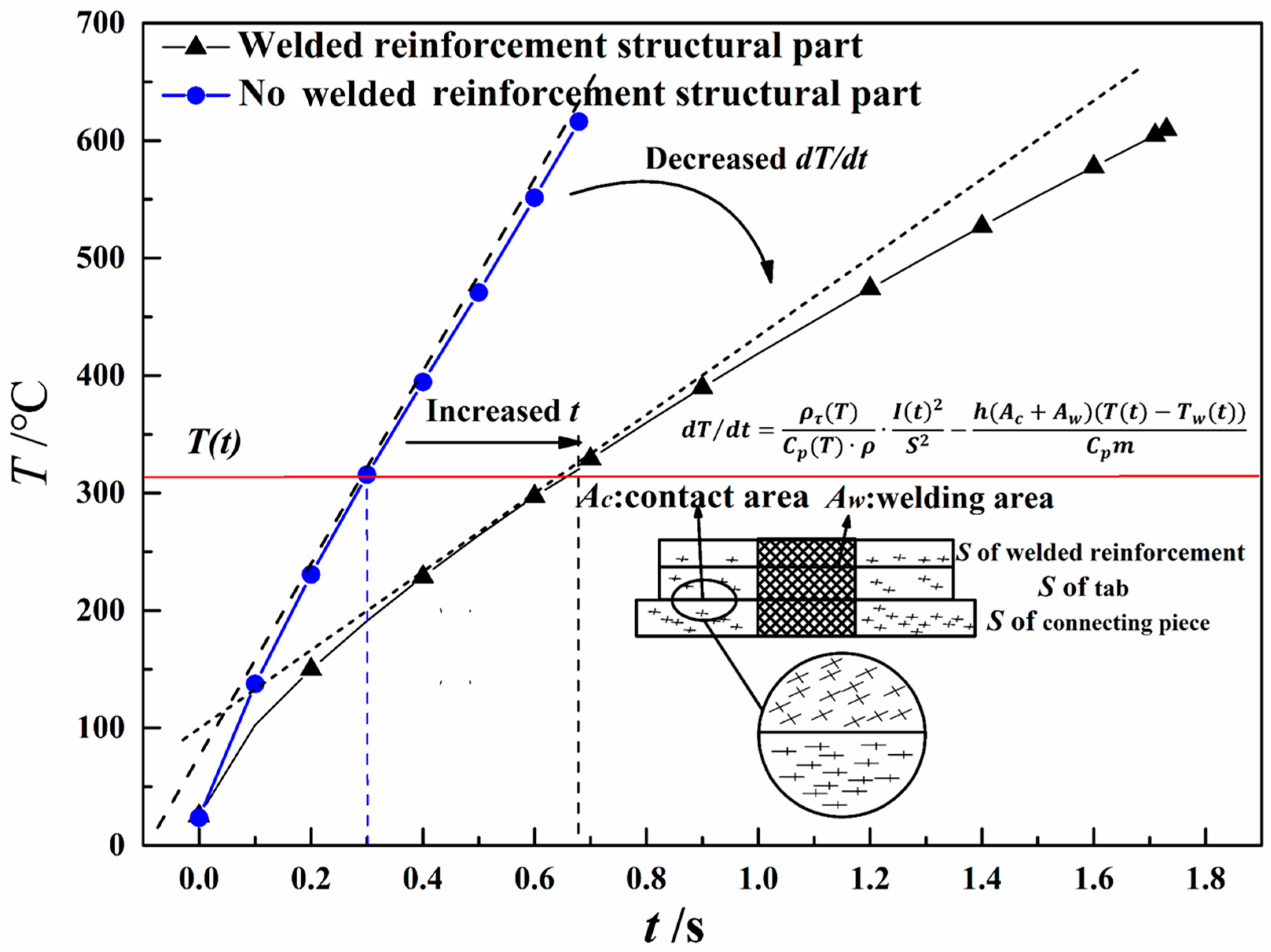
| Structural Parts | Density (kg/m3) | Specific Heat Capacity (J/kg°C) | Thermal Conductivity (w/m2·K) |
|---|---|---|---|
| Positive connecting piece | 2.7 × 103 | 8.8 × 102 | 237 |
| Negative connecting piece | 8.9 × 103 | 3.9 × 102 | 394 |
| Positive tab | 2.7 × 103 | 8.8 × 102 | 237 |
| Negative tab | 8.9 × 103 | 3.9 × 102 | 394 |
| Positive welded reinforcement structural part | 2.7 × 103 | 8.8 × 102 | 237 |
| Negative welded reinforcement structural part | 8.9 × 103 | 3.9 × 102 | 394 |
| Structural Parts | Dimensions (L × W × D/mm) |
|---|---|
| Unthickened connecting piece | 54 × 40 × 1 |
| Thickened connecting piece | 54 × 40 × 1.5 |
| Welded reinforcement structural part | 38 × 37.5 × 0.2 (thickness before welding) |
| Configuration Groups | Cross-Sectional Area of Overflow (mm2) | Solder Area (mm2) | Contact Area of Overflow (mm2) |
|---|---|---|---|
| C0 | 54 | 100 | 348 |
| T1 | 81 | 100 | 348 |
| T2 | 88.5 | 100 | 580 |
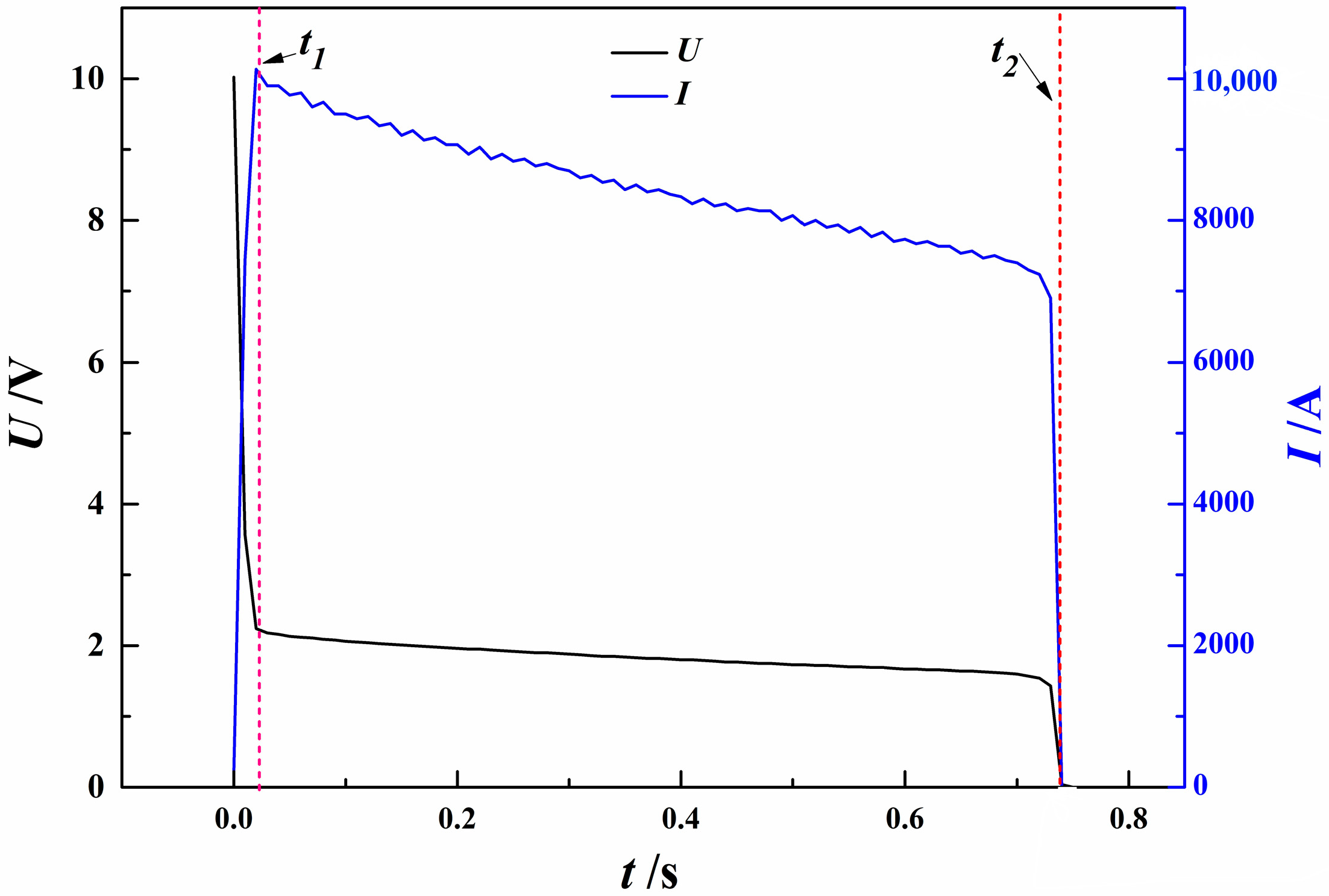
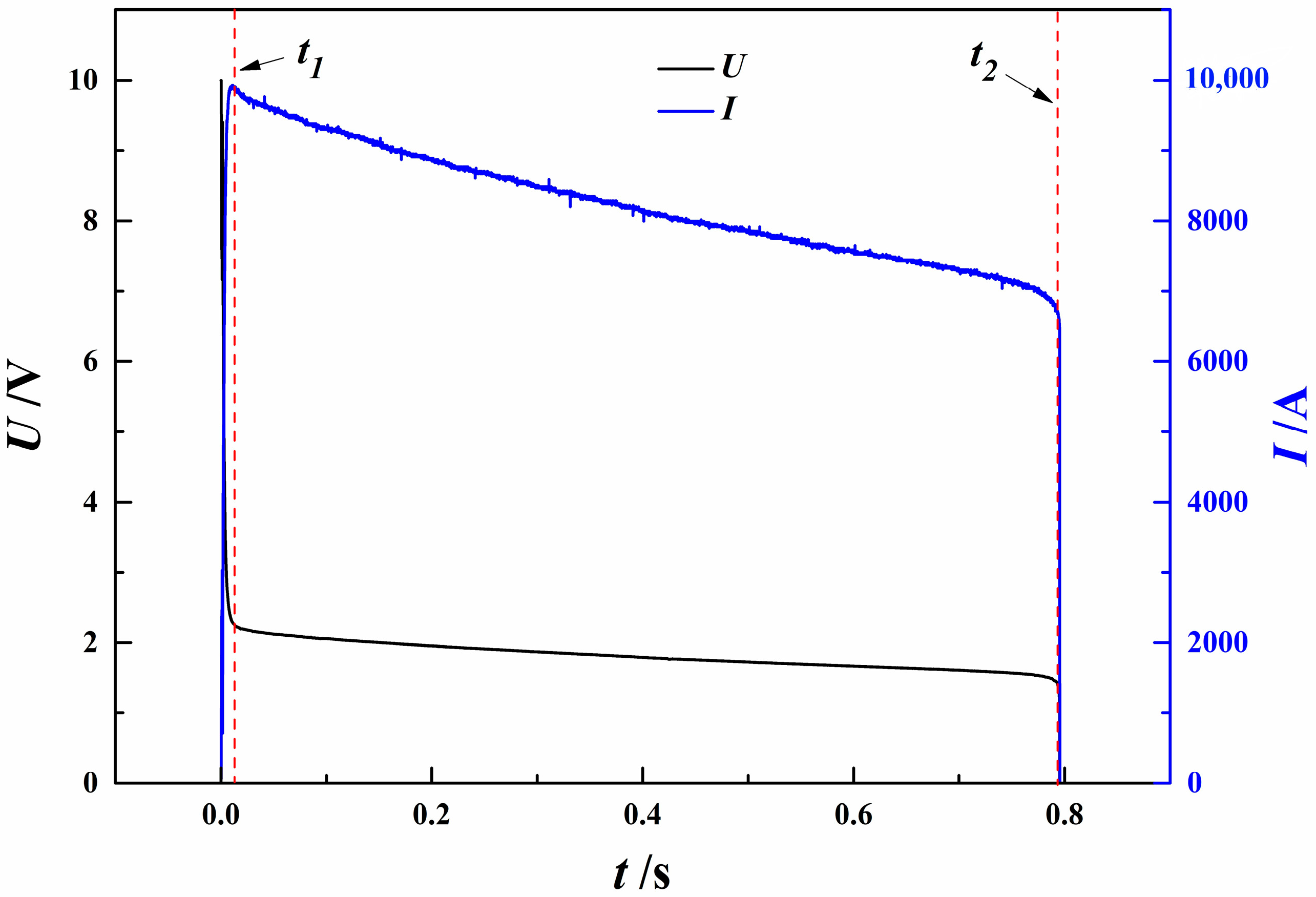
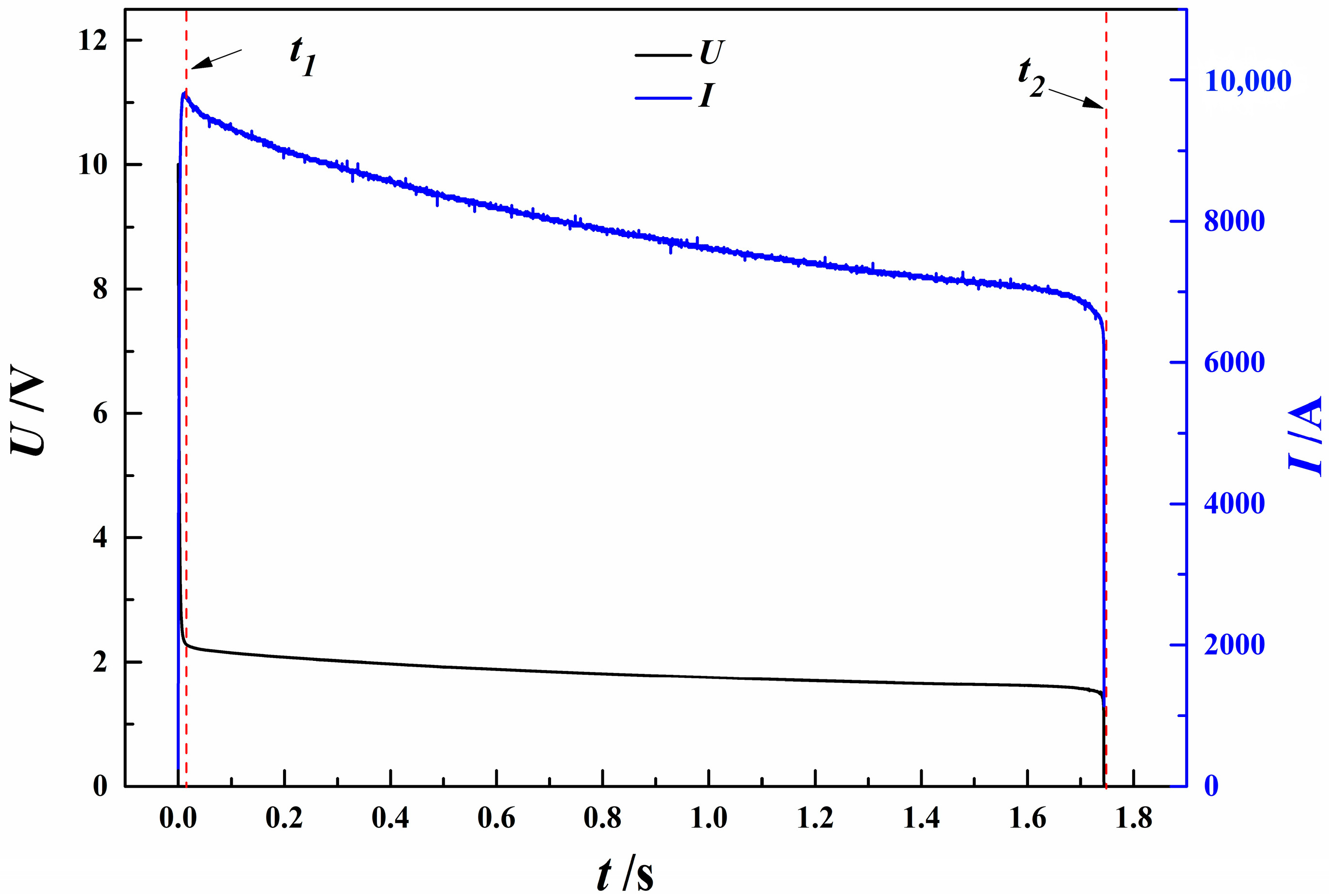
| Configuration Group | Test Current-Carrying Time (s) | Simulated Current-Carrying Time (s) |
|---|---|---|
| Control Group (C0) | 0.75 | 0.44 |
| 0.98 | ||
| 0.79 | ||
| Test Group 1 (T1) | 0.80 | 0.68 |
| 1.2 | ||
| 0.95 | ||
| Test Group 2 (T2) | 1.73 | 1.73 |
| 1.89 | ||
| 1.74 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Wang, Y.; Liu, H.; Liu, Y.; Yang, X. Thermal–Electrical Optimization of Lithium-Ion Battery Conductor Structures Under Extreme High Amperage Current. Appl. Sci. 2025, 15, 5338. https://doi.org/10.3390/app15105338
Guo J, Wang Y, Liu H, Liu Y, Yang X. Thermal–Electrical Optimization of Lithium-Ion Battery Conductor Structures Under Extreme High Amperage Current. Applied Sciences. 2025; 15(10):5338. https://doi.org/10.3390/app15105338
Chicago/Turabian StyleGuo, Jingdi, Yiran Wang, He Liu, Yahui Liu, and Xiaokang Yang. 2025. "Thermal–Electrical Optimization of Lithium-Ion Battery Conductor Structures Under Extreme High Amperage Current" Applied Sciences 15, no. 10: 5338. https://doi.org/10.3390/app15105338
APA StyleGuo, J., Wang, Y., Liu, H., Liu, Y., & Yang, X. (2025). Thermal–Electrical Optimization of Lithium-Ion Battery Conductor Structures Under Extreme High Amperage Current. Applied Sciences, 15(10), 5338. https://doi.org/10.3390/app15105338






