New Methods of Series Expansions between Three Anomalies
Abstract
1. Introduction
2. Materials and Methods
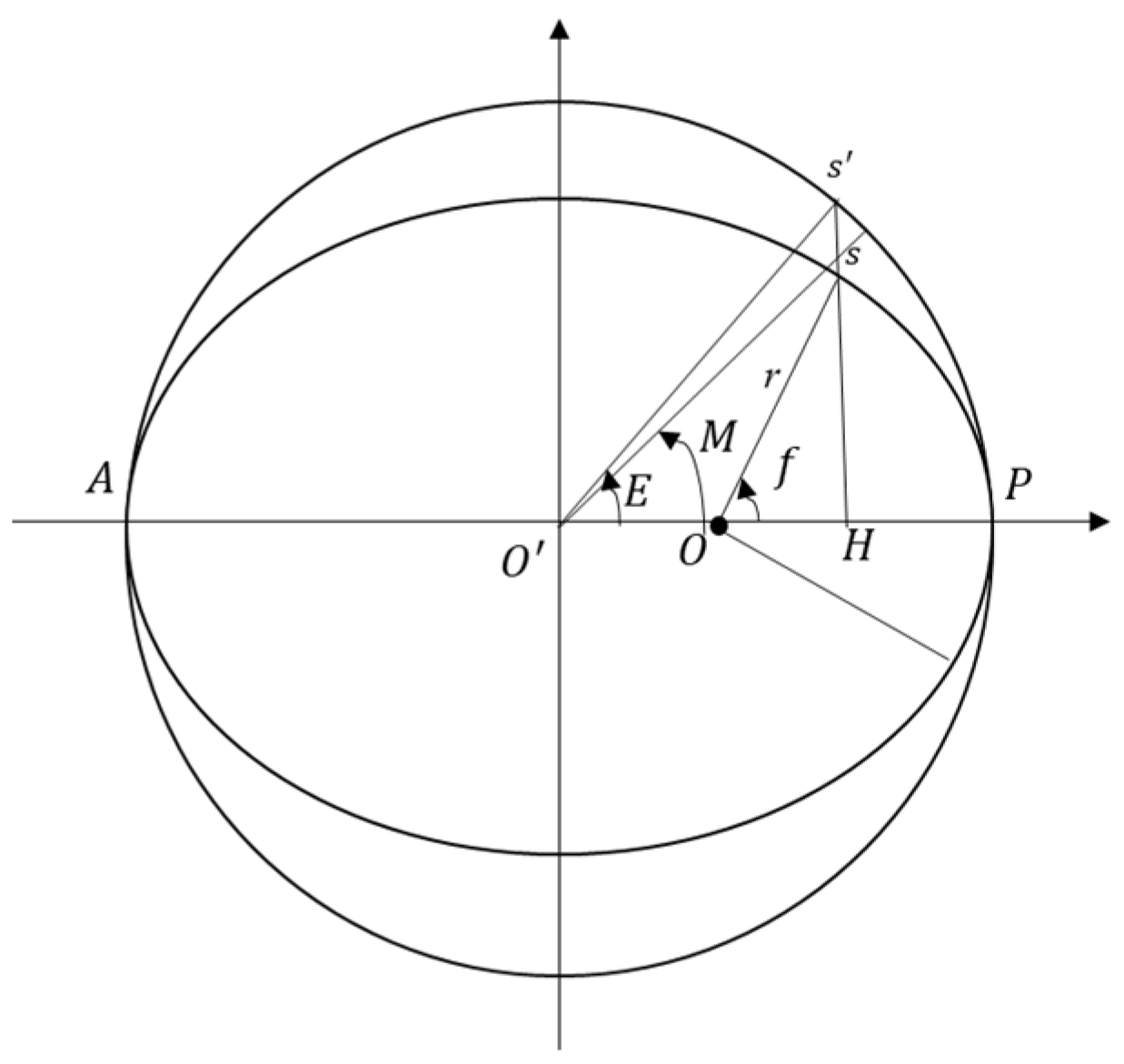
2.1. The Geometric Relationship among True Anomaly, Elliptic Anomaly, and Mean Anomaly
2.2. The Derivation of the Expansion Formula for the Relationship between True Anomaly and Elliptic Anomaly
2.3. The Derivation of the Expansion Formula for the Relationship between Elliptic Anomaly and Mean Anomaly
Comparison of Efficiency
2.4. The Derivation of the Expansion Formula for the Relationship between True Anomaly and Mean Anomaly
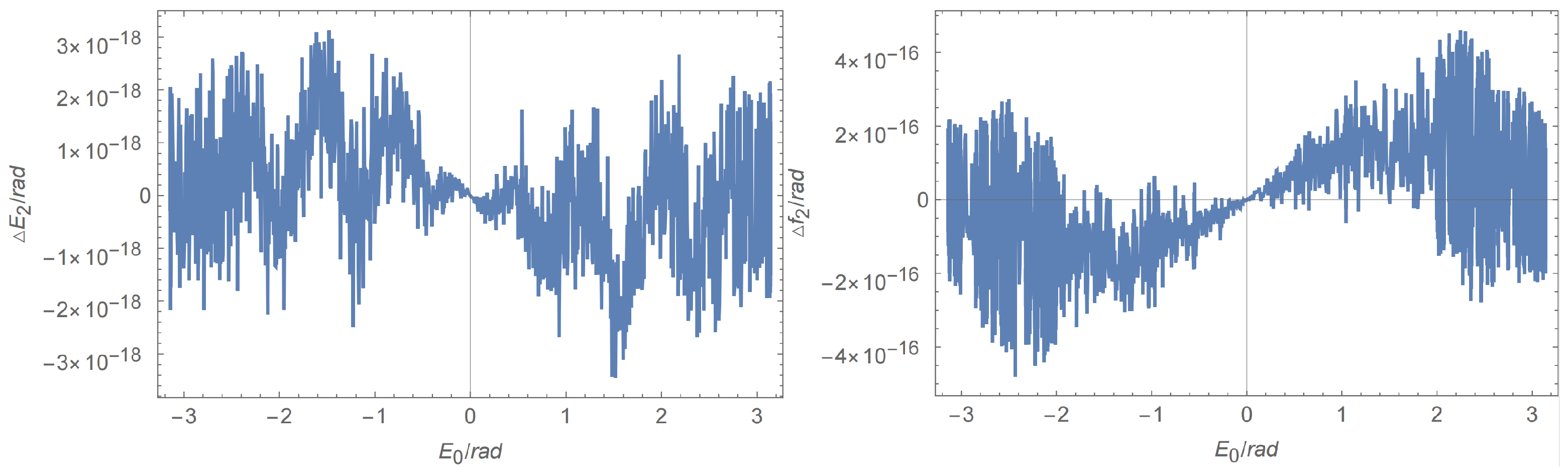
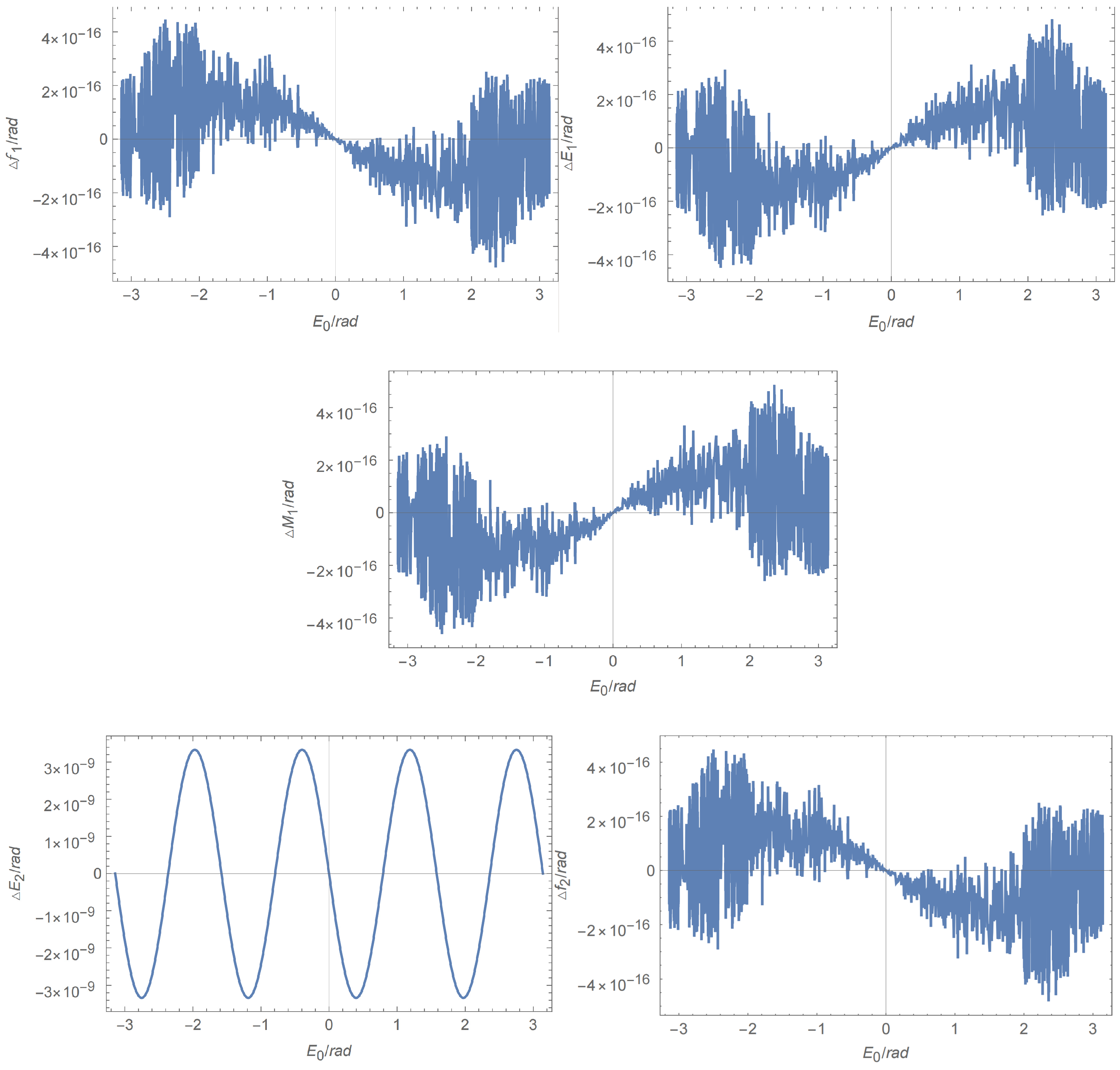
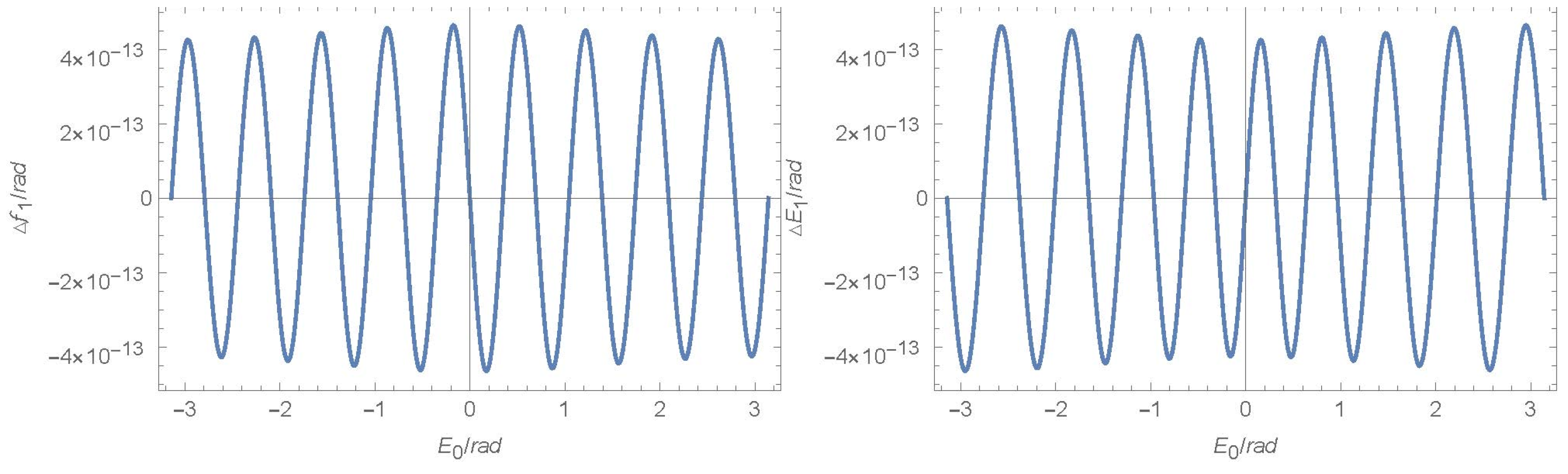
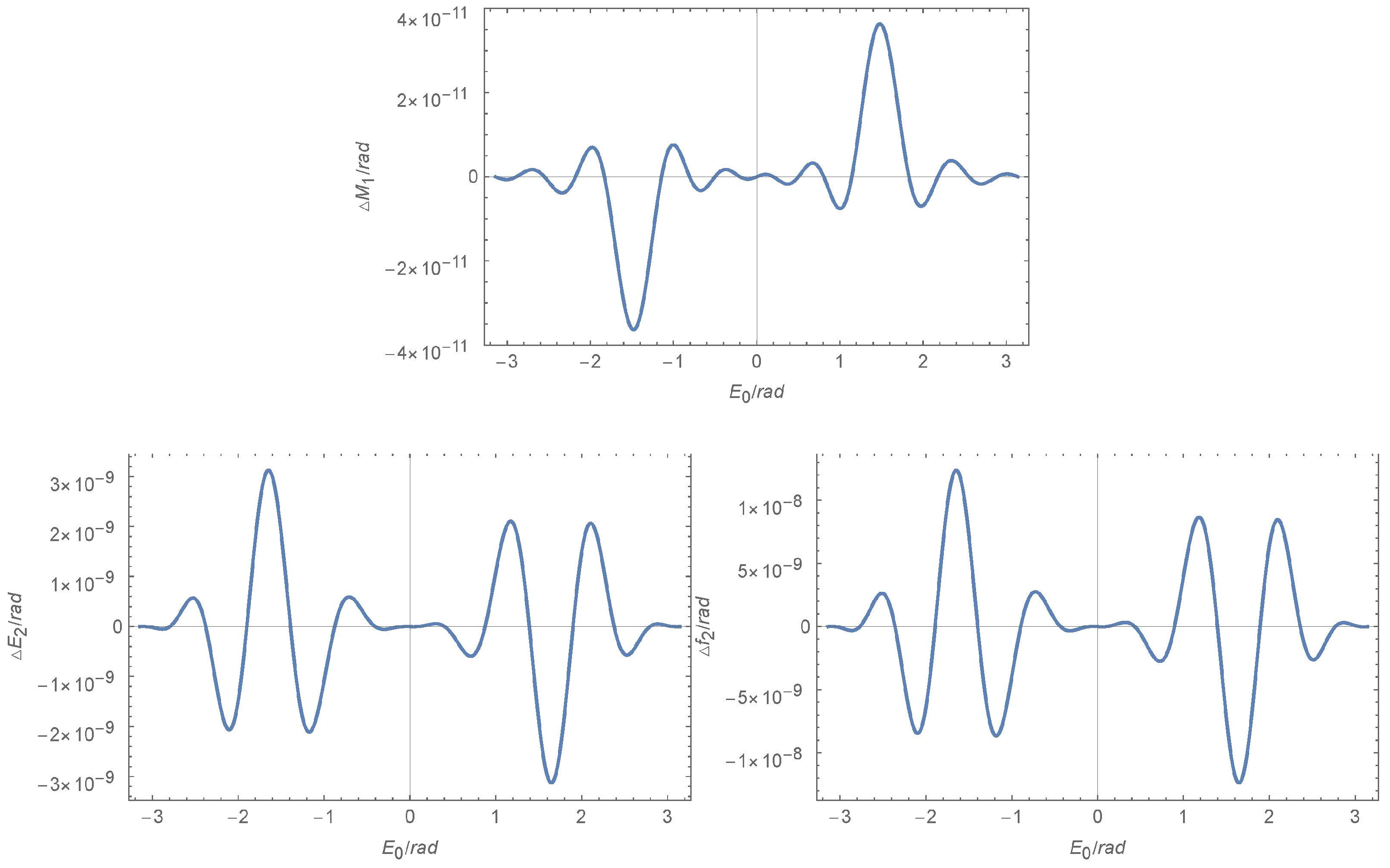
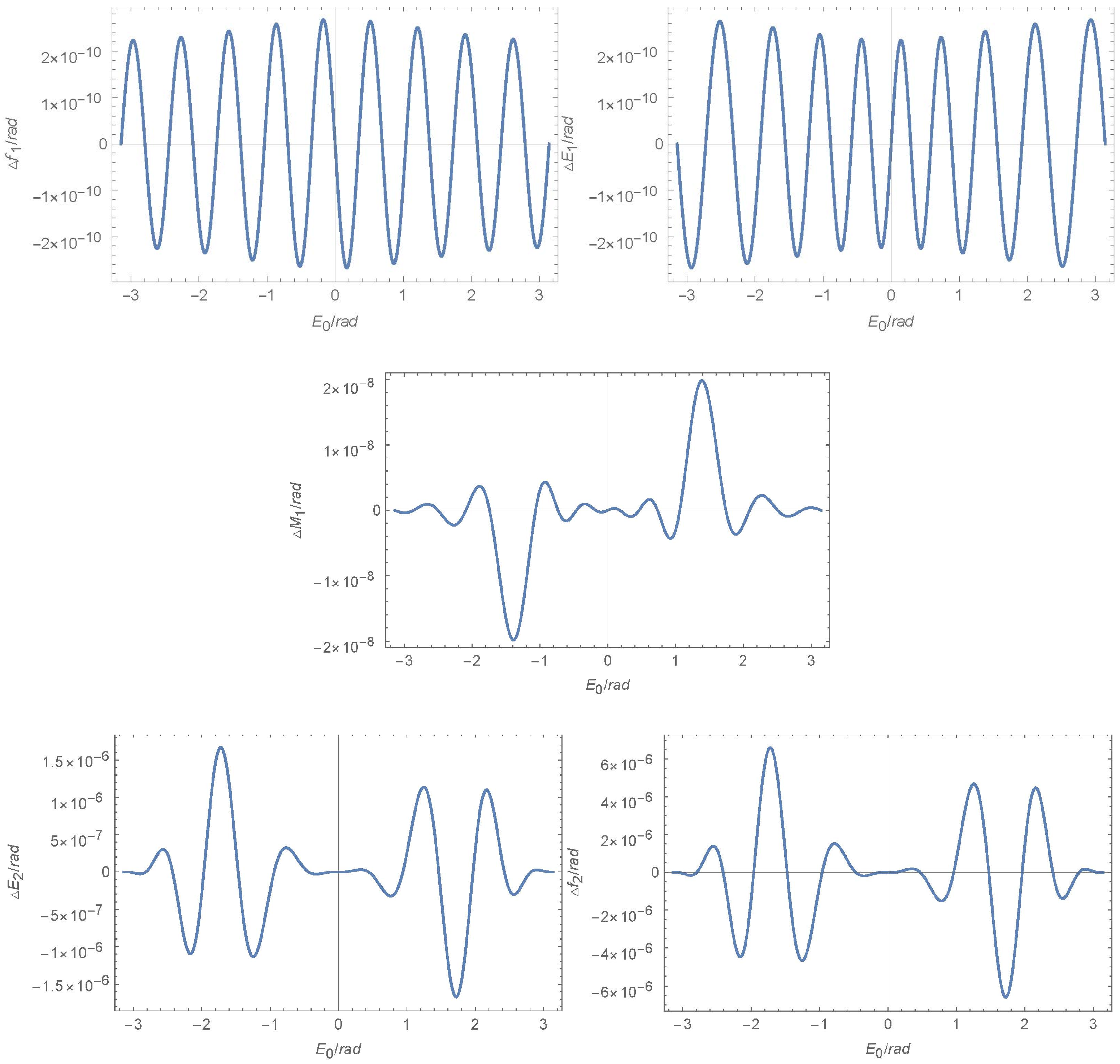
2.5. Error Analysis
- These errors of the trigonometric series expansion of the three anomalies increase with the increase in eccentricity e.
- These error plots of the trigonometric series expansions with parameters m and e for three anomalies are different, but they all exhibit central symmetry.
- When e = 0.01, these errors , , , , and calculated with parameter m are better than 10−15. When , these errors are better than 10−7. When e = 0.2, these errors are better than 10−5.
- When e = 0.01, these errors , , , , and calculated with parameter e are better than 10−15. When , these errors are better than 10−8. When e = 0.2, the errors are better than 10−5.
- The transformation formula between the true anomaly f and the elliptic anomaly E with the parameter m is simpler in form than the formula with the parameter e.
- The transformation formula between the true anomaly f and the mean anomaly M with the parameter m is simpler in form than the formula with the parameter e.
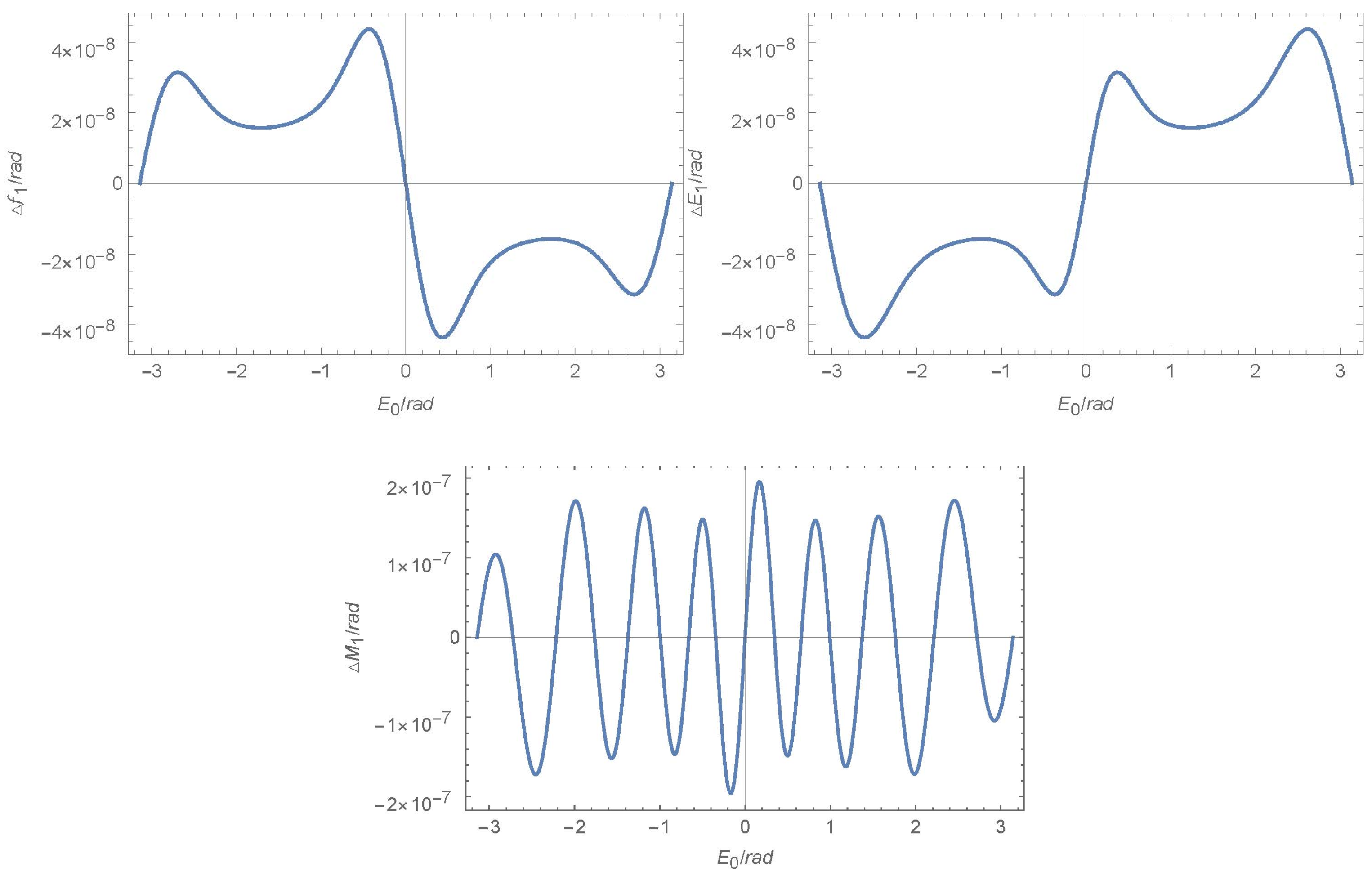
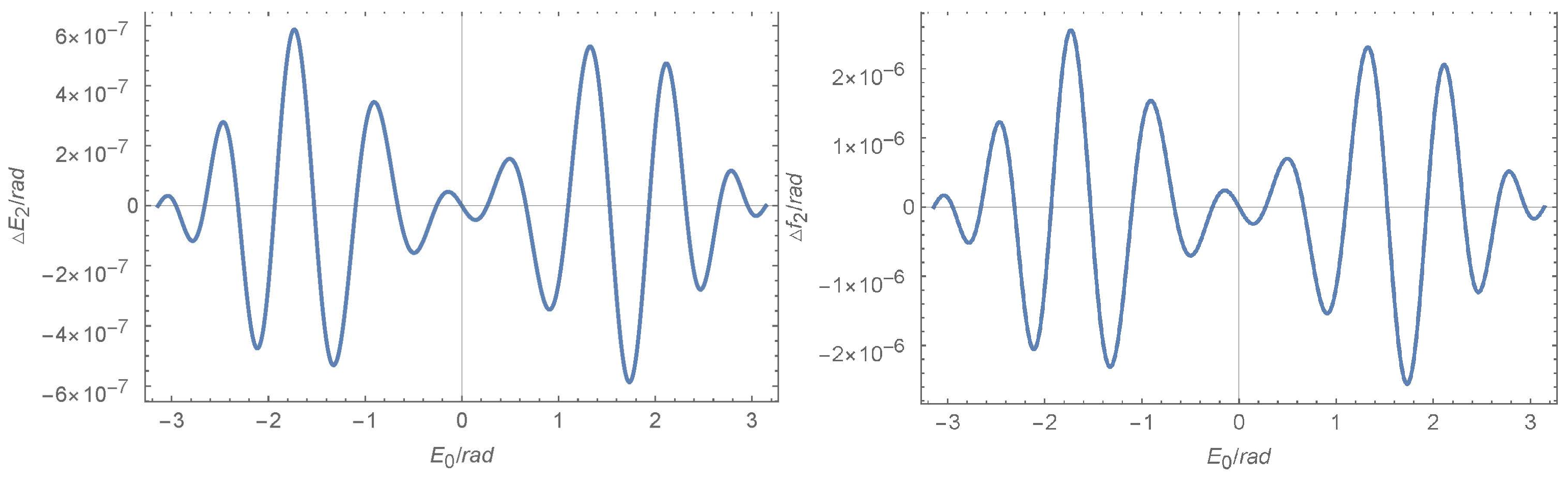
2.6. Symbolic Expression for Extreme Value of Differences among Three Anomalies
2.6.1. Symbolic Expression for Extreme Value of Difference between Elliptic and Mean Anomalies
2.6.2. Symbolic Expression for Extreme Value of Difference between True Anomaly and Elliptic Anomaly
2.6.3. Symbolic Expression for Extreme Value of Difference between True and Elliptic Anomalies
- The absolute values of the extreme values of the difference among the three anomalies increase with the increase in eccentricity.
- The absolute values of the extreme values of the difference between the elliptic anomaly and the mean anomaly, the difference between the true anomaly and the elliptic anomaly, and the difference between the true anomaly and the mean anomaly gradually increase.
- When the eccentricity is small, the extreme absolute value of the difference E − M between the elliptic anomaly and the mean anomaly, as well as the extreme value of the difference f − E between the true anomaly and the elliptic anomaly, is approximately equal.
- The absolute value of the extreme value of the difference f − M between the true anomaly and the mean anomaly is approximately twice as large as the absolute value of the extreme value of the difference E − M between the elliptic anomaly and the mean anomaly, and the absolute value of the extreme value of the difference f − E between the true anomaly and the elliptic anomaly.
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhao, Q.; Guo, J.; Wang, C.; Lyu, Y.; Xu, X.; Yang, C.; Li, J. Precise orbit determination for BDS satellites. Satell. Navig. 2022, 3, 1–24. [Google Scholar] [CrossRef]
- Chen, G.; Zhou, R.; Hu, Z.; Lv, Y.; Wei, N.; Zhao, Q. Statistical characterization of the signal-in-space errors of the BDS: A comparison between BDS-2 and BDS-3. GPS Solut. 2021, 25, 112. [Google Scholar] [CrossRef]
- Cui, H.; Wang, J.; Wang, M.; Wang, H.; Zhu, W.; Hou, Y. Service Performance Assessment of BDS⁃3 Using Satellite Distribution Probability. Geomat. Inf. Sci. Wuhan Univ. 2021, 45, 938–946. [Google Scholar]
- Shao, B.; Xin, J.; Lu, H.; Mao, Y.; Yuan, H.; Liu, C.; Hu, X.; Lou, Y. BDS-3 performance assessment: PNT, SBAS, PPP, SMC, and SAR. Acta Geod. Cartogr. Sin. 2021, 50, 181–193. [Google Scholar]
- Li, J. Solar Radiation Pressure Modeling for Beidou-3 Satellites. Ph.D. Thesis, Wuhan University, Wuhan, China, 2022. [Google Scholar]
- Yang, Y.; Mao, Y.; Sun, B. Basic performance and future developments of BeiDou global navigation satellite system. Satell. Navig. 2020, 1, 1. [Google Scholar] [CrossRef]
- Han, C.; Liu, L.; Cai, Z.; Lin, Y. The space–time references of BeiDou navigation satellite system. Satell. Navig. 2021, 2, 18. [Google Scholar] [CrossRef]
- Li, P.; Zhang, H. Research on BDS satellites precision orbit determination based on global MGEX observation stations. J. Navig. Position 2021, 9, 25–32. [Google Scholar]
- Gao, D.; Li, H.; Bian, S. The Series Expansions of Transformations between Eccentric, Mean and True Anomalies. J. Navig. Position 2015, 3, 57–61. [Google Scholar]
- Markley, F.L. Kepler Equation solver. Celest. Mech. Dyn. Astron. 1995, 63, 101–111. [Google Scholar] [CrossRef]
- Abubekerov, M.K.; Gostev, N.Y. Solution of Kepler’s Equation with Machine Precision. Astron. Rep. 2020, 64, 1060–1066. [Google Scholar] [CrossRef]
- Zhang, R.; Bian, S.; Li, H. Symbolic iteration method based on computer algebra analysis for Kepler’s equation. Sci. Rep. 2022, 12, 2957. [Google Scholar] [CrossRef] [PubMed]
- Wu, B.; Zhou, Y.; Lim, C.W.; Zhong, H. A new solution approach via analytical approximation of the elliptic Kepler equation. Acta Astronaut. 2023, 202, 303–310. [Google Scholar] [CrossRef]
- Stumpff, K. On the Application of Lie—Series to the Problems of Celestial Mechanics; National Aeronautics and Space Administration: Washington, DC, USA, 1968.
- Gooding, R.H.; Odell, A.W. The hyperbolic Kepler equation (and the elliptic equation revisited). Celest. Mech. 1988, 44, 267–282. [Google Scholar] [CrossRef]
- Calvo, M.; Elipe, A.; Montijano, J.I. A monotonic starter for solving the hyperbolic Kepler equation by Newton’s method. Celest. Mech. Dyn. Astron. 2019, 131, 18. [Google Scholar] [CrossRef]
- Zechmeister, M. Solving Kepler’s equation with CORDIC double iterations. Mon. Not. R. Astron. Soc. 2021, 500, 109–117. [Google Scholar] [CrossRef]
- González-Gaxiola, O.; Hernández-Linares, S. An Efficient Iterative Method for Solving the Elliptical Kepler’s Equation. Int. J. Appl. Comput. Math. 2021, 7, 42. [Google Scholar] [CrossRef]
- Philcox, O.H.E.; Jeremy, G.; Zachary, S. Kepler’s Goat Herd: An exact solution to Kepler’s equation for elliptical orbits. Mon. Not. R. Astron. Soc. 2021, 506, 6111–6116. [Google Scholar] [CrossRef]
- Tommasini, D.; Olivieri, D.N. Two fast and accurate routines for solving the elliptic Kepler equation for all values of the eccentricity and mean anomaly. Astron. Astrophys. 2022, 658, A196. [Google Scholar] [CrossRef]
- Bian, S.; Li, H. Computer Algebra Analysis on Geodesy, 1st ed.; China Science Publishing & Media Ltd.: Beijing, China, 2018. [Google Scholar]
- Li, Q.; Cui, C. Principles of Satellite Geodesy, 1st ed.; Surveying and Mapping Publishing: Beijing, China, 1989. [Google Scholar]
- Zhao, Y. Fundamentals of Astromechanics, 1st ed.; Nanjing University Press: Nanjing, China, 1993. [Google Scholar]
- Gu, X. A Calculating Method for Epicyclic Motion of Spacecraft. J. UEST China 2003, 32, 635–637. [Google Scholar]
- Gu, X.; Tan, Z. Calculating Method of Series for the True Anomaly of a Spacecraft Elliptic Orbit. Appl. Math. Mech. 2005, 26, 45–50. [Google Scholar] [CrossRef]
- Liu, L.; Hou, Y. Fundamentals of Orbital Mechanics, 1st ed.; Higher Education Press: Beijing, China, 2018. [Google Scholar]
- Liu, L. Orbital Mechanics of Artificial Earth Satellites, 1st ed.; Higher Education Press: Beijing, China, 1992. [Google Scholar]
- Clemence, G.M. Methods of Celestial Mechanics, 1st ed.; Academic Press: New York, NY, USA, 1961. [Google Scholar]
- Farnocchia, D.; Cioci, D.B.; Milani, A. Robust resolution of Kepler’s equation in all eccentricity regimes. Celest. Mech. Dyn. Astron. 2013, 116, 21–34. [Google Scholar] [CrossRef]
- Liu, L. Theory and Application of Spacecraft Orbit Determination, 1st ed.; Publishing House of Electronics Industry: Beijing, China, 2015. [Google Scholar]
- Liu, L. Satellite Orbit Theory and Applications, 1st ed.; Publishing House of Electronics Industry: Beijing, China, 2015. [Google Scholar]
- Da, S.F.S. Extension of the solution of Kepler’s equation to high eccentricities. Celest. Mech. Dyn. Astron. 1994, 58, 297–308. [Google Scholar]
- Siewert, C.E.; Burniston, E.E. An exact analytical solution of Kepler’s equation. Celest. Mech. 1972, 6, 294–304. [Google Scholar] [CrossRef]
- Xiong, J. Ellipsoidal Geodesy, 1st ed.; The People’s Liberation Army Press: Beijing, China, 1988. [Google Scholar]
- Li, Z.; Bian, S.; Kong, H. Symbolic Iterative Method for Solving Inverse Problems in Ellipsoidal Geodesy. Hydrogr. Surv. Charting 2013, 33, 27–29. [Google Scholar]
- Murray, C.D.; Dermott, S.F. Solar System Dynamics; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Danby, J.M.A. Fundamentals of Celestial Mechanics; Willmann-Bell: Richmond, VA, USA, 1988. [Google Scholar]
- Gao, D.; Bian, S.; Li, H. Symbolic Expressions of Difference Extrema Between Anomalies for Satellite Movement. Hydrogr. Surv. Charting 2015, 35, 8–11. [Google Scholar]


| Method | e = 0.01 | e = 0.05 | e = 0.1 | e = 0.2 | ||||
|---|---|---|---|---|---|---|---|---|
| N | Time | N | Time | N | Time | N | Time | |
| Kepler’s Goat Herd | 3 | 137.5 | 5 | 269.3 | 5 | 249.2 | 5 | 219.3 |
| This Work | 5 | 114.6 | 8 | 155.6 | 11 | 194.4 | 18 | 299.0 |
| Eccentricities | Parameter | /rad | /rad | /rad | /rad | /rad |
|---|---|---|---|---|---|---|
| e = 0.01 | m | |||||
| e | ||||||
| e = 0.1 | m | |||||
| e | ||||||
| e = 0.2 | m | |||||
| e |
| Function | Argument | Extreme Point | Extreme Value |
|---|---|---|---|
| E − M | E | ||
| M | |||
| f | |||
| f − E | E | ||
| M | |||
| f | |||
| f − M | E | ||
| M | |||
| f |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, D.; Li, H.; Bian, S.; Chen, Y.; Li, W. New Methods of Series Expansions between Three Anomalies. Appl. Sci. 2024, 14, 3873. https://doi.org/10.3390/app14093873
Zhao D, Li H, Bian S, Chen Y, Li W. New Methods of Series Expansions between Three Anomalies. Applied Sciences. 2024; 14(9):3873. https://doi.org/10.3390/app14093873
Chicago/Turabian StyleZhao, Dongfang, Houpu Li, Shaofeng Bian, Yongbing Chen, and Wenkui Li. 2024. "New Methods of Series Expansions between Three Anomalies" Applied Sciences 14, no. 9: 3873. https://doi.org/10.3390/app14093873
APA StyleZhao, D., Li, H., Bian, S., Chen, Y., & Li, W. (2024). New Methods of Series Expansions between Three Anomalies. Applied Sciences, 14(9), 3873. https://doi.org/10.3390/app14093873






