A Story-Based Random Structure Modeling Method for Regional Buildings Earthquake Loss Estimation
Abstract
1. Introduction
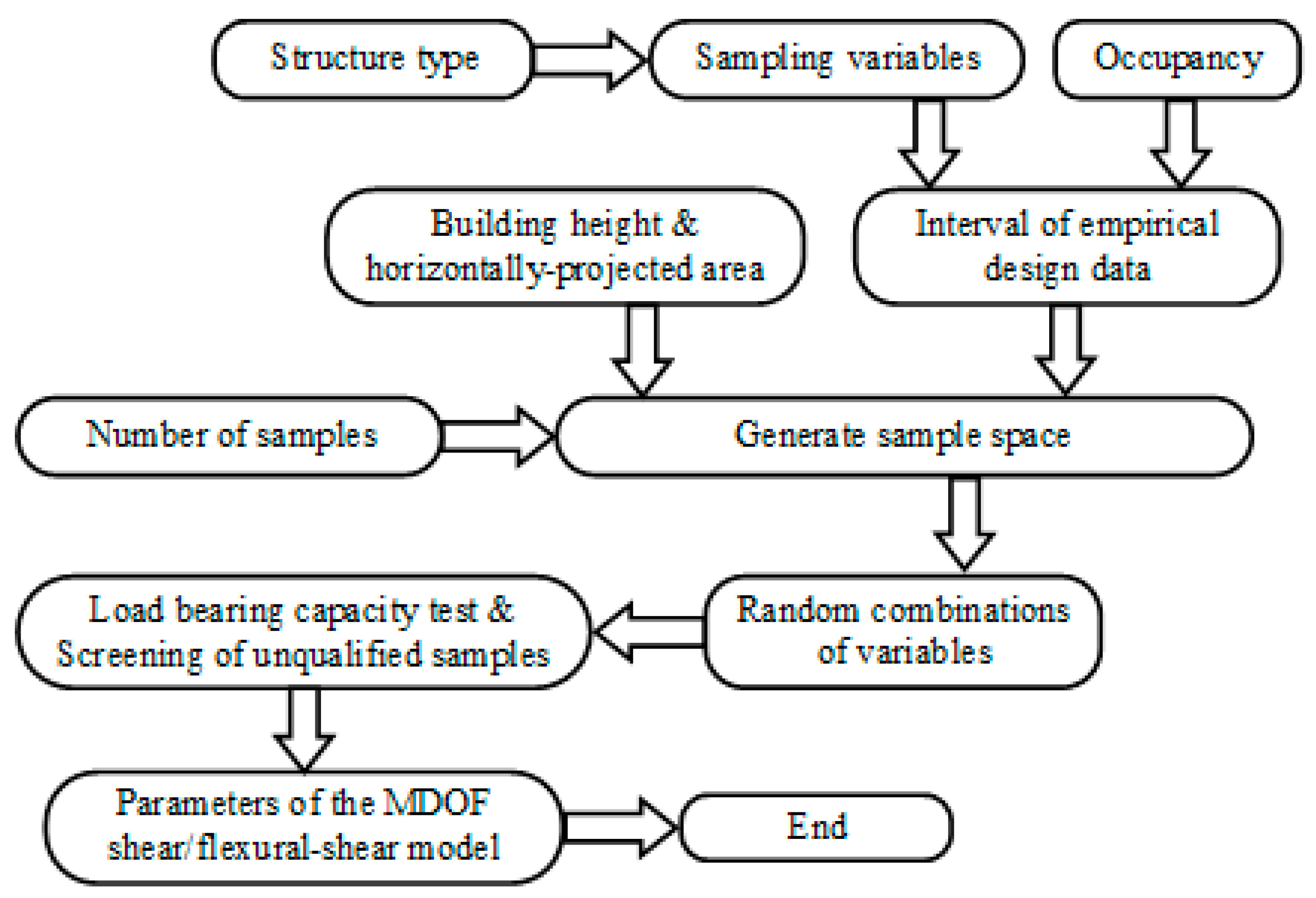
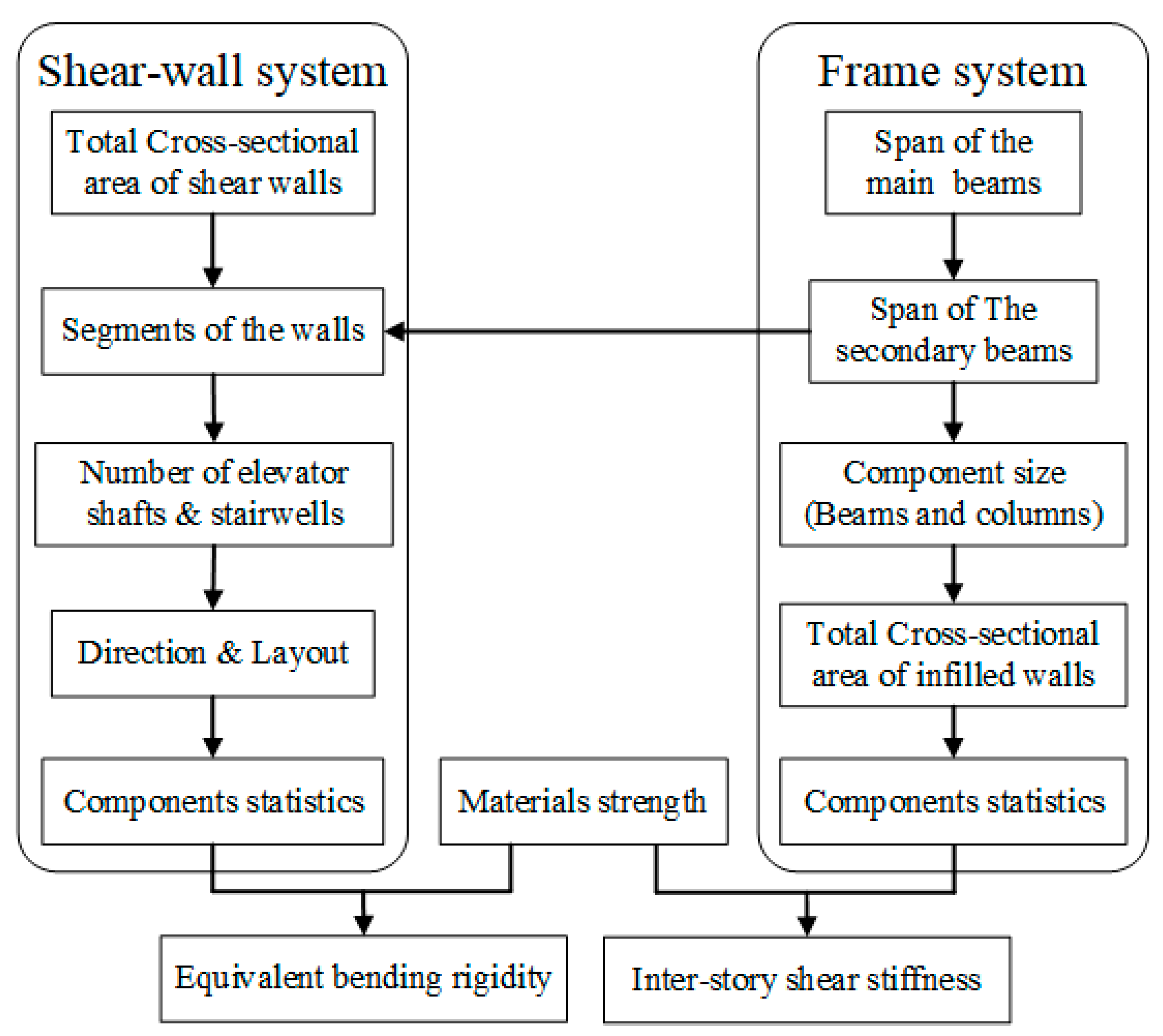
2. Basic Framework of the Methodology
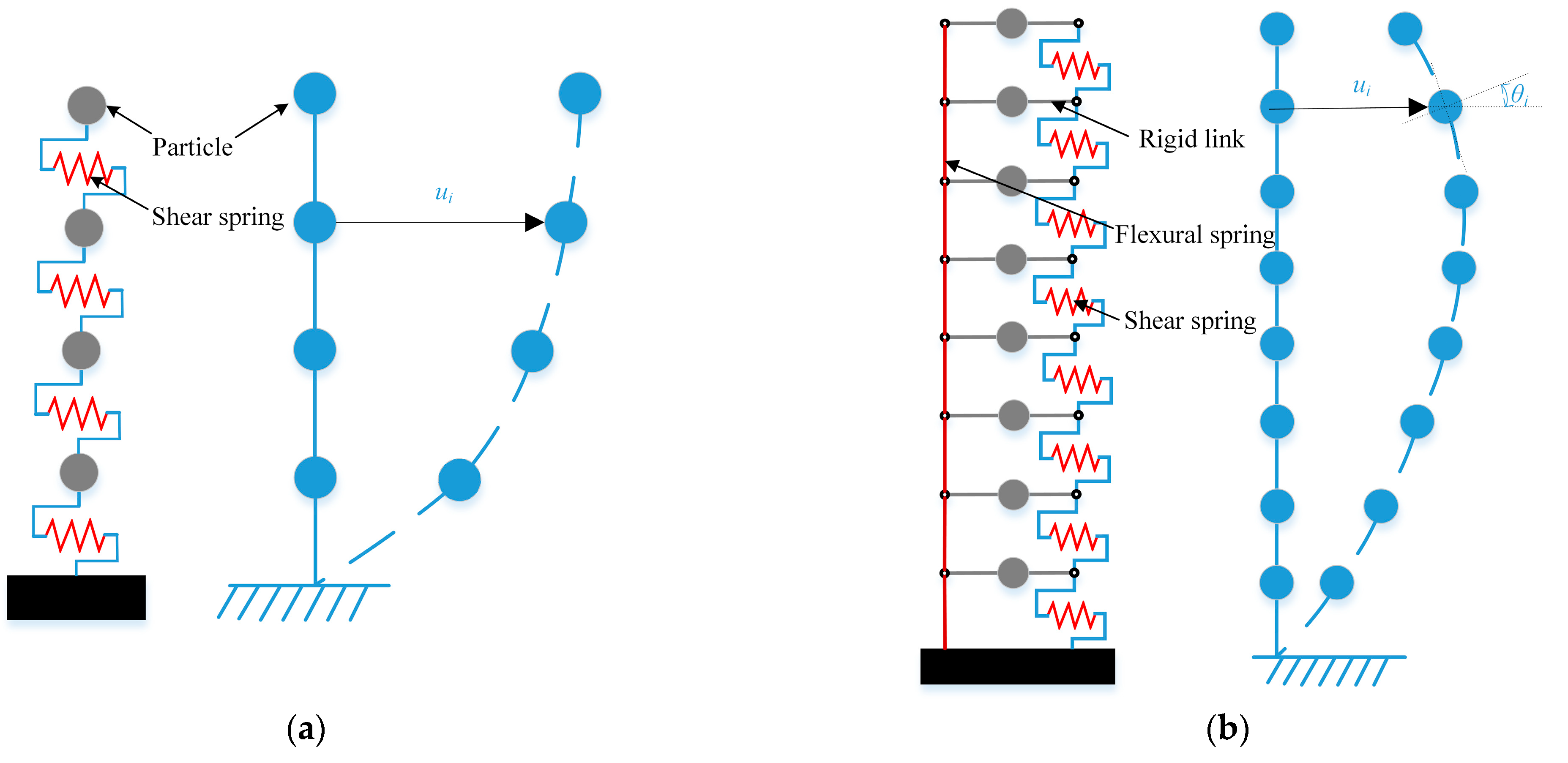
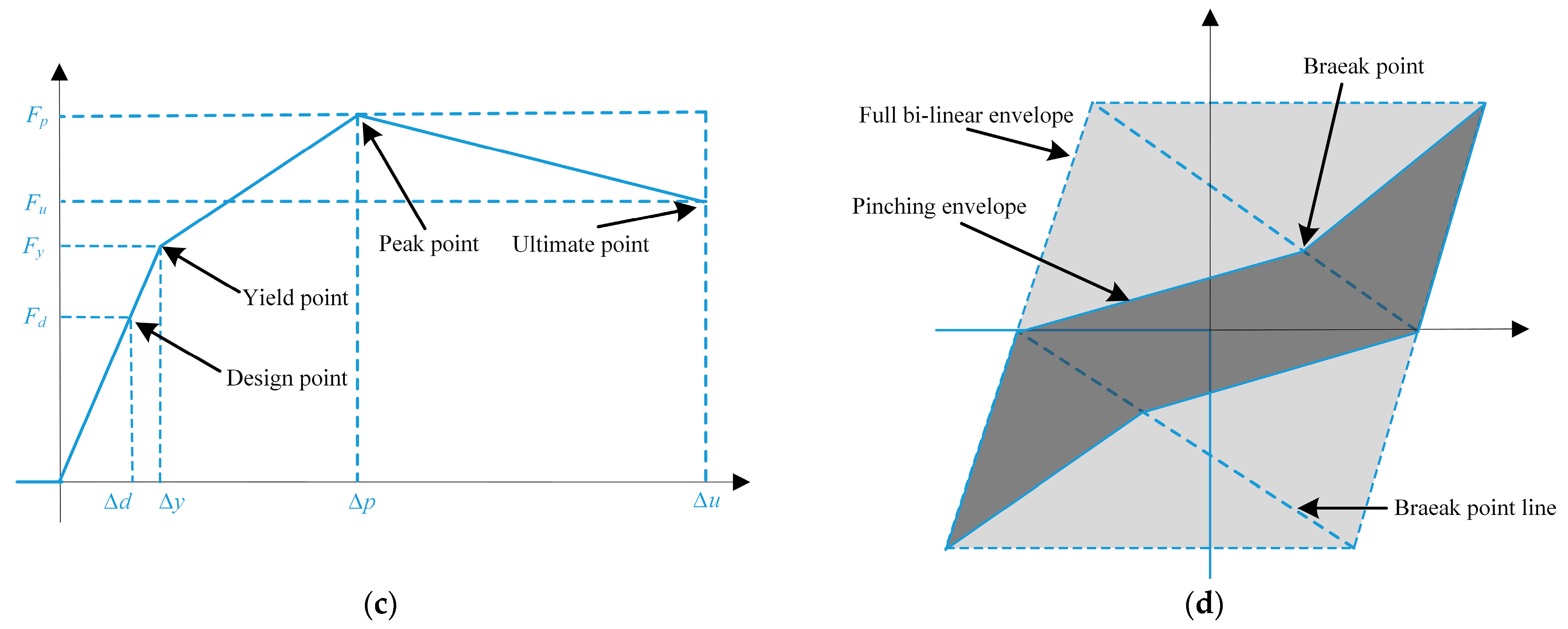
2.1. The Definition of the SBRS Model
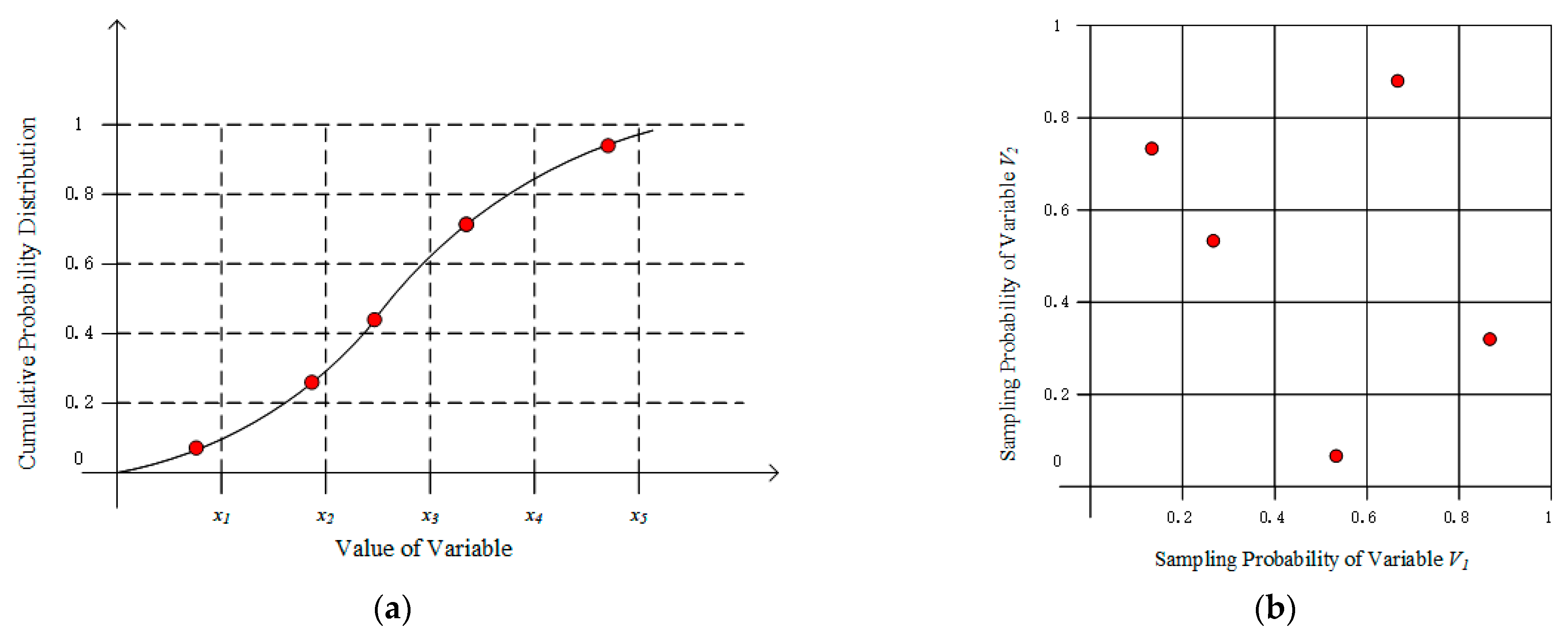
2.2. Random Sampling Method
2.3. Methods for Building the Sample Space
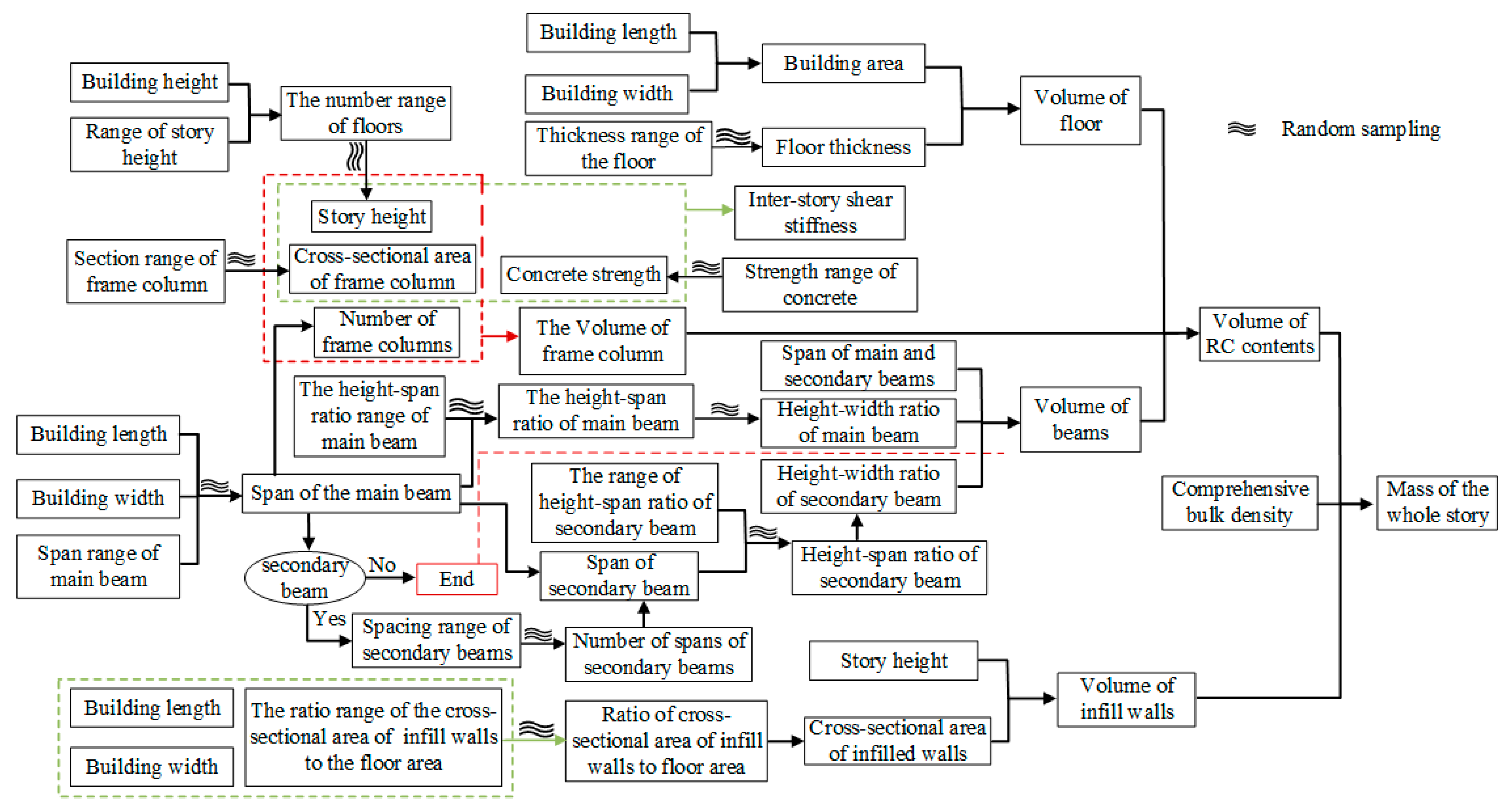
3. SBRS Model Sampling Process
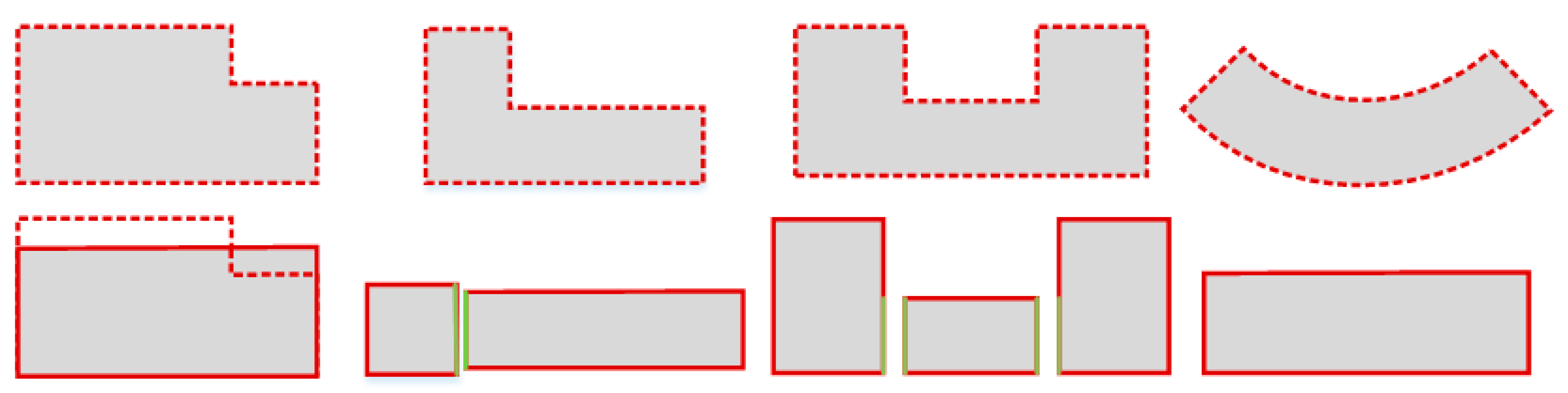
3.1. Treatment of Building Plan
- (1)
- For buildings where the concave dimension of the plane does not exceed 30% of the total size of the corresponding projection direction, the plane is equivalently reconstructed as a regular rectangle equal to its area [33].
- (2)
- When the plane is concave by more than 30%, the layout form of the internal structure and the number of components are estimated, respectively, and summarized after removing the overlapped parts.
- (3)
- For curved buildings, they are equivalently reconfigured as equal-area rectangles of the same width.
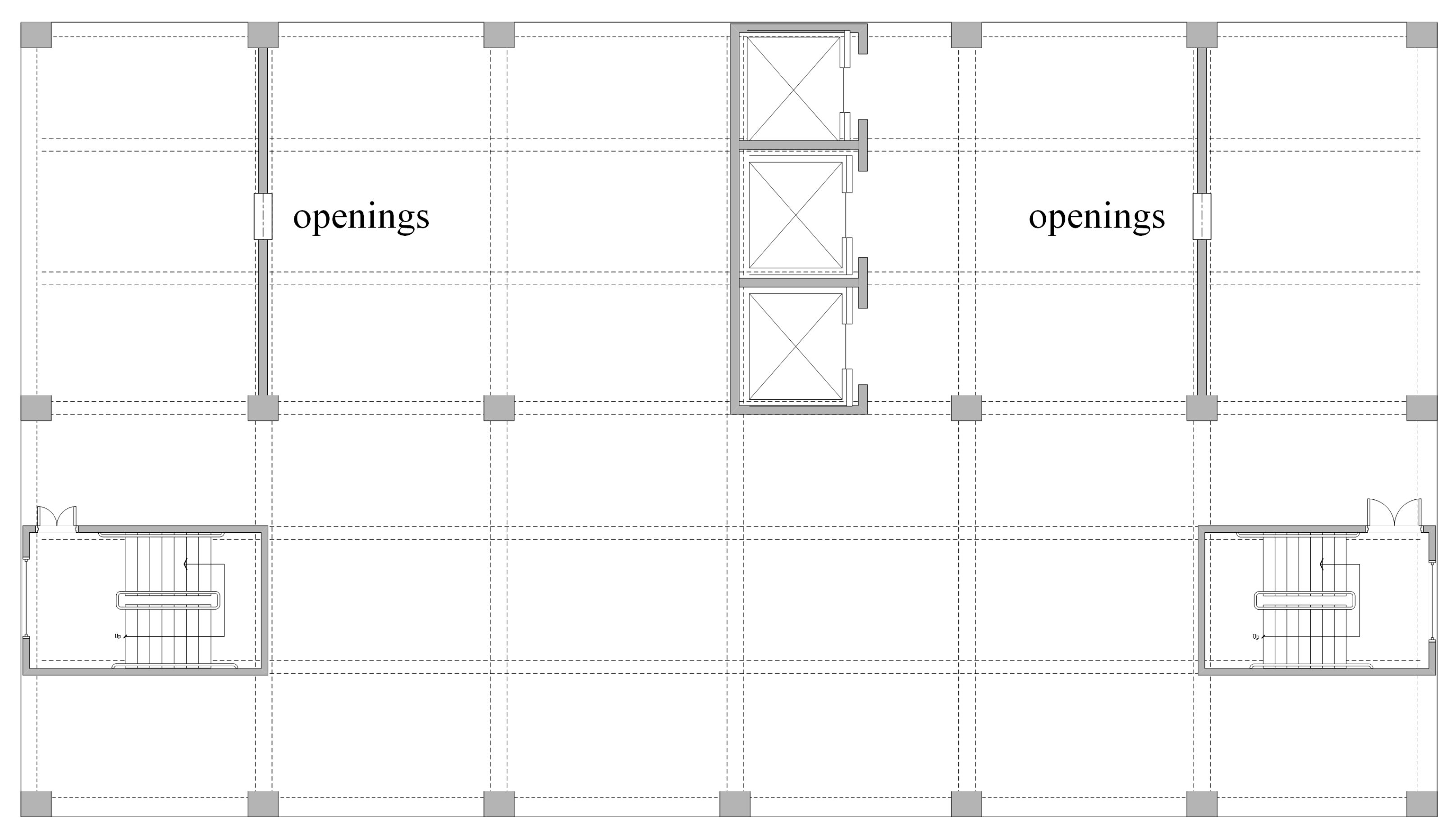
3.2. Common Layout for Structures
- (1)
- Shear walls should be arranged bi-directionally along the main axis of the building, and both should have a similar total lateral stiffness.
- (2)
- Shear walls should be evenly distributed in the floor plane, and the spacing should be manageable to ensure that the building cover has sufficient stiffness in its plane.
- (3)
- For a regular rectangular floor plan, the shear wall should be suitable to maintain basic symmetry along the longitudinal and transverse bi-directional plane centerline.
- (4)
- Shear walls should be set through the entire building height to avoid sudden changes in stiffness to form a weak structure story.
- (5)
- The cross-section of the shear wall should be simple and regular to avoid the appearance of a complex and shaped cross-section.
- (6)
- The ratio of the total height of the shear wall to its section height should be at least 3, and the section height of the wall limb should generally not be more than 8 m.
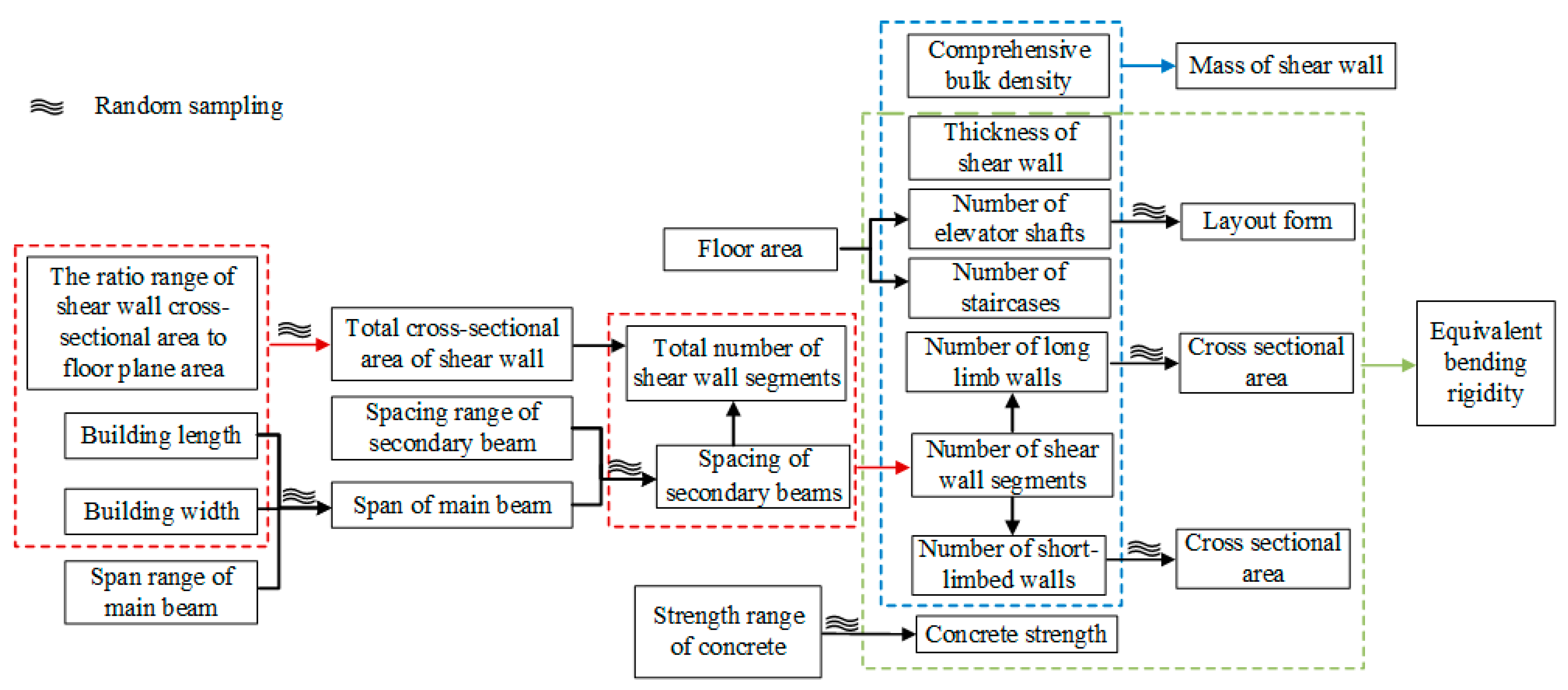
3.3. Generate the SBRS Model
- (1)
- The spacing of secondary beams is the basic unit of measurement of shear wall section height, and the number of wall segments is obtained based on the total cross-sectional area of the shear wall. Usually, the number of wall segments obtained from the calculation is partial. After rounding up, the total shear wall section height in the direction of the short side of the building is slightly larger than that in the direction of the long side.
- (2)
- After deducting the total number of shear wall sections in the direction of the short side of the building from the elevator shafts and stairwells, this is divided by the spacing of the framing columns on the short side of the building to obtain the number of long sections of shear walls; if it is not possible to divide the whole number, the spacing of the secondary beams is used as the height of the section to obtain the number of remaining wall sections.
- (3)
- The direction of the short side of the building is the seismically unfavorable direction, and the cross-sectional area and equivalent bending stiffness of the shear wall in that direction are calculated.
- (4)
- Except for connecting beams in elevator shafts, stairwells, and long sections of shear wall openings, the number of connecting beams between shear walls is determined by random sampling. If the number of shear walls is N, the upper limit of the number of connecting beams is N − 1.
4. Case Study
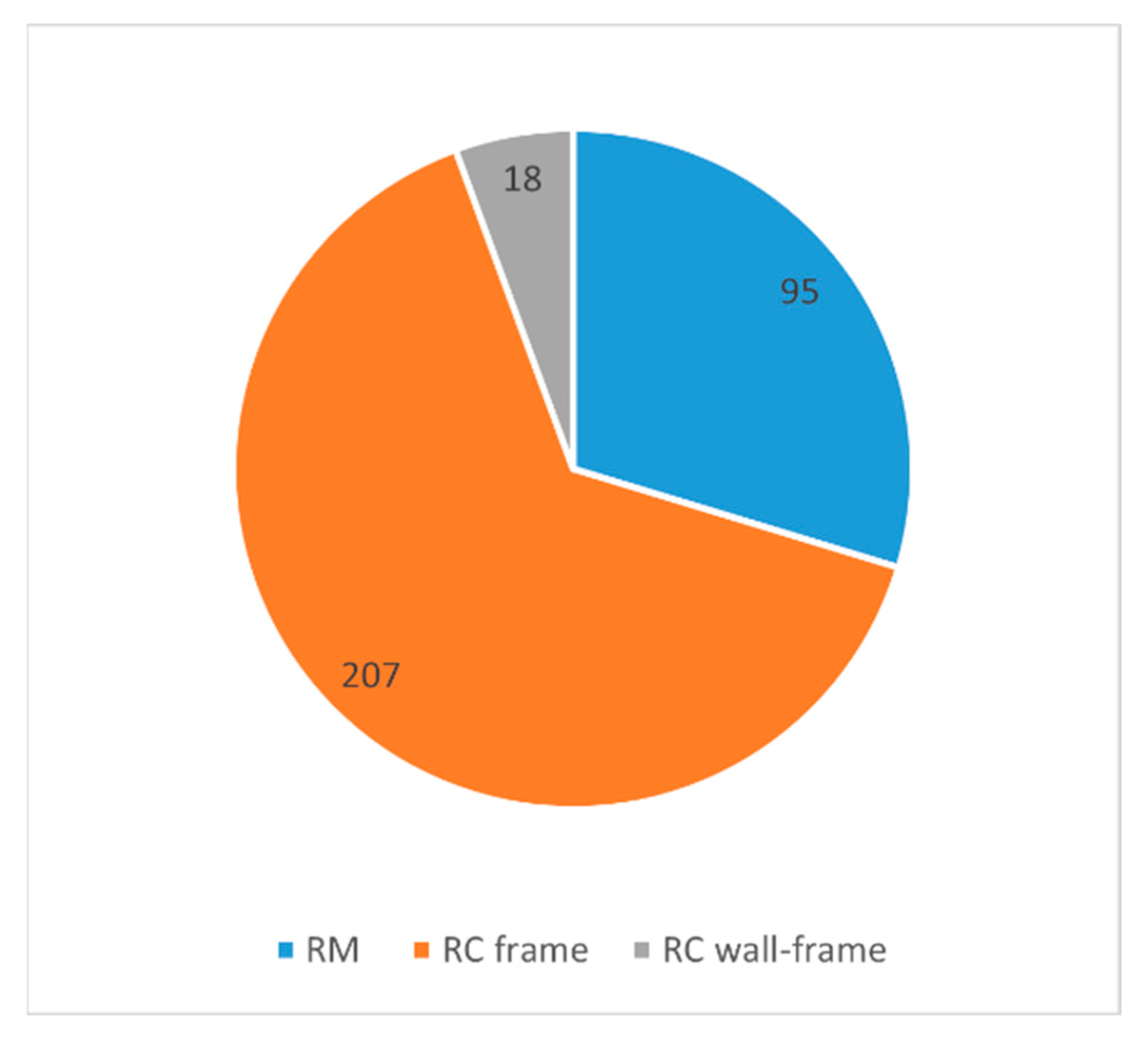
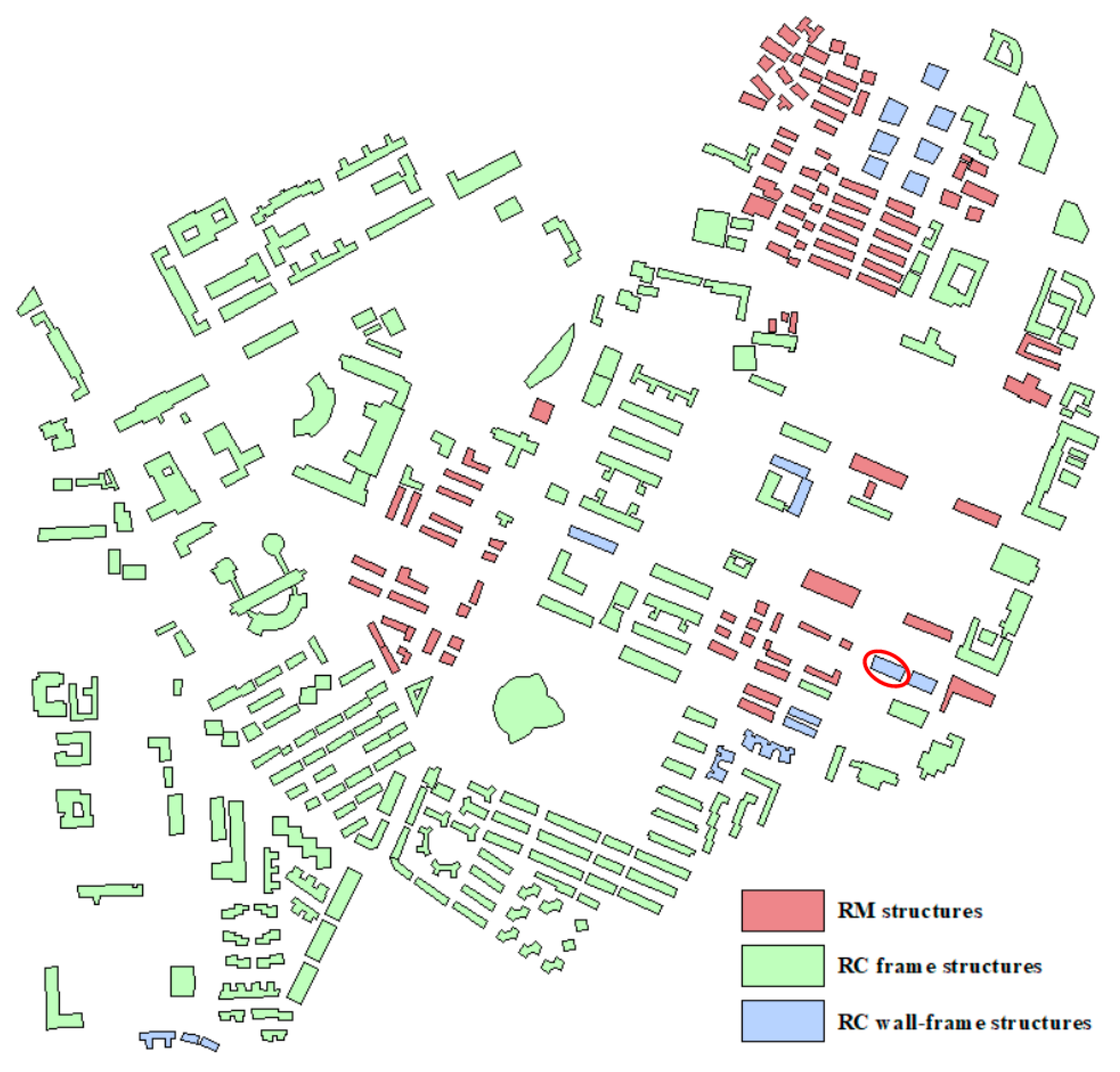
4.1. Basic Information on Regional Buildings
4.2. SBRS Models Generation
- (1)
- Story height. The story heights of office buildings are generally in the range from 3.0 to 3.6 m.
- (2)
- Bidirectional main and secondary beam spans. The main beam’s span range in the building’s short side direction is usually from 6 to 8 m, the long side direction is from 4 to 6 m, and the secondary beams are laid out at spacings from 2 to 3 m.
- (3)
- The cross-sectional area of the frame column. It can be assumed that the square columns have side lengths ranging from 600 to 800 mm, with 50 mm as the basic unit of increase or decrease.
- (4)
- Material strength. The concrete strength grade is C30~C40, and the thickness of the infill wall is uniformly taken at 200 mm. The ratio of the cross-section to the floor plane is taken as 5~8% according to engineering experience. According to GB 11968-2020, the Standard for Autoclaved Aerated Concrete Blocks [35], the commonly used grade markings for autoclaved aerated concrete blocks are B05 and B06. The corresponding dry weights are 500 kg/m3 and 600 kg/m3, considering mortar, plaster, and other factors, and the integrated volume weight is 900 kg/m3.
- (5)
- Shear wall section size. The shear wall thickness is 200 mm, and the ratio of the shear wall cross-sectional area to the floor area is from 2% to 3%; for office buildings, in addition to stairwells and elevator shafts outside, the other shear wall section is simplified to take the “I” type column–wall combination section.
- (6)
- Number of stairwells and elevator shafts. According to the building area, two sets of evacuation stairs should be set up to meet the requirement that the straight distance to the evacuation door between the two safety exits is not less than 40 m in the Code for Fire Protection Design of Buildings [36]. According to the floor area projection of three elevators, the short side of the building, in the direction of the main beam span range, meets the unilateral layout of the space requirements.
- (7)
- Other parameters. According to the provisions of Article 3.1.1 of GB 50009-2012, the Load code for the design of building structures [37], the loads of the building structure are divided into three categories: permanent load, variable load, and accidental load, and only the first two categories are considered in this paper. Among them, the constant loads such as fixed equipment and long-term storage outside the building body are considered as 1.1 times the load of the building body, and for variable loads, standard, combined, frequent, or quasi-permanent values should be used as representative values according to the design requirements. The standard value of floor distributed live load is 2.0 kN/m2, the combination value coefficient is 0.7, the frequency value coefficient is 0.5, the coefficient of live load reduction according to the stories is 0.65, and the structural damping ratio is 0.05.
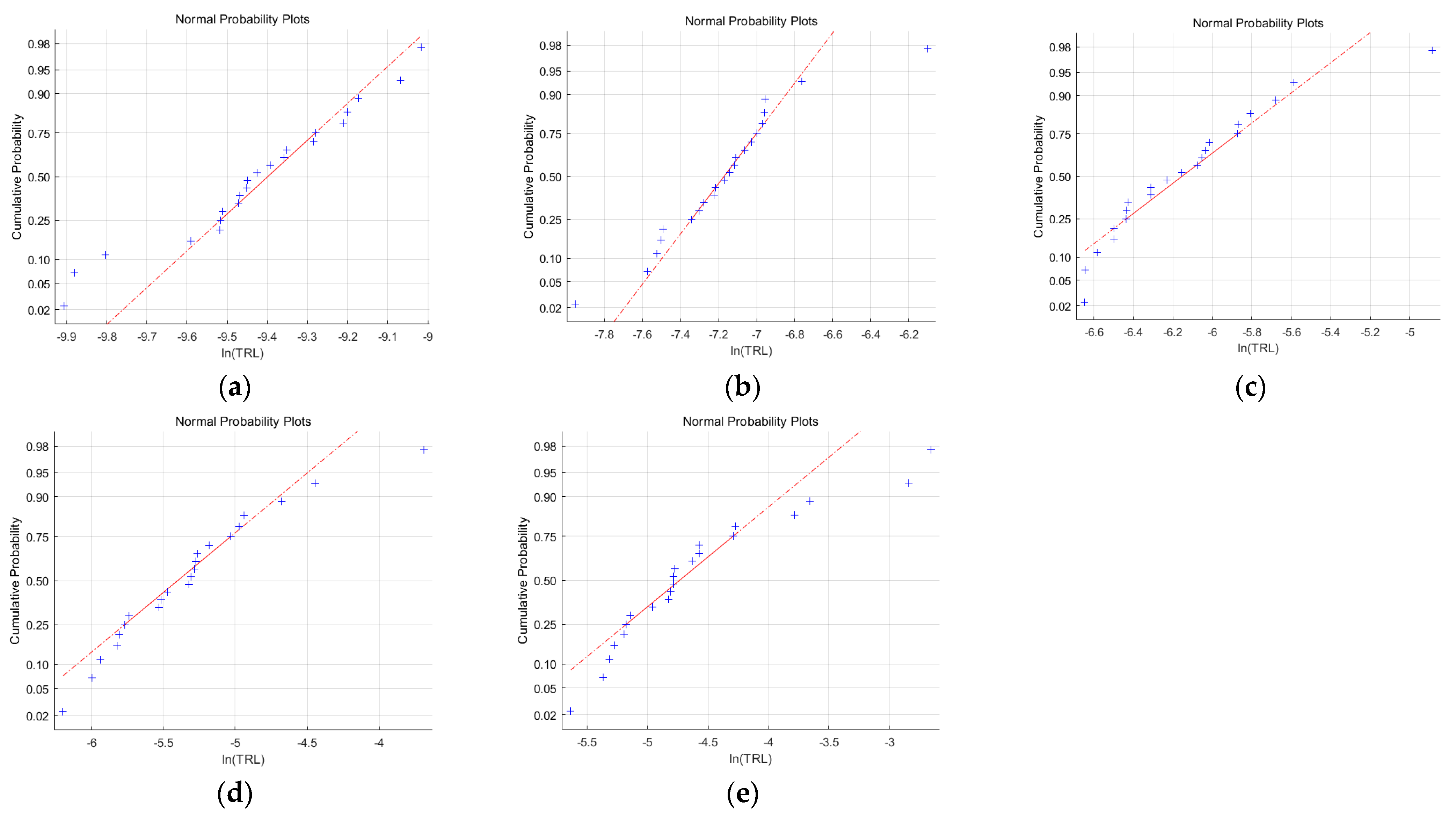
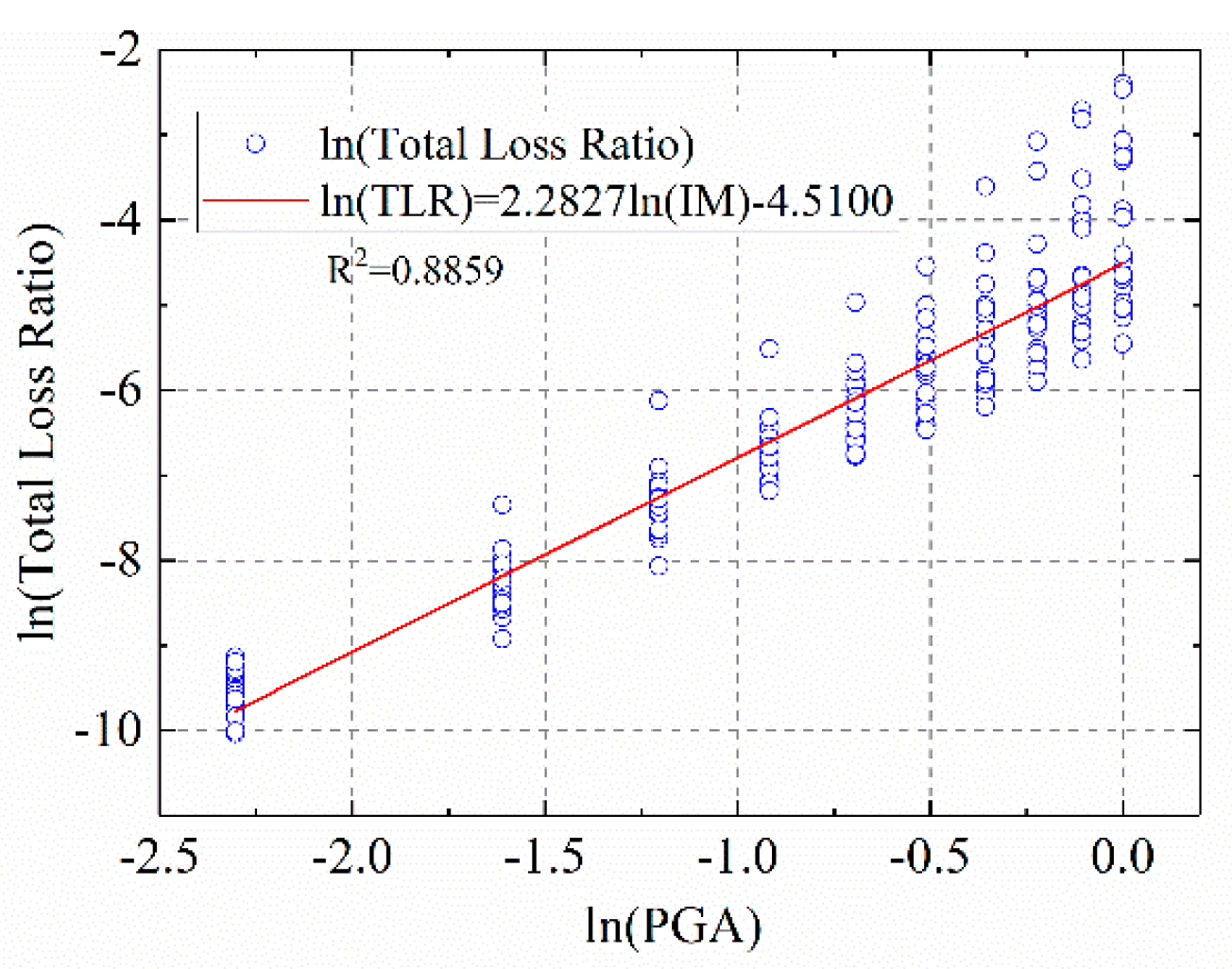
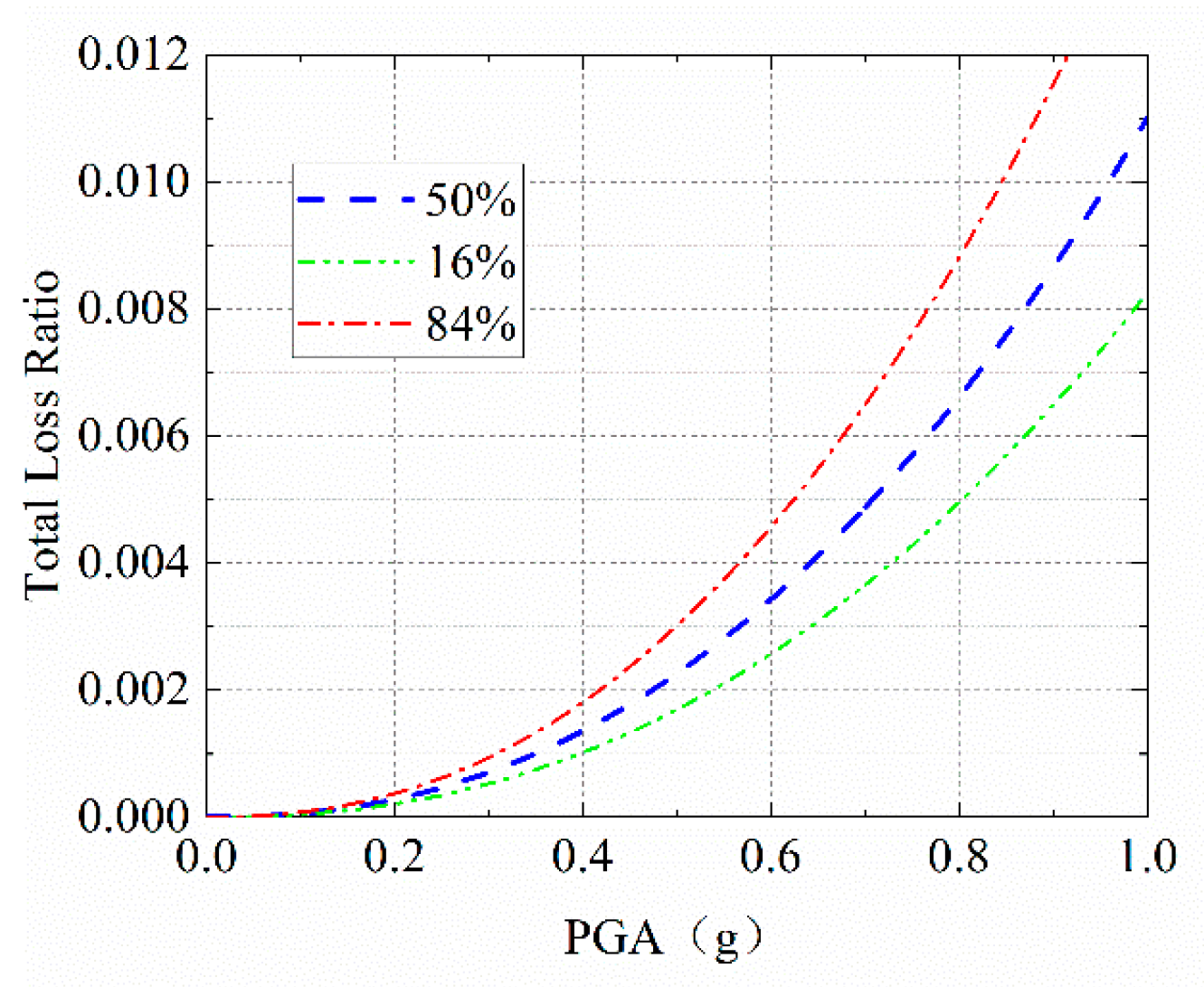
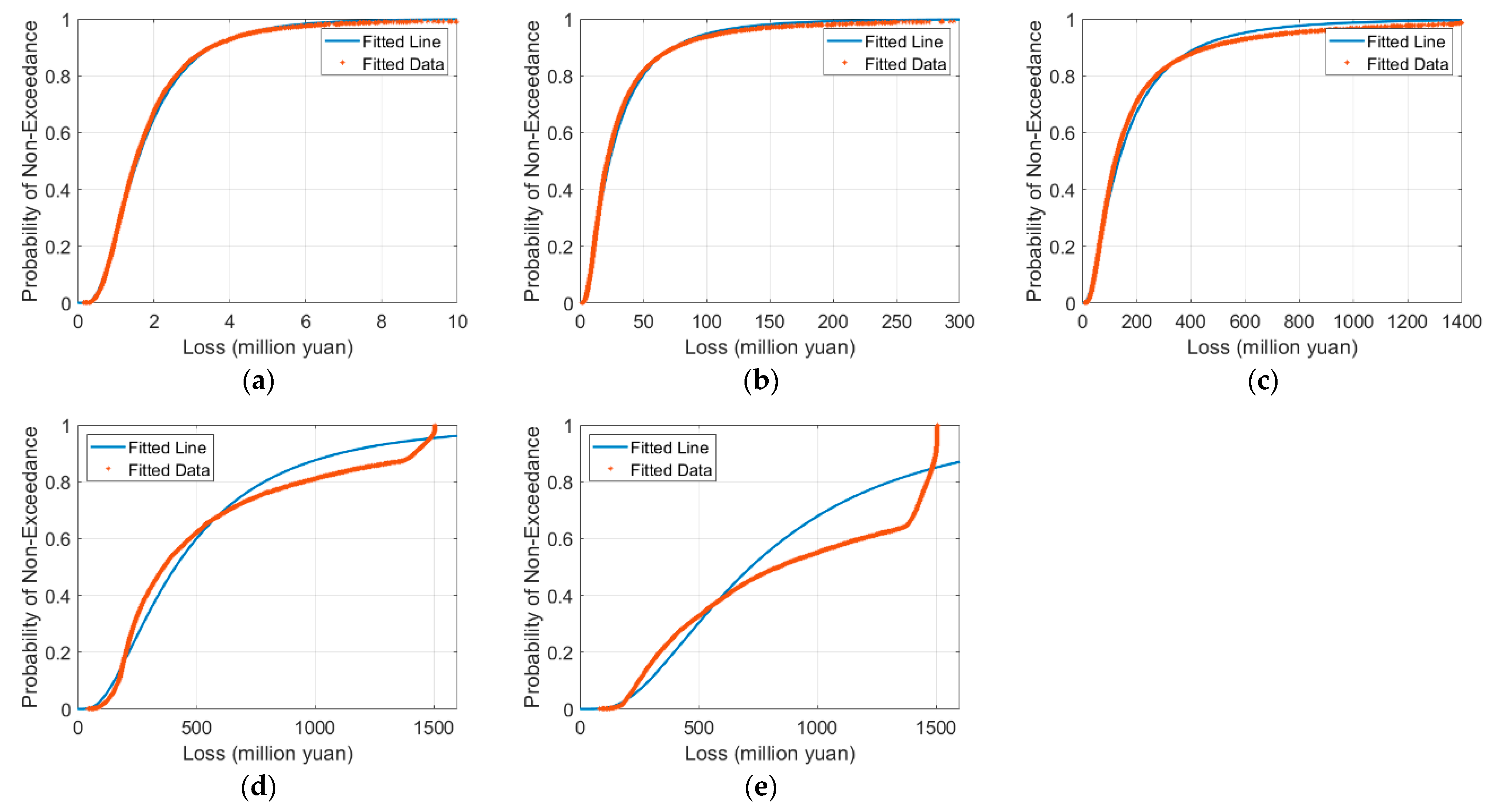
4.3. Earthquake Loss of Single Buildings
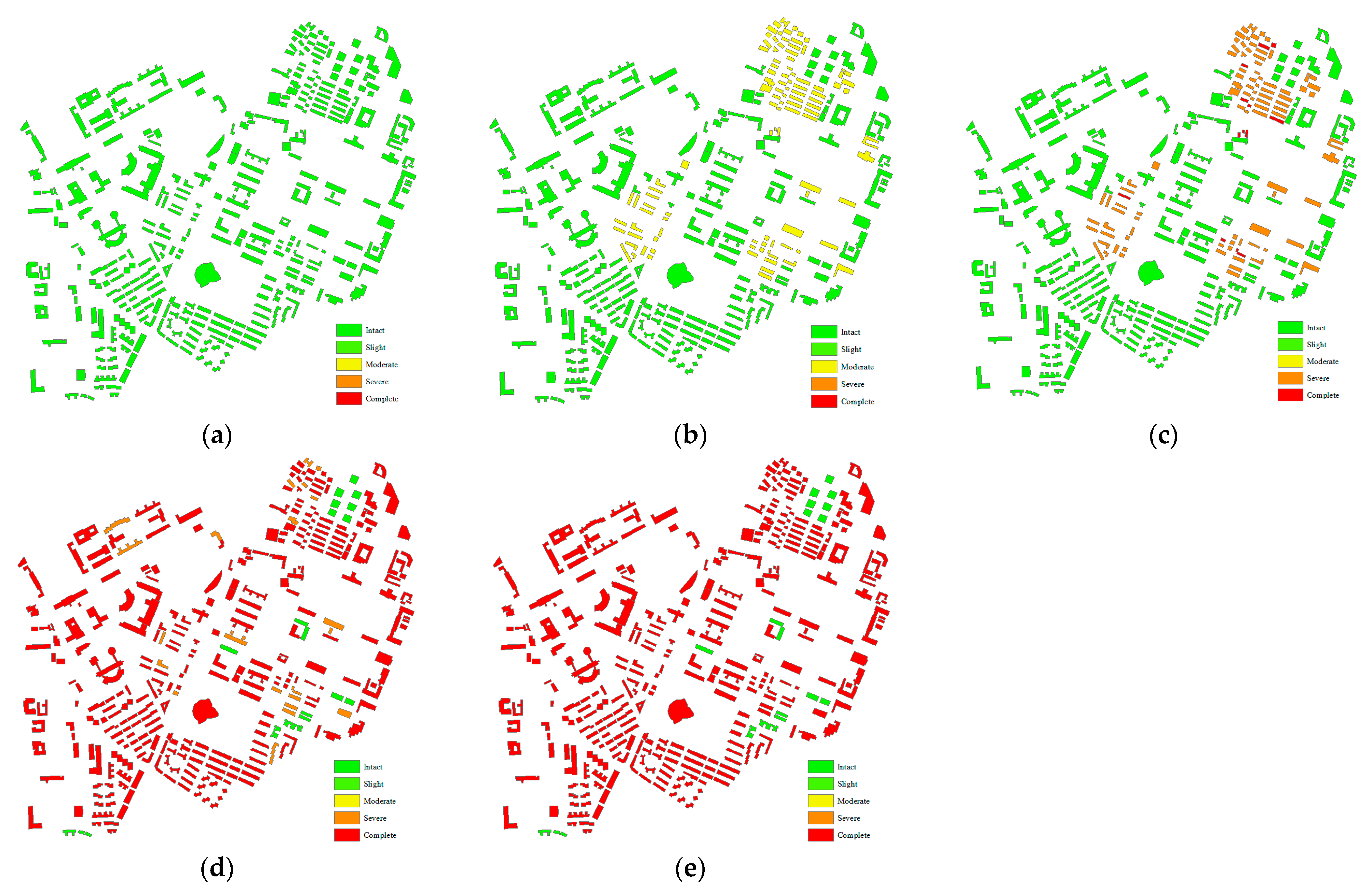
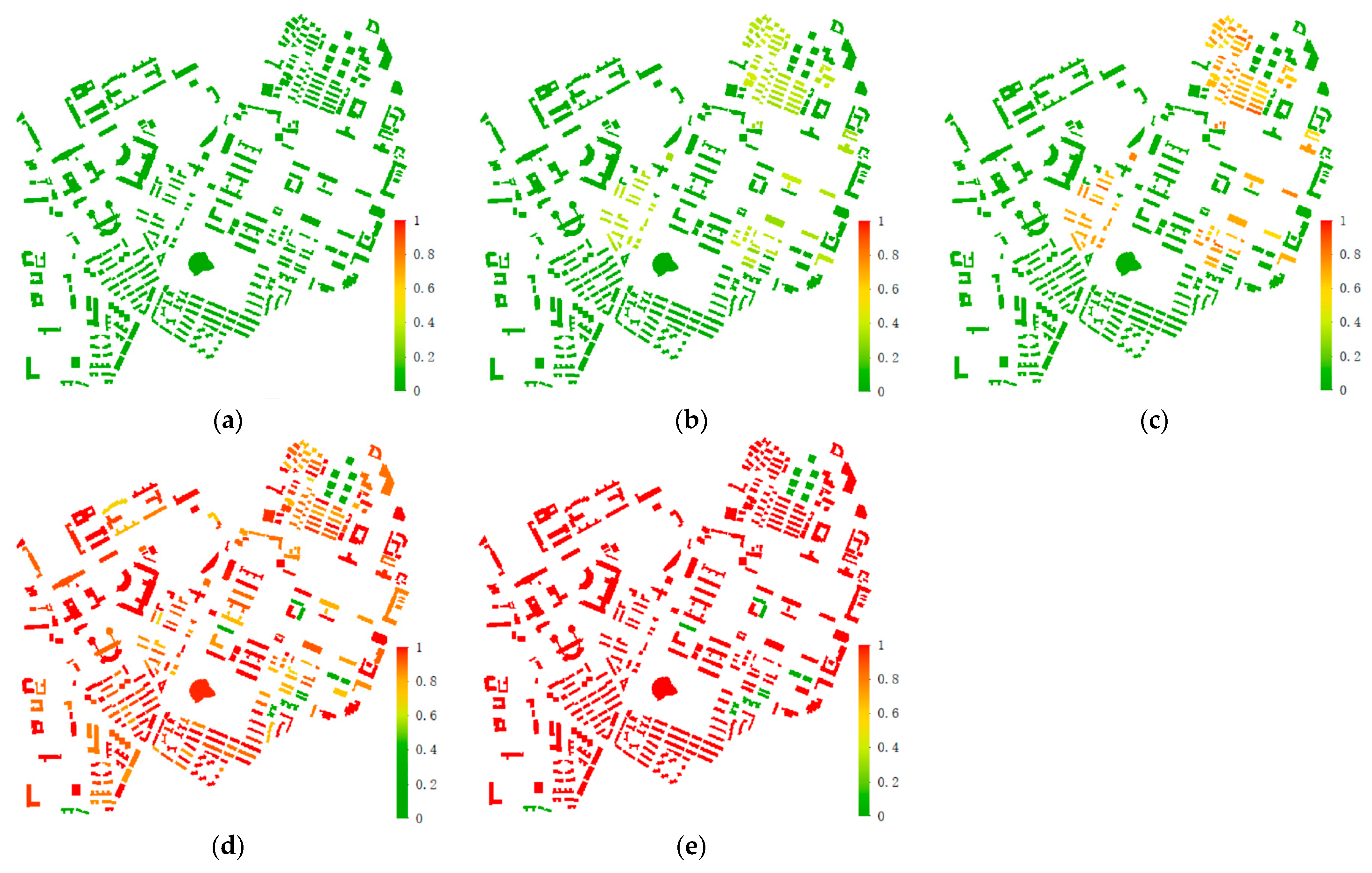
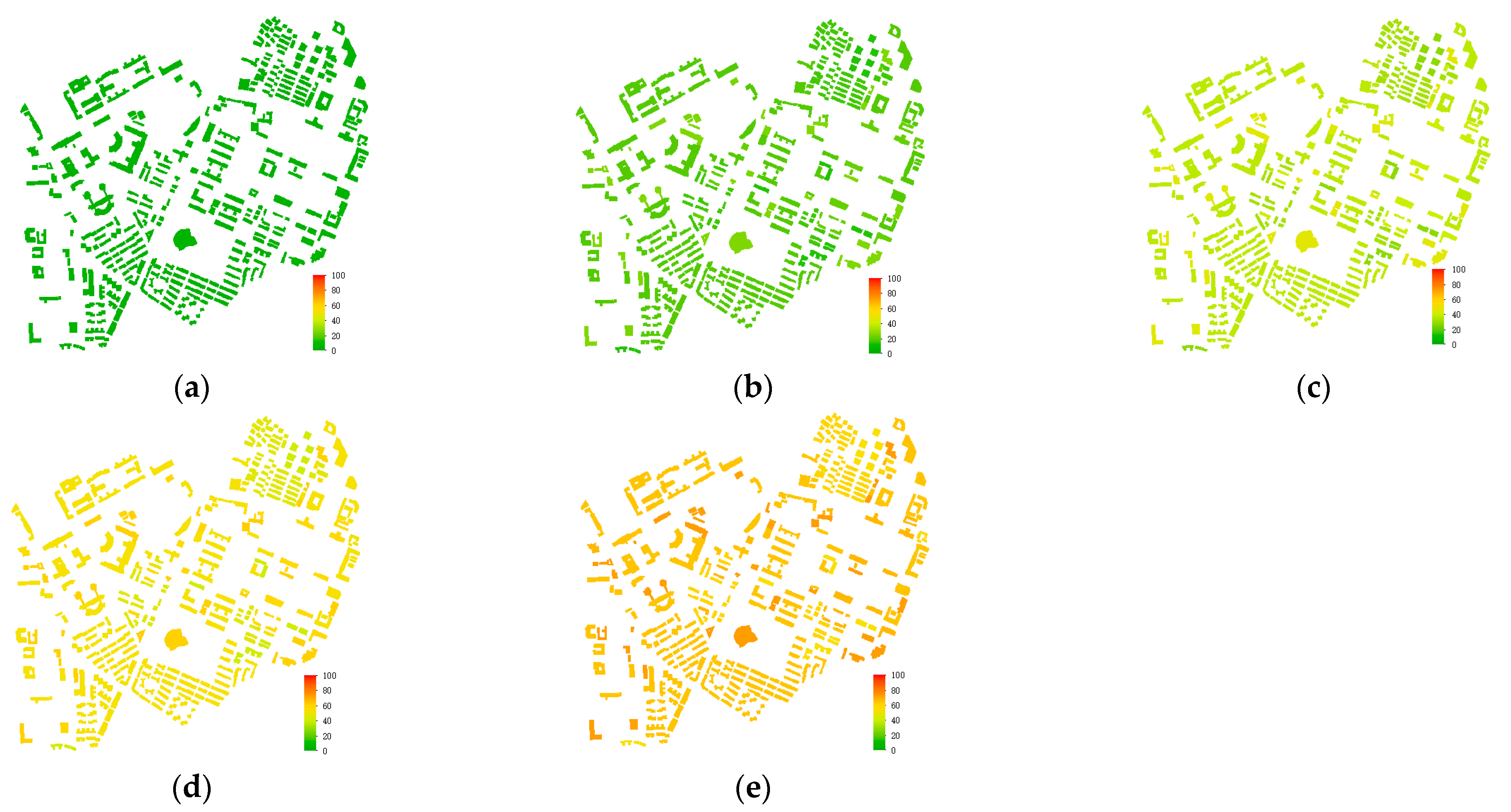
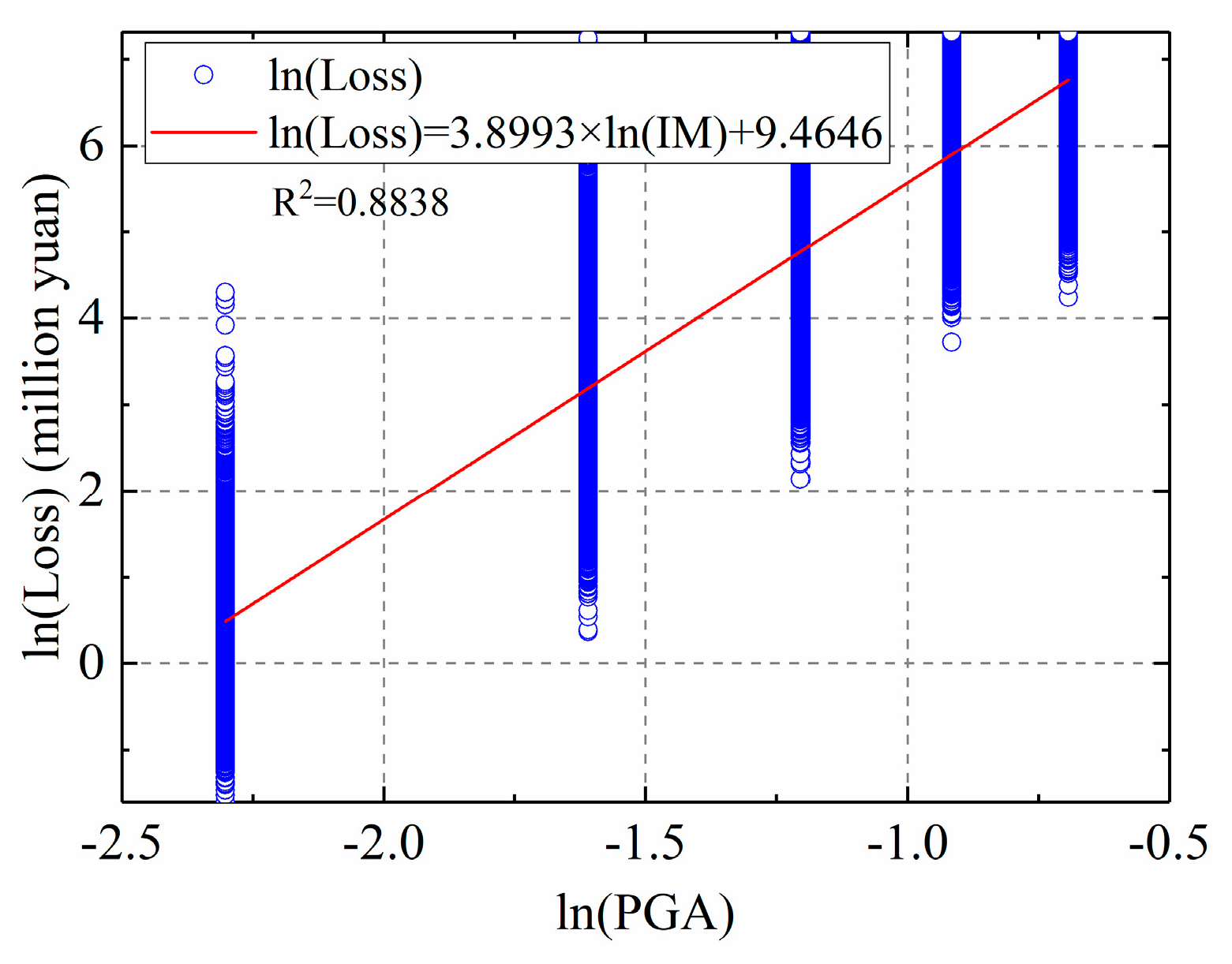
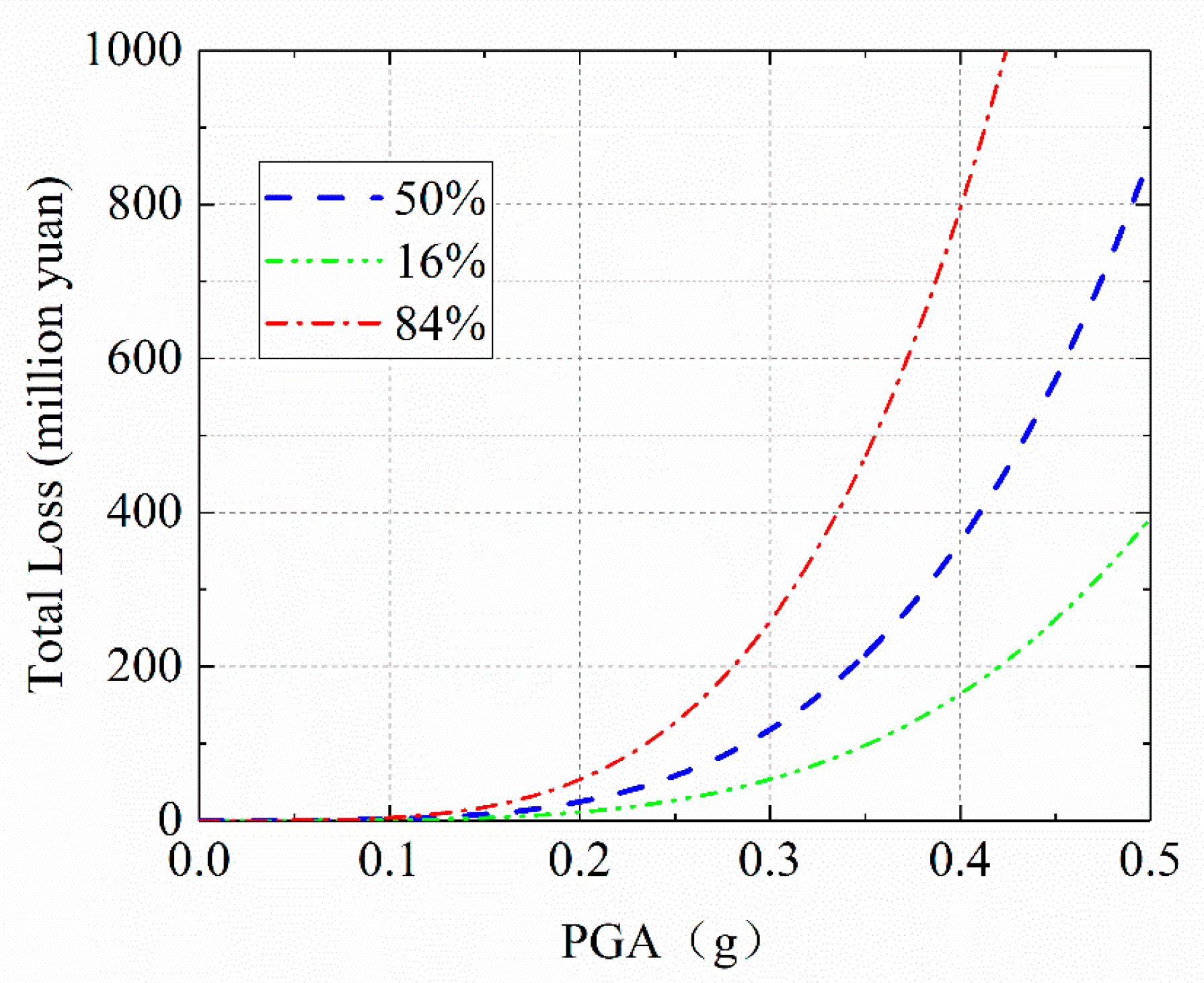
4.4. Earthquake Loss of Regional Buildings
5. Conclusions
- (1)
- It can establish a structural analysis model with probabilistic accuracy when it is difficult to obtain the specific structural design information of the building, which solves the problem of the rapid and refined modeling of a large number of buildings based on the component level at the urban area scale.
- (2)
- Simulating the structural arrangement and component information of different types of buildings based on the probabilistic approach and combining this with an MDOF centralized mass model makes it possible to use a regional building earthquake loss estimation method based on the seismic capacity of the structure and a dynamic time history analysis, which considers estimation accuracy and computational efficiency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yu, S.Z.; Mu, A.D. Preliminary discussion on the earthquake loss assessment method. Earthq. Eng. Eng. Vib. 2017, 37, 144–151. (In Chinese) [Google Scholar] [CrossRef]
- Zheng, S.S.; Sun, L.F.; Long, L. Urban Earthquake Disaster Loss Assessment: Theoretical Methods, System Development and Application; Science Press: Beijing, China, 2019; pp. 9–13. [Google Scholar]
- Zhou, G.Q.; Mao, Y.; Shi, W.H. Relation between the Earthquake—Affected Population and the Earthquake Losses in Yunnan Area. J. Seismol. Res. 2004, 1, 88–93. (In Chinese) [Google Scholar]
- Chen, Y.; Lin, J.Q.; Liu, J.L.; Cai, Y.J.; Huang, X.K.; Lin, Q.L. Study on rapid assessment method of earthquake direct economic loss. World Earthq. Eng. 2017, 33, 188–193. (In Chinese) [Google Scholar]
- Lu, X.Z.; Cheng, Q.L.; Xu, Z.; Xu, Y.J.; Sun, C.J. Real-time city-scale time-history analysis and its application in resilience-oriented earthquake emergency responses. Appl. Sci. 2019, 9, 3497. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). Multi-Hazard Loss Estimation Methodology—Earthquake Model Technical Manual (HAZUS-MH 2.1); Federal Emergency Management Agency: Washington, DC, USA, 2012.
- Kircher, C.A.; Whitman, R.V.; Holmes, W.T. HAZUS earthquake loss estimation methods. Nat. Hazards Rev. 2006, 7, 45–59. [Google Scholar] [CrossRef]
- Hosseinpour, V.; Saeidi, A.; Nollet, M.J.; Nastev, M. Seismic loss estimation software: A comprehensive review of risk assessment steps, software development and limitations. Eng. Struct. 2021, 232, 111866. [Google Scholar] [CrossRef]
- Yeh, C.H.; Loh, C.H.; Tsai, K.C. Overview of Taiwan earthquake loss estimation system. Nat Hazards 2006, 37, 23–37. [Google Scholar] [CrossRef]
- Chen, H.F.; Sun, B.T.; Chen, X.; Zhong, Y.Z. HAZ-China earthquake disaster loss estimation system. China Civ. Eng. J. 2013, 46 (Suppl. S2), 294–300. (In Chinese) [Google Scholar] [CrossRef]
- Ezz, A.A.E.; Smirnoff, A.; Nastev, M.; Nollet, M.J.; Mcgrath, H. ER2-Earthquake: Interactive web-application for urban seismic risk assessment. Int. J. Disaster Risk Reduct. 2019, 34, 326–336. [Google Scholar] [CrossRef]
- Lu, X.Z.; Han, B.; Hori, M.; Xiong, C.; Xu, Z. A coarse-grained parallel approach for seismic damage simulations of urban areas based on refined models and GPU/CPU cooperative computing. Adv. Eng. Softw. 2014, 70, 90–103. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Zheng, S.S.; Sun, L.F.; Li, L.; Yang, W.; Li, L. Developing GIS-based earthquake loss model: A case study of Baqiao District, China. Bull. Earthq. Eng. 2021, 19, 2045–2079. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). Next-Generation Performance-Based Seismic Design Guidelines Program Plan for New and Existing Buildings; Technical Report 445; Federal Emergency Management Agency: Washington, DC, USA, 2006.
- Yu, X.H. Global Probabilistic Seismic Capacity Analysis of Reinforced Concrete Frame Structures. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2007. [Google Scholar]
- Lu, D.G.; Liu, Y.; Yu, X.H. Seismic fragility models and forward-backward probabilistic risk analysis in second-generation performance-based earthquake engineering. Eng. Mech. 2019, 36, 12. (In Chinese) [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). Seismic Performance Assessment of Buildings: Volume 1—Methodology; Technical Report P58; Federal Emergency Management Agency: Washington, DC, USA, 2012.
- Baradaran Shoraka, M.; Yang, T.Y.; Elwood, K.J. Seismic loss estimation of non-ductile reinforced concrete buildings. Earthq. Eng. Struct. Dyn. 2013, 42, 297–310. [Google Scholar] [CrossRef]
- Shome, N.; Jayaram, N.; Krawinkler, H.; Rahnama, M. Loss estimation of tall buildings designed for the PEER tall building initiative project. Earthq. Spectra 2015, 31, 1309–1336. [Google Scholar] [CrossRef]
- Ramirez, C.; Miranda, E. Building-Specific Loss Estimation Methods and Tools for Simplified Performance-Based Earthquake Engineering; Report 171; the John A; Blume Earthquake Engineering Center, Stanford University: Stanford, CA, USA, 2009. [Google Scholar]
- T/SSC 1-2021; Seismic Destructive Power Evaluation Based on Strong Motion Records. Seismological Society of China Seismological Society of China (SSC): Beijing, China, 2021.
- Zeng, X.; Liu, S.X.; Xu, Z.; Lu, X.Z. Earthquake loss prediction for campus buildings based on FEMA-P58 method: A case study. Eng. Mech. 2016, 33 (Suppl. S1), 113–118. (In Chinese) [Google Scholar] [CrossRef]
- Zeng, X.; Lu, X.Z.; Yang, T.Y.; Xu, Z. Application of the FEMA-P58 methodology for regional earthquake loss prediction. Nat. Hazards 2016, 83, 177–192. [Google Scholar] [CrossRef]
- Xu, Z.; Zhang, H.Z.; Lu, X.Z.; Xu, Y.J.; Zhang, Z.C.; Li, Y. A prediction method of building seismic loss based on BIM and FEMA P-58. Autom. Constr. 2019, 102, 245–257. [Google Scholar] [CrossRef]
- Zhuang, Y.Y.; Xiong, F.; Lu, Y.; Zhang, B.W. Research on modeling method of GIS-based dynamic response analysis model for urban building clusters. World Earthq. Eng. 2021, 7, 137–147. (In Chinese) [Google Scholar] [CrossRef]
- Ruggieri, S.; Liguori, F.S.; Leggieri, V.; Bilotta, A.; Madeo, A.; Casolo, S.; Uva, G. An archetype-based automated procedure to derive global-local seismic fragility of masonry building aggregates: META-FORMA-XL. Int. J. Disaster Risk Reduct. 2023, 95, 103903. [Google Scholar] [CrossRef]
- Spence, R.; So, E.; Ameri, G.; Akinci, A.; Cocco, M.; Cultrera, G.; Franceschina, G.; Pacor, F.; Pessina, V.; Lombardi, A.W.; et al. Earthquake Disaster Scenario Prediction and Loss Modeling for Urban Areas; LESSLOSS, Istituto Universitario di Studi Superiori di Pavia: Pavia, Italy, 2007. [Google Scholar]
- Li, L. Study on Earthquake Damage Prediction of Urban Buildings and Earthquake Economic Losses. Master’s Thesis, Dalian University of Technology, Dalian, China, 2007. [Google Scholar]
- Xiong, C.; Lu, X.Z.; Lin, X.C.; Xu, Z.; Ye, L.P. Parameter determination and damage assessment for THA-based regional seismic damage prediction of multi-story buildings. J. Earthq. Eng. 2016, 21, 461–485. [Google Scholar] [CrossRef]
- Xiong, C.; Lu, X.Z.; Guan, H.; Xu, Z. A nonlinear computational model for regional seismic simulation of tall buildings. Bull. Earthq. Eng. 2016, 14, 1047–1069. [Google Scholar] [CrossRef]
- Steelman, J.S.; Hajjar, J.F. Influence of inelastic seismic response modeling on regional loss estimation. Eng. Struct. 2009, 31, 2976–2987. [Google Scholar] [CrossRef]
- GB 50011-2010; Code for Seismic Design of Buildings. Ministry of Housing and Urban-Rural Development of China (MOHURD): Beijing, China, 2010.
- Huang, J.L. Research on Prediction Method of Seismic Direct Economic Loss of Building Complex. Master’s Thesis, South China University of Technology, Guangzhou, China, 2020. [Google Scholar]
- Su, Q.W.; Liu, Y.H.; Zhao, S.C. Seismic performance of masonry buildings based on wall area. China Civ. Eng. J. 2010, 43 (Suppl. S1), 473–478. (In Chinese) [Google Scholar] [CrossRef]
- GB11968-2020; Standard for Autoclaved Aerated Concrete Blocks. National Standardization Committee (NSC): Beijing, China, 2020.
- GB 50016-2014; Code for Fire Protection Design of Buildings. Ministry of Housing and Urban-Rural Development (MOHURD): Beijing, China, 2014.
- GB 50009-2012; Load Code for the Design of Building Structures. Ministry of Housing and Urban-Rural Development (MOHURD): Beijing, China, 2012.
- Li, G.S. Design Handbook of High-Rise Reinforced Concrete Structure; China Building Industry Press: Beijing, China, 2011; pp. 390–433. [Google Scholar]
- Vamvatsikos, D.; Cornell, C. Incremental dynamic analysis. Earthq. Eng. Struct. Dyn. 2002, 31, 491–514. [Google Scholar] [CrossRef]
- Federal Emergency Management Agency (FEMA). Quantification of Building Seismic Performance Factors; Technical Report 695; Federal Emergency Management Agency: Washington, DC, USA, 2009.
- Structural Engineers Association of California (SEAOC). Performance-Based Seismic Engineering of Buildings: Conceptual Framework, Volume I and II, Vision 2000; Structural Engineers Association of California: Sacramento, CA, USA, 1995. [Google Scholar]
- Grunthal, G. European Macroseismic Scale 1998 (EMS-98); Cahiers du Centre European de Geodynamic et de Seismology 15; Centre European de Geodynamique et de Seismology: Luxembourg, 1998. [Google Scholar]
- GB/T 24335-2009; Classification of Earthquake Damage to Buildings and Special Structures. Ministry of Housing and Urban-Rural Development (MOHURD): Beijing, China, 2009.
- Federal Emergency Management Agency (FEMA). NEHRP Guidelines for the Seismic Rehabilitation of Buildings; Technical Report 273; Federal Emergency Management Agency: Washington, DC, USA, 1997.
- JGJ/T 415-2017; Technical Specification for Post-Earthquake Urgent Assessment and Repair of Buildings. Ministry of Housing and Urban-Rural Development (MOHURD): Beijing, China, 2017.
- Lin, S.B.; Xie, L.L.; Gong, M.S.; Li, M. Performance-based methodology for assessing seismic vulnerability and capacity of buildings. Earthq. Eng. Eng. Vib. 2010, 9, 157–165. (In Chinese) [Google Scholar] [CrossRef]






| Design Conditions | ||
|---|---|---|
| Intensity 7, Class II soil | 3~5% | 2~3% |
| Intensity 8, Class II soil | 4~6% | 3~4% |
| Type | Variable | Sample Values |
|---|---|---|
| Discrete | Story height | 3.3 m |
| Two-way main beam span | 6.3 m, 5.7 m | |
| Span of the secondary beam | 3.1 m | |
| The cross-sectional area of the frame column | 0.75 × 0.75 m2 | |
| Section height of the shear wall | 6.2 m | |
| Section thickness of the shear wall | 200 mm | |
| Strength of materials | C40 | |
| Number of stairwells and elevator shafts | 2, 3 | |
| Continuous | The ratio of the cross-sectional area of infill walls to the floor area | 0.0765 |
| The ratio of the shear wall cross-sectional area to the floor area | 0.0272 | |
| The height–span ratio of the main and secondary beam section | 0.1159, 0.0672 | |
| The height–width ratio of the main and secondary beam section | 0.4836, 0.4597 |
| Parameters | Statistics | ||||
|---|---|---|---|---|---|
| Max | Min | Mean | Standard Deviation | Dispersion Coefficient | |
| GA (1010 ) | 1.8290 | 0.4833 | 1.0163 | 0.3745 | 0.3685 |
| EI (109 ) | 6.5899 | 2.9024 | 4.5731 | 0.8160 | 0.1784 |
| λ | 1.9502 | 1.0938 | 1.4673 | 0.2093 | 0.1427 |
| m (105 kg) | 9.8294 | 6.2419 | 7.5343 | 0.7310 | 0.0970 |
| Damage Index | Damage State | ||||
|---|---|---|---|---|---|
| Intact | Slight | Moderate | Severe | Complete | |
| Inter-story drift ratio | <1/550 | 1/550~1/100 | 1/100~1/50 | 1/50~1/25 | >1/25 |
| Component Types | Damage Degree | |||
|---|---|---|---|---|
| 1 | 2 | 3 | Unrepairable | |
| Infilled wall | 0.15 | 0.5 | 1 | 1 |
| Shear wall | 0.1 | 0.3 | 0.7 | 1 |
| Frame beam | 0.1 | 0.3 | 0.8 | 1 |
| Frame column | 0.1 | 0.3 | 0.7 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Lu, Y.; Xiong, F. A Story-Based Random Structure Modeling Method for Regional Buildings Earthquake Loss Estimation. Appl. Sci. 2024, 14, 1849. https://doi.org/10.3390/app14051849
Yang Z, Lu Y, Xiong F. A Story-Based Random Structure Modeling Method for Regional Buildings Earthquake Loss Estimation. Applied Sciences. 2024; 14(5):1849. https://doi.org/10.3390/app14051849
Chicago/Turabian StyleYang, Zhi, Yang Lu, and Feng Xiong. 2024. "A Story-Based Random Structure Modeling Method for Regional Buildings Earthquake Loss Estimation" Applied Sciences 14, no. 5: 1849. https://doi.org/10.3390/app14051849
APA StyleYang, Z., Lu, Y., & Xiong, F. (2024). A Story-Based Random Structure Modeling Method for Regional Buildings Earthquake Loss Estimation. Applied Sciences, 14(5), 1849. https://doi.org/10.3390/app14051849






