Seismic Response Analysis of Concrete Box-Type Subgrade in High-Speed Railways
Abstract
1. Introduction
2. Basic Requirements for Seismic Design of Box-Type Subgrades
3. Computational Methods and Models
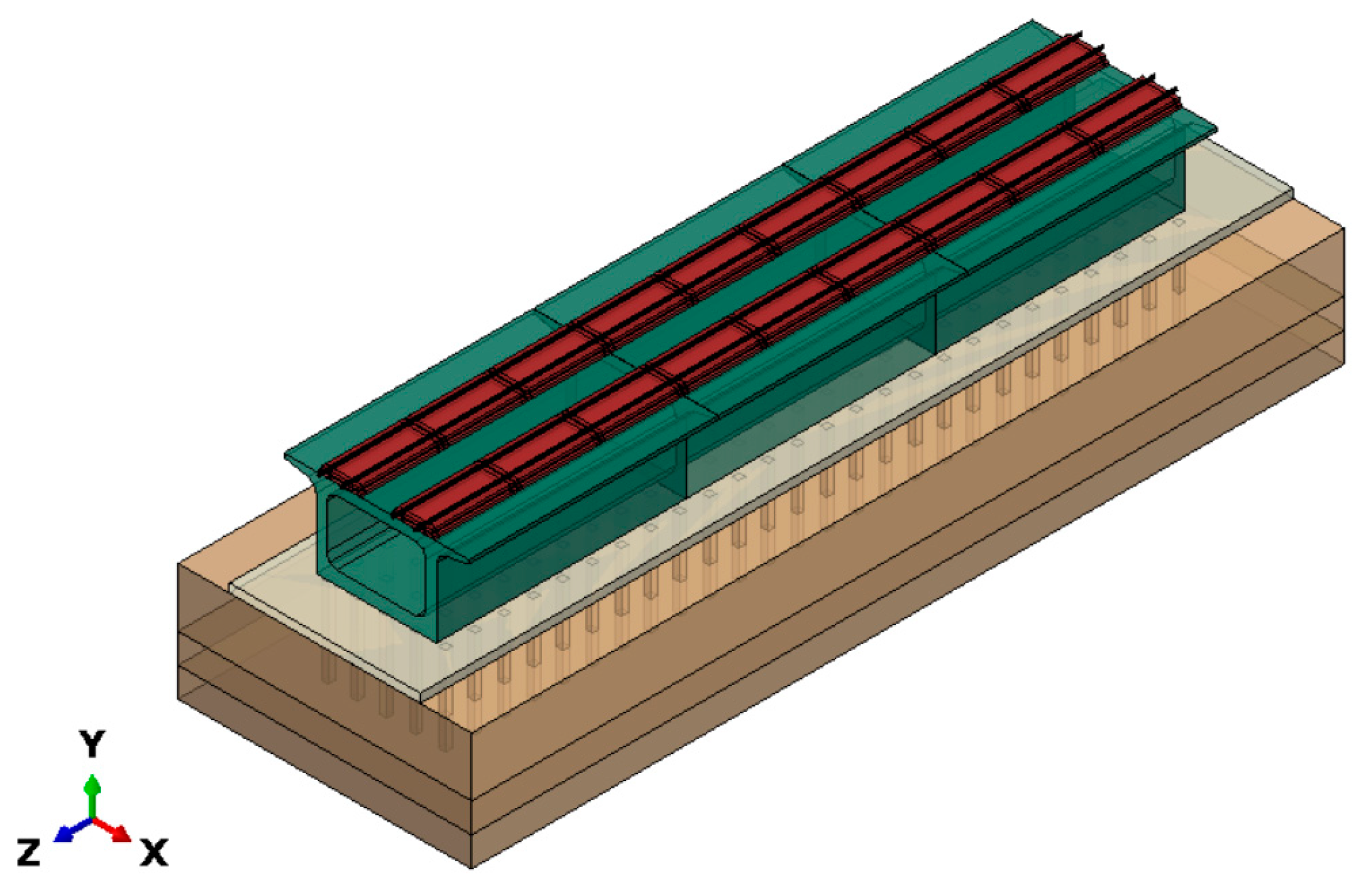
3.1. Finite Element Model
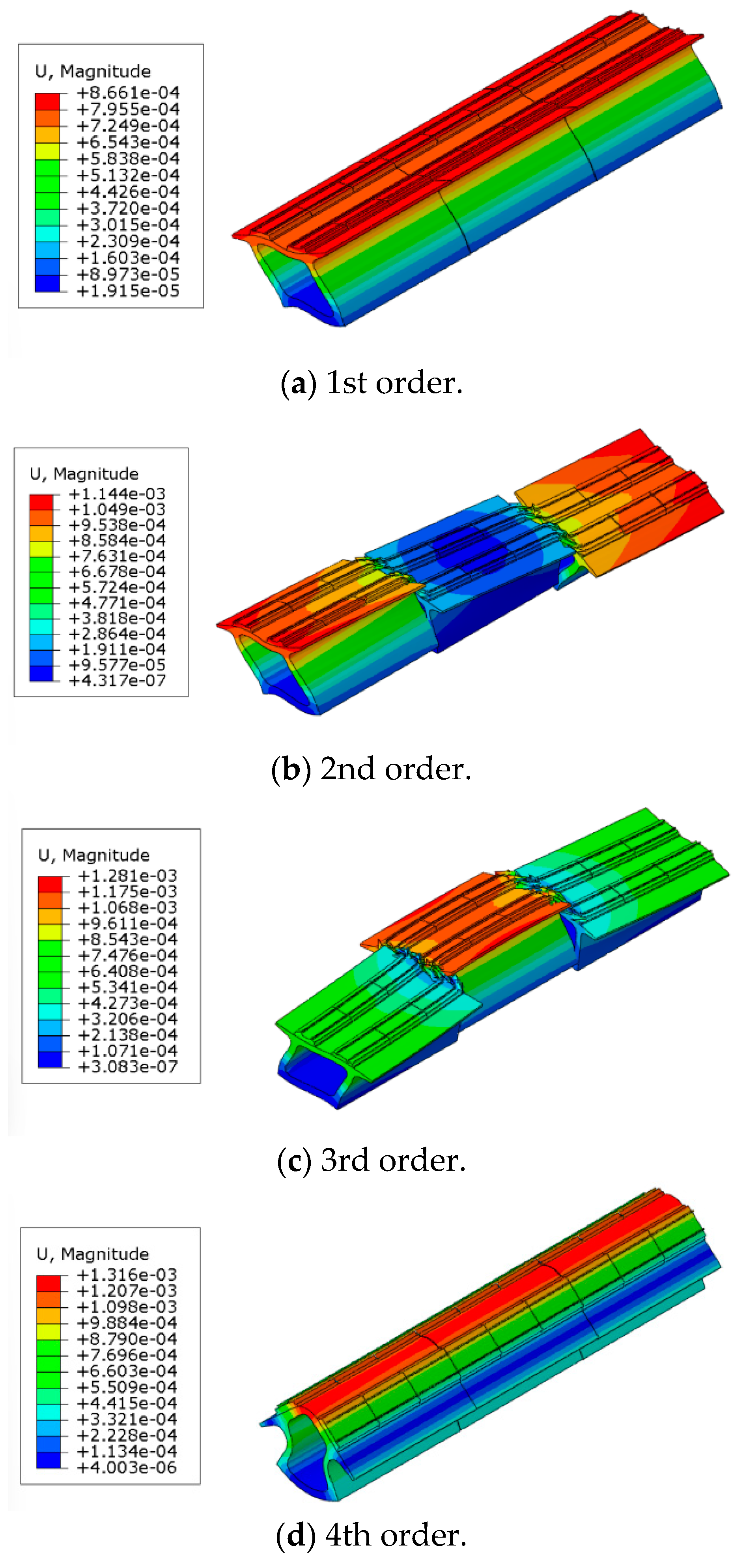
3.2. Analysis of the Self-Vibration Characteristics of Box-Type Subgrade Structures
3.3. Seismic Wave Input
4. Seismic Response of Box-Type Subgrade
4.1. System Dynamic Response Characteristics Under Multi-Dimensional Seismic Coupling
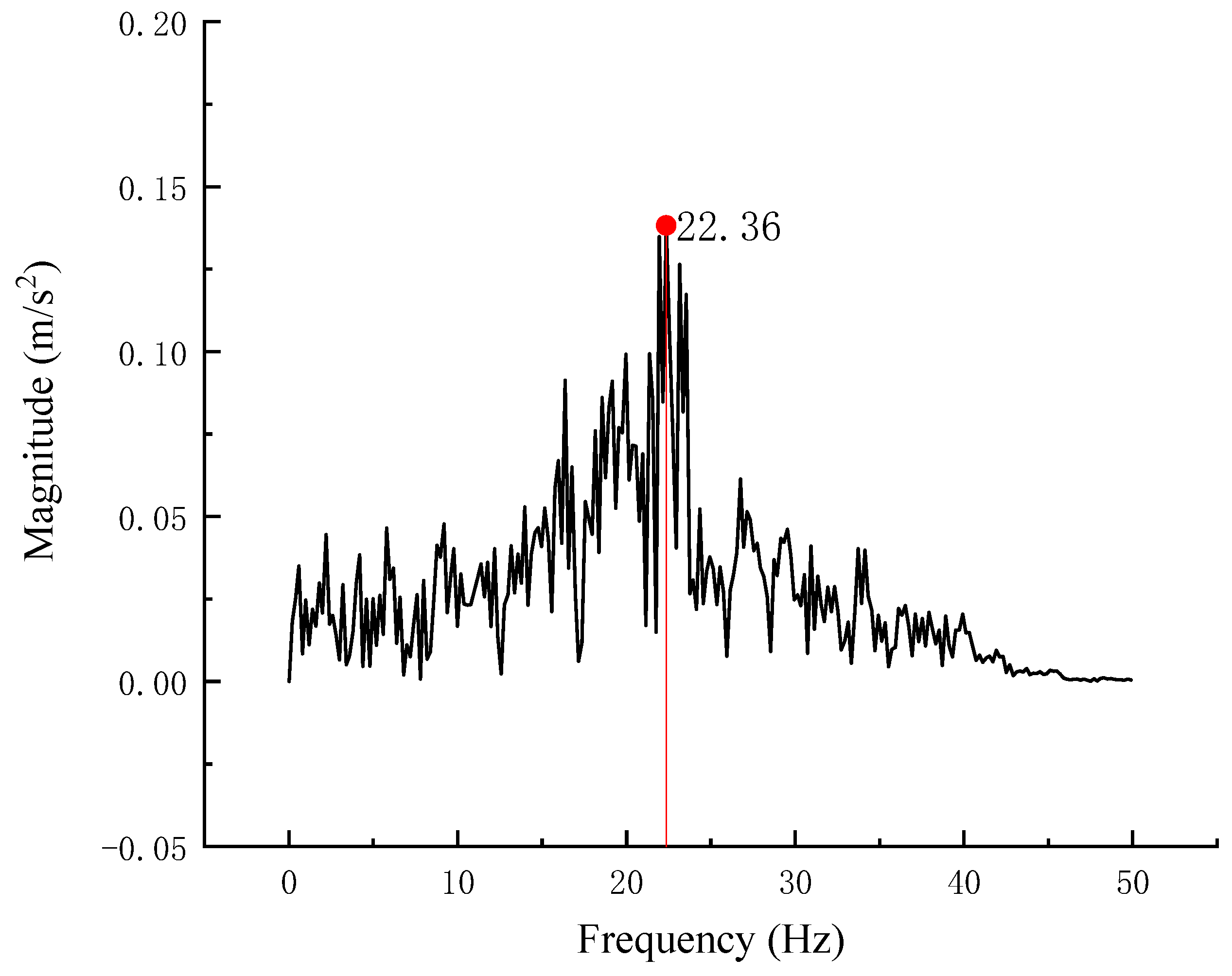
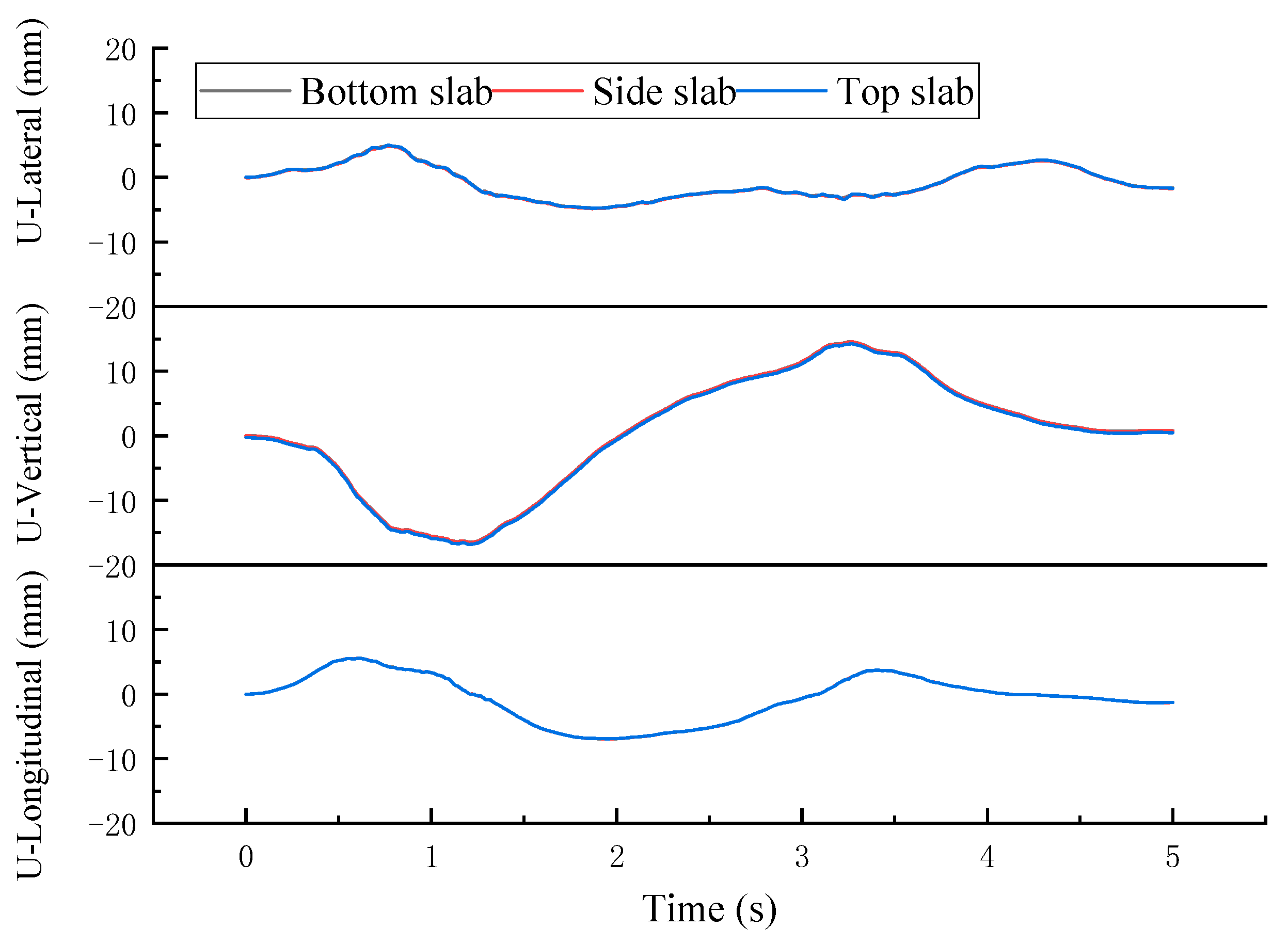
4.1.1. Vibration and Response Analysis of Box Foundation Based on the Time–Frequency Domain Method
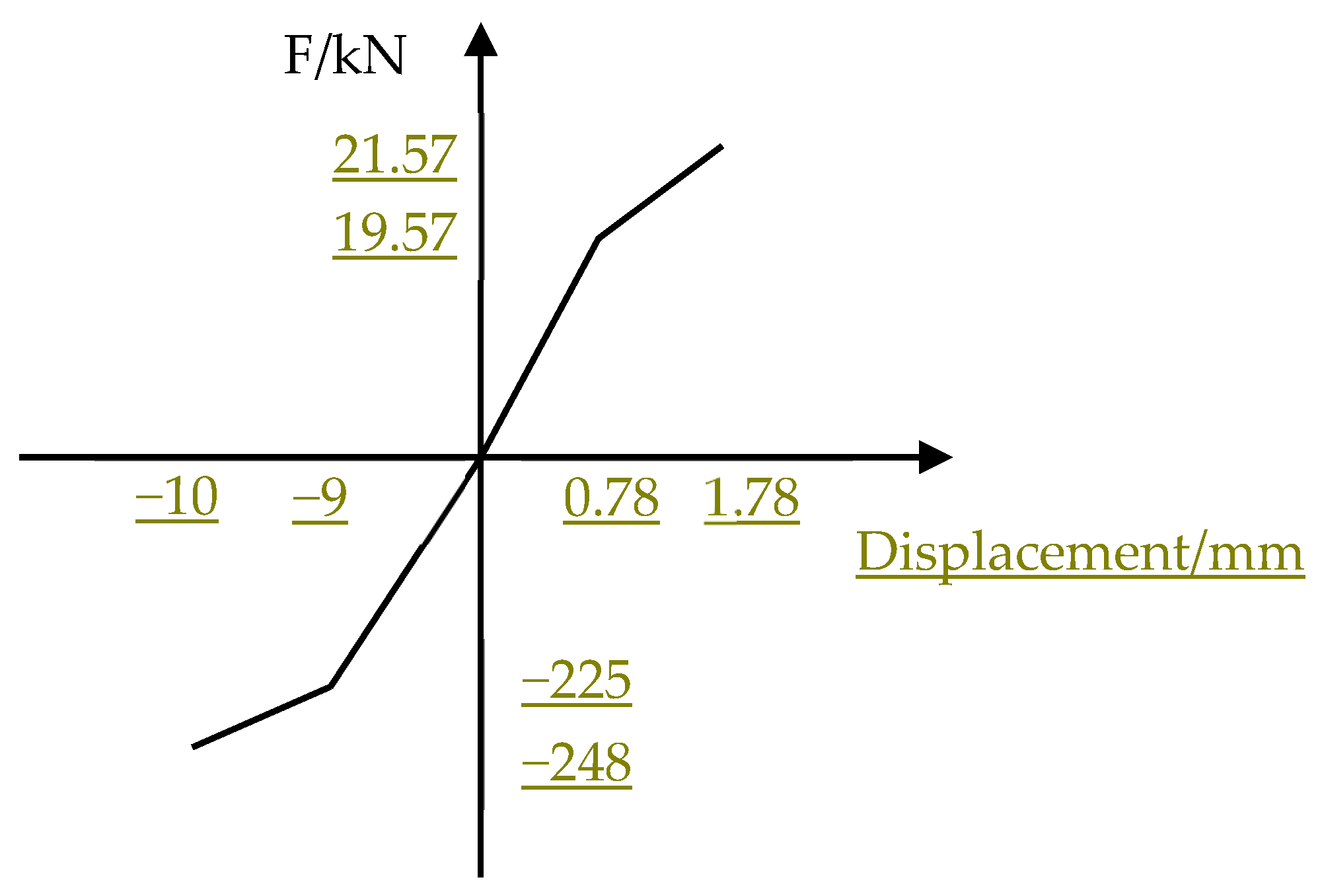
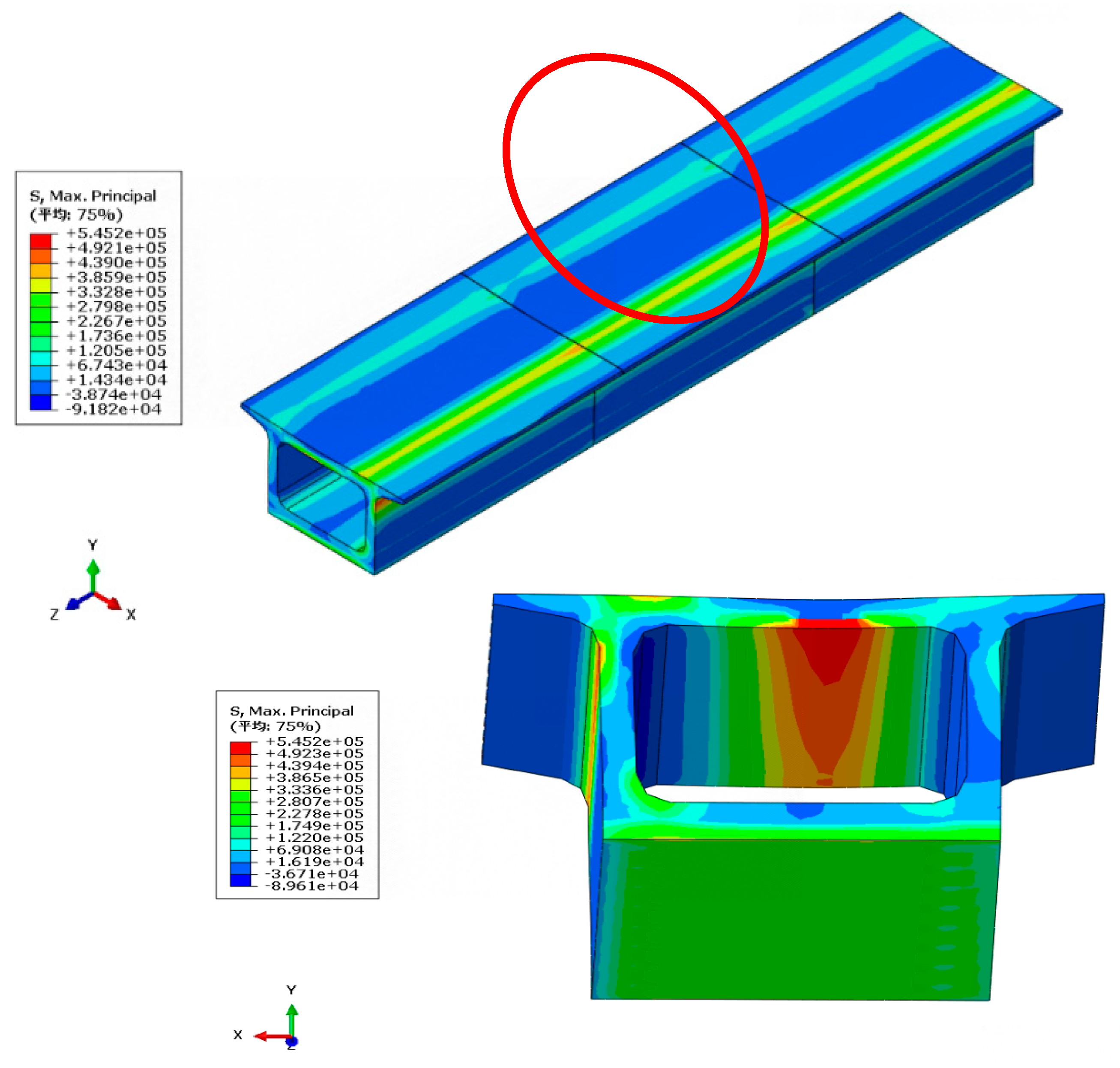
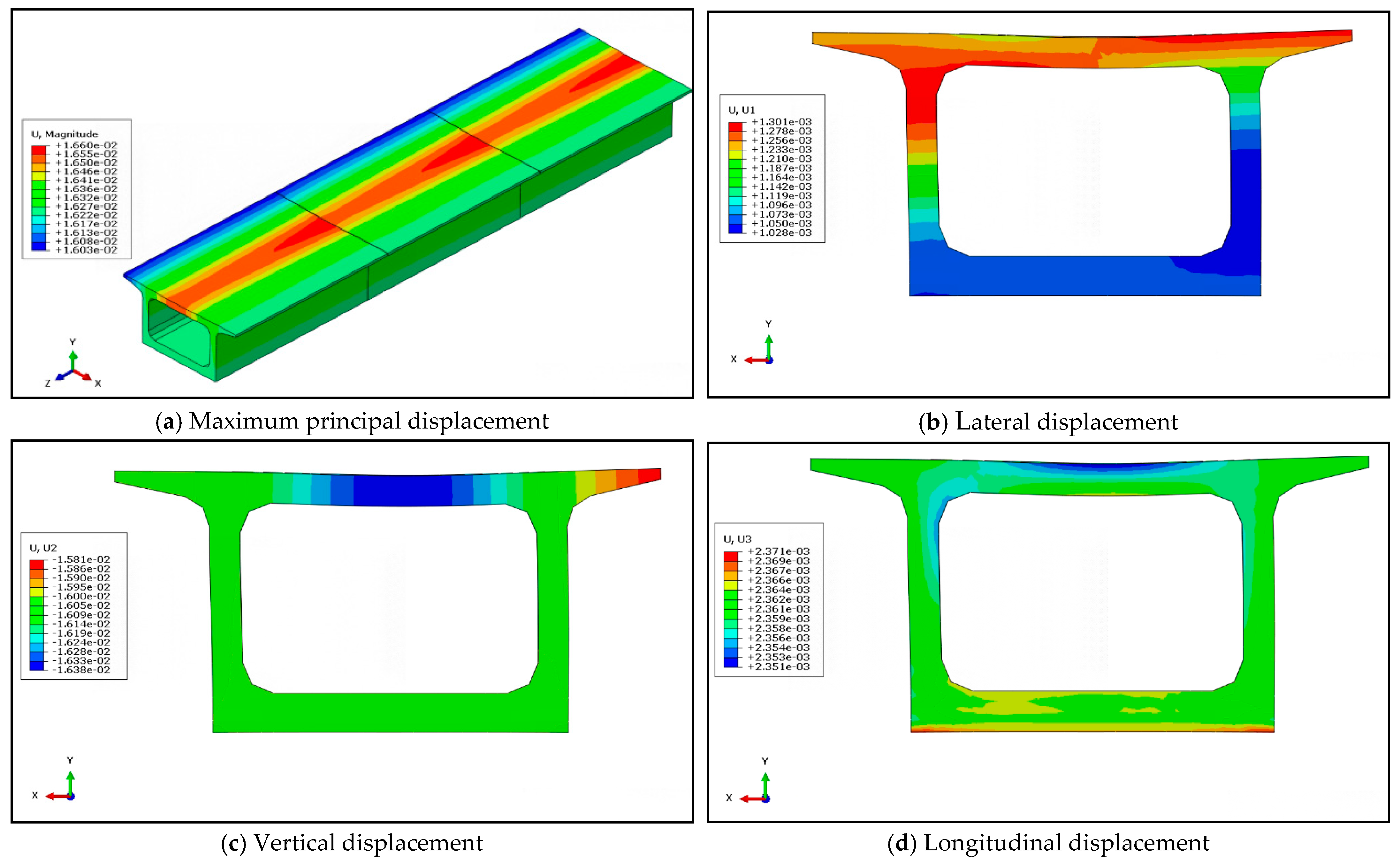
4.1.2. Force and Deformation Characteristics of Box-Type Subgrade
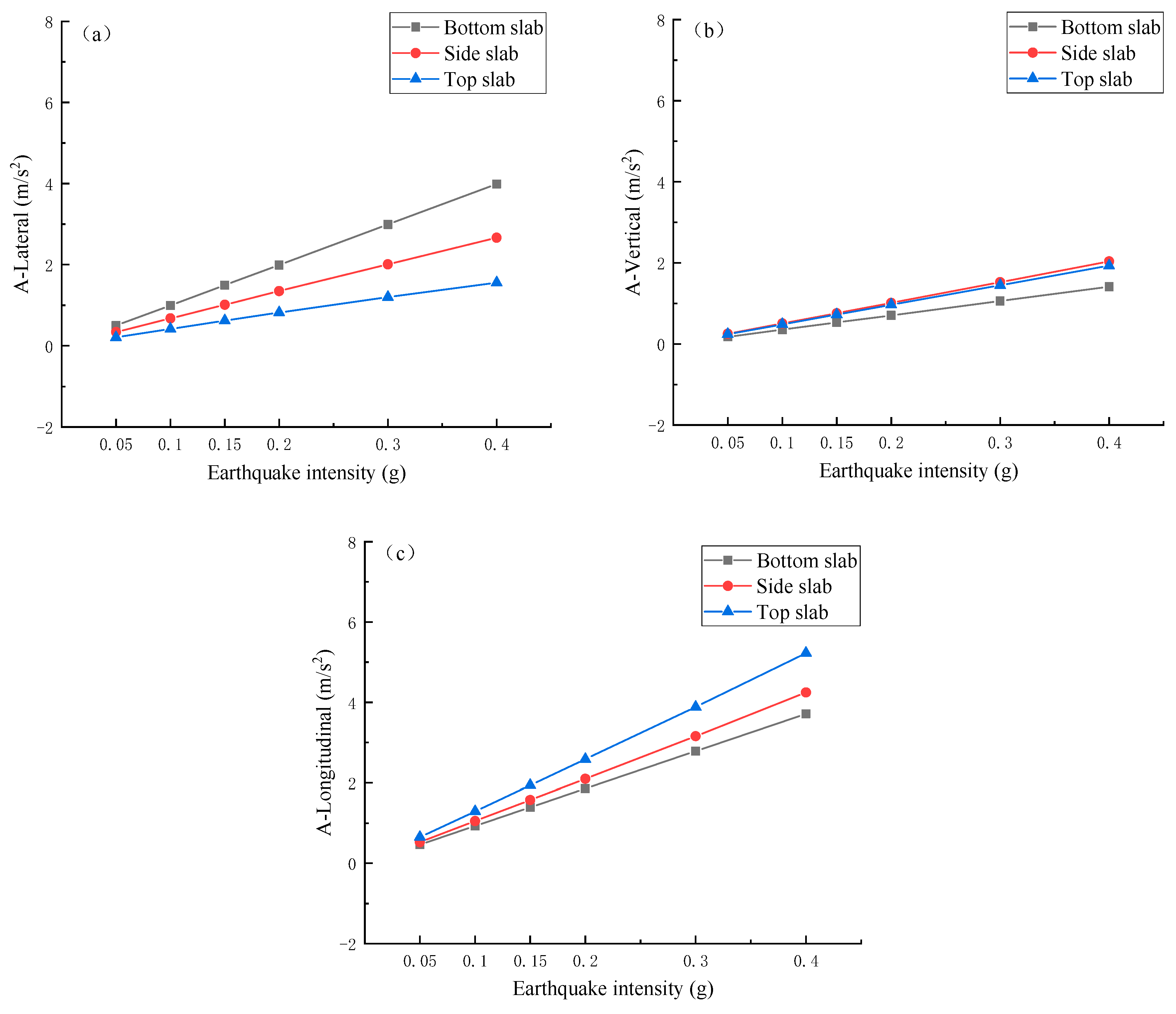
4.2. Dynamic Response of Box Culvert Foundations Under Different Earthquake Intensities
5. Conclusions
- (1)
- Due to the contact connection between the box-type subgrade and the underlying foundation, the vibration response of the box-type subgrade under seismic action is primarily the rigid body motion. The deformation of various components, such as the top slab, side walls, and bottom slab, of the box-type subgrade under seismic action is small. Overall, it can meet the seismic defense requirements of “no damage in minor earthquakes, repairable in moderate earthquakes, and no collapse in major earthquakes”.
- (2)
- Under seismic action, there is tensile stress concentration at the contact area between the bottom of the top slab and the side walls of the box-type subgrade, but it is far below the designed strength value, with the bottom slab being in compression. In terms of vibration amplification factors, the acceleration amplification factor of the box-type subgrade is just 1.44 at resonance. Compared with traditional simply supported beams, the seismic amplification factor of the box-type subgrade is relatively small, indicating better adaptability in strong earthquakes.
- (3)
- The analysis shows that the frequency characteristics of the acceleration response time–history curve of the box-type subgrade under seismic action are basically consistent with the input seismic wave; therefore, it is suggested that the seismic alarm threshold setting for the box-type subgrade system uses the original acceleration ground motion parameters.
- (4)
- With an increase in seismic intensity, the vibration and deformation of the box-type subgrade change linearly. The deformation occurs mainly in the vertical displacement, with the horizontal displacement being relatively small and the growth rate relatively low. However, the structural force increases nonlinearly, exhibiting a slower growth at low seismic intensities. Once the design basic acceleration peak crosses 0.2 g, the force on the box-type subgrade structure increases significantly, and the rate of damage accelerates.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- GB 18306-2015; China’s Ground Motion Parameter Zoning Map. Standard Press: Beijing, China, 2015.
- Jiang, L.; Kang, X.; Li, C.; Shao, G. Earthquake response of continuous girder bridge for high-speed railway: A shaking table test study. Eng. Struct. 2019, 180, 249–263. [Google Scholar] [CrossRef]
- Yu, L.; Zhu, Z.; Qin, Y.; Wang, X.; Wang, Y.; Zheng, W. Study on the settlement limit of high-speed railway box subgrade. J. Railw. Sci. Eng. 2023, 20, 3203–3216. [Google Scholar] [CrossRef]
- Zongyu, Z.; Lei, Y.; Dijia, T.; Junjie, H.; Qian, S. Analysis of static and dynamic characteristics of high-speed railway flange frame box subgrade. Subgrade Eng. 2023, 4, 6–12. [Google Scholar] [CrossRef]
- Xie, H.; Xiao, S. Calculation method for high-fill box-type embankment structures of railways. J. Shandong Univ. Eng. Sci. 2022, 4, 166–174. [Google Scholar]
- Nilimaa, J. Smart materials and technologies for sustainable concrete construction. Dev. Built Environ. 2023, 15, 100177. [Google Scholar] [CrossRef]
- Tullini, N.; Traili, A.; Baraldi, D. Stability of slender beams and frames resting on 2D elastic half-space. Arch. Appl. Mech. 2013, 83, 467–482. [Google Scholar] [CrossRef]
- Tezzon, E.; Tullini, N.; Minghini, F. Static analysis of shear flexible beams and frames in adhesive contact with an isotropic elastic half-plane using a coupled fe-bie model. Eng. Struct. 2015, 104, 32–50. [Google Scholar] [CrossRef]
- Baraldi, D.; Tullini, N. Incremental analysis of elastoplastic beams and frames resting on an elastic half-plane. J. Eng. Mech. 2017, 143, 04017101. [Google Scholar] [CrossRef]
- Mylona, E.K.V.; Sextos, A.G.; Mylonakis, G.E. Rotational seismic excitation effects on CIDH pile-supported bridge piers. Eng. Struct. 2017, 138, 181–194. [Google Scholar] [CrossRef][Green Version]
- Liu, Z.-N.; Chen, X.-C.; Zhang, Y.-L. Longitudinal seismic response of ballastless railway bridges considering traveling wave effect. J. Vib. Shock 2020, 39, 142–149. [Google Scholar]
- Toyooka, A.; Ikeda, M.; Yanagawa, H.; Kataoka, H.; Iemura, H.; Murata, K. Effects of track structure on seismic behavior of isolation system bridges. Q. Rep. RTRI 2005, 46, 238–243. [Google Scholar] [CrossRef]
- Zhang, Y.-L.; Yang, S.-J.; Chen, X.-C. Seismic response analysis of high-speed railway bridges based on rail-bridge integrated model. Bridge Constr. 2016, 46, 23–28. [Google Scholar]
- Yang, M.-G.; Meng, D.-L.; Dai, L.-Y. Transverse seismic pounding effect for simply-supported girder bridges of high-speed railway considering track constraint. J. Cent. South Univ. Sci. Technol. 2018, 49, 916–924. [Google Scholar]
- Zhang, P.-F.; Cai, K.; Wang, C.-L.; Lei, X.-Y. Dynamic response of CRTSIII slab ballastless track system on bridge with multi-dimensional earthquake action. Sci. China Technol. Sci. 2024, 54, 924–939. [Google Scholar]
- Ai, Z.-L. Study of nonlinear time-history analysis on railway portal pier. J. Railw. Eng. Soc. 2014, 31, 61–66. [Google Scholar]
- Sun, Z.-F. Design and research of large span steel box portal pier for high speed railway. J. Railw. Eng. Soc. 2019, 36, 43–47. [Google Scholar]
- GB50111-2006; Code for Seismic Design of Railway Engineering. Plan Press: Beijing, China, 2009.
- Liu, Z.-W. Research on seismic performance and design method for high-speed railway bridges based on track-bridge integrated model. Chin. J. Rock Mech. Eng. 2020, 39, 1080. (In Chinese) [Google Scholar]
- Yan, B.; Kuang, W.-F.; Yu, L.-M.; Luo, B.-F. Seismic response characteristics of high-speed railway portal piers, bridge-track systems. J. Cent. South Univ. Nat. Sci. Ed. 2023, 54, 3544–3550. [Google Scholar]
- Maleska, T.; Beben, D.; Vaslestad, J.; Sergei Sukuvara, D. Application of EPS Geofoam below Soil–Steel Composite Bridge Subjected to Seismic Excitations. J. Geotech. Geoenvironmental Eng. 2024, 150, 12674. [Google Scholar] [CrossRef]
- Lu, R. Comparative Analysis of Simplified Seismic Calculation Methods for Pile Group Bridge Structures. Master’s Thesis, Tongji University, Shanghai, China, 2001. [Google Scholar]
- Lu, J.; Chen, X.; Ding, M.; Ma, H.; Zhang, X. Research on simplified seismic calculation models for railway gravity piers. Vib. Shock 2021, 9, 71–76. [Google Scholar] [CrossRef]
- Du, X.-T.; Xia, H.; Yu, Z. Research on the Excitation Input Mode in Seismic Analysis of Bridges. J. Railw. Sci. Eng. 2011, 33, 86–90. [Google Scholar]
- Ding, Y.; Zhang, G.; Zhao, X.; Lu, Z.; Li, X. Shear performance of horizontally curved steel box bridge girders under hydrocarbon fire exposure conditions: Numerical investigation and design implications. Thin-Walled Struct. 2024, 205, 112479. [Google Scholar] [CrossRef]
- TB0015-2012; Specifications for the Design of Railway Track without Joints. Railway Publishing House: Beijing, China, 2012.
- Zhang, Y.; Xu, J.; Liu, C.; Chen, X. Seismic response of railway bridge pile foundations under cap-pier-soil interaction. J. Railw. Eng. Soc. 2020, 5, 30–35. [Google Scholar]
- Gao, G.; Pan, T.; Geng, J.; Chen, S. Vibration characteristics of pile-net composite foundation under the combined action of earthquakes and high-speed trains. Noise Vib. Control. 2024, 1, 7–14. [Google Scholar]
- Luo, Y.; Liu, X.; Cui, J.; Wang, H.; Huang, L.; Zeng, W.; Zhang, H. Lifetime fatigue cracking behavior of weld defects in orthotropic steel bridge decks: Numerical and experimental study. Eng. Fail. Anal. 2025, 167, 108993. [Google Scholar] [CrossRef]
- Embaby, M.; El Naggar, M.H. Experimental and numerical investigation for steel shear panels of modular bridge. J. Constr. Steel Res. 2024, 219, 108743. [Google Scholar] [CrossRef]
- Nicoletti, R.S.; Rossi, A.; de Souza AS, C.; Martins, C.H. Numerical assessment of effective width in steel-concrete composite box girder bridges with partial interaction. Eng. Struct. 2021, 239, 112333. [Google Scholar] [CrossRef]
- Wei, B.; Yang, T.-H.; Jiang, L.-Z. The Influence of the Refinement of Track Structure Modeling on the Seismic Vulnerability of High-Speed Railway Continuous Beam Bridges. Eng. Mech. 2018, 35, 16–23+51. [Google Scholar]
- Yang, Y.-Q.; Yao, J.-C.; Liu, P.-H.; Ke, Z. Experimental Study on Dynamic Performance of Ballastless Track Railway Bridges with Common Spans. China Railw. Sci. 2008, 29, 47–52. [Google Scholar]
- Yan, B.; Liu, S.; Dai, G.-L.; Pu, H.; Tang, J.-F. Nonlinear Interaction between Bridge and Ballastless Track under Multi-Dimensional Seismic Actions. J. Railw. Eng. 2016, 38, 74–80. [Google Scholar]
- Zhan, Y.-X.; Jiang, G.-L.; Wei, Y.-X. Dynamic Response Analysis of Ballastless Track Pile Slab Structure Subgrade under Seismic Load. China Railw. Sci. 2006, 6, 22–26. [Google Scholar]
- Liu, Z.; Guo, E.-D.; He, Q.; Shengjian, L. Seismic Response Analysis of High-Speed Railway Coupled Vehicle-Bridge System. Earthq. Eng. Eng. Vib. 2015, 35, 119–124. [Google Scholar] [CrossRef]
- Meng, X. Analysis of Vertical Resonance Conditions for Simplified Supported Box Beams with Common Spans in High-Speed Railways. Vib. Shock 2019, 38, 19–24+32. [Google Scholar] [CrossRef]
- Xiao, W.-J. National Earthquake Intensity Rapid Reporting and Early Warning Project. Disaster Prev. Mitig. 2023, 1, 18–23. [Google Scholar]
- Lin, L.; Li, C.-H.; Wei, Y.-M. Selection of Computational Parameters for Earthquake Intensity Rapid Reporting and Early Warning System. J. Heilongjiang Coll. Eng. 2019, 33, 20–23. [Google Scholar] [CrossRef]
- Wang, Y.; Xiao, H.; Zhang, Z.; Guo, X.; Liu, Q. Self-supervised representation learning of metro interior noise based on variational autoencoder and deep embedding clustering. Comput.-Aided Civ. Infrastruct. Eng. 2024, 1–20. [Google Scholar] [CrossRef]









| Fortification Intensity | 6 | 7 | 8 | 9 | ||
|---|---|---|---|---|---|---|
| Frequent Earthquakes | 0.02 g | 0.04 g | 0.05 g | 0.07 g | 0.10 g | 0.14 g |
| Design Earthquakes | 0.05 g | 0.10 g | 0.15 g | 0.20 g | 0.30 g | 0.40 g |
| Rare Earthquakes | 0.11 g | 0.21 g | 0.32 g | 0.38 g | 0.57 g | 0.64 g |
| Seismic Performance Standards | Structural Working Conditions | Remarks |
|---|---|---|
| Performance Requirement I | Normal Operating State | The structure can be considered an elastic system, and under the expected seismic action, the structure generally suffers no damage or minor damage without interrupting traffic. |
| Performance Requirement II | Inelastic Operating State | The inelastic deformation of the structure or the damage to the structural system should be controlled within a repairable range. Under the expected seismic action, the structure should not suffer significant damage and can be a limited-speed operation open to traffic after repair. |
| Performance Requirement III | Elastoplastic Working Phase | The structure undergoes significant inelastic deformation, but it should be controlled within the specified range. Under the expected seismic action, the structure may suffer considerable damage but should not collapse entirely. It can be a limited-speed operation open to traffic after emergency repair. |
| Seismic Ground Motion Level | Frequent Earthquakes | Design Earthquakes | Rare Earthquakes |
|---|---|---|---|
| Structures | Bridges | Subgrades, retaining walls, tunnels, abutments, and connections between upper and lower bridge structures | Bridges with reinforced concrete piers |
| Seismic Design Objectives | Meet Seismic Performance Requirement I | Meet Seismic Performance Requirement II | Meet Seismic Performance Requirement III |
| Analysis Methods | General Bridges: Response Spectrum Method | Static method | Simplified method for ductility design of reinforced concrete piers |
| Name | Density (kg/m3) | Elastic Modulus (Pa) | Poisson’s Ratio | Dimensions (m) |
|---|---|---|---|---|
| Rebar | 7800 | 1.9 × 109 | 0.3 | HRB400 |
| Rail | 7850 | 2.1 × 1011 | 0.3 | 60 kg/m |
| Track Slab | 2500 | 3.6 × 1010 | 0.167 | 2.5 × 2.0 × 5.6 |
| Self-Compacting Concrete | 2000 | 3 × 109 | 0.167 | 2.5 × 0.9 × 5.6 |
| Base Plate | 2500 | 3.25 × 1010 | 0.167 | 2.95 × 2.0 × 5.65 |
| Order | Frequency (Hz) | Box-Type Subgrade Deformation State |
|---|---|---|
| 1 | 9.24 | Lateral deformation in the same direction |
| 2 | 10.74 | Lateral deformation in opposite directions |
| 3 | 13.84 | Lateral deformation in opposite directions |
| 4 | 23.10 | Vertical deformation in the upward and downward directions |
| 5 | 23.21 | Vertical torsional deformation |
| 6 | 24.64 | Vertical torsional deformation |
| 7 | 25.00 | Horizontal torsional deformation |
| 8 | 26.15 | Horizontal torsional deformation |
| 9 | 27.19 | Vertical torsional deformation |
| 10 | 27.50 | Vertical torsional deformation |
| Beam Structure | Vertical Natural Frequency (Hz) | Horizontal Natural Frequency (Hz) |
|---|---|---|
| 16.99 m Box-Type Subgrade | 23.10 | 9.24 |
| 24 m Simply Supported Beam | 11.25 | 21.34 |
| 32 m Simply Supported Beam | 8.08 | 15.59 |
| Vibration Amplification Factors | Longitudinal | Transverse | Vertical |
|---|---|---|---|
| Peak Value of Input Seismic Wave | 3.714 | 3.987 | 1.413 |
| Bottom Slab (Peak Acceleration/Amplification Factor) | 3.714/1 | 3.987/1 | 1.413/1 |
| Side Slab (Peak Acceleration/Amplification Factor) | 4.235/1.14 | 2.673/0.67 | 2.039/1.44 |
| Top Slab (Peak Acceleration/Amplification Factor) | 5.151/1.39 | 1.559/0.39 | 1.945/1.38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-Y.; Xiao, H.; Song, X.-G.; Guo, S.-J.; Luo, B.-E.; Nadakatti, M.M. Seismic Response Analysis of Concrete Box-Type Subgrade in High-Speed Railways. Appl. Sci. 2024, 14, 11899. https://doi.org/10.3390/app142411899
Chen Y-Y, Xiao H, Song X-G, Guo S-J, Luo B-E, Nadakatti MM. Seismic Response Analysis of Concrete Box-Type Subgrade in High-Speed Railways. Applied Sciences. 2024; 14(24):11899. https://doi.org/10.3390/app142411899
Chicago/Turabian StyleChen, Ying-Ying, Hong Xiao, Xu-Guo Song, Shuai-Jie Guo, Bei-Er Luo, and Mahantesh M. Nadakatti. 2024. "Seismic Response Analysis of Concrete Box-Type Subgrade in High-Speed Railways" Applied Sciences 14, no. 24: 11899. https://doi.org/10.3390/app142411899
APA StyleChen, Y.-Y., Xiao, H., Song, X.-G., Guo, S.-J., Luo, B.-E., & Nadakatti, M. M. (2024). Seismic Response Analysis of Concrete Box-Type Subgrade in High-Speed Railways. Applied Sciences, 14(24), 11899. https://doi.org/10.3390/app142411899






