Experimental Study of Material Proportioning for Similar Modeling of Brittle Rocks
Abstract
1. Introduction
2. Materials and Methods
2.1. Selection of Material Components
2.2. Orthogonal Design of Material Proportions
2.3. Material Mixing and Specimen Preparation
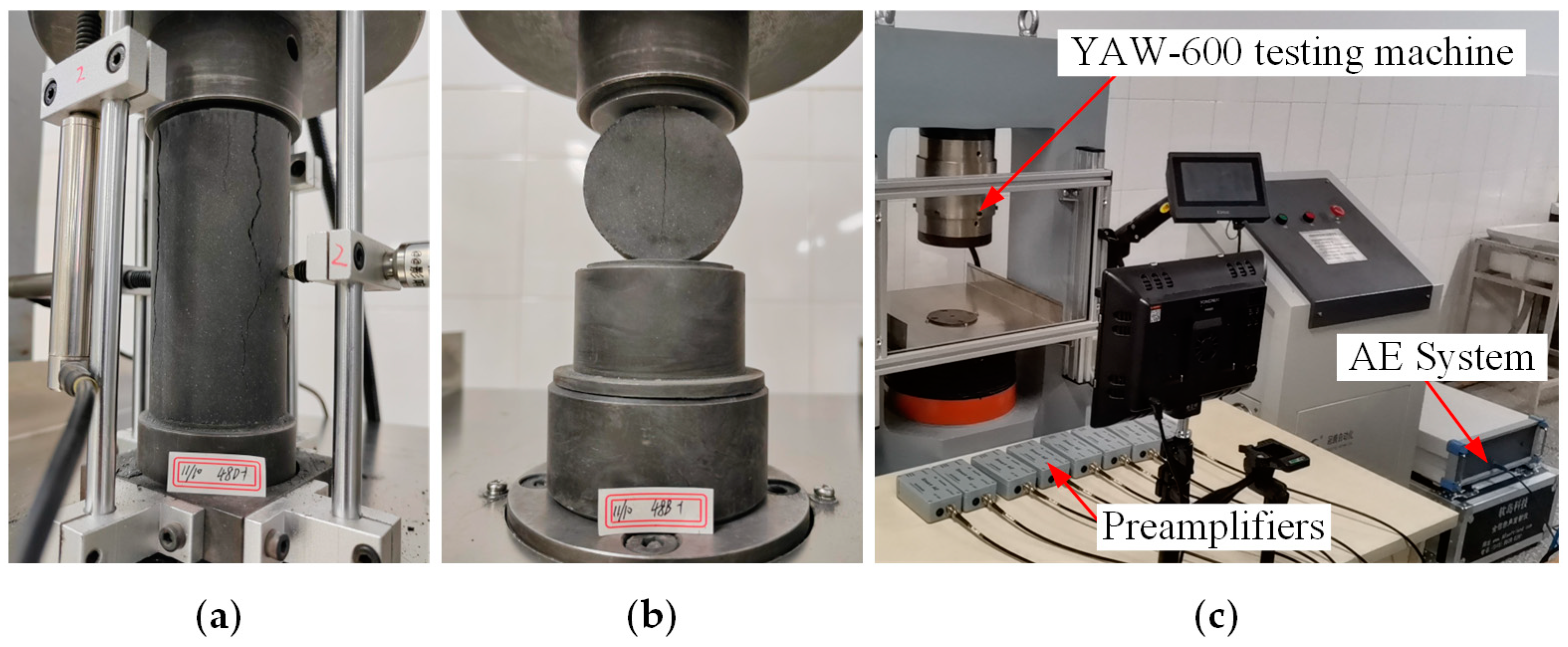
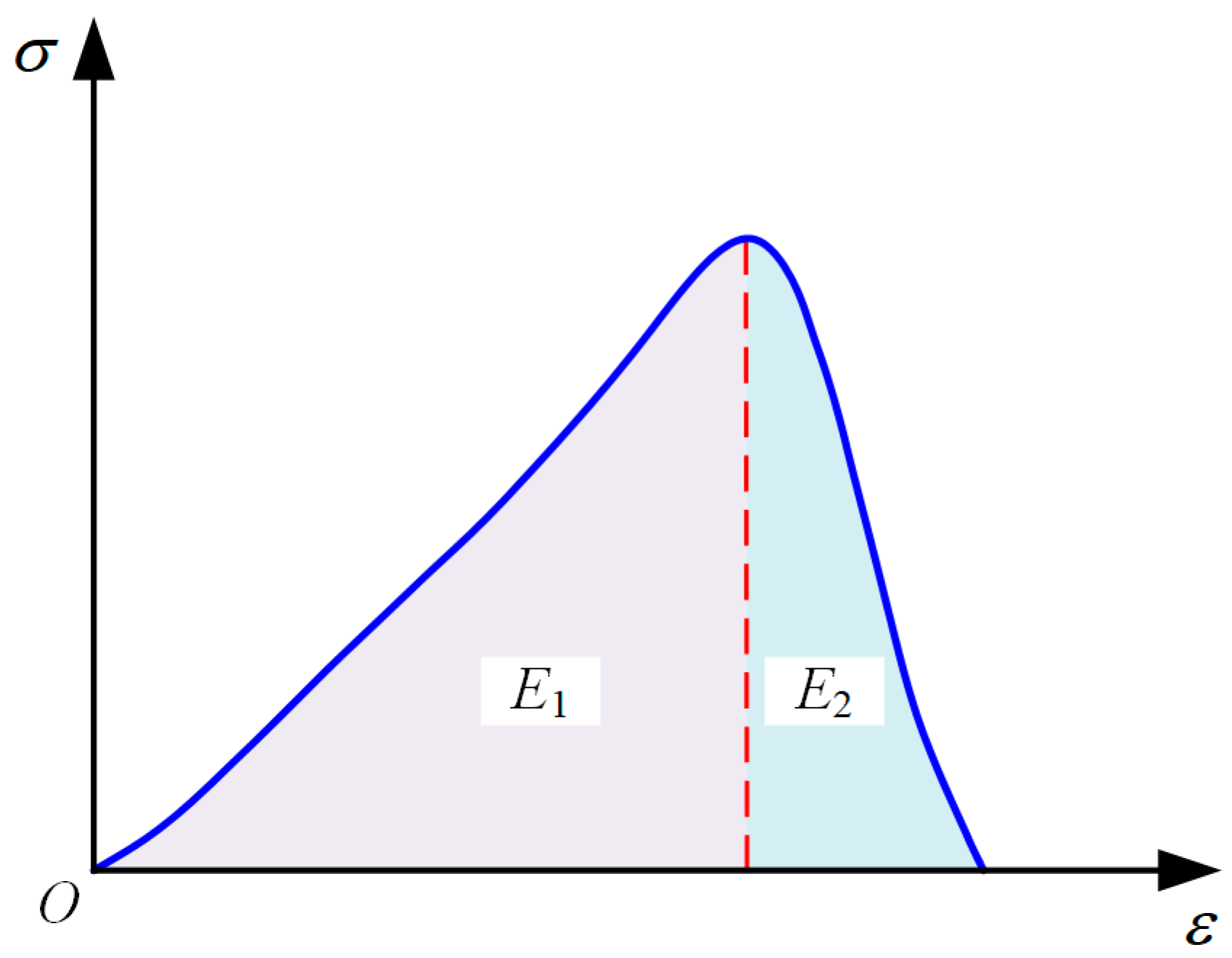
2.4. Testing Methods and Evaluation Indicators
3. Experimental Results and Analysis
3.1. Physical and Mechanical Parameters of the Materials
3.2. Sensitivity Analysis of Factors
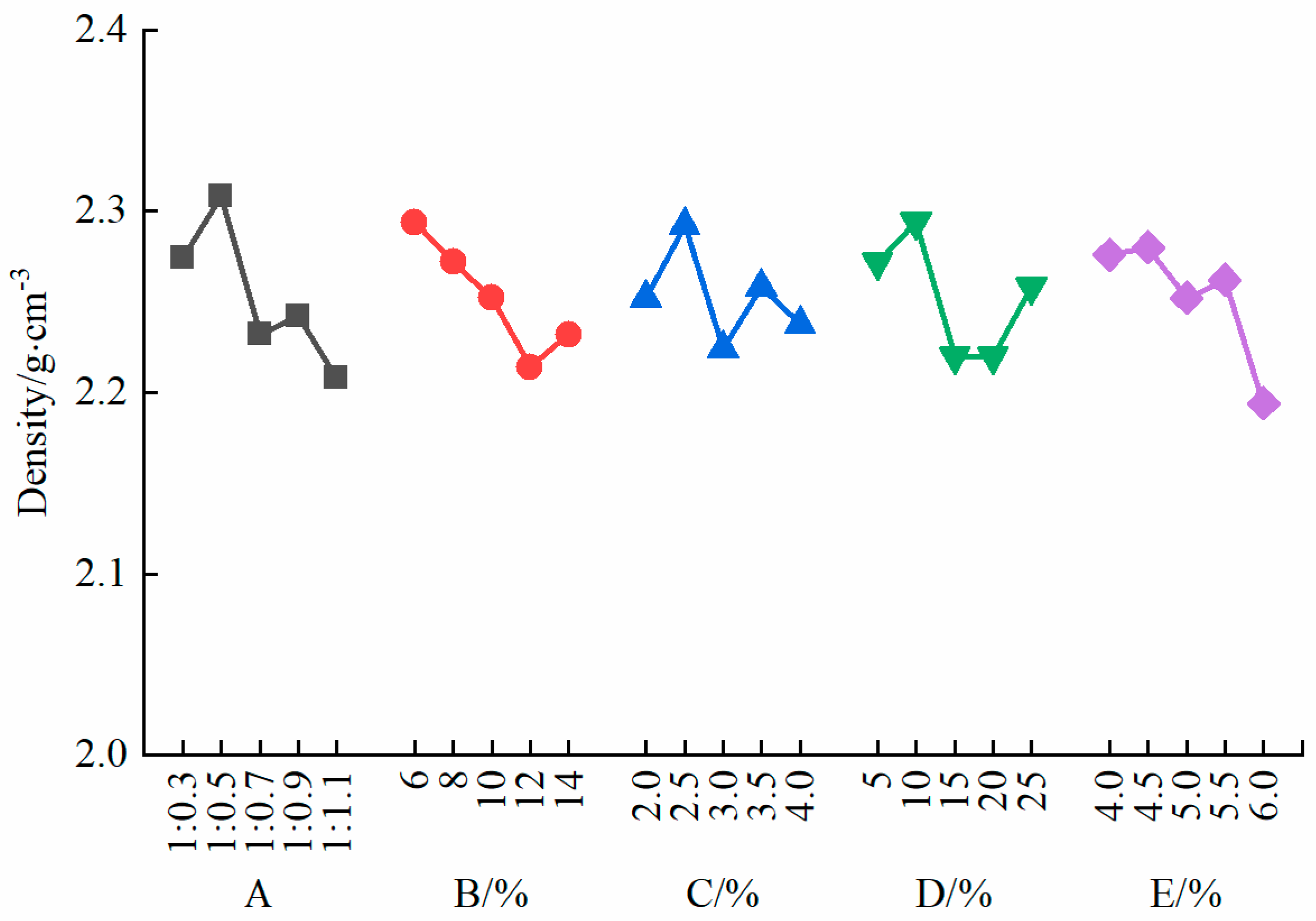
3.2.1. Sensitivity Analysis of Density
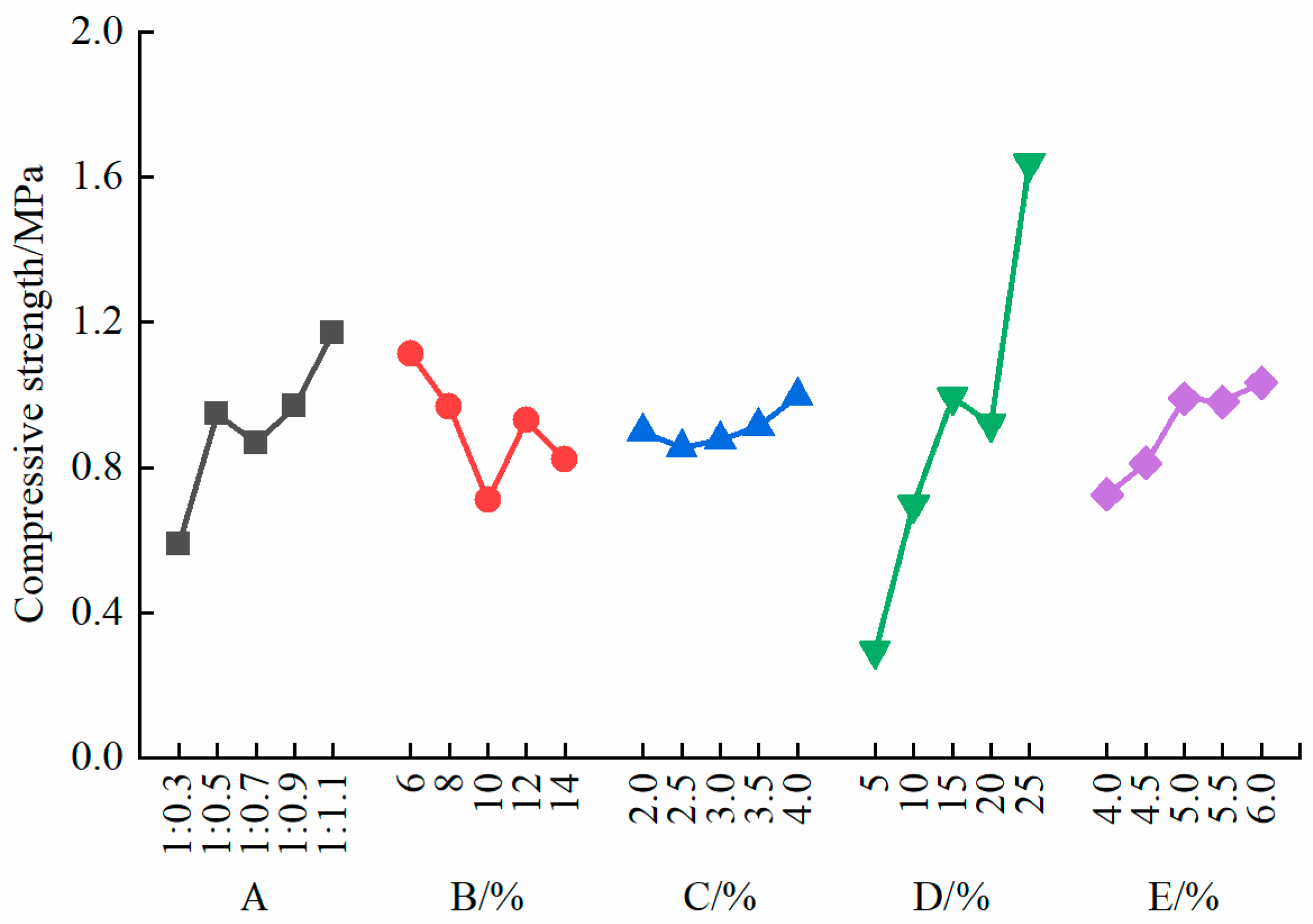
3.2.2. Sensitivity Analysis of Compressive Strength
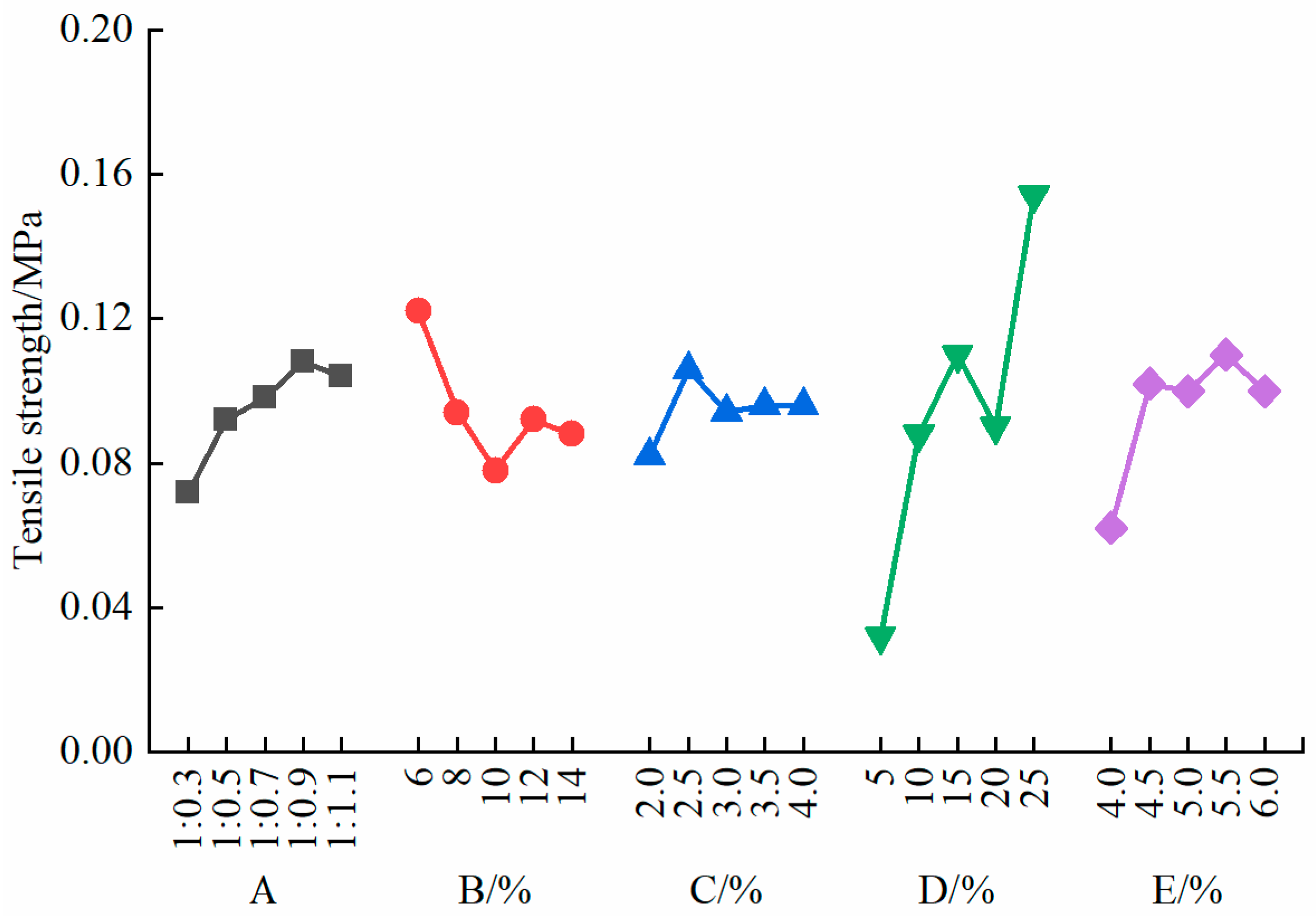
3.2.3. Sensitivity Analysis of Tensile Strength
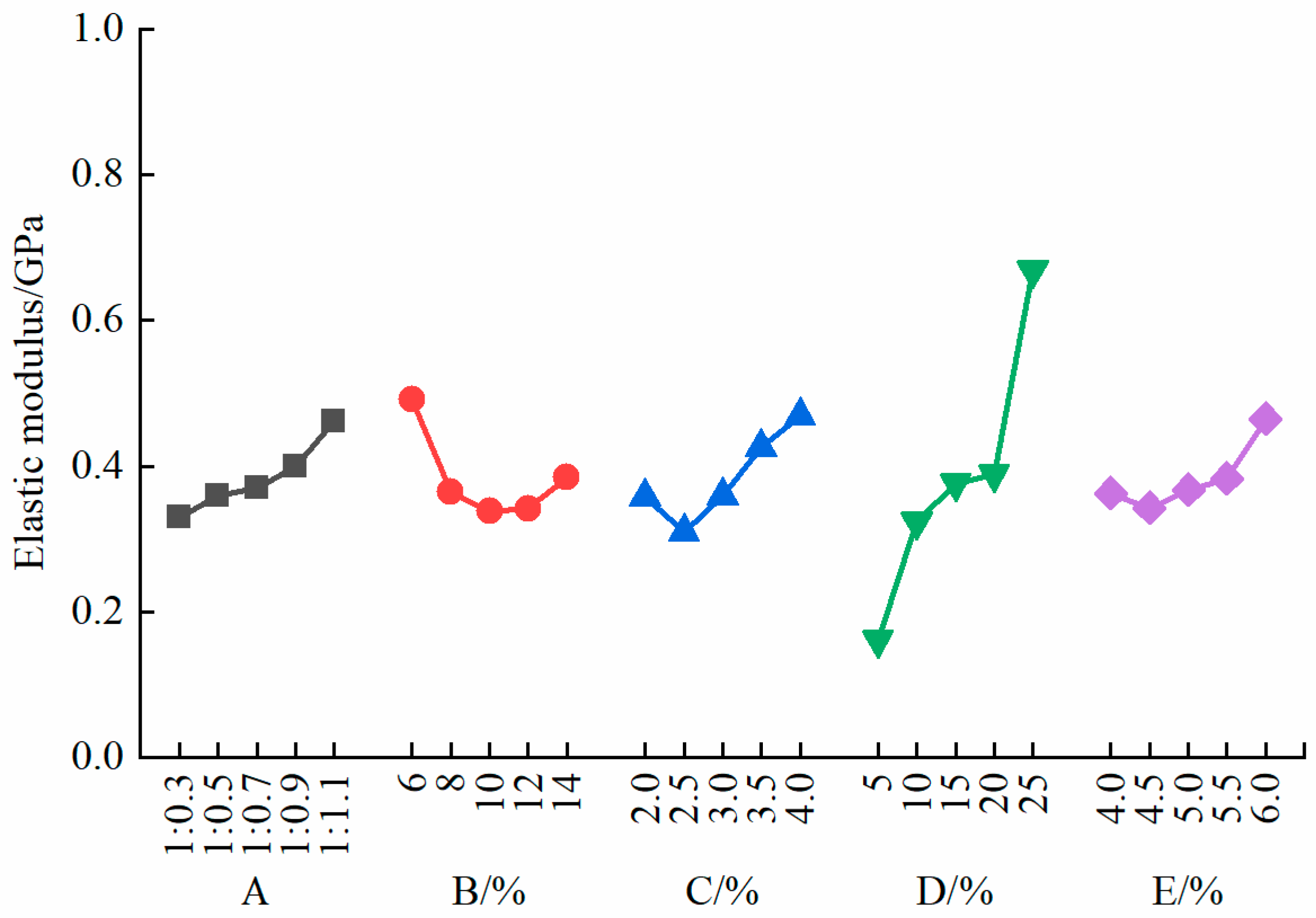
3.2.4. Sensitivity Analysis of the Elastic Modulus
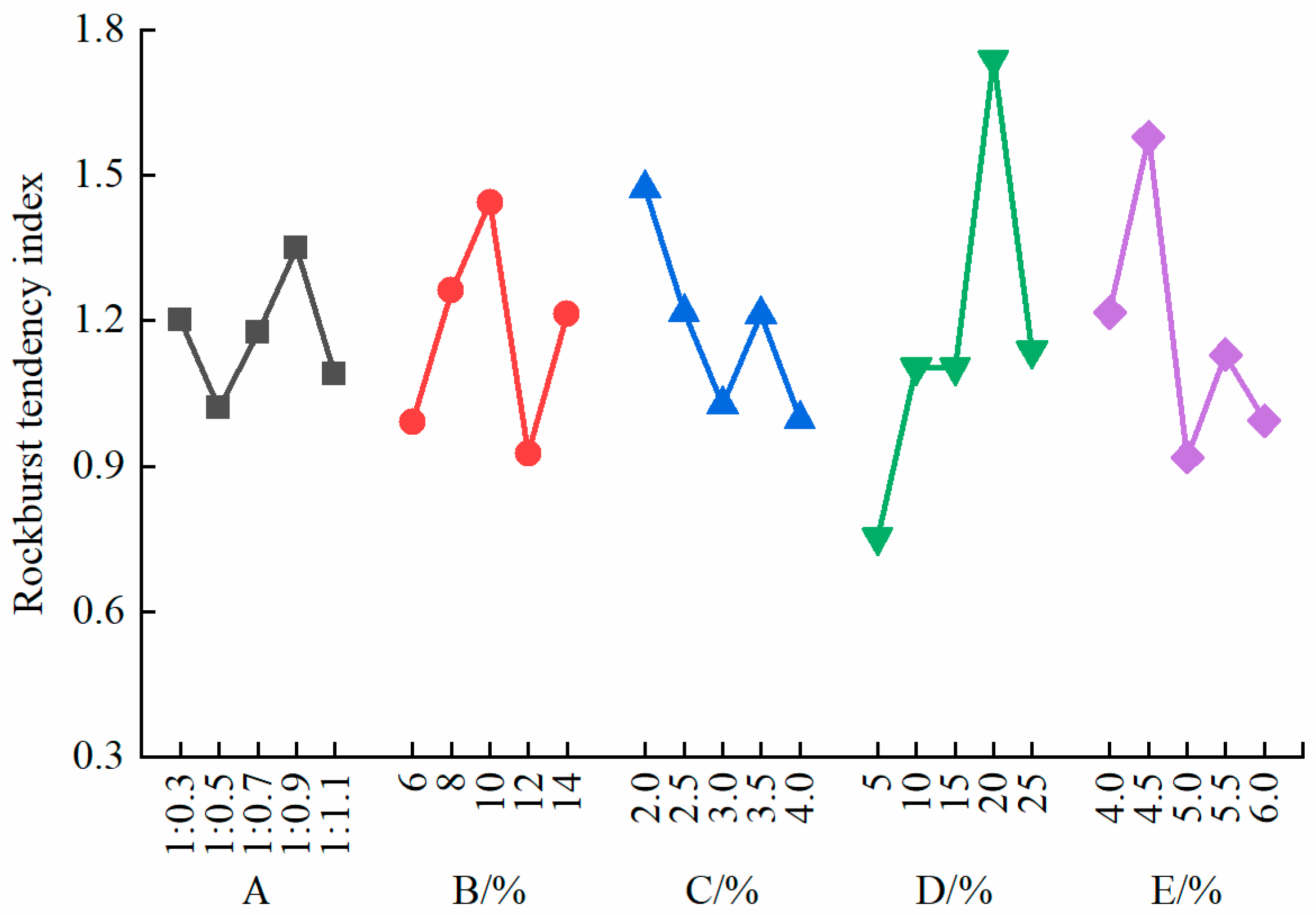
3.2.5. Sensitivity Analysis of the Rockburst Tendency Index
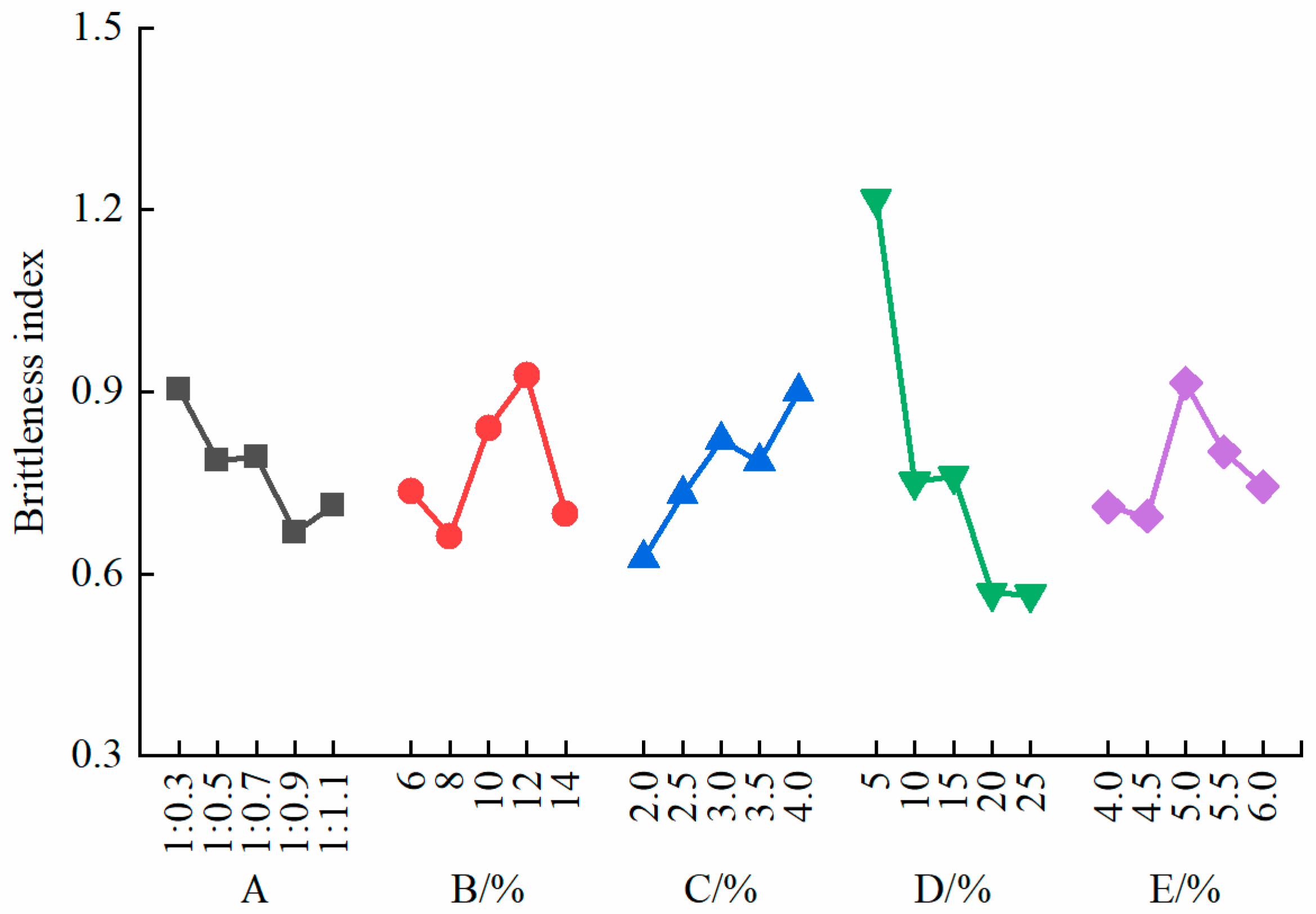
3.2.6. Sensitivity Analysis of the Brittleness Index
4. Multiple Linear Regression Analysis
5. Proportioning and Validation of Brittle Rock Analogs
5.1. Jinping Marble Analogous Material
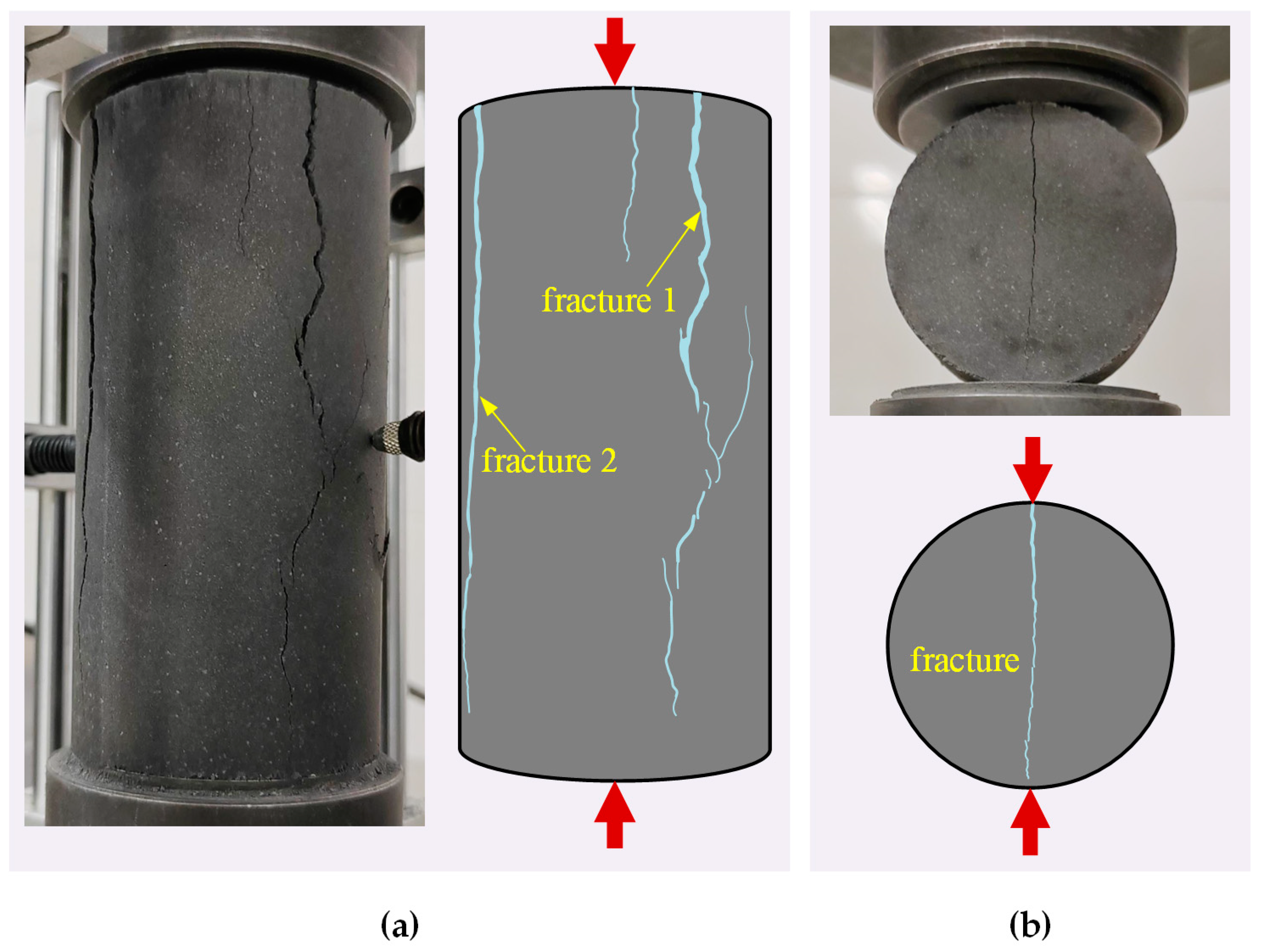
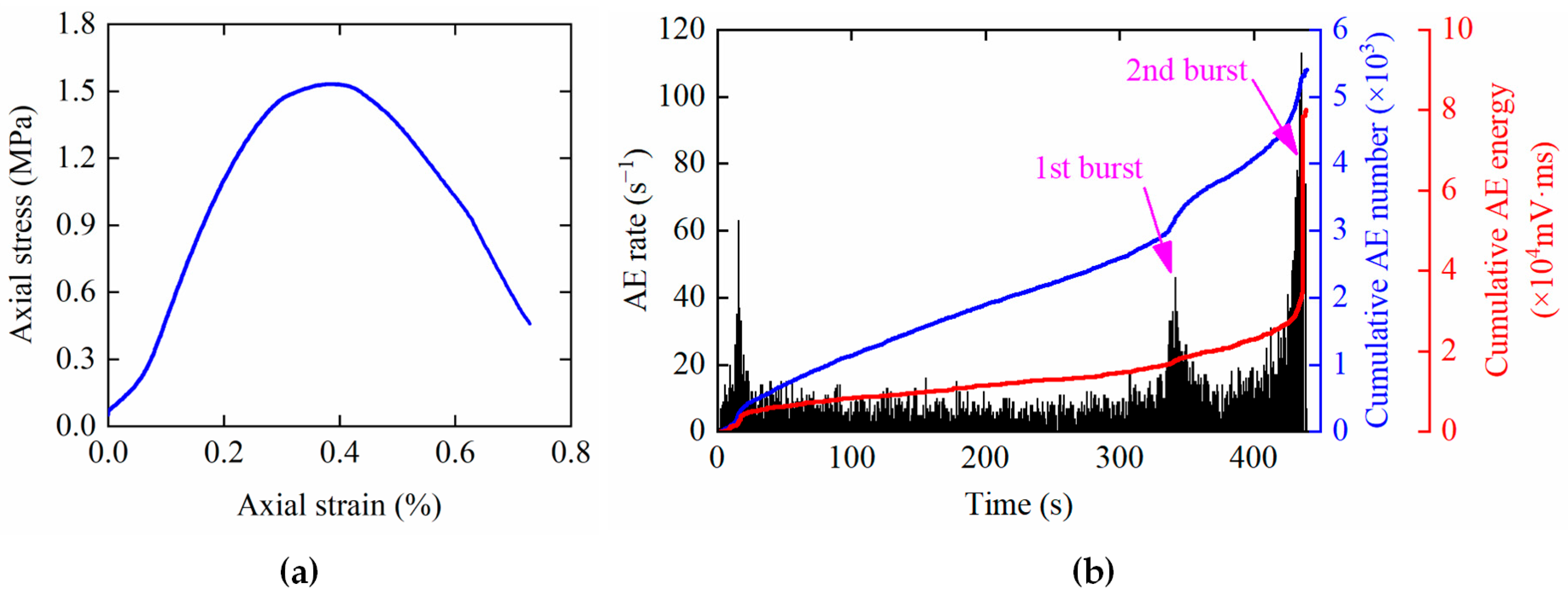
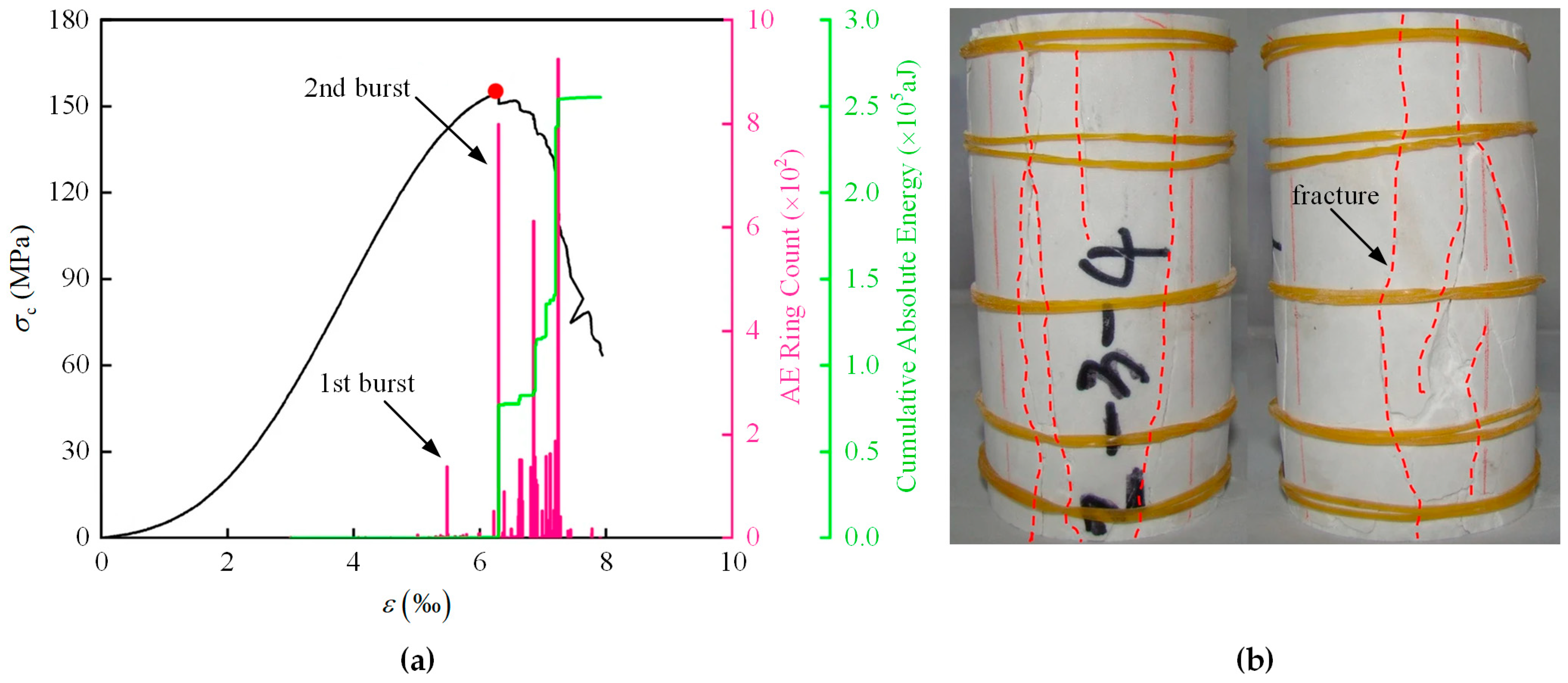
5.2. Failure Modes and Acoustic Emission Characteristics
6. Conclusions
- (1)
- In the analogous material, the refined iron powder, barite powder, and quartz sand serve as aggregates; the rosin–alcohol solution acts as a binder; and the gypsum functions as a brittleness modifier. The orthogonal test results showed that the density of the specimen ranges from 2.10 to 2.40 g/cm3; the compressive strength ranges from 0.12 to 1.94 MPa; the tensile strength ranges from 0.01 to 0.20 MPa; the elastic modulus ranges from 0.120 to 0.772 GPa; the rockburst tendency index ranges from 0.59 to 3.29; and the brittleness index ranges from 0.141 to 1.578. The prepared material offers a wide range of parameter variability, rapid drying, stable performance, and a straightforward manufacturing process.
- (2)
- The sensitivity analysis indicated that the refined iron powder–barite powder ratio significantly impacts the density of the analogous material, while the concentration of the rosin–alcohol solution primarily controls the material’s compressive strength, tensile strength, elastic modulus, rockburst tendency index, and brittleness index. Therefore, when preparing the brittle rock analog, it is more effective to appropriately adjust the iron powder–barite powder ratio and the concentration of the rosin–alcohol solution.
- (3)
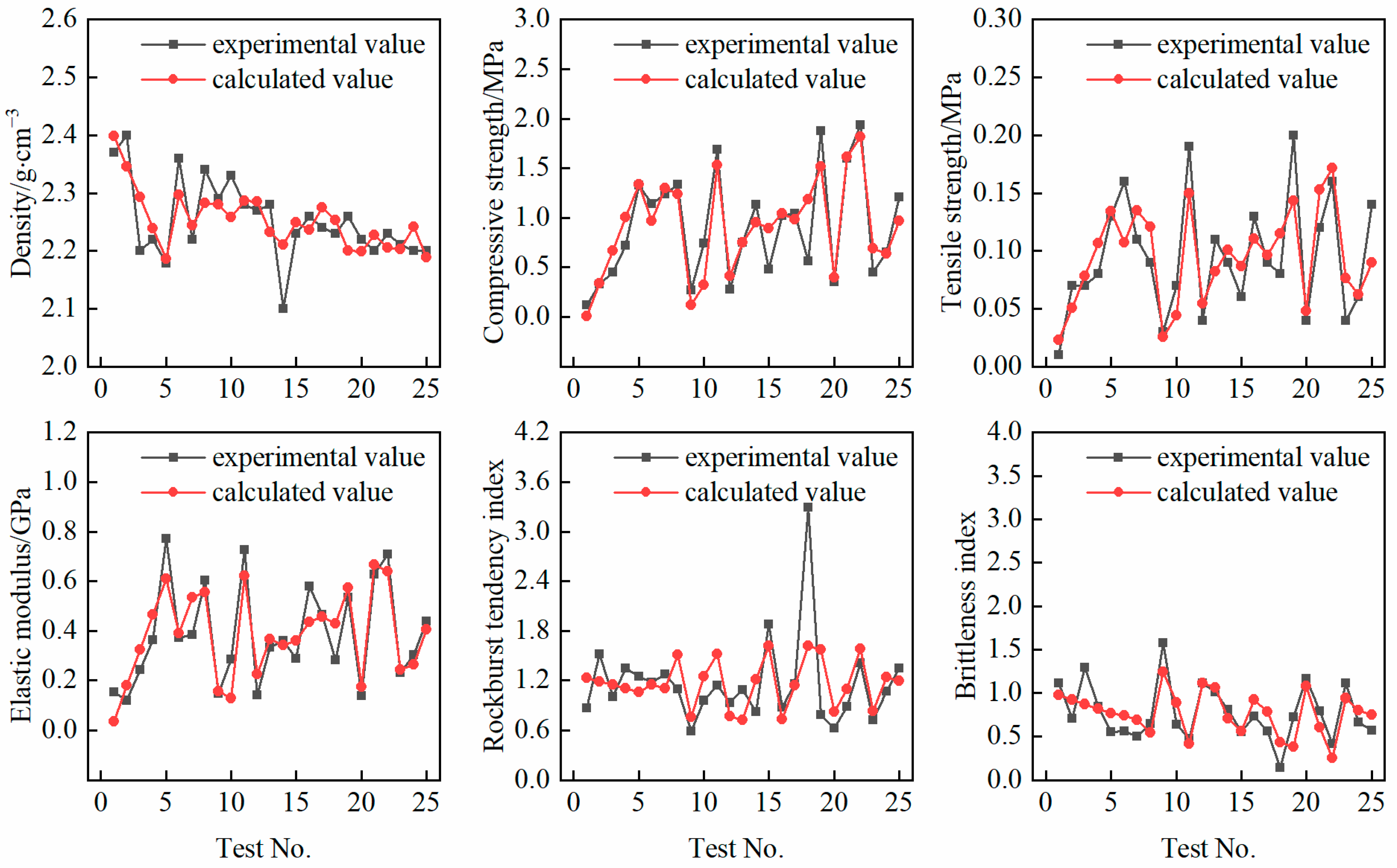
- The multiple linear regression equation effectively simulates and accurately predicts the physical and mechanical parameters of the specimen, improving the efficiency of material proportion and preparation. The equation provides a scientific basis and theoretical support for the rapid formulation of rock analogs.
- (4)
- An analogous material was prepared for T2b marble, and mechanical tests and acoustic emission monitoring were conducted on the specimens. Under uniaxial compression and Brazilian splitting conditions, the fracture mode and acoustic emission characteristics of the specimens were found to closely resemble those of brittle rock. This also validated the feasibility of the preparation method for rock analogs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, C.; Feng, X.; Zhou, H.; Qiu, S.; Wu, W. Case histories of four extremely intense rockbursts in deep tunnels. Rock Mech. Rock Eng. 2012, 45, 275–288. [Google Scholar] [CrossRef]
- Keneti, A.; Sainsbury, B. Review of published rockburst events and their contributing factors. Eng. Geol. 2018, 246, 361–373. [Google Scholar] [CrossRef]
- Gong, F.; Dai, J.; Xu, L. A strength-stress coupling criterion for rockburst: Inspirations from 1114 rockburst cases in 197 underground rock projects. Tunn. Undergr. Space Technol. 2023, 142, 105396. [Google Scholar] [CrossRef]
- Ortlepp, W.D.; Stacey, T.R. Rockburst mechanisms in tunnels and shafts. Tunn. Undergr. Space Technol. 1994, 9, 59–65. [Google Scholar] [CrossRef]
- Qian, Q.H. Definition, mechanism, classification and quantitative forecast model for rockburst and pressure bump. Rock Soil Mech. 2014, 35, 1–6. [Google Scholar]
- Li, C.C.; Zhao, T.; Zhang, Y.; Wan, W. A study on the energy sources and the role of the surrounding rock mass in strain burst. Int. J. Rock Mech. Min. Sci. 2022, 154, 105114. [Google Scholar] [CrossRef]
- Cook, N.G.W. The basic mechanics of rockbursts. J. S. Afr. Inst. Min. Metall. 1963, 64, 71–81. [Google Scholar]
- Askaripour, M.; Saeidi, A.; Rouleau, A.; Mercier-Langevin, P. Rockburst in underground excavations: A review of mechanism, classification, and prediction methods. Undergr. Space 2022, 7, 577–607. [Google Scholar] [CrossRef]
- Li, T.; Ma, C.; Zhu, M.; Meng, L.; Chen, G. Geomechanical types and mechanical analyses of rockbursts. Eng. Geol. 2017, 222, 72–83. [Google Scholar] [CrossRef]
- Xiao, P.; Li, D.; Zhao, G.; Zhu, Q. Characteristics and mechanism of rockburst at five deep gold mines in Jiaodong Peninsula of China. Int. J. Rock Mech. Min. Sci. 2023, 171, 105574. [Google Scholar] [CrossRef]
- Ortlepp, W.D. Observation of mining-induced faults in an intact rock mass at depth. Int. J. Rock Mech. Min. Sci. 2000, 37, 423–436. [Google Scholar] [CrossRef]
- Stacey, T.R. Addressing the consequences of dynamic rock failure in underground excavations. Rock Mech. Rock Eng. 2016, 49, 4091–4101. [Google Scholar] [CrossRef]
- Mazaira, A.; Konicek, P. Intense rockburst impacts in deep underground construction and their prevention. Can. Geotech. J. 2015, 52, 1426–1439. [Google Scholar] [CrossRef]
- Chen, L.; Bai, S.; Yin, X.; Zhao, Y. Plane-strain model tests on rock-burst of horseshoe section caverns in hard and brittle rockmass. Chin. J. Geotech. Eng. 2008, 30, 1520–1526. [Google Scholar]
- Kusui, A.; Villaescusa, E.; Funatsu, T. Mechanical behaviour of scaled-down unsupported tunnel walls in hard rock under high stress. Tunn. Undergr. Space Technol. 2016, 60, 30–40. [Google Scholar] [CrossRef]
- Feng, X.; Li, Z.; Mei, S.; Tian, J.; Yang, C.; Yao, Z.; Gao, J. A novel large-scale three-dimensional physical model experimental system for deep underground engineering. Rock Mech. Rock Eng. 2023, 56, 8395–8413. [Google Scholar] [CrossRef]
- Burgert, W.; Lippmann, H. Models of translatory rock bursting in coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 285–294. [Google Scholar] [CrossRef]
- Pan, Y.; Zhang, M.; Wang, L.; Li, G. Study on rockburst by equivalent material simulation tests. Chin. J. Geotech. Eng. 1997, 19, 49–56. [Google Scholar]
- Li, T.; Wang, X.; Meng, L. Physical simulation study of similar materials for rockburst. Chin. J. Rock Mech. Eng. 2011, 30, 2610–2616. [Google Scholar]
- Xu, W.; Xu, Y.; Wang, Y.; Than, L.G.; Lee, P.K.K.; Tsui, Y. Experimental study on simulation materials of rockburst. Chin. J. Rock Mech. Eng. 2000, 19, 873–877. [Google Scholar]
- Chen, L.; Bai, S. Proportioning test study on similar material of rockburst tendency of brittle rockmass. Rock Soil Mech. 2006, 27, 1050–1054. [Google Scholar]
- Zhou, H.; Chen, J.; Zhang, C.; Zhu, Y.; Lu, J.; Jiang, Y. Experimental study of the rockburst model material with low-strength and high-brittleness. Rock Soil Mech. 2019, 40, 2039–2049. [Google Scholar]
- Wu, J.; Zhang, X.Y.; Yu, L.Y.; Zhang, L.W.; Wu, T. Rockburst mechanism of rock mass with structural planes in underground chamber excavation. Eng. Fail. Anal. 2022, 139, 106501. [Google Scholar] [CrossRef]
- Ma, C.; Zhu, Z.; Fang, Z.; Li, Z.; Liu, L. Optimal proportion of similar materials and rockburst tendency of white sandstone. Adv. Mater. Sci. Eng. 2021, 2021, 6590779. [Google Scholar] [CrossRef]
- Jiang, Y.Z.; Wang, R.H.; Tang, T.C.; Guo, J.L. Research on plane strain model test of rockburst of underground cavern in hard brittle rockmass. Appl. Mech. Mater. 2014, 501-504, 1810–1814. [Google Scholar] [CrossRef]
- Shi, Y.; Ye, Y.; Hu, N.; Jiao, Y.; Wang, X. Physical simulation test on surrounding rock deformation of roof rockburst in continuous tunneling roadway. Minerals 2021, 11, 1335. [Google Scholar] [CrossRef]
- Ma, F.; Li, Z.; Luo, G. NIOS model material and its use in geo-mechanical similarity model test. J. Hydroelectr. Eng. 2004, 23, 48–51. [Google Scholar]
- Zhang, Q.; Li, S.; Guo, X.; Li, Y.; Wang, H. Research and development of new typed cementitious geotechnical similar material for iron crystal sand and its application. Rock Soil Mech. 2008, 29, 2126–2130. [Google Scholar]
- Liu, R.; Zhang, Y.; Wen, C.; Tang, J. Study on the design and analysis methods of orthogonal experiment. Exp. Technol. Manag. 2010, 27, 52–55. [Google Scholar]
- Zhang, C.; Lu, J.; Chen, J.; Zhou, H.; Yang, F. Discussion on rock burst proneness indexes and their relation. Rock Soil Mech. 2017, 38, 1397–1404. [Google Scholar]
- Kidybiński, A. Bursting liability indices of coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 295–304. [Google Scholar] [CrossRef]
- Tan, Y.A. Discussion on energy impact index of rock-bursting rock. Hydrogeol. Eng. Geol. 1992, 19, 10–12. [Google Scholar]
- Xia, Y.J.; Li, L.C.; Tang, C.A.; Li, X.Y.; Ma, S.; Li, M. A new method to evaluate rock mass brittleness based on stress–strain curves of class I. Rock Mech. Rock Eng. 2017, 50, 1123–1139. [Google Scholar] [CrossRef]
- Mei, S.; Feng, X.; Li, Z.; Yang, C.; Gao, J. A novel 3D printing technology for synthetic hard rock and the fabrication of Jinping marble. Rock Mech. Rock Eng. 2022, 55, 7695–7714. [Google Scholar] [CrossRef]

| Level | A Refined Iron Powder–Barite Powder Ratio | B Quartz Sand (%) | C Gypsum Powder (%) | D Rosin–Alcohol Solution Concentration (%) | E Rosin–Alcohol Solution Dosage (%) |
|---|---|---|---|---|---|
| 1 | 1:0.3 | 6 | 2.0 | 5 | 4.0 |
| 2 | 1:0.5 | 8 | 2.5 | 10 | 4.5 |
| 3 | 1:0.7 | 10 | 3.0 | 15 | 5.0 |
| 4 | 1:0.9 | 12 | 3.5 | 20 | 5.5 |
| 5 | 1:1.1 | 14 | 4.0 | 25 | 6.0 |
| Test No. | A Refined Iron Powder–Barite Powder Ratio | B Quartz Sand (%) | C Gypsum Powder (%) | D Rosin–Alcohol Solution Concentration (%) | E Rosin–Alcohol Solution Dosage (%) |
|---|---|---|---|---|---|
| 1 | 1:0.3 | 6 | 2.0 | 5 | 4.0 |
| 2 | 1:0.3 | 8 | 2.5 | 10 | 4.5 |
| 3 | 1:0.3 | 10 | 3.0 | 15 | 5.0 |
| 4 | 1:0.3 | 12 | 3.5 | 20 | 5.5 |
| 5 | 1:0.3 | 14 | 4.0 | 25 | 6.0 |
| 6 | 1:0.5 | 6 | 2.5 | 15 | 5.5 |
| 7 | 1:0.5 | 8 | 3.0 | 20 | 6.0 |
| 8 | 1:0.5 | 10 | 3.5 | 25 | 4.0 |
| 9 | 1:0.5 | 12 | 4.0 | 5 | 4.5 |
| 10 | 1:0.5 | 14 | 2.0 | 10 | 5.0 |
| 11 | 1:0.7 | 6 | 3.0 | 25 | 4.5 |
| 12 | 1:0.7 | 8 | 3.5 | 5 | 5.0 |
| 13 | 1:0.7 | 10 | 4.0 | 10 | 5.5 |
| 14 | 1:0.7 | 12 | 2.0 | 15 | 6.0 |
| 15 | 1:0.7 | 14 | 2.5 | 20 | 4.0 |
| 16 | 1:0.9 | 6 | 3.5 | 10 | 6.0 |
| 17 | 1:0.9 | 8 | 4.0 | 15 | 4.0 |
| 18 | 1:0.9 | 10 | 2.0 | 20 | 4.5 |
| 19 | 1:0.9 | 12 | 2.5 | 25 | 5.0 |
| 20 | 1:0.9 | 14 | 3.0 | 5 | 5.5 |
| 21 | 1:1.1 | 6 | 4.0 | 20 | 5.0 |
| 22 | 1:1.1 | 8 | 2.0 | 25 | 5.5 |
| 23 | 1:1.1 | 10 | 2.5 | 5 | 6.0 |
| 24 | 1:1.1 | 12 | 3.0 | 10 | 4.0 |
| 25 | 1:1.1 | 14 | 3.5 | 15 | 4.5 |
| Test No. | ρ (g·cm−3) | σc (MPa) | σt (MPa) | E (GPa) | Wcf | Bi |
|---|---|---|---|---|---|---|
| 1 | 2.37 | 0.12 | 0.01 | 0.152 | 0.87 | 1.111 |
| 2 | 2.40 | 0.33 | 0.07 | 0.120 | 1.52 | 0.706 |
| 3 | 2.20 | 0.45 | 0.07 | 0.243 | 1.01 | 1.292 |
| 4 | 2.22 | 0.72 | 0.08 | 0.364 | 1.35 | 0.848 |
| 5 | 2.18 | 1.33 | 0.13 | 0.772 | 1.25 | 0.556 |
| 6 | 2.36 | 1.14 | 0.16 | 0.373 | 1.17 | 0.561 |
| 7 | 2.22 | 1.24 | 0.11 | 0.385 | 1.28 | 0.500 |
| 8 | 2.34 | 1.34 | 0.09 | 0.604 | 1.10 | 0.650 |
| 9 | 2.29 | 0.27 | 0.03 | 0.148 | 0.59 | 1.578 |
| 10 | 2.33 | 0.74 | 0.07 | 0.286 | 0.96 | 0.642 |
| 11 | 2.28 | 1.69 | 0.19 | 0.727 | 1.14 | 0.475 |
| 12 | 2.27 | 0.28 | 0.04 | 0.142 | 0.94 | 1.116 |
| 13 | 2.28 | 0.75 | 0.11 | 0.333 | 1.09 | 1.006 |
| 14 | 2.10 | 1.13 | 0.09 | 0.360 | 0.83 | 0.813 |
| 15 | 2.23 | 0.48 | 0.06 | 0.289 | 1.88 | 0.555 |
| 16 | 2.26 | 1.02 | 0.13 | 0.579 | 0.88 | 0.736 |
| 17 | 2.24 | 1.04 | 0.09 | 0.464 | 1.16 | 0.567 |
| 18 | 2.23 | 0.56 | 0.08 | 0.281 | 3.29 | 0.141 |
| 19 | 2.26 | 1.88 | 0.20 | 0.536 | 0.79 | 0.727 |
| 20 | 2.22 | 0.35 | 0.04 | 0.137 | 0.63 | 1.168 |
| 21 | 2.20 | 1.60 | 0.12 | 0.628 | 0.89 | 0.791 |
| 22 | 2.23 | 1.94 | 0.16 | 0.708 | 1.41 | 0.422 |
| 23 | 2.21 | 0.45 | 0.04 | 0.230 | 0.73 | 1.113 |
| 24 | 2.20 | 0.65 | 0.06 | 0.304 | 1.07 | 0.666 |
| 25 | 2.20 | 1.21 | 0.14 | 0.438 | 1.35 | 0.570 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 2.27 | 2.29 | 2.25 | 2.27 | 2.28 |
| Average value 2 | 2.31 | 2.27 | 2.29 | 2.29 | 2.28 |
| Average value 3 | 2.23 | 2.25 | 2.22 | 2.22 | 2.25 |
| Average value 4 | 2.24 | 2.21 | 2.26 | 2.22 | 2.26 |
| Average value 5 | 2.21 | 2.23 | 2.24 | 2.26 | 2.19 |
| Range | 0.10 | 0.08 | 0.07 | 0.07 | 0.09 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 0.59 | 1.11 | 0.90 | 0.29 | 0.73 |
| Average value 2 | 0.95 | 0.97 | 0.86 | 0.70 | 0.81 |
| Average value 3 | 0.87 | 0.71 | 0.88 | 0.99 | 0.99 |
| Average value 4 | 0.97 | 0.93 | 0.91 | 0.92 | 0.98 |
| Average value 5 | 1.17 | 0.82 | 1.00 | 1.64 | 1.03 |
| Range | 0.65 | 0.40 | 0.14 | 1.34 | 0.31 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 0.07 | 0.12 | 0.08 | 0.03 | 0.06 |
| Average value 2 | 0.09 | 0.09 | 0.11 | 0.09 | 0.10 |
| Average value 3 | 0.10 | 0.08 | 0.09 | 0.11 | 0.10 |
| Average value 4 | 0.11 | 0.09 | 0.10 | 0.09 | 0.11 |
| Average value 5 | 0.10 | 0.09 | 0.10 | 0.15 | 0.10 |
| Range | 0.04 | 0.04 | 0.03 | 0.12 | 0.05 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 0.330 | 0.492 | 0.357 | 0.162 | 0.363 |
| Average value 2 | 0.359 | 0.364 | 0.310 | 0.324 | 0.343 |
| Average value 3 | 0.370 | 0.338 | 0.359 | 0.376 | 0.367 |
| Average value 4 | 0.399 | 0.342 | 0.425 | 0.389 | 0.383 |
| Average value 5 | 0.462 | 0.384 | 0.469 | 0.669 | 0.465 |
| Range | 0.132 | 0.154 | 0.159 | 0.507 | 0.122 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 1.20 | 0.99 | 1.47 | 0.75 | 1.22 |
| Average value 2 | 1.02 | 1.26 | 1.22 | 1.10 | 1.58 |
| Average value 3 | 1.18 | 1.44 | 1.03 | 1.10 | 0.92 |
| Average value 4 | 1.35 | 0.93 | 1.21 | 1.74 | 1.13 |
| Average value 5 | 1.09 | 1.21 | 1.00 | 1.14 | 0.99 |
| Range | 0.33 | 0.51 | 0.47 | 0.99 | 0.66 |
| Average Value | A | B (%) | C (%) | D (%) | E (%) |
|---|---|---|---|---|---|
| Average value 1 | 0.903 | 0.735 | 0.626 | 1.217 | 0.710 |
| Average value 2 | 0.786 | 0.662 | 0.732 | 0.751 | 0.694 |
| Average value 3 | 0.793 | 0.840 | 0.820 | 0.761 | 0.914 |
| Average value 4 | 0.668 | 0.926 | 0.784 | 0.567 | 0.801 |
| Average value 5 | 0.712 | 0.698 | 0.900 | 0.566 | 0.744 |
| Range | 0.235 | 0.264 | 0.274 | 0.651 | 0.220 |
| Statistical Metrics | ρ | σc | σt | E | Wcf | Bi |
|---|---|---|---|---|---|---|
| R2 | 0.652 | 0.809 | 0.666 | 0.798 | 0.580 | 0.708 |
| Adjusted R2 | 0.623 | 0.758 | 0.579 | 0.745 | 0.523 | 0.682 |
| F | 7.124 | 16.073 | 7.590 | 15.015 | 5.240 | 9.220 |
| p-Value | <0.001 | <0.001 | <0.001 | <0.001 | 0.003 | <0.001 |
| Variable | p-Value for coefficient | |||||
| Constant | <0.001 | 0.045 | 0.029 | 0.043 | 0.036 | 0.022 |
| Barite powder–refined iron powder ratio | 0.014 | 0.005 | 0.032 | 0.030 | 0.058 | 0.031 |
| Quartz sand | 0.022 | 0.036 | 0.035 | 0.037 | 0.055 | 0.043 |
| Gypsum powder | 0.047 | 0.049 | 0.054 | 0.026 | 0.031 | 0.027 |
| Rosin–alcohol solution concentration | 0.035 | <0.001 | <0.001 | <0.001 | 0.021 | <0.001 |
| Rosin–alcohol solution | 0.022 | 0.024 | 0.027 | 0.039 | 0.037 | 0.046 |
| Unit Weight (kN/m3) | Uniaxial Compressive Strength (MPa) | Tensile Strength (MPa) | Elastic Modulus (GPa) | Cohesion (MPa) | Internal Friction Angle (°) | Poisson’s Ratio |
|---|---|---|---|---|---|---|
| 27.7 | 101 | 6.02 | 42.8 | 34.47 | 29.0 | 0.26 |
| Refined Iron Powder–Barite Powder Ratio | Quartz Sand (%) | Gypsum Powder (%) | Rosin–Alcohol Solution Concentration (%) | Rosin–Alcohol Solution Dosage (%) |
|---|---|---|---|---|
| 1:0.5 | 17 | 2 | 10 | 5.1 |
| Unit Weight (kN/m3) | Uniaxial Compressive Strength (MPa) | Tensile Strength (MPa) | Elastic Modulus (GPa) | Cohesion (MPa) | Internal Friction Angle (°) | Poisson’s Ratio | |
|---|---|---|---|---|---|---|---|
| Calculated value | 25.2 | 1.53 | 0.09 | 0.649 | 0.522 | 29.0 | 0.26 |
| Measured value | 24.2 | 1.53 | 0.10 | 0.659 | 0.428 | 29.0 | 0.26 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Chu, C.; Wu, S.; Pang, R.; Xia, Z. Experimental Study of Material Proportioning for Similar Modeling of Brittle Rocks. Appl. Sci. 2024, 14, 11694. https://doi.org/10.3390/app142411694
Zhang C, Chu C, Wu S, Pang R, Xia Z. Experimental Study of Material Proportioning for Similar Modeling of Brittle Rocks. Applied Sciences. 2024; 14(24):11694. https://doi.org/10.3390/app142411694
Chicago/Turabian StyleZhang, Chaojun, Chaoqun Chu, Shunchuan Wu, Rui Pang, and Zhiyuan Xia. 2024. "Experimental Study of Material Proportioning for Similar Modeling of Brittle Rocks" Applied Sciences 14, no. 24: 11694. https://doi.org/10.3390/app142411694
APA StyleZhang, C., Chu, C., Wu, S., Pang, R., & Xia, Z. (2024). Experimental Study of Material Proportioning for Similar Modeling of Brittle Rocks. Applied Sciences, 14(24), 11694. https://doi.org/10.3390/app142411694






