Interpretation of a 3D Magnetotellurics Model of the Aceh and Seulimeum Segments of the Sumatran Fault Zone
Abstract
1. Introduction
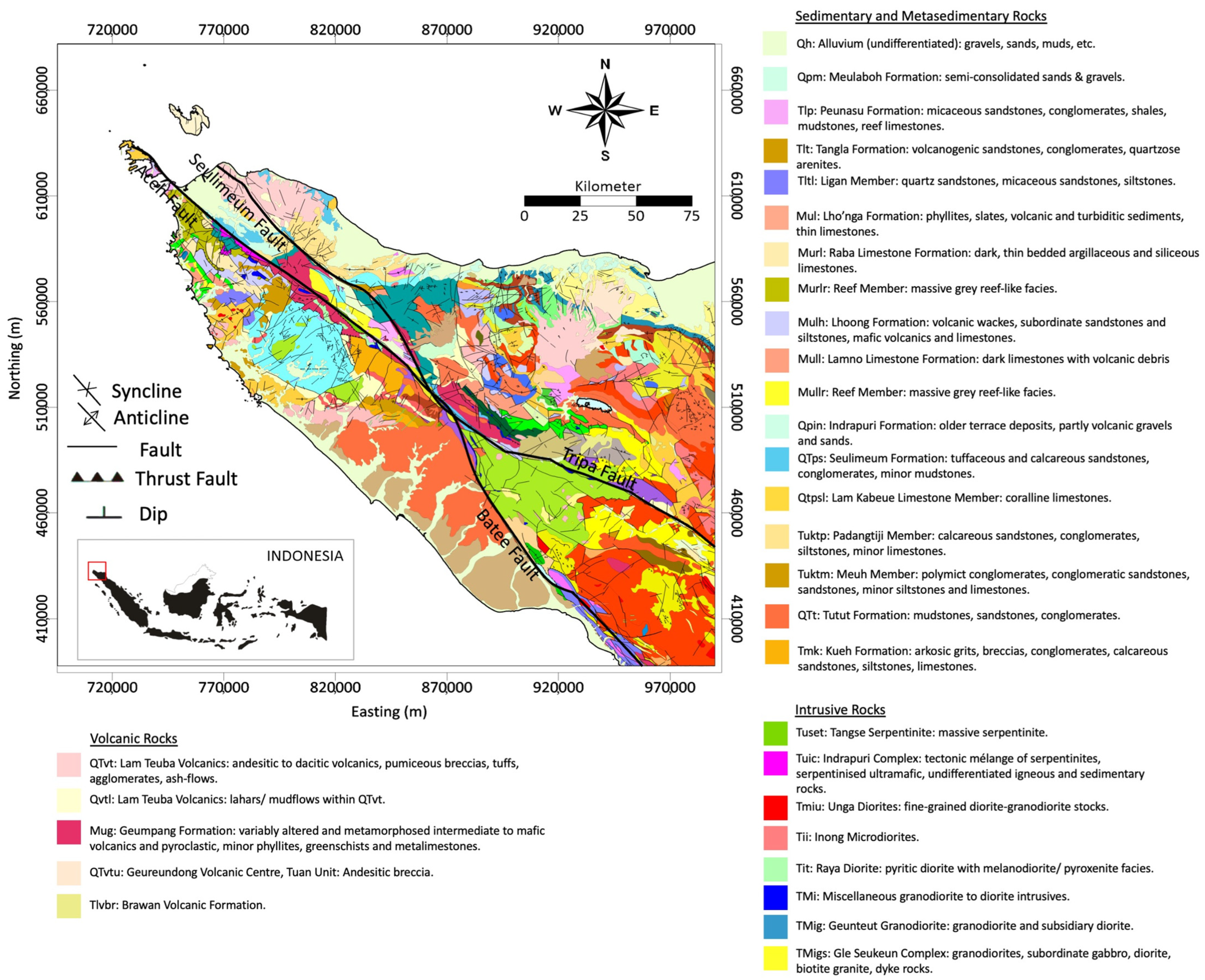
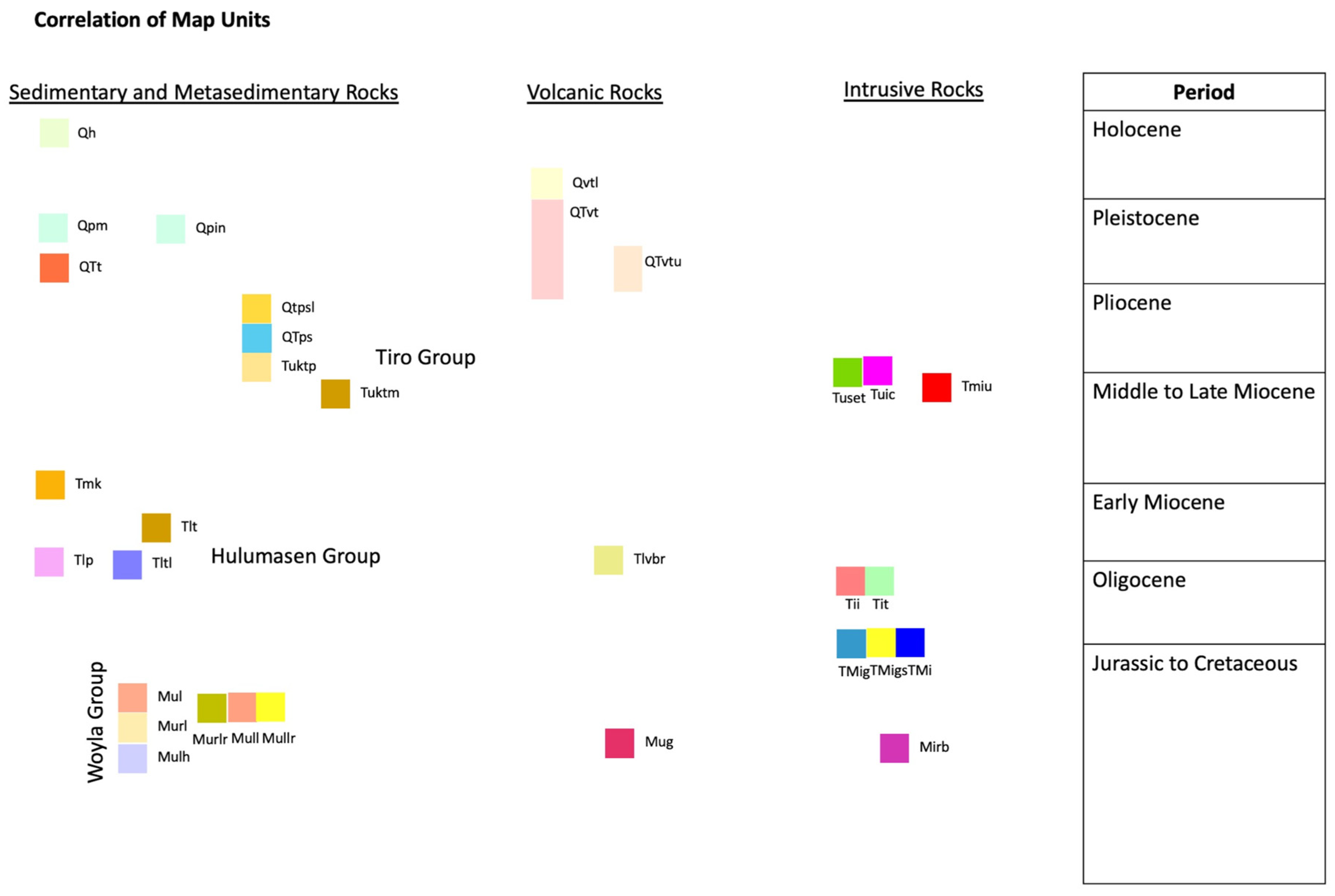
2. Geological Setting
3. MT Method
3.1. MT Method Theory
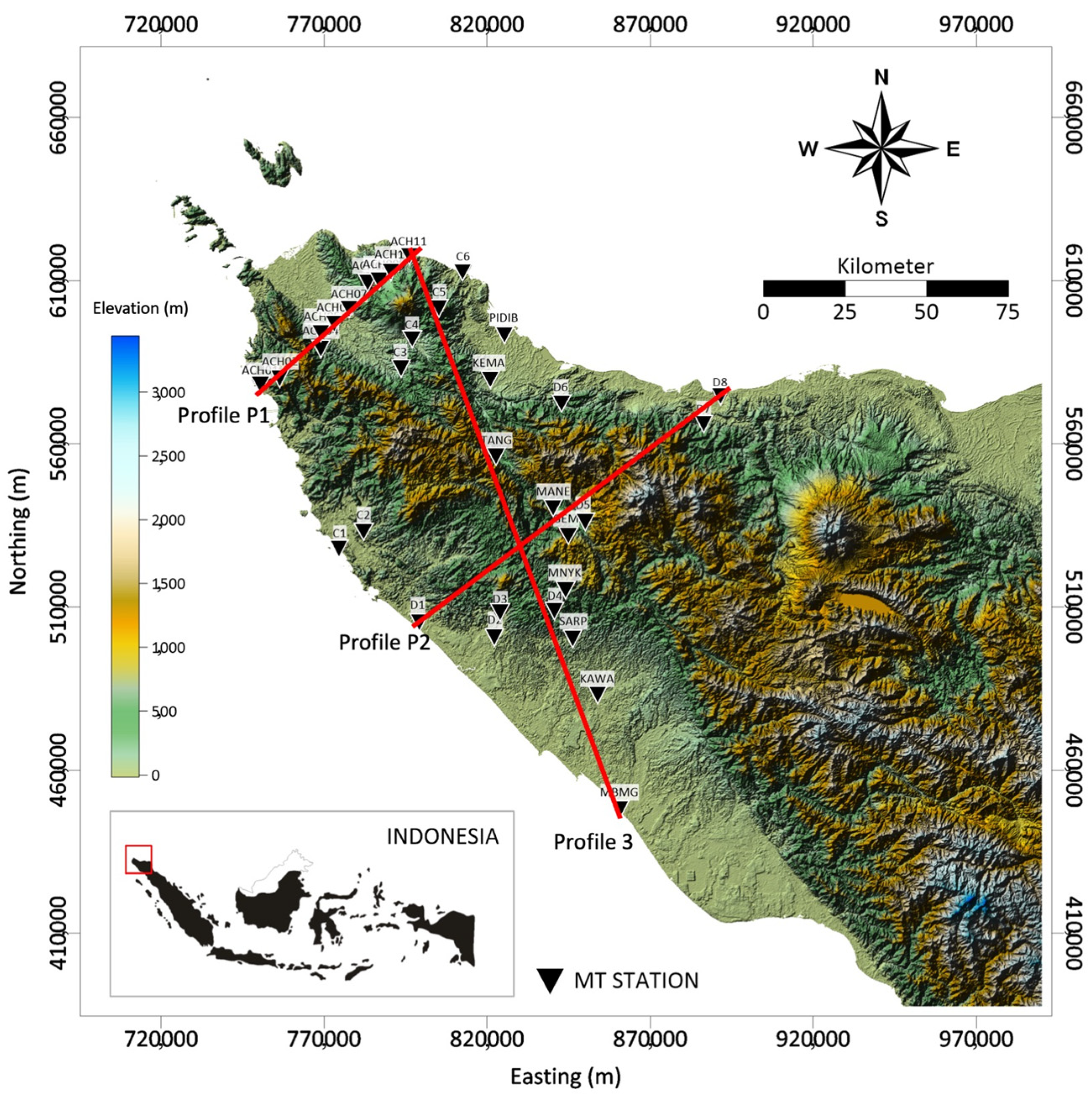
3.2. MT Data Acquisition and Processing
3.3. Dimensionality Analysis
3.4. Three-Dimensional MT Inversion
4. Results
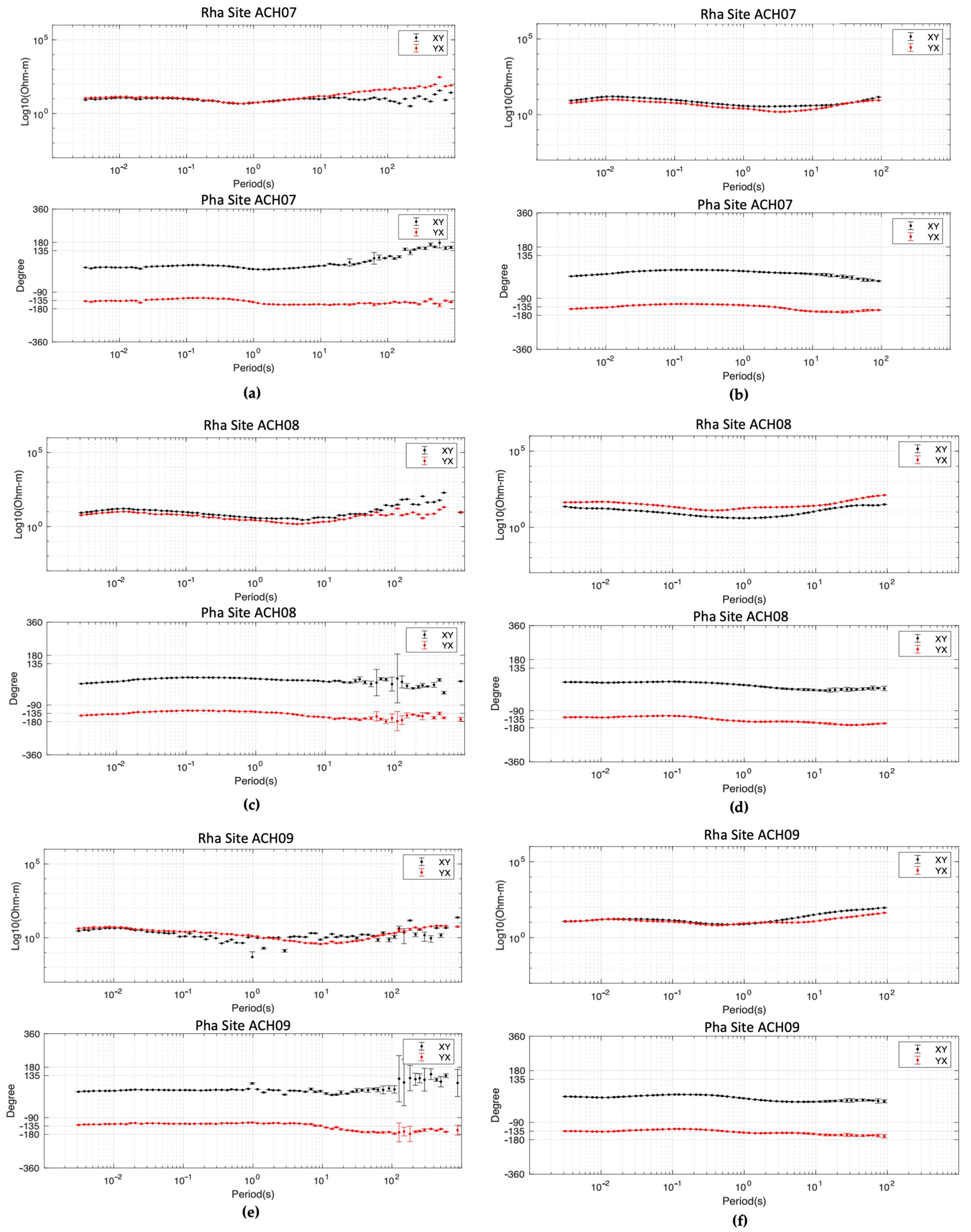
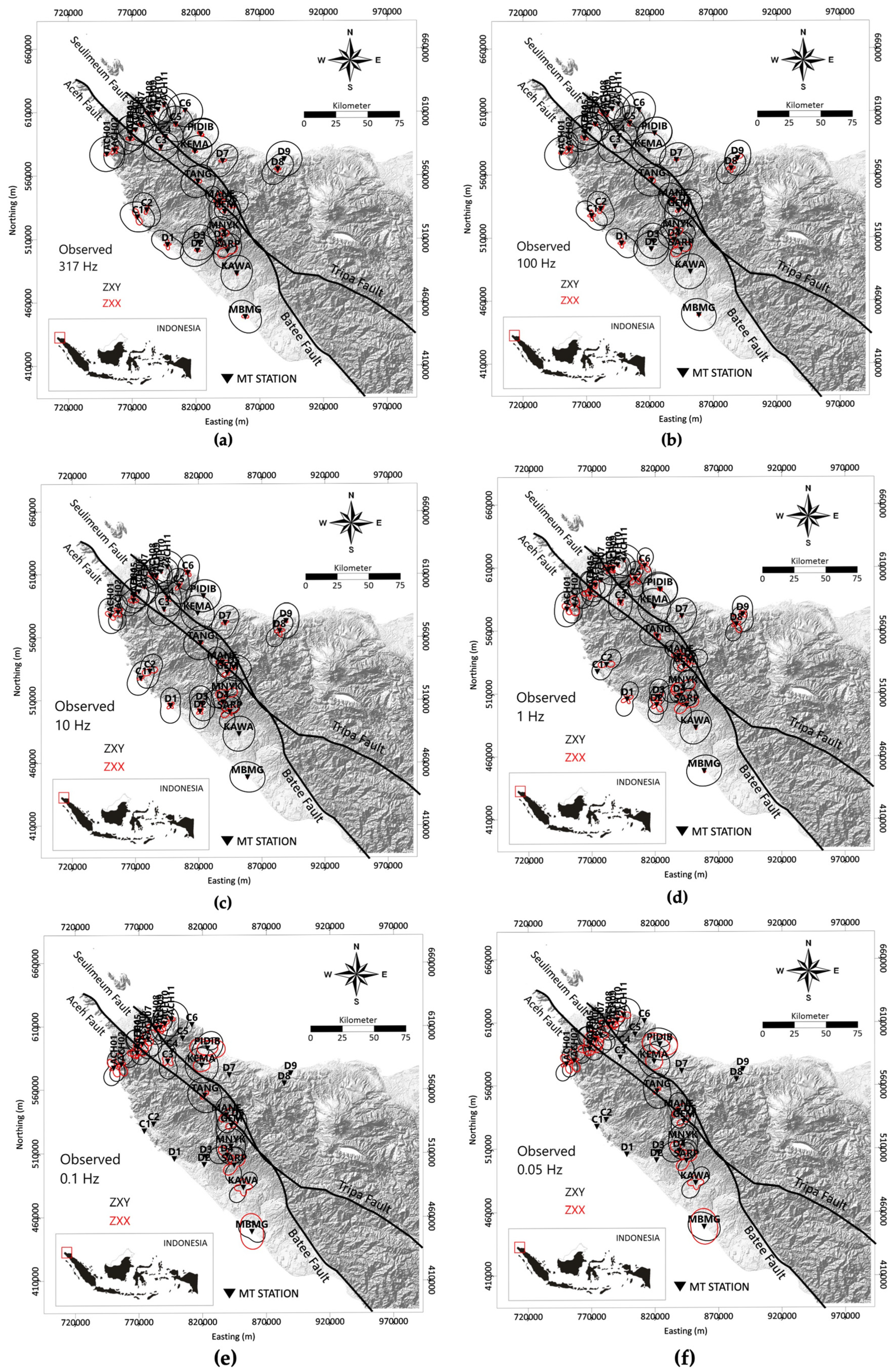
4.1. Dimensionality Analysis: Polar Diagram
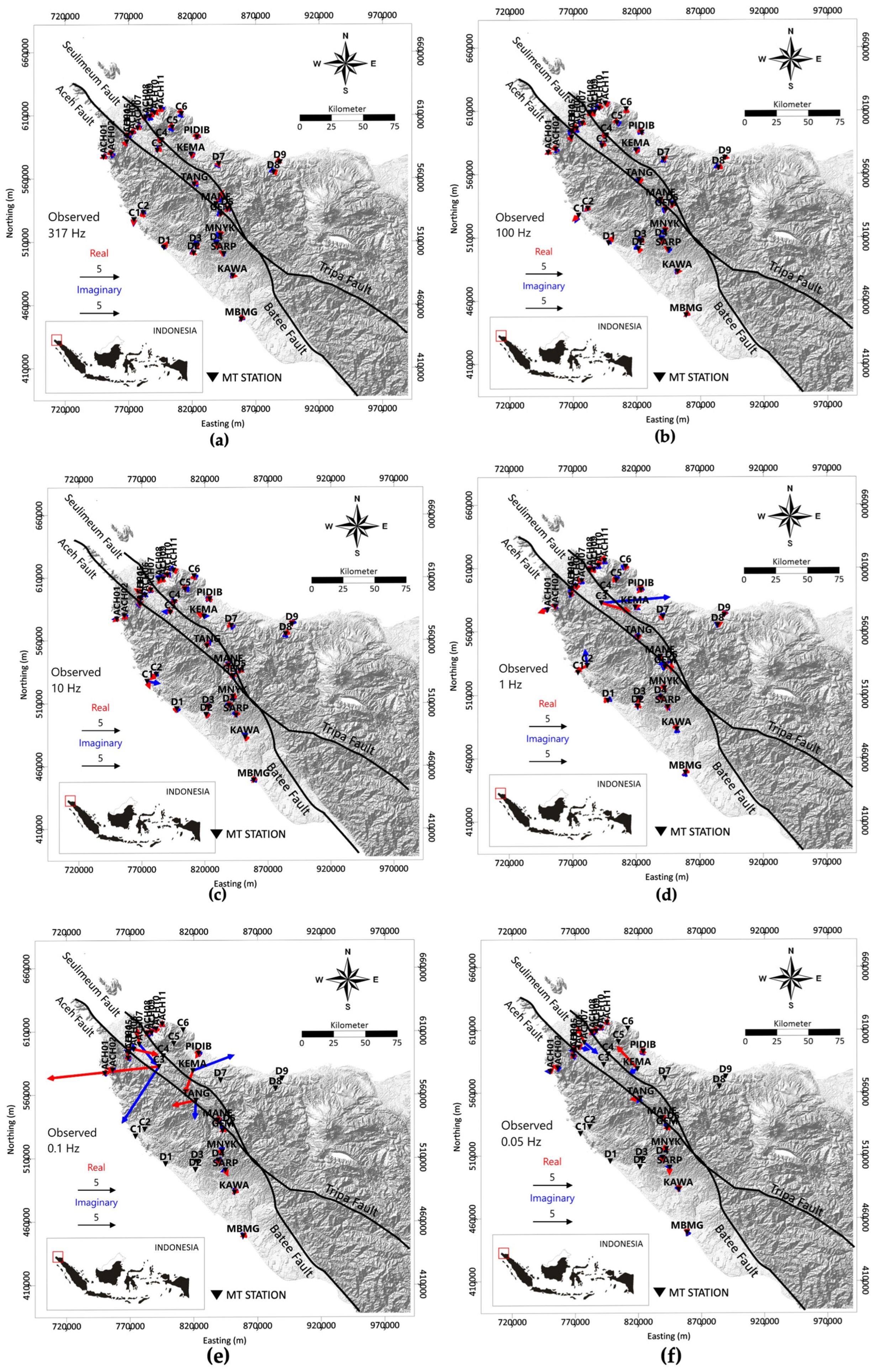
4.2. Dimensionality Analysis: Induction Arrows
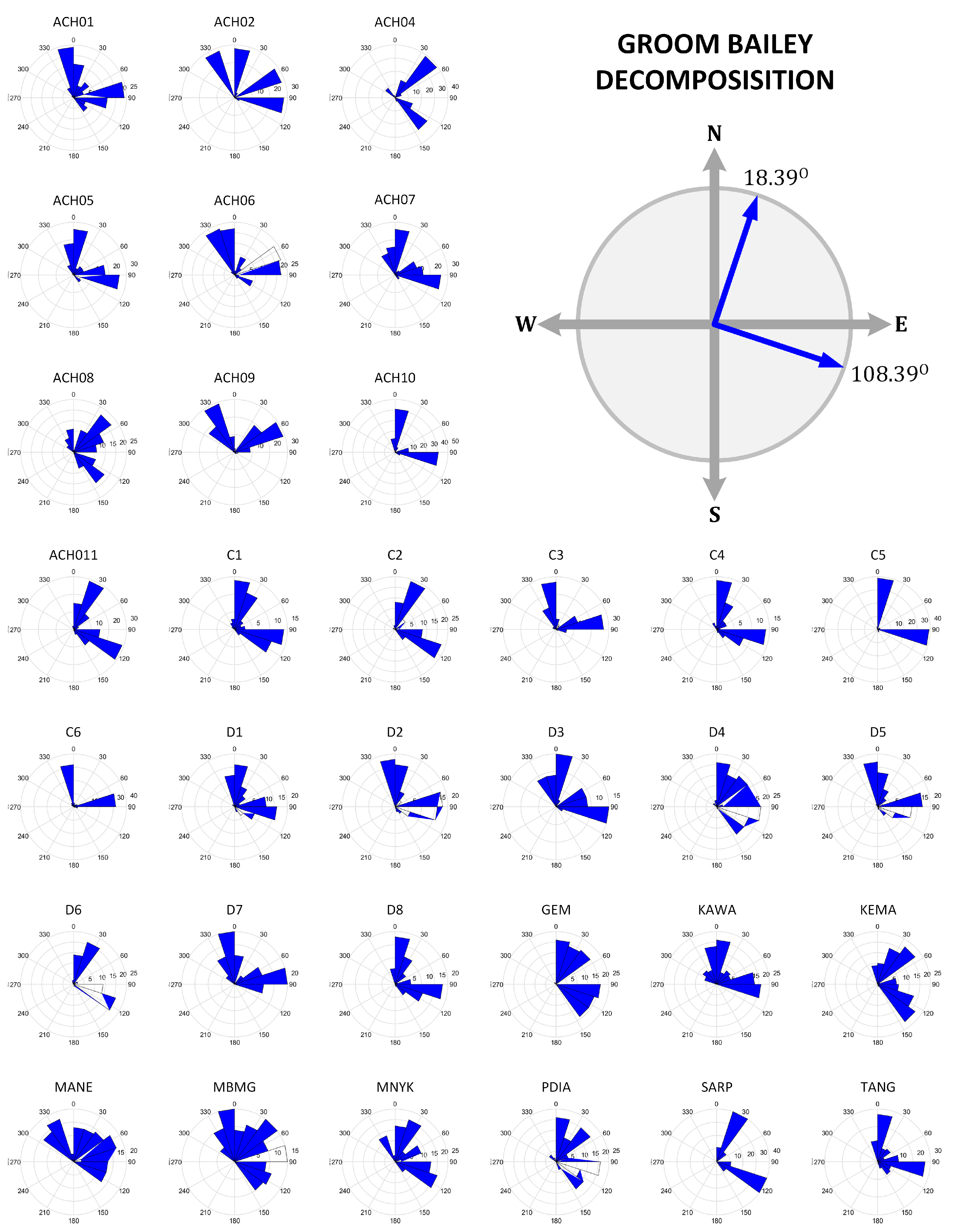
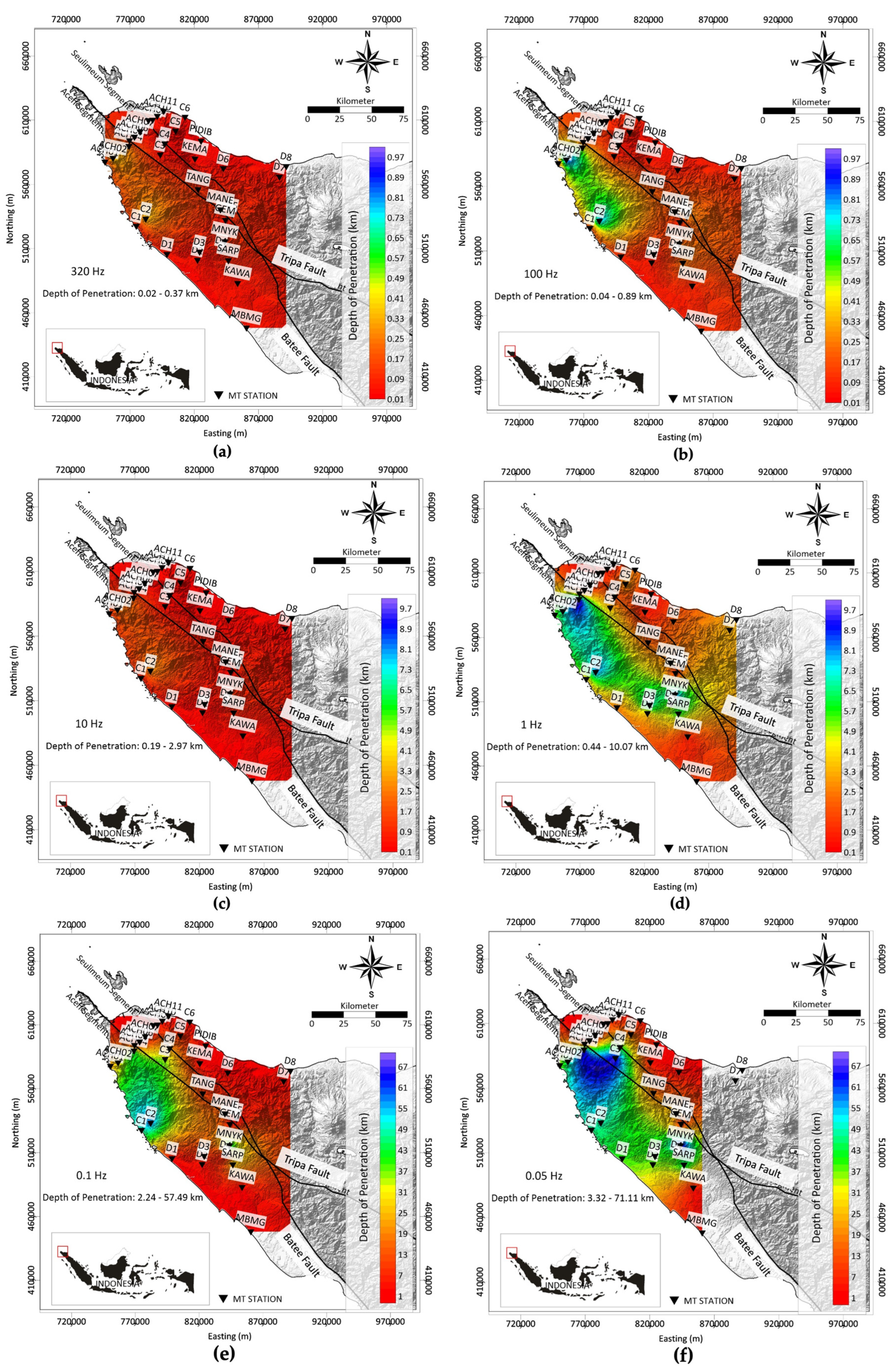
4.3. Groom–Bailey Decomposition and the Depth of Penetration (DOP)
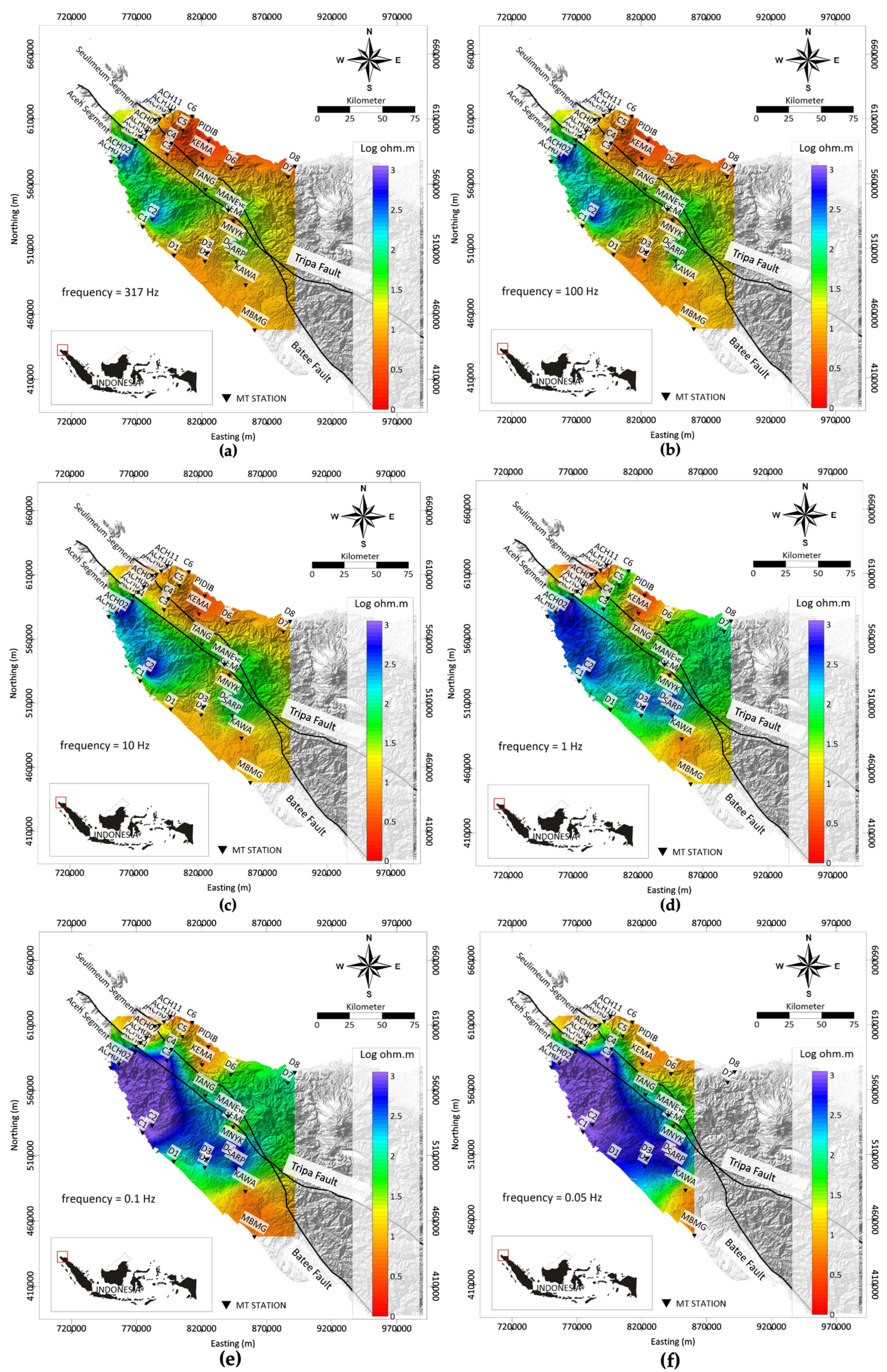
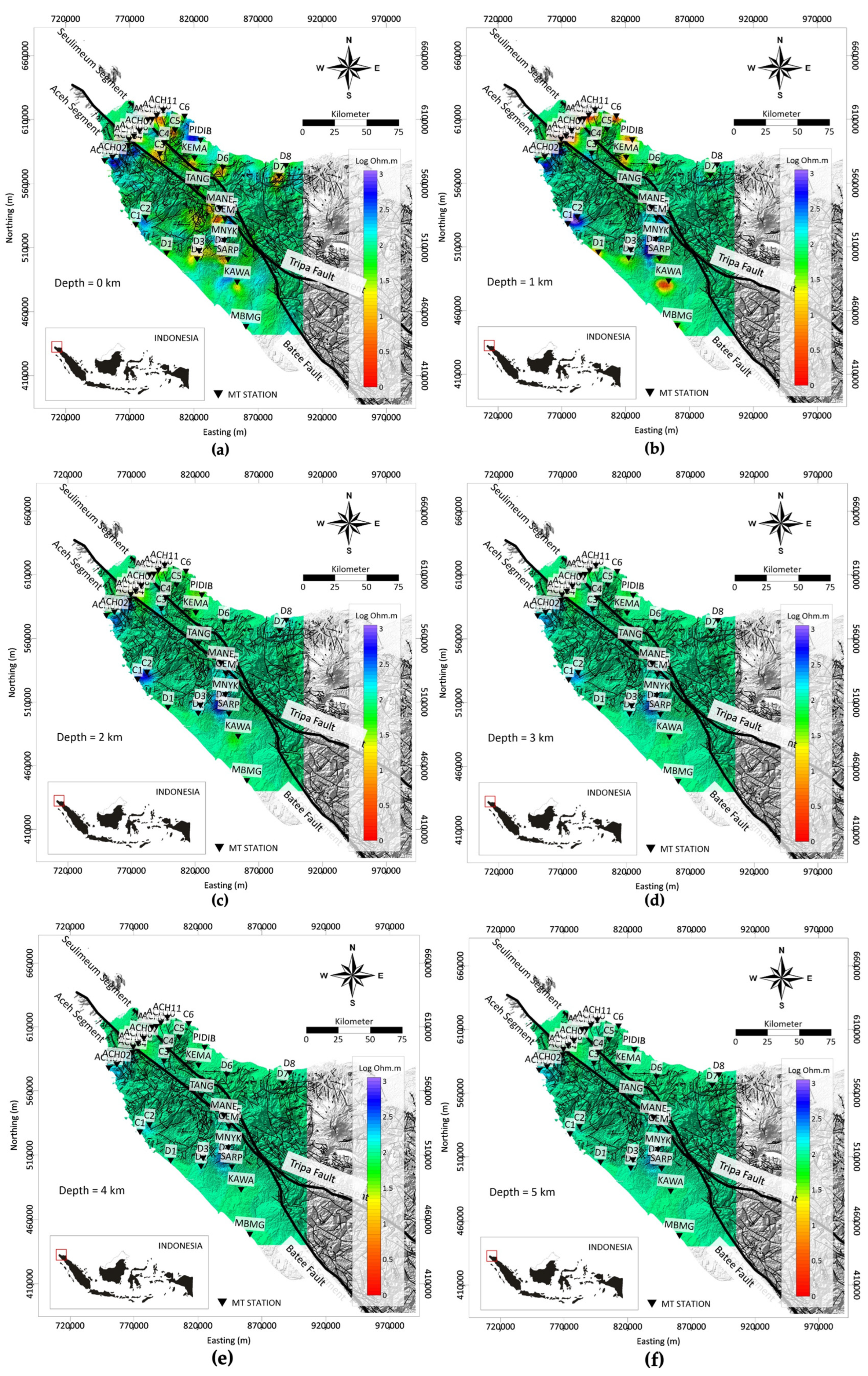
4.4. Three-Dimensional Inversion Model of MT Data
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- DeMets, C.; Gordon, R.G.; Argus, D.F. Geologically Current Plate Motions. Geophys. J. Int. 2010, 181, 1–80. [Google Scholar] [CrossRef]
- Fitch, T.J. Plate Convergence, Transcurrent Faults, and Internal Deformation Adjacent to Southeast Asia and the Western Pacific. J. Geophys. Res. 1972, 77, 4432–4460. [Google Scholar] [CrossRef]
- Hamilton, W. Tectonics of the Indonesian Region; U.S. Government Publishing Office: Washington, DC, USA, 1979.
- Genrich, J.F.; Bock, Y.; McCaffrey, R.; Prawirodirdjo, L.; Stevens, C.W.; Puntodewo, S.S.O.; Subarya, C.; Wdowinski, S. Distribution of Slip at the Northern Sumatran Fault System. J. Geophys. Res. Solid Earth 2000, 105, 28327–28341. [Google Scholar] [CrossRef]
- Curray, J.R. Tectonics and History of the Andaman Sea Region. J. Asian Earth Sci. 2005, 25, 187–232. [Google Scholar] [CrossRef]
- Fernández-Blanco, D.; Philippon, M.; von Hagke, C. Structure and Kinematics of the Sumatran Fault System in North Sumatra (Indonesia). Tectonophysics 2016, 693, 453–464. [Google Scholar] [CrossRef][Green Version]
- Sieh, K.; Natawidjaja, D. Neotectonics of the Sumatran Fault, Indonesia. J. Geophys. Res. Solid Earth 2000, 105, 28295–28326. [Google Scholar] [CrossRef]
- Febriansyah Putra, A.; Chenrai, P. Geomorphic Interpretation on the Formation of Strike-Slip Basins along the Northern Sumatran Fault. J. Asian Earth Sci. X 2023, 10, 100167. [Google Scholar] [CrossRef]
- Ito, T.; Gunawan, E.; Kimata, F.; Tabei, T.; Simons, M.; Meilano, I.; Agustan; Ohta, Y.; Nurdin, I.; Sugiyanto, D. Isolating Along-strike Variations in the Depth Extent of Shallow Creep and Fault Locking on the Northern Great Sumatran Fault. J. Geophys. Res. Solid Earth 2012, 117, B06409. [Google Scholar] [CrossRef]
- Natawidjaja, D.; Sieh, K. Slip Rate along the Sumatran Transcurrent Fault and Its Tectonic Significance. In Proceedings of the Conference on Tectonic Evolution of Southeast Asia, London, UK, 21–24 August 1994; Volume 7–8. [Google Scholar]
- Sieh, K.; Zachariasen, J.; Bock, Y.; Edwards, L.; Taylor, F.; Gans, P. Active Tectonics of Sumatra. Geol. Soc. Am. Abstr. Programs 1994, 26, 382. [Google Scholar]
- Bellier, O.; Sébrier, M. Is the Slip Rate Variation on the Great Sumatran Fault Accommodated by Fore-arc Stretching? Geophys. Res. Lett. 1995, 22, 1969–1972. [Google Scholar] [CrossRef]
- Bennet, J.D.; Brige, D.M.C.; Cameron, N.R.; Djunuddin, A.; Ghazali, S.A.; Jeffery, D.H.; Kartawa, W.; Keats, W.; Rock, N.M.S.; Thosmson, S.J.; et al. Geological Map of Banda Aceh Sheet, Scale 1: 250.000; Indonesian Geological Research and Development Center: Bandung, Indonesia, 1981. [Google Scholar]
- Hurukawa, N.; Wulandari, B.R.; Kasahara, M. Earthquake History of the Sumatran Fault, Indonesia, since 1892, Derived from Relocation of Large Earthquakes. Bull. Seismol. Soc. Am. 2014, 104, 1750–1762. [Google Scholar] [CrossRef]
- Cagniard, L. Basic theory of the magneto-telluric method of geophysical prospecting. Geophysics 1953, 18, 605–635. [Google Scholar] [CrossRef]
- Tikhonov, A.N. On Determining Electrical Characteristics of the Deep Layers of the Earth’s Crust. Doklady 1950, 73, 295–297. [Google Scholar]
- Simpson, F.; Bahr, K. Practical Magnetotellurics; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Berdichevsky, M.; Dmitriev, V. Models and Methods of Magnetotellurics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Zhang, L.; Unsworth, M.; Jin, S.; Wei, W.; Ye, G.; Jones, A.G.; Jing, J.; Dong, H.; Xie, C.; Le Pape, F.; et al. Structure of the Central Altyn Tagh Fault Revealed by Magnetotelluric Data: New Insights into the Structure of the Northern Margin of the India–Asia Collision. Earth Planet. Sci. Lett. 2015, 415, 67–79. [Google Scholar] [CrossRef]
- Oryński, S.; Jóźwiak, W.; Nowożyński, K. An Integrative 3-D Model of the Deep Lithospheric Structure beneath Dolsk and Odra Fault Zones as a Result of Magnetotelluric Data Interpretation. Geophys. J. Int. 2021, 227, 1917–1936. [Google Scholar] [CrossRef]
- He, M.; Xu, Y.; Fang, H.; Zhang, X. Deep Structure and Formation Model of the Southernmost Tanlu Fault Zone, Eastern China: Constrained from Magnetotelluric Imaging. J. Asian Earth Sci. 2023, 256, 105805. [Google Scholar] [CrossRef]
- Nurhasan; Ogawa, Y.; Kimata, F.; Sutarno, D.; Sugiyanto, D.; Ismail, N. Identification of Sumatran Fault Zone Using Magnetotelluric and Garvity Data. In Proceedings of the 13th SEGJ International Symposium, Tokyo, Japan, 12–14 November 2018; Society of Exploration Geophysicists and Society of Exploration Geophysicists of Japan: Tokyo, Japan, 2019; pp. 182–185. [Google Scholar]
- Ogawa, Y.; Uchida, T. A Two-Dimensional Magnetotelluric Inversion Assuming Gaussian Static Shift. Geophys. J. Int. 1996, 126, 69–76. [Google Scholar] [CrossRef]
- Sutarno, D.; Ogawa, Y.; Prihantoro, R.; Sugiyanto, D.; Ismail, N.; Kimata, F. Electrical Resistivity Imaging of Sumatran Fault Based on Magnetotelluric Data. In Proceedings of the 22nd EM Induction Workshop, Weimar, Germany, 24–30 August 2014. [Google Scholar]
- Yanis, M.; Abdullah, F.; Zaini, N.; Ismail, N. The Northernmost Part of the Great Sumatran Fault Map and Images Derived from Gravity Anomaly. Acta Geophys. 2021, 69, 795–807. [Google Scholar] [CrossRef]
- Syukri, M.; Saad, R. Seulimeum Segment Characteristic Indicated by 2-D Resistivity Imaging Method. NRIAG J. Astron. Geophys. 2017, 6, 210–217. [Google Scholar] [CrossRef][Green Version]
- Natawidjaja, D.H. Neotectonics of the Sumatran Fault and Paleogeodesy of the Sumatran Subduction Zone; California Institute of Technology: Pasadena, CA, USA, 2003. [Google Scholar]
- Barber, A.; Crow, M.; Milsom, J. Introduction and Previous Research. In Sumatra: Geology, Resources and Tectonic Evolution; Geological Society of London: London, UK, 2005. [Google Scholar]
- Singh, S.C.; Moeremans, R.; McArdle, J.; Johansen, K. Seismic Images of the Sliver Strike-slip Fault and Back Thrust in the Andaman-Nicobar Region. J. Geophys. Res. Solid. Earth 2013, 118, 5208–5224. [Google Scholar] [CrossRef]
- Fontaine, H.; Gafoer, S. The Pre-Tertiary Fossils of Sumatra and Their Environments; CCOP Technical Secretariat: Bangkok, Thailand, 1989. [Google Scholar]
- Cameron, N.R.; Bennett, J.D.; Bridge, D.M.; Clarke, M.C.G.; Djunuddin, A.; Ghazali, S.A.; Harahap, H.; Jeffery, D.H.; Kartawa, W.; Keats, W.; et al. Geological Map of Takengon Sheet, Sumatra, Scale 1: 250.000; Indonesian Geological Research and Development Center: Bandung, Indonesia, 1983. [Google Scholar]
- Hady, A.K.; Marliyani, G.I. Updated Segmentation Model of the Aceh Segment of the Great Sumatran Fault System in Northern Sumatra, Indonesia. J. Appl. Geol. 2020, 5, 84–100. [Google Scholar] [CrossRef]
- Natawidjaja, D.H.; Triyoso, W. The Sumatran Fault Zone—From Source to Hazard. J. Earthq. Tsunami 2007, 1, 21–47. [Google Scholar] [CrossRef]
- Curray, J.R.; Moore, D.G.; Lawver, L.A.; Emmel, F.J.; Raitt, R.W.; Henry, M.; Kieckhefer, R. Tectonics of Andaman Sea and Burma, in Geological and Geophysical Investigations of Continental Margins. Amer. Assoc. Petrol. Geol. Mem. 1979, 29, 189–198. [Google Scholar]
- Moechtar, H.; Subiyanto, S.; Sugianto, D. Geologi Aluvium Dan Karakter Endapan Pantai/Pematang Pantai di Lembah Krueng Aceh, Aceh Besar (Prov. NAD). J. Geol. Dan. Sumberd. Miner. 2009, 19, 273–283. [Google Scholar] [CrossRef]
- Kumar, S. Tipper Magnitude: A Possible Indicator of Anomalous Conducting Zone. J. Geophys. 2017, XXXV, 83–87. [Google Scholar]
- Muksin, U.; Irwandi; Rusydy, I.; Muzli; Erbas, K.; Marwan; Asrillah; Muzakir; Ismail, N. Investigation of Aceh Segment and Seulimeum Fault by Using Seismological Data; A Preliminary Result. J. Phys. Conf. Ser. 2018, 1011, 012031. [Google Scholar] [CrossRef]
- Wait, J.R. Theory of Magnetotelluric Fields. J. Res. NBS D 1962, 66, 509–541. [Google Scholar] [CrossRef]
- Berdichevsky, M.N.; Dmitriev, V.I. Magnetotellurics in the Context of the Theory of Ill-Posed Problems; Society of Exploration Geophysicists: Tulsa, OK, USA, 2002. [Google Scholar]
- Sutarno, D.; Vozoff, K. Robust M-Estimation of Magnetotelluric Impedance Tensors. Explor. Geophys. 1989, 20, 383–398. [Google Scholar] [CrossRef]
- Egbert, G.D.; Booker, J.R. Robust Estimation of Geomagnetic Transfer Functions. Geophys. J. Int. 1986, 87, 173–194. [Google Scholar] [CrossRef]
- Zorin, N.; Aleksanova, E.; Shimizu, H.; Yakovlev, D. Validity of the Dispersion Relations in Magnetotellurics: Part I—Theory. Earth Planets Space 2020, 72, 9. [Google Scholar] [CrossRef]
- Weidelt, P. The Inverse Problem of Geomagnetic Induction. Z. Geophys. 1972, 38, 257–289. [Google Scholar] [CrossRef][Green Version]
- Caldwell, T.G.; Bibby, H.M.; Brown, C. The Magnetotelluric Phase Tensor. Geophys. J. Int. 2004, 158, 457–469. [Google Scholar] [CrossRef]
- Berdichevsky, M.N.; Vanyan, L.L.; Koshurnikov, A.V. The Baikal Rift Zone: Magnetotelluric Arbitration of Geodynamic Models. In Proceedings of the XIV Workshop on EM induction in the Earth, Sinaia, Romania, 16–22 August 1998. [Google Scholar]
- Berdichevsky, M.N.; Vanyan, L.L.; Nguen, T.V. Phase Polar Diagrams of the Magnetotelluric Impedance. Izv. Akad. Nauk. SSSR Ser. Fiz. Zemli 1993, 2, 19–23. [Google Scholar]
- Berdichevsky, M.N. Electrical Prospecting by the Method of Magnetotelluric Profiling: Moscow; Nedra Publishing House: Moscow, Russia, 1968. [Google Scholar]
- Chave, A.D.; Jone, A.G. The Magnetotelluric Method: Theory and Practice; Cambridge University Press: New York, NY, USA, 2012. [Google Scholar]
- Parkinson, W.D. The Influence of Continents and Oceans on Geomagnetic Variations. Geophys. J. R. Astron. Soc. 1962, 6, 441–449. [Google Scholar] [CrossRef]
- Wiese, H. Geomagnetishce Tiefentellurik. Geofis. Pura Appl. 1962, 52, 83–103. [Google Scholar] [CrossRef]
- Kelbert, A.; Meqbel, N.; Egbert, G.D.; Tandon, K. ModEM: A Modular System for Inversion of Electromagnetic Geophysical Data. Comput. Geosci. 2014, 66, 40–53. [Google Scholar] [CrossRef]
- Egbert, G.D.; Kelbert, A. Computational recipes for electromagnetic inverse problems. Geophys. J. Int. 2012, 189, 251–267. [Google Scholar] [CrossRef]
- Evans, R.L.; Chave, A.D.; Jones, A.G.; Mackie, R.; Rodi, W. Conductivity of Earth Materials. In The Magnetotelluric Method: Theory and Practice; Chave, A.D., Jones, A.G., Eds.; Cambridge University Press: Cambridge, UK, 2012; ISBN 9781139020138. [Google Scholar]
- Selway, K. Electrical Discontinuities in the Continental Lithosphere Imaged with Magnetotellurics; AGU Publications: Washington, DC, USA, 2018; pp. 89–109. [Google Scholar]
- Glover, P.W.J. Geophysical Properties of the Near Surface Earth: Electrical Properties. In Treatise on Geophysics; Elsevier: Amsterdam, The Netherlands, 2015; pp. 89–137. [Google Scholar]
- Slezak, K.; Jozwiak, W.; Nowozynski, K.; Orynski, S.; Brasse, H. 3-D Studies of MT Data in the Central Polish Basin: Influence of Inversion Parameters, Model Space and Transfer Function Selection. J. Appl. Geophys. 2019, 161, 26–36. [Google Scholar] [CrossRef]
- Siripunvaraporn, W.; Egbert, G.; Uyeshima, M. WSINV3DMT Version 1.0.0 for Single Processor Machine. pp. 1–21. Available online: https://manualzz.com/doc/6812773/wsinv3dmt-magnetotelluric-inversion-user-manual (accessed on 5 August 2020).
- Hady, A.K.; Marliyani, G.I. Updated Segmentation Model and Cummulative Offset Measurement of the Aceh Segment of the Sumatran Fault System in West Sumatra, Indonesia. J. Appl. Geol. 2021, 5, 84. [Google Scholar] [CrossRef]
- Hoffmann-Rothe, A.; Ritter, O.; Haak, V. Magnetotelluric and Geomagnetic Modelling Reveals Zones of Very High Electrical Conductivity in the Upper Crust of Central Java. Phys. Earth Planet. Inter. 2001, 124, 131–151. [Google Scholar] [CrossRef]
- d’Erceville, I.; Kunetz, G. The effect of a fault on the earth’s natural electromagnetic field. Geophysics 1962, 27, 651–665. [Google Scholar] [CrossRef]
- Halliday, D.; Resnick, R.; Walker, J. Fundamentals of Physics, 6th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1991. [Google Scholar]
- Telford, W.M.; Geldart, L.P.; Sheriff, R.E. Applied Geophysics; Cambridge University Press: Cambridge, UK, 1976. [Google Scholar]
- Ghorbani, A.; Revil, A.; Coperey, A.; Soueid Ahmed, A.; Roque, S.; Heap, M.J.; Grandis, H.; Viveiros, F. Complex Conductivity of Volcanic Rocks and the Geophysical Mapping of Alteration in Volcanoes. J. Volcanol. Geotherm. Res. 2018, 357, 106–127. [Google Scholar] [CrossRef]
- Rahmadi, R.; Setiawan, B.; Aziz, M. Analisis Karakteristik Endapan Emas Plaser di Daerah Kecamatan Sungai Mas dan Sekitarnya, Kabupaten Aceh Barat, Provinsi Aceh. Acta Geosci. Energy Min. 2023, 2, 32–38. [Google Scholar]
- Park, C.-S.; Jeong, J.-H.; Park, H.-W.; Kim, K. Experimental Study on Electrode Method for Electrical Resistivity Survey to Detect Cavities under Road Pavements. Sustainability 2017, 9, 2320. [Google Scholar] [CrossRef]
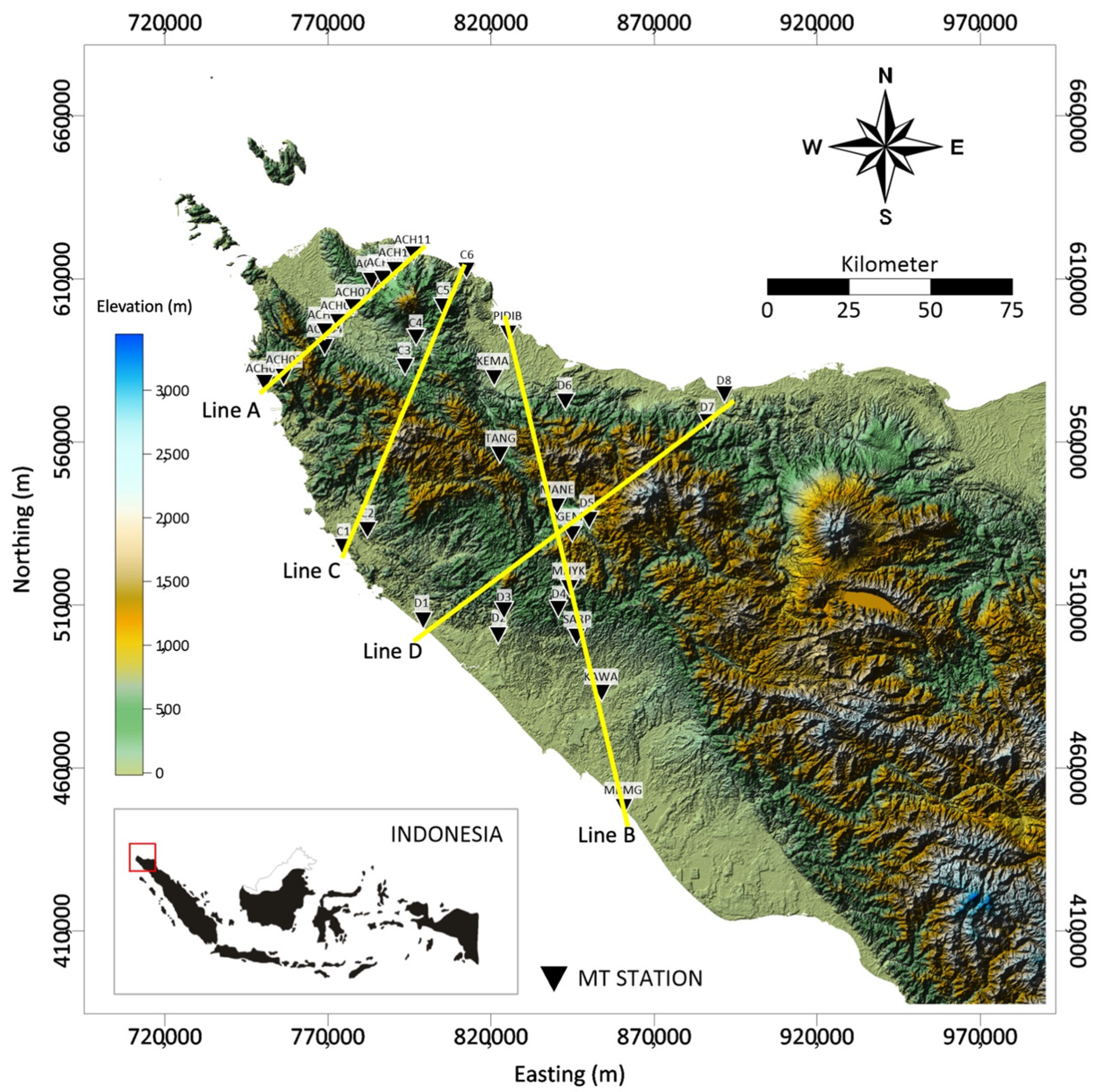







| Line | Acquisition Time | Recording Time | Spacing (km) | Direction | Site Name |
|---|---|---|---|---|---|
| A | October and November 2008 | 12 h | 6–10 | SW-NE | ACH01, ACH02, ACH04, ACH05, ACH06, ACH07, ACH08, ACH09, ACH10, ACH11 |
| B | August 2009 | 12 h | 9–50 | SW-NE | MBMG, KAWA, SARP, MNYK, GEM, MANE, TANG, KEMA, PIDIB |
| C | July–August 2012 | 12 h | 9–13 | N-N | C1, C2, C3, C4, C5, C6 |
| D | July–August 2012 | 17 h | 9–50 | SE-NW | D1, D2, D3, D4, D5, D6, D7, D8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roodhiyah, L.Y.; Nurhasan; Tiffany; Pratomo, P.M.; Susilawati, A.; Supriyadi; Ogawa, Y.; Sugiyanto, D.; Sutarno, D.; Srigutomo, W. Interpretation of a 3D Magnetotellurics Model of the Aceh and Seulimeum Segments of the Sumatran Fault Zone. Appl. Sci. 2024, 14, 11335. https://doi.org/10.3390/app142311335
Roodhiyah LY, Nurhasan, Tiffany, Pratomo PM, Susilawati A, Supriyadi, Ogawa Y, Sugiyanto D, Sutarno D, Srigutomo W. Interpretation of a 3D Magnetotellurics Model of the Aceh and Seulimeum Segments of the Sumatran Fault Zone. Applied Sciences. 2024; 14(23):11335. https://doi.org/10.3390/app142311335
Chicago/Turabian StyleRoodhiyah, Lisa Yihaa, Nurhasan, Tiffany, Prihandhanu Mukti Pratomo, Anggie Susilawati, Supriyadi, Yasuo Ogawa, Didik Sugiyanto, Doddy Sutarno, and Wahyu Srigutomo. 2024. "Interpretation of a 3D Magnetotellurics Model of the Aceh and Seulimeum Segments of the Sumatran Fault Zone" Applied Sciences 14, no. 23: 11335. https://doi.org/10.3390/app142311335
APA StyleRoodhiyah, L. Y., Nurhasan, Tiffany, Pratomo, P. M., Susilawati, A., Supriyadi, Ogawa, Y., Sugiyanto, D., Sutarno, D., & Srigutomo, W. (2024). Interpretation of a 3D Magnetotellurics Model of the Aceh and Seulimeum Segments of the Sumatran Fault Zone. Applied Sciences, 14(23), 11335. https://doi.org/10.3390/app142311335