Research on the Uplift Characteristics of Pantographs of High-Speed Trains Under Crosswind Conditions

Abstract

Through the geometric relationships and force analysis of the main components of pantographs on high-speed trains, the coefficients of aerodynamic forces and lift transmission between the pantograph and main components under crosswind conditions were derived. Based on the aerodynamic forces acting on the pantograph at different crosswind speeds, wind angles, and operating speeds, the aerodynamic lift of the pantograph and main components was ultimately determined. The results indicate that the aerodynamic lift of the pantograph is mainly distributed on the bow structure, with the aerodynamic lift of the upper frame all being negative values, while the absolute value of the aerodynamic lift of the lower arm rod is the smallest. The operating speed of the pantograph and the wind angle of the crosswind have a significant impact on the aerodynamic lift of the main components, while the impact of the crosswind speed is relatively small. At the same operating speed of the pantograph, the lower the corresponding crosswind speed, the smaller the aerodynamic lift of the pantograph. The aerodynamic lift of the pantograph tends to decrease gradually with the increase in crosswind speed, and the impact of crosswind speed decreases gradually with the increase in the pantograph operating speed. A comprehensive relationship formula between the aerodynamic lift of the pantograph and the operating speed, crosswind speed, and wind angle is obtained, and the empirical formula for the contact force of the bow net and train operating speed is modified. The research results are of great significance and value for the study and application of lift forces on pantographs under crosswind conditions.

1. Introduction

The good quality of current collection performed by the pantograph is an important factor in ensuring the stable operation of high-speed trains. Research on the uplift characteristics of pantographs under crosswind conditions has been advanced through significant contributions from scientists worldwide. Brandani, V. [1] laid the groundwork by identifying the increased uplift forces caused by crosswinds, leading to further investigations using wind tunnel models by Sanquer, Stéphane et al. [2]. Latorre Iglesias et al. [3] focused on the role of individual components but did not as comprehensively explore the variations in aerodynamic lift across different components. In this study, this is extended by showing that the bow collector experiences the most significant uplift, while the upper frame experiences negative lift, an observation that was not detailed in the previous literature. Li et al. [4] provided wind tunnel studies on crosswind effects but did not capture this non-linear behavior. In this study, their experimental work is developed by using advanced numerical simulations (DES) to show how aerodynamic lift behaves at extreme wind angles and speeds, offering new insights that were not previously reported. Kan et al. [5] previously identified the importance of the pantograph head; in this study, this is expanded by detailing how crosswind angles affect different components, offering more precise guidance for optimizing pantograph design. European researchers have since focused on integrating aerodynamic analyses with overall train stability and control, contributing to the development of robust pantograph designs that maintain reliable contact with the catenary under varying wind conditions [6]. Meanwhile, American scientists have emphasized advanced CFD modeling and experimental studies, which have deepened the understanding of complex airflow patterns and dynamic responses of pantographs [7]. These global efforts have collectively improved the safety and efficiency of high-speed rail systems, although challenges remain, particularly in addressing extreme crosswind conditions as train speeds continue to rise. In this study, we seek to build on these global contributions by offering a comprehensive analysis of pantograph uplift under crosswind conditions, aiming to enhance the overall understanding and mitigation strategies.

The contact force of the bow net includes two parts, the static lift force and the aerodynamic lift force generated by the pantograph, collectively referred to as the dynamic contact force of the bow net. The static lift force is generally around 70 N, typically not exceeding 90 N [8,9,10]. During high-speed operation, the contact pressure between the bow net and the pantograph is the sum of the static lift force and the aerodynamic lift force generated by the pantograph, known as the dynamic contact force of the bow net. During the sliding contact between the pantograph and the contact line, if the dynamic contact force is too small, the contact resistance increases, leading to phenomena such as the pantograph lifting and arcing during operation [11]. Conversely, if the contact pressure is too high, local grooves may be formed on the sliding block, resulting in serious accidents such as the contact wire bouncing and pantograph scraping. Therefore, it is necessary to ensure a reasonable aerodynamic lift force for the pantograph during operation, which requires the pantograph itself to have good aerodynamic characteristics [12,13]. A large amount of research has been conducted based on the dynamics of the bow net, but there is relatively little research on the impact of aerodynamic forces on the contact force of the bow net, especially without considering the change in aerodynamic effects of the pantograph under crosswind conditions [14,15,16]. Furthermore, as the train speed increases, the impact of aerodynamic forces cannot be ignored. Combined with the effect of crosswind, the law of aerodynamic lift force of the pantograph also changes. Therefore, further in-depth analysis is needed.

When the pantograph operates at high speed, the average value $p_v$ of the experimental bow net contact force can be obtained through $p_v=p_1+p_2=0.00097V^2+70$, where the static contact force $p_2$ of the bow net is 70 N, as shown in Figure 1, and $p_1$ represents the aerodynamic lift force of the pantograph [17]. Under the influence of crosswind, the aerodynamic load of the pantograph changes with the speed of the train, wind speed, and wind angle, resulting in a change in aerodynamic lift force. Therefore, it is not appropriate to simply use the train speed as the criterion for determining the aerodynamic lift force. Consequently, an in-depth study of the aerodynamic lift force of the pantograph under crosswind conditions is necessary. In this paper, by establishing a model of the aerodynamic lift force of the pantograph under crosswind conditions and combining it with numerical simulation methods of aerodynamic forces acting on the pantograph, the lift force of the pantograph and its main components are analyzed. The results have certain engineering practical value.

2. Pantograph Aerodynamic Lift Calculation Model

The pantograph prototype consists of components such as the sliding block, bracket, balance lever, upper arm rod, hinge seat, lower arm rod, push rod, and base frame, all considered rigid bodies. In the analysis of pantograph lift force, the main components of the pantograph are selected, and the geometric model of the pantograph is simplified. It can be observed from the physical model that the pantograph structure is complex, and it is feasible to describe its motion and mechanical analysis using an equivalent two-dimensional framework in practice. For clarity, here, the pantograph sliding block and bracket are referred to as the bow head. Components with relatively small aerodynamic forces, such as the balance lever, are neglected, resulting in the final geometric model of the pantograph used for lift force calculation, as shown in Figure 2.

The aerodynamic lift generated by the pantograph during high-speed operation is the combined effect of aerodynamic drag, lift, lateral force, and aerodynamic moments on each component. It results in a vertical force at the bow head, which is equivalent to the top end of the upper arm rod. Neglecting the deformation of the bow head spring under the aerodynamic action of the pantograph, the bow head can only undergo vertical motion. Therefore, a vertical constraint is applied at the top end of the upper arm rod to calculate the vertical constraint reaction forces generated by each component of the pantograph under aerodynamic forces, thereby obtaining the aerodynamic lift of the pantograph.

3. Methods for Calculating the Aerodynamic Lift of the Pantograph

The method adopted in this paper for calculating the aerodynamic lift of the pantograph begins with a rational simplification and force analysis of the pantograph on high-speed trains, deriving the conversion relationship between the aerodynamic forces and lift forces of the main components of the pantograph. Based on the analysis of the aerodynamic characteristics of the pantograph, the aerodynamic forces acting on each component are determined. These aerodynamic forces are then applied to the lift force calculation model, establishing the mechanical equilibrium equations of the model, and ultimately solving for the aerodynamic lift of the pantograph and its main components. The geometric model of the pantograph used in this paper is consistent with that in references, and similar simplifications have been applied. Initially, the case without crosswind is considered, and a comparison with the calculated values from references shows good agreement. The calculated values from the references align well with the experimental results, with deviations within acceptable limits, as depicted in Figure 3. Therefore, adopting the calculation method from these references for determining the aerodynamic lift of the pantograph in this paper is reliable and accurate.

3.1. Geometric Relationships and Force Analysis of Pantograph Components

To analyze the transfer coefficients of the resistance and lift of each component of the pantograph into aerodynamic lift, the aerodynamic forces are equivalent to a point where the aerodynamic moment is zero. Figure 4 illustrates the geometric relationships of the pantograph components during operation [18]. In the figure, L₁, L₂, L₃, L₄, L₅, S₁, S₂, and S₃ represent the distances of segments GF, FC, CB, CD, DE, AG, AC, and BE, respectively. α, β, γ, ε, and θ represent the angles between the lines of the push rod, hinge A, line connecting points G and F, lower arm rod, and segments BC and CE of the upper arm rod with the x-axis. Points D and F represent the points on the upper and lower arm rods where the aerodynamic moment is zero.

According to the design requirements, the pantograph bow head moves vertically. Neglecting the deformation caused by the aerodynamic lift force on the spring, only vertical displacement occurs at the pantograph bow head and the top of the upper arm rod. After applying a vertical constraint at the top end of the upper arm rod, the vertical constraint reaction forces generated by each component of the pantograph under aerodynamic forces can be calculated, thereby obtaining the aerodynamic lift of the pantograph, which is equal in magnitude but opposite in direction to this force. Figure 5 illustrates the force analysis of the upper arm rod and lower arm rod. In the figure, Fe represents the vertical constraint reaction force; F₇, F₅, and F₁₀ are the resistances acting on the bow head, upper arm rod, and lower arm rod, respectively; F₆, F₄, and F₁₁ are the lifts acting on the bow head, upper arm rod, and lower arm rod, respectively; F₂ and F₃ represent the forces exerted by the lower arm rod on the upper arm rod at the hinge C; F₈ and F₉ represent the forces exerted by the upper arm rod on the lower arm rod at the hinge C′; and F₁ represents the internal force of the push rod.

3.2. Calculation of Transfer Coefficients

Based on the geometric relationships and force analysis of the pantograph components described above, the force balance equation for the lower arm rod and the moment balance equation for point G in the Cartesian coordinate system are established, as shown in Equation (1). Similarly, the force balance equation for the upper arm rod and the moment balance equation for point C in the Cartesian coordinate system are established, as shown in Equation (2). From the force analysis of the upper and lower arm rods, it is known that F₂ equals F₉ and F₃ equals F₈. The segments L₄ and L₅ of the upper arm rod are denoted as L₄₅, while the segments L₁ and L₂ of the lower arm rod are denoted as L₁₂. By solving the above two equations simultaneously, the vertical constraint force Fe can be obtained, as shown in Equation (3).

The angles α, β, γ, ε, and θ are determined based on the geometric relationships of the pantograph components, while L₁ and L₄ are obtained based on the equivalent points with zero aerodynamic moment. As indicated by the equations above, once the aerodynamic force equivalent positions and angles of the pantograph components are determined, the transfer coefficients for converting the aerodynamic drag and lift into aerodynamic lift force can be obtained. The effect of crosswind is considered in the form of resultant force. A positive transfer coefficient indicates that the force increases the aerodynamic lift force, while a negative transfer coefficient indicates that the force reduces the aerodynamic lift force. The transfer coefficient reflects the efficiency of converting the aerodynamic force of the pantograph into aerodynamic lift force. Once the transfer coefficients are determined, the aerodynamic lift forces of the pantograph and its main components can be calculated.

$$\left[{(\mathrm{L}}_1{+\mathrm{L}}_2)\sin \gamma { -(\mathrm{L}}_1{+\mathrm{L}}_2)\cos \gamma { \mathrm{L}}_2\sin \gamma { \mathrm{L}}_2\cos \gamma \right]\left[\begin{array}{l}{\mathrm{F}}_8\\ {\mathrm{F}}_9\\ {\mathrm{F}}_{10}\\ {\mathrm{F}}_{11}\end{array}\right]=0$$

(1)

$$\left[\begin{array}{llllllll}0& 0& -1& 0& -\cos \mathsf{\alpha }& 1& 0& 0\\ 1& 0& 0& 1& \sin \mathsf{\alpha }& 0& -1& -1& \\ {(\mathrm{L}}_4{+\mathrm{L}}_5)\sin \mathsf{\theta }& ({\mathrm{L}}_4{+\mathrm{L}}_5)\cos \mathsf{\theta }& {\mathrm{L}}_5\sin \mathsf{\theta }& {\mathrm{L}}_5\cos \mathsf{\theta }& -{\mathrm{L}}_3\sin (\alpha +\epsilon )& 0& 0& -({\mathrm{L}}_4+{\mathrm{L}}_5)\cos \mathsf{\theta }\end{array}\right]\left[\begin{array}{l}{\mathrm{F}}_1\\ {\mathrm{F}}_2\\ {\mathrm{F}}_3\\ {\mathrm{F}}_4\\ {\mathrm{F}}_5\\ {\mathrm{F}}_6\\ {\mathrm{F}}_7\\ {\mathrm{F}}_{\mathrm{e}}\end{array}\right]=\left[\begin{array}{l}0\\ 0\\ 0\end{array}\right]$$

(2)

$$\left[\begin{array}{cccccc}{\displaystyle \frac{a}{m}}& 1& {\displaystyle \frac{b}{m}}& {\displaystyle \frac{c}{m}}& {\displaystyle \frac{d}{n}}& {\displaystyle \frac{e}{n}}\end{array}\right]\left[\begin{array}{c}{F}_7\\ F_6\\ F_5\\ F_4\\ F_{10}\\ F_{11}\end{array}\right]={\mathrm{F}}_e$$

(3)

$$a=\sin \gamma \sin (\alpha +\epsilon )L_3-\sin \theta \sin (\alpha -\gamma )L_{45}$$

$$b=\sin \gamma \sin (\alpha +\epsilon )L_3-\sin \theta \sin (\alpha -\gamma )L_2$$

$$c=-\sin \theta \sin (\alpha -\gamma )L_2-\sin \gamma \sin (\alpha +\epsilon )L_3$$

$$d=\sin \gamma \sin (\alpha +\epsilon )L_1{L}_3$$

$$e=\cos \gamma \sin (\alpha +\epsilon )L_1{L}_3$$

$$m=\cos \theta \sin (\alpha -\gamma )L_{45}+\cos \gamma \sin (\alpha +\epsilon )L_3$$

$$n=[\cos \theta \sin (\alpha -\gamma )L_{45}+\cos \gamma \sin (\alpha +\epsilon )L_3]L_{12}$$

3.3. Pantograph Aerodynamics Model

The pantograph consists of components such as the sliding block, sliding block bracket (bow head), upper arm rod, balance lever, lower arm rod, push rod (pull rod), base frame, damper, support insulators, airbags, etc. In numerical simulation calculations, the focus is on the aerodynamic characteristics and flow field characteristics of the main components of the pantograph. Therefore, the pantograph is appropriately simplified, as shown in Figure 6.

A model of the electric train set, including the pantograph, train, and overhead contact system, was built to scale at a 1:1 ratio. Considering that the pantograph is mainly affected by the wall area on the top of the train, a train model with three carriages was used, with the bogie part simplified, and the pantograph was positioned in the middle of the train. The lengths of the front, middle, and rear cars were 25.7 m, 25 m, and 25.7 m, respectively, with a width of 3 m, and the contact line was 6.0 m above the ground. The dimensions of the computational domain were length × width × height = 267 m × 192 m × 34 m, as shown in Figure 7.

It is specified that the pantograph moves along the $+x$ direction in the computational domain, indicating that the main airflow moves along the $-x$ direction, with $-V_t$ specified as the main airflow velocity. The crosswind moves along the $+z$ direction, with $V_w$ specified as the crosswind velocity, and $\theta$ representing the angle between the crosswind and the main airflow direction, specified as the crosswind angle. This is illustrated in Figure 8.

The computational domain grid uses the Trim grid, and the surfaces of the pantograph and the train body are treated using the wall function method. To ensure a smooth connection between the boundary grid and the main flow area, the boundary grid thickness is set to 6.8 mm, and the boundary layer grid is divided into 6 layers, with the first layer grid near the wall set at a distance of 0.2 mm from the wall. Regions with significant changes in flow field, such as the pantograph, train surface, and wake, are refined. The total number of grids is 13.97 million, ensuring the accuracy of the calculation, as shown in Figure 9.

3.4. Numerical Simulation Method

The detached eddy simulation (DES) method combines the advantages of Reynolds-Averaged Navier–Stokes (RANS) and large eddy simulation (LES). In this study, the DES simulation method is employed, with the SST turbulence model selected. The SST model is based on the assumption of shear stress transport (SST) and combines the characteristics of the k-ε and k-ω turbulence models through a blending function [19]. The numerical simulation results are compared and analyzed against the experimental results of a 1:8 scaled model of high-speed train aerodynamic performance conducted in the 8 m × 6 m wind tunnel at the China Aerodynamics Research and Development Center, as shows in Figure 10. The aim was to validate the accuracy of the numerical simulation method. The results show that the error between the numerical simulation and model experiments was within a reasonable range, demonstrating the feasibility of analyzing the aerodynamic loads on high-speed train pantographs using numerical simulation.

4. Aerodynamic Lift of the Pantograph

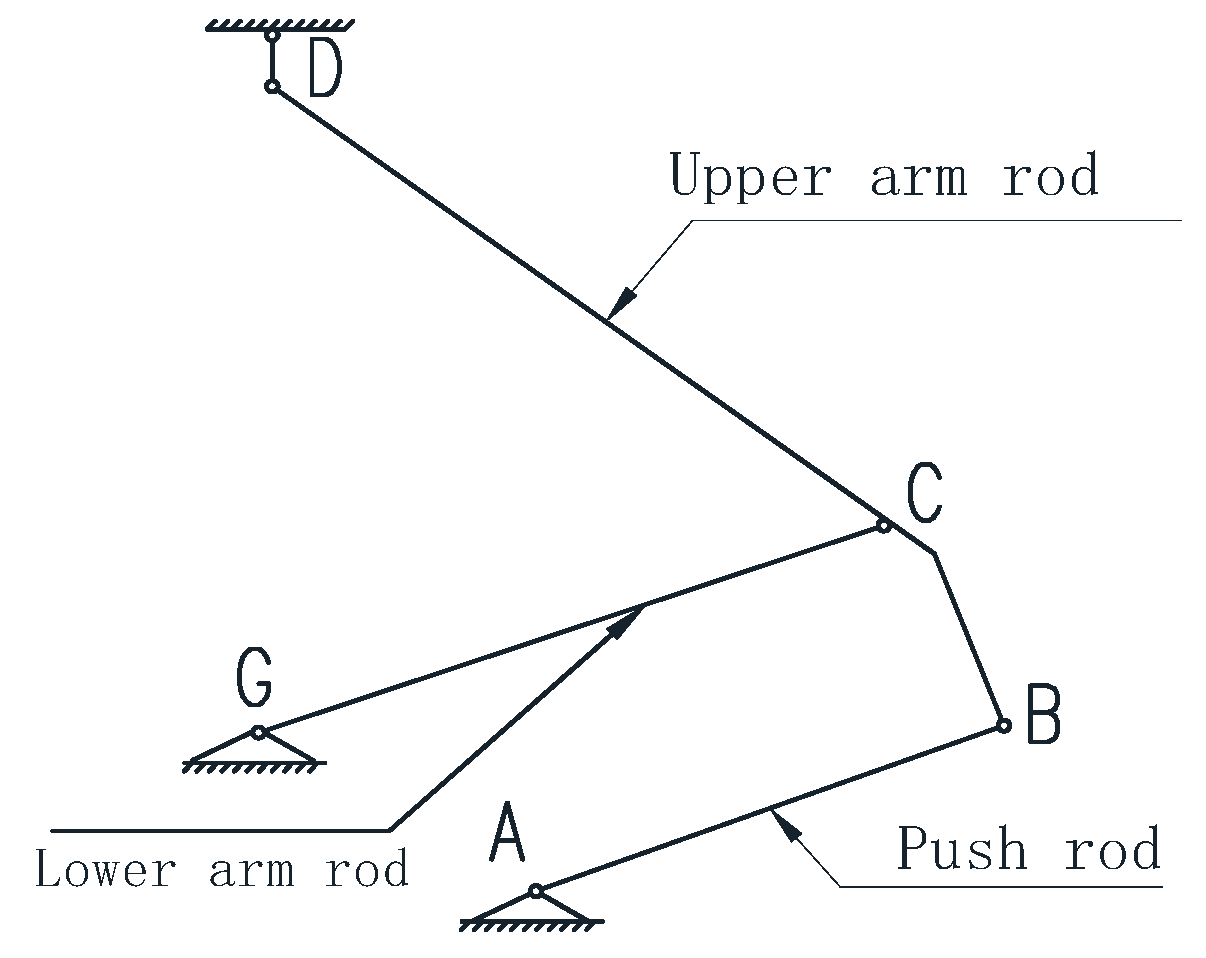
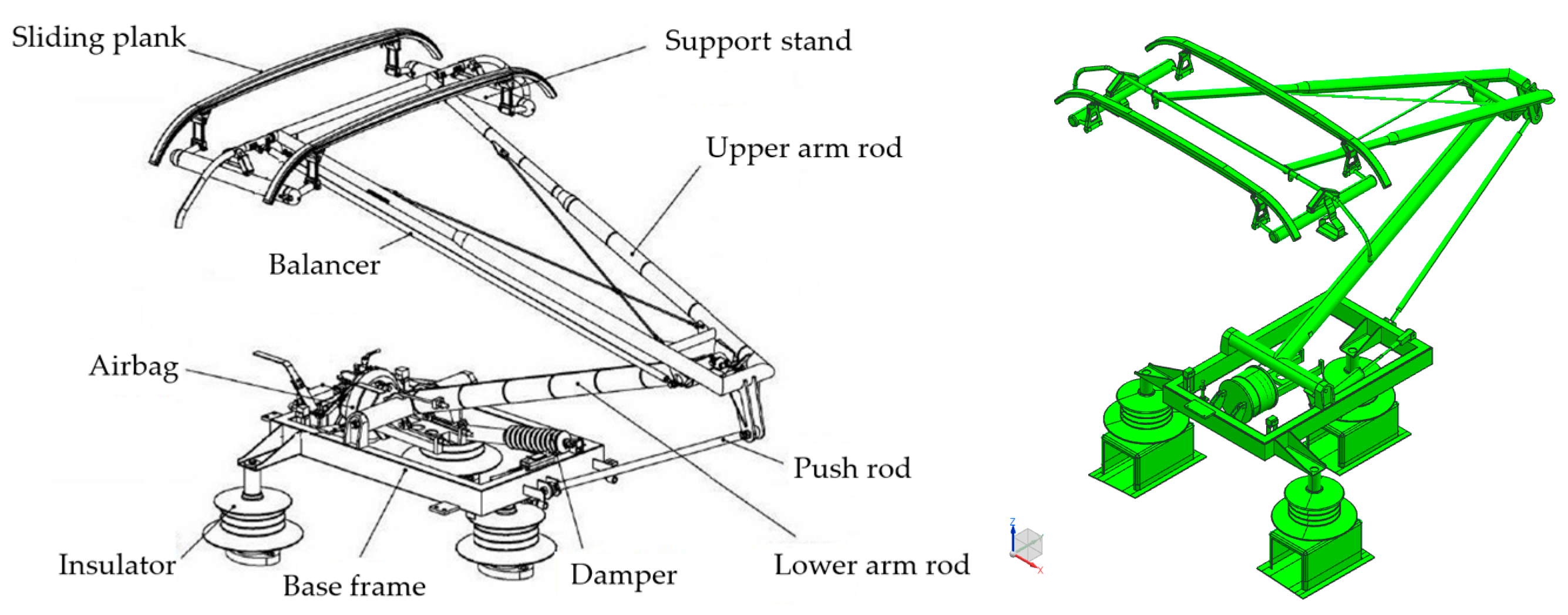
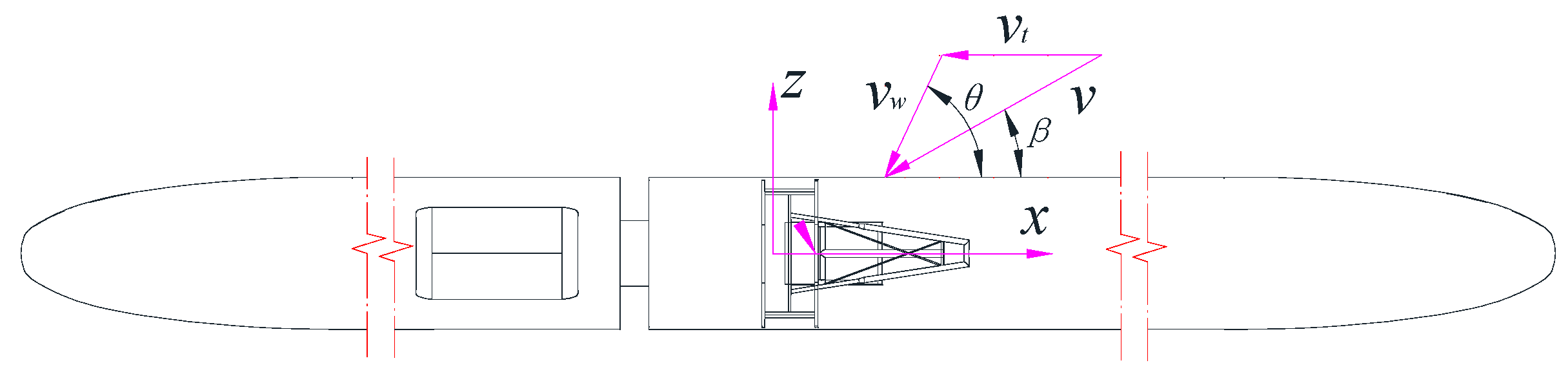
4.1. Aerodynamic Loads on the Pantograph

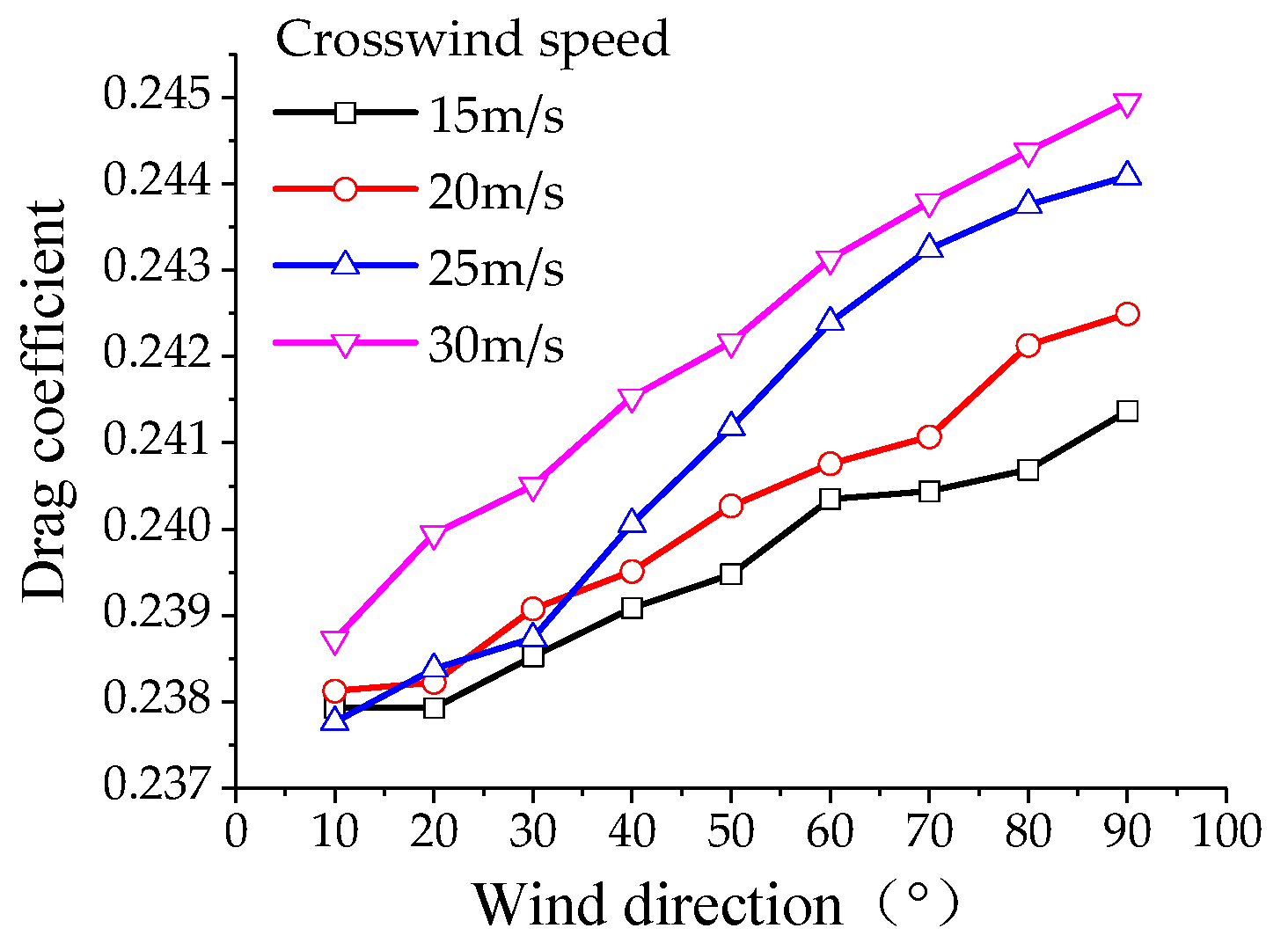
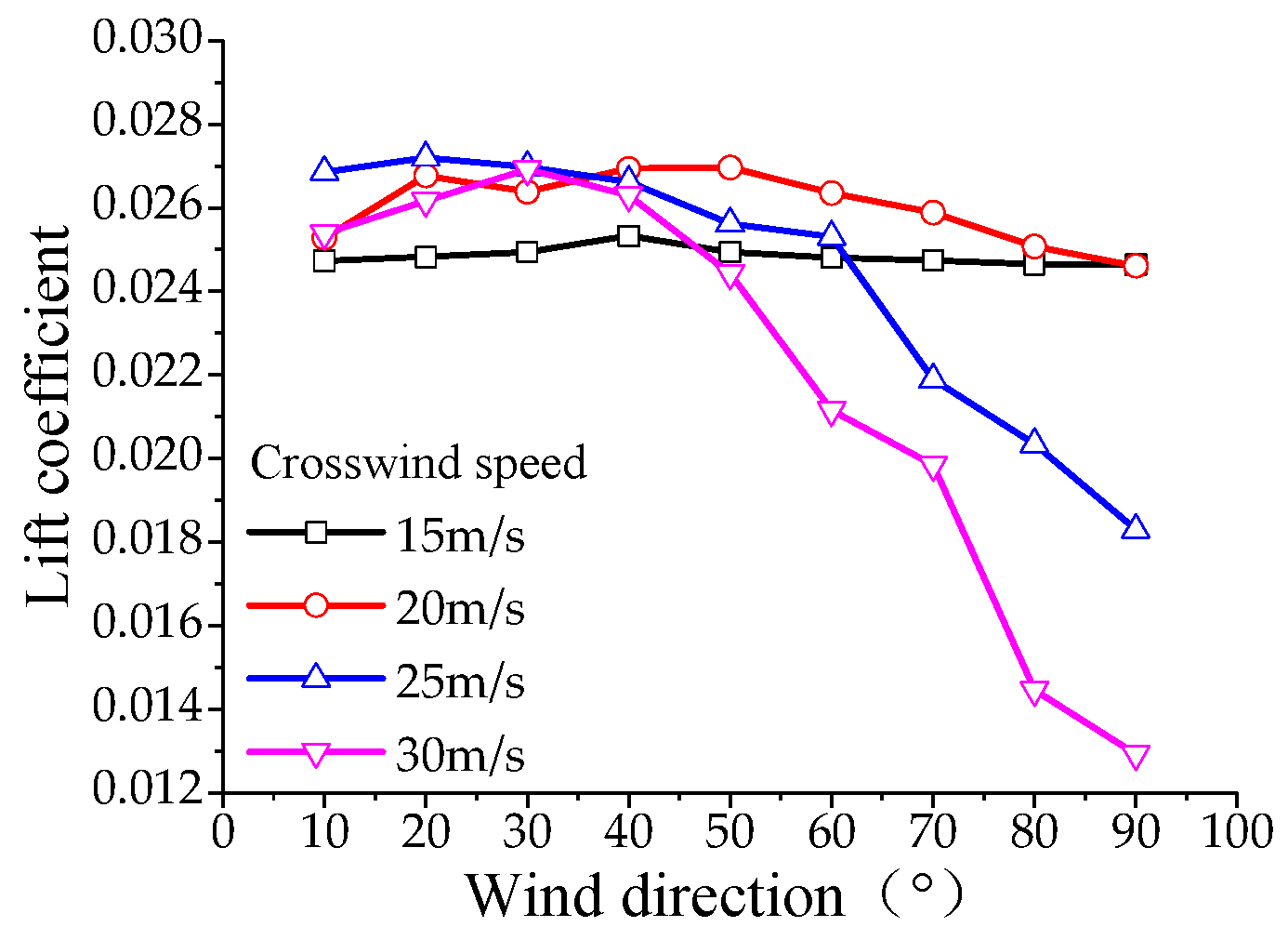
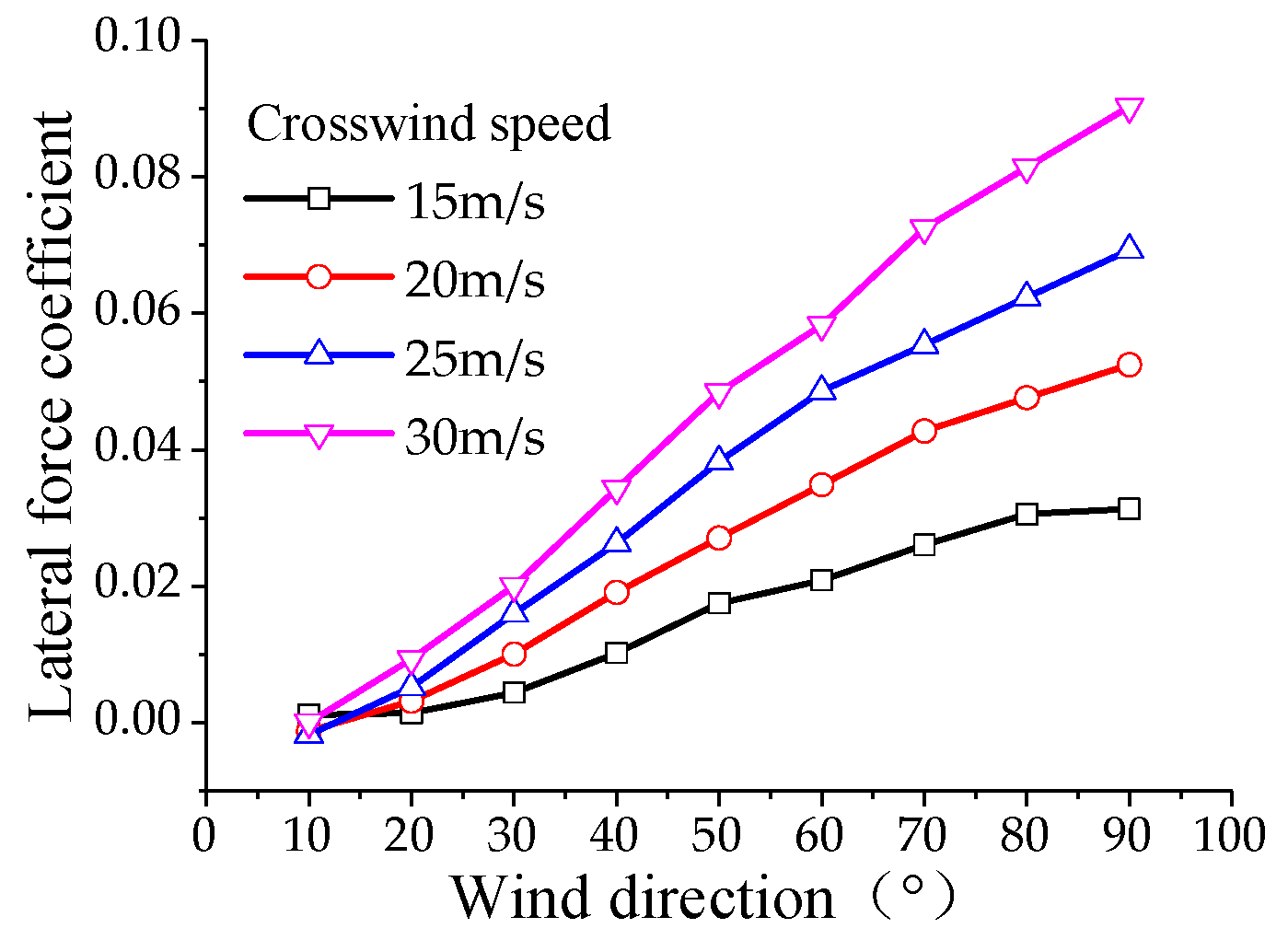
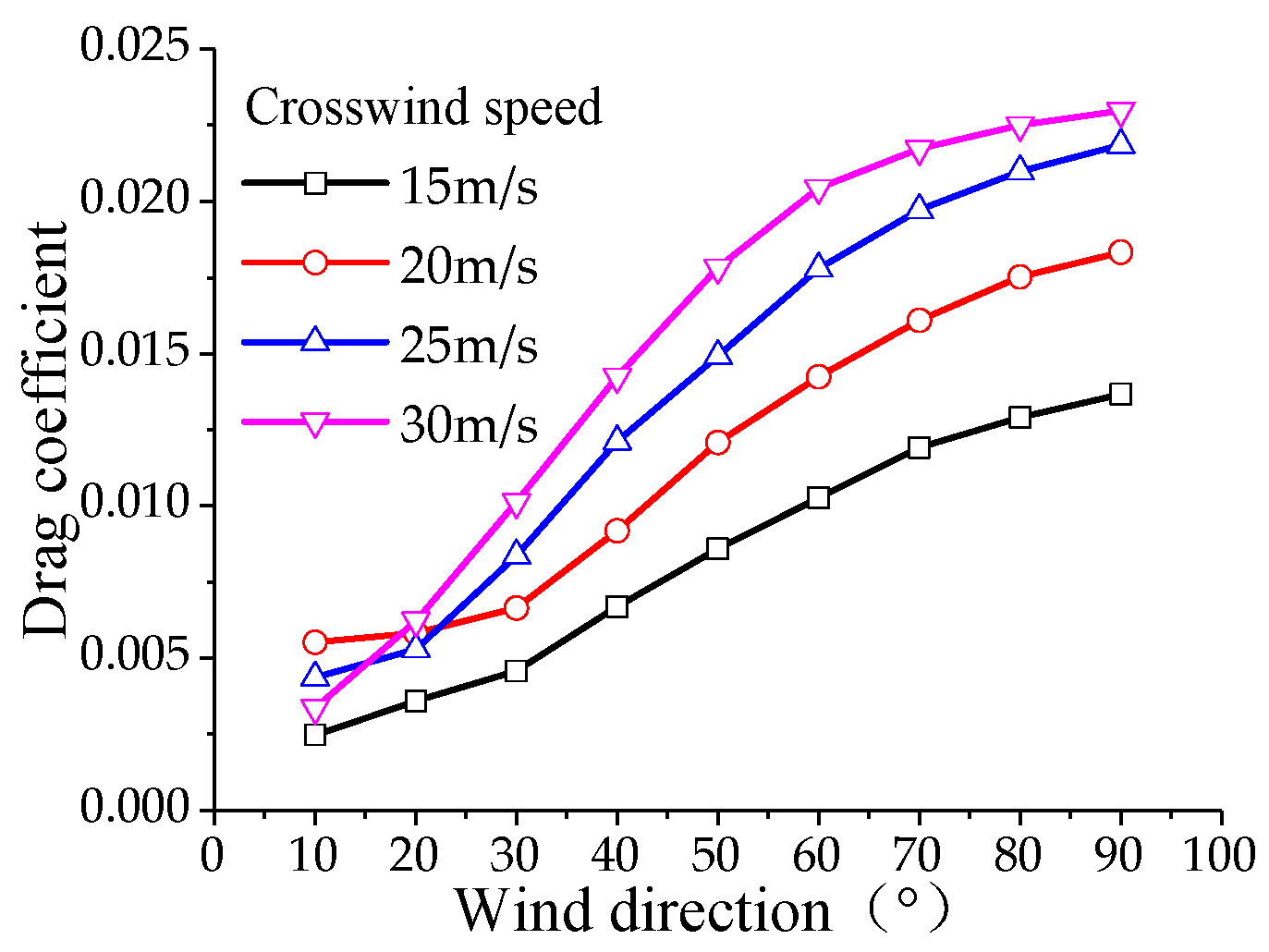
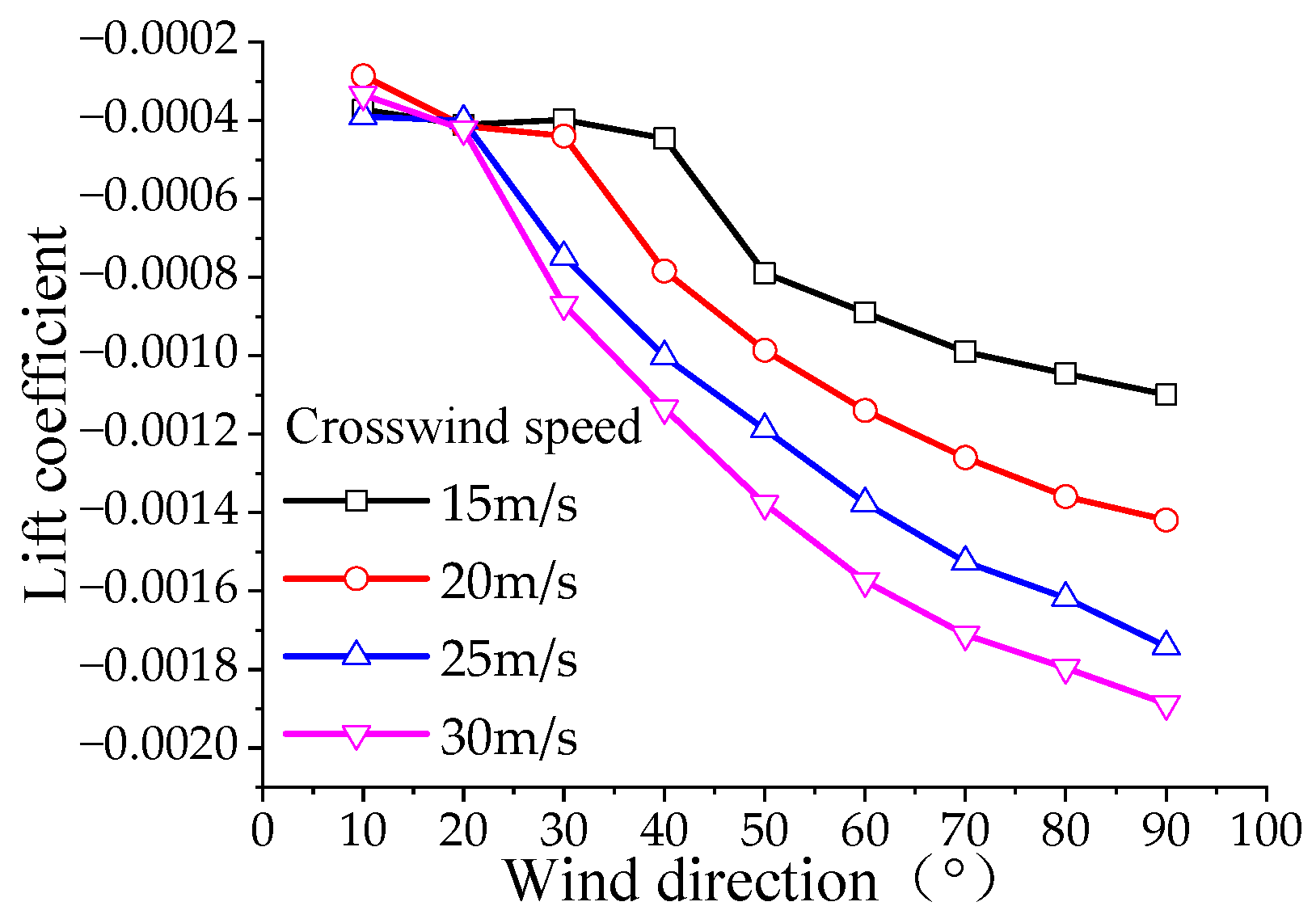
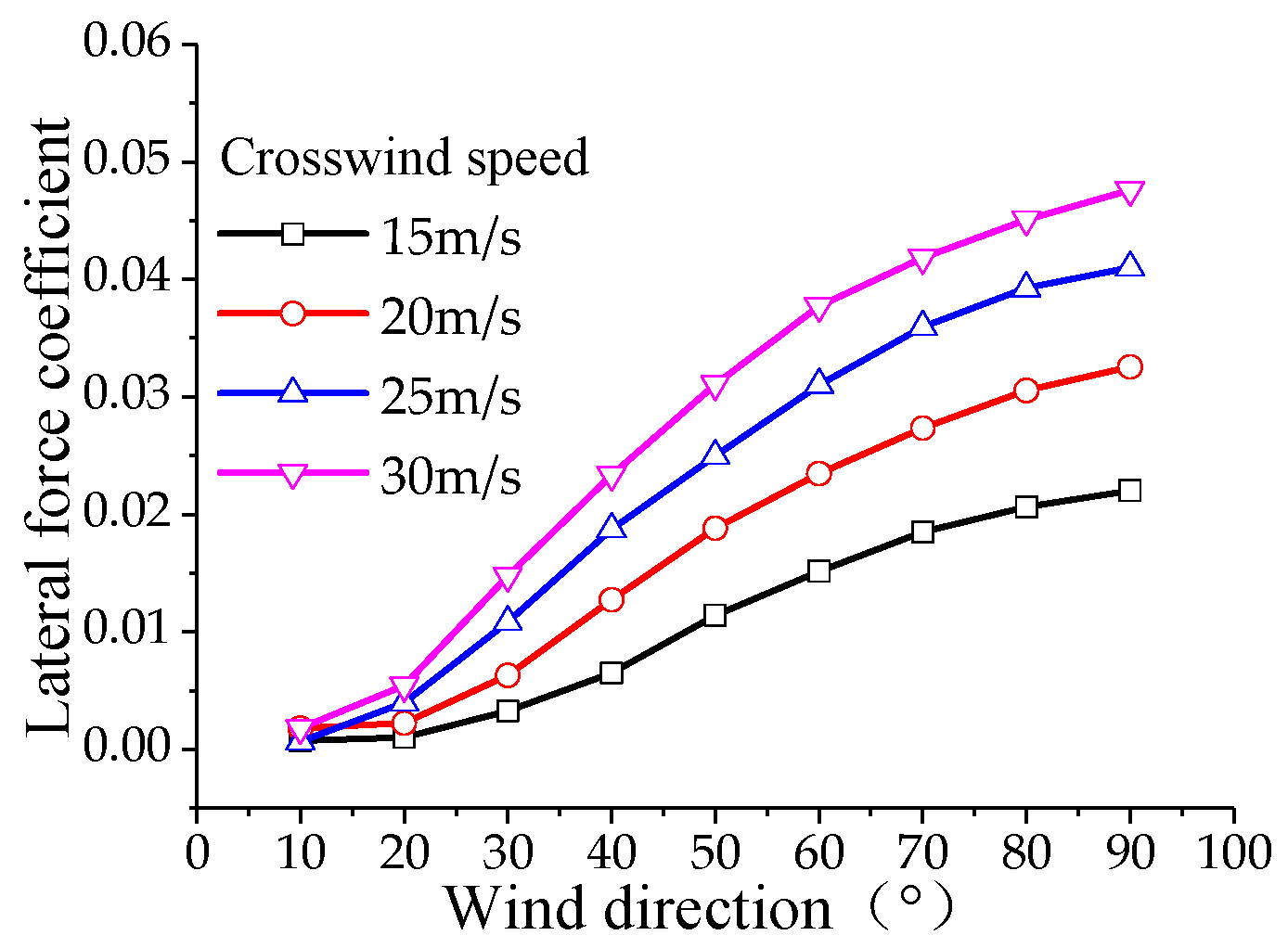
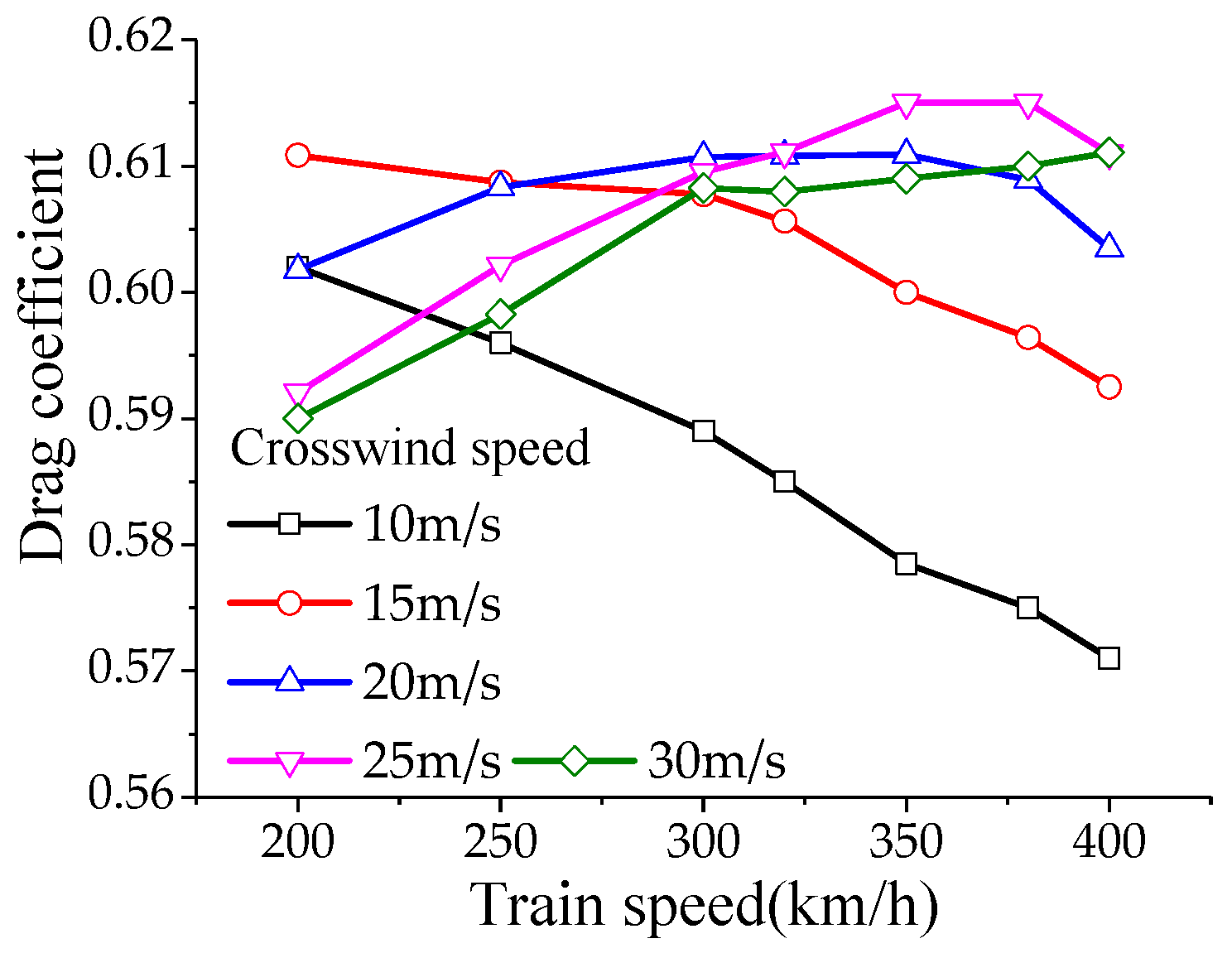
To analyze the aerodynamic lift experienced by the pantograph, the calculation conditions for the aerodynamic loads on the pantograph were as follows: the operating speed of the high-speed train ranges from 200 km/h to 400 km/h (in increments of 50 km/h), with crosswind speeds of 10, 15, 20, 25, and 30 m/s, and crosswind angles ranging from 10° to 90° (in increments of 10°). According to the results of the numerical simulations, under the influence of crosswind, the drag coefficient of the pantograph varies non-monotonically with increasing train speed, reaching its maximum value at a speed of 350 m/s and then decreasing. The lift and lateral force coefficients decrease monotonically with increasing train speed. The drag coefficient of the pantograph varies non-monotonically with increasing crosswind speed, reaching its maximum value at a crosswind speed of 25 m/s and then decreasing. The lift and lateral force coefficients increase monotonically with increasing crosswind speed. The drag, lift, and lateral force coefficients increase monotonically with increasing crosswind angle, with the maximum drag coefficient occurring at a crosswind speed of 30 m/s and a crosswind angle of 90°, as shown in Figure 11, Figure 12 and Figure 13.

Figure 11 shows the drag coefficient variation with train speed. The drag coefficient increases non-linearly, peaking at around 350 km/h and then decreasing. This behavior is due to the complex interaction between aerodynamic forces and the pantograph structure, where certain speeds maximize aerodynamic resistance.

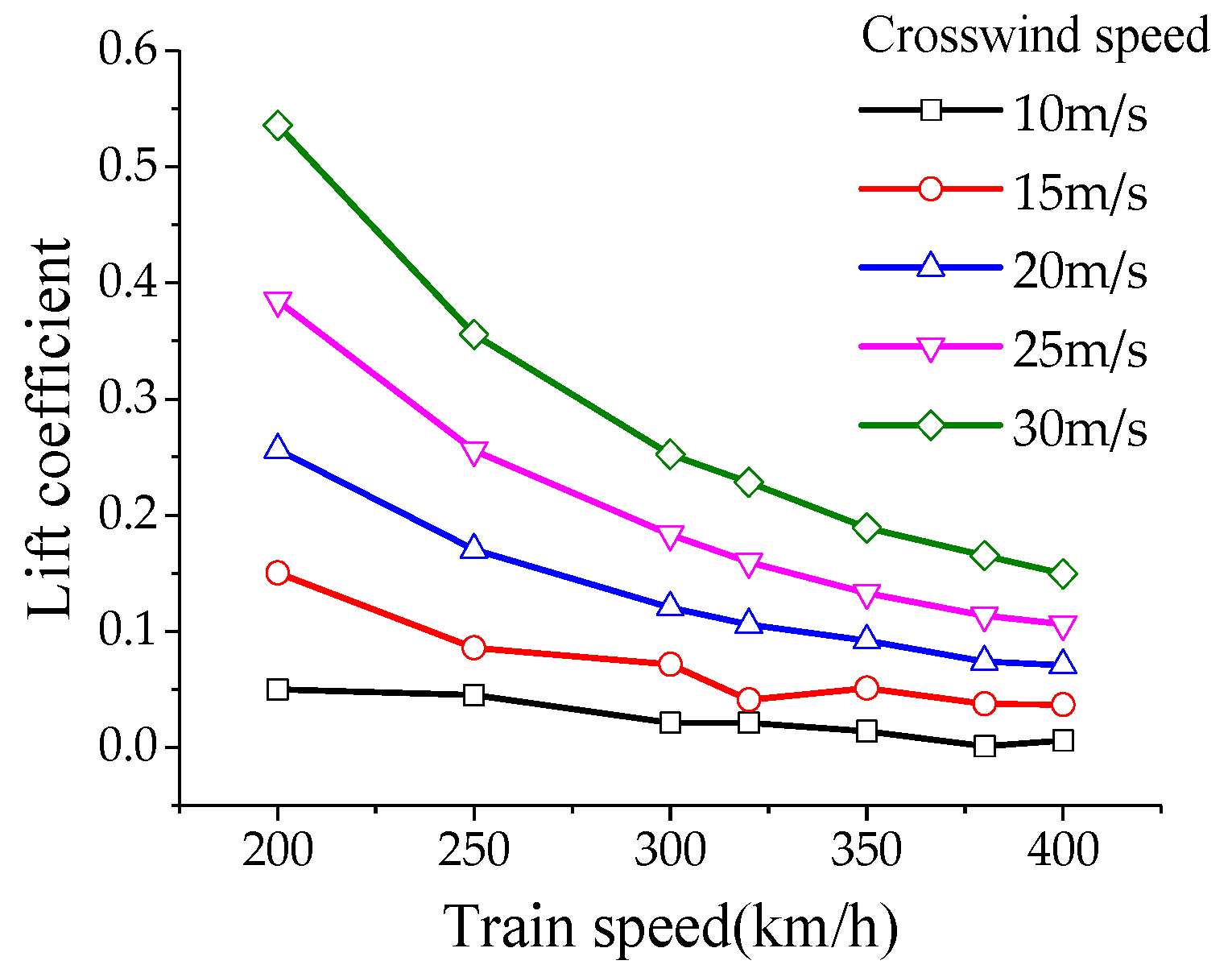
Figure 12 depicts the variation in the lift coefficient with train speed. The lift coefficient decreases steadily as the train speed increases, indicating that the aerodynamic lift acting on the pantograph becomes less pronounced at higher speeds, which helps maintain stable contact with the overhead line.

Figure 13 presents the lateral force coefficient’s response to changes in train speed and crosswind speed. As both factors increase, the lateral force coefficient also increases, highlighting the destabilizing effect of crosswinds on the pantograph, particularly at higher speeds.

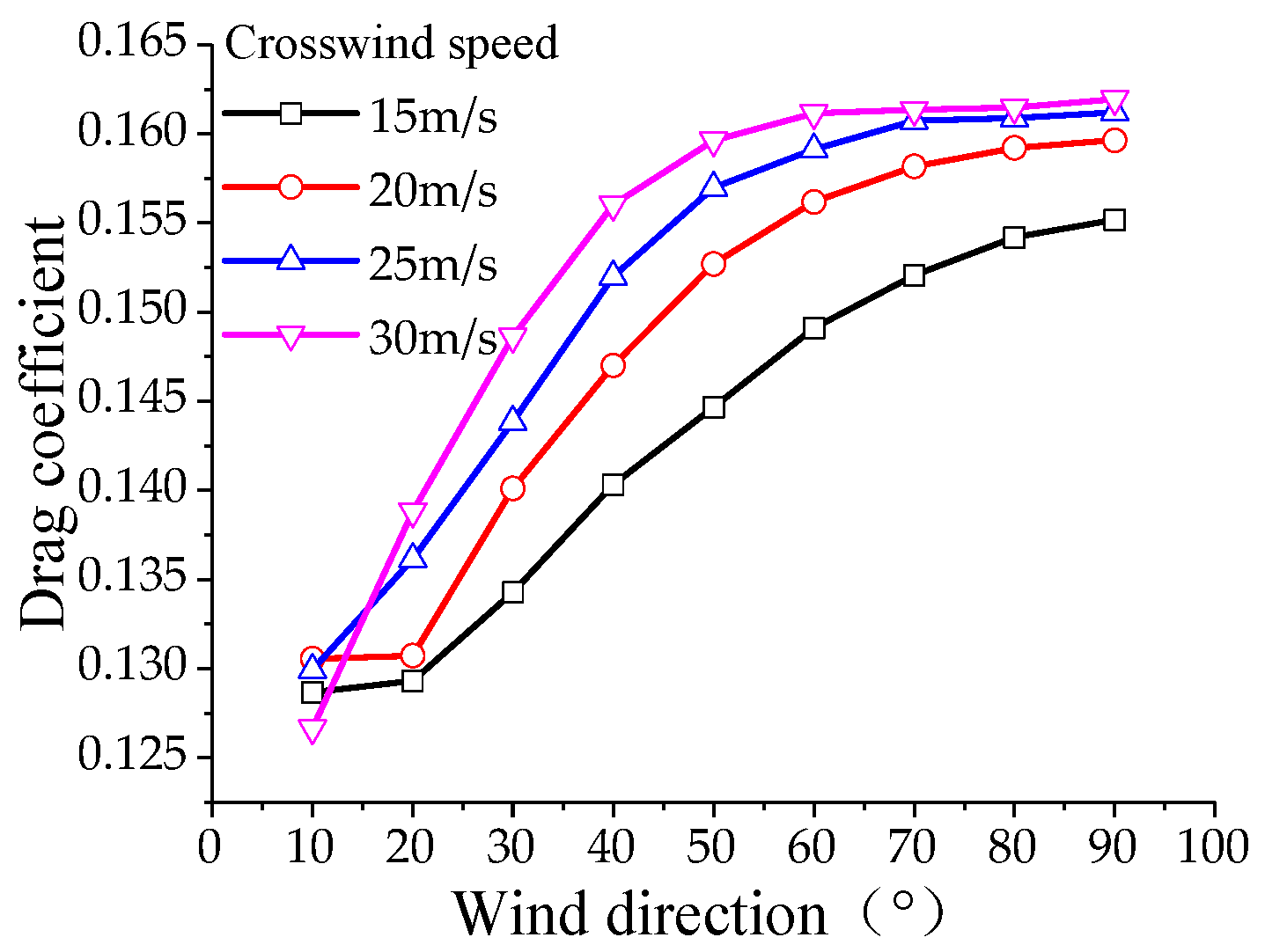
Figure 14 shows that the drag coefficient seems to generally decrease as the train speed increases for most crosswind conditions (especially at higher speeds). The fitting lines for crosswind speeds show different trends, where some lines have positive slopes (e.g., 15 m/s and 30 m/s crosswinds) and others have negative slopes (e.g., 10 m/s, 20 m/s, and 25 m/s crosswinds). The confidence bands (shaded regions) around the linear fits suggest the certainty in the model’s estimates. Wider confidence bands at lower and higher speeds, particularly for 30 m/s and 15 m/s, indicate higher uncertainty. The 95% prediction bands suggest expected variability in new data points, showing the drag coefficient fluctuates more in certain speed intervals (lower for faster speeds).

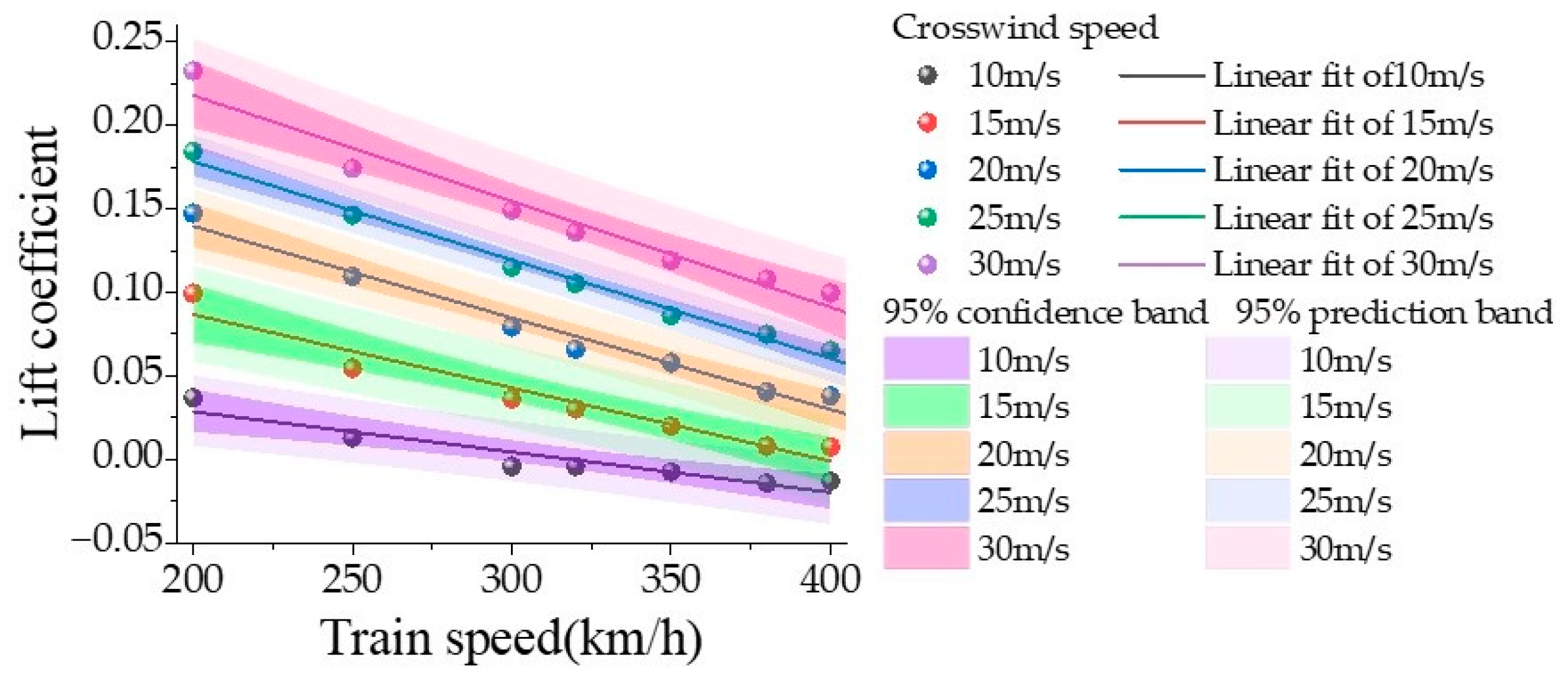
Figure 15 shows the lift coefficient generally decreases with increasing train speed across all crosswind speeds (10 m/s to 30 m/s). The linear fits for the various crosswind speeds follow almost parallel paths, indicating a consistent trend across wind conditions. Confidence intervals (shaded regions) are tighter for lower crosswind speeds (10 m/s and 15 m/s), indicating a higher certainty in the model’s fit for these conditions. In contrast, for higher crosswind speeds (25 m/s and 30 m/s), the confidence and prediction intervals are wider, showing greater uncertainty and variability at these higher wind speeds.

Figure 16 illustrates the lateral force coefficient tends to decrease as the train speed increases, which is consistent across all crosswind speeds. There is a clear separation between the different crosswind speeds, with higher crosswind speeds (e.g., 30 m/s) showing larger lateral force coefficients. The confidence intervals (shaded regions) show relatively narrow bands for lower wind speeds (10 m/s, 15 m/s, 20 m/s), suggesting a reliable model fit. The prediction bands show that variability is relatively low, even for higher wind speeds. Notably, the confidence bands for 30 m/s are wide, reflecting uncertainty in the lateral force coefficient at high crosswind speeds.

Based on the numerical simulation, a comprehensive relationship equation for the aerodynamic forces and moment coefficients of the pantograph with respect to the train speed, crosswind speed, and wind direction angle is proposed. Taking the aerodynamic drag of the pantograph as an example, the aerodynamic drag F_x is related to the pantograph’s operating speed (i.e., train speed) $V_t$_, crosswind speed $V_w$, air density $\rho$, dynamic viscosity coefficient $\mu$, characteristic size of the pantograph $h$, and surface roughness of the pantograph $k_s$, and can be expressed by the following equation:

$$f(F_x,V_t,V_w,\theta ,\rho ,\mu ,h,k_s)=0$$

(4)

Based on $Re=V_t\rho h/\mu$, therefore, as follows:

$${\displaystyle \frac{F_x}{V_t^2\rho h^2}}=f({\displaystyle \frac{V_w}{V_t}},{\displaystyle \frac{1}{Re}},{\displaystyle \frac{k_s}{h}},\theta )$$

(5)

The drag coefficient of the pantograph is given by $C_x=F_x/V^2\rho h^2{S}_x$ where $V^2=V_t^2+V_w^2+2V_t{V}_w\cos \theta$, thus can derive, as follows:

$$C_x=F_x/V^2\rho h^2{S}_x,V^2=V_t^2+V_w^2+2V_t{V}_w\cos \theta$$

(6)

Therefore,

$$C_x={\displaystyle \frac{1}{1+{(\frac{V_w}{V_t})}^2+2(\frac{V_w}{V_t})\cos \theta }}f({\displaystyle \frac{V_w}{V_t}},{\displaystyle \frac{1}{Re}},{\displaystyle \frac{k_s}{h}},\theta )$$

(7)

Since the geometric dimensions of the pantograph remain constant, and $k_s/h$ is a constant, the drag coefficient of the pantograph is only related to $V_w/V_t$ and $\theta$. Expressing $\theta$ in the form of $cos\theta$, where $V_t$ and $V_w$ represent the train speed and crosswind speed, respectively, and their ratio is dimensionless, defined as the wind speed to train speed ratio ${\lambda }_{wt}$, ${\lambda }_{wt}=V_w/V_t$. Thus, we have the following:

$$C_x={\displaystyle \frac{1}{1+{({\lambda }_{wt})}^2+2({\lambda }_{wt})\cos \theta }}f({\lambda }_{wt},\cos \theta )$$

(8)

From the above equation, it is evident that the drag coefficient of the pantograph is only related to the wind speed to train speed ratio ${\lambda }_{wt}$ and the cosine of the crosswind angle cos θ. Based on the variation patterns of the aerodynamic force and moment coefficients of the pantograph, a functional form $f({\lambda }_{wt},\cos \theta )$ can be obtained, thus providing the specific expression of the drag coefficient. By fitting the data, the values of the coefficients in the equation can be determined, leading to the final comprehensive relationship between the drag coefficient and the train speed, crosswind speed, and wind direction angle.

Let $f({\lambda }_{wt},\cos \theta )=a{\lambda }_{wt}^b\left(1+m{\lambda }_{wt}^c{\left|\cos \theta \right|}^n\right)$, substituting it into Equation (7), we can obtain the specific expression of the drag coefficient, as follows:

$$C_x={\displaystyle \frac{a{\lambda }_{wt}^b\left(1+m{\lambda }_{wt}^c{\left|\cos \theta \right|}^n\right)}{1+{\lambda }_{wt}^2+2{\lambda }_{wt}\cos \theta }}$$

(9)

In the above equation, a, b, c, m, and n are undetermined coefficients, which are solved through data fitting to obtain $a=0.779$, $b=0.128$, $c=1.267$, $m=1.951$, and $n=0.683$, thereby obtaining the drag coefficient, as follows:

$$C_x={\displaystyle \frac{0.779{\lambda }_{wt}^{0.128}\left(1+1.951{\lambda }_{wt}^{1.267}{\left|\cos \theta \right|}^{0.683}\right)}{1+{\lambda }_{wt}^2+2{\lambda }_{wt}\cos \theta }}$$

(10)

When the wind direction angle θ = 90°, the drag coefficient is $C_x=0.779{\lambda }_{wt}^{0.128}/(1+{\lambda }_{wt}^2)$; when the wind direction angle θ = 0°, the drag coefficient is $C_x=(0.779{\lambda }_{wt}^{0.128}+1.519{\lambda }_{wt}^{1.395})/(1+2{\lambda }_{wt}+{\lambda }_{wt}^2)$. The above equation considers the special conditions when the wind direction is 0° and 90°. For situations without crosswind (${\nu }_w=0$), a separate treatment is required. Similarly, the comprehensive relationship equations for the lift coefficient, lateral force coefficient, and aerodynamic coefficient with respect to vehicle speed, crosswind speed, and wind direction angle can be obtained.

Lift coefficient:

$$C_y={\displaystyle \frac{2.653{\lambda }_{wt}^{2.171}\left(1-3.063{\lambda }_{wt}^{1.170}|\cos \theta {|}^{1.506}\right)}{1+{\lambda }_{wt}^2+2{\lambda }_{wt}\cos \theta }}$$

(11)

Sideways force coefficient:

$$C_z={\displaystyle \frac{-0.087+0.975{\lambda }_{wt}-1.59{\lambda }_{wt}^2+2.097{\lambda }_{wt}^3+0.388\cos \theta -0.600{\cos }^2\theta +0.166{\cos }^3\theta }{1+{\lambda }_{wt}^2+2{\lambda }_{wt}\cos \theta }}$$

(12)

To analyze the aerodynamic lift forces acting on the components of the high-speed train pantograph, aerodynamic loads for the head, upper framework, and lower arm were obtained through numerical simulations. 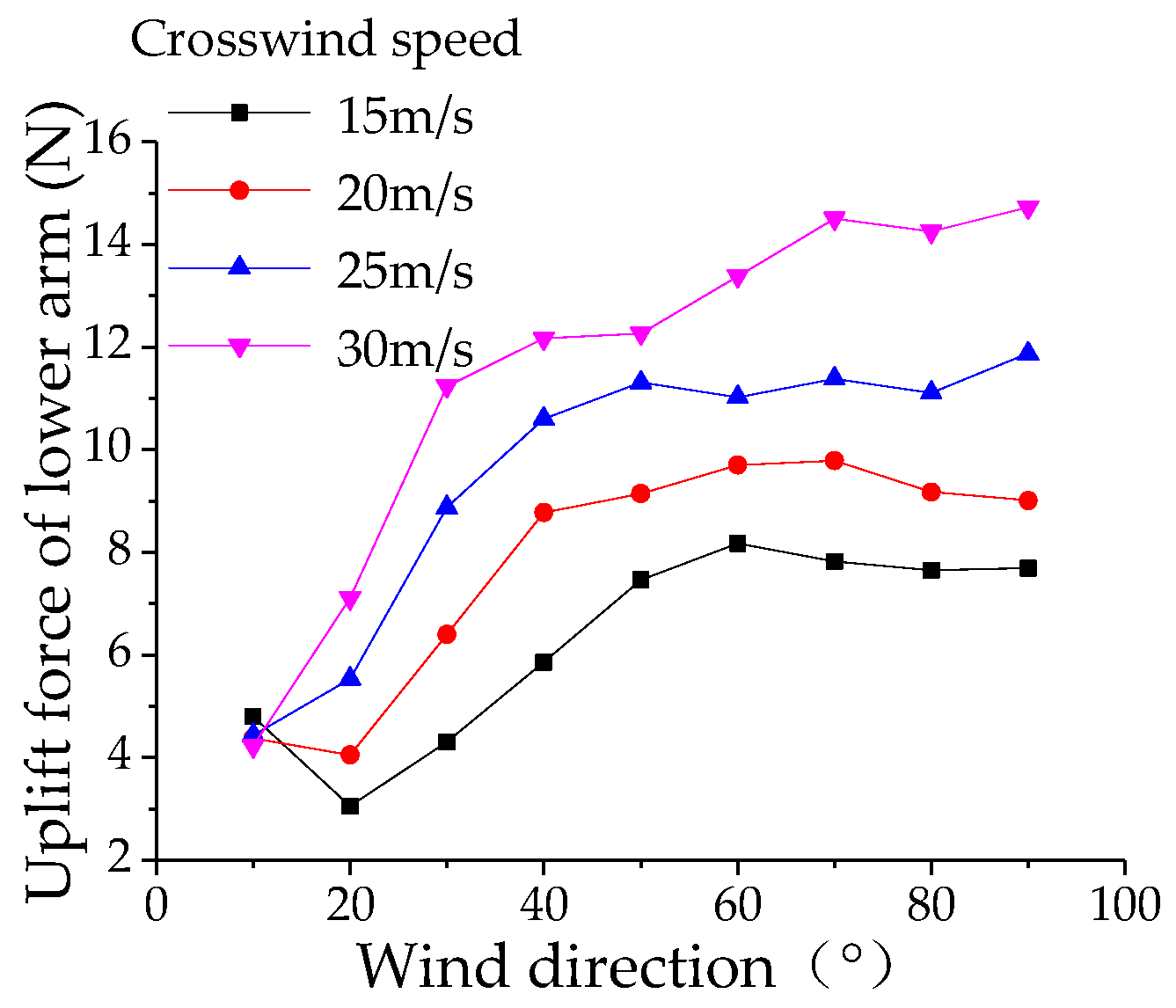The variation patterns of aerodynamic loads for the head are shown in Figure 17, Figure 18 and Figure 19, for the upper framework in Figure 20, Figure 21 and Figure 22, and for the lower arm in Figure 23, Figure 24 and Figure 25. Under the influence of crosswinds, with increasing wind speed and wind direction angle, the resistance, lateral force coefficients, overturning moment, and lateral moment coefficients of the head, upper framework, and lower arm exhibit consistent trends with those of the pantograph, but with values lower than those experienced by the pantograph under corresponding crosswind speeds and angles. Among these components, the head exhibits the highest resistance coefficient, indicating a clear direction for reducing drag on a per-component basis. Compared to the pantograph, the lift coefficient patterns for the head, upper framework, and lower arm show significant changes. Therefore, in the design and optimization process of the pantograph, special attention should be paid to the lift forces acting on the head, upper framework, and lower arm to prevent excessive aerodynamic lift from causing catenary accidents and excessive pitching moments from enhancing longitudinal section vibrations of the pantograph, thereby affecting the stability of current collection from the overhead lines.

4.2. Aerodynamic Lift of the Main Components of the Pantograph

Analysis of the aerodynamic lift of the main components of the pantograph reveals the variation in the aerodynamic lift with the operating speed, crosswind speed, and wind direction angle of the pantograph. Figure 17, Figure 18, Figure 19, Figure 20, Figure 21, Figure 22, Figure 23, Figure 24 and Figure 25 depict the variation in aerodynamic lift of the bow collector, upper frame, and lower arm with the wind direction angle, where the operating speed of the pantograph is 97.22 m/s and the wind direction angle ranges from 10° to 90°. Figure 26 illustrates the variation in the aerodynamic lift of the bow collector with the vehicle speed and crosswind speed at a wind direction angle of 90°. The aerodynamic lift of the bow collector gradually decreases with an increase in the wind direction angle. When the wind direction angle reaches 60° and the crosswind speed is 30 m/s, the aerodynamic lift of the bow collector sharply decreases and becomes lower than that for the crosswind speeds of 15, 20, and 25 m/s. This indicates that the conditions of maximum crosswind speed and wind direction angle do not necessarily result in the maximum aerodynamic lift.

Figure 17. Curve of variation in bow head drag coefficient.

Figure 17. Curve of variation in bow head drag coefficient.

Figure 17 shows the variation in the drag coefficient with wind direction angle. As the wind direction angle increases, the drag coefficient rises due to the increased surface area exposed to the crosswind, leading to higher aerodynamic resistance.

Figure 18. Curve of variation in bow head lift coefficient.

Figure 18. Curve of variation in bow head lift coefficient.

Figure 18 illustrates the lift coefficient’s behavior with changing wind direction. The lift coefficient decreases as the wind direction angle increases because the crosswind reduces the vertical upward force acting on the bow head. At higher angles, the airflow around the bow head becomes less efficient at generating lift.

Figure 19 shows the lateral force coefficient increasing with wind direction. The increase occurs because, as the wind angle widens, a larger portion of the wind force is directed horizontally, generating greater lateral force, which could affect the stability of the pantograph.

Figure 19. Curve of variation in bow head lateral force coefficient.

Figure 19. Curve of variation in bow head lateral force coefficient.

Figure 20 demonstrates how the drag coefficient for the upper frame increases with wind direction. Although similar to the bow head, the magnitude of drag is smaller due to the upper frame’s smaller frontal area and different geometry, which results in less aerodynamic drag.

Figure 21 presents the lift coefficient for the upper frame, which decreases with the increasing wind direction angle. This reduction in lift is primarily due to the weakening of the vertical airflow component acting on the upper frame at higher wind angles.

Figure 20. Curve of variation in upper frame drag coefficient.

Figure 20. Curve of variation in upper frame drag coefficient.

Figure 21. Curve of variation in upper frame lift coefficient.

Figure 21. Curve of variation in upper frame lift coefficient.

Figure 22. Curve of variation in upper frame lateral force coefficient.

Figure 22. Curve of variation in upper frame lateral force coefficient.

Figure 22 highlights the lateral force coefficient’s increase with wind direction angle. Like the bow head, the upper frame also experiences higher lateral forces at greater wind angles due to the shift of wind pressure from vertical to horizontal components. However, the upper frame generates less lateral force than the bow head due to its smaller surface area.

Figure 23. Curve of variation in lower arm rod drag coefficient.

Figure 23. Curve of variation in lower arm rod drag coefficient.

Figure 23 shows the drag coefficient for the lower arm rod increasing with wind direction angle, though at a lower rate than the bow head or upper frame. This is because the lower arm rod is smaller and positioned lower, leading to less aerodynamic interaction with the crosswind.

Figure 24. Curve of variation in lower arm rod Lift coefficient.

Figure 24. Curve of variation in lower arm rod Lift coefficient.

Figure 24 illustrates the lift coefficient for the lower arm rod, which decreases with wind direction angle. The lower arm rod plays a minimal role in generating lift, as its design is more focused on structural support rather than aerodynamic efficiency.

Figure 25. Curve of variation in lower arm rod lateral force coefficient.

Figure 25. Curve of variation in lower arm rod lateral force coefficient.

Figure 25 shows the lateral force coefficient increasing with wind direction angle, but remaining the smallest among all the pantograph components. This is because the lower arm rod, positioned low on the pantograph, encounters less wind exposure, resulting in reduced lateral forces.

Figure 26. Aerodynamic lift of the bow section.

Figure 26. Aerodynamic lift of the bow section.

Figure 26 illustrates how the aerodynamic lift of the bow section changes with the crosswind speed and wind direction angle. The lift decreases significantly at wind angles of 60° and above, particularly at higher crosswind speeds (30 m/s), because, at these angles, the airflow’s vertical component diminishes, reducing the bow section’s ability to generate lift. The sharp drop in lift at high angles suggests a critical threshold where the bow section can no longer maintain efficient aerodynamic performance.

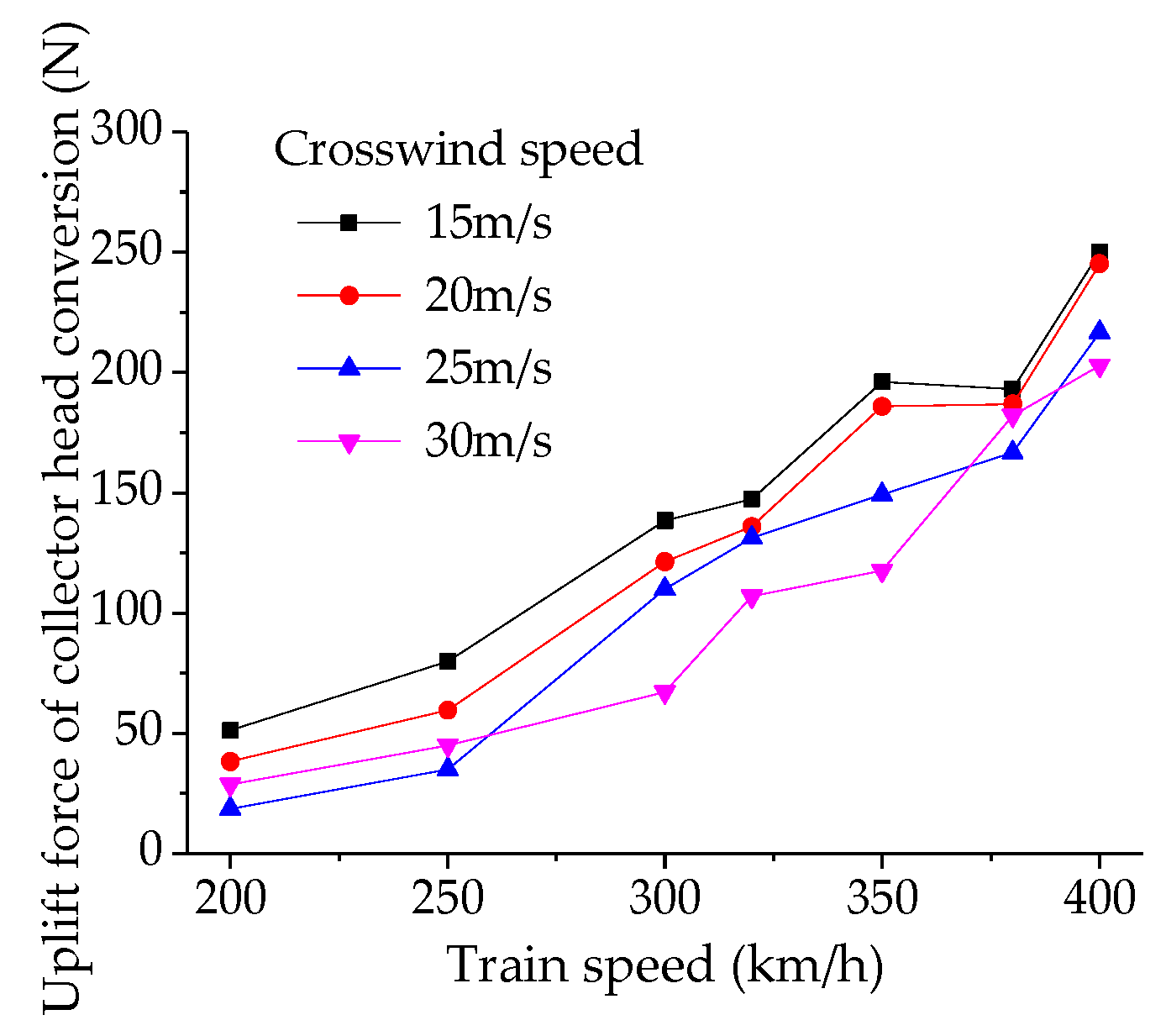
Figure 27, Figure 28 and Figure 29 illustrate the variation in the aerodynamic lift of the bow collector, upper frame, and lower arm with the operating speed of the pantograph. The operating speed ranges from 200 km/h to 400 km/h, crosswind speeds range from 15 m/s to 30 m/s, and the wind direction angle is 90°. The aerodynamic lift of the bow collector increases with the operating speed of the pantograph. Under conditions where the wind direction angle is 90°, the aerodynamic lift of the bow collector reaches its maximum value when the pantograph operating speed is 400 km/h at crosswind speeds of 15 m/s and 20 m/s. The aerodynamic lift of the upper frame decreases linearly with an increase in the operating speed of the pantograph, with little influence from the crosswind speed. As the crosswind speed increases, the influence of the operating speed of the pantograph on the aerodynamic lift of the lower arm gradually decreases, highlighting the importance of considering the aerodynamic lift of the bow collector.

Figure 27. Aerodynamic lift of the upper framework.

Figure 27. Aerodynamic lift of the upper framework.

Figure 27 shows that the aerodynamic lift of the bow section increases with the pantograph’s operating speed. This is because higher speeds intensify the airflow around the bow section, leading to greater upward aerodynamic forces. At 400 km/h, the lift reaches its maximum, indicating that the bow section is highly sensitive to increases in train speed.

Figure 28. Aerodynamic lift of the lower arm rod.

Figure 28. Aerodynamic lift of the lower arm rod.

Figure 28 demonstrates that the aerodynamic lift of the upper frame decreases linearly as operating speed increases. This is due to the negative lift forces acting on the upper frame, which become more pronounced at higher speeds as the airflow around the frame stabilizes.

Figure 29. Aerodynamic lift of the bowhead.

Figure 29. Aerodynamic lift of the bowhead.

Figure 29 presents the aerodynamic lift of the lower arm rod, which decreases as the crosswind speed increases. The lower arm rod experiences less aerodynamic lift compared to the bow head and upper frame, and its contribution to the overall lift diminishes further as the crosswind disrupts airflow patterns around the pantograph.

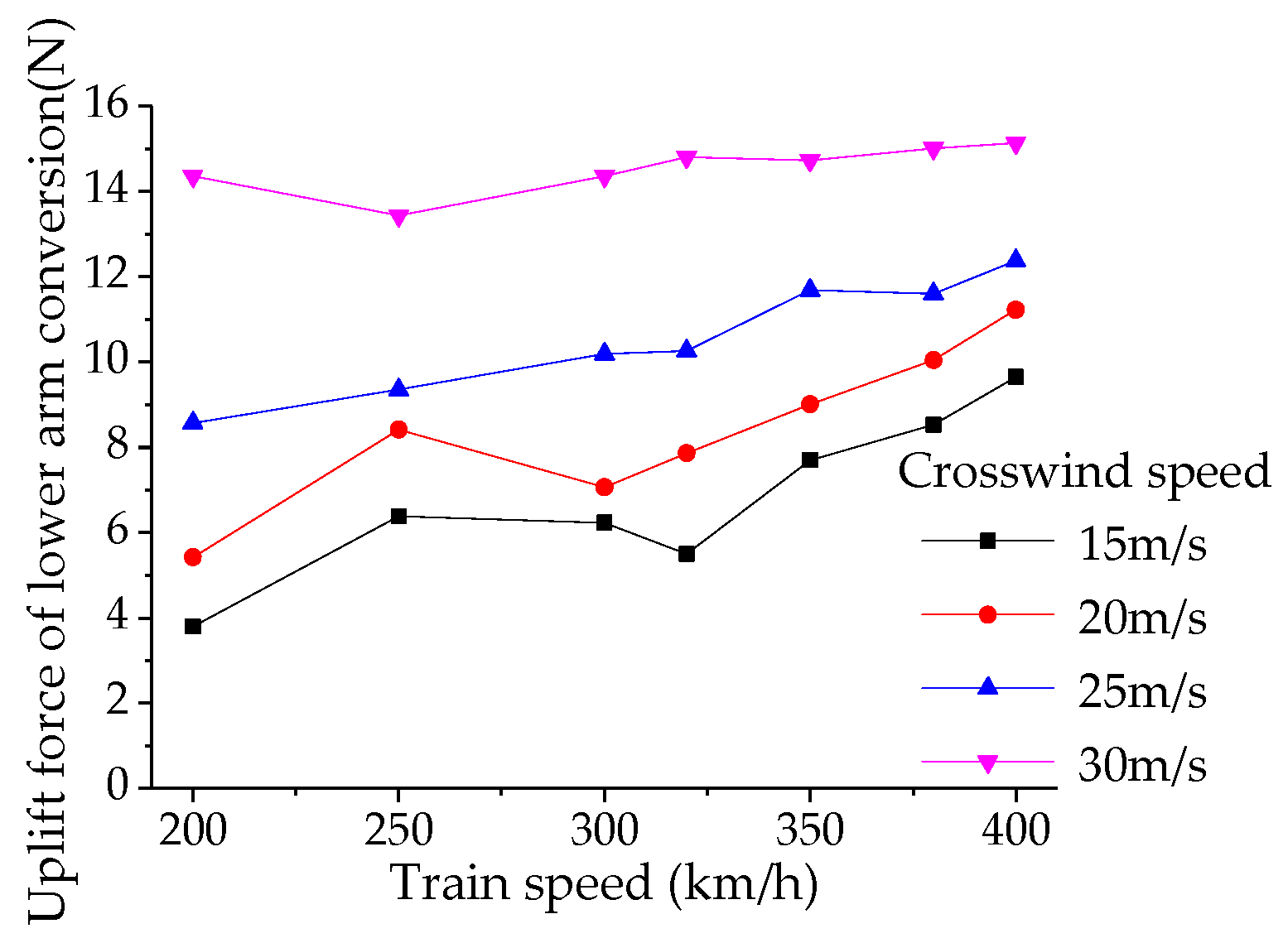
Figure 30, Figure 31 and Figure 32 show the variation in the aerodynamic lift of the bow collector, upper frame, and lower arm with the operating speed of the pantograph, where the operating speed ranges from 200 km/h to 400 km/h, the crosswind speed ranges from 15 m/s to 30 m/s, and the wind direction angle is 90°. The aerodynamic lift of the bow collector increases with the operating speed of the pantograph, reaching its maximum value when the pantograph operating speed is 400 km/h at crosswind speeds of 15 m/s and 20 m/s in conditions where the wind direction angle is 90°. The aerodynamic lift of the upper frame decreases linearly with an increase in the operating speed of the pantograph, with little influence from the crosswind speed. As the crosswind speed increases, the influence of the operating speed of the pantograph on the aerodynamic lift of the lower arm gradually decreases, highlighting the importance of considering the aerodynamic lift of the bow collector.

Figure 30 shows that the aerodynamic lift of the upper frame decreases as the pantograph speed increases. This is because higher train speeds stabilize the airflow around the upper frame, reducing any upward lift forces.

Figure 31. Lift of the lower arm rod.

Figure 31. Lift of the lower arm rod.

Figure 31 demonstrates that the aerodynamic lift of the lower arm decreases with increasing crosswind speed, as higher crosswinds reduce the vertical aerodynamic forces acting on the lower arm.

Figure 32. Lift force of the bowhead.

Figure 32. Lift force of the bowhead.

Figure 32 shows that the aerodynamic lift of the bow head increases with pantograph speed. At higher speeds, the bow head generates stronger lift forces due to the intensified airflow, especially at a wind direction angle of 90°, where the effect of speed is most significant.

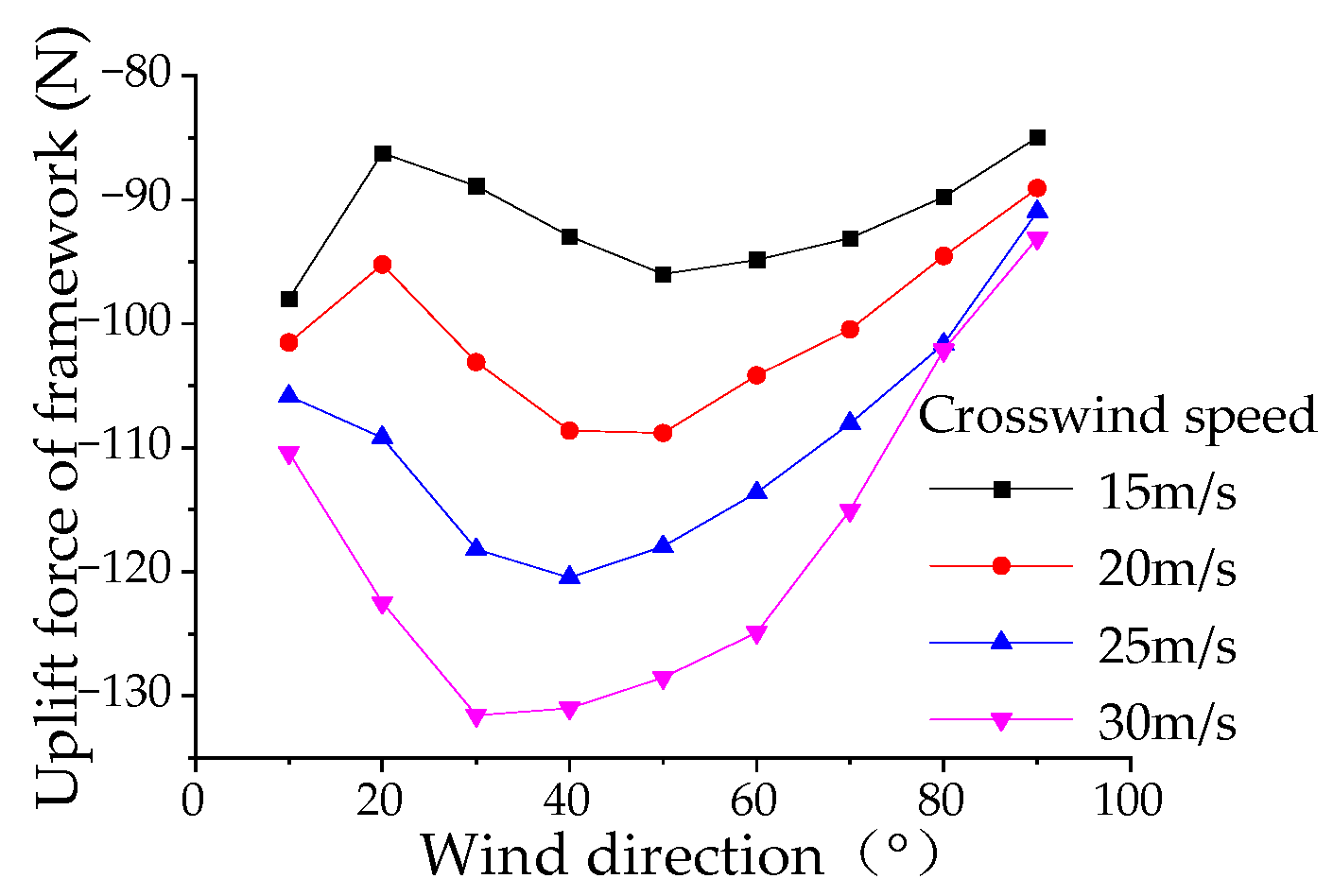
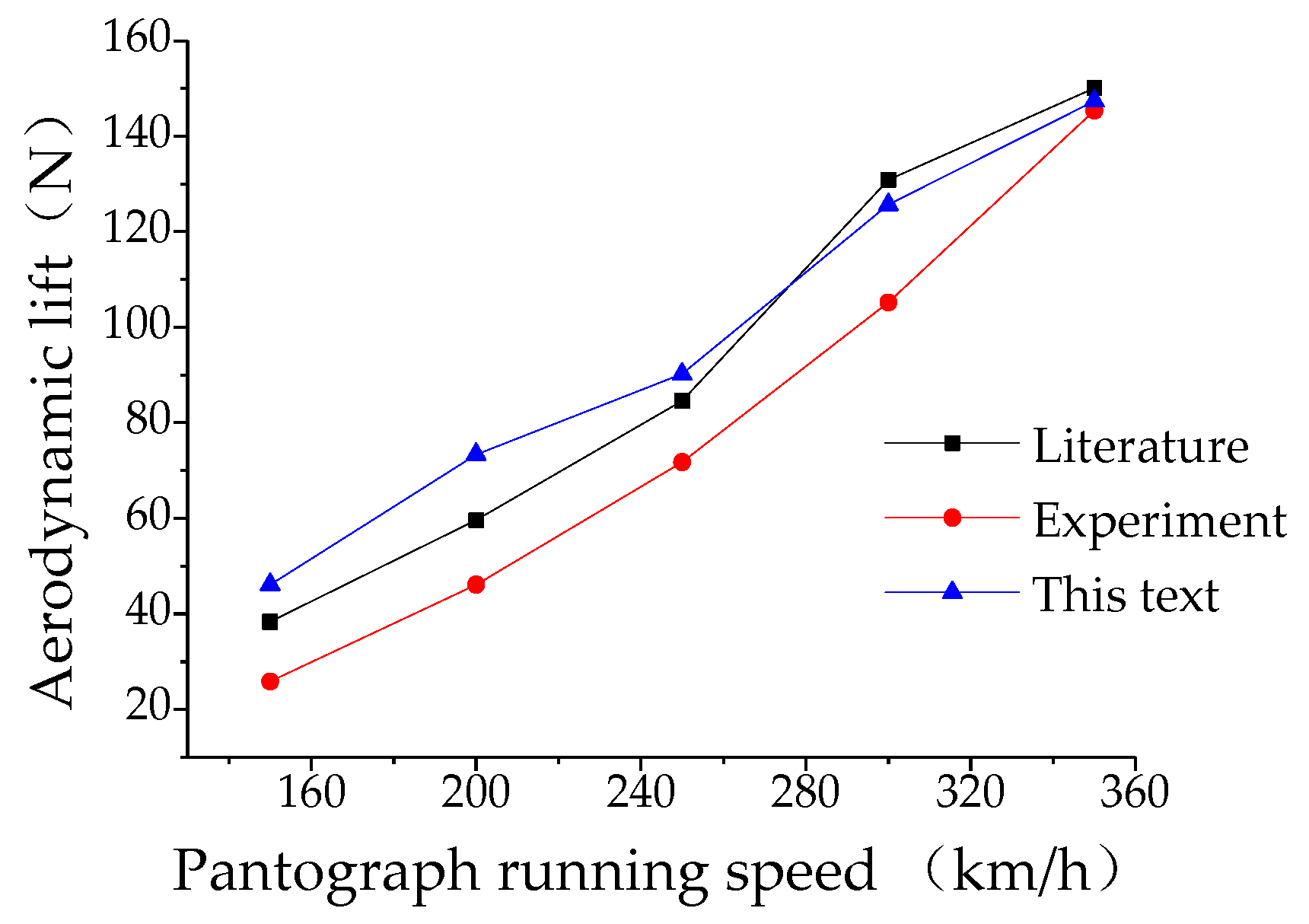
From the analysis of the aerodynamic lift of the main components of the pantograph, it can be observed that the aerodynamic lift of the bow collector head is the largest, while the absolute value of the aerodynamic lift of the lower arm is the smallest. Additionally, the aerodynamic lift of the upper arm is consistently negative, which conforms to the flow characteristics of the pantograph’s collector head, upper arm, and lower arm in the unsteady external flow field. The running speed of the pantograph significantly affects the collector head and upper frame, while the crosswind speed has a greater impact on the lower arm and minimal effect on the aerodynamic lift of the upper frame. The combined influence of the running speed and crosswind speed, with a certain yaw angle (or crosswind direction angle), acts on the main components of the pantograph, determining the variation in the aerodynamic forces and torques based on the flow characteristics of each component, thereby determining the distribution of aerodynamic lift.

4.3. Analysis of Pantograph Aerodynamic Lift

The pantograph, composed of various components, forms a structurally complex three-dimensional geometry. Under aerodynamic forces, each component of the pantograph generates vertical constraint forces, known as the pantograph aerodynamic lift. Figure 33, Figure 34 and Figure 35 illustrate the variation in the pantograph aerodynamic lift with wind direction, train operating speed, and crosswind speed, with wind direction ranging from 10° to 90°, train speed (vehicle speed) from 200 km/h to 400 km/h, and crosswind speed from 15 m/s to 30 m/s. When the train speed is 350 km/h, the pantograph aerodynamic lift decreases with the increasing wind direction angle. Within the range of wind direction angles from 10° to 50°, there is a rapid decrease in lift when the wind direction angle reaches 50° under a crosswind speed of 30 m/s. The slopes of the curves increase with increasing crosswind speed, and the variation pattern of pantograph lift changes significantly when the wind direction angle reaches 50°. The minimum aerodynamic lift occurs in the condition with a wind direction angle of 90° and a crosswind speed of 30 m/s. The aerodynamic lift of the pantograph generally increases with the train speed. In conditions with crosswind speeds of 15 m/s, 20 m/s, and 25 m/s, there is a significant inflection point in pantograph aerodynamic lift when the train speed reaches 380 km/h, while this phenomenon is not observed in conditions with a crosswind speed of 30 m/s.

Figure 33 indicates that the pantograph lift force decreases sharply with increasing wind direction angle, especially between 10° and 50°. This occurs because, at higher wind angles, the vertical component of the crosswind reduces the upward aerodynamic forces acting on the pantograph, leading to a drop in lift.

Figure 34. Lift force of the lower arm rod.

Figure 34. Lift force of the lower arm rod.

Figure 34 shows that pantograph lift force increases with train speed. As train speed rises, the airflow over the pantograph becomes stronger, generating higher aerodynamic lift forces.

Figure 35. Pantograph lift force.

Figure 35. Pantograph lift force.

Figure 35 shows that the pantograph lift force decreases significantly with increasing crosswind speed. At high crosswind speeds (30 m/s), the lift force reaches its minimum, as the crosswind disrupts the pantograph’s ability to generate upward lift, reducing contact stability with the overhead lines.

Figure 36 demonstrates that pantograph lift force peaks at high vehicle speeds, especially around 380 km/h. This is because faster speeds increase the dynamic pressure on the pantograph, resulting in greater lift forces.

Figure 37 shows that the pantograph lift force decreases as crosswind speed increases. High crosswinds reduce the vertical lift forces acting on the pantograph, potentially causing performance issues such as insufficient contact with the overhead lines.

Figure 36. Pantograph lift force (vehicle speed).

Figure 36. Pantograph lift force (vehicle speed).

Figure 37. Pantograph lift force (crosswind).

Figure 37. Pantograph lift force (crosswind).

The aerodynamic uplift force of the pantograph gradually decreases with the increase in crosswind speed. In operating conditions where the pantograph speeds are 200, 250, 300, 320, 350, 380, and 400 km/h, the aerodynamic uplift force of the pantograph decreases by 96.7%, 93.9%, 81.4%, 41.1%, 31.5%, 24.0%, and 35.9%, respectively, with the increase in crosswind speed. It can be observed that as the pantograph operating speed increases, the influence of crosswind speed gradually decreases.

5. Comprehensive Analysis of Pantograph Uplift Force

In order to comprehensively analyze the factors affecting the aerodynamic lifting force of the pantograph, we derive a comprehensive relationship between the lifting force, train speed, crosswind speed, and wind direction angle based on the calculated values obtained in this section. Here, we define the ratio of crosswind speed $V_w$ to train speed $V_{\mathrm{t}}$ as the wind speed to velocity ratio ${\lambda }_{wt}$, and represent the influence of wind direction angle $\theta$ using $\cos \theta$. Through a data-fitting process, we obtain the functional form of the comprehensive formula for aerodynamic lifting force, train speed, wind speed, and wind direction angle. Thus, we ultimately derive the comprehensive relationship between the pantograph aerodynamic lifting force $F_{\mathrm{e}}$ and the wind speed to velocity ratio ${\lambda }_{wt}$, as well as the wind direction angle $\theta$.

$$F_{\mathrm{e}}=20.216\times {\lambda }_{wt}^{0.787}\left(1+22.151{\lambda }_{wt}^{-1.660}{\left|\cos \theta \right|}^{0.933}\right)$$

(13)

Without considering the effect of crosswind, the relative air density $p_v$ can be obtained through $p_v=0.00097V^2+70$ Taking into account the influence of train speed, crosswind speed, and wind direction angle, the aerodynamic uplift force of the pantograph is no longer a simple quadratic function of the cosine of the wind speed-to-vehicle speed ratio cosθ. Therefore, using the above formula to calculate the contact force of the pantograph is inaccurate. Empirical calculations suggest that by considering the vector sum of train speed and crosswind speed ($V=\overrightarrow{V_t}+\overrightarrow{V_w}=\sqrt{V_t^2+V_w^2+2V_t{V}_w\cos \theta }$), the calculated values using this formula have errors within 10% when the train speed is 350 km/h and crosswind speed is within 20 m/s. However, when the crosswind speed increases to 25 m/s and 30 m/s, the formula deviates from the real situation. In the calculation of the aerodynamic uplift force of the pantograph, the transmission coefficient is determined by establishing the balance equation based on the geometric relationship of each component of the pantograph and force analysis. Since aerodynamic forces include the influence of crosswind, under the effect of crosswind, the uplift force of the pantograph should be determined by the following equation:

$$F_m=F_e+70=20.216\times {\lambda }_{wt}^{0.787}\left(1+22.151{\lambda }_{wt}^{-1.660}{\left|\cos \theta \right|}^{0.933}\right)+70$$

(14)

Figure 38 clearly outlines the process from analyzing the main factors influencing pantograph uplift force, to detailed investigations of each sub-factor, culminating in a final synthesis and design recommendations for optimizing performance.

6. Discussion

Pantographs are key factors in current collection in high-speed railways, but oscillations from wire interactions can hinder performance, as highlighted in the T2006 prototype study [20]. Active suspension systems have been proposed to optimize pantograph dynamics. In this study, it builds on such efforts by analyzing aerodynamic forces (drag, lift, and lateral force coefficients) under various crosswind and train speeds to enhance pantograph stability and performance. Extensive research has been conducted both domestically and internationally on the aerodynamic characteristics and behavior of pantographs under various conditions, establishing a robust foundation for comparative analysis with the results of the present study. Xiao, C [21] identified that the aerodynamic lift acting on the pantograph is predominantly concentrated on the head structure. Building upon this finding, in the current research, the variation in aerodynamic lift as a function of crosswind angles is investigated, providing critical insights for the design and operational safety of high-speed trains. Li, X [18] demonstrated that the operating speed and wind angle significantly influence the aerodynamic lift on the primary components of the pantograph. In this study, their work is extended by deriving a comprehensive formula that correlates aerodynamic lift with variables such as operating speed, crosswind speed, and wind angle. Additionally, Abbas-Bayoumi [22] employed both empirical and numerical methods to predict key aspects of aerodynamic behavior. Building on their methodology, in the present study, a relationship is formulated between pantograph aerodynamic lift, pantograph operating speed $V_t$, crosswind speed $V_w$, and wind angle $\theta$, while optimizing the empirical formula for pantograph contact force and train operating speed. Through a comparative analysis of these key findings with the existing literature, this research not only affirms the consistency of the results but also underscores significant advancements and contributions in the field.

However, while a detailed model of pantograph uplift is successfully developed in this study, there remain several areas that require further research. Future work should focus on the effects of transient wind conditions and turbulence on pantograph uplift, as real-world conditions often involve rapid shifts in wind speed and direction. Moreover, more extensive experimental validation, particularly at higher train speeds and more extreme crosswind conditions, will be crucial for refining the accuracy of the numerical model.

7. Conclusions

(1) The aerodynamic uplift force of the pantograph is primarily distributed on the pantograph head structure, where the aerodynamic uplift forces on the upper framework are all negative, while the absolute values of the aerodynamic uplift forces on the lower arm rods are the smallest. The aerodynamic uplift force on the pantograph head gradually decreases with increasing wind direction angle. Within the range of wind directions from 10° to 50°, under the same crosswind angle, the aerodynamic uplift force on the pantograph head increases with increasing crosswind speed. However, when the wind direction angle reaches 60° and the crosswind speed is 30 m/s, the aerodynamic uplift force on the pantograph head sharply decreases. Additionally, the aerodynamic uplift force of the pantograph gradually increases with the increasing pantograph operating speed or crosswind speed.

This result indicates that the bow collector should be reinforced in pantograph designs to withstand high uplift forces, especially during high-speed operations in windy conditions. On the other hand, the negative lift on the upper frame could be exploited to stabilize the pantograph and reduce the overall aerodynamic load. Design optimization should focus on improving the structural strength of the bow collector while possibly streamlining the upper frame to minimize negative lift-induced instability.

(2) The operating speed of the pantograph and the wind direction angle have a significant impact on the aerodynamic uplift force of the main components, while the crosswind speed has a relatively minor effect on both the pantograph and main components’ aerodynamic uplift forces. When the wind direction angle is small or the pantograph operating speed is high, the aerodynamic uplift force reaches higher values, increasing the likelihood of pantograph catenary contact and even bow scraping accidents. Conversely, in conditions with high crosswind speeds and low pantograph operating speeds, the pantograph is prone to experiencing insufficient aerodynamic uplift force, leading to arcing and disconnection phenomena.

This finding challenges the conventional design assumption that uplift forces always increase with wind speed. In practical terms, this suggests that pantograph designs need to account for specific wind angles and speeds rather than relying on linear aerodynamic models. For instance, safety protocols can be developed to monitor critical wind angles and speeds, allowing for dynamic adjustment or emergency braking in high-wind scenarios to prevent damage or detachment from the catenary.

(3) Through a comprehensive analysis of the factors influencing the aerodynamic uplift force of the pantograph, a relationship formula is derived that considers the aerodynamic uplift force of the pantograph in relation to the pantograph operating speed $V_t$, crosswind speed $V_w$, and wind direction angle $\theta$. Furthermore, adjustments are made to empirical formulas relating to pantograph contact force and train operating speed.

This formula provides a practical tool for railway engineers to forecast aerodynamic uplift forces under a wide range of operational conditions. It can be integrated into simulation software or used for real-time monitoring of pantograph performance during high-speed train operations, enabling engineers to make informed decisions about speed adjustments, pantograph positioning, and even maintenance schedules based on predicted aerodynamic loads.