Modeling of Coupled Structural Electromagnetic Statistical Concept for Examining Performance Sensitivity of Antenna Array to Distortion at Millimeter-Wave
Abstract
1. Introduction
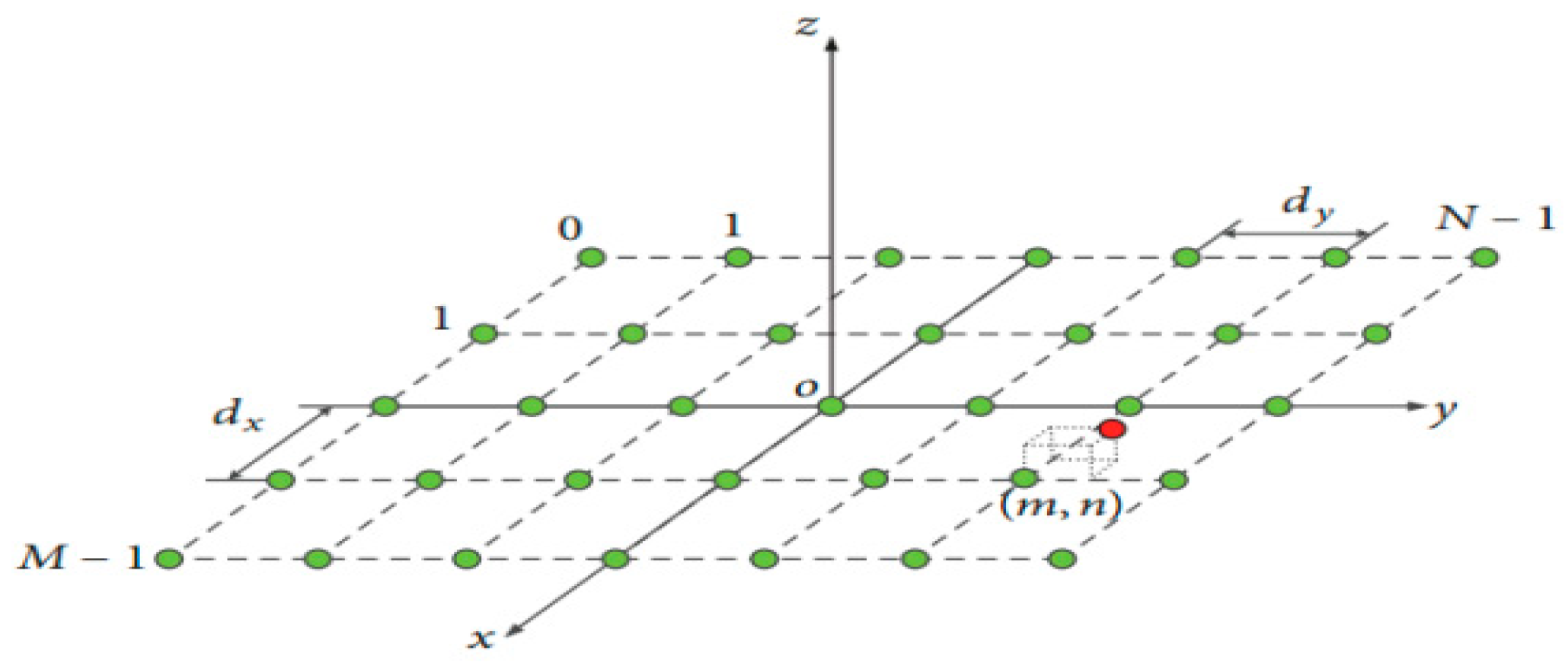
2. Proposed Coupled Structure–Electromagnetic Statistical Model
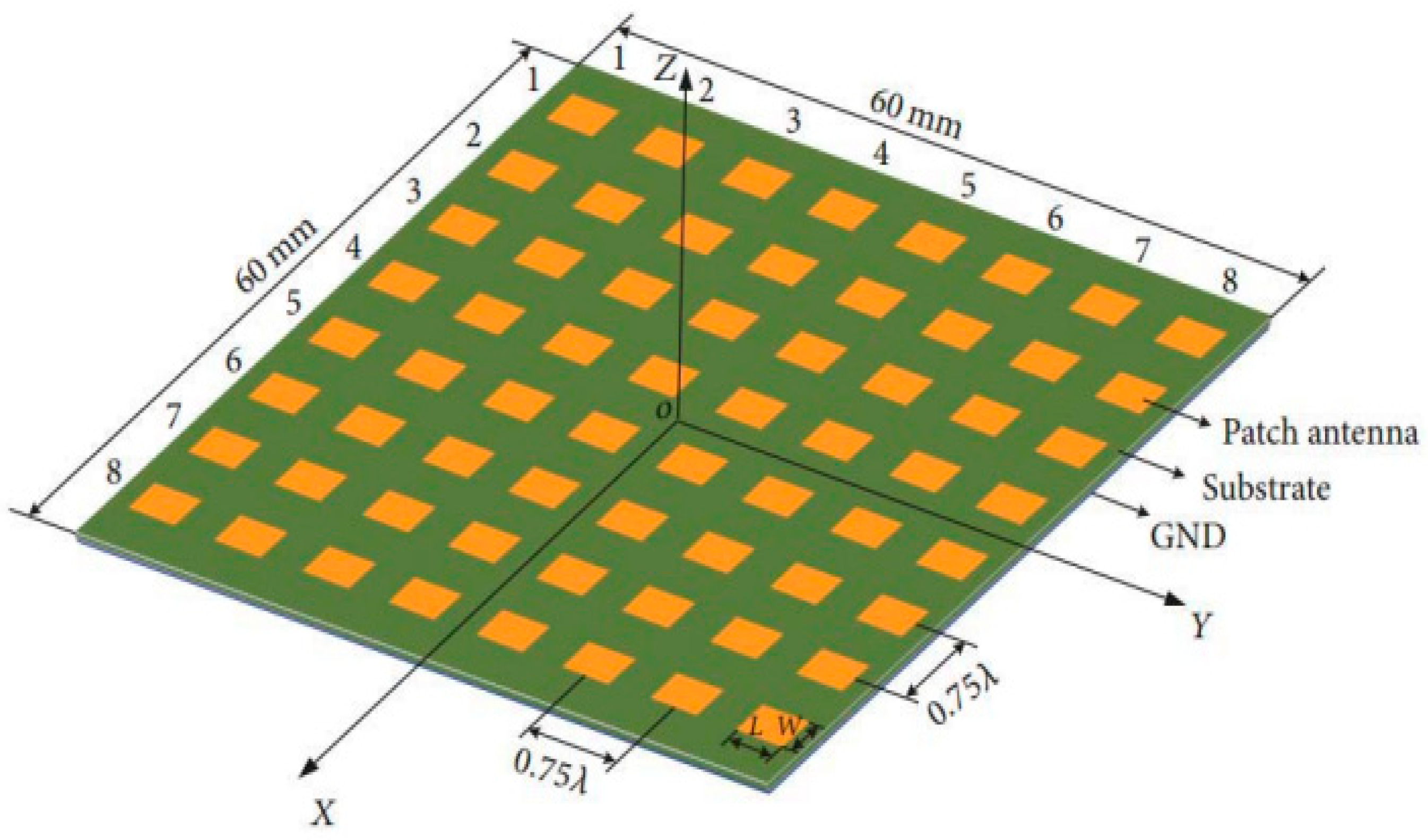
3. Coupled Structure–Electromagnetic Statistical Model Validation
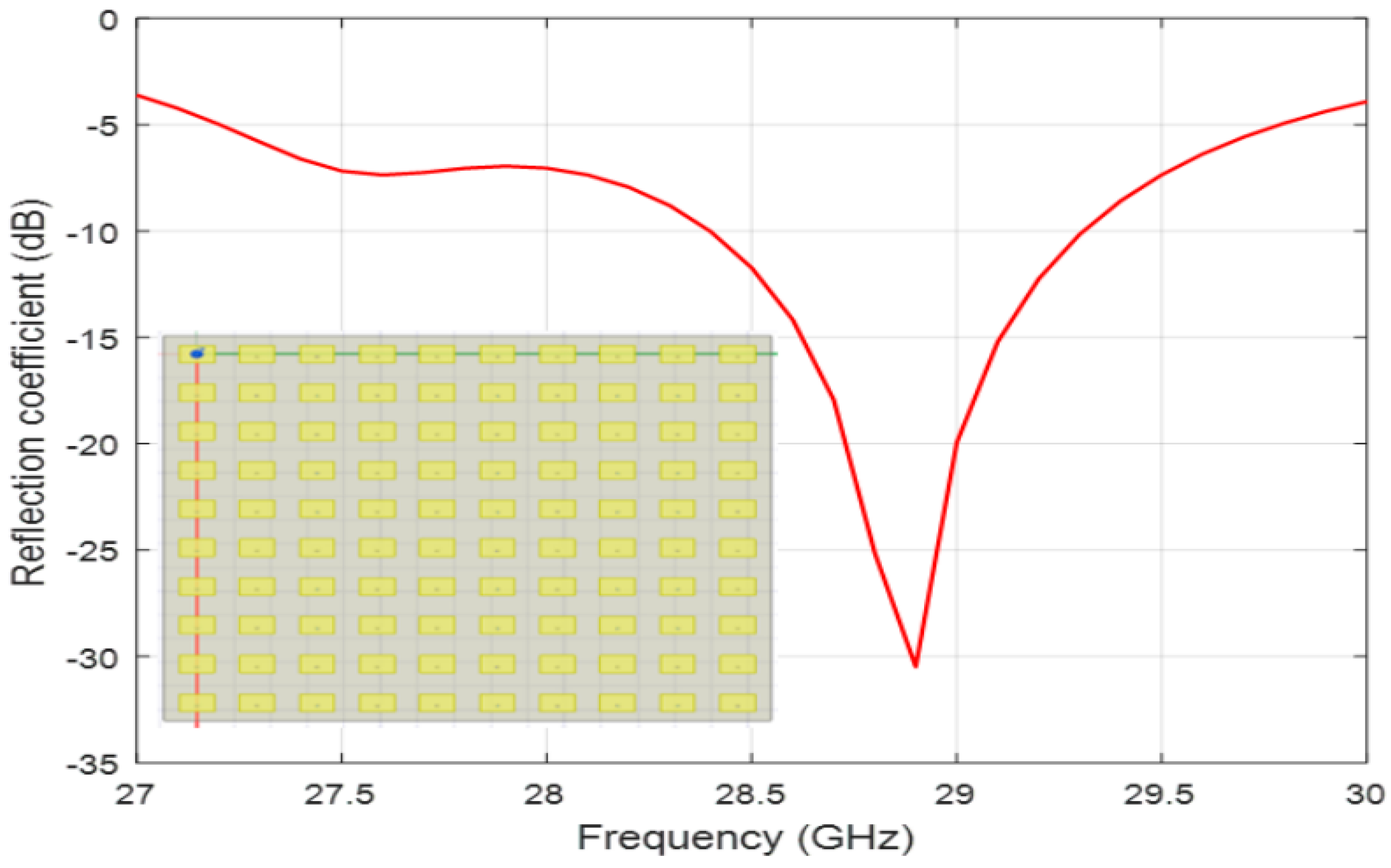
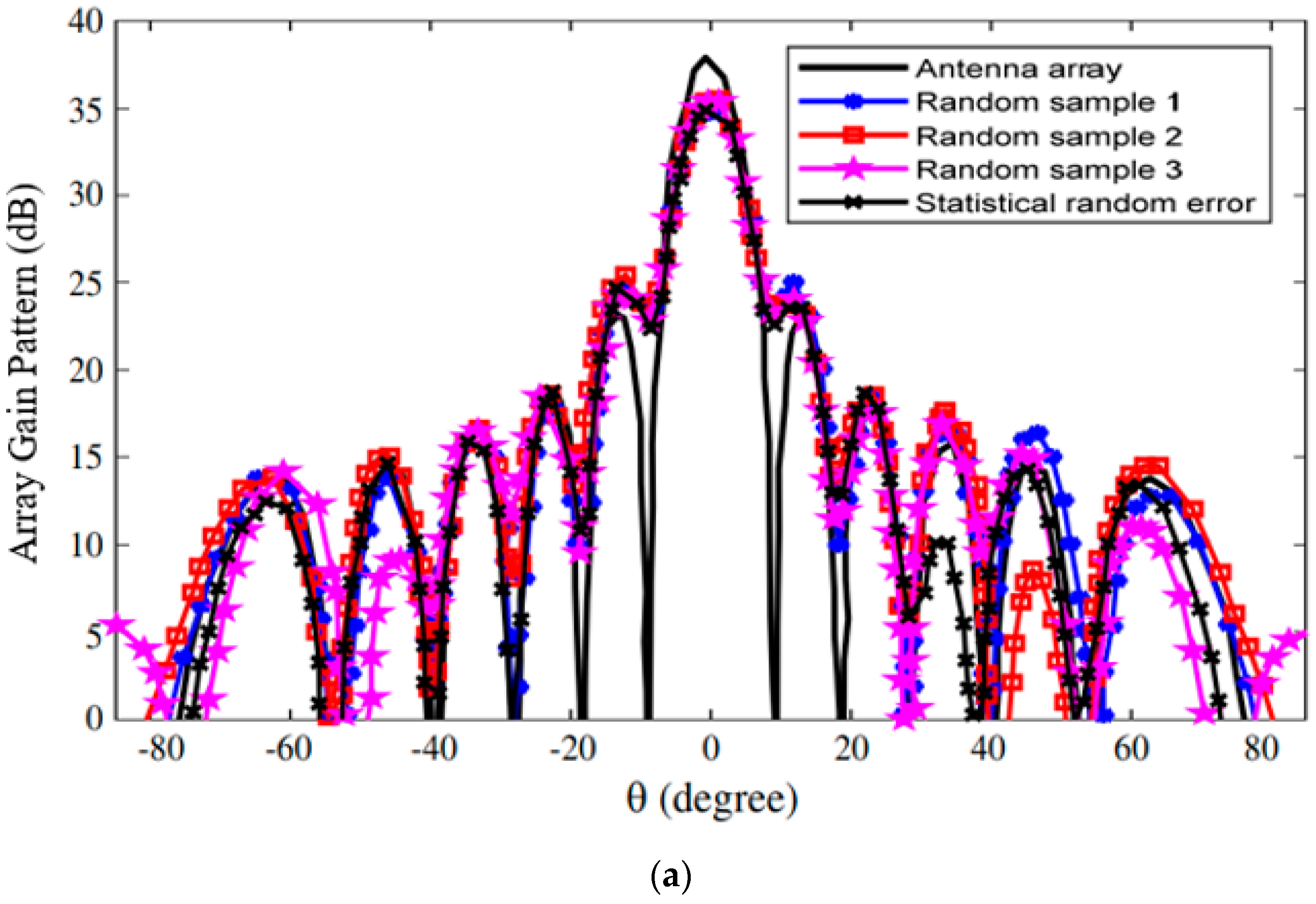
4. Simulation Example and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Haupt, R.L.; Rahmat-Samii, Y. Antenna array developments: A perspective on the past, present and future. IEEE Antennas Propag. Mag. 2015, 57, 86–96. [Google Scholar] [CrossRef]
- Farina, A.; Timmoneri, L. Phased array systems for air, land and naval defence applications in Selex ES. In Proceedings of the 8th European Conference on Antennas and Propagation (EuCAP), The Hague, The Netherlands, 6–11 April 2014; pp. 560–564. [Google Scholar]
- Famoriji, O.J.; Yang, S.; Li, Y.; Chen, W.; Fadamiro, A.; Zhang, Z.; Lin, F. Design of a simple circularly polarized dual-frequency reconfigurable microstrip patch antenna array for millimeter-wave applications. IET Microw. Antennas Propag. 2019, 13, 1671–1677. [Google Scholar] [CrossRef]
- Famoriji, O.J.; Xu, Z. Antenna feed array synthesis for effective communication system. IEEE Sens. J. 2020, 20, 15085–15098. [Google Scholar] [CrossRef]
- Zhou, J.; Song, Z.L.W.; Huang, J.; Wang, C. Performance of structurally integrated antennas subjected to dynamical loads. Int. J. Appl. Electromagn. Mech. 2015, 48, 409–422. [Google Scholar] [CrossRef]
- Famoriji, O.J.; Shongwe, T. Spherical Atomic Norm-Inspired Approach for Direction-of-Arrival Estimation of EM Waves Impinging on Spherical Antenna Array with Undefined Mutual Coupling. Appl. Sci. 2013, 13, 3067. [Google Scholar] [CrossRef]
- Sutinjo, A.; Hall, P. Antenna rotation error tolerance for a low-frequency aperture array polarimeter. IEEE Trans. Antennas Propag. 2014, 62, 3401–3406. [Google Scholar] [CrossRef]
- Oliveri, G.; Zardi, F.; Gottardi, G.; Massa, A. Optically-Transparent EM Skins for Outdoor-to-Indoor mm-Wave Wireless Communications. IEEE Access 2024, 12, 65178–65191. [Google Scholar] [CrossRef]
- Freni, A.; Beccaria, M.; Mazzinghi, A.; Massaccesi, A.; Pirinoli, P. Low-Profile and Low-Visual Impact Smart Electromagnetic Curved Passive Skins for Enhancing Connectivity in Urban Scenarios. Electronics 2023, 12, 4491. [Google Scholar] [CrossRef]
- Famoriji, O.J.; Akingbade, K.F.; Ogunti, E.O.; Apena, W.; Fadamiro, A.O.; Lin, F. Analysis of phased array antenna system via spherical harmonics decomposition. IET Commun. 2019, 13, 3097–3104. [Google Scholar] [CrossRef]
- Rocca, P.; Manica, L.; Anselmi, N.; Massa, A. Analysis of the pattern tolerances in linear arrays with arbitrary amplitude errors. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 639–642. [Google Scholar] [CrossRef]
- Duan, B.Y.; Wang, M. Multidisciplinary optimization of microwave antennas. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Boston, MA, USA, 8–11 April 2013. [Google Scholar]
- Laskar, J.; Pinel, S.; Sarkar, S.; Sen, P.; Perumana, B.; Dawn, D.; Leung, M.; Barale, F.; Yeh, D.; Shin, S.C.; et al. On the development of CMOS mmW and sub-THz phased array technology for communication/sensing nodes. In Proceedings of the 2010 IEEE MTT-S International Microwave Symposium, Anaheim, CA, USA, 23–28 May 2010; pp. 1312–1315. [Google Scholar]
- Zhang, Z.Y.; Li, S.; Zuo, S.L.; Zhao, J.Y.; Yang, X.-D.; Fu, G. Dual-polarized crossed bowtie dipole array for wireless communication applications. Int. J. Antennas Propag. 2014, 2014, 349516. [Google Scholar] [CrossRef]
- Famoriji, O.J.; Shongwe, T. Electromagnetic machine learning for estimation and mitigation of mutual coupling in strongly coupled arrays. ICT Express 2023, 9, 8–15. [Google Scholar] [CrossRef]
- Li, G.; Ai, B.; He, D.; Zhong, Z.; Hui, B.; Kim, J. On the feasibility of high speed railway mmWave channels in tunnel scenario. Wirel. Commun. Mob. Comput. 2017, 2017, 7135896. [Google Scholar] [CrossRef]
- Fadamiro, A.; Semomhe, A.; Famoriji, O.J.; Lin, F. A multiple element calibration algorithm for active phased array antenna. IEEE J. Multiscale Multiphys. 2019, 4, 163–170. [Google Scholar] [CrossRef]
- Kamoda, H.; Tsumochi, J.; Kuki, T.; Suginoshita, F. A study on antenna gain degradation due to digital phase shifter in phased array antennas. Microw. Opt. Technol. Lett. 2011, 53, 1743–1746. [Google Scholar] [CrossRef]
- Wang, C.; Wang, Y.; Wang, W.; Zhou, J.; Wang, M.; Wang, Z. Electromechanical coupling based influence of structural error on radiation and scattering performance of array antennas. IEEE Electron. Lett. 2017, 53, 904–906. [Google Scholar] [CrossRef]
- Famoriji, O.J.; Ogundepo, O.Y.; Qi, X. An intelligent deep learning-based direction-of-arrival estimation scheme using spherical antenna array with unknown mutual coupling. IEEE Access 2020, 8, 179259–179271. [Google Scholar] [CrossRef]
- Ruze, J. The effect of aperture errors on the antenna radiation pattern. Il Nuovo C. 1952, 9 (Suppl. S3), 364–380. [Google Scholar] [CrossRef]
- Rondinelli, L.A. Effects of random errors on the performance of antenna arrays of many elements. IRE Int. Conv. Rec. 1959, 7, 174–189. [Google Scholar]
- Elliott, R.E. Mechanical and electrical tolerances for two-dimensional scanning antenna arrays. IRE Trans. Antennas Propag. 1958, 6, 114–120. [Google Scholar] [CrossRef]
- Hsiao, J.K. Array sidelobes, error tolerance, gain and beamwidth. In Naval Research Lab Report; Naval Research Lab: Washington, DC, USA, 1984. [Google Scholar]
- Ossowska, A.; Kim, J.H.; Wiesbec, W.K. Influence of mechanical antenna distortions on the performance of the HRWS SAR system. In Proceedings of the 2007 IEEE International Geoscience 74 and Remote Sensing Symposium, Barcelona, Spain, 23–28 July 2007; pp. 2152–2155. [Google Scholar]
- Zaitsev, E.; Hofman, J. Phased array fatness effects on antenna system performance. In Proceedings of the 4th IEEE International Symposium on Phased Array Systems and Technology Array, Waltham, MA, USA, 12–15 October 2010; pp. 121–125. [Google Scholar]
- Wang, C.; Kang, M.; Wang, Y.; Wang, W.; Du, J. Coupled structural-electromagnetic modeling and analysis of hexagonal active phased array antennas with random errors. AEU Int. J. Electron. Commun. 2016, 70, 592–598. [Google Scholar] [CrossRef]
- Lange, M. Impact of statistical errors on active phased-array antenna performance. In Proceedings of the Military Communications Conference, Orlando, FL, USA, 29–31 October 2007; pp. 1–5. [Google Scholar]
- Schediwy, S.W.; Price, D.; Dulwich, F.; Mort, B. A quantitative analysis of how phase errors affect the beam quality of phased arrays. In Proceedings of the 4th IEEE International Symposium on Phased Array Systems and Technology, Waltham, MA, USA, 12–15 October 2010; pp. 256–260. [Google Scholar]
- Lanne, M. Design aspects and pattern prediction for phased arrays with subarray position errors. In Proceedings of the 4th IEEE International Symposium on Phased Array Systems and Technology, Waltham, MA, USA, 12–15 October 2010; pp. 440–446. [Google Scholar]
- Wang, C.; Kang, M.; Wang, W.; Duan, B.; Lin, L.; Ping, L. On the performance of array antennas with mechanical distortion errors considering element numbers. Int. J. Electron. 2017, 104, 462–484. [Google Scholar] [CrossRef]
- Kang, M.K.; Wang, Y.; Yin, L.; Wang, C.S.; Tang, B.F.; Zhong, J.F. Performance prediction for array antennas with element position error based on coupled structural-electromagnetic model. In Proceedings of the Fifth Asia International Symposium on Mechatronics, Guilin, China, 7–10 October 2015; pp. 34–38. [Google Scholar]
- Wang, C.; Wang, Y.; Yang, X.; Gao, W.; Jiang, C.; Zhang, L.Y.; Wang, M. Effect of randomness in element position on the performance of communication array antennas in internet of things. Wirel. Commun. Mob. Comput. 2018, 2018, 6492143. [Google Scholar] [CrossRef]
- Wang, C.S.; Duan, B.Y.; Zhang, F.S.; Zhu, M.B. Coupled structural-electromagnetic-thermal modelling and analysis of active phased array antennas. IET Microw. Antennas Propag. 2010, 4, 247–257. [Google Scholar] [CrossRef]
- Gao, X.; Ma, Q.; Gu, Z.; Cui, W.Y.; Liu, C.; Zhang, J.; Cui, T.J. Programmable surface plasmonic neural networks for microwave detection and processing. Nat. Electron. 2023, 6, 319–328. [Google Scholar] [CrossRef]
- Liu, C.; Ma, Q.; Luo, Z.J.; Hong, Q.R.; Xiao, Q.; Zhang, H.C.; Miao, L.; Yu, W.M.; Cheng, Q.; Li, L.; et al. A programmable diffractive deep neural network based on a digital-coding metasurface array. Nat. Electron. 2022, 5, 113–122. [Google Scholar] [CrossRef]


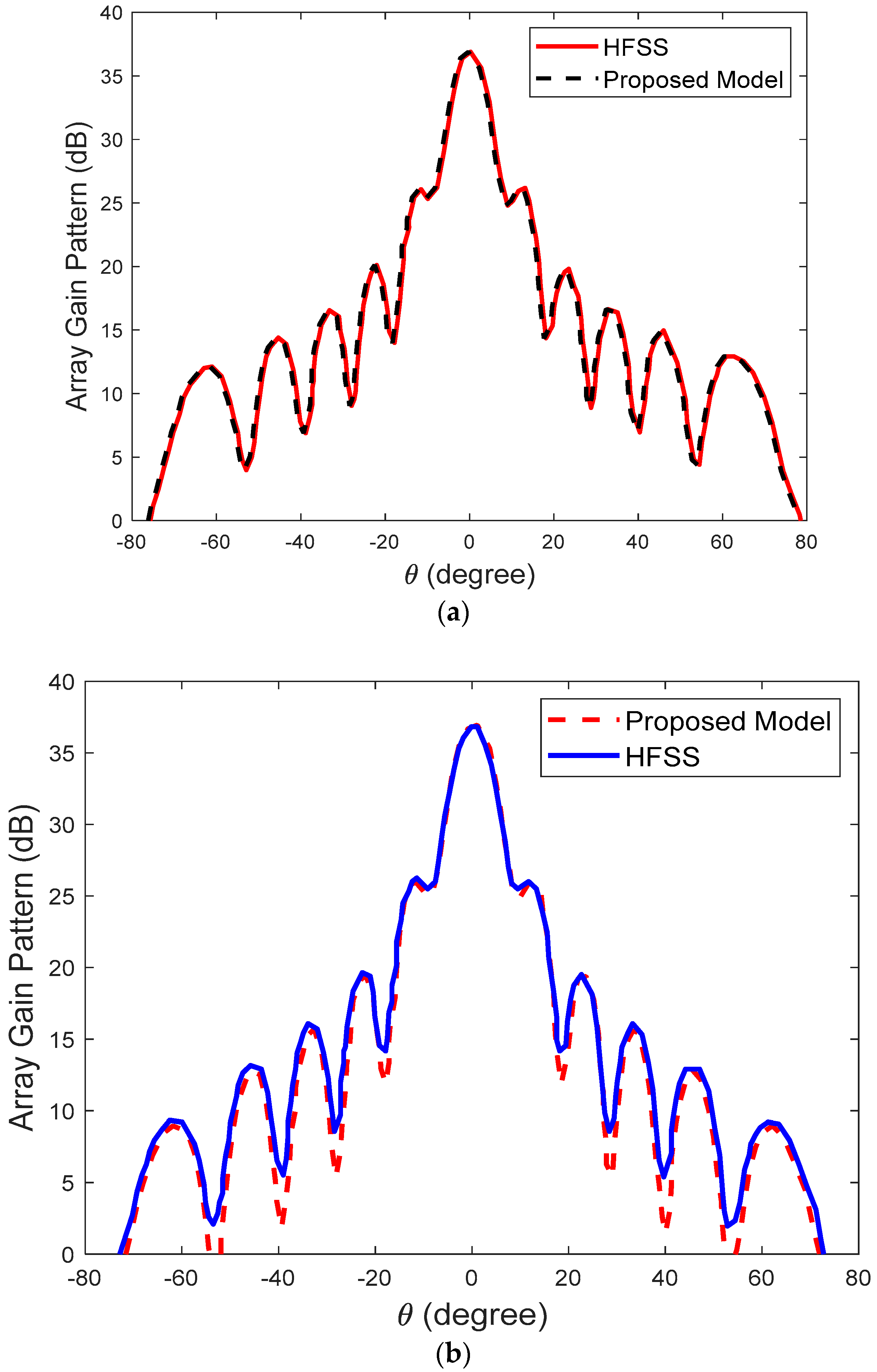

| Performance | Coupled Structure Model Result | HFSS-Based Result |
|---|---|---|
| Boresight pointing (°) = 0° = 90° | 0.14 0.12 | 0.14 0.12 |
| Beam width (°) = 0° = 90° | 8.70 8.69 | 8.70 8.69 |
| Gain (dB) | 61.32 | 61.32 |
| First SLL (dB) = 0° = 90° | 51.17 50.87 | 51.17 50.97 |
| Second SLL (dB) = 0° = 90° | 45.20 44.88 | 45.63 45.05 |
| Third SLL (dB) = 0° = 90° | 42.43 41.82 | 42.81 41.95 |
| Fourth SLL (dB) = 0° = 90° | 40.56 38.71 | 40.66 38.13 |
| Fifth SLL (dB) = 0° = 90° | 38.55 34.81 | 39.04 35.09 |
| Performance | Sample 1 | Sample 2 | Sample 3 | Statistical Random Error |
|---|---|---|---|---|
| Boresight pointing (°) ϕ = 0° ϕ = 90° | +0.09 −0.21 | +0.23 +0.23 | −0.22 +0.28 | +0.25 +0.31 |
| Beam width (°) ϕ = 0° ϕ = 90° | +0.12 +0.17 | +0.20 +0.30 | +0.23 +0.23 | +0.25 +0.33 |
| Gain loss (dB) | −1.99 | −2.44 | −1.78 | −2.14 |
| First SLL (dB) ϕ = 0° ϕ = 90° | +0.45 +0.53 | +0.31 +1.54 | +0.31 +1.96 | +0.03 +0.03 |
| Second SLL (dB) ϕ = 0° ϕ = 90° | −0.41 +0.82 | −1.22 −0.13 | −0.09 −1.52 | −0.64 −0.89 |
| Third SLL (dB) ϕ = 0° ϕ = 90° | +0.52 −1.31 | −3.82 +0.17 | +1.14 +0.57 | −0.41 −0.41 |
| Fourth SLL (dB) ϕ = 0° ϕ = 90° | +1.65 −0.81 | −0.23 +1.15 | −4.54 −0.43 | −0.24 −0.24 |
| Fifth SLL (dB) ϕ = 0° ϕ = 90° | −0.76 −2.72 | −0.43 −1.78 | +0.44 +1.12 | −0.11 −0.12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Famoriji, O.J.; Shongwe, T. Modeling of Coupled Structural Electromagnetic Statistical Concept for Examining Performance Sensitivity of Antenna Array to Distortion at Millimeter-Wave. Appl. Sci. 2024, 14, 7111. https://doi.org/10.3390/app14167111
Famoriji OJ, Shongwe T. Modeling of Coupled Structural Electromagnetic Statistical Concept for Examining Performance Sensitivity of Antenna Array to Distortion at Millimeter-Wave. Applied Sciences. 2024; 14(16):7111. https://doi.org/10.3390/app14167111
Chicago/Turabian StyleFamoriji, Oluwole John, and Thokozani Shongwe. 2024. "Modeling of Coupled Structural Electromagnetic Statistical Concept for Examining Performance Sensitivity of Antenna Array to Distortion at Millimeter-Wave" Applied Sciences 14, no. 16: 7111. https://doi.org/10.3390/app14167111
APA StyleFamoriji, O. J., & Shongwe, T. (2024). Modeling of Coupled Structural Electromagnetic Statistical Concept for Examining Performance Sensitivity of Antenna Array to Distortion at Millimeter-Wave. Applied Sciences, 14(16), 7111. https://doi.org/10.3390/app14167111






