Comparative Analysis of the Irradiation with Medium Fluences of High-Energy Electrons and Pr Doping on the Fluctuation Conductivity of YBa2Cu3O7–δ Single Crystals
Abstract
:1. Introduction
2. Experimental Methods
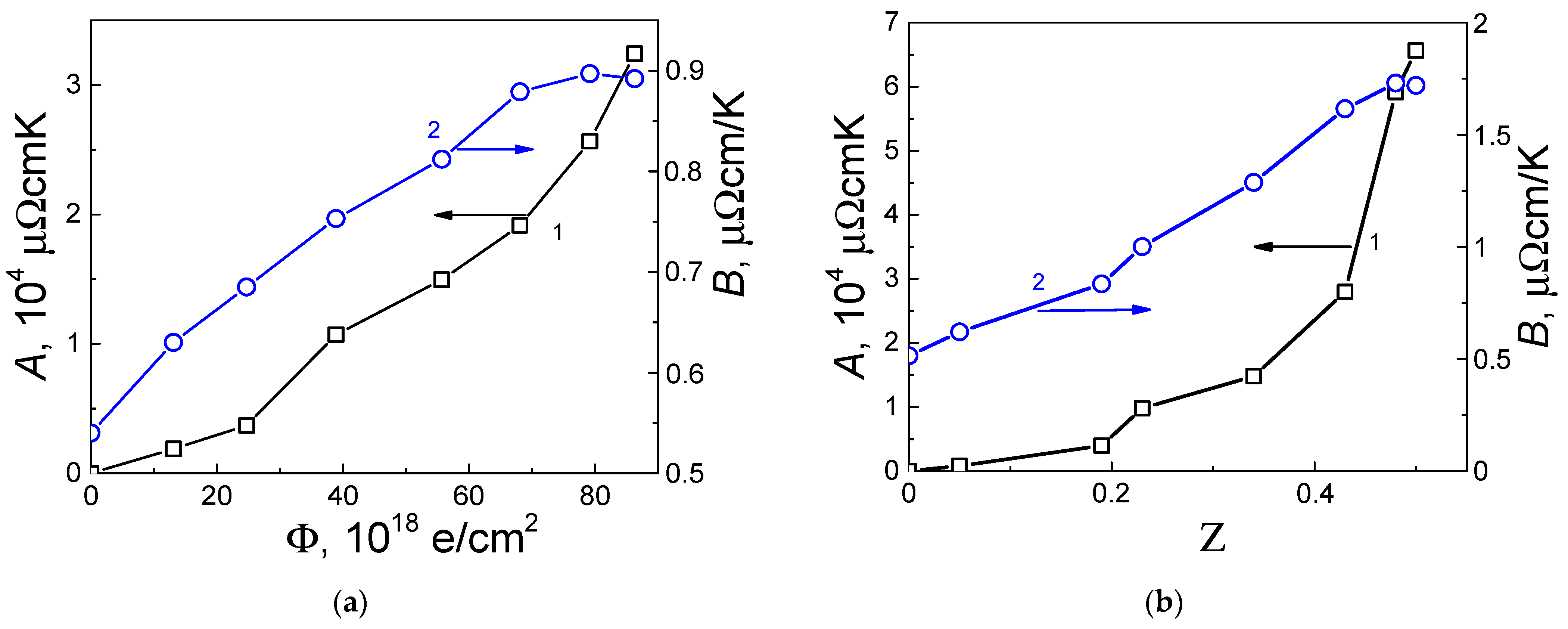
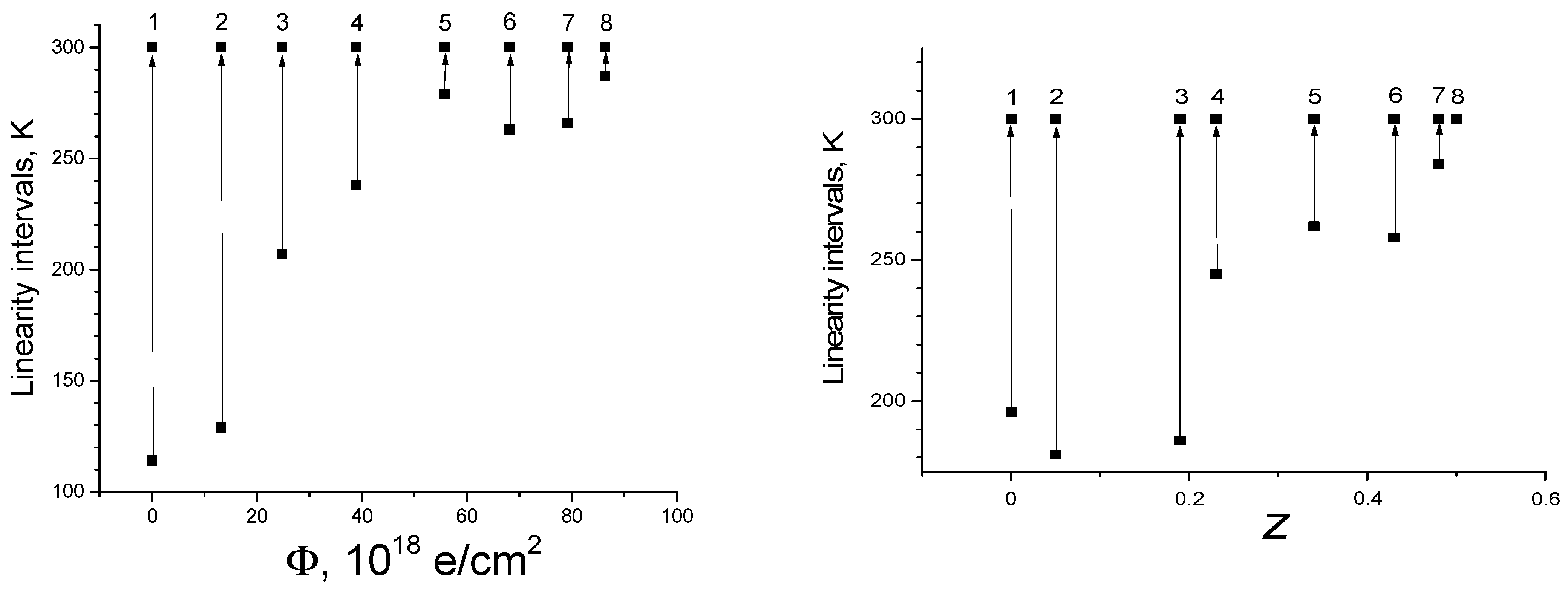
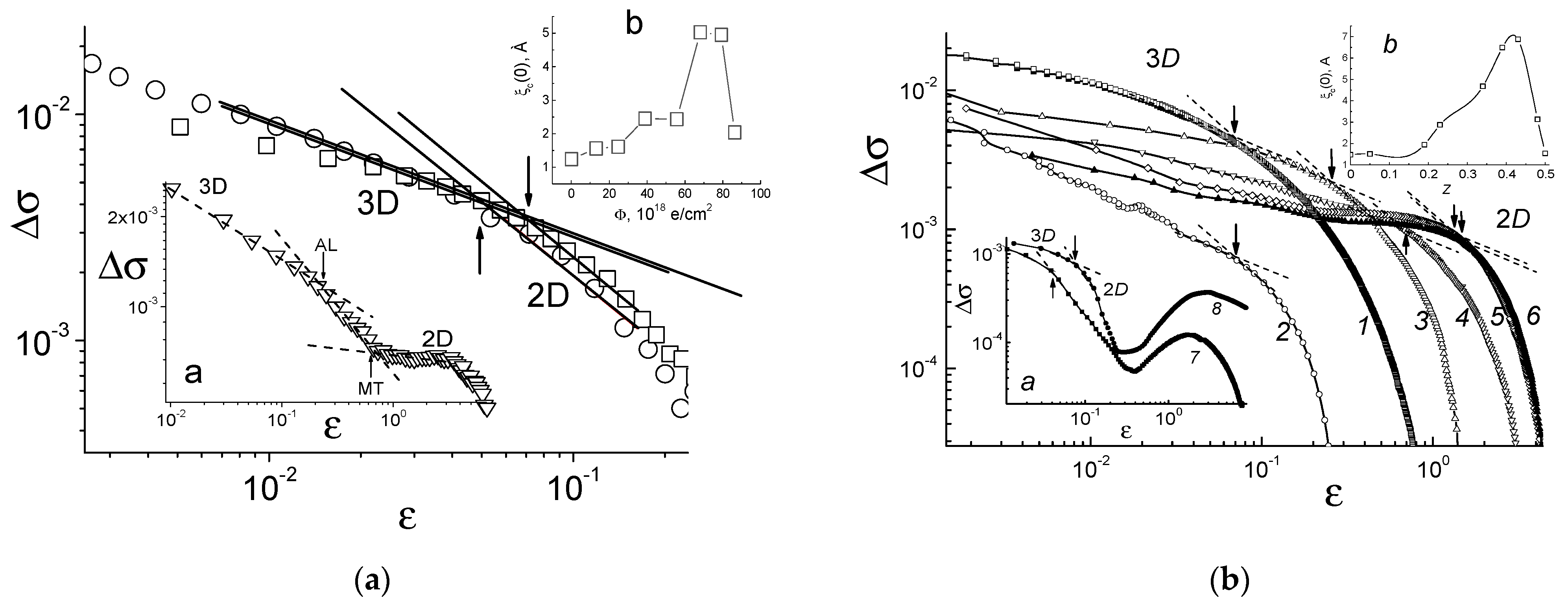
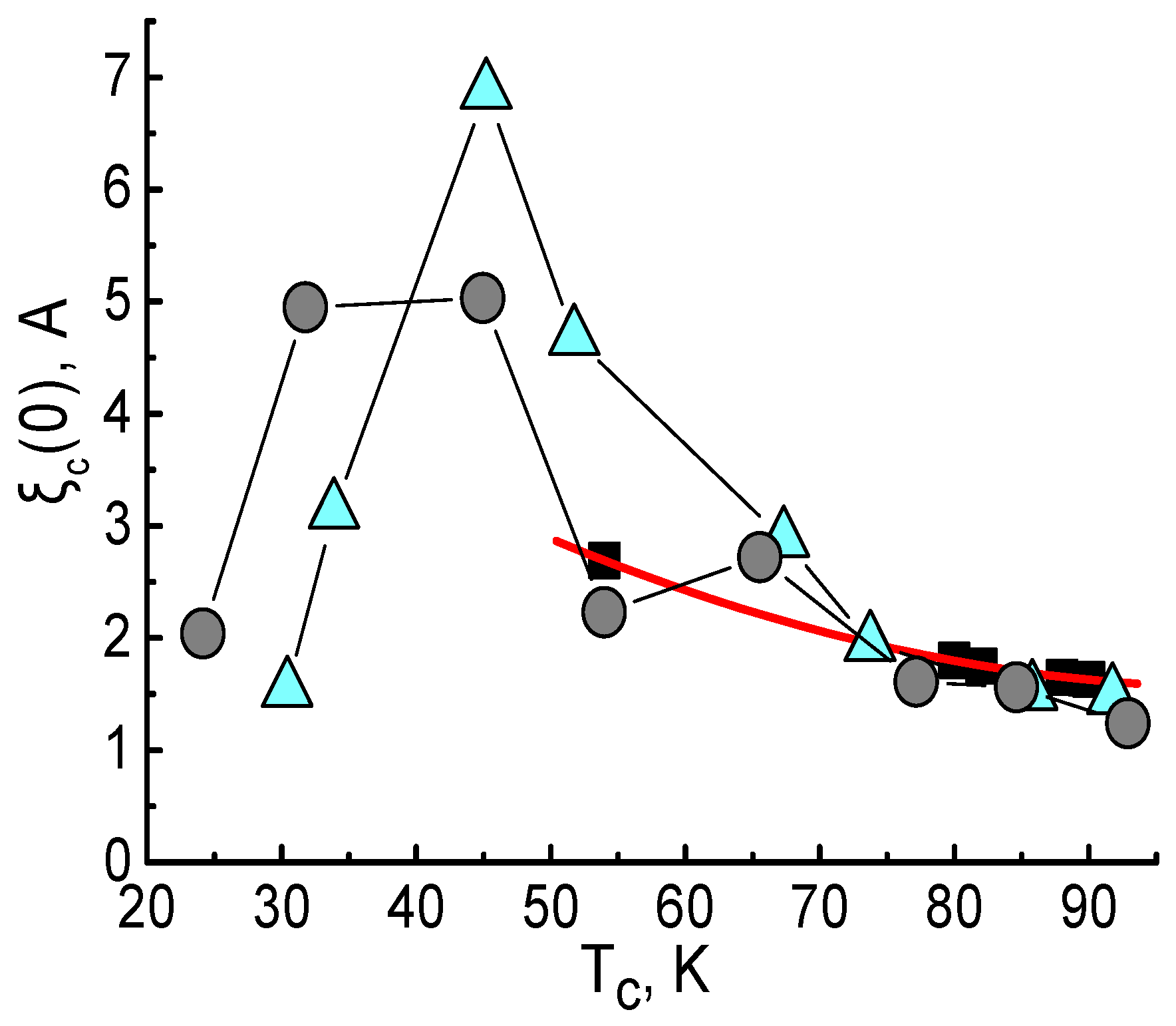
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vovk, R.V.; Solovyov, A.L. Electric transport and the pseudogap in the 1-2-3 HTSC system, under all-around compression (Review Article). Low Temp. Phys. 2018, 44, 111–153. [Google Scholar] [CrossRef]
- Friedman, T.A.; Rice, J.P.; Giapintzakis, J.; Ginzberg, D.M. In-plane paraconductivity in a single crystal of superconducting YBa2Cu3O7−x. Phys. Rev. B 1989, 39, 4258–4266. [Google Scholar] [CrossRef]
- Jorgensen, D.; Pes, S.; Lightfoot, P.; Shi, H.; Paulikas, A.P.; Veal, B.W. Time-dependent structural phenomena at room temperature in quenched YBa2Cu3O6.41: Local oxygen ordering and superconductivity. Phys. C 1990, 17, 571–578. [Google Scholar] [CrossRef]
- Mendonca Ferreira, L.; Pureur, P.; Borges, H.A.; Lejay, P. Effects of pressure on the fluctuation conductivity of YBa2Cu3O7. Phys. Rev. B 2004, 69, 212505. [Google Scholar] [CrossRef]
- Petrusenko, Y.T.; Neklyudov, I.M.; Sleptsov, A.N.; Yakovlev, V.F.; Bondarenko, A.V.; Obolensky, M.A. Recovery processes in YBa2Cu3O7−x single crystals after low-temperature irradiation. Phys. B Condens. Matter 1991, 169, 711–712. [Google Scholar] [CrossRef]
- Borges, H.A.; Continentino, M.A. Pressure study of the paraconductivity of high Tc superconductors. Solid State Commun. 1991, 80, 197–199. [Google Scholar] [CrossRef]
- Solovjov, A.L.; Omelchenko, L.V.; Vovk, R.V.; Dobrovolskiy, O.V.; Kamchatnaya, S.N.; Sergeyev, D.M. Peculiarities in the pseudogap behavior in optimally doped YBa2Cu3O7−δ single crystals under pressure up to 1 GPa. Curr. Appl. Phys. 2016, 16, 931–938. [Google Scholar] [CrossRef]
- Solovjov, A.L.; Habermeier, H.-U.; Haage, T. Fluctuation conductivity in YBa2Cu3O7−y films with different oxygen content. I. Optimally and lightly doped YBCO films. Low Temp. Phys. 2002, 28, 17–24. [Google Scholar] [CrossRef]
- Vovk, R.V.; Vovk, N.R.; Khadzhai, G.Y.; Goulatis, I.L.; Chroneos, A. Effect of high pressure on the electrical resistivity of optimally doped YBa2Cu3O7−δ single crystals with unidirectional planar defects. Phys. B Condes. Matter 2013, 422, 33–35. [Google Scholar] [CrossRef]
- Pan, V.M.; Svechnikov, V.L.; Solovjov, V.F. YBa2Cu3O7- delta single-crystal microstructure related to transport critical current density. Supercond. Sci. Technol. 1992, 5, 707. [Google Scholar] [CrossRef]
- Timusk, T.; Statt, B. The pseudogap in high-temperature superconductors: An experimental survey. Rep. Prog. Phys. 1999, 62, 61–122. [Google Scholar] [CrossRef]
- Aslamazov, L.G.; Larkin, A.I. The influence of fluctuation pairing of electrons on the conductivity of normal metal. Phys. Lett. A 1968, 26, 238–239. [Google Scholar] [CrossRef]
- Lawrence, W.E.; Doniach, S. Theory of layer-structure superconductors. In Proceedings of the 12th International Conference on Low Temperature Physics, Kyoto, Japan, 4–10 September 1970; Kanda, E., Ed.; Keigaku: Tokyo, Japan, 1970; p. 361. [Google Scholar]
- Bieri, J.B.; Maki, K.; Thompson, R.S. Nonlocal effect in magnetoconductivity of high-Tc superconductors. Phys. Rev. B 1991, 44, 4709–4711. [Google Scholar] [CrossRef] [PubMed]
- Vovk, R.V.; Obolenskii, M.A.; Zavgorodniy, A.A.; Goulatis, I.L.; Chroneos, A.; Biletskiy, E.V. Resistive investigation of pseudogap state in non-stoichiometric ReBa2Cu3O7−δ (Re = Y, Ho) single crystals with account for BCS–BEC crossover. J. Alloys Compd. 2009, 485, L21–L23. [Google Scholar] [CrossRef]
- Siegrist, T.; Sunshince, S.; Murphy, D.W.; Cava, R.J.; Zahurak, S.M. Crystal structure of the high-Tc superconductor Ba2YCu3O9−δ. Phys. Rev. B 1987, 35, 7137–7139. [Google Scholar] [CrossRef] [PubMed]
- Bondarenko, A.V.; Prodan, A.A.; Petrusenko, Y.T.; Borisenko, V.N.; Dworschak, F.; Dedek, U. Effect of electron irradiation on vortex dynamics in YBa2Cu3O7−δ single crystals. Phys. Rev. B 2001, 64, 092513. [Google Scholar] [CrossRef]
- Akhavan, M. The question of Pr in HTSC. Phys. B Condes. Matter 2002, 321, 265–282. [Google Scholar] [CrossRef]
- Solovjov, A.L.; Omelchenko, L.V.; Petrenko, E.V.; Vovk, R.V.; Khotkevych, V.V.; Chroneos, A. Peculiarities of pseudogap in Y0.95Pr0.05Ba2Cu3O7−δ single crystals under pressure up to 1.7 GPa. Sci. Rep. 2019, 9, 20424. [Google Scholar] [CrossRef]
- Frishherz, M.C.; Kirk, M.A.; Zhang, G.P.; Weber, H.W. Transmission electron microscopy of defect cascades in YBa2Cu3O7−δ produced by ion irradiation. Philos. Mag. A 1993, 67, 1347–1363. [Google Scholar] [CrossRef]
- Ashkenazi, J. A Theory for the High-T c Cuprates: Anomalous Normal-State and Spectroscopic Properties, Phase Diagram, and Pairing. J. Supercond. Nov. Magn. 2011, 24, 1281–1308. [Google Scholar] [CrossRef]
- Vovk, R.V.; Vovk, N.R.; Shekhovtsov, O.V.; Goulatis, I.L.; Chroneos, A. c-axis hopping conductivity in heavily Pr-doped YBCO single crystals. Supercond. Sci. Technol. 2013, 26, 085017. [Google Scholar] [CrossRef]
- Oduleye, O.O.; Penn, S.J.; Alford, N.M.; Lay, L.L.; Beales, T. Thermal cycling, critical current vs. strain, and finite element modelling of 1, 7, 19 and 37 filament Ag/Bi-Sr-Ca-Cu-O (BSCCO) tapes. IEEE Trans. Appl. Supercond. 1999, 9, 2621–2624. [Google Scholar] [CrossRef]
- Wang, Q.; Saunders, G.A.; Liu, H.J.; Acres, M.S.; Almond, D.P. Electrical resistance under pressure in textured Bi2Sr2CaCu2O8+y: Enhancement of the energy gap and thermodynamic fluctuations. Phys. Rev. B 1997, 55, 8529–8543. [Google Scholar] [CrossRef]
- Chroneos, A.I.; Goulatis, I.L.; Vovk, R.V. Atomic Scale Models for RBa2Cu3O6.5 and R1–XPrXBa2Cu3O6.5 Compounds (R=Y and Lanthanides). Acta Chim. Slov. 2007, 54, 179–184. [Google Scholar]
- Chroneos, A.; Kolesnikov, D.D.; Taranova, I.A.; Matsepulin, A.V.; Vovk, R.V. Composition variation and electron irradiation effects on the fluctuation conductivity in Y1–zPrzBa2Cu3O7−δ single crystals. J. Mater. Sci. Mater. Electron. 2020, 31, 19429–19436. [Google Scholar] [CrossRef]
- Khadzhai, G.Y.; Sklyar, V.V.; Vovk, R.V. Suppression of superconductivity in YBa2Cu3O7−δ single crystals upon irradiation with fast electrons. Low Temp. Phys. 2022, 48, 271–273. [Google Scholar] [CrossRef]
- Anderson, P.W.; Zou, Z. “Normal” Tunneling and “Normal” Transport: Diagnostics for the Resonating-Valence-Bond State. Phys. Rev. Lett. 1988, 60, 132–135. [Google Scholar] [CrossRef]
- Stojkovic, B.P.; Pines, D. Theory of the longitudinal and Hall conductivities of the cuprate superconductors. Phys. Rev. B 1997, 55, 8576–8595. [Google Scholar] [CrossRef]
- Valles, J.M., Jr.; White, A.E.; Short, K.T.; Dynes, R.C.; Garno, J.P.; Levi, A.F.J.; Anzlowar, M.; Baldwin, K. Ion-beam-induced metal-insulator transition in YBa2Cu3O7−δ: A mobility edge. Phys. Rev. B 1989, 39, 11599–11602. [Google Scholar] [CrossRef] [PubMed]
- Chryssikos, G.D.; Kamitsos, E.I.; Kapoutsis, J.A.; Patsis, A.P.; Psycharis, V.; Koufoudakis, A.; Mitros, C.; Kallias, G.; Gamari-Seale, E.; Niarchos, D. X-ray diffraction and infrared investigation of RBa2Cu3O7 and R0.5Pr0.5Ba2Cu3O7 compounds (RY and lanthanides). Phys. C Supercond. 1995, 254, 44–62. [Google Scholar] [CrossRef]
- De Gennes, P.G. Superconductivity of Metals and Alloys; W.A. Benjamin, Inc.: New York, NY, USA; Amsterdam, The Netherlands, 1966. [Google Scholar]
- Matthews, A.J.; Kavokin, K.V.; Usher, A.; Portnoi, M.E.; Zhu, M.; Gething, J.D.; Elliot, M.; Herrenden-Harker, W.G.; Phillips, K.; Ritchie, D.A.; et al. Temperature dependence of the breakdown of the quantum Hall effect studied by induced currents. Phys. Rev. B 2004, 70, 075317. [Google Scholar] [CrossRef]
- Adamenko, I.N.; Nemchenko, K.E.; Tsyganok, V.I.; Chervanev, A.I. Interaction of quasiparticles and stationary states of superfluid solutions of helium isotopes. Low Temp. Phys. 1994, 20, 498–504. [Google Scholar]
- Curran, P.G.; Khotkevych, V.V.; Bending, S.J.; Gibbs, A.S.; Lee, S.L.; Mackenzie, A.P. Vortex imaging and vortex lattice transitions in superconducting Sr2RuO4 single crystals. Phys. Rev. B 2011, 84, 104507. [Google Scholar] [CrossRef]
- Randeria, M. Pre-pairing for condensation. Nat. Phys. 2010, 6, 561–562. [Google Scholar] [CrossRef]
- Badoux, S.; Tabis, W.; Laliberte, F.; Grissonnanche, G.; Vignolle, B.; Vignolles, D.; Beard, J.; Bonn, D.A.; Hardy, W.N.; Liang, R.; et al. Change of carrier density at the pseudogap critical point of a cuprate superconductor. Nature 2016, 531, 210–214. [Google Scholar] [CrossRef] [PubMed]
- Kordyuk, A.A. Pseudogap from ARPES experiment: Three gaps in cuprates and topological superconductivity (Review Article). Low Temp. Phys. 2015, 41, 319–341. [Google Scholar] [CrossRef]
- Solovjov, A.L. Chapter 7: Pseudogap and local pairs in high-Tc superconductors. In Superconductors—Materials, Properties and Applications; Gabovich, A.M., Ed.; InTech: Rijeka, Croatia, 2012; pp. 137–170. [Google Scholar]
- He, R.-H.; Hashimoto, M.; Karapetyan, H.; Koralek, J.D.; Hinton, J.P.; Testaud, J.P.; Nathan, V.; Yoshida, Y.; Yao, H.; Tanaka, K.; et al. From a Single-Band Metal to a High-Temperature Superconductor via Two Thermal Phase Transitions. Science 2011, 331, 1579–1583. [Google Scholar] [CrossRef] [PubMed]
- Anderson, P.W. The Theory of Superconductivity in the High Cuprates; Princeton University Press: Princeton, NJ, USA, 1997; p. 446. [Google Scholar]
- Emery, V.J.; Kivelson, S.A. Importance of phase fluctuations in superconductors with small superfluid density. Nature 1995, 374, 434–437. [Google Scholar] [CrossRef]
- Corson, J.; Mallozzi, R.; Orenstein, J.; Eckstein, J.N.; Bozovic, I. Vanishing of phase coherence in underdoped Bi2Sr2CaCu2O8+δ. Nature 1999, 398, 221–223. [Google Scholar] [CrossRef]
- Varma, C.M. Theory of the pseudogap state of the cuprates. Phys. Rev. B 2006, 73, 155113. [Google Scholar] [CrossRef]
- Cava, R.J. Structural Chemistry and the Local Charge Picture of Copper Oxide Superconductors. Science 1990, 247, 656–662. [Google Scholar] [CrossRef] [PubMed]
- Chen, Q.; Kosztin, I.; Janko, B.; Levin, K. Pairing Fluctuation Theory of Superconducting Properties in Underdoped to Overdoped Cuprates. Phys. Rev. Lett. 1998, 81, 4708–4711. [Google Scholar] [CrossRef]
- Efetov, K.; Meier, H.; Pépin, C. Pseudogap state near a quantum critical point. Nat. Phys. 2013, 9, 442–446. [Google Scholar] [CrossRef]
- Imada, M.; Fujimori, A.; Tokura, Y. Metal-insulator transitions. Rev. Mod. Phys. 1998, 70, 1039. [Google Scholar] [CrossRef]
- Suzuki, M.; Watanabe, T. Discriminating the Superconducting Gap from the Pseudogap in Bi2Sr2CaCu2O8+δ by Interlayer Tunneling Spectroscopy. Phys. Rev. Lett. 2000, 85, 4787–4790. [Google Scholar] [CrossRef] [PubMed]
- Habermeier, H.-U. In Proceedings of the ESF International Workshop on Superconductivity in Reduced Dimensions, Sulzburg, Austria, May 2010; p. 33.
- Birgineau, R.J.; Shirane, G. Neutron Scattering Studies of Structural and Magnetic Excitations in Lamellar Copper Oxides. A Review. In Physical Properties of High Temperature Superconductors I; Ginsberg, D.M., Ed.; World Scientific: Singapore, 1989; pp. 152–212. [Google Scholar]
- Gawalek, W.; Schueppel, W.; Hergt, R.; Andra, W.; Fischer, K.; Gornert, P. High critical currents in peritectically grown YBa2Cu3O7-x single crystals. Supercond. Sci. Technol. 1992, 5, S407–S410. [Google Scholar] [CrossRef]
- Ando, Y.; Komiya, S.; Segawa, K.; Ono, S.; Kurita, Y. Electronic Phase Diagram of High-Tc Cuprate Superconductors from a Mapping of the In-Plane Resistivity Curvature. Phys. Rev. Lett. 2004, 93, 267001. [Google Scholar] [CrossRef]
- Fehrenbacher, R.; Rice, T.M. Unusual electronic structure of PrBa2Cu3O7. Phys. Rev. Lett. 1993, 70, 3471–3474. [Google Scholar] [CrossRef]
- Liechtenstein, A.I.; Mazin, I.I. Quantitative model for the superconductivity suppression in R1-xPrxBa2Cu3O7 with different rare earths. Phys. Rev. Lett. 1995, 74, 1000–1003. [Google Scholar] [CrossRef]
- Chu, C.W.; Hor, P.H.; Meng, R.L.; Gao, L.; Huang, A.J.; Wang, Y.Q. Evidence for superconductivity above 40 K in the La-Ba-Cu-O compound system. Phys. Rev. Lett. 1988, 58, 405–407. [Google Scholar] [CrossRef]
- Loktev, V.M. Particularities of Superconductivity in 2D Metals: Transition from Cooper Pairing to the Local One. Low Temp. Phys. 1996, 22, 488–491. [Google Scholar]
- Gusynin, P.; Loktev, V.M.; Sharapov, S.G. Phase diagram of a 2D metal system with a variable number of carriers. Sov. ZETF Let. 1997, 65, 182. [Google Scholar] [CrossRef]
- Kondo, T.; Hamaya, Y.; Palczewski, A.D.; Takeuchi, T.; Wen, J.S.; Xu, Z.J.; Gu, G.; Schmalian, J.; Kaminski, A. Disentangling Cooper-pair formation above the transition temperature from the pseudogap state in the cuprates. Nat. Phys. 2011, 7, 21–25. [Google Scholar] [CrossRef]

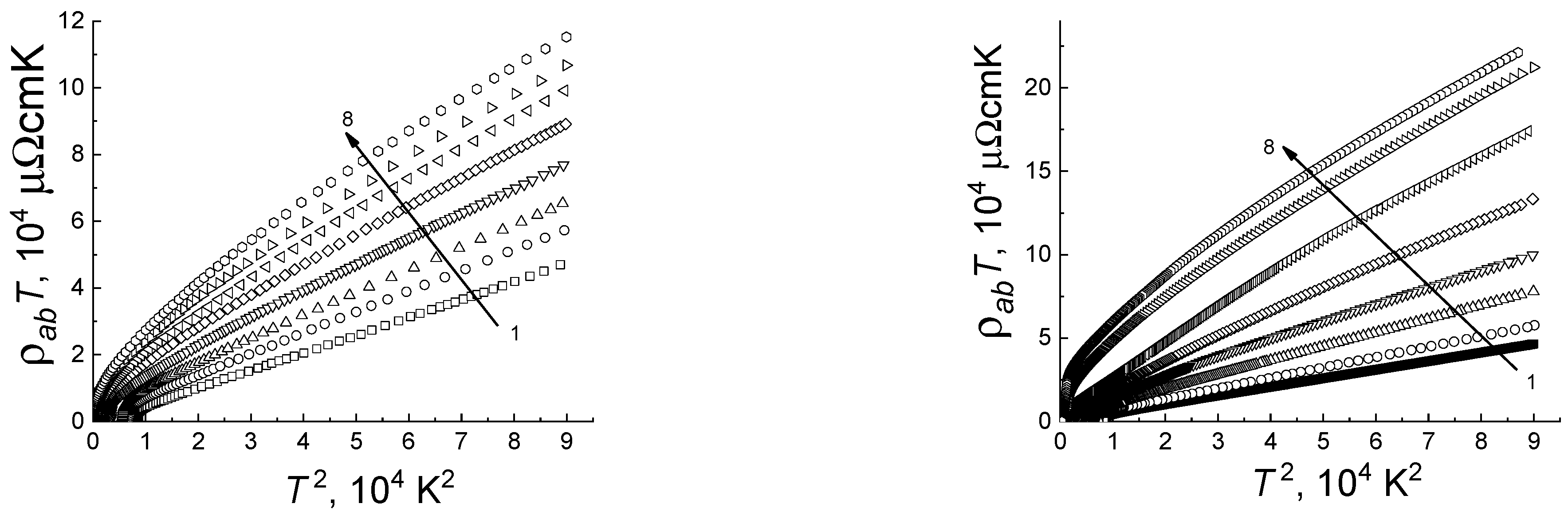
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khadzhai, G.; Goulatis, I.; Chroneos, A.; Feher, A.; Vovk, R. Comparative Analysis of the Irradiation with Medium Fluences of High-Energy Electrons and Pr Doping on the Fluctuation Conductivity of YBa2Cu3O7–δ Single Crystals. Appl. Sci. 2024, 14, 6536. https://doi.org/10.3390/app14156536
Khadzhai G, Goulatis I, Chroneos A, Feher A, Vovk R. Comparative Analysis of the Irradiation with Medium Fluences of High-Energy Electrons and Pr Doping on the Fluctuation Conductivity of YBa2Cu3O7–δ Single Crystals. Applied Sciences. 2024; 14(15):6536. https://doi.org/10.3390/app14156536
Chicago/Turabian StyleKhadzhai, George, Ioannis Goulatis, Alexander Chroneos, Alexander Feher, and Ruslan Vovk. 2024. "Comparative Analysis of the Irradiation with Medium Fluences of High-Energy Electrons and Pr Doping on the Fluctuation Conductivity of YBa2Cu3O7–δ Single Crystals" Applied Sciences 14, no. 15: 6536. https://doi.org/10.3390/app14156536
APA StyleKhadzhai, G., Goulatis, I., Chroneos, A., Feher, A., & Vovk, R. (2024). Comparative Analysis of the Irradiation with Medium Fluences of High-Energy Electrons and Pr Doping on the Fluctuation Conductivity of YBa2Cu3O7–δ Single Crystals. Applied Sciences, 14(15), 6536. https://doi.org/10.3390/app14156536







