Comparison between the Dynamic Responses of Steel Buildings with Medium and Deep Columns under Several Seismic Intensities
Abstract
1. Introduction and Literature Review
2. Justification and Objectives
3. Procedure, Structural Models, and Strong Motions
3.1. Parameters of the Study
3.2. Structural Models with W14 Columns
3.3. Structural Models with Deep Columns
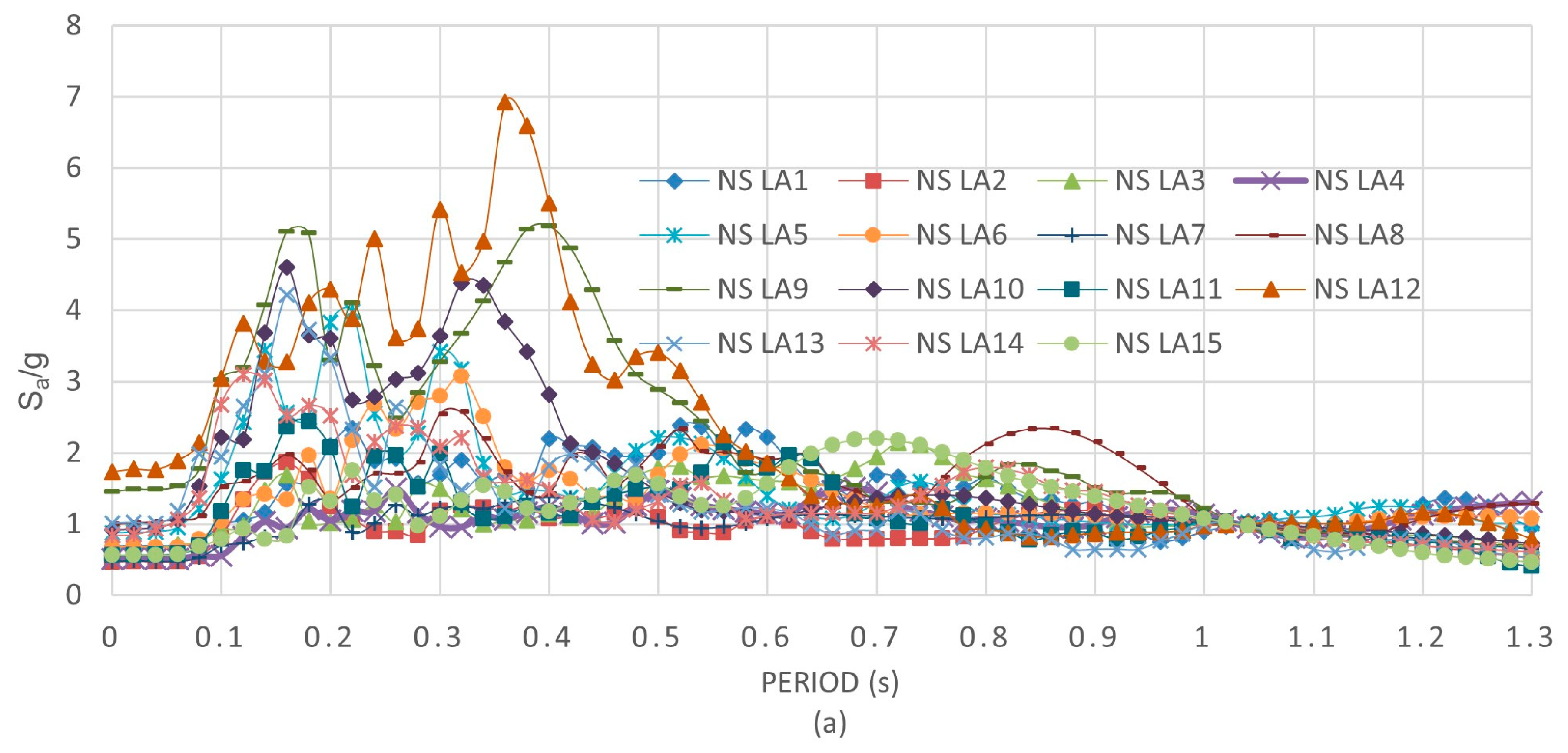
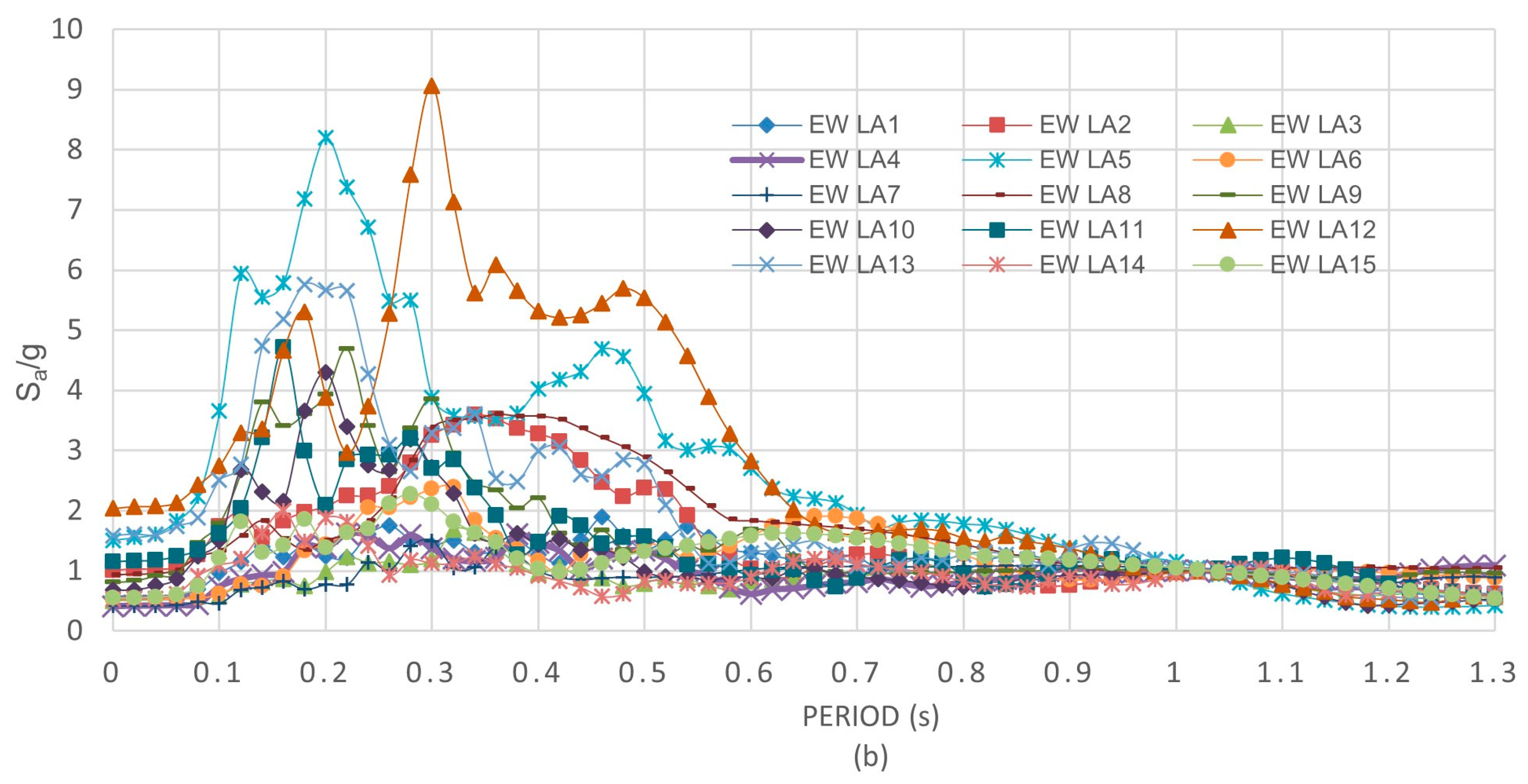
3.4. Strong Motion Records
3.5. Local, Shear, and Lateral–Torsional Buckling of Deep Columns
4. Results and Discussion
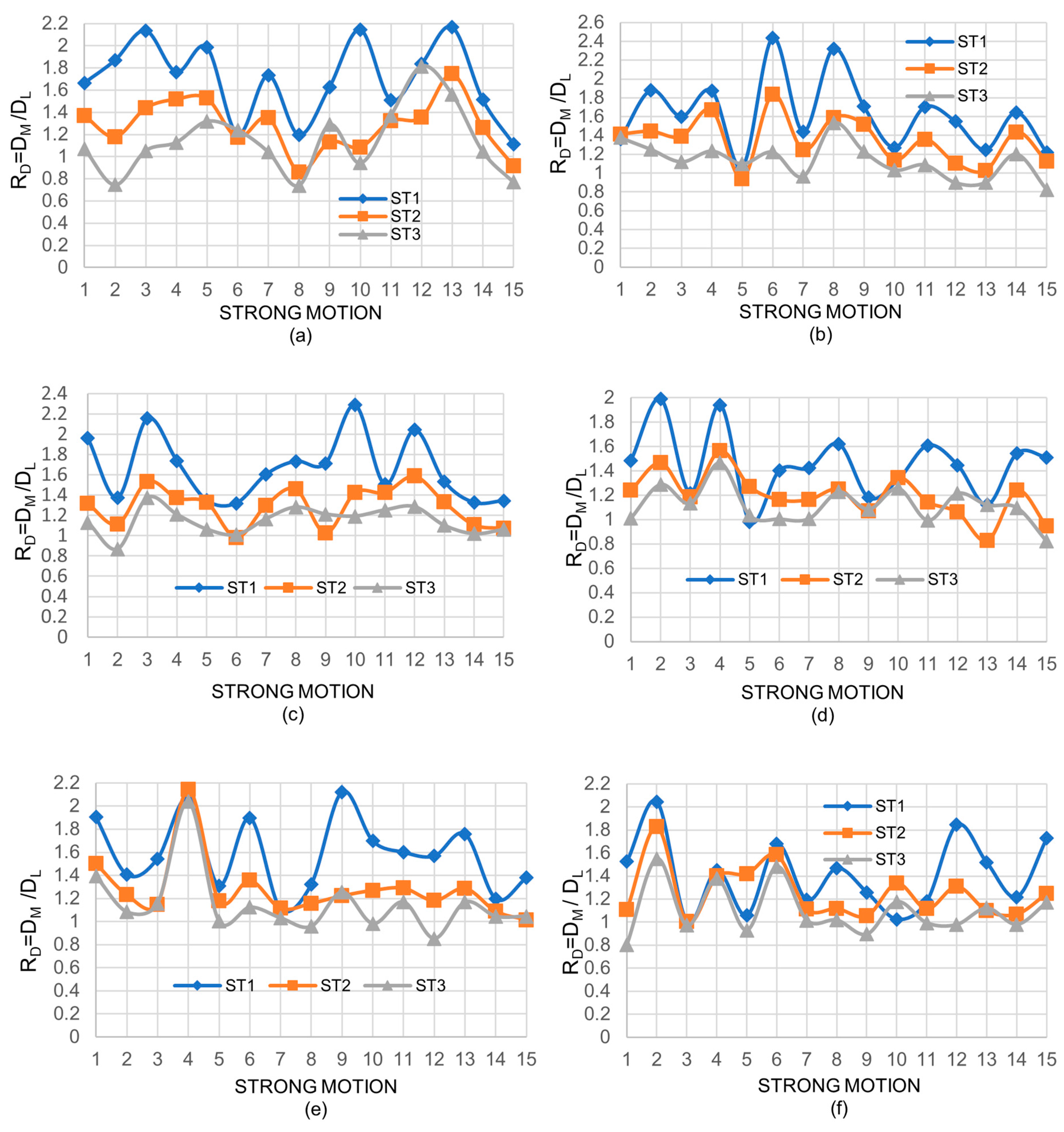
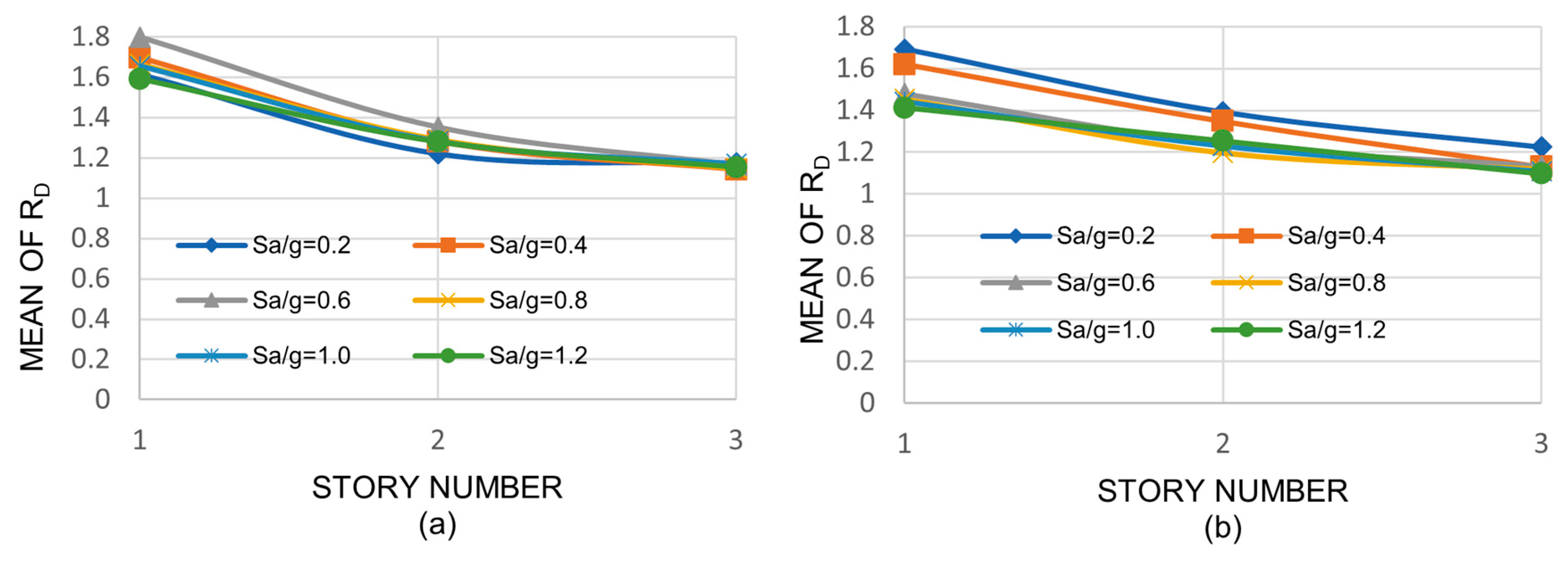
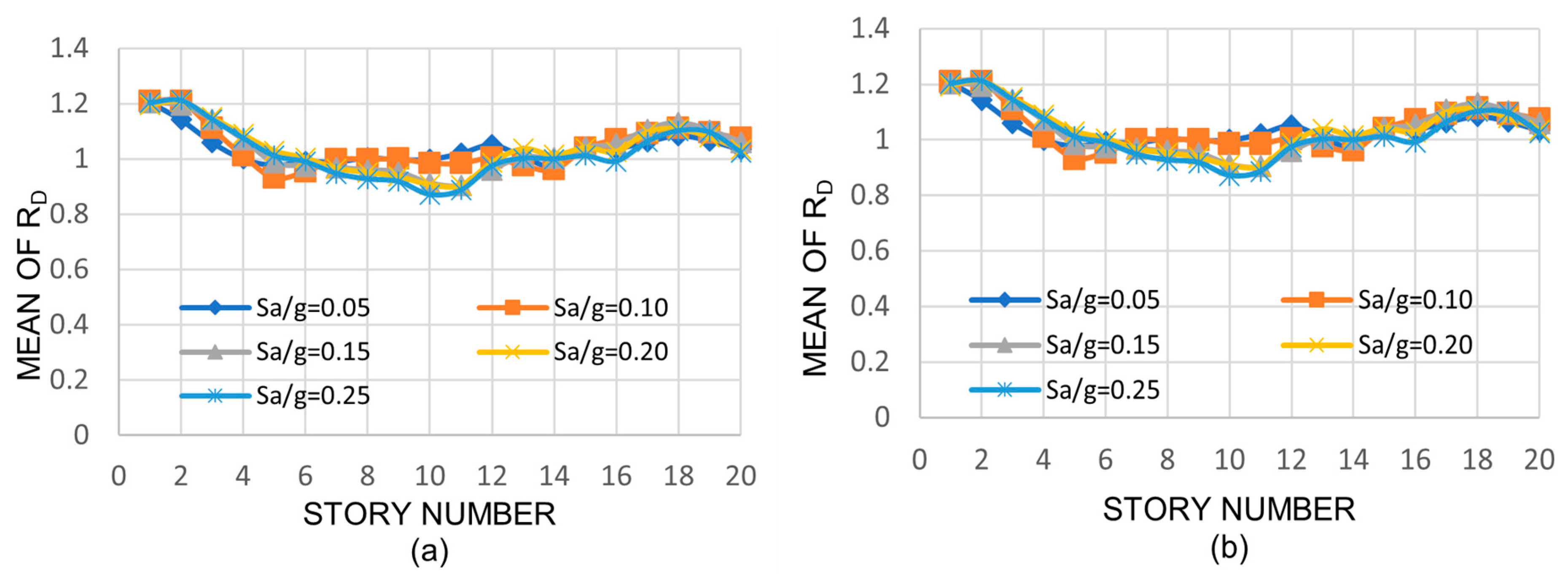
4.1. Objective 1: Results in Terms of Displacements
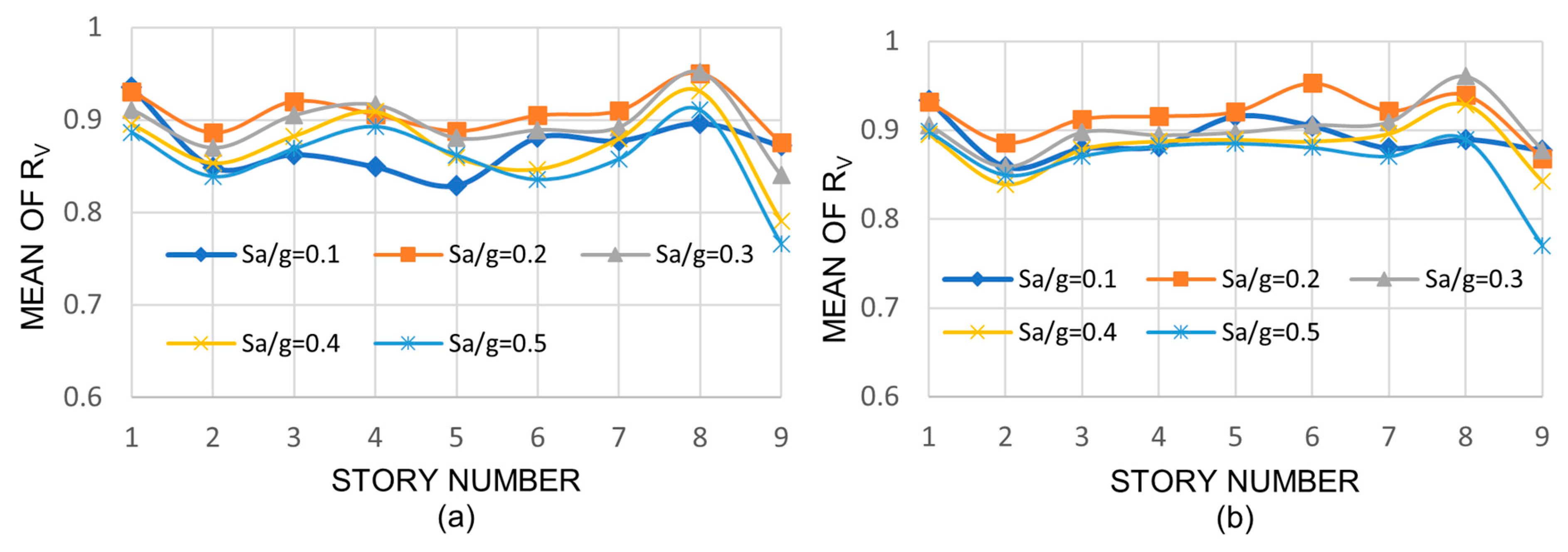
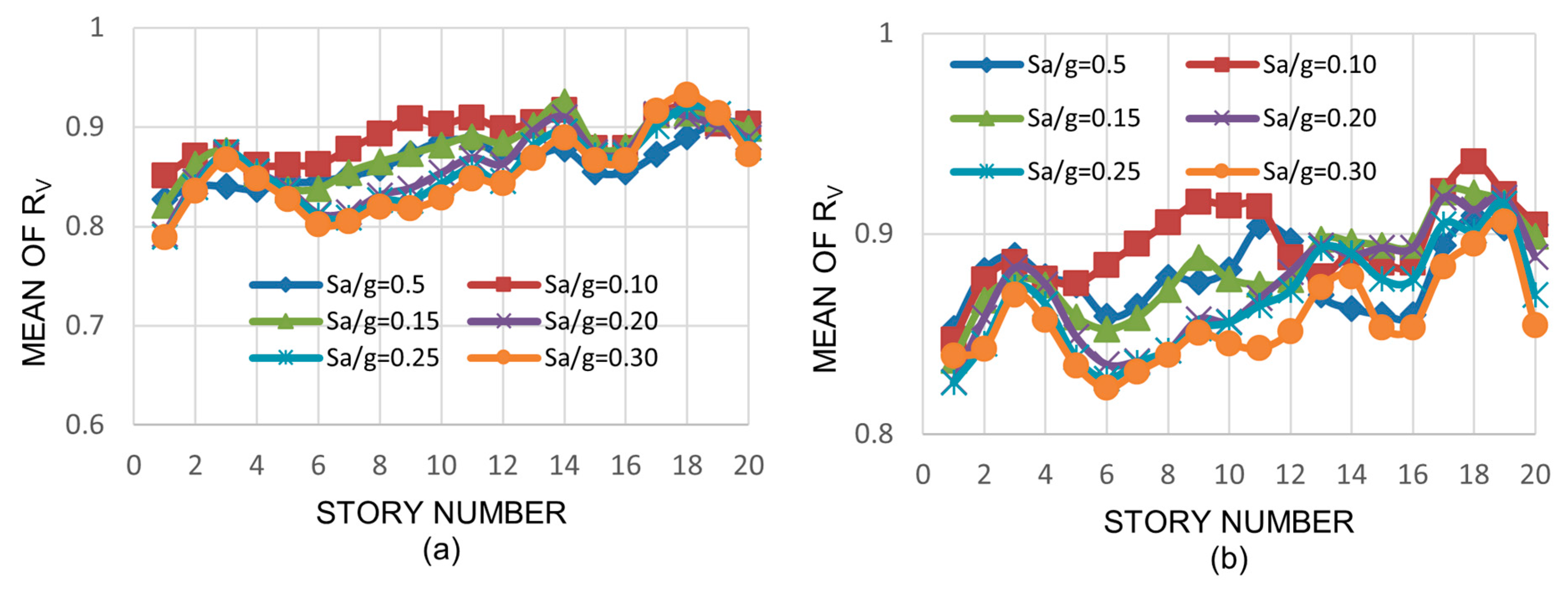
4.2. Objective 2: Results in Terms of Resultant Stresses: Interstory Shears, Axial Loads, and Bending Moments
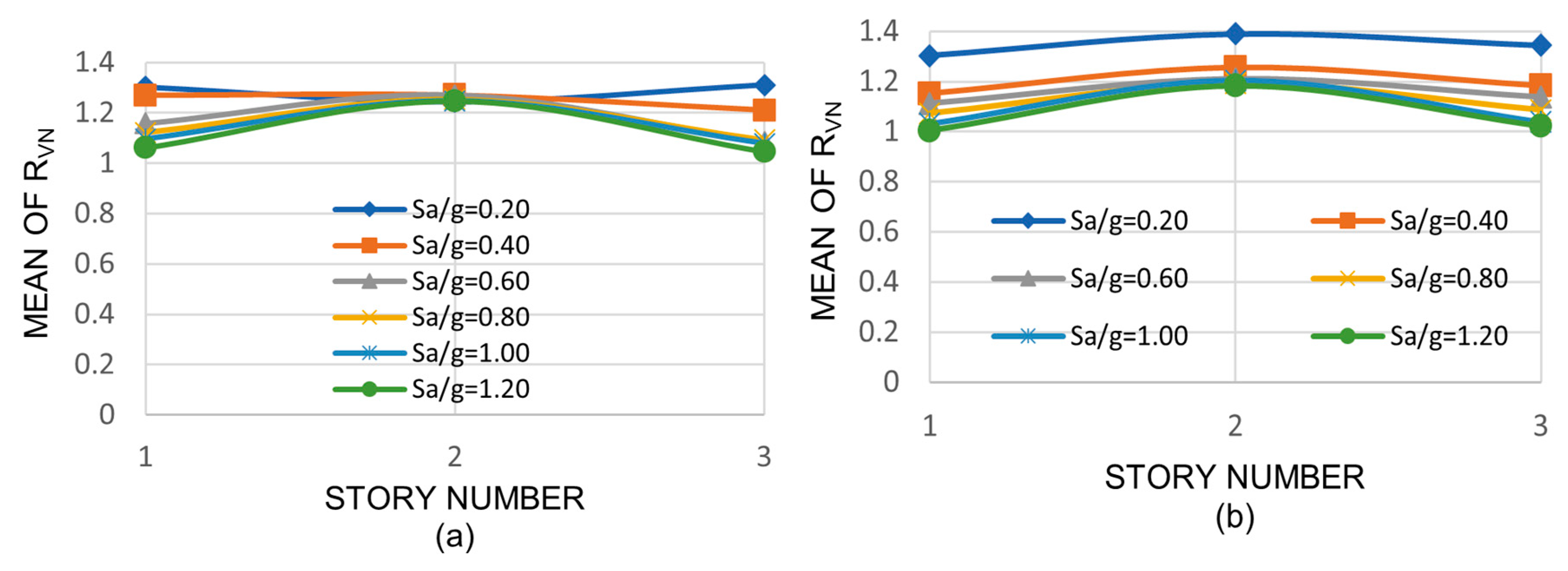
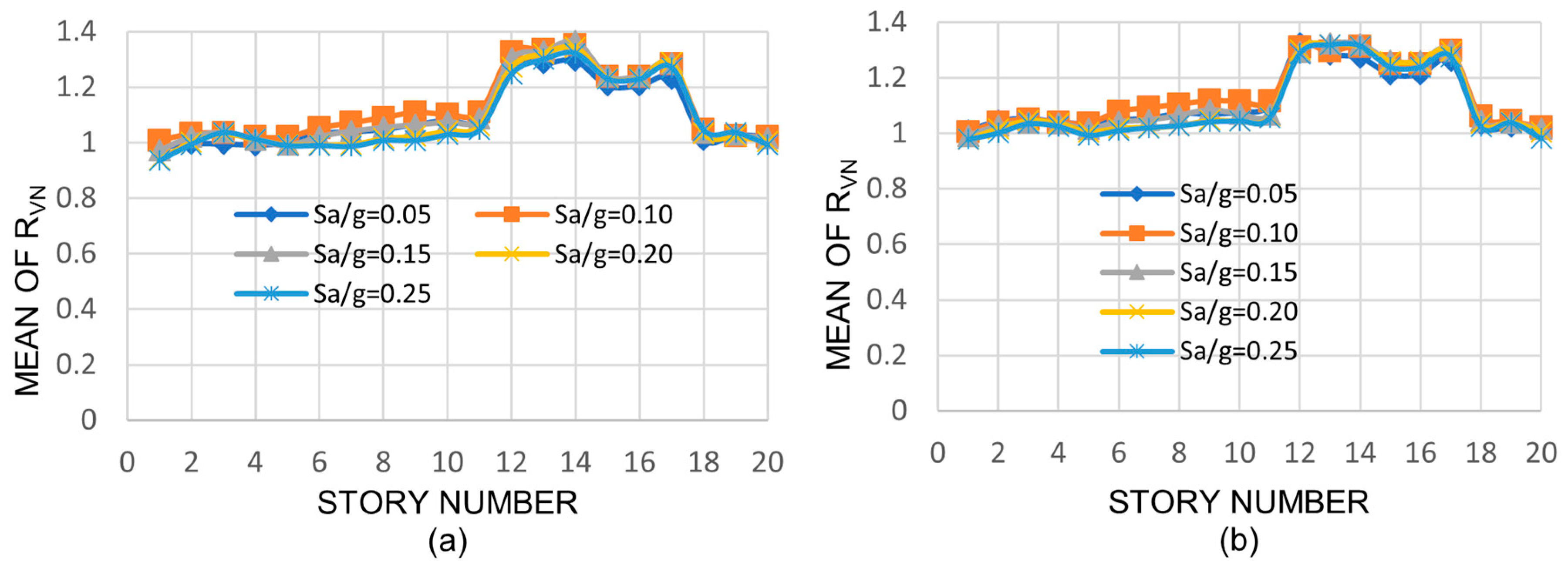
4.3. Objective 3: Results in Terms of Normalized Single Overall and Normalized Combined Local Resultant Stresses
4.3.1. Normalized Shear Strength
4.3.2. Combined Normalized Axial Loads and Bending Moments
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gupta, A.; Krawinkler, H. Behavior of Ductile SMRFs at Various Seismic Hazard Levels. J. Struct. Eng. 2000, 126, 98–107. [Google Scholar] [CrossRef]
- Lee, K.; Foutch, D. Performance Evaluation of New Steel Frame Buildings for Seismic Loads. Earthq. Eng. Struct. Dyn. 2001, 31, 653–670. [Google Scholar] [CrossRef]
- Foutch, A.; Yun, S. Modeling of Steel Moment Frames for Seismic Loads. J. Constr. Steel Res. 2002, 58, 529–564. [Google Scholar] [CrossRef]
- Mele, E.; Di Sarno, L.; De Luca, A. Seismic Behavior of Perimeter and Spatial Steel Frames. J. Earthq. Eng. 2004, 8, 457–496. [Google Scholar] [CrossRef]
- Fathi, M.; Daneshjoo, F.; Melchers, R. A Method for Determining the Behaviour Factor of Moment-Resisting Steel Frames with Semi-Rigid Connections. Eng. Struct. 2006, 28, 514–531. [Google Scholar] [CrossRef]
- Lee, K.; Foutch, D. Seismic Evaluation of Steel Moment Frames Buildings Designed Using Different R-Values. J. Struct. Eng. Div. ASCE 2006, 132, 1461–1472. [Google Scholar] [CrossRef]
- Krishnan, S.; Ji, C.; Komatitsch, D.; Tromp, J. Performance of Two 18-Storey Steel Moment-Frame Building in Southern California during Two Large Simulated San Andres Earthquakes. Earthq. Spectra 2006, 22, 1035–1061. [Google Scholar] [CrossRef]
- Black, E. Use of Stability Coefficients for Evaluating the P–Δ Effect in Regular Steel Moment Resisting Frames. Eng. Struct. 2011, 33, 1205–1216. [Google Scholar] [CrossRef]
- Sejal, P.; Vasanwala, S.; Desai, A. Comparison of Steel Moment Resisting Frame Designed by Elastic Design and Performance Based Plastic Design Method Based on the Inelastic Response Analysis. Int. J. Civ. Struct. Eng. 2012, 2, 1081–1097. [Google Scholar]
- Teran-Gilmore, A.; Diaz, G.; Reyes, C. Displacement-Based Conception of Moment-Resisting Frames That House Essential Facilities. Soil Dyn. Earthq. Eng. 2013, 46, 96–113. [Google Scholar] [CrossRef]
- Athanasios, I.; Dimopoulos, A.; Bazeos, N.; Beskos, D. Seismic Yield Displacements of Plane Moment Resisting and X-Braced Steel Frames. Soil Dyn. Earthq. Eng. 2012, 41, 128–140. [Google Scholar]
- Formisano, A.; Landolfo, R.; Mazzolani, F. Robustness Assessment Approaches for Steel Framed Structures under Catastrophic Events. Comput. Struct. 2015, 147, 216–228. [Google Scholar] [CrossRef]
- Liu, W.; Rasmussen, K.J.R.; Zhang, H. Systems Reliability for 3D Steel Frames Subject to Gravity Loads. Structures 2016, 8, 170–182. [Google Scholar] [CrossRef]
- Samanta, A.; Huang, Y. Ground-Motion Scaling for Seismic Performance Assessment of High-Rise Moment-Resisting Frame Building. Soil Dyn. Earthq. Eng. 2017, 94, 125–135. [Google Scholar] [CrossRef]
- Loulelis, D.; Papagiannopoulos, G.; Beskos, D. Modal Strength Reduction Factors for Seismic Design of Steel Moment Resisting Frames. Eng. Struct. 2018, 154, 23–37. [Google Scholar] [CrossRef]
- Ruiz-García, J.; Yaghmaei-Sabegh, S.; Bojórquez, E. Three-Dimensional Response of Steel Moment-Resisting Buildings under Seismic Sequences. Eng. Struct. 2018, 175, 399–414. [Google Scholar] [CrossRef]
- Fogarty, J.; Wu, T.Y.; El-Tawil, S. Collapse Response and Design of Deep Steel Columns Subjected to Lateral Displacement. J. Struct. Eng. 2017, 143, 04017130. [Google Scholar] [CrossRef]
- Elkady, A.; Lignos, D.G. Full-Scale Testing of Deep Wide-Flange Steel Columns under Multiaxis Cyclic Loading: Loading Sequence, Boundary Effects, and Lateral Stability Bracing Force Demands. J. Struct. Eng. 2018, 144, 04017189. [Google Scholar] [CrossRef]
- Sediek, O.A.; Wu, T.-Y.; McCormick, J.; El-Tawil, S. Prediction of Seismic Collapse Behavior of Deep Steel Columns Using Machine Learning. Structures 2022, 40, 163–175. [Google Scholar] [CrossRef]
- Wu, T.-Y.; Pal, P.S.; Wang, H.-C. Collapse Risk of Steel Framed Buildings with Deep Columns under Tri-Directional Excitation. J. Constr. Steel Res. 2023, 208, 108030. [Google Scholar] [CrossRef]
- Sepulveda, C. Seismic Behavior of Deep and Slender Steel Columns through Full-Scale Cyclic and Hybrid Testing. Ph.D. Thesis, University of California San Diego, La Jolla, CA, USA, 2024. [Google Scholar]
- Gilton, C.; Chi, B.; Uang, C. Cyclic Response of RBS Moment Connections: Weak-Axis Configuration and Deep Column Effects; University of California San Diego: La Jolla, CA, USA, 2000. [Google Scholar]
- Chi, B.; Uang, C. Cyclic Response and Design Recommendations of Reduced Beam Section Moment Connections with Deep Columns. J. Struct. Eng. 2002, 128, 464–473. [Google Scholar] [CrossRef]
- Shen, J.; Astaneh-Asl, A.; McCallen, D. Use of Deep Columns in Special Steel Moment Frames. Steel Tip Rep. 2002. [Google Scholar]
- Zhang, X.; Ricles, J.; LuL, W.; Fisher, J. Analytical and Experimental Studies on Seismic Behavior of Deep Column-to-Beam Welded Reduced Beam Section Moment Connections. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. Paper No 1599. [Google Scholar]
- Shao, D.; Hale, T. Full Scale Testing and Project Application of Sideplate Moment Connection for SMRF Using Deep Columns. In Proceedings of the 2004 SEAOC Convention, Monterey, CA, USA, 25–28 August 2004. [Google Scholar]
- Ricles, J.; Zhang, X. Seismic Performance of Reduced Beam Section Moment Connections to Deep Columns. In Proceedings of the Structures Congress, St Louis, MI, USA, 18–21 May 2006. [Google Scholar]
- Zhang, X.; Ricles, J. Experimental Evaluation of Reduced Beam Section Connections to Deep Columns. J. Struct. Eng. 2006, 132, 346–357. [Google Scholar] [CrossRef]
- Zhang, X.; Ricles, J. Seismic Behavior of Reduced Beam Section Moment Connections to Deep Columns. J. Struct. Eng. 2006, 132, 358–367. [Google Scholar] [CrossRef]
- Elkady, A.; Lignos, D. Collapse Assessment of Steel Moment Resisting Frames Designed with Deep Members. In Proceedings of the Vienna Congress on Recent Advances in Earthquake Engineering and Structural Dynamics, Vienna, Austria, 28–30 August 2013; p. 249. [Google Scholar]
- Islam, A.; Imanpour, A. Stability of Wide-Flange Columns in Steel Moment-Resisting Frames: Evaluation of the Canadian Seismic Design Requirements. Bull. Earthq. Eng. 2022, 20, 1591–1617. [Google Scholar] [CrossRef]
- Monjardin-Quevedo, J.G.; Reyes-Salazar, A.; Tolentino, D.; Gaxiola-Camacho, O.D.; Vazquez-Becerra, G.E.; Gaxiola-Camacho, J.R. Seismic Reliability of Steel SMFs with Deep Columns Based on PBSD Philosophy. Structures 2022, 42, 1–15. [Google Scholar] [CrossRef]
- Boushehri, K.; Tsavdaridis, K.D.; Cai, G. Seismic Behaviour of RWS Moment Connections to Deep Columns with European Sections. J. Constr. Steel Res. 2019, 161, 416–435. [Google Scholar] [CrossRef]
- Carr, A. RUAUMOKO, Inelastic Dynamic Analysis Program 2016.
- Preserve, L.; Chen, W.F.; Atsuta, T. Interaction Equations for Biaxially Loaded Sections. J. Struct. Div. 1972, 98, 1035–1052. [Google Scholar]
- Federal Emergency Management Agency. State of the Art Report on Systems Performance of Steel Moment Frames Subjected to Earthquake Ground Shaking. 2000. Available online: https://www.atcouncil.org/pdfs/FEMA355Ctoc.pdf (accessed on 11 December 2023).
- UBC. Structural Engineering Design Provisions, Uniform Building Code. In Proceedings of the International Conference of Building Officials, Whittier, CA, USA, 16–19 April 1997. [Google Scholar]
- AISC Steel Construction Manual; American Institute of Steel Construction: Chicago, IL, USA, 2010.
- Roeder, C.W.; Schneider, S.P.; Carpenter, J.E. Seismic Behavior of Moment-Resisting Steel Frames: Analytical Study. J. Struct. Eng. 1993, 119, 1866–1884. [Google Scholar] [CrossRef]
- Leon, R.T.; Shin, K.J. Performance of Semi-Rigid Frames. In Proceedings of the Structures XIII Congress, Boston, MA, USA, 2–5 April 1995; ASCE: New York, NY, USA, 1995; pp. 1020–1035. [Google Scholar]
- Nader, M.N.; Astaneh, A. Dynamic Behavior of Flexible, Semirigid and Rigid Steel Frames. J. Constr. Steel Res. 1991, 18, 179–192. [Google Scholar] [CrossRef]
- Osman, A.; Ghobarah, A.; Korol, R.M. Implications of Design Philosophies for Seismic Response of Steel Moment Frames. Earthq. Eng. Struct. Dyn. 1995, 24, 127–143. [Google Scholar] [CrossRef]
- Schneider, S.P.; Roeder, C.W.; Carpenter, J.E. Seismic Behavior of Moment-Resisting Steel Frames: Experimental Study. J. Struct. Eng. 1993, 119, 1885–1902. [Google Scholar] [CrossRef]
- AISC Specification for Structural Steel Buildings; American Institute of Steel Construction: Chicago, IL, USA, 2010.
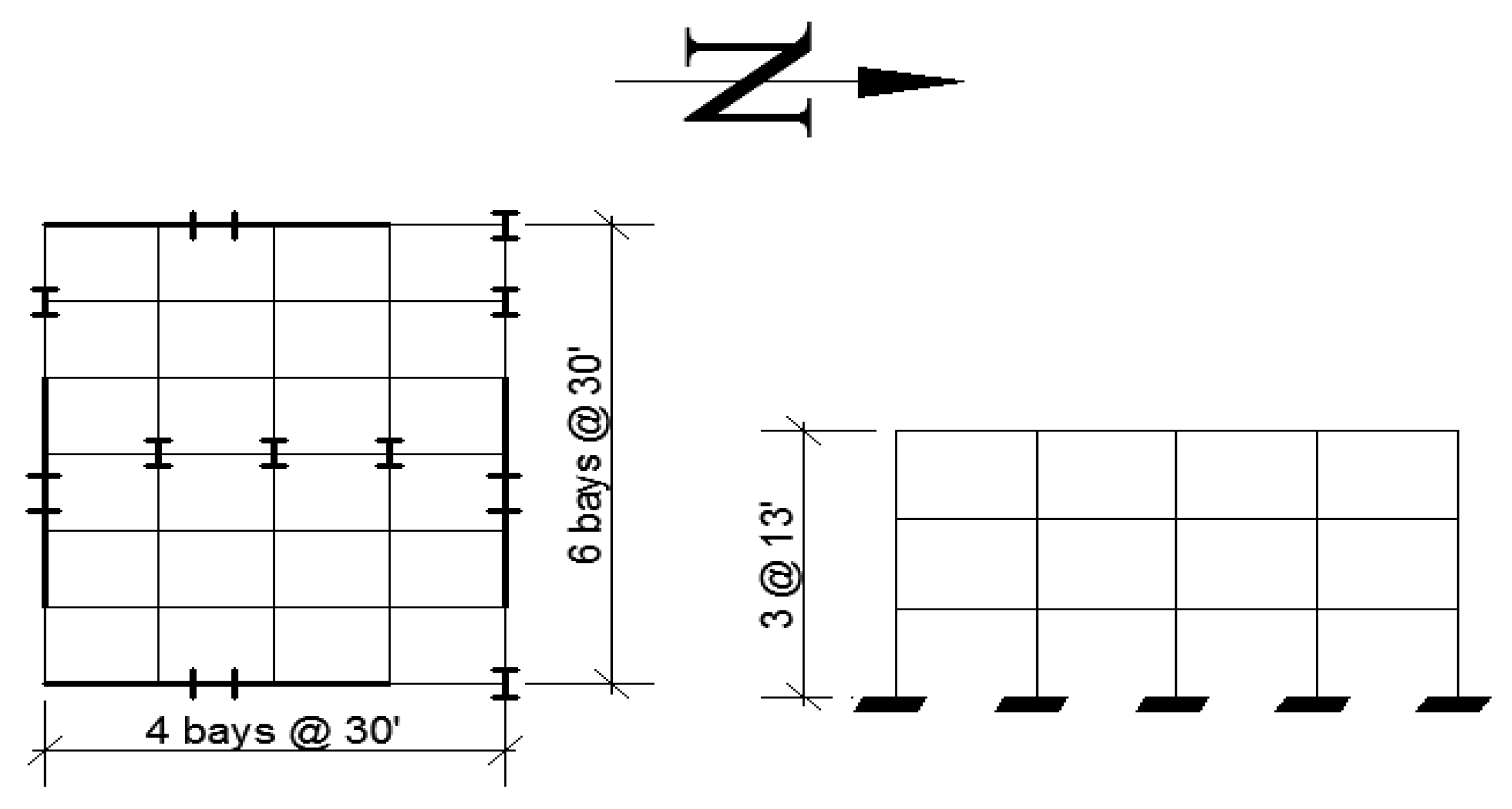
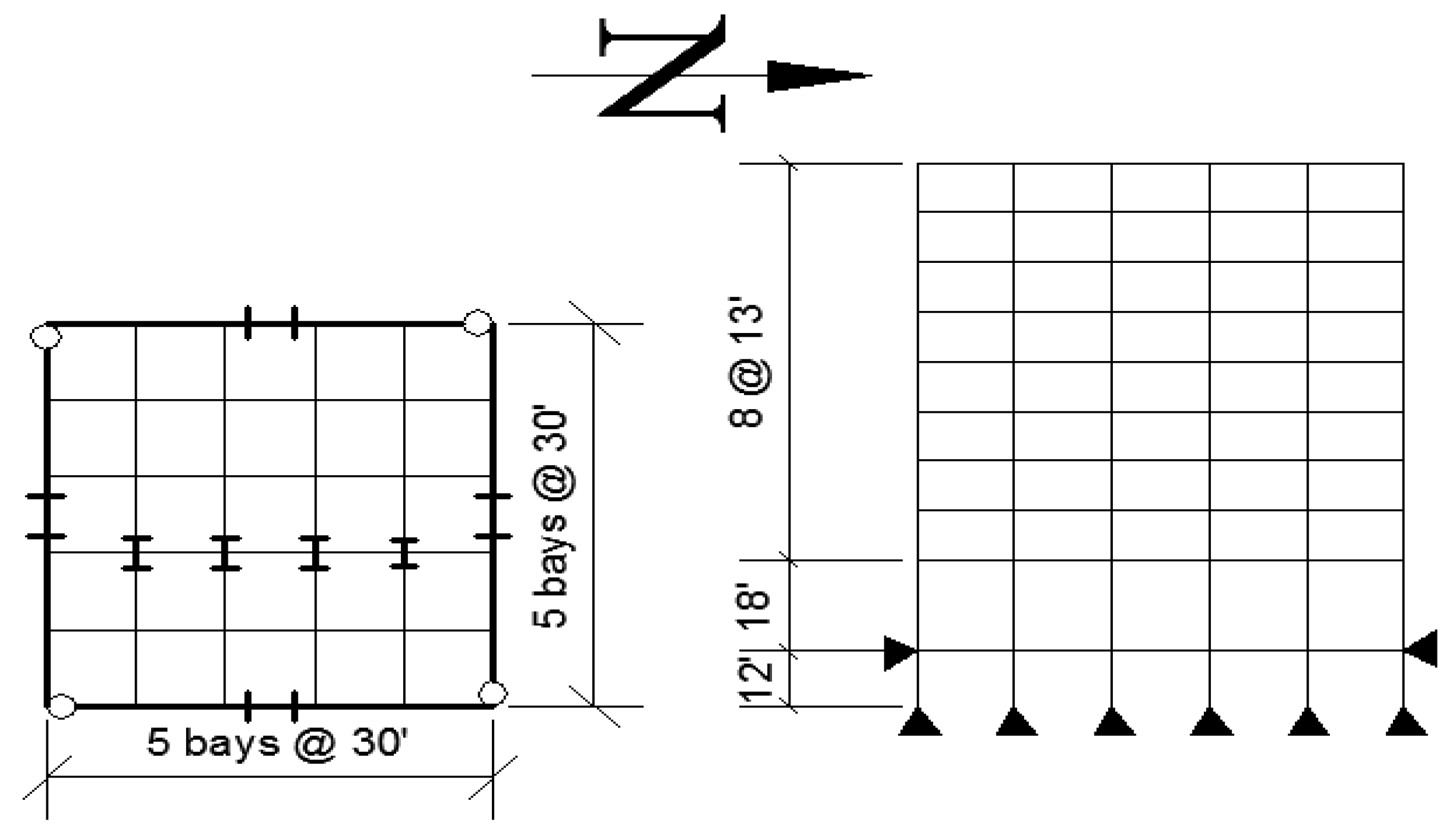




| Model | Story | Columns | Girders | |
|---|---|---|---|---|
| Outside | Inside | |||
| 3-story | 1 | W14 × 257 | W14 × 311 | W33 × 118 |
| 2 | W14 × 257 | W14 × 311 | W30 × 116 | |
| 3/Roof | W14 × 257 | W14 × 311 | W24 × 68 | |
| 9-story | Basement-1 | W14 × 370 | W14 × 500 | W36 × 160 |
| 1 | W14 × 370 | W14 × 500 | W36 × 160 | |
| 2 | W14 × 370 | W14 × 500 | W36 × 160 | |
| 3 | W14 × 370 | W14 × 455 | W36 × 135 | |
| 4 | W14 × 370 | W14 × 455 | W36 × 135 | |
| 5 | W14 × 283 | W14 × 370 | W36 × 135 | |
| 6 | W14 × 283 | W14 × 370 | W36 × 135 | |
| 7 | W14 × 257 | W14 × 283 | W30 × 99 | |
| 8 | W14 × 257 | W14 × 283 | W27 × 84 | |
| 9/roof | W14 × 233 | W14 × 257 | W24 × 68 | |
| Story | Columns | Girders | |
|---|---|---|---|
| Outside | Inside | ||
| Basement-1 | 15 × 15 × 2.00 | W24 × 335 | W14 × 22 |
| Basement-2 | 15 × 15 × 2.00 | W24 × 335 | W30 × 99 |
| 1 | 15 × 15 × 2.00 | W24 × 335 | W30 × 99 |
| 2 | 15 × 15 × 2.00 | W24 × 335 | W30 × 99 |
| 3 | 15 × 15 × 1.25 | W24 × 335 | W30 × 99 |
| 4 | 15 × 15 × 1.25 | W24 × 335 | W30 × 99 |
| 5 | 15 × 15 × 1.25 | W24 × 335 | W30 × 108 |
| 6 | 15 × 15 × 1.00 | W24 × 229 | W30 × 108 |
| 7 | 15 × 15 × 1.00 | W24 × 229 | W30 × 108 |
| 8 | 15 × 15 × 1.00 | W24 × 229 | W30 × 108 |
| 9 | 15 × 15 × 1.00 | W24 × 229 | W30 × 108 |
| 10 | 15 × 15 × 1.00 | W24 × 229 | W30 × 108 |
| 11 | 15 × 15 × 1.00 | W24 × 229 | W30 × 99 |
| 12 | 15 × 15 × 1.00 | W24 × 192 | W30 × 99 |
| 13 | 15 × 15 × 1.00 | W24 × 192 | W30 × 99 |
| 14 | 15 × 15 × 1.00 | W24 × 192 | W30 × 99 |
| 15 | 15 × 15 × 0.75 | W24 × 131 | W30 × 99 |
| 16 | 15 × 15 × 0.75 | W24 × 131 | W30 × 99 |
| 17 | 15 × 15 × 0.75 | W24 × 131 | W27 × 84 |
| 18 | 15 × 15 × 0.75 | W24 × 117 | W27 × 84 |
| 19 | 15 × 15 × 0.75 | W24 × 117 | W24 × 62 |
| 20/Roof | 15 × 15 × 0.50 | W24 × 84 | W21 × 50 |
| Model | Story | Columns | |
|---|---|---|---|
| Outside | Inside | ||
| 1 | 1/2 | W27 × 258 | W27 × 307 |
| 2/3 | W27 × 258 | W27 × 307 | |
| 3/roof | W27 × 258 | W27 × 307 | |
| 2 | −1/1 | W27 × 368 | W27 × 494 |
| 1/2 | W27 × 368 | W27 × 494 | |
| 2/3 | W27 × 368 | W27 × 494, W27 × 448 | |
| 3/4 | W27 × 368 | W27 × 448 | |
| 4/5 | W27 × 368, W27 × 281 | W27 × 448, W27 × 368 | |
| 5/6 | W27 × 281 | W27 × 368 | |
| 6/7 | W27 × 281, W27 × 258 | W27 × 368, W27 × 281 | |
| 7/8 | W27 × 258 | W27 × 281 | |
| 8/9 | W27 × 258, W27 × 235 | W27 × 281, W27 × 258 | |
| 9/roof | W27 × 235 | W27 × 258 | |
| Model | Story | Columns |
|---|---|---|
| Inside | ||
| 3 | Basement-1 | W40 × 328 |
| Basement-2 | W40 × 328 | |
| 1/2 | W40 × 328 | |
| 2/3 | W40 × 328 | |
| 3/4 | W40 × 328 | |
| 4/5 | W40 × 328 | |
| 5/6 | W40 × 328 | |
| 6/7 | W40 × 221 | |
| 7/8 | W40 × 221 | |
| 8/9 | W40 × 221 | |
| 9/10 | W40 × 221 | |
| 10/11 | W40 × 221 | |
| 11/12 | W40 × 221 | |
| 12/13 | W40 × 192 | |
| 13/14 | W40 × 192 | |
| 14/15 | W40 × 192 | |
| 15/16 | W40 × 131 | |
| 16/17 | W40 × 131 | |
| 17/18 | W40 × 131 | |
| 18/19 | W40 × 117 | |
| 19/20 | W40 × 117 | |
| 20/roof | W40 × 84 |
| Designation | Record Information | Magnitude (Mw) | PGA (m/s2) | Period (s) | ||
|---|---|---|---|---|---|---|
| NS | EW | NS | EW | |||
| LA1 | Imperial Valley, 1940 | 6.9 | 4.52 | 6.63 | 0.53 | 0.46 |
| LA2 | Imperial Valley, 1979 | 6.5 | 3.86 | 4.78 | 0.16 | 0.34 |
| LA3 | Landers, 1992 | 7.3 | 4.14 | 4.17 | 0.73 | 0.33 |
| LA4 | Kern, 1952 | 7.3 | 5.11 | 3.53 | 0.25 | 0.23 |
| LA5 | Loma Prieta, 1989 | 7 | 6.53 | 9.50 | 0.21 | 0.2 |
| LA6 | Northridge, 1994, Newhall | 6.7 | 6.65 | 6.45 | 0.31 | 0.31 |
| LA7 | Northridge, 1994, Rinaldi | 6.7 | 5.23 | 5.69 | 0.39 | 0.29 |
| LA8 | Northridge, 1994, Sylmar | 6.7 | 5.59 | 8.03 | 0.31 | 0.36 |
| LA9 | North Palm Springs, 1986 | 6 | 10.01 | 9.68 | 0.17 | 0.21 |
| LA10 | Coyote Lake, 1979 | 5.7 | 5.79 | 3.28 | 0.15 | 0.21 |
| LA11 | Morgan Hill, 1984 | 6.2 | 3.12 | 5.36 | 0.18 | 0.16 |
| LA12 | Parkfield, 1966, Cholame 5W | 6.1 | 7.65 | 6.20 | 0.37 | 0.3 |
| LA13 | Parkfield, 1966, Cholame 8W | 6.1 | 6.81 | 7.75 | 0.17 | 0.21 |
| LA14 | North Palm Springs, 1986 | 6 | 5.08 | 3.71 | 0.13 | 0.21 |
| LA15 | Whittier, 1987 | 6 | 7.54 | 4.70 | 0.7 | 0.28 |
| Model | Direction | Sa/g (1) | RDT (2) | RA | RM | ||
|---|---|---|---|---|---|---|---|
| EXT (3) | INT (4) | EXT (5) | INT (6) | ||||
| 1 | NS | 0.2 | 1.21 | 0.99 | 0.97 | 0.66 | 0.70 |
| 0.4 | 1.24 | 0.98 | 0.95 | 0.66 | 0.68 | ||
| 0.6 | 1.33 | 0.95 | 0.94 | 0.60 | 0.62 | ||
| 0.8 | 1.29 | 0.93 | 0.94 | 0.57 | 0.59 | ||
| 1.0 | 1.29 | 0.91 | 0.93 | 0.56 | 0.58 | ||
| 1.2 | 1.27 | 0.91 | 0.93 | 0.55 | 0.57 | ||
| EW | 0.2 | 1.38 | 1.01 | 0.98 | 0.68 | 0.73 | |
| 0.4 | 1.29 | 0.96 | 0.95 | 0.61 | 0.65 | ||
| 0.6 | 1.20 | 0.94 | 0.94 | 0.58 | 0.60 | ||
| 0.8 | 1.17 | 0.93 | 0.94 | 0.56 | 0.58 | ||
| 1.0 | 1.19 | 0.93 | 0.93 | 0.55 | 0.57 | ||
| 1.2 | 1.20 | 0.93 | 0.93 | 0.54 | 0.56 | ||
| 2 | NS | 0.1 | 1.22 | 0.99 | 0.98 | 0.78 | 0.84 |
| 0.2 | 1.16 | 0.98 | 0.97 | 0.78 | 0.83 | ||
| 0.3 | 1.17 | 0.98 | 0.97 | 0.74 | 0.79 | ||
| 0.4 | 1.11 | 0.97 | 0.97 | 0.71 | 0.75 | ||
| 0.5 | 1.06 | 0.98 | 0.97 | 0.69 | 0.72 | ||
| EW | 0.1 | 1.15 | 0.99 | 0.98 | 0.78 | 0.85 | |
| 0.2 | 1.13 | 0.98 | 0.98 | 0.77 | 0.82 | ||
| 0.3 | 1.13 | 0.97 | 0.97 | 0.71 | 0.76 | ||
| 0.4 | 1.08 | 0.96 | 0.97 | 0.68 | 0.72 | ||
| 0.5 | 1.06 | 0.96 | 0.97 | 0.68 | 0.70 | ||
| 3 | NS | 0.05 | 0.97 | 0.93 | 0.99 | 0.67 | 0.70 |
| 0.10 | 1.02 | 0.95 | 0.98 | 0.68 | 0.70 | ||
| 0.15 | 1.01 | 0.97 | 0.99 | 0.66 | 0.68 | ||
| 0.20 | 1.01 | 0.96 | 0.98 | 0.64 | 0.65 | ||
| 0.25 | 1.01 | 0.96 | 0.98 | 0.65 | 0.66 | ||
| EW | 0.05 | 0.99 | 0.95 | 1.00 | 0.70 | 0.74 | |
| 0.10 | 1.00 | 0.96 | 0.99 | 0.69 | 0.71 | ||
| 0.15 | 1.00 | 0.99 | 0.99 | 0.66 | 0.68 | ||
| 0.20 | 1.01 | 0.98 | 0.99 | 0.66 | 0.67 | ||
| 0.25 | 1.01 | 0.98 | 0.98 | 0.65 | 0.65 | ||
| Model | Direction | Sa/g | RAM | |
|---|---|---|---|---|
| EXT | INT | |||
| 1 | NS | 0.2 | 1.14 | 1.19 |
| 0.4 | 1.13 | 1.15 | ||
| 0.6 | 1.05 | 1.05 | ||
| 0.8 | 0.99 | 1.00 | ||
| 1.0 | 0.98 | 0.99 | ||
| 1.2 | 0.96 | 0.97 | ||
| EW | 0.2 | 1.17 | 1.23 | |
| 0.4 | 1.06 | 1.10 | ||
| 0.6 | 1.01 | 1.02 | ||
| 0.8 | 0.97 | 0.98 | ||
| 1.0 | 0.96 | 0.97 | ||
| 1.2 | 0.95 | 0.96 | ||
| 2 | NS | 0.1 | 1.24 | 1.31 |
| 0.2 | 1.24 | 1.31 | ||
| 0.3 | 1.19 | 1.24 | ||
| 0.4 | 1.13 | 1.19 | ||
| 0.5 | 1.10 | 1.14 | ||
| EW | 0.1 | 1.24 | 1.32 | |
| 0.2 | 1.22 | 1.30 | ||
| 0.3 | 1.14 | 1.19 | ||
| 0.4 | 1.08 | 1.13 | ||
| 0.5 | 1.08 | 1.11 | ||
| 3 | NS | 0.05 | 1.03 | 1.03 |
| 0.10 | 0.98 | 1.05 | ||
| 0.15 | 0.99 | 1.03 | ||
| 0.20 | 0.97 | 0.99 | ||
| 0.25 | 0.98 | 0.99 | ||
| 0.30 | 0.98 | 0.99 | ||
| EW | 0.05 | 1.07 | 1.10 | |
| 0.10 | 1.00 | 1.06 | ||
| 0.15 | 1.01 | 1.02 | ||
| 0.20 | 1.00 | 1.01 | ||
| 0.25 | 0.99 | 0.99 | ||
| 0.30 | 0.99 | 0.99 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Valenzuela-Beltrán, F.; Llanes-Tizoc, M.D.; Bojorquez, E.; Bojorquez, J.; Leal-Graciano, J.M.; Baca, V.; Chavez, R.; Reyes-Salazar, A. Comparison between the Dynamic Responses of Steel Buildings with Medium and Deep Columns under Several Seismic Intensities. Appl. Sci. 2024, 14, 5067. https://doi.org/10.3390/app14125067
Valenzuela-Beltrán F, Llanes-Tizoc MD, Bojorquez E, Bojorquez J, Leal-Graciano JM, Baca V, Chavez R, Reyes-Salazar A. Comparison between the Dynamic Responses of Steel Buildings with Medium and Deep Columns under Several Seismic Intensities. Applied Sciences. 2024; 14(12):5067. https://doi.org/10.3390/app14125067
Chicago/Turabian StyleValenzuela-Beltrán, Federico, Mario D. Llanes-Tizoc, Eden Bojorquez, Juan Bojorquez, J. M. Leal-Graciano, Victor Baca, Robespierre Chavez, and Alfredo Reyes-Salazar. 2024. "Comparison between the Dynamic Responses of Steel Buildings with Medium and Deep Columns under Several Seismic Intensities" Applied Sciences 14, no. 12: 5067. https://doi.org/10.3390/app14125067
APA StyleValenzuela-Beltrán, F., Llanes-Tizoc, M. D., Bojorquez, E., Bojorquez, J., Leal-Graciano, J. M., Baca, V., Chavez, R., & Reyes-Salazar, A. (2024). Comparison between the Dynamic Responses of Steel Buildings with Medium and Deep Columns under Several Seismic Intensities. Applied Sciences, 14(12), 5067. https://doi.org/10.3390/app14125067








