1. Introduction
In the process of producing, maintaining, and servicing concrete components, it is challenging to completely eliminate initial defects, such as pores, voids, and micro-cracks, due to various factors, including production technology, maintenance conditions, and the external environment [
1,
2,
3]. As a result of applied loads and exposure to external environmental conditions, these microscopic defects within the component progressively expand and interconnect, leading to the formation of macroscopic cracks and, in severe cases, damage to the entire component.
Indeed, the cracking of the concrete protective layer represents only one form of structural crack. The presence and propagation of various types of cracks not only lead to stress redistribution within concrete structures but also alter the distribution of influencing factors, such as chloride ions and temperature, among others. These changes can significantly impact the corrosion of steel bars within the structure [
4,
5,
6].
The shear failure of reinforced concrete beams typically constitutes a brittle failure, presenting a challenge in providing early warnings as there are minimal discernible signs prior to failure. The risk associated with shear failure is greater than that of bending failure due to the lack of obvious warnings before the occurrence of failure [
7,
8,
9]. Moreover, the propagation and evolution of concrete cracks contribute to the deterioration of the physical and mechanical properties of concrete members, including load-bearing capacity and deformation performance. Consequently, this degradation can impact the safety, applicability, and durability of the structure. Given these considerations, it becomes essential to thoroughly investigate the propagation of cracks during the failure process of reinforced concrete beams.
Rombach [
10] performed numerical and continuum media mechanics simulations of flexural shear crack propagation in concrete beams without transverse reinforcement. The study involved using numerical and continuum media mechanics simulations to investigate flexural shear crack propagation in concrete beams without transverse reinforcement. The researchers utilized concrete damage plastic material modeling to develop the model, incorporating the crack propagation method and the diffuse cracking method. By comparing the crack patterns observed in actual beam tests with the results obtained from different finite element models, the study aimed to gain insights into crack propagation and internal force redistribution within concrete members. Crack width, interface roughness between cracks, and concrete strength have an effect on the shear transfer capacity of the cracked interface [
11,
12]. Yang [
13] conducted an experimental study on the shear performance of continuous beams of lightweight aggregate concrete and fitted a discount factor for the shear capacity, which was related to the maximum aggregate particle size, density, and splitting tensile strength of lightweight aggregate concrete.
Yin [
14] derived an expression for crack propagation resistance in ordinary three-point bending concrete specimens and investigated the influence of the span/height ratio on the fracture process of three-point bending concrete beams. The findings indicate that the crack propagation resistance curve exhibits an increase with crack propagation and both the curve and the fracture process zone curve are unaffected by the span/height ratio. However, they do demonstrate an increase with the specimen height. Wu [
15] presents an analytical method for determining the resistance curve to crack extension in concrete. It is assumed that crack extension starts when the difference between the stress intensity factor due to external force and the stress intensity factor due to cohesive force in the fracture process zone is equal to the initial crack toughness. Based on this assumption, the crack extension resistance curve of concrete can be analytically expressed as the sum of the stress intensity factor induced by the external force, or the initial crack toughness, and the stress intensity factor obtained from the bond stresses within the fracture process zone.
According to the development pattern, the surface cracks of reinforced concrete are mostly open-type (type I, opening mode) cracks: under the action of tensile stress orthogonal to the crack surface, the crack surface produces opening displacement (displacement is orthogonal to the crack surface) and the upper and lower displacements are opposite; sliding mode (type II, sliding mode) cracks: under the shear stress parallel to the crack surface and perpendicular to the crack tip line, the crack surface produces relative slip (displacement parallel to the shear stress direction); and tear-type (type III, anti-plane shear mode) cracks: under the shearing stress perpendicular to the crack surface and parallel to the crack tip line, the crack surface produces three kinds of relative slip (displacement parallel shear stress direction) along the crack surface. In the process of shear failure, the internal stress state of reinforced concrete beams is complicated and the surface cracks are mostly type I-II compound cracks. The propagation of opening-type cracks (type I) and sliding-type cracks (type II) is prone to low-stress brittle fracture [
16], which accords with the characteristics of brittle materials, such as concrete and rock.
By exploring the propagation law of damaged crack defects, it is hoped to describe their mechanical properties in essence. The direct way to solve this problem is to study the propagation law of damage fracture defects. However, it is difficult for classical fracture mechanics to describe the damage degree of a concrete crack tip through the mesoscopic level [
17]. Therefore, by considering the mechanical characteristics of cracks and pores inside concrete as a damage field with coordinated deformation and continuous force, the development and propagation law of damage cracks inside concrete based on the above ideas can fully reflect the bearing capacity and failure characteristics of concrete materials [
18].
The innovation of this paper is that a prediction model for crack development and the evolution of concrete beams is developed by considering the nonlinear damage constitutive model of concrete. Through the damage distribution law in the finite element model, the development and evolution of concrete cracks are predicted, so as to identify the damage process of concrete beams and provide design references for complex projects. In order to accurately simulate the mechanical properties of reinforced concrete under reciprocating loads, a nonlinear finite element model of Abaqus was established based on the constitutive relation considering the cyclic loading characteristics of steel. In order to verify the reliability of the finite element analysis results in this paper, the finite element analysis results of simple supported beams are compared with the test results. The reciprocating loading modes of beam end-controlled load and displacement-controlled load are proposed, respectively, which can simulate the effects of horizontal earthquake, gravity load, and axial pressure on reinforced concrete frame joints. The law of shear crack propagation in reinforced concrete frame joints in practical engineering is investigated systematically.
2. Comparison of Finite Element Model and Experiment Results
2.1. Overview of the Test
To validate the accuracy of the finite element modeling, an Abaqus model is created based on the experimental investigation of crack propagation patterns in reinforced concrete simply supported beams documented in the literature [
19]. The test beams have cross-sectional dimensions of 150 mm × 250 mm, a total length of 1800 mm, and a calculated span of 1500 mm. The pads used in the experiment have cross-sectional dimensions of 150 mm × 60 mm × 20 mm. The reinforcement details are provided in
Table 1 and the dimensions and reinforcement arrangement of the test beams can be seen in
Figure 1, based on the results of the experimental study.
Table 2 shows the reinforced concrete beams of different sizes. The effective spans are all 1500 mm but the shear span to effective depth ratios and the stirrup ratio are different. Taking JL-1.5-6-150 as an example, it indicates that the shear span to effective depth ratio is 1.5, the stirrup diameter is 6 mm, and the stirrup spacing is 150 mm.
Table 2.
Parameters of reinforced concrete beams.
Table 2.
Parameters of reinforced concrete beams.
| Species | Effective Span/mm | Shear Span to Effective Depth Ratio | Concentrated Load Span/mm | Stirrup Spacing | Stirrup Ratio/% |
|---|
| JL-1.5-6-150 | 1500 | 1.5 | 338 | 6@150 | 0.25 |
| JL-1.5-8-200 | 1500 | 1.5 | 338 | 8@200 | 0.36 |
| JL-1.5-8-150 | 1500 | 1.5 | 338 | 8@150 | 0.45 |
| JL-2.0-6-150 | 1500 | 2.0 | 450 | 6@150 | 0.25 |
| JL-2.0-8-200 | 1500 | 2.0 | 450 | 8@200 | 0.36 |
| JL-2.0-8-150 | 1500 | 2.0 | 450 | 8@150 | 0.45 |
| JL-2.5-6-150 | 1500 | 2.5 | 563 | 6@150 | 0.25 |
| JL-2.5-8-200 | 1500 | 2.5 | 563 | 8@200 | 0.36 |
| JL-2.5-8-150 | 1500 | 2.5 | 563 | 8@150 | 0.45 |
Figure 2.
Test beam stirrup strain gauge position.
Figure 2.
Test beam stirrup strain gauge position.
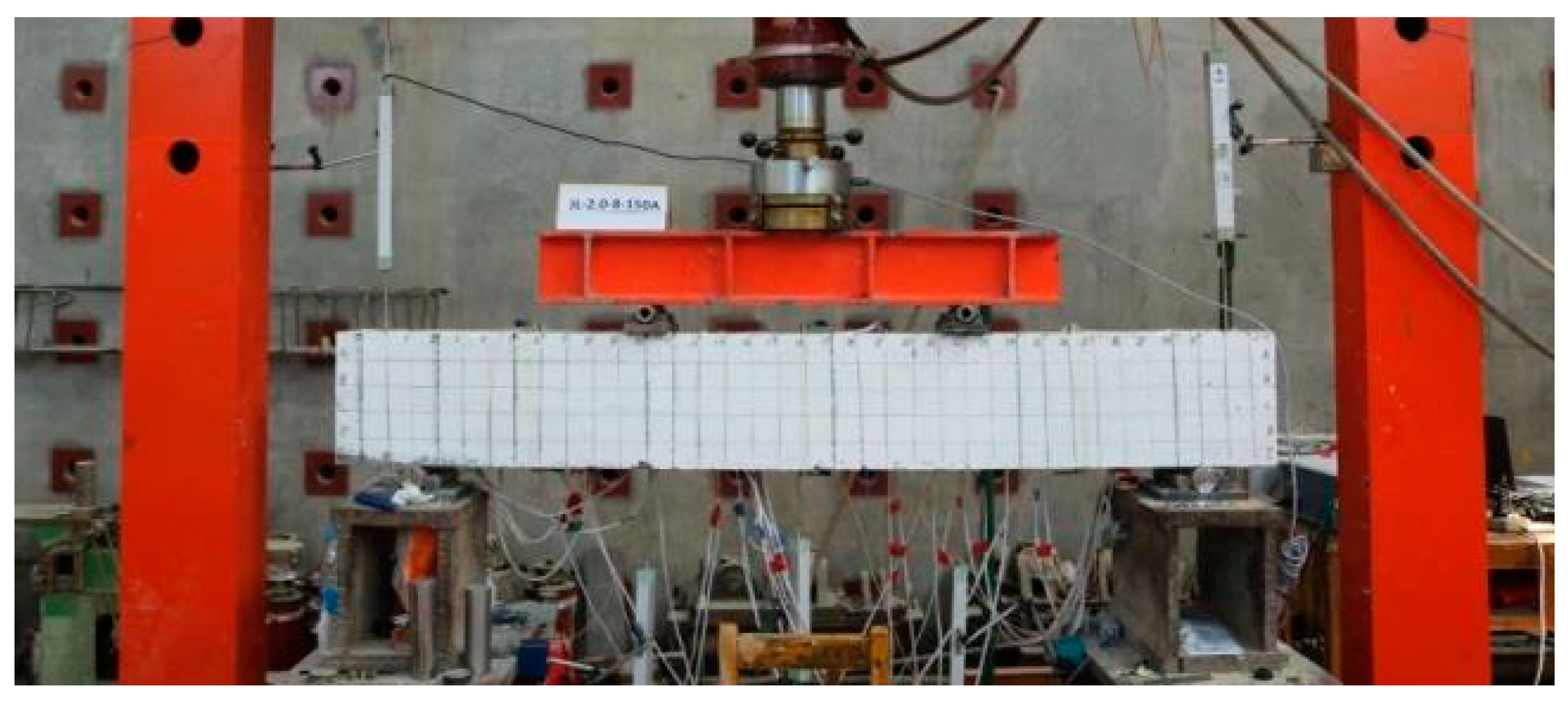
Figure 3.
Reinforced concrete beam loading test device from reference [
19].
Figure 3.
Reinforced concrete beam loading test device from reference [
19].
It should be noted that the experimental designs all used over-reinforced beams. The failure mode of concrete beams under bending is ductile; however, shear failure, if it occurs, tends to be brittle [
20]. Shear failure poses a significantly higher risk compared to bending failure as it often lacks clear warning signs [
21]. In practical projects, controlling the shear-to-span ratio and stirrup ratio helps mitigate the occurrence of shear failure in the inclined section of concrete beams. To elucidate the evolution of crack propagation in shear beams, it becomes necessary to conduct experimental studies on beams regarding more dangerous shear failure. Consequently, the tests use different shear-to-span ratios and stirrup ratios, employing an over-reinforced beam design. By doing so, it can be ensured that the concrete beam would not experience bending failure but rather shear-compression failure.
As shown in
Figure 4, each test beam was damaged in shear-compression damage. Critical diagonal cracks were formed along the line between the loading point and the support, the concrete at the upper end of the diagonal cracks was crushed by the pressure, and obvious flexural deformation occurred. The cracks in the pure bending section in the span of the beam basically extended vertically upward but none of them developed to the top of the test beam. The cracks in the shear-bending section were more abundant and denser and intersected and penetrated each other to form a crack that ran through the height of the test beam.
2.2. Material Constitute of Concrete in the FE Model
The stress–strain relationship employed in the finite element model corresponds to the constitutive relation of concrete, as specified in the Code for the Design of Concrete Structures (GB 50010-2010) [
22]. During the elastic phase, the stress–strain relationship of concrete is determined by its Young’s modulus
E and Poisson’s ratio
λ. In the inelastic phase, the stress–strain relationship of concrete is determined by the aforementioned code. The stress–strain relation of concrete under axial compression can be described by Equations (1)–(5):
where
represents the representative value of the axial compressive strength of concrete, which is measured at 22.4 MPa according to [
19];
represents the modulus of elasticity of concrete, which is taken as 30,904.1 MPa;
represents the descending parameter value of the axial compression stress–strain curve of concrete, set as 1.563;
is the peak compressive strain of concrete corresponding to the representative value of the axial compressive strength
of concrete, set as 0.001696; and
is the propagation parameter of concrete axial compression damage.
Figure 5 shows the stress–strain curves and axial compressive damage–strain curves of concrete under axial compression, respectively.
The axial tensile stress–strain curve of concrete can be calculated according to Equations (6)–(9):
where
represents the representative value of the axial tensile strength of concrete, which is taken as 2.2 MPa according to the code [
22];
represents the descending parameter value of the axial tensile stress–strain curve of concrete, set as 1.53;
is the peak tensile strain of concrete corresponding to the representative value of axial tensile strength
, set as 0.0000998; and
is the propagation parameter of concrete axial tensile damage.
Figure 6 shows the axial tensile stress–strain curve of concrete and the axial tensile damage–strain curve of concrete, respectively.
By combining the data calculated from the above formulas with the plastic damage constitutive model provided by Abaqus [
23], the shear failure test results of the simply supported beams are verified accordingly. The hardening model in Abaqus follows the von Mises yield criterion and has a good ability to simulate the nonlinear material of concrete.
2.3. Finite Element Model Building
In Abaqus, three-dimensional solid C3D8R elements are used for concrete and two-node three-dimensional linear truss T3D2 elements are used for reinforcement. Rigid pads are used to transfer the concentrated forces and the pads are C3D8R elements. The reinforcement cage is embedded in the concrete.
The mesh type for the pads and concrete is a hexahedral mesh with a minimum size of 20 mm and the reinforcement is a linear element with a minimum size of 30 mm to ensure sufficient accuracy. Previously, in order to more accurately simulate the dynamic damage evolution of concrete beams, an attempt was made to control the concrete mesh within 10 mm. However, due to the small mesh size, iterative calculations were difficult and the results did not converge and could not be completed. For the mesh of concrete materials, it is not the smaller the better. The smaller the mesh, the smaller the peak strain exhibited by the concrete model in Abaqus and the faster the bearing capacity decreases after reaching the peak value; the simulated bearing capacity of the concrete structure is obviously too large and the gap between the simulated stress–strain relationship of the concrete and the actual is too large. At the same time, too small a mesh also creates the problems of computational convergence and high cost of computational time. Therefore, a mesh size of 30–100 mm is generally selected in the simulation. On the other hand, too large a mesh size also affects the simulated damage cracking effect. Therefore, a grid size of 20 mm is the most appropriate in this paper. The meshing results of reinforced concrete beams are shown in
Figure 7.
2.4. Comparison of the Experimental and Finite Element Modeling of Strains
The load–strain curves of the bottom longitudinal reinforcement in the test are compared with the finite element simulation results, as shown in
Figure 8, and the test results are in good agreement with the finite element simulation structure. It can be seen that for the RC beams with different shear-to-span ratios, the load–strain curves of the bottom longitudinal reinforcement are close to the linear relationship without inflection points, indicating that the longitudinal reinforcement at the bottom of the reinforced concrete beams has not yet reached the yield state. The error of finite element simulation in predicting the strain–load curve of the longitudinal reinforcement, compared to the test, is reflected in the specimen JL-2.5-8-200, which is 9.83%.
Based on the data from both the test and the finite element simulation, as shown in
Figure 9, it is evident that the JL-2.5-8-200 test beam enters the elastic–plastic state earlier than the test beams with shear span to effective depth ratios of 1.5 and 2.0. When the load reaches approximately 70 kN, the test beam transitions into the elastic–plastic state, which aligns well with the test results. Throughout the entire loading process, the test beam exhibits a complete elastic–elastic–plastic damage progression. Initially, before the test beam develops cracks (elastic stage), the deflection increases linearly with the applied load. Once cracking initiates (elastic–plastic stage), the rate of deflection increase accelerates. As the load continues to increase (failure stage), the deflection grows rapidly.
The load–stirrup strain curve shows that in the stage of slight damage, before the concrete cracks, the shear force in this stage is mainly borne by the concrete and the shear force borne by the stirrup is very weak; thus, the stirrup strain of the test beam is extremely small and fluctuates around 0.
As the beam transitions into the stage of stable damage growth, the shear force is gradually transferred from the concrete to the stirrup, particularly when the bending shear inclined crack appears. As a result of the shear stress, the stirrup strain increases gradually. With the continued increase in shear force, multiple abdominal shear oblique cracks appear and their widths continue to expand. The shear force borne by the stirrup increases significantly and reaches a second inflection point where the stirrup strain increases rapidly.
Figure 10 indicates that the strain at stirrup-1 agrees well with the test results; whereas, the strain growth rate at stirrup-2 is lower than the test results in the comparison between the original test and finite element simulation results but the trend remains the same.
The longitudinal strain–load curve, stirrup strain–load curve, and deflection curve are in good agreement with those obtained by Abaqus finite element analysis; however, the interaction and slip between concrete and steel bars are not considered, resulting in a small strain and the calculated deflection of steel bars, that is, a large calculated stiffness.
2.5. Comparison of the Experimental and Finite Element Modeling of Cracks
In the process of concrete propagation, cracks often bifurcate and skew due to the lack of coordination in deformation among different materials within the concrete. The development direction of cracks is uncertain. In concrete members, the presence and propagation of cracks serve as direct indicators of damage to the member. Therefore, evaluating the degree of damage to concrete based on the progression of cracks holds practical significance.
As shown in
Figure 11, in the process of the shear failure of the test beam, due to the stress concentration at the loading point and support, the cracking phenomenon occurred at the support. Stress concentration at the loading point and support led to cracking in these areas. As the load increased, the mid-span bending moment reached the cracking moment, resulting in the appearance of the first crack in the pure bending section of the concrete beam. The test beam entered the stage of damage stabilization development, with small cracks appearing at the bottom of the shear bending section and relatively stable development. New cracks appeared on the surface of the test beam at the beginning of loading and expanded steadily as the load increased. At the 60 kN load, cracks in the pure bending section extended upwards and a bending shear inclined crack developed adjacent to this section due to the combined effect of shear force and bending moment. At the 120 kN load, the depth of the web shear inclined crack near the upper surface of the test beam continued to increase. When the load reached 140 kN, most of the concrete in the test beam was crushed and new cracks appeared in the shear section, expanding continuously. Eventually, the diagonal cracks in the shear section connected with each other and there was a slight increase in the depth of the cracks in the pure section.
Figure 11 illustrates the damage process of the beam. At the stage of stable damage development, the small cracks appearing at the bottom of the beam bending section do not have a large increase in width, stabilizing at about 0.3–0.4 mm; however, the number of them keeps increasing, which results in a smaller spacing of the cracks and a denser crack region. The concrete plastic damage model, while useful for quantitatively deriving the percentage of concrete damage, may not fully capture the width, spacing, and depth of macroscopic cracks. However, it can qualitatively demonstrate the development direction and propagation process of cracks through the DAMAGET distribution. It provides quantitative information on the extent of concrete damage; a complementary qualitative assessment of crack development and propagation is facilitated by the DAMAGET distribution.