Reliability Assessment of Statistical Distributions for Analyzing Dielectric Breakdown Strength of Polypropylene
Abstract
:1. Introduction
2. Statistical Distribution
2.1. Normal Distribution
2.2. Log-Normal Distribution
2.3. Exponential Distribution
2.4. Weibull Distribution
3. Evaluation of the Fit of Estimated Distributions
4. Data Acquisition
5. Results
6. Discussion
7. Conclusions
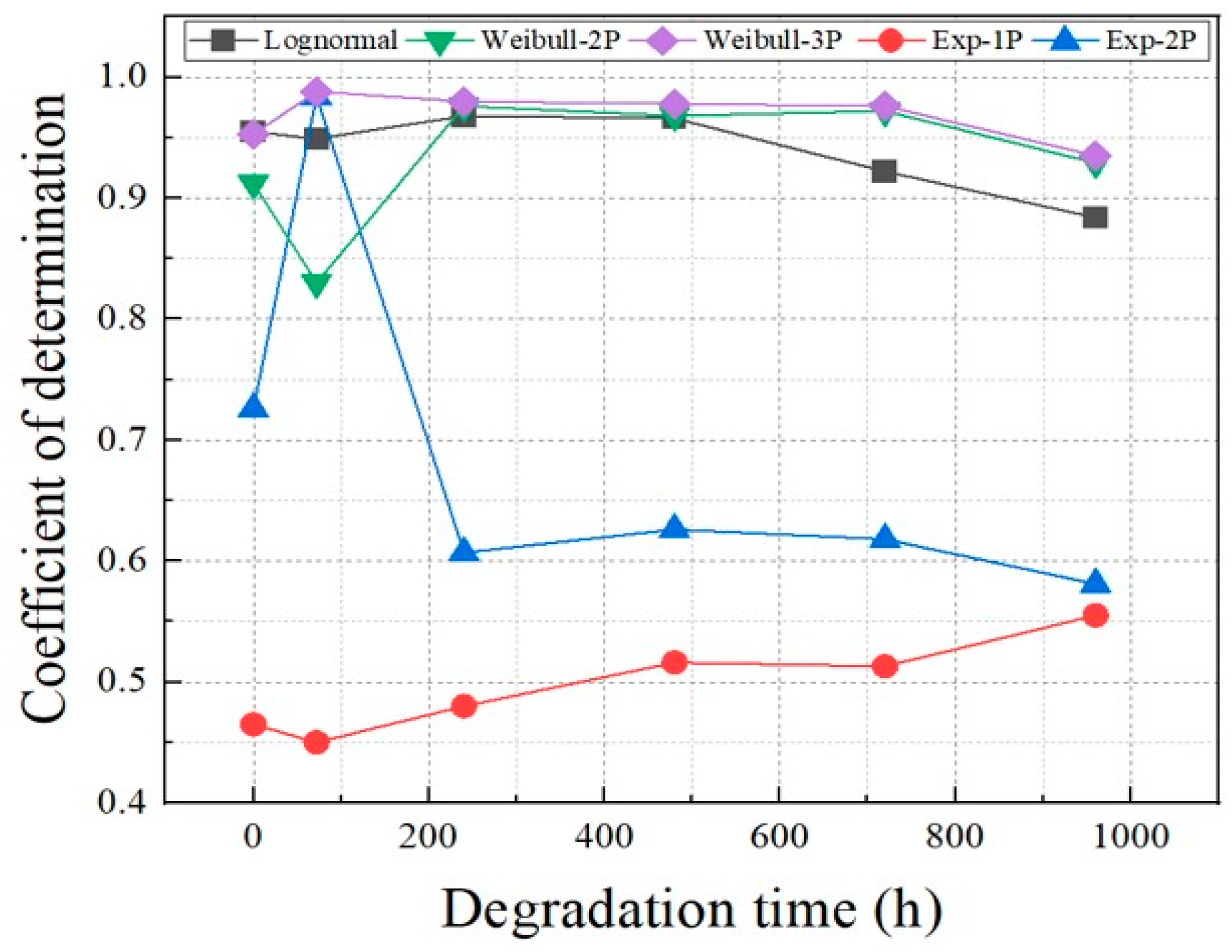
- The optimized statistical distribution may vary before and after degradation, emphasizing the importance of interpreting data based on this distinction.
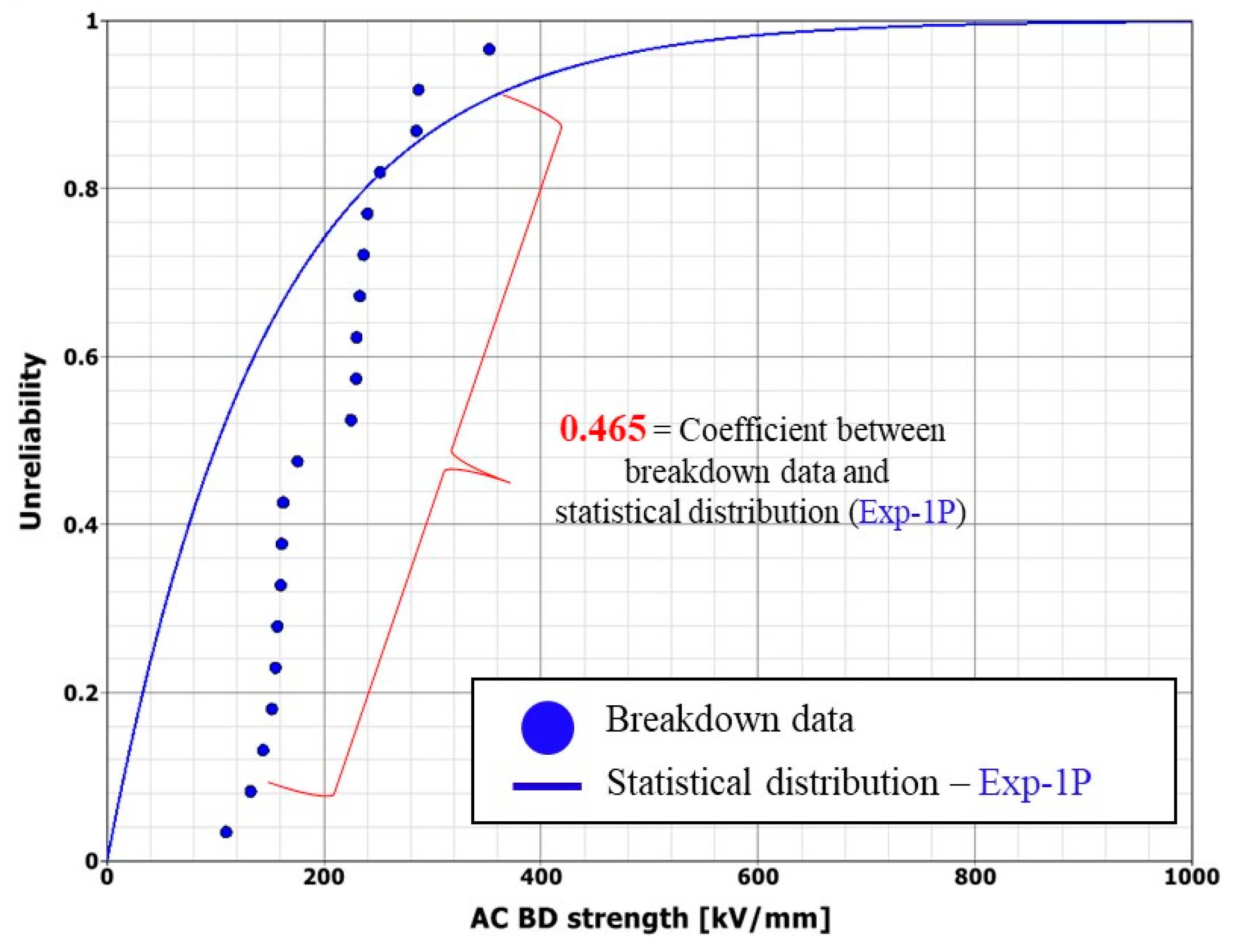
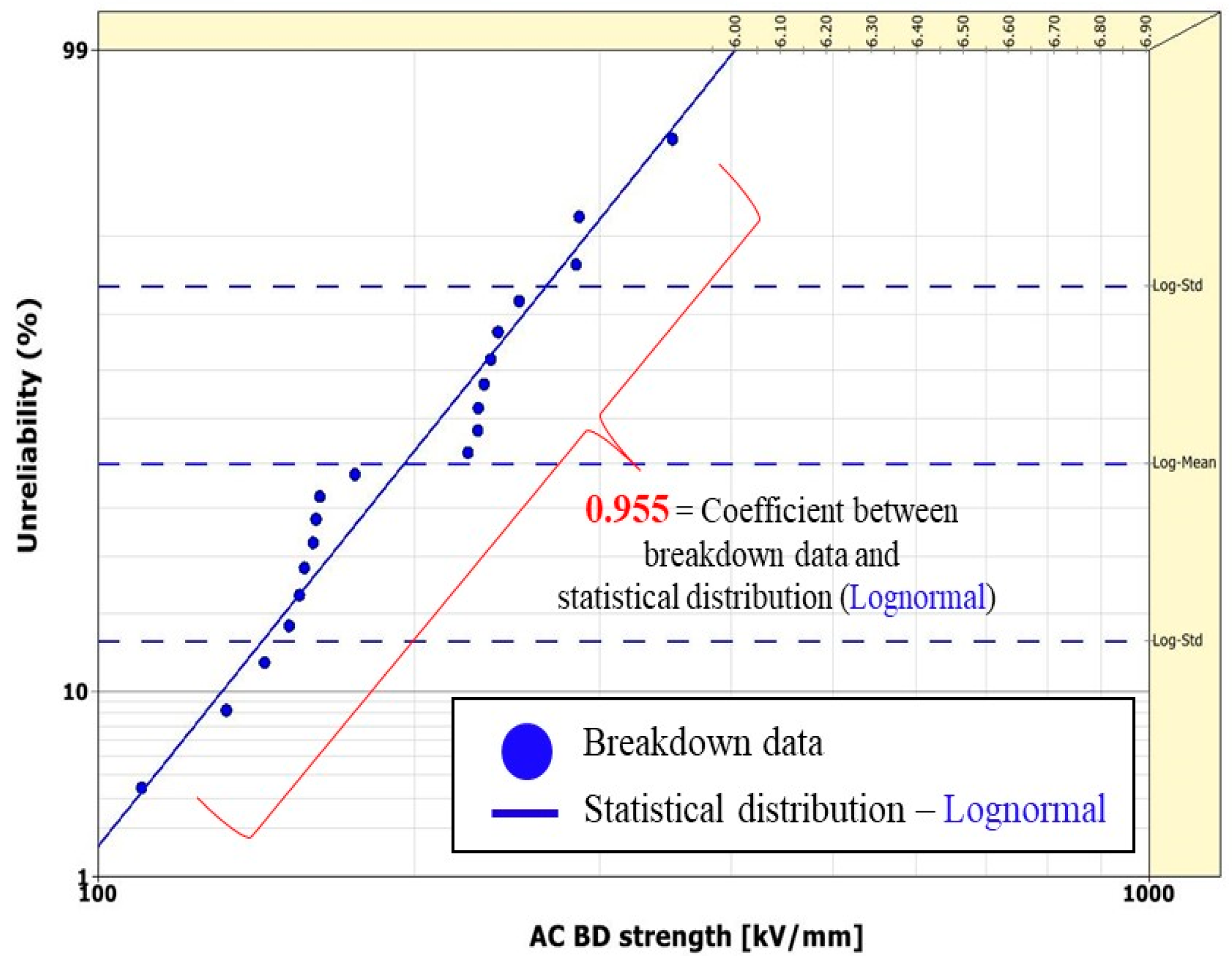
- A statistical distribution suitable for degradation conditions was selected using the coefficient of determination. Before degradation, the log-normal distribution proved suitable with a coefficient of determination of 0.955. After 960 h of degradation at 130 °C, the Weibull distribution exhibited the most suitable coefficient of determination at 0.929. Consequently, interpreting the breakdown data after degradation by using the Weibull distribution is reasonable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Preetha, P.; Joy Thomas, M. AC breakdown characteristics of epoxy nanocomposites. IEEE Trans. Dielectr. Electr. Insul. 2011, 18, 1526–1534. [Google Scholar] [CrossRef]
- Martin, D.; Wang, Z.D. Statistical analysis of the AC breakdown voltages of ester based transformer oils. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 1044–1050. [Google Scholar] [CrossRef]
- Imai, T.; Sawa, F.; Ozaki, T.; Inoue, Y.; Shimizu, T.; Tanaka, T. Comparison of insulation breakdown properties of epoxy nanocomposites under homogeneous and divergent electric fields. In Proceedings of the IEEE Conference on Electrical Insulation and Dielectric Phenomena, Auburn, AL, USA, 6–9 October 2006; Volume 2006. [Google Scholar] [CrossRef]
- Hallinan, A.J., Jr. A review of the Weibull distribution. J. Qual. Technol 1993, 25, 85–93. [Google Scholar] [CrossRef]
- Rinne, H. The Weibull Distribution: A Handbook; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Khalili, A.; Kromp, K. Statistical properties of Weibull estimators. J. Mater. Sci. 1991, 26, 6741–6752. [Google Scholar] [CrossRef]
- Beroual, A.; Khaled, U.; Mbolo Noah, P.; Sitorus, H. Comparative study of breakdown voltage of mineral, synthetic and natural oils and based mineral oil mixtures under AC and DC voltages. Energies 2017, 10, 511. [Google Scholar] [CrossRef]
- Khan, A.A.; Malik, N.; Al-Arainy, A.; Alghuwainem, S. A review of condition monitoring of underground power cables. In Proceedings of the IEEE International Conference on Condition Monitoring and Diagnosis, Bali, Indonesia, 23–27 September 2012; Volume 2012. [Google Scholar] [CrossRef]
- Goegebeur, Y.; Guillou, A. Goodness-of-fit testing for Weibull-type behavior. J. Stat. Plan. Inference 2010, 140, 1417–1436. [Google Scholar] [CrossRef]
- Evans, J.W.; Johnson, R.A.; Green, D.W. Two- and Three-Parameter Weibull Goodness-of-Fit Tests; United States Department of Agriculture, Forest Service, Forest Products Laboratory: Madison, WI, USA, 1989; Volume 493. [Google Scholar]
- Krit, M.; Gaudoin, O.; Remy, E. Goodness-of-fit tests for the Weibull and extreme value distributions: A review and comparative study. Commun. Stat.-Simul. Comput. 2021, 50, 1888–1911. [Google Scholar] [CrossRef]
- Littell, R.C.; Mc Clave, J.T.M.; Offen, W.W. Goodness-of-fit tests for the two parameter Weibull distribution. Commun. Stat.-Simul. Comput. 1979, 8, 257–269. [Google Scholar] [CrossRef]
- Parsons, F.G.; Wirsching, P.H. A Kolmogorov-Smirnov goodness-of-fit test for the two-parameter Weibull distribution when the parameters are estimated from the data. Microelectron. Reliab. 1982, 22, 163–167. [Google Scholar] [CrossRef]
- IEC 60243–1:2013; Electric Strength of Insulating Materials–Test Methods–Part 1: Tests at Power Frequencies. IEC: Geneva, Switzerland, 2013.
- Kurimoto, M.; Takenaka, Y.; Murakami, Y.; Nagao, M. Compatibility evaluation between dielectric breakdown strength and thermal conduction in epoxy/boron-nitride composite. In Proceedings of the Annual Report Conference on Electrical Insulation and Dielectric Phenomena IEEE, Cancun, Mexico, 16–19 October 2011; Volume 2011. [Google Scholar] [CrossRef]
- Lim, J.-S.; Kim, S.H.; Lee, S.W.; Kim, Y.H.; Jung, Y.H. A Weibull Statistical Analysis of High Thermal Resistance Insulation Material through AC Breakdown Characteristic of Mckewon Electrode. In Proceedings of the KIEE Conference; The Korean Institute of Electrical Engineers: Gangnam-gu, Republic of Korea, 2015. [Google Scholar]
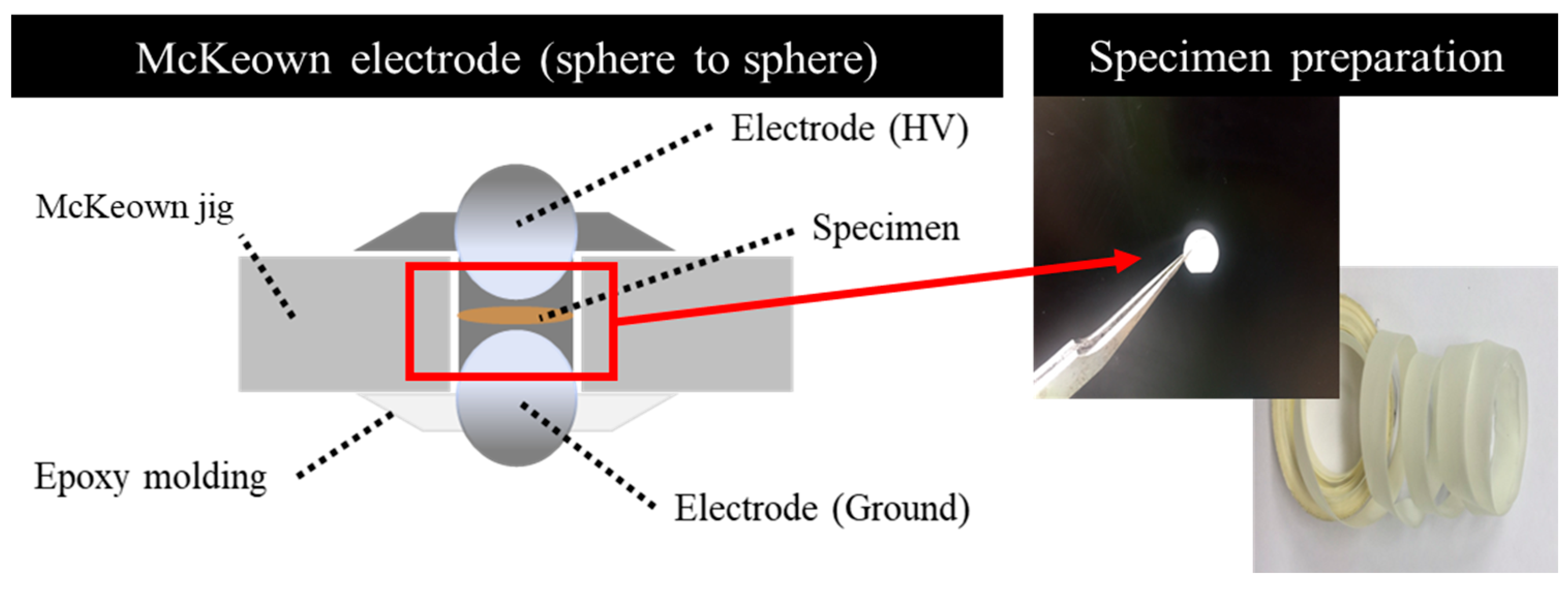
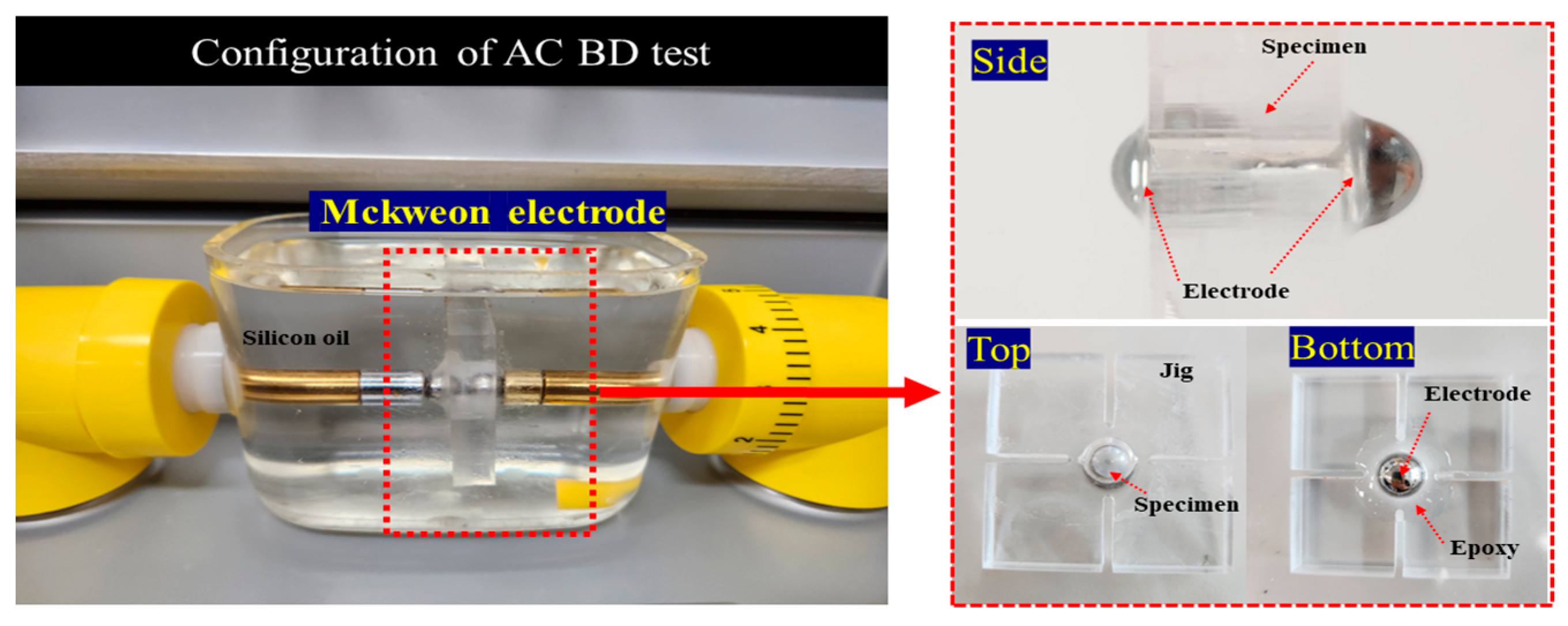

| Distribution | When to Use (Failure Rate Type) | Characteristics |
|---|---|---|
| Normal | Wear-out (IFR) | Real-number range Could be unsuitable for lifetime analysis |
| Log-normal | Wear-out (IFR) | Failure of natural deterioration |
| Exponential | Useful life (CFR) | Constant probability of failure |
| Weibull | Early, Useful, Wear-out (DFR, CFR, IFR) | Failure rate changes according to time |
| Item | Contents | |
|---|---|---|
| Testing condition | Maximum voltage | 60 [kV] |
| Applied voltage | AC ramp | |
| Ramp rate | 1 [kV/s] | |
| Material of electrode | Stainless steel | |
| Electrode type | McKeown (sphere to sphere) | |
| Electrode size | 10 Φ | |
| Specimen | Material | PP |
| Size | 8 Φ | |
| Thickness | 120 ± 8 [μm] | |
| Environmental condition | Temperature | 20 [°C] |
| Relative humidity | 35 [%] | |
| Degradation Condition | Coefficient of Determination | |||||
|---|---|---|---|---|---|---|
| Temp. [°C] | Time [h] | Log-Normal | Exp-1P | Exp-2P | Weibull-2P | Weibull-3P |
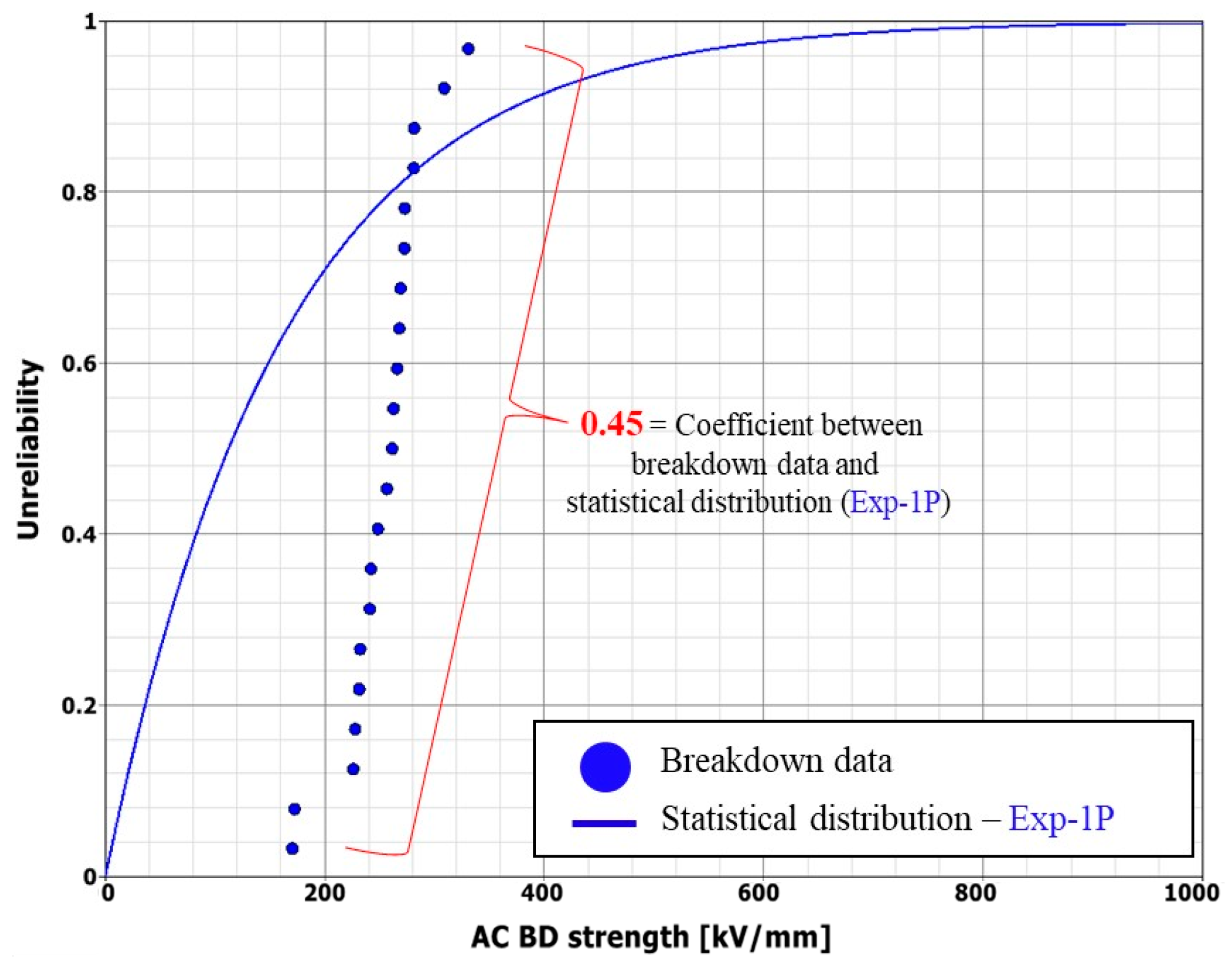
| - | 0 | 0.955 | 0.465 | 0.726 | 0.912 | 0.953 |
| 110 | 72 | 0.966 | 0.469 | 0.771 | 0.937 | 0.964 |
| 240 | 0.815 | 0.520 | 0.629 | 0.872 | 0.863 | |
| 480 | 0.960 | 0.500 | 0.774 | 0.916 | 0.970 | |
| 720 | 0.897 | 0.533 | - | 0.897 | - | |
| 960 | 0.956 | 0.516 | 0.561 | 0.982 | 0.982 | |
| 130 | 72 | 0.949 | 0.450 | 0.984 | 0.830 | 0.988 |
| 240 | 0.968 | 0.480 | 0.607 | 0.976 | 0.980 | |
| 480 | 0.966 | 0.516 | 0.626 | 0.968 | 0.978 | |
| 720 | 0.922 | 0.513 | 0.618 | 0.972 | 0.976 | |
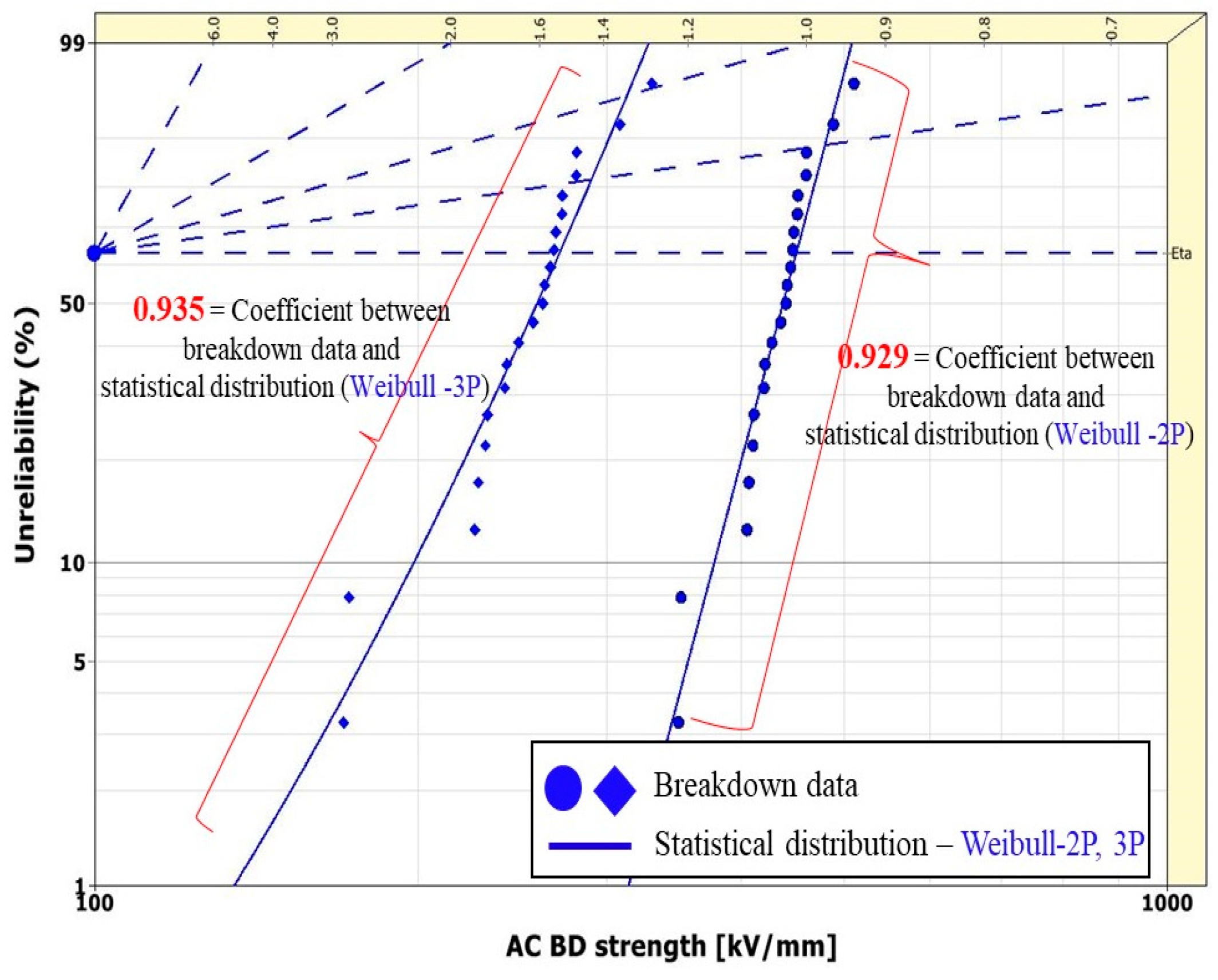
| 960 | 0.884 | 0.555 | 0.581 | 0.929 | 0.935 | |
| Degradation Condition | Mean Difference | |||||
|---|---|---|---|---|---|---|
| Temp. [°C] | Time [h] | Log-Normal | Exp-1P | Exp-2P | Weibull-2P | Weibull-3P |
| - | 0 | 5.3 | 24.2 | 10.1 | 6.1 | 5.2 |
| 110 | 72 | 4.4 | 25.7 | 8.5 | 3.8 | 3.8 |
| 240 | 10.3 | 27.1 | 22.6 | 8.4 | - | |
| 480 | 3.8 | 29.1 | 8.8 | 6.2 | 3.6 | |
| 720 | 13.8 | 26.3 | - | 11.8 | - | |
| 960 | 3.0 | 29.3 | 18.3 | 2.4 | 2.1 | |
| 130 | 72 | 4.0 | 23.4 | 2.9 | 6.7 | 2.5 |
| 240 | 3.0 | 27.2 | 15.9 | 3.5 | 3.2 | |
| 480 | 3.7 | 29.6 | 14.8 | 2.5 | 3.2 | |
| 720 | 5.2 | 28.6 | 16.5 | 3.1 | 2.6 | |
| 960 | 7.4 | 31.3 | 20.7 | 4.8 | - | |
| Degradation Condition | Weibull-2P | Weibull-3P | Weibull-3P Available | |||
|---|---|---|---|---|---|---|
| Temp. [°C] | Time [h] | AIC | BIC | AIC | BIC | |
| - | 0 | 226 | 228 | 225 | 228 | O |
| 110 | 72 | 221 | 223 | 221 | 224 | X |
| 240 | 227 | 229 | - | - | X | |
| 480 | 196 | 198 | 195 | 198 | O | |
| 720 | 222 | 224 | - | - | X | |
| 960 | 207 | 209 | 209 | 212 | X | |
| 130 | 72 | 214 | 216 | 201 | 203 | O |
| 240 | 214 | 216 | 216 | 219 | X | |
| 480 | 199 | 201 | 200 | 203 | X | |
| 720 | 206 | 208 | 208 | 210 | X | |
| 960 | 199 | 201 | - | - | X | |
| Degradation Condition | Selected Distribution | Coefficient of Determination | Log-Normal | Weibull | ||||
|---|---|---|---|---|---|---|---|---|
| Temp. [°C] | Time [h] | μ′ | σ′ | η | β | γ | ||
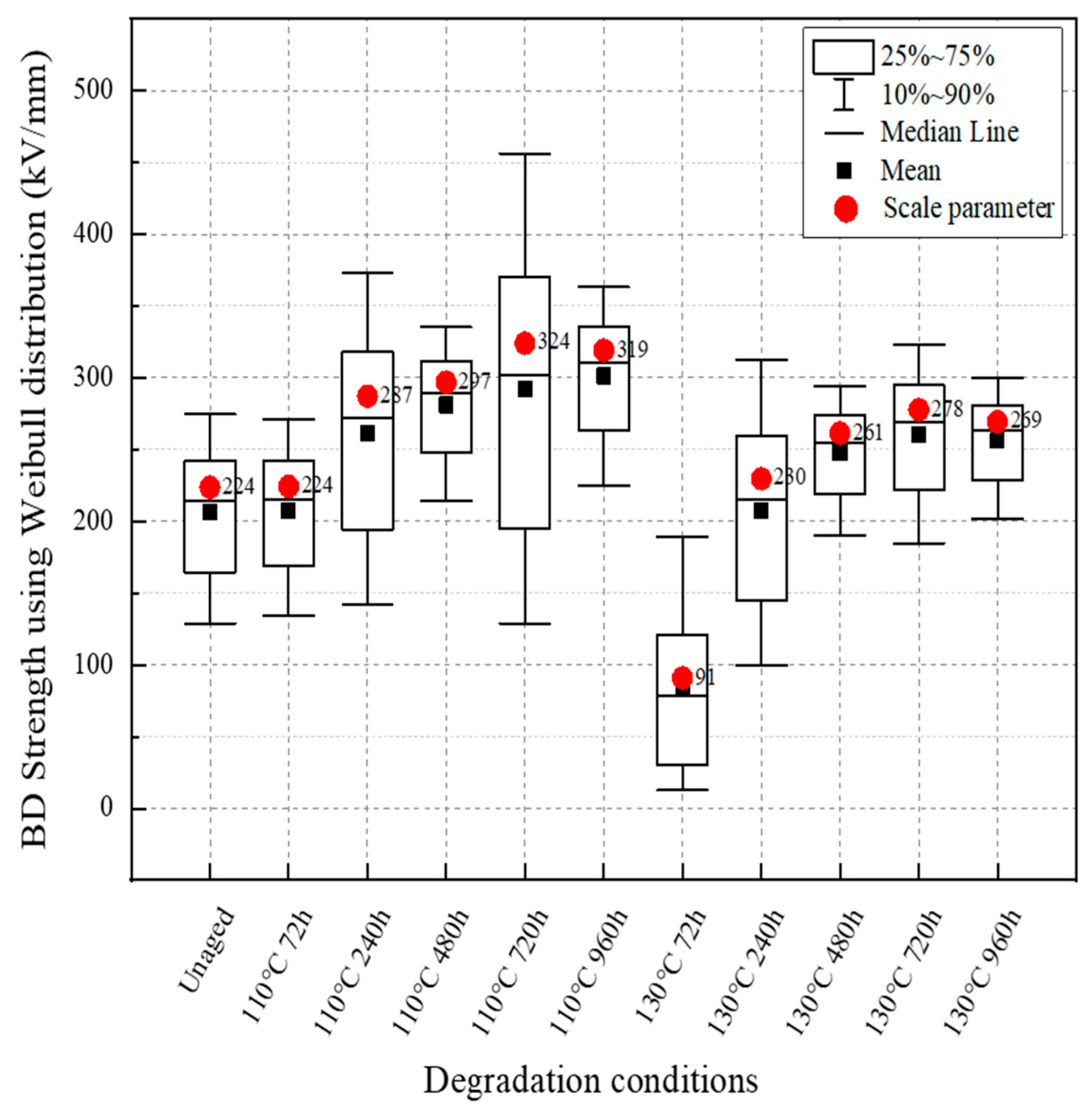
| - | 0 | Log-normal | 0.955 | 5.27 | 0.31 | |||
| 110 | 72 | Log-normal | 0.966 | 5.28 | 0.28 | |||
| 240 | Weibull-2P | 0.872 | 287.17 | 3.19 | ||||
| 480 | Weibull-3P | 0.970 | 95.32 | 1.51 | 195.25 | |||
| 720 | Weibull-2P | 0.897 | 324.02 | 2.44 | ||||
| 960 | Weibull-2P | 0.982 | 319.09 | 6.43 | ||||
| 130 | 72 | Weibull-3P | 0.988 | 90.93 | 1.14 | 141.02 | ||
| 240 | Weibull-2P | 0.976 | 229.60 | 2.70 | ||||
| 480 | Weibull-2P | 0.968 | 261.31 | 7.08 | ||||
| 720 | Weibull-2P | 0.972 | 277.68 | 5.51 | ||||
| 960 | Weibull-2P | 0.929 | 269.30 | 7.72 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, K.-H.; Lee, S.-W.; Kim, H.-J.; Lim, J.-S. Reliability Assessment of Statistical Distributions for Analyzing Dielectric Breakdown Strength of Polypropylene. Appl. Sci. 2024, 14, 3. https://doi.org/10.3390/app14010003
Park K-H, Lee S-W, Kim H-J, Lim J-S. Reliability Assessment of Statistical Distributions for Analyzing Dielectric Breakdown Strength of Polypropylene. Applied Sciences. 2024; 14(1):3. https://doi.org/10.3390/app14010003
Chicago/Turabian StylePark, Keon-Hee, Seung-Won Lee, Hae-Jong Kim, and Jang-Seob Lim. 2024. "Reliability Assessment of Statistical Distributions for Analyzing Dielectric Breakdown Strength of Polypropylene" Applied Sciences 14, no. 1: 3. https://doi.org/10.3390/app14010003
APA StylePark, K.-H., Lee, S.-W., Kim, H.-J., & Lim, J.-S. (2024). Reliability Assessment of Statistical Distributions for Analyzing Dielectric Breakdown Strength of Polypropylene. Applied Sciences, 14(1), 3. https://doi.org/10.3390/app14010003






