Reduced-Order Model Based on Volterra Series for Aerodynamics of the Bridge Deck Section and Flutter Critical Wind Speed Prediction
Abstract
Featured Application
Abstract
1. Introduction
2. Volterra Model and FSI Method
2.1. Volterra Series Theory
2.2. Volterra Kernel Identification Based on Impulse Function
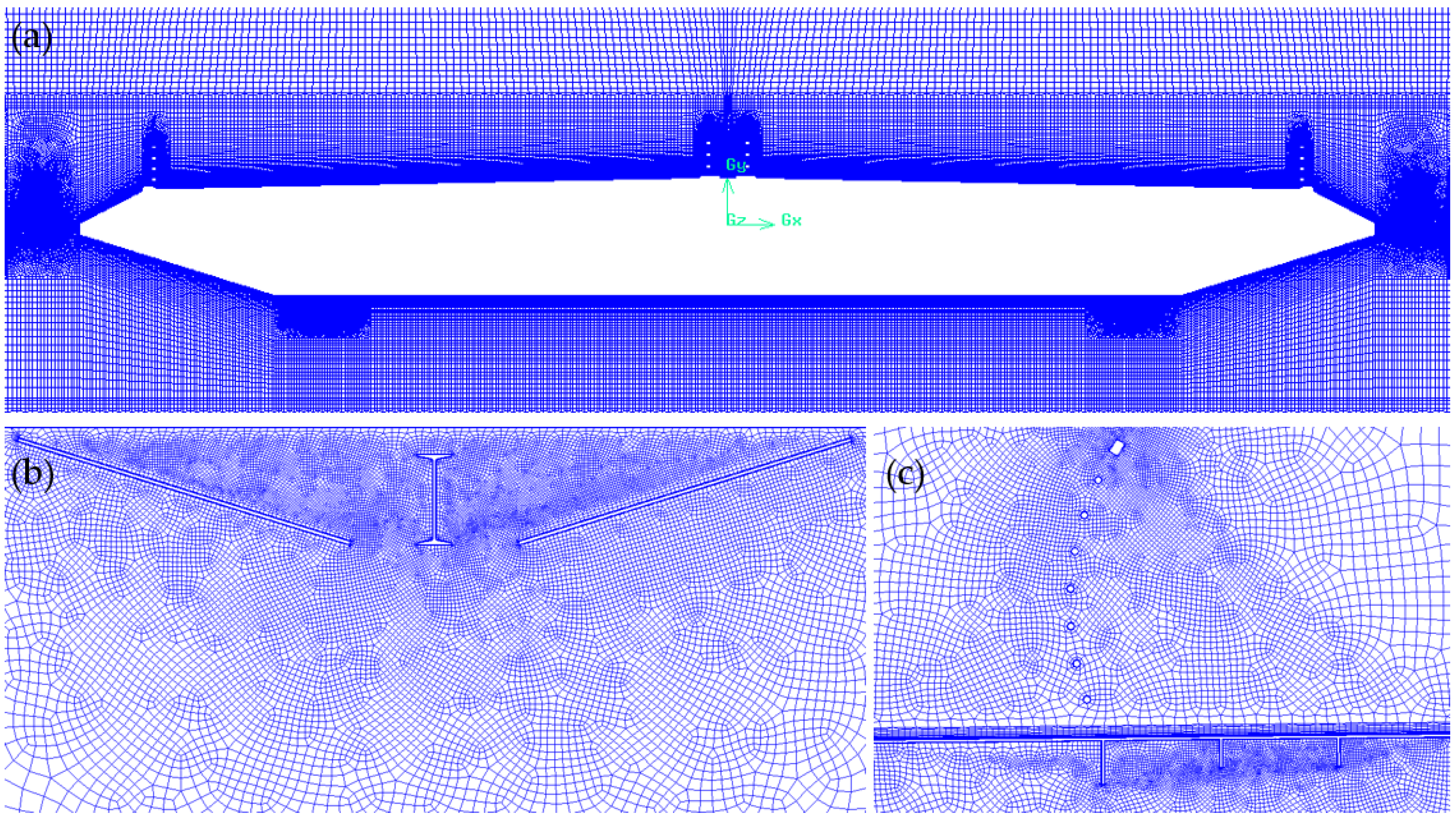
2.3. Computational Approach
2.4. Aeroelastic Analysis Based on the Volterra Model and Newmark-β Method
3. Validations and Applications
3.1. Validations
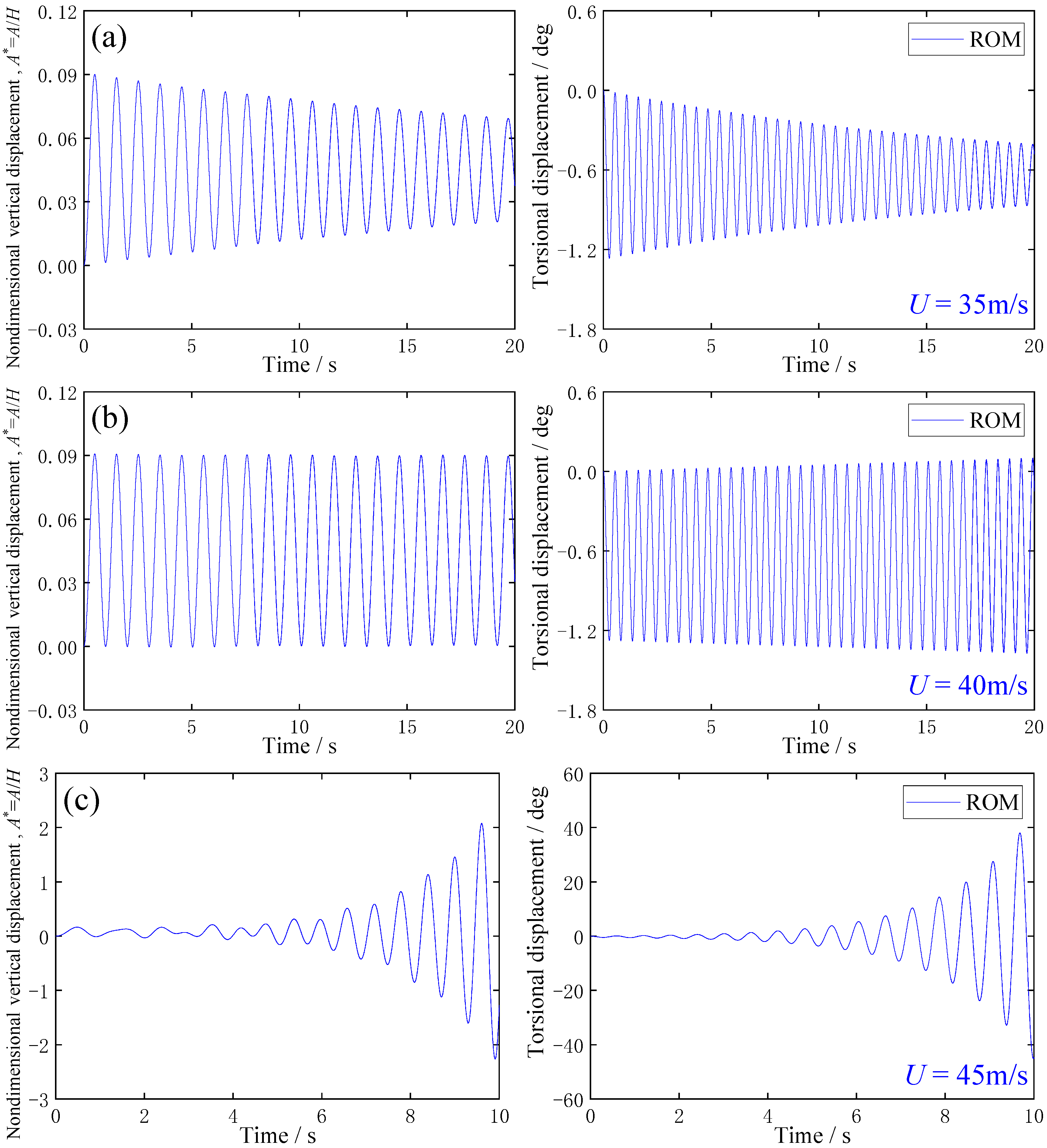
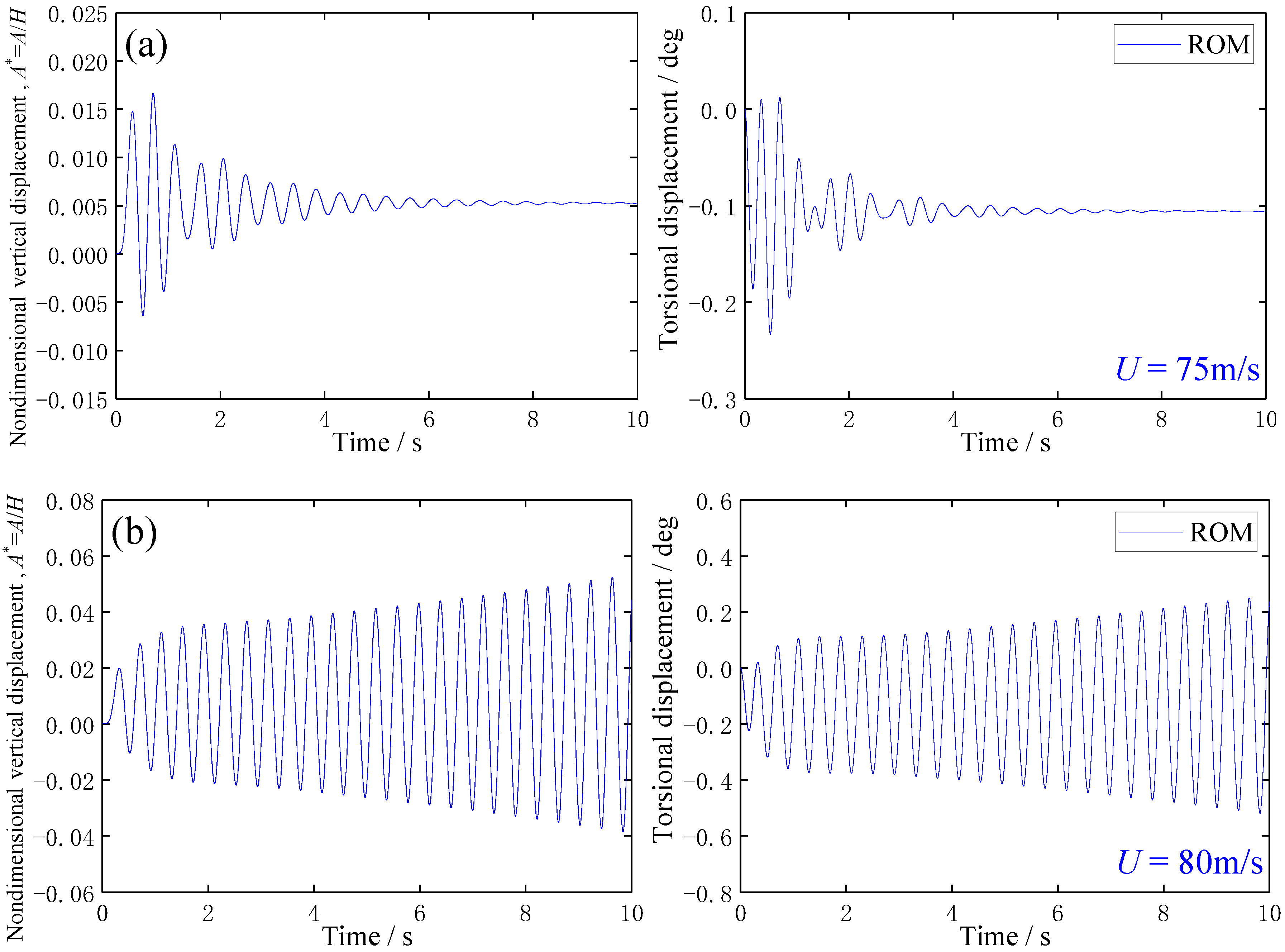
3.2. Numerical Results of Wind-Induced Vibration Responses
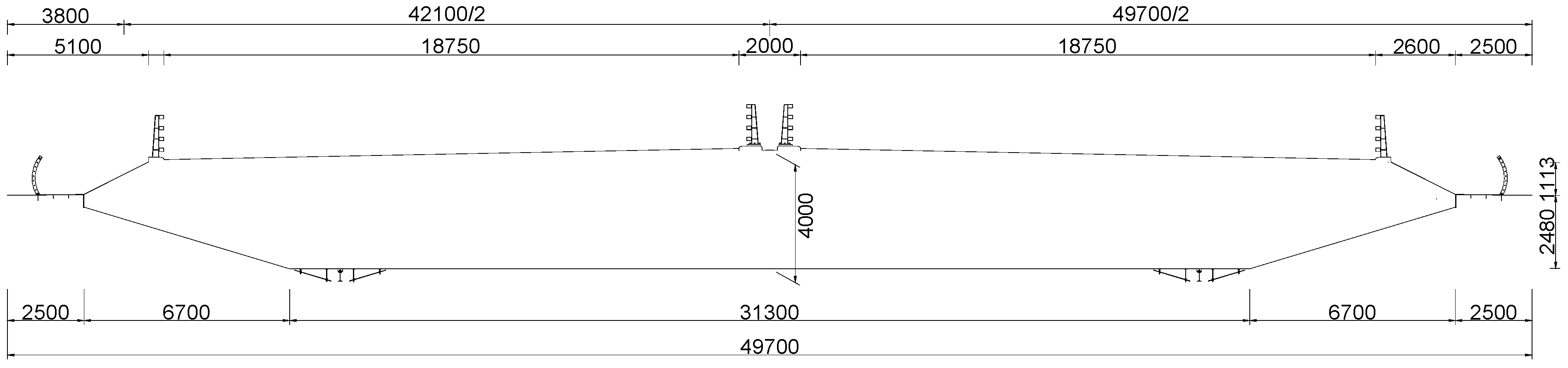
3.3. Practical Application
4. Discussion and Conclusions
- The aerodynamic ROM of the main girder section is established based on the first-order Volterra series through the CFD numerical simulation. Moreover, the aerodynamic identification accuracy of the main girder is found to be in good agreement with the results of the forced vibration based on CFD numerical simulation. Additionally, the established aerodynamic ROM can consider the nonlinear aerodynamic effect caused by the certain amplitude and frequency of the streamlined main beam section.
- An aeroelastic analysis method for the main girder section is established based on the Volterra series-based aerodynamic ROM and the Newmark-β method, which enabled efficient FSI calculations and the determination of the flutter critical wind speed and flutter frequency. Moreover, the accuracy and feasibility of the methods in this study are verified by comparing the numerical results of different main deck sections obtained from FSI simulations based on ANSYS Fluent and the existing numerical and experimental results.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Farquharson, F.B. The Collapse of the Tacoma Narrows Bridge. Sci. Mon. 1940, 51, 574–578. [Google Scholar]
- Scanlan, R.H.; Tomko, J.J. Airfoil and Bridge Deck Flutter Derivatives. J. Eng. Mech. Div. 1971, 97, 1717–1733. [Google Scholar] [CrossRef]
- Patil, M.J.; Hodges, D.H.; Cesnik, C.E.S. Limit-Cycle Oscillation in High-Aspect-Ratio Wings. J. Fluids Struct. 2001, 15, 107–132. [Google Scholar] [CrossRef]
- Scanlan, R.H. Amplitude and Turbulence Effects on Bridge Flutter Derivatives. J. Struct. Eng. 1997, 123, 232–236. [Google Scholar] [CrossRef]
- Dowell, E.H. Some Recent Advances in Nonlinear Aeroelasticity: Fluid-Structure Interaction in the 21st Century. In Proceedings of the 51st AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Orlando, FL, USA, 12–15 April 2010. [Google Scholar]
- Silva, W.A. Identification of Linear and Nonlinear Aerodynamic Impulse Responses Using Digital Filter Techniques. In Proceedings of the 22nd AIAA Atmospheric Flight Mechanics Conference, New Orleans, LA, USA, 11–13 August 1997. [Google Scholar]
- Silva, W.A.; Bartels, R.E. Development of Reduced-Order Models for Aeroelastic Analysis and Flutter Prediction Using the CFL3Dv6.0 Code. J. Fluids Struct. 2004, 19, 729–745. [Google Scholar] [CrossRef]
- Hall, K.C.; Thomas, J.P.; Clark, W.S. Computation of Unsteady Nonlinear Flows in Cascades Using a Harmonic Balance Technique. AIAA J. 2002, 40, 879–886. [Google Scholar] [CrossRef]
- Romanowski, M. Reduced-Order Unsteady Aerodynamic and Aeroelastic Models Using Karhunen-Lo’eve Eigenmodes. In Proceedings of the 6th AIAA Symposium on Multidisciplinary Analysis and Optimization, Bellevue, WA, USA, 4–6 September 1996. [Google Scholar]
- Li, T.; Wu, T.; Liu, Z. Nonlinear unsteady bridge aerodynamics: Reduced-order modeling based on deep LSTM networks. J. Wind. Eng. Ind. Aerodyn. 2020, 198, 104116. [Google Scholar] [CrossRef]
- Wiener, N. Response of a Nonlinear Device to Noise. MIT Radiation Lab. Report No. 129. 1942. Available online: https://apps.dtic.mil/sti/citations/ADA800212 (accessed on 25 January 2023).
- Clancy, S.J.; Rugh, W.J. A Note on the Identification of Discrete-Time Polynomial Systems. IEEE Trans. Automat. Contr. 1979, 24, 975–978. [Google Scholar] [CrossRef]
- Tromp, J.; Jenkins, J. A Volterra Kernel Identification Scheme Applied to Aerodynamic Reactions. In Proceedings of the 17th Atmospheric Flight Mechanics Conference, Portland, OR, USA, 20–22 August 1990. [Google Scholar]
- Silva, W. Recent Enhancements to the Development of CFD-Based Aeroelastic Reduced-Order Models. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, HI, USA, 23–26 April 2013. [Google Scholar]
- Wu, T.; Kareem, A. Simulation of Nonlinear Bridge Aerodynamics: A Sparse Third-Order Volterra Model. J. Sound Vib. 2014, 333, 178–188. [Google Scholar] [CrossRef]
- Liu, K.; Li, D.; Xiang, J. Reduced-order modeling of unsteady aerodynamics of a flapping wing based on the Volterra theory. Results Phys. 2017, 7, 2451–2457. [Google Scholar] [CrossRef]
- Skyvulstad, H.; Petersen, Ø.W.; Argentini, T.; Zasso, A.; Øiseth, O. The use of a Laguerrian expansion basis as Volterra kernels for the efficient modeling of nonlinear self-excited forces on bridge decks. J. Wind. Eng. Ind. Aerodyn. 2021, 219, 104805. [Google Scholar] [CrossRef]
- Ali, K.; Katsuchi, H.; Yamada, H. Development of nonlinear framework for simulation of Typhoon-induced buffeting response of Long-span bridges using Volterra series. Eng. Struct. 2021, 244, 112721. [Google Scholar] [CrossRef]
- Ruiz, C.; Acosta, J.A.; Ollero, A. Aerodynamic reduced-order Volterra model of an ornithopter under high-amplitude flapping. Aerosp. Sci. Technol. 2022, 121, 107331. [Google Scholar] [CrossRef]
- Xu, K.; Zhao, L.; Ge, Y. Reduced-order modeling and calculation of vortex-induced vibration for large-span bridges. J. Wind. Eng. Ind. Aerodyn. 2017, 167, 228–241. [Google Scholar] [CrossRef]
- Li, L.Z.; Yang, M.; Luo, X.; Zhang, J.; Yuan, M.N. A spline ROM of blade aerodynamic force to upstream wake. Aerosp. Sci. Technol. 2018, 84, 650–660. [Google Scholar] [CrossRef]
- Lin, R.M.; Ng, T.Y. Identification of Volterra kernels for improved predictions of nonlinear aeroelastic vibration responses and flutter. Eng. Struct. 2018, 171, 15–28. [Google Scholar] [CrossRef]
- Volterra, V. Theory of Functionals and of Integral and Integro-Differential Equations, 1st ed.; Dover Publications: New York, NY, USA, 1959; pp. 1–226. [Google Scholar]
- Wu, T.; Kareem, A. A nonlinear convolution scheme to simulate bridge aerodynamics. Comput. Struct. 2013, 128, 259–271. [Google Scholar] [CrossRef]
- Wu, T.; Kareem, A. A nonlinear analysis framework for bluff-body aerodynamics: A Volterra representation of the solution of Navier-Stokes equations. J. Fluids Struct. 2015, 54, 479–502. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Zhang, M.; Xu, F.; Wu, T.; Zhang, Z. Postflutter Analysis of Bridge Decks Using Aerodynamic-Describing Functions. J. Bridge Eng. 2020, 25, 04020046. [Google Scholar] [CrossRef]
- Gao, G.; Zhu, L.; Li, J.; Han, W.; Wei, L.; Yan, Q. Nonlinear post-flutter bifurcation of a typical twin-box bridge deck: Experiment and empirical modeling. J. Fluids Struct. 2022, 112, 103583. [Google Scholar] [CrossRef]
- Fujiwara, A. Numerical simulation of flow field around an oscillating bridge using finite difference method. J. Wind. Eng. Ind. Aerodyn. 1993, 46, 567–575. [Google Scholar] [CrossRef]
- Walther, J.H. Discrete Vortex Method for Two-dimensional Flow past Bodies of Arbitrary Shape Undergoing Prescribed Rotary and Translational Motion. Ph.D. Dissertation, Technical University of Denmark, Copenhagen, Denmark, 1 September 1994. [Google Scholar]
- Poulsen, N.K.; Damsgaard, A.; Reinhold, T.A. Determination of flutter derivatives for the Great Belt Bridge. J. Wind. Eng. Ind. Aerodyn. 1992, 41, 153–164. [Google Scholar] [CrossRef]
- Wang, L. Numerical Simulation of Aerodynamic Stability of Large Span Bridges with Closed Streamlined Box Girder. Master Dissertation, Hunan University, Changsha, China, 23 May 2017. (In Chinese). [Google Scholar]

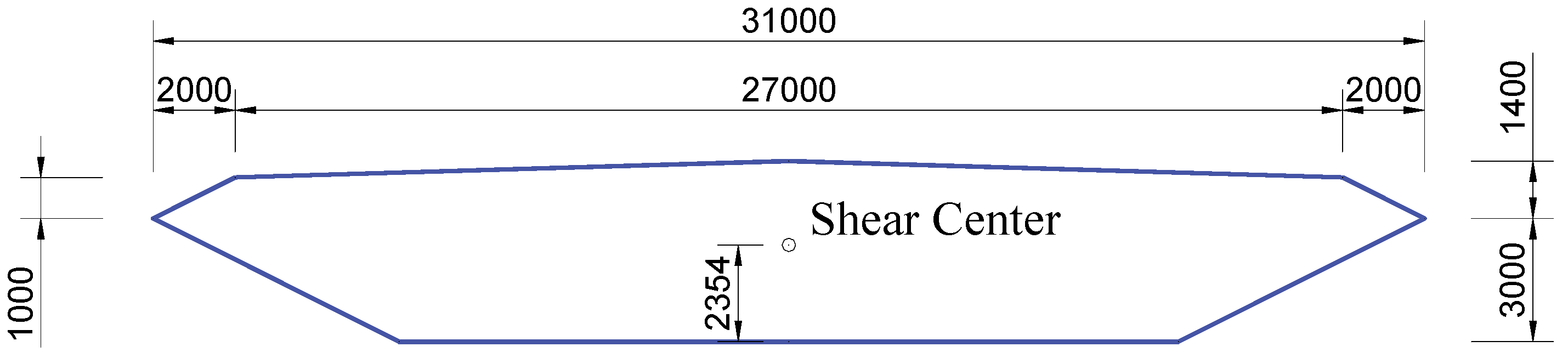

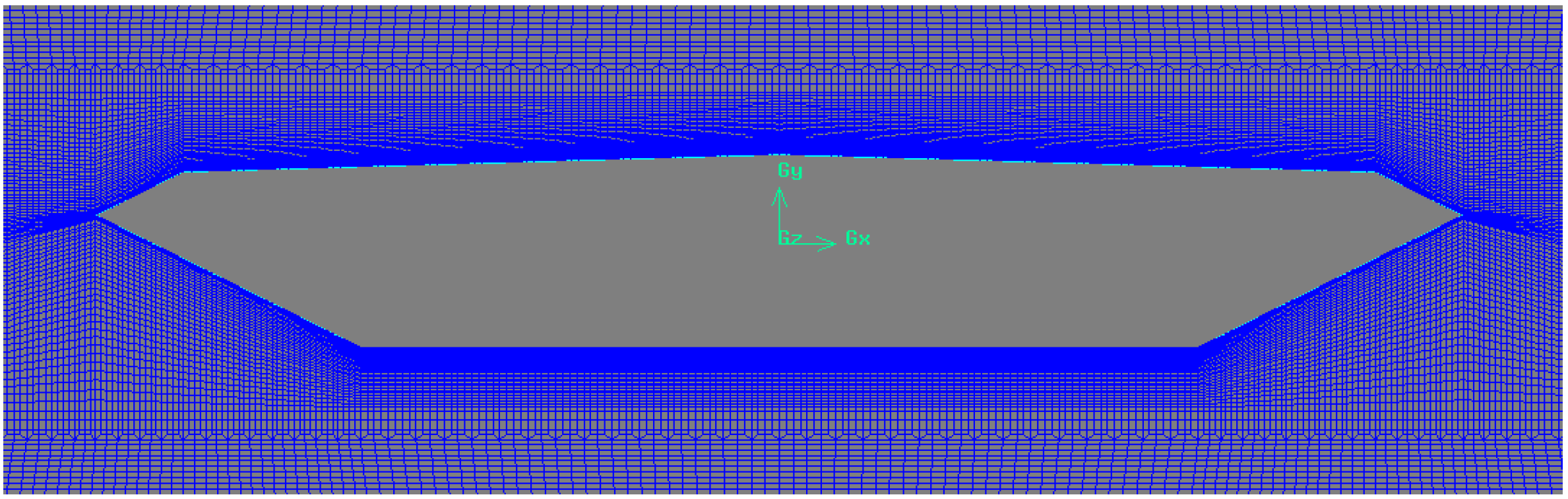

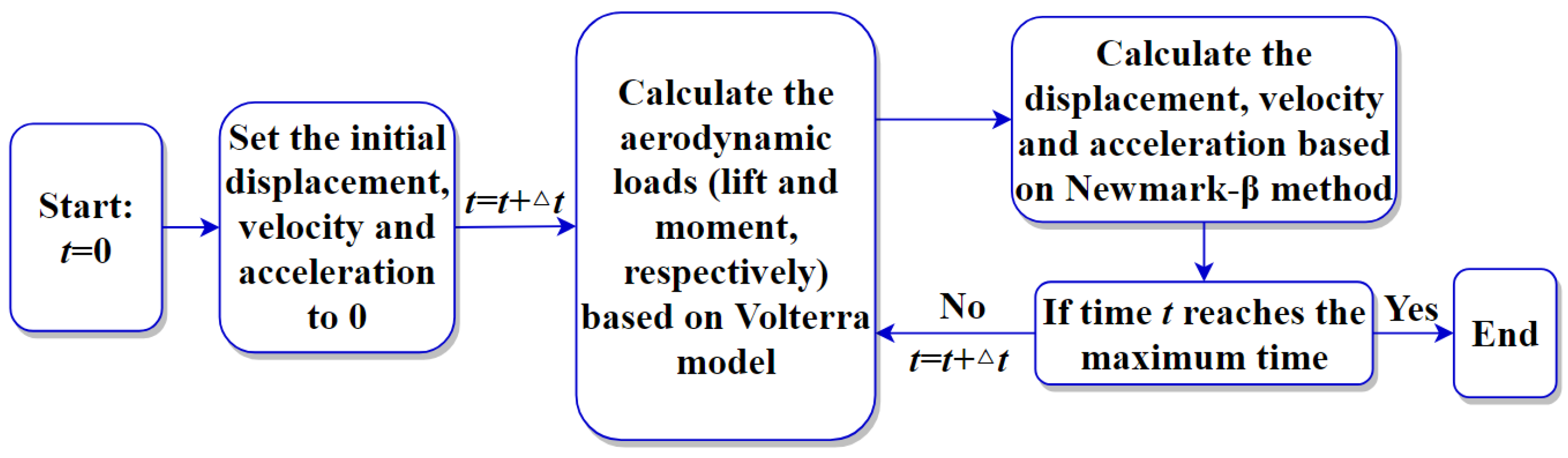
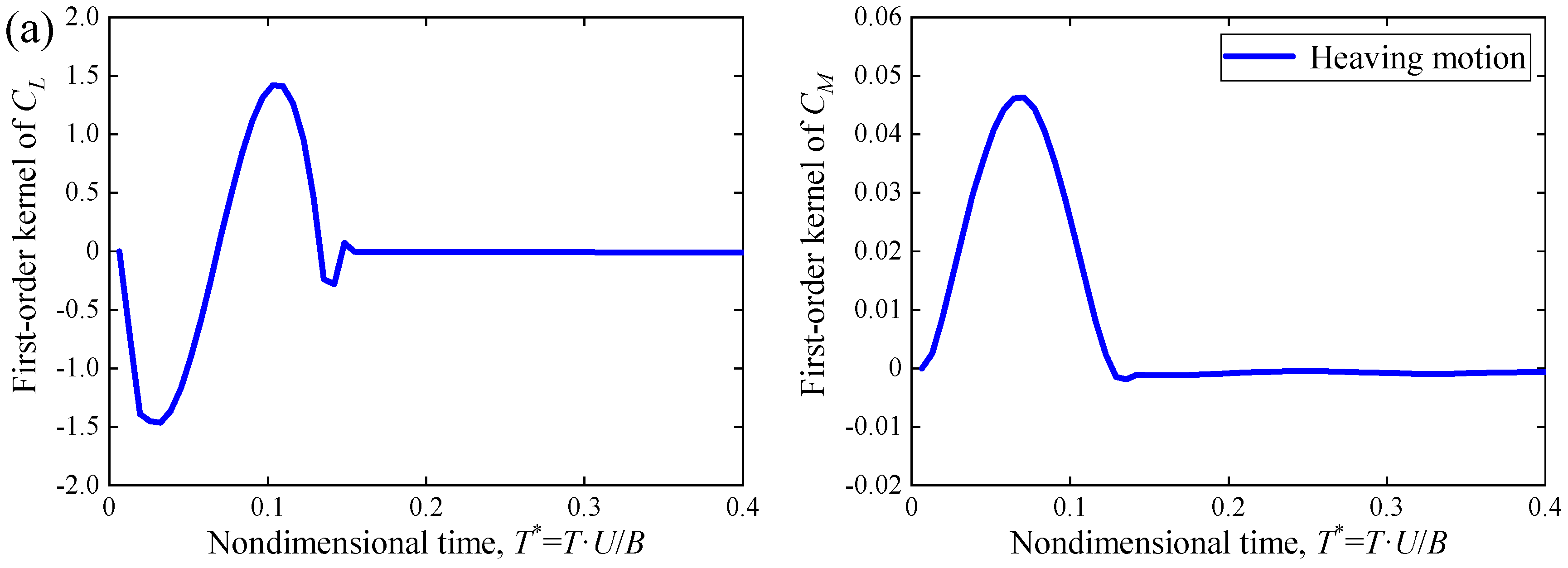
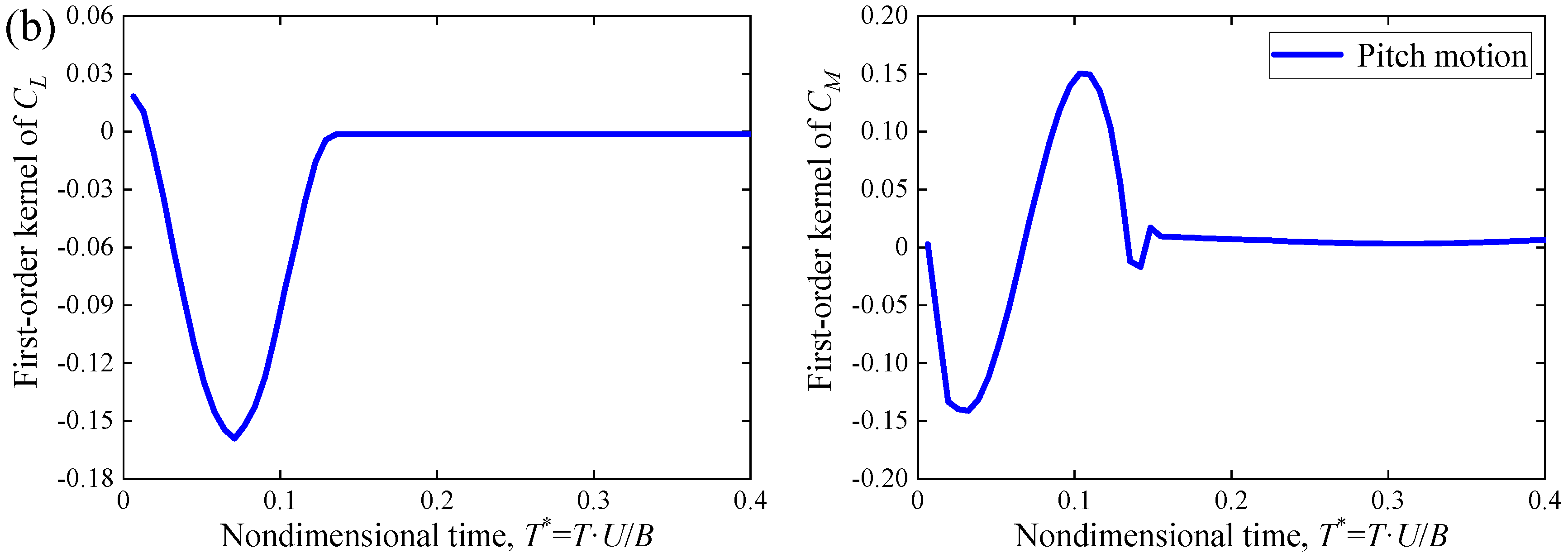
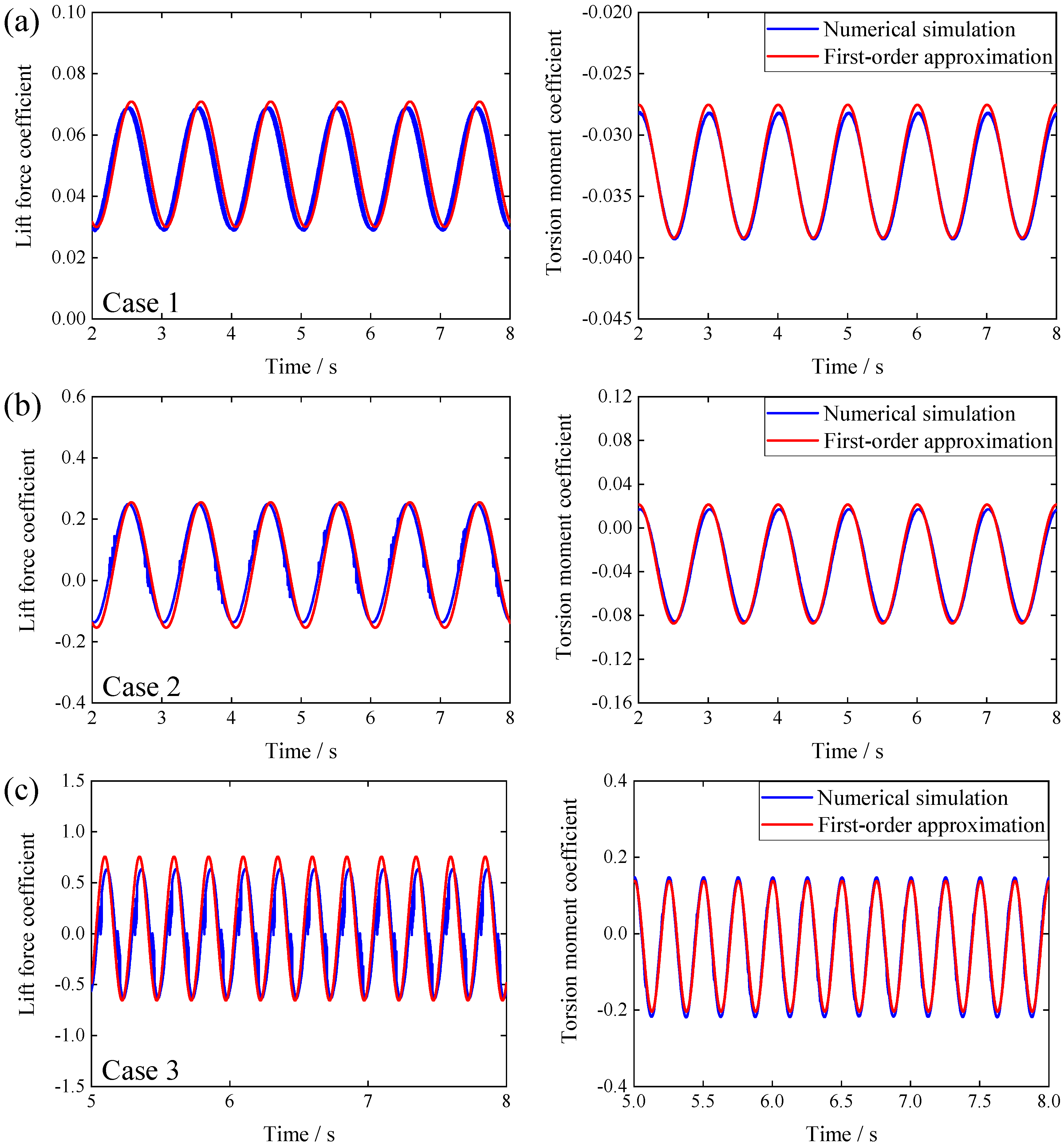
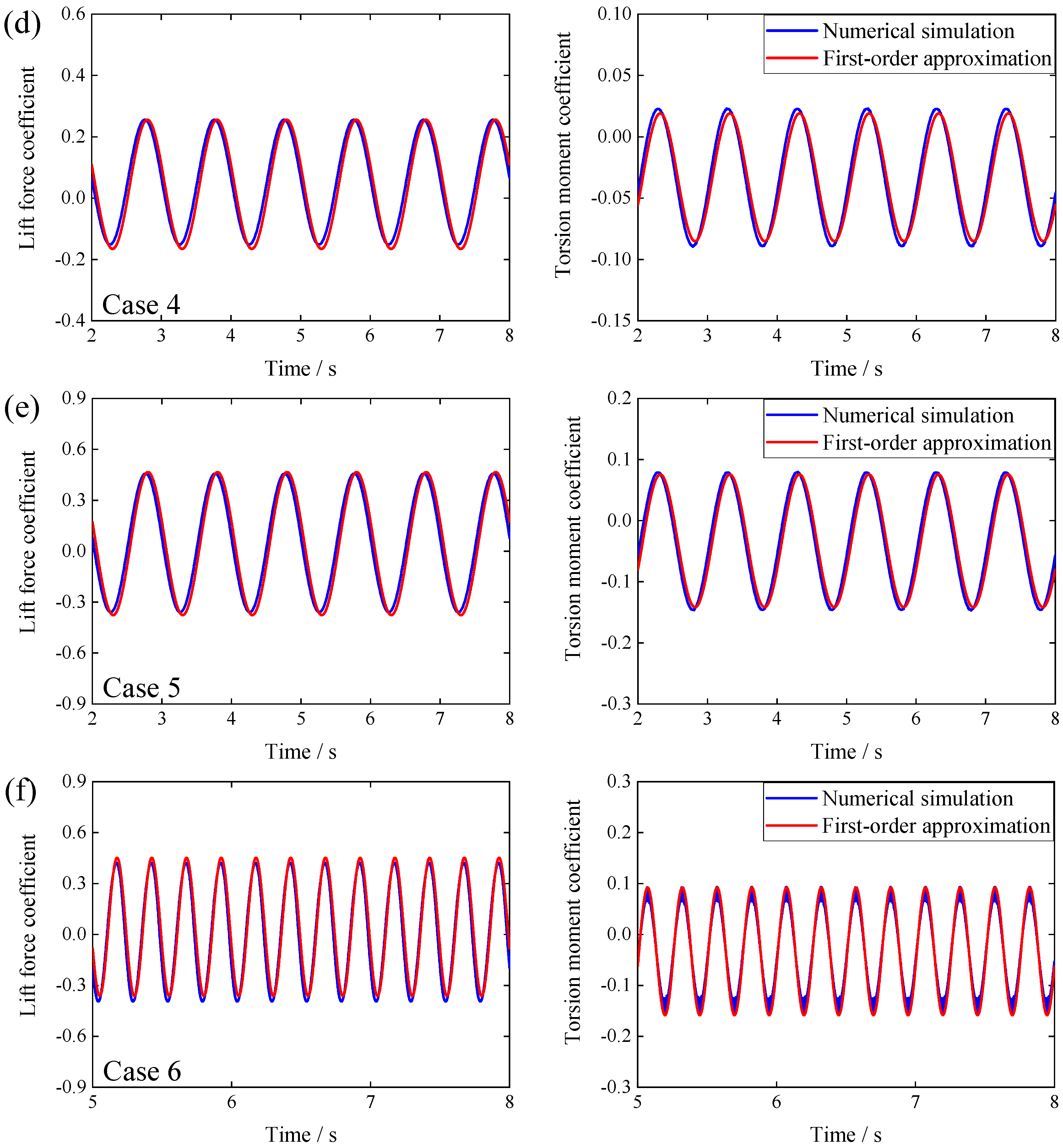
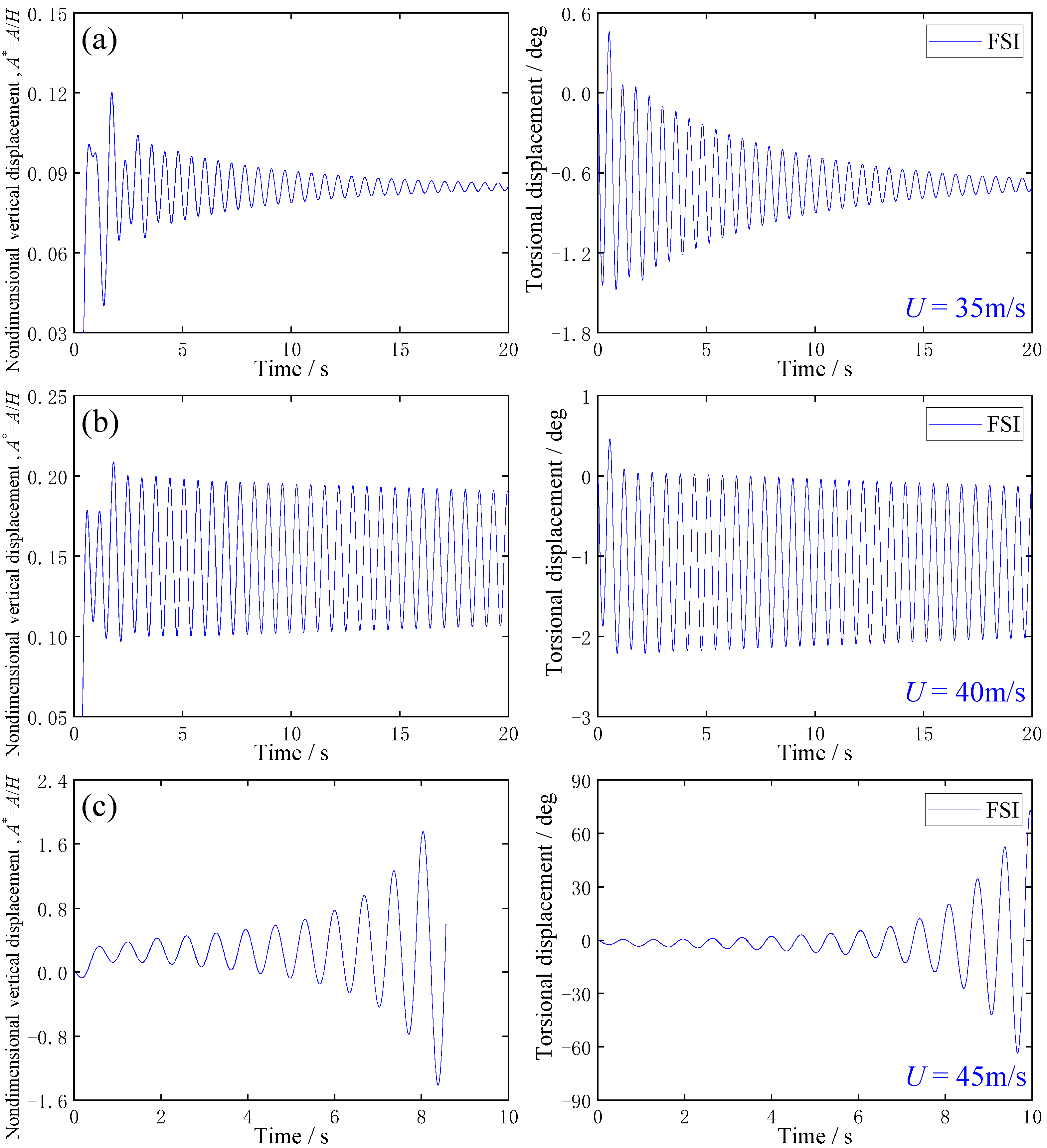
| Case | yh/m | fh/Hz | Root Variance of Force Coefficient | First-Order Approximation | Numerical Simulation | Relative Error, δ/% |
|---|---|---|---|---|---|---|
| 1 | 0.004 | 1 | CL’ | 0.0145 | 0.0140 | 3.57 |
| CM’ | 0.0038 | 0.0036 | 5.55 | |||
| 2 | 0.04 | 1 | CL’ | 0.1447 | 0.1396 | 3.65 |
| CM’ | 0.0378 | 0.0359 | 5.29 | |||
| 3 | 0.04 | 4 | CL’ | 0.4990 | 0.4796 | 4.05 |
| CM’ | 0.1209 | 0.1252 | 1.91 |
| Case | θα/° | fα/Hz | Root Variance of Force Coefficient | First-Order Approximation | Numerical Simulation | Relative Error, δ/% |
|---|---|---|---|---|---|---|
| 4 | 3 | 1 | CL’ | 0.1460 | 0.1474 | 0.95 |
| CM’ | 0.0371 | 0.0392 | 5.36 | |||
| 5 | 6 | 1 | CL’ | 0.2919 | 0.2963 | 1.48 |
| CM’ | 0.0743 | 0.0786 | 5.47 | |||
| 6 | 6 | 4 | CL’ | 0.2761 | 0.2910 | 5.12 |
| CM’ | 0.0919 | 0.0871 | 5.51 |
| Parameters | Unit | Prototype Main Deck Section | Scale Ratio | Main Deck Section MODEL |
|---|---|---|---|---|
| Width, B | m | 31.0 | 1:80 | 0.3875 |
| Height, H | m | 4.4 | 1:80 | 0.0550 |
| Mass per unit length, m | kg/m | 17.8 × 103 | 1:802 | 2.781 |
| Torsion mass moment of inertia per unit length, I | kg·m2/m | 2.173 × 106 | 1:804 | 0.053 |
| Vertical bending frequency, fh | Hz | 0.099 | 10:1 | 0.99 |
| Torsional frequency, fα | Hz | 0.186 | 10:1 | 1.86 |
| Vertical bending–damping ratio, ζh | % | 0.5 | / | 0.5 |
| Torsional damping ratio, ζα | % | 0.5 | / | 0.5 |
| Wind velocity, U | m/s | / | 1:8 | / |
| Method | Flutter Critical Wind Speed (m/s) | Flutter Frequency (Hz) |
|---|---|---|
| Finite volume method (Fujiwara [29]) | 40.5 | 0.160 |
| Discrete-vortex model (Walther [30]) | 37.6 | 0.165 |
| Wind tunnel test (Poulsen et al. [31]) | 36.0 | 0.163 |
| FSI (present) | 40.0 | 0.164 |
| ROM (present) | 35.0~40.0 | 0.160 |
| Parameters | Unit | Prototype Main Deck Section | Scale Ratio | Main Deck Section Model |
|---|---|---|---|---|
| Width, B | m | 49.7 | 1:70 | 7.10 |
| Height, H | m | 4.4 | 1:70 | 0.057 |
| Mass per unit length, m | kg/m | 6.95 × 104 | 1:702 | 14.184 |
| Torsion mass moment of inertia per unit length, I | kg•m2/m | 1.16 × 107 | 1:704 | 0.483 |
| Vertical bending frequency, fh | Hz | 0.103 | 16.83:1 | 1.7334 |
| Torsional frequency, fα | Hz | 0.225 | 16.83:1 | 3.7842 |
| Vertical bending–damping ratio, ζh | % | 0.5 | / | 0.5 |
| Torsional damping ratio, ζα | % | 0.5 | / | 0.5 |
| Wind velocity, U | m/s | / | 1:4.16 | / |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, Z.; Liu, Z.; He, F. Reduced-Order Model Based on Volterra Series for Aerodynamics of the Bridge Deck Section and Flutter Critical Wind Speed Prediction. Appl. Sci. 2023, 13, 3486. https://doi.org/10.3390/app13063486
Wei Z, Liu Z, He F. Reduced-Order Model Based on Volterra Series for Aerodynamics of the Bridge Deck Section and Flutter Critical Wind Speed Prediction. Applied Sciences. 2023; 13(6):3486. https://doi.org/10.3390/app13063486
Chicago/Turabian StyleWei, Ziran, Zhiwen Liu, and Fawei He. 2023. "Reduced-Order Model Based on Volterra Series for Aerodynamics of the Bridge Deck Section and Flutter Critical Wind Speed Prediction" Applied Sciences 13, no. 6: 3486. https://doi.org/10.3390/app13063486
APA StyleWei, Z., Liu, Z., & He, F. (2023). Reduced-Order Model Based on Volterra Series for Aerodynamics of the Bridge Deck Section and Flutter Critical Wind Speed Prediction. Applied Sciences, 13(6), 3486. https://doi.org/10.3390/app13063486







